Effect of Intraluminal Thrombus Burden on the Risk of Abdominal Aortic Aneurysm Rupture
Abstract
1. Introduction
2. Materials and Methods
2.1. Model Geometry
2.2. Modeling of the Fluid Domain
2.2.1. Boundary Conditions in the Fluid Domain
2.2.2. Material Properties of Blood
2.3. FSI Modeling
2.3.1. Governing Equations and Material Properties in Solid Domain
2.3.2. Boundary Conditions in Solid Domain
3. Results
3.1. Mesh Independency in Fluid Domain
3.2. Mesh Independency in Solid Domain
3.3. Determination of Effective Stresses
3.4. The Maximum Effective Stresses on Artery
3.5. The Maximum Displacement Magnitudes on Artery
3.6. Statistical Analyses on Maximum Effective Stresses
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Salman, H.E.; Ramazanli, B.; Yavuz, M.M.; Yalcin, H.C. Biomechanical Investigation of Disturbed Hemodynamics-Induced Tissue Degeneration in Abdominal Aortic Aneurysms Using Computational and Experimental Techniques. Front. Bioeng. Biotechnol. 2019, 7, 111. [Google Scholar] [CrossRef] [PubMed]
- McGloughlin, T.M.; Doyle, B.J. New Approaches to Abdominal Aortic Aneurysm Rupture Risk Assessment. Arterioscler. Thromb. Vasc. Biol. 2010, 30, 1687–1694. [Google Scholar] [CrossRef]
- Arzani, A.; Shadden, S.C. Transport and Mixing in Patient Specific Abdominal Aortic Aneurysms with Lagrangian Coherent Structures. In Proceedings of the Summer Bioengineering Conference, Fajardo, Puerto Rico, 20–23 June 2012; pp. 9–10. [Google Scholar]
- Arzani, A.; Shadden, S.C. Characterizations and Correlations of Wall Shear Stress in Aneurysmal Flow. J. Biomech. Eng. 2015, 138, 014503. [Google Scholar] [CrossRef]
- Arzani, A.; Suh, G.-Y.; Dalman, R.L.; Shadden, S.C. A longitudinal comparison of hemodynamics and intraluminal thrombus deposition in abdominal aortic aneurysms. Am. J. Physiol. Heart Circ. Physiol. 2014, 307, H1786–H1795. [Google Scholar] [CrossRef]
- Bengtsson, H.; Bergqvist, D. Ruptured abdominal aortic aneurysm: A population-based study. J. Vasc. Surg. 1993, 18, 74–80. [Google Scholar] [CrossRef] [PubMed]
- Salman, H.E.; Yalcin, H.C. Computational Investigation of the Effect of Wall Thickness on Rupture Risk in Abdominal Aortic Aneurysms. J. Appl. Fluid Mech. 2020, 14, 499–513. [Google Scholar] [CrossRef]
- Fillinger, M.F.; Raghavan, M.L.; Marra, S.P.; Cronenwett, J.L.; Kennedy, F.E. In vivo analysis of mechanical wall stress and abdominal aortic aneurysm rupture risk. J. Vasc. Surg. 2002, 36, 589–597. [Google Scholar] [CrossRef]
- Mutlu, O.; Salman, H.E.; Al-Thani, H.; El-Menyar, A.; Qidwai, U.A.; Yalcin, H.C. How does hemodynamics affect rupture tissue mechanics in abdominal aortic aneurysm: Focus on wall shear stress derived parameters, time-averaged wall shear stress, oscillatory shear index, endothelial cell activation potential, and relative residence time. Comput. Biol. Med. 2023, 154, 106609. [Google Scholar] [CrossRef]
- Salman, H.E.; Yalcin, H.C. Computational Modeling of Blood Flow Hemodynamics for Biomechanical Investigation of Cardiac Development and Disease. J. Cardiovasc. Dev. Dis. 2021, 8, 14. [Google Scholar] [CrossRef]
- Doyle, B.J.; McGloughlin, T.M.; Kavanagh, E.G.; Hoskins, P.R. From Detection to Rupture: A Serial Computational Fluid Dynamics Case Study of a Rapidly Expanding, Patient-Specific, Ruptured Abdominal Aortic Aneurysm. In Computational Biomechanics for Medicine; Springer: New York, NY, USA, 2014; pp. 53–68. [Google Scholar]
- Les, A.S.; Shadden, S.C.; Figueroa, C.A.; Park, J.M.; Tedesco, M.M.; Herfkens, R.J.; Dalman, R.L.; Taylor, C.A. Quantification of Hemodynamics in Abdominal Aortic Aneurysms during Rest and Exercise Using Magnetic Resonance Imaging and Computational Fluid Dynamics. Ann. Biomed. Eng. 2010, 38, 1288–1313. [Google Scholar] [CrossRef]
- Soudah, E.; Ng, E.Y.K.; Loong, T.H.; Bordone, M.; Pua, U.; Narayanan, S. CFD Modelling of Abdominal Aortic Aneurysm on Hemodynamic Loads Using a Realistic Geometry with CT. Comput. Math. Methods Med. 2013, 2013, 472564. [Google Scholar] [CrossRef] [PubMed]
- Boyd, A.J.; Kuhn, D.C.S.; Lozowy, R.J.; Kulbisky, G.P. Low wall shear stress predominates at sites of abdominal aortic aneurysm rupture. J. Vasc. Surg. 2016, 63, 1613–1619. [Google Scholar] [CrossRef] [PubMed]
- Belkacemi, D.; Al-Rawi, M.; Abbes, M.T.; Laribi, B. Flow Behaviour and Wall Shear Stress Derivatives in Abdominal Aortic Aneurysm Models: A Detailed CFD Analysis into Asymmetry Effect. CFD Lett. 2022, 14, 60–74. [Google Scholar] [CrossRef]
- Franck, G.; Dai, J.; Fifre, A.; Ngo, S.; Justine, C.; Michineau, S.; Allaire, E.; Gervais, M. Reestablishment of the Endothelial Lining by Endothelial Cell Therapy Stabilizes Experimental Abdominal Aortic Aneurysms. Circulation 2013, 127, 1877–1887. [Google Scholar] [CrossRef]
- Zambrano, B.A.; Gharahi, H.; Lim, C.; Jaberi, F.A.; Choi, J.; Lee, W.; Baek, S. Association of Intraluminal Thrombus, Hemodynamic Forces, and Abdominal Aortic Aneurysm Expansion Using Longitudinal CT Images. Ann. Biomed. Eng. 2016, 44, 1502–1514. [Google Scholar] [CrossRef] [PubMed]
- Zambrano, B.A.; Gharahi, H.; Lim, C.Y.; Lee, W.; Baek, S. Association of vortical structures and hemodynamic parameters for regional thrombus accumulation in abdominal aortic aneurysms. Int. J. Numer. Methods Biomed. Eng. 2022, 38, e3555. [Google Scholar] [CrossRef] [PubMed]
- Di Achille, P.; Tellides, G.; Figueroa, C.A.; Humphrey, J.D. A haemodynamic predictor of intraluminal thrombus formation in abdominal aortic aneurysms. Proc. R. Soc. A Math. Phys. Eng. Sci. 2014, 470, 20140163. [Google Scholar] [CrossRef]
- Meng, H.; Tutino, V.M.; Xiang, J.; Siddiqui, A. High WSS or Low WSS? Complex Interactions of Hemodynamics with Intracranial Aneurysm Initiation, Growth, and Rupture: Toward a Unifying Hypothesis. Am. J. Neuroradiol. 2014, 35, 1254. [Google Scholar] [CrossRef]
- Fanni, B.M.; Antonuccio, M.N.; Pizzuto, A.; Berti, S.; Santoro, G.; Celi, S. Uncertainty Quantification in the In Vivo Image-Based Estimation of Local Elastic Properties of Vascular Walls. J. Cardiovasc. Dev. Dis. 2023, 10, 109. [Google Scholar] [CrossRef]
- Xie, J.; Zhou, J.; Fung, Y.C. Bending of Blood Vessel Wall: Stress-Strain Laws of the Intima-Media and Adventitial Layers. J. Biomech. Eng. 1995, 117, 136–145. [Google Scholar] [CrossRef]
- Peña, J.A.; Martínez, M.A.; Peña, E. Failure damage mechanical properties of thoracic and abdominal porcine aorta layers and related constitutive modeling: Phenomenological and microstructural approach. Biomech. Model. Mechanobiol. 2019, 18, 1709–1730. [Google Scholar] [CrossRef] [PubMed]
- Simsek, F.G.; Kwon, Y.W. Investigation of material modeling in fluid–structure interaction analysis of an idealized three-layered abdominal aorta: Aneurysm initiation and fully developed aneurysms. J. Biol. Phys. 2015, 41, 173–201. [Google Scholar] [CrossRef] [PubMed]
- O’Leary, S.A.; Mulvihill, J.J.; Barrett, H.E.; Kavanagh, E.G.; Walsh, M.T.; McGloughlin, T.M.; Doyle, B.J. Determining the influence of calcification on the failure properties of abdominal aortic aneurysm (AAA) tissue. J. Mech. Behav. Biomed. Mater. 2015, 42, 154–167. [Google Scholar] [CrossRef]
- Marra, S.P.; Daghlian, C.P.; Fillinger, M.F.; Kennedy, F.E. Elemental composition, morphology and mechanical properties of calcified deposits obtained from abdominal aortic aneurysms. Acta Biomater. 2006, 2, 515–520. [Google Scholar] [CrossRef] [PubMed]
- Fillinger, M.F.; Marra, S.P.; Raghavan, M.L.; Kennedy, F.E. Prediction of rupture risk in abdominal aortic aneurysm during observation: Wall stress versus diameter. J. Vasc. Surg. 2003, 37, 724–732. [Google Scholar] [CrossRef]
- Gasser, T.C. Biomechanical Rupture Risk Assessment. Aorta 2016, 4, 42–60. [Google Scholar] [CrossRef]
- Wang, D.H.J.; Makaroun, M.S.; Webster, M.W.; Vorp, D.A. Effect of intraluminal thrombus on wall stress in patient-specific models of abdominal aortic aneurysm. J. Vasc. Surg. 2002, 36, 598–604. [Google Scholar] [CrossRef]
- Georgakarakos, E.; Ioannou, C.V.; Kamarianakis, Y.; Papaharilaou, Y.; Kostas, T.; Manousaki, E.; Katsamouris, A.N. The Role of Geometric Parameters in the Prediction of Abdominal Aortic Aneurysm Wall Stress. Eur. J. Vasc. Endovasc. Surg. 2010, 39, 42–48. [Google Scholar] [CrossRef]
- Wolters, B.J.B.M.; Rutten, M.C.M.; Schurink, G.W.H.; Kose, U.; de Hart, J.; van de Vosse, F.N. A patient-specific computational model of fluid–structure interaction in abdominal aortic aneurysms. Med. Eng. Phys. 2005, 27, 871–883. [Google Scholar] [CrossRef]
- Smith, C.R.; Leon, M.B.; Mack, M.J.; Miller, D.C.; Moses, J.W.; Svensson, L.G.; Tuzcu, E.M.; Webb, J.G.; Fontana, G.P.; Makkar, R.R.; et al. Transcatheter versus Surgical Aortic-Valve Replacement in High-Risk Patients. N. Engl. J. Med. 2011, 364, 2187–2198. [Google Scholar] [CrossRef]
- Raghavan, M.L.; Kratzberg, J.; Castro de Tolosa, E.M.; Hanaoka, M.M.; Walker, P.; da Silva, E.S. Regional distribution of wall thickness and failure properties of human abdominal aortic aneurysm. J. Biomech. 2006, 39, 3010–3016. [Google Scholar] [CrossRef] [PubMed]
- Malkawi, A.H.; Hinchliffe, R.J.; Xu, Y.; Holt, P.J.; Loftus, I.M.; Thompson, M.M. Patient-specific biomechanical profiling in abdominal aortic aneurysm development and rupture. J. Vasc. Surg. 2010, 52, 480–488. [Google Scholar] [CrossRef] [PubMed]
- Scotti, C.M.; Jimenez, J.; Muluk, S.C.; Finol, E.A. Wall stress and flow dynamics in abdominal aortic aneurysms: Finite element analysis vs. fluid–structure interaction. Comput. Methods Biomech. Biomed. Eng. 2008, 11, 301–322. [Google Scholar] [CrossRef] [PubMed]
- Doyle, B.J.; Callanan, A.; McGloughlin, T.M. A comparison of modelling techniques for computing wall stress in abdominal aortic aneurysms. Biomed. Eng. Online 2007, 6, 38. [Google Scholar] [CrossRef]
- Ene, F.; Delassus, P.; Morris, L. The influence of computational assumptions on analysing abdominal aortic aneurysm haemodynamics. Proc. Inst. Mech. Eng. H 2014, 228, 768–780. [Google Scholar] [CrossRef]
- Baieth, H.E. Physical parameters of blood as a non-newtonian fluid. Int. J. Biomed. Sci. 2008, 4, 323–329. [Google Scholar]
- Tzirakis, K.; Kamarianakis, Y.; Kontopodis, N.; Ioannou, C.V. The Effect of Blood Rheology and Inlet Boundary Conditions on Realistic Abdominal Aortic Aneurysms under Pulsatile Flow Conditions. Bioengineering 2023, 10, 272. [Google Scholar] [CrossRef]
- Gijsen, F.J.H.; van de Vosse, F.N.; Janssen, J.D. The influence of the non-Newtonian properties of blood on the flow in large arteries: Steady flow in a carotid bifurcation model. J. Biomech. 1999, 32, 601–608. [Google Scholar] [CrossRef]
- Bernsdorf, J.; Wang, D. Non-Newtonian blood flow simulation in cerebral aneurysms. Comput. Math. Appl. 2009, 58, 1024–1029. [Google Scholar] [CrossRef]
- Khanafer, K.M.; Gadhoke, P.; Berguer, R.; Bull, J.L. Modeling pulsatile flow in aortic aneurysms: Effect of non-Newtonian properties of blood. Biorheology 2006, 43, 661–679. [Google Scholar]
- Jahangiri, M.; Saghafian, M.; Sadeghi, M.R. Numerical simulation of non-Newtonian models effect on hemodynamic factors of pulsatile blood flow in elastic stenosed artery. J. Mech. Sci. Technol. 2017, 31, 1003–1013. [Google Scholar] [CrossRef]
- Qiu, Y.; Yuan, D.; Wen, J.; Fan, Y.; Zheng, T. Numerical identification of the rupture locations in patient-specific abdominal aortic aneurysmsusing hemodynamic parameters. Comput. Methods Biomech. Biomed. Eng. 2018, 21, 1–12. [Google Scholar] [CrossRef] [PubMed]
- Salman, H.E.; Saltik, L.; Yalcin, H.C. Computational Analysis of Wall Shear Stress Patterns on Calcified and Bicuspid Aortic Valves: Focus on Radial and Coaptation Patterns. Fluids 2021, 6, 287. [Google Scholar] [CrossRef]
- Wilson, J.S.; Virag, L.; Di Achille, P.; Karšaj, I.; Humphrey, J.D. Biochemomechanics of Intraluminal Thrombus in Abdominal Aortic Aneurysms. J. Biomech. Eng. 2013, 135, 021011. [Google Scholar] [CrossRef]
- Vande Geest, J.P.; Sacks, M.S.; Vorp, D.A. A planar biaxial constitutive relation for the luminal layer of intra-luminal thrombus in abdominal aortic aneurysms. J. Biomech. 2006, 39, 2347–2354. [Google Scholar] [CrossRef] [PubMed]
- Chandra, S.; Raut, S.S.; Jana, A.; Biederman, R.W.; Doyle, M.; Muluk, S.C.; Finol, E.A. Fluid-Structure Interaction Modeling of Abdominal Aortic Aneurysms: The Impact of Patient-Specific Inflow Conditions and Fluid/Solid Coupling. J. Biomech. Eng. 2013, 135, 081001. [Google Scholar] [CrossRef]
- Raut, S.S.; Jana, A.; De Oliveira, V.; Muluk, S.C.; Finol, E.A. The Effect of Uncertainty in Vascular Wall Material Properties on Abdominal Aortic Aneurysm Wall Mechanics. In Computational Biomechanics for Medicine; Springer: New York, NY, USA, 2014; pp. 69–86. [Google Scholar]
- Reeps, C.; Gee, M.; Maier, A.; Gurdan, M.; Eckstein, H.-H.; Wall, W.A. The impact of model assumptions on results of computational mechanics in abdominal aortic aneurysm. J. Vasc. Surg. 2010, 51, 679–688. [Google Scholar] [CrossRef]
- Di Martino, E.S.; Guadagni, G.; Fumero, A.; Ballerini, G.; Spirito, R.; Biglioli, P.; Redaelli, A. Fluid–structure interaction within realistic three-dimensional models of the aneurysmatic aorta as a guidance to assess the risk of rupture of the aneurysm. Med. Eng. Phys. 2001, 23, 647–655. [Google Scholar] [CrossRef]
- Kelsey, L.J.; Powell, J.T.; Norman, P.E.; Miller, K.; Doyle, B.J. A comparison of hemodynamic metrics and intraluminal thrombus burden in a common iliac artery aneurysm. Int. J. Numer. Methods Biomed. Eng. 2017, 33, e2821. [Google Scholar] [CrossRef]
- Skalak, T.C.; Price, R.J. The Role of Mechanical Stresses in Microvascular Remodeling. Microcirculation 1996, 3, 143–165. [Google Scholar] [CrossRef]
- Budynas, R.G.; Nisbett, J.K. Shigley’s Mechanical Engineering Design; McGraw-Hill: New York, NY, USA, 2011; Volume 9. [Google Scholar]
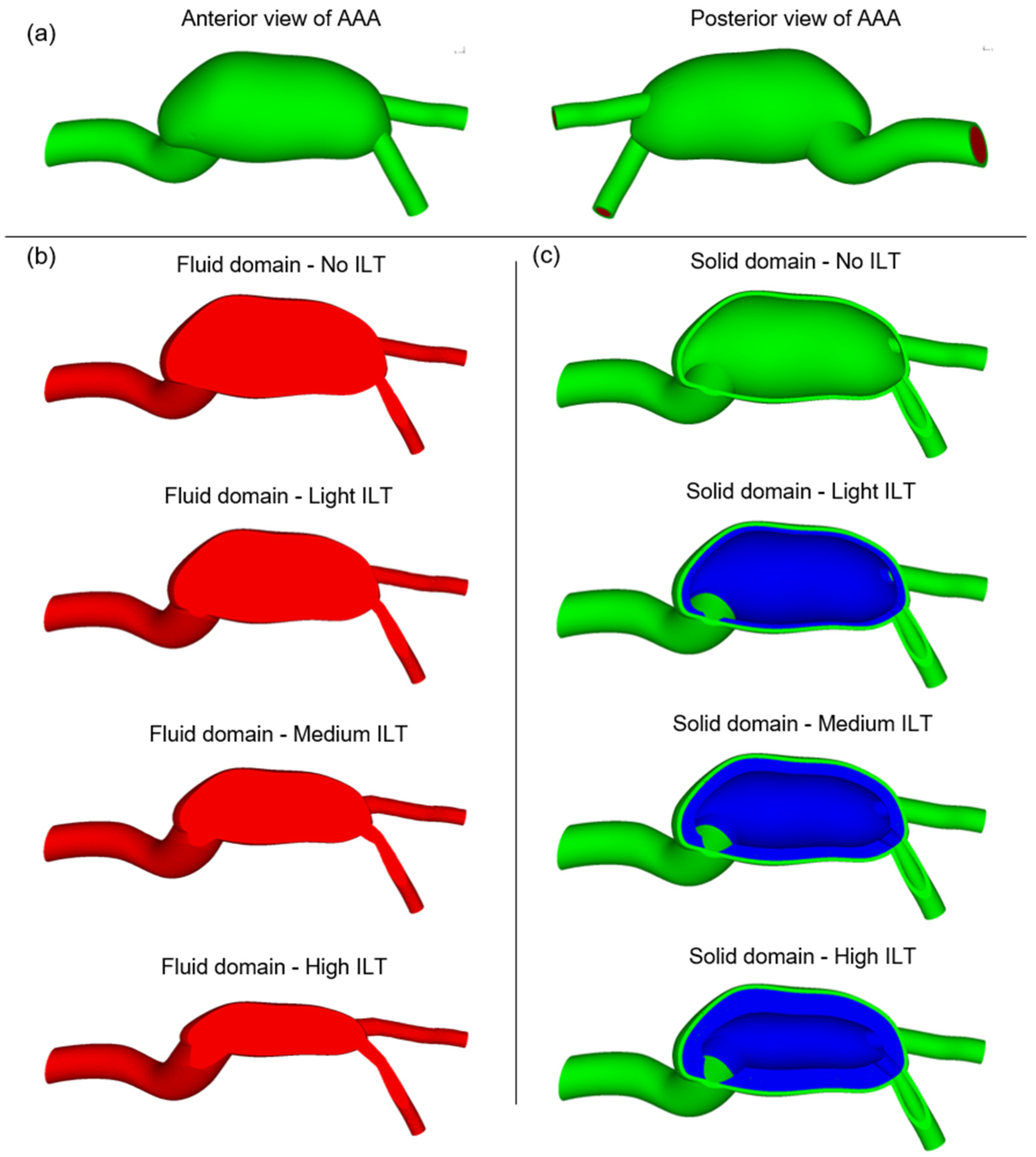

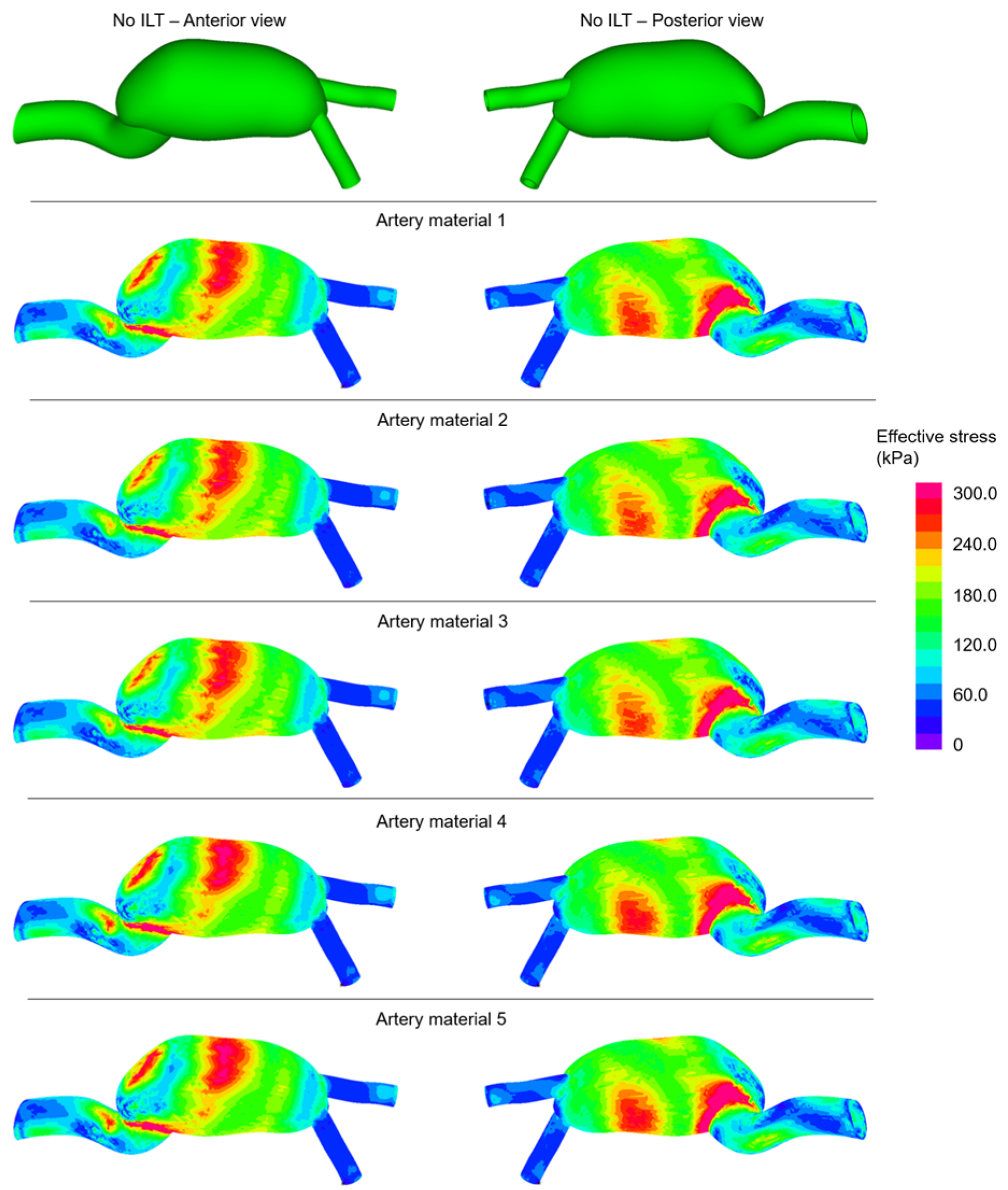
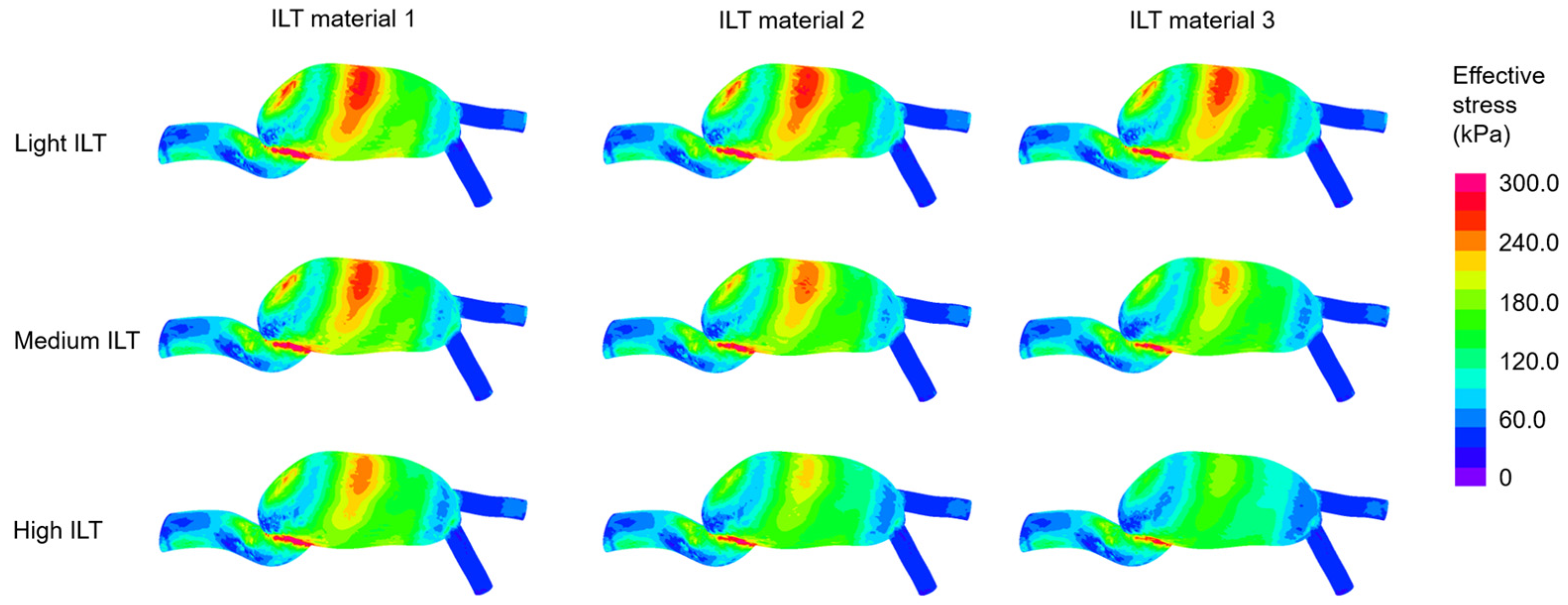
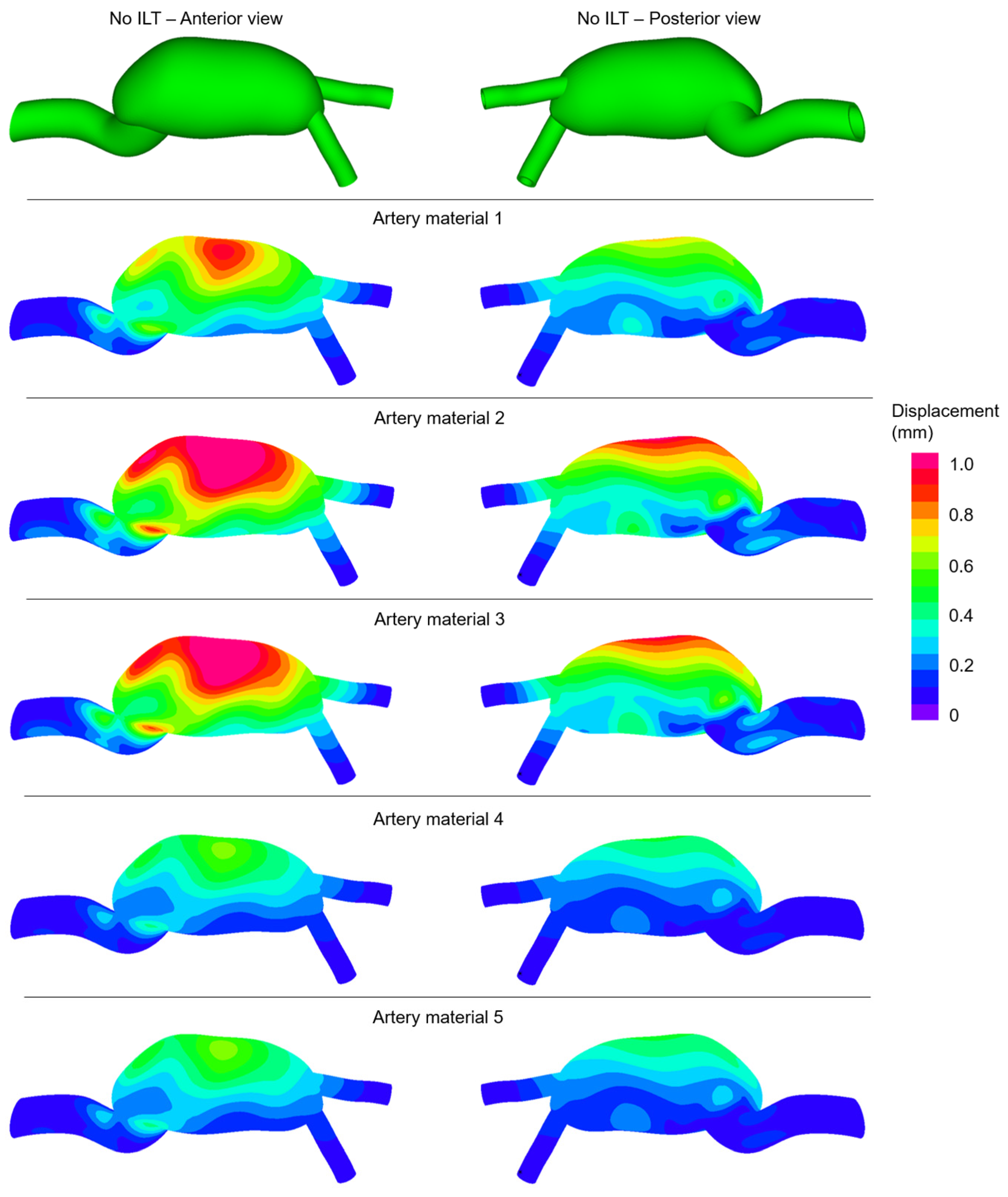

| Case | ILT Burden | ILT Material | Artery Material | Case | ILT Burden | ILT Material | Artery Material |
|---|---|---|---|---|---|---|---|
| 1 | No ILT | 1 | 1 | 26 | Medium | 2 | 1 |
| 2 | No ILT | 1 | 2 | 27 | Medium | 2 | 2 |
| 3 | No ILT | 1 | 3 | 28 | Medium | 2 | 3 |
| 4 | No ILT | 1 | 4 | 29 | Medium | 2 | 4 |
| 5 | No ILT | 1 | 5 | 30 | Medium | 2 | 5 |
| 6 | Light | 1 | 1 | 31 | Medium | 3 | 1 |
| 7 | Light | 1 | 2 | 32 | Medium | 3 | 2 |
| 8 | Light | 1 | 3 | 33 | Medium | 3 | 3 |
| 9 | Light | 1 | 4 | 34 | Medium | 3 | 4 |
| 10 | Light | 1 | 5 | 35 | Medium | 3 | 5 |
| 11 | Light | 2 | 1 | 36 | High | 1 | 1 |
| 12 | Light | 2 | 2 | 37 | High | 1 | 2 |
| 13 | Light | 2 | 3 | 38 | High | 1 | 3 |
| 14 | Light | 2 | 4 | 39 | High | 1 | 4 |
| 15 | Light | 2 | 5 | 40 | High | 1 | 5 |
| 16 | Light | 3 | 1 | 41 | High | 2 | 1 |
| 17 | Light | 3 | 2 | 42 | High | 2 | 2 |
| 18 | Light | 3 | 3 | 43 | High | 2 | 3 |
| 19 | Light | 3 | 4 | 44 | High | 2 | 4 |
| 20 | Light | 3 | 5 | 45 | High | 2 | 5 |
| 21 | Medium | 1 | 1 | 46 | High | 3 | 1 |
| 22 | Medium | 1 | 2 | 47 | High | 3 | 2 |
| 23 | Medium | 1 | 3 | 48 | High | 3 | 3 |
| 24 | Medium | 1 | 4 | 49 | High | 3 | 4 |
| 25 | Medium | 1 | 5 | 50 | High | 3 | 5 |
| ILT Material Model | Elastic Modulus (kPa) |
|---|---|
| 1 | 50 |
| 2 | 100 |
| 3 | 200 |
| Artery Material Model | (N/cm2) | (N/cm2) |
|---|---|---|
| 1 | 17.4 | 188.1 |
| 2 | 15.2 | 117.6 |
| 3 | 21.9 | 117.6 |
| 4 | 21.9 | 355.7 |
| 5 | 15.2 | 355.7 |
| Case | ILT Burden | ILT Material | Artery Material | Maximum Stress on Artery (kPa) | Case | ILT Burden | ILT Material | Artery Material | Maximum Stress on Artery (kPa) |
|---|---|---|---|---|---|---|---|---|---|
| 1 | No ILT | - | 1 | 719.8 | 26 | Medium | 2 | 1 | 610.2 |
| 2 | No ILT | - | 2 | 715.4 | 27 | Medium | 2 | 2 | 610.2 |
| 3 | No ILT | - | 3 | 714.5 | 28 | Medium | 2 | 3 | 609.0 |
| 4 | No ILT | - | 4 | 730.7 | 29 | Medium | 2 | 4 | 639.1 |
| 5 | No ILT | - | 5 | 730.5 | 30 | Medium | 2 | 5 | 637.2 |
| 6 | Light | 1 | 1 | 670.1 | 31 | Medium | 3 | 1 | 588.3 |
| 7 | Light | 1 | 2 | 666.2 | 32 | Medium | 3 | 2 | 580.3 |
| 8 | Light | 1 | 3 | 665.6 | 33 | Medium | 3 | 3 | 580.2 |
| 9 | Light | 1 | 4 | 671.0 | 34 | Medium | 3 | 4 | 599.4 |
| 10 | Light | 1 | 5 | 671.2 | 35 | Medium | 3 | 5 | 597.3 |
| 11 | Light | 2 | 1 | 664.2 | 36 | High | 1 | 1 | 619.2 |
| 12 | Light | 2 | 2 | 661.7 | 37 | High | 1 | 2 | 613.6 |
| 13 | Light | 2 | 3 | 660.9 | 38 | High | 1 | 3 | 613.2 |
| 14 | Light | 2 | 4 | 661.9 | 39 | High | 1 | 4 | 624.2 |
| 15 | Light | 2 | 5 | 662.2 | 40 | High | 1 | 5 | 624.3 |
| 16 | Light | 3 | 1 | 656.1 | 41 | High | 2 | 1 | 579.5 |
| 17 | Light | 3 | 2 | 649.7 | 42 | High | 2 | 2 | 564.5 |
| 18 | Light | 3 | 3 | 649.5 | 43 | High | 2 | 3 | 565.5 |
| 19 | Light | 3 | 4 | 656.9 | 44 | High | 2 | 4 | 593.6 |
| 20 | Light | 3 | 5 | 657.1 | 45 | High | 2 | 5 | 593.4 |
| 21 | Medium | 1 | 1 | 635.9 | 46 | High | 3 | 1 | 540.6 |
| 22 | Medium | 1 | 2 | 641.6 | 47 | High | 3 | 2 | 513.4 |
| 23 | Medium | 1 | 3 | 639.5 | 48 | High | 3 | 3 | 516.0 |
| 24 | Medium | 1 | 4 | 676.6 | 49 | High | 3 | 4 | 567.7 |
| 25 | Medium | 1 | 5 | 674.9 | 50 | High | 3 | 5 | 567.2 |
| Case | ILT Burden | ILT Material | Artery Material | Maximum Displacement on Artery (mm) | Case | ILT Burden | ILT Material | Artery Material | Maximum Displacement on Artery (mm) |
|---|---|---|---|---|---|---|---|---|---|
| 1 | No ILT | - | 1 | 1.03 | 26 | Medium | 2 | 1 | 1.09 |
| 2 | No ILT | - | 2 | 1.44 | 27 | Medium | 2 | 2 | 1.41 |
| 3 | No ILT | - | 3 | 1.38 | 28 | Medium | 2 | 3 | 1.37 |
| 4 | No ILT | - | 4 | 0.64 | 29 | Medium | 2 | 4 | 0.77 |
| 5 | No ILT | - | 5 | 0.65 | 30 | Medium | 2 | 5 | 0.78 |
| 6 | Light | 1 | 1 | 0.97 | 31 | Medium | 3 | 1 | 0.92 |
| 7 | Light | 1 | 2 | 1.33 | 32 | Medium | 3 | 2 | 1.21 |
| 8 | Light | 1 | 3 | 1.28 | 33 | Medium | 3 | 3 | 1.17 |
| 9 | Light | 1 | 4 | 0.62 | 34 | Medium | 3 | 4 | 0.63 |
| 10 | Light | 1 | 5 | 0.63 | 35 | Medium | 3 | 5 | 0.64 |
| 11 | Light | 2 | 1 | 0.91 | 36 | High | 1 | 1 | 1.76 |
| 12 | Light | 2 | 2 | 1.24 | 37 | High | 1 | 2 | 2.10 |
| 13 | Light | 2 | 3 | 1.20 | 38 | High | 1 | 3 | 2.05 |
| 14 | Light | 2 | 4 | 0.59 | 39 | High | 1 | 4 | 1.44 |
| 15 | Light | 2 | 5 | 0.59 | 40 | High | 1 | 5 | 1.45 |
| 16 | Light | 3 | 1 | 0.88 | 41 | High | 2 | 1 | 1.20 |
| 17 | Light | 3 | 2 | 1.19 | 42 | High | 2 | 2 | 1.48 |
| 18 | Light | 3 | 3 | 1.16 | 43 | High | 2 | 3 | 1.45 |
| 19 | Light | 3 | 4 | 0.57 | 44 | High | 2 | 4 | 0.92 |
| 20 | Light | 3 | 5 | 0.58 | 45 | High | 2 | 5 | 0.92 |
| 21 | Medium | 1 | 1 | 1.38 | 46 | High | 3 | 1 | 0.89 |
| 22 | Medium | 1 | 2 | 1.73 | 47 | High | 3 | 2 | 1.12 |
| 23 | Medium | 1 | 3 | 1.69 | 48 | High | 3 | 3 | 1.09 |
| 24 | Medium | 1 | 4 | 1.03 | 49 | High | 3 | 4 | 0.65 |
| 25 | Medium | 1 | 5 | 1.04 | 50 | High | 3 | 5 | 0.66 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Arslan, A.C.; Salman, H.E. Effect of Intraluminal Thrombus Burden on the Risk of Abdominal Aortic Aneurysm Rupture. J. Cardiovasc. Dev. Dis. 2023, 10, 233. https://doi.org/10.3390/jcdd10060233
Arslan AC, Salman HE. Effect of Intraluminal Thrombus Burden on the Risk of Abdominal Aortic Aneurysm Rupture. Journal of Cardiovascular Development and Disease. 2023; 10(6):233. https://doi.org/10.3390/jcdd10060233
Chicago/Turabian StyleArslan, Aykut Can, and Huseyin Enes Salman. 2023. "Effect of Intraluminal Thrombus Burden on the Risk of Abdominal Aortic Aneurysm Rupture" Journal of Cardiovascular Development and Disease 10, no. 6: 233. https://doi.org/10.3390/jcdd10060233
APA StyleArslan, A. C., & Salman, H. E. (2023). Effect of Intraluminal Thrombus Burden on the Risk of Abdominal Aortic Aneurysm Rupture. Journal of Cardiovascular Development and Disease, 10(6), 233. https://doi.org/10.3390/jcdd10060233






