Analytical Study of Stress Distributions around Screws in Flat Mandibular Bone under In-Plane Loading
Abstract
1. Introduction
2. Stress Analysis
- (1).
- The screws are supposed to be symmetrically loaded herein. As reported, through-thickness bicortical screw placement is often used for the rigid fixation of mandibular fractures [19]. Thus, an in-plane loaded rigid pin in a through hole was considered reasonable here. The effects of transverse pull-out loads and contributions from large moments deserve special attention and necessitate a full three-dimensional finite element study.
- (2).
- (3).
- The screw was assumed to be infinitely rigid. According to Hyer et al. [22], the stiffness of the pin has a minor effect on stress distribution around the hole.
- (4).
- Threads on the screw and screw hole were neglected.
- (5).
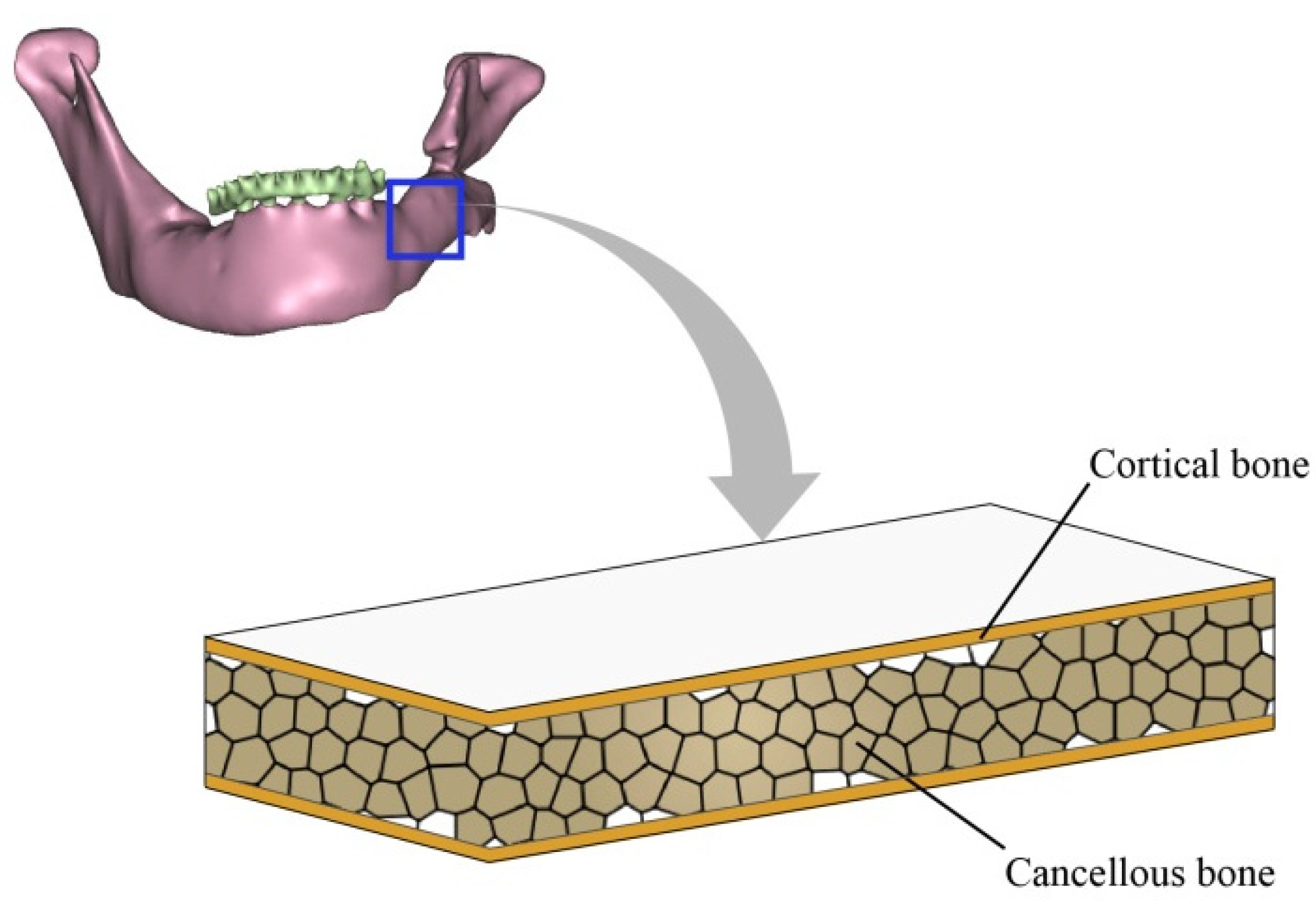
- The location of the screw hole in the bone was assumed to be far away from the edge of the bone. The bone was considered to be of uniform thickness and flat in the vicinity of the screw.
- (6).
- The contact between the screw and the bone was considered, and the friction coefficient was assumed to be constant over the contact region.
2.1. Elastic Properties of Human Bones
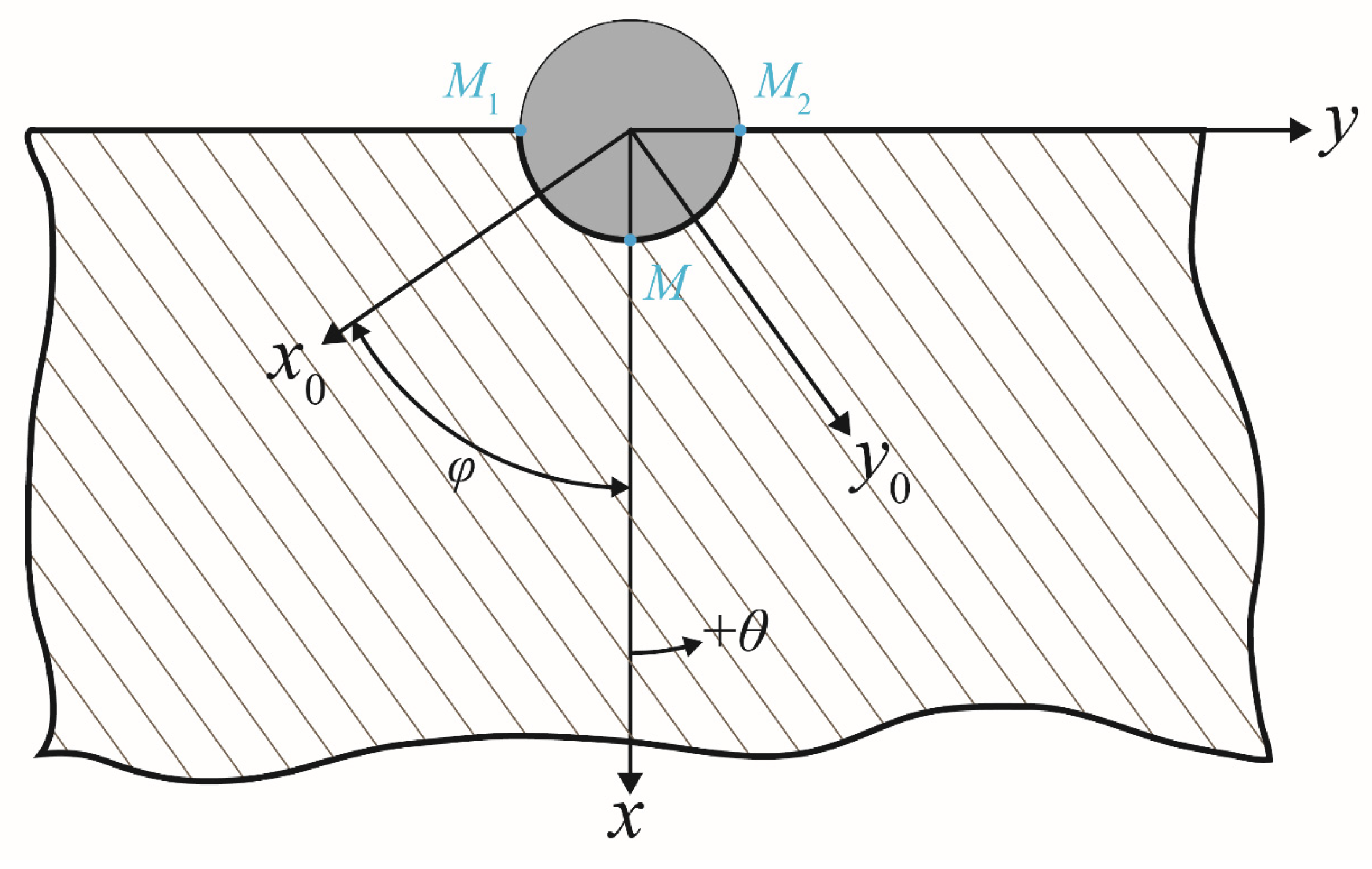
2.2. Stress Analysis Based on Complex Stress Functions
2.2.1. General Solution of Stress Functions
2.2.2. Boundary Conditions
2.2.3. Exact Solution of Stresses
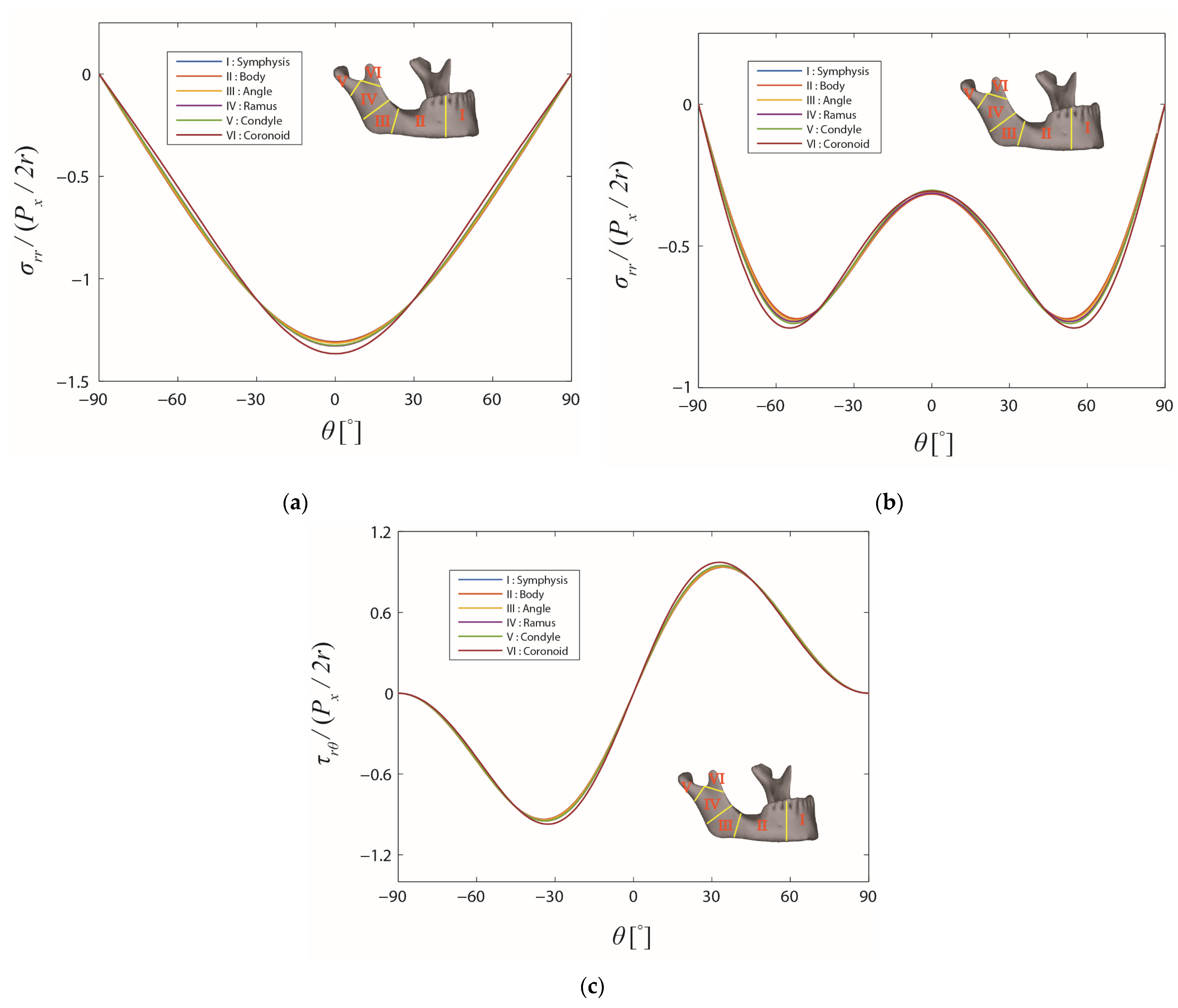
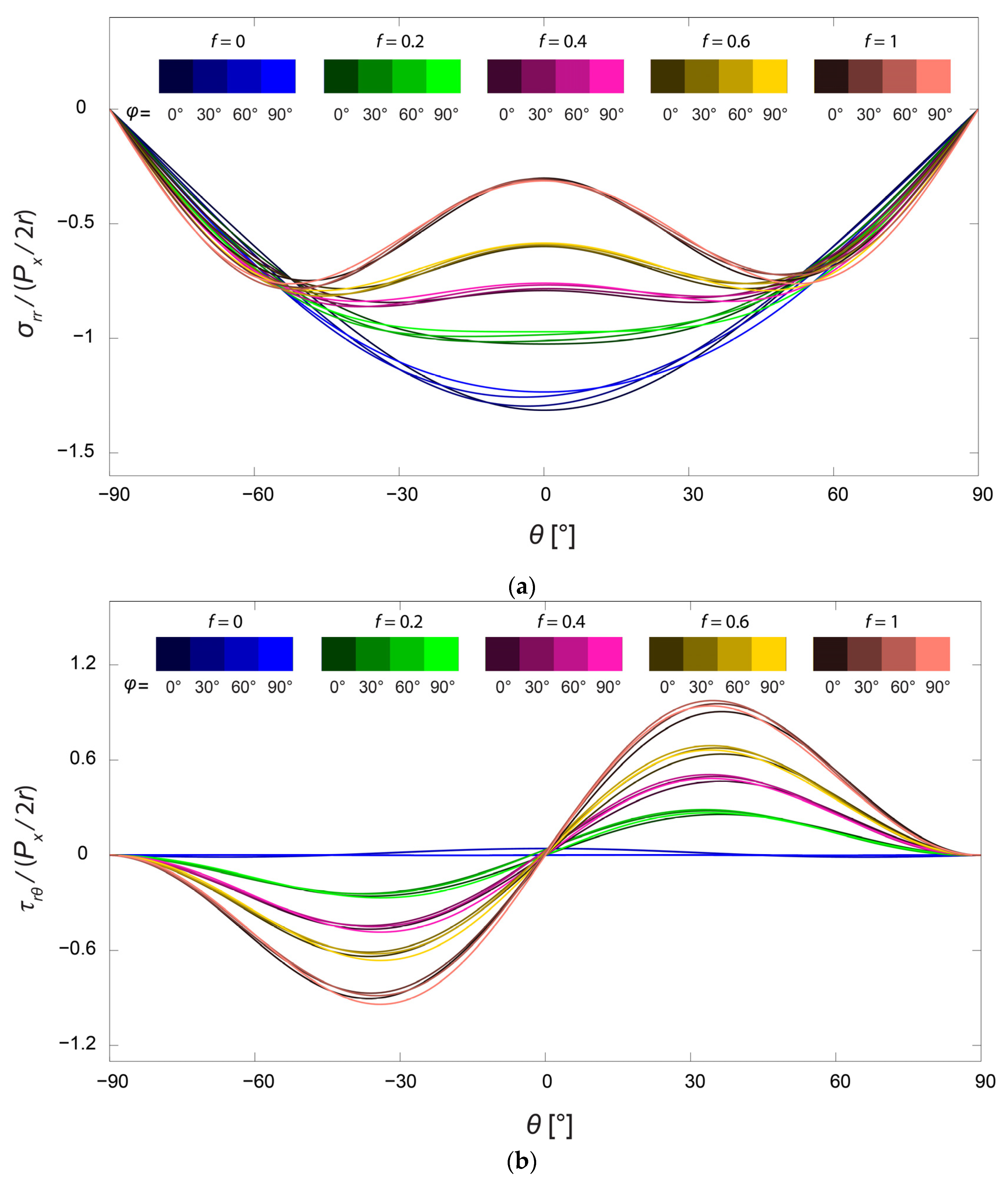
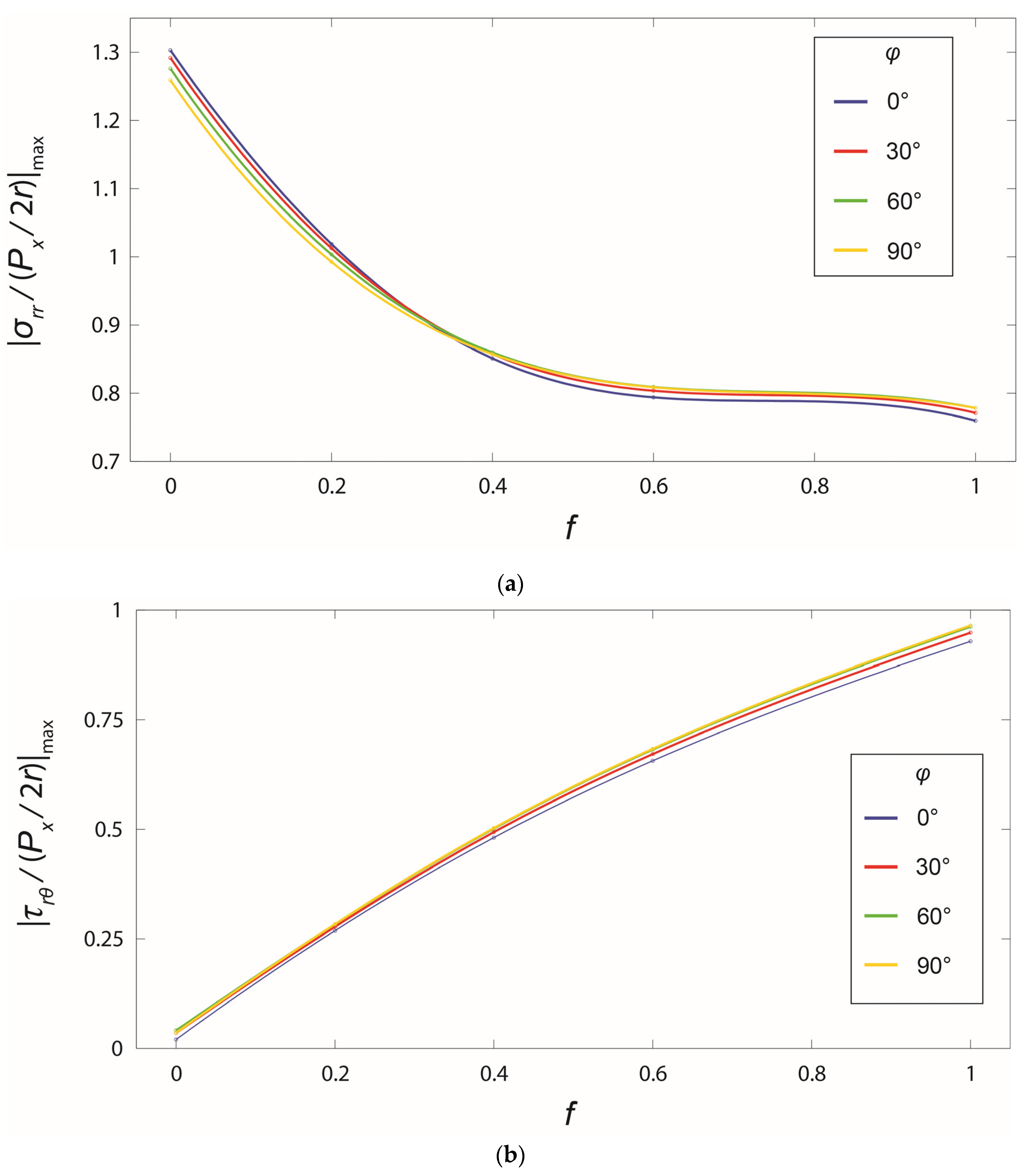
3. Results and Discussions
3.1. Average Stresses over the Cross-Section of the Laminated Bone
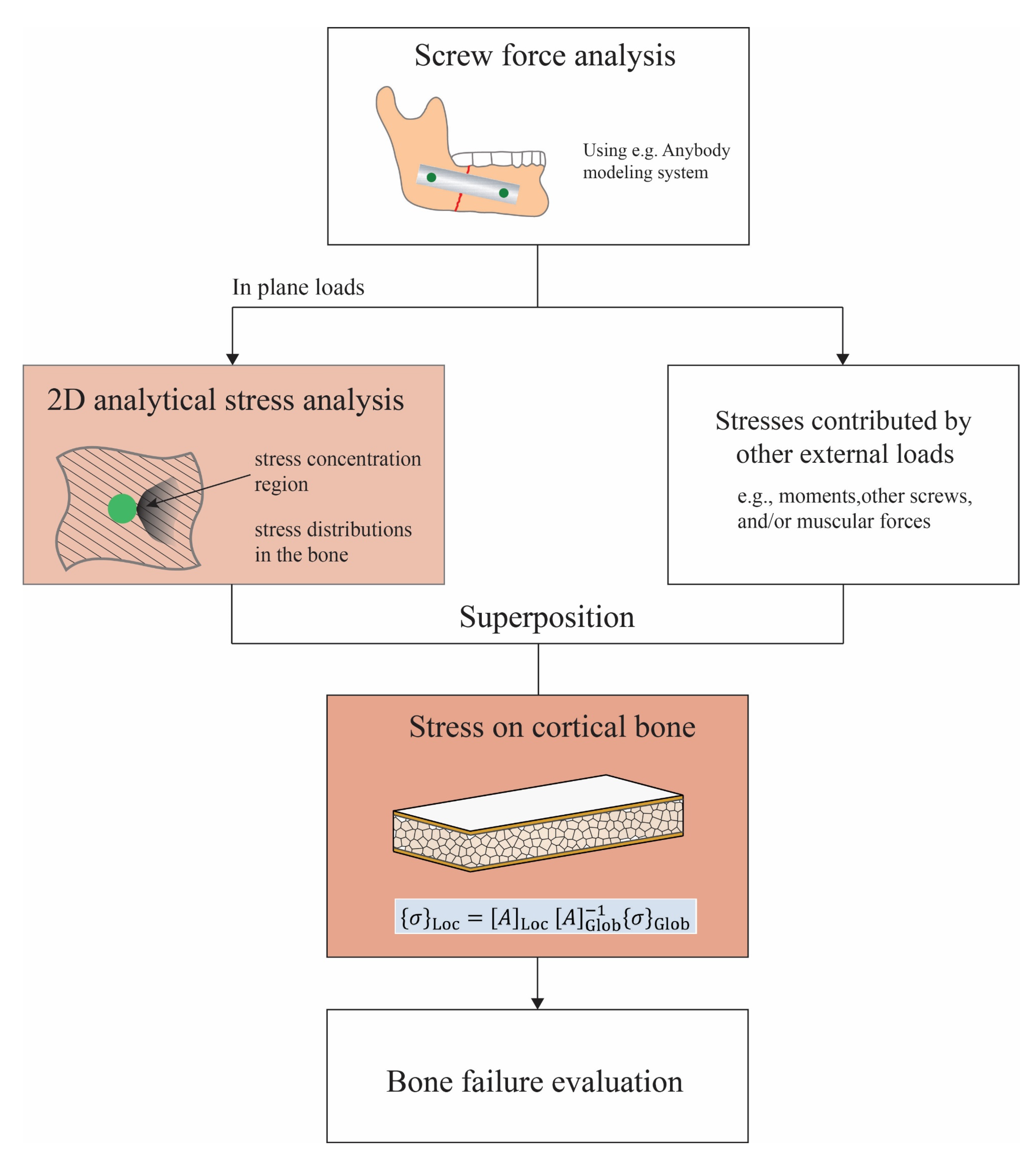
3.2. Potential Applications of the Analytical Model
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Brumback, R.J. The Rationales of Interlocking Nailing of the Femur, Tibia, and Humerus: An overview. Clin. Orthop. Relat. Res. 1996, 324, 292–320. [Google Scholar] [CrossRef]
- Lowery, G.L.; McDonough, R.F. The Significance of Hardware Failure in Anterior Cervical Plate Fixation. Patients with 2- to 7-year follow-up. Spine 1998, 23, 181–186. [Google Scholar] [CrossRef]
- Wimmer, C.; Gluch, H. Aseptic loosening after CD instrumentation in the treatment of scoliosis: A report about eight cases. J. Spinal Disord. 1998, 11, 440–443. [Google Scholar] [CrossRef] [PubMed]
- Xie, S.; Manda, K.; Pankaj, P. Time-dependent behaviour of bone accentuates loosening in the fixation of fractures using bone-screw systems. Bone Jt. Res. 2018, 7, 580–586. [Google Scholar] [CrossRef] [PubMed]
- Yamamoto, M.K.; D’Avila, R.P.; De Cerqueira Luz, J.G. Evaluation of surgical retreatment of mandibular fractures. J. Craniomaxillofac. Surg. 2013, 41, 42–46. [Google Scholar] [CrossRef]
- Soltesz, U.; Siegele, D.; Riedmueller, J.; Schulz, P. Stress concentration and bone resorption in the jaw for dental implants with shoulders. In Proceedings of the European Conference on Biomaterials, Gothenburg, Sweden, 27–29 August 1981. [Google Scholar]
- Huiskes, R.; Nunamaker, D. Local stresses and bone adaption around orthopedic implants. Calcif. Tissue Int. 1984, 36, S110–S117. [Google Scholar] [CrossRef]
- Crupi, V.; Guglielmino, E.; La Rosa, G.; Vander Sloten, J.; Van Oosterwyck, H. Numerical analysis of bone adaptation around an oral implant due to overload stress. Proc. Inst. Mech. Eng. Part H J. Eng. Med. 2004, 218, 407–415. [Google Scholar] [CrossRef] [PubMed]
- Li, J.; Li, H.; Shi, L.; Fok, A.S.; Ucer, C.; Devlin, H.; Horner, K.; Silikas, N. A mathematical model for simulating the bone remodeling process under mechanical stimulus. Dent. Mater. 2007, 23, 1073–1078. [Google Scholar] [CrossRef]
- Kozlovsky, A.; Tal, H.; Laufer, B.Z.; Leshem, R.; Rohrer, M.D.; Weinreb, M.; Artzi, Z. Impact of implant overloading on the peri-implant bone in inflamed and non-inflamed peri-implant mucosa. Clin. Oral Implant. Res. 2007, 18, 601–610. [Google Scholar] [CrossRef]
- Gefen, A. Computational simulations of stress shielding and bone resorption around existing and computer-designed orthopaedic screws. Med. Biol. Eng. Comput. 2002, 40, 311–322. [Google Scholar] [CrossRef]
- Kitamura, E.; Stegaroiu, R.; Nomura, S.; Miyakawa, O. Biomechanical aspects of marginal bone resorption around osseointegrated implants: Considerations based on a three-dimensional finite element analysis. Clin. Oral Implant. Res. 2004, 15, 401–412. [Google Scholar] [CrossRef] [PubMed]
- Baggi, L.; Cappelloni, I.; Di Girolamo, M.; Maceri, F.; Vairo, G. The influence of implant diameter and length on stress distribution of osseointegrated implants related to crestal bone geometry: A three-dimensional finite element analysis. J. Prosthet. Dent. 2008, 100, 422–431. [Google Scholar] [CrossRef]
- De Jong, T. Stresses Around Pin-Loaded Holes in Elastically Orthotropic or Isotropic Plates. J. Compos. Mater. 1977, 11, 313–331. [Google Scholar] [CrossRef]
- Hyer, M.W.; Liu, D. Photoelastic determination of stresses in multiple-pin connectors. Exp. Mech. 1983, 23, 249–256. [Google Scholar] [CrossRef]
- Chang, F.-K.; Scott, R.A. Springer GS. Failure of composite laminates containing pin loaded holes—Method of solution. J. Compos. Mater. 1984, 18, 255–278. [Google Scholar] [CrossRef]
- Hyer, M.W.; Klang, E.C. Contact stresses in pin-loaded orthotropic plates. Int. J. Solids Struct. 1985, 21, 957–975. [Google Scholar] [CrossRef]
- Zhang, K.D.; Ueng, C.E.S. Stresses around a pin-loaded hole in orthotropic plates with arbitrary loading direction. Compos. Struct. 1985, 3, 119–143. [Google Scholar] [CrossRef]
- Beaty, N.B.; Le, T.T. Mandibular thickness measurements in young dentate adults. Arch. Otolaryngol. Head Neck Surg. 2009, 135, 920–923. [Google Scholar] [CrossRef]
- Matthews, F.L.; Hirst, I.R. The variation of bearing strength with load direction. In Proceedings of the Symposium: Jointing in Fibre Reinforced Plastics, London, UK, 4–5 September 1978. [Google Scholar]
- Godwin, E.; Matthews, F. A review of the strength of joints in fibre-reinforced plastics: Part 1. Mechanically fastened joints. Composites 1980, 11, 155–160. [Google Scholar] [CrossRef]
- Hyer, M.W.; Klang, E.C.; Cooper, D.E. The effects of pin elasticity, clearance, and friction on the stresses in a pin-loaded orthotropic plate. J. Compos. Mater. 1987, 21, 190–206. [Google Scholar] [CrossRef]
- Richmond, B.G.; Wright, B.W.; Grosse, I.; Dechow, P.C.; Ross, C.F.; Spencer, M.A.; Strait, D.S. Finite element analysis in functional morphology. Anat. Rec. Part A Discov. Mol. Cell. Evol. Biol. 2005, 283, 259–274. [Google Scholar] [CrossRef] [PubMed]
- Ichim, I.; Swain, M.V.; Kieser, J.A. Mandibular stiffness in humans: Numerical predictions. J. Biomech. 2006, 39, 1903–1913. [Google Scholar] [CrossRef] [PubMed]
- Natali, A.N.; Hart, R.T.; Pavan, P.G.; Knets, I. Mechanics of bone tissue. In Dental Biomechanics, 1st ed.; Natali, A.N., Ed.; Taylor & Francis: London, UK, 2003; pp. 1–19. [Google Scholar]
- O’Mahony, A.M.; Williams, J.L.; Katz, J.O.; Spencer, P. Anisotropic elastic properties of cancellous bone from a human edentulous mandible. Clin. Oral Implant. Res. 2000, 11, 415–421. [Google Scholar] [CrossRef] [PubMed]
- Giesen, E.B.W.; Ding, M.; Dalstra, M.; van Eijden, T.M.G.J. Mechanical properties of cancellous bone in the huaman mandibular condyle are anisotropic. J. Biomech. 2001, 34, 799–803. [Google Scholar] [CrossRef]
- Chaudhary, N.; Lovald, S.T.; Wagner, J.; Khraishi, T.; Baack, B. Experimental and Numerical Modeling of Screws Used for Rigid Internal Fixation of Mandibular Fractures. Model. Simul. Eng. 2008, 2008, 628120. [Google Scholar] [CrossRef]
- Schwartz-Dabney, C.; Dechow, P. Variations in cortical material properties throughout the human dentate mandible. Am. J. Phys. Anthropol. 2003, 120, 252–277. [Google Scholar] [CrossRef]
- Cruz, M.; Wassall, T.; Toledo, E.M.; Barra, L.P.; Lemonge, A.C. Three-dimensional finite element stress analysis of a cuneiform-geometry implant. Int. J. Oral Maxillofac. Implant. 2003, 18, 675–684. [Google Scholar]
- Kitamura, E.; Stegaroiu, R.; Nomura, S.; Miyakawa, O. Influence of marginal bone resorption on stress around an implant—A three-dimensional finite element analysis. J. Oral Rehabil. 2005, 32, 279–286. [Google Scholar] [CrossRef]
- Hyer, M.W. Stress Analysis of Fiber-Reinforced Composite Materials; DEStech Publications: Lancaster, PA, USA, 2009. [Google Scholar]
- Lekhnitskii, S.G. Anisotropic Plates, 2nd ed.; Gordon and Breach: New York, NY, USA, 1968. [Google Scholar]
- Tan, S.C. Laminated composites containing an elliptical opening. II. Experiment and model modification. J. Compos. Mater. 1987, 21, 949–968. [Google Scholar] [CrossRef]
- Tan, S.C. Finite-width correction factors for anisotropic plate containing a central opening. J. Compos. Mater. 1988, 22, 1080–1097. [Google Scholar] [CrossRef]
- Biemond, J.E.; Aquarius, R.; Verdonschot, N.; Buma, P. Frictional and bone ingrowth properties of engineered surface topographies produced by electron beam technology. Arch. Orthop. Trauma Surg. 2011, 131, 711–718. [Google Scholar] [CrossRef] [PubMed]
- Perez, R.; Oeltjen, J.C.; Thaller, S.R. A Review of Mandibular Angle Fractures. Craniomaxillofac. Trauma Reconstr. 2011, 4, 69–72. [Google Scholar] [CrossRef] [PubMed]
- Huang, H.-L.; Tsai, M.-T.; Hsu, J.-T.; Fuh, L.-J.; Tu, M.-G.; Wu, A.Y.-J. Do Threaded Size and Surface Roughness Affect the Bone Stress and Bone-Implant Interfacial Sliding of Titanium Dental Implant? In Proceedings of the World Congress on Engineering, London, UK, 6–8 July 2011.
- Basafa, E.; Murphy, R.J.; Gordon, C.R.; Armand, M. Modeling the biomechanics of swine mastication—An inverse dynamics approach. J. Biomech. 2014, 47, 2626–2632. [Google Scholar] [CrossRef]
- Doblaré, M.; Garcia, J.M.; Gómez-Benito, M.J. Modelling bone tissue fracture and healing: A review. Eng. Fract. Mech. 2004, 71, 1809–1840. [Google Scholar] [CrossRef]

| Positions in Mandible | Elastic Moduli (GPa) | Poisson Ratios | Shear Moduli (GPa) | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Symphysis | 20.49 | 16.35 | 12.09 | 0.34 | 0.22 | 0.43 | 6.91 | 4.83 | 5.32 |
| Body | 21.73 | 17.83 | 12.70 | 0.34 | 0.20 | 0.45 | 7.45 | 5.51 | 5.53 |
| Angle | 23.79 | 19.01 | 12.76 | 0.3 | 0.22 | 0.41 | 7.58 | 4.99 | 5.49 |
| Ramus | 24.61 | 18.36 | 12.97 | 0.28 | 0.23 | 0.38 | 7.41 | 5.01 | 5.39 |
| Condyle | 23.50 | 17.85 | 12.65 | 0.24 | 0.25 | 0.32 | 7.15 | 5.15 | 5.50 |
| Coronoid | 28.00 | 17.50 | 14.00 | 0.23 | 0.28 | 0.28 | 7.15 | 5.30 | 5.75 |
| Mandible | (GPa) | (GPa) | (GPa) | |
|---|---|---|---|---|
| Symphysis | 5.20 | 4.37 | 1.80 | 0.33 |
| Body | 9.51 | 7.95 | 3.30 | 0.34 |
| Angle | 12.58 | 10.20 | 4.06 | 0.30 |
| Ramus | 15.31 | 11.57 | 4.44 | 0.28 |
| Condyle | 19.08 | 14.56 | 5.83 | 0.24 |
| Coronoid | 28.00 | 17.50 | 7.15 | 0.23 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huo, J.; Hirsch, J.-M.; Gamstedt, E.K. Analytical Study of Stress Distributions around Screws in Flat Mandibular Bone under In-Plane Loading. Bioengineering 2023, 10, 786. https://doi.org/10.3390/bioengineering10070786
Huo J, Hirsch J-M, Gamstedt EK. Analytical Study of Stress Distributions around Screws in Flat Mandibular Bone under In-Plane Loading. Bioengineering. 2023; 10(7):786. https://doi.org/10.3390/bioengineering10070786
Chicago/Turabian StyleHuo, Jinxing, Jan-Michaél Hirsch, and E. Kristofer Gamstedt. 2023. "Analytical Study of Stress Distributions around Screws in Flat Mandibular Bone under In-Plane Loading" Bioengineering 10, no. 7: 786. https://doi.org/10.3390/bioengineering10070786
APA StyleHuo, J., Hirsch, J.-M., & Gamstedt, E. K. (2023). Analytical Study of Stress Distributions around Screws in Flat Mandibular Bone under In-Plane Loading. Bioengineering, 10(7), 786. https://doi.org/10.3390/bioengineering10070786







