Biomechanical Evaluation of Temporomandibular Joint Reconstruction Using Individual TMJ Prosthesis Combined with a Fibular Free Flap in a Pediatric Patient
Abstract
1. Introduction
2. Materials and Methods
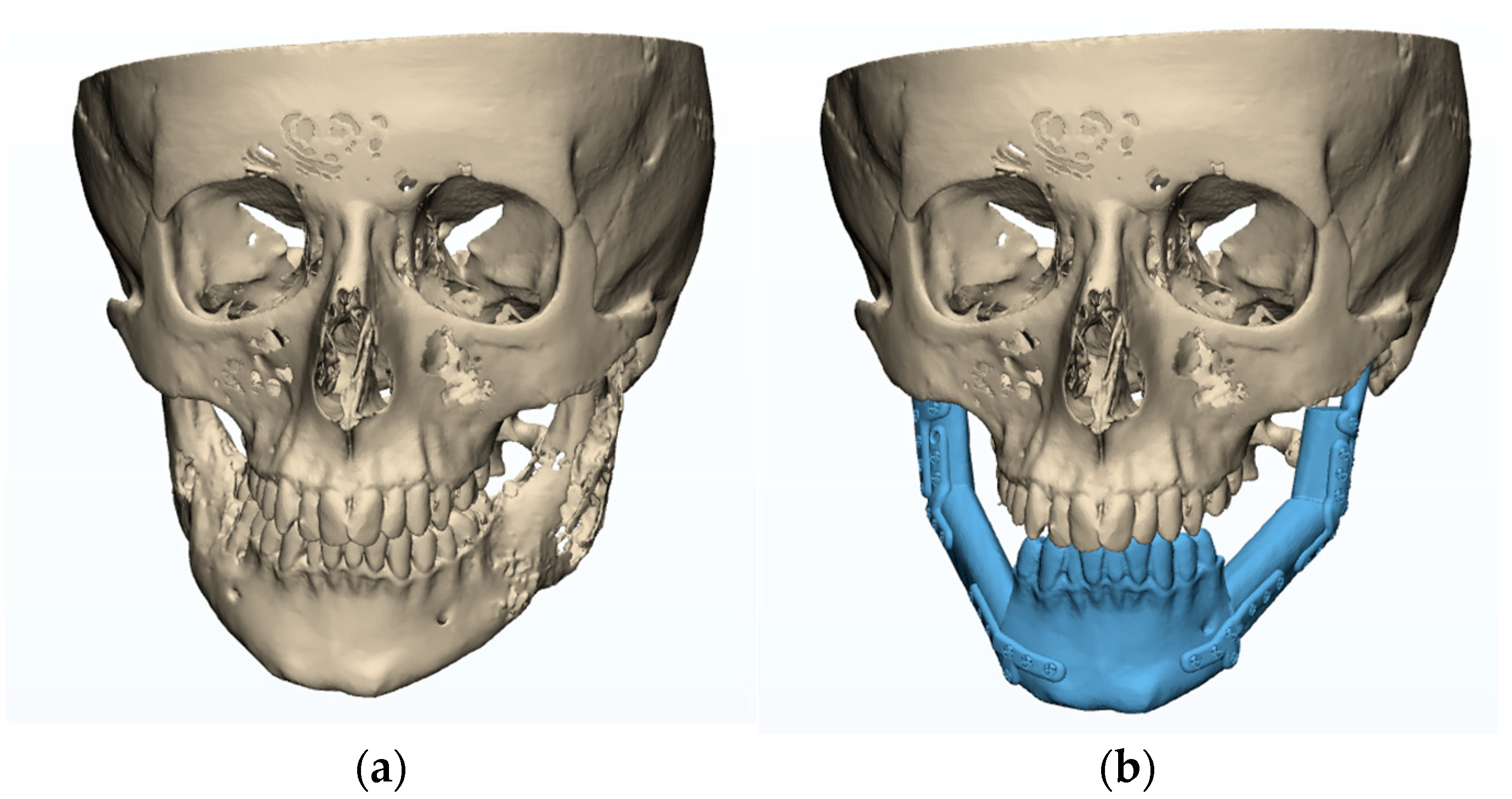
2.1. The Planning/Modelling of TMJ Reconstruction
- The development of a fibula model in 3D based on CT data (in DICOM format). The 3D segmentation process was performed using Mimics v16 (Materialise Co, Leuven, Belgium).
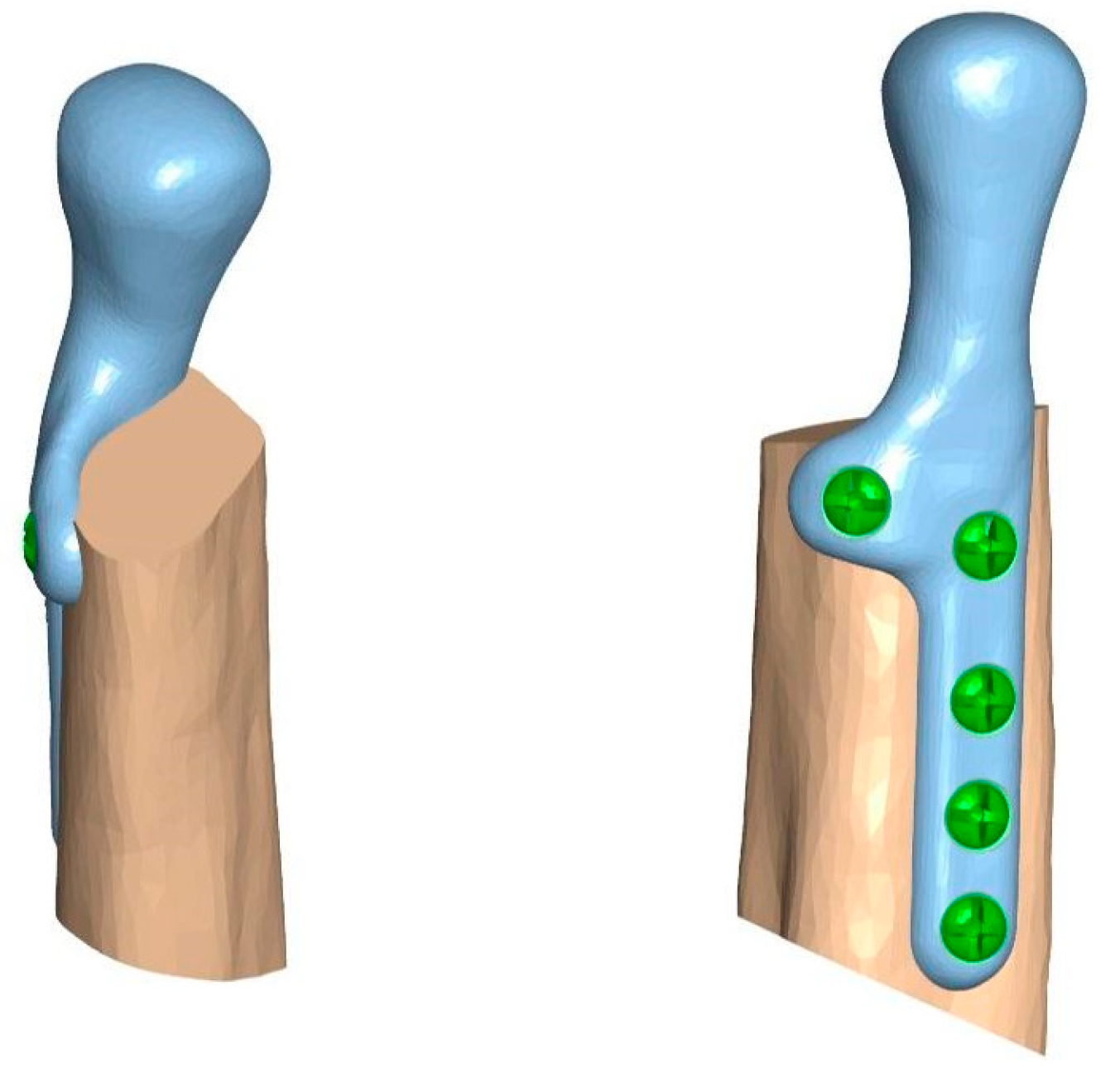
- Adjusting prostheses used in the clinical case (composed of the condylar head and mandibular ramus elements) to match the surface of fibular fragments dedicated o biomechanical testing, using Mimics (Figure 2).
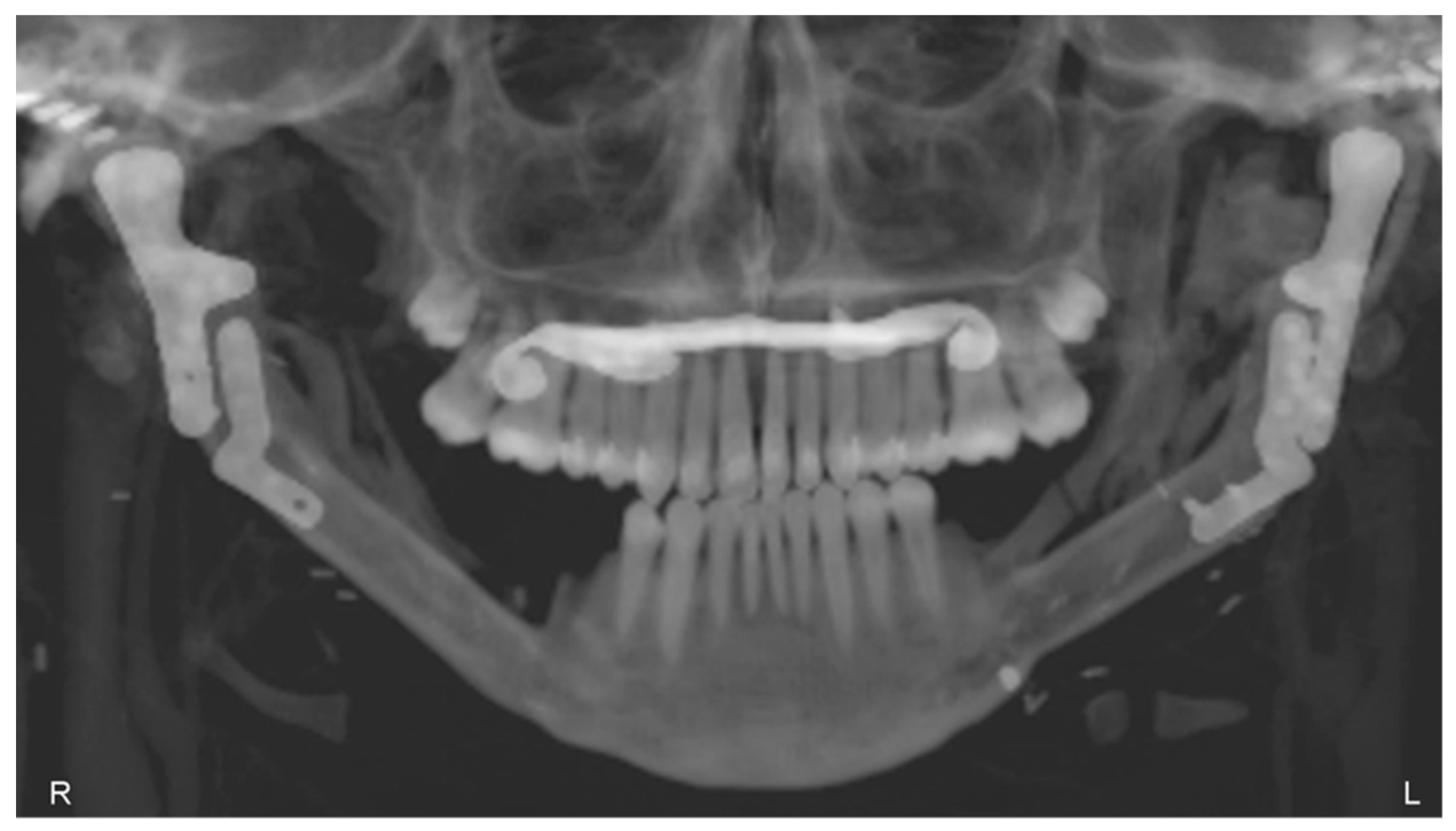
2.2. The Preoperative Evaluation Phase
2.3. Biomechanical Testing—Experimental Study
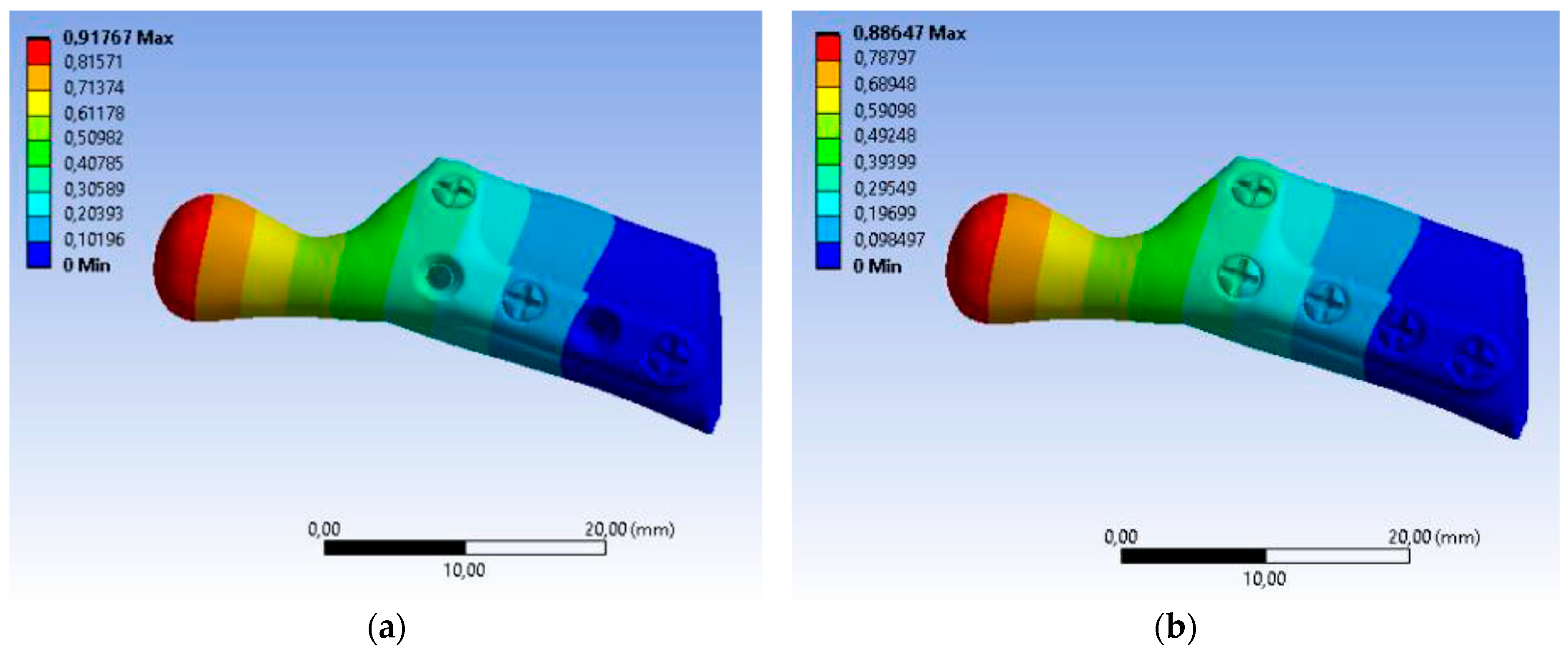
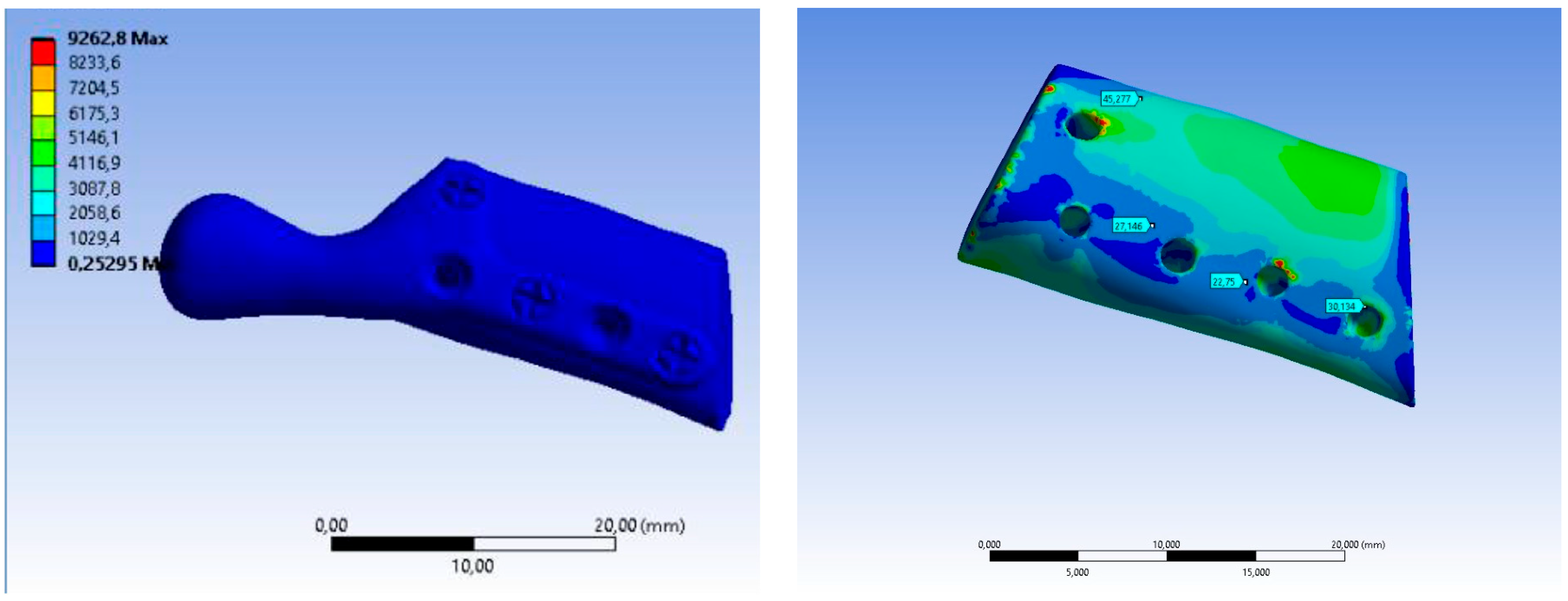
2.4. Finite Element Analysis—Numerical Simulation
3. Results
3.1. Results of the Experimental Tests
3.2. Results of the Numerical Analysis
3.3. Postoperative Evaluation of Patient
4. Discussion
5. Limitations
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Dowgierd, K.; Pokrowiecki, R.; Borowiec, M.; Kozakiewicz, M.; Smyczek, D.; Krakowczyk, Ł. A Protocol for the Use of a Combined Microvascular Free Flap with Custom-Made 3D-Printed Total Temporomandibular Joint (TMJ) Prosthesis for Mandible Reconstruction in Children. Appl. Sci. 2021, 11, 2176. [Google Scholar] [CrossRef]
- Sinn, D.P.; Tandon, R.; Tiwana, P.S. Can Alloplastic Total Temporomandibular Joint Reconstruction be Used in the Growing Patient? A Preliminary Report. J. Oral Maxillofac. Surg. 2021, 79, 2267.e1–2267.e16. [Google Scholar] [CrossRef]
- Shawky, M.; Elbehairy, M.S.; Atef, M.; Amr, K. A single-stage computer-guided gap arthroplasty with simultaneous patient-specific total joint replacement with a novel flat fossa design: A case report. Int. J. Surg. Case Rep. 2021, 79, 440–449. [Google Scholar] [CrossRef]
- Roychoudhury, A.; Yadav, P.; Alagarsamy, R.; Bhutia, O.; Goswami, D. Outcome of Stock Total Joint Replacement with Fat Grafting in Adult Temporomandibular Joint Ankylosis Patients. J. Oral Maxillofac. Surg. 2020, 79, 75–87. [Google Scholar] [CrossRef] [PubMed]
- Zimmerer, R.M.; Sander, A.K.; Schönfeld, A.; Lethaus, B.; Gellrich, N.-C.; Neuhaus, M.-T. Congenital Mandibular Hypoplasia: Patient-Specific Total Joint Replacement as a Line Extension in the Treatment of Complex Craniofacial Anomalies. J. Maxillofac. Oral Surg. 2022. [Google Scholar] [CrossRef]
- Wolford, L.M. Surgical correction of the severe jaw deformity in teenagers with juvenile idiopathic arthritis. Plast. Aesthetic Res. 2021, 8, 31. [Google Scholar] [CrossRef]
- Lypka, M.; Shah, K.; Jones, J. Prosthetic temporomandibular joint reconstruction in a cohort of adolescent females with juve-nile idiopathic arthritis. Pediatr. Rheumatol. 2020, 18, 68. [Google Scholar] [CrossRef] [PubMed]
- Brown, Z.; Rushing, D.C.; Perez, D.E. Alloplastic Temporomandibular Joint Reconstruction for Patients with Juvenile Idiopathic Arthritis. J. Oral Maxillofac. Surg. 2020, 78, 1492–1498. [Google Scholar] [CrossRef] [PubMed]
- Henry, A.; Mehra, P. Reconstruction of the TMJ and condyle in inflammatory arthritis. J. Oral Biol. Craniofacial Res. 2022, 12, 623–632. [Google Scholar] [CrossRef] [PubMed]
- Resnick, C.M. Temporomandibular Joint Reconstruction in the Growing Child. Oral Maxillofac. Surg. Clin. N. Am. 2018, 30, 109–121. [Google Scholar] [CrossRef] [PubMed]
- Andrade, N.N.; Kapoor, P.; Mathai, P.; Gupta, V.; Lakshmi, V.K.; Sharma, S. Management of paediatric ankylosis. J. Oral Biol. Craniofacial Res. 2023, 13, 191–201. [Google Scholar] [CrossRef]
- Sidebottom, A.J. Alloplastic or autogenous reconstruction of the TMJ. J. Oral Biol. Craniofacial Res. 2013, 3, 135–139. [Google Scholar] [CrossRef]
- Resnick, C.M.; Genuth, J.; Calabrese, C.E.; Taghinia, A.; Labow, B.I.; Padwa, B.L. Temporomandibular Joint Ankylosis After Ramus Construction with Free Fibula Flaps in Children with Hemifacial Microsomia. J. Oral Maxillofac. Surg. 2018, 76, 2001.e1–2001.e15. [Google Scholar] [CrossRef] [PubMed]
- Kim, J.-H.; Park, B.-H.; Yoo, M.-S.; Lee, B.-K. Stability of the Natural Joint Side in Unilateral Alloplastic Total Temporomandibular Joint Replacement Using a Ready-Made System. Appl. Sci. 2021, 11, 3935. [Google Scholar] [CrossRef]
- Linsen, S.S.; Schön, A.; Mercuri, L.G.; Teschke, M. Unilateral, Alloplastic Temporomandibular Joint Reconstruction, Biomechani-cally What Happens to the Contralateral Temporomandibular Joint?—A Prospective Cohort Study. J. Oral Maxillofac. Surg. 2021, 79, 2016–2029. [Google Scholar] [CrossRef] [PubMed]
- Hirsch, D.L.; Garfein, E.S.; Christensen, A.M.; Weimer, K.A.; Saddeh, P.B.; Levine, J.P. Use of computer-aided design and computer-aided manufacturing to produce orthognathically ideal surgical outcomes: A paradigm shift in head and neck reconstruction. J. Oral Maxillofac. Surg. 2009, 67, 2115–2122. [Google Scholar] [CrossRef] [PubMed]
- Ciocca, L.; Mazzoni, S.; Fantini, M.; Persiani, F.; Baldissara, P.; Marchetti, C.; Scotti, R. A CAD/CAM-prototyped anatomical condy-lar prosthesis connected to a custom-made bone plate to support a fibula free flap. Med. Biol. Eng. Comput. 2012, 50, 743–749. [Google Scholar] [CrossRef] [PubMed]
- Rodby, K.A.; Turin, S.; Jacobs, R.J.; Cruz, J.F.; Hassid, V.J.; Kolokythas, A.; Antony, A.K. Advances in oncologic head and neck reconstruction: Systematic review and future considerations of virtual surgical planning and computer aided design/computer aided modeling. Plast. Reconstr. Aesthetic Surg. 2014, 67, 1171–1185. [Google Scholar] [CrossRef]
- Ramos, A.; Duarte, R.J.; Mesnard, M. Strain induced in the condyle by self-tapping screws in the Biomet alloplastic temporomandibular joint: A preliminary experimental study. Int. J. Oral Maxillofac. Surg. 2015, 44, 1376–1382. [Google Scholar] [CrossRef]
- Ramos, A.; Gonzalez-Perez, L.M.; Infante-Cossio, P.; Mesnard, M. Ex-vivo and in vitro validation of an innovative mandibular condyle implant concept. J. Craniomaxillofacial Surg. 2019, 47, 112–119. [Google Scholar] [CrossRef]
- Mesnard, M.; Ramos, A. Experimental and numerical predictions of Biomet(®) alloplastic implant in a cadaveric mandibular ramus. J. Craniomaxillofacial Surg. 2016, 44, 608–615. [Google Scholar] [CrossRef]
- Park, S.M.; Lee, J.W.; Noh, G. Which plate results in better stability after segmental mandibular resection and fibula free flap reconstruction? Biomechanical analysis. Oral Surg. Oral Med. Oral Pathol. Oral Radiol. 2018, 126, 380–389. [Google Scholar] [CrossRef] [PubMed]
- Pinheiro, M.; Krairi, A.; Willaert, R.; Costa, M.C.; Van Paepegem, W. Structural optimization of patient-specific temporomandibular joint replacement implants for additive manufacturing: Novel metrics for safety evaluation and biomechanical performance. Bio-Design Manuf. 2022, 5, 333–347. [Google Scholar] [CrossRef]
- Pinto-Borges, H.; Pinto, J.; Carvalho, O.; Henriques, B.; Silva, F.; Gomes, J.; Ramos, A.; Souza, J.C. Stresses, friction, and wear on different materials and design for emporomandibular joint total joint replacement (TMJ TJR). Tribol. Int. 2022, 178, 108051. [Google Scholar] [CrossRef]
- Tiwari, A.; Gupta, V.K.; Haldkar, R.K.; Parinov, I.A. Biomechanical Analysis of Patient-Specific Temporomandibular Joint Implant and Comparison with Natural Intact Jaw Bone Using Finite Element Method. Appl. Sci. 2022, 12, 3003. [Google Scholar] [CrossRef]
- Huys, S.E.; Pastor-Alonso, D.; Theuns, P.; van Lenthe, G.H.; Vander Sloten, J.; Mommaerts, M.Y. A novel 3D-printed, patient-specific alloplastic temporomandibular joint replacement allowing enthesis reconstruction: A finite element analysis. Ann. 3d Print. Med. 2022, 6, 100058. [Google Scholar] [CrossRef]
- Pinheiro, M.; Willaert, R.; Khan, A.; Krairi, A.; Van Paepegem, W. Biomechanical evaluation of the human mandible after temporomandibular joint replacement under different biting conditions. Sci. Rep. 2021, 11, 14034. [Google Scholar] [CrossRef] [PubMed]
- Guo, F.; Huang, S.; Hu, M.; Yang, C.; Li, D.; Liu, C. Biomechanical evaluation of a customized 3D-printed polyetheretherketone condylar prosthesis. Exp. Ther Med. 2021, 21, 348. [Google Scholar] [CrossRef]
- Prasadh, S.; Suresh, S.; Hong, K.L.; Bhargav, A.; Rosa, V.; Wong, R.C.W. Biomechanics of alloplastic mandible reconstruction using biomaterials: The effect of implant design on stress concentration influences choice of material. J. Mech. Behav. Biomed. Mater. 2020, 103, 103548. [Google Scholar] [CrossRef]
- Xu, X.; Zhang, J.; Luo, D.; Guo, C.; Rong, Q. Biomechanical comparison between the custom-made mandibular condyle prosthesis and total temporomandibular joint prosthesis in finite element analysis. Acta Bioeng. Biomech. 2020, 22, 4. [Google Scholar] [CrossRef]
- Lipowicz, A.; Wolański, W.; Kawlewska, E.; Zwolska, P.; Kulesa-Mrowiecka, M.; Dowgierd, K.; Linek, P.; Myśliwiec, A. Evaluation of Mandibular Growth and Symmetry in Child with Congenital Zygomatic-Coronoid Ankylosis. Symmetry 2021, 13, 1634. [Google Scholar] [CrossRef]
- Joszko, K.; Gzik, M.; Wolański, W.; Gzik-Zroska, B.; Kawlewska, E. Biomechanical evaluation of human lumbar spine in spondylolisthesis. J. Appl. Biomed. 2018, 16, 51–58. [Google Scholar] [CrossRef]
- Kajzer, W.; Kajzer, A.; Gzik-Zroska, B.; Wolanski, W.; Janicka, I.; Dzielicki, J. Comparison of Numerical and Experimental Analysis of Plates Used in Treatment of Anterior Surface Deformity of Chest; Piętka, E., Kawa, J., Eds.; Information Technologies in Biomedicine; Lecture Notes in Computer Science; Springer: Berlin/Heidelberg, Germany, 2012; Volume 7339. [Google Scholar] [CrossRef]
- Kiel, M.; Marciniak, J.; Szewczenko, J.; Basiaga, M.; Wolański, W. Biomechanical analysis of plate stabilization on cervical part of spine. Arch. Mater. Sci. Eng. 2009, 38, 41–47. [Google Scholar]
- Gzik-Zroska, B.; Tejszerska, D.; Wolański, W.; Gzik, M. Biomechanical analysis of funnel chest after correction of deformation by stabilizing plate. In World Congress on Medical Physics and Biomedical Engineering, September 7–12, 2009, Munich, Germany: Vol. 25/4 Image Processing, Biosignal Processing, Modelling and Simulation, Biomechanics; Springer: Berlin/Heidelberg, Germany, 2010; pp. 117–120. [Google Scholar]
- Gzik-Zroska, B.; Wolański, W.; Gzik, M. Engineering-aided treatment of chest deformities to improve the process of breathing. Int. J. Numer. Method Biomed. Eng. 2013, 29, 926–937. [Google Scholar] [CrossRef]
- Mian, M.; Ackland, D.; Fink, S.; Wang, N.; Dimitroulis, G. Accuracy of custom temporomandibular joint replacement surgery using a virtual surgical planning protocol. Oral Maxillofac. Surg. 2021, 25, 367–371. [Google Scholar] [CrossRef]
- Sivam, A.; Ong, Y.L.R.; Garg, A.; Sillifant, P. The Use of Virtual Surgical Planning for Management of Ameloblastoma: A Case Report. Res. Rep. Oral Maxillofac. Surg. 2022, 6, 062. [Google Scholar] [CrossRef]
- Shu, D.L.; Liu, X.Z.; Guo, B.; Ran, W.; Liao, X.; Zhang, Y.Y. Accuracy of using computer-aided rapid prototyping templates for man-dible reconstruction with an iliac crest graft. World J. Surg. Oncol. 2014, 12, 190. [Google Scholar] [CrossRef]
- Gomez, N.L.; Boccalatte, L.A.; Ruiz, L.; Nassif, M.G.; Figari, M.F.; Ritacco, L. Total Temporomandibular Joint Replacement and Simultaneous Orthognath-ic Surgery Using Computer-Assisted Surgery. J. Maxillofac. Oral Surg. 2021, 20, 394–403. [Google Scholar] [CrossRef]
- Han, H.H.; Kim, H.Y.; Lee, J.Y. The Pros and Cons of Computer-Aided Surgery for Segmental Mandibular Reconstruction after Oncological Surgery. Arch. Craniofac. Surg. 2017, 18, 149–154. [Google Scholar] [CrossRef]
- Van Baar, G.J.C.; Forouzanfar, T.; Liberton, N.P.T.J.; Winters, H.A.H.; Leusink, F.K.J. Accuracy of computer-assisted surgery in man-dibular reconstruction: A systematic review. Oral Oncol. 2018, 84, 52–60. [Google Scholar] [CrossRef] [PubMed]
- Van Baar, G.J.C.; Liberton, N.P.T.J.; Winters, H.A.H.; Leeuwrik, L.; Forouzanfar, T.; Leusink, F.K.J. A Postoperative Evaluation Guide-line for Computer-Assisted Reconstruction of the Mandible. J. Vis. Exp. 2020, 28, 155. [Google Scholar] [CrossRef]
- Chole, R.H.; Patil, R.N.; Balsaraf Chole, S.; Gondivkar, S.; Gadbail, A.R.; Yuwanati, M.B. Association of mandible anatomy with age, gender, and dental status: A radiographic study. ISRN Radiol. 2013, 2013, 453763. [Google Scholar] [CrossRef]
- Lee, H.H. Finite Element Simulations with ANSYS Workbench 15; Chuan Hwa Book Co.: Taiwan, China, 2014. [Google Scholar]
- De Maesschalck, T.; Courvoisier, D.S.; Scolozzi, P. Computer-assisted versus traditional freehand technique in fibular free flap mandibular reconstruction: A morphological comparative study. Eur. Arch. Otorhinolaryngol. 2017, 274, 517–526. [Google Scholar] [CrossRef]
- Schepers, R.H.; Kraeima, J.; Vissink, A.; Lahoda, L.U.; Roodenburg, J.L.; Reintsema, H.; Raghoebar, G.M.; Witjes, M.J. Accuracy of secondary maxillofacial reconstruction with prefabricated fibula grafts using 3D planning and guided reconstruction. J. Craniomaxillofac. Surg. 2016, 44, 392–399. [Google Scholar] [CrossRef] [PubMed]
- Kieser, D.C.; Kanade, S.; Waddell, N.J.; Kieser, J.A.; Theis, J.C.; Swain, M.V. The deer femur—A morphological and biomechanical animal model of the human femur. Biomed. Mater. Eng. 2014, 24, 1693–1703. [Google Scholar] [CrossRef] [PubMed]
- Berteau, J.P.; Pithioux, M.; Baron, C.; Gineyts, E.; Follet, H.; Lasaygues, P.; Chabrand, P. Characterisation of the difference in fracture mechanics between children and adult cortical bone. Comput. Methods Biomech. Biomed. Eng. 2012, 15 (Suppl. S1), 281–282. [Google Scholar] [CrossRef] [PubMed]
- Seikaly, H.; Chau, J.; Li, F.; Driscoll, B.; Seikaly, D.; Calhoun, J.; Calhoun, K.H. Bone that best matches the properties of the mandible. J. Otolaryngol. 2003, 32, 262–265. [Google Scholar] [CrossRef]
- Royhman, D.; Radhakrishnan, R.; Yuan, J.C.; Mathew, M.T.; Mercuri, L.G.; Sukotjo, C. An electrochemical investigation of TMJ implant metal alloys in an artificial joint fluid environment: The influence of pH variation. J. Craniomaxillofac. Surg. 2014, 42, 1052–1061. [Google Scholar] [CrossRef]
- Dowgierd, K.; Pokrowiecki, R.; Borowiec, M.; Sokolowska, Z.; Dowgierd, M.; Wos, J.; Kozakiewicz, M.; Krakowczyk, Ł. Protocol and Evaluation of 3D-Planned Microsurgical and Dental Implant Reconstruction of Maxillary Cleft Critical Size Defects in Adolescents and Young Adults. J. Clin. Med. 2021, 10, 2267. [Google Scholar] [CrossRef]

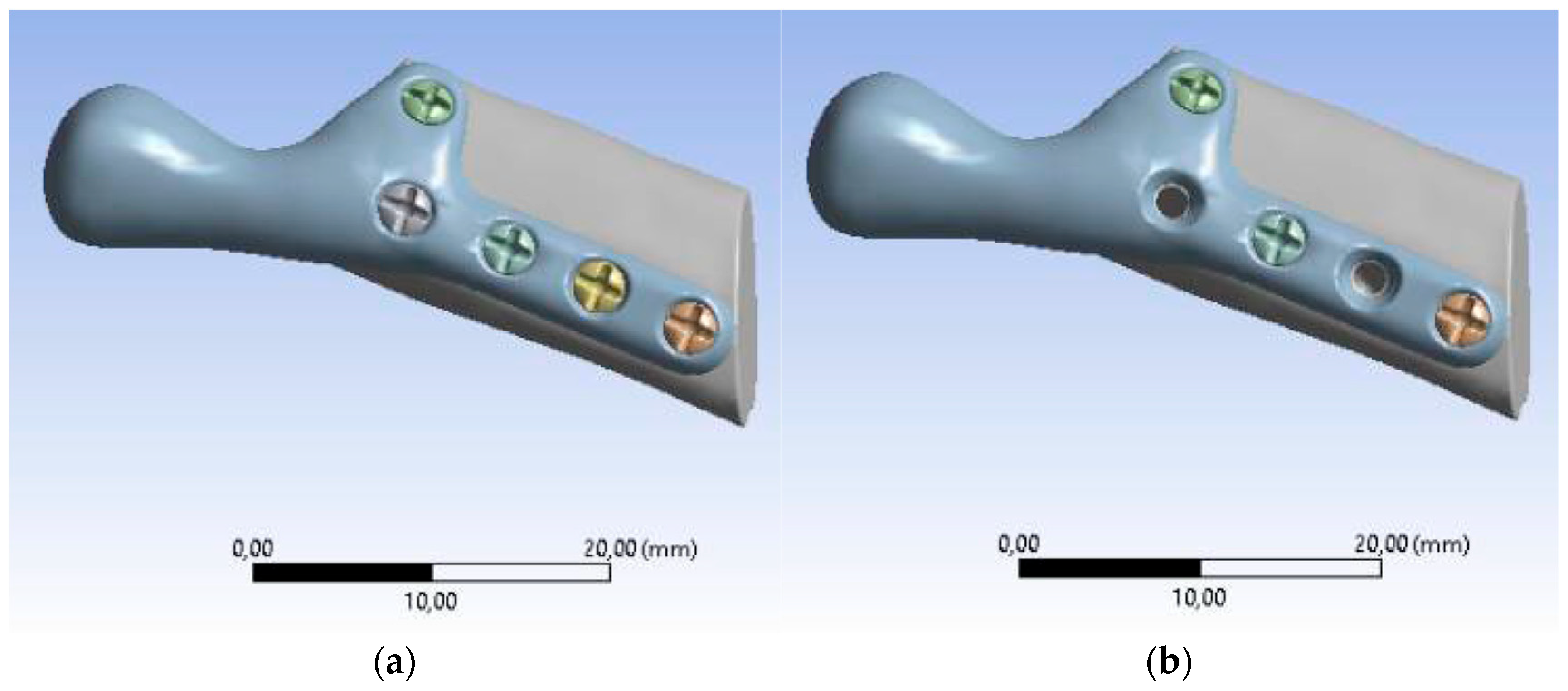
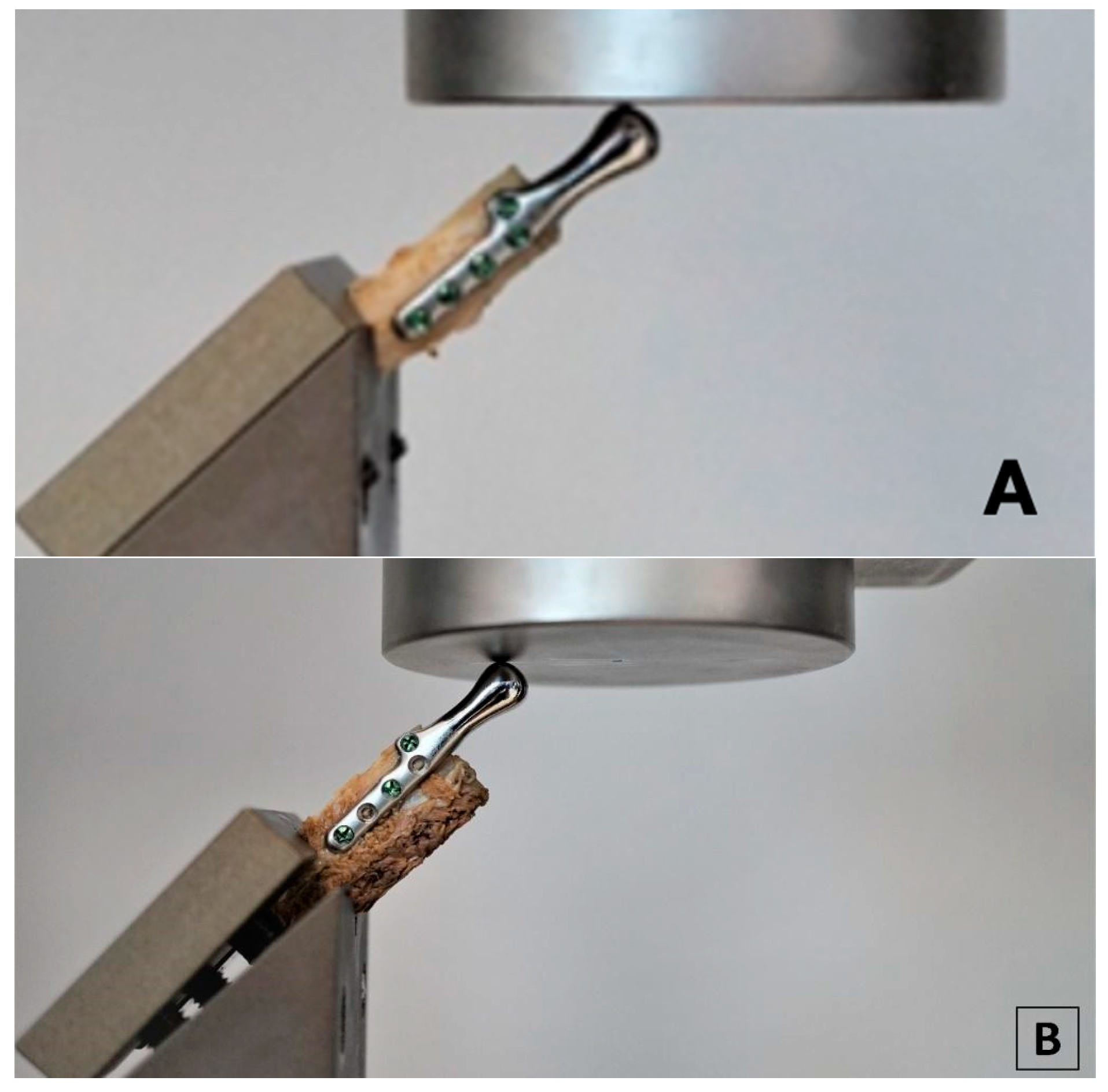
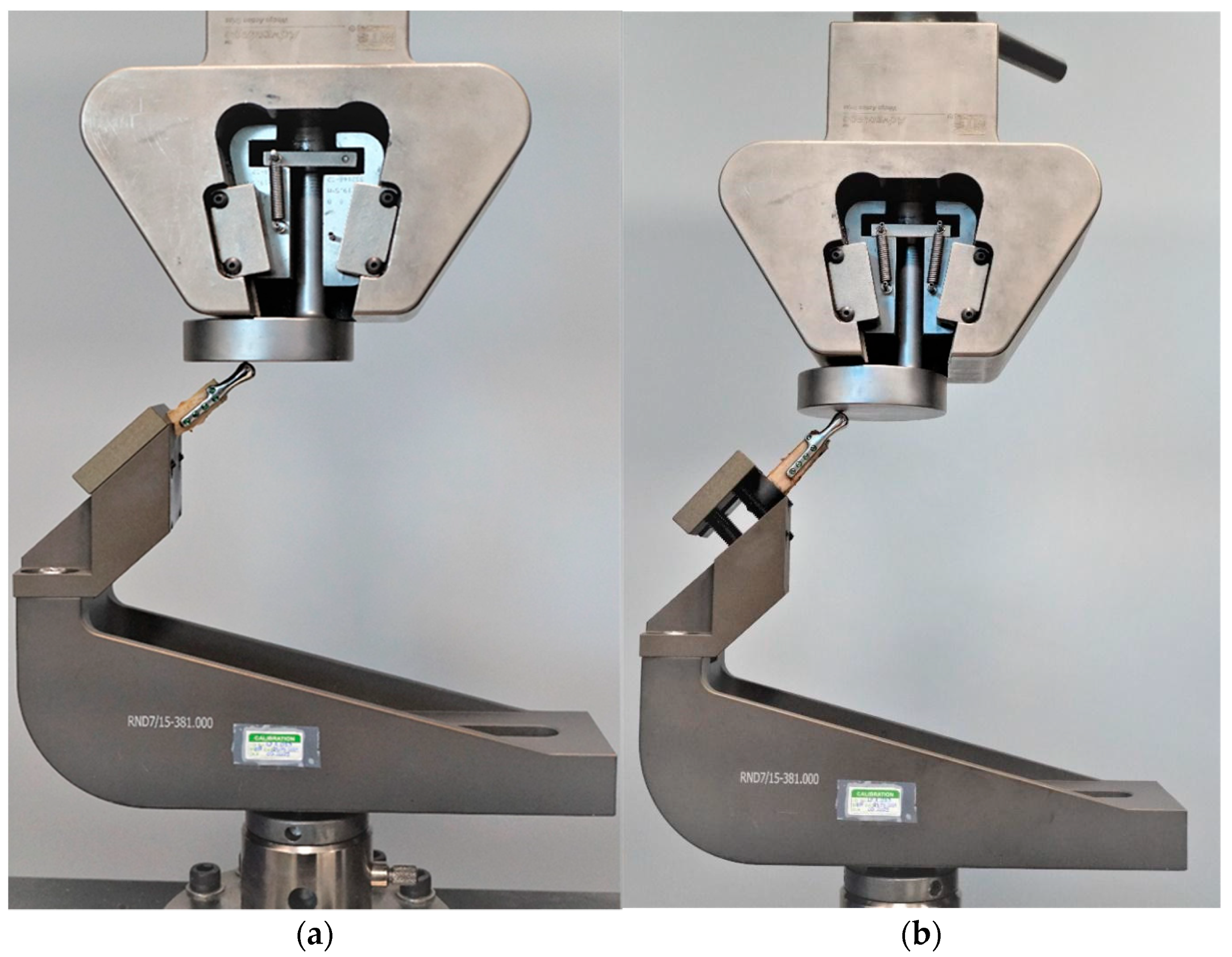





| Sample | Length [mm] | Width [mm] | Cross-Sectional Area [mm2] | Number of Screws |
|---|---|---|---|---|
| SAMPLE 1 | 48 | 9.7 | 53 | 5 |
| SAMPLE 2 | 48 | 9.4 | 41 | 5 |
| SAMPLE 3 | 46.5 | 14.5 | 100 | 5 |
| SAMPLE 4 | 50.6 | 10.3 | 66 | 3 |
| SAMPLE 5 | 74.9 | 14 | 106 | 3 |
| SAMPLE 6_B | 65.5 | 16.9 | 95 | 5 |
| SAMPLE 7_B | 58.3 | 47.8 | 183.6 | 5 |
| Component/ Type of Material | Density | Tensile Strength | Young’s Modulus | Poisson’s Ratio |
|---|---|---|---|---|
| The fibula/ Human bone | 2.1 g/cm3 | 193 MPa | 14.4 GPa | 0.4 |
| The fibula/graft Porcine bone | 1.6 g/cm3 | 68 MPa | 2.6 GPa | 0.33 |
| TMJ prosthesis/ CoCrMo (ISO 5832-12) | 8.4 g/cm3 | 780 to 1280 MPa | 210 to 250 GPa | 0.3 |
| Screws/ Ti6Al4V (ISO 5832-3) | 4.4 g/cm3 | 1000 to 1190 MPa | 110 GPa | 0.32 |
| Sample | Peak Load [N] | Load of Offset Yield [N] | Displacement at Offset Yield [mm] | Stiffness (Peak Load/Displacement) [N/mm] |
|---|---|---|---|---|
| SAMPLE 1 | 316 | 312 | 5.97 | 52.93 |
| SAMPLE 2 | 185 | 120 | 3.08 | 60.06 |
| SAMPLE 3 | 397 | 354 | 5.03 | 78.92 |
| SAMPLE 4 | 115 | 112 | 1.33 | 86.46 |
| SAMPLE 5 | 280 | 52 | 1.63 | 171.78 |
| SAMPLE 6 | 228 | 154 | 1.67 | 136.52 |
| SAMPLE 7 | 493 | 375 | 3.99 | 123.55 |
| Sample (Human) | Equivalent Stress Von-Mises Maximum (Average—Bone) [MPa] | Maximum Deformation [mm] |
|---|---|---|
| SAMPLE A | 9262 (50.05) | 0.91 |
| SAMPLE B | 9262 (49.86) | 0.88 |
| Sample | Experimental Test | Numerical Study | The Deviations [%] | ||
|---|---|---|---|---|---|
| Peak Load [N] | Displacement Yield—x [mm] | Maximum Equivalent Stress Implant (Bone)[MPa] | Maximum Deformation—x0 [mm] | ||
| SAMPLE 1 | 316 | 5.97 | 5984 (49.98) | 5.32 | 10.88 |
| SAMPLE 2 | 185 | 3.08 | 3503 (29.26) | 3.11 | 0.97 |
| SAMPLE 3 | 397 | 5.03 | 7517 (62.79) | 6.68 | 32.80 |
| SAMPLE 4 | 115 | 1.33 | 2355 (18.06) | 2.10 | 57.89 |
| SAMPLE 5 | 280 | 1.63 | 5261 (35.78) | 2.30 | 41.10 |
| SAMPLE 6 | 228 | 1.67 | 4283 (29.31) | 1.76 | 5.38 |
| SAMPLE 7 | 493 | 3.99 | 9262 (63.38) | 3.81 | 4.51 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dowgierd, K.; Kawlewska, E.; Joszko, K.; Kropiwnicki, J.; Wolanski, W. Biomechanical Evaluation of Temporomandibular Joint Reconstruction Using Individual TMJ Prosthesis Combined with a Fibular Free Flap in a Pediatric Patient. Bioengineering 2023, 10, 541. https://doi.org/10.3390/bioengineering10050541
Dowgierd K, Kawlewska E, Joszko K, Kropiwnicki J, Wolanski W. Biomechanical Evaluation of Temporomandibular Joint Reconstruction Using Individual TMJ Prosthesis Combined with a Fibular Free Flap in a Pediatric Patient. Bioengineering. 2023; 10(5):541. https://doi.org/10.3390/bioengineering10050541
Chicago/Turabian StyleDowgierd, Krzysztof, Edyta Kawlewska, Kamil Joszko, Jacek Kropiwnicki, and Wojciech Wolanski. 2023. "Biomechanical Evaluation of Temporomandibular Joint Reconstruction Using Individual TMJ Prosthesis Combined with a Fibular Free Flap in a Pediatric Patient" Bioengineering 10, no. 5: 541. https://doi.org/10.3390/bioengineering10050541
APA StyleDowgierd, K., Kawlewska, E., Joszko, K., Kropiwnicki, J., & Wolanski, W. (2023). Biomechanical Evaluation of Temporomandibular Joint Reconstruction Using Individual TMJ Prosthesis Combined with a Fibular Free Flap in a Pediatric Patient. Bioengineering, 10(5), 541. https://doi.org/10.3390/bioengineering10050541