Fabrication of Hydrogel-Based Composite Fibers and Computer Simulation of the Filler Dynamics in the Composite Flow
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Synthesis of GelMA and ADA
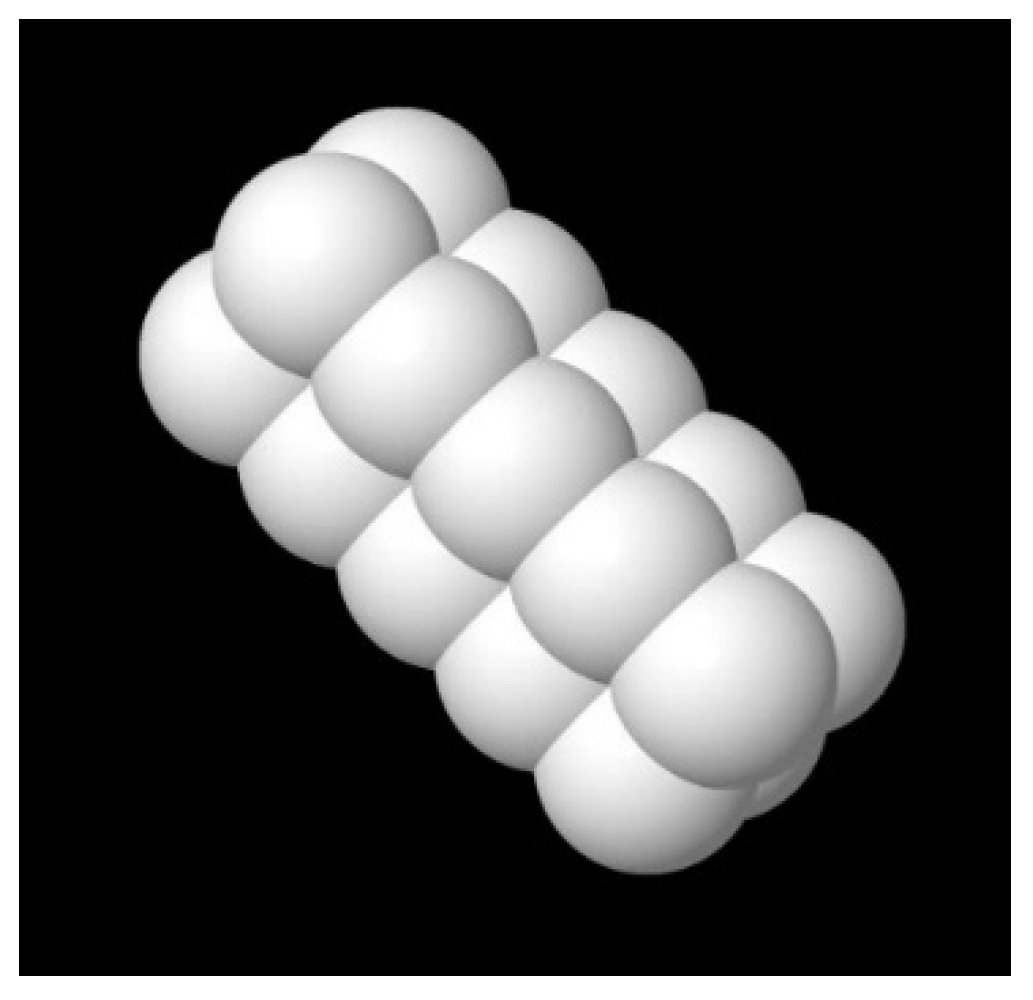
2.3. Fabrication of Rod-Shaped Fillers
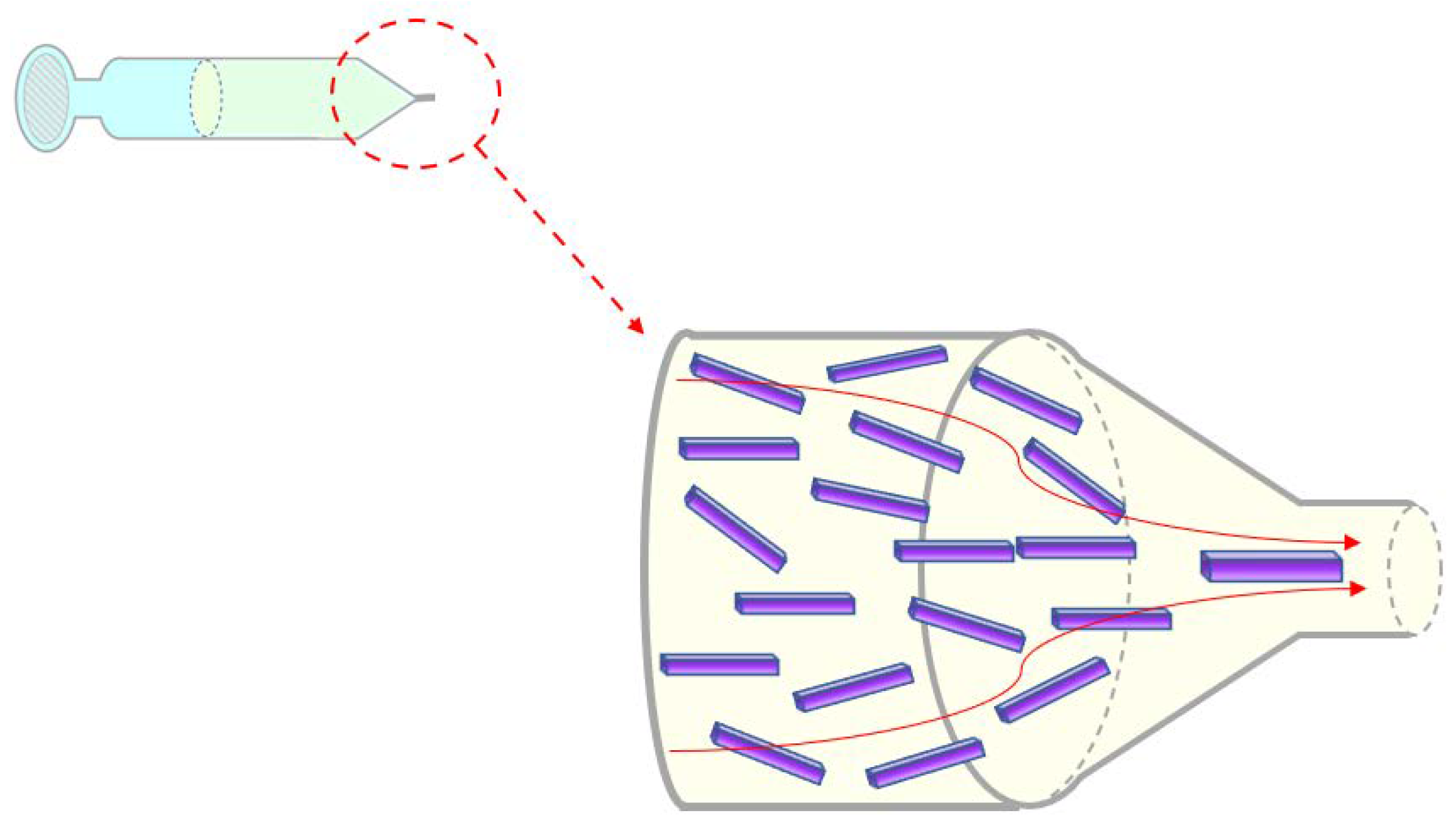
2.4. Wet Spinning of ADA-GelMA Filaments with Rod-Shaped Fillers
2.5. Viscosity Measurement of the Spinning Solution
2.6. Extrusion of GelMA Hydrogel with Rod-Shaped Fillers Using a 3D Printer
2.7. Statistical Analysis
3. Computational Simulation
3.1. Simulation of the Composite Flow
3.2. Simulation of the Filler Dynamics in the Composite Flow
4. Results and Discussion
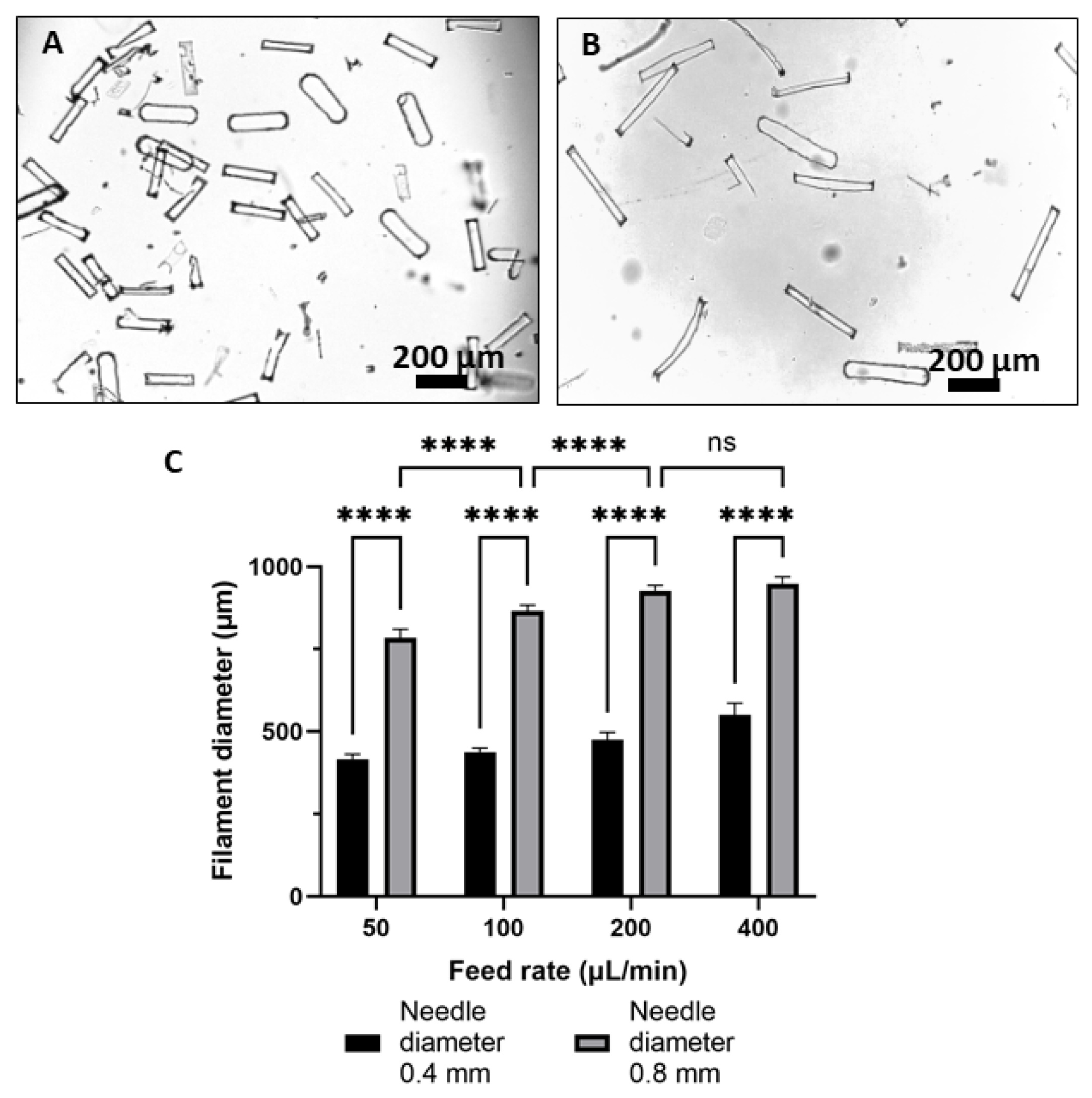
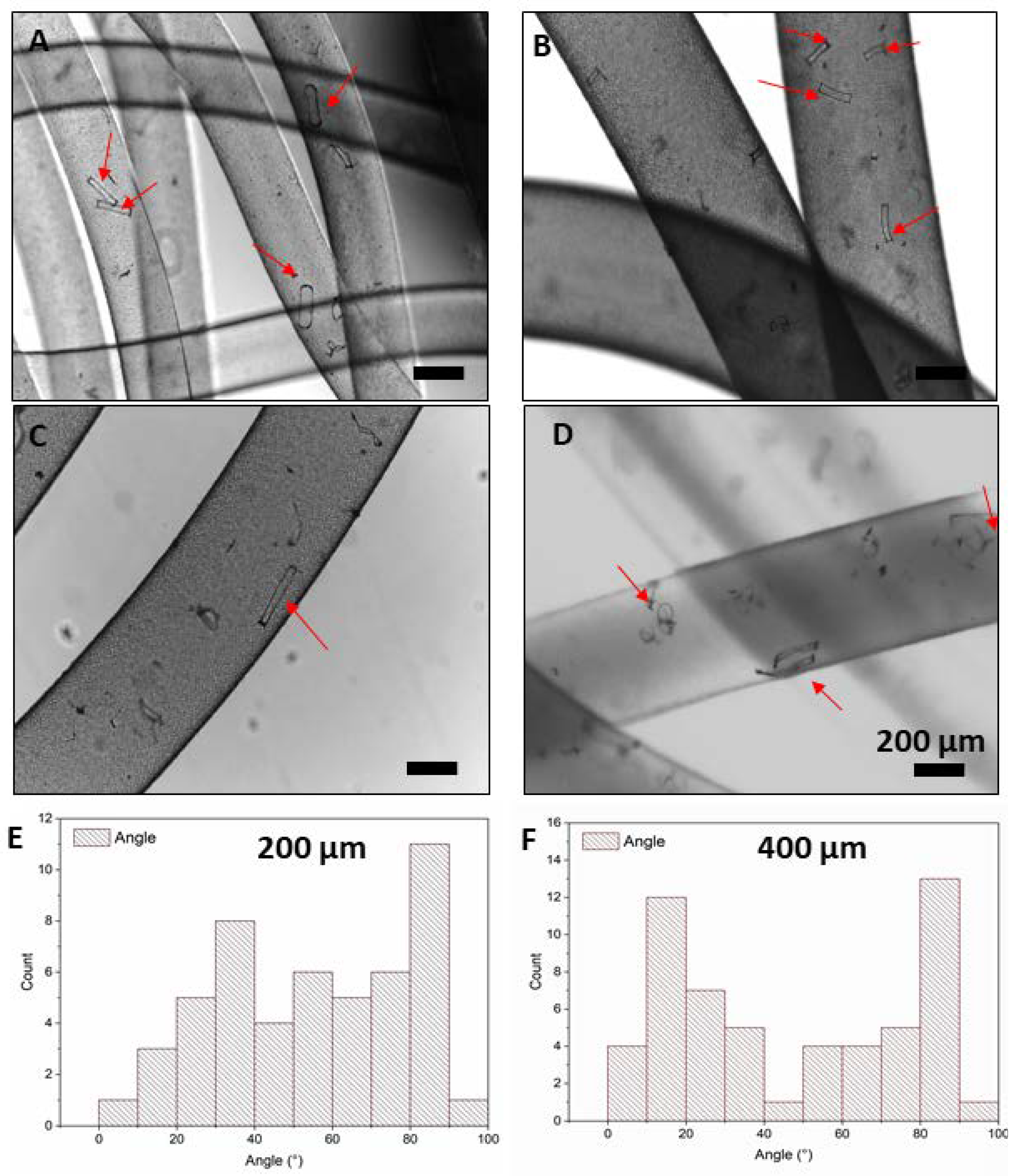
4.1. Microrod Productions
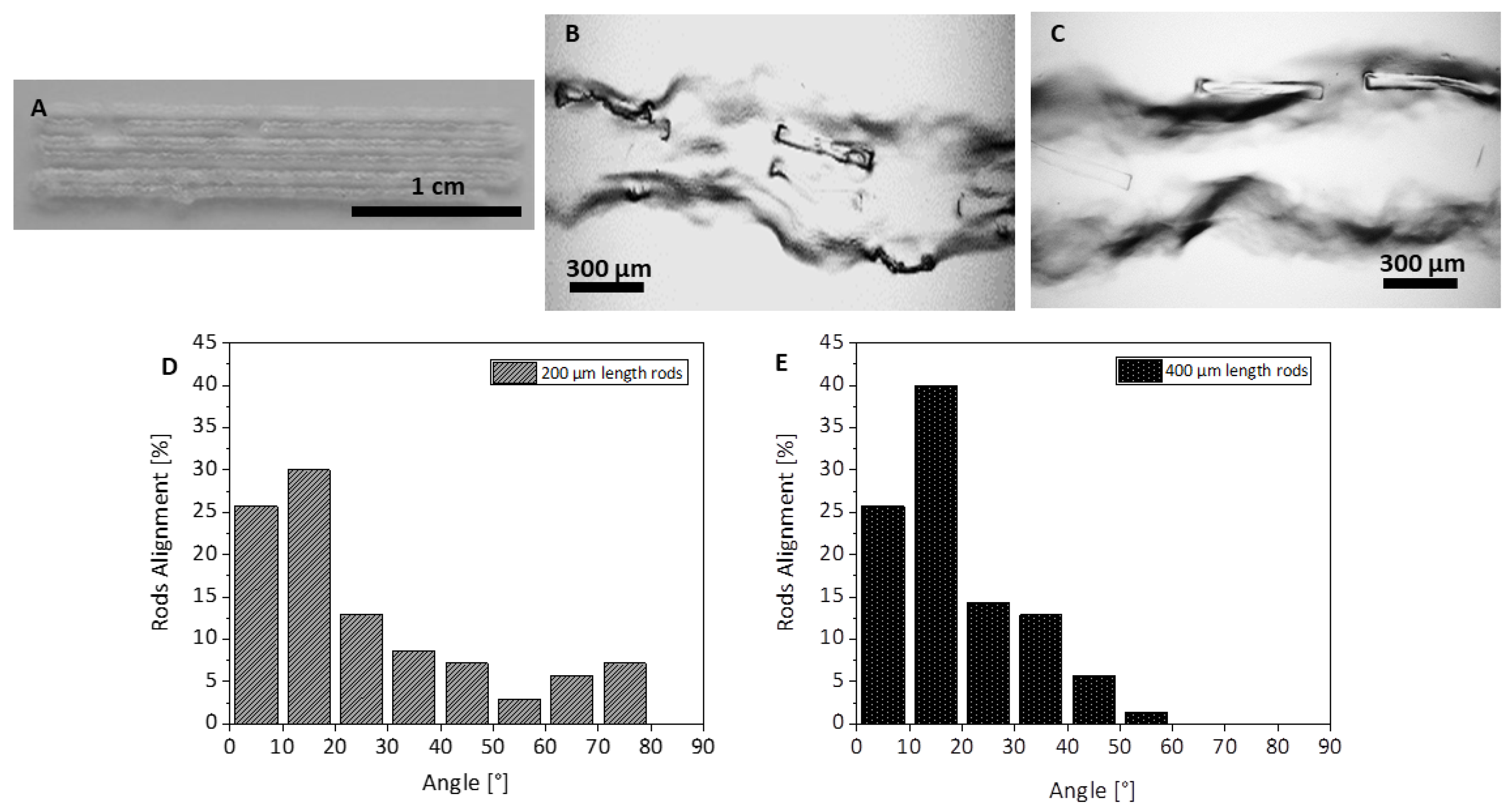
4.2. Wet Spinning of the Filaments
4.3. Extruded Filaments Using a 3D Printer
4.4. Computational Simulation
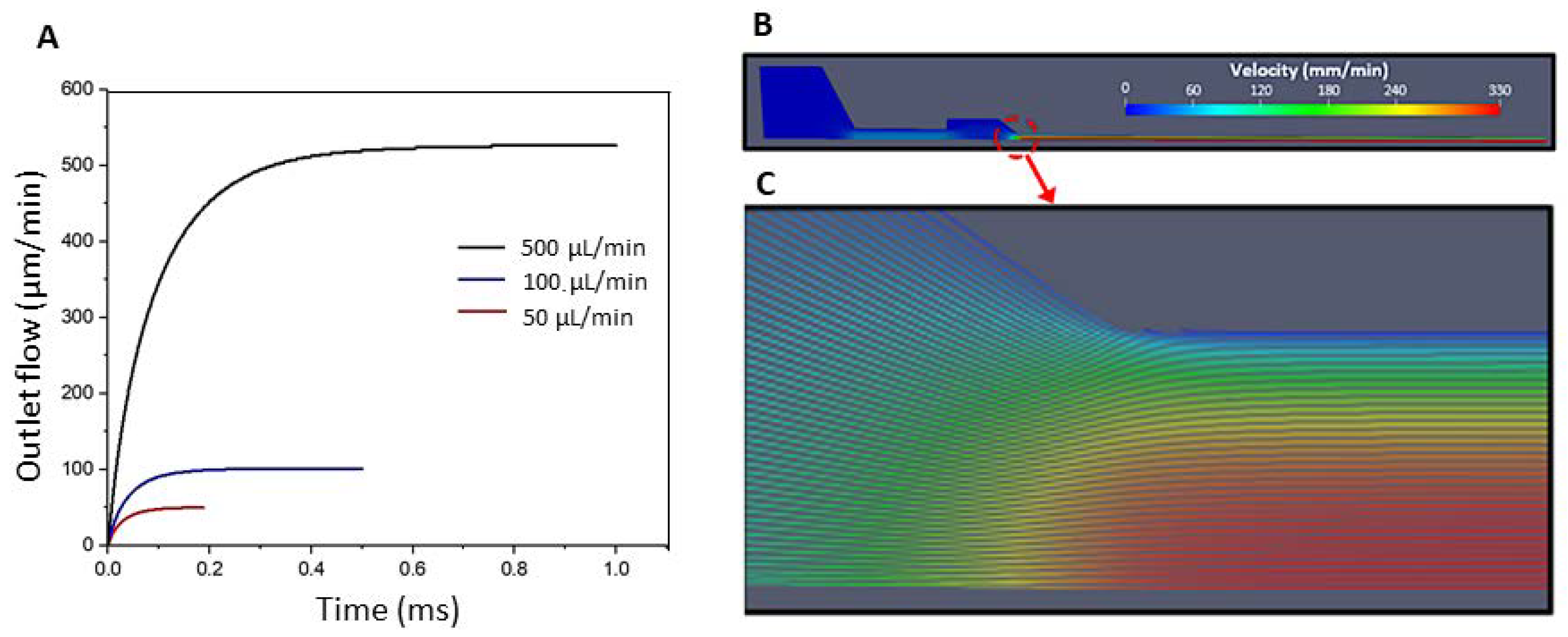
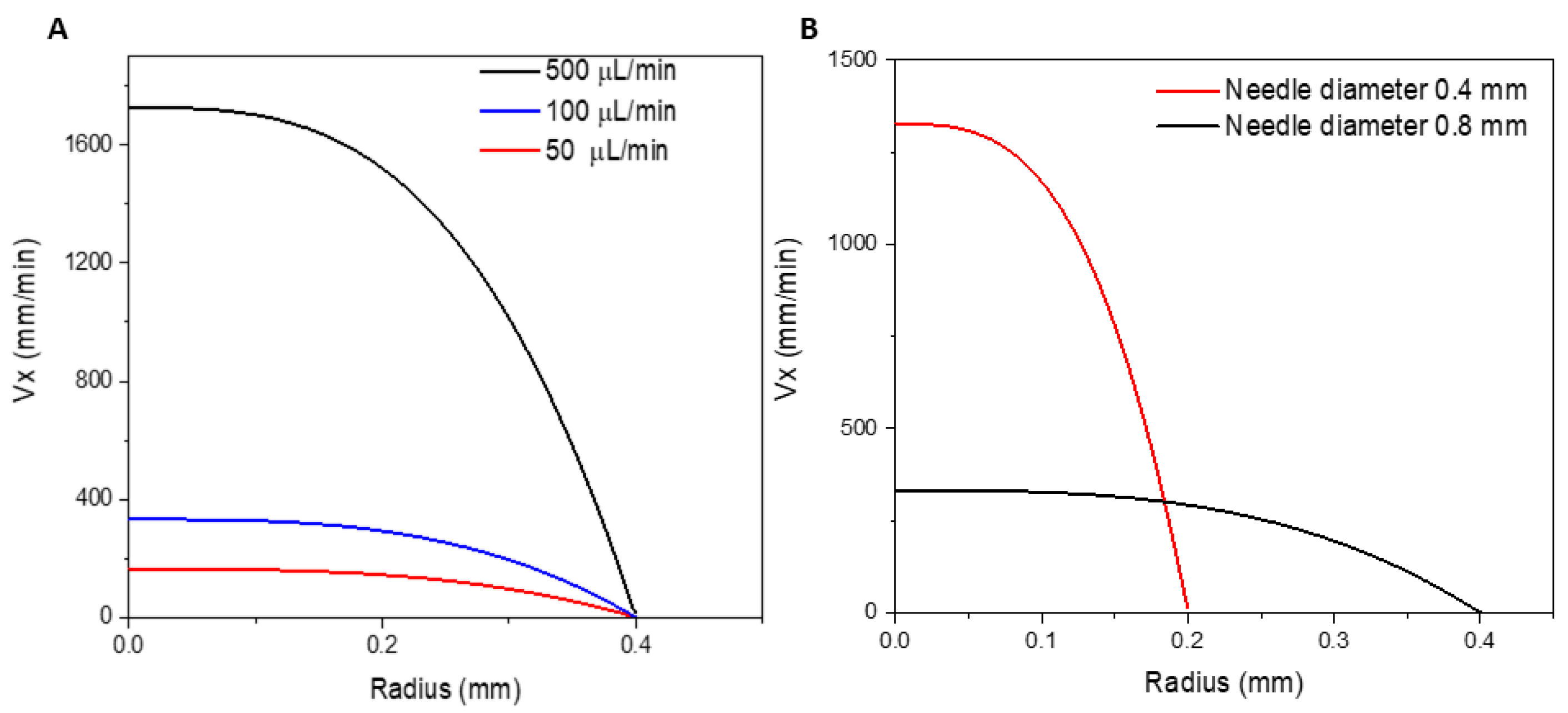
4.5. Flow Simulation
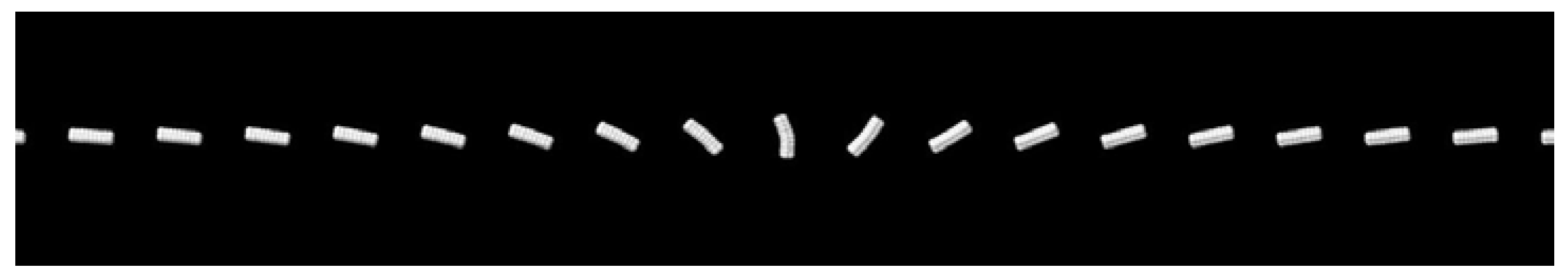
4.6. Filler Dynamics
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ahadian, S.; Sadeghian, R.B.; Salehi, S.; Ostrovidov, S.; Bae, H.; Ramalingam, M.; Khademhosseini, A. Bioconjugated Hydrogels for Tissue Engineering and Regenerative Medicine. Bioconjugate Chem. 2015, 26, 1984–2001. [Google Scholar] [CrossRef] [PubMed]
- Xiao, W.; Li, J.; Qu, X.; Wang, L.; Tan, Y.; Li, K.; Li, H.; Yue, X.; Li, B.; Liao, X. Cell-laden interpenetrating network hydrogels formed from methacrylated gelatin and silk fibroin via a combination of sonication and photocrosslinking approaches. Mater. Sci. Eng. C 2019, 99, 57–67. [Google Scholar] [CrossRef] [PubMed]
- Jeon, O.; Shin, J.-Y.; Marks, R.; Hopkins, M.; Kim, T.-H.; Park, H.-H.; Alsberg, E. Highly Elastic and Tough Interpenetrating Polymer Network-Structured Hybrid Hydrogels for Cyclic Mechanical Loading-Enhanced Tissue Engineering. Chem. Mater. 2017, 29, 8425–8432. [Google Scholar] [CrossRef]
- Han, W.T.; Jang, T.; Chen, S.; Chong, L.S.H.; Jung, H.-D.; Song, J. Improved cell viability for large-scale biofabrication with photo-crosslinkable hydrogel systems through a dual-photoinitiator approach. Biomater. Sci. 2020, 8, 450–461. [Google Scholar] [CrossRef] [PubMed]
- Choi, J.R.; Yong, K.W.; Choi, J.Y.; Cowie, A.C. Recent advances in photo-crosslinkable hydrogels for biomedical applications. BioTechniques 2019, 66, 40–53. [Google Scholar] [CrossRef] [PubMed]
- Chen, M.H.; Wang, L.L.; Chung, J.J.; Kim, Y.-H.; Atluri, P.; Burdick, J.A. Methods To Assess Shear-Thinning Hydrogels for Application As Injectable Biomaterials. ACS Biomater. Sci. Eng. 2017, 3, 3146–3160. [Google Scholar] [CrossRef] [PubMed]
- Lee, J.; Cuddihy, M.J.; Kotov, N.A. Three-Dimensional Cell Culture Matrices: State of the Art. Tissue Eng. Part B Rev. 2008, 14, 61–86. [Google Scholar] [CrossRef] [PubMed]
- Farasatkia, A.; Kharaziha, M.; Ashrafizadeh, F.; Salehi, S. Transparent silk/gelatin methacrylate (GelMA) fibrillar film for corneal regeneration. Mater. Sci. Eng. C 2021, 120, 111744. [Google Scholar] [CrossRef] [PubMed]
- An, Y.; Gao, L.; Wang, T. Graphene Oxide/Alginate Hydrogel Fibers with Hierarchically Arranged Helical Structures for Soft Actuator Application. ACS Appl. Nano Mater. 2020, 3, 5079–5087. [Google Scholar] [CrossRef]
- Wang, Q.-Q.; Liu, Y.; Zhang, C.-J.; Zhang, C.; Zhu, P. Alginate/gelatin blended hydrogel fibers cross-linked by Ca2+ and oxidized starch: Preparation and properties. Mater. Sci. Eng. C 2019, 99, 1469–1476. [Google Scholar] [CrossRef] [PubMed]
- Krishnamoorthy, S.; Zhang, Z.; Xu, C. Biofabrication of three-dimensional cellular structures based on gelatin methacrylate–alginate interpenetrating network hydrogel. J. Biomater. Appl. 2019, 33, 1105–1117. [Google Scholar] [CrossRef] [PubMed]
- Reakasame, S.; Boccaccini, A.R. Oxidized Alginate-Based Hydrogels for Tissue Engineering Applications: A Review. Biomacromolecules 2018, 19, 3–21. [Google Scholar] [CrossRef] [PubMed]
- Jeon, O.; Wolfson, D.W.; Alsberg, E. In-Situ Formation of Growth-Factor-Loaded Coacervate Microparticle-Embedded Hydrogels for Directing Encapsulated Stem Cell Fate. Adv. Mater. 2015, 27, 2216–2223. [Google Scholar] [CrossRef] [PubMed]
- Distler, T.; Solisito, A.A.; Schneidereit, D.; Friedrich, O.; Detsch, R.; Boccaccini, A.R. 3D printed oxidized alginate-gelatin bioink provides guidance for C2C12 muscle precursor cell orientation and differentiation via shear stress during bioprinting. Biofabrication 2020, 12, 045005. [Google Scholar] [CrossRef]
- Mohamed, M.A.; Fallahi, A.; El-Sokkary, A.M.A.; Salehi, S.; Akl, M.A.; Jafari, A.; Tamayol, A.; Fenniri, H.; Khademhosseini, A.; Andreadis, S.T.; et al. Stimuli-responsive hydrogels for manipulation of cell microenvironment: From chemistry to biofabrication technology. Prog. Polym. Sci. 2019, 98, 101147. [Google Scholar] [CrossRef]
- Rezwan, K.; Chen, Q.Z.; Blaker, J.J.; Boccaccini, A.R. Biodegradable and bioactive porous polymer/inorganic composite scaffolds for bone tissue engineering. Biomaterials 2006, 27, 3413–3431. [Google Scholar] [CrossRef]
- Ostrovidov, S.; Shi, X.; Sadeghian, R.B.; Salehi, S.; Fujie, T.; Bae, H.; Ramalingam, M.; Khademhosseini, A. Stem Cell Differentiation Toward the Myogenic Lineage for Muscle Tissue Regeneration: A Focus on Muscular Dystrophy. Stem Cell Rev. Rep. 2015, 11, 866–884. [Google Scholar] [CrossRef]
- Ostrovidov, S.; Ebrahimi, M.; Bae, H.; Nguyen, H.K.; Salehi, S.; Kim, S.B.; Kumatani, A.; Matsue, T.; Shi, X.; Nakajima, K.; et al. Gelatin–Polyaniline Composite Nanofibers Enhanced Excitation–Contraction Coupling System Maturation in Myotubes. ACS Appl. Mater. Interfaces 2017, 9, 42444–42458. [Google Scholar] [CrossRef]
- Datta, P.; Vyas, V.; Dhara, S.; Chowdhury, A.R.; Barui, A. Anisotropy Properties of Tissues: A Basis for Fabrication of Biomimetic Anisotropic Scaffolds for Tissue Engineering. J. Bionic Eng. 2019, 16, 842–868. [Google Scholar] [CrossRef]
- Sano, K.; Ishida, Y.; Aida, T. Synthesis of Anisotropic Hydrogels and Their Applications. Angew. Chem. Int. Ed. 2018, 57, 2532–2543. [Google Scholar] [CrossRef]
- Apsite, I.; Salehi, S.; Ionov, L. Materials for Smart Soft Actuator Systems. Chem. Rev. 2022, 122, 1349–1415. [Google Scholar] [CrossRef] [PubMed]
- Koeck, K.S.; Salehi, S.; Humenik, M.; Scheibel, T. Processing of Continuous Non-Crosslinked Collagen Fibers for Microtissue Formation at the Muscle-Tendon Interface. Adv. Funct. Mater. 2022, 32, 2112238. [Google Scholar] [CrossRef]
- Jenkins, T.L.; Little, D. Synthetic scaffolds for musculoskeletal tissue engineering: Cellular responses to fiber parameters. Npj Regen. Med. 2019, 4, 15. [Google Scholar] [CrossRef] [PubMed]
- Apsite, I.; Uribe, J.M.; Posada, A.F.; Rosenfeldt, S.; Salehi, S.; Ionov, L. 4D biofabrication of skeletal muscle microtissues. Biofabrication 2020, 12, 015016. [Google Scholar] [CrossRef]
- Tamayol, A.; Najafabadi, A.H.; Aliakbarian, B.; Arab-Tehrany, E.; Akbari, M.; Annabi, N.; Juncker, D.; Khademhosseini, A. Hydrogel Templates for Rapid Manufacturing of Bioactive Fibers and 3D Constructs. Adv. Healthc. Mater. 2015, 4, 2146–2153. [Google Scholar] [CrossRef]
- Tuzlakoglu, K.; Alves, C.M.; Mano, J.F.; Reis, R.L. Production and Characterization of Chitosan Fibers and 3-D Fiber Mesh Scaffolds for Tissue Engineering Applications. Macromol. Biosci. 2004, 4, 811–819. [Google Scholar] [CrossRef]
- Ng, P.F.; Lee, K.I.; Meng, S.; Zhang, J.; Wang, Y.; Fei, B. Wet Spinning of Silk Fibroin-Based Core–Sheath Fibers. ACS Biomater. Sci. Eng. 2019, 5, 3119–3130. [Google Scholar] [CrossRef]
- Ebrahimi, M.; Ostrovidov, S.; Salehi, S.; Kim, S.B.; Bae, H.; Khademhosseini, A. Enhanced skeletal muscle formation on microfluidic spun gelatin methacryloyl (GelMA) fibres using surface patterning and agrin treatment. J. Tissue Eng. Regen. Med. 2018, 12, 2151–2163. [Google Scholar] [CrossRef]
- Puppi, D.; Chiellini, F. Wet-spinning of biomedical polymers: From single-fibre production to additive manufacturing of three-dimensional scaffolds. Polym. Int. 2017, 66, 1690–1696. [Google Scholar] [CrossRef]
- Stone, R.; Hipp, S.; Barden, J.; Brown, P.J.; Mefford, O.T. Highly scalable nanoparticle–polymer composite fiber via wet spinning. J. Appl. Polym. Sci. 2013, 130, 1975–1980. [Google Scholar] [CrossRef]
- Ostrovidov, S.; Salehi, S.; Costantini, M.; Suthiwanich, K.; Ebrahimi, M.; Sadeghian, R.B.; Fujie, T.; Shi, X.; Cannata, S.; Gargioli, C.; et al. 3D Bioprinting in Skeletal Muscle Tissue Engineering. Small 2019, 15, 1805530. [Google Scholar] [CrossRef] [PubMed]
- Constante, G.; Apsite, I.; Alkhamis, H.; Dulle, M.; Schwarzer, M.; Caspari, A.; Synytska, A.; Salehi, S.; Ionov, L. 4D Biofabrication Using a Combination of 3D Printing and Melt-Electrowriting of Shape-Morphing Polymers. ACS Appl. Mater. Interfaces 2021, 13, 12767–12776. [Google Scholar] [CrossRef] [PubMed]
- Groll, J.; Boland, T.; Blunk, T.; Burdick, J.A.; Cho, D.-W.; Dalton, P.D.; Derby, B.; Forgacs, G.; Li, Q.; Mironov, V.A.; et al. Biofabrication: Reappraising the definition of an evolving field. Biofabrication 2016, 8, 013001. [Google Scholar] [CrossRef] [PubMed]
- Chen, X.-Y.; Chen, J.-Y.; Tong, X.-M.; Mei, J.-G.; Chen, Y.-F.; Mou, X.-Z. Recent advances in the use of microcarriers for cell cultures and their ex vivo and in vivo applications. Biotechnol. Lett. 2020, 42, 1–10. [Google Scholar] [CrossRef] [PubMed]
- Huang, L.; Abdalla, A.M.E.; Xiao, L.; Yang, G. Biopolymer-Based Microcarriers for Three-Dimensional Cell Culture and Engineered Tissue Formation. Int. J. Mol. Sci. 2020, 21, 1895. [Google Scholar] [CrossRef] [PubMed]
- Salehi, S.; Ostrovidov, S.; Ebrahimi, M.; Sadeghian, R.B.; Liang, X.; Nakajima, K.; Bae, H.; Fujie, T.; Khademhosseini, A. Development of Flexible Cell-Loaded Ultrathin Ribbons for Minimally Invasive Delivery of Skeletal Muscle Cells. ACS Biomater. Sci. Eng. 2017, 3, 579–589. [Google Scholar] [CrossRef] [PubMed]
- Sonnleitner, D.; Schrüfer, S.; Berglund, L.; Schubert, D.W.; Lang, G. Correlating rheology and printing performance of fiber-reinforced bioinks to assess predictive modelling for biofabrication. J. Mater. Res. 2021, 36, 3821–3832. [Google Scholar] [CrossRef]
- Benwood, C.; Chrenek, J.; Kirsch, R.L.; Masri, N.Z.; Richards, H.; Teetzen, K.; Willerth, S.M. Natural Biomaterials and Their Use as Bioinks for Printing Tissues. Bioengineering 2021, 8, 27. [Google Scholar] [CrossRef] [PubMed]
- Prendergast, M.E.; Davidson, M.D.; Burdick, J.A. A biofabrication method to align cells within bioprinted photocrosslinkable and cell-degradable hydrogel constructs via embedded fibers. Biofabrication 2021, 13, 044108. [Google Scholar] [CrossRef] [PubMed]
- Omidinia-Anarkoli, A.; Boesveld, S.; Tuvshindorj, U.; Rose, J.C.; Haraszti, T.; De Laporte, L. An Injectable Hybrid Hydrogel with Oriented Short Fibers Induces Unidirectional Growth of Functional Nerve Cells. Small 2017, 13, 1702207. [Google Scholar] [CrossRef] [PubMed]
- Rose, J.C.; Fölster, M.; Kivilip, L.; Gerardo-Nava, J.L.; Jaekel, E.E.; Gehlen, D.B.; Rohlfs, W.; De Laporte, L. Predicting the orientation of magnetic microgel rods for soft anisotropic biomimetic hydrogels. Polym. Chem. 2020, 11, 496–507. [Google Scholar] [CrossRef]
- Weller, H.G.; Tabor, G.; Jasak, H.; Fureby, C. A tensorial approach to computational continuum mechanics using object-oriented techniques. Comput. Phys. 1998, 12, 620–631. [Google Scholar] [CrossRef]
- Frenkel, D.; Smit, B. Chapter 1—Introduction. In Understanding Molecular Simulation, 2nd ed.; Frenkel, D., Smit, B., Eds.; Academic Press: San Diego, CA, USA, 2002; pp. 1–6. [Google Scholar]
- Heid, S.; Becker, K.; Byun, J.; Biermann, I.; Neščáková, Z.; Zhu, H.; Groll, J.; Boccaccini, A.R. Bioprinting with bioactive alginate dialdehyde-gelatin (ADA-GEL) composite bioinks: Time-dependent in-situ crosslinking via addition of calcium-silicate particles tunes in vitro stability of 3D bioprinted constructs. Bioprinting 2022, 26, e00200. [Google Scholar] [CrossRef]
- Tao, Y.-G.; den Otter, W.K.; Briels, W.J. Kayaking and Wagging of Rods in Shear Flow. Phys. Rev. Lett. 2005, 95, 237802. [Google Scholar] [CrossRef] [PubMed]
- Einarsson, J.; Candelier, F.; Lundell, F.; Angilella, J.R.; Mehlig, B. Rotation of a spheroid in a simple shear at small Reynolds number. Phys. Fluids 2015, 27, 063301. [Google Scholar] [CrossRef]
- Palanisamy, D.; Otter, W.K.d. Efficient Brownian Dynamics of rigid colloids in linear flow fields based on the grand mobility matrix. J. Chem. Phys. 2018, 148, 194112. [Google Scholar] [CrossRef]
- Meyer, N.; Saburow, O.; Hohberg, M.; Hrymak, A.N.; Henning, F.; Kärger, L. Parameter Identification of Fiber Orientation Models Based on Direct Fiber Simulation with Smoothed Particle Hydrodynamics. J. Compos. Sci. 2020, 4, 77. [Google Scholar] [CrossRef]
- Weller, C.G.a.H.G. Notes on Computational Fluid Dynamics: General Principles; CFD Direct Ltd.: Reading, UK, 2002. [Google Scholar]
- Emmermacher, J.; Spura, D.; Cziommer, J.; Kilian, D.; Wollborn, T.; Fritsching, U.; Steingroewer, J.; Walther, T.; Gelinsky, M.; Lode, A. Engineering considerations on extrusion-based bioprinting: Interactions of material behavior, mechanical forces and cells in the printing needle. Biofabrication 2020, 12, 025022. [Google Scholar] [CrossRef]
- Weeks, J.D.; Chandler, D.; Andersen, H.C. Role of Repulsive Forces in Determining the Equilibrium Structure of Simple Liquids. J. Chem. Phys. 1971, 54, 5237–5247. [Google Scholar] [CrossRef]
- Advani, S.G.; III, C.L.T. The Use of Tensors to Describe and Predict Fiber Orientation in Short Fiber Composites. J. Rheol. 1987, 31, 751–784. [Google Scholar] [CrossRef]
- Lee, B.R.; Lee, K.H.; Kang, E.; Kim, D.-S.; Lee, S.-H. Microfluidic wet spinning of chitosan-alginate microfibers and encapsulation of HepG2 cells in fibers. Biomicrofluidics 2011, 5, 022208. [Google Scholar] [CrossRef] [PubMed]
- Rubio, L.D.; Potomkin, M.; Baker, R.D.; Sen, A.; Berlyand, L.; Aranson, I.S. Self-Propulsion and Shear Flow Align Active Particles in Nozzles and Channels. Adv. Intell. Syst. 2021, 3, 2000178. [Google Scholar] [CrossRef]
- Rose, J.C.; Cámara-Torres, M.; Rahimi, K.; Köhler, J.; Möller, M.; De Laporte, L. Nerve Cells Decide to Orient inside an Injectable Hydrogel with Minimal Structural Guidance. Nano Lett. 2017, 17, 3782–3791. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.; Wang, Q.; Xu, C. Nanocellulose-Based Inks for 3D Bioprinting: Key Aspects in Research Development and Challenging Perspectives in Applications—A Mini Review. Bioengineering 2020, 7, 40. [Google Scholar] [CrossRef]
- Kosik-Kozioł, A.; Costantini, M.; Bolek, T.; Szöke, K.; Barbetta, A.; Brinchmann, J.; Święszkowski, W. PLA short sub-micron fiber reinforcement of 3D bioprinted alginate constructs for cartilage regeneration. Biofabrication 2017, 9, 044105. [Google Scholar] [CrossRef] [PubMed]
- Davidson, M.D.; Prendergast, M.E.; Ban, E.; Xu, K.L.; Mickel, G.; Mensah, P.; Dhand, A.; Janmey, P.A.; Shenoy, V.B.; Burdick, J.A. Programmable and contractile materials through cell encapsulation in fibrous hydrogel assemblies. Sci. Adv. 2021, 7, eabi8157. [Google Scholar] [CrossRef] [PubMed]
- Blaeser, A.; Duarte Campos, D.F.; Puster, U.; Richtering, W.; Stevens, M.M.; Fischer, H. Controlling Shear Stress in 3D Bioprinting is a Key Factor to Balance Printing Resolution and Stem Cell Integrity. Adv. Healthc. Mater. 2016, 5, 326–333. [Google Scholar] [CrossRef]
- Paxton, N.; Smolan, W.; Böck, T.; Melchels, F.; Groll, J.; Jungst, T. Proposal to assess printability of bioinks for extrusion-based bioprinting and evaluation of rheological properties governing bioprintability. Biofabrication 2017, 9, 044107. [Google Scholar] [CrossRef]
- Sonnleitner, D.; Schäfer, N.; Wieland, A.; Fischer, L.; Pasberg, P.; Thievessen, I.; Lang, G. PCL micro-dumbbells—A new class of polymeric particles reveals morphological biofunctionality. Appl. Mater. Today 2021, 24, 101097. [Google Scholar] [CrossRef]
- Ma, Z.; Wang, Y.; Ren, N.; Shi, W. A coupled CFD-DEM simulation of upward seepage flow in coarse sands. Mar. Georesources Geotechnol. 2019, 37, 589–598. [Google Scholar] [CrossRef]
- Blais, B.; Lassaigne, M.; Goniva, C.; Fradette, L.; Bertrand, F. Development of an unresolved CFD–DEM model for the flow of viscous suspensions and its application to solid–liquid mixing. J. Comput. Phys. 2016, 318, 201–221. [Google Scholar] [CrossRef]
- Bérard, A.; Patience, G.S.; Blais, B. Experimental methods in chemical engineering: Unresolved CFD-DEM. Can. J. Chem. Eng. 2020, 98, 424–440. [Google Scholar] [CrossRef]
- Aguado, B.A.; Mulyasasmita, W.; Su, J.; Lampe, K.J.; Heilshorn, S.C. Improving Viability of Stem Cells During Syringe Needle Flow Through the Design of Hydrogel Cell Carriers. Tissue Eng. Part A 2012, 18, 806–815. [Google Scholar] [CrossRef] [PubMed]
- Wilkes, G.L. An overview of the basic rheological behavior of polymer fluids with an emphasis on polymer melts. J. Chem. Educ. 1981, 58, 880. [Google Scholar] [CrossRef]
- Jeffery, G.B. The Motion of Ellipsoidal Particles Immersed in a Viscous Fluid. Proc. R. Soc. London. Ser. A Contain. Pap. A Math. Phys. Character 1922, 102, 161–179. [Google Scholar]
- Kim, T.; Trangkanukulkij, R.; Kim, W.S. Nozzle Shape Guided Filler Orientation in 3D Printed Photo-curable Nanocomposites. Sci. Rep. 2018, 8, 3805. [Google Scholar] [CrossRef]
- Lewicki, J.P.; Rodriguez, J.N.; Zhu, C.; Worsley, M.A.; Wu, A.S.; Kanarska, Y.; Horn, J.D.; Duoss, E.B.; Ortega, J.M.; Elmer, W.; et al. 3D-Printing of Meso-structurally Ordered Carbon Fiber/Polymer Composites with Unprecedented Orthotropic Physical Properties. Sci. Rep. 2017, 7, 43401. [Google Scholar] [CrossRef]
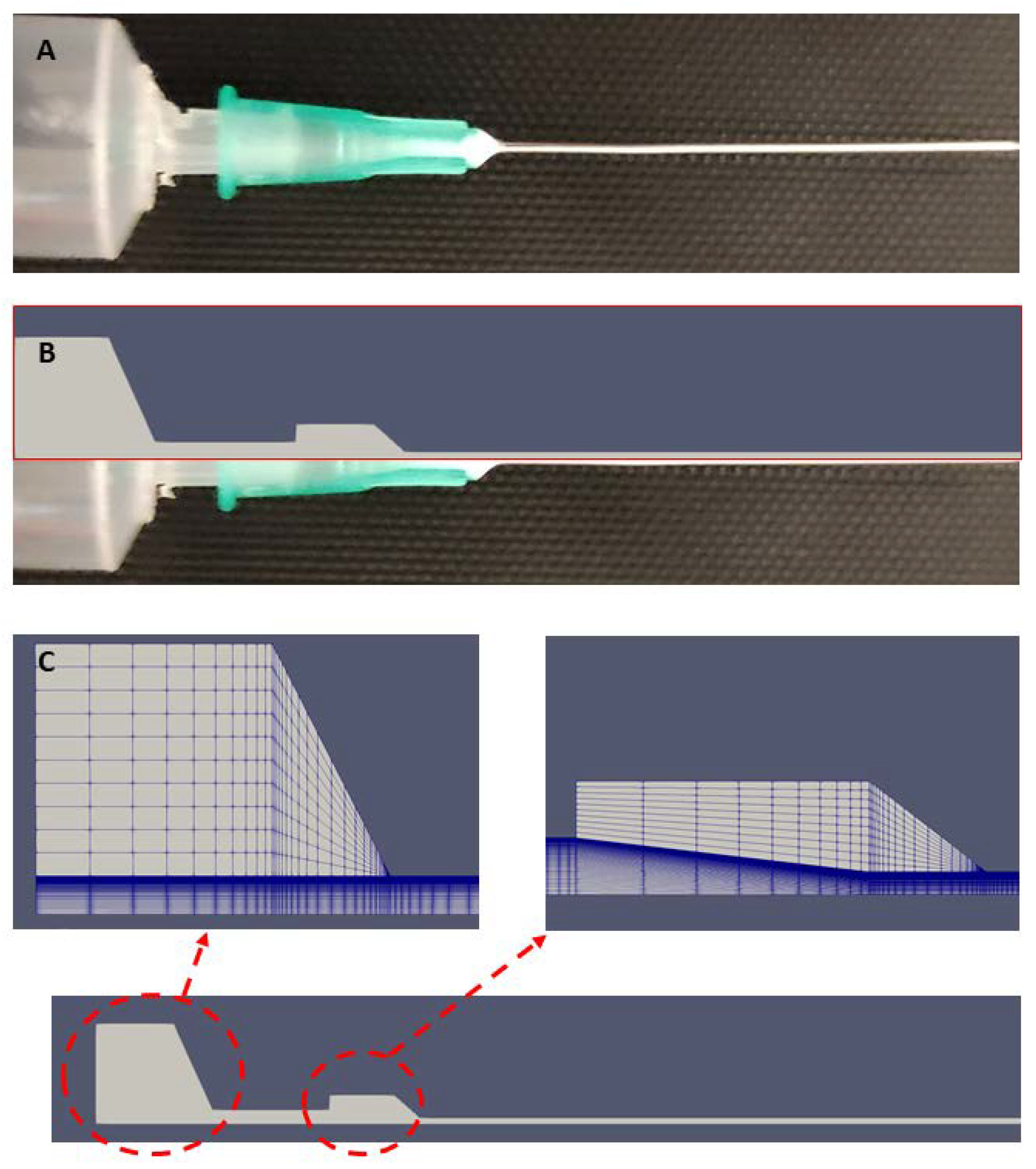

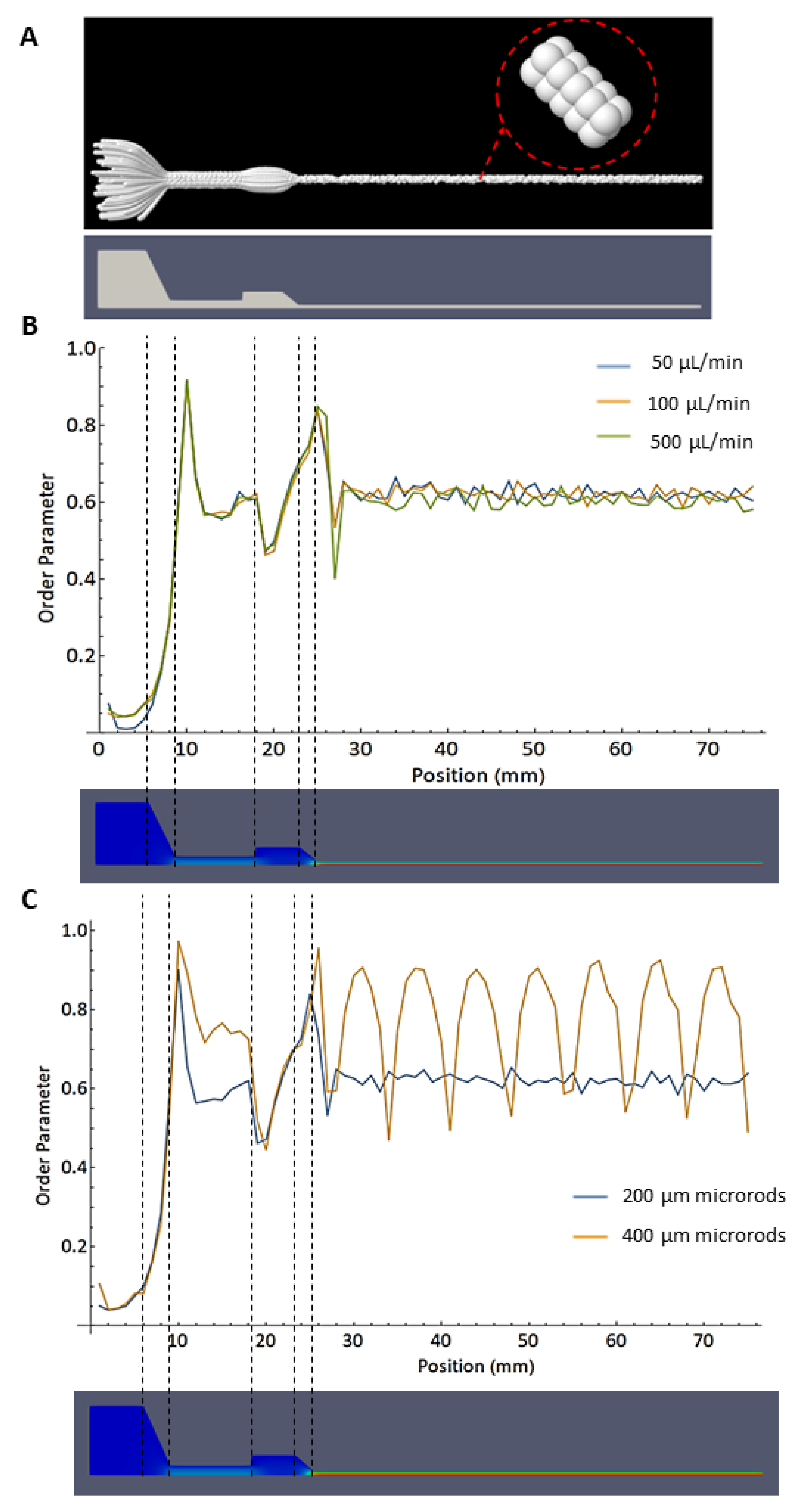
| Geometry Section | Distance, x (mm) | Diameter (mm) |
|---|---|---|
| Syringe (container) | 0–6 | 14.14 |
| Syringe cone | 6–9 | from 14.14 to 2 |
| Syringe (junction) | 9–18 | 2 |
| Needle (junction) | 18–23 | 4 |
| Needle cone | 23–25 | from 4 to dn |
| Needle | 25–75 | dn = 0.4 or dn = 0.8 |
| Desired Flow Rate (µL/min) | Needle Diameter (mm) | Kinematic Inlet Pressure (m2/s2) |
|---|---|---|
| 50 | 0.8 | 6.4 |
| 100 | 0.8 | 9 |
| 500 | 0.8 | 20 |
| 100 | 0.4 | 10 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gruhn, T.; Monsalve, C.O.; Müller, C.; Heid, S.; Boccaccini, A.R.; Salehi, S. Fabrication of Hydrogel-Based Composite Fibers and Computer Simulation of the Filler Dynamics in the Composite Flow. Bioengineering 2023, 10, 448. https://doi.org/10.3390/bioengineering10040448
Gruhn T, Monsalve CO, Müller C, Heid S, Boccaccini AR, Salehi S. Fabrication of Hydrogel-Based Composite Fibers and Computer Simulation of the Filler Dynamics in the Composite Flow. Bioengineering. 2023; 10(4):448. https://doi.org/10.3390/bioengineering10040448
Chicago/Turabian StyleGruhn, Thomas, Camilo Ortiz Monsalve, Claudia Müller, Susanne Heid, Aldo R. Boccaccini, and Sahar Salehi. 2023. "Fabrication of Hydrogel-Based Composite Fibers and Computer Simulation of the Filler Dynamics in the Composite Flow" Bioengineering 10, no. 4: 448. https://doi.org/10.3390/bioengineering10040448
APA StyleGruhn, T., Monsalve, C. O., Müller, C., Heid, S., Boccaccini, A. R., & Salehi, S. (2023). Fabrication of Hydrogel-Based Composite Fibers and Computer Simulation of the Filler Dynamics in the Composite Flow. Bioengineering, 10(4), 448. https://doi.org/10.3390/bioengineering10040448








