Computational Study of Hemodynamic Field of an Occluded Artery Model with Anastomosis
Abstract
1. Introduction
2. Materials and Methods
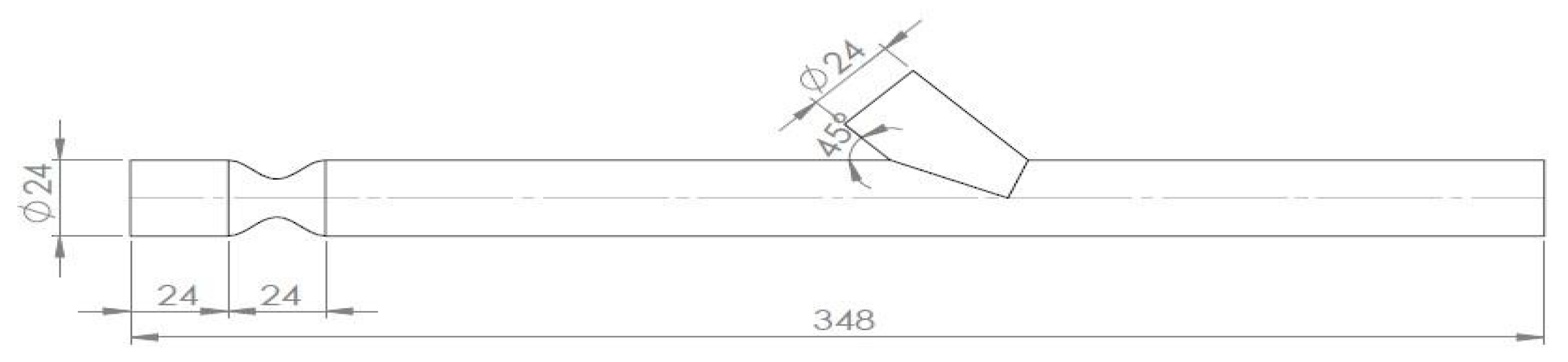
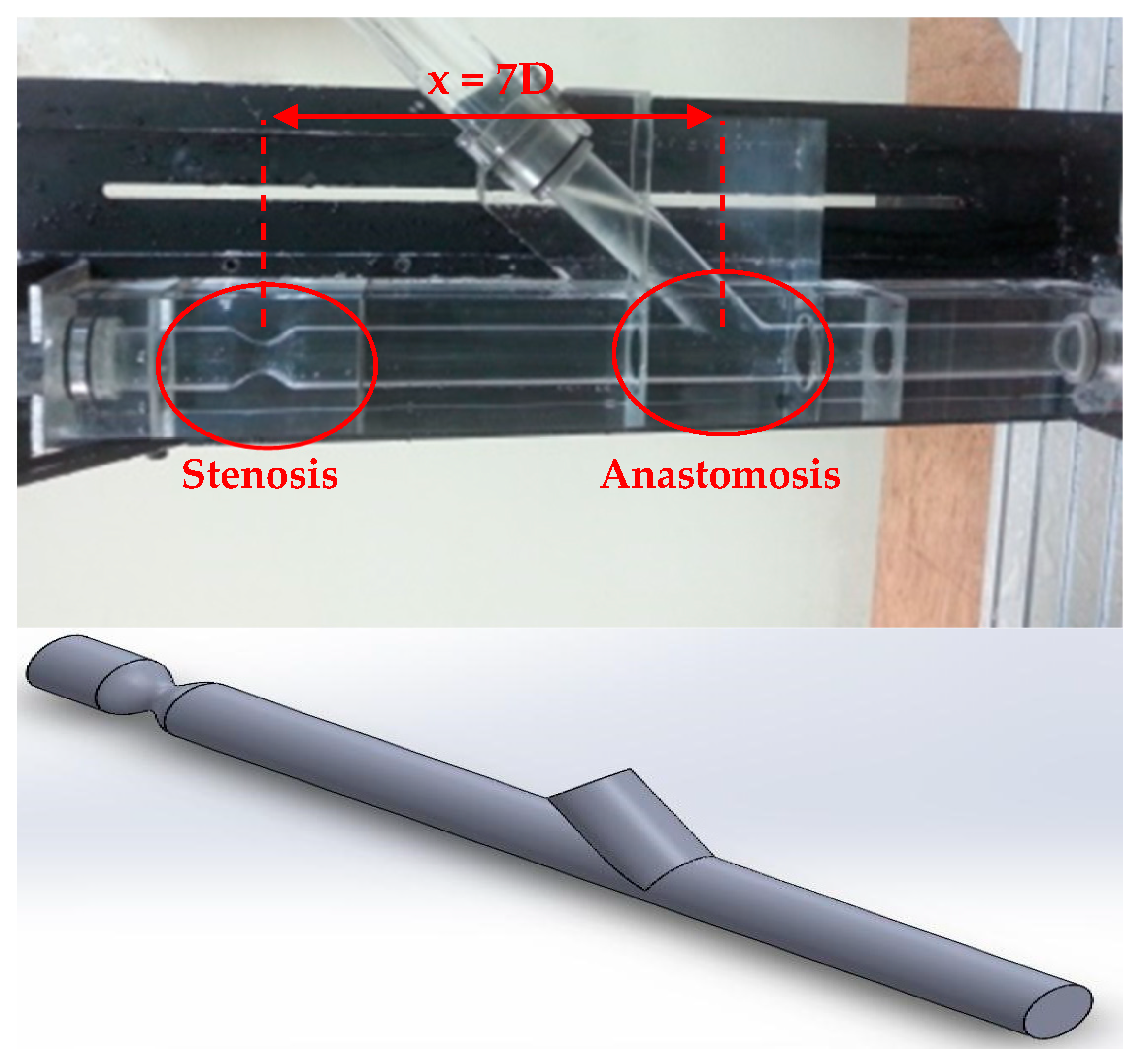
2.1. Model Geometry
2.2. Governing Equations
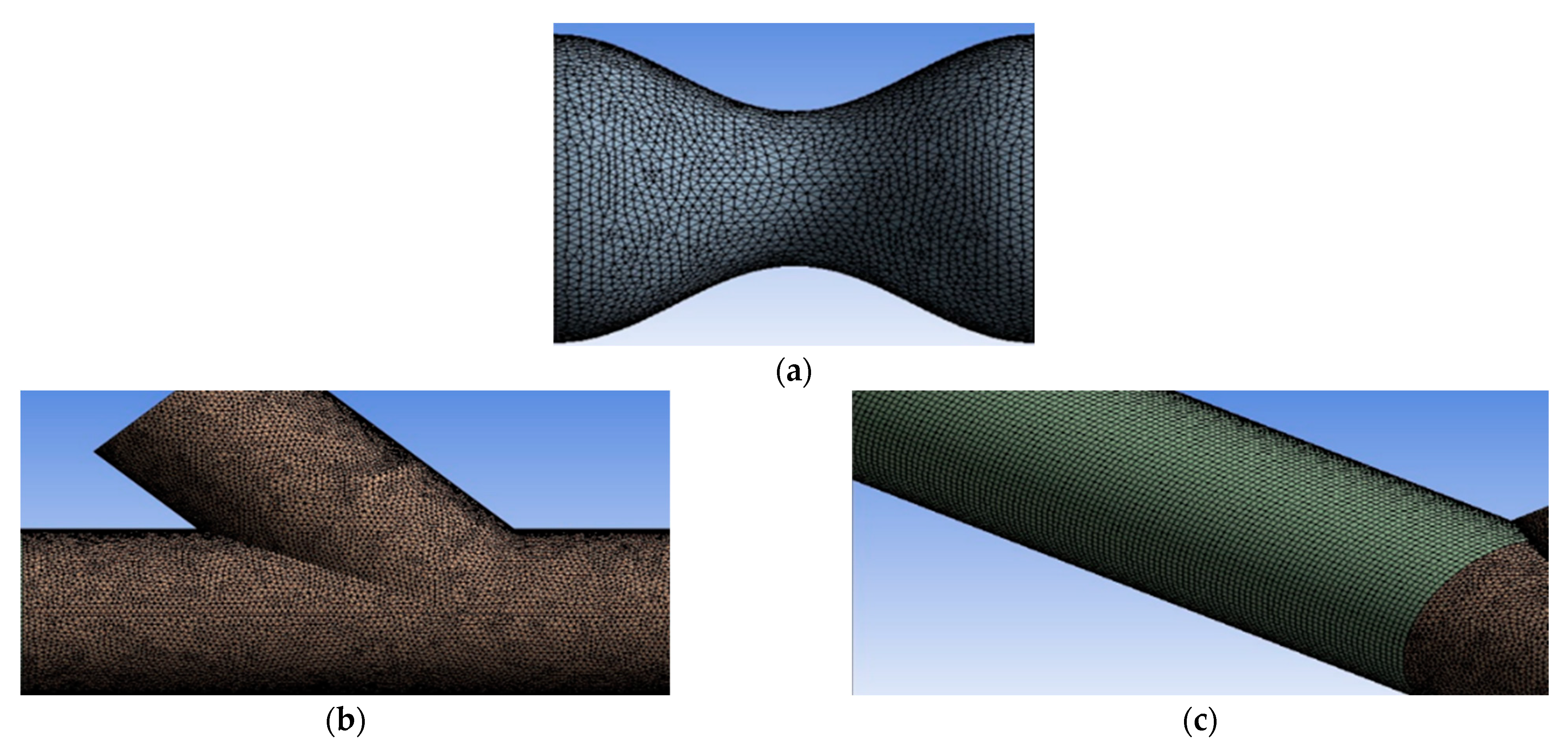
2.3. Numerical Model
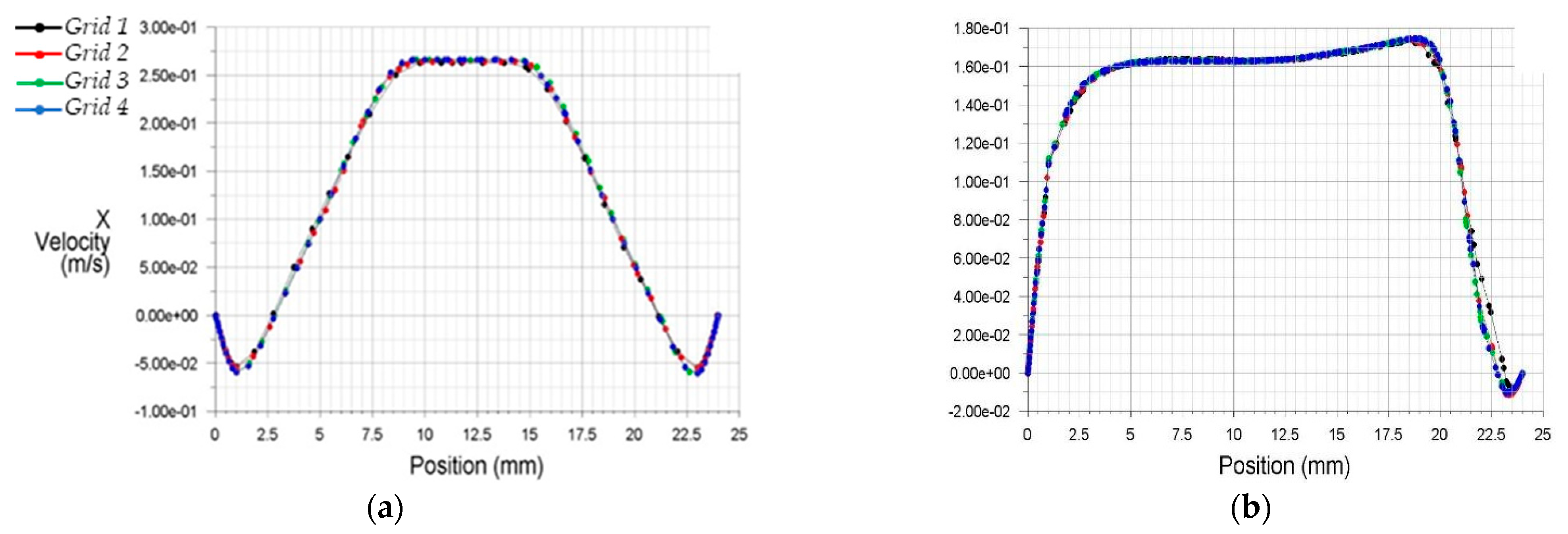
2.4. Grid Sensitivity Analysis
2.5. Boundary Conditions
3. Results
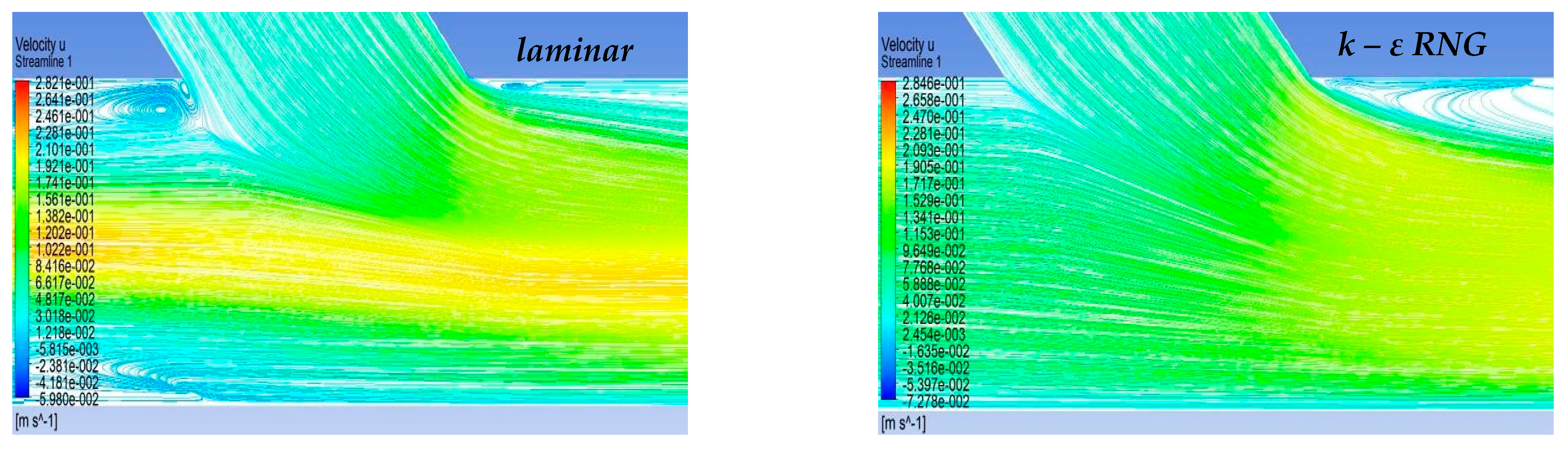
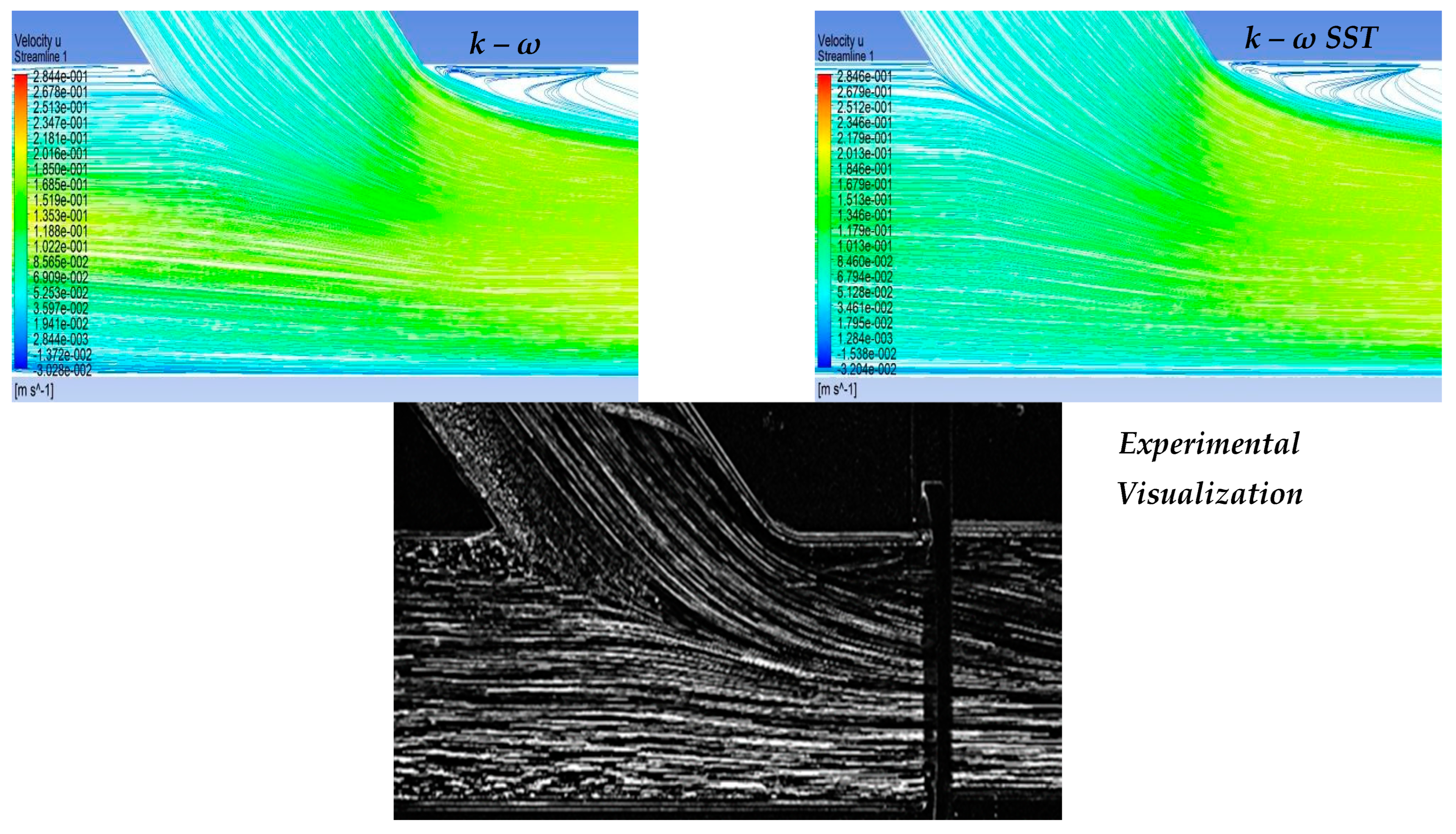
3.1. Qualitative Comparison of Flow Models
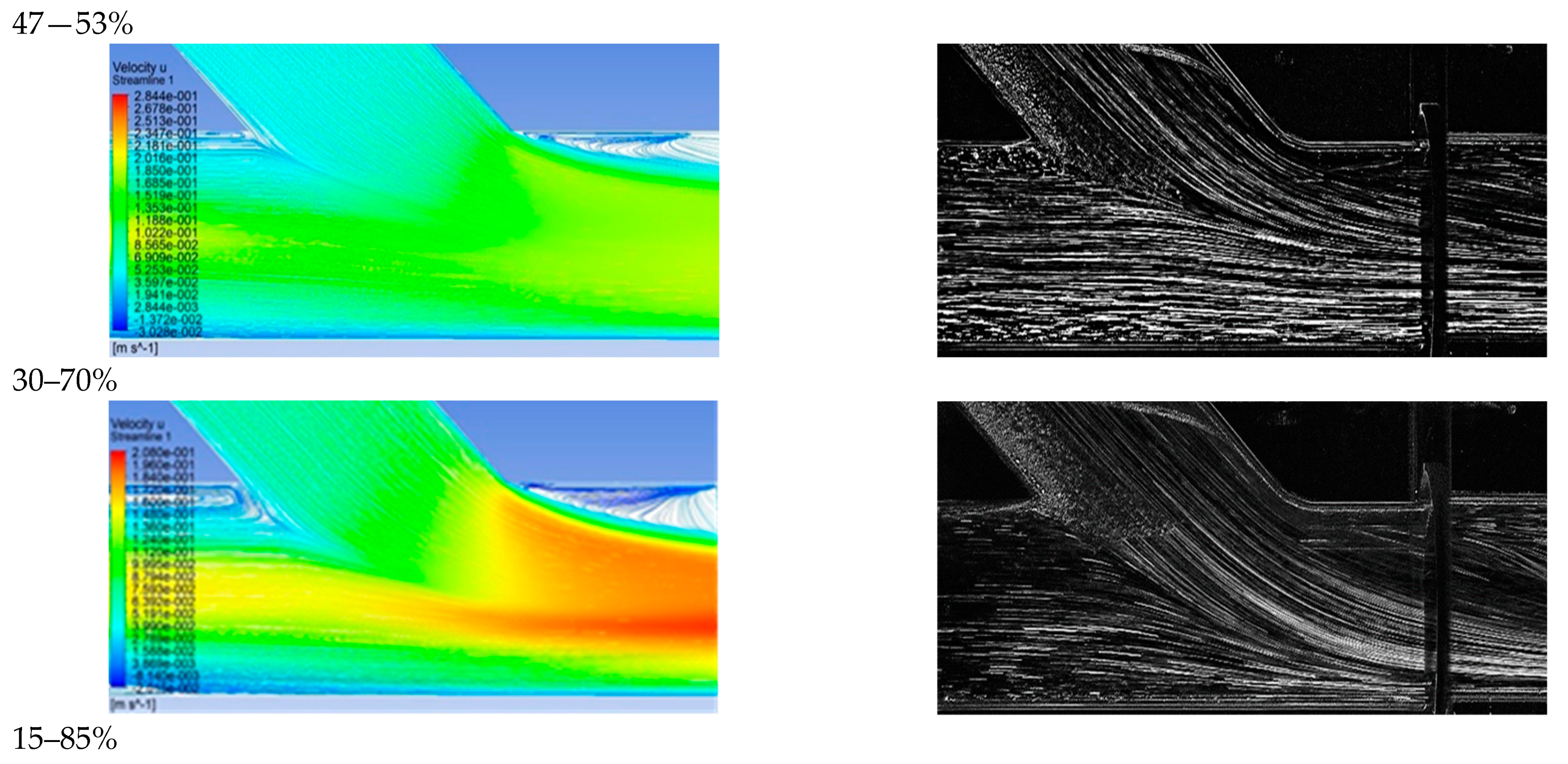
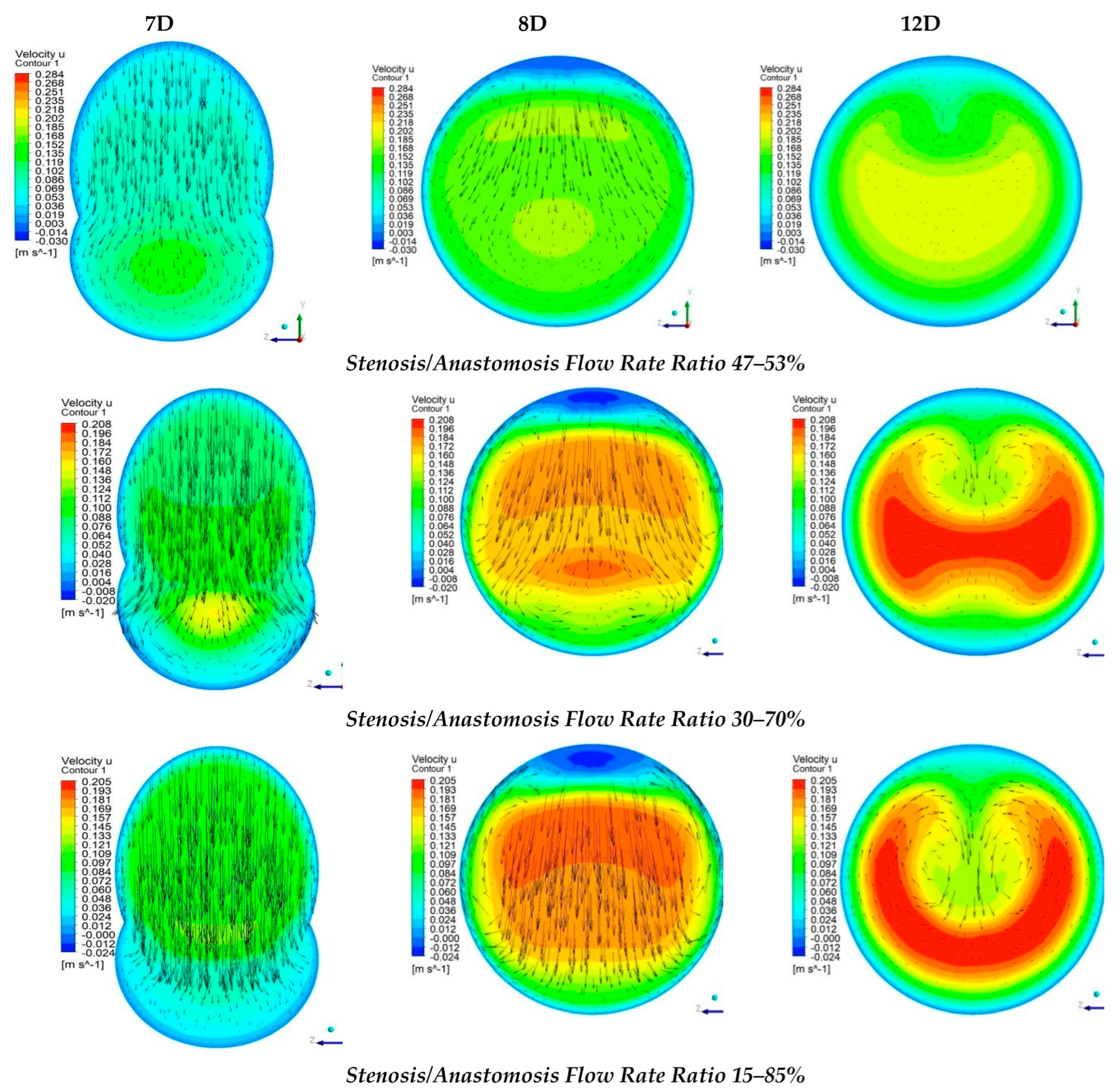
3.2. Velocity Field in the Artery Anastomosis Region
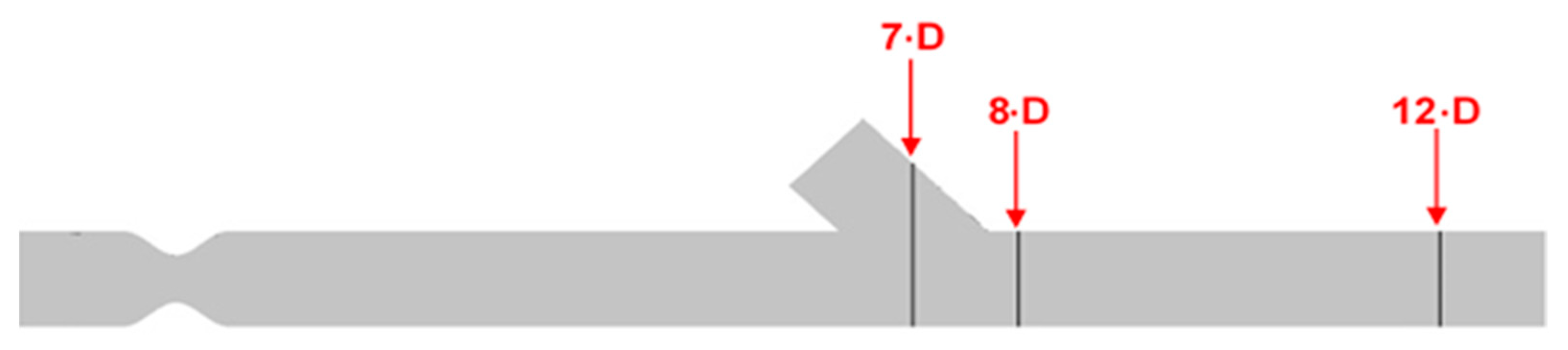
3.3. Cross Sectional Velocity Distribution
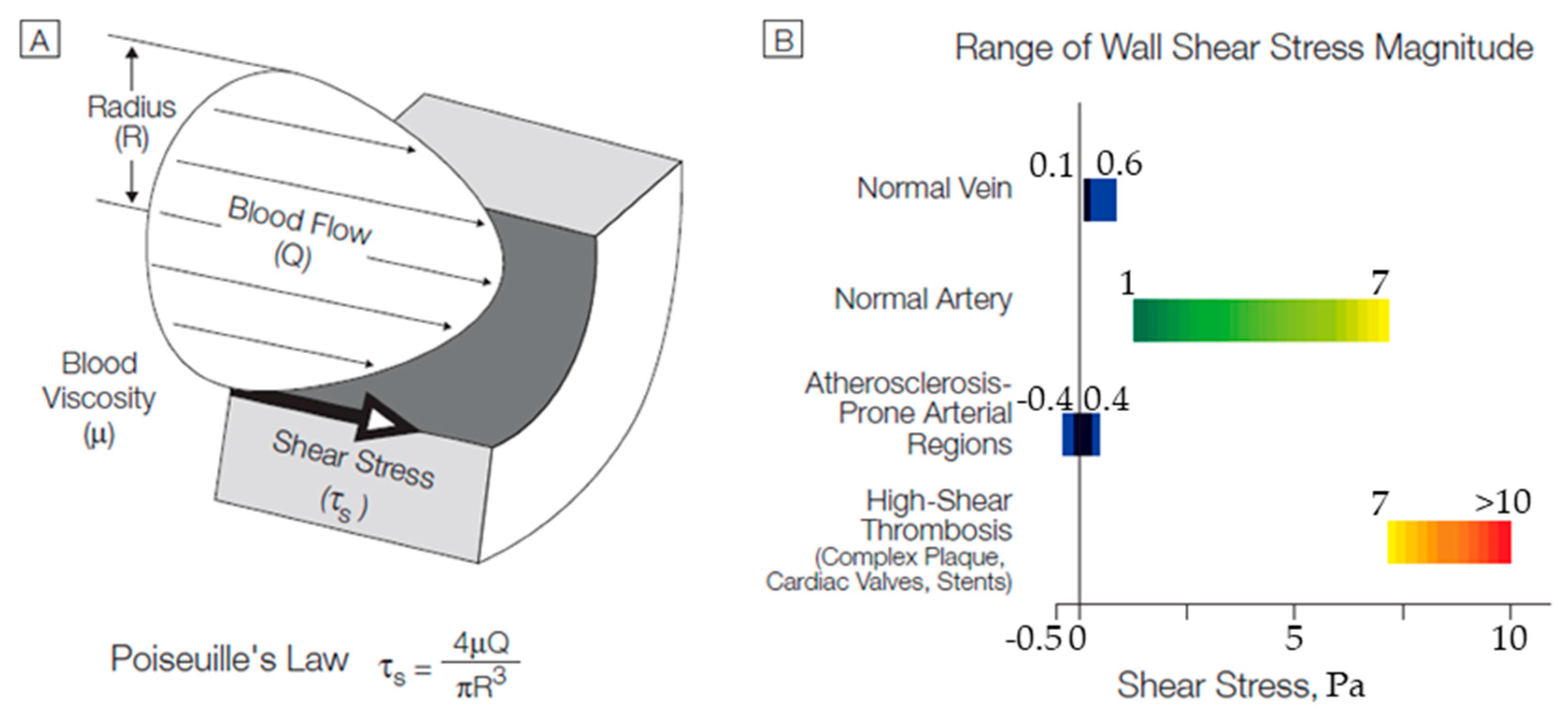
3.4. Wall Shear Stresses (WSS)
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Carvalho, V.; Pinho, D.; Lima, R.A.; Teixeira, J.C.; Teixeira, S. Blood Flow Modeling in Coronary Arteries: A Review. Fluids 2021, 6, 53–67. [Google Scholar] [CrossRef]
- Badimon, L.; Vilahur, G. Thrombosis formation on atherosclerotic lesions and plaque rupture. J. Intern. Med. 2014, 276, 618–632. [Google Scholar] [CrossRef]
- Lusis, A.J. Atherosclerosis. Nature 2000, 407, 233–241. [Google Scholar] [CrossRef] [PubMed]
- Kashyap, V.; Arora, B.B.; Bhattacharjee, S. A computational study of branch-wise curvature in idealized coronary artery bifurcations. Appl. Eng. Sci. 2020, 4, 100027. [Google Scholar] [CrossRef]
- Sun, Y.; Guan, X. Autophagy: A new target for the treatment of atherosclerosis. Front. Lab. Med. 2018, 2, 68–71. [Google Scholar] [CrossRef]
- Carpenter, H.J.; Gholipour, A.; Ghayesh, M.H.; Zander, A.C.; Psaltis, P.J. A review on the biomechanics of coronary arteries. Int. J. Eng. Sci. 2020, 147, 103201. [Google Scholar] [CrossRef]
- Lopes, D.; Puga, H.; Teixeira, J.; Lima, R. Blood flow simulations in patient-specific geometries of the carotid artery: A systematic review. J. Biomech. 2020, 111, 110019. [Google Scholar] [CrossRef]
- Zaromytidou, J.M.; Siasos, G.; Coskun, A.U.; Lucier, M.; Antoniadis, A.P.; Papafaklis, M.I.; Koskinas, K.C.; Andreou, I.; Feldman, C.L.; Stone, P.H. Intravascular hemodynamics and coronary artery disease: New insights and clinical implications. Hell. J. Cardiol. 2016, 57, 389–400. [Google Scholar] [CrossRef]
- Doutel, E.; Carneiro, J.; Campos, J.B.L.M.; Miranda, J.M. Experimental and numerical methodology to analyze flows in a coronary bifurcation. Eur. J. Mech. B Fluids 2018, 67, 341–356. [Google Scholar] [CrossRef]
- Abdelsalam, S.I.; Zaher, A.Z. On behavioral response of ciliated cervical canal on the development of electroosmotic forces in spermatic fluid, Modelling and Simulations of Fluid Flows. Math. Model. Nat. Phenom. 2022, 17, 27. [Google Scholar] [CrossRef]
- Alsharif, A.M.; Abdellateef, A.I.; Elmaboud, Y.A. Performance enhancement of a DC-operated micropump with electroosmosis in a hybrid nanofluid: Fractional Cattaneo heat flux problem. Appl. Math. Mech. 2022, 43, 931–944. [Google Scholar] [CrossRef]
- Abdelsalam, S.I.; Mekheimer, K.S.; Zaher, A.Z. Dynamism of a hybrid Casson nanofluid with laser radiation and chemical reaction through sinusoidal channels. Waves Random Complex Media 2022. [CrossRef]
- Nisco, G.D.; Hoogendoorn, A.; Chiastra, C.; Gallo, D.; Kok, A.M.; Morbiducci, U.; Wentzel, J.J. The impact of helical flow on coronary atherosclerotic plaque development. Atherosclerosis 2020, 300, 39–46. [Google Scholar] [CrossRef] [PubMed]
- Samady, H.; Eshtehardi, P.; McDaniel, M.C.; Suo, J.; Dhawan, S.S.; Maynard, C.; Timmins, L.H.; Quyyumi, A.A.; Giddens, D.P. Coronary artery wall shear stress is associated with progression and transformation of atherosclerotic plaque and arterial remodeling in patients with coronary artery disease. Circulation 2011, 124, 779–788. [Google Scholar] [CrossRef]
- Han, D.; Starikov, A.; Hartaigh, B.; Gransar, H.; Kolli, K.K.; Lee, J.H.; Rizvi, A.; Baskaran, L.; Schulman-Marcus, J.; Lin, F.Y.; et al. Relationship between endothelial wall shear stress and high-risk atherosclerotic plaque characteristics for identification of coronary lesions that cause ischemia: A direct comparison with fractional flow reserve. J. Am. Heart Assoc. 2016, 5, e004186. [Google Scholar] [CrossRef]
- Siasos, G.G.; Sara, J.D.; Zaromytidou, M.; Park, K.H.; Coskun, A.U.; Lerman, L.O.; Oikonomou, E.; Maynard, C.C.; Fotiadis, D.; Stefanou, K.; et al. Local Low Shear Stress and Endothelial Dysfunction in PatientsWith Nonobstructive Coronary Atherosclerosis. J. Am. Coll. Cardiol. 2018, 71, 2092–2102. [Google Scholar] [CrossRef]
- Soulis, J.V.; Fytanidis, D.K.; Seralidou, K.V.; Giannoglou, G.D. Wall shear stress oscillation and its gradient in the normal left coronary artery tree bifurcations. Hippokratia 2014, 18, 12–16. [Google Scholar]
- Pandey, R.; Kumar, M.; Majdoubi, J.; Rahimi-Gorji, M.; Srivastav, V.K. A review study on blood in human coronary artery: Numerical approach. Comput. Methods Program. Biomed. 2020, 187, 105243. [Google Scholar] [CrossRef]
- Carvalho, V.; Maia, I.; Souza, A.; Ribeiro, J.; Costa, P.; Puga, H.; Teixeira, S.F.C.F.; Lima, R.A. In vitro stenotic arteries to perform blood analogues flow visualizations and measurements: A Review. Open Biomed. Eng. J. 2020, 14, 87–102. [Google Scholar] [CrossRef]
- Zuo, Y.; Estes, S.K.; Ali, R.A.; Gandhi, A.A.; Yalavarthi, S.; Shi, H.; Sule, G.; Gockman, K.; Madison, J.A.; Zuo, M.; et al. Prothrombotic autoantibodies in serum from patients hospitalized with COVID-19. Sci. Transl. Med. 2020, 3876, 1–17. [Google Scholar] [CrossRef]
- LaDisa, J.F.; Olson, L.E.; Douglas, H.A.; Warltier, D.C.; Kersten, J.R.; Pagel, P.S. Alterations in regional vascular geometry produced by theoretical stent implantation influence distributions of wall shear stress: Analysis of a curved coronary artery using 3D computational fluid dynamics modeling. Biomed. Eng. Online 2006, 5, 40. [Google Scholar] [CrossRef] [PubMed]
- Griggs, R.; Wing, E.F.G. Cecil Essentials of Medicine, 9th ed.; Elsevier: New York, NY, USA, 2016; ISBN 9781437718997. [Google Scholar]
- Loth, F.; Jones, S.A.; Zarins, C.K.; Giddens, D.P.; Nassar, R.F.; Glagov, S.; Bassiouny, H.S. Relative Contribution of Wall Shear Stress and Injury in Experimental Intimal Thickening at PTFE End-to-Side Arterial Anastomoses. J. Biomech. Eng. 2002, 124, 44–51. [Google Scholar] [CrossRef] [PubMed]
- Bassiouny, H.S.; White, S.; Glagov, S.; Choi, E.; Giddens, D.P.; Zarins, C.K. Anastomotic intimal hyperplasia: Mechanical injury or flow induced. J. Vasc. Surg. 1992, 15, 708–716. [Google Scholar] [CrossRef] [PubMed]
- Longest, P.W.; Kleinstreuer, C. Computational hemodynamics analysis and comparison study of arterio-venous grafts. J. Med. Eng. Technol. 2000, 24, 102–110. [Google Scholar] [CrossRef]
- David, N.K. Blood Flow in Arteries. Annu. Rev. Fluid Mech. 1997, 29, 399–434. [Google Scholar] [CrossRef]
- Fillinger, M.F.; Reinitz, E.R.; Schwartz, R.A.; Resetarits, D.E.; Paskanik, A.M. Graft geometry and venous intimal-medial hyperplasia in arteriovenous loop grafts. J. Vasc. Surg. 1990, 11, 556–566. [Google Scholar] [CrossRef]
- Giordana, S.; Sherwin, S.J.; Peiro, J.; Doorly, D.J.; Crane, J.S.; Lee, K.E.; Cheshire, N.J.W.; Caro, C.G. Local and global geometric influence on steady flow in distal anastomoses of peripheral bypass grafts. J. Biomech. Eng. 2005, 127, 1087–1089. [Google Scholar] [CrossRef]
- Keynton, R.S.; Evancho, M.M.; Sims, R.L.; Rodway, N.V.; Gobin, A.; Rittgers, S.E. Intimal hyperplasia and wall shear in arterial bypass graft distal anastomoses: An in vivo model study. J. Biomech. Eng. 2001, 123, 464–467. [Google Scholar] [CrossRef]
- Rittgers, S.E.; Karayannacos, P.E.; Guy, J.F.; Nerem, R.M.; Shaw, G.M. Velocity distribution and intimal proliferation in autologous vein graft in dogs. Circ. Res. 1978, 42, 792–801. [Google Scholar] [CrossRef]
- Sottiurai, V.S.; Yao, J.S.; Batson, R.C.; Sue, S.L.; Jones, R.; Nakamura, Y.A. Distal anastomotic intimal hyperplasia: Histopathologic character and biogenesis. Annu. Rev. Fluid Mech. 1989, 3, 26–33. [Google Scholar] [CrossRef]
- Kamiya, A.; Togawa, T. Adaptive regulation of wall shear stress to flow change in the canine carotid artery. Am. J. Physiol. 1980, 239, H14–H21. [Google Scholar] [CrossRef] [PubMed]
- Giannadakis, A.; Perrakis, K.; Panidis, T.; Romeos, A. Experimental investigation of the Hemodynamic Field of Occluded Arteries with Double Stenosis. In Proceedings of the 10th IEEE International Workshop of Biomedical Engineering, Kos Island, Greece, 14 November 2011. [Google Scholar] [CrossRef]
- Kalogirou, I.D.; Romeos, A.; Giannadakis, A.; Perrakis, K.; Panidis, T. Flow patterns in an occluded artery with an end to side anastomosis model. A visualization study. NAUN Int. J. Biol. Biomed. Eng. 2016, 10, 159–167. [Google Scholar]
- Romeos, A.; Giannadakis, A.; Kalogirou, I.; Perrakis, K.; Panidis, T.H. Visualization study of an occluded artery with an end-to-side anastomosis, INASE. In Proceedings of the 19th International Conference on Circuits, Systems, Communications and Computers—Continuum Mechanics, Zakynthos Island, Greece, 16–20 July 2015. [Google Scholar]
- Loth, F.; Fischer, P.F.; Bassiouny, H.S. Blood Flow in End to Side Anastomosis. Annu. Rev. Fluid Mech. 2008, 40, 367–393. [Google Scholar] [CrossRef]
- Keynton, R.S.; Rittgers, S.E.; Shu, M.C.S. The effect of angle and flow rate upon hemodynamics in distal vascular graft anastomoses: An in vitro model study. J. Biomech. Eng. 1991, 113, 458–463. [Google Scholar] [CrossRef]
- Politis, A.K.; Stavropoulos, G.P.; Christolis, M.N.; Panagopoulos, P.G.; Vlachos, N.S.; Markatos, N.C. Numerical modeling of simulated blood flow in idealized composite arterial coronary grafts: Steady state simulations. J. Biomech. 2007, 40, 1125–1136. [Google Scholar] [CrossRef]
- Sui, Y.; Ma, B.; Chu, B.; Qiao, A. Optimization of Anastomotic Configuration in CABG Surgery. Commun. Numer. Methods Eng. 2009, 25, 1097–1106. [Google Scholar] [CrossRef]
- Politis, A.K.; Stavropoulos, G.P.; Christolis, M.N.; Panagopoulos, P.G.; Vlachos, N.S.; Markatos, N.C. Numerical modelling of simulated blood flow in idealized composite arterial coronary grafts: Transient flow. J. Biomech. 2008, 41, 25–39. [Google Scholar] [CrossRef]
- Ansys Fluent Theory Guide, ANSYS Inc.,Release 2021R2, July 2021. Available online: www.ansys.com (accessed on 12 November 2022).
- Ghalichi, F.; Deng, X.; De Champlain, A.; Douville, Y.; King, M.; Guidoin, R. Low Reynolds number turbulence modeling of blood flow in arterial stenosis. Biorheology 1998, 35, 281–294. [Google Scholar] [CrossRef]
- Skouras, E.D.; Paraskeva, C.A.; Valavanides, M.S.; Kalarakis, A.N.; Kalogirou, I.; Mavridis, C. Computational Investigation of Flow Field in Biological Liquids at Clinically Significant Conditions using Meshless Methods. In Proceedings of the 10th Panhellenic Science Congress Chemistry Engineering, Patras, Greece, 4–6 June 2015. [Google Scholar]
- Frankel, S.H.; Varghese, S.S. Numerical Modeling of Pulsatile Turbulent Flow in Stenotic Vessels. J. Biomech. Eng. 2003, 125, 445–460. [Google Scholar] [CrossRef]
- Kabir, M.A.; Alam, M.F.; Uddin, M.A. A numerical study on the effects of Reynolds number on blood flow with spiral velocity through regular arterial stenosis. Chiang Mai J. Sci. 2018, 45, 2515–2527. [Google Scholar]
- Carvalho, V.; Rodrigues, N.; Ribeiro, R.; Costa, P.; Teixeira, J.C.F.; Lima, R.; Teixeira, S.F.C.F. Hemodynamic study in 3D printed stenotic coronary artery models: Experimental validation and transient simulation. Comput. Methods Biomech. Biomed. Eng. 2020, 24, 623–626. [Google Scholar] [CrossRef] [PubMed]
- Carvalho, V.; Rodrigues, N.; Lima, R.A.; Teixeira, S.F.C.F. Modeling blood pulsatile turbulent flow in stenotic coronary arteries. Int. J. Biol. Biomed. Eng. 2020, 14, 1998–4510. [Google Scholar] [CrossRef]
- Kamangar, S.; Salman Ahmed, N.J.; Badruddin, I.A.; Al-Rawahi, N.; Husain, A.; Govindaraju, K.; Yunus Khan, T.M. Effect of stenosis on hemodynamics in left coronary artery based on patient-specific CT scan. Biomed. Mater. Eng. 2019, 30, 463–473. [Google Scholar] [CrossRef] [PubMed]
- Banks, J.; Bressloff, N.W. Turbulence Modeling in Three-Dimensional Stenosed Arterial Bifurcations. J. Biomech. Eng. 2007, 129, 40–50. [Google Scholar] [CrossRef] [PubMed]
- Bertolotti, C.; Deplano, V. Three-dimensional numerical simulations of flow through a stenosed coronary bypass. J. Biomech. 1999, 33, 1011–1022. [Google Scholar] [CrossRef]
- Lakin, W.D.; Stevens, S.A.; Goetz, W. A Differentiable, Periodic Function for Pulsatile Cardiac Output Based on Heart Rate and Stroke Volume. Math. Biosci. 2003, 182, 201–211. [Google Scholar] [CrossRef]
- Malek, A.M.; Alper, S.L.; Izumo, S. Hemodynamic Shear Stress and Its Role in Atherosclerosis. JAMA 1999, 282, 235–242. [Google Scholar] [CrossRef]







| Grids | Number of Elements | Number of Nodes |
|---|---|---|
| Grid 1 | 7.65·105 | 4.28·105 |
| Grid 2 | 1.15·106 | 5.81·105 |
| Grid 3 | 2.45·106 | 1.23·106 |
| Grid 4 | 3.17·106 | 1.63·106 |
| Cases | Flow Rate Proportion | Calculation Positions | Velocity (m/s) | Re |
|---|---|---|---|---|
| 1st | 47% | A | 0.063 | 482 |
| 53% | B | 0.072 | 544 | |
| 47% | C | 0.254 | 964 | |
| 100% | D | 0.135 | 1026 | |
| 2nd | 30% | A | 0.041 | 308 |
| 70% | B | 0.095 | 718 | |
| 30% | C | 0.162 | 616 | |
| 100% | D | 0.135 | 1026 | |
| 3rd | 15% | A | 0.02 | 154 |
| 85% | B | 0.115 | 872 | |
| 15% | C | 0.081 | 308 | |
| 100% | D | 0.135 | 1026 | |
| 4th | 0% | A | 0 | 0 |
| 100% | B | 0.135 | 1026 | |
| 0% | C | 0 | 0 | |
| 100% | D | 0.135 | 1026 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Parissis, P.; Romeos, A.; Giannadakis, A.; Kalarakis, A.; Peroulis, M. Computational Study of Hemodynamic Field of an Occluded Artery Model with Anastomosis. Bioengineering 2023, 10, 146. https://doi.org/10.3390/bioengineering10020146
Parissis P, Romeos A, Giannadakis A, Kalarakis A, Peroulis M. Computational Study of Hemodynamic Field of an Occluded Artery Model with Anastomosis. Bioengineering. 2023; 10(2):146. https://doi.org/10.3390/bioengineering10020146
Chicago/Turabian StyleParissis, Panagiotis, Alexandros Romeos, Athanasios Giannadakis, Alexandros Kalarakis, and Michail Peroulis. 2023. "Computational Study of Hemodynamic Field of an Occluded Artery Model with Anastomosis" Bioengineering 10, no. 2: 146. https://doi.org/10.3390/bioengineering10020146
APA StyleParissis, P., Romeos, A., Giannadakis, A., Kalarakis, A., & Peroulis, M. (2023). Computational Study of Hemodynamic Field of an Occluded Artery Model with Anastomosis. Bioengineering, 10(2), 146. https://doi.org/10.3390/bioengineering10020146






