Impact of Coastal Wetland Restoration Plan on the Water Balance Components of Heeia Watershed, Hawaii
Abstract
1. Introduction
2. Materials and Methods
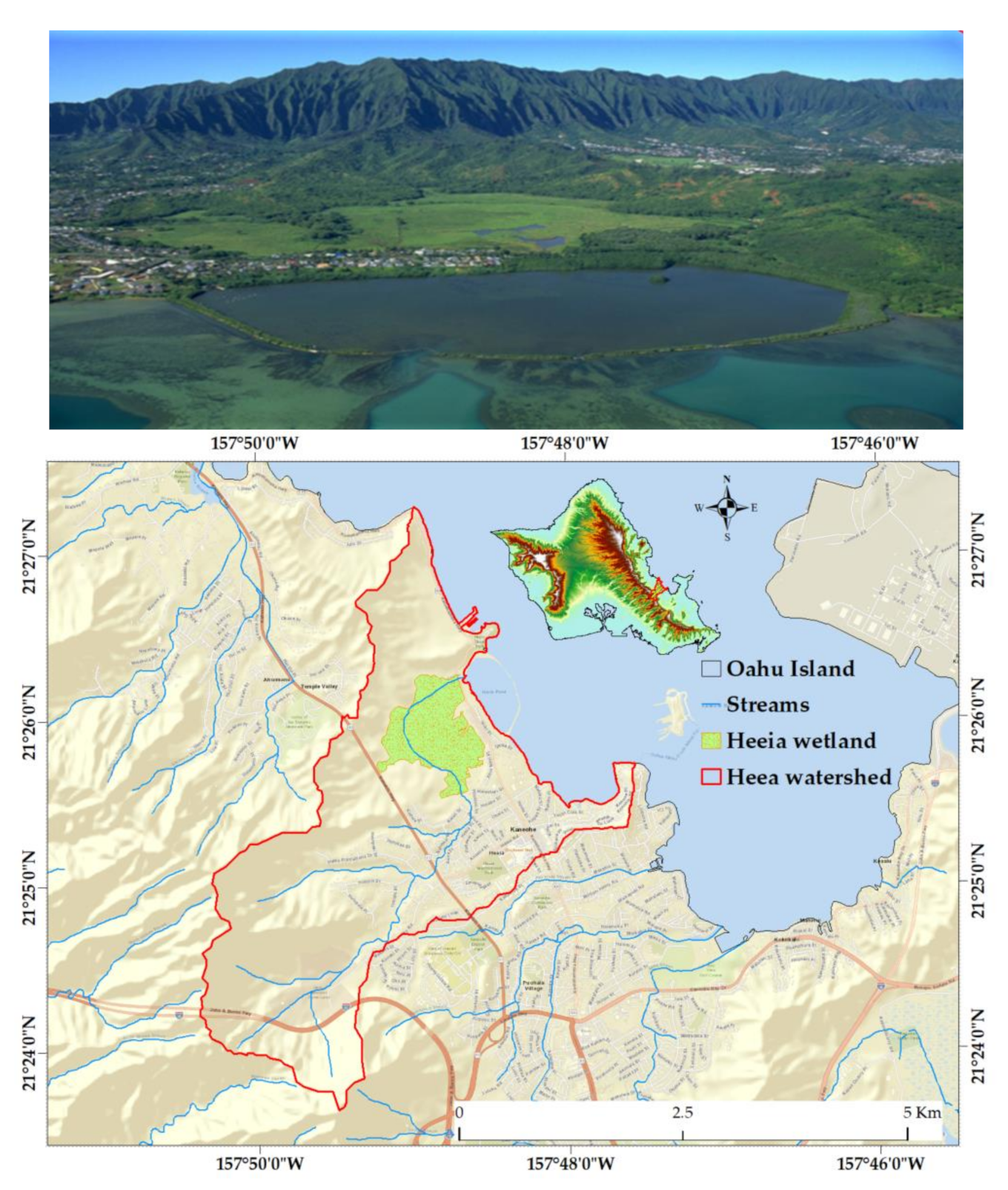
2.1. Study Area
2.2. Available Data
- A 10 × 10 m Digital Elevation Model (DEM) obtained from the Department of Commerce (DOC), National Oceanic and Atmospheric Administration (NOAA), Center for Coastal Monitoring and Assessment (CCMA).
- A 1:24,000 scale soil map was obtained from the Soil Survey Geographic (SSURGO) database as provided by the US Department of Agriculture, Natural Resources Conservation Service (USDA-NRCS).
- A 2.4 × 2.4 m land use map data was downloaded from the NOAA Coastal Change Analysis Program (C-CAP), http://coast.noaa.gov/ccapftp/.
- Due to lack of hydro-meteorological data within the watershed, we utilized various approaches, including interpolation, rescaling, and estimation based on the observed data and contour maps. For instance, fifteen virtual stations (Figure 3) were created within the watershed based on the spatial variability of rainfall. Rainfall values were generated for each station using the closest rain gauge station and isohyets of the Rainfall Atlas of Hawaii [26]. To fill the other missing variables (temperature, wind speed, solar radiation, and relative humidity), a method proposed by Leta et al. [27] was used.
- Also used in the study is the daily streamflow data recorded at the Haiku station (U.S. Geological Survey (USGS) gauging station: 16275000) and others measured by this study at the coastal plain and estuary at the wetland flow sampling station for the period from 2012 to 2013. For the coastal plain, long-term and continuous streamflows were estimated based on the method developed by Leta et al. [27].
2.3. SWAT Model Description and Setup
2.4. Model Sensitivity, Calibration, Validation, and Uncertainty Analysis
2.5. Model Performance Evaluation
2.6. Land Cover Change Scenario
2.7. Wetland Taro Management
3. Results and Discussion
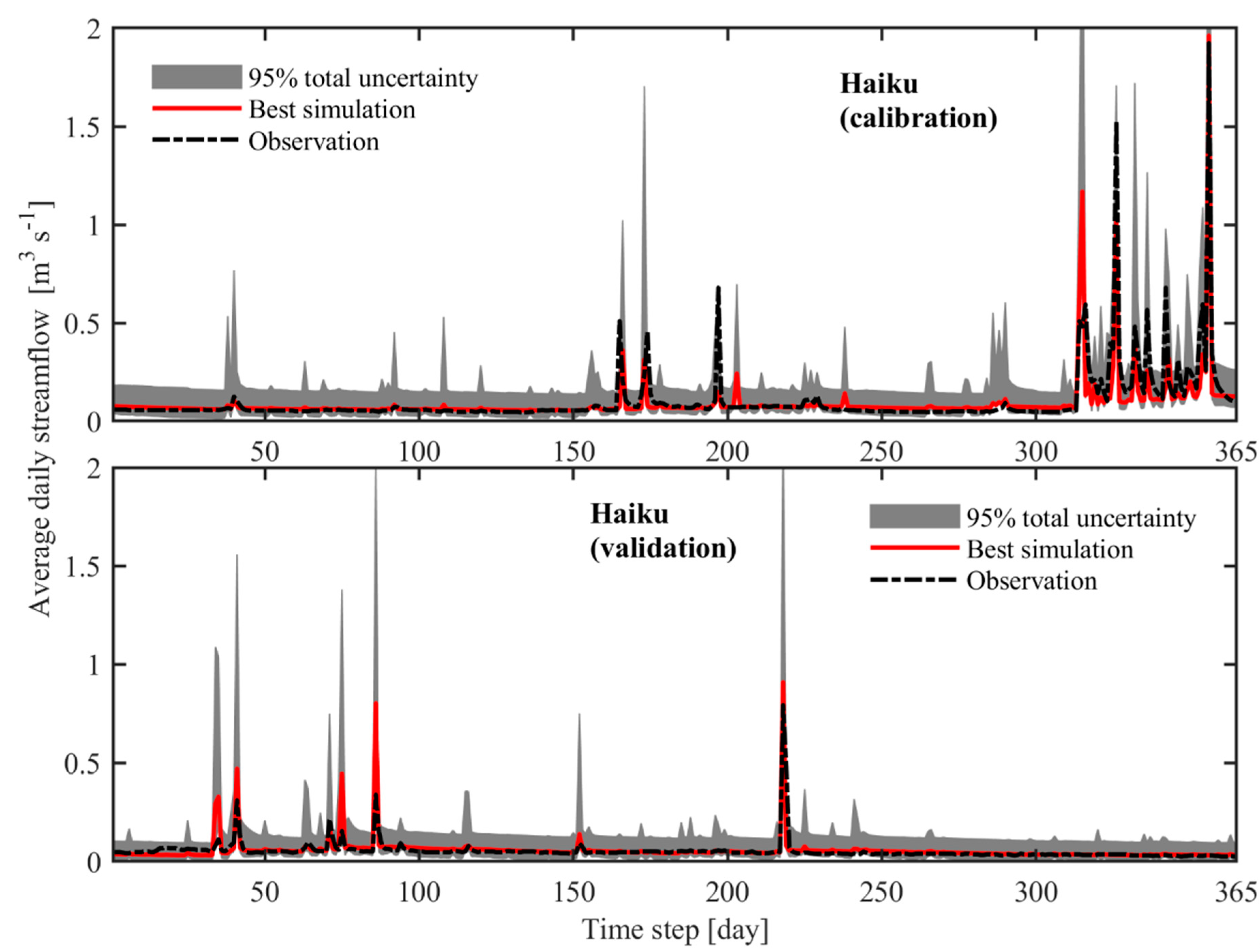
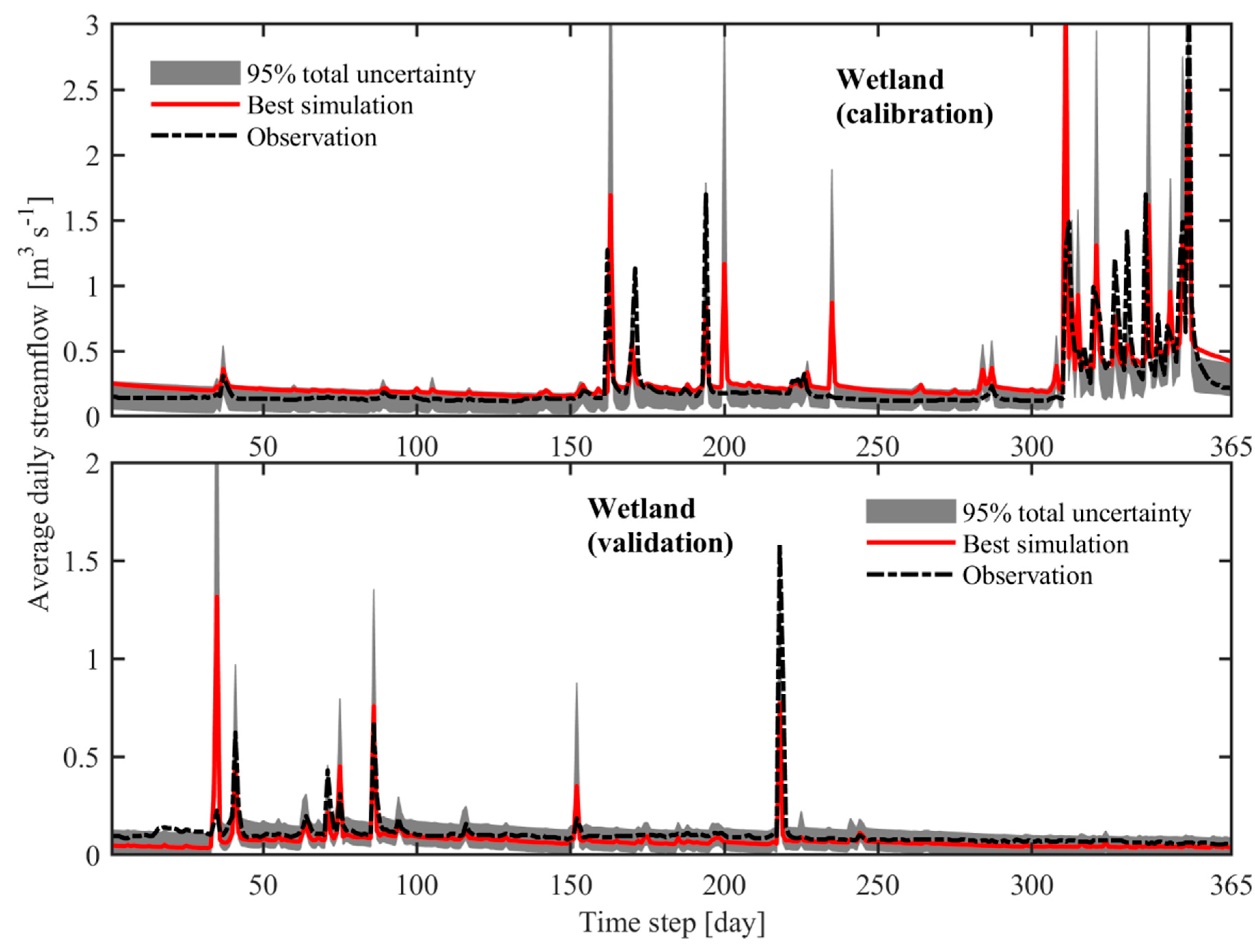
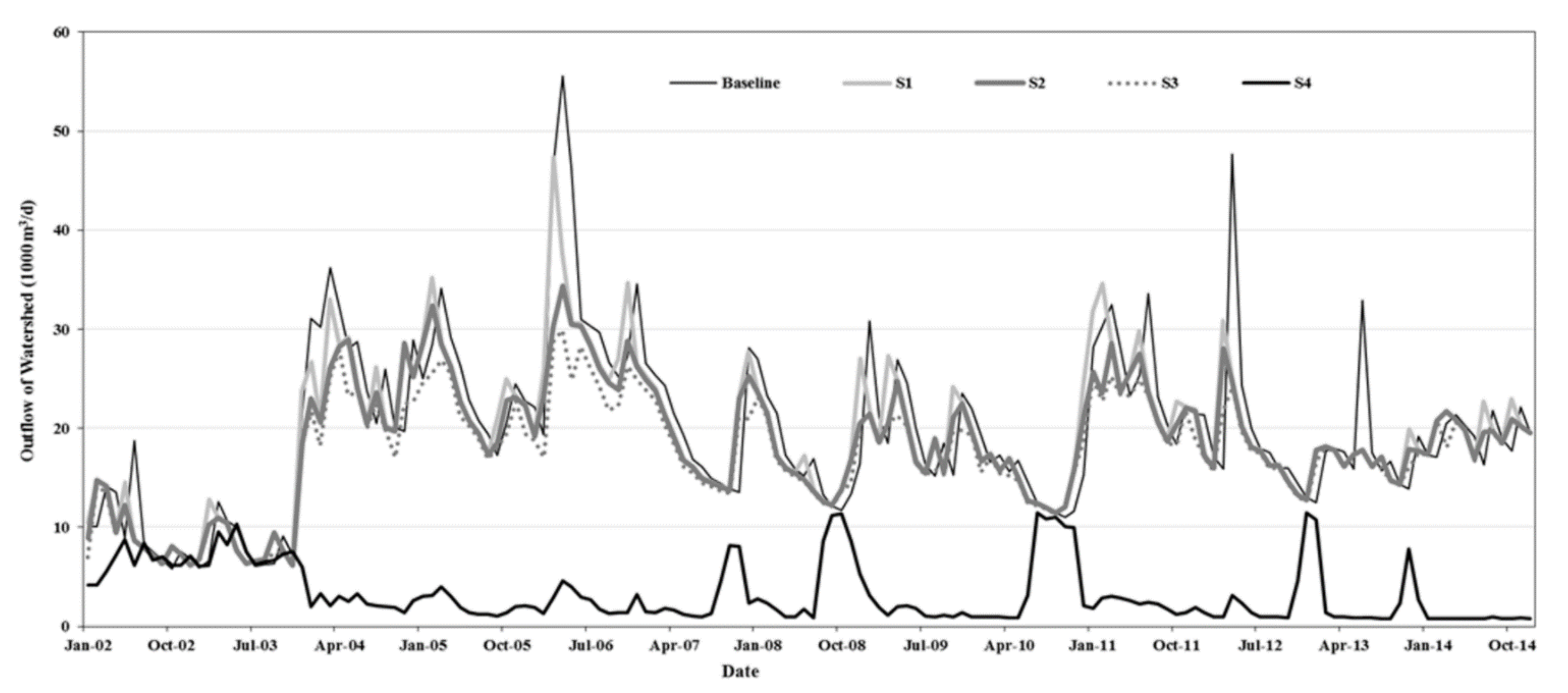
3.1. Daily Streamflow Simulation and Uncertainty Analysis
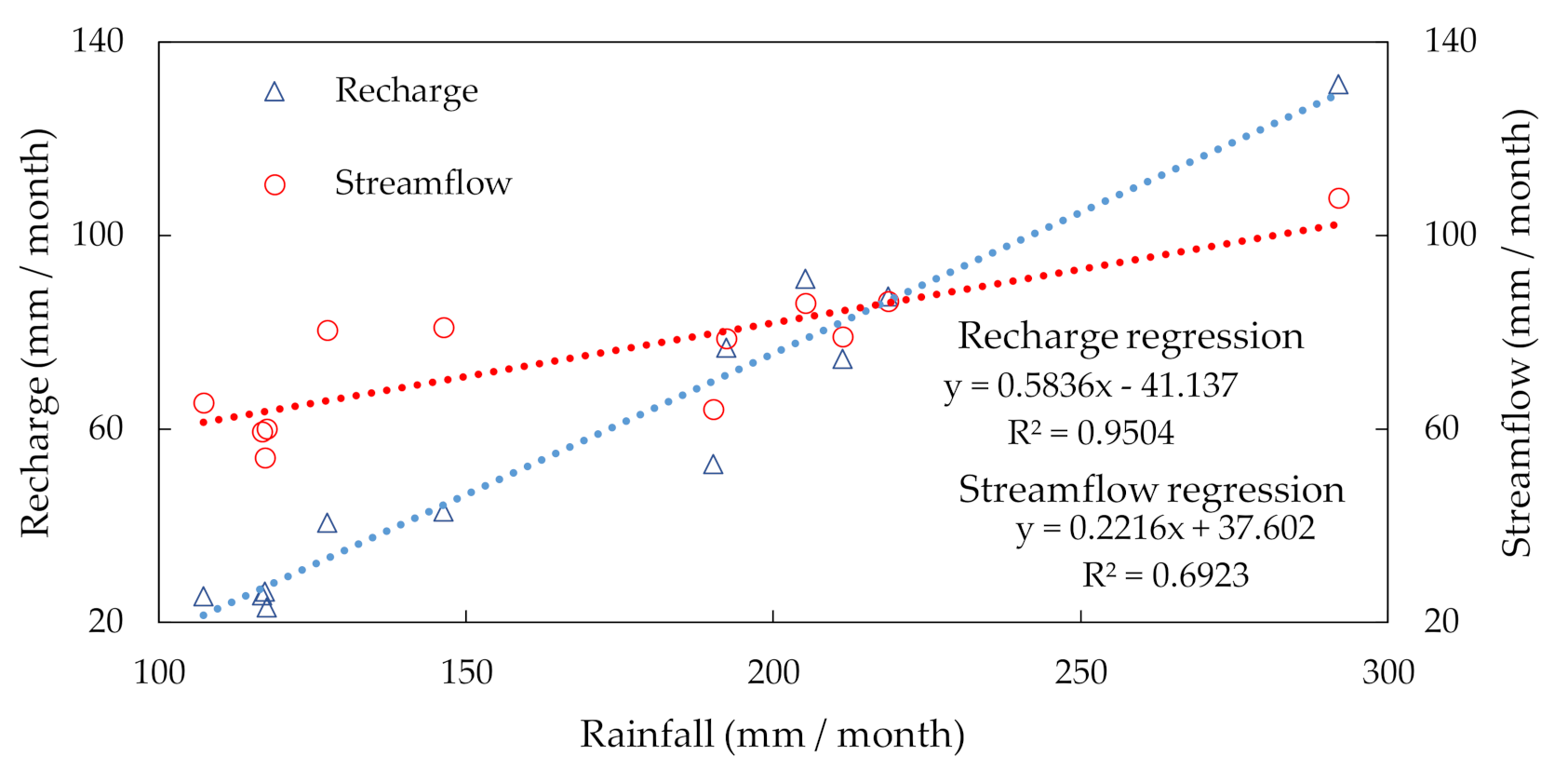
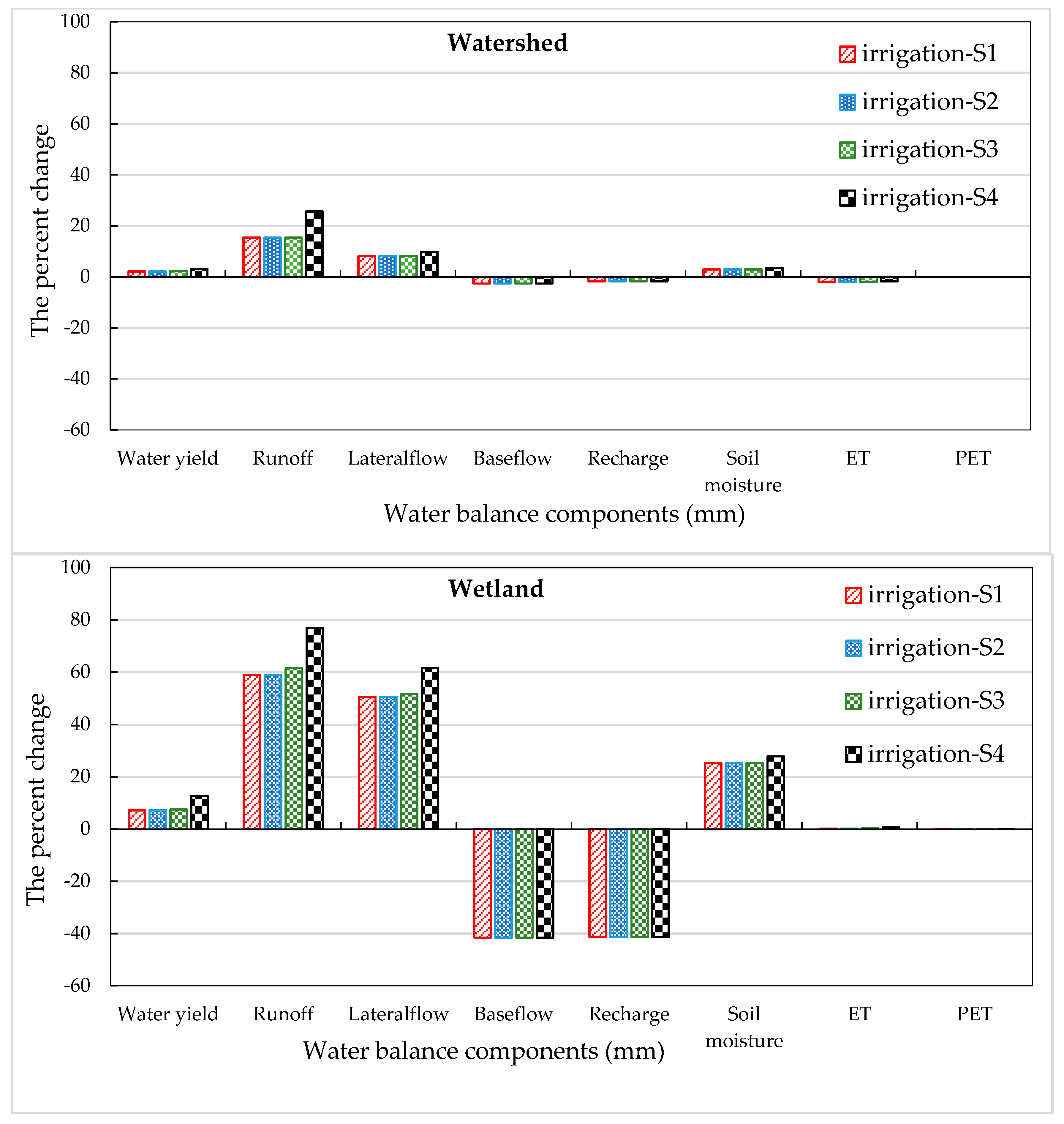
3.2. The Watershed Water Balance
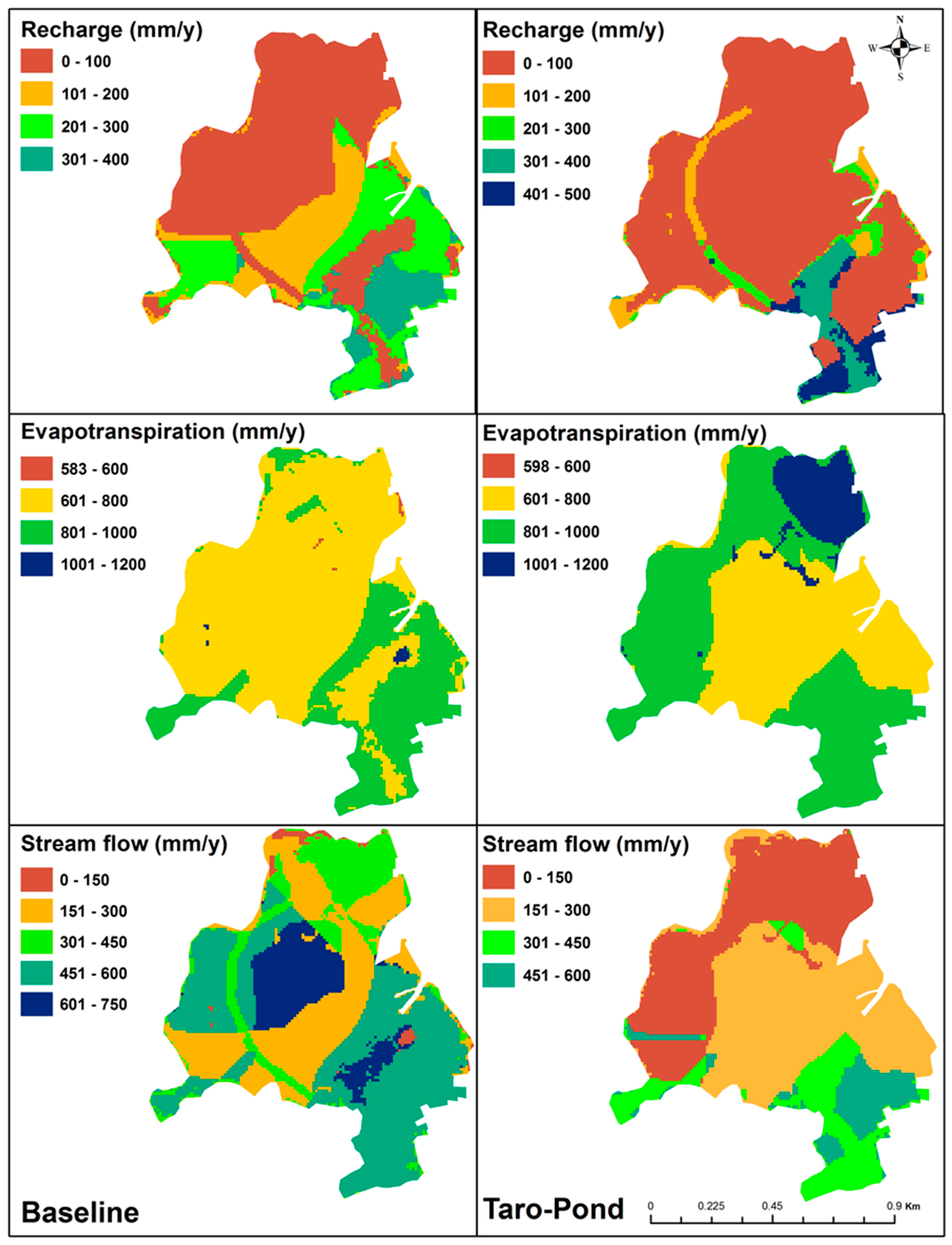
3.3. The Coastal Wetland Water Balance
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Mitsch, W.J.; Gosselink, J.G. Wetlands, 4th ed.; John Wiley & Sons, Inc.: New York, NY, USA, 2007. [Google Scholar]
- Bantilan-Smith, M.; Bruland, G.L.; MacKenzie, R.A.; Henry, A.R.; Ryder, C.R. A comparison of the vegetation and soils of natural, restored, and created coastal lowland wetlands in Hawaii. Wetlands 2009, 29, 1023–1035. [Google Scholar] [CrossRef]
- Bruland, G. Coastal wetlands: Function and role in reducing impact of land-based management. Coast. Watershed Manag. 2008, 13, 85. [Google Scholar]
- Henry, A.R. Strategic Plan for Wetland Conservation in Hawaii; Pacific Coast Joint Venture: Honolulu, HI, USA, 2006. [Google Scholar]
- Kailua Bay Advisory Council. Ko’olaupoko Watershed Restoration Action Strategy Kailua Bay Advisory Council (KBAC); Hawaii’s Department of Health: Honolulu, HI, USA, 2007. [Google Scholar]
- Jaradat, A. Agriculture in Iraq: Resources, potentials, constraints, and research needs and priorities. Food Agric. Environ. 2003, 1, 160–166. [Google Scholar]
- Oiwi, K. Heeia Wetlands Restoration, File Number: POH-2010-00159; Townscape, Inc: Käneÿohe, HI, USA, 2011. [Google Scholar]
- Oiwi, K. Heeia Wetland Restoration Strategic Plan 2010–2015; Käneÿohe, HI, USA, 2010; p. 16. [Google Scholar]
- Abu Hammad, A.; Tumeizi, A. Land degradation: Socioeconomic and environmental causes and consequences in the eastern Mediterranean. Land Degrad. Dev. 2012, 23, 216–226. [Google Scholar] [CrossRef]
- Shamrukh, M. Assessment of riverbank filtration for potable water supply in upper egypt. J. Eng. Sci. 2006, 34, 1175–1184. [Google Scholar]
- Ghodeif, K.; Paufler, S.; Grischek, T.; Wahaab, R.; Souaya, E.; Bakr, M.; Abogabal, A. Riverbank filtration in Cairo, Egypt—part I: Installation of a new riverbank filtration site and first monitoring results. Environ. Earth Sci. 2018, 77, 270. [Google Scholar] [CrossRef]
- Martin-Jézéquel, V.; Hildebrand, M.; Brzezinski, M.A. Silicon metabolism in diatoms: Implications for growth. J. Phycol. 2000, 36, 821–840. [Google Scholar] [CrossRef]
- Simpson, T.L.; Volcani, B.E. Silicon and Siliceous Structures in Biological Systems; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Bruijnzeel, L. Hydrological Impacts of Converting Tropical Montane Cloud Forest to Pasture, with Initial Reference to Northern Costa Rica; Final Technical Report DFID-FRP Project no. R7991; Vrije Universiteit: Amsterdam, The Netherlands, 2006. [Google Scholar]
- Schmidt, C.K.; Lange, F.; Brauch, H.; Kühn, W. Experiences with riverbank filtration and infiltration in Germany. DVGW-Water Technol. Cent. (TZW) Karlsr. Ger. 2003, 1–17. [Google Scholar]
- Arnold, J.G.; Srinivasan, R.; Muttiah, R.S.; Williams, J.R. Large area hydrologic modeling and assessment part I: Model development. AWRA J. Am. Water Resour. Assoc. 1998, 34, 73–89. [Google Scholar] [CrossRef]
- Hunter, C.L.; Evans, C.W. Coral reefs in Kaneohe Bay, Hawaii: Two centuries of western influence and two decades of data. Bull. Mar. Sci. 1995, 57, 501–515. [Google Scholar]
- Arnold, J.; Kiniry, J.; Srinivasan, R.; Williams, J.; Haney, E.; Neitsch, S. Soil and Water Assessment Tool Input/Output File Documentation: Version 2012; Technical Report; Texas Water Resources Institute: College Station, TX, USA, 2012. [Google Scholar]
- Gingerich, S.B.; Yeung, C.W.; Ibarra, T.-J.N.; Engott, J.A. Water Use in Wetland Kalo Cultivation in Hawaii; U. S. Geological Survey: Reston, VA, USA, 2007.
- Mat, N.; Hamzah, Z.; Maskin, M.; Wood, A.K. Mineral uptake by taro (colocasia esculenta) in swamp agroecosystem following gramoxone®(paraquat) herbicide spraying. J. Nucl. Relat. Technol. 2006, 3, 59–68. [Google Scholar]
- Onwueme, I. Taro cultivation in Asia and the Pacific. Rap Publ. 1999, 16, 1–9. [Google Scholar]
- Penn, D.C. Water and Energy Flows in Hawaii Taro Pondfields; University of Hawaii at Manoa: Honolulu, HI, USA, 1997. [Google Scholar]
- Nejadhashemi, A.P.; Shen, C.; Wardynski, B.J.; Mantha, P.S. Evaluating the impacts of land use changes on hydrologic responses in the agricultural regions of Michigan and Wisconsin. In Proceedings of the 2010 American Society of Agricultural and Biological Engineers, Pittsburgh, PA, USA, 20–23 June 2010; American Society of Agricultural and Biological Engineers: St. Joseph, MI, USA, 2010; p. 1. [Google Scholar]
- Pratiwi, S.H.; Soelistyono, R.; Maghfoer, M.D. The Growth and Yield of Taro (Colocasia. esculenta (L.) Schott) var. Antiquorum in Diverse. Sizes of Tuber and Numbers of Leaf. Int. J. Sci. Res. 2014, 3, 2289–2292. [Google Scholar]
- Shih, S.; Snyder, G. Leaf area index and dry biomass of taro. Agron. J. 1984, 76, 750–753. [Google Scholar] [CrossRef]
- Giambelluca, T.; Chen, Q.; Frazier, A.; Price, J.P.; Chen, Y.-L.; Chu, P.-S.; Eischeid, J.; Delparte, D. The Rainfall Atlas of Hawai‘i. Bull. Amer. Meteor. Soc 2013, 94, 313–316. [Google Scholar] [CrossRef]
- Leta, O.T.; El-Kadi, A.I.; Dulai, H.; Ghazal, K.A. Assessment of climate change impacts on water balance components of Heeia watershed in Hawaii. J. Hydrol. Reg. Stud. 2016, 8, 182–197. [Google Scholar] [CrossRef]
- Gassman, P.W.; Reyes, M.R.; Green, C.H.; Arnold, J.G. The soil and water assessment tool: Historical development, applications, and future research directions. Trans. ASABE 2007, 50, 1211–1250. [Google Scholar] [CrossRef]
- Gassman, P.W.; Sadeghi, A.M.; Srinivasan, R.J. Applications of the SWAT model special section: Overview and insights. J. Environ. Qual. 2014, 43, 1–8. [Google Scholar] [CrossRef] [PubMed]
- Neitsch, S.L.; Arnold, J.G.; Kiniry, J.R.; Williams, J.R. Soil and Water Assessment Tool Theoretical Documentation Version 2009; Texas Water Resources Institute: College Station, TX, USA, 2011. [Google Scholar]
- Leuning, R.; Zhang, Y.; Rajaud, A.; Cleugh, H.; Tu, K. A simple surface conductance model to estimate regional evaporation using MODIS leaf area index and the Penman-Monteith equation. Water Resour. Res. 2008, 44. [Google Scholar] [CrossRef]
- Bai, Z.; Dent, D.; Olsson, L.; Schaepman, M. Global Assessment of Land Degradation and Improvement: 1. Identification by Remote Sensing; ISRIC-World Soil Information: Wageningen, The Netherlands, 2008. [Google Scholar]
- CSO. Environmental Statistics Report of Iraq for 2009; Central Statistical Organisation: Baghdad, Iraq, 2010. [Google Scholar]
- King, K.W.; Arnold, J.; Bingner, R. Comparison of Green-Ampt and curve number methods on Goodwin Creek watershed using SWAT. Trans. ASAE 1999, 42, 919. [Google Scholar] [CrossRef]
- Abbaspour, K.C.; Yang, J.; Maximov, I.; Siber, R.; Bogner, K.; Mieleitner, J.; Zobrist, J.; Srinivasan, R. Modelling hydrology and water quality in the pre-alpine/alpine Thur watershed using SWAT. J. Hydrol. 2007, 333, 413–430. [Google Scholar] [CrossRef]
- Abbaspour, K.; Johnson, C.; Van Genuchten, M.T. Estimating uncertain flow and transport parameters using a sequential uncertainty fitting procedure. Vadose Zone J. 2004, 3, 1340–1352. [Google Scholar] [CrossRef]
- Abbaspour, K. SWAT-CUP 2012: SWAT Calibration and Uncertainty Programs—A User Manual; Swiss Federal Institute of Aquatic Science and Technology: Zurich, Switzerland, 2014. [Google Scholar]
- Dürr, H.; Meybeck, M.; Hartmann, J.; Laruelle, G.; Roubeix, V. Global spatial distribution of natural riverine silica inputs to the coastal zone. Biogeosciences 2011, 8, 597–620. [Google Scholar] [CrossRef]
- Pritchard, D. Wise use of wetlands. In Ramsar Handbooks for the Wise Use of Wetlands, 4th ed.; Ramsar Convention Secretariat: Gland, Switzerland, 2010. [Google Scholar]
- Nash, J.E.; Sutcliffe, J.V. River flow forecasting through conceptual models part I—A discussion of principles. J. Hydrol. 1970, 10, 282–290. [Google Scholar] [CrossRef]
- Sorooshian, S.; Duan, Q.; Gupta, V.K. Calibration of rainfall-runoff models: Application of global optimization to the Sacramento soil moisture accounting model. Water Resour. Res. 1993, 29, 1185–1194. [Google Scholar] [CrossRef]
- Allen, R.; Pruitt, W.; Businger, J.; Fritschen, L.; Jensen, M.; Quinn, F. Hydrology Handbook: ASCE Manuals and Reports on Engineering Practice, 2nd ed.; ASCE: Reston, VA, USA, 1996; pp. 125–252. [Google Scholar]
- Legates, D.R.; McCabe, G.J. Evaluating the use of “goodness-of-fit” measures in hydrologic and hydroclimatic model validation. Water Resour. Res. 1999, 35, 233–241. [Google Scholar] [CrossRef]
- Miyasaka, S.C.; Ogoshi, R.M.; Tsuji, G.Y.; Kodani, L.S. Site and planting date effects on taro growth. Agron. J. 2003, 95, 545–557. [Google Scholar]
- Struyf, E.; Conley, D.J. Silica: An essential nutrient in wetland biogeochemistry. Front. Ecol. Environ. 2009, 7, 88–94. [Google Scholar] [CrossRef]
- Allen, J.; Newman, M.E.; Riford, M.; Archer, G.H. Blood and plant residues on Hawaiian stone tools from two archaeological sites in Upland Kāne’ohe, Ko’olau Poko district, O’ahu Island. Asian Perspect. 1995, 34, 283–302. [Google Scholar]
- Arnold, J.; Moriasi, D.; Gassman, P.; Abbaspour, K.; White, M.; Srinivasan, R.; Santhi, C.; Harmel, R.; Van Griensven, A.; Van Liew, M. SWAT: Model use, calibration, and validation. Trans. ASABE 2012, 55, 1491–1508. [Google Scholar] [CrossRef]
- Shih, S.; Snyder, G. Leaf area index and evapotranspiration of Taro. Agron. J. 1985, 77, 554–556. [Google Scholar] [CrossRef]
- Evans, D. Taro, Mauka to Makai: A Taro Production and Business Guide for Hawaiian Growers; CTAHR University of Hawaii at Manoa: Honolulu, HI, USA, 2008. [Google Scholar]
- Xie, X.; Cui, Y. Development and test of SWAT for modeling hydrological processes in irrigation districts with paddy rice. J. Hydrol. 2011, 396, 61–71. [Google Scholar] [CrossRef]
- Moriasi, D.N.; Arnold, J.G.; Van Liew, M.W.; Bingner, R.L.; Harmel, R.D.; Veith, T.L. Model evaluation guidelines for systematic quantification of accuracy in watershed simulations. Trans. ASABE 2007, 50, 885–900. [Google Scholar] [CrossRef]
- Ndomba, P.; Mtalo, F.; Killingtveit, A. SWAT model application in a data scarce tropical complex catchment in Tanzania. Phys. Chem. Earth, Parts A/B/C 2008, 33, 626–632. [Google Scholar] [CrossRef]
- Khoi, D.N.; Thom, V.T. Parameter uncertainty analysis for simulating streamflow in a river catchment of Vietnam. Global Ecol. Conserv. 2015, 4, 538–548. [Google Scholar] [CrossRef]
- Pervez, M.S.; Henebry, G.M. Assessing the impacts of climate and land use and land cover change on the freshwater availability in the Brahmaputra River basin. J. Hydrol. Reg. Stud. 2015, 3, 285–311. [Google Scholar] [CrossRef]
- Ghazal, K.A.; Leta, O.; El-Kadi, A.; Dulai, H. Assessment of Wetland Restoration and Climate Change Impacts on Water Balance Components of the Heeia Coastal Wetland in Hawaii. Hydrology 2019, 6, 37–10. [Google Scholar] [CrossRef]
- Izuka, S.K.; Hill, B.R.; Shade, P.J.; Tribble, G.W. Geohydrology and Possible Transport Routes of Polychlorinated Biphenyls in Haiku Valley, Oahu, Hawaii; US Department of the Interior, US Geological Survey: Reston, VA, USA, 1993. [Google Scholar]
- Shade, P.J.; Nichols, W.D. Water Budget and the Effects of Land-Use Changes on Ground-Water Recharge, Oahu, Hawaii; US Government Printing Office: Washington, DC, USA, 1996.
- Takasaki, K.J.; Hirashima, G.T.; Lubke, E.R. Water Resources of Windward Oahu, Hawaii; US Government Printing Office: Washington, DC, USA, 1969.
- Osorio, J.; Jeong, J.; Bieger, K.; Arnold, J. Influence of potential evapotranspiration on the water balance of sugarcane fields in Maui, Hawaii. J. Water Resour. Prot. 2014, 6, 852. [Google Scholar] [CrossRef]


| Variable Name | Code and Values | Definition | Reference |
|---|---|---|---|
| ICNUM | 142 | Land cover/plant code | This study |
| CPNM | TARO | Four character code of land name | This study |
| IDC | 6 | Herbaceous perennial crop code | [18,44] |
| CROPNAME | Wetland Taro | Name of flooded Taro | This study |
| BIO_E | 47 | Radiation-use efficiency of Herbaceous | [18,44] |
| HVSTI | 0.01 | Harvest index for optimal growth | This study |
| BLAI | 2.5 | Maximum potential leaf area index (LAI) | [25,44] |
| FRGRW1 | 0.11 | Fraction of the plant growing season | [25,47] |
| LAIMX1 | 0.13 | Fraction of the maximum LAI (first point) | [18,48] |
| FRGRW2 | 0.24 | Fraction of the plant growing season | [18,25] |
| LAIMX2 | 0.91 | Fraction of the maximum LAI (second point) | [18,25] |
| DLAI | 0.89 | Fraction of growing season (decline leaf area) | [18,25] |
| CHTMX | 0.7 | Maximum canopy height (meter) | This study |
| RDMX | 0.6 | Maximum root depth (meter) | This study |
| TOPT | 25 | Optimal temperature for plant growth (°C) | This study |
| TBASE | 21 | Minimum temperature for plant growth (°C) | This study |
| Reach Number | S1 | S2 | S3 | S4 |
|---|---|---|---|---|
| 1 | 0.02 | 0.01 | 0.005 | 0.002 |
| 2 | 1.5 | 0.75 | 0.375 | 0.15 |
| 3 | 1.5 | 0.75 | 0.375 | 0.15 |
| 4 | 0.06 | 0.03 | 0.015 | 0.006 |
| 5 | 1.6 | 0.8 | 0.4 | 0.16 |
| 6 | 0.06 | 0.03 | 0.015 | 0.006 |
| 7 | 0.2 | 0.1 | 0.05 | 0.02 |
| 8 | 2 | 1 | 0.5 | 0.2 |
| Station | Period | Time Span | NSE | PBIAS (%) | RSR | r | P-Factor | R-Factor |
|---|---|---|---|---|---|---|---|---|
| Haiku | Calibration | 2002–2008 | 0.60 | 4.60 | 0.66 | 0.69 | 0.96 | 1.36 |
| Validation | 2009–2014 | 0.51 | 8.00 | 0.70 | 0.54 | 0.96 | 0.89 | |
| Wetland | Calibration | 2002–2008 | 0.51 | 13.00 | 0.63 | 0.67 | 0.81 | 0.81 |
| Validation | 2009–2014 | 0.50 | −2.59 | 0.67 | 0.50 | 0.95 | 0.67 |
| Scale | Scenario | Rainfall | Streamflow | Runoff | LF | BF | Recharge | Soil Moisture | ET | PET |
|---|---|---|---|---|---|---|---|---|---|---|
| Wetland | baseline | 1065 | 292 | 39 | 91 | 130 | 140 | 115 | 791 | 1533 |
| irrigation-S1 | 1065 | 313 | 62 | 137 | 76 | 82 | 144 | 792 | 1534 | |
| irrigation-S2 | 1065 | 313 | 62 | 137 | 76 | 82 | 144 | 792 | 1534 | |
| irrigation-S3 | 1065 | 314 | 63 | 138 | 76 | 82 | 144 | 793 | 1534 | |
| irrigation-S4 | 1065 | 329 | 69 | 147 | 76 | 82 | 147 | 796 | 1534 | |
| Watershed | baseline | 2043 | 904 | 119 | 306 | 459 | 699 | 171 | 916 | 1412 |
| irrigation-S1 | 2043 | 923 | 125 | 331 | 447 | 687 | 176 | 898 | 1412 | |
| irrigation-S2 | 2043 | 923 | 125 | 331 | 447 | 687 | 176 | 898 | 1412 | |
| irrigation-S3 | 2043 | 924 | 125 | 331 | 447 | 687 | 176 | 898 | 1412 | |
| irrigation-S4 | 2043 | 932 | 129 | 336 | 447 | 687 | 177 | 900 | 1412 |
| Month | Rainfall | WY | Runoff | LF | BF | Recharge | Soil Moisture | ET | PET |
|---|---|---|---|---|---|---|---|---|---|
| Jan | 192 | 79 | 10 | 31 | 35 | 77 | 179 | 61 | 91 |
| Feb | 205 | 86 | 19 | 31 | 34 | 91 | 176 | 63 | 95 |
| Mar | 292 | 108 | 23 | 43 | 40 | 131 | 179 | 83 | 109 |
| Apr | 127 | 80 | 5 | 31 | 42 | 41 | 148 | 96 | 124 |
| May | 146 | 81 | 10 | 25 | 44 | 43 | 126 | 95 | 129 |
| Jun | 107 | 66 | 4 | 18 | 42 | 25 | 104 | 89 | 142 |
| Jul | 118 | 60 | 2 | 15 | 41 | 23 | 101 | 82 | 145 |
| Aug | 117 | 60 | 3 | 16 | 39 | 26 | 100 | 75 | 144 |
| Sep | 117 | 54 | 3 | 14 | 36 | 27 | 104 | 69 | 130 |
| Oct | 190 | 64 | 8 | 19 | 36 | 53 | 135 | 70 | 115 |
| Nov | 211 | 79 | 15 | 29 | 34 | 75 | 155 | 69 | 98 |
| Dec | 219 | 86 | 16 | 33 | 36 | 88 | 171 | 62 | 89 |
| Scale | Scenario | Season | Rainfall | Streamflow | Runoff | LF | BF | Recharge | Soil Moisture | ET | PET |
|---|---|---|---|---|---|---|---|---|---|---|---|
| Wetland | irrigation-S1 | wet | 0 | 18.94 | 80.19 | 40.5 | −42.07 | −41.42 | 23.97 | −4.31 | −0.27 |
| dry | 0 | −12.22 | 13.18 | 84.99 | −41.37 | −43.07 | 57.46 | 5.53 | 0.26 | ||
| irrigation-S2 | wet | 0 | 19.22 | 80.95 | 40.78 | −42.07 | −41.42 | 24.01 | −4.29 | −0.27 | |
| dry | 0 | −12.17 | 13.32 | 85.22 | −41.37 | −43.07 | 57.49 | 5.54 | 0.26 | ||
| irrigation-S3 | wet | 0 | 19.86 | 82.64 | 41.46 | −42.07 | −41.42 | 24.13 | −4.26 | −0.27 | |
| dry | 0 | −11.94 | 13.79 | 86.15 | −41.37 | −43.07 | 57.6 | 5.57 | 0.26 | ||
| irrigation-S4 | wet | 0 | 25.7 | 95.58 | 48.8 | −42.07 | −41.42 | 25.24 | −3.94 | −0.27 | |
| dry | 0 | −5.62 | 35.46 | 108.34 | −41.37 | −43.07 | 59.95 | 6.21 | 0.26 | ||
| Watershed | irrigation-S1 | wet | 0 | 2.29 | 6.86 | 5.72 | −2.49 | −2.01 | 3.05 | −2.66 | −0.06 |
| dry | 0 | 1.93 | 1.85 | 12.3 | −2.69 | −0.98 | 5.1 | −1.36 | 0.05 | ||
| irrigation-S2 | wet | 0 | 2.32 | 6.93 | 5.74 | −2.49 | −2.01 | 3.05 | −2.66 | −0.06 | |
| dry | 0 | 1.93 | 1.59 | 12.32 | −2.69 | −0.98 | 5.11 | −1.36 | 0.05 | ||
| irrigation-S3 | wet | 0 | 2.39 | 7.16 | 5.83 | −2.49 | −2.01 | 3.09 | −2.65 | −0.06 | |
| dry | 0 | 1.95 | 1.66 | 12.37 | −2.96 | −0.98 | 5.13 | −1.35 | 0.05 | ||
| irrigation-S4 | wet | 0 | 3.29 | 9.51 | 7.14 | −2.94 | −2.01 | 3.53 | −2.5 | −0.06 | |
| dry | 0 | 2.77 | 5.25 | 14.3 | −2.69 | −0.98 | 5.73 | −1.12 | 0.05 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ghazal, K.A.; Leta, O.T.; El-Kadi, A.I.; Dulai, H. Impact of Coastal Wetland Restoration Plan on the Water Balance Components of Heeia Watershed, Hawaii. Hydrology 2020, 7, 86. https://doi.org/10.3390/hydrology7040086
Ghazal KA, Leta OT, El-Kadi AI, Dulai H. Impact of Coastal Wetland Restoration Plan on the Water Balance Components of Heeia Watershed, Hawaii. Hydrology. 2020; 7(4):86. https://doi.org/10.3390/hydrology7040086
Chicago/Turabian StyleGhazal, Kariem A., Olkeba Tolessa Leta, Aly I. El-Kadi, and Henrietta Dulai. 2020. "Impact of Coastal Wetland Restoration Plan on the Water Balance Components of Heeia Watershed, Hawaii" Hydrology 7, no. 4: 86. https://doi.org/10.3390/hydrology7040086
APA StyleGhazal, K. A., Leta, O. T., El-Kadi, A. I., & Dulai, H. (2020). Impact of Coastal Wetland Restoration Plan on the Water Balance Components of Heeia Watershed, Hawaii. Hydrology, 7(4), 86. https://doi.org/10.3390/hydrology7040086







