Partial Replacement of Carbon Black with Graphene in Tire Compounds: Transport Properties, Thermal Stability and Dynamic Mechanical Analysis
Abstract
1. Introduction
2. Experimental
2.1. Materials and Compound Formulations
2.2. Transport Characteristics
Swelling Studies
- m0 = initial mass of the vulcanizate samples
- m1 = mass of the swollen samples.
- Vr = volume fraction of swollen rubber
- m1 = mass of the swollen sample
- m2 = final dried mass of the sample
- mf = mass of filler
- ρp = density of the polymer
- ρs = density of solvent toluene (0.866 g/cm3)
- Mc = molecular weight between crosslinks
- Vs = molar volume of toluene (106.4 cm3/mol)
2.3. Equilibrium Sorption Experiments
2.4. Dynamic Mechanical Properties
2.5. Thermogravimetric Analysis (TGA)
3. Results and Discussion
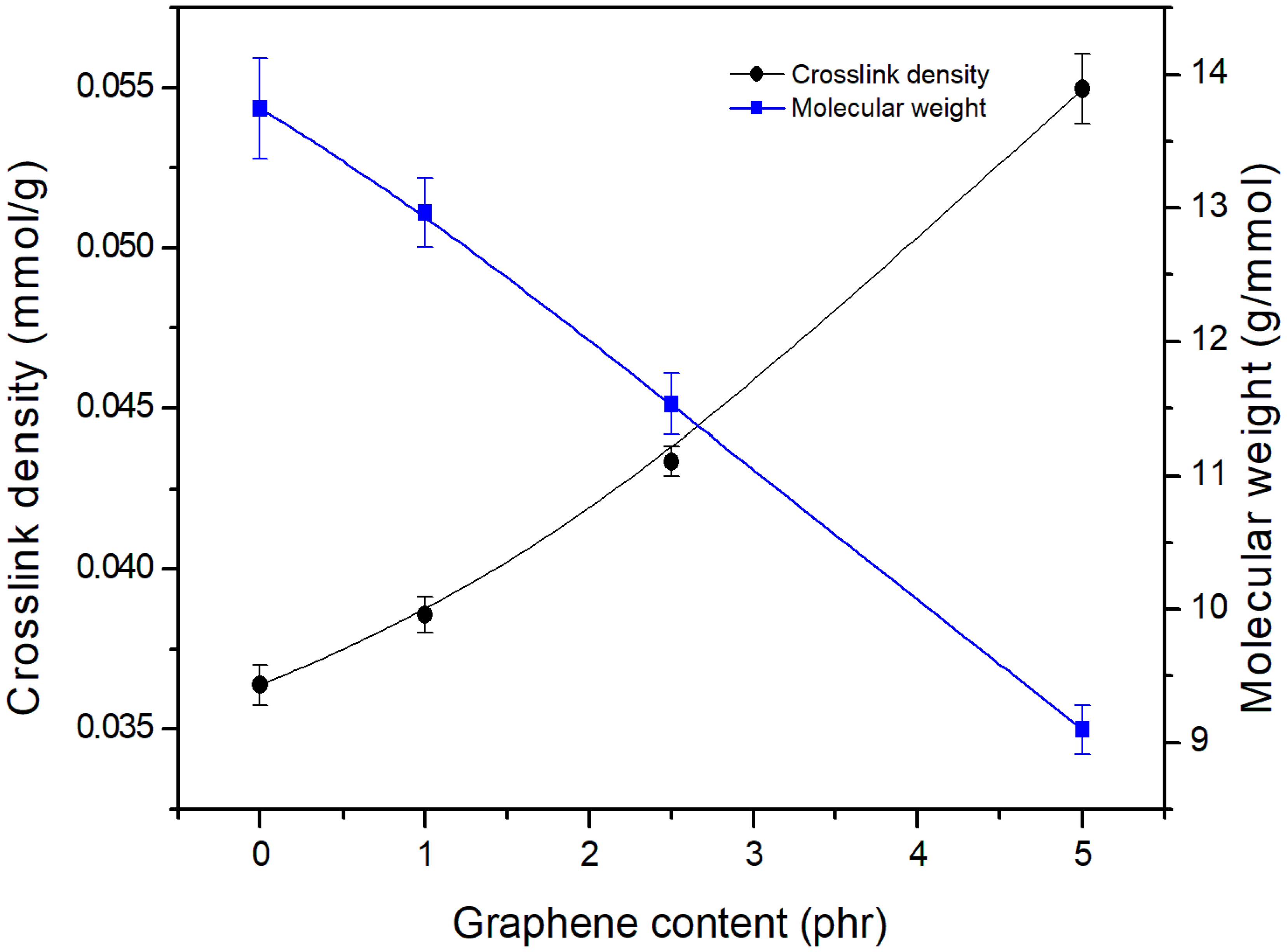
3.1. Swelling Studies
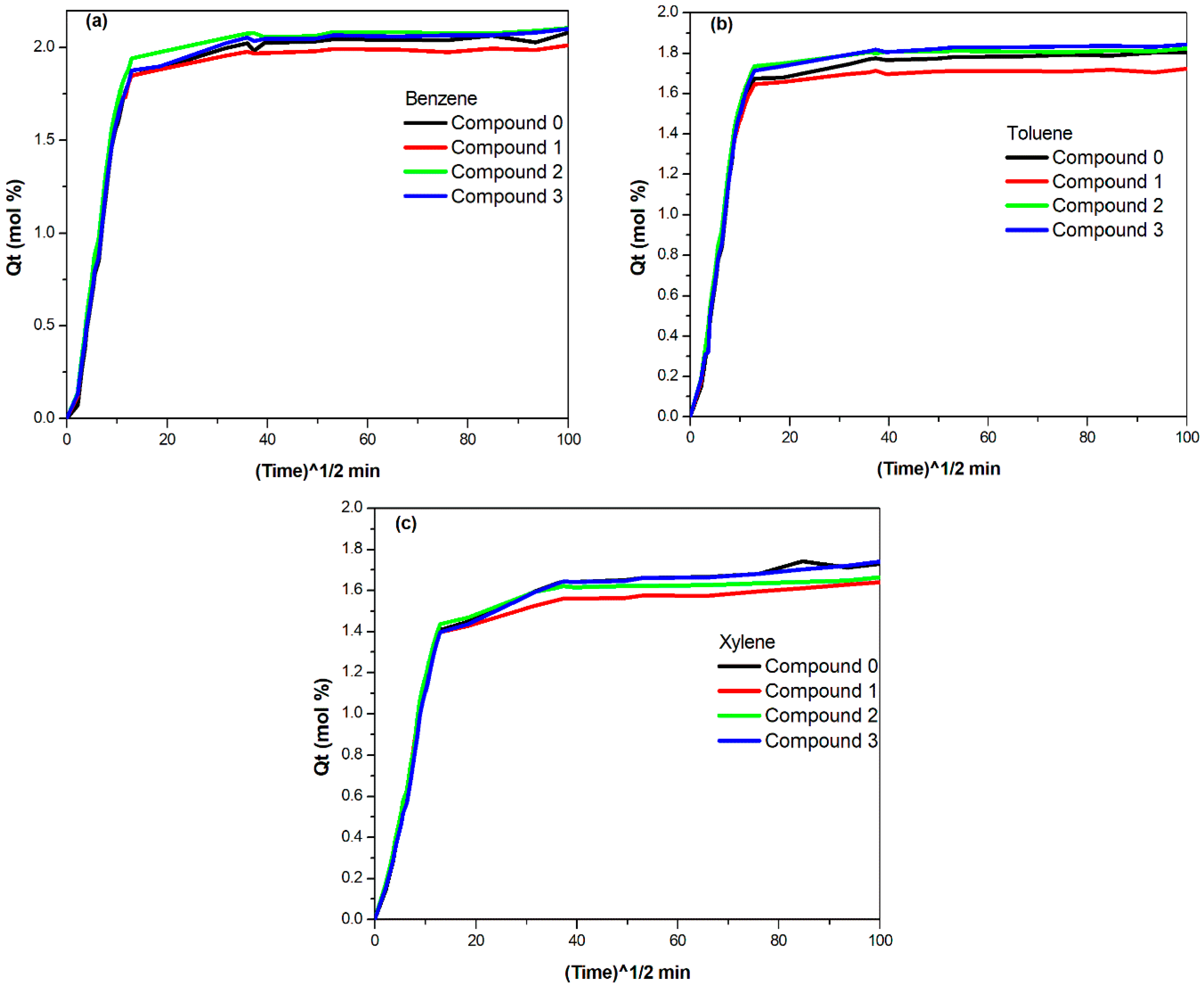
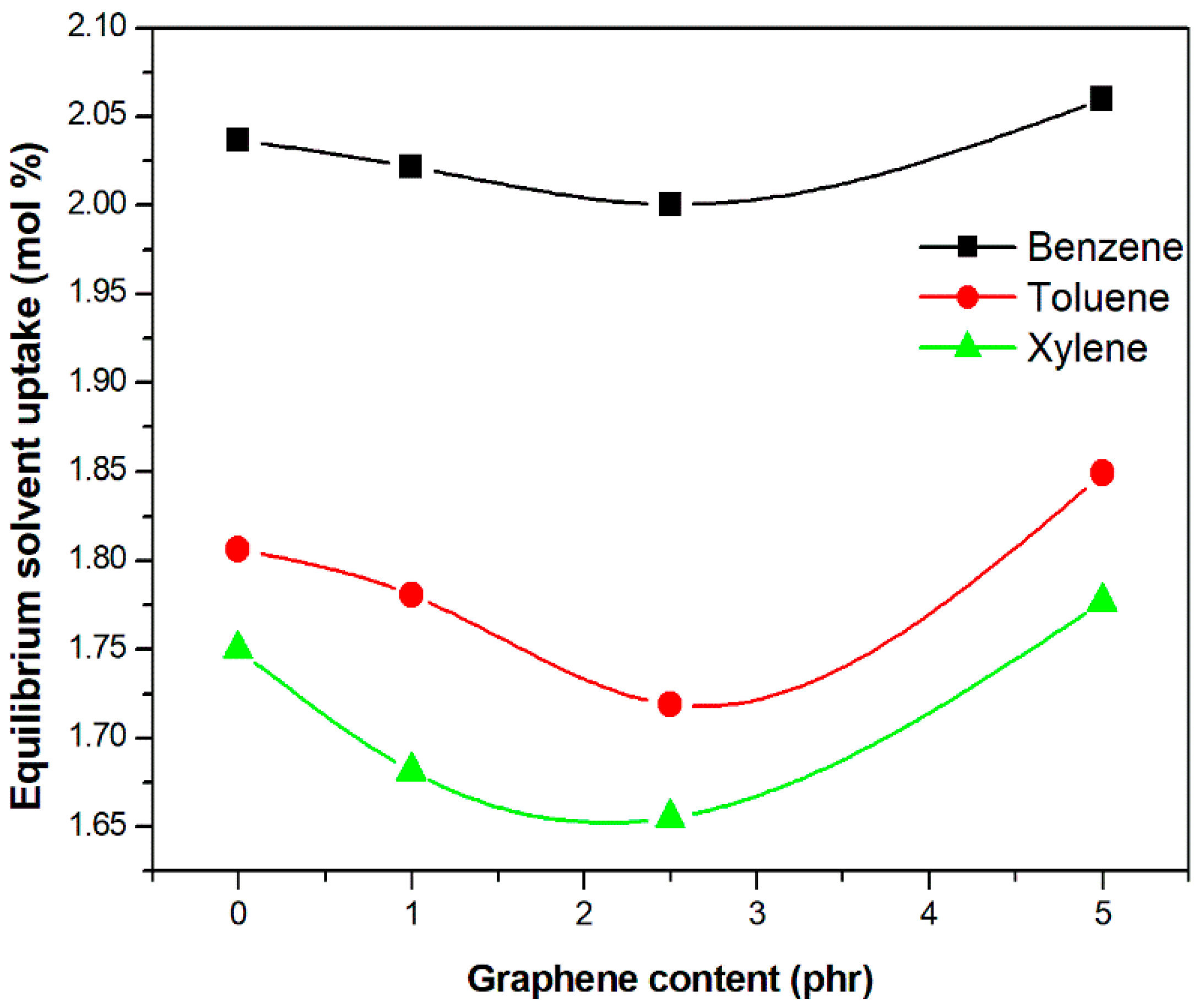
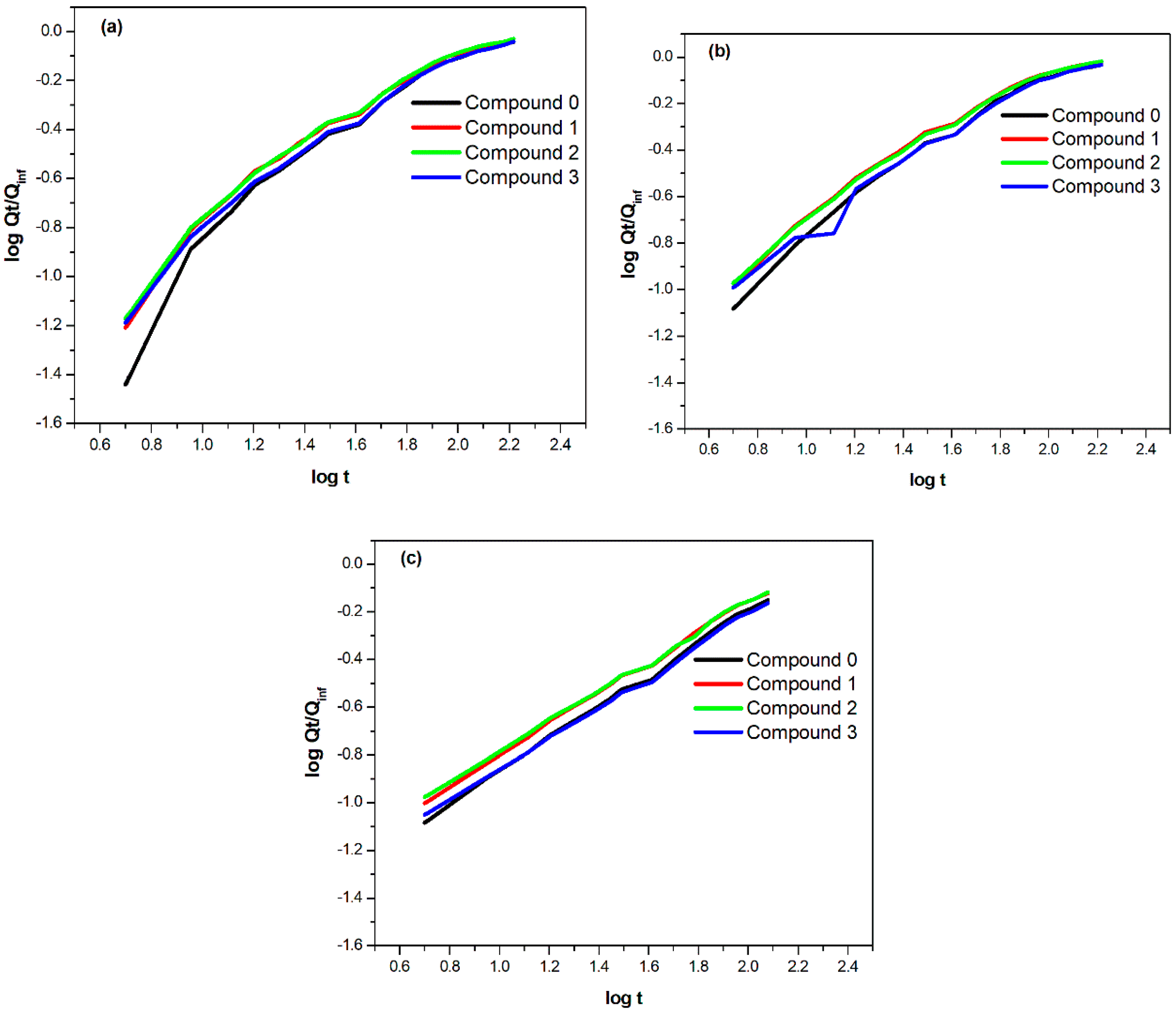
3.2. Sorption Characteristics
- h = thickness of the specimen
- θ = slope of the initial linear portion of sorption curves
- Q∞ = equilibrium solvent uptake
- M∞ = mass of solvent uptake at equilibrium
- M0 = initial mass of the sample
- D = diffusivity
- S = solubility.
3.3. Dynamic Mechanical Analysis (DMA)
3.4. Thermogravimetric Analysis (TGA)
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Thomas, J.; Patil, R. The Road to Sustainable Tire Materials: Current State-of-the-Art and Future Prospectives. Environ. Sci. Technol. 2023, 57, 2209–2216. [Google Scholar] [CrossRef] [PubMed]
- Railsback, H.E.; Cooper, W.T.; Stumpe, N.A. Cis-Polybutadiene-Natural Rubber Blends. Rubber Chem. Technol. 1959, 32, 308–320. [Google Scholar] [CrossRef]
- Kaliyathan, A.V.; Rane, A.V.; Huskic, M.; Kunaver, M.; Kalarikkal, N.; Rouxel, D.; Thomas, S. Carbon Black Distribution in Natural Rubber/Butadiene Rubber Blend Composites: Distribution Driven by Morphology. Compos. Sci. Technol. 2020, 200, 108484. [Google Scholar] [CrossRef]
- Hess, W.M.; Vegvari, P.C.; Swor, R.A. Carbon Black in NR/BR Blends for Truck Tires. Rubber Chem. Technol. 1985, 58, 350–382. [Google Scholar] [CrossRef]
- Polgar, L.M.; Van Essen, M.; Pucci, A.; Picchioni, F. Smart Rubbers: Synthesis and Applications; Walter de Gruyter GmbH & Co. KG: Berlin, Germany, 2019; ISBN 3110639017. [Google Scholar]
- Parameswaran, S.K.; Bhattacharya, S.; Mukhopadhyay, R.; Naskar, K.; Bhowmick, A.K. Excavating the Unique Synergism of Nanofibers and Carbon Black in Natural Rubber Based Tire Tread Composition. J. Appl. Polym. Sci. 2021, 138, 49682. [Google Scholar] [CrossRef]
- Poikelispää, M.; Das, A.; Dierkes, W.; Vuorinen, J. The Effect of Partial Replacement of Carbon Black by Carbon Nanotubes on the Properties of Natural Rubber/Butadiene Rubber Compound. J. Appl. Polym. Sci. 2013, 130, 3153–3160. [Google Scholar] [CrossRef]
- Poikelispää, M.; Shakun, A.; Sarlin, E. Nanodiamond—Carbon Black Hybrid Filler System for Demanding Applications of Natural Rubber—Butadiene Rubber Composite. Appl. Sci. 2021, 11, 10085. [Google Scholar] [CrossRef]
- Ghosh, B.; Paul, S.; Kar, S.; Ghosal, R.; Roy, A.; Mondal, T.; Bhowmick, A.K. Graphene-Based Hybrid Fillers as New Reinforcing Agents in Rubber Compounds for the Tire Industry. In Graphene-Rubber Nanocomposites; CRC Press: Boca Raton, FL, USA, 2022; pp. 199–223. [Google Scholar]
- Tabandeh-Khorshid, M.; Kumar, A.; Omrani, E.; Kim, C.; Rohatgi, P. Synthesis, Characterization, and Properties of Graphene Reinforced Metal-Matrix Nanocomposites. Compos. Part B Eng. 2020, 183, 107664. [Google Scholar] [CrossRef]
- Innes, J.R.; Young, R.J.; Papageorgiou, D.G. Graphene Nanoplatelets as a Replacement for Carbon Black in Rubber Compounds. Polymers 2022, 14, 1204. [Google Scholar] [CrossRef] [PubMed]
- Sethulekshmi, A.S.; Jayan, J.S.; Saritha, A.; Joseph, K. Recent Developments in Natural Rubber Nanocomposites Containing Graphene Derivatives and Its Hybrids. Ind. Crops Prod. 2022, 177, 114529. [Google Scholar] [CrossRef]
- Marinho, B.; Ghislandi, M.; Tkalya, E.; Koning, C.E.; de With, G. Electrical Conductivity of Compacts of Graphene, Multi-Wall Carbon Nanotubes, Carbon Black, and Graphite Powder. Powder Technol. 2012, 221, 351–358. [Google Scholar] [CrossRef]
- Ke, K.; Sang, Z.; Chen, X.; Rohm, K.; Manas-Zloczower, I. Tuning Mechanical and Electrical Properties of Elastomer Composites with Hybrid Filler Network Containing Graphene for Stretchable Strain Sensors. Adv. Eng. Mater. 2022, 24, 2100703. [Google Scholar] [CrossRef]
- Leong, Y.L.; Lim, H.N.; Ibrahim, I. Graphene in Rubber Formulations: A Comprehensive Review and Performance Optimization Insights. Mol. Syst. Des. Eng. 2023, 8, 1229–1251. [Google Scholar] [CrossRef]
- Wang, J.; Li, S.; Yang, L.; Liu, B.; Xie, S.; Qi, R.; Zhan, Y.; Xia, H. Graphene-Based Hybrid Fillers for Rubber Composites. Molecules 2024, 29, 1009. [Google Scholar] [CrossRef] [PubMed]
- Capezza, A.; Andersson, R.L.; Ström, V.; Wu, Q.; Sacchi, B.; Farris, S.; Hedenqvist, M.S.; Olsson, R.T. Preparation and Comparison of Reduced Graphene Oxide and Carbon Nanotubes as Fillers in Conductive Natural Rubber for Flexible Electronics. ACS Omega 2019, 4, 3458–3468. [Google Scholar] [CrossRef] [PubMed]
- Valentini, L.; Bon, S.B.; López-Manchado, M.A.; Verdejo, R.; Pappalardo, L.; Bolognini, A.; Alvino, A.; Borsini, S.; Berardo, A.; Pugno, N.M. Synergistic Effect of Graphene Nanoplatelets and Carbon Black in Multifunctional EPDM Nanocomposites. Compos. Sci. Technol. 2016, 128, 123–130. [Google Scholar] [CrossRef]
- Song, P.; Wang, G.; Zhang, Y. Preparation and Performance of Graphene/Carbon Black Silicone Rubber Composites Used for Highly Sensitive and Flexible Strain Sensors. Sens. Actuators A Phys. 2021, 323, 112659. [Google Scholar] [CrossRef]
- Khalifa, M.; Murugesan, S.; Anandhan, S. Graphene-Based Elastomer Nanocomposites: A Fascinating Material for Flexible Sensors in Health Monitoring. In Graphene-Rubber Nanocomposites; CRC Press: Boca Raton, FL, USA, 2022; pp. 347–375. [Google Scholar]
- Yin, F.; Niu, H.; Kim, E.; Shin, Y.K.; Li, Y.; Kim, N. Advanced Polymer Materials-based Electronic Skins for Tactile and Non-contact Sensing Applications. InfoMat 2023, 5, e12424. [Google Scholar] [CrossRef]
- Abbasi, S.; Peerzada, M.H.; Nizamuddin, S.; Mubarak, N.M. Functionalized Nanomaterials for the Aerospace, Vehicle, and Sports Industries. In Handbook of Functionalized Nanomaterials for Industrial Applications; Elsevier: Amsterdam, The Netherlands, 2020; pp. 795–825. [Google Scholar]
- Mirizzi, L.; Carnevale, M.; D’Arienzo, M.; Milanese, C.; Di Credico, B.; Mostoni, S.; Scotti, R. Tailoring the Thermal Conductivity of Rubber Nanocomposites by Inorganic Systems: Opportunities and Challenges for Their Application in Tires Formulation. Molecules 2021, 26, 3555. [Google Scholar] [CrossRef] [PubMed]
- Jayalakshmy, M.S.; Mishra, R.K. Applications of Carbon-Based Nanofiller-Incorporated Rubber Composites in the Fields of Tire Engineering, Flexible Electronics and EMI Shielding. In Carbon-Based Nanofillers and Their Rubber Nanocomposites; Elsevier: Amsterdam, The Netherlands, 2019; pp. 441–472. [Google Scholar]
- Mondal, S.; Khastgir, D. Elastomer Reinforcement by Graphene Nanoplatelets and Synergistic Improvements of Electrical and Mechanical Properties of Composites by Hybrid Nano Fillers of Graphene-Carbon Black & Graphene-MWCNT. Compos. Part A Appl. Sci. Manuf. 2017, 102, 154–165. [Google Scholar]
- Debnath, M.; Adhikary, A. Use of Graphene in Rubber Nanocomposite, Its Processability, and Commercial Advantages. In Rubber Products: Technology and Cost Optimisation; Walter de Gruyter GmbH & Co. KG: Berlin, Germany, 2024; p. 151. [Google Scholar]
- Battig, A.; Fadul, N.A.-R.; Frasca, D.; Schulze, D.; Schartel, B. Multifunctional Graphene Nanofiller in Flame Retarded Polybutadiene/Chloroprene/Carbon Black Composites. e-Polymers 2021, 21, 244–262. [Google Scholar] [CrossRef]
- Roy, A.; Kar, S.; Ghosal, R.; Mukhopadhyay, R.; Naskar, K.; Bhowmick, A.K. Unique Graphene-carbon Black Hybrid Nanofiller by a Micromechanical Cleavage Technique as a Reinforcing Agent in Elastomers: Fundamental and Experimental Studies. J. Appl. Polym. Sci. 2023, 140, e53575. [Google Scholar] [CrossRef]
- Döscher, H.; Schmaltz, T.; Neef, C.; Thielmann, A.; Reiss, T. Graphene Roadmap Briefs (No. 2): Industrialization Status and Prospects 2020. 2D Mater. 2021, 8, 22005. [Google Scholar] [CrossRef]
- Mathew, M.; Midhun Dominic, C.D.; Neenu, K.V.; Begum, P.M.S.; Dileep, P.; Kumar, T.G.A.; Sabu, A.A.; Nagane, D.; Parameswaranpillai, J.; Badawi, M. Carbon Black and Chitin Nanofibers for Green Tyres: Preparation and Property Evaluation. Carbohydr. Polym. 2023, 310, 120700. [Google Scholar] [CrossRef]
- Shiva, M.; Akhtari, S.S.; Shayesteh, M. Effect of Mineral Fillers on Physico-Mechanical Properties and Heat Conductivity of Carbon Black-Filled SBR/Butadiene Rubber Composite. Iran. Polym. J. 2020, 29, 957–974. [Google Scholar] [CrossRef]
- Dominic, M.; Joseph, R.; Begum, P.M.S.; Kanoth, B.P.; Chandra, J.; Thomas, S. Green Tire Technology: Effect of Rice Husk Derived Nanocellulose (RHNC) in Replacing Carbon Black (CB) in Natural Rubber (NR) Compounding. Carbohydr. Polym. 2020, 230, 115620. [Google Scholar] [CrossRef] [PubMed]
- Jung, J.; Sodano, H.A. Aramid Nanofiber Reinforced Rubber Compounds for the Application of Tire Tread with High Abrasion Resistance and Fuel Saving Efficiency. ACS Appl. Polym. Mater. 2020, 2, 4874–4884. [Google Scholar] [CrossRef]
- Mohamad Aini, N.A.; Othman, N.; Hussin, M.H.; Sahakaro, K.; Hayeemasae, N. Effect of Hybrid Carbon Black/Lignin on Rheological, Mechanical and Thermal Stability Properties of NR/BR Composites. Plast. Rubber Compos. 2022, 51, 293–305. [Google Scholar] [CrossRef]
- Ercan Kalkan, M.; Karakaya, N.; Özkoç, G. POSS Nanoparticles as a Potential Compatibilizer for Natural Rubber/Butadiene Rubber Blends. Polym. Adv. Technol. 2020, 31, 2290–2300. [Google Scholar] [CrossRef]
- Sreelekshmi, R.V.; Sudha, J.D.; Menon, A.R.R. Novel Organomodified Kaolin/Silica Hybrid Fillers in Natural Rubber and Its Blend with Polybutadiene Rubber. Polym. Bull. 2017, 74, 783–801. [Google Scholar] [CrossRef]
- Nayeeif, A.A.; Abed, M.S.; Abdulkhalik, H. Morphology, rheology and mechanical properties of NR, NR/BR and NR nanocomposite. J. Eng. Sci. Technol. 2022, 17, 3512–3525. [Google Scholar]
- Ma, L.; Zhang, Z.; Peng, Z.; Formela, K.; Wang, S. Dynamic Mechanical Properties and Flexing Fatigue Resistance of Tire Sidewall Rubber as Function of Waste Tire Rubber Reclaiming Degree. J. Appl. Polym. Sci. 2021, 138, 51290. [Google Scholar] [CrossRef]
- Kaliyathan, A.V.; Rane, A.V.; Jackson, S.; Thomas, S. Analysis of Diffusion Characteristics for Aromatic Solvents through Carbon Black Filled Natural Rubber/Butadiene Rubber Blends. Polym. Compos. 2021, 42, 375–396. [Google Scholar] [CrossRef]
- Rajan, K.P.; Gopanna, A.; Theravalappil, R.; Abdelghani, E.A.M.; Thomas, S.P. Partial Replacement of Carbon Black with Graphene in Natural Rubber/Butadiene Rubber Based Tire Compound: Investigation of Critical Properties. J. Polym. Res. 2022, 29, 1–17. [Google Scholar] [CrossRef]
- Flory, P.J.; Rehner, J., Jr. Statistical Mechanics of Cross-linked Polymer Networks I. Rubberlike Elasticity. J. Chem. Phys. 1943, 11, 512–520. [Google Scholar] [CrossRef]
- Hergenrother, W.L.; Hilton, A.S. Use of χ as a Function of Volume Fraction of Rubber to Determine Crosslink Density by Swelling. Rubber Chem. Technol. 2003, 76, 832–845. [Google Scholar] [CrossRef]
- Moon, B.; Lee, J.; Park, S.; Seok, C.-S. Study on the Aging Behavior of Natural Rubber/Butadiene Rubber (NR/BR) Blends Using a Parallel Spring Model. Polymers 2018, 10, 658. [Google Scholar] [CrossRef] [PubMed]
- Joseph, R.; George, K.E.; Francls, D.J.; Thomas, K.T. Polymer-Solvent Interaction Parameter for NR/SBR and NR/BR Blends. Int. J. Polym. Mater. 1987, 12, 29–34. [Google Scholar] [CrossRef]
- Farsouni Eydi, E.; Shariati, A.; Khosravi-Nikou, M.R. Separation of BTEX Compounds (Benzene, Toluene, Ethylbenzene and Xylenes) from Aqueous Solutions Using Adsorption Process. J. Dispers. Sci. Technol. 2019, 40, 453–463. [Google Scholar] [CrossRef]
- Al-Kandary, J.A.; Al-Jimaz, A.S.; Abdul-Latif, A.-H.M. Viscosities, Densities, and Speeds of Sound of Binary Mixtures of Benzene, Toluene, o-Xylene, m-Xylene, p-Xylene, and Mesitylene with Anisole at (288.15, 293.15, 298.15, and 303.15) K. J. Chem. Eng. Data 2006, 51, 2074–2082. [Google Scholar] [CrossRef]
- Niziolek, A.M.; Onel, O.; Floudas, C.A. Production of Benzene, Toluene, and Xylenes from Natural Gas via Methanol: Process Synthesis and Global Optimization. AIChE J. 2016, 62, 1531–1556. [Google Scholar] [CrossRef]
- Mathai, A.E.; Thomas, S. Transport of Aromatic Hydrocarbons through Crosslinked Nitrile Rubber Membranes. J. Macromol. Sci. Part B Phys. 1996, 35, 229–253. [Google Scholar] [CrossRef]
- Augustine, A.; Joseph, J.; Thomas, S.P.; Stephen, R. Effect of Halloysite Nanotubes and Organically Modified Bentonite Clay Hybrid Filler System on the Properties of Natural Rubber. J. Elastomers Plast. 2019, 0095244319865573. [Google Scholar]
- Bijina, V.; Jandas, P.J.; Jose, J.; Abhitha, K.; John, H. Tailoring of Performance Characteristics of Green Tyre Tread Formulation Using Thermally Exfoliated Graphite/Carbon Black Binary Filler System via Scalable and Competent Method. Res. Sq. 2022. [Google Scholar] [CrossRef]
- Thomas, P.C.; Tomlal Jose, E.; Selvin Thomas, P.; Thomas, S.; Joseph, K. High-performance Nanocomposites Based on Arcylonitrile-butadiene Rubber with Fillers of Different Particle Size: Mechanical and Morphological Studies. Polym. Compos. 2010, 31, 1515–1524. [Google Scholar] [CrossRef]
- Balachandran, M.; Bhagawan, S.S. Mechanical, Thermal and Transport Properties of Nitrile Rubber (NBR)—Nanoclay Composites. J. Polym. Res. 2012, 19, 9809. [Google Scholar] [CrossRef]
- Igwe, I.O.; Ezeani, O.E. Studies on the Transport of Aromatic Solvents through Filled Natural Rubber. Int. J. Polym. Sci. 2012, 2012, 212507. [Google Scholar] [CrossRef]
- Nair, M.V.; Kumar, A.; Joseph, S.; Zachariah, A.K.; Maria, H.J.; Thomas, S. Synergistic Effect of Hybrid Fillers on Transport Behavior of NR/EPDM Blends. J. Integr. Sci. Technol. 2021, 9, 9–21. [Google Scholar]
- Abhisha, V.S.; Sisanth, K.S.; Thomas, S.; Stephen, R. Kinetic Studies on the Transport Behavior of Hybrid Filler Incorporated Natural Rubber (NR). Express Polym. Lett. 2023, 17, 1070. [Google Scholar] [CrossRef]
- Ravindran, A.; Kamaraj, M.; Vasanthmurali, N.; Meghavarshini, V.; Balachandran, M. Nanosilica Reinforced EPDM Silicone Rubber Blends: Experimental and Theoretical Evaluation of Mechanical and Solvent Sorption Properties. Mater. Today Proc. 2021, 46, 4381–4386. [Google Scholar] [CrossRef]
- Maria, H.J.; Lyczko, N.; Nzihou, A.; Mathew, C.; George, S.C.; Joseph, K.; Thomas, S. Transport of Organic Solvents through Natural Rubber/Nitrile Rubber/Organically Modified Montmorillonite Nanocomposites. J. Mater. Sci. 2013, 48, 5373–5386. [Google Scholar] [CrossRef]
- Korsmeyer, R.W.; Gurny, R.; Doelker, E.; Buri, P.; Peppas, N.A. Mechanisms of Solute Release from Porous Hydrophilic Polymers. Int. J. Pharm. 1983, 15, 25–35. [Google Scholar] [CrossRef]
- Jose, J.P.; Thomas, S. XLPE Based Al2O3–Clay Binary and Ternary Hybrid Nanocomposites: Self-Assembly of Nanoscale Hybrid Fillers, Polymer Chain Confinement and Transport Characteristics. Phys. Chem. Chem. Phys. 2014, 16, 20190–20201. [Google Scholar] [CrossRef] [PubMed]
- Peppas, N.A.; Sahlin, J.J. A Simple Equation for the Description of Solute Release. III. Coupling of Diffusion and Relaxation. Int. J. Pharm. 1989, 57, 169–172. [Google Scholar] [CrossRef]
- Zachariah, A.K.; Kumar Chandra, A.; Mohamed, P.K.; Parameswaranpillai, J.; Thomas, S. Mixed Mode Morphology in Elastomeric Blend Nanocomposites: Effect on Vulcanization, Thermal Stability and Solvent Permeability. Polym. Compos. 2018, 39, E1659–E1668. [Google Scholar] [CrossRef]
- Kumar, N.; Singh, K.P.; Giri, A.; Singh, S.P. Transport Mechanism and Diffusion Kinetics of Kerosene through Polynorbornene Rubber/Natural Rubber Blends. Polym. Bull. 2021, 79, 5305–5325. [Google Scholar] [CrossRef]
- Prajitha, V.; Jibin, K.P.; Abitha, V.K.; Sisanth, K.S.; Huskic, M.; Meera, A.P.; George, J.S.; Thomas, S. Advancing Mechanical Performance in Sustainable Engineering: Synergistic Effects of Graphene Oxide/Nano-silica Hybrid Nanofiller via Latex Coagulation in Natural Rubber Composites. Polym. Compos. 2024, 45, 5980–5991. [Google Scholar] [CrossRef]
- Bindu, P.; Thomas, S. Viscoelastic Behavior and Reinforcement Mechanism in Rubber Nanocomposites in the Vicinity of Spherical Nanoparticles. J. Phys. Chem. B 2013, 117, 12632–12648. [Google Scholar] [CrossRef] [PubMed]
- Balakrishnan, P.; Sreekala, M.S.; Kunaver, M.; Huskić, M.; Thomas, S. Morphology, Transport Characteristics and Viscoelastic Polymer Chain Confinement in Nanocomposites Based on Thermoplastic Potato Starch and Cellulose Nanofibers from Pineapple Leaf. Carbohydr. Polym. 2017, 169, 176–188. [Google Scholar] [CrossRef] [PubMed]
- Oommen, Z.; Groeninckx, G.; Thomas, S. Dynamic Mechanical and Thermal Properties of Physically Compatibilized Natural Rubber/Poly (Methyl Methacrylate) Blends by the Addition of Natural Rubber-graft-poly (Methyl Methacrylate). J. Polym. Sci. Part B Polym. Phys. 2000, 38, 525–536. [Google Scholar] [CrossRef]
- Gopi, J.A.; Patel, S.K.; Chandra, A.K.; Tripathy, D.K. SBR-Clay-Carbon Black Hybrid Nanocomposites for Tire Tread Application. J. Polym. Res. 2011, 18, 1625–1634. [Google Scholar] [CrossRef]
- Hua, J.; Liu, K.; Wang, Z.; Geng, J.; Wang, X. Effect of Vinyl and Phenyl Group Content on the Physical and Dynamic Mechanical Properties of HVBR and SSBR. J. Appl. Polym. Sci. 2018, 135, 45975. [Google Scholar] [CrossRef]
- Sadat-Mansouri, S.N.; Hamrahjou, N.; Taghvaei-Ganjali, S.; Zadmard, R. Synthesis and Application of Silica Supported Calix [4] Arene Derivative as a New Processing Aid Agent for Reducing Hysteresis of Tread Rubber Compounds Used in Low Rolling Resistance Tires. Acta Chim. Slov. 2022, 69, 98–107. [Google Scholar] [PubMed]
- Mondal, T.; Bhowmick, A.K.; Ghosal, R.; Mukhopadhyay, R. Expanded Graphite as an Agent towards Controlling the Dispersion of Carbon Black in Poly (Styrene–Co-Butadiene) Matrix: An Effective Strategy towards the Development of High Performance Multifunctional Composite. Polymer 2018, 146, 31–41. [Google Scholar] [CrossRef]
- Thaptong, P.; Sae-Oui, P.; Sirisinha, C. Effects of Silanization Temperature and Silica Type on Properties of Silica-filled Solution Styrene Butadiene Rubber (SSBR) for Passenger Car Tire Tread Compounds. J. Appl. Polym. Sci. 2016, 133, 43342. [Google Scholar] [CrossRef]
- Gabriel, C.F.S.; Gabino, A.d.A.P.; de Sousa, A.M.F.; Furtado, C.R.G.; Nunes, R.C.R. Tire Tread Rubber Compounds with Ternary System Filler Based on Carbon Black, Silica, and Metakaolin: Contribution of Silica/Metakaolin Content on the Final Properties. J. Elastomers Plast. 2019, 51, 712–726. [Google Scholar] [CrossRef]
- Sae-oui, P.; Suchiva, K.; Thepsuwan, U.; Intiya, W.; Yodjun, P.; Sirisinha, C. Effects of Blend Ratio and SBR Type on Properties of Silica-Filled SBR/NR Tire Tread Compounds. Rubber Chem. Technol. 2016, 89, 240–250. [Google Scholar] [CrossRef]
- Schuring, D.J.; Futamura, S. Rolling Loss of Pneumatic Highway Tires in the Eighties. Rubber Chem. Technol. 1990, 63, 315–367. [Google Scholar] [CrossRef]
- Harper, M.; Tardiff, J.; Haakenson, D.; Joandrea, M.; Knych, M. Tire Tread Performance Modification Utilizing Polymeric Additives. SAE Int. J. Veh. Dyn. Stab. NVH 2017, 1, 179–189. [Google Scholar] [CrossRef]
- Sarkawi, S.S.; Aziz, A.A.; Kifli, A. Properties of Graphene Nano-Filler Reinforced Epoxidized Natural Rubber Composites. J. Polym. Sci. Technol. 2017, 2, 36–44. [Google Scholar]
- Prime, R.B.; Bair, H.E.; Vyazovkin, S.; Gallagher, P.K.; Riga, A. Thermogravimetric Analysis (TGA). Therm. Anal. Polym. Fundam. Appl. 2009, 241–317. [Google Scholar]
- Yahya, Y.S.R.; Azura, A.R.; Ahmad, Z. Effect of Curing Systems on Thermal Degradation Behaviour of Natural Rubber (SMR CV 60). J. Phys. Sci. 2011, 22, 1–14. [Google Scholar]
- De, D.; Panda, P.K.; Roy, M.; Bhunia, S. Reinforcing Effect of Reclaim Rubber on Natural Rubber/Polybutadiene Rubber Blends. Mater. Des. 2013, 46, 142–150. [Google Scholar] [CrossRef]
- Sircar, A.K. Analysis of Elastomer Vulcanizate Composition by TG-DTG Techniques. Rubber Chem. Technol. 1992, 65, 503–526. [Google Scholar] [CrossRef]
- Wunderlich, B. Thermal Analysis of Polymeric Materials; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2005; ISBN 3540236295. [Google Scholar]

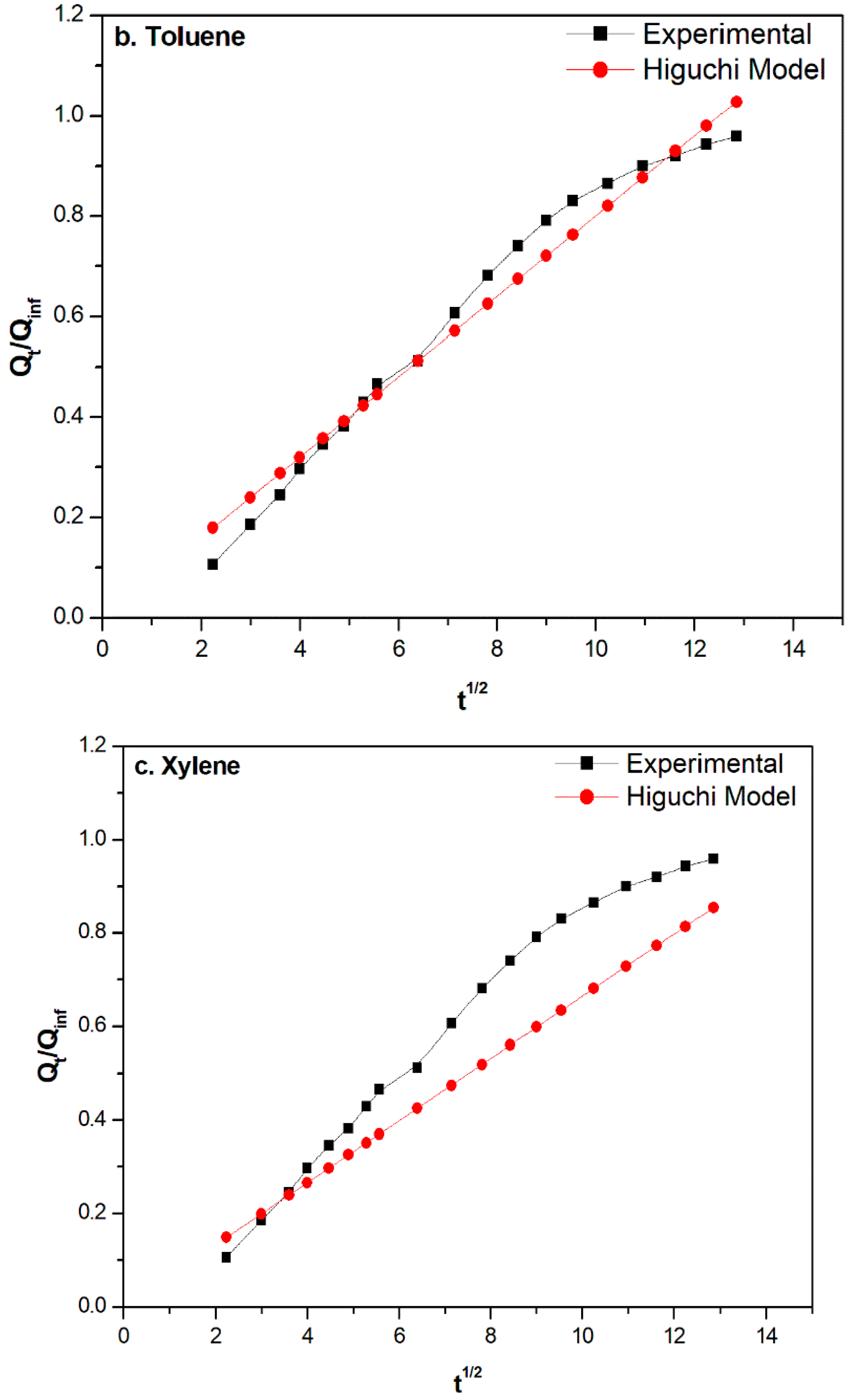


| Sample | Diffusion Coefficient (D * 108) m2/s | Sorption Coefficient (S) g/g | Permeability Coefficient (P * 108) m2/s | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Benzene | Toluene | Xylene | Benzene | Toluene | Xylene | Benzene | Toluene | Xylene | |
| Compound 0 | 0.1053 | 7.1619 | 3.3768 | 1.5887 | 1.6645 | 1.8581 | 0.1673 | 1.1921 | 6.2743 |
| Compound 1 | 0.1021 | 6.1783 | 3.195 | 1.5603 | 1.5839 | 1.7569 | 0.1593 | 0.9786 | 5.6134 |
| Compound 2 | 0.1196 | 7.4653 | 3.3464 | 1.6165 | 1.6685 | 1.7849 | 0.1933 | 1.2456 | 5.9731 |
| Compound 3 | 0.1056 | 7.4328 | 3.2952 | 1.6067 | 1.7039 | 1.8864 | 0.1697 | 1.2664 | 6.2161 |
| Sample | Benzene | Toluene | Xylene | |||
|---|---|---|---|---|---|---|
| n | k | n | k | n | k | |
| Compound 0 | 0.8518 | −1.752 | 0.6587 | −1.4061 | 0.6753 | −1.5414 |
| Compound 1 | 0.7446 | −1.537 | 0.6051 | −1.28 | 0.6439 | −1.4413 |
| Compound 2 | 0.735 | −1.518 | 0.6037 | −1.281 | 0.6273 | −1.4107 |
| Compound 3 | 0.7446 | −1.563 | 0.6371 | −1.371 | 0.6509 | −1.5121 |
| Model | Compound 0 | Compound 1 | Compound 2 | Compound 3 | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Benzene | Toluene | Xylene | Benzene | Toluene | Xylene | Benzene | Toluene | Xylene | Benzene | Toluene | Xylene | ||
| Higuchi Model | kh | 0.0727 | 0.0768 | 0.0617 | 0.0746 | 0.0809 | 0.0662 | 0.0767 | 0.0800 | 0.0665 | 0.0719 | 0.0767 | 0.0612 |
| R2 | 0.8777 | 0.8779 | 0.9184 | 0.872 | 0.8529 | 0.9092 | 0.8727 | 0.8577 | 0.9122 | 0.8794 | 0.8883 | 0.9269 | |
| Korsemayer–Peppas Model | n | 0.1250 | 0.0985 | 0.0985 | 0.1093 | 0.0898 | 0.0898 | 0.1091 | 0.0899 | 0.0899 | 0.1199 | 0.1083 | 0.1083 |
| k | 0.3523 | 0.4340 | 0.4340 | 0.3989 | 0.4729 | 0.4731 | 0.3999 | 0.4709 | 0.4709 | 0.3665 | 0.4063 | 0.4063 | |
| R2 | 0.7115 | 0.6892 | 0.6892 | 0.6963 | 0.6641 | 0.6641 | 0.6926 | 0.6719 | 0.6719 | 0.7231 | 0.6867 | 0.6867 | |
| Peppas–Sahlin Model | m | −0.1075 | −0.0999 | −0.0941 | −0.1101 | −0.0999 | −0.0976 | −0.1111 | −0.109 | −0.0999 | −0.1072 | −0.1107 | −0.1016 |
| k1 | 5.1025 | 4.985 | 4.873 | 5.0021 | 4.9934 | 4.809 | 5.092 | 4.917 | 4.8531 | 5.0650 | 5.0006 | 4.9398 | |
| k2 | −6.408 | −6.0934 | −6.0009 | −6.2502 | −6.05 | −5.8960 | −6.4005 | −6.0009 | −5.9991 | −6.3233 | −6.269 | −6.2999 | |
| R2 | 0.9495 | 0.9457 | 0.9411 | 0.9511 | 0.9442 | 0.9421 | 0.9482 | 0.9460 | 0.9373 | 0.9469 | 0.9410 | 0.9298 | |
| Sample | The Volume of the Constrained Region (Cv) | Degree of Entanglement (N) |
|---|---|---|
| Compound 0 | 0 | 8823 |
| Compound 1 | 0.0042 | 9900 |
| Compound 2 | 0.1829 | 39,599 |
| Compound 3 | 0.0025 | 9422 |
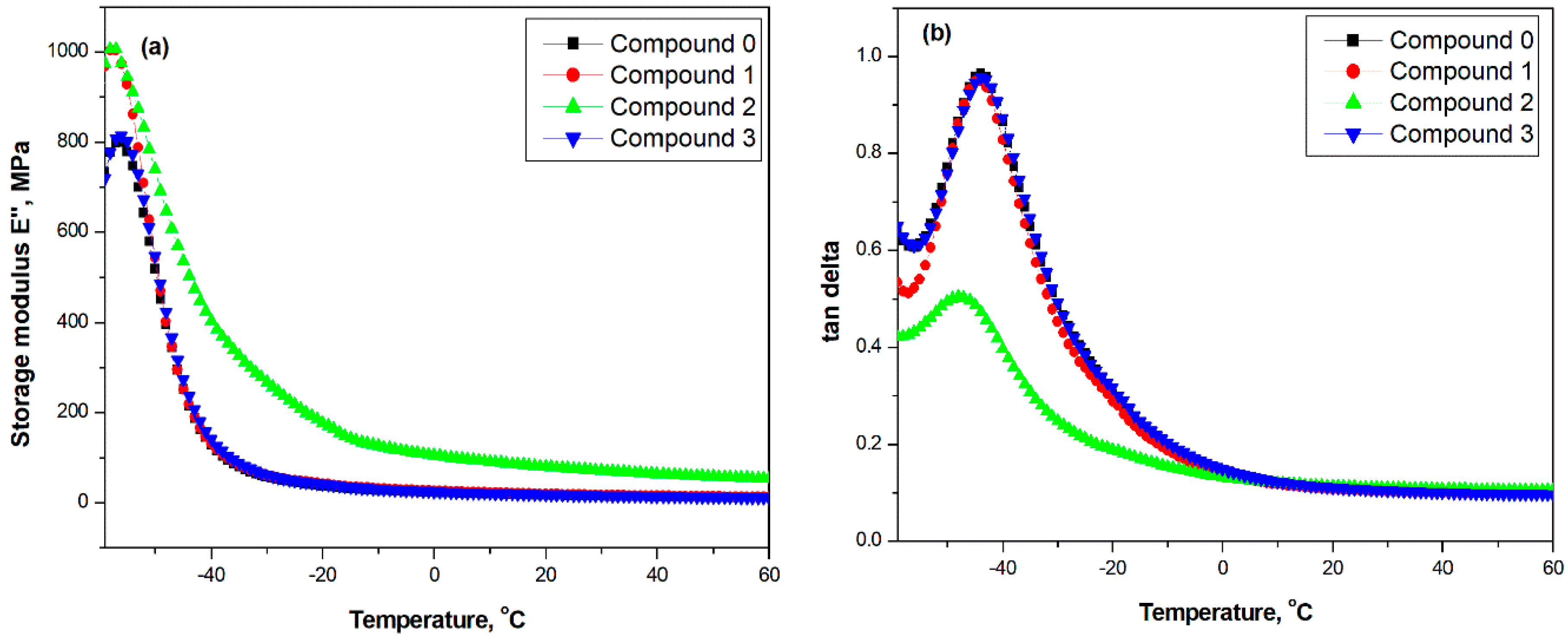
| Sample | Storage Modulus@30 °C [MPa] | tanδ@−20 °C | tanδ@0 °C | tanδ@+20 °C | tanδ@+60 °C | Tanδ Peak (Tg) °C |
|---|---|---|---|---|---|---|
| Compound 0 | 15.42 | 0.302 | 0.143 | 0.110 | 0.100 | −43.86 |
| Compound 1 | 16.91 | 0.289 | 0.140 | 0.108 | 0.099 | −44.31 |
| Compound 2 | 71.04 | 0.317 | 0.149 | 0.114 | 0.103 | −46.83 |
| Compound 3 | 15.94 | 0.189 | 0.132 | 0.111 | 0.097 | −43.56 |
| Sample | First Degradation Peak (°C) | Weight Loss at Peak-1 (%) | Second Degradation Peak (°C) | Weight Loss at Peak-2 (%) | Residue at 750 °C (%) |
|---|---|---|---|---|---|
| Compound 0 | 370 | 29.38 | 447 | 53.51 | 0.86 |
| Compound 1 | 363 | 19.09 | 447.16 | 39.07 | 4.22 |
| Compound 2 | 373.5 | 12.80 | 447.8 | 40.95 | 4.91 |
| Compound 3 | 358 | 25.60 | 443 | 48.10 | 7.90 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rajan, K.P.; Gopanna, A.; Rafic, M.; Theravalappil, R.; Thomas, S.P. Partial Replacement of Carbon Black with Graphene in Tire Compounds: Transport Properties, Thermal Stability and Dynamic Mechanical Analysis. ChemEngineering 2024, 8, 57. https://doi.org/10.3390/chemengineering8030057
Rajan KP, Gopanna A, Rafic M, Theravalappil R, Thomas SP. Partial Replacement of Carbon Black with Graphene in Tire Compounds: Transport Properties, Thermal Stability and Dynamic Mechanical Analysis. ChemEngineering. 2024; 8(3):57. https://doi.org/10.3390/chemengineering8030057
Chicago/Turabian StyleRajan, Krishna Prasad, Aravinthan Gopanna, Mohammed Rafic, Rajesh Theravalappil, and Selvin P. Thomas. 2024. "Partial Replacement of Carbon Black with Graphene in Tire Compounds: Transport Properties, Thermal Stability and Dynamic Mechanical Analysis" ChemEngineering 8, no. 3: 57. https://doi.org/10.3390/chemengineering8030057
APA StyleRajan, K. P., Gopanna, A., Rafic, M., Theravalappil, R., & Thomas, S. P. (2024). Partial Replacement of Carbon Black with Graphene in Tire Compounds: Transport Properties, Thermal Stability and Dynamic Mechanical Analysis. ChemEngineering, 8(3), 57. https://doi.org/10.3390/chemengineering8030057









