Statistical Analysis of Wood Durability Data and Its Effect on a Standardised Classification Scheme
Abstract
:1. Introduction
2. Materials and Methods
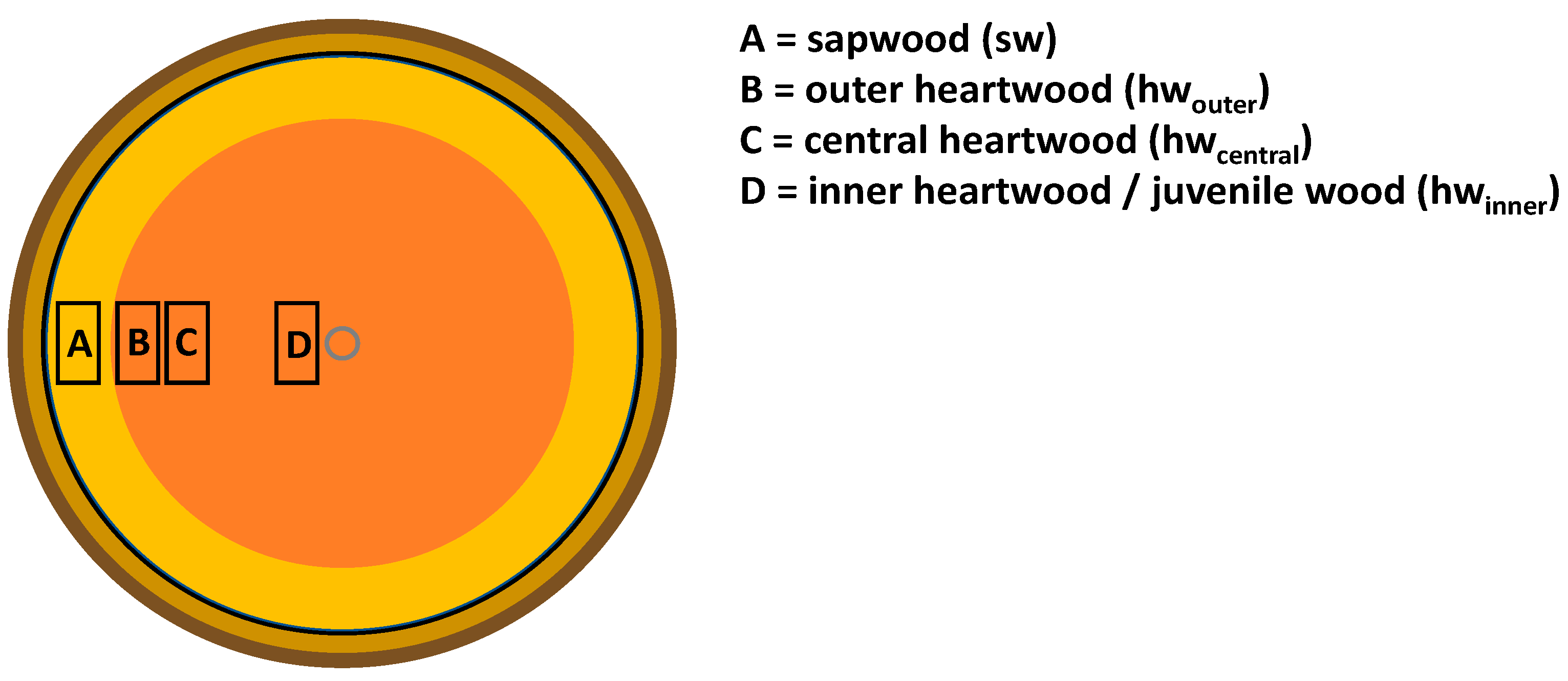
2.1. Wood Species, Treatments, and Sampling
2.2. Agar Plate Tests with Basidiomycetes
2.3. Durability Classification
2.4. Statistical Analysis
Fitted Probability Density Functions
3. Results and Discussion
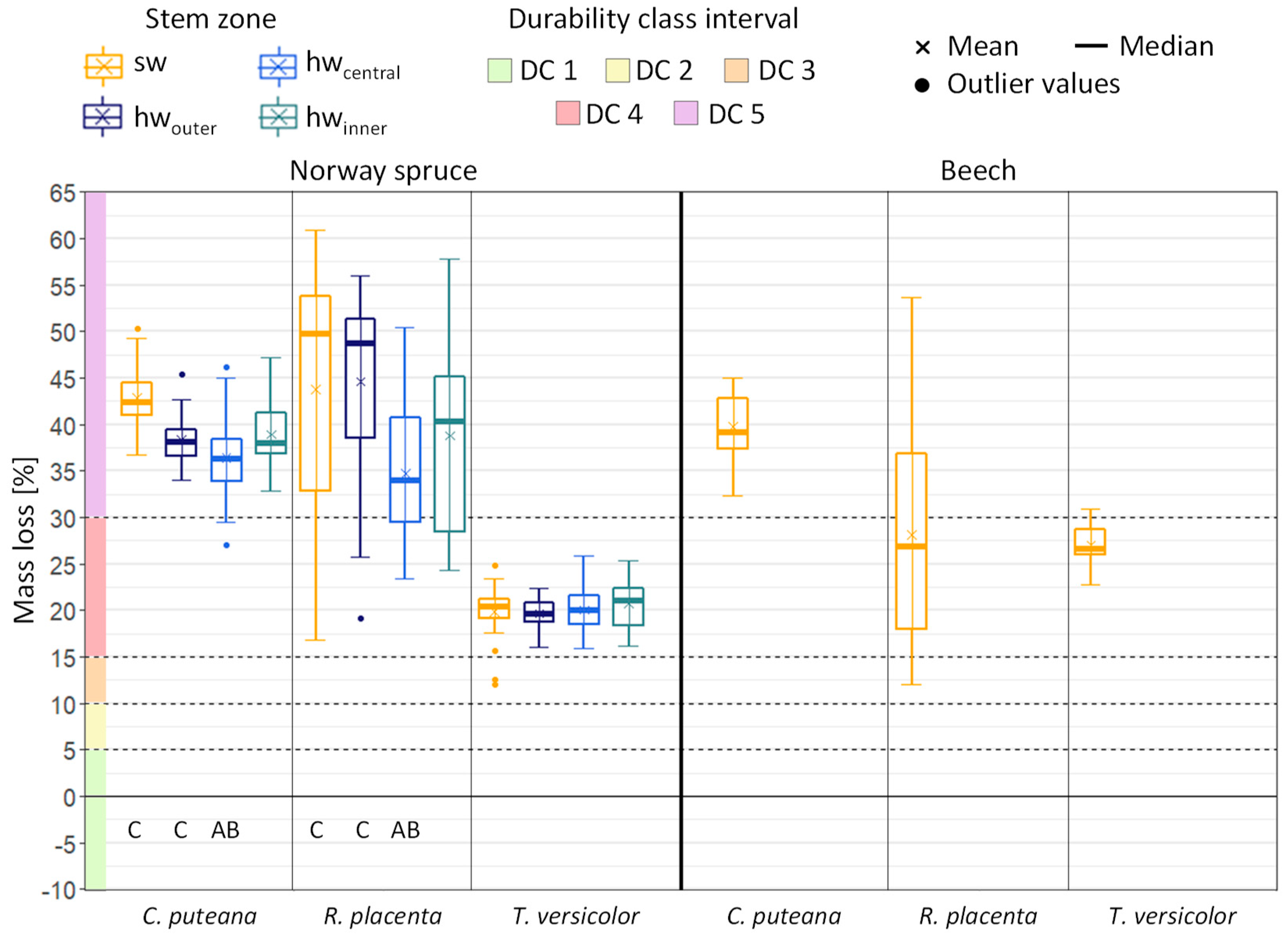
3.1. Mass Loss
3.2. Sampling from Different Stem Zones
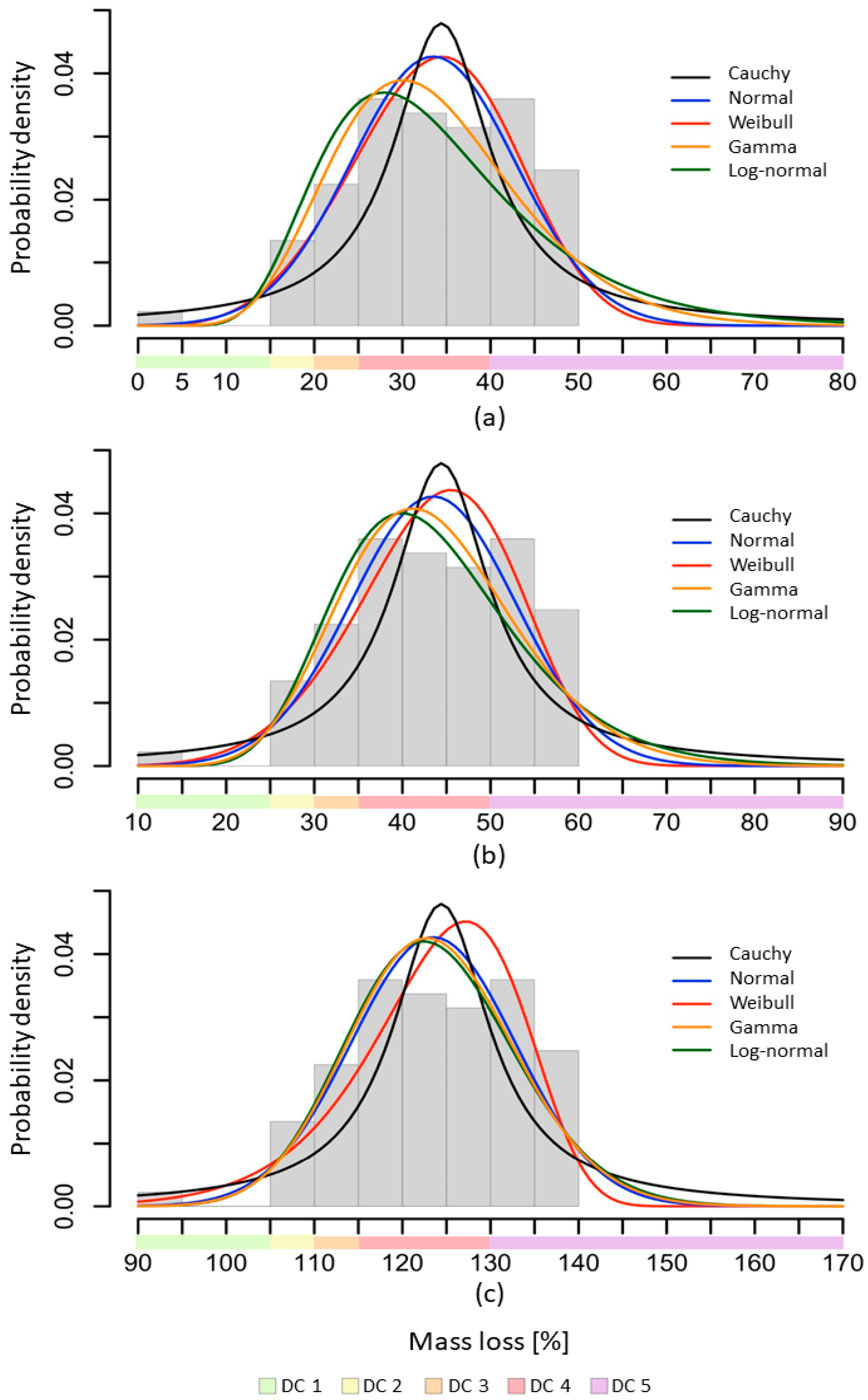
3.3. Applying Fitted Probability Density Functions to Mass Loss Data
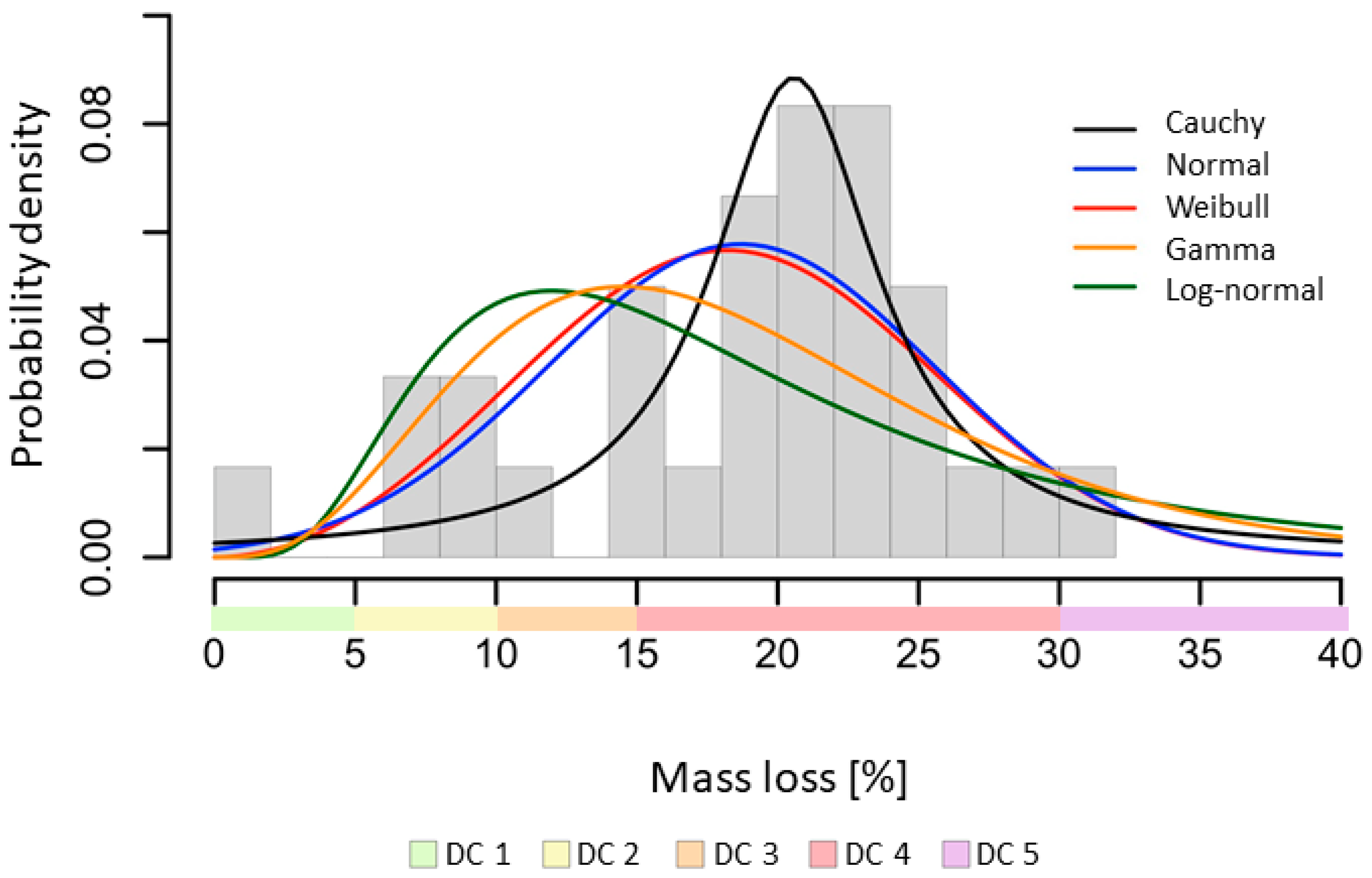
3.3.1. Handling Negative Mass Loss Values
- (1)
- The substitution of negative ML values by very small, but still positive operating values of 0.1e−10% does not lead to significant changes in the data set’s median value, which is crucial since the latter defines the DC. However, the mean value and the standard deviation are influenced by substituting negative values. Nevertheless, these values are parameter estimators for the fitting of affected distributions. An unspecific shift of these values due to substitution cannot be seen as a valid procedure, because this shift directly influences the calculation of the fitted probability function.
- (2)
- A low (10%), medium (20%), and high (100%) data translation along the x-axis was exemplarily performed for the ML data (hwtotal) of Scots pine incubated with C. puteana. As shown in Figure 5, the data translation led to changes in the overall appearance of the fitted distributions; especially for Weibull, Gamma, and Log-normal distributions. The latter does not equally continue to ± ∞, but is limited to zero. Since the transformation of the fitted probability density function to the DC distribution is performed via the calculation of the integral area of the graph within the DC intervals, any compression or shift of the graph can lead to a non-transparent impact on the integral proportion within the DC intervals. The restriction of certain distributions to 0 (0 to +∞) in comparison to distributions with no limitation (−∞ to +∞) can cause non-transparent differences in the integral area per DC interval. Once the ML data analysis is completed, a reconstruction of empirical or fitted ML data via the integral percentage is impossible.
3.3.2. Data Fitting
3.4. Durability Classification
3.5. Further Aspects under Debate
4. Conclusions
- Since the durability can vary not only between sapwood, heartwood, and juvenile wood but also between outer and central heartwood, more precise guidance is needed on the sampling procedure. Especially when sampling boards, planks, and wood products, it is difficult to differentiate between stem zones which are not adequately addressed by the current standards.
- Showing the spread of individual ML data using fitted probability density functions is an optional but recommended element of the test protocol according to EN 113-2 [9]. However, the proposed statistical treatment is inadequately described and thus hardly reproducible. In particular, the standard lacks a description of the selection procedure of the best-fitting density function.
- The application of probability density functions is demanding and laborious. The comparison of DCs based on empirical distributions and those derived from best-fitted density functions showed that only marginal differences could be expected. The additional information about the variability of wood durability is rather limited. Furthermore, the statistical procedure is highly complex, and its application may cause further sources of error.
- Generally, the assignment of dispersion indicators appeared meaningful since it could provide additional information about the variability of wood durability. However, using two different and non-complementary indicators may cause confusion. Preference should be given to indicators that provide both qualitative and quantitative information. A range of DCs should take precedence over the variability index ‘v’.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Van Acker, J.; Stevens, M.; Carey, J.; Sierra-Alvarez, R.; Militz, H.; Le Bayon, I.; Kleist, G.; Peek, R.D. Biological durability of wood in relation to end-use. Holz als Roh- und Werkstoff. 2003, 61, 35–45. [Google Scholar] [CrossRef]
- Augusta, U. Untersuchung der Natürlichen Dauerhaftigkeit Wirtschaftlich Bedeutender Holzarten bei Verschiedener Beanspruchung im Außenbereich. Ph.D. Thesis, University of Hamburg, Hamburg, Germany, 2007. [Google Scholar]
- Brischke, C.; Alfredsen, G.; Humar, M.; Conti, E.; Cookson, L.; Emmerich, E.; Flæte, P.O.; Fortino, S.; Francis, L.; Hundhausen, U.; et al. Modelling the material resistance of wood—Part 2: Validation and Optimization of the Meyer-Veltrup Model. Forests 2021, 12, 590. [Google Scholar] [CrossRef]
- Brischke, C.; Alfredsen, G.; Humar, M.; Conti, E.; Cookson, L.; Emmerich, E.; Flæte, P.O.; Fortino, S.; Francis, L.; Hundhausen, U.; et al. Modelling the material resistance of wood—Part 3: Relative resistance in above and in ground situations—Results of a global survey. Forests 2021, 12, 576. [Google Scholar] [CrossRef]
- Brischke, C.; Alfredsen, G. Biological durability of pine wood. Wood Mat. Sci. Eng. 2022, 18, 1050–1064. [Google Scholar] [CrossRef]
- EN 350; Durability of Wood and Wood-Based Products—Testing and Classification of the Durability to Biological Agents of Wood and Wood-Based Materials. European Committee for Standardization: Brussels, Belgium, 2016.
- CEN/TS 15083-2; Durability of Wood and Wood-Based Products—Determination of the Natural Durability of Solid Wood against Wood-Destroying Fungi, Test. Methods—Part 2: Soft Rotting Micro-Fungi. European Committee for Standardization (CEN): Brussels, Belgium, 2005.
- EN 252; Field Test Method for Determining the Relative Protective Effectiveness of a Wood Preservative in Ground Contact. European Committee for Standardization: Brussels, Belgium, 2015.
- EN 113-2; Durability of Wood and Wood-Based Products—Test. Method against Wood Destroying Basidiomycetes—Part. 2: Assessment of Inherent or Enhanced Durability. European Committee for Standardization: Brussels, Belgium, 2021.
- Van Acker, J.; Palanti, S. Durability. In Performance of Bio-Based Building Materials; Jones, D., Brischke, C., Eds.; Elsevier: Duxford, UK, 2017; pp. 257–277. [Google Scholar]
- Alfredsen, G.; Brischke, C.; Marais, B.N.; Stein, R.F.; Zimmer, K.; Humar, M. Modelling the material resistance of wood—Part 1: Utilizing durability test data based on different reference wood species. Forests 2021, 12, 558. [Google Scholar] [CrossRef]
- Bollmus, S.; Bächle, L.; Militz, H.; Brischke, C. Durability classification of preservative treated and modified wood. In Proceedings of the IRG Annual Meeting, IRG/WP 19-20659, Quebec City, QC, Canada, 12–16 May 2019; p. 17. [Google Scholar]
- Brischke, C.; Bollmus, S.; Melcher, E.; Stephan, I. Biological durability and moisture dynamics of Dawn redwood (Metasequoia glyptostroboides) and Port Orford cedar (Chamaecyparis lawsoniana). Wood Mat. Sci. Eng. 2022, 18, 1024–1034. [Google Scholar] [CrossRef]
- Meyer, L.; Brischke, C.; Treu, A.; Larsson-Brelid, P. Critical moisture conditions for fungal decay of modified wood by basidiomycetes as detected by pile tests. Holzforschung 2016, 70, 331–339. [Google Scholar] [CrossRef]
- R Core Team. R: A Language and Environment for Statistical Computing; R Foundation for Statistical Computing: Vienna, Austria, 2020; Available online: http://www.r-project.org/index.html (accessed on 11 April 2023).
- Brischke, C.; Welzbacher, C.R.; Gellerich, A.; Bollmus, S.; Humar, M.; Plaschkies, K.; Scheiding, W.; Alfredsen, G.; Van Acker, J.; De Windt, I. Wood natural durability testing under laboratory conditions: Results from a round-robin test. Eur. J. Wood Wood Prod. 2014, 72, 129–133. [Google Scholar] [CrossRef]
- Emmerich, L.; Ehrmann, A.; Brischke, C.; Militz, H. Comparative studies on the durability and moisture performance of wood modified with cyclic N-methylol and N-methyl compounds. Wood Sci. Technol. 2021, 55, 1531–1554. [Google Scholar] [CrossRef]
- Nilsson, T.; Daniel, G. On the use of% weight loss as a measure for expressing results of laboratory decay experiments. In Proceedings of the IRG Annual Meeting, IRG/WP 92-2394, Harrogate, UK, 10–15 May 1992; p. 6. [Google Scholar]
- Brischke, C.; Bayerbach, R.; Rapp, A.O. Decay-influencing factors: A basis for service life prediction of wood and wood-based products. Wood Mat. Sci. Eng. 2006, 1, 91–107. [Google Scholar] [CrossRef]
- Despot, R.; Hasan, M.; Brischke, C.; Welzbacher, C.R.; Rapp, A.O. Changes in physical, mechanical and chemical properties of wood during sterilisation by gamma radiation. Holzforschung 2007, 61, 267–271. [Google Scholar] [CrossRef]
- Despot, R.; Hasan, M.; Glavaš, M.; Rep, G. On the changes of natural durability of wood sterilised by gamma radiation. In Proceedings of the IRG Annual Meeting, IRG/WP 06-10571, Tromsø, Norway, 18–22 June 2006; p. 12. [Google Scholar]
- Brischke, C.; von Boch-Galhau, N.; Bollmus, S. Impact of different sterilization techniques and mass loss measurements on the durability of wood against wood-destroying fungi. Eur. J. Wood Wood Prod. 2022, 80, 35–44. [Google Scholar] [CrossRef]
- Donath, S.; Militz, H.; Mai, C. Creating water repellent effects on wood by treatment with silanes. Holzforschung 2006, 60, 40–46. [Google Scholar] [CrossRef]
- Beck, G.; Thybring, E.E.; Thygesen, L.G. Brown-rot fungal degradation and de-acetylation of acetylated wood. Int. Biodeter. Biodegr. 2018, 135, 62–70. [Google Scholar] [CrossRef]
- Thygesen, L.G.; Beck, G.; Nagy, N.E.; Alfredsen, G. Cell wall changes during brown rot degradation of furfurylated and acetylated wood. Int. Biodeter. Biodegr. 2021, 162, 105257. [Google Scholar] [CrossRef]
- Brischke, C.; Soetbeer, A.; Meyer-Veltrup, L. The minimum moisture threshold for wood decay by basidiomycetes revisited. A review and modified pile experiments with Norway spruce and European beech decayed by Coniophora puteana and Trametes versicolor. Holzforschung 2017, 71, 893–903. [Google Scholar] [CrossRef]
- Emmerich, L.; Bleckmann, M.; Strohbusch, S.; Brischke, C.; Bollmus, S.; Militz, H. Growth behavior of wood-destroying fungi in chemically modified wood: Wood degradation and translocation of nitrogen compounds. Holzforschung 2021, 75, 786–797. [Google Scholar] [CrossRef]


| Wood Species | Botanical Name | Stem Zone * | |||
|---|---|---|---|---|---|
| sw | hwouter | hwcentral | hwinner | ||
| European larch | Larix decidua | n.a. | 90 | 90 | 90 |
| Norway spruce | Picea abies | 90 | 90 | 90 | 90 |
| Scots pine | Pinus sylvestris | 90 | 90 | 90 | 90 |
| European beech | Fagus sylvatica | 90 | n.a. | n.a. | n.a. |
| English oak | Quercus robur | n.a. | 90 | 90 | 90 |
| Black locust | Robinia pseudoacacia | n.a. | 90 | 90 | 90 |
| Durability Class | Description | Median Percent Mass Loss (ML) 1 |
|---|---|---|
| DC 1 | Very durable | ≤5 |
| DC 2 | Durable | >5 to ≤10 |
| DC 3 | Moderately durable | >10 to ≤15 |
| DC 4 | Less durable | >15 to ≤30 |
| DC 5 | Not durable | >30 2 |
| Wood Species | Test Fungi | Stem Zone | ||||
|---|---|---|---|---|---|---|
| sw | hwouter | hwcentral | hwinner | hwtotal | ||
| European larch | C. puteana | n.a. | * | - | - | - |
| R. placenta | n.a. | *** | - | ** | *** | |
| T. versicolor | n.a. | - | - | - | - | |
| Norway spruce | C. puteana | - | - | - | - | - |
| R. placenta | ** | ** | - | * | ** | |
| T. versicolor | *** | - | - | - | - | |
| Scots pine | C. puteana | * | - | - | - | * |
| R. placenta | - | * | - | - | ** | |
| T. versicolor | - | - | - | - | - | |
| European beech | C. puteana | - | n.a. | n.a. | n.a. | n.a. |
| R. placenta | * | n.a. | n.a. | n.a. | n.a. | |
| T. versicolor | - | n.a. | n.a. | n.a. | n.a. | |
| English oak | C. puteana | n.a. | - | *** | *** | *** |
| R. placenta | n.a. | *** | ** | - | *** | |
| T. versicolor | n.a. | ** | - | - | *** | |
| Black locust | C. puteana | n.a. | *** | - | - | *** |
| R. placenta | n.a. | ** | - | - | *** | |
| T. versicolor | n.a. | *** | *** | - | *** | |
| Empirical Distribution ** | Best Fit Density Function *** | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Wood Species | Stem Zone | Test Fungus | Median ML [%] | DC * | DC 1 [%] | DC 2 [%] | DC 3 [%] | DC 4 [%] | DC 5 [%] | DC | DC 1 [%] | DC 2 [%] | DC 3 [%] | DC 4 [%] | DC 5 [%] | DC |
| Scots pine | sw | C.p. | 43.4 | 5 | - | - | - | - | 100.0 | 5 | - | - | - | 1.2 | 98.8 | 5 2 |
| R.p. | 35.7 | 5 | 3.3 | - | - | 33.3 | 63.3 | 5 | 0.6 | 1.2 | 2.6 | 25.4 | 70.2 | 5 1 | ||
| T.v. | 24.1 | 4 | - | - | - | 100.0 | - | 4 | - | - | 0.1 | 99.9 | - | 4 2 | ||
| hwouter | C.p. | 25.2 | 4 | - | 3.3 | 10.0 | 53.3 | 33.3 | 4 | 0.3 | 3.1 | 9.5 | 62.9 | 24.2 | 4 2 | |
| R.p. | 23.1 | 4 | - | - | 3.3 | 60.0 | 36.7 | 4 | 0.2 | 2.0 | 6.3 | 53.9 | 37.6 | 4 4 | ||
| T.v. | 10.7 | 3 | - | 4.0 | 60.0 | - | - | 2–3 | 1.3 | 41.9 | 56.3 | 0.5 | - | 2–3 2 | ||
| hwcentral | C.p. | 23.9 | 4 | - | 13.3 | 10.0 | 50.0 | 26.7 | 4v | 1.1 | 5.9 | 12.6 | 56.7 | 23.7 | 4 2 | |
| R.p. | 31.9 | 5 | - | - | - | 36.7 | 63.3 | 5 | - | 0.5 | 2.4 | 42.8 | 54.3 | 4–5 3 | ||
| T.v. | 10.7 | 3 | - | 40.0 | 60.0 | - | - | 2–3 | 1.3 | 41.9 | 56.3 | 0.5 | - | 2–3 2 | ||
| hwinner | C.p. | 23.2 | 4 | 3.4 | 3.4 | 13.8 | 48.3 | 31.1 | 4 | 0.9 | 5.3 | 12.1 | 58.2 | 23.5 | 4 2 | |
| R.p. | 24.1 | 4 | - | - | 3.3 | 73.3 | 23.3 | 4 | 0.1 | 1.7 | 6.8 | 66.9 | 24.5 | 4 3 | ||
| T.v. | 10.9 | 3 | 10.0 | 30.0 | 50.0 | 10.0 | - | 3v | 8.6 | 37.8 | 41.8 | 11.8 | - | 3 1 | ||
| hwtotal | C.p. | 23.7 | 4 | 1.1 | 6.7 | 11.2 | 50.6 | 30.4 | 4 | 1.7 | 5.3 | 10.2 | 55.4 | 27.4 | 4 2 | |
| R.p. | 25.2 | 4 | - | - | 2.2 | 56.7 | 41.1 | 4–5 | - | 0.2 | 3.2 | 61.9 | 34.7 | 4 3 | ||
| T.v. | 10.8 | 3 | 3.3 | 35.6 | 56.7 | 4.4 | - | 3 | 3.2 | 39.9 | 51.8 | 5.1 | - | 3 2 | ||
| Empirical Distribution ** | Best Fit Density Function *** | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Wood Species | Stem Zone | Test Fungus | Median ML [%] | DC * | DC 1 [%] | DC 2 [%] | DC 3 [%] | DC 4 [%] | DC 5 [%] | DC | DC 1 [%] | DC 2 [%] | DC 3 [%] | DC 4 [%] | DC 5 [%] | DC |
| European larch | hwouter | C.p. | 20.7 | 4 | - | - | 3.3 | 76.7 | 20.0 | 4 | - | 0.2 | 8.2 | 78.0 | 13.6 | 4 4 |
| R.p. | 19.7 | 4 | - | - | 13.3 | 73.3 | 13.3 | 4 | - | 2.1 | 15.5 | 65.5 | 17.0 | 3–5 4 | ||
| T.v. | 9.6 | 2 | 10.0 | 43.3 | 33.3 | 13.3 | - | 2v | 5.5 | 48.1 | 36.2 | 10.2 | - | 2v 3 | ||
| hwcentral | C.p. | 28.0 | 4 | - | - | 10.0 | 46.7 | 43.3 | 4–5 | 0.1 | 1.1 | 4.7 | 56.4 | 37.7 | 4 2 | |
| R.p. | 32.0 | 5 | 6.6 | - | - | 36.7 | 56.7 | 5 | 4.3 | 3.5 | 5.3 | 27.7 | 59.2 | 5 1 | ||
| T.v. | 7.9 | 2 | 13.3 | 60.0 | 26.7 | - | - | 2 | 15.2 | 55.5 | 27.4 | 1.9 | - | 1–3 2 | ||
| hwinner | C.p. | 24.5 | 4 | - | - | 3.3 | 93.4 | 3.3 | 4 | - | 0.2 | 2.5 | 91.3 | 6.0 | 4 2 | |
| R.p. | 30.6 | 5 | - | - | - | 46.7 | 53.3 | 4–5 | - | - | 1.4 | 44.5 | 54.1 | 4–5 4 | ||
| T.v. | 9.4 | 2 | 3.3 | 63.3 | 33.3 | - | - | 2 | 5.8 | 59.4 | 34.4 | 0.4 | - | 2 2 | ||
| hwtotal | C.p. | 24.5 | 4 | - | - | 5.6 | 72.2 | 22.2 | 4 | - | 0.3 | 5.4 | 73.6 | 20.7 | 4 3 | |
| R.p. | 24.8 | 4 | 2.2 | - | 4.4 | 52.2 | 41.1 | 4–5 | 1.4 | 5.0 | 8.7 | 38.8 | 46.1 | 4 2 | ||
| T.v. | 9.3 | 2 | 8.9 | 55.6 | 31.1 | 4.4 | - | 2 | 10.7 | 50.3 | 35.1 | 3.9 | - | 2 2 | ||
| Empirical Distribution ** | Best Fit Density Function *** | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Wood Species | Stem Zone | Test Fungus | Median ML [%] | DC * | DC 1 [%] | DC 2 [%] | DC 3 [%] | DC 4 [%] | DC 5 [%] | DC | DC 1 [%] | DC 2 [%] | DC 3 [%] | DC 4 [%] | DC 5 [%] | DC |
| Norway spruce | sw | C.p. | 42.4 | 5 | - | - | - | - | 100.0 | 5 | - | - | - | - | 100.0 | 5 4 |
| R.p. | 49.8 | 5 | - | - | - | 13.3 | 86.7 | 5 | - | 0.1 | 0.5 | 11.3 | 88.1 | 5 2 | ||
| T.v. | 20.5 | 4 | - | - | 6.7 | 93.3 | - | 4 | - | 0.8 | 2.3 | 92.2 | 2.9 | 4 5 | ||
| hwouter | C.p. | 38.1 | 5 | - | - | - | - | 100.0 | 5 | - | - | - | - | 100.0 | 5 4 | |
| R.p. | 48.7 | 5 | - | - | - | 6.7 | 93.3 | 5 | - | - | - | 4.3 | 95.7 | 5 2 | ||
| T.v. | 19.7 | 4 | - | - | - | 100.0 | - | 4 | - | - | 0.1 | 99.9 | - | 4 1 | ||
| hwcentral | C.p. | 36.3 | 5 | - | - | - | 6.7 | 93.3 | 5 | - | - | - | 5.8 | 94.2 | 5 3 | |
| R.p. | 34.0 | 5 | - | - | - | 36.7 | 63.3 | 5 | - | - | - | 27.7 | 72.3 | 5 4 | ||
| T.v. | 20.1 | 4 | - | - | - | 100.0 | - | 4 | - | - | 0.3 | 99.7 | - | 4 4 | ||
| hwinner | C.p. | 38.1 | 5 | - | - | - | - | 100.0 | 5 | - | - | - | 0.1 | 99.9 | 5 4 | |
| R.p. | 40.3 | 5 | - | - | - | 33.3 | 66.7 | 5 | - | - | 0.1 | 18.4 | 81.5 | 5 3 | ||
| T.v. | 21.1 | 4 | - | - | - | 100.0 | - | 4 | - | - | 1.5 | 98.5 | - | 4 1 | ||
| hwtotal | C.p. | 37.6 | 5 | - | - | - | 2.2 | 97.8 | 5 | - | - | - | 1.2 | 98.8 | 5 1 | |
| R.p. | 40.0 | 5 | - | - | - | 25.8 | 74.2 | 5 | - | 0.1 | 0.6 | 16.0 | 83.3 | 5 2 | ||
| T.v. | 19.9 | 4 | - | - | - | 100.0 | - | 4 | - | - | 0.4 | 99.6 | - | 4 4 | ||
| Empirical Distribution ** | Best Fit Density Function *** | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Wood Species | Stem Zone | Test Fungus | Median ML [%] | DC * | DC 1 [%] | DC 2 [%] | DC 3 [%] | DC 4 [%] | DC 5 [%] | DC | DC 1 [%] | DC 2 [%] | DC 3 [%] | DC 4 [%] | DC 5 [%] | DC |
| English oak | hwouter | C.p. | 0.7 | 1 | 100.0 | - | - | - | - | 1 | 99.3 | 0.4 | 0.1 | 0.1 | 0.1 | 1 5 |
| R.p. | 0.7 | 1 | 100.0 | - | - | - | - | 1 | 99.3 | 0.6 | 0.1 | - | - | 1 4 | ||
| T.v. | 5.4 | 2 | 46.7 | 26.7 | 13.3 | 13.3 | - | 2v | 40.9 | 37.1 | 13.3 | 7.8 | 0.9 | 2 4 | ||
| hwcentral | C.p. | 0.6 | 1 | 100.0 | - | - | - | - | 1 | 99.3 | 0.4 | 0.1 | 0.1 | 0.1 | 1 4 | |
| R.p. | 0.8 | 1 | 100.0 | - | - | - | - | 1 | 100.0 | - | - | - | - | 1 4 | ||
| T.v. | 6.4 | 2 | 36.7 | 30.0 | 26.7 | 6.7 | - | 1–3 | 34.2 | 39.0 | 19.2 | 7.6 | - | 1–3 2 | ||
| hwinner | C.p. | 1.0 | 1 | 76.7 | 10.0 | 10.0 | 3.3 | - | 1v | 83.3 | 10.0 | 3.2 | 2.6 | 0.9 | 1 4 | |
| R.p. | 7.5 | 2 | 23.3 | 50.0 | 23.3 | 6.7 | - | 1–3 | 24.5 | 49.4 | 22.6 | 3.5 | - | 1–3 2 | ||
| T.v. | 20.1 | 4 | 3.3 | 13.3 | 10.0 | 70.0 | 3.3 | 4v | 2.4 | 8.0 | 19.2 | 65.3 | 5.1 | 4 1 | ||
| hwtotal | C.p. | 0.7 | 1 | 92.2 | 3.3 | 3.3 | 1.1 | - | 1 | 99.0 | 0.5 | 0.2 | 0.2 | 0.1 | 1 5 | |
| R.p. | 1.2 | 1 | 74.4 | 16.7 | 7.8 | 1.1 | - | 1 | 83.0 | 10.5 | 3.2 | 2.5 | 0.8 | 1 4 | ||
| T.v. | 9.7 | 2 | 28.9 | 23.3 | 16.7 | 30.0 | 1.1 | 1–4/2v | 23.3 | 28.5 | 21.5 | 24.1 | 2.6 | 1–4/2v 2 | ||
| Empirical Distribution ** | Best Fit Density Function *** | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Wood Species | Stem Zone | Test Fungus | Median ML [%] | DC * | DC 1 [%] | DC 2 [%] | DC 3 [%] | DC 4 [%] | DC 5 [%] | DC | DC 1 [%] | DC 2 [%] | DC 3 [%] | DC 4 [%] | DC 5 [%] | DC |
| Black locust | hwouter | C.p. | 0.8 | 1 | 100.0 | - | - | - | - | 1 | 99.1 | 0.9 | - | - | - | 1 4 |
| R.p. | 2.3 | 1 | 86.7 | 13.3 | - | - | - | 1 | 92.1 | 7.6 | 0.3 | - | - | 1 4 | ||
| T.v. | 3.8 | 1 | 63.3 | 30.0 | 6.7 | - | - | 1 | 59.8 | 34.6 | 4.7 | 0.9 | - | 1 4 | ||
| hwcentral | C.p. | 1.4 | 1 | 100.0 | - | - | - | - | 1 | 100.0 | - | - | - | - | 1 3 | |
| R.p. | 4.0 | 1 | 83.3 | 16.7 | - | - | - | 1 | 89.5 | 10.5 | - | - | - | 1 2 | ||
| T.v. | 4.5 | 1 | 63.3 | 33.3 | 3.3 | 6.7 | - | 1 | 52.5 | 46.8 | 0.7 | - | - | 1–2 4 | ||
| hwinner | C.p. | 4.4 | 1 | 63.3 | 36.7 | - | - | - | 1 | 63.1 | 33.2 | 3.6 | 0.1 | - | 1 2 | |
| R.p. | 8.2 | 2 | - | 76.7 | 23.3 | - | - | 2 | 0.8 | 78.5 | 20.6 | 0.1 | - | 2 3 | ||
| T.v. | 20.7 | 4 | - | 3.3 | - | 90.0 | 6.7 | 4 | 0.1 | 1.8 | 9.6 | 83.0 | 5.5 | 4 2 | ||
| hwtotal | C.p. | 1.5 | 1 | 87.8 | 12.2 | - | - | - | 1 | 90.4 | 7.8 | 1.3 | 0.5 | - | 1 4 | |
| R.p. | 4.2 | 1 | 56.7 | 35.5 | 7.8 | - | - | 1 | 57.5 | 35.4 | 6.2 | 0.9 | - | 1 3 | ||
| T.v. | 6.3 | 2 | 42.2 | 22.2 | 3.4 | 30.0 | 2.2 | 1–4/2v | 29.7 | 33.2 | 16.9 | 15.7 | 4.5 | 1–4/2v 4 | ||
| Empirical Distribution ** | Best Fit Density Function *** | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Wood Species | Stem Zone | Test Fungus | Median ML [%] | DC * | DC 1 [%] | DC 2 [%] | DC 3 [%] | DC 4 [%] | DC 5 [%] | DC | DC 1 [%] | DC 2 [%] | DC 3 [%] | DC 4 [%] | DC 5 [%] | DC |
| Beech | sw | C.p. | 39.2 | 5 | - | - | - | - | 100.0 | 5 | - | - | - | - | 100.0 | 5 1 |
| R.p. | 26.9 | 4 | - | - | 10.0 | 43.3 | 46.7 | 4–5 | - | 1.6 | 7.6 | 52.6 | 38.2 | 4 3 | ||
| T.v. | 26.7 | 4 | - | - | - | 93.3 | 6.7 | 4 | - | - | - | 92.6 | 7.4 | 4 1 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brischke, C.; Haase, F.; Bächle, L.; Bollmus, S. Statistical Analysis of Wood Durability Data and Its Effect on a Standardised Classification Scheme. Standards 2023, 3, 210-226. https://doi.org/10.3390/standards3020017
Brischke C, Haase F, Bächle L, Bollmus S. Statistical Analysis of Wood Durability Data and Its Effect on a Standardised Classification Scheme. Standards. 2023; 3(2):210-226. https://doi.org/10.3390/standards3020017
Chicago/Turabian StyleBrischke, Christian, Felix Haase, Lea Bächle, and Susanne Bollmus. 2023. "Statistical Analysis of Wood Durability Data and Its Effect on a Standardised Classification Scheme" Standards 3, no. 2: 210-226. https://doi.org/10.3390/standards3020017
APA StyleBrischke, C., Haase, F., Bächle, L., & Bollmus, S. (2023). Statistical Analysis of Wood Durability Data and Its Effect on a Standardised Classification Scheme. Standards, 3(2), 210-226. https://doi.org/10.3390/standards3020017








