A New Perspective on Fluorapatite Dissolution in Hydrochloric Acid: Thermodynamic Calculations and Experimental Study

Abstract

Apatite (Ap) dissolution in diluted acids is well described in the literature, but in technological processes which use more concentrated acids, the reaction is fast, and it is complicated to follow the process kinetics. The relationship between pH change and the apatite dissolution rate depending on HCl concentration was studied by thermodynamic calculations and experiments with synthetic fluorapatite (FAp). On the basis of experimental pH measurements, the kinetics of dissolution was analyzed. The solution composition (P, Ca, F) was determined by wet chemical methods and the solid part was characterized by XRD and FTIR. It was shown that the amount of HCl needed for FAp dissolution depends on acid concentration. FAp dissolution rate cannot be deduced from solubility data of P, Ca or F as the secondary reactions of CaF₂ and CaHPO₄ formation take place simultaneously. It was found that the Ap dissolution rate can be followed by pH change.

1. Introduction

The main source of phosphorus for mankind is natural apatites. The biggest part of natural calciumfluorapatite (CaFAp) is used for the production of phosphorus fertilizers and phosphoric acid via acidic digestion processes [1].

The studies of CaAp dissolution processes in acids have been comprehensively reviewed by Dorozkin up to the year 2010, and a general description of the dissolution stages has been given [2]. The dissolution of apatite is described by different models: a diffusion and kinetical model, a mono- and poly-nuclear model, a self-inhibition model, a stoichiometric/non-stoichiometric dissolution, an ion-exchange model, a hydrogen catalytic model, and an etch pit formation model, which all depend on the experiment conditions and sample composition. Most of the models have been elaborated for apatite dissolution in either slightly acidic or nearly neutral (4 < pH < 8) aqueous solutions and at relatively low concentrations. Therefore, the validity of these models for apatite dissolution in strong acids (HCl, HNO₃, H₂SO₄) at pH < 2 used in the production of fertilizers and phosphorus acid as well as in the recovery technologies of rare earth elements needs specification.

The chemical model developed for the description of Ap dissolution in strong acidic media based on indirect measurements describes the dissolution of Ap as follows [2]:

Ca₅(PO₄)₃(F,OH) + H₂O + H⁺ → Ca₅(PO₄)₃(H₂O)⁺ + HF,H₂O

(1)

2Ca₅(PO₄)₃(H₂O)⁺ → 3Ca₃(PO₄)₂ + Ca²⁺ + 2H₂O

(2)

Ca₃(PO₄)₂ + 2H⁺ → Ca²⁺ + 2CaHPO₄

(3)

CaHPO₄ + H⁺ → Ca²⁺ + H₂PO₄⁻

(4)

Net-reaction 5Ca²⁺ + 3PO₄³⁻ + F⁻,OH⁻ + 7H⁺ → 5Ca²⁺ + 3H₂PO₄⁻ + HF,H₂O

(5)

According to this model, chemical dissolution of apatite occurs step by step on the surface via intermediate formation of a monoatomic layer of some virtual compounds, like Ca₃(PO₄)₂ and CaHPO₄.

In the latest studies of the dissolution of various natural [3,4,5,6,7] and some synthetic carbonate apatites [8,9] using chemical and thermochemical methods, the main controlling factors discussed are the acid concentration, apatite particle size, time, and solid/liquid ratio. The amounts of HCl used in these experiments have been much higher than needed in a stoichiometric reaction. However, the conclusion has been made that the rapid leaching of Ca, P, and F in strong acid solutions of high concentration (up to 1 M) in a short time interval of 10–30 min is problematic for proper investigation of kinetics and detailed reaction mechanism [7,10].

The thermochemical method used for the study of kinetics of FAp dissolution in HCl at the concentration of 1–2 M and acid dose 28 mole HCl per 1 mole of FAp revealed a very fast reaction, which is affected by apatite composition [9]. The reaction of apatite decomposition is generally described by the following equation

Ca₅(PO₄)₃F + 10H⁺ + 10Cl⁻ → 5Ca²⁺ + 3H₃PO₄ + 10Cl⁻ + HF

(6)

Based on the activation energies, the dissolution mechanism of Ca and P in HCl is stated to be mainly controlled by chemical kinetics [9,11]. However, a conclusion that the dissolution is limited by the product layer diffusion process [12] has also been made. In addition, the counteraction of the reaction products of apatite dissolution in technological processes at these relatively high saturation conditions should be considered as well.

Thus, there is no clear understanding of how to describe the rate of apatite dissolution at its initial stage in strong acid solutions of high concentrations.

The aim of this study was to find dependence between pH change and the apatite dissolution rate in fluorapatite dissolution reactions by thermodynamic calculations and experiments with synthetic flourapatite at near-stoichiometric conditions in hydrochloric acid media.

2. Results and Discussion

2.1. Thermodynamic Calculations

The thermodynamic calculations (HsC) were performed for HCl solutions with concentrations from 0.05 to 2 M adding FAp by the step of 0.02 mol and with different compositions of the solid phase formed in the reaction: HsC1—with the formation of CaF₂, and HsC2—with the formation of CaF₂, together with CaHPO₄·2H₂O. In earlier studies, the formation of CaHPO₄ has been proposed [2], and in the system also with H₃PO₄, the formation of CaF₂ has been found [13,14]. The results of the calculations with 1 M HCl are shown in Figure 1 and Figure 2.

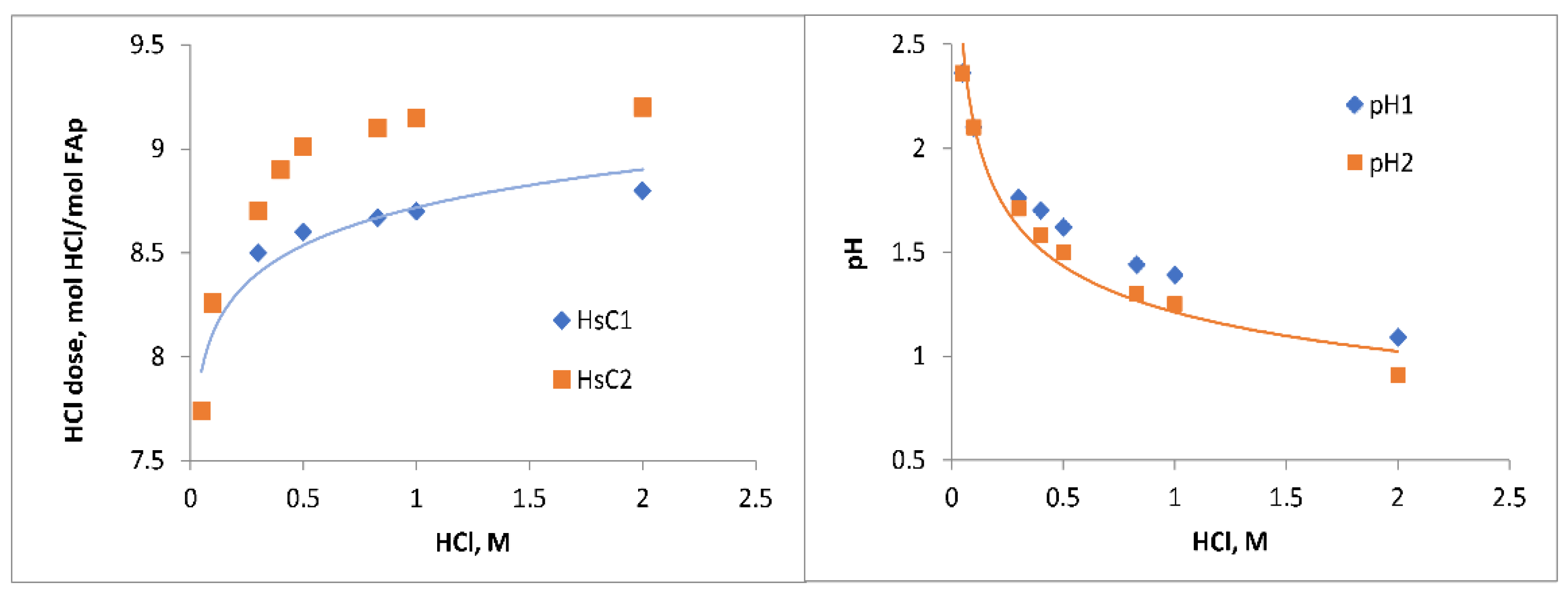
In Figure 1, the amounts (moles) of ions in aqua and solid phases in the case of 1 M HCl are presented depending on the amount of FAp added and on the solid-phase composition assumed. At the stoichiometric point (maximum of phosphorus dissolved) the aqua phase contains Ca²⁺, H⁺, Cl⁻, H₂PO₄⁻ ions and H₃PO₄ and CaCl₂ aqua complexes. The latter is formed in the amount below 0.15 mol that is not visible in a figure. The amount of HCl needed for the dissolution of 1 mole of FAp is 8.7 (HsC1) or 9.0 moles (HsC2).

The amount of HCl needed for the dissolution of 1 mole of FAp (and later the acid dose) changes from 7.6 to 8.8 (HsC1) or 9.2 (HsC2) according to the increase in HCl concentration from 0.05 to 2 M (Figure 2) and the selected reaction products. This phenomenon can be explained by the change in H⁺ consumption depending on the equilibrium of H₃PO₄ and HPO₄²⁻ species in solution.

The formation of CaF₂ takes place at any HCl concentration. CaHPO₄·2H₂O formation is possible at HCl concentrations above 0.2 M. In Figure 2 it is seen that at HCl concentrations above 0.2 M and in the case of CaF₂ formation alone, the dose of HCl is 0.4–0.5 moles lower than in the case of simultaneous formation of CaHPO₄·2H₂O and CaF₂.

The pH value differs slightly depending on the solid phase formed (Figure 2) as the amount of CaHPO₄·2H₂O is small. According to the calculations, pH increases in correlation with the quantity of apatite dissolved (Figure 1). In real conditions, the amount of apatite dissolved increases with time, and therefore, it could be described by the change of pH.

2.2. Experiments with Synthetic FAp

The experiments of FAp dissolution in HCl at different concentrations and doses (HCl/FAp mole ratio) were performed under the conditions described in

Table 1. pH and solubility of P₂O₅, CaO, and F in the experiments and by calculations.

Exp. No.	Calculation Mode or Exp. Time	HCl Dose by HsC Calculation, mol	HCl/FAp Mole Ratio	pH	Solubility, %
					P2O5	CaO	F
HCl Concentration 0.05 M
		7.94
	HsC		12.42	1.87	100	93.4	32.8
1	5 h		12.42	1.90	100	95.6	95.97
	HsC		10.24	2.01	100	92.4	37.90
2	5 h		10.24	2.08	100	98.2	83.86
HCl Concentration 0.1 M
		8.26
	HsC		10.2	1.82	100	91.4	13.5
5	5 h		10.20	1.79	99.40	98.5	26.22
	3.5 months a			-	-	98.2	24.21
	HsC		8.62	2.02	100	90.3	5.30
6	5 h		8.62	1.95	99.71	97.5	12.10
HCl Concentration 0.5 M
	HsC 1	8.55
	HsC 2	9.01
	HsC		12.98	0.95	100	91.4	14.10
7	5 h		12.98	0.98	100	93.1	17.86
	HsC 1		9.99	1.25	100	90.5	5.30
	HsC 2		9.99	1.30	100	90.5	4.50
8	5 h		9.99	1.17	100	92.8
	3.5 months a			-	-	100 ± 2	17.00
	HsC 1		6.47	1.65	77.4	70.0	1.35
	HsC 2		6.47	1.60	60.9	66.7	1.55
9	5 h			1.85	99.42	-	0.86
HCl Concentration 0.83 M
	HsC 1	8.7
	HsC 2	9.01
	HsC 1		8.24	1.48	96.5	87.0	1.24
	HsC 2		8.24	1.34	88.1	83.0	1.71
10	24 + 72 h a		8.24	1.25	96.62	88.3	16.71
HCl Concentration 1 M
	HsC 1	8.7
	HsC 2	9.01
	HsC 1		10.21	0.92	100	90.4	4.50
11	60 min		10.21	0.72	95.24	92.1	29.11
	HsC 1		8.71	1.39	100	90.1	1.3
	HsC 2		8.71	1.28	94.80	87.0	1.67
12	5 + 45 h a		8.71	1.09	100 ± 2	97.6	11.53

^a storage time without mixing.

. The minimum time used was limited by the very fine solid phase separation conditions. The pH value, Ca, P, and F content in the solutions were compared with the HsC calculations. pH measurements of the HCl solution mixtures with synthetic FAp revealed that the pH change is very fast and the speed increases with the increase in acid concentration (Figure 3). The value of pH stabilizes at 3000 s in 0.05 M HCl but in 0.5 M solution only 1000 s is needed for stabilization, and in 1 M solution even 600–700 s. These results are in accordance with the thermochemical measurements performed in 3% (1 M) HCl with synthetic apatites described in [9,12].

The pH values measured in the experiments at longer times are closer to the calculated values and, at that, even closer in less-concentrated solutions (Table 1). The latter can be explained by the lack of precision of activity values in the calculations of strong acids. Additionally, the adsorption processes at solid surfaces are not taken into account in the equilibrium calculations that could slightly change the hydrogen ion consumption profile and rate in real experiments.

The solubility of F and Ca determined in the experiments is higher than calculated, but these values decrease with time. The difference is bigger for diluted HCl solutions (0.05–0.1 M) and in the case of HCl doses above stoichiometric. Solubility of Ca and F is lower than calculated if the dose of HCl is below stoichiometric, but P solubility is higher, which indicates the formation of the secondary solid phase CaF₂. Therefore, the composition of the soluble part is affected not only by the acid concentration and time but also by the acid dose.

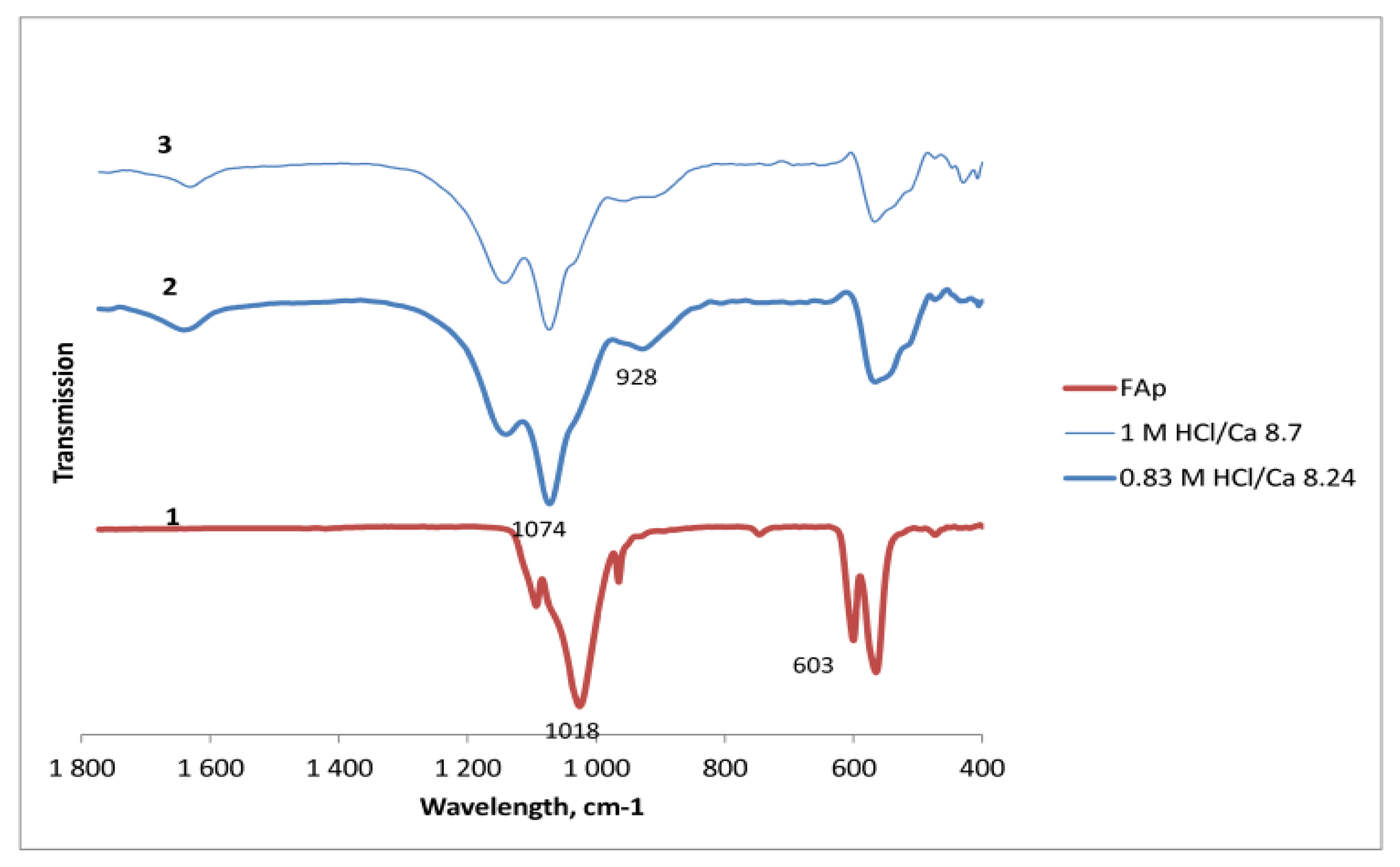
The XRD analysis of the solid phase separated after the reaction approved the formation of CaF₂ (

Table 2. Solid phase composition in the reaction of FAp with HCl by XRD analysis.

Exp. No.	Exp. Time	HCl Conc., M	HCl/FAp Mole Ratio	Solid Phase Composition, %
				CaF2	FAp	CaHPO4
5	5 h + 3.5 months a	0.10	10.20	100	0	0
8	5 h + 3.5 months a	0.50	9.99	98	nd	0
9	10 min b	0.50	6.47	6.7	93	nd
	60 min	0.50	6.47	8	92	nd
	300 min	0.50	6.47	8	92	nd
	24 h	0.50	6.47	22	74	4
	72 h	0.50	6.47	19	81	nd
10	24 + 72 min a	0.83	8.24			FTIR
11	10 min b	1.00	10.21	11	89	nd
13	10 min b	1.00	6.50	15	80	2
	60 min	1.00	6.50	11	83	7
	300 min	1.00	6.50	11	88	1
	24 h	1.00	6.50	28	72	1
	72 h	1.00	6.50	35	58	7
12	5 + 45 h a	1.00	8.71	23	77	FTIR

^a storage time without mixing; ^b here filtration time exceeded the mixing time.

, Figure 4). In the case of an HCl dose above 10, the solid phase consisted only of CaF₂. In the experiments at an almost stoichiometric amount of HCl (exp. 10 and 12), some amorphous phase is also detected in the solid part that, according to FTIR analysis, could be attributed to hydrogen phosphate. In Figure 5, the absorption bands at 1140, 1074, and 928 cm⁻¹, characteristic of H-PO₄ vibrations [15], are seen. At the same time, the absorption bands of PO₄ in FAp at 1089, 1018, and 603 cm⁻¹ have been shifted or disappeared.

In the experiments with HCl/FAp = 6.5 (below stoichiometric), the solid phase consisted of unreacted FAp and CaF₂ (Table 2). The amount of CaF₂ increased with time.

As a result of the experiments, the formation of CaF₂ was proved. Speed of CaF₂ crystallization depends on HCl concentration and dose, being higher at lower acid dose and higher concentration (Table 2). CaF₂ was found in the HCl solutions of 0.1 M only after some months, whilst in the experiments with 1 M HCl, it was found after only 10 min. In the reactions describing the dissolution of FAp in HCl discussed in [1,2,3,4,5,6], the formation of CaF₂ is not mentioned, but it is mentioned in the studies with H₃PO₄ [13,14]. Possibly, CaF₂ is not found in weak acid as the formation of it is much slower than FAp dissolution, but it occurs with remarkable speed at HCl concentrations of 5–25% used in the real phosphate rock treatment technology.

Crystalline CaHPO₄·2H₂O was not detected, but a transition phase containing Ca-HPO₄ groups was present in the mixtures with a stoichiometric dose of HCl, as was proposed by Dorozkin [2]. It was also not noticed in previous studies in which the HCl dose exceeded the stoichiometric dose, because in these conditions CaHPO₄ dissolves.

Thus, by the information given above, it was shown that the proposed earlier net reaction for FAp dissolution in acids is a simplified description of the process.

2.3. Kinetic Analysis

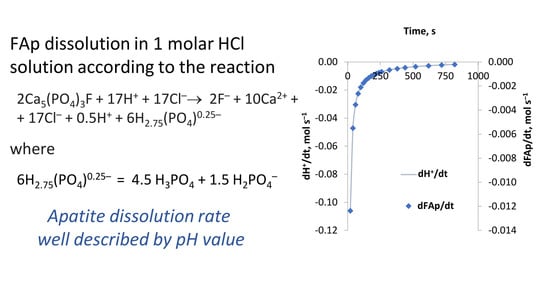
According to HsC equilibrium calculations where apatite reacts with 1 M HCl solution (amounts of species less than two orders of magnitudes are eliminated), the dissolution process at the stoichiometric point of apatite dissolution can be described by the following equation:

2Ca₅(PO₄)₃F + 17H⁺ + 17Cl⁻ → 2F⁻ + 10Ca²⁺ + 17Cl⁻ + 0.5H⁺ + 6H_2.75(PO₄)^0.25−

(7)

with

6H_2.75(PO₄)^0.25− = 4.5H₃PO₄ + 1.5H₂PO₄⁻

(8)

The reactions above fulfill the elements and charge balances. It is also assumed that new solid compounds CaF₂ and CaHPO₄, presented in the paper earlier, are formed slowly, and that in the initial stage only apatite dissolution Reaction (7) occurs.

For the batch reactor, the change in the total number of H⁺ -ions n_H+ can be expressed as:

$$\frac{d{n}_{{\mathrm{H}}^{+}}}{dt}=r_{{\mathrm{H}}^{+}}V,$$

(9)

$$\frac{d(V M_{{\mathrm{H}}^{+}})}{dt}=r_{{\mathrm{H}}^{+}}V,$$

(10)

giving

$$\frac{d M_{{\mathrm{H}}^{+}}}{dt}=r_{{\mathrm{H}}^{+}},$$

(11)

where

$r_{{\mathrm{H}}^{+}}$—consumption rate of H⁺ ions, mol s⁻¹ L⁻¹

V—solution volume, L

$M_{{\mathrm{H}}^{+}}$— molar concentration of hydrogen ions, mol L⁻¹

and according to Reaction (7), the reaction rates for apatite dissolution and ions formed are $\frac{d M_{\mathrm{FAp}}}{dt}=r_{\mathrm{FAp}}=\frac{2}{17}{r}_{{\mathrm{H}}^{+}}$, $\frac{d M_{{\mathrm{Ca}}^{2+}}}{dt}=r_{{\mathrm{Ca}}^{2+}}=\frac{10}{17}{r}_{{\mathrm{H}}^{+}}$, $\frac{d M_{{\mathrm{F}}^{-}}}{dt}=r_{{\mathrm{F}}^{-}}=-\frac{2}{17}{r}_{{\mathrm{H}}^{+}}$, and $\frac{d M_{{\mathrm{H}}_{2.75}{({\mathrm{PO}}_4)}^{0.25-}}}{dt}=r_{{\mathrm{H}}_{2.75}{({\mathrm{PO}}_4)}^{0.25-}}=-\frac{6}{17}{r}_{{\mathrm{H}}^{+}}$.

Here, the apatite (single solid phase) dissolution reaction rate is calculated in mol s⁻¹ L⁻¹, and later its concentrations are calculated as mol L⁻¹. From the experimental data and calculations, it can be shown that the pH value of the solution is suitable for the description of the kinetics of the apatite dissolution process.

The pH change from the experiment with 1 M HCl solution (dose 8.7 mol HCl/1 mol apatite) is presented in Figure 3. In the calculations, the averaged datapoint values were used. The initial amount of apatite was 1.39 mmols, liquid volume was equal with the added 1 M HCl volume, 12.35 mL, and the temperature was 25 °C.

The exact concentrations of H⁺ ions can be calculated from the pH values knowing the activity coefficient ^γ_H⁺. On the basis of HsC calculations, the molar activity coefficient values are related to pH by the following equation (R = 0.99)

$${\gamma }_{{\mathrm{H}}^{+}}=0.843-0.032 \mathrm{pH}$$

(12)

and from the definition of pH

$$\mathrm{pH}=-\log ({\gamma }_{{\mathrm{H}}^{+}}{M}_{{\mathrm{H}}^{+}})$$

(13)

the more exact molar concentration of hydrogen ions is then expressed as

$$M_{{\mathrm{H}}^{+}}={10}^{-\mathrm{pH}-\log ({\gamma }_{{\mathrm{H}}^{+}})}$$

(14)

The time-change of the molar concentration of H⁺ ions in the time interval from 10 s to 820 s was fitted with numerous different functions and the mathematically most appropriate (regression coefficient R = 0.99) was found to be

$$\ln (M_{{\mathrm{H}}^{+}})=a+b \ln (t)+c {[\ln (t)]}^2$$

(15)

with a = 1.94; b = −1.131; c = 0.076.

In this experiment, after 820 s, the pH remains constant. From Equation (15), the molar concentration of H⁺ ions at any time moment t is

$$M_{{\mathrm{H}}^{+}}=\exp \{a+b \ln (t)+c {[\ln (t)]}^2\}$$

(16)

The consumption rate of H⁺ ions can now be given as

$$r_{{\mathrm{H}}^{+}}=\frac{d{M}_{{\mathrm{H}}^{+}}}{dt}=(\exp \{a+b \ln (t)+c {[\ln (t)]}^2\}) (\frac{b}{t}+2c\frac{\ln (t)}{t})$$

(17)

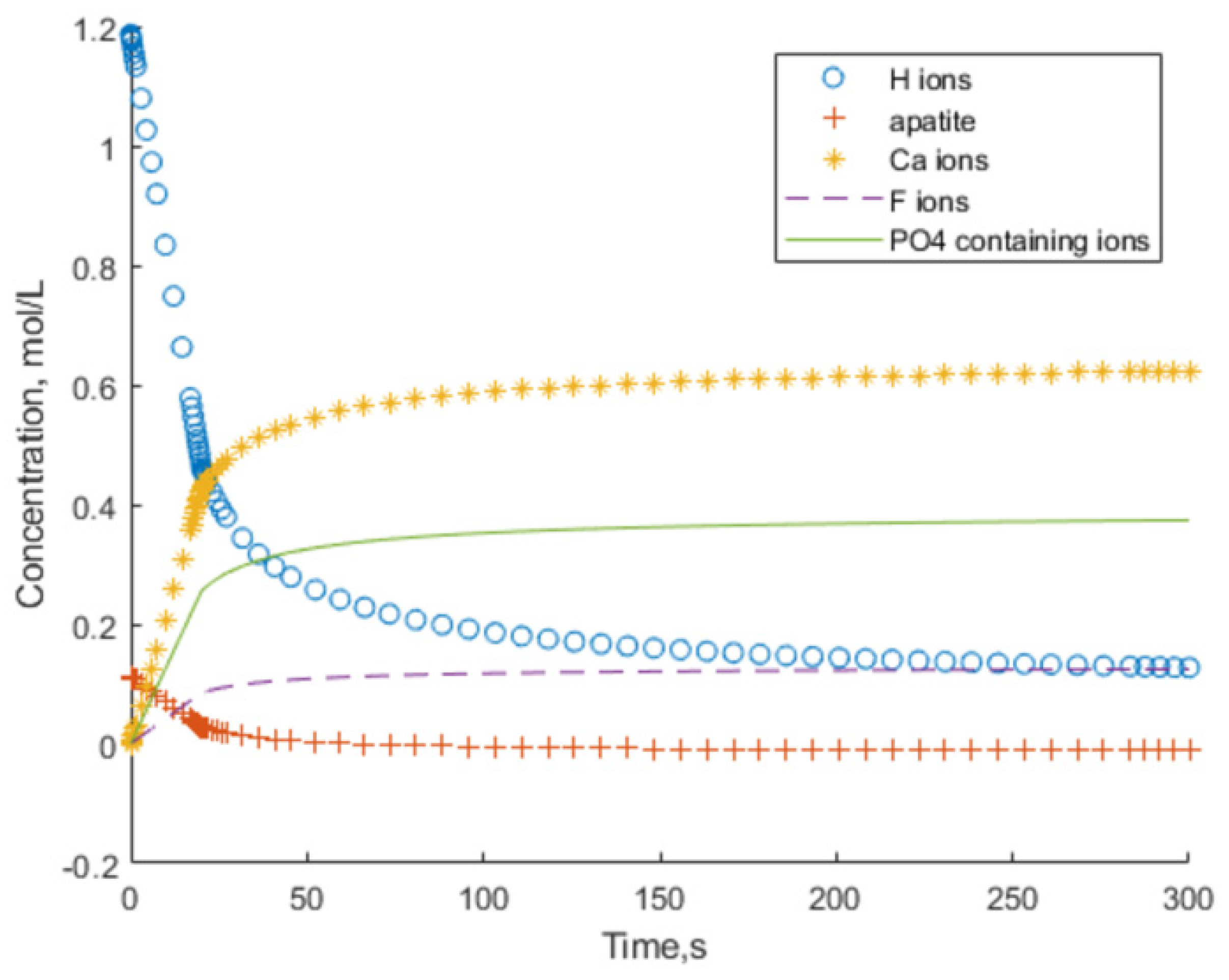
Using the Relationship (11) the dissolution rate of apatite and the concentrations of H⁺ and other ions (Ca²⁺, F⁻, H_2.75(PO₄)^0.25−) can now be calculated by solving the ordinary differential equation system (Equations (10) and (11)). The initial conditions are: t = 0 s: M_H+ = 1.186, M_FAp = 0.113, M_Ca2+ = 0, M_F⁻ = 0, M_H2.75(PO4) = 0 (mol/L). The very first seconds are excluded as not suitable for Equation (15). The first period of dissolution, until 300 s, is used for the graphical illustration of the calculation results, shown in Figure 6.

The other parameters of the solution (P, Ca, and F concentrations) are affected by the secondary reactions of the formation of CaF₂ and CaHPO₄, and therefore, the only comparable parameter to describe the FAp dissolution kinetics in calculations and experiments is pH.

3. Materials and Methods

FAp was synthesized by precipitation from aqueous solution. Two solutions—one containing Ca(NO₃)₂ (0.5 M), the other containing (NH₄)₂HPO₄ (0.6 M) and NH₄F (0.2 M)—were added simultaneously to NH₄OH–NH₄NO₃ solution under N₂ flow and 80 °C temperature. The pH of the solution was adjusted and maintained at 9. The precipitate was washed thoroughly with distilled water and dried after separation of the mother liquor and finally calcined at 800 °C. The chemical composition of the FAp obtained was P₂O₅ 41.35, CaO 54.11, and F 3.47%. The FTIR and XRD spectra of the product corresponded to pure FAp (ICDD, DB card nr 00-060-0667). Ap unit cell parameters were a = 9.3705(16) and c = 6.8818(15) Å.

All reagents for synthesis and analyses were bought from ACROS organics (Acros Organics B.V.B.A., Fair Lawn, NJ, USA), Sigma-Aldrich (St. Louis, MO, USA), and Merck (Merck & Co., Kenilworth, NJ, USA) and used as-bought.

The surface area of the FAp (3.6 m²∙g⁻¹) was measured by the BET-method (adsorptive gas N₂, carrier gas He, heating temperature 150 °C) using a sorptometer KELVIN 1040/1042 (Costech International, Tallinn, Estonia).

In the batch experiments of the dissolution process, different amounts of FAp were added to the exactly measured amounts of 0.05, 0.1, 0.5, and 1.0 M HCl solution and the suspension was mixed in an overhead mixture up to 72 h or by an automatic titrator that allowed to follow the change of pH in short time intervals up to 5 h. After solid-phase separation, the composition of the solution was analyzed. The solid-phase composition was determined by XRD and FTIR spectroscopy.

pH was measured with Mettler Toledo electrode DG-117 Water, calibrated with Mettler Toledo pH buffer solutions (2.0; 4.01; 7.00), and connected to a Mettler Toledo automatic titrator T90 (Greifensee, Switzerland) in the propeller-mixed suspension every 20 s. In the solutions after solid-part separation by filtration, the concentration of Ca²⁺ ions was determined by atomic absorption spectrometry (Spectra AA 55B, Varian BV, Varian Australia Pty Ltd., Belrose, Australia) and the concentration of phosphorus was determined spectrophotometrically (Biochrom Libra S70PC, Cambridge, UK) as the phosphomolybdate yellow complex (λ = 430 nm). The fluoride concentration in the solution was determined by an F-selective electrode (Mettler Toledo, Greifensee, Switzerland).

XRD patterns were obtained with a difractometer Rigaku Ultima IV, Wilmington, MA, USA (40 kV, 40 mA, scan speed 5 deg min⁻¹, step 0.0200 deg, K-beta filter). The cell dimensions were determined by Rigaku PDXL 2 software.

FTIR spectra were recorded with a Bruker 55/S/NIR FTIR ATR spectrometer (Bremen, Germany) as an average of 32 scans at a resolution of 4 cm⁻¹.

Computational Methods

An equilibrium composition was calculated using HSC Chemistry^® software version 10.0.1.8 (Outotec, Finland) which uses the Gibbs energy minimization method. The input species for calculations are given in

Table 3. The input species for calculations of the equilibrium composition.

Phase	Formula
Mixture	N2(g); HCl(g); HF(g)
Aqua	H2O; OH(−a); HCl(a); Cl(−a); CaCl2(a); F(−a); HF(a);
	HPO4(−2a); H3PO4(a); H2PO4(−a); PO4(−3a)
	H(+a); Ca(+2a)
Pure solids	Ca5(PO4)3F; CaF2(s); CaHPO4·2H2O

, their thermochemical data was taken from the program database.

In the simulations of the dissolution process, Matlab R2020b (update 4), MathWorks, Inc., Natick, MA, USA was used.

4. Conclusions

The correlation between the pH change and the apatite dissolution rate depending on HCl concentration was studied by thermodynamic calculations and experiments with synthetic fluorapatite.

A mathematical description of the studied dissolution process was composed, according to which the kinetics of dissolution can be determined by measuring the pH values of the system.

According to thermodynamics calculations, the stoichiometric amount of HCl needed for FAp dissolution depends on acid concentration. The experiments showed, as expected, that the dissolution of FAp is very fast and depends also on acid concentration.

The formation of secondary solid phases (CaF₂ and CaHPO₄) in the FAp-HCl system was proved and should be considered as a possible prerequisite for diffusion limitation at dissolution.

The reaction rate cannot be deduced from the calcium, fluorine, and phosphorus content of the solution as the secondary reactions of CaF₂ and CaHPO₄ formation (notably at higher acid concentration) take place simultaneously.

The next stage of the studies will involve natural apatites.