Molecular S = 2 High-Spin, S = 0 Low-Spin and S = 0 ⇄ 2 Spin-Transition/-Crossover Nickel(II)-Bis(nitroxide) Coordination Compounds
Abstract
1. Introduction
2. Results and Discussion
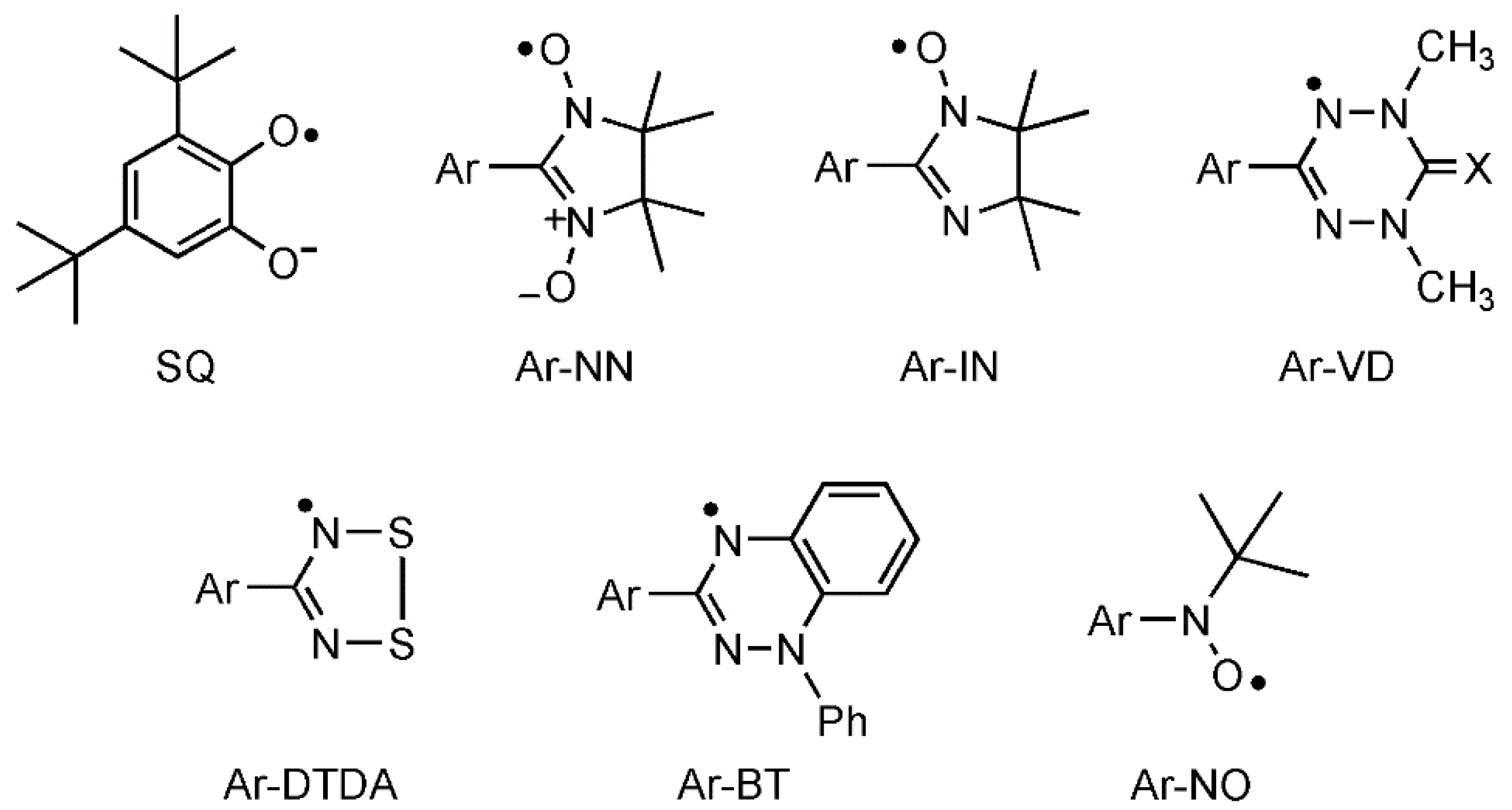
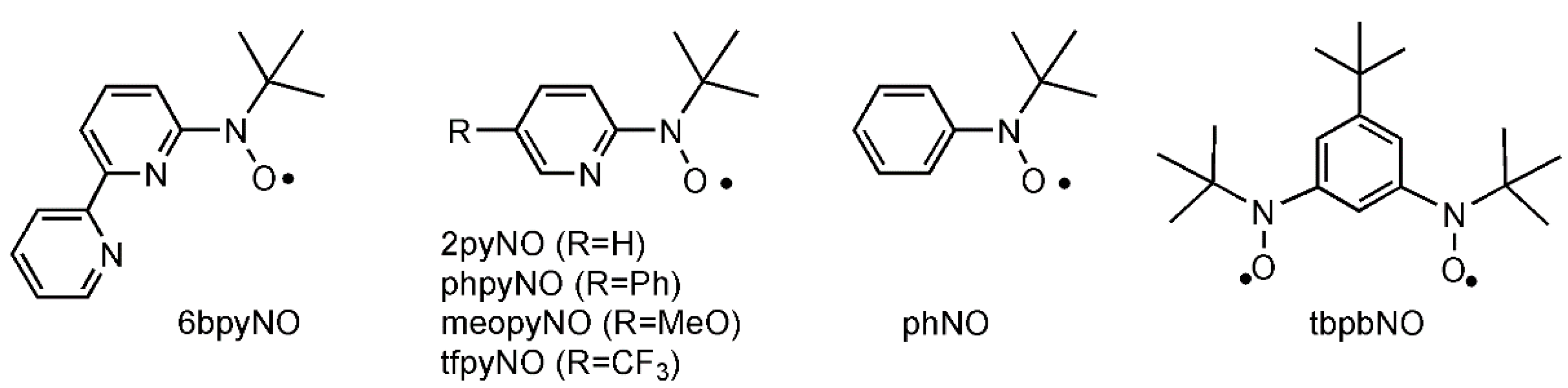
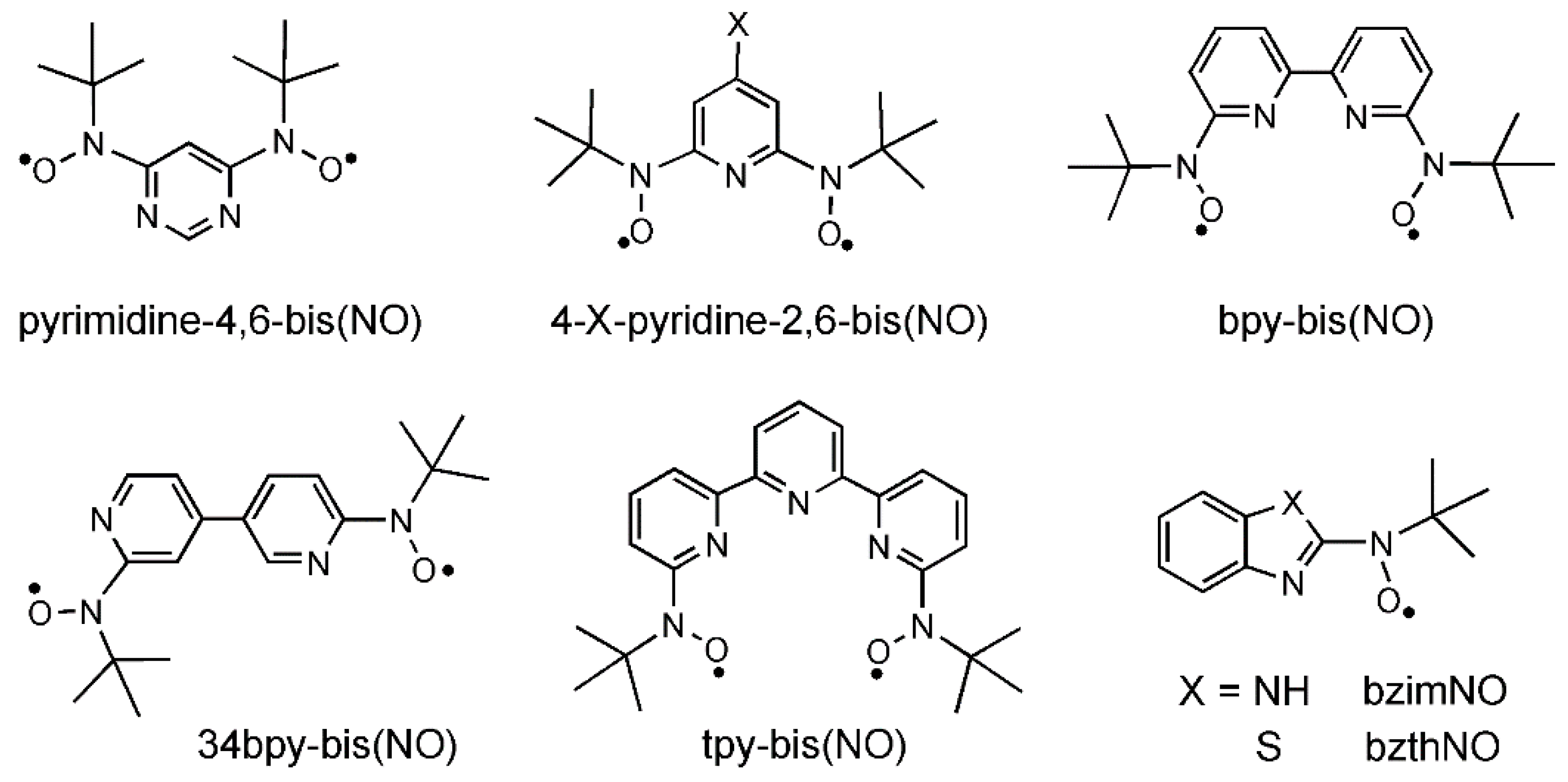
2.1. Advantage of Nitroxides
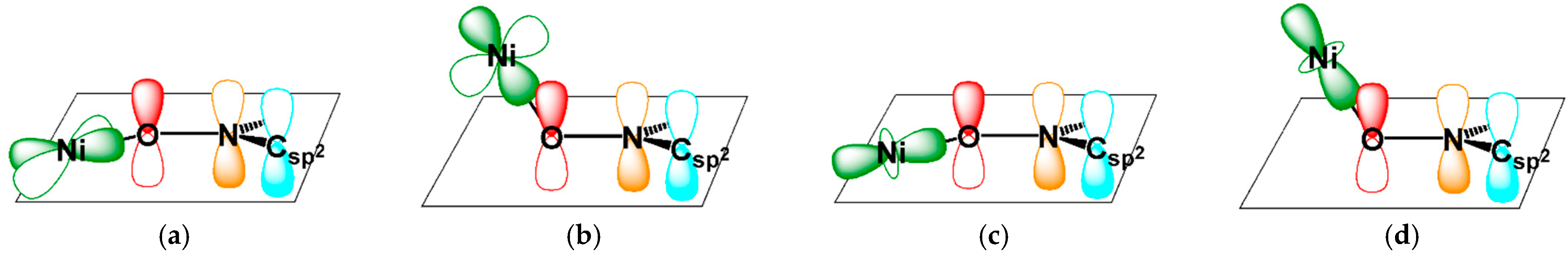
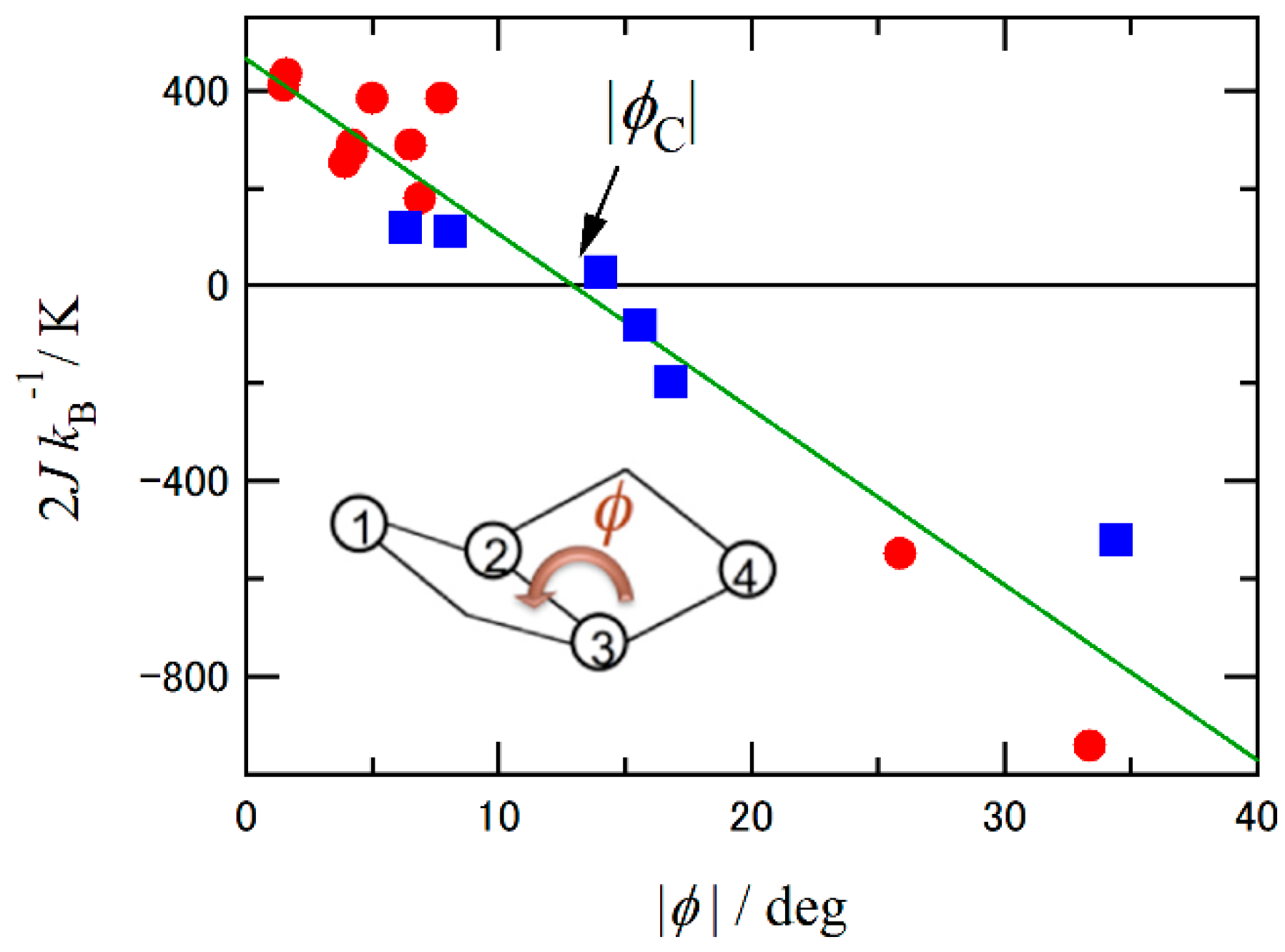
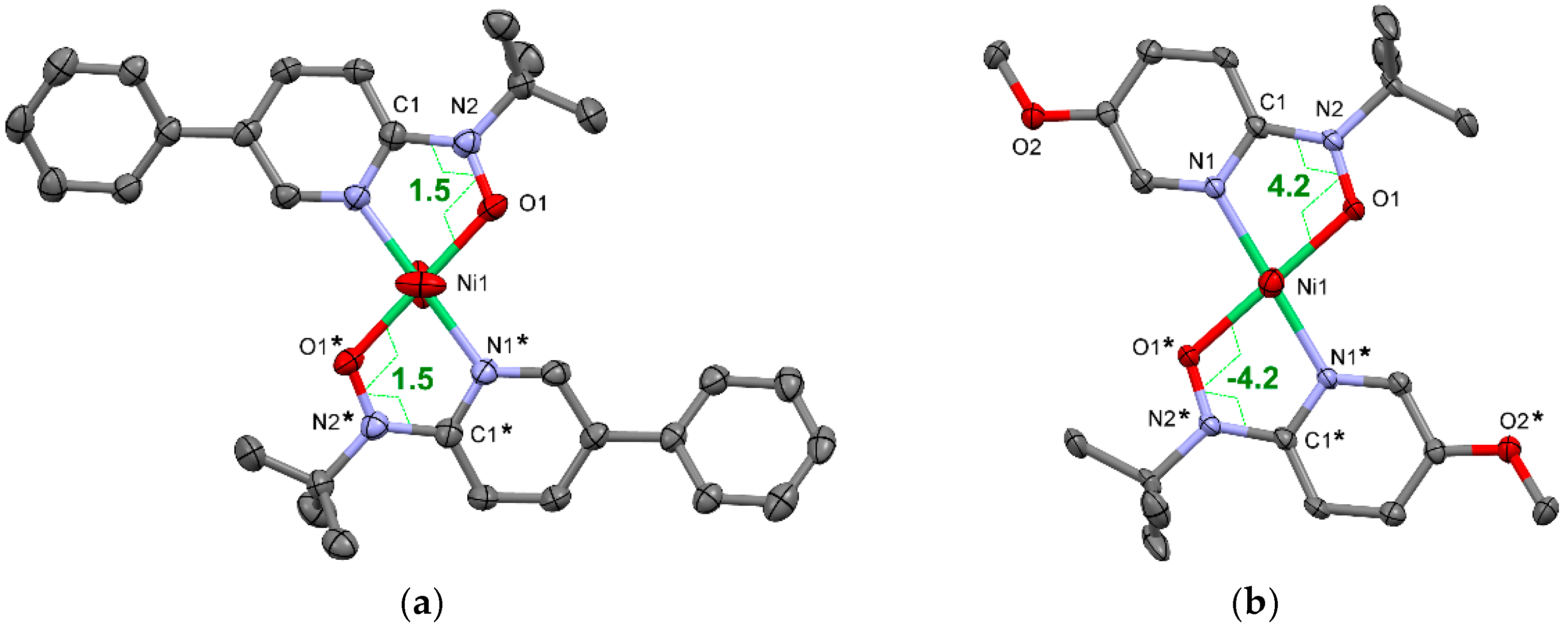
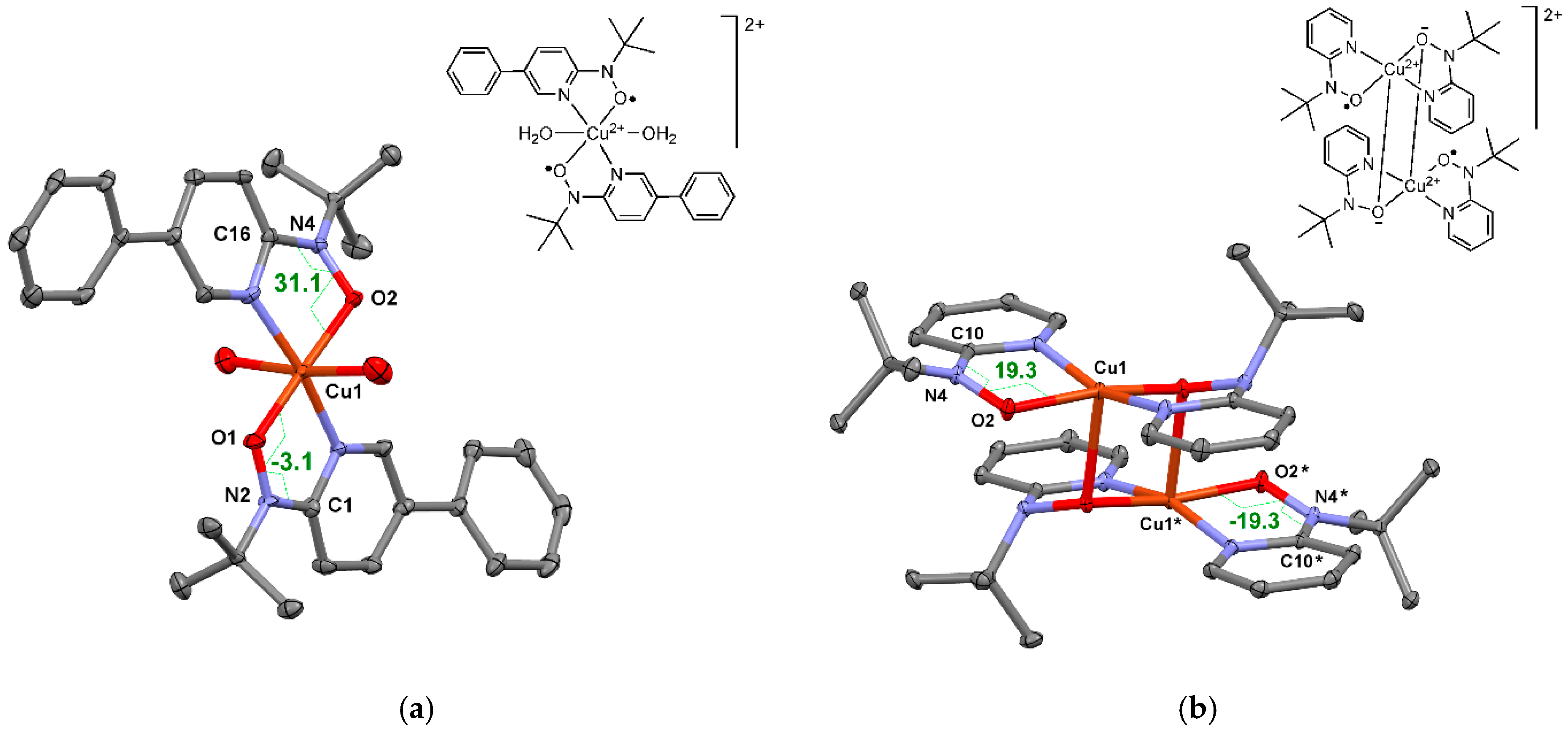
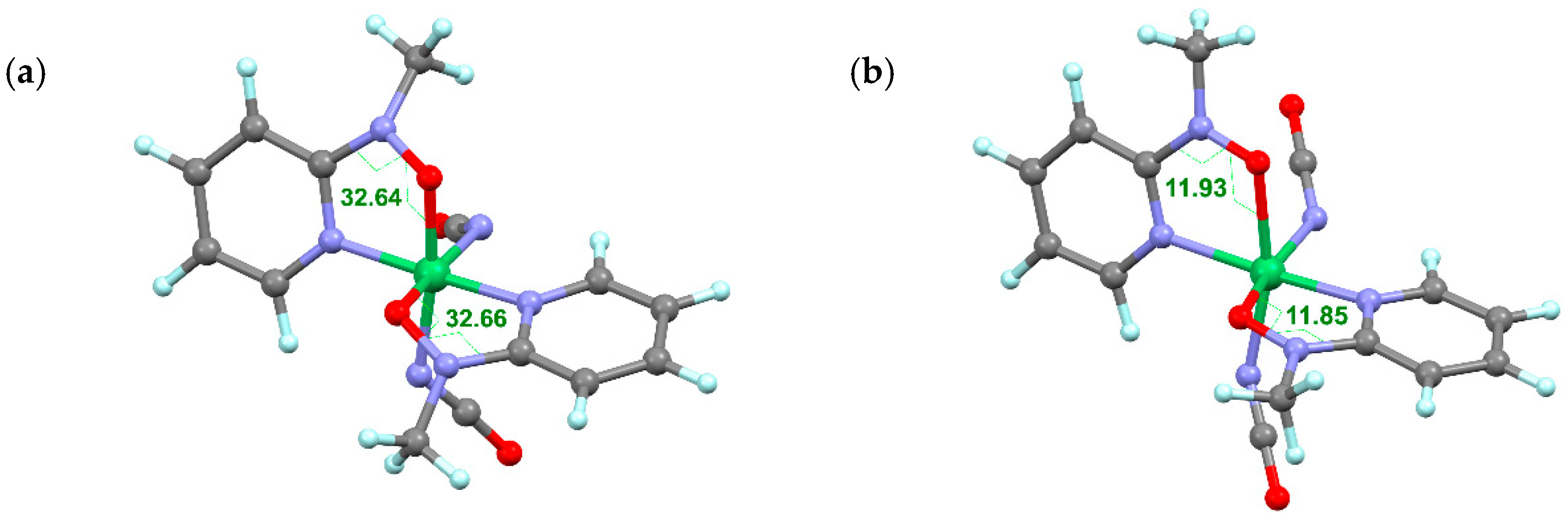
2.2. Magneto-Structural Relationship and Molecular Design
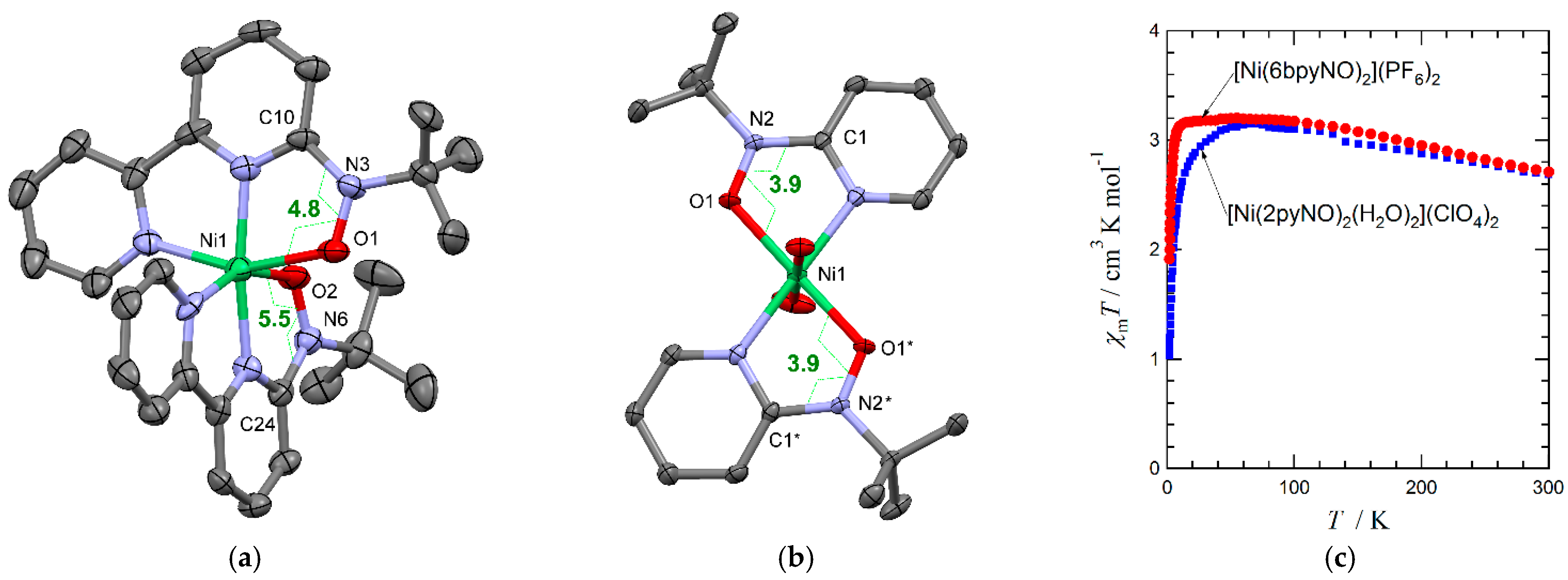
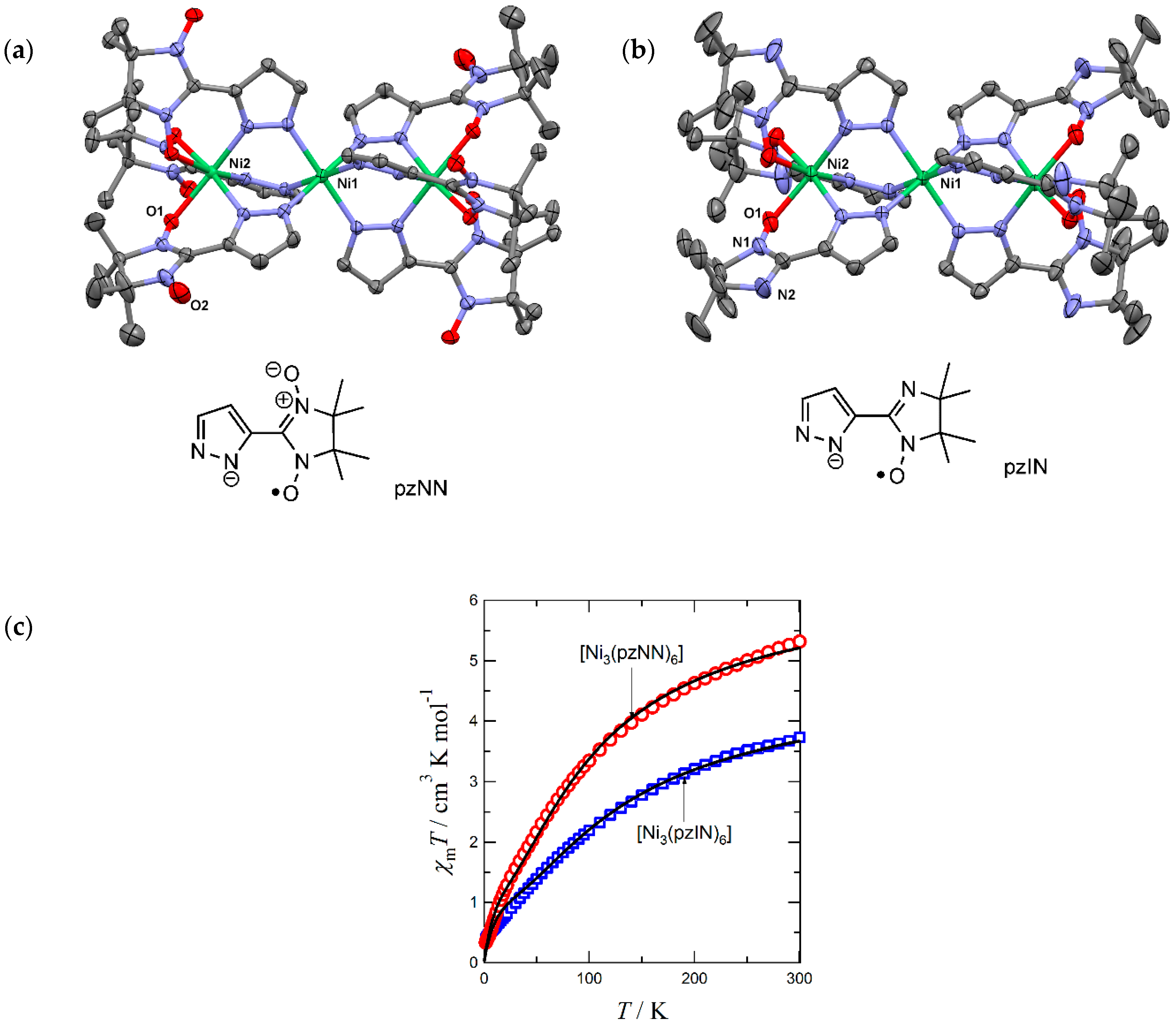
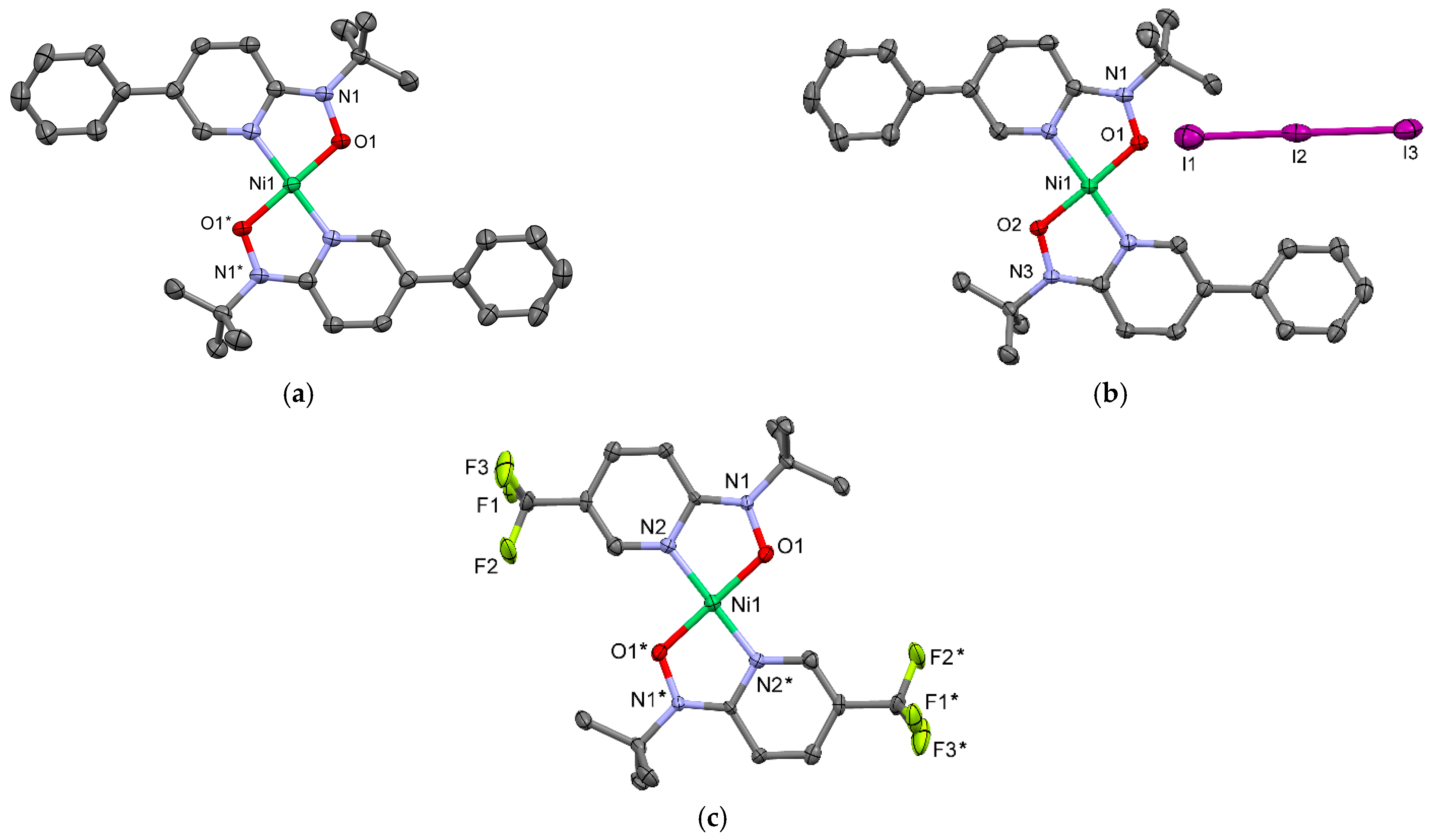
2.3. High-Spin Molecules
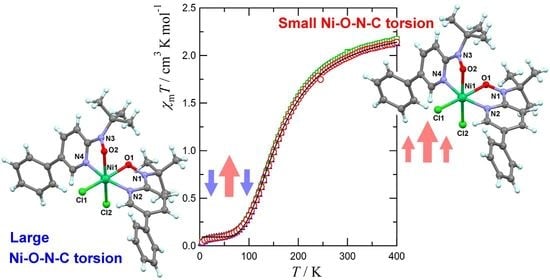
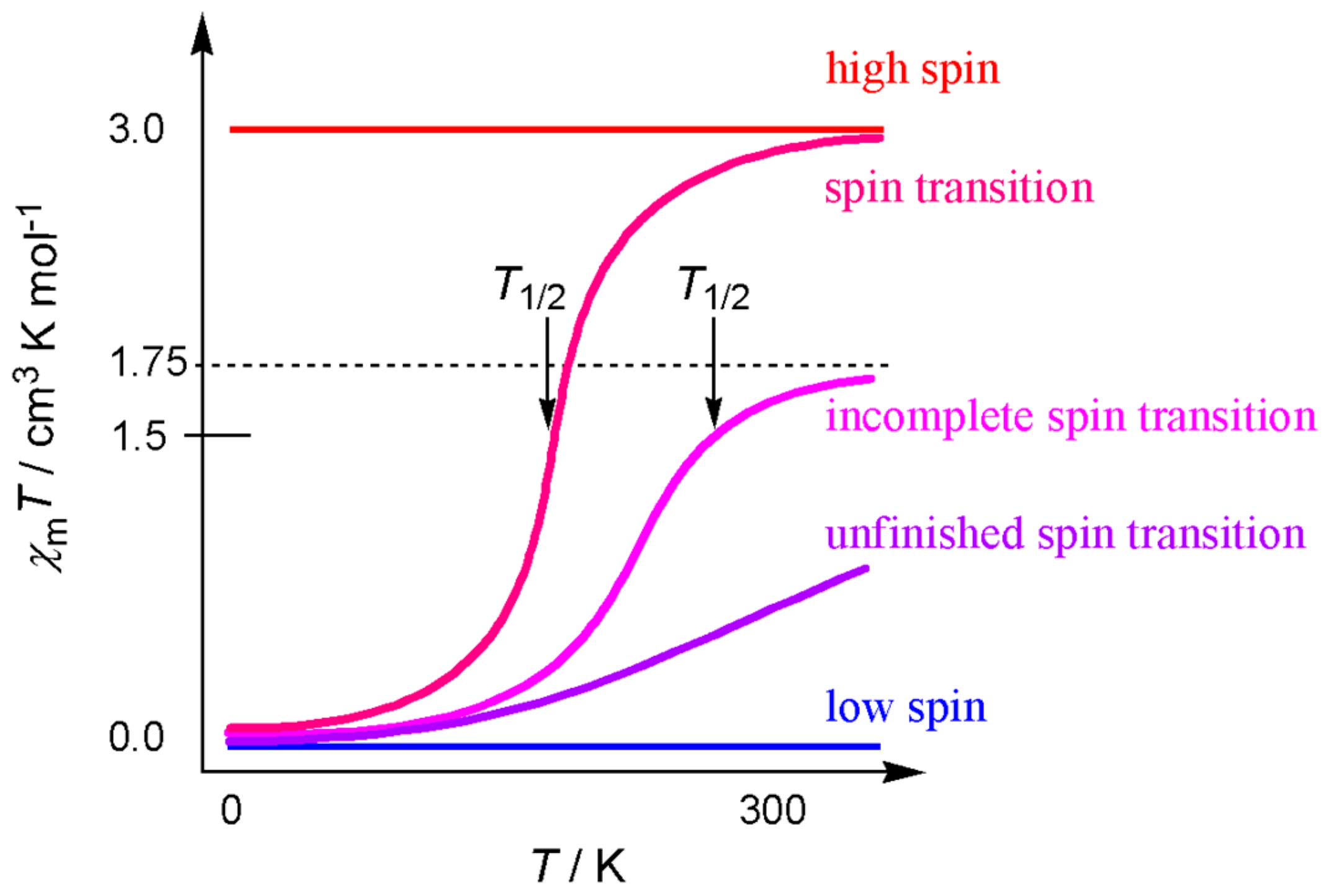
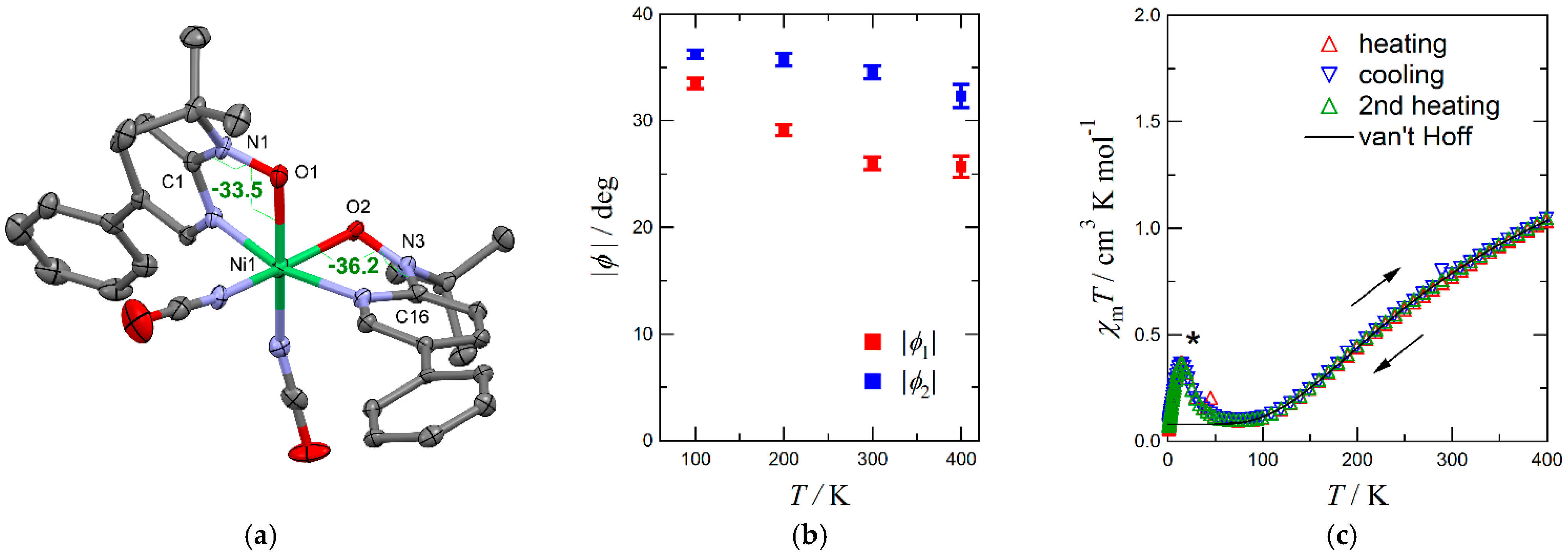
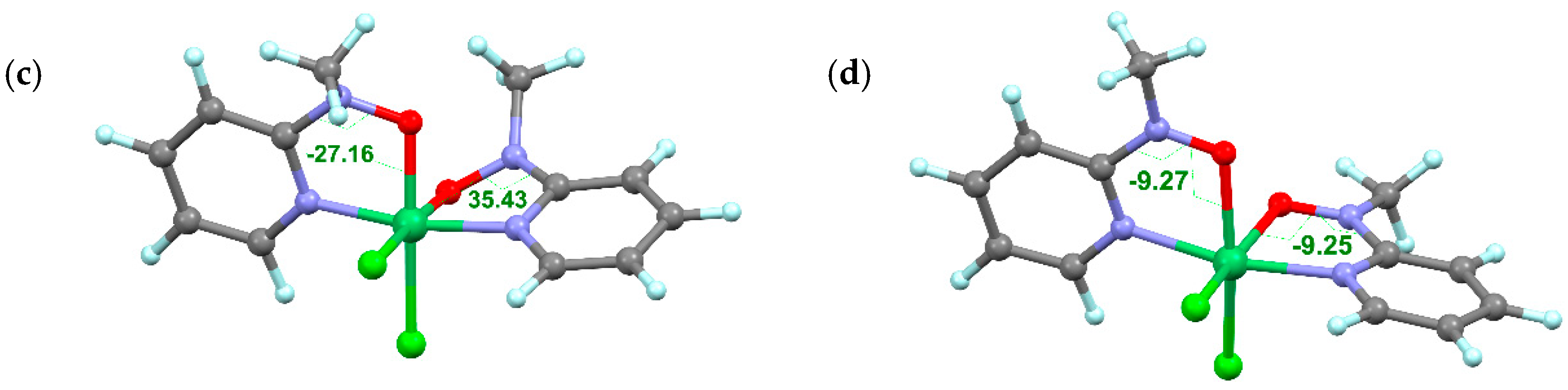
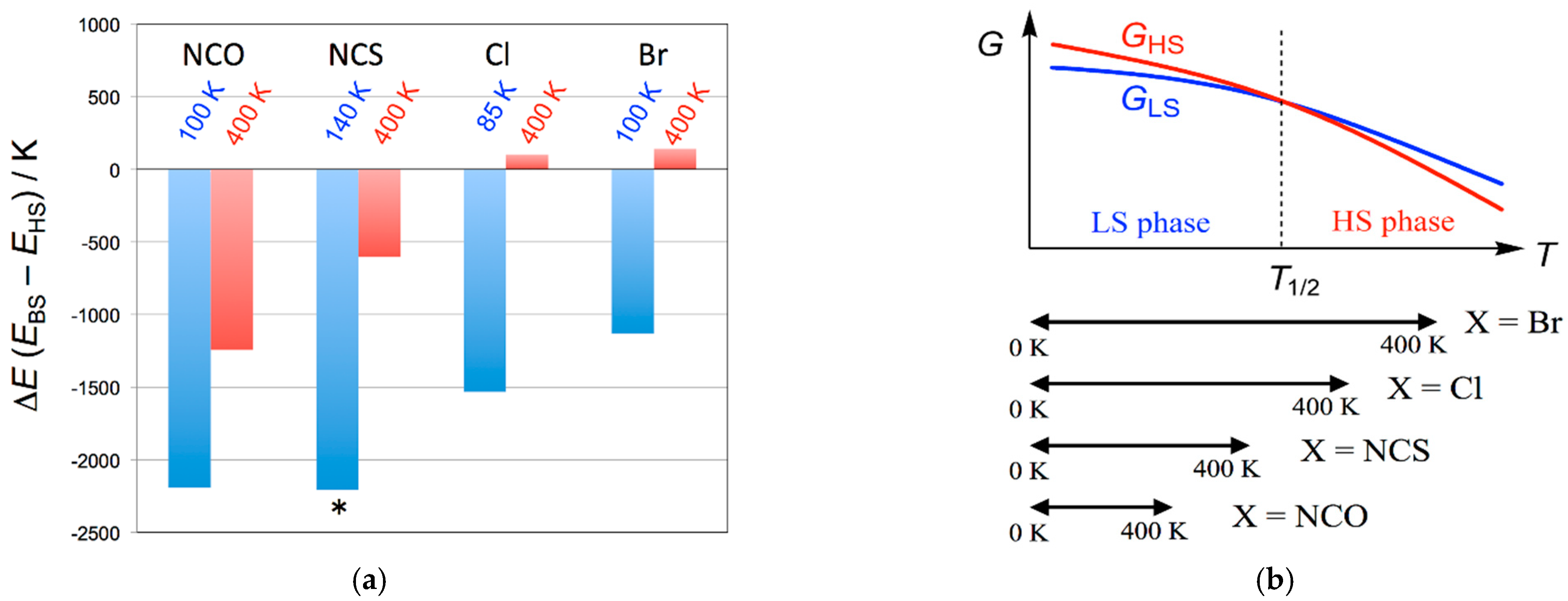
2.4. Spin-Transition Molecules
2.5. Unfinished Spin-Transition Molecules
2.6. Low-Spin Molecules
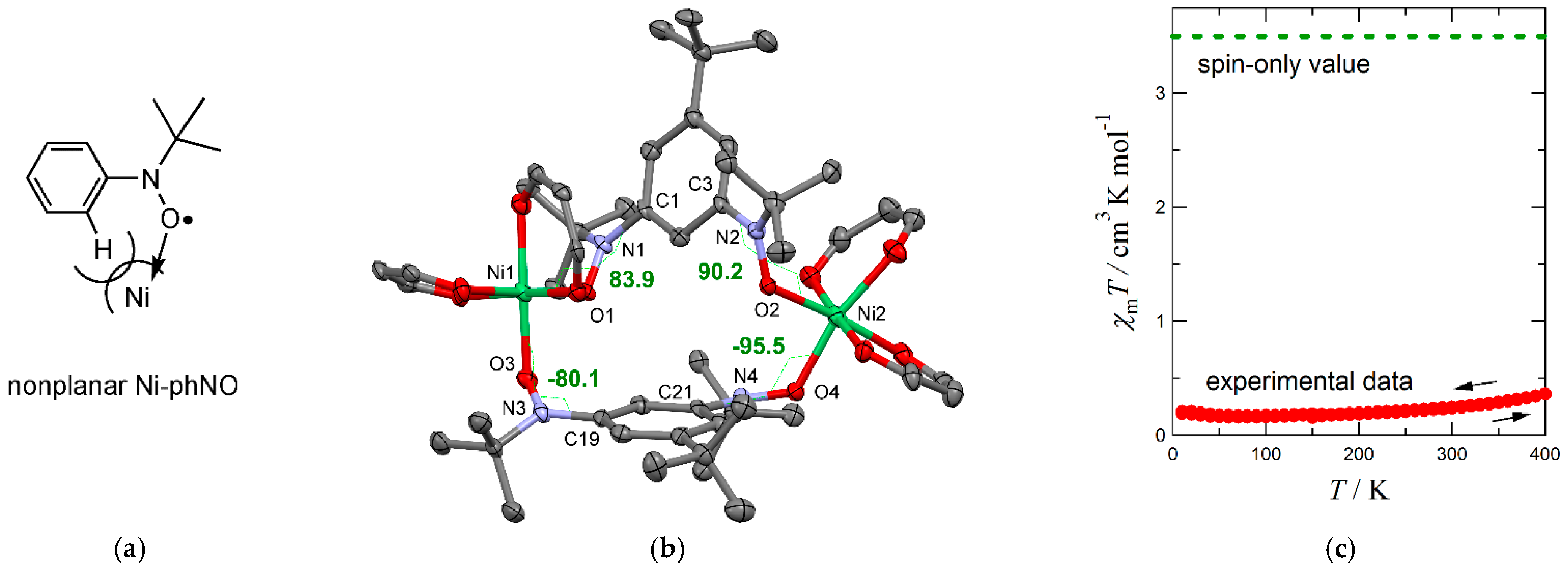
2.7. Diamagnetic Molecules
2.8. Miscellaneous
3. Concluding Remarks
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Gütlich, P.; Goodwin, H.A. (Eds.) Spin Crossover in Transition Metal Compounds I, II, and III; Springer: Berlin, Germany, 2004. [Google Scholar]
- Halcrow, M.A. (Ed.) Spin-Crossover Materials: Properties and Applications; John Wiley & Sons, Inc.: Oxford, UK, 2013. [Google Scholar]
- Molnár, G.; Rat, S.; Salmon, L.; Nicolazzi, W.; Bousseksou, A. Spin Crossover Nanomaterials: From Fundamental Concepts to Devices. Adv. Mater. 2018, 30, 1703862. [Google Scholar] [CrossRef]
- Coronado, E. Molecular magnetism: From chemical design to spin control in molecules, materials and devices. Nat. Rev. Mater. 2020, 5, 87–104. [Google Scholar] [CrossRef]
- Kumar, K.S.; Ruben, M. Emerging trends in spin crossover (SCO) based functional materials and devices. Coord. Chem. Rev. 2017, 346, 176–205. [Google Scholar] [CrossRef]
- Sato, O.; Tao, J.; Zhang, Y.Z. Control of magnetic properties through external stimuli. Angew. Chem. Int. Ed. 2007, 46, 2152–2187. [Google Scholar] [CrossRef] [PubMed]
- Ratera, I.; Veciana, J. Playing with organic radicals as building blocks for functional molecular materials. Chem. Soc. Rev. 2012, 41, 303–349. [Google Scholar] [CrossRef] [PubMed]
- Iwamura, H. What role has organic chemistry played in the development of molecule-based magnets? Polyhedron 2013, 66, 3–14. [Google Scholar] [CrossRef]
- Caneschi, A.; Gatteschi, D.; Sessoli, R.; Rey, P. Toward molecular magnets: The metal–radical approach. Acc. Chem. Res. 1989, 22, 392–398. [Google Scholar] [CrossRef]
- Meng, X.; Shi, W.; Cheng, P. Magnetism in one-dimensional metal–nitronyl nitroxide radical system. Coord. Chem. Rev. 2019, 378, 134–150. [Google Scholar] [CrossRef]
- Faust, T.B.; D’Alessandro, D.M. Radicals in metal–organic frameworks. RSC Adv. 2014, 4, 17498–17512. [Google Scholar] [CrossRef]
- Lü, B.; Chen, Y.; Li, P.; Wang, B.; Müllen, K.; Yin, M. Stable radical anions generated from a porous perylenediimide metal–organic framework for boosting near-infrared photothermal conversion. Nat. commun. 2019, 10, 1–8. [Google Scholar] [CrossRef]
- Castellano, M.; Ruiz-García, R.; Cano, J.; Ferrando-Soria, J.; Pardo, E.; Fortea-Pérez, F.R.; Stiriba, S.-E.; Barros, W.P.; Stumpf, H.O.; Cañadillas-Delgado, L.; et al. Metallosupramolecular approach toward multifunctional magnetic devices for molecular spintronics. Coord. Chem. Rev. 2015, 303, 110–138. [Google Scholar] [CrossRef]
- Kurmoo, M. Magnetic metal–organic frameworks. Chem. Soc. Rev. 2009, 38, 1353–1379. [Google Scholar] [CrossRef] [PubMed]
- Thorarinsdottir, A.E.; Harris, T.D. Metal–Organic Framework Magnets. Chem. Rev. 2020, 120, 8716–8789. [Google Scholar] [CrossRef] [PubMed]
- Kitazawa, T.; Gomi, Y.; Takahashi, M.; Takeda, M.; Enomoto, M.; Miyazaki, A.; Enoki, T. Spin-crossover behaviour of the coordination polymer FeII(C5H5N)2NiII(CN)4. J. Mater. Chem. 1996, 6, 119–121. [Google Scholar] [CrossRef]
- Murphy, M.J.; Zenere, K.A.; Ragon, F.; Southon, P.D.; Kepert, C.J.; Neville, S.M. Guest programmable multistep spin crossover in a porous 2-D Hofmann-type material. J. Am. Chem. Soc. 2017, 139, 1330–1335. [Google Scholar] [CrossRef]
- Takahashi, K.; Cui, H.B.; Okano, Y.; Kobayashi, H.; Mori, H.; Tajima, H.; Einaga, Y.; Sato, O. Evidence of the chemical uniaxial strain effect on electrical conductivity in the spin-crossover conducting molecular system: [FeIII(qnal)2][Pd(dmit)2]5·acetone. J. Am. Chem. Soc. 2008, 130, 6688–6689. [Google Scholar] [CrossRef]
- Djukic, B.; Lemaire, M.T. Hybrid Spin-crossover conductor exhibiting unusual variable-temperature electrical conductivity. Inorg. Chem. 2009, 48, 10489–10491. [Google Scholar] [CrossRef]
- Aromí, G.; Real, J.A. Special Issue “Spin Crossover (SCO) Research”. Magnetochemistry 2016, 2, 28. [Google Scholar] [CrossRef]
- Takahashi, K. Spin-Crossover Complexes. Inorganics 2018, 6, 32. [Google Scholar] [CrossRef]
- Kitazawa, T. Synthesis and Applications of New Spin Crossover Compounds. Crystals 2019, 9, 382. [Google Scholar] [CrossRef]
- Ovchinnikov, S.G. Spin Crossover (SCO) Research 2020. Molecules 2020, 25. Available online: https://www.mdpi.com/journal/molecules/special_issues/SCO_Research (accessed on 1 January 2021).
- Hayami, S.; Holmes, S.M.; Halcrow, M.A. Spin-State Switches in Molecular Materials Chemistry. J. Mater. Chem. C 2015, 3, 7775–7778. [Google Scholar] [CrossRef]
- Kimura, A.; Ishida, T. Spin-crossover temperature predictable from DFT calculation for iron(II) complexes with 4-substituted pybox and related heteroaromatic ligands. ACS Omega 2018, 3, 6737–6747. [Google Scholar] [CrossRef] [PubMed]
- Kimura, A.; Ishida, T. Pybox-iron(II) spin-crossover complexes with substituent effects from the 4-position of the pyridine ring (Pybox= 2,6-Bis(oxazolin-2-yl)pyridine). Inorganics 2017, 5, 52. [Google Scholar] [CrossRef]
- Yamasaki, M.; Ishida, T. Heating-rate dependence of spin-crossover hysteresis observed in an iron(II) complex having tris(2-pyridyl)methanol. J. Mater. Chem. C 2015, 3, 7784–7787. [Google Scholar] [CrossRef]
- Hirosawa, N.; Oso, Y.; Ishida, T. Spin crossover and light-induced excited spin-state trapping observed for an iron(II) complex chelated with tripodal tetrakis(2-pyridyl)methane. Chem. Lett. 2012, 41, 716–718. [Google Scholar] [CrossRef]
- Ishida, T.; Kanetomo, T.; Yamasaki, M. An iron(II) complex tripodally chelated with 1,1,1-tris(pyridin-2-yl)ethane showing room-temperature spin-crossover behaviour. Acta Crystallogr. Sect. C Struct. Chem. 2016, 72, 797–801. [Google Scholar] [CrossRef]
- Ondo, A.; Ishida, T. Cobalt(II) terpyridin-4′-yl nitroxide complex as an exchange-coupled spin-crossover material. Crystal 2018, 8, 155. [Google Scholar] [CrossRef]
- Mochida, N.; Kimura, A.; Ishida, T. Spin-Crossover Hysteresis of [FeII(LHiPr)2(NCS)2] (LHiPr = N-2-Pyridylmethylene- 4-Isopropylaniline) Accompanied by Isopropyl Conformation Isomerism. Magnetochemistry 2015, 1, 17–27. [Google Scholar] [CrossRef]
- Oso, Y.; Kanatsuki, D.; Saito, S.; Nogami, T.; Ishida, T. Spin-crossover Transition Coupled with Another Solid–Solid Phase Transition for Iron(II) Thiocyanate Complexes Chelated with Alkylated N-(Di-2-pyridylmethylene)anilines. Chem. Lett. 2008, 37, 760–761. [Google Scholar] [CrossRef]
- Oso, Y.; Ishida, T. Spin-crossover transition in a mesophase iron(II) thiocyanate complex chelated with 4-hexadecyl-N-(2-pyridylmethylene)aniline. Chem. Lett. 2009, 38, 604–605. [Google Scholar] [CrossRef]
- Boca, R. (Ed.) Theoretical Foundations of Molecular Magnetism: Current Methods in Inorganic Chemistry; Elsevier: Amsterdam, The Netherlands, 1999; Volume 1, pp. 541–563. [Google Scholar]
- Cirera, J.; Via-Nadal, M.; Ruiz, E. Benchmarking density functional methods for calculation of state energies of first row spin-crossover molecules. Inorg. Chem. 2018, 57, 14097–14105. [Google Scholar] [CrossRef] [PubMed]
- Olguín, J. Unusual metal centres/coordination spheres in spin crossover compounds. Coord. Chem. Rev. 2020, 407, 213148. [Google Scholar] [CrossRef]
- Yamasaki, M.; Ishida, T. First Iron(II) spin-crossover complex with an N5S coordination sphere. Chem. Lett. 2015, 44, 920–921. [Google Scholar] [CrossRef]
- Likhtenshtein, G.I. (Ed.) Nitroxides. Brief History, Fundamentals, and Recent Developments; Springer: Cham, Switzerland, 2020. [Google Scholar]
- Hicks, R.G. (Ed.) Stable Radicals: Fundamentals and Applied Aspects of Odd- Electron Compounds; Wiley: Chichester, UK, 2011. [Google Scholar]
- Haugland, M.M.; Lovett, J.E.; Anderson, E.A. Advances in the synthesis of nitroxide radicals for use in biomolecule spin labelling. Chem. Soc. Rev. 2018, 47, 668–680. [Google Scholar] [CrossRef] [PubMed]
- Klare, J.P.; Steinhoff, H.J. Spin labeling EPR. Photosynth. Res. 2009, 102, 377–390. [Google Scholar] [CrossRef] [PubMed]
- Nishimaki, H.; Ishida, T. Organic two-step spin-transition-like behavior in a linear S = 1 array: 3′-methylbiphenyl-3,5-diyl bis(tert-butylnitroxide) and related compounds. J. Am. Chem. Soc. 2010, 132, 9598–9599. [Google Scholar] [CrossRef]
- Kanetomo, T.; Ishida, T. Notably Strong Antiferromagnetic Interaction in a Methylene-bridged Bis(dihydrophenanthridin-N-oxyl). Chem. Lett. 2017, 46, 188–190. [Google Scholar] [CrossRef]
- Kanetomo, T.; Ichihashi, K.; Enomoto, M.; Ishida, T. Ground Triplet Spirobiradical: 2,2′,7,7′-Tetra(tert-butyl)-9,9′(10H,10′H)- spirobiacridine-10,10′-dioxyl. Org. Lett. 2019, 21, 3909–3912. [Google Scholar] [CrossRef]
- Koizumi, N.; Ishida, T. Forced proximity of nitroxide groups in pincer compounds with a xanthene spacer. Tetrahedron Lett. 2017, 58, 2804–2808. [Google Scholar] [CrossRef]
- Ishida, T.; Shinozuka, K.; Kubota, M.; Ohashi, M.; Nogami, T. Fullerene spin label. Synthesis and characterization of the [60] fullerene-substituted TEMPO radical. J. Chem. Soc. Chem. Commun. 1995, 1841–1842. [Google Scholar] [CrossRef]
- Nogami, T.; Ishida, T.; Yasui, M.; Iwasaki, F.; Takeda, N.; Ishikawa, M.; Kawakami, T.; Yamaguchi, K. Proposed mechanism of ferromagnetic interaction of organic ferromagnets: 4-(arylmethyleneamino)-2,2,6,6-tetramethylpiperidin-1-oxyls and related compounds. Bull. Chem. Soc. Jpn. 1996, 69, 1841–1848. [Google Scholar] [CrossRef]
- Ovcharenko, V.; Fokin, S.; Chubakova, E.; Romanenko, G.; Bogomyakov, A.; Dobrokhotova, Z.; Lukzen, N.; Morozov, V.; Petrova, M.; Petrova, M.; et al. A Copper−Nitroxide Adduct Exhibiting Separate Single Crystal-to-Single Crystal Polymerization−Depolymerization and Spin Crossover Transitions. Inorg. Chem. 2016, 55, 5853–5861. [Google Scholar] [CrossRef] [PubMed]
- Fedin, M.V.; Verber, S.L.; Bagryanskaya, E.; Ovcharenko, V.I. Electron Paramagnetic Resonance of Switchable Copper-Nitroxide-Based Molecular Magnets: An Indispensable Tool for Intriguing Systems. Coord. Chem. Rev. 2015, 289–290, 341–356. [Google Scholar] [CrossRef]
- Ovcharenko, V.I.; Maryunina, K.Y.; Fokin, S.V.; Tretyakov, E.V.; Romanenko, G.V.; Ikorskii, V.N. Spin transitions in non-classical systems. Russ. Chem. Bull. 2004, 53, 2406–2427. [Google Scholar] [CrossRef]
- Lanfranc de Panthou, F.; Belorizky, E.; Calemczuk, R.; Luneau, D.; Marcenat, C.; Ressouche, E.; Turek, P.; Rey, P. A New Type of Thermally Induced Spin Transition Associated with an Equatorial Axial Conversion in a Copper(II)–Nitroxide Cluster. J. Am. Chem. Soc. 1995, 117, 11247–11253. [Google Scholar] [CrossRef]
- Hirel, C.; Li, L.; Brough, P.; Vostrikova, K.; Pécaut, J.; Mehdaoui, B.; Bernard, M.; Turek, P.; Rey, P. New Spin-Transition-Like Copper(II)−Nitroxide Species. Inorg. Chem. 2007, 46, 7545–7552. [Google Scholar] [CrossRef]
- Lanfranc de Panthou, F.; Luneau, D.; Musin, R.; Öhrström, L.; Grand, A.; Turek, P.; Rey, P. Spin-Transition and Ferromagnetic Interactions in Copper(II) Complexes of a 3-Pyridyl-Substituted Imino Nitroxide. Dependence of the Magnetic Properties upon Crystal Packing. Inorg. Chem. 1996, 35, 3484–3491. [Google Scholar] [CrossRef]
- Kahn, O. Molecular Magnetism; VCH: New York, NY, USA, 1993. [Google Scholar]
- Miller, J.S.; Gatteschi, D. Molecule-based magnets. Chem. Soc. Rev. 2011, 40, 3065–3066. [Google Scholar] [CrossRef]
- Lemaire, M.T. Recent developments in the coordination chemistry of stable free radicals. Pure Appl. Chem. 2004, 76, 277–293. [Google Scholar] [CrossRef]
- Ferrando-Soria, J.; Vallejo, J.; Castellano, M.; Martinez-Lillo, J.; Pardo, E.; Cano, J.; Castro, I.; Lloret, F.; Ruiz-Garcia, R.; Julve, M. Molecular magnetism, quo vadis? A historical perspective from a coordination chemist viewpoint. Coord. Chem. Rev. 2017, 339, 17–103. [Google Scholar] [CrossRef]
- Rota, J.B.; Calzado, C.J.; Train, C.; Robert, V. Microscopic origins of the ferromagnetic exchange coupling in oxoverdazyl-based Cu(II) complex. J. Chem. Phys. 2010, 132, 154702. [Google Scholar] [CrossRef] [PubMed]
- McConnell, H.M. Ferromagnetism in Solid Free Radicals. J. Chem. Phys. 1963, 39, 1910. [Google Scholar] [CrossRef]
- Romero, F.M.; Ziessel, R.; Luneau, D. Structural control of ferromagnetic interactions in nickel(II) complexes based on a tetradentate biradical. Chem. Commun. 1998, 551–552. [Google Scholar] [CrossRef]
- Luneau, D.; Rey, P.; Laugier, J.; Fries, P.; Caneschi, A.; Gatteschi, D.; Sessoli, R. Nitrogen-bonded copper(II)–imino nitroxide complexes exhibiting large ferromagnetic interactions. J. Am. Chem. Soc. 1991, 113, 1245–1251. [Google Scholar] [CrossRef]
- Luneau, D.; Rey, P.; Laugier, J.; Belorizky, E.; Cogne, A. Ferromagnetic behavior of nickel(II)–imino nitroxide derivatives. Inorg. Chem. 1992, 31, 3578–3584. [Google Scholar] [CrossRef]
- Hicks, R.G.; Lemaire, M.T.; Thompson, L.K.; Barclay, T.M. Strong ferromagnetic and antiferromagnetic exchange coupling between transition metals and coordinated verdazyl radicals. J. Am. Chem. Soc. 2000, 122, 8077–8078. [Google Scholar] [CrossRef]
- Hearns, N.G.; Preuss, K.E.; Richardson, J.F.; Bin-Salamon, S. Design and Synthesis of a 4-(2′-Pyridyl)-1,2,3,5-Dithiadiazolyl Cobalt Complex. J. Am. Chem. Soc. 2004, 126, 9942–9943. [Google Scholar] [CrossRef]
- Rawson, J.M.; Alberola, A.; Whalley, A. Thiazyl radicals: Old materials for new molecular devices. J. Mater. Chem. 2006, 16, 2560–2575. [Google Scholar] [CrossRef]
- Morgan, I.S.; Peuronen, A.; Hanninen, M.M.; Reed, R.W.; Clérac, R.; Tuononen, H.M. 1-Phenyl-3-(pyrid-2-yl)benzo[e][1,2,4]triazinyl: The first “Blatter Radical” for coordination chemistry. Inorg. Chem. 2014, 53, 33–35. [Google Scholar] [CrossRef]
- Sidharth, T.N.S.; Nasani, R.; Gupta, A.; Sooraj, B.N.S.; Roy, S.; Mondal, A.; Konar, S. Reversal of magnetic exchange coupling between copper(II) and Blatter radical depending on the coordination environment. Inorg. Chim. Acta 2020, 503, 119395. [Google Scholar] [CrossRef]
- Blatter, H.M.; Lukaszewski, H. A new stable free radical. Tetrahedron Lett. 1968, 9, 2701–2705. [Google Scholar] [CrossRef]
- Kahn, O.; Prins, R.; Reedijk, J.; Thompson, J.S. Orbital symmetries and magnetic interaction between copper(II) ions and the o-semiquinone radical. Magnetic studies of (di-2-pyridylamine)(3,5-di-tert-butyl- o-semiquinonato)copper(II) perchlorate and bis(bis(3,5-di-tert-butyl-o-semiquinonato)copper(II)). Inorg. Chem. 1987, 26, 3557–3561. [Google Scholar] [CrossRef]
- Benelli, C.; Dei, A.; Gatteschi, D.; Pardi, L. Synthesis, redox behavior, magnetic properties, and crystal structure of a nickel(II)-semiquinone adduct with an unusually strong ferromagnetic coupling. Inorg. Chem. 1988, 27, 2831–2836. [Google Scholar] [CrossRef]
- Adams, D.M.; Dei, A.; Rheingold, A.L.; Hendrickson, D.N. Bistability in the [CoII(semiquinonate)2] to [CoIII(catecholate)(semiquinonate)] valence-tautomeric conversion. J. Am. Chem. Soc. 1993, 115, 8221–8229. [Google Scholar] [CrossRef]
- Chaudhuri, P.; Verani, C.N.; Bill, E.; Bothe, E.; Weyhermüller, T.; Wieghardt, K. Electronic structure of bis(o-iminobenzosemiquinonato)metal complexes (Cu, Ni, Pd). The art of establishing physical oxidation states in transition-metal complexes containing radical ligands. J. Am. Chem. Soc. 2001, 123, 2213–2223. [Google Scholar] [CrossRef]
- Pierpont, C.G. Studies on charge distribution and valence tautomerism in transition metal complexes of catecholate and semiquinonate ligands. Coord. Chem. Rev. 2001, 216, 99–125. [Google Scholar] [CrossRef]
- Okazawa, A.; Nagaichi, Y.; Nogami, T.; Ishida, T. Magneto-structure relationship in copper(II) and nickel(II) complexes chelated with stable tert-butyl 5-phenyl-2-pyridyl nitroxide and related radicals. Inorg. Chem. 2008, 47, 8859–8868. [Google Scholar] [CrossRef]
- Ali, A.; Dhar, D.; Barman, S.K.; Lloret, F.; Mukherjee, R. Nickel(II) complex of a hexadentate ligand with two o-iminosemiquinonato(1−) π-radical units and its monocation and dication. Inorg. Chem. 2016, 55, 5759–5771. [Google Scholar] [CrossRef]
- Lemes, M.A.; Brunet, G.; Pialat, A.; Ungur, L.; Korobkov, I.; Murugesu, M. Strong ferromagnetic exchange coupling in a {NiII4} cluster mediated through an air-stable tetrazine-based radical anion. Chem. Commun. 2017, 53, 8660–8663. [Google Scholar] [CrossRef]
- Murugesu, M.; Mavragani, N.; Kitos, A.A.; Brusso, J.L. Enhancing magnetic communication between metal centres: The role of s-tetrazine based radicals as ligands. Chem. Eur. J. 2021, in press. [Google Scholar] [CrossRef] [PubMed]
- Woods, T.J.; Stout, H.D.; Dolinar, B.S.; Vignesh, K.R.; Ballesteros-Rivas, M.F.; Achim, C.; Dunbar, K.R. Strong ferromagnetic exchange coupling mediated by a bridging tetrazine radical in a dinuclear nickel complex. Inorg. Chem. 2017, 56, 12094–12097. [Google Scholar] [CrossRef] [PubMed]
- Bonanno, N.M.; Lough, A.J.; Lemaire, M.T. Polynuclear Cu4L4 Copper(II) Aminyl Radical Coordination Complexes. Inorg. Chem. 2018, 57, 4837–4840. [Google Scholar] [CrossRef] [PubMed]
- De Souza, M.S.; Briganti, M.; Reis, S.G.; Stinghen, D.; Bortolot, C.S.; Cassaro, R.A.A.; Guedes, G.P.; Da Silva, F.C.; Ferreira, V.F.; Novak, M.A.; et al. Magnetic Cationic Copper(II) Chains and a Mononuclear Cobalt(II) Complex Containing [Ln(hfac)4]− Blocks as Counterions. Inorg. Chem. 2019, 58, 1976–1987. [Google Scholar] [CrossRef]
- Bubnov, M.P.; Teplova, I.A.; Cherkasova, A.V.; Baranov, E.V.; Fukin, G.K.; Romanenko, G.V.; Bogomyakov, A.S.; Straikov, A.G.; Cherkasov, V.K. Metal-ligand ferromagnetic exchange interactions in heteroligand bis-o-semiquinonato nickel complexes with 2,2′-dipyridine and 1,10-phenanthroline. Polyhedron 2019, 158, 262–269. [Google Scholar] [CrossRef]
- Richardson, P.F.; Kreilick, R.W. Copper Complexes with Free-Radical Ligands. J. Am. Chem. Soc. 1977, 99, 8183–8187. [Google Scholar] [CrossRef]
- Luneau, D.; Risoan, G.; Rey, P.; Grand, A.; Caneschi, A.; Gatteschi, D.; Laugier, J. Transition metal derivatives of a chelating nitronyl nitroxide ligand. Nickel(II) and manganese(II) complexes. Inorg. Chem. 1993, 32, 5616–5622. [Google Scholar] [CrossRef]
- Aoki, C.; Ishida, T.; Nogami, T. Metamagnetic behavior of [Ni(4ImNNH)2(NO3)2] having a ground high-spin state (4ImNNH = 4-imidazolyl nitronyl nitroxide). Inorg. Chem. Commun. 2003, 6, 1122–1125. [Google Scholar] [CrossRef]
- Aoki, C.; Ishida, T.; Nogami, T. Molecular metamagnet [Ni(4ImNNH)2(NO3)2] (4ImNNH= 4-imidazolyl nitronyl nitroxide) and the related compounds showing supramolecular H-bonding interactions. Inorg. Chem. 2003, 42, 7616–7625. [Google Scholar] [CrossRef]
- Luneau, D.; Rey, P. Magnetism of metal–nitroxide compounds involving bis-chelating imidazole and benzimidazole substituted nitronyl nitroxide free radicals. Coord. Chem. Rev. 2005, 249, 2591–2611. [Google Scholar] [CrossRef]
- Yoshioka, N.; Irisawa, M.; Aizawa, N.; Aoki, T.; Inoue, H.; Ohba, S. Structure and magnetic property of 2-imidazolylnitronyl nitroxide and its metal complexes. Mol. Cryst. Liq. Cryst. Sci. Technol. Sect. A 1996, 286, 165–170. [Google Scholar] [CrossRef]
- Okazawa, A.; Nogami, T.; Ishida, T. Strong intramolecular ferromagnetic couplings in nickel(II) and copper(II) complexes chelated with tert-butyl 5-methoxy-2-pyridyl nitroxide. Polyhedron 2009, 28, 1917–1921. [Google Scholar] [CrossRef]
- Kyoden, Y.; Homma, Y.; Ishida, T. High-Spin and Incomplete Spin-Crossover Polymorphs in Doubly Chelated [Ni(L)2Br2] (L = tert-Butyl 5-Phenyl-2-pyridyl Nitroxide). Inorg. Chem. 2019, 58, 10743–10755. [Google Scholar] [CrossRef]
- Ondo, A.; Ishida, T. Structures and Magnetic Properties of Transition Metal Complexes Involving 2,2′-Bipyridin-6-yl Nitroxide. AIP Conf. Proc. 2017, 1807, 020023. [Google Scholar]
- Okazawa, A. Magneto-Structural Relationship on Strong Exchange Interactions between Chelating Nitroxide Radical and Transition-Metal Spins. IOP Conf. Ser. Mater. Sci. Eng. 2017, 202, 012002. [Google Scholar] [CrossRef]
- Venkataraman, L.; Klare, J.E.; Nuckolls, C.; Hyberstsen, M.S.; Steigerwald, M.L. Dependence of single-molecule junction conductance on molecular conformation. Nature 2006, 442, 904–907. [Google Scholar] [CrossRef]
- Itoh, K.; Kinoshita, M. (Eds.) Molecular Magnetism—New Magnetic Materials–Chapter 2; Kodansha-Gordon and Breach: Tokyo, Japan, 2000. [Google Scholar]
- Mallah, T.; Thiébaut, S.; Verdaguer, M.; Veillet, P. High-Tc molecular-based magnets: Ferrimagnetic mixed-valence chromium(III)-chromium(II) cyanides with Tc at 240 and 190 Kelvin. Science 1993, 262, 1554–1557. [Google Scholar] [CrossRef] [PubMed]
- Kahn, O.; Charlot, M.F. Overlap density in binuclear complexes; a topological approach of the exchange interaction. In Quantum Theory of Chemical Reactions; Springer: Dordrecht, the Netherlands, 1980; pp. 215–240. [Google Scholar]
- Osanai, K.; Okazawa, A.; Nogami, T.; Ishida, T. Strong Ferromagnetic Exchange Couplings in Copper(II) and Nickel(II) Complexes with a Paramagnetic Tridentate Chelate Ligand, 2,2′-Bipyridin-6-yl tert-Butyl Nitroxide. J. Am. Chem. Soc. 2006, 128, 14008–14009. [Google Scholar] [CrossRef]
- Okazawa, A.; Nogami, T.; Ishida, T. tert-Butyl 2-Pyridyl Nitroxide Available as a Paramagnetic Chelate Ligand for Strongly Exchange-Coupled Metal−Radical Compounds. Chem. Mater. 2007, 19, 2733–2735. [Google Scholar] [CrossRef]
- Keana, J.F. Newer aspects of the synthesis and chemistry of nitroxide spin labels. Chem. Rev. 1978, 78, 37–64. [Google Scholar] [CrossRef]
- Miller, J.S.; Epstein, A.J.; Reiff, W.M. Ferromagnetic molecular charge-transfer complexes. Chem. Rev. 1988, 88, 201–220. [Google Scholar] [CrossRef]
- Lahti, P.M. Structure–property relationships for metal-free organic magnetic materials. Adv. Phys. Org. Chem. 2011, 45, 93–169. [Google Scholar]
- Landee, C.P.; Turnbull, M.M. A gentle introduction to magnetism: Units, fields, theory, and experiment. J. Coord. Chem. 2014, 67, 375–439. [Google Scholar] [CrossRef]
- Gruber, S.J.; Harris, C.M.; Sinn, E. Metal Complexes as Ligands. VI. Antiferromagnetic Interactions in Trinuclear Complexes Containing Similar and Dissimilar Metals. J. Chem. Phys. 1968, 9, 2183–2191. [Google Scholar] [CrossRef]
- Homma, Y.; Ishida, T. A new S = 0 ⇄ S = 2 “Spin-crossover” scenario found in a Nickel(II) Bis(nitroxide) system. Chem. Mater. 2018, 30, 1835–1838. [Google Scholar] [CrossRef]
- Lluncll, M.; Casanova, D.; Circra, J.; Bofill, J.M.; Alcmany, P.; Alvarez, S.; Pinsky, M.; Avnir, D. SHAPE, v2.1; University of Barcelona: Barcelona, Spain, 2005. [Google Scholar]
- Hogue, R.W.; Singh, S.; Brooker, S. Spin crossover in discrete polynuclear iron(II) complexes. Chem. Soc. Rev. 2018, 47, 7303–7338. [Google Scholar] [CrossRef]
- Halcrow, M.A. Structure:function relationships in molecular spin-crossover complexes. Chem. Soc. Rev. 2011, 40, 4119–4142. [Google Scholar] [CrossRef]
- Sheppard, C.L.; Tandon, S.S.; Thompson, L.K.; Bridson, J.N.; Miller, D.O.; Handa, M.; Lloret, F. Polynuclear copper(II) complexes with μ2-1,1-azide bridges. Structural and magnetic properties. Inorg. Chim. Acta 1996, 250, 227–239. [Google Scholar] [CrossRef]
- Sánchez-de-Armas, R.; Hernández, N.C.; Calzado, C.J. Copper–nitroxide based breathing crystals: A unified mechanism of gradual magnetostructural transition supported by quantum chemistry calculations. Inorg. Chem. Front. 2019, 6, 1228–1237. [Google Scholar] [CrossRef]
- Kashiro, A.; Kohno, W.; Ishida, T. Odd–Even Effect on the Spin-Crossover Temperature in Iron(II) Complex Series Involving an Alkylated or Acyloxylated Tripodal Ligand. Inorg. Chem. 2020, 59, 10163–10171. [Google Scholar] [CrossRef]
- Gütlich, P.; Gaspar, A.B.; Garcia, Y. Spin state switching in iron coordination compounds. Beilstein J. Org. Chem. 2013, 9, 342–391. [Google Scholar] [CrossRef] [PubMed]
- Okazawa, A.; Ishida, T. Spin-Transition-Like Behavior on One Side in a Nitroxide–Copper(II)–Nitroxide Triad System. Inorg. Chem. 2010, 49, 10144–10147. [Google Scholar] [CrossRef] [PubMed]
- Okazawa, A.; Hashizume, D.; Ishida, T. Ferro- and Antiferromagnetic Coupling Switch Accompanied by Twist Deformation around the Copper(II) and Nitroxide Coordination Bond. J. Am. Chem. Soc. 2010, 132, 11516–11524. [Google Scholar] [CrossRef] [PubMed]
- Letard, J.-F.; Real, J.A.; Moliner, N.; Gaspar, A.B.; Capes, L.; Cador, O.; Kahn, O. Light Induced Excited Pair Spin State in an Iron(II) Binuclear Spin-Crossover Compound. J. Am. Chem. Soc. 1999, 121, 10630–10631. [Google Scholar] [CrossRef]
- Tretyakov, E.V.; Tolstikov, S.E.; Suvorova, A.O.; Polushkin, A.V.; Romanenko, G.V.; Bogomyakov, A.S.; Veber, S.L.; Fedin, M.V.; Stass, D.V. Crucial role of paramagnetic ligands for magnetostructural anomalies in “breathing crystals”. Inorg. Chem. 2012, 51, 9385–9394. [Google Scholar] [CrossRef]
- Chaudhary, A.; Mohammad, A.; Mobin, S.M. Recent advances in single-crystal-to-single-crystal transformation at the discrete molecular level. Cryst. Growth Des. 2017, 17, 2893–2910. [Google Scholar] [CrossRef]
- Ishida, T. Moving Molecules in Crystalline Solids: Gradual Structure Transition and Spin Transition/ Crossover. IOP Conf. Ser.: Mater. Sci. Engineer. 2019, 515, 012001. [Google Scholar] [CrossRef]
- Kashiro, A.; Kyoden, Y.; Okazawa, A.; Ishida, T. Moving Organic Molecules in Crystalline Solids: Gradual Structural Transition and Spin Transition/Crossover. J. Synth. Org. Chem. Jpn. 2019, 77, 684–695. [Google Scholar] [CrossRef]
- Molnaŕ, G.; Mikolasek, M.; Ridier, K.; Fahs, A.; Nicolazzi, W.; Bousseksou, A. Molecular Spin Crossover Materials: Review of the Lattice Dynamical Properties. Ann. Phys. (Berlin Ger.) 2019, 531, 1900076. [Google Scholar] [CrossRef]
- Valtiner, M.; Paulsen, H.; Weinberger, P.; Linert, W. Theoretical investigations of a series of [hexakis(1-(tetrazol-1-yl)alkane-N4)iron(II)] bis(tetrafluoroborate) spin crossover complexes: Methyl- to pentyl substituted species in the approximation of free cations. MATCH Commun. Math. Comput. Chem. 2007, 57, 749–761. [Google Scholar]
- Kepp, K.P. Theoretical study of spin crossover in 30 iron complexes. Inorg. Chem. 2016, 55, 2717–2727. [Google Scholar] [CrossRef]
- Kyoden, Y.; Ishida, T. An indication of spin-transition accompanied by an order-disorder structural transformation in [Ni(phpyNO)2(NCS)2] (phpyNO = tert-butyl 5-phenyl-2-pyridyl nitroxide). RSC Adv. 2020, 10, 16009–16015. [Google Scholar] [CrossRef]
- Kyoden, Y.; Ishida, T. A Hidden Coordination-Bond Torsional Deformation as a Sign of Possible Spin Transition in Nickel(II)-Bis(nitroxide) Compounds. Molecules 2020, 25, 3790. [Google Scholar] [CrossRef] [PubMed]
- Ortega-Villar, N.; Muñoz, M.C.; Real, J.A. Symmetry breaking in iron(II) spin-crossover molecular crystals. Magnetochem. 2016, 2, 16. [Google Scholar] [CrossRef]
- Griffin, M.; Shakespeare, S.; Shepherd, H.J.; Harding, C.J.; Létard, J.F.; Desplanches, C.; Goeta, A.E.; Howard, J.A.K.; Powell, A.K.; Mereacre, V.; et al. A Symmetry-Breaking Spin-State Transition in Iron(III). Angew. Chem. Int. Ed. 2011, 50, 896–900. [Google Scholar] [CrossRef]
- Sheu, C.F.; Pillet, S.; Lin, Y.C.; Chen, S.M.; Hsu, I.J.; Lecomte, C.; Wang, Y. Magnetostructural Relationship in the Spin-Crossover Complex t-{Fe(abpt)2[N(CN)2]2}: Polymorphism and Disorder Phenomenon. Inorg. Chem. 2008, 47, 10866–10874. [Google Scholar] [CrossRef]
- Kashiro, A.; Some, K.; Kobayashi, Y.; Ishida, T. Iron(II) and 1,1,1-Tris(2-pyridyl)nonadecane Complex Showing an Order–Disorder-Type Structural Transition and Spin-Crossover Synchronized over Both Conformers. Inorg. Chem. 2019, 58, 7672–7676. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 16, Revision C.01; Gaussian Inc.: Wallingford, CT, USA, 2019. [Google Scholar]
- Becke, A.D. Density- functional thermochemistry. III. The role of exact exchange. J. Chem. Phys. 1993, 98, 5648–5652. [Google Scholar] [CrossRef]
- Becke, A.D. Density-functional thermochemistry. V. Systematic optimization of exchange-correlation functionals. J. Chem. Phys. 1997, 107, 8554–8560. [Google Scholar] [CrossRef]
- Lee, C.; Yang, W.; Parr, R.G. Development of the Colle-Salvetti correlation-energy formula into a functional of the electron density. Phys. Rev. B 1988, 37, 785. [Google Scholar] [CrossRef]
- Neese, F. Prediction of molecular properties and molecular spectroscopy with density functional theory: From fundamental theory to exchange-coupling. Coord. Chem. Rev. 2009, 253, 526–563. [Google Scholar] [CrossRef]
- Ruiz, E.; Cano, J.; Alvarez, S.; Alemany, P. Broken symmetry approach to calculation of exchange coupling constants for homobinuclear and heterobinuclear transition metal complexes. J. Comput. Chem. 1999, 20, 1391–1400. [Google Scholar] [CrossRef]
- Noodleman, L. Valence bond description of antiferromagnetic coupling in transition metal dimers. J. Chem. Phys. 1981, 74, 5737–5743. [Google Scholar] [CrossRef]
- Yamaguchi, K.; Kawakami, T.; Takano, Y.; Kitagawa, Y.; Yamashita, Y.; Fujita, H. Analytical and ab initio studies of effective exchange interactions, polyradical character, unpaired electron density, and information entropy in radical clusters (R)N: Allyl radical cluster (N = 2−10) and hydrogen radical cluster (N = 50). Int. J. Quantum Chem. 2002, 90, 370–385. [Google Scholar] [CrossRef]
- Ciofini, I.; Daul, C.A. DFT calculations of molecular magnetic properties of coordination compounds. Cood. Chem. Rev. 2003, 238, 187–209. [Google Scholar] [CrossRef]
- Bondi, A.V. van der Waals Volumes and Radii. J. Phys. Chem. 1964, 68, 441–451. [Google Scholar] [CrossRef]
- Fegy, K.; Vostrikova, K.E.; Luneau, D.; Rey, P. New nitroxide based molecular magnetic materials. Mol. Cryst. Liq. Cryst. Sci. Technol. Sect. A 1997, 305, 69–80. [Google Scholar] [CrossRef]
- Wang, C.; Ma, Y.; Wang, Y.; Wang, Q.; Li, L.; Cheng, P.; Liao, D. A New Quinoxalinyl-Substituted Nitronyl Nitroxide Radical and its Five-Spin CuII and Four-Spin MnII Complexes: Syntheses, Crystal Structures, and Magnetic Properties. Aust. J. Chem. 2012, 65, 672–679. [Google Scholar] [CrossRef]
- Wang, J.; Li, J.N.; Zhang, S.L.; Zhao, X.H.; Shao, D.; Wang, X.Y. Syntheses and magnetic properties of a pyrimidyl-substituted nitronyl nitroxide radical and its cobalt(II) complexes. Chem. Commun. 2016, 52, 5033–5036. [Google Scholar] [CrossRef]
- Omata, J.; Ishida, T.; Hashizume, D.; Iwasaki, F.; Nogami, T. Radical-copper macrocycles and related compounds. Mol. Cryst. Liq. Cryst. 2002, 376, 455–462. [Google Scholar] [CrossRef]
- Omata, J.; Ishida, T.; Hashizume, D.; Iwasaki, F.; Nogami, T. Radical-copper wheels: Structure and magnetism of hexanuclear hybrid arrays. Inorg. Chem. 2001, 40, 3954–3958. [Google Scholar] [CrossRef] [PubMed]
- Ishida, T.; Omata, J.; Nogami, T. Host–guest chemistry of radical-copper wheels. A supramolecular control of magnetic exchange coupling. Polyhedron 2003, 22, 2133–2138. [Google Scholar] [CrossRef]
- Yamada, S.; Yasui, M.; Nogami, T.; Ishida, T. Self-assembled meso-helicates of linear trinuclear nickel(II)–radical complexes with triple pyrazolate bridges. Dalton Trans. 2006, 1622–1626. [Google Scholar] [CrossRef]
- Albrecht, M. Catecholate-Based Helicates. Eur. J. Inorg. Chem. 2020, 2227–2237. [Google Scholar] [CrossRef]
- Ousaka, N.; Shimizu, K.; Suzuki, Y.; Iwata, T.; Itakura, M.; Taura, D.; Iida, H.; Furusho, Y.; Mori, T.; Yashima, E. Spiroborate-based double-stranded helicates: Meso-to-racemo isomerization and ion-triggered springlike motion of the racemo-helicate. J. Am. Chem. Soc. 2018, 140, 17027–17039. [Google Scholar] [CrossRef]
- Caulder, D.L.; Raymond, K.N. The rational design of high symmetry coordination clusters. J. Chem. Soc. Dalton Trans. 1999, 1185–1200. [Google Scholar] [CrossRef]
- Kahn, M.L.; Sutter, J.P.; Golhen, S.; Guionneau, P.; Ouahab, L.; Kahn, O.; Chasseau, D. Systematic investigation of the nature of the coupling between a Ln(III) ion (Ln = Ce(III) to Dy(III)) and its aminoxyl radical ligands. Structural and magnetic characteristics of a series of {Ln(organic radical)2} compounds and the related {Ln(Nitrone)2} derivatives. J. Am. Chem. Soc. 2000, 122, 3413–3421. [Google Scholar]
- Kanetomo, T.; Yoshii, S.; Nojiri, H.; Ishida, T. Single-molecule magnet involving strong exchange coupling in terbium(III) complex with 2,2′-bipyridin-6-yl tert-butyl nitroxide. Inorg. Chem. Front. 2015, 2, 860–866. [Google Scholar] [CrossRef]
- Kanetomo, T.; Ishida, T. Luminescent single-ion magnets from Lanthanoid(III) complexes with monodentate ketone ligands. AIP Conf. Proc. 2016, 1709, 020015. [Google Scholar]
- Mochizuki, T.; Nogami, T.; Ishida, T. Ferromagnetic Superexchange Coupling through a Diamagnetic Iron(II) Ion in a Mixed-Valent Iron(III, II, III) meso-Helicate. Inorg. Chem. 2009, 48, 2254–2259. [Google Scholar] [CrossRef]
- Chiarelli, R.; Gambarelli, S.; Rassat, A. Exchange interactions in nitroxide biradicals. Mol. Cryst. Liq. Cryst. Sci. Technol. Sect. A. 1997, 305, 455–478. [Google Scholar] [CrossRef]
- Ito, S.; Ishida, T. Practically Diamagnetic Macrocycle Consisting of Nickel–Biradical Heterospins with the Largest Out-of-Plane Torsion at Coordination Bonds. Chem. Lett. 2020, 49, 1062–1065. [Google Scholar] [CrossRef]
- Görlitz, G.; Hayamizu, T.; Itoh, T.; Matsuda, K.; Iwamura, H. Synthesis, Structure, and Magnetic Properties of a Cyclic Dimer of Bis(hexafluoroacetylacetonato){1,3-bis(N-tert-butyl-N-oxylamino)-5-tert-butylbenzene}manganese(II). Inorg. Chem. 1998, 37, 2083–2085. [Google Scholar] [CrossRef]
- Rabu, P.; Drillon, M.; Iwamura, H.; Görlitz, G.; Itoh, T.; Matsuda, K.; Koga, N.; Inoue, K. Exchange Coupling Parameters and Energy Levels for Cyclic Metal–Radical Complexes of Bis(hexafluoroacetylacetonato)manganese(II) with 5-tert-Butyl-1,3-phenylenebis(N-tert-butylaminoxyl) and (4-N-tert-Butyl-N-oxyamino)pyridine. Eur. J. Inorg. Chem. 2000, 211–216. [Google Scholar] [CrossRef]
- Numata, Y.; Inoue, K. Synthesis, crystal structure and magnetic properties of a cyclic dimer of [{Co(hfac)2}·BNOt-Bu]2. J. Mag. Mag. Mater. 2007, 310, 1847–1848. [Google Scholar] [CrossRef]
- Borrás-Almenar, J.J.; Clemente-Juan, J.M.; Coronado, E.; Tsukerblat, B.S. MAGPACK1 A package to calculate the energy levels, bulk magnetic properties, and inelastic neutron scattering spectra of high nuclearity spin clusters. J. Comput. Chem. 2001, 22, 985–991. [Google Scholar] [CrossRef]
- Nutting, J.E.; Rafiee, M.; Stahl, S.S. Tetramethylpiperidine N-oxyl (TEMPO), phthalimide N-oxyl (PINO), and related N-oxyl species: Electrochemical properties and their use in electrocatalytic reactions. Chem. Rev. 2018, 118, 4834–4885. [Google Scholar] [CrossRef] [PubMed]
- Siu, J.C.; Sauer, G.S.; Saha, A.; Macey, R.L.; Fu, N.; Chauviré, T.; Lancaster, K.M.; Lin, S. Electrochemical azidooxygenation of alkenes mediated by a Tempo–N3 charge-transfer complex. J. Am. Chem. Soc. 2018, 140, 12511–12520. [Google Scholar] [CrossRef] [PubMed]
- Wang, F.; Rafiee, M.; Stahl, S.S. Electrochemical Functional-Group-Tolerant Shono-type Oxidation of Cyclic Carbamates Enabled by Aminoxyl Mediators. Angew. Chem. Int. Ed. 2018, 57, 6686–6690. [Google Scholar] [CrossRef] [PubMed]
- Mollin, J.; Kasparek, F.; Lasovsky, J. On the basicity of hydroxylamine and its derivatives. Chem. Zvesti 1975, 1, 39–43. [Google Scholar]
- Kirsh, J.M.; Woodside, A.J.; Manor, B.C.; Carroll, P.J.; Rablen, P.R.; Graves, C.R. Synthesis and Characterization of (pyNO−)2GaCl: A Redox-Active Gallium Complex. Inorganics 2018, 6, 50. [Google Scholar] [CrossRef]
- Poitras, A.M.; Bogart, J.A.; Cole, B.E.; Carroll, P.J.; Schelter, E.J.; Graves, C.R. Synthesis and characterization of aluminum complexes of redox-active pyridyl nitroxide ligands. Inorg. Chem. 2015, 54, 10901–10908. [Google Scholar] [CrossRef]
- Bogart, J.A.; Lewis, A.J.; Boreen, M.A.; Lee, H.B.; Medling, S.A.; Carroll, P.J.; Booth, C.H.; Schelter, E.J. A ligand field series for the 4f-block from experimental and DFT computed Ce(IV/III) electrochemical potentials. Inorg. Chem. 2015, 54, 2830–2837. [Google Scholar] [CrossRef] [PubMed]
- Bogart, J.A.; Lewis, A.J.; Medling, S.A.; Piro, N.A.; Carroll, P.J.; Booth, C.H.; Schelter, E.J. Homoleptic Cerium(III) and Cerium(IV) Nitroxide Complexes: Significant Stabilization of the 4+ Oxidation State. Inorg. Chem. 2013, 52, 11600–11607. [Google Scholar] [CrossRef] [PubMed]
- McSkimming, A.; Su, J.; Cheisson, T.; Gau, M.R.; Carroll, P.J.; Batista, E.R.; Yang, P.; Schelter, E.J. Coordination Chemistry of a Strongly-Donating Hydroxylamine with Early Actinides: An Investigation of Redox Properties and Electronic Structure. Inorg. Chem. 2018, 57, 4387–4394. [Google Scholar] [CrossRef]
- Brese, N.E.; O’keeffe, M. Bond-valence parameters for solids. Acta Crystallogr. B Struct. Sci. 1991, 47, 192–197. [Google Scholar] [CrossRef]
- Bogart, J.A.; Lee, H.B.; Boreen, M.A.; Jun, M.; Schelter, E.J. Fine-tuning the oxidative ability of persistent radicals: Electrochemical and computational studies of substituted 2-pyridylhydroxylamines. J. Org. Chem. 2013, 78, 6344–6349. [Google Scholar] [CrossRef]
- Lannes, A.; Suffren, Y.; Tommasino, J.B.; Chiriac, R.; Toche, F.; Khrouz, L.; Molton, F.; Duboc, C.; Kieffer, I.; Hazemann, J.L.; et al. Room Temperature Magnetic Switchability Assisted by Hysteretic Valence Tautomerism in a Layered Two-Dimensional Manganese Radical Coordination Framework. J. Am. Chem. Soc. 2016, 138, 16493–16501. [Google Scholar] [CrossRef]
- Luneau, D. Coordination Chemistry of Nitronyl Nitroxide Radicals Has Memory. Eur. J. Inorg. Chem. 2020, 597–604. [Google Scholar] [CrossRef]
- Homma, Y.; Okazawa, A.; Ishida, T. Ground triplet pyrimidine-4,6-diyl bis(tert-butyl nitroxide) as a paramagnetic building block for metal–organic frameworks. Tetrahedron Lett. 2013, 54, 3120–3123. [Google Scholar] [CrossRef]
- Kawakami, H.; Tonegawa, A.; Ishida, T. A designed room-temperature triplet ligand from pyridine-2,6-diyl bis(tert-butyl nitroxide). Dalton Trans. 2016, 45, 1306–1309. [Google Scholar] [CrossRef] [PubMed]
- Kawakami, H.; Tonegawa, A.; Ishida, T. Pyridine-2,6-diyl dinitroxides as room-temperature triplet ligands. AIP Conf. Proc. 2016, 1709, 020017. [Google Scholar]
- Koide, K.; Ishida, T. Biradical chelating host 2,2′-bipyridine-6,6′-diyl bis(tert-butyl nitroxide) showing tunable exchange magnetic coupling. Inorg. Chem. Commun. 2011, 14, 194–196. [Google Scholar] [CrossRef]
- Koide, K.; Ishida, T. 2,2′-Bipyridine-6,6′-diyl bisnitroxide as a paramagnetic host: Encapsulation of a zinc(II) ion. Polyhedron 2011, 30, 3034–3037. [Google Scholar] [CrossRef]
- Konno, T.; Koide, K.; Ishida, T. A supramolecular switch between ground high-and low-spin states using 2,2′:6′,2′′-terpyridine-6,6′′-diyl bis(tert-butyl nitroxide). Chem. Commun. 2013, 49, 5156–5158. [Google Scholar] [CrossRef] [PubMed]
- Okazawa, A.; Terakado, Y.; Ishida, T.; Kojima, N. A triplet biradical with double bidentate sites based on tert-butyl pyridyl nitroxide as a candidate for strong ferromagnetic couplers. New J. Chem. 2018, 42, 17874–17878. [Google Scholar] [CrossRef]
- Gallagher, N.M.; Olankitwanit, A.; Rajca, A. High-spin organic molecules. J. Org. Chem. 2015, 80, 1291–1298. [Google Scholar] [CrossRef]
- Barone, V.; Cacelli, I.; Ferretti, A.; Monti, S.; Prampolini, G. An integrated protocol for the accurate calculation of magnetic interactions in organic magnets. J. Chem. Theory Comput. 2011, 7, 699–706. [Google Scholar] [CrossRef]
- Yoshitake, T.; Kudo, H.; Ishida, T. Thermally Activated Paramagnets from Diamagnetic Polymers of Biphenyl-3,5-diyl Bis(tert-butyl Nitroxides) Carrying Methyl and Fluoro Groups at the 2′-and 5′-Positions. Crystals 2016, 6, 30. [Google Scholar] [CrossRef]
- Ishida, T.; Iwamura, H. Bis[3-tert-butyl-5-(N-oxy-tert-butylamino)phenyl] nitroxide in a quartet ground state: A prototype for persistent high-spin poly[(oxyimino)-1,3-phenylenes]. J. Am. Chem. Soc. 1991, 113, 4238–4241. [Google Scholar] [CrossRef]
- Ferrer, J.R.; Lahti, P.M.; George, C.; Antorrena, G.; Palacio, F. Synthesis, crystallography, and magnetic properties of 2-tert-butylaminoxylbenzimidazole. Chem. Mater. 1999, 11, 2205–2210. [Google Scholar] [CrossRef]
- Taylor, P.; Ghalsasi, P.; Lahti, P.M. Modulating spin delocalization in conjugated nitroxides: 2-(N-aminoxyl-N-tert-butyl)-benzothiazole. Tetrahedron Lett. 2004, 45, 6295–6298. [Google Scholar] [CrossRef]
- Ishida, T.; Murakami, R.; Kanetomo, T.; Nojiri, H. Magnetic study on radical-gadolinium(III) complexes. Relationship between the exchange coupling and coordination structure. Polyhedron 2013, 66, 183–187. [Google Scholar] [CrossRef]
- Ikegaya, N.; Kanetomo, T.; Murakami, R.; Ishida, T. Triply Radical-coordinated Gadolinium(III) Complex as a High-spin S = 5 Assembly. Chem. Lett. 2012, 41, 82–83. [Google Scholar] [CrossRef]
- Kanetomo, T.; Ishida, T. Strongest exchange coupling in gadolinium(III) and nitroxide coordination compounds. Inorg. Chem. 2014, 53, 10794–10796. [Google Scholar] [CrossRef] [PubMed]
- Kanetomo, T.; Yoshitake, T.; Ishida, T. Strongest Ferromagnetic Coupling in Designed Gadolinium(III)–Nitroxide Coordination Compounds. Inorg. Chem. 2016, 55, 8140–8146. [Google Scholar] [CrossRef] [PubMed]
- Kanetomo, T.; Kihara, T.; Miyake, A.; Matsuo, A.; Tokunaga, M.; Kindo, K.; Nojiri, H.; Ishida, T. Giant Exchange Coupling Evidenced with a Magnetization Jump at 52 T for a Gadolinium-Nitroxide Chelate. Inorg. Chem. 2017, 56, 3310–3314. [Google Scholar] [CrossRef]
- Nakamura, T.; Kanetomo, T.; Ishida, T. Strong Antiferromagnetic Interaction in a Gadolinium(III) Complex with Methoxy-TEMPO Radical: A Relation between the Coupling and the Gd–O–N Angle. Inorg. Chem. 2021, 60. [Google Scholar] [CrossRef]
- Gupta, T.; Rajeshkumar, T.; Rajaraman, G. Magnetic exchange in {GdIII–radical} complexes: Method assessment, mechanism of coupling and magneto-structural correlations. Phys. Chem. Chem. Phys. 2014, 16, 14568–14577. [Google Scholar] [CrossRef]
- Zhu, M.; Li, C.; Wang, X.; Li, L.; Sutter, J.P. Thermal Magnetic Hysteresis in a Copper–Gadolinium–Radical Chain Compound. Inorg. Chem. 2016, 55, 2676–2678. [Google Scholar] [CrossRef] [PubMed]
- Xi, L.; Han, J.; Huang, X.; Li, L. Nitronyl Nitroxide Biradical-Based Binuclear Lanthanide Complexes: Structure and Magnetic Properties. Magnetochem. 2020, 6, 48. [Google Scholar] [CrossRef]
- Kanetomo, T.; Naoi, Y.; Enomoto, M. Gadolinium-Triradical Complex with Ground S = 10 State: Synthesis, Structural Characterization and Magnetic Studies. Eur. J. Inorg. Chem. 2021, in press. [Google Scholar] [CrossRef]
- Baker, M.L.; Tanaka, T.; Murakami, R.; Ohira-Kawamura, S.; Nakajima, K.; Ishida, T.; Nojiri, H. Relationship between torsion and anisotropic exchange coupling in a TbIII–radical-based single-molecule magnet. Inorg. Chem. 2015, 54, 5732–5738. [Google Scholar] [CrossRef] [PubMed]
- Kanetomo, T.; Yasui, M.; Ishida, T. Exchange-coupled terbium–radical complex Tb-phNO showing slow reversal of magnetization. Polyhedron 2017, 136, 30–34. [Google Scholar] [CrossRef]
- Patrascu, A.A.; Calancea, S.; Briganti, M.; Soriano, S.; Madalan, A.M.; Cassaro, R.A.A.; Caneschi, A.; Totti, F.; Vaz, M.G.F.; Andruh, M. A chimeric design of heterospin 2p–3d, 2p–4f, and 2p–3d–4f complexes using a novel family of paramagnetic dissymmetric compartmental ligands. Chem. Commun. 2017, 53, 6504–6507. [Google Scholar] [CrossRef]
- Xi, L.; Sun, J.; Li, H.; Han, J.; Huang, X.; Li, L. Chain versus Discrete Assembly of Nitronyl Nitroxide Radical-Lanthanide Complexes: Regulating Magnetization Dynamics by Modifying Coordination Symmetry. Cryst. Growth Des. 2020, 20, 3785–3794. [Google Scholar] [CrossRef]
- Kato, M.; Ito, H.; Hasegawa, M.; Ishii, K. Soft crystals: Flexible response systems with high structural order. Chem. Eur. J. 2019, 25, 5105–5112. [Google Scholar] [CrossRef]
- Bencini, A.; Gatteschi, D. EPR of Exchange Coupled Systems; Dover Pub. Inc.: Nineola, NY, USA, 2012; Chapter 1. [Google Scholar]
- Anderson, P.W. Theory of magnetic exchange interactions: Exchange in insulators and semiconductors. Solid State Phys. 1963, 14, 99–214. [Google Scholar]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ishida, T.; Ito, S.; Homma, Y.; Kyoden, Y. Molecular S = 2 High-Spin, S = 0 Low-Spin and S = 0 ⇄ 2 Spin-Transition/-Crossover Nickel(II)-Bis(nitroxide) Coordination Compounds. Inorganics 2021, 9, 10. https://doi.org/10.3390/inorganics9020010
Ishida T, Ito S, Homma Y, Kyoden Y. Molecular S = 2 High-Spin, S = 0 Low-Spin and S = 0 ⇄ 2 Spin-Transition/-Crossover Nickel(II)-Bis(nitroxide) Coordination Compounds. Inorganics. 2021; 9(2):10. https://doi.org/10.3390/inorganics9020010
Chicago/Turabian StyleIshida, Takayuki, Saki Ito, Yuta Homma, and Yukiya Kyoden. 2021. "Molecular S = 2 High-Spin, S = 0 Low-Spin and S = 0 ⇄ 2 Spin-Transition/-Crossover Nickel(II)-Bis(nitroxide) Coordination Compounds" Inorganics 9, no. 2: 10. https://doi.org/10.3390/inorganics9020010
APA StyleIshida, T., Ito, S., Homma, Y., & Kyoden, Y. (2021). Molecular S = 2 High-Spin, S = 0 Low-Spin and S = 0 ⇄ 2 Spin-Transition/-Crossover Nickel(II)-Bis(nitroxide) Coordination Compounds. Inorganics, 9(2), 10. https://doi.org/10.3390/inorganics9020010