Phases and Interfaces in the Cr–Fe–Si Ternary System: X-ray Diffraction and Electron Microscopy Study
Abstract
1. Introduction
2. Results
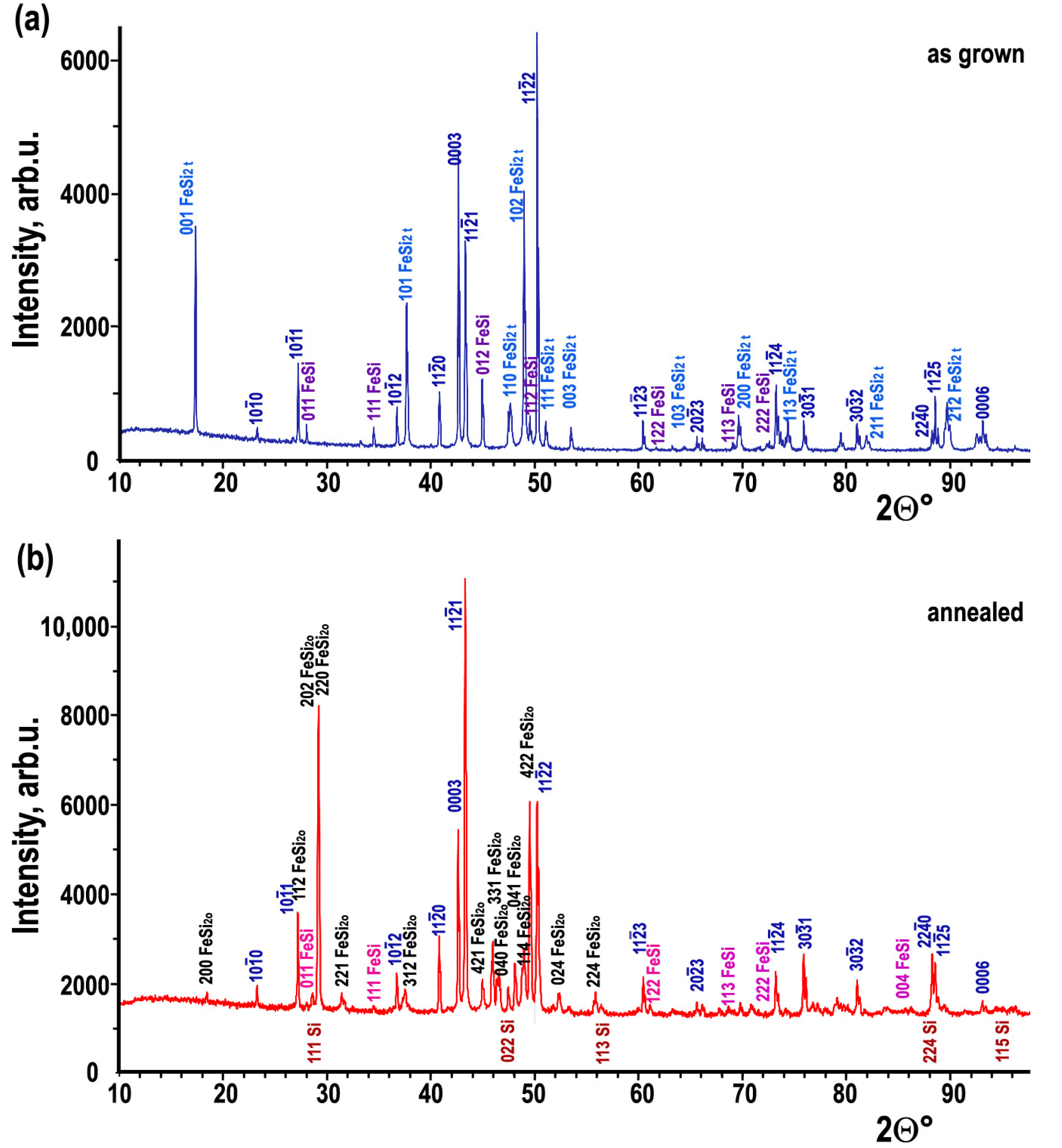
2.1. Powder X-ray Diffraction Study
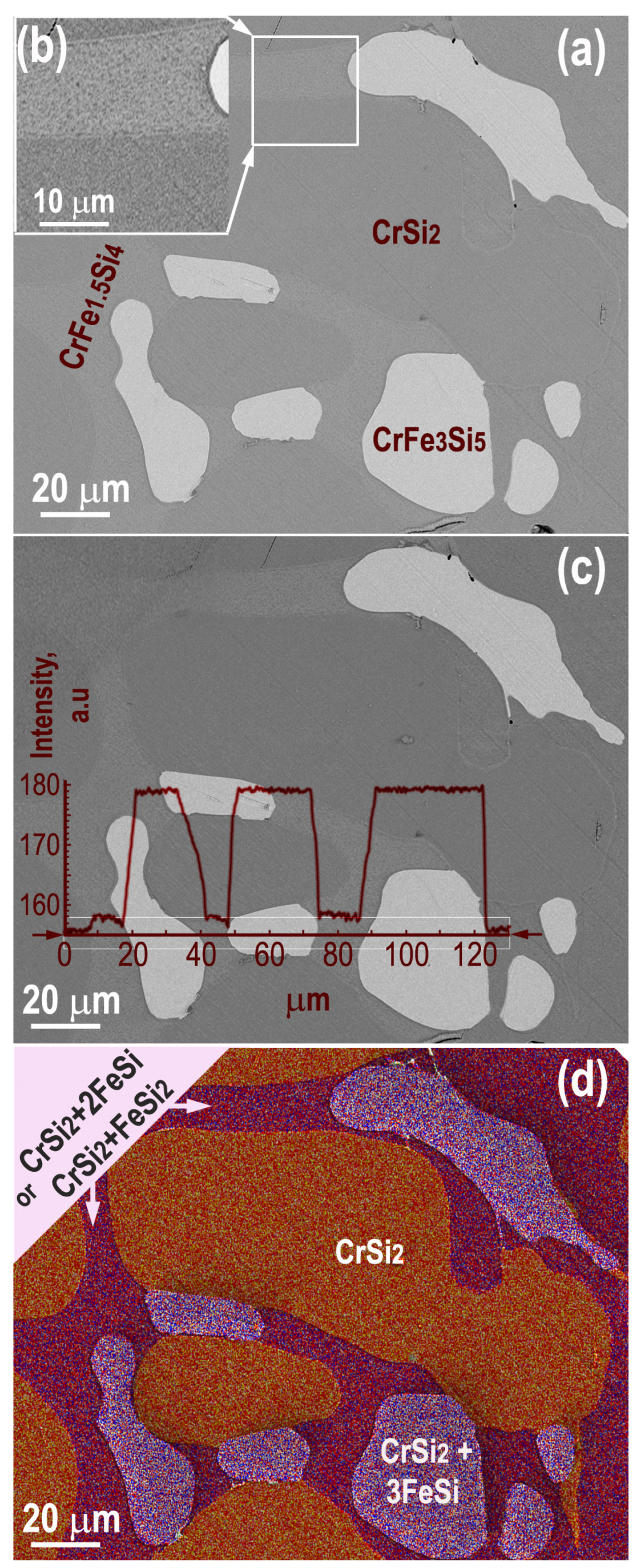
2.2. SEM and EDXS Analysis
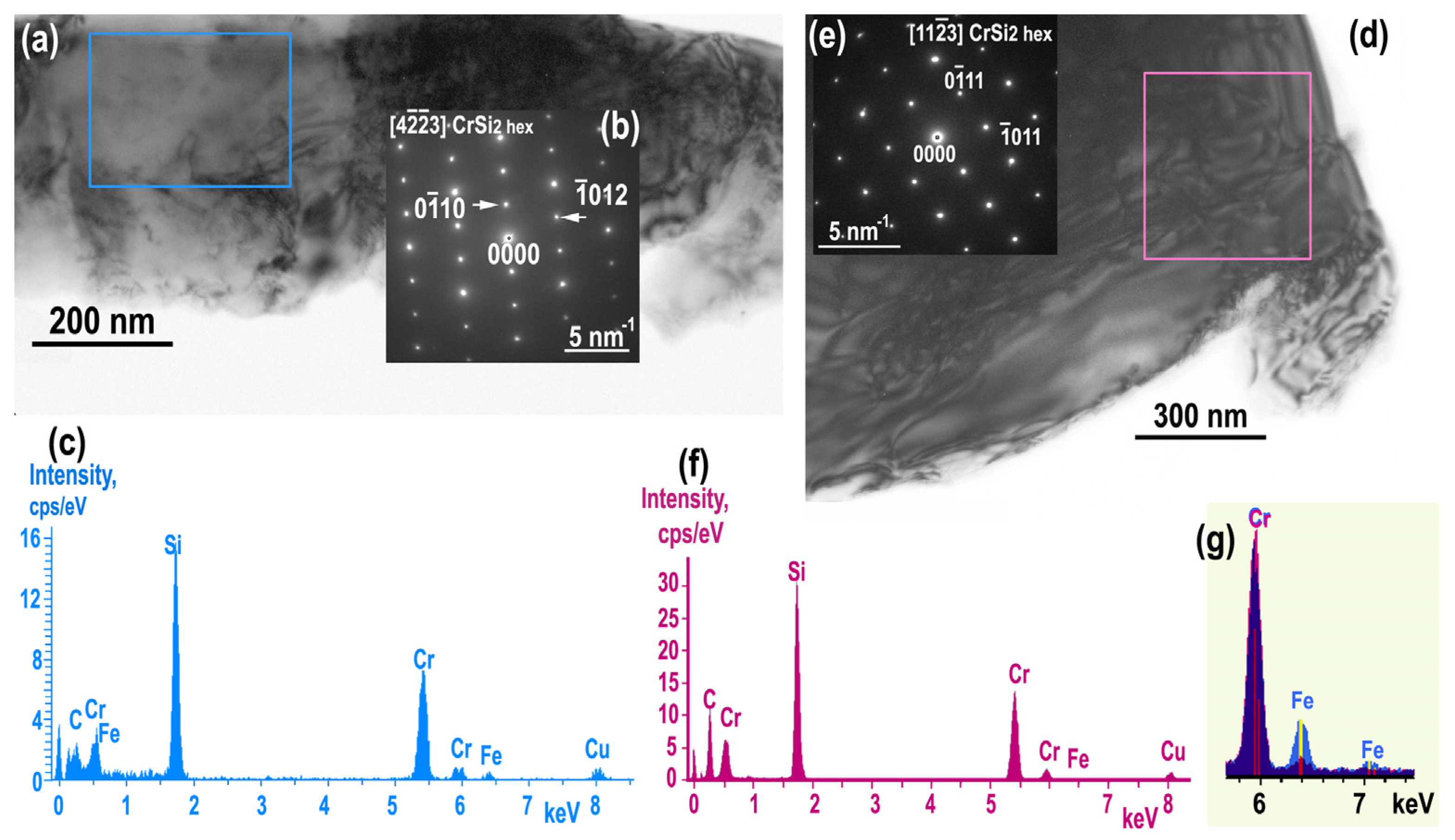
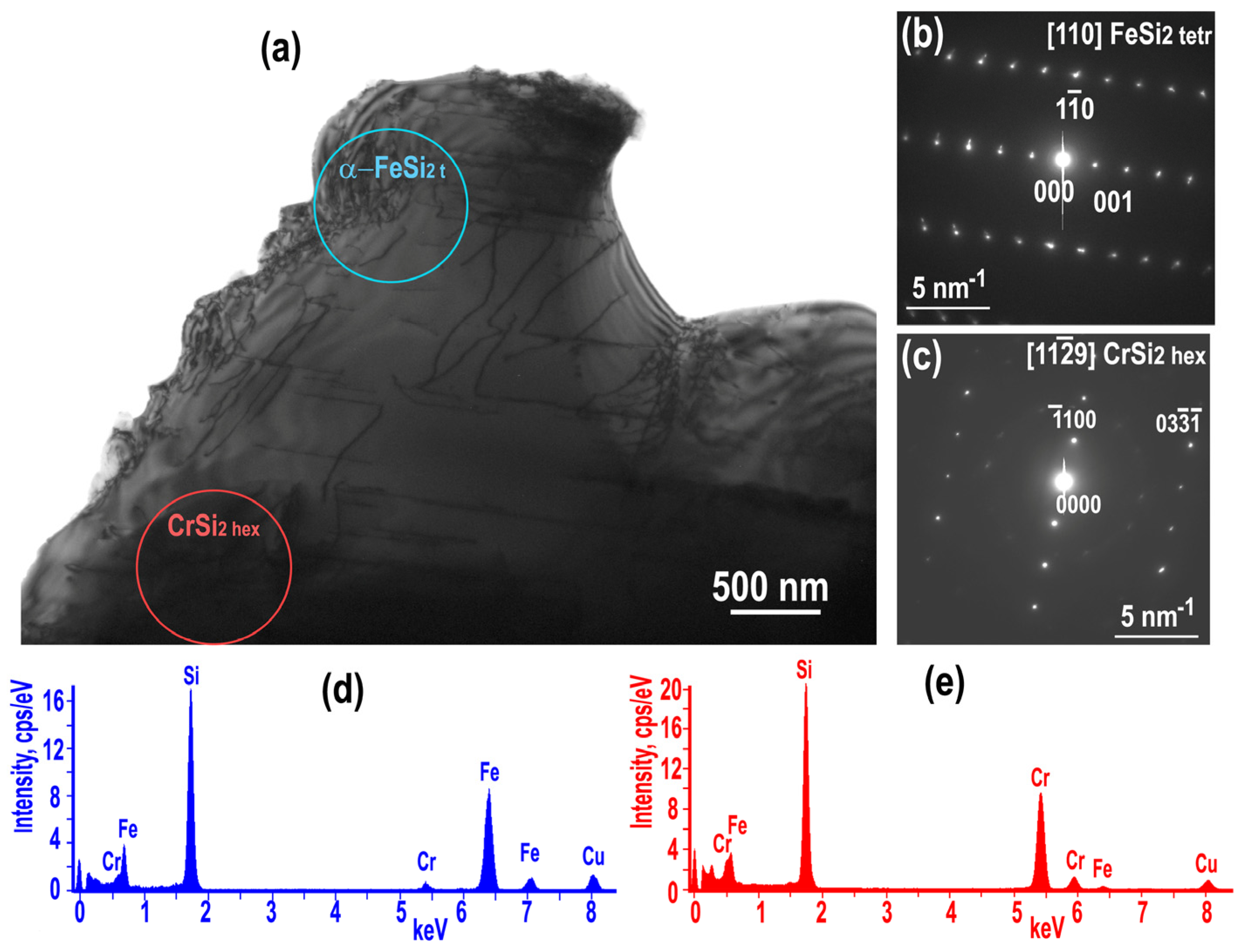
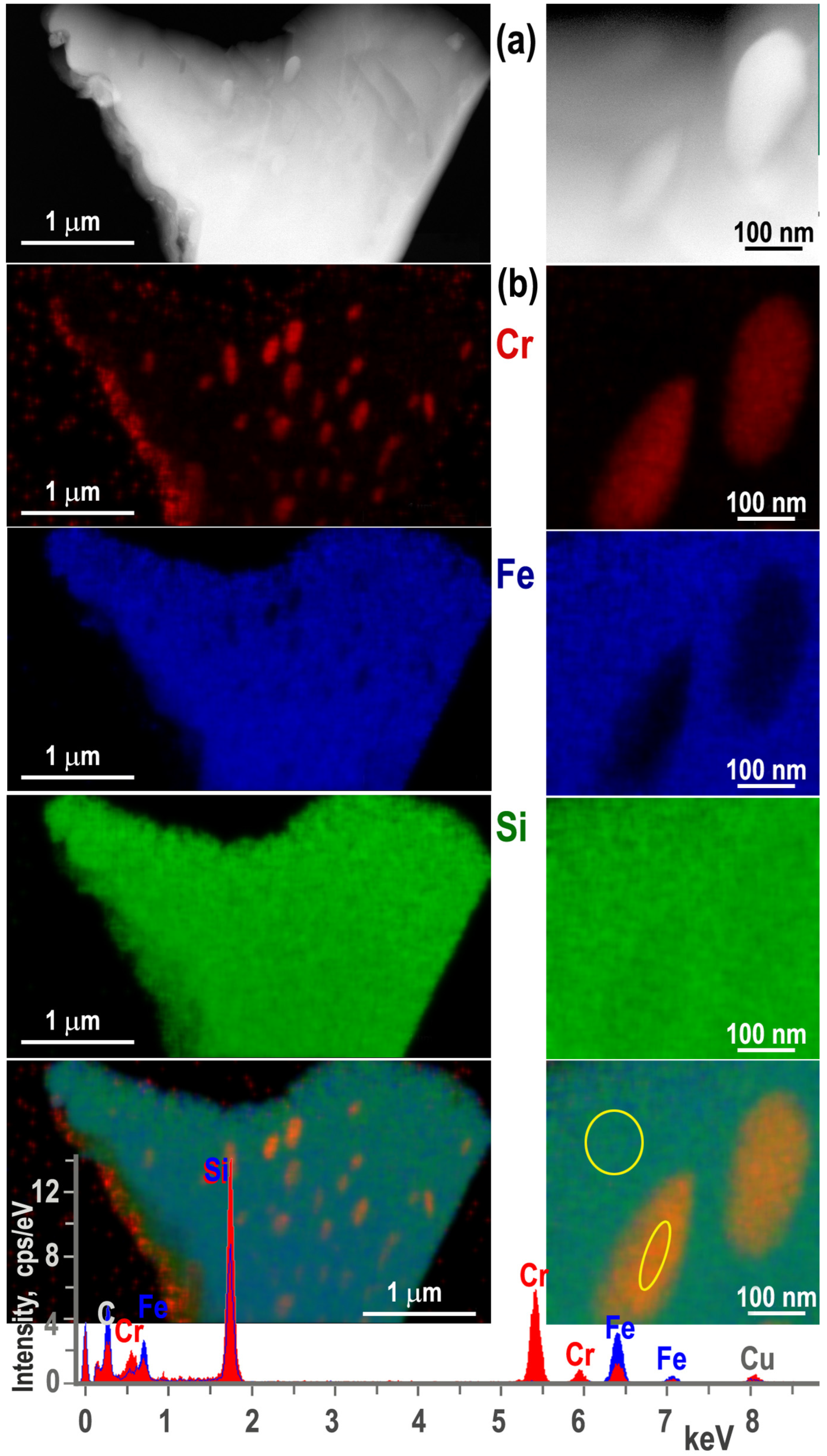
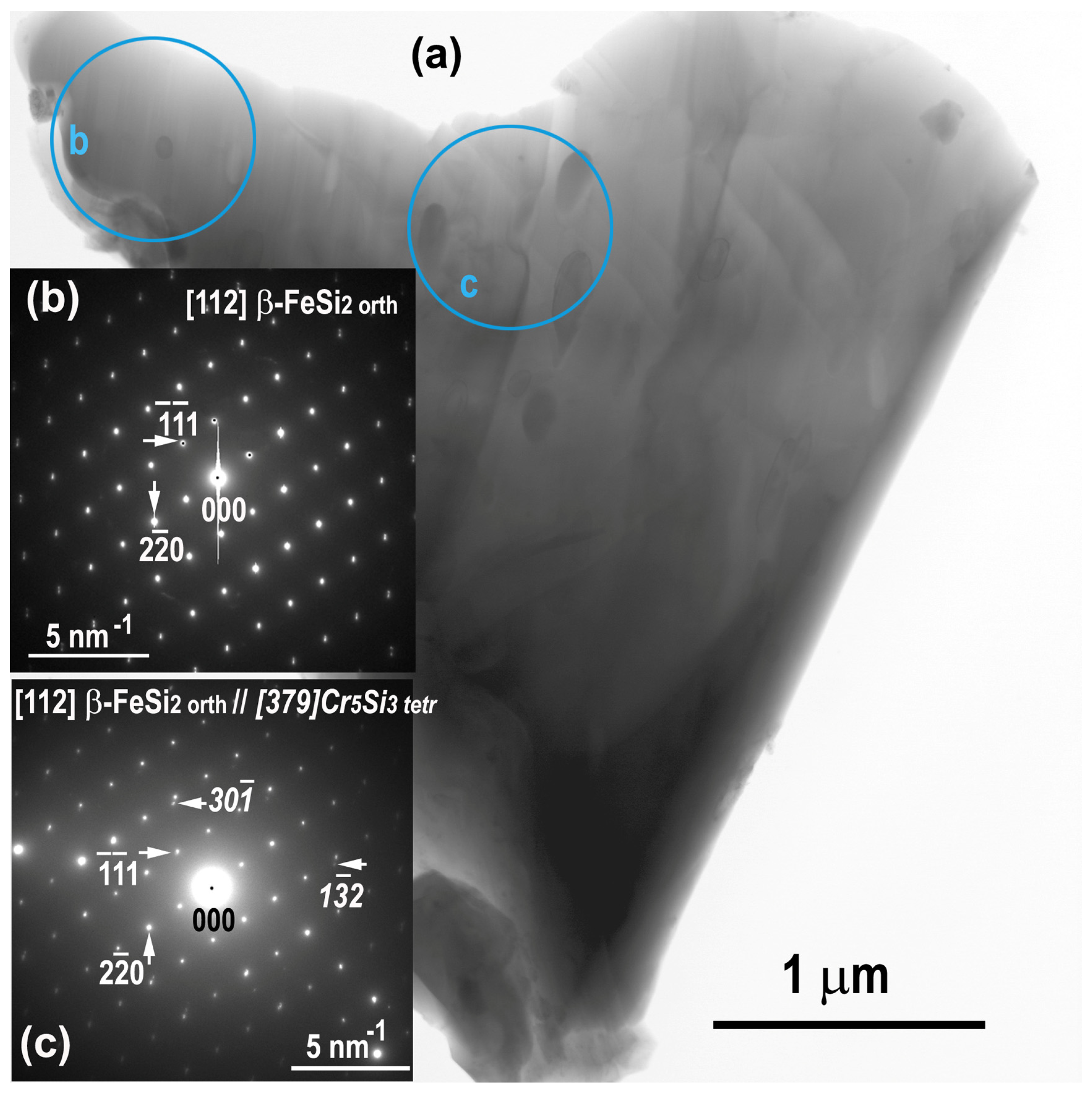
2.3. TEM, EDXS and Electron Diffraction Study
2.4. Crystallographic Orientation Relationships, Lattice Mismatch, Thermal Expansion Coefficient Mismatch
3. Materials and Methods
4. Discussion and Conclusions
Future Research Directions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yamamoto, K.; Kimura, Y.; Mishima, Y. Phase constitution and microstructure of the Fe-Si-Cr ternary ferritic alloys. Scr. Mater. 2004, 50, 977–981. [Google Scholar] [CrossRef]
- Han, H.K.; Loka, C.; Yang, Y.M.; Kim, J.H.; Moon, S.W.; Cho, J.S.; Lee, K.-S. High capacity retention Si/silicide nanocomposite anode materials fabricated by high-energy mechanical milling for lithium-ion rechargeable batteries. J. Power Sources 2015, 281, 293–300. [Google Scholar] [CrossRef]
- Min, E.H.; Kim, M.S.; Koh, J.G. Study of an Electromagnetic Wave Absorber Prepared with Fe-Si-Cr for Mobile Phones. J. Korean Phys. Soc. 2008, 53, 2086–2089. [Google Scholar] [CrossRef]
- Han, H.; Pan, D.; Li, D. Study on Fe-Si-Cr Soft magnetic composite coated with silicon dioxide. Mater. Res. Express 2019, 6, 026104. [Google Scholar] [CrossRef]
- Kim, S.W.; Cho, M.K.; Mishima, Y.; Choi, D.C. High temperature thermoelectric properties of p- and n-type β-FeSi2 with some dopants. Intermetallics 2003, 11, 399–405. [Google Scholar] [CrossRef]
- Isoda, Y.; Udono, H. Preparation and Thermoelectric Properties of Iron Disilicide. In Thermoelectrics and Its Energy Harvesting Materials, Preparation, and Characterization in Thermoelectrics; Rowe, D.M., Ed.; CRS Press; Taylor & Francis Group: Boca Raton, FL, USA, 2012; pp. 18-1–18-25. [Google Scholar]
- Nishida, I. The crystal growth and thermoelectric properties of chromium disilicide. J. Mater. Sci. 1972, 7, 1119–1124. [Google Scholar] [CrossRef]
- Khalil, M.; Moll, A.; Godfroy, M.; Letrouit-Lebranchu, A.; Villeroy, B.; Alleno, E.; Viennois, R.; Beaudhuin, M. Thermoelectric properties and stability of nanostructured chromium disilicide CrSi2. J. Appl. Phys. 2019, 126, 135103. [Google Scholar] [CrossRef]
- Chen, G.; Dresselhaus, M.S.; Dresselhaus, G.; Fleurial, J.-P.; Caillat, T. Recent developments in thermoelectric materials. Int. Mater. Rev. 2003, 48, 45–66. [Google Scholar] [CrossRef]
- Popescu, A.; Haney, P.M. Interface scattering in polycrystalline thermoelectrics. J. Appl. Phys. 2014, 115, 123701. [Google Scholar] [CrossRef]
- Chart, T.; Putland, F.; Dinsdale, A. Calculated phase equilibria for the Cr-Fe-Ni-Si system—I ternary equilibria. CALPHAD 1980, 4, 27–46. [Google Scholar] [CrossRef]
- Lindholm, M. A Thermodynamic description of the Fe-Cr-Si system with emphasis on the equilibria of the sigma (σ) phase. J. Phase Equilibria 1997, 18, 432–440. [Google Scholar] [CrossRef]
- Raghavan, V. Cr-Fe-Si (Chromium-Iron-Silicon). J. Phase Equilibria Diffus. 2004, 25, 545–546. [Google Scholar] [CrossRef]
- Wen, A.; Zhang, L.; Liu, L.; Masset, P.J.; Zhao, Y.; Zheng, L.; Zhao, F. Experimental Investigation of Phase Equilibria in the Fe-Cr-Si. J. Phase Equilibria Diffus. 2020, 41, 587–597. [Google Scholar] [CrossRef]
- Murarka, S.P. Transition metal silicides. Ann. Rev. Mater. Sci. 1983, 13, 117–137. [Google Scholar] [CrossRef]
- Nicolet, M.-A.; Lau, S.S. Formation and Characterization of Transition-Metal Silicides. In Materials and Process Characterization; Einspruch, N.G., Larrabee, G.B., Eds.; VLSI Electronics Microstructure Science; Elsevier: Amsterdam, The Netherlands, 1983; Volume 6, pp. 329–464. [Google Scholar]
- D’Heurle, F.; Gas, P. Kinetics of formation of silicides: A review. J. Mater. Res. 1986, 1, 205–221. [Google Scholar] [CrossRef]
- Burkov, A.T. Silicide Thermoelectrics: Materials for Energy Harvesting. Phys. Status Solidi A 2018, 215, 1800105. [Google Scholar] [CrossRef]
- Pshenay-Severin, D.A.; Burkov, A.T. Electronic structure of B20 (FeSi-Type) transition-metal monosilicides. Materials 2019, 12, 2710. [Google Scholar] [CrossRef]
- Ferrer-Argemi, L.; Yu, Z.; Lee, J. Effects of metal silicide inclusion interface and shape on thermal transport in silicon nanocomposites. J. Appl. Phys. 2019, 126, 035106. [Google Scholar] [CrossRef]
- Singh, S.; Sharma, Y.C. A review on silicide based materials for thermoelectric applications. IJAERS 2021, 8, 160–168. [Google Scholar] [CrossRef]
- Abbassi, L.; Mesguich, D.; Berthebaud, D.; Le Tonquesse, S.; Srinivasan, B.; Mo-ri, T.; Coulomb, L.; Chevallier, G.; Estournès, C.; Flahaut, E.; et al. Effect of Nanostructuring on the Thermoelectric Properties of β-FeSi2. Nanomaterials 2021, 11, 2852. [Google Scholar] [CrossRef]
- Tarani, E.; Stathokostopoulos, D.; Tsipas, S.A.; Chrissafis, K.; Vourlias, G. Effect of the Deposition Time and Heating Temperature on the Structure of Chromium Sili-cides Synthesized by Pack Cementation Process. Corros. Mater. Degrad. 2021, 2, 12. [Google Scholar] [CrossRef]
- Lue, J.-T.; Mu, S.-J. Ellipsometry and structure studies of chromium, molybdenum, and platinum silicides. Phys. Rev. B 1987, 36, 1657–1661. [Google Scholar] [CrossRef] [PubMed]
- Bedeker, C.J.; Kritzinger, S.; Lombaard, J.C. Formation and microstructure of various thin film chromium silicide phases. Thin Solid Films 1986, 141, 117–127. [Google Scholar] [CrossRef]
- Otogawaa, N.; Wanga, S.; Kihara, S.; Liub, Z.; Fukuzawa, Y.; Suzuki, Y.; Osamura, M.; Ootsuka, T.; Miyake, T.; Nakayama, Y.; et al. Semiconductor–metal phase transition of iron disilicide by laser annealing and its application to form device electrodes. Thin Solid Films 2004, 461, 223–226. [Google Scholar] [CrossRef]
- Liang, S.; Islam, R.; Smith, D.J.; Bennett, P.A. Phase transformation in FeSi2 nanowires. J. Cryst. Growth 2006, 295, 166–171. [Google Scholar] [CrossRef]
- Igarashi, S.; Haraguchi, M.; Aihara, J.; Saito, T.; Yamaguchi, K.; Yamamoto, H.; Hojou, K. Observation of iron silicide formation by plan-view transmission electron microscopy. J. Electron Microsc. 2004, 53, 223–228. [Google Scholar] [CrossRef]
- Moll, A.; Hermet, P.; Bantignies, J.-L.; Candolfi, C.; Lenoir, B.; Maurin, D.; Ra-monda, M.; Oliviero, E.; Fréty, N. Influence of nanostructurationonthe vibrational, electronic and optical properties of CrSi2 thin films. J. Phys. Chem. C 2020, 124, 28267–28276. [Google Scholar] [CrossRef]
- Schuette, M.; Wartchow, R.; Binnewies, M. Shape controlling synthesis—Formation of Fe3Si by the reaction of iron with silicon tetrachloride and crystal structure refinement. ZAAC 2003, 629, 1846–1850. [Google Scholar] [CrossRef]
- Joergensen, J.E.; Rasmussen, S.E. A neutron diffraction study of Cr3Si. Acta Cryst. B 1982, 38, 346–347. [Google Scholar] [CrossRef]
- Buschow, K.H.J.; van Engen, P.G.; Jongebreur, R. Magneto-optical properties of metallic ferromagnetic materials. J. Magn. Magn. Mater. 1983, 38, 1–22. [Google Scholar] [CrossRef]
- Goldschmidt, H.J.; Brand, J.A. The constitution of the chromium-niobium-silicon system. J. Less-Common Met. 1961, 3, 34–43. [Google Scholar] [CrossRef]
- Weill, A.R. Structure of the Eta Phase of the Iron-Silicon System. Nature 1943, 152, 413. [Google Scholar] [CrossRef]
- Dauben, C.H.; Templeton, D.H.; Myers, C.E. The crystal structure of Cr5Si3. J. Phys. Chem. 1956, 60, 443–445. [Google Scholar] [CrossRef]
- Aronsson, B. An investigation of the Me5Si3-MeSi region of the Mn-Fe-Si and some related systems. Acta Chem. Scand. 1958, 12, 308–313. [Google Scholar] [CrossRef]
- Brukl, C.; Nowotny, H.; Benesovsky, F. Untersuchungen in den Dreistoffsystemen: V-Al-Si, Nb-Al -Si, Cr-Al-Si, Mo-Al-Si bzw. Cr(Mo)-Al-Si. Mon. Chem. 1961, 92, 967–980. [Google Scholar] [CrossRef]
- Johnson, V.; Weiher, J.F.; Frederick, C.G.; Rogers, D.B. Magnetic and Moessbauer effect studies of Mn5Si3:Fe5Si3 solid solutions. J. Solid State Chem. 1972, 4, 311–323. [Google Scholar] [CrossRef]
- Vočadlo, L.; Knight, K.S.; Price, G.D.; Wood, I.G. Thermal expansion and crystal structure of FeSi between 4 and 1173 K determined by time-of-flight neutron powder diffraction. Phys. Chem. Miner. 2002, 29, 132–139. [Google Scholar] [CrossRef]
- Pauling, L.; Soldate, A.M. The nature of the bonds in the iron silicide FeSi and related crystals. Acta Cryst. 1948, 1, 212–216. [Google Scholar] [CrossRef]
- Dusausoy, Y.; Protas, J. Structure cristalline du disilicure de fer, FeSi2 beta. Acta Cryst. B 1971, 27, 1209–1218. [Google Scholar] [CrossRef]
- Nishida, I.; Sakata, T. Semiconducting properties of pure and Mn-doped chromium disilicides. J. Phys. Chem. Solids 1978, 39, 499–505. [Google Scholar] [CrossRef]
- Yamane, H.; Yamada, T. Effects of stacking fault on the diffraction intensities of beta-(FeSi2). J. Alloys Compd. 2009, 476, 282–287. [Google Scholar] [CrossRef]
- Zelenin, L.P.; Radovskii, I.Z.; Sidorenko, F.A.; Gel’d, P.V.; Rabinovich, B.S. Structural characteristics of solid solutions of chromium disilicide with vanadium and titanium disilicides. Powder Metall. Met. Ceram. 1966, 5, 896–900. [Google Scholar] [CrossRef]
- Mason, K.; Mueller-Vogt, G. Osmium disilicide: Preparation, crystal growth, and physical properties of a new semiconducting compound. J. Cryst. Growth 1983, 63, 34–38. [Google Scholar] [CrossRef]
- Tanaka, K.; Nawata, K.; Inui, H.; Yamaguchi, M.; Koiwa, M. Refinement of crystallographic parameters in refractory metal disilicides. MRS Online Proc. Libr. (OPL) 2001, 646, N4.3.1–N4.3.5. [Google Scholar] [CrossRef]
- Li, G.; Nicheng, S.; Ming, X.; Zhesheng, M.; Wenji, B.; Qingsong, F. X-ray diffraction investigation of native Si-Fe alloy minerals from Luobusha, Tibet. Front. Earth Sci. China 2007, 1, 21–25. [Google Scholar] [CrossRef]
- Bond, W.L.; Kaiser, W. Interstitial versus substitutional oxygen in silicon. J. Phys. Chem. Solids 1960, 16, 44–45. [Google Scholar] [CrossRef]
- Wittmann, A.; Burger, K.O.; Nowotny, H. Untersuchungen im Dreistoff: Ni-Al-Si sowie von Mono- und Disilicidsystemen einiger Uebergangsmetalle. Mon. Chem. 1962, 93, 674–680. [Google Scholar] [CrossRef]
- Straumanis, M.E.; Aka, E.Z. Lattice parameters, coefficients of thermal expansion and atomic weights of purest silicon and germanium. J. Appl. Phys. 1952, 23, 330–334. [Google Scholar] [CrossRef]
- Robinson, V.N.E. Imaging with backscattered electrons in a scanning electron microscope. Scanning 1980, 3, 15–26. [Google Scholar] [CrossRef]
- Lloyd, G.E. Atomic number and crystallographic contrast images with the SEM: A review of backscattered electron techniques. Mineral. Mag. 1987, 51, 3–19. [Google Scholar] [CrossRef]
- Goldstein, J.I.; Newbury, D.E.; Michael, J.R.; Ritchie, N.W.M.; Scott, J.H.J.; Joy, D.C. Backscattered Electrons. In Scanning Electron Microscopy and X-ray Microanalysis; Goldstein, J.I., Newbury, D.E., Michael, J.R., Ritchie, N.W.M., Scott, J.H.J., Joy, D.C., Eds.; Springer: New York, NY, USA, 2017; pp. 15–28. [Google Scholar]
- Gladyshevskii, E.I. Crystal structure of compounds and phase equilibria in ternary systems of two transition metals and silicon. Powder Metall. Met. Ceram. 1962, 1, 262–265. [Google Scholar] [CrossRef]
- Vecherskii, S.I.; Sidorenko, F.A. Effect of phase transformation in higher iron silicide on the electrical conductivity of the multiphase system iron silicide-glass. II. Effect of structural transformation in lebeauite on the temperature dependence for composite resistivity. Sov. Powder Metall. Met. Ceram. 1992, 31, 835–838. [Google Scholar] [CrossRef]
- Stadelmann, P.A. EMS—A software package for electron diffraction analysis and HREM image simulation in materials science. Ultramicroscopy 1987, 21, 131–146. [Google Scholar] [CrossRef]
- Imamov, R.M.; Klechkovskaya, V.V.; Suvorova, E.I. High-Resolution Transmission Electron Microscopy for Crystallographic Study of Nanomaterials. Crystallogr. Rep. 2011, 56, 650–661. [Google Scholar] [CrossRef]
- Howe, J.M.; Aaronson, H.I.; Hirth, J.P. Aspects of interphase boundary structure in diffusional phase transformations. Acta Mater. 2000, 48, 3977–3984. [Google Scholar] [CrossRef]
- Rabinovich, B.S.; Radovskii, I.Z.; Gel’d, P.V. Coefficients of thermal expansion of chromium disilicide and its solid solutions with TiSi2 and VSi2. Sov. Powder Metall. Met. Ceram. 1968, 7, 879–883. [Google Scholar] [CrossRef]
- Imai, M.; Isoda, Y.; Udono, H. Thermal expansion of semiconducting silicides β-FeSi2 and Mg2Si. Intermetallics 2015, 67, 75–80. [Google Scholar] [CrossRef]
- Krentsis, R.P.; Kalishevich, G.I.; Gel’d, P.V.; Andreeva, L.P. Thermal expansion of monosilicides of chromium, manganese, iron, and cobalt. Sov. Phys. J. Vol. 1972, 15, 143–145. [Google Scholar] [CrossRef]
- Mazur, A.V.; Gasik, M.M. Thermal expansion of silicon at temperatures up to 1100 °C. J. Mater. Process. Tech. 2009, 209, 723–727. [Google Scholar] [CrossRef]
- Kladko, V.; Kuchuk, A.; Lytvyn, P.; Yefanov, O.; Safriuk, N.; Belyaev, A.; Mazur, Y.I.; DeCuir, E.A., Jr.; Ware, M.E.; Salamo, G.J. Substrate effects on the strain relaxation in GaN/AlN short-period superlattices. Nanoscale Res. Lett. 2012, 7, 289. [Google Scholar] [CrossRef]
- Liu, H.; Cheng, X.; Valanoor, N. A universal approach for predicting crystallography of heterogeneous epitaxial nanocrystals with multiple orientation relationships. ACS Appl. Mater. Interfaces 2016, 8, 34844–34853. [Google Scholar] [CrossRef] [PubMed]
- Hoeft, H.; Schwaab, P. Investigations towards optimizing EDS analysis by the Cliff-Lorimer method in scanning transmission electron microscopy. X-ray Spectrom. 1988, 17, 201–208. [Google Scholar] [CrossRef]
- Yakowitz, H. Methods of quantitative analysis. In Practical Scanning Electron Microscopy; Goldstein, J.I., Yakowitz, H., Newbury, D.E., Lifshin, E., Colby, J.W., Coleman, J.R., Eds.; Plenum Press: New York, NY, USA, 1975; pp. 327–332. [Google Scholar]
- Newbury, D.E.; Ritchie, N.W.M. Electron-Excited X-ray Microanalysis by Energy Dispersive Spectrometry at 50: Analytical Accuracy, Precision, Trace Sensitivity, and Quantitative Compositional Mapping. Microsc. Microanal. 2019, 25, 1075–1105. [Google Scholar] [CrossRef] [PubMed]
- Stadelmann, P. JEMS. 2021. Available online: https://www.jems-swiss.ch/ (accessed on 19 January 2023).
- Setton, M.; van der Spiegel, J. Silicide formation from ternary metal-metal-silicon systems. Thin Solid Film. 1988, 156, 351–363. [Google Scholar] [CrossRef]
- Cui, S.; Jung, I.-H. Thermodynamic assessments of the Fe-Si-Cr and Fe-Si-Mg systems. Metall. Mater. Trans. A 2017, 48, 4342–4355. [Google Scholar] [CrossRef]




| Fe–Si | Cr–Si | ||||
|---|---|---|---|---|---|
| Phase | Space Group; Lattice Parameters (nm) | # ICSD | Phase | Space Group; Lattice Parameters (nm) | # ICSD |
| Fe3Si | Fmm; a = 0.5665 [30]; | 412838 | Cr3Si | Pmn; a = 0.45599 [31]; | 32509 |
| Fmm; a = 0.5662 [32]. | 633537 | Pmn; a = 0.4539 [33]. | 199130 | ||
| Fe5Si3 | P63/mcm; a = 0.67552, c = 0.47174 [34]; | 42585 | Cr5Si3 | I4/mcm; a = 0.917, c = 0.4636 [35]; | 15683 |
| P63/mcm; a = 0.6755, c = 0.4715 [36]; | 633525 | I4/mcm; a = 0.915, c = 0.464 [37]. | 626782 | ||
| P63/mcm; a = 0.6756, c = 0.4718 [38]. | 633540 | ||||
| ε-FeSi | P213; a= 0.445507 [39]; | 5250 | CrSi | P213; a = 0.4629 [33]. | 626772 |
| P213; a= 0.4489 [40]; | 76945 | ||||
| β-FeSi2 | Cmca, a= 0.9863, b = 0.7791, c = 0.7833 [41]; | 9119 | CrSi2 | P6222; a = 0.4428, c = 0.6364 [42]; | 626776 |
| Cmca, a= 0.9876, b = 0.7798, c = 0.7836 [43]; | 163384 | P6222; a = 0.4428, c = 0.6364 [44]; | 626787 | ||
| Cmca, a= 0.988, b = 0.7798, c = 0.7839 [45]. | 603890 | P6422; a = 0.4283, c = 0.6368 [46]; | 96026 | ||
| α-FeSi2 | P4/mmm, a = 0.2725, c = 0.5202 [47]; | 5258 | Si | Fdm, a = 0.54307 [48]; | 29287 |
| P4/mmm, a = 0.269, c = 0.5133 [49]. | 633544 | Fdm, a = 0.543086 [50]. | 76268 | ||
| As Grown (Non-Annealed) | Annealed | ||
|---|---|---|---|
| Rwp = 13.4%, GOF = 6.4 | Rwp = 11.5%, GOF = 5.2 | ||
| phase | weight % | Phase | weight % |
| CrSi2 | 50.5 (4) | CrSi2 | 50.7 (3) |
| α−FeSi2 | 41.9 (4) | β−FeSi2 | 46.8 (3) |
| ε−FeSi | 7.5 (2) | ε−FeSi | 1.3 (1) |
| Si | Not found | Si | 1.2 (1) |
| Material | hkl | d, nm | α·10−6 K−1 |
|---|---|---|---|
| CrSi2 hex | (200) (024) (0003) | 0.19148 0.1222 0.2117 | 12.87 |
| α–FeSi2 tetr | (10) | 0.19057 | 12.0 |
| β–FeSi2 orth | (713) (3) (8) | 0.12246 0.13259 0.09325 | 11.8 |
| ε–FeSi cub | (02) (10) | 0.20351 0.20351 | 15.5 |
| Cr5Si3 tetr | (233) | 0.13207 | 14.07 |
| Si cub | (60) | 0.08617 | 4.3 |
| Interfaces | Misfit, δ therm | Mismatch, δ lattice |
|---|---|---|
| CrSi2 hex–α-FeSi2 tetr | 0–2.9·10−2% | 0.5% |
| CrSi2 hex–β-FeSi2 tetr | 7.8·10−2% | 0.2% |
| CrSi2 hex–ε-FeSi cub | 0.2% | 4.1% |
| β-FeSi2 tetr–Cr5Si3 tetr | 0.2% | 0.4% |
| β-FeSi2 tetr–Si cub | 0.6% | 7.9% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Suvorova, E.I.; Arkharova, N.A.; Ivanova, A.G.; Solomkin, F.Y.; Buffat, P.A. Phases and Interfaces in the Cr–Fe–Si Ternary System: X-ray Diffraction and Electron Microscopy Study. Inorganics 2023, 11, 73. https://doi.org/10.3390/inorganics11020073
Suvorova EI, Arkharova NA, Ivanova AG, Solomkin FY, Buffat PA. Phases and Interfaces in the Cr–Fe–Si Ternary System: X-ray Diffraction and Electron Microscopy Study. Inorganics. 2023; 11(2):73. https://doi.org/10.3390/inorganics11020073
Chicago/Turabian StyleSuvorova, Elena I., Natalya A. Arkharova, Anna G. Ivanova, Fedor Yu. Solomkin, and Philippe A. Buffat. 2023. "Phases and Interfaces in the Cr–Fe–Si Ternary System: X-ray Diffraction and Electron Microscopy Study" Inorganics 11, no. 2: 73. https://doi.org/10.3390/inorganics11020073
APA StyleSuvorova, E. I., Arkharova, N. A., Ivanova, A. G., Solomkin, F. Y., & Buffat, P. A. (2023). Phases and Interfaces in the Cr–Fe–Si Ternary System: X-ray Diffraction and Electron Microscopy Study. Inorganics, 11(2), 73. https://doi.org/10.3390/inorganics11020073






