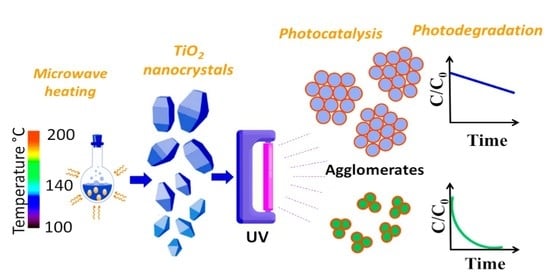
Synergistic Correlation in the Colloidal Properties of TiO2 Nanoparticles and Its Impact on the Photocatalytic Activity
Abstract
:1. Introduction
2. Results and Discussion
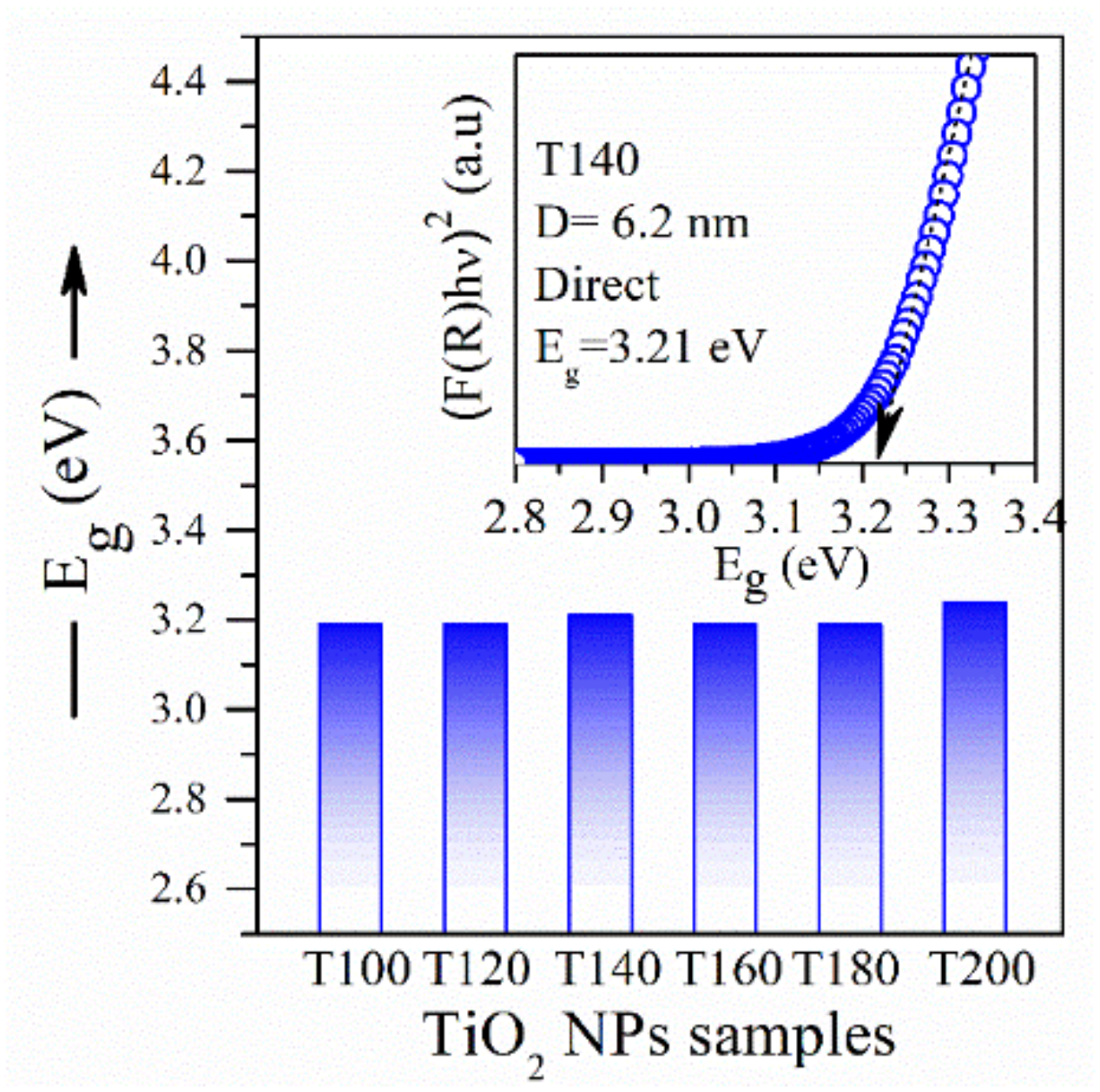
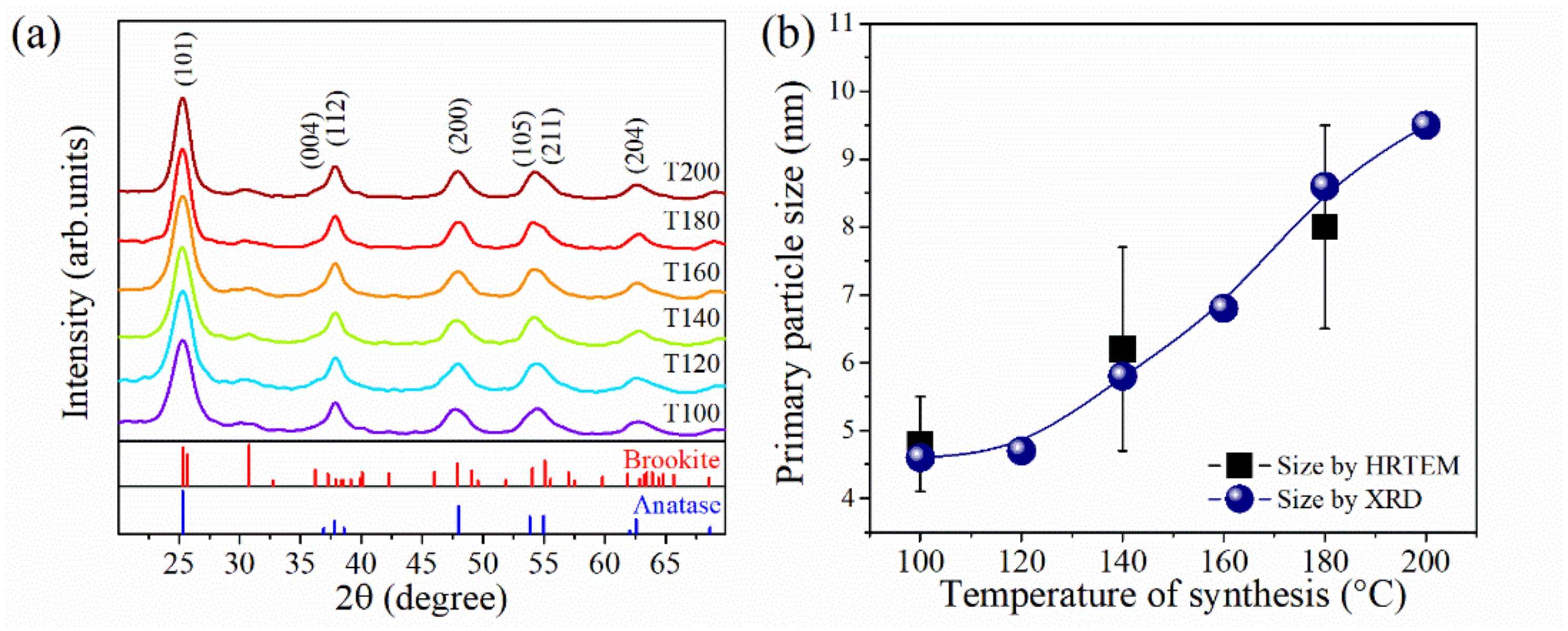
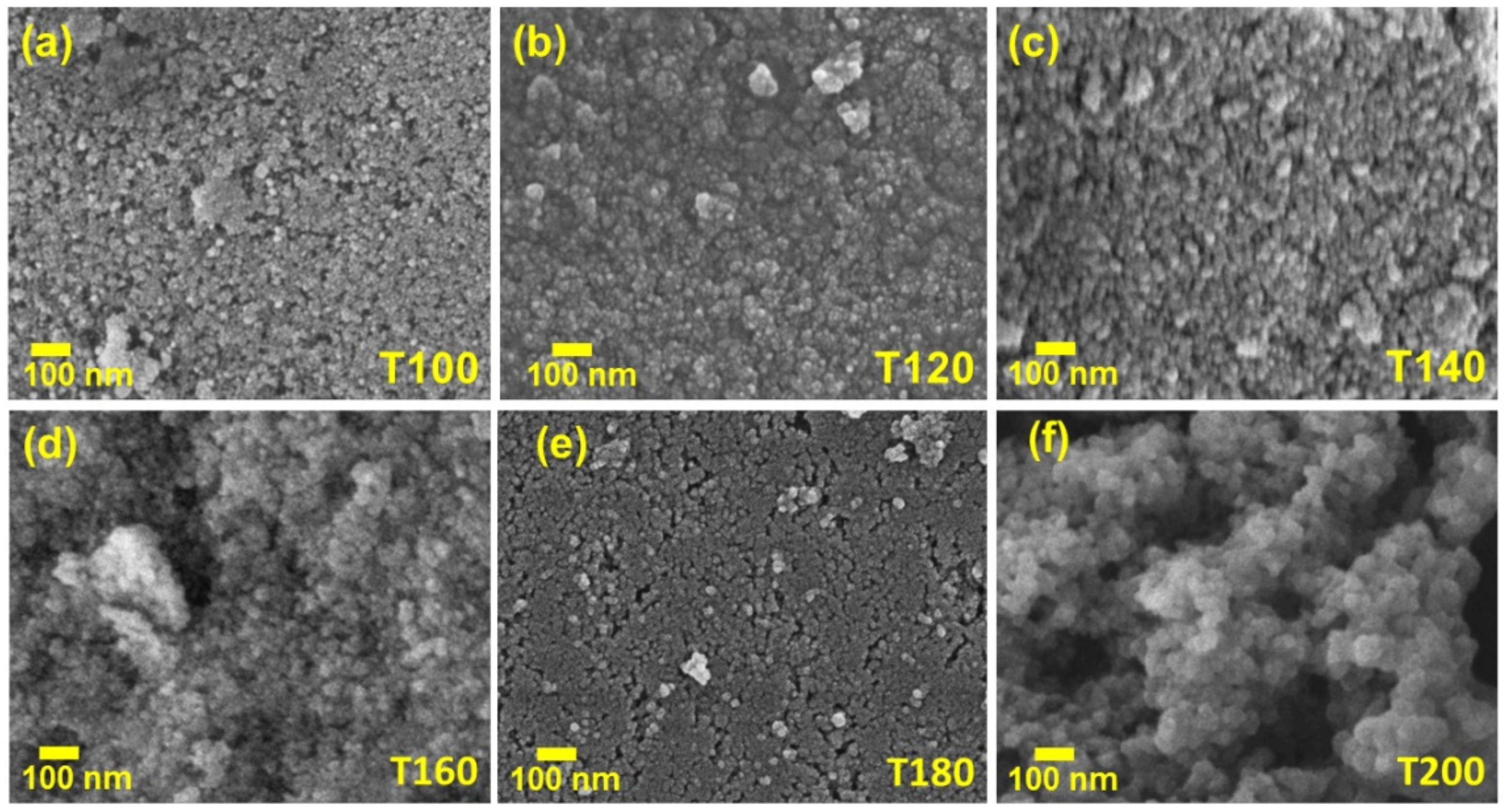
2.1. Crystal Structure and Morphology of TiO2 NPs
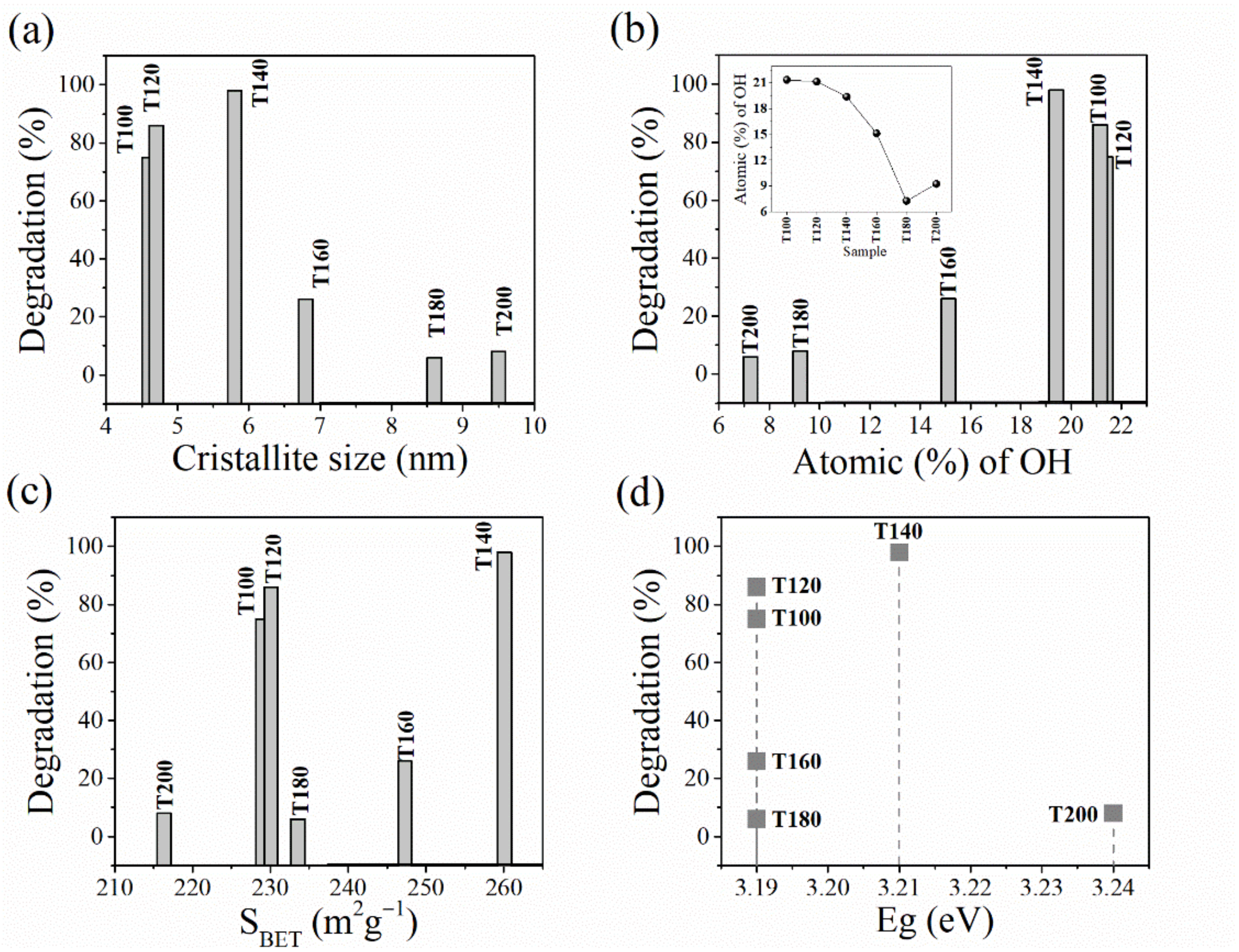
2.2. Elemental Analysis and Textural Surface Characterization of TiO2 NPs
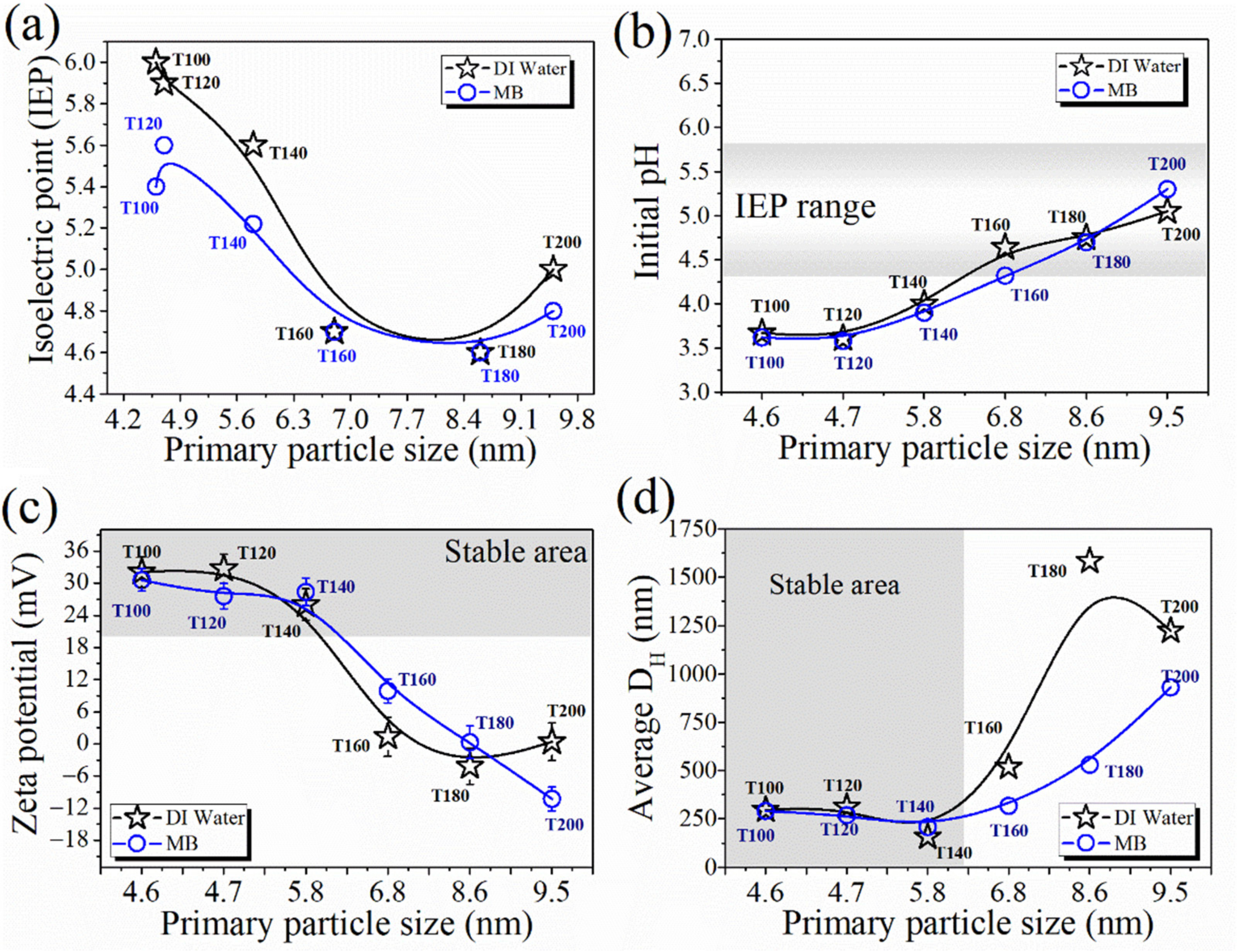
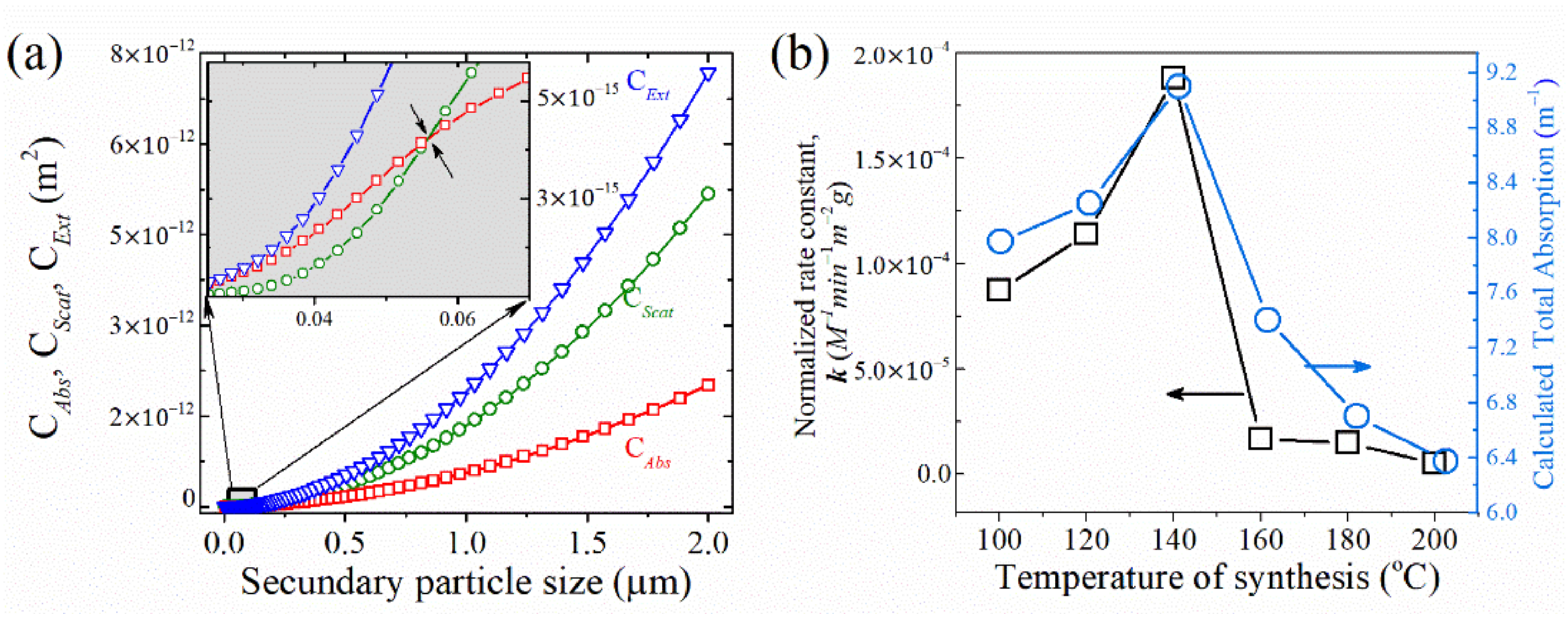
2.3. Colloidal Dispersion Properties on the Photocatalytic Activity
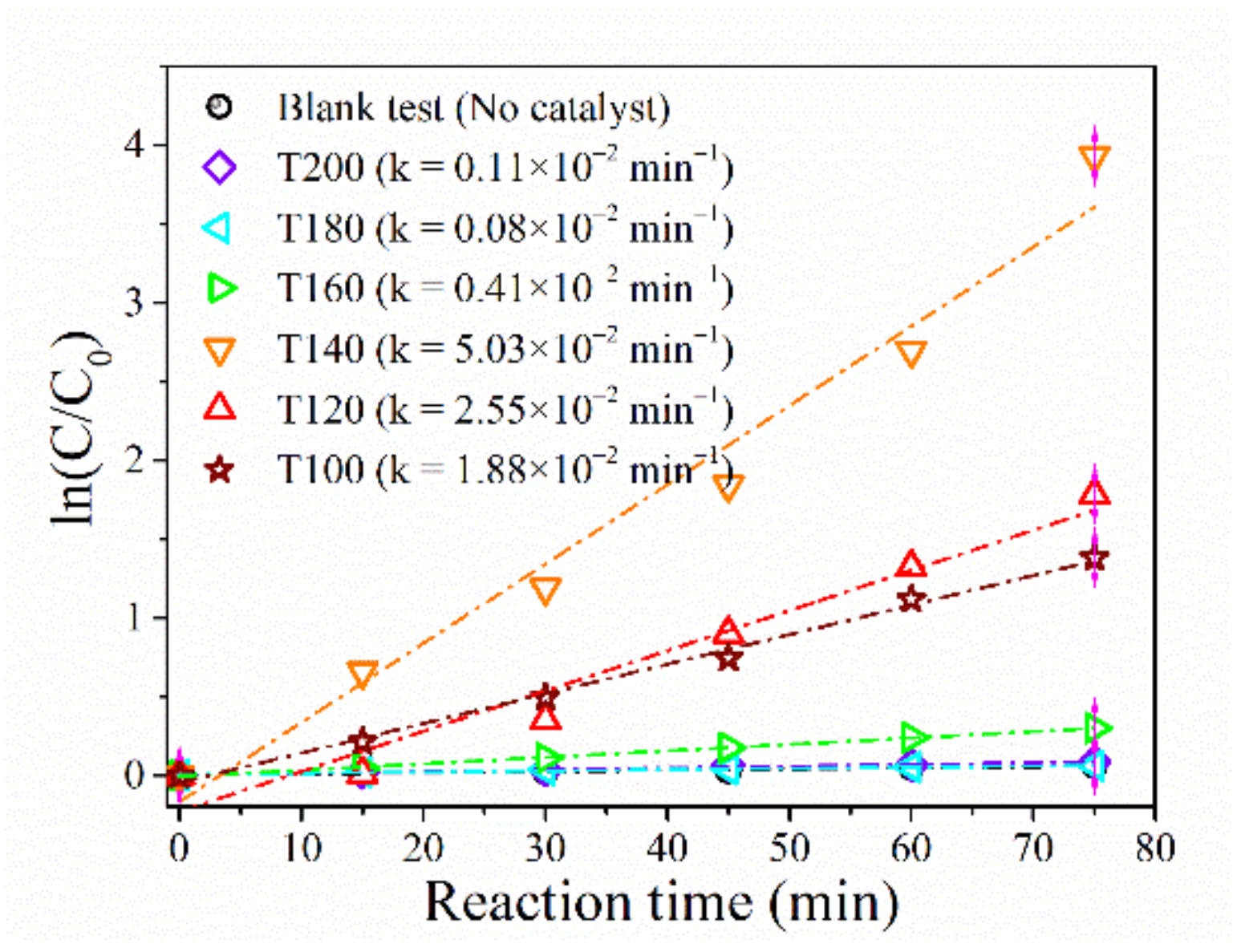
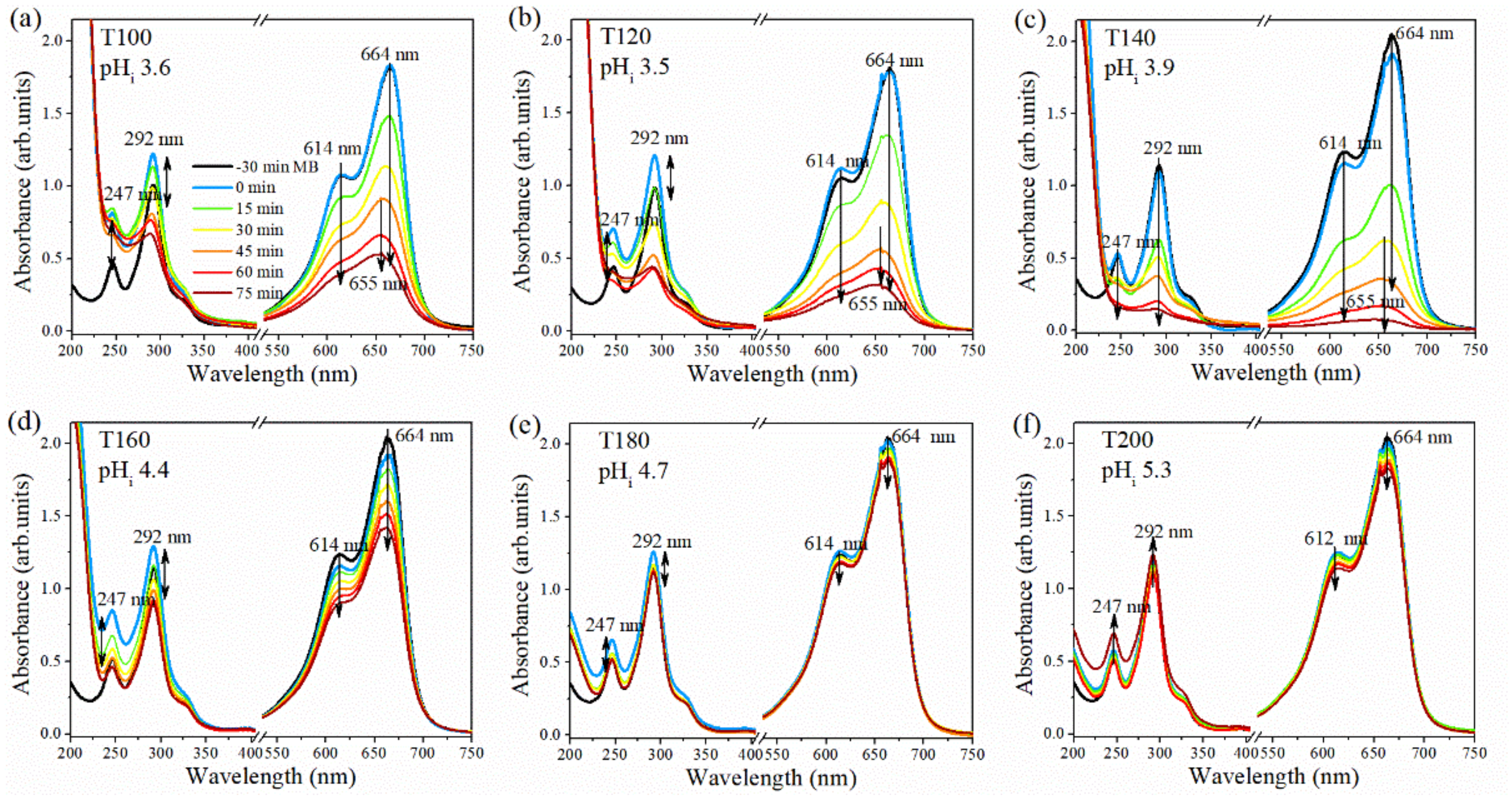
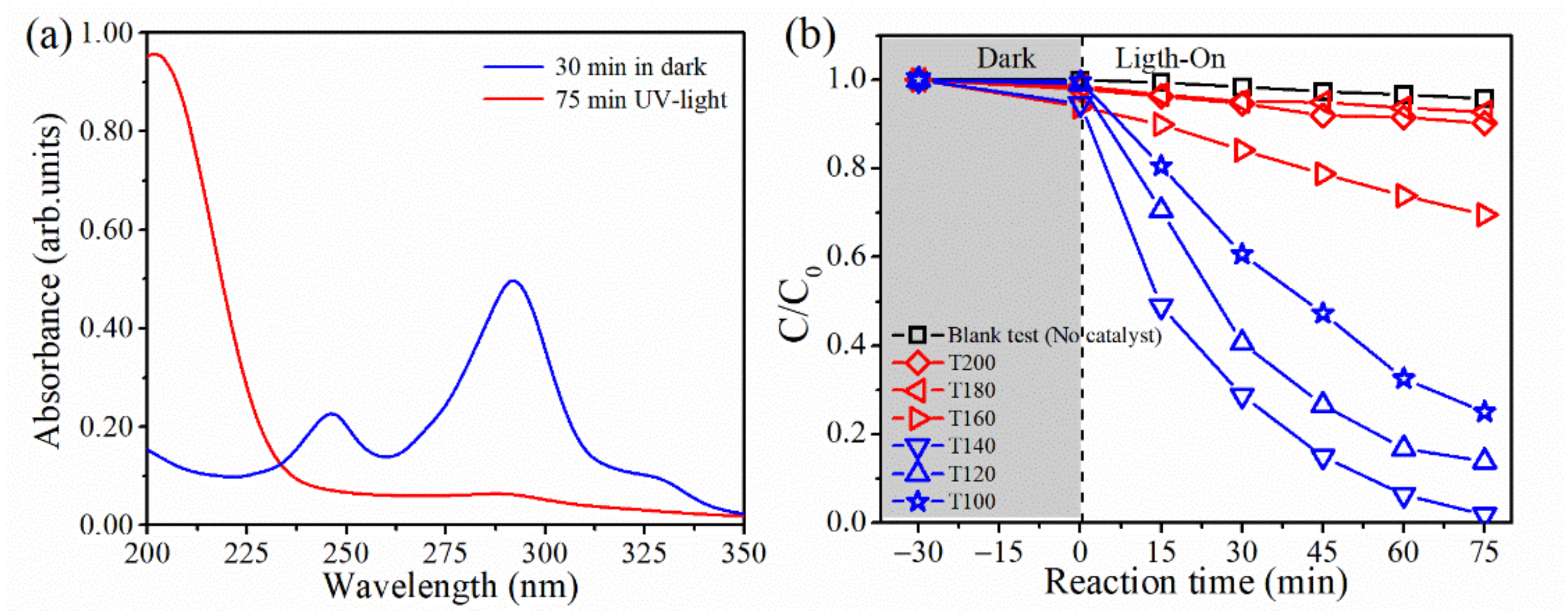
2.4. Photocatalytic Activity Measurements
3. Materials and Methods
3.1. TiO2 NP Powder Synthesis and Characterization
3.2. Characterization of TiO2 Dispersions
3.3. Photodegradation of MB
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A


References
- Kim, D.S.; Han, S.J.; Kwak, S.Y. Synthesis and photocatalytic activity of mesoporous TiO2 with the surface area, crystallite size, and pore size. J. Colloid Interface Sci. 2007, 316, 85–91. [Google Scholar] [CrossRef] [PubMed]
- Lazar, M.; Varghese, S.; Nair, S. Photocatalytic water treatment by titanium dioxide: Recent updates. Catalysts 2012, 2, 572–601. [Google Scholar] [CrossRef]
- Salameh, C.; Nogier, J.-P.; Launay, F.; Boutros, M. Dispersion of colloidal TiO2 nanoparticles on mesoporous materials targeting photocatalysis applications. Catal. Today 2015, 257, 35–40. [Google Scholar] [CrossRef]
- Tiwari, V.; Jiang, J.; Sethi, V.; Biswas, P. One-step synthesis of noble metal–titanium dioxide nanocomposites in a flame aerosol reactor. Appl. Catal. A-Gen. 2008, 345, 241–246. [Google Scholar] [CrossRef]
- Biswas, P.; Wu, C.Y. Control of toxic metal emissions from combustors using sorbents: A review. J. Air Waste Manag. Assoc. 2011, 48, 113–127. [Google Scholar] [CrossRef] [PubMed]
- Savage, N.; Diallo, M.S. Nanomaterials and water purification: Opportunities and challenges. J. Nanopart. Res. 2005, 7, 331–342. [Google Scholar] [CrossRef]
- Długosz, O.; Staroń, A.; Brzoza, P.; Banach, M. Synergistic effect of sorption and photocatalysis on the degree of dye removal in single and multicomponent systems on ZnO-SnO2. Environ. Sci. Pollut. Res. 2022, 29, 27042–27050. [Google Scholar] [CrossRef] [PubMed]
- Luna, M.; Delgado, J.J.; Romero, I.; Montini, T.; Almoraima Gil, M.L.; Martínez-López, J.; Fornasiero, P.; Mosquera, M.J. Photocatalytic TiO2 nanosheets-SiO2 coatings on concrete and limestone: An enhancement of de-polluting and self-cleaning properties by nanoparticle design. Constr. Build. Mater. 2022, 338, 127349. [Google Scholar] [CrossRef]
- Almquist, C.B.; Biswas, P. Role of synthesis method and particle size of nanostructured TiO2 on its photoactivity. J. Catal. 2002, 212, 145–156. [Google Scholar] [CrossRef]
- Lin, H.; Huang, C.; Li, W.; Ni, C.; Shah, S.; Tseng, Y. Size dependency of nanocrystalline TiO2 on its optical property and photocatalytic reactivity exemplified by 2-chlorophenol. Appl. Catal. B-Environ. 2006, 68, 1–11. [Google Scholar] [CrossRef]
- Xu, N.; Shi, Z.; Fan, Y.; Dong, J.; Shi, J.; Hu, M.Z.-C. Effects of particle size of TiO2 on photocatalytic degradation of methylene blue in aqueous suspensions. Ind. Eng. Chem. Res. 1999, 38, 373–379. [Google Scholar] [CrossRef]
- Behnajady, M.A.; Modirshahla, N.; Shokri, M.; Elham, H.; Zeininezhad, A. The effect of particle size and crystal structure of titanium dioxide nanoparticles on the photocatalytic properties. J. Environ. Sci. Health Part A 2008, 43, 460–467. [Google Scholar] [CrossRef] [PubMed]
- Wu, Z.; Cao, S.; Zhang, C.; Piao, L. Effects of bulk and surface defects on the photocatalytic performance of size-controlled TiO2 nanoparticles. Nanotechnology 2017, 28, 275706. [Google Scholar] [CrossRef] [PubMed]
- Byrne, J.A.; Eggins, B.R.; Linquette-Mailleyb, S.; Dunlopa, P.S.M. The effect of hole acceptors on the photocurrent response of particulate TiO2 anodes. Analyst 1998, 123, 2007–2012. [Google Scholar] [CrossRef]
- Du, Y.; Rababi, J. The measure of TiO2 photocatalytic efficiency and the comparison of different photocatalytic titania. J. Phys. Chem. B 2003, 107, 11970–11978. [Google Scholar] [CrossRef]
- Štengl, V.; Králová, D. Photoactivity of brookite–rutile TiO2 nanocrystalline mixtures obtained by heat treatment of hydrothermally prepared brookite. Mater. Chem. Phys. 2011, 129, 794–801. [Google Scholar] [CrossRef]
- Yu, Y.; Yu, J.C.; Yu, J.-G.; Kwok, Y.-C.; Che, Y.-K.; Zhao, J.-C.; Ding, L.; Ge, W.-K.; Wong, P.-K. Enhancement of photocatalytic activity of mesoporous TiO2 by using carbon nanotubes. Appl. Catal. A-Gen. 2005, 289, 186–196. [Google Scholar] [CrossRef]
- Meire, M.; Verbruggen, S.W.; Lenaerts, S.; Lommens, P.; Van Der Voort, P.; Van Driessche, I. Microwave-assisted synthesis of mesoporous titania with increased crystallinity, specific surface area, and photocatalytic activity. J. Mater. Sci. 2016, 51, 9822–9829. [Google Scholar] [CrossRef]
- Schwarze, M.; Klingbeil, C.; Do, H.U.; Kutorglo, E.M.; Parapat, R.Y.; Tasbihi, M. Highly active TiO2 photocatalysts for hydrogen production through a combination of commercial TiO2 material selection and platinum co-catalyst deposition using a colloidal approach with green reductants. Catalysts 2021, 11, 1027. [Google Scholar] [CrossRef]
- Siwińska-Ciesielczyk, K.; Bartlewicz, O.; Bartczak, P.; Piasecki, A.; Jesionowski, T. Functional titania–silica/chlorophyllin hybrids: Design, fabrication, comprehensive physicochemical characteristic and photocatalytic test. Adsorption 2019, 25, 485–499. [Google Scholar] [CrossRef] [Green Version]
- Li, W.; Zhao, Y.; Yuan, S.; Wang, Z.; Fang, J.; Shi, L.; Zhang, M. Synthesis and characterization of highly dispersed TiO2 nanocrystal colloids by microwave-assisted hydrothermal method. J. Mater. Sci. 2012, 47, 7999–8006. [Google Scholar] [CrossRef]
- Maira, A.J.; Yeung, K.L.; Lee, C.Y.; Yue, P.L.; Chan, C.K. Size effects in gas-phase photo-oxidation of trichloroethylene using nanometer-sized TiO2 catalysts. J. Catal. 2000, 192, 185–196. [Google Scholar] [CrossRef]
- Kočí, K.; Obalová, L.; Matějová, L.; Plachá, D.; Lacný, Z.; Jirkovský, J.; Šolcová, O. Effect of TiO2 particle size on the photocatalytic reduction of CO2. Appl. Catal. B-Environ. 2009, 89, 494–502. [Google Scholar] [CrossRef]
- Akpan, U.G.; Hameed, B.H. Parameters affecting the photocatalytic degradation of dyes using TiO2-based photocatalysts: A review. J. Hazard. Mater. 2009, 170, 520–529. [Google Scholar] [CrossRef] [PubMed]
- Lakshminarasimhan, N.; Kim, W.; Choi, W. Effect of the agglomerated state on the photocatalytic hydrogen production with in situ agglomeration of colloidal TiO2 nanoparticles. J. Phys. Chem. C 2008, 112, 20451–20457. [Google Scholar] [CrossRef]
- Tang, W.Z.; Zhang, Z.; An, H.; Quintana, M.O.; Torres, D.F. TiO2/UV photodegradation of azo dyes in aqueous solutions. Environ. Sci. Technol. 1997, 18, 1–12. [Google Scholar] [CrossRef]
- Stylidi, M.; Kondarides, D.I.; Verykios, X.E. Visible light-induced photocatalytic degradation of Acid Orange 7 in aqueous TiO2 suspensions. Appl. Catal. B-Environ. 2004, 47, 189–201. [Google Scholar] [CrossRef]
- Sienkiewicz, A.; Rokicka-Konieczna, P.; Wanag, A.; Kusiak-Nejman, E.; Morawski, A.W. Artificial solar light-driven APTES/TiO2 photocatalysts for methylene blue removal from water. Molecules 2022, 27, 947. [Google Scholar] [CrossRef]
- Wen, J.; Li, X.; Liu, W.; Fang, Y.; Xie, J.; Xu, Y. Photocatalysis fundamentals and surface modification of TiO2 nanomaterials. Chin. J. Catal. 2015, 36, 2049–2070. [Google Scholar] [CrossRef]
- Dong, H.; Zeng, G.; Tang, L.; Fan, C.; Zhang, C.; He, X.; He, Y. An overview on limitations of TiO2-based particles for photocatalytic degradation of organic pollutants and the corresponding countermeasures. Water Res. 2015, 79, 128–146. [Google Scholar] [CrossRef]
- Wang, N.; Li, J.; Zhu, L.; Dong, Y.; Tang, H. Highly photocatalytic activity of metallic hydroxide/titanium dioxide nanoparticles prepared via a modified wet precipitation process. J. Photochem. Photobiol. A 2008, 198, 282–287. [Google Scholar] [CrossRef]
- Azeez, F.; Al-Hetlani, E.; Arafa, M.; Abdelmonem, Y.; Nazeer, A.A.; Amin, M.O.; Madkour, M. The effect of surface charge on photocatalytic degradation of methylene blue dye using chargeable titania nanoparticles. Sci. Rep. 2018, 8, 7104. [Google Scholar] [CrossRef] [PubMed]
- Bubacz, K.; Choina, J.; Dolat, D.; Morawski, A.W. Methylene blue and phenol photocatalytic degradation on nanoparticles of anatase TiO2. Pol. J. Environ. Stud. 2010, 19, 685–691. [Google Scholar]
- Kim, W.; Tachikawa, T.; Moon, G.-h.; Majima, T.; Choi, W. Molecular-level understanding of the photocatalytic activity difference between anatase and rutile nanoparticles. Angew. Chem. Int. Ed. 2014, 53, 14036–14041. [Google Scholar] [CrossRef]
- Harris, J.; Silk, R.; Smith, M.; Dong, Y.; Chen, W.-T.; Waterhouse, G.I.N. Hierarchical TiO2 nanoflower photocatalysts with remarkable activity for aqueous methylene blue photo-oxidation. ACS Omega 2020, 5, 18919–18934. [Google Scholar] [CrossRef]
- Egerton, T.A. UV-Absorption—The primary process in photocatalysis and some practical consequences. Molecules 2014, 19, 18192–18214. [Google Scholar] [CrossRef]
- Tolosana-Moranchel, A.; Pecharromán, C.; Faraldos, M.; Bahamonde, A. Strong effect of light scattering by distribution of TiO2 particle aggregates on photocatalytic efficiency in aqueous suspensions. Chem. Eng. J. 2021, 403, 126186. [Google Scholar] [CrossRef]
- Ruan, Z.-H.; Gao, X.; Yuan, Y.; Tan, H.-P. Theoretical insight into the effect of Br, Na co-doping on electronic structure, photocatalytic and optical characteristics of g-C3N4 using first-principles and optical simulations. J. Mater. Sci. 2021, 56, 10382–10392. [Google Scholar] [CrossRef]
- Egerton, T.A.; Purnama, H. Does hydrogen peroxide really accelerate TiO2 UV-C photocatalyzed decolouration of azo-dyes such as Reactive Orange 16? Dye. Pigment. 2014, 101, 280–285. [Google Scholar] [CrossRef]
- Baxendale, J.H.; Wilson, J.A. The photolysis of hydrogen peroxide at high light intensities. Trans. Faraday Soc. 1957, 53, 344–356. [Google Scholar] [CrossRef]
- Kormann, C.; Bahnemann, D.W.; Hoffmann, M.R. Photolysis of chloroform and other organic molecules in aqueous titanium dioxide suspensions. Environ. Sci. Technol. 1991, 25, 494–500. [Google Scholar] [CrossRef]
- Ceballos-Chuc, M.C.; Ramos-Castillo, C.M.; Alvarado-Gil, J.J.; Oskam, G.; Rodríguez-Gattorno, G. Influence of brookite impurities on the Raman spectrum of TiO2 anatase nanocrystals. J. Phys. Chem. C 2018, 122, 19921–19930. [Google Scholar] [CrossRef]
- León, A.; Reuquen, P.; Garín, C.; Segura, R.; Vargas, P.; Zapata, P.; Orihuela, P. FTIR and Raman characterization of TiO2 nanoparticles coated with polyethylene glycol as carrier for 2-methoxyestradiol. Appl. Sci. 2017, 7, 49. [Google Scholar] [CrossRef]
- Oskam, G.; Hu, Z.; Penn, R.L.; Pesika, N.; Searson, P.C. Coarsening of metal oxide nanoparticles. Phys. Rev. E Stat. Nonlinear Soft Matter Phys. 2002, 66, 011403. [Google Scholar] [CrossRef] [PubMed]
- Sreekantan, S.; Saharudin, K.A.; Lockman, Z.; Tzu, T.W. Fast-rate formation of TiO2 nanotube arrays in an organic bath and their applications in photocatalysis. Nanotechnology 2010, 21, 365603. [Google Scholar] [CrossRef] [PubMed]
- Zhou, X.; Yang, F.; Jin, B.; Huang, Y.; Wu, Z. Hydrothermal fabrication of Ti3+ self-doped TiO2 nanorods with high visible light photocatalytic activity. Mater. Lett. 2013, 112, 145–148. [Google Scholar] [CrossRef]
- Choi, H.; Lee, J.; Kim, D.; Kumar, A.; Jeong, B.; Kim, K.-J.; Lee, H.; Park, J.Y. Influence of lattice oxygen on the catalytic activity of blue titania supported Pt catalyst for CO oxidation. Catal. Sci. Technol. 2021, 11, 1698–1708. [Google Scholar] [CrossRef]
- He, Z.; Que, W.; Chen, J.; He, Y.; Wang, G. Surface chemical analysis on the carbon-doped mesoporous TiO2 photocatalysts after post-thermal treatment: XPS and FTIR characterization. J. Phys. Chem. Solids 2013, 74, 924–928. [Google Scholar] [CrossRef]
- Lee, H.U.; Lee, S.C.; Choi, S.; Son, B.; Kim, H.; Lee, S.M.; Kim, H.J.; Lee, J. Influence of visible-light irradiation on physicochemical and photocatalytic properties of nitrogen-doped three-dimensional (3D) titanium dioxide. J. Hazard. Mater. 2013, 258, 10–18. [Google Scholar] [CrossRef]
- Im, J.H.; Yang, S.J.; Yun, C.H.; Park, C.R. Simple fabrication of carbon/TiO2 composite nanotubes showing dual functions with adsorption and photocatalytic decomposition of Rhodamine, B. Nanotechnology 2012, 23, 035604. [Google Scholar] [CrossRef]
- Mutuma, B.K.; Shao, G.N.; Kim, W.D.; Kim, H.T. Sol-gel synthesis of mesoporous anatase-brookite and anatase-brookite-rutile TiO2 nanoparticles and their photocatalytic properties. J. Colloid Interface Sci 2015, 442, 1–7. [Google Scholar] [CrossRef]
- Houas, A.; Lachheb, H.; Ksibi, M.; Elaloui, E.; Guillard, C.; Herrmann, J.-M. Photocatalytic degradation pathway of methylene blue in water. Appl. Catal. B-Environ. 2001, 31, 145–157. [Google Scholar] [CrossRef]
- Suttiponparnit, K.; Jiang, J.; Sahu, M.; Suvachittanont, S.; Charinpanitkul, T.; Biswas, P. Role of surface area, primary particle size, and crystal phase on titanium dioxide nanoparticle dispersion properties. Nanoscale Res. Lett. 2011, 6, 27. [Google Scholar] [CrossRef] [PubMed]
- Berg, J.M.; Romoser, A.; Banerjee, N.; Zebda, R.; Sayes, C.M. The relationship between pH and zeta potential of ∼30 nm metal oxide nanoparticle suspensions relevant toin vitrotoxicological evaluations. Nanotoxicology 2009, 3, 276–283. [Google Scholar] [CrossRef]
- Parks, G.a. The isoelectric points of solid oxides, solid hydroxides, and aqueous hydroxo complex systems. Chem. Rev. 1965, 65, 177–198. [Google Scholar] [CrossRef]
- Kosmulski, M. pH-dependent surface charging and points of zero charge. IV. Update and new approach. J. Colloid Interface Sci. 2009, 337, 439–448. [Google Scholar] [CrossRef]
- Kosmulski, M. The pH-dependent surface charging and the points of zero charge. J. Colloid Interface Sci. 2002, 253, 77–87. [Google Scholar] [CrossRef]
- Liao, D.L.; Liao, B.Q. Shape, size and photocatalytic activity control of TiO2 nanoparticles with surfactants. J. Photochem. Photobiol. A Chem. 2007, 187, 363–369. [Google Scholar] [CrossRef]
- Katafias, A.; Lipińska, M.; Strutyński, K. Alkaline hydrogen peroxide as a degradation agent of methylene blue kinetic and mechanistic studies. React. Kinet. Mech. Catal. 2010, 101, 251–266. [Google Scholar] [CrossRef]
- Hiroshi, O. Photoreduction of methylene blue by visible light in the aqueous solution containing certain kinds of inorganic salts. II. photobleached product. Bull. Chem. Soc. Jpn. 1961, 34, 1057–1063. [Google Scholar] [CrossRef]
- De Tacconi, N.R.; Carmona, J.; Rajeshwar, K. Reversibility of photoelectrochromism at the TiO2/methylene blue interface. J. Electrochem. Soc. 1997, 144, 2486–2490. [Google Scholar] [CrossRef]
- Dang, T.T.T.; Le, S.T.T.; Channei, D.; Khanitchaidecha, W.; Nakaruk, A. Photodegradation mechanisms of phenol in the photocatalytic process. Res. Chem. Intermed. 2016, 42, 5961–5974. [Google Scholar] [CrossRef]
- Ortiz-Gomez, A.; Serrano-Rosales, B.; Salaices, M.; de Lasa, H. Photocatalytic oxidation of phenol: reaction network, kinetic modeling, and parameter estimation. Ind. Eng. Chem. Res. 2007, 46, 7394–7409. [Google Scholar] [CrossRef]
- Albiss, B.; Abu-Dalo, M. Photocatalytic degradation of methylene blue using zinc oxide nanorods grown on activated carbon fibers. Sustainability 2021, 13, 4729. [Google Scholar] [CrossRef]
- Reyes-Coronado, D.; Rodriguez-Gattorno, G.; Espinosa-Pesqueira, M.E.; Cab, C.; de Coss, R.; Oskam, G. Phase-pure TiO2 nanoparticles: Anatase, brookite and rutile. Nanotechnology 2008, 19, 145605. [Google Scholar] [CrossRef]
- Serpone, N.; Lawless, D.; Khairutdinov, R. Size effects on the photophysical properties of colloidal anatase TiO2 particles: Size quantization or direct transitions in this indirect semiconductor? J. Phys. Chem. B 1995, 99, 16646–16654. [Google Scholar] [CrossRef]
- Wachs, I.E.; Phivilay, S.P.; Roberts, C.A. Reporting of reactivity for heterogeneous photocatalysis. ACS Catal. 2013, 3, 2606–2611. [Google Scholar] [CrossRef]
- Tsai, M.-C.; Lee, J.-Y.; Chen, P.-C.; Chang, Y.-W.; Chang, Y.-C.; Yang, M.-H.; Chiu, H.-T.; Lin, I.N.; Lee, R.-K.; Lee, C.-Y. Effects of size and shell thickness of TiO2 hierarchical hollow spheres on photocatalytic behavior: An experimental and theoretical study. Appl. Catal. B-Environ. 2014, 147, 499–507. [Google Scholar] [CrossRef]
- Glasstone, S. Textbook of Physical Chemistry, 2nd ed.; D. Van Nostrand Company, Inc.: London, UK, 1962. [Google Scholar]
- Delgado, A.V.; Gonzalez-Caballero, F.; Hunter, R.J.; Koopal, L.K.; Lyklema, J.; International Union of, P.; Applied Chemistry, P.; Biophysical Chemistry Division, I.T.R. Measurement and interpretation of electrokinetic phenomena. J. Colloid Interface Sci. 2007, 309, 194–224. [Google Scholar] [CrossRef]
- Jiang, J.; Oberdörster, G.; Biswas, P. Characterization of size, surface charge, and agglomeration state of nanoparticle dispersions for toxicological studies. J. Nanopart. Res. 2008, 11, 77–89. [Google Scholar] [CrossRef]
- Kosmulski, M. Surface Charging and Points of Zero Charge, 1st ed.; CRC Press: Boca Raton, FL, USA, 2009; p. 1092. [Google Scholar]
- Bohren, C.F.; Huffman, D.R. Absorption and Scattering by a Sphere. In Absorption and Scattering of Light by Small Particles; Chapter 4; Wiley-VCH: Weinheim, Germany, 1998; pp. 82–129. [Google Scholar]
- Haynes, W.M. CRC Handbook of Chemistry and Physics, 96th ed.; CRC Press: Boca Raton, FL, USA, 2015. [Google Scholar]
- Shi, H.; Zhang, T.; An, T.; Li, B.; Wang, X. Enhancement of photocatalytic activity of nano-scale TiO2 particles co-doped by rare earth elements and heteropolyacids. J. Colloid Interface Sci. 2012, 380, 121–127. [Google Scholar] [CrossRef] [PubMed]

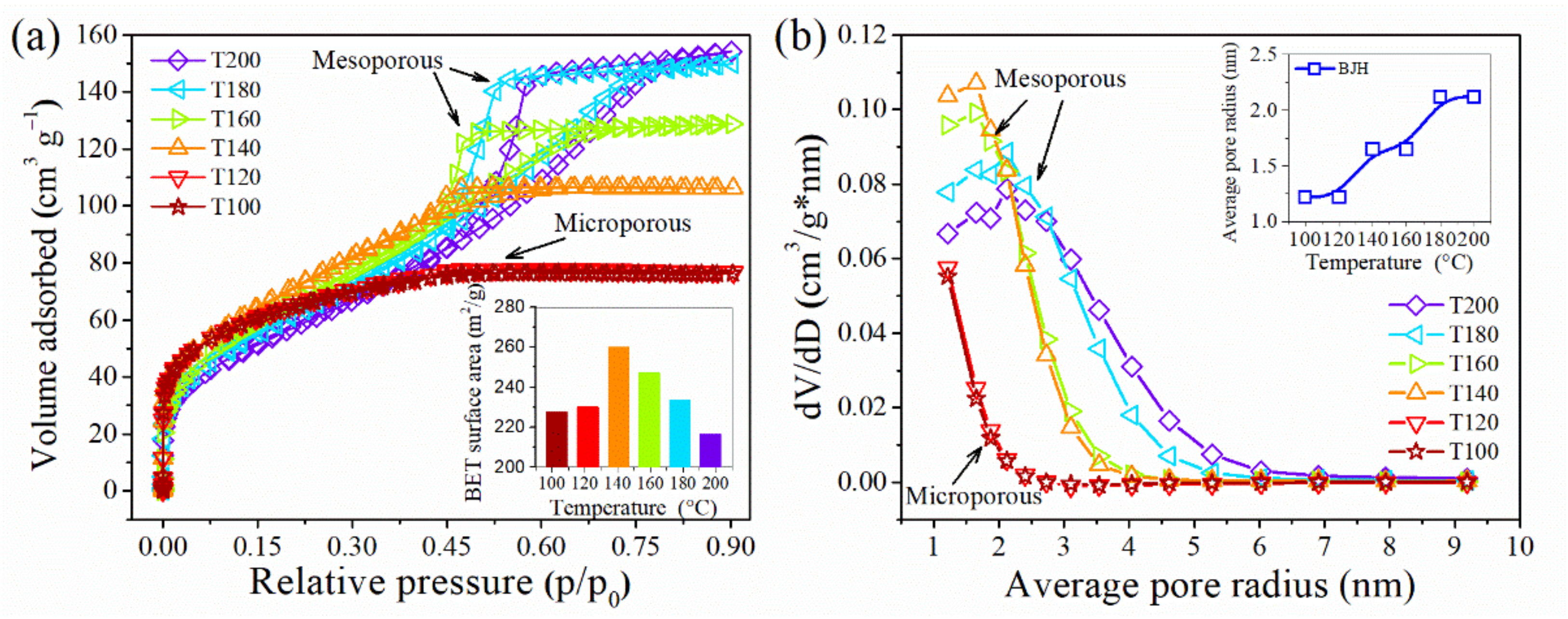


| Photocatalyst | SBET (m2 g−1) | Pore Volume (cm3 g−1) | Mean Pore Diameter (nm) |
|---|---|---|---|
| T100 | 229 | 0.118 | 2.07 |
| T120 | 230 | 0.119 | 2.06 |
| T140 | 260 | 0.165 | 2.53 |
| T160 | 247 | 0.199 | 3.22 |
| T180 | 233 | 0.232 | 3.97 |
| T200 | 216 | 0.239 | 4.41 |
| Sample | Rate Constant (10−2 min−1) | TORs(10−4 M−1 min−1 m−2 g1) | Conversion (%, 75 min) | R2 |
|---|---|---|---|---|
| T100 | 1.88 | 0.82 | 75 | 0.99 |
| T120 | 2.55 | 1.20 | 86 | 0.99 |
| T140 | 5.03 | 1.93 | 98 | 0.98 |
| T160 | 0.41 | 0.17 | 26 | 1.00 |
| T180 | 0.08 | 0.03 | 6 | 0.96 |
| T200 | 0.11 | 0.05 | 8 | 0.97 |
| Global Test | ||||
|---|---|---|---|---|
| R-multiple | Square-R | Std. Error | F | p-Value |
| 0.90 | 0.95 | 0.00002 | 39.89 | 0.003 |
| Coefficient test | ||||
| Std. error | Coefficients | p-value | t-test | |
| Intercept | 0.000082 | −0.00044 | 0.005 | −5.40 |
| Slope | 0.000010 | 0.00006 | 0.003 | 6.31 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ceballos-Chuc, M.C.; Ramos-Castillo, C.M.; Rodríguez-Pérez, M.; Ruiz-Gómez, M.Á.; Rodríguez-Gattorno, G.; Villanueva-Cab, J. Synergistic Correlation in the Colloidal Properties of TiO2 Nanoparticles and Its Impact on the Photocatalytic Activity. Inorganics 2022, 10, 125. https://doi.org/10.3390/inorganics10090125
Ceballos-Chuc MC, Ramos-Castillo CM, Rodríguez-Pérez M, Ruiz-Gómez MÁ, Rodríguez-Gattorno G, Villanueva-Cab J. Synergistic Correlation in the Colloidal Properties of TiO2 Nanoparticles and Its Impact on the Photocatalytic Activity. Inorganics. 2022; 10(9):125. https://doi.org/10.3390/inorganics10090125
Chicago/Turabian StyleCeballos-Chuc, María Concepción, Carlos Manuel Ramos-Castillo, Manuel Rodríguez-Pérez, Miguel Ángel Ruiz-Gómez, Geonel Rodríguez-Gattorno, and Julio Villanueva-Cab. 2022. "Synergistic Correlation in the Colloidal Properties of TiO2 Nanoparticles and Its Impact on the Photocatalytic Activity" Inorganics 10, no. 9: 125. https://doi.org/10.3390/inorganics10090125
APA StyleCeballos-Chuc, M. C., Ramos-Castillo, C. M., Rodríguez-Pérez, M., Ruiz-Gómez, M. Á., Rodríguez-Gattorno, G., & Villanueva-Cab, J. (2022). Synergistic Correlation in the Colloidal Properties of TiO2 Nanoparticles and Its Impact on the Photocatalytic Activity. Inorganics, 10(9), 125. https://doi.org/10.3390/inorganics10090125