Nanoscale Optical Trapping by Means of Dielectric Bowtie
Abstract
:1. Introduction
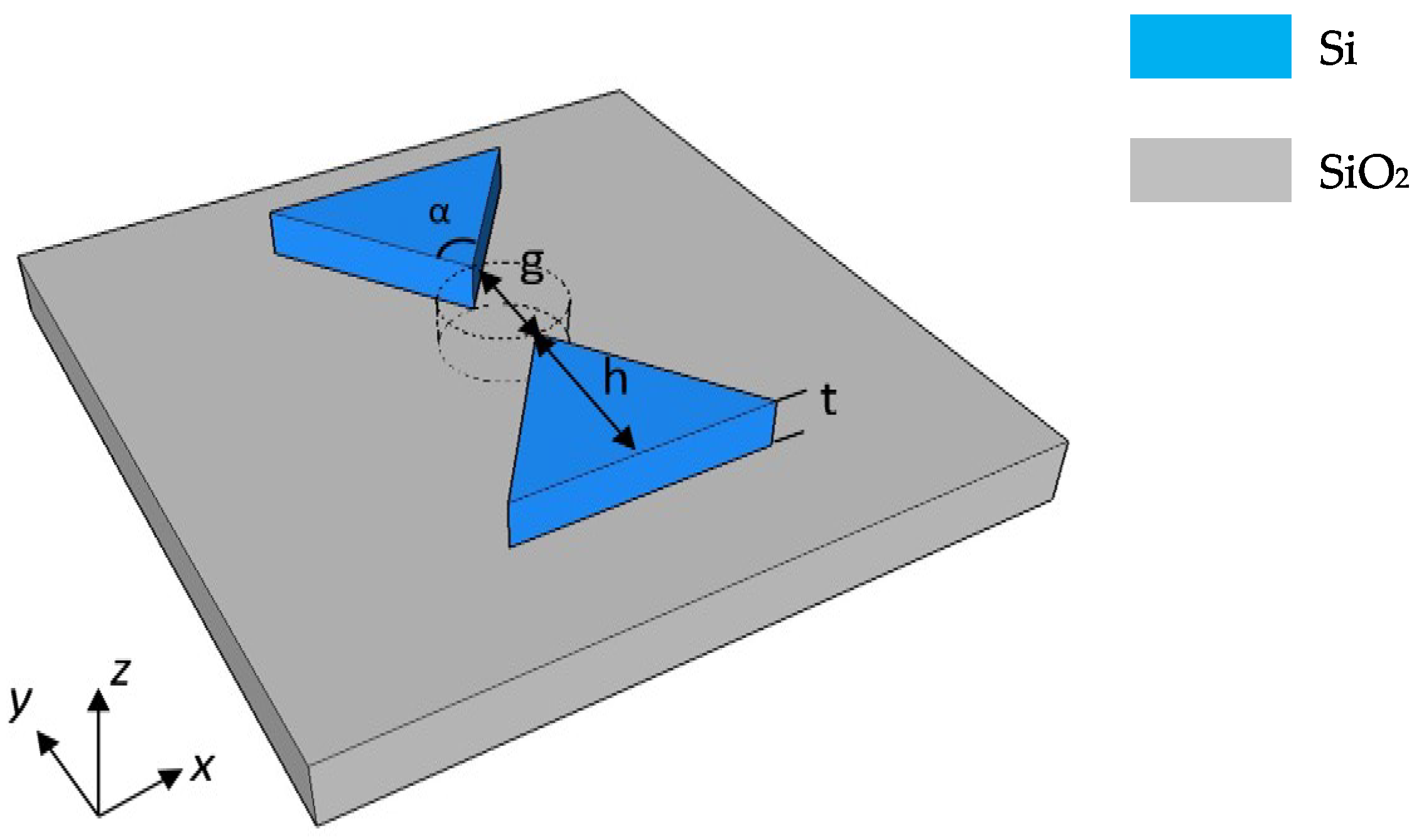
2. Dielectric Bowtie
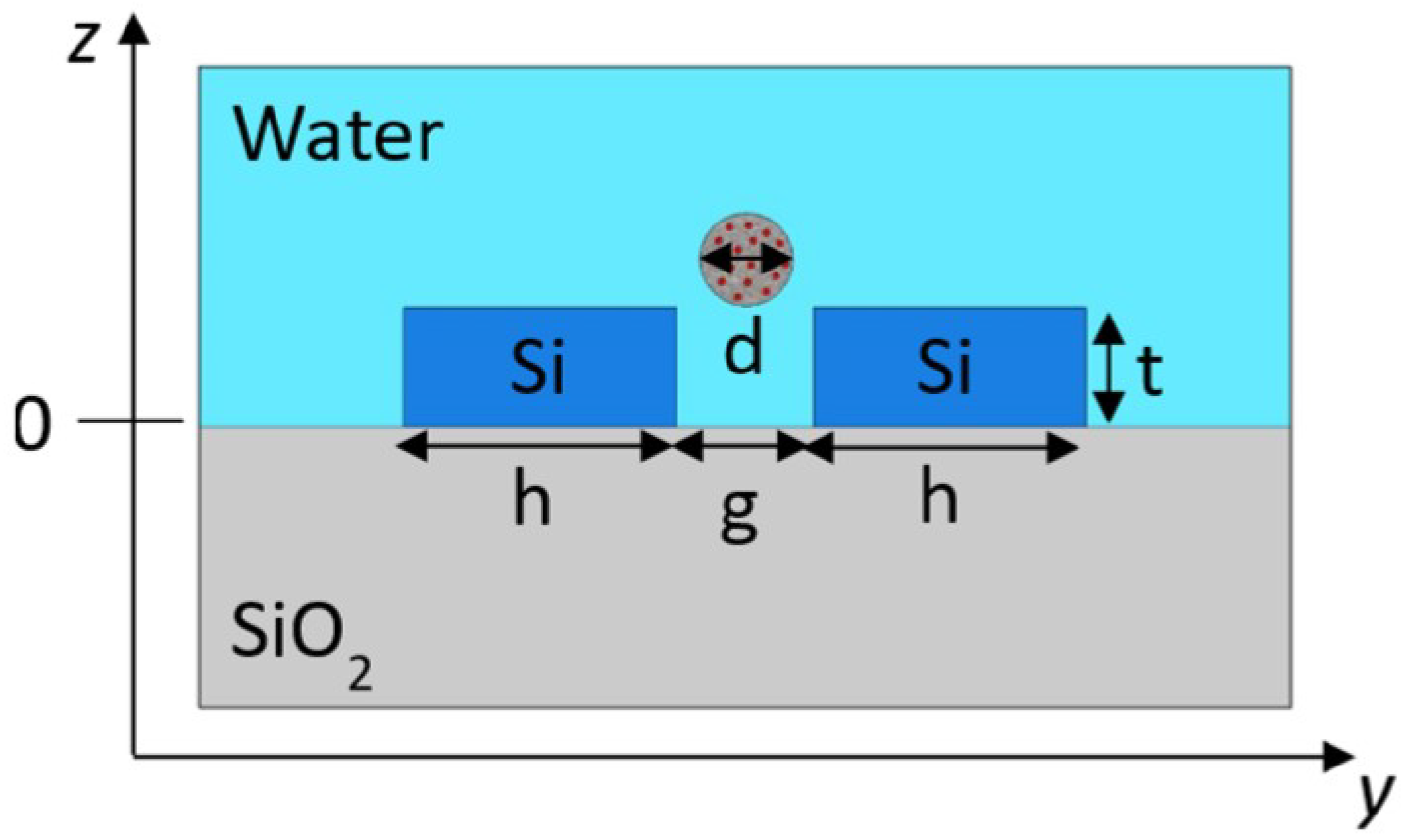
3. Design to Enhance the Energy within the Trapping Site
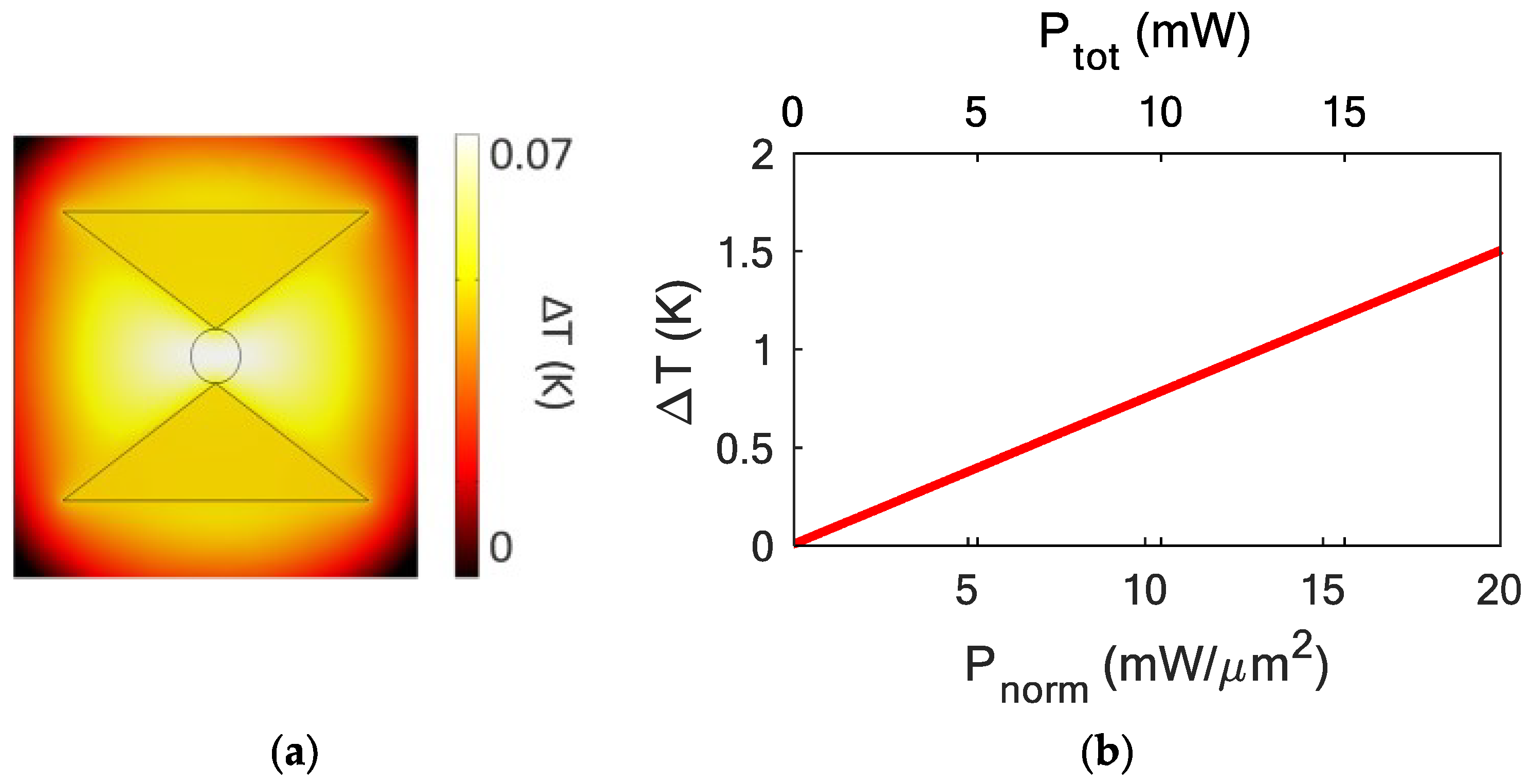
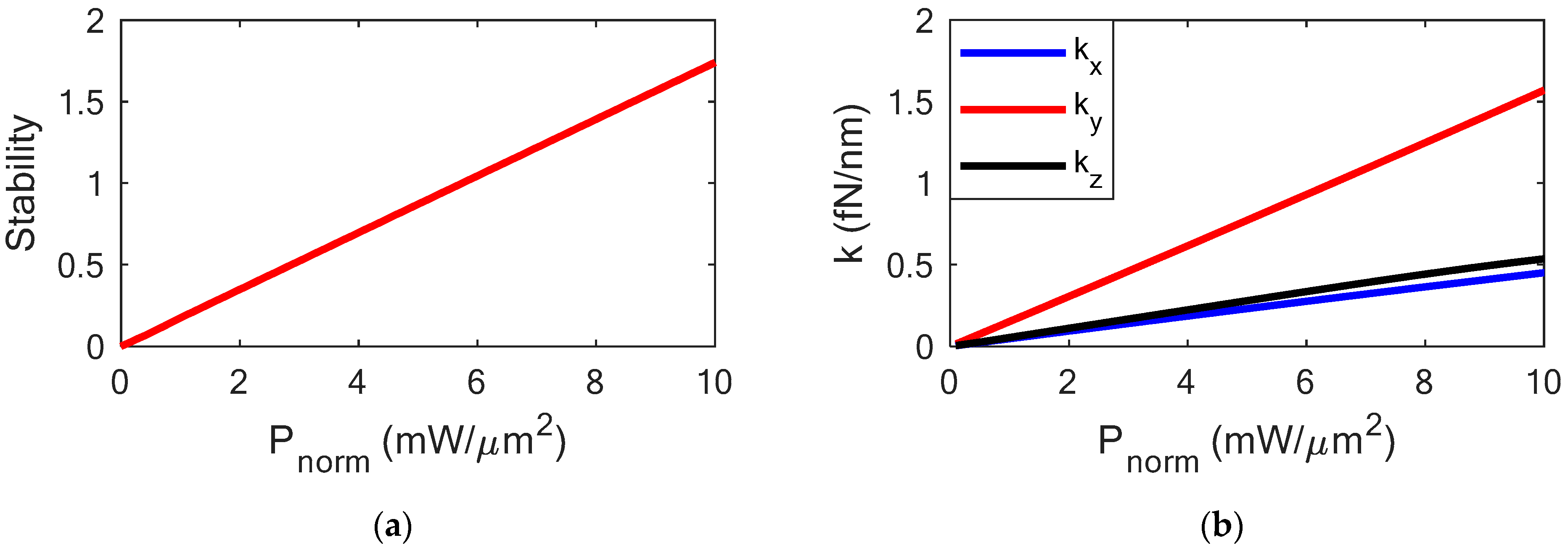
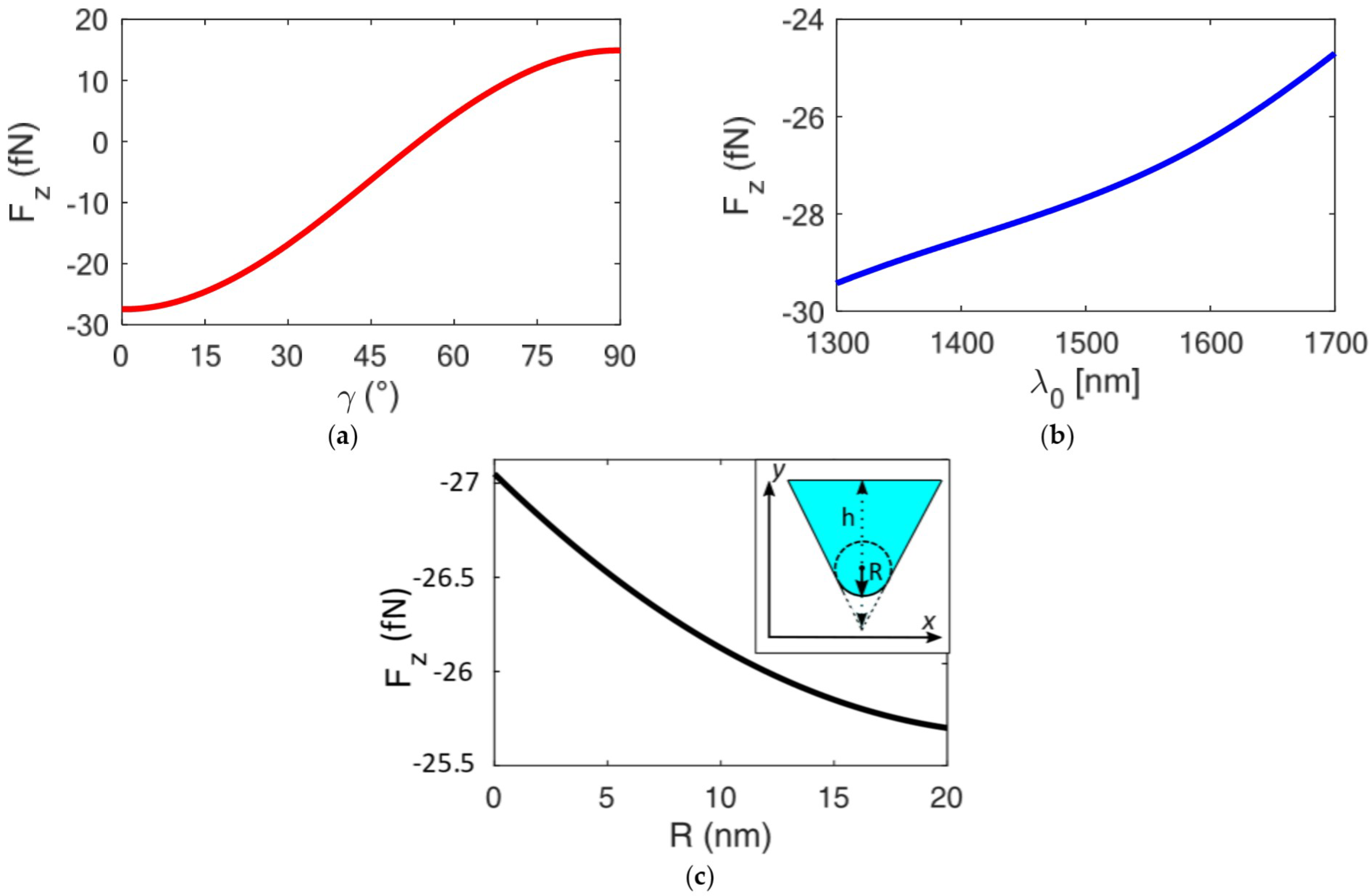
4. Numerical Results on the Optical Trapping and Discussions
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kirchain, R.; Kimerling, L. A roadmap for nanophotonics. Nat. Phot. 2007, 1, 303–305. [Google Scholar] [CrossRef]
- Grier, D.G. A revolution in optical manipulation. Nature 2003, 424, 810–816. [Google Scholar] [CrossRef] [PubMed]
- Choi, M.S.; Lee, G.H.; Yu, Y.J.; Lee, D.Y.; Lee, S.H.; Kim, P.; Hone, J.; Yoo, W.J. Controlled charge trapping by molybdenum disulphide and graphene in ultrathin heterostructured memory devices. Nat. Comm. 2013, 4, 1624. [Google Scholar] [CrossRef] [PubMed]
- Hohenester, U. Nano and Quantum Optics: An Introduction to Basic Principles and Theory; Springer: Cham, Switzerland, 2020. [Google Scholar]
- Ashkin, A.; Dziedzic, J.M. Optical trapping and manipulation of viruses and bacteria. Science 1987, 235, 1517–1520. [Google Scholar] [CrossRef] [PubMed]
- Ashkin, A. Acceleration and trapping of particles by radiation pressure. Phys. Rev. Lett. 1970, 24, 56. [Google Scholar] [CrossRef] [Green Version]
- Ashkin, A.; Dziedzic, J.M.; Bjorkholm, J.E.; Chu, S. Observation of a single-beam gradient force optical trap for dielectric particles. Opt. Lett. 1986, 11, 288–290. [Google Scholar] [CrossRef] [Green Version]
- Conteduca, D.; Dell’Olio, F.; Krauss, T.F.; Ciminelli, C. Photonic and plasmonic nanotweezing of nano-and microscale particles. Appl. Spectr. 2017, 71, 367–390. [Google Scholar] [CrossRef] [Green Version]
- Neuman, K.C.; Block, S.M. Optical trapping. Rev. Sci. Instrum. 2004, 75, 2787–2809. [Google Scholar] [CrossRef]
- Maragò, O.M.; Jones, P.H.; Gucciardi, P.G.; Volpe, G.; Ferrari, A.C. Optical trapping and manipulation of nanostructures. Nat. Nanotech. 2013, 8, 807. [Google Scholar] [CrossRef] [Green Version]
- Mandal, S.; Serey, X.; Erickson, D. Nanomanipulation using silicon photonic crystal resonators. Nano Lett. 2010, 10, 99–104. [Google Scholar] [CrossRef]
- Conteduca, D.; Brunetti, G.; Dell’Olio, F.; Armenise, M.N.; Krauss, T.F.; Ciminelli, C. Monitoring of individual bacteria using electro-photonic traps. Biomed. Opt. Express 2019, 10, 3463–3471. [Google Scholar] [CrossRef] [PubMed]
- Lin, S.; Schonbrun, E.; Crozier, K. Optical manipulation with planar silicon microring resonators. Nano Lett. 2010, 10, 2408–2411. [Google Scholar] [CrossRef] [PubMed]
- Cai, H.; Poon, A.W. Optical manipulation of microparticles using whispering-gallery modes in a silicon nitride microdisk resonator. Opt. Lett. 2011, 36, 4257–4259. [Google Scholar] [CrossRef] [PubMed]
- Koya, A.N.; Cunha, J.; Guo, T.L.; Toma, A.; Garoli, D.; Wang, T.; Juodkazis, S.; Cojoc, D.; Proietti Zaccaria, R. Novel Plasmonic Nanocavities for Optical Trapping—Assisted Biosensing Applications. Adv. Opt. Mat. 2020, 8, 1901481. [Google Scholar] [CrossRef]
- Conteduca, D.; Reardon, C.; Scullion, M.G.; Dell’Olio, F.; Armenise, M.N.; Krauss, T.F.; Ciminelli, C. Ultra-high Q/V hybrid cavity for strong light-matter interaction. APL Phot. 2017, 2, 086101. [Google Scholar] [CrossRef] [Green Version]
- Dantham, V.R.; Holler, S.; Barbre, C.; Keng, D.; Kolchenko, V.; Arnold, S. Label-free detection of single protein using a nanoplasmonic-photonic hybrid microcavity. Nano Lett. 2013, 13, 3347–3351. [Google Scholar] [CrossRef]
- Chen, Y.F.; Serey, X.; Sarkar, R.; Chen, P.; Erickson, D. Controlled photonic manipulation of proteins and other nanomaterials. Nano Lett. 2012, 12, 1633–1637. [Google Scholar] [CrossRef] [Green Version]
- Lin, S.; Crozier, K.B. Trapping-assisted sensing of particles and proteins using on-chip optical microcavities. ACS Nano 2013, 7, 1725–1730. [Google Scholar] [CrossRef]
- Conteduca, D.; Brunetti, G.; Pitruzzello, G.; Tragni, F.; Dholakia, K.; Krauss, T.F.; Ciminelli, C. Exploring the limit of multiplexed near-field optical trapping. ACS Phot. 2021, 8, 2060–2066. [Google Scholar] [CrossRef]
- Kotnala, A.; DePaoli, D.; Gordon, R. Sensing nanoparticles using a double nanohole optical trap. Lab A Chip 2013, 13, 4142–4146. [Google Scholar] [CrossRef]
- Yoo, D.; Gurunatha, K.L.; Choi, H.K.; Mohr, D.A.; Ertsgaard, C.T.; Gordon, R.; Oh, S.H. Low-power optical trapping of nanoparticles and proteins with resonant coaxial nanoaperture using 10 nm gap. Nano Lett. 2018, 18, 3637–3642. [Google Scholar] [CrossRef] [PubMed]
- Kim, J.D.; Lee, Y.G. Trapping of a single DNA molecule using nanoplasmonic structures for biosensor applications. Biomed. Opt. Exp. 2014, 5, 2471–2480. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhang, P.; Song, G.; Yu, L. Optical trapping of single quantum dots for cavity quantum electrodynamics. Phot. Res. 2018, 6, 182–185. [Google Scholar] [CrossRef]
- Lu, Y.; Du, G.; Chen, F.; Yang, Q.; Bian, H.; Yong, J.; Hou, X. Tunable potential well for plasmonic trapping of metallic particles by bowtie nano-apertures. Sci. Rep. 2016, 6, 20594. [Google Scholar] [CrossRef] [PubMed]
- Bendix, P.M.; Reihani, S.N.S.; Oddershede, L.B. Direct measurements of heating by electromagnetically trapped gold nanoparticles on supported lipid bilayers. ACS Nano 2010, 4, 2256–2262. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, K.; Schonbrun, E.; Steinvurzel, P.; Crozier, K.B. Trapping and rotating nanoparticles using a plasmonic nano-tweezer with an integrated heat sink. Nat. Comm. 2011, 2, 469. [Google Scholar] [CrossRef] [Green Version]
- Xu, Z.; Song, W.; Crozier, K.B. Direct particle tracking observation and Brownian dynamics simulations of a single nanoparticle optically trapped by a plasmonic nanoaperture. ACS Phot. 2018, 5, 2850–2859. [Google Scholar] [CrossRef]
- Xu, Z.; Song, W.; Crozier, K.B. Optical trapping of nanoparticles using all-silicon nanoantennas. ACS Phot. 2018, 5, 4993–5001. [Google Scholar] [CrossRef]
- Yue, W.C.; Yao, P.J.; Xu, L.X.; Ming, H. All-dielectric bowtie waveguide with deep subwavelength mode confinement. Front. Phys. 2018, 13, 134207. [Google Scholar] [CrossRef] [Green Version]
- Lu, Q.; Shu, F.J.; Zou, C.L. Dielectric bow-tie nanocavity. Opt. Lett. 2013, 38, 5311–5314. [Google Scholar] [CrossRef] [Green Version]
- Zotev, P.G.; Wang, Y.; Sortino, L.; Severs Millard, T.; Mullin, N.; Conteduca, D.; Shagar, M.; Genco, A.; Hobbs, J.K.; Krauss, T.F.; et al. Transition Metal Dichalcogenide Dimer Nanoantennas for Tailored Light–Matter Interactions. ACS Nano 2022, 16, 6493–6505. [Google Scholar] [CrossRef] [PubMed]
- Pesce, G.; Jones, P.H.; Maragò, O.M.; Volpe, G. Optical tweezers: Theory and practice. Eur. Phys. J. Plus 2020, 135, 949. [Google Scholar] [CrossRef]
- Gan, Q.; Ding, Y.J.; Bartoli, F.J. “Rainbow” trapping and releasing at telecommunication wavelengths. Phys. Rev. Lett. 2009, 102, 056801. [Google Scholar] [CrossRef] [PubMed]
- Hale, G.M.; Querry, M.R. Optical constants of water in the 200-nm to 200-μm wavelength region. Appl. Opt. 1973, 12, 555–563. [Google Scholar] [CrossRef]
- Malitson, I.H. Interspecimen comparison of the refractive index of fused silica. J. Opt. Soc. Am. 1965, 55, 1205–1209. [Google Scholar] [CrossRef]
- Salzberg, C.D.; Villa, J.J. Infrared refractive indexes of silicon germanium and modified selenium glass. JOSA 1957, 47, 244–246. [Google Scholar] [CrossRef]
- Dobrzynski, L.; Maradudin, A.A. Electrostatic edge modes in a dielectric wedge. Phys. Rev. B 1972, 6, 3810. [Google Scholar] [CrossRef]
- Almeida, V.R.; Xu, Q.; Barrios, C.A.; Lipson, M. Guiding and confining light in void nanostructure. Opt. Lett. 2004, 29, 1209–1211. [Google Scholar] [CrossRef]
- Fehr, A.R.; Perlman, S. Coronaviruses: An overview of their replication and pathogenesis. Coronaviruses 2015, 1282, 1–23. [Google Scholar]
- Kingsbury, D.W. Paramyxoviridae. Intervirology 1978, 10, 137–152. [Google Scholar] [CrossRef]
- Wang, S.; Shan, X.; Patel, U.; Huang, X.; Lu, J.; Li, J.; Tao, N. Label-free imaging, detection, and mass measurement of single viruses by surface plasmon resonance. Proc. Natl. Acad. Sci. USA 2010, 107, 16028–16032. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Vollmer, F.; Arnold, S.; Keng, D. Single virus detection from the reactive shift of a whispering-gallery mode. Proc. Natl. Acad. Sci. USA 2008, 105, 20701–20704. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Block, O.; Mitra, A.; Novotny, L.; Dykes, C. A rapid label-free method for quantitation of human immunodeficiency virus type-1 particles by nanospectroscopy. J. Virol. Methods 2012, 182, 70–75. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ciminelli, C.; Conteduca, D.; Dell’Olio, F.; Armenise, M.N. Design of an optical trapping device based on an ultra-high Q/V resonant structure. IEEE Phot. J. 2014, 6, 1–16. [Google Scholar] [CrossRef]
- Serey, X.; Mandal, S.; Erickson, D. Comparison of silicon photonic crystal resonator designs for optical trapping of nanomaterials. Nanotechnology 2010, 21, 305202. [Google Scholar] [CrossRef]
- Svoboda, K.; Block, S.M. Optical Trapping of Metallic Rayleigh Particles. Opt. Lett. 1994, 19, 930–932. [Google Scholar] [CrossRef]
- Pelton, M.; Liu, M.; Kim, H.Y.; Smith, G.; Guyot-Sionnest, P.; Scherer, N.F. Optical trapping and alignment of single gold nanorods by using plasmon resonance. Opt. Lett. 2006, 31, 2075–2077. [Google Scholar] [CrossRef]
- Yang, A.H.; Moore, S.D.; Schmidt, B.S.; Klug, M.; Lipson, M.; Erickson, D. Optical manipulation of nanoparticles and biomolecules in sub-wavelength slot waveguides. Nature 2009, 457, 71–75. [Google Scholar] [CrossRef]
- Grigorenko, A.N.; Roberts, N.W.; Dickinson, M.R.; Zhang, Y. Nanometric optical tweezers based on nanostructured substrates. Nat. Phot. 2008, 2, 365–370. [Google Scholar] [CrossRef] [Green Version]
- Bian, Y.; Zheng, Z.; Liu, Y.; Liu, J.; Zhu, J.; Zhou, T. Hybrid wedge plasmon polariton waveguide with good fabrication-error-tolerance for ultra-deep-subwavelength mode confinement. Opt. Exp. 2011, 19, 22417–22422. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brunetti, G.; Sasanelli, N.; Armenise, M.N.; Ciminelli, C. Nanoscale Optical Trapping by Means of Dielectric Bowtie. Photonics 2022, 9, 425. https://doi.org/10.3390/photonics9060425
Brunetti G, Sasanelli N, Armenise MN, Ciminelli C. Nanoscale Optical Trapping by Means of Dielectric Bowtie. Photonics. 2022; 9(6):425. https://doi.org/10.3390/photonics9060425
Chicago/Turabian StyleBrunetti, Giuseppe, Nicola Sasanelli, Mario Nicola Armenise, and Caterina Ciminelli. 2022. "Nanoscale Optical Trapping by Means of Dielectric Bowtie" Photonics 9, no. 6: 425. https://doi.org/10.3390/photonics9060425
APA StyleBrunetti, G., Sasanelli, N., Armenise, M. N., & Ciminelli, C. (2022). Nanoscale Optical Trapping by Means of Dielectric Bowtie. Photonics, 9(6), 425. https://doi.org/10.3390/photonics9060425






