Controlling Dispersion Characteristic of Focused Vortex Beam Generation
Abstract
:1. Introduction
2. Method
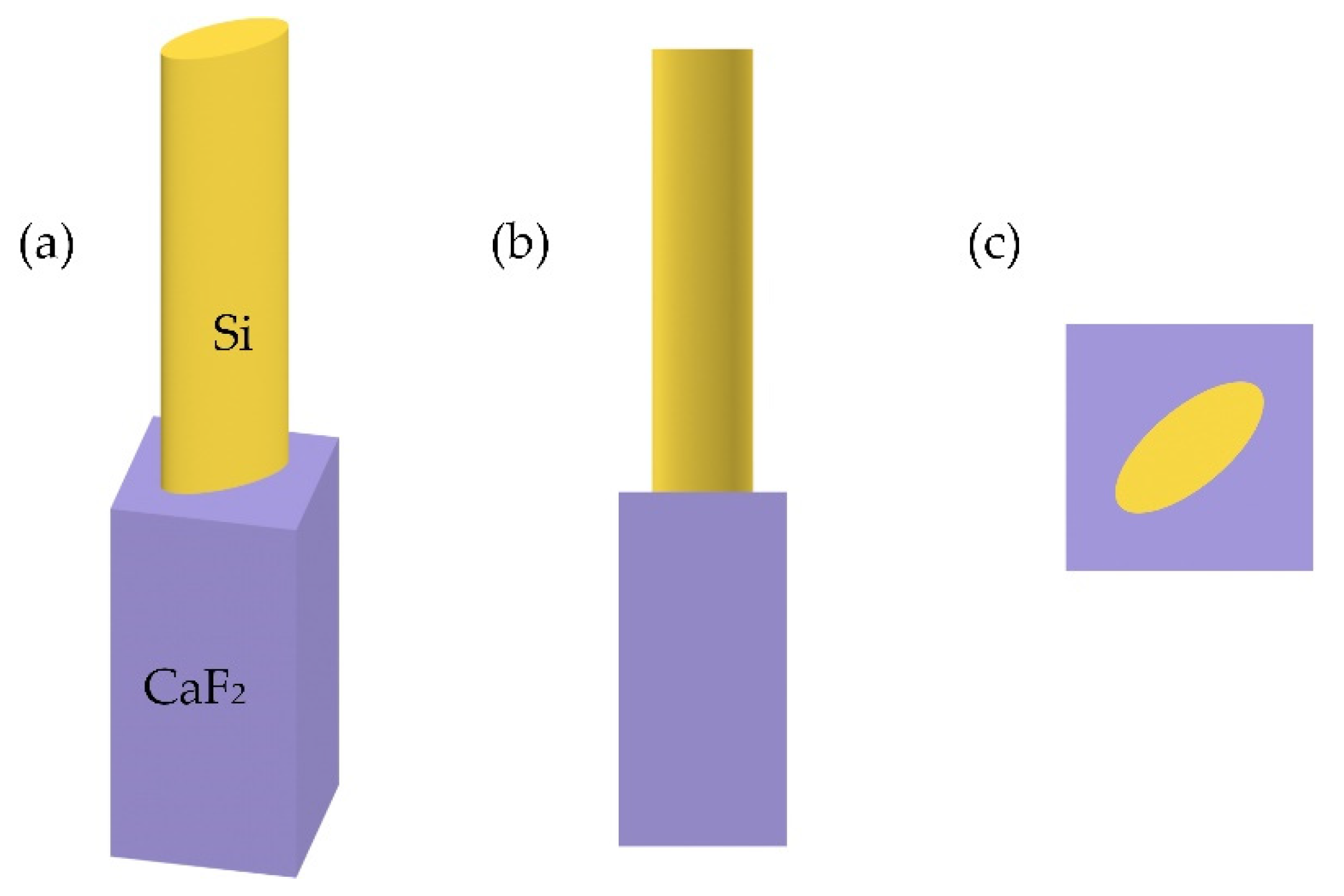
3. Meta-Atom
4. Result and Discussion
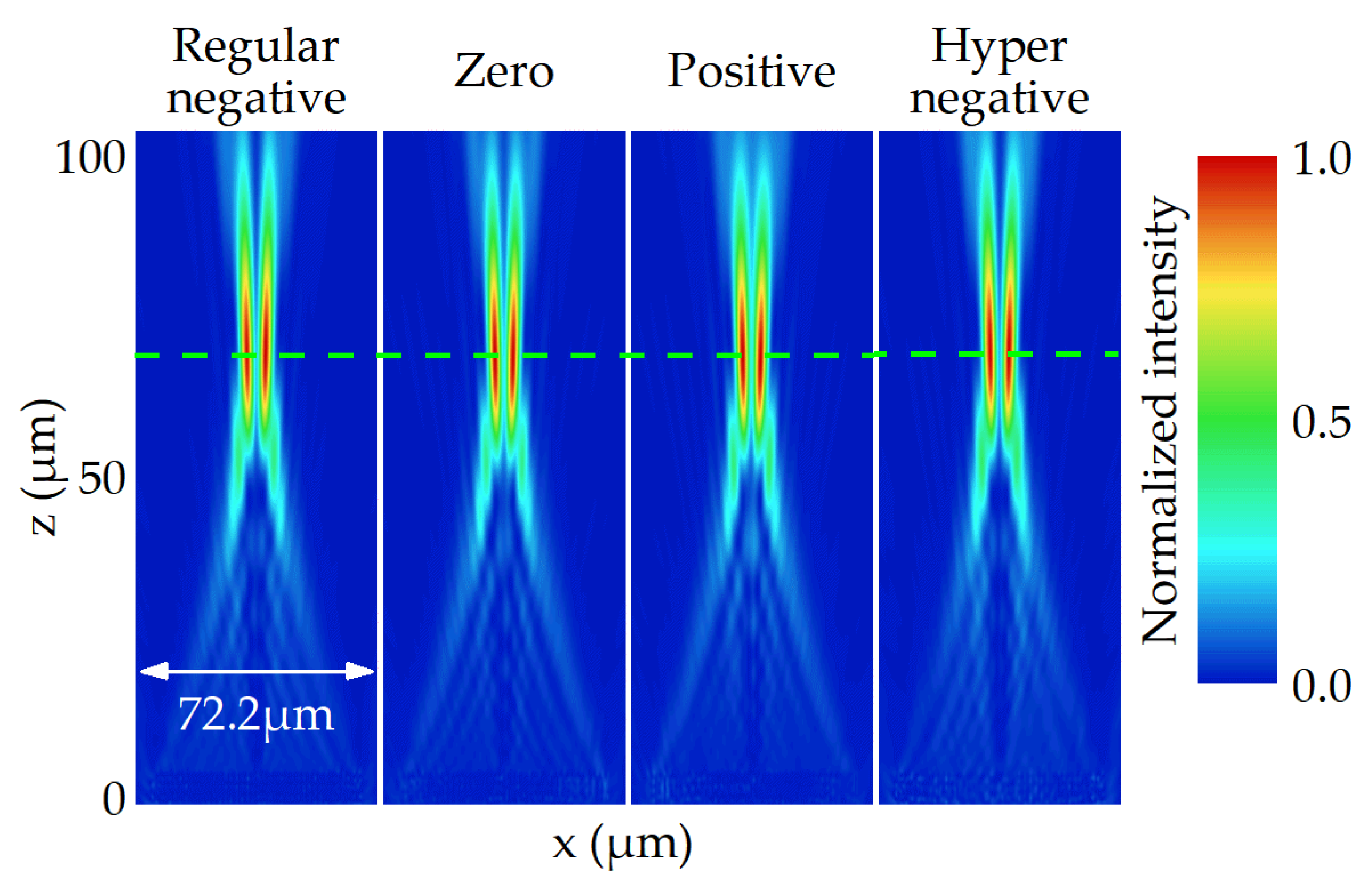
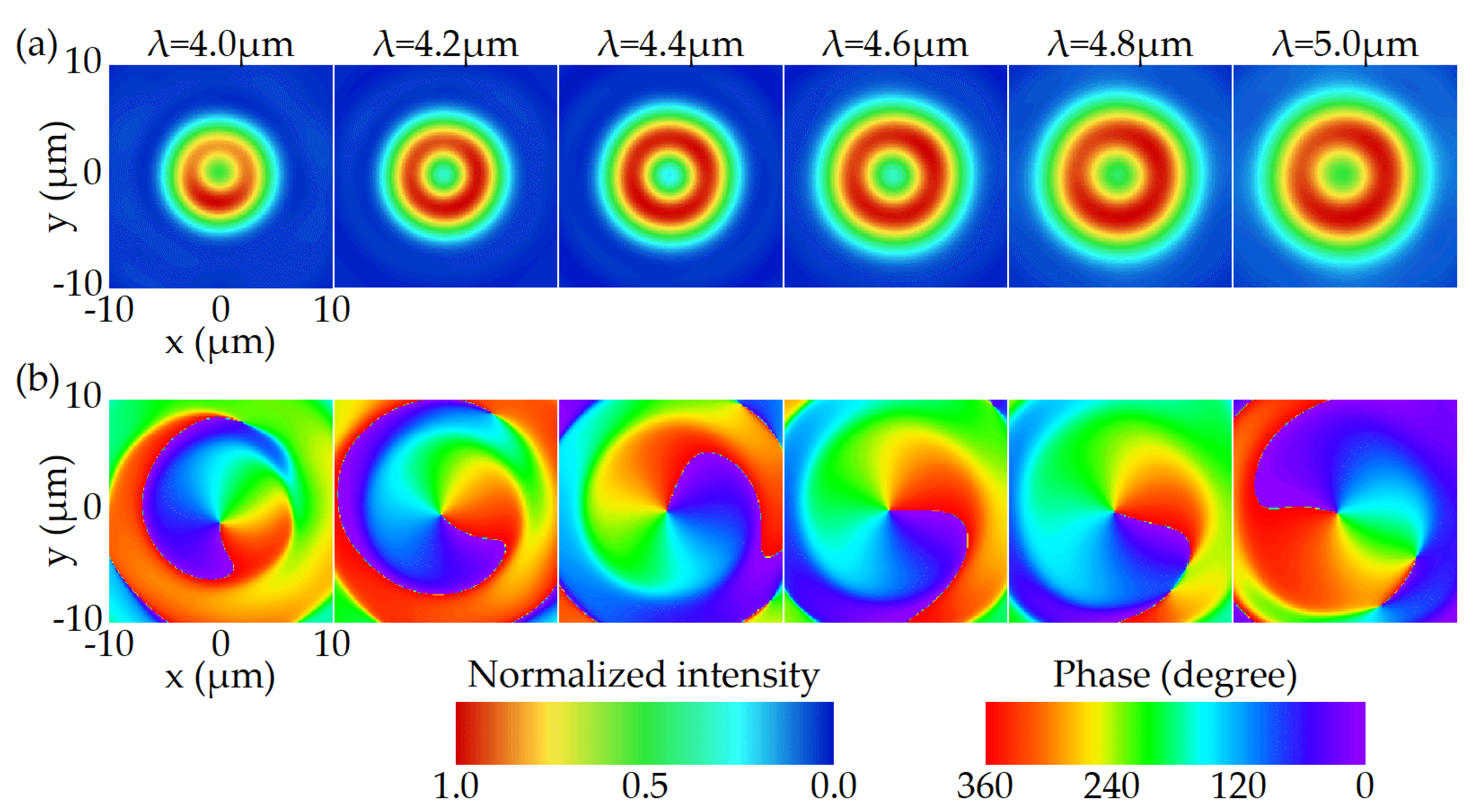
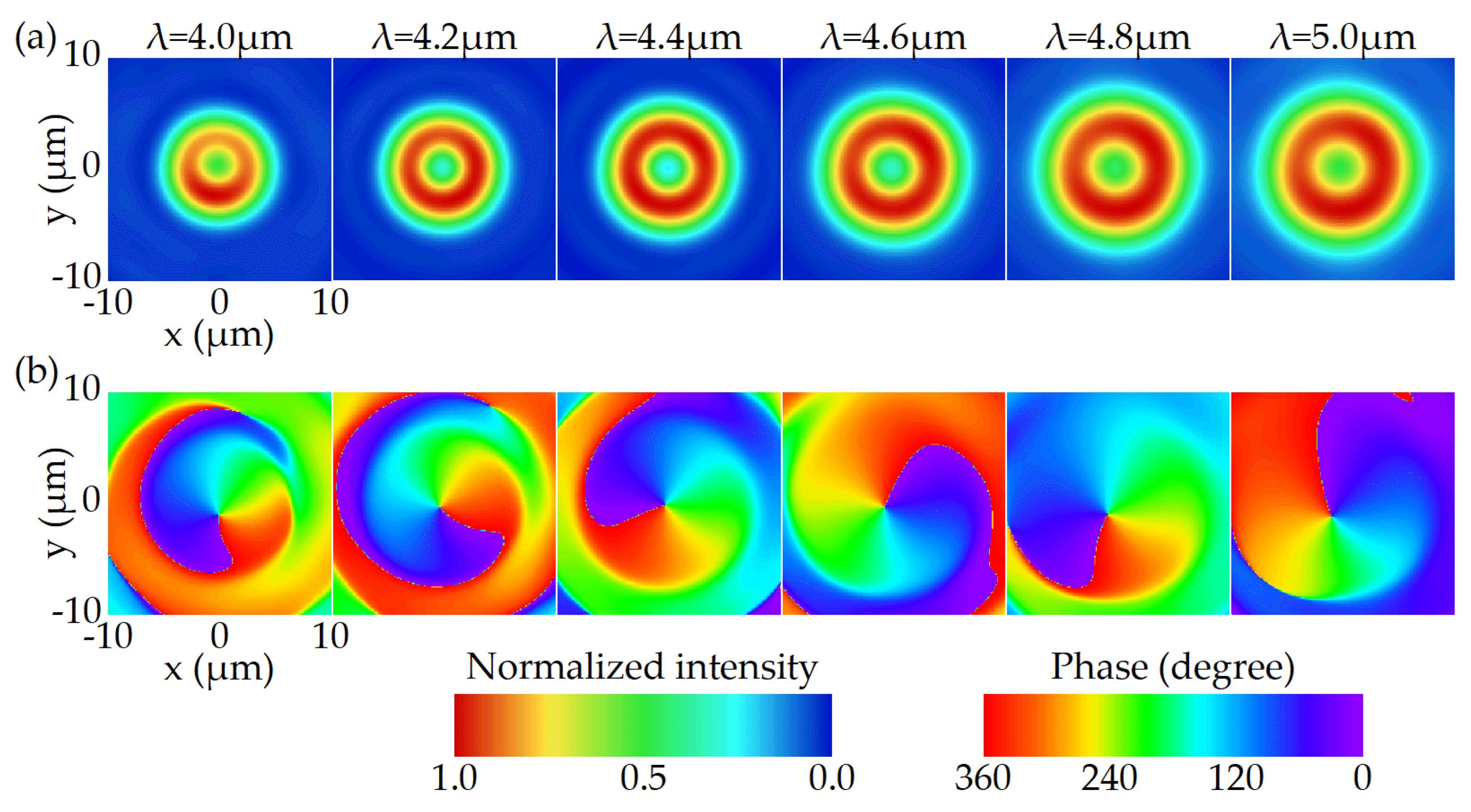
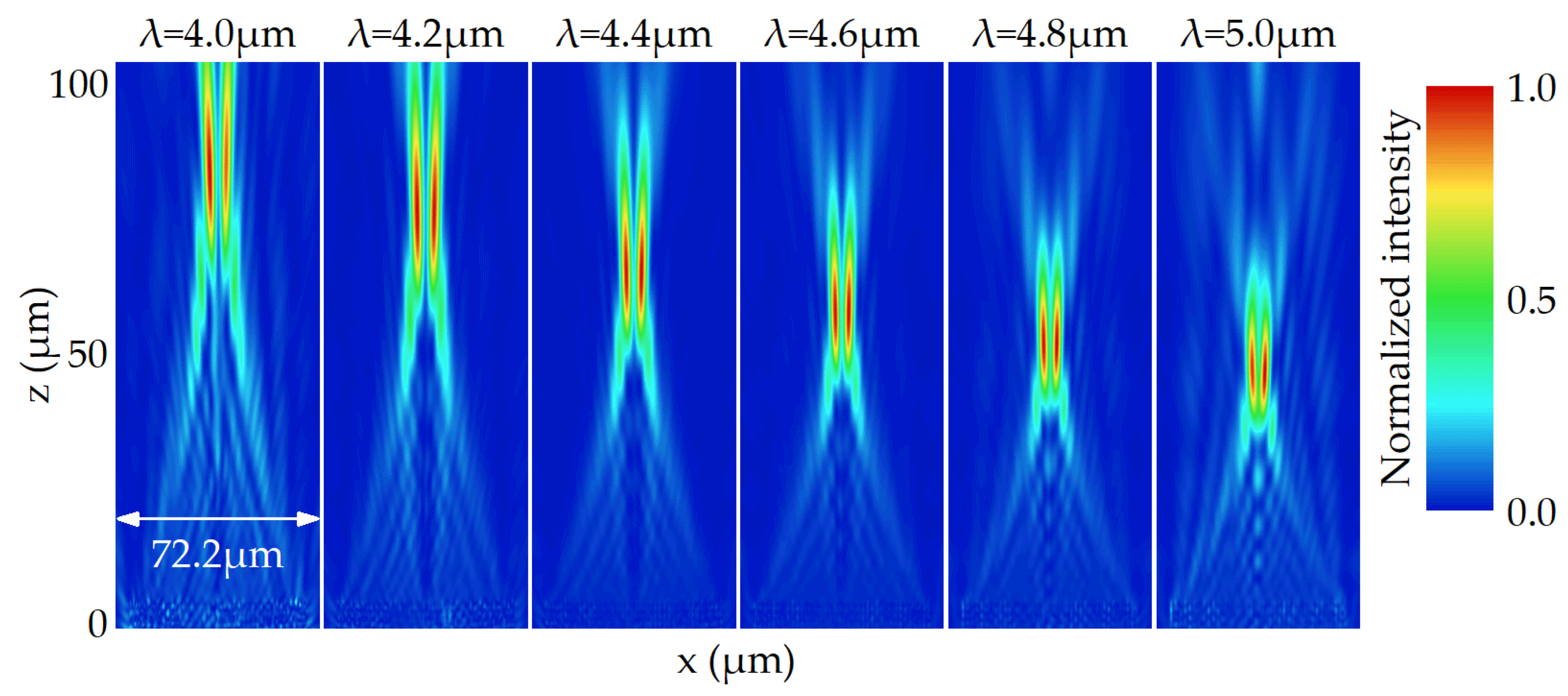
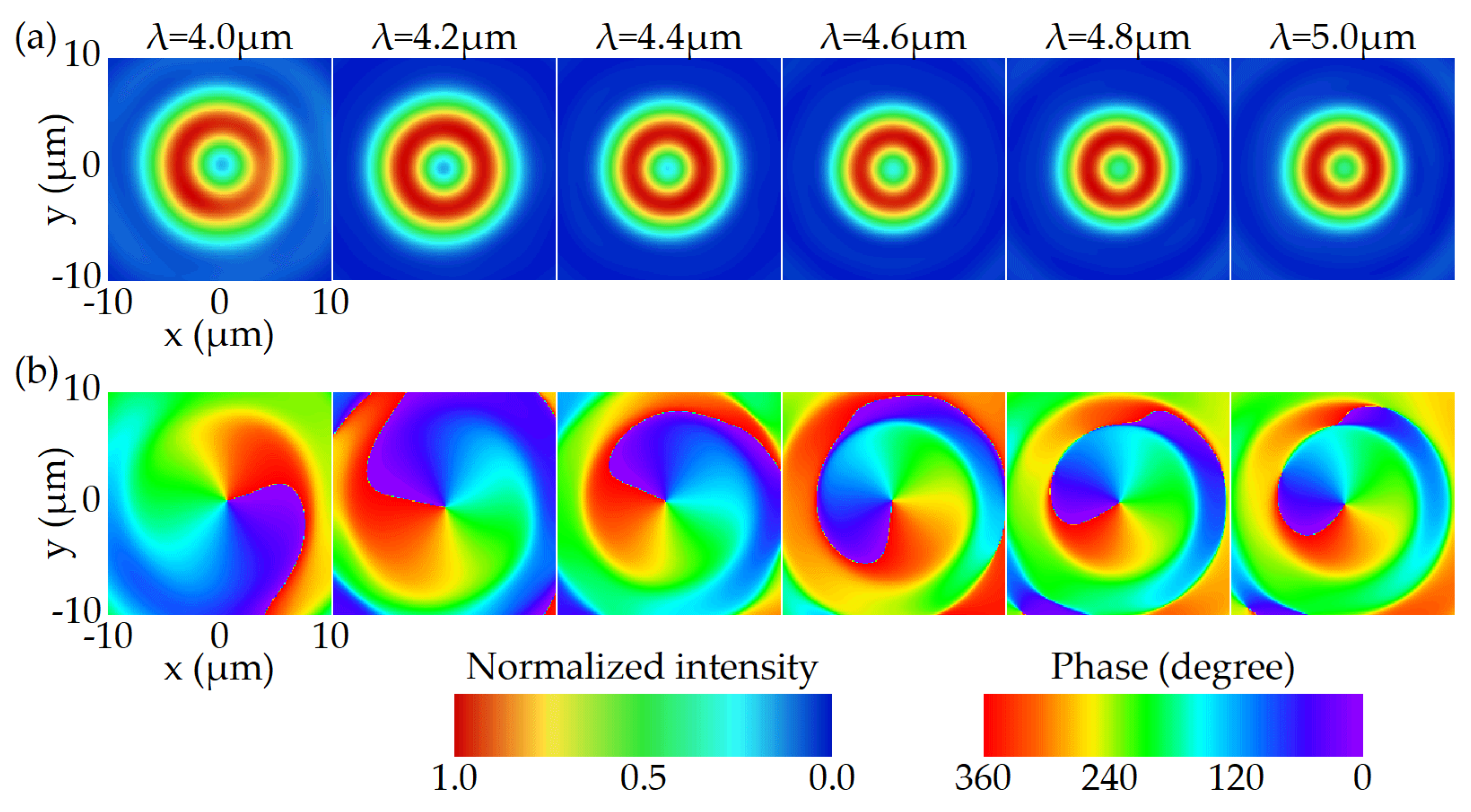
4.1. Regular Negative Dispersion
4.2. Zero Dispersion
4.3. Positive Dispersion
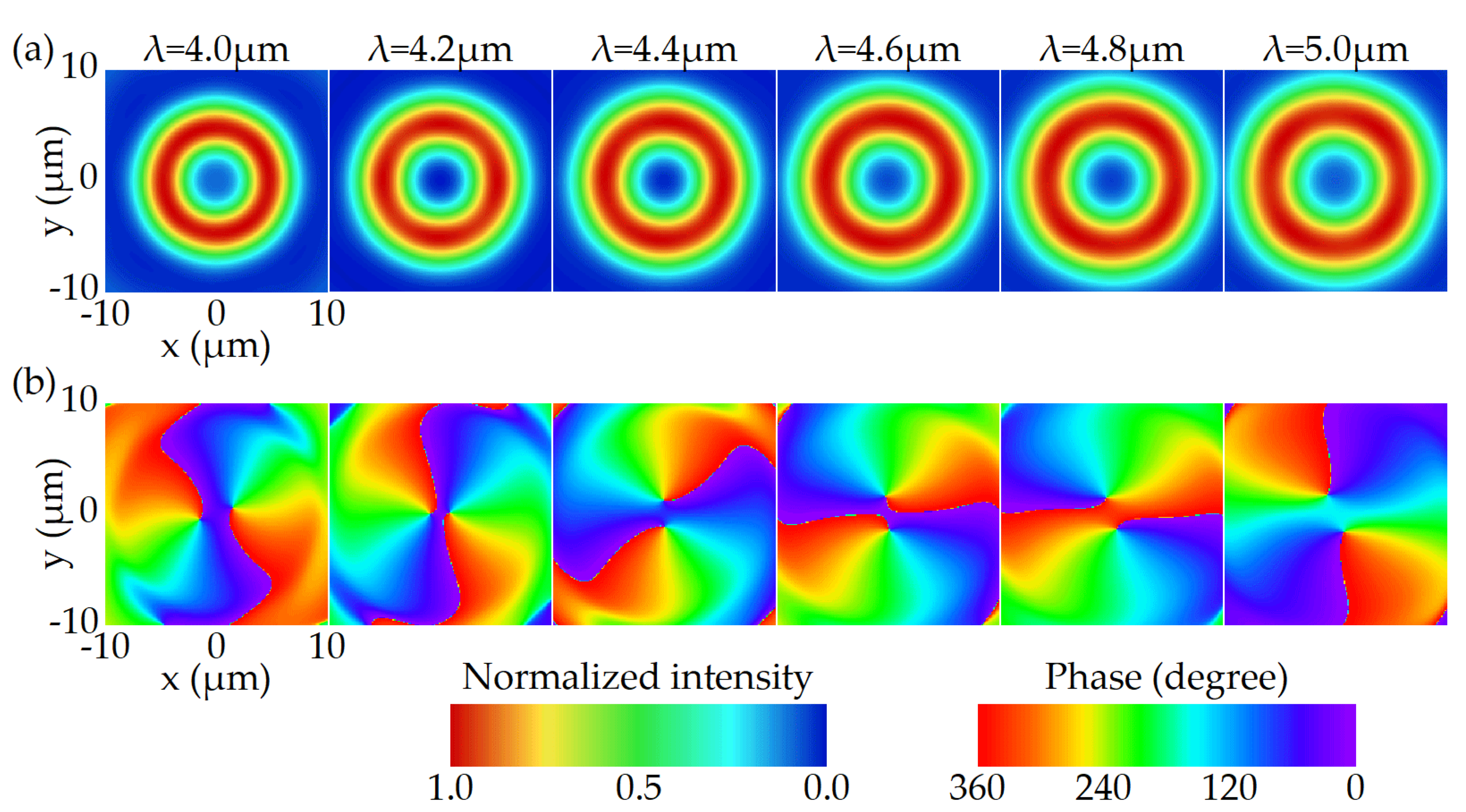
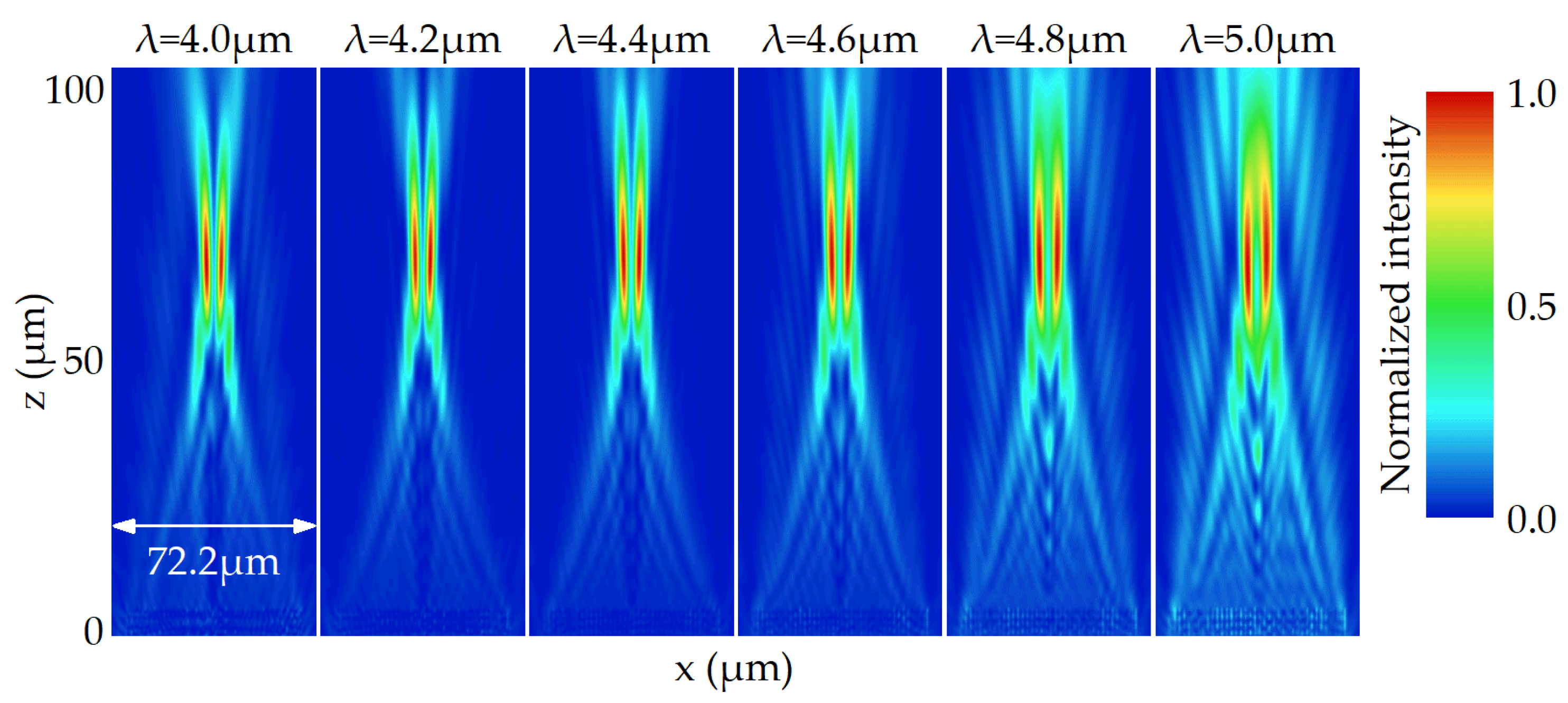
4.4. Hyper-Negative Dispersion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A

References
- Zhao, D.; Jin, Y.; Wang, B.Z.; Zang, R. Time reversal based broadband synthesis method for arbitrarily structured beam-steering arrays. IEEE Trans. Antennas Propag. 2011, 60, 164–173. [Google Scholar] [CrossRef]
- Sharma, S.; Dey, T.N. Phase-induced transparency-mediated structured-beam generation in a closed-loop tripod configuration. Phys. Rev. A 2017, 96, 033811. [Google Scholar] [CrossRef] [Green Version]
- Auyeung, R.C.; Kim, H.; Mathews, S.; Piqué, A. Laser forward transfer using structured light. Opt. Express 2015, 23, 422–430. [Google Scholar] [CrossRef] [PubMed]
- Sharma, V.; Kumar, S.C.; Samanta, G.K.; Ebrahim-Zadeh, M. Multi-structured-beam optical parametric oscillator. Opt. Express 2020, 28, 21650–21658. [Google Scholar] [CrossRef] [PubMed]
- Allen, L.; Beijersbergen, M.W.; Spreeuw, R.J.C.; Woerdman, J.P. Orbital angular momentum of light and the transformation of Laguerre-Gaussian laser modes. Phys. Rev. A 1992, 45, 8185. [Google Scholar] [CrossRef] [PubMed]
- Ng, J.; Lin, Z.; Chan, C.T. Theory of optical trapping by an optical vortex beam. Phys. Rev. Lett. 2010, 104, 103601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Liu, K.; Cheng, Y.; Gao, Y.; Li, X.; Qin, Y.; Wang, H. Super-resolution radar imaging based on experimental OAM beams. Appl. Phys. Lett. 2017, 110, 164102. [Google Scholar] [CrossRef]
- Shao, W.; Huang, S.; Liu, X.; Chen, M. Free-space optical communication with perfect optical vortex beams multiplexing. Opt. Commun. 2018, 427, 545–550. [Google Scholar] [CrossRef]
- Milione, G.; Nguyen, T.A.; Nolan, D.A.; Karimi, E.; Slussarenko, S.; Marrucci, L.; Alfano, R.R. Superdense coding with vector vortex beams: A classical analogy of entanglement. In Proceedings of the Frontiers in Optics 2013, Orlando, FL, USA, 6–10 October 2013. [Google Scholar] [CrossRef]
- Khonina, S.N.; Ustinov, A.V.; Logachev, V.I.; Porfirev, A.P. Properties of vortex light fields generated by generalized spiral phase plates. Phys. Rev. A 2020, 101, 043829. [Google Scholar] [CrossRef]
- Chen, R.; Wang, J.; Zhang, X.; Yao, J.; Ming, H.; Wang, A. Fiber-based mode converter for generating optical vortex beams. Opto-Electron. Adv. 2018, 1, 180003. [Google Scholar] [CrossRef] [Green Version]
- Khonina, S.N.; Karpeev, S.V.; Butt, M.A. Spatial-light-modulator-based multichannel data transmission by vortex beams of various orders. Sensors 2021, 21, 2988. [Google Scholar] [CrossRef] [PubMed]
- Aieta, F.; Genevet, P.; Kats, M.A.; Yu, N.; Blanchard, R.; Gaburro, Z.; Capasso, F. Aberration-free ultrathin flat lenses and axicons at telecom wavelengths based on plasmonic metasurfaces. Nano Lett. 2012, 12, 4932–4936. [Google Scholar] [CrossRef] [PubMed]
- Khorasaninejad, M.; Zhu, A.Y.; Roques-Carmes, C.; Chen, W.T.; Oh, J.; Mishra, I.; Devlin, R.C.; Capasso, F. Polarization-insensitive metalenses at visible wavelengths. Nano Lett. 2016, 16, 7229–7234. [Google Scholar] [CrossRef] [PubMed]
- Yu, N.; Genevet, P.; Kats, M.A.; Aieta, F.; Tetienne, J.P.; Capasso, F.; Gaburro, Z. Light propagation with phase discontinuities: Generalized laws of reflection and refraction. Science 2011, 334, 333–337. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Abdelsalam, M.; Mahmoud, A.M.; Swillam, M.A. Polarization independent dielectric metasurface for infrared beam steering applications. Sci. Rep. 2019, 9, 1–7. [Google Scholar] [CrossRef]
- Ni, X.; Kildishev, A.V.; Shalaev, V.M. Metasurface holograms for visible light. Nat. Commun. 2013, 4, 1–6. [Google Scholar] [CrossRef]
- Wen, D.; Yue, F.; Li, G.; Zheng, G.; Chan, K.; Chen, S.; Chen, M.; Li, K.F.; Wong, P.W.H.; Cheah, K.W.; et al. Helicity multiplexed broadband metasurface holograms. Nat. Commun. 2015, 6, 1–7. [Google Scholar] [CrossRef] [PubMed]
- Ma, X.; Pu, M.; Li, X.; Huang, C.; Wang, Y.; Pan, W.; Zhao, B.; Cui, J.; Wang, C.; Zhao, Z.; et al. A planar chiral meta-surface for optical vortex generation and focusing. Sci. Rep. 2015, 5, 1–7. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mehmood, M.Q.; Mei, S.; Hussain, S.; Huang, K.; Siew, S.Y.; Zhang, L.; Zhang, T.; Ling, X.; Liu, H.; Teng, J.; et al. Visible-frequency metasurface for structuring and spatially multiplexing optical vortices. Adv. Mater. 2016, 28, 2533–2539. [Google Scholar] [CrossRef] [PubMed]
- Shi, Z.; Khorasaninejad, M.; Huang, Y.W.; Roques-Carmes, C.; Zhu, A.Y.; Chen, W.T.; Sanjeev, V.; Ding, Z.W.; Tamagone, M.; Chaudhary, K.; et al. Single-layer metasurface with controllable multiwavelength functions. Nano Lett. 2018, 18, 2420–2427. [Google Scholar] [CrossRef] [PubMed]
- Liu, X.; Deng, J.; Jin, M.; Tang, Y.; Zhang, X.; Li, K.F.; Li, G. Cassegrain metasurface for generation of orbital angular momentum of light. Appl. Phys. Lett. 2019, 115, 221102. [Google Scholar] [CrossRef]
- Ding, F.; Chen, Y.; Bozhevolnyi, S.I. Focused vortex-beam generation using gap-surface plasmon metasurfaces. Nanophotonics 2020, 9, 371–378. [Google Scholar] [CrossRef] [Green Version]
- Tang, S.; Ding, F. High-efficiency focused optical vortex generation with geometric gap-surface plasmon metalenses. Appl. Phys. Lett. 2020, 117, 011103. [Google Scholar] [CrossRef]
- Yuan, Y.; Ding, X.; Zhang, K.; Wu, Q. Planar efficient metasurface for vortex beam generating and converging in microwave region. IEEE Trans. Magn. 2017, 53, 1–4. [Google Scholar] [CrossRef]
- Ou, K.; Li, G.; Li, T.; Yang, H.; Yu, F.; Chen, J.; Zhao, Z.; Cao, G.; Chen, X.; Lu, W. High efficiency focusing vortex generation and detection with polarization-insensitive dielectric metasurfaces. Nanoscale 2018, 10, 19154–19161. [Google Scholar] [CrossRef] [PubMed]
- Wang, S.; Wu, P.C.; Su, V.C.; Lai, Y.C.; Chu, C.H.; Chen, J.W.; Lu, S.H.; Chen, J.; Xu, B.; Kuan, C.H.; et al. Broadband achromatic optical metasurface devices. Nat. Commun. 2017, 8, 1–9. [Google Scholar] [CrossRef] [PubMed]
- Chen, W.T.; Zhu, A.Y.; Sanjeev, V.; Khorasaninejad, M.; Shi, Z.; Lee, E.; Capasso, F. A broadband achromatic metalens for focusing and imaging in the visible. Nat. Nanotechnol. 2018, 13, 220–226. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kang, M.; Feng, T.; Wang, H.T.; Li, J. Wave front engineering from an array of thin aperture antennas. Opt. Express 2012, 20, 15882–15890. [Google Scholar] [CrossRef] [PubMed]
- Khorasaninejad, M.; Chen, W.T.; Devlin, R.C.; Oh, J.; Zhu, A.Y.; Capasso, F. Metalenses at visible wavelengths: Diffraction-limited focusing and subwavelength resolution imaging. Science 2016, 352, 1190–1194. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mueller, J.P.B.; Rubin, N.A.; Devlin, R.C.; Groever, B.; Capasso, F. Metasurface polarization optics: Independent phase control of arbitrary orthogonal states of polarization. Phys. Rev. Lett. 2017, 118, 113901. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Arbabi, A.; Horie, Y.; Ball, A.J.; Bagheri, M.; Faraon, A. Subwavelength-thick lenses with high numerical apertures and large efficiency based on high-contrast transmitarrays. Nat. Commun. 2015, 6, 1–6. [Google Scholar] [CrossRef] [PubMed]
- Khorasaninejad, M.; Shi, Z.; Zhu, A.Y.; Chen, W.T.; Sanjeev, V.; Zaidi, A.; Capasso, F. Achromatic metalens over 60 nm bandwidth in the visible and metalens with reverse chromatic dispersion. Nano Lett. 2017, 17, 1819–1824. [Google Scholar] [CrossRef] [PubMed]
- Arbabi, E.; Arbabi, A.; Kamali, S.M.; Horie, Y.; Faraon, A. Controlling the sign of chromatic dispersion in diffractive optics with dielectric metasurfaces. Optica 2017, 4, 625–632. [Google Scholar] [CrossRef]
- Li, L.; Yuan, Q.; Chen, R.; Zou, X.; Zang, W.; Li, T.; Zheng, G.; Wang, S.; Wang, Z.; Zhu, S. Chromatic dispersion manipulation based on metasurface devices in the mid-infrared region. Chin. Opt. Lett. 2020, 18, 082401. [Google Scholar] [CrossRef]
- Yee, K. Numerical solution of initial boundary value problems involving Maxwell’s equations in isotropic media. IEEE Trans. Antennas Propag. 1966, 14, 302–307. [Google Scholar] [CrossRef] [Green Version]
- Mur, G. Absorbing boundary conditions for the finite-difference approximation of the time-domain electromagnetic-field equations. IEEE Trans. Electromagn. Compat. 1981, 4, 377–382. [Google Scholar] [CrossRef]
- Chaudhury, B.; Boeuf, J.P. Computational studies of filamentary pattern formation in a high power microwave breakdown generated air plasma. IEEE Trans. Plasma Sci. 2010, 38, 2281–2288. [Google Scholar] [CrossRef]
- Moxley III, F.I.; Chuss, D.T.; Dai, W. A generalized finite-difference time-domain scheme for solving nonlinear Schrödinger equations. Comput. Phys. Commun. 2013, 184, 1834–1841. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, X.; Fan, Z. Controlling Dispersion Characteristic of Focused Vortex Beam Generation. Photonics 2022, 9, 179. https://doi.org/10.3390/photonics9030179
Li X, Fan Z. Controlling Dispersion Characteristic of Focused Vortex Beam Generation. Photonics. 2022; 9(3):179. https://doi.org/10.3390/photonics9030179
Chicago/Turabian StyleLi, Xueshen, and Zhigang Fan. 2022. "Controlling Dispersion Characteristic of Focused Vortex Beam Generation" Photonics 9, no. 3: 179. https://doi.org/10.3390/photonics9030179
APA StyleLi, X., & Fan, Z. (2022). Controlling Dispersion Characteristic of Focused Vortex Beam Generation. Photonics, 9(3), 179. https://doi.org/10.3390/photonics9030179




