Performance Improvement of Graded Bandgap Solar Cell via Optimization of Energy Levels Alignment in Si Quantum Dot, TiO₂ Nanoparticles, and Porous Si

Abstract

Charge carriers’ generation from zinc includes silicon quantum dots (ZnSiQDs) layer sandwiched in-between porous silicon (PSi) and titania nanoparticles (TiO₂NPs) layer-based solar cell is an efficient way to improve the cell’s performance. In this view, ZnSiQDs layer with various QDs sizes have been inserted, separating the PSi and TiO₂NPs layers to achieve some graded bandgap quantum dot solar cells (GBQDSCs). In this process, ZnSiQDs of mean diameter 1.22 nm is first prepared via the top-down method. Next, ZnSiQDs have been re-grown using the bottom-up approach to get various mean diameters of 2.1, 2.7 and 7.4 nm. TiO₂NPs of mean diameter in the range of 3.2 to 33.94 nm have been achieved via thermal annealing. The influence of different ZnSiQDs sizes on the designed GBGQDSCs performance has been determined. The proposed cell attains a short circuit current of 40 mA/cm² and an efficiency of 4.9%. It has been shown that the cell performance enhances by optimizing the energy levels alignment in the PSi, ZnSiQDs, TiO₂NPs layers.

1. Introduction

Around 0.2% of the total radiation from the sun is sufficient to meet the annual global energy demand [1]. Presently, more than 90% of the commercially available solar cells are established on silicon (Si) materials with the maximum efficiency of monocrystalline and polycrystalline Si solar cells are 22–25% and 18–21%, respectively. The manufacturing costs of the Si-based solar cells and their limited efficiency of the photon to current conversion are the two key issues that must be addressed. The low photogeneration in crystalline Si (c-Si) is due to its high reflectance (over 40%) and high refractive index (approximately 3.5) [2], which appreciably affects the solar cell performance unless inhibited [3,4]. Amongst all other types of solar cells, the c-Si solar cell is still the best choice for photovoltaic customers due to its highest efficiency, long stability, and abundance of Si on earth crust. However, despite Si’s high recombination rate and high thermal and optical losses, it remains the workhorse in the photovoltaic industry [5]. To reduce the total losses from c-Si growth of different nanostructures such as Si nanowires [6,7], micro-and mesopores and quantum dots [6,8] on c-Si have been strategized. These nanostructures have been utilized to minimize the reflection over a broadband of incident light via the figuration density graded layer with tinier features compared to the incident light wavelength. These additive layers can serve as an antireflection film, eliminating the need for vacuum-deposited costly antireflection coatings, thereby reducing the manufacturing cost of the cell [9].

In recent years, Si quantum dots (SiQDs) have widely been applied in biotechnology, solar cells, and light-emitting diode (LED) [10,11]. The phenomena called the quantum confinement effect (QCE) in SiQDs that arises due to the size reduction can limit the movement of the charge carriers (like confined electrons in the atom) [12]. This QCE can be exploited to convert indirect bandgap Si to direct energy bandgap, making nanoSi effective for optoelectronic applications. SiQDs can be produced via the top-down, bottom-up, and combination of the other methods. Colloidal suspension if SiQDs can be achieved using electrochemical etching of porous Si (PSi) assisted with ultrasonication process [12,13]. Electrochemical etching and wet etching processes can produce highly crystalline SiQDs due to well-crystallized starting material, wherein they use hydrofluoric (HF) acid, ethanol (ET), deionized water (DIW), hydrogen peroxide (H₂O₂) and HF-HNO₃. In this process, Si-O and Si-H are created on the surface. The density of these bonds relies on the etching parameters, including the etching times, HF acid concentrations, and current densities. For instance, an increase in the concentration of HF acid can lead to the dissolution of the silicon oxide layers, making Si-H bonds predominant. Due to the generation of new states via the stress at the interface of the oxide/SiQDs, Si-O surface bond produces a pair of energy states within the band gap region of SiQDs. One of these energy levels lies near the edge of the conduction band (CB) and another occurs near to valance band (VB) edge, acting as the trap levels.

As an anti-reflectance (AR) layer, PSi or black silicon (b-Si) have been utilized in solar cells [2]. High AR of PSi is related to the high porosity that absorbs the short wavelength of solar irradiation and converts it to the long-wavelength wherein the efficiency of the solar cell fabricated from PSi exceeded 22% [3,14]. The reflection of PSi proportionally gets inversed with the etching time due to its increased porosity, increasing the surface roughness. In contrast, the power conversion efficiency (PCE) increased with the etching time up to a specific value due to the increased absorbance or decreased reflectance by PSi. With longer etching time, the cell’s PCE has been reduced due to the surface oxidation-mediated increase in the trap density and low carriers mobility resulting from the generation of the intrinsic layer in the etching process. The observed enhancement in the porosity led to the reduction in the crystallite size, thus increasing the transparency of PSi [6]. The Fermi energy level of PSi positioned at the middle of the energy bandgap was vanished with the decrease in dopant concentration due to the generation of pores by the electrochemical etching [15,16]. Furthermore, due to QCE (reduction in the crystallite size of Si nanostructures), the bandgap of PSi became wider compared to their crystalline bulk counterpart [2,17]. Despite few studies, the mechanism of charge carriers’ generation from zinc included silicon quantum dots (ZnSiQDs) layer sandwiched in-between PSi and titania nanoparticles (TiO₂NPs) layer-based solar cell and its effect on the improvement of the cell’s performance has poorly been understood. Therefore, it is vital to examine the impact of various ZnSiQDs layer thicknesses on the performance of graded bandgap quantum dot solar cells (GBQDSCs) by inserting such layer in-between PSi and TiO₂NPs layers.

Quantum dot sensitized solar cells (QDSSCs) are considered one of the third generation’s innovative solar cells due to the excellent optoelectronic of QDs light absorber properties, such as significant absorption coefficient, flexible light-harvesting capability, high stability and low-cost availability. In comparison, the theoretical limit of QDSSC power conversion efficiency (PCE) can be beyond the level of Shockley-Queisser (33.7%) due to the multiexciton generation (MEG) [18,19]. Shockley-Quiesser limit is the maximum PCE for the p-n solar cell at 1.5 air mass (AM), and the bandgap is 1.34 eV, where the loss only is radiative recombination. In reality, the maximum PCE does not reach to Shockley-Queisser limit due to the high amount of light reflected from the surface [20,21]. Figure 1 shows the pathways of the energy loss through the homogeneous junction. The two most important causes of power loss in single-band photovoltaic devices are photons with less energy than the bandgap and photons above the bandgap [22].

Therefore, three solutions were proposed to solve those problems [18]: (a) increasing the number of bandgaps, (b) extraction of the carriers before thermalization and (c) MEG per high energy photon [23].

Over the last decades, QDs-sensitized solar cells (QDSSCs) became promising wherein QDs of PbS, CdSe, CdSeTe, ZnS, and CdS have broadly been used to enhance the cell’s PCE (η) over 13% and current density (J_SC) approximately 30 mA/cm² [24,25]. However, these solar cells are made up of highly hazardous heavy metals, including lead and cadmium, which are controlled in electronic consumer goods by various countries [24,26]. It is a major disadvantage of QDSSCs acceptance at the commercial level. Consequently, less toxic elements in solar cell manufacturing became demanding, which is the main motivation of this work. Based on these factors, we incorporated SiQDs of different sizes in Zn to get ZnSiQDs which was then utilized as an active layer (for light absorption at different wavelengths in the solar spectrum) to fabricate GBQDSCs. The bottom-up method was used to produce these ZnSiQDs of homogeneous morphologies (shapes and sizes). The performance of the proposed cells was evaluated in terms of η and J_SC. A very high value of Jsc (40 mA/ cm²) and PCE (4.99%) was obtained. Triple active layers of ZnSiQDs (TL-ZnSiQDs) with the thicknesses of 7.8 nm (band gap energy of 3.3 eV), 2.7 nm (band gap energy of 2.5 eV), and 2.1 nm (band gap energy of 2.3 eV) were deposited on the PSi layer. Photovoltaic devices of various architectures were studied to achieve the optimum performance in terms of the cells parameters such as fill factor (FF), open circuit voltage (V_OC), short circuit current (I_SC), η and so forth. The achieved best cell structure had the configuration of Al/p-c-Si/PSi/ZnSiQDs (2.1 nm)/ZnSiQDs (2.7 nm)/ZnSiQDs (7.4 nm)/TiO₂NPs/Ag. The obtained results were analyzed, interpreted, discussed and validated with state-of-the-art existing reports in the literature.

Therefore, this research did not aim to develop high-efficiency GBQDSCs, but rather to (1) synthesis and characterize non-toxic and low-cost quantum dots, which will be used in solar cells. In addition, (2) to understand better the mechanisms that control the performance of the solar cells based on SiQDs, PSi, and TiO2NPs.

2. Materials and Methods

2.1. Synthesis of Colloidal ZnSiQDs

Zinc (Zn) incorporated PSi (ZnPSi) and colloidal silicon quantum dots (ZnSiQDs) were prepared using high purity HF (purity of 48%), ET (purity of 99.9%), Zn powder (purity of 99.9%), acetone (C₃H₆O), ammonium hydroxide (NH₄OH, purity range of 28.0–30.0%), and n-type Si (100) (resistivity in-between 0.002 to 0.005 Ω.cm). The detailed preparation protocol has been reported elsewhere [27,28]. The ZnPSi was used as a resource to produce the ZnSiQDs. The n-type Si (100) wafer of dimension 1.5 cm × 2.5 cm were cleaned via the RCA method [29]. A Teflon cell was used to produce ZnPSi; wherein zinc powder (0.17 g) was mixed to a solution made of HF and ET (volume ratio of 1:1). First, the n-type Si (100) as an anode and platinum (Pt) wire as cathode was immersed in the resultant solution to make the ZnPSi film. Next, the ethanoic HF within the Teflon cell was lit with a tungsten lamp, and the etching process was carried out at different current densities (in the range of 5 to 45 mA/cm² at an incremental step of 5 mA/cm². The solution concentration ratio (1:1) and etching time (20 min) were kept constant. The obtained film was electro-polished to extract the ZnPSi from the n-Si substrate. The brown fragments of ZnPSi film were collected by centrifugation (at 1000 rpm to 5 min) from the HF and ET solution. Later, the brown fragments were ultrasonicated in acetone for 1 h to produce a grey solution followed by filtering and centrifugation (at 1500 rpm for 30 min) to synthesize the colloidal ZnSiQDs (diameter of 1.22 nm) in acetone. The bottom-up approach was used for the re-growth of ZnSiQDs wherein different volumes of NH₄OH (15, 20, and 25 μL) were added to the colloidal ZnSiQDs suspension in acetone before being left in a dark environment for 72 h. Under ultraviolet light illumination, the colloidal ZnSiQDs suspension with NH₄OH revealed various colors. About 20 mL of colloidal ZnSiQDs with NH₄OH in acetone were mixed with 1 μL of polyvinylpyrrolidone (PVP) in 40 μL of DIW to regulate the size of the colloidal ZnSiQDs with NH₄OH, wherein the resultant mixture was constantly agitated for 30 min. All the prepared samples were characterized at room temperature.

2.2. Synthesis of TiO₂NPs

Titanium tetrachloride (TiCl₄ of 0.09 M in 20% of HCl), NH₄OH (purity range of 28.0 to 30.0%), and acetic acid (C₂H₄O₂, purity of 99.7%) were obtained from Sigma Aldrich (Hong Kong, China) and used to make TiO₂NPs. First, around 3 mL of TiCl₄ was combined with 20 mL of DIW followed by vigorous stirring until the complete dissolution of TiCl₄. Then, NH₄OH was added drop by drop to the resulting mixture until the pH became 8. The white gel of Ti(OH)₄ that appeared after a few minutes was filtrated and washed many times by DIW to remove the chloride ions [30,31]. Then, about 6 mL of Ti(OH)₄ gel was added to 36 mL of DI with constant agitated stirring until the complete dissolution. Later, approximately 12 mL of C₂H₅O₂ was added to Ti(OH)₄ and DIW to restrict the degree of hydrolysis, resulting in (CH₃COO)₄Ti heated on a hotplate at 60 °C for 2 h. Finally, (CH₃COO)₄Ti was heated at 400 °C for 3 h to get high-quality TiO₂ NPs-based thin film.

2.3. Synthesis of PSi Layers

A p-type crystalline Si (100) (p-c-Si, resistivity in the range of 0.002 to 0.005 Ω.cm) was used as substrate. First, the p-c-Si wafer was cut into small sections of the dimension of 1 cm × 1 cm and then cleaned by the RCA method. Then, PSi was grown by the electrochemical etching process at various etching times in 30 s to 120 s. The other parameters, such as HF and ET ratio (1:1), current density (10 mA/cm²), were kept fixed. The etching process was carried out in a Teflon ring cell.

2.4. Synthesis of GBQDSCs

First, thin films of ZnSiQDs and TiO₂NPs were deposited on the PSi layer using the combined spray and spin-coating techniques to get the triple-layer solar cell. The layers deposition arrangement has relied on the bandgap energy values of various layers. Next, the DC sputtering technique was used to deposit the metal electrodes of Al (using Aluminum target, purity of 99.99%) and Ag (using silver target, purity of 99.9%) on the triple layers to make the contacts on the solar cell. The sputtering machine was operated at 5 × 10⁻⁵ mbar and 30 W for Ag target and 150 W for Al target. The forward contacts Ag were finger mask shaped (the fixed active region with 0.5 mm × 3 mm). Finally, the obtained solar cells were annealed at 400 °C for 3 h.

2.5. Charactrization

A field emission scanning electron microscope was used to investigate the samples’ morphology (FESEM, FEI Nova SEM 450, FEI Company, Hillsboro, OR, USA). An energy dispersive X-ray diffraction (EDX, FEI Nova SEM 450, FEI Company, Hillsboro, OR, USA) spectrometer was used to evaluate the elemental compositions of the samples. An energy-filtered transmission electron microscope was used to investigate the morphologies of colloidal TiO₂NPs and ZnSiQDs (EFTEM, Libra 120, Zeiss GmbH, Oberkochen, Germany). The UV–Vis–NIR absorption spectra of the samples were recorded using an Agilent Carry 5000 absorption spectrophotometer. With a tiny physical probe or sharp tip, the atomic force microscopic (AFM, Model: Dimension EDGE, BRUKER, Ltd., Manchester, UK) was used to scan the surface of a material. X-ray diffraction (XRD, Bruker D8 Advance, AXS GmbH, Karlsruhe, Germany) is a non-destructive characterization method for determining the crystalline phases of nanomaterials as well as structural information such preferred orientation and crystallite size. X-ray diffractometry is based on the Bragg equation. The thin film of TiO₂NPs and ZnSiQDs were deposited using a spin coating device, whereas the Auto HHV500 Sputter Coater or Radio Frequency (RF) and Direct Current (DC) Sputtering Magnetron was utilized to deposit the thin film of conductive materials such as Ag and Al. The PCE, FF, J_SC, and V_OC were measured using an electrical circuit measurement device (LED simulator).

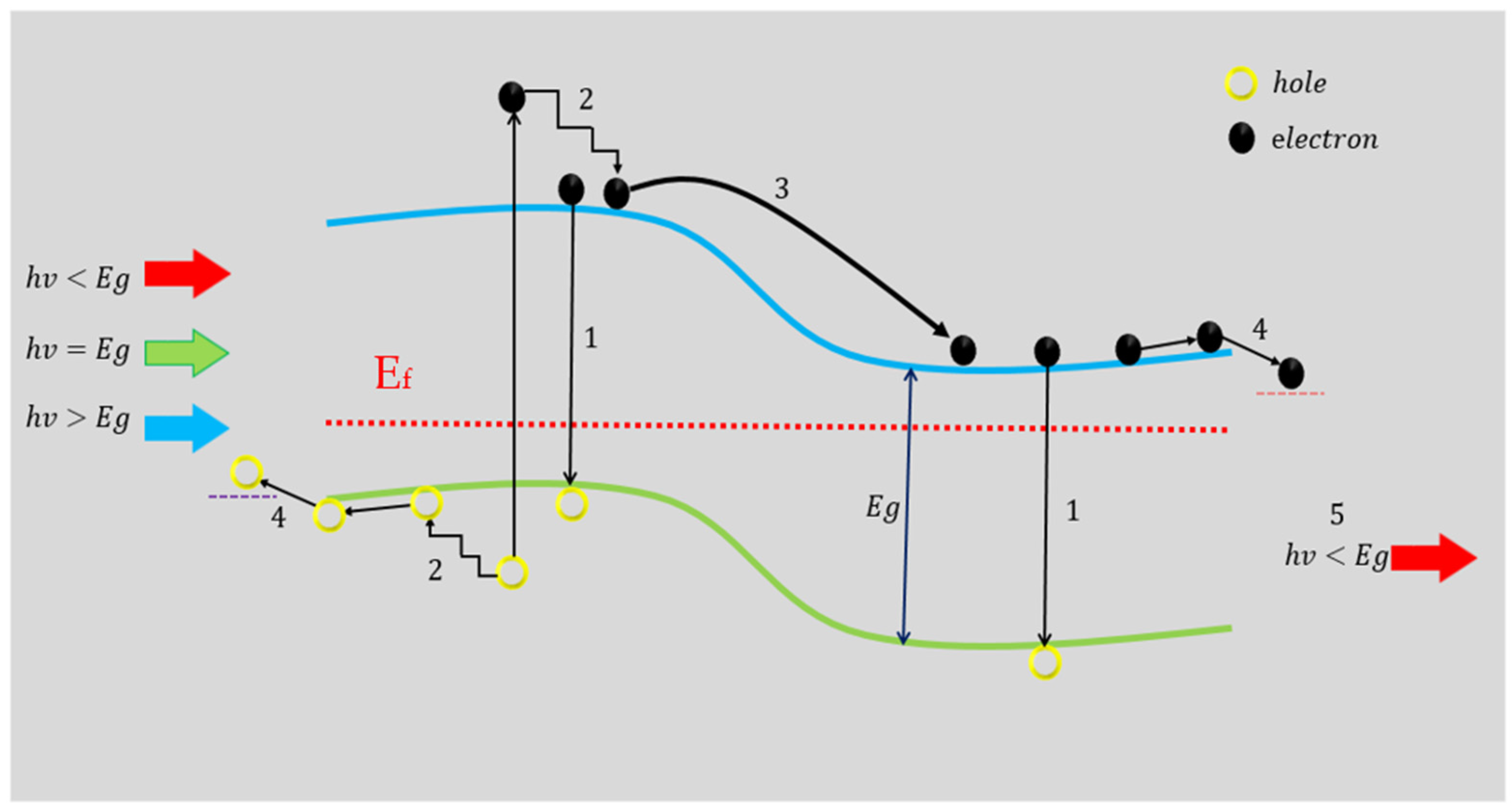
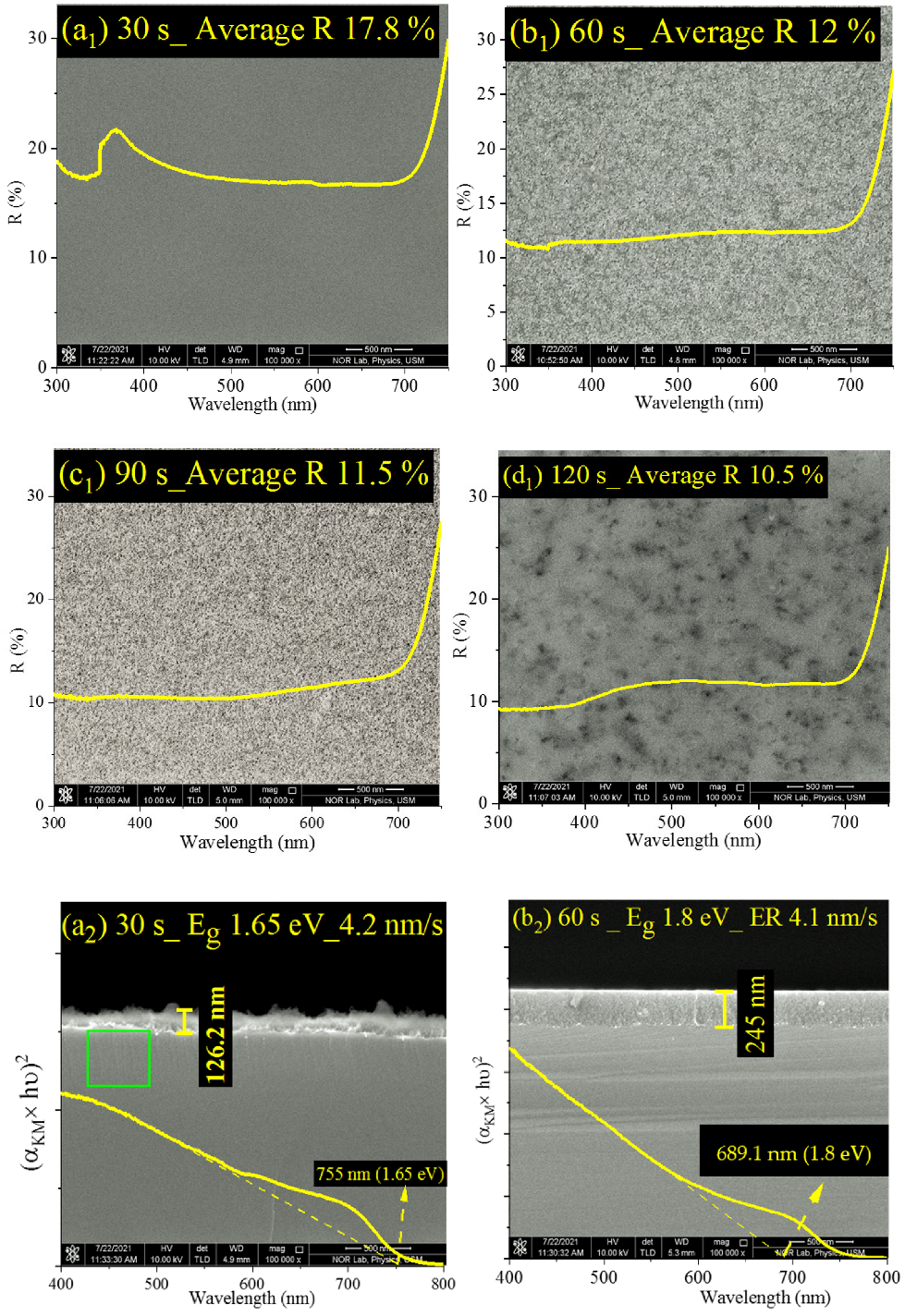
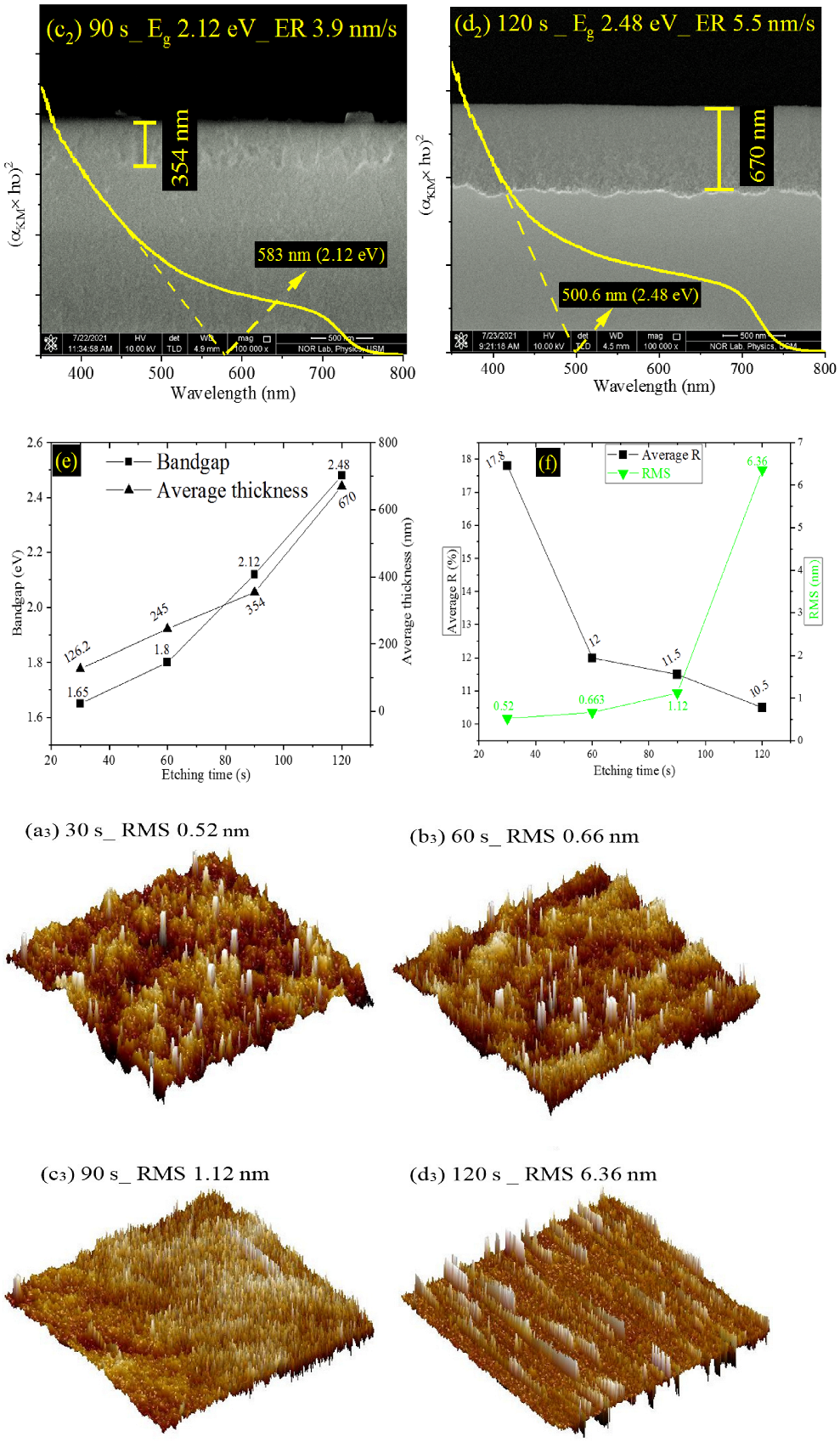
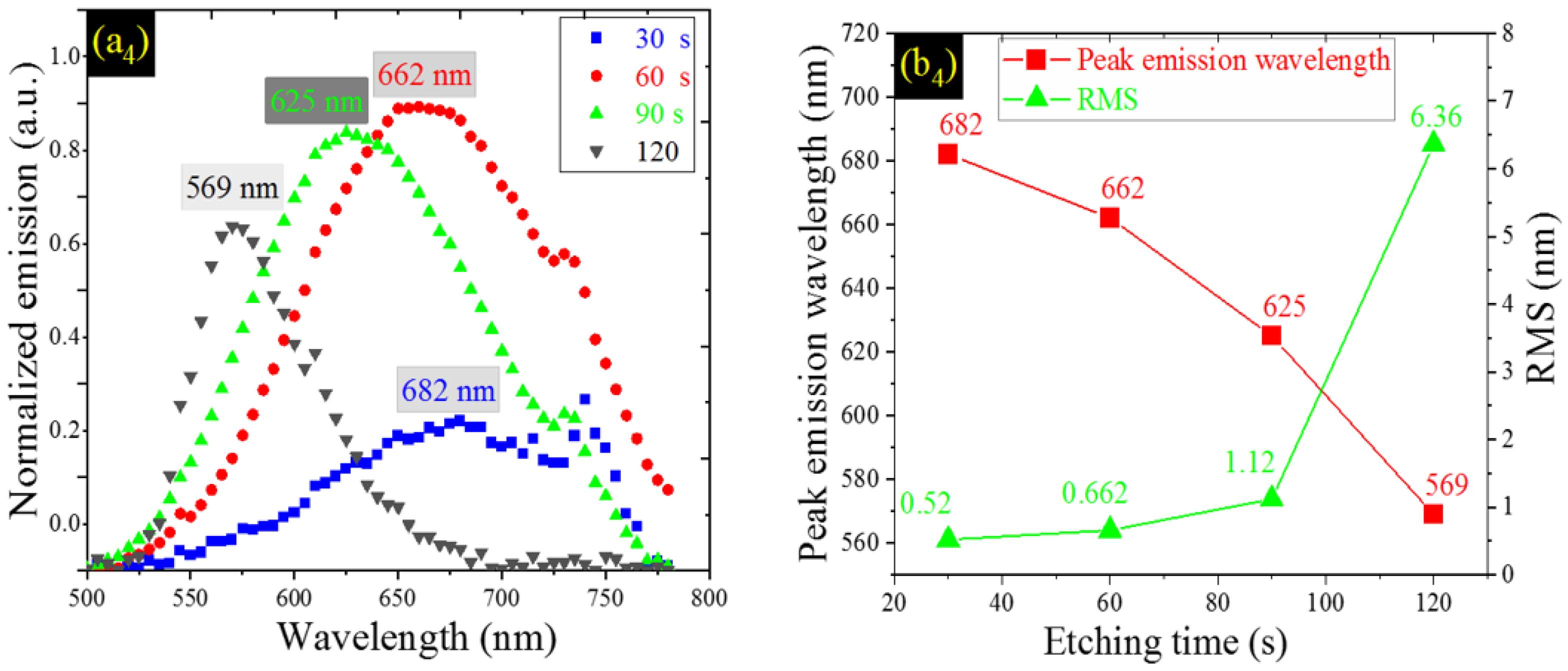
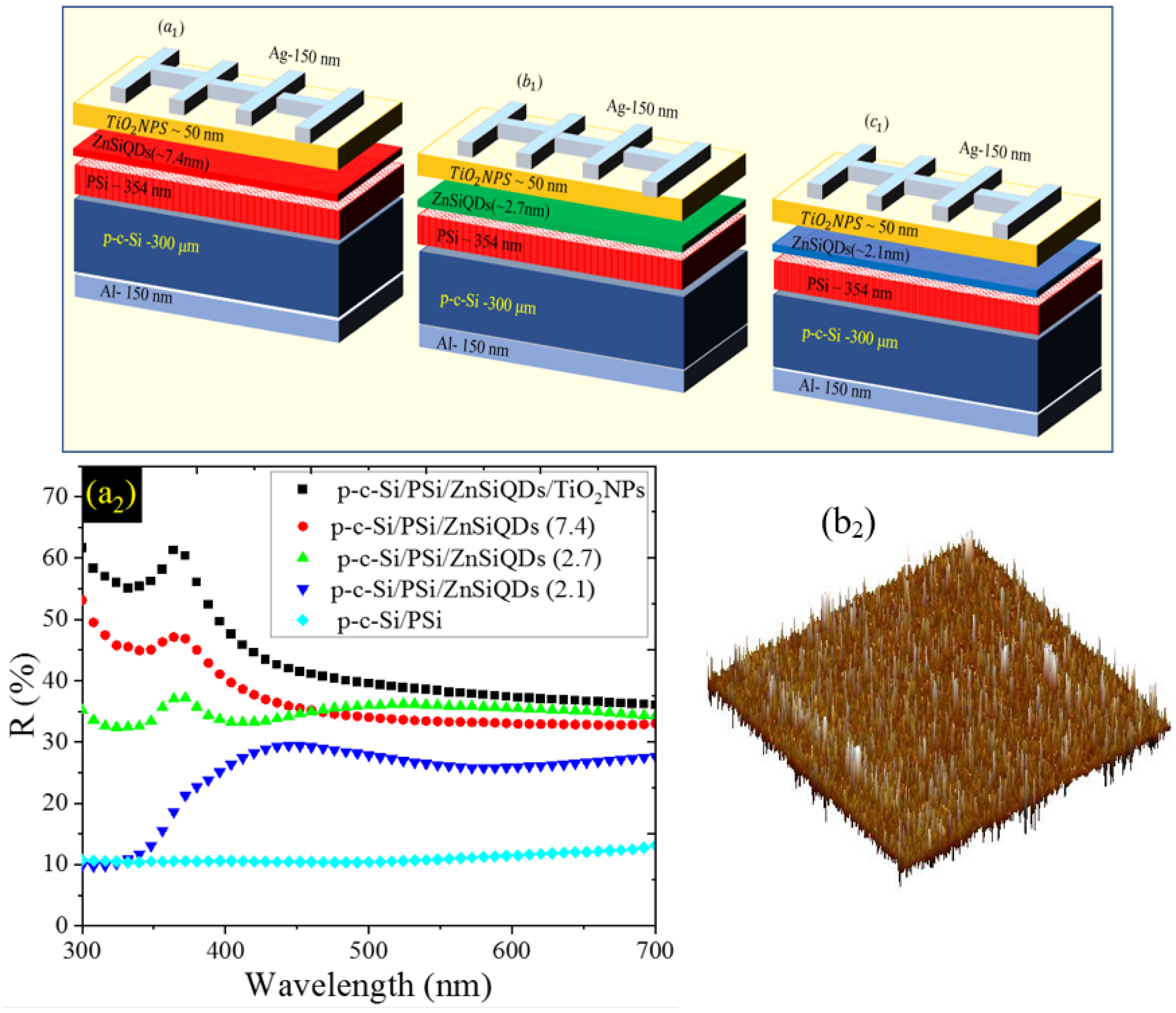
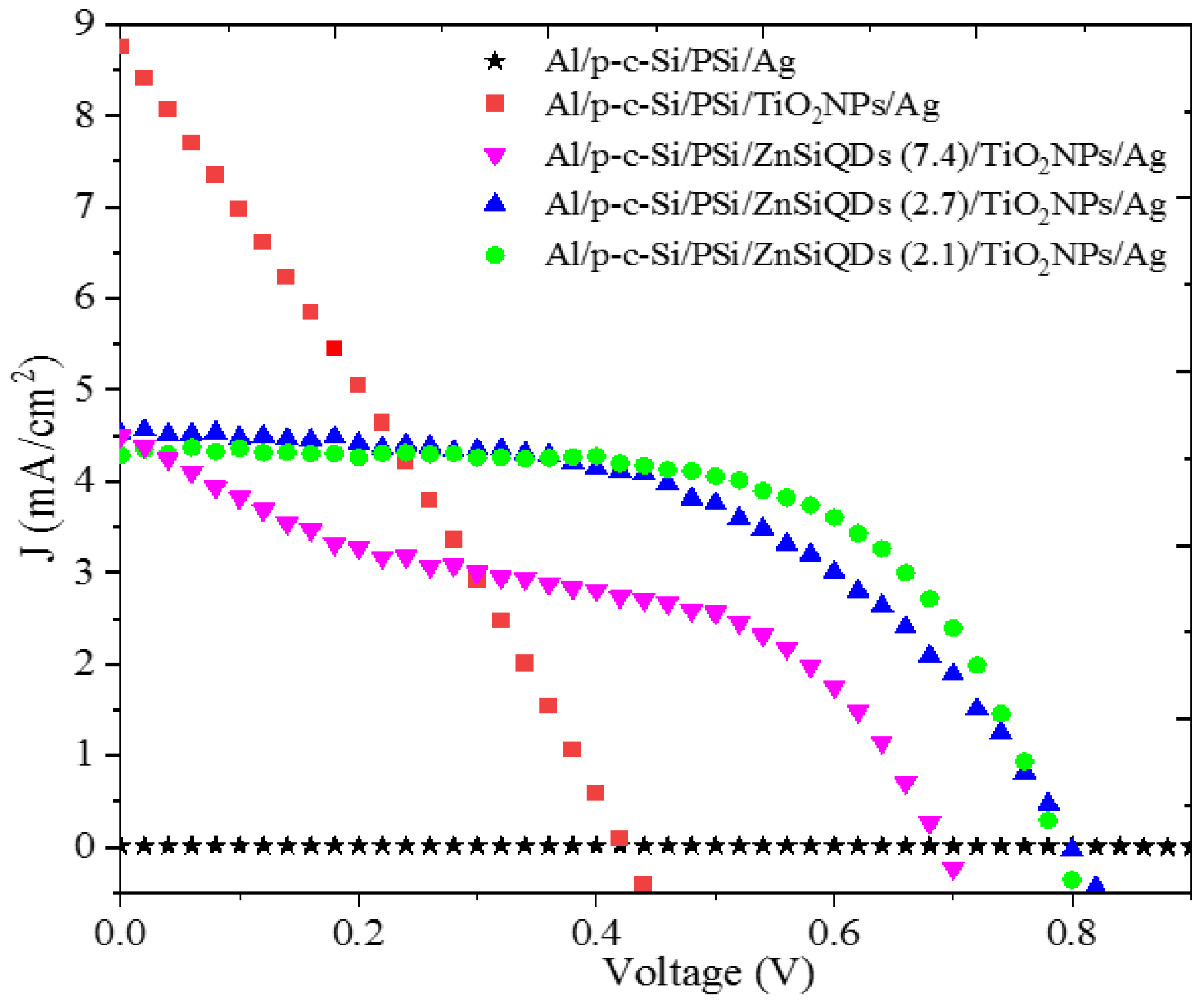
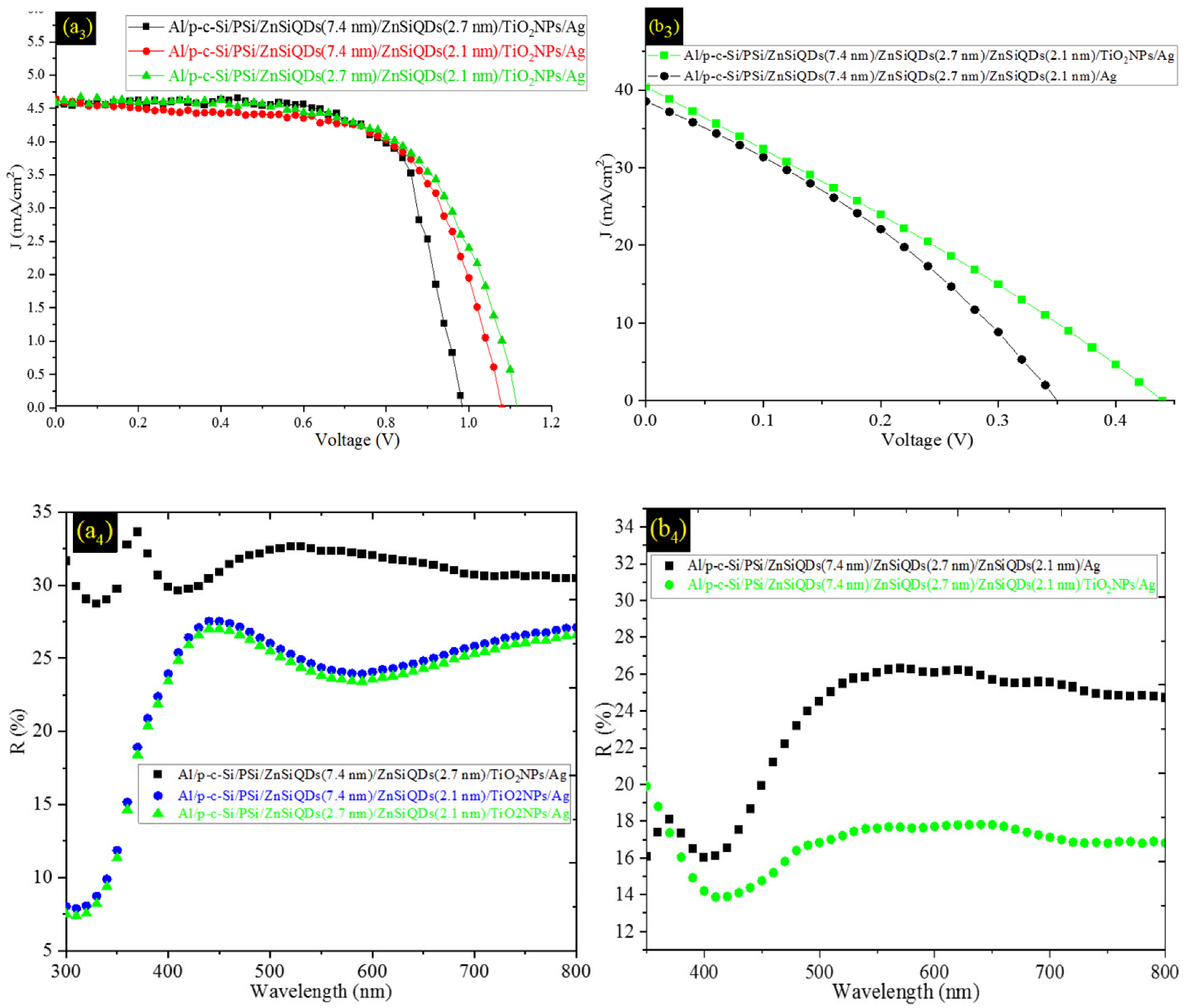
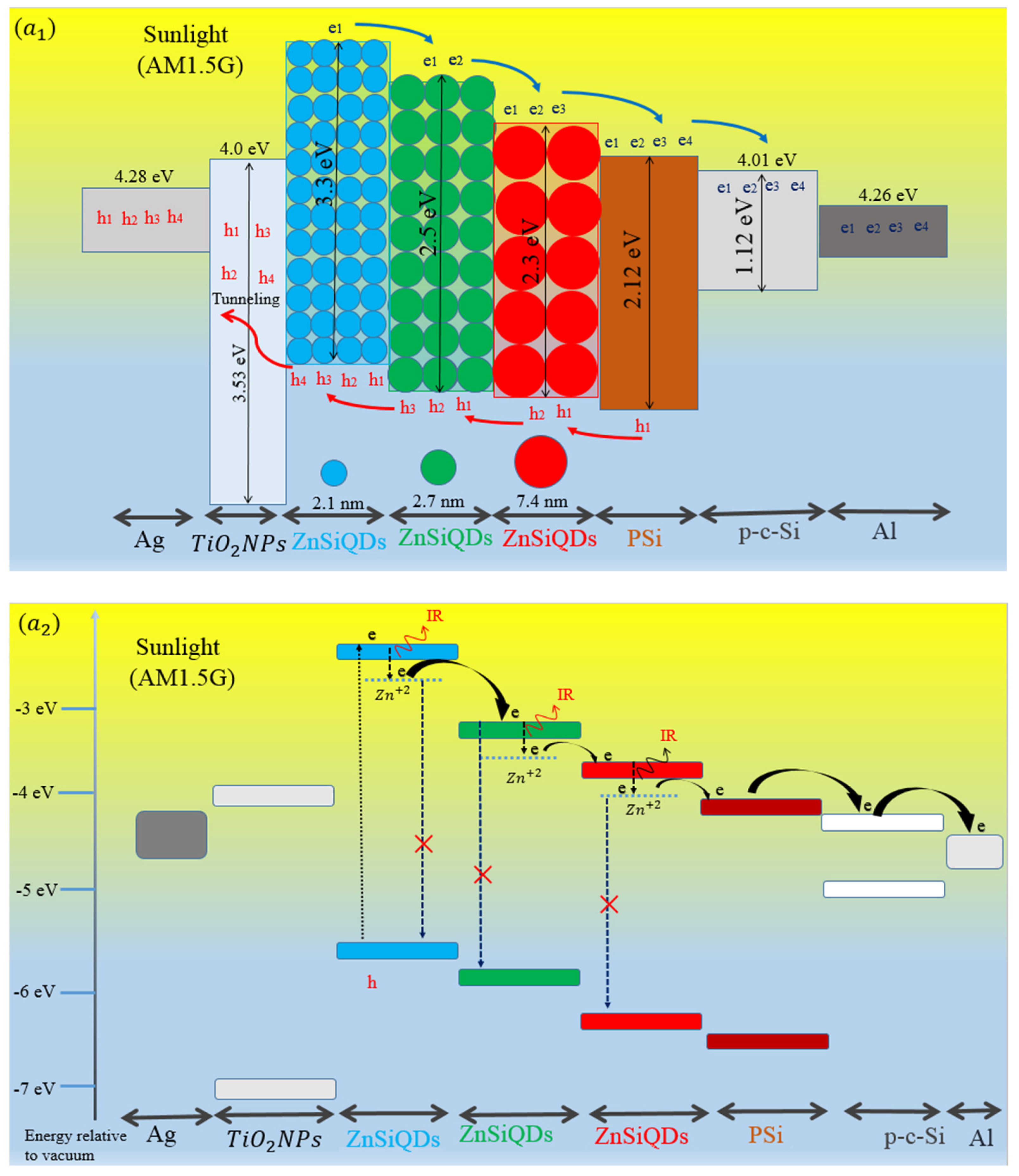
3. Results and Discussion

3.1. Structure and Morphology of ZnPSi and ZnSiQDs

Figure 2a,b shows the FESEM micrographs of ZnPSi etched at 5 mA/cm². In addition, PL spectra and XRD profiles of the film are shown as yellow curves. The porosity of the film was obtained using the weight measurement [32]. With the increase in porosity, the PL peak was blue-shifted, ascribing to the bandgap widening caused by the smaller crystallite size [33,34]. After the etching, the sample emitted red light under UV illumination, which was ascribed to the strong QCE due to particle size reduction (less than the exciton Bohr radius). Figure 2b depicts the measured Si crystallite size less than 10 nm [34,35]. The sharp XRD peaks corresponding to ZnO and Si indicated the purity and high crystallinity of the produced film. Debye-Scherrer equation was used to estimate the mean size of Si crystallites present in the film in which intense XRD data was considered [36]. After etching, the dimensions of crystallites were appreciably due to the pores generation on the substrate. Figure 2b shows the formation of ZnO and Si nanostructures with the corresponding mean crystallite size of approximately 21 nm and 6 nm.

Figure 2c–f illustrates the EFTEM micrographs of various ZnSiQDs samples combined with their respective nanocrystallites size distribution. ZnSiQDs of 20 mL suspended in acetone added with different volumes of NH₄OH are shown. ImageJ software was utilized to estimate the mean size of the nanoparticles. Spherical and semi-hexagonal shaped ZnSiQDs (yellow circle) with the mean size of 1.22, 2.1, 2.77, and 7.4 nm were obtained with the corresponding NH₄OH volumes of 0, 15, 20, and 25 µL. The variation of NH₄OH amount considerably affected the morphologies (sizes and shapes) of ZnSiQDs, causing the QDs size distribution to become more uniform with reduced inter-particle spacing. In short, the addition of NH₄OH into the colloidal ZnSiQDs suspension enabled the re-growth of tinier nanoparticles and the formation chain through a central core [37,38].

Figure 2g displays the UV-Vis absorption spectra of the colloidal ZnSiQDs suspended in acetone added with different volumes of NH₄OH (15, 20, and 25 μL). The inset shows the NH₄OH volume-dependent optical band gap energies (E_g) of ZnSiQDs. With the increase in NH₄OH volume from 15 to 25 μL, the corresponding value of E_g was decreased from 3.6 to 2.2 eV. The observed narrowing of the energy bandgap with the increase in NH₄OH volume was attributed to the generation of large number of OH⁻ and NH₄⁺ that in turn facilitated the formation of substantial ZnSiQDs [39].

Because of its superior mechanical and thermal qualities, transparent Si-based film shows excellent performance and can be obtained at a low cost, making PSi a promising material for solar cells design. Due to its wide surface areas in the compact volumes, PSi was transformed to the direct bandgap energy material, ensuring the most extensive incident light absorption from the solar spectrum, resulting in a rise in solar cell efficiency. In addition, the unique optical characteristics of PSi make it a versatile candidate for diverse optoelectronic applications [40,41,42]. Meanwhile, the electrical characteristics and performance of PSi-based solar cells were determined by measuring the I-V curve using a solar simulator. The solar cell was fabricated by depositing three layers of ZnSiQDs (different E_g values of 2.2, 2.4, and 3.4 eV) onto PSi. Unified spin coating and spray technique was used to deposit the active layer, effectively absorbing solar radiation at various wavelengths. The surface properties of PSi etched at 30 s to 120 s revealed that the applied parameters (current densities, etching times, and HF concentrations) were optimum to generate the uniform micro-and mesoporous structures (Figure 2).

Figure 3a₁,b₁,c₁,d₁ depicts the FESEM images of PSi produced at different etching times with the corresponding reflection spectra (yellow curves). Both porosity and sizes of the pores were increased with the increase in etching times accompanied by the merging of neighboring nanopores, generating larger pores. Irrespective of the etching times, the diameters (D) of the mesopores were less than 50 nm. The total reflectance of PSi was inversely related to the etching time. The increase in the porosity of PSi with increasing etching time was responsible for the refractive index reduction. The reflection coefficient (R) of PSi was estimated using Fresnel’s equation [43]:

$$R={\left(\frac{{\mathrm{n}}_1-{\mathrm{n}}_2}{{\mathrm{n}}_1+{\mathrm{n}}_2}\right)}^2$$

(1)

where n₁ and n₂ are the refractive index of the incident and refracting medium, respectively. The sample etched at 120 s showed the weakest reflectance, maximum thickness, and widest bandgap (Figure 3d₁), which was due to the highest porosity in this sample [44]. Briefly, it was asserted that the pore sizes and porosity of PSi layers could be customized by controlling the etching current densities and etching times [45,46].

Figure 3a₂,b₂,c₂,d₂ presents the FESEM images (cross-section) of PSi films produced at various etching times together with their corresponding Tauc plots (yellow curves). The thickest PSi film was achieved for the longest etching time due to the enhanced chemical disintegration of the PSi layer grew in HF acid. At constant HF concentration and current density, the porosity of the PSi layer was increased with the increase in thickness at different etching times. However, the etching rate (ER) was unchanged [47]. This observation can be attributed to the chemical disintegration-assisted formation of several tiny channels below the PSi layer (marked by the green square in Figure 3a₂). The varying current density during the etching process allowed the formation of multi-layers of PSi with varying thicknesses. Figure 3e displays the time-dependent etching variation of versus bandgap energy and average thickness of PSi layers. Kubelka–Munk (K-M) function was used to calculate the values of Eg of the PSi films.

Figure 3a₃,b₃,c₃,d₃ shows the time-dependent etching variation of the AFM images of PSi which comprised of tiny needles on the surface. The number of needles was increased with the increase in etching time due to two reasons: improved porosity in PSi and increased chemical degradation rate, thus lowering the separation between the pores [48]. The roughness of PSi was proportional to the etching time [49] and this roughness can measure the distribution and deepness of the pores. In other words, the higher roughness suggested the higher number of pores on the sample surface [49,50]. The sample etched at 120 s showed the highest surface roughness and the maximum pores with varying morphologies in the corresponding FESEM and AFM images.

Figure 3f shows the time-dependent etching variation in the root mean square (RMS) roughness and percentage reflectance (R%) of PSi. The value of R was decreased, and RMS roughness was increased with the increase in etching time, indicating an increasing porosity of PSi. In turn, it caused an increase in the optical band gap energy and thickness of PSi, enabling the most extended channels for the light path length within the PSi layer [51].

Figure 3a₄ displays the etching time-dependent PL emission spectra of PSi. The sample etched at 30, 60, 90, and 120 s showed a broad emission spectral peak centered at 682, 662, 625 and 569 nm, respectively. Furthermore, the PL peak of PSi was shifted to a shorter wavelength (blue-shift) with the increase in etching time, which was mainly due to the quantum size effect called QCE. The value of Eg for PSi estimated from the peak value of PL spectra was 1.81, 1.87, 1.98, and 2.18 eV for samples etched at 30, 60, 90, and 120 s, respectively. Sample etched at 30, and 60 s showed the lowest and highest PL peak intensity. Indicated the low (weak QCE) and high (strong QCE) radiative recombination rate in PSi etched at 30 and 60 s, respectively. The observed increase in the PL peak intensity for PSi etched at 60, 90, and 120 s was due to the rise in the strong QCE-mediated radiative recombination rate (tinier nanocrystallites at longer etching time) [52]. Figure 3b₄ illustrates the etching time-dependent peak emission wavelength and RMS roughness of PSi. The PL spectra of PSi exhibited a strong blue shift and increased surface roughness with the increase in etching times which was majorly ascribed to the strong QCE of tiny nanocrystallites [53]. Based on these results, a correlation among etching time, RMS roughness and Eg of PSi can be established. It was inferred that with the increase in etching times, the number of the pores in PSi was increased, which enhanced the surface roughness, reduced the separation between the pores, and made the Si nanocrystallites tinier and widened the bandgap, thus causing a blue shift in the PL peak.

3.2. Effect of PSi on Cell’s Performance

It is vital to understand both the microscopic and electrical properties of solar cells to enhance their performance [54]. As aforementioned, the PSi derived from p-c-Si was utilized to improve the solar cell performance. First, the optimum parameters of PSi were determined to see their influence on the simple photovoltaic structure. Second, PSi was used as a substrate to deposit other layers such as TiO₂NPs and ZnSiQDs of various morphologies. Third, the I-V curve studied the electrical properties of PSi etched at 30, 60, 90, and 120 s to determine the optimum PSi and its feasibility of use to enhance the cell’s performance. The rear and top contact were made by depositing Al and Ag via the RF sputtering on the p-c-Si and PSi, respectively.

Figure 4a illustrates the current density versus applied voltage characteristics (J-V) of Al/c-Si/PSi/Ag. PSi etched at 90 s showed the best performance and thus was chosen to construct the solar cells. With the increase in etching times, the reflection from PSi was decreased, thus increasing the resistivity and decreasing the carriers’ concentration of PSi. Figure 4b depicts the etching time-dependent carriers concentration of PSi obtained from the Hall measurement. The carrier concentration of PSi was varied from 2.45 × 10¹³ cm⁻³ (at etching time of 30 s) to 7.32×10¹¹ cm⁻³ (at etching time of 300 s) as opposed to the high value of c-Si (2.99 × 10¹⁶ cm⁻³). The observed decrease in the carrier concentration of PSi with the increase in etching times was mainly due to the formation of the large number of pores at the defects and dopant sites of Si that could shift the Fermi level of PSi at the center of the energy bandgap [6,15,55].

Table 1. Performance of Al/p-c-Si/PSi/Ag photovoltaic cell at different etching times.

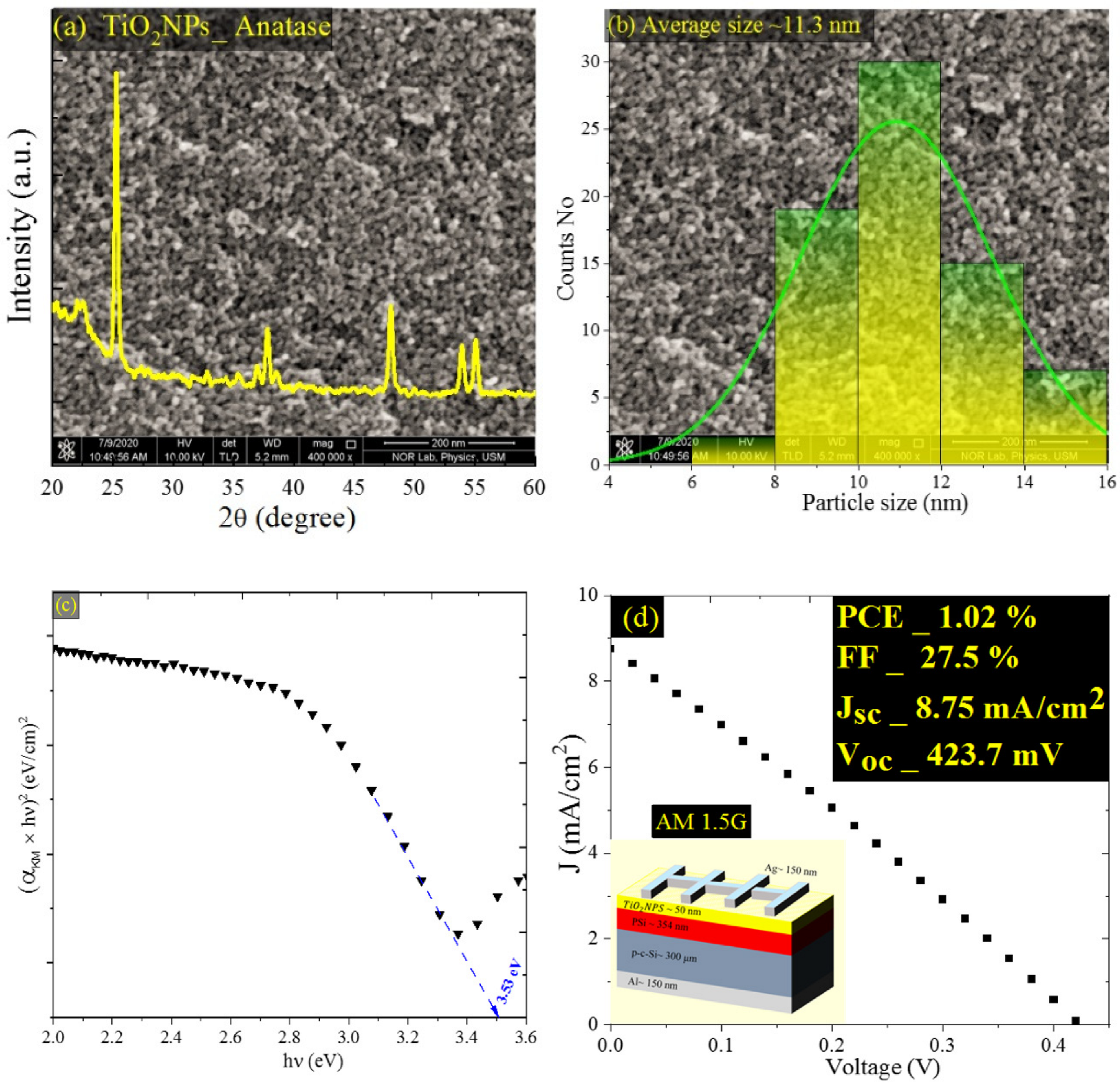
Etching Time (s)	JSC (mA/cm2)	VOC (V)	FF (%)	PCE or η (%)
0	3.6 × 10−3	0.54	61	1.19 × 10−3
30	6.41 × 10−3	0.85	50	2.74 × 10−3
60	6.37 × 10−3	0.98	64	4.05 × 10−3
90	1.54 × 10−2	0.88	54	7.39 × 10−3
120	2.59 × 10−3	0.48	47	5.92 × 10−4

presents the performance of PSi etched at various times (0 to 120 s) after incorporating into the photovoltaic device. The performance of PSi etched at 90 s was the optimum in terms of J_SC, V_OC, FF (%) and η (%). This was due to the lowest average reflectance and carrier recombination rate of PSi etched at 90 s, suggesting its potency as an antireflection layer. The shunt resistance (R_sh) was around 3.8 × 10⁶ Ω.cm² and ideally, it should be infinite in the optimum cell, thus restricting any alternative route for the flow of leakage current. Conversely, the series resistance (R_S) should be zero so that there is no loss of voltage [56].

3.3. Effect of TiO₂ NPs on Cell’s Performance

Figure 5a,b illustrates the FESEM images (top view) of TiO₂NPs-based film annealed at 500 °C together with their corresponding XRD pattern and particles size distribution fitted to Gaussian (yellow and green curves). XRD analysis confirmed the anatase TiO₂NPs as the primary phase. The estimated mean nanocrystallites size obtained from the intense XRD peak analyses via Debye-Scherrer relation was 12.5 nm. In addition, the average size of the TiO₂NPs obtained from the ImageJ software was 11.3 nm (Figure 5b), indicating the tally of these two estimates. The value of bandgap energy of TiO₂NPs obtained using the Kubelka–Munk plot was 3.53 eV (Figure 5c) which is very close to the theoretical value and other estimates in the works of literature.

Figure 5d shows the I-V characteristics of Al/p-c-Si/PSi/TiO₂NPs/Ag photovoltaic cells. Inset shows the cell’s layout, values of J_SC, V_OC, FF (%) and PCE or η (%). The PCE of the cell made using TiO₂NPs can be enhanced or quenched depending on the decrease or increase in carriers’ recombination rate. The recombination rate must be reduced to improve the solar cell’s efficiency. The existence of oxygen defects/vacancies that functioned as the trap levels across the bandgap region of PSi was the primary source of charge carrier recombination on the PSi surface. The deposition of TiO₂NPs (with the mean size of 11.3 nm) on top of the PSi layer resulted in surface passivation, thus lowering the oxygen vacancies by covering the surface and infiltrating into the and macro-pores (D > 50 nm) and mesopores (2 nm < D < 50 nm) [57,58] of the PSi layer. However, the TiO₂NPs with sizes close to PSi nanocrystallites could ease their infiltration into those pores. Hence, the improvement of PSi passivation enabled the reduction in the recombination rates of carriers through the oxygen vacancies. At the Ag and P-Si contact, the TiO₂NPs thin-film acted as a tunnelling layer for electrons. Therefore, the film containing TiO₂NPs of mean size 11.3 nm was chosen as the ideal electron transport layer (ETL) to coat PSi film for achieving the best photovoltaic performance in terms of PCE and FF (Figure 5d).

3.4. Effect of Deposited Single Layer of ZnSiQDs (SL-ZnSiQDs) on Cell’s Performance

Figure 6a₁,b₁,c₁ depict the cross-sectional view of the Al/p-c-Si/PSi/ZnSiQDs /TiO₂NPs/Ag (ZnSiQDs mean size of 7.4 nm), Al/p-c-Si/PSi/ZnSiQDs/TiO₂NPs/Ag (QDs mean size of 2.7 nm) and Al/p-c-Si/PSi/ZnSiQDs/TiO₂NPs/Ag (QDs mean size of 2.1 nm) solar cells, respectively. Films containing uniform ZnSiQDs were sandwiched between the PSi and TiO₂NPs layers to fabricate the proposed solar cells. Electrochemically etched PSi with optimum nanopores and electrical properties was selected to construct the solar cell, ensuring excellent contacts between the TiO₂NPs and ZnSiQDs junctions [35].

Figure 6a₂ shows the reflection spectra of p-c-Si/PSi, p-c-Si/PSi/ZnSiQDs (ZnSiQDs mean size of 7.4 nm), p-c-Si/PSi/ZnSiQDs (QDs mean size of 2.7 nm), p-c-Si/PSi/ZnSiQDs (QDs mean size of 2.1 nm) and p-c-Si/PSi/ZnSiQDs/TiO₂NPs. The average reflection of PSi before the deposition of layers (SL-ZnSiQDs, and TiO₂NPs) was lower than after the deposition (Figure 6a₂), which was due to the filling of pores due to deposition, making the surface more homogeneous and smoother. Figure 6b₂ shows the AFM image of p-c-Si/PSi/ZnSiQDs/TiO₂NPs (RMS roughness of 0.859 nm). The RMS roughness of PSi was reduced by 23% after the single layer of ZnSiQDs (2.1 nm). The deposition reduced the number of pores and made them tinier than without deposition. In turn, it yielded a high coverage area of NPs on the PSi surface, thus improving the surface morphology and making it comparatively regular [59].

Figure 7 compares the photovoltaic performance of the cells made of Al/p-c-Si/PSi/Ag, Al/p-c-Si/PSi/ TiO₂NPs/Ag, Al/p-c-Si/PSi/ZnSiQDs/TiO₂NPs/Ag (ZnSiQDs mean size of 7.4 nm), Al/p-c-Si/PSi/ZnSiQDs/TiO₂NPs/Ag (QDs mean size of 2.7 nm) and Al/p-c-Si/PSi/ZnSiQDs/TiO₂NPs/Ag (QDs mean size of 2.1 nm). The values of PCE, FF, and I-V properties of the GBQDSCs were much higher compared to those designed without ZnSiQDs. Compared to the PCE value of the reference cell (Al/p-c-Si/PSi/Ag), the PCE value of the GBQDSCs made from ZnSiQDs layer of average QDs size of 2.1 nm, 2.7 nm, and 7.4 nm was 2.17%, 1.88% and 1.28%, respectively. This indicated an enhancement of PCE by approximately 114% than the reference cell (

Table 2. Photovoltaic performance comparison of the cells Al/p-c-Si/PSi/ TiO₂NPs/Ag, Al/p-c-Si/PSi/ZnSiQDs/TiO₂NPs/Ag (ZnSiQDs mean size of 7.4 nm), Al/p-c-Si/PSi/ZnSiQDs/TiO₂NPs/Ag (QDs mean size of 2.7 nm) and Al/p-c-Si/PSi/ZnSiQDs/TiO₂NPs/Ag (QDs mean size of 2.1 nm).

Solar Cell Structure	Jsc (mA/cm2)	Voc (V)	FF (%)	PCE (%)
Al/p-c-Si/PSi/TiO2NPs/Ag	8.75	423.7	27.5	1.02
Al/p-c-Si/PSi/ZnSiQDs (7.4 nm)/TiO2NPs/Ag	4.49	695	41.03	1.28
Al/p-c-Si/PSi/ZnSiQDs (2.7 nm)/TiO2NPs/Ag	4.52	801.1	51.88	1.88
Al/p-c-Si/PSi/ZnSiQDs (2.1 nm)/TiO2NPs/Ag	4.28	789.6	64.11	2.17

). The cell designed with ZnSiQDs and TiO₂NPs layers had a lower short circuit current (J_SC) than the cell with TiO₂NPs only. It can be argued that solar radiation with higher energy was successfully absorbed by ZnSiQDs and then transmitted to the underneath p-c-Si layer via the non-radiative energy transfer (NRET) mechanism. In addition, TiO₂NPs and PSi have effectively segregated the produced carriers’ in the p-c-Si region, thus decreasing the value of J_SC, increasing V_OC, FF and PCE values with the decrease in QDs mean size. Briefly, the capacity of ZnSiQDs to serve as a hydrophilic layer by modifying the electronic band structures, gaining higher coverage of TiO₂NPs, minimizing the series resistance and improving both FF and PCE was verified [35].

Table 2 shows the comparative performance evaluation of the cells Al/p-c-Si/PSi/ TiO₂NPs/Ag, Al/p-c-Si/PSi/ZnSiQDs/TiO₂NPs/Ag (ZnSiQDs mean size of 7.4 nm), Al/p-c-Si/PSi/ZnSiQDs/TiO₂NPs/Ag (QDs mean size of 2.7 nm) and Al/p-c-Si/PSi/ZnSiQDs/TiO₂NPs/Ag (QDs mean size of 2.1 nm). It is worth noting that the solar cell performance is heavily reliant on the collection efficiency of the photo-generated carriers [60] and the size of ZnSiQDs. Thus, the ZnSiQDs layer that contained the tiniest QDs produced the highest efficiency. In this work, the thickness of ZnSiQDs was fixed for all samples; only the QDs sizes were customized to enhance the cells’ efficiency. It was further assumed that the concentration of colloidal ZnSiQDs in the solvent were almost the same. However, the solvent volume with higher volume enclosed a larger number of ZnSiQDs (smaller sizes) than the one with lower volume (larger sizes of ZnSiQDs). Volumes of 5, 3.9, and 1.4 mL were used to obtain the corresponding QDs sizes of 2.1, 2.7, and 7.4 nm, wherein such volumes were estimated from the smallest particle size to another size ratio. Because of the weak interface recombination, the cell containing ZnSiQDs had the highest performance [61]. The lowest solar cell performance of the cell was achieved for ZnSiQDs largest sizes that were utilized as an active layer. This was mainly due to the reduction in the electric fields that administered the carriers extraction, resulting in poor charge carrier transit in the cell, thus lowering the current and hampered charge recombination [62].

3.5. Effect of Deposited ZnSiQDs Double and Triple Layers (DL-ZnSiQDs and TL-ZnSiQDs) on Cell’s Performance

Figure 8a₁–c₁ show the proposed GBQDSCs comprised of DL-ZnSiQDs (double layers of ZnSiQDs). These layers were deposited in the order of Al/p-c-Si/PSi/ZnSiQDs (7.4 nm)/ZnSiQDs (2.7 nm)/TiO₂NPs/Ag, Al/p-c-Si/PSi/ZnSiQDs (7.4 nm)/ZnSiQDs (2.1 nm)/TiO₂NPs/Ag, and Al/p-c-Si/PSi/ZnSiQDs (2.7 nm)/ZnSiQDs (2.1 nm)/TiO₂NPs/Ag. Figure 8d₁,e₁ shows the GBQDSCs consisted of TL-ZnSiQDs (triple layers of ZnSiQDs) wherein the structure of TL-ZnSiQDs was Al/p-c-Si/PSi/ZnSiQDs (7.4 nm)/ZnSiQDs (2.7 nm)/ZnSiQDs (2.1 nm)/TiO₂NPs/Ag with and without TiO₂NPs, respectively.

Figure 8a₂ shows the cross-section FESEM image of the p-c-Si/ PSi/TL-ZnSiQDs/TiO₂NPs cell with various dimensions (yellow). Figure 8b₂ shows the top view of PSi and pores size distribution (histogram) with Gaussian fit (blue curve). Layer containing ZnSiQDs of mean size 7.4 nm was the first layer deposited on the PSi layer so that ZnSiQDs can penetrate the pores having a mean diameter of 12.4 nm (Figure 8b₂). The layer containing ZnSiQDs of mean size 7.4 nm cannot be seen because it was incorporated with PSi layer. The structure of the solar cell that contained the (SL-DL-TL)-ZnSiQDs was comparable to the rainbow or tandem solar cell architecture. It consisted of sub-cell wherein each one of these contains a particular active layer of quantum dot materials with a particular bandgap. The PCE values of the tandem solar cells depend on the number of sub-cells [62]. Many reports indicated that the intermediate layer, such as Gr [63], Au [62,64], PEDOT:PSS [62] was used to connect different sub-cells. In the current study, the GBQDSCs did not contain an intermediate layer.

Figure 8a₃,b₃ show the performance of the solar cell designed with DL-ZnSiQDs and TL-ZnSiQDs. Each layer absorbed a particular wavelength from the solar spectra, enhancing the production of the photo-generated carriers. Additionally, utilizing the graded bandgap of ZnSiQDs led to the enhancement of the extraction of the photo-generated carriers, hence improving the solar cell performance [62]. All parameters of the solar cell incorporated with DL-ZnSiQDs was enhanced compared to the one designed with SL-ZnSiQDs wherein the obtained values of J_SC, V_OC, fill factor, and efficiency showed an improvement by 2.6%, 39.4%, 11.3%, and 52.1%, respectively. Conversely, the values of J_SC, V_OC, FF and PCE for the solar cell designed with TL-ZnSiQDs showed an enhancement of 843.9%, −44%, −57.1%, and 116%, respectively.

Figure 8a₄,b₄ illustrates the reflectance spectra of the cell designed with DL-ZnSiQDs and TL-ZnSiQDs. The average reflection of SL-ZnSiQDs, DL-ZnSiQDs, and TL-ZnSiQDs was 22.3%, 19.7%, and 17.5%, respectively. The value of J_SC in DL-ZnSiQDs was increased slower than that of SL-ZnSiQDs, which was due to the higher recombination rate at the interface for the former one than the latter cell.

Figure 9a₁ displays the mechanism of electron tunnelling and carrier production-recombination across the energy band gap of a typical solar cell in band structure with a graded band alignment. Because the Hall concentration dropped to ~10¹¹ cm⁻³, the PSi layer after etching became an intrinsic layer with the Fermi level positioned at the middle of the energy bandgap [15,55]. The difference in the CB energy (ΔEc) between Si and TiO₂ was minimal, allowing the photo-generated electrons to be extracted in the ETL [65]. On the other hand, the VB energy difference (ΔEv) was very high (~2.15 eV), preventing the holes from being extracted at the Si-TiO₂ interface [66]. The work functions (Ф) of Ag and Al is 4.28 eV and 4.26 eV, respectively. The CB and VB were shifted increasingly with the continual QDs size reduction [62]. In the case of bulk Si and TiO₂, the difference in the CB energy is very small. However, when the size becomes comparable to the exciton Bohr radius, the energy bandgap becomes wider, shifting both CB and VB energy to a higher value. All active layers of the GBQDSCs containing ZnSiQDs of sizes of 2.1, 2.7 and 7.4 nm could successfully absorb various wavelengths from the solar spectrum. After absorbing the suitable solar radiation, the layers with bandgap energy values of 3.3, 2.5, and 2.3 eV could generate numerous electron-hole (e–h) pairs, including e₁–h₁, e₂–h₂, and e₃–h₃, respectively. Later, these electrons and holes moved in opposite directions. The energy alignment of the CB and VB between different layers allowed the transfer of these carriers from layer to layer before being eventually collected in electrodes, thus improving the photocurrent in the cell and efficiency. It was affirmed that the utilization of the graded bandgap could enhance both the extraction of the charge carriers and diffusion length because of the increasing electric fields provided by the graded structures in the cell [5,62,67].

Table 3. Performance parameters comparison of the studied solar cells.

Solar Cell Structure	PCE (%)	FF (%)	JSC (mA/cm2)	VOC (mV)
Aluminum/p-c-Si/PSi/Silver	0.0074	54.52	0.0154	879.6
Al/p-c-Si/PSi/TiO2NP/Ag	1.02	27.5	8.75	423.7
Al/p-c-Si/PSi/ZnSiQDs (7.4 nm)/TiO2NP/Ag	1.28	41.03	4.49	695
Al/p-c-Si/PSi/ZnSiQDs (2.7 nm)/TiO2NP/Ag	1.88	51.88	4.52	801.1
Al/p-c-Si/PSi/ZnSiQDs (2.1 nm)/TiO2NP/Ag	2.17	64.11	4.28	789.6
Al/p-c-Si/PSi/ZnSiQDs(7.4 nm)/ZnSiQDs(2.7 nm)/TiO2NPs/Ag	3.19	71.35	4.55	983.32
Al/p-c-Si/PSi/ZnSiQDs(7.4 nm)/ZnSiQDs(2.1 nm)/TiO2NPs/Ag	3.23	64.34	4.64	1079.7
Al/p-c-Si/PSi/ZnSiQDs(2.7 nm)/ZnSiQDs(2.1 nm)/TiO2NPs/Ag	3.3	64.48	4.58	1116.93
Al/p-c-Si/PSi/ZnSiQDs(7.4 nm)/ZnSiQDs(2.7 nm)/ZnSiQDs(2.1 nm)/Ag	4.4	32.6	38.6	351.9
Al/p-c-Si/PSi/ZnSiQDs(7.4 nm)/ZnSiQDs(2.7 nm)/ZnSiQDs(2.1 nm)/TiO2NPs/Ag	4.9	27.6	40.4	440.8

summarizes various performance parameters of the designed solar cells. PCE was increased from 0.0074% for PSi as an active layer to 4.9% for TL-ZnSiQDs, corresponding to the PCE enhancement of 661.2 times.

Figure 9a₂ shows the mechanism of the inserted Zn⁺² responsible for enhancing the electron extraction by reducing the recombination rate. The deleted path of the recombination route was mentioned with a dashed line with the delete signal. The maximum efficiency for SL, DL and TL were at the smallest size of the ZnSiQDs. However, the production of Zn-O and Si-O-Zn can demonstrate that Zn⁺² ions were successfully doped into the inner SiQDs layer and that the SiQDs layer was fully coated [59].

Figure 9a₃ displays that depositing the layers in reverse led to a sharply reduced J_SC due to the loss of alignment of the energy levels and consequently lowering efficiency. The PCE and J_SC values were correspondingly reduced to 0.42% and 2.8 mA/cm². Figure 9a₄ shows the direction of electron transfer was reversed, and the PSi served as a blocking layer to extract the holes.

Table 4. Comparison of the performance of the proposed cells with other state-of-the-art literature reports.

QD	VOC (V)	JSC (mA/cm2)	FF (%)	η (%)	Ref.
M-ZnS/CdSeS	0.6	17.57	49	5.15	[59]
CdS/CdSe/ZnS	0.614	15.37	45.1	4.25	[68]
CdS	0.69	3.93	62.5	1.73	[69]
ZnS/CdSe/ZnS	0.559	14.8	49.45	4.34	[70]
ZnPh	0.54	18.03	46.22	4.5	[71]
CdS/CdSe/ZnS	0.56	17.2	44	4.26	[72]
CdS/CdSe	0.53	11.86	34	2.15	[73]
CdS/CdSe	0.59	17.18	55	5.59	[74]
CdSe	0.636	16	54	5.5	[75]
CuSe/CdSe	0.55	17.29	51	4.83	[76]
N-G/CdSe	0.48	17.78	51.88	4.88	[77]
Bi2S3	0.21	21.37	37	1.63	[24]
CdSe/ZnO	0.48	15.7	37.4	2.82	[78]
TL-ZnSiQDs	0.44	40.4	27.6	4.9	Present

compares the performance of the proposed cell with other state-of-the-art literature reports.

4. Conclusions

For the first time, we report the design, characterization, and performance evaluation of GBQDSCs made from ZnSiQDs as an active layer. The values of Eg for ZnSiQDs of different sizes were 2.3, 2.5, and 3.3 eV, PSi was 2.2 eV, and TiO₂NPs was 3.6 eV. The effect of various Eg values on the energy level alignment of the different layers and the performance of the solar cells were examined. The TL-ZnSiQDs structure consisted of three sizes with other Eg, where each size of ZnSiQDs absorbed a particular wavelength. Thus, using such an active ZnSiQDs layer, the solar cell collectively absorbed a broad wavelength region from the solar spectra and acted like a rainbow quantum dot solar cell. The energy level alignment between the different sizes of QDs improved the electron injection and J_SC by minimizing the thermal (non-radiative) loss. As a result, the proposed cells demonstrated excellent performance. However, for any energy level misalignment, the electrons and holes injection became inhibited, reducing the J_SC value minimal and producing the lowest PCE. It was shown that by properly aligning the energy levels of various semiconducting layers; it is possible to customize the efficiency of the GBQDSCs.