Study of the Effect of Nematic Order Degradation in Liquid Crystal-Based Surface Plasmon Resonance Sensors
Abstract
:1. Introduction
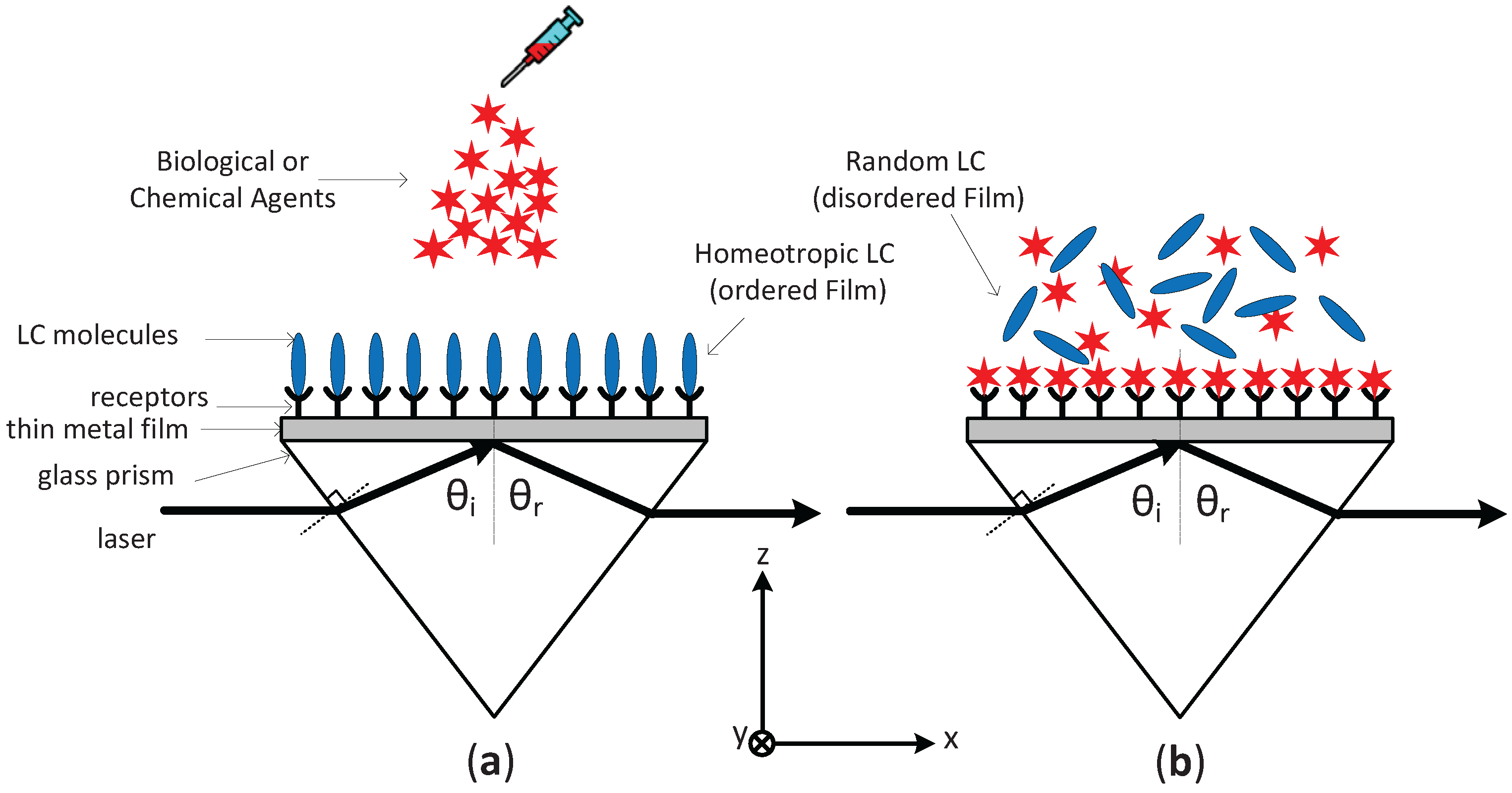
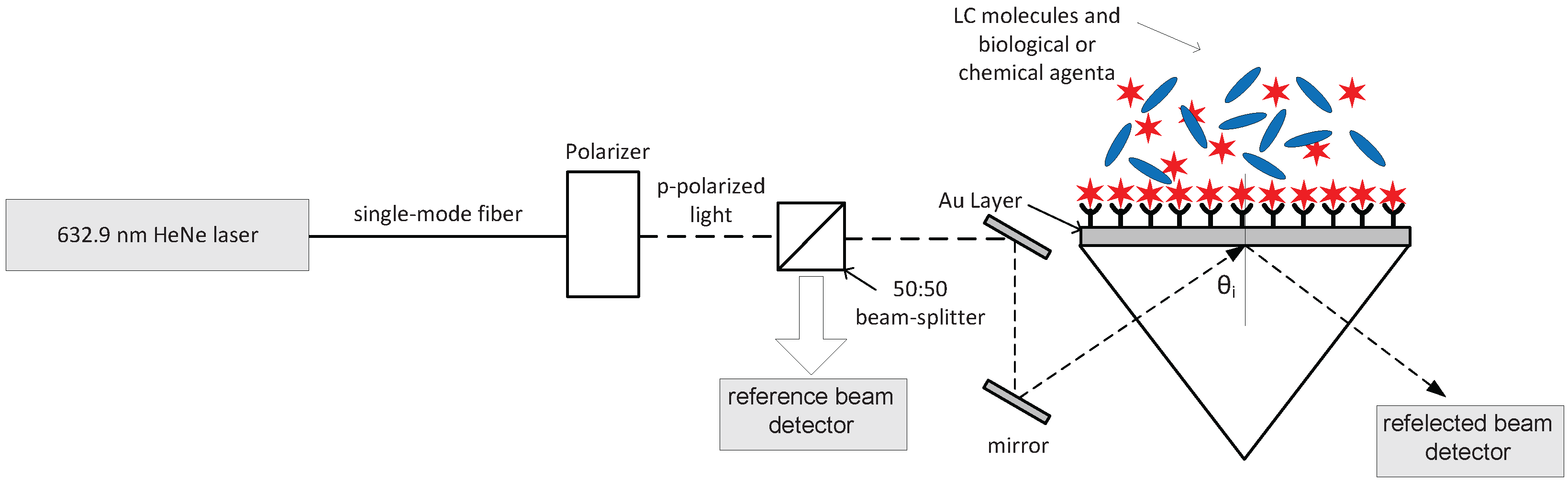
2. SPR-LC Sensor Fundamentals and Analysis
2.1. The Fundamentals
2.2. The Analysis
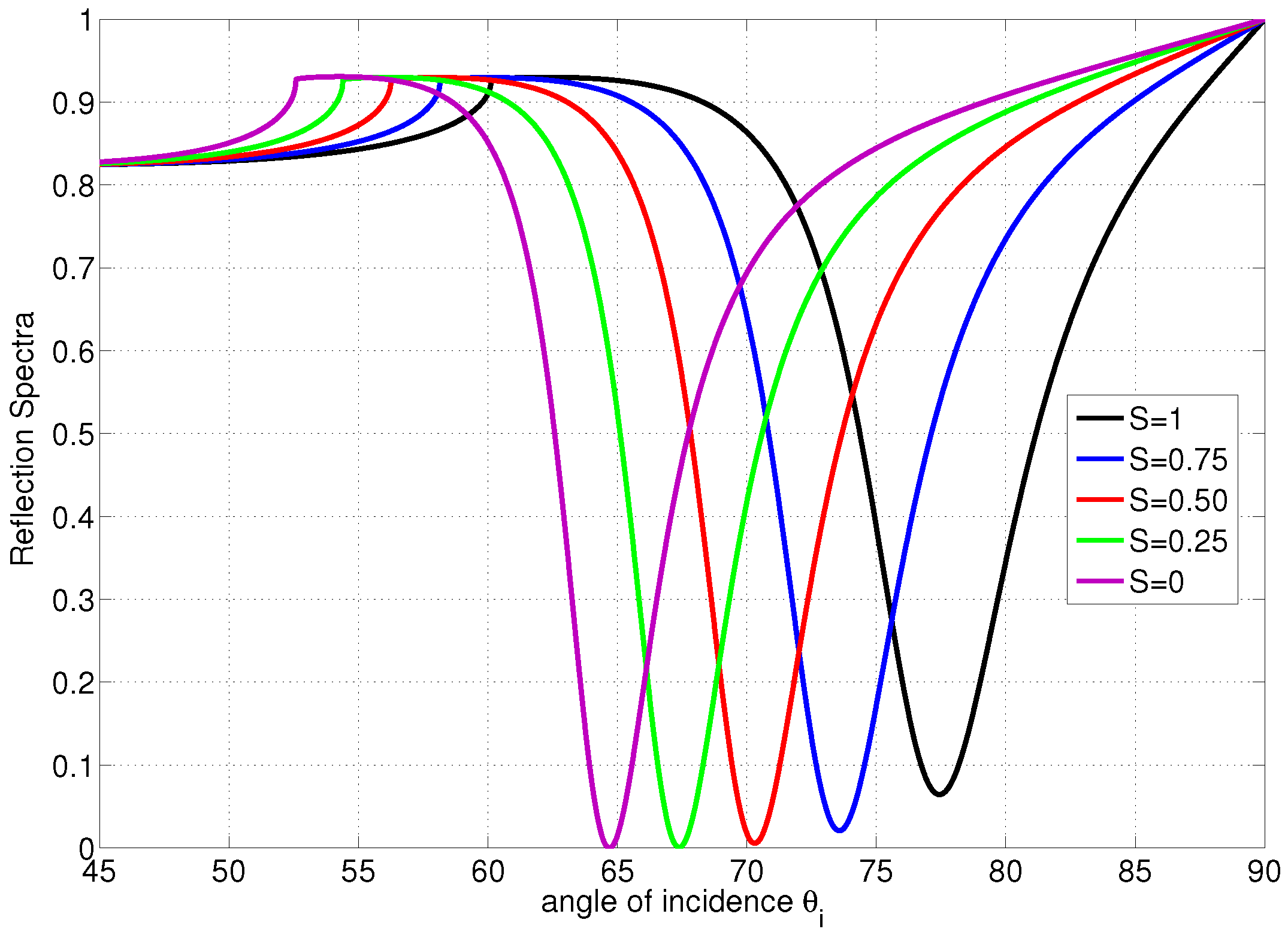
3. Simulation Results
4. Conclusions
Conflicts of Interest
References
- Brake, J.; Daschner, M.; Abbott, N.L. Biomolecular interactions at phospholipid decorated surfaces of thermotropic liquid crystals. Science 2003, 302, 2094–2097. [Google Scholar] [CrossRef] [PubMed]
- Liang, B.-J.; Hsu, J.-S.; Hsu, P.-F.; Liu, D.-G. Electrostatic Discharge Sensor Based on Brightness Retention of Liquid Crystals. IEEE Sens. J. 2016, 4, 918–923. [Google Scholar] [CrossRef]
- Ferhat, M.; Hameed, O.; Azab, Y.; Heikal, A.M.; El-Hefnawy, S.M.; Obayya, S.S.A. Highly Sensitive Plasmonic Photonic Crystal Temperature Sensor Filled With Liquid Crystal. IEEE Photonics Technol. Lett. 2016, 28, 59–62. [Google Scholar] [CrossRef]
- Feng, J.; Zhao, Y.; Li, S.-S.; Lin, X.-W.; Lu, Y.-Q. Fiber-Optic Pressure Sensor Based on Tunable Liquid Crystal Technology. IEEE Photonics J. 2010, 3, 292–298. [Google Scholar] [CrossRef]
- Abu-Abed, A.S.; Lindquist, R.G. Capacitive transduction for liquid crystal based sensors, part II: Partially disordered systems. IEEE Sens. J. 2008, 8, 1557–1564. [Google Scholar] [CrossRef]
- Carlton, R.J.; Hunter, J.T.; Miller, D.S.; Abbasi, R.; Mushenheim, P.C.; Tan, L.N.; Abbott, N.L. Chemical and biological sensing using liquid crystals. Liq. Cryst. Rev. 2013, 1, 29–51. [Google Scholar] [CrossRef] [PubMed]
- Sridharamurthy, S.; Cadwell, K.; Abbott, N.L.; Jiang, H. A Liquid crystal based gas sensor using microfabricated pillar arrays as a support structure. In Proceedings of the IEEE Sensors Conference, Atlanta, GA, USA, 28–31 October 2007; pp. 1044–1047. [Google Scholar]
- Shah, R.; Abbott, N.L. Principles for measurment of chemical exposure based on recognition-driven anchoring transitions in liquid crystals. Science 2001, 293, 1296–1299. [Google Scholar] [CrossRef] [PubMed]
- Raether, H. Surface Plasmons on Smooth and Rough Surfaces and on Gratings; Springer: New York, NY, USA, 1988. [Google Scholar]
- Srid, D.; Challene, W. Modern Introduction to Surface Plasmons: Theory, Mathematica Modeling, and Applications; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Liu, Y.; Liu, X.; Chen, S.; Liu, Q.; Peng, W. Investigation of a Capillary-Based Surface Plasmon Resonance Sensor for Biosensing. J. Lightwave Technol. 2016, 17, 4036–4042. [Google Scholar] [CrossRef]
- Shalaev, V.M.; Kawata, S. Nanophotonics with Surface Plasmons; Elsevier Science: New York, NY, USA, 2007. [Google Scholar]
- Byun, K.M.; Kim, D.; Kim, S.J. Investigation of the profile effect on the sensitivity enhancement of nanowire-mediated localized surface plasmon resonance biosensors. Sens. Actuators B Chem. 2007, 117, 401–407. [Google Scholar] [CrossRef]
- Chinowsky, T.M.; Quinn, J.G.; Bartholomew, D.; Kaiser, R.; Elkind, J. Performance of the spreeta 2000 integrated surface plasmon resonance affinity sensor. Sens. Actuators B Chem. 2003, 91, 266–274. [Google Scholar] [CrossRef]
- Hodnik, V.; Anderluh, G. Toxin detection by surface plasmon resonance. Sensors 2009, 9, 1339–1354. [Google Scholar] [CrossRef] [PubMed]
- Ruan, L.; Yang, F.; Sambles, J.R. Otto-coupled surface plasmons in a liquid crystal cell. Appl. Phys. Lett. 2009, 95, 171102. [Google Scholar] [CrossRef]
- Lu, M.; Zhang, X.; Liang, Y.; Li, L.; Masson, J.-F.; Peng, W. Liquid crystal filled surface plasmon resonance thermometer. Opt. Express 2016, 10, 10904–10911. [Google Scholar] [CrossRef] [PubMed]
- Kieser, B.; Pauluth, D.; Gauglitz, G. Nematic liquid crystals as sensitive layers for surface plasmon resonance sensors. Anal. Chim. Acta 2001, 434, 231–237. [Google Scholar] [CrossRef]
- De Gennes, P.G.; Prost, J. The Physics of Liquid Crystals; Oxford Science: Oxford, UK, 1993. [Google Scholar]
- Abu-Abed, A.S. Optical waves in partially ordered anisotropic media. Opt. Express 2009, 17, 1646–1651. [Google Scholar] [CrossRef] [PubMed]
- Buka, A.; de Jeu, W.H. Diamagnetism and orientational order of nematic liquid crystals. J. Phys. Fr. 1982, 43, 361–367. [Google Scholar] [CrossRef]
- Jun, N.; Zou, Y.; Abu-Abed, A.S.; Lindquist, R.G. Capacitive Techniques to Monitor of Anchoring Energy for Liquid Crystal Sensors. IEEE Sens. J. 2009, 10, 1479–1485. [Google Scholar]


© 2017 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abuabed, A.S.A. Study of the Effect of Nematic Order Degradation in Liquid Crystal-Based Surface Plasmon Resonance Sensors. Photonics 2017, 4, 24. https://doi.org/10.3390/photonics4020024
Abuabed ASA. Study of the Effect of Nematic Order Degradation in Liquid Crystal-Based Surface Plasmon Resonance Sensors. Photonics. 2017; 4(2):24. https://doi.org/10.3390/photonics4020024
Chicago/Turabian StyleAbuabed, Alaeddin S. A. 2017. "Study of the Effect of Nematic Order Degradation in Liquid Crystal-Based Surface Plasmon Resonance Sensors" Photonics 4, no. 2: 24. https://doi.org/10.3390/photonics4020024
APA StyleAbuabed, A. S. A. (2017). Study of the Effect of Nematic Order Degradation in Liquid Crystal-Based Surface Plasmon Resonance Sensors. Photonics, 4(2), 24. https://doi.org/10.3390/photonics4020024




