A Detailed Study of a Resonant-Assisted Segmented Cladding Fiber for Large Mode Area Applications
Abstract
1. Introduction
2. Analysis Method and Fiber Structure
3. Results and Discussion
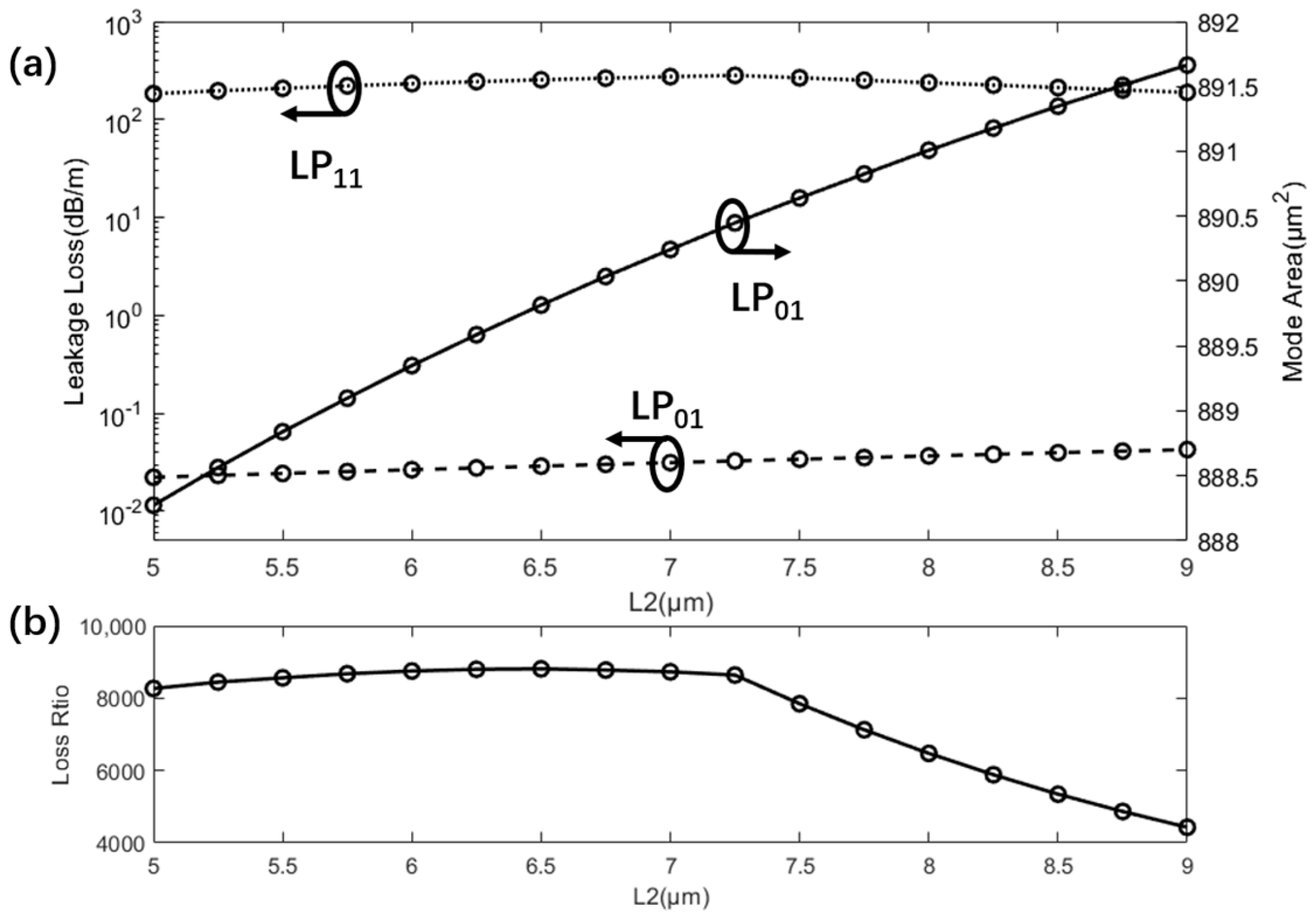
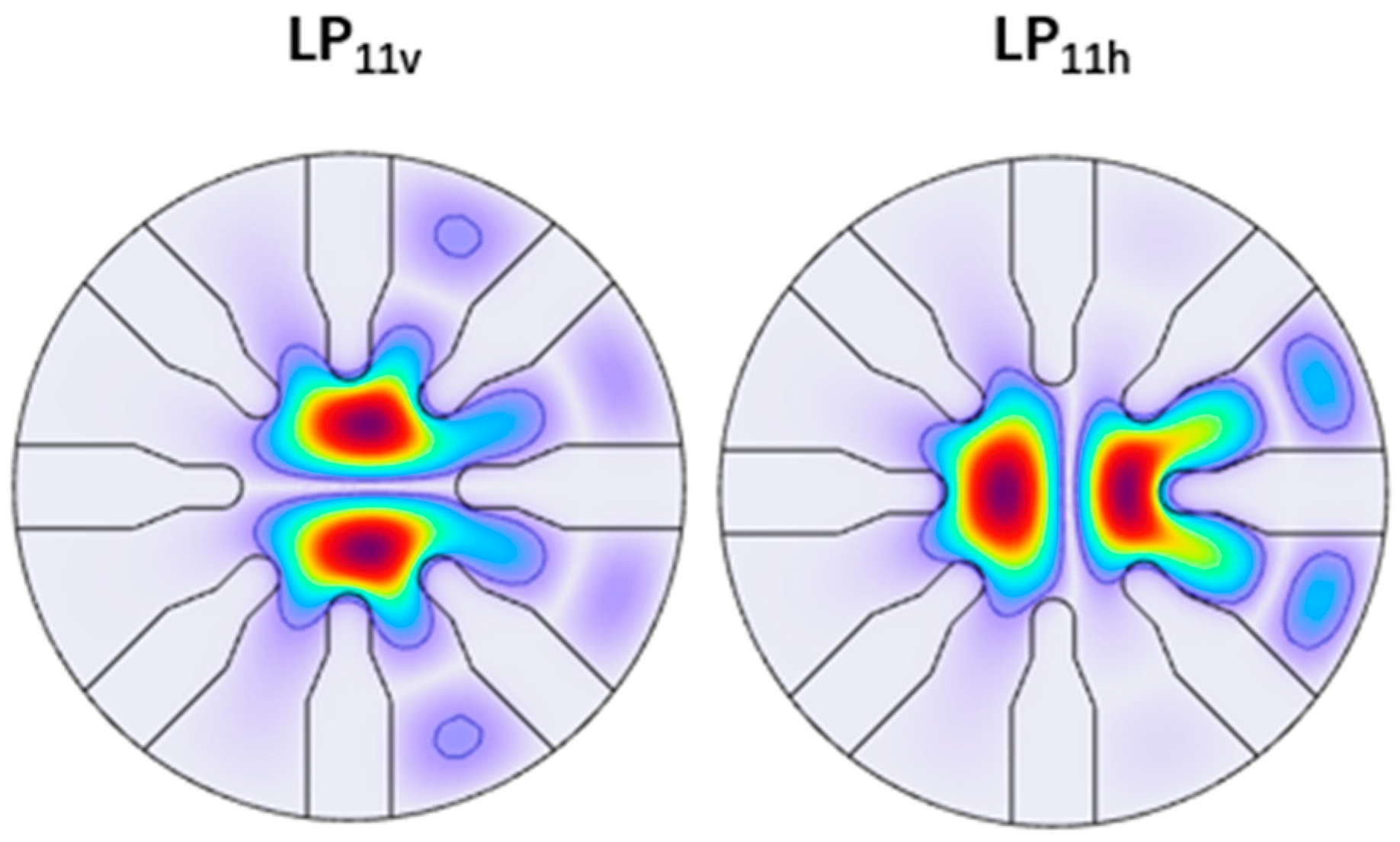
3.1. Properties of the RA-SCF
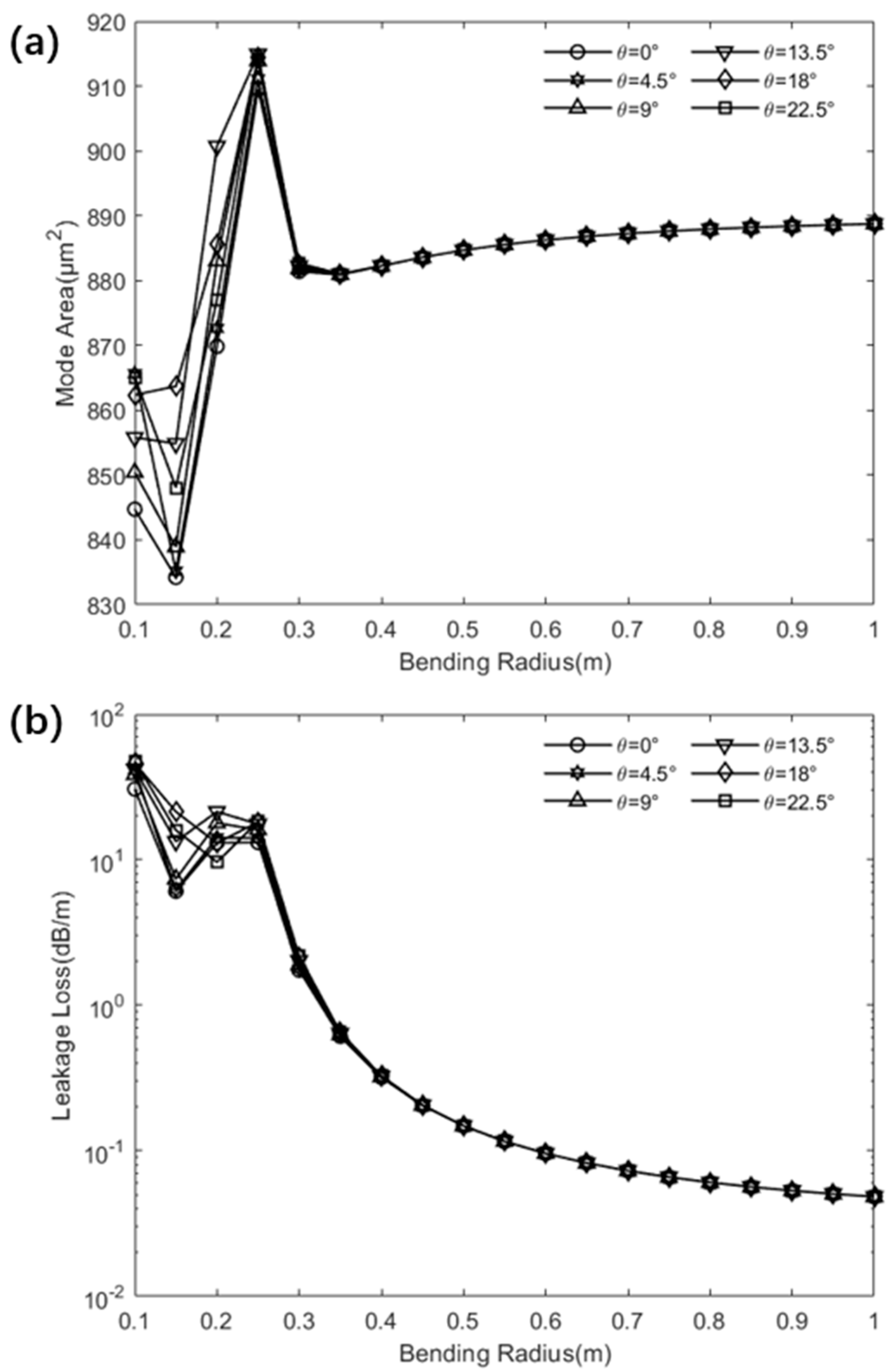
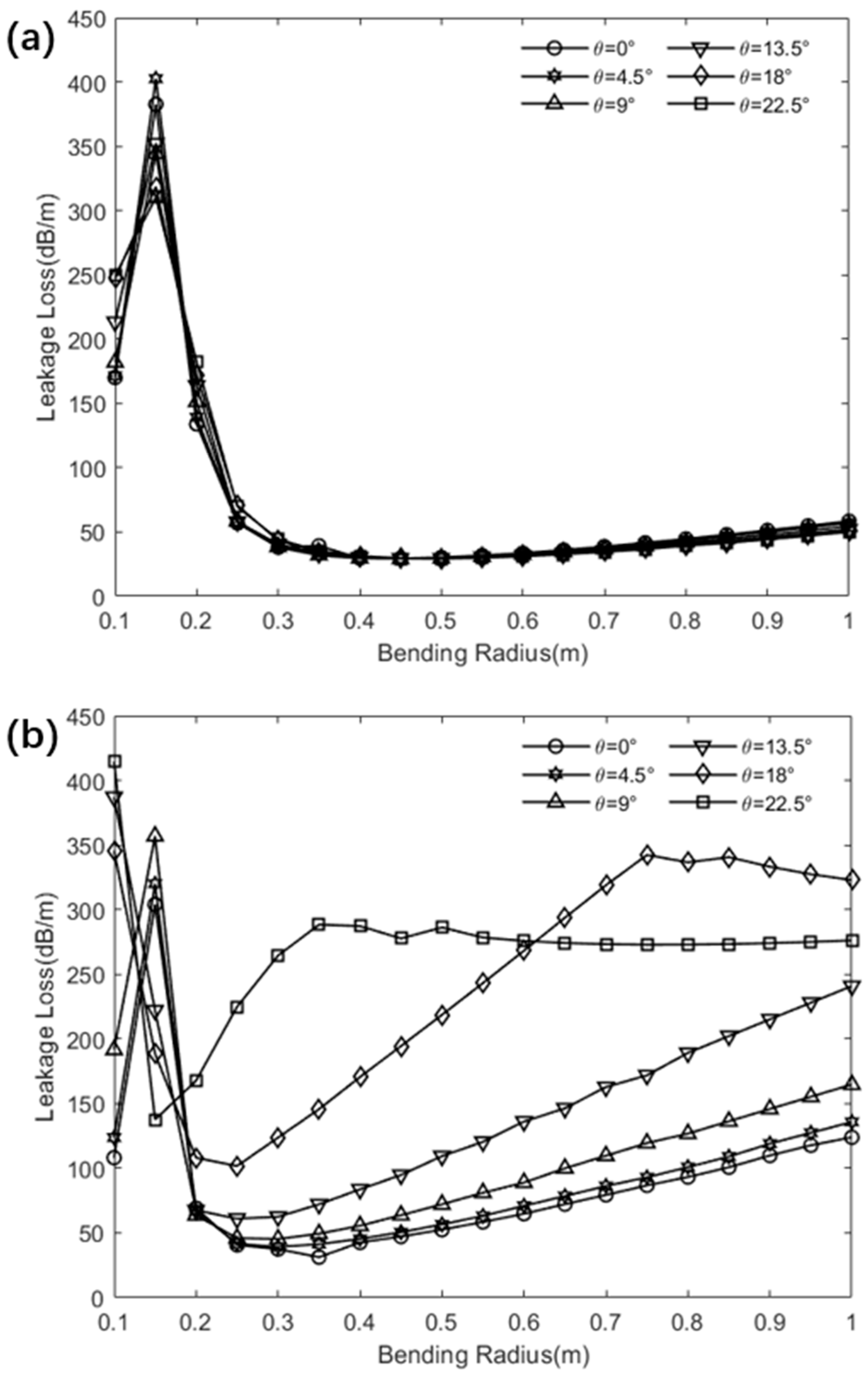
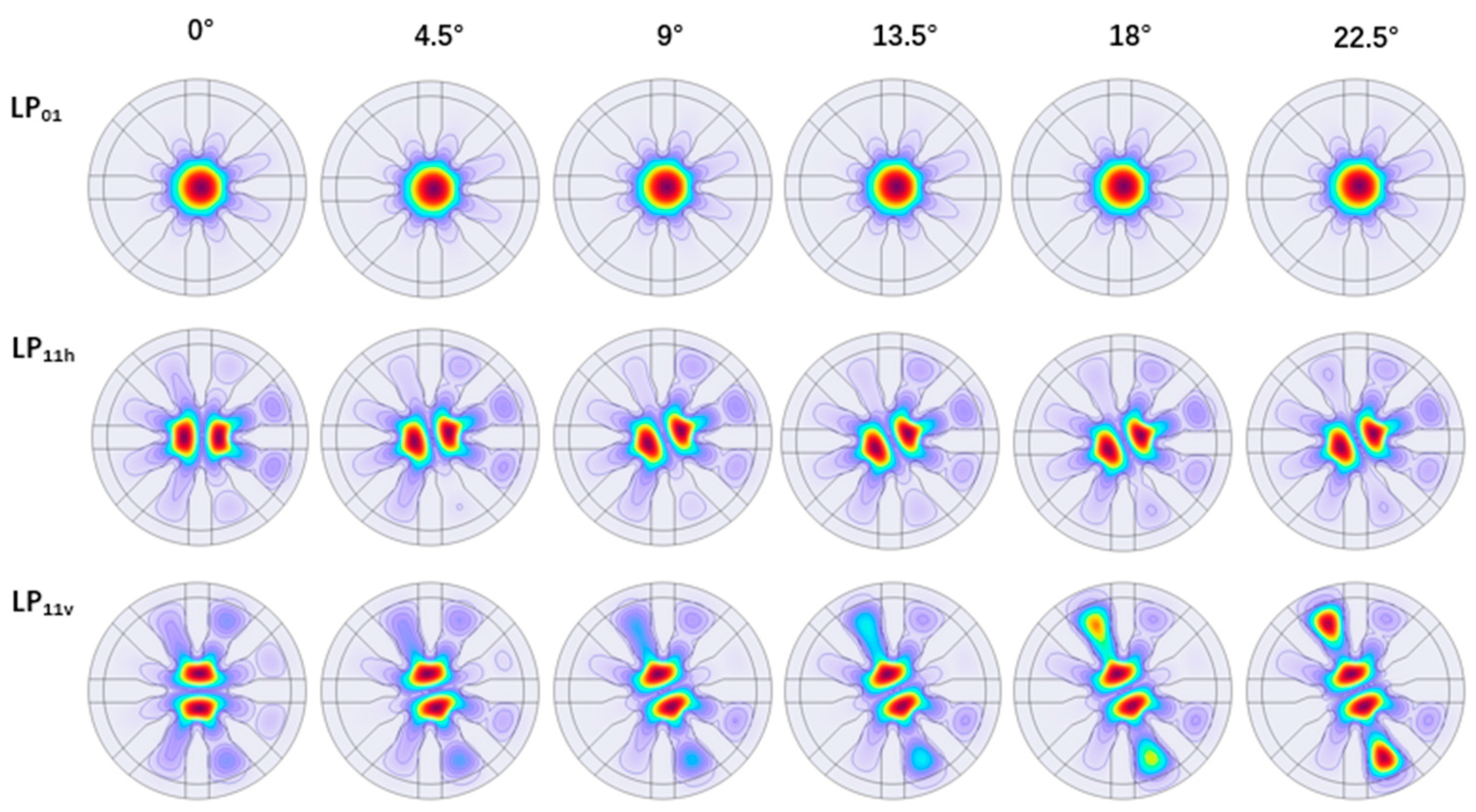
3.2. Bending Performance of the RA-SCF
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Snitzer, E. Optical maser action of Nd+3 in a barium crown glass. Phys. Rev. Lett. 1961, 7, 444–446. [Google Scholar] [CrossRef]
- Coffey, V. High-energy lasers: New advances in defense applications. Opt. Photonics News 2014, 25, 28–35. [Google Scholar] [CrossRef]
- Shi, W.; Schulzgen, A.; Amezcua, R.; Zhu, X.; Alam, S.-U. Fiber lasers and their applications: Introduction. J. Opt. Soc. Am. B 2017, 34, FLA1. [Google Scholar] [CrossRef]
- Shiner, B. The impact of fiber laser technology on the world wide material processing market. In Proceedings of the Conference on Lasers and Electro-Optics 2013, San Jose, CA, USA, 9–14 June 2013; Optical Society of America: Washington, DC, USA; p. AF2J.1. [Google Scholar]
- Dawson, J.W.; Messerly, M.J.; Beach, R.J.; Shverdin, M.Y.; Stappaerts, E.A.; Sridharan, A.K.; Pax, P.H.; Heebner, J.E.; Siders, C.W.; Barty, C. Analysis of the scalability of diffraction-limited fiber lasers and amplifiers to high average power. Opt. Express 2008, 16, 13240–13266. [Google Scholar] [CrossRef]
- Smith, A.V.; Smith, J.J. Mode instability in high power fiber amplifiers. Opt. Express 2011, 19, 10180–10192. [Google Scholar] [CrossRef] [PubMed]
- Jain, D.; Jung, Y.; Barua, P.; Alam, S.; Sahu, J.K. Demonstration of ultra-low NA rare-earth doped step index fiber for applications in high power fiber lasers. Opt. Express 2015, 23, 7407–7415. [Google Scholar] [CrossRef] [PubMed]
- Petit, V.; Tumminelli, R.P.; Minelly, J.D.; Khitrov, V. Extremely low NA Yb doped preforms (<0.03) fabricated by MCVD. In Fiber Lasers XIII: Technology, Systems, and Applications; SPIE: Bellingham, WA, USA, 2016; Volume 9728. [Google Scholar]
- Wang, S.; Xu, W.; Wang, F.; Lou, F.; Zhang, L.; Zhou, Q.; Chen, D.; Feng, S.; Wang, M.; Yu, C.; et al. Yb3+-doped silica glass rod with high optical quality and low optical attenuation prepared by modified sol-gel technology for large mode area fiber. Opt. Mater. Express 2017, 7, 2012–2022. [Google Scholar] [CrossRef]
- Knight, J.C.; Birks, T.A.; Cregan, R.F.; Russell, P.S.J.; De Sandro, J.P. Large Mode Area Photonic Crystal Fiber. Opt. Photonics News 1998, 9, 34–35. [Google Scholar] [CrossRef]
- Jain, D.; Baskiotis, C.; Sahu, J.K. Mode area scaling with multi-trench rod-type fibers. Opt. Express 2013, 21, 1448–1455. [Google Scholar] [CrossRef]
- Dong, L.; Peng, X.; Li, J. Leakage channel optical fibers with large effective area. Opt. Soc. Am. B 2007, 24, 1689–1697. [Google Scholar] [CrossRef]
- Becker, M.; Lorenz, A.; Elsmann, T.; Latka, I.; Schwuchow, A.; Dochow, S.; Spittel, R.; Kobelke, J.; Bierlich, J.; Schuster, K.; et al. Single-mode multi-core fibers with integrated Bragg filters. Light. Technol. 2016, 34, 4572–4578. [Google Scholar] [CrossRef]
- Franczyk, M.; Pysz, D.; Pucko, P.; Michalik, D.; Biduś, M.; Dłubek, M.; Buczyński, R. Yb3+ doped silica nanostructured core fiber laser. Opt. Express 2019, 27, 35108–35119. [Google Scholar] [CrossRef]
- Baskiotis, C.; Molin, D.; Bouwmans, G.; Gooijer, F.; Sillard, P.; Quiquempois, Y.; Douay, M. Bending behaviors of all solid silica large mode area Bragg fibers. In Fiber Lasers VI: Technology, Systems, and Applications; SPIE: Bellingham, WA, USA, 2009; Volume 7195, p. 719520. [Google Scholar]
- Ma, S.; Ning, T.; Li, J.; Pei, L.; Zhang, C.; Wen, X. Detailed study of bending effects in large mode area segmented cladding fibers. Appl. Opt. 2016, 55, 9954–9960. [Google Scholar] [CrossRef]
- Yu-Lai, S.; De-Jian, Z.; Xiao-Yong, C.; Jia-Ming, N. Bend-resistant low bending loss and large mode area single-mode fiber with low NA. Opt. Fiber Technol. 2019, 51, 101–106. [Google Scholar] [CrossRef]
- Rastogi, V.; Chiang, K.S. Propagation characteristics of a segmented cladding fiber. Opt. Lett. 2001, 26, 491–493. [Google Scholar] [CrossRef]
- Ma, S.; Ning, T.; Lu, S.; Zheng, J.; Li, J.; Pei, L. Bending-Resistant Design of a Large Mode Area Segmented Cladding Fiber with Resonant Ring. J. Light. Technol. 2018, 36, 2844–2849. [Google Scholar] [CrossRef]
- Yang, S.; Zhang, W.; She, Y.; Du, H.; Tu, S. A Large Mode Area Parabolic-Profile Core Fiber with Modified Segmented in Cladding. Photonics 2022, 9, 783. [Google Scholar] [CrossRef]
- Pournoury, M.; Zamiri, A.; Ghasemi, M.; Kim, D. The Optimization of a Segmented Cladding Fiber via the Response Surface Methodology Approach for a Large Mode Area. Photonics 2023, 10, 935. [Google Scholar] [CrossRef]
- Duan, J.; Teng, C.; Han, K.; Yu, M.; Wu, W.; Zhang, Q.; Chiang, K.S. Fabrication of segmented cladding fiber by bicomponent spinning. Polym. Eng. Sci. 2009, 49, 1865–1870. [Google Scholar] [CrossRef]
- Hooda, B.; Pal, A.; Rastogi, V.; Sen, R.; Gandhi, J.; Kobelke, J. Segmented cladding fiber fabricated in silica-based glass. Opt. Eng. 2015, 54, 075103. [Google Scholar] [CrossRef]
- Millo, A.; Naeh, I.; Katzir, A. Single-Mode Segmented Cladding Fibers for the Middle Infrared. Light. Technol. 2007, 25, 2115–2121. [Google Scholar] [CrossRef]
- Pournoury, M.; Han, S.R.; Ghasemi, M.; Lee, H.; Kim, D.; Oh, K. Silica Segmented Cladding Fiber Design and Its Fabrication Using a Powder-in-Tube Technique. Light. Technol. 2021, 39, 7251–7258. [Google Scholar] [CrossRef]
- Peng, Q.; Sheng, J.; Yang, K.; Wang, X.; Sun, W.; Wang, Y.; Liang, X.; Jiao, K.; Bai, S.; Zhao, Z.; et al. Single-Mode Segmented Cladding Chalcogenide Glass Fiber with Ultra-Large Mode Area. Light. Technol. 2023, 41, 5722–5728. [Google Scholar] [CrossRef]
- Yang, J.; Ming, H.; Chiang, K.S. Analysis of Erbium-Doped Ultralarge-Core Segmented-Cladding Fibers for Optical Amplification. Light. Technol. 2008, 26, 3098–3103. [Google Scholar] [CrossRef]
- Gao, K.; Yang, J.; Wang, A.; Ming, H.; Chiang, K.S. Numerical analysis of high power ytterbium-doped segmented-cladding fiber amplifier. In Proceedings of the Photonics and Optoelectronics Meetings, Wuhan, China, 24–27 November 2008. [Google Scholar]
- Wang, Y.; Hou, S.; Xie, G.Y.Z. Bend-resistant stress-type segmented cladding large-mode-area single-mode fibers. Appl. Opt. 2023, 62, 2218–2226. [Google Scholar] [CrossRef]
- Ma, S.; Ning, T.; Pei, L.; Li, J.; Zheng, J. Bend-resistant large mode area fiber with novel segmented cladding. Opt. Laser Technol. 2018, 98, 113–120. [Google Scholar] [CrossRef]
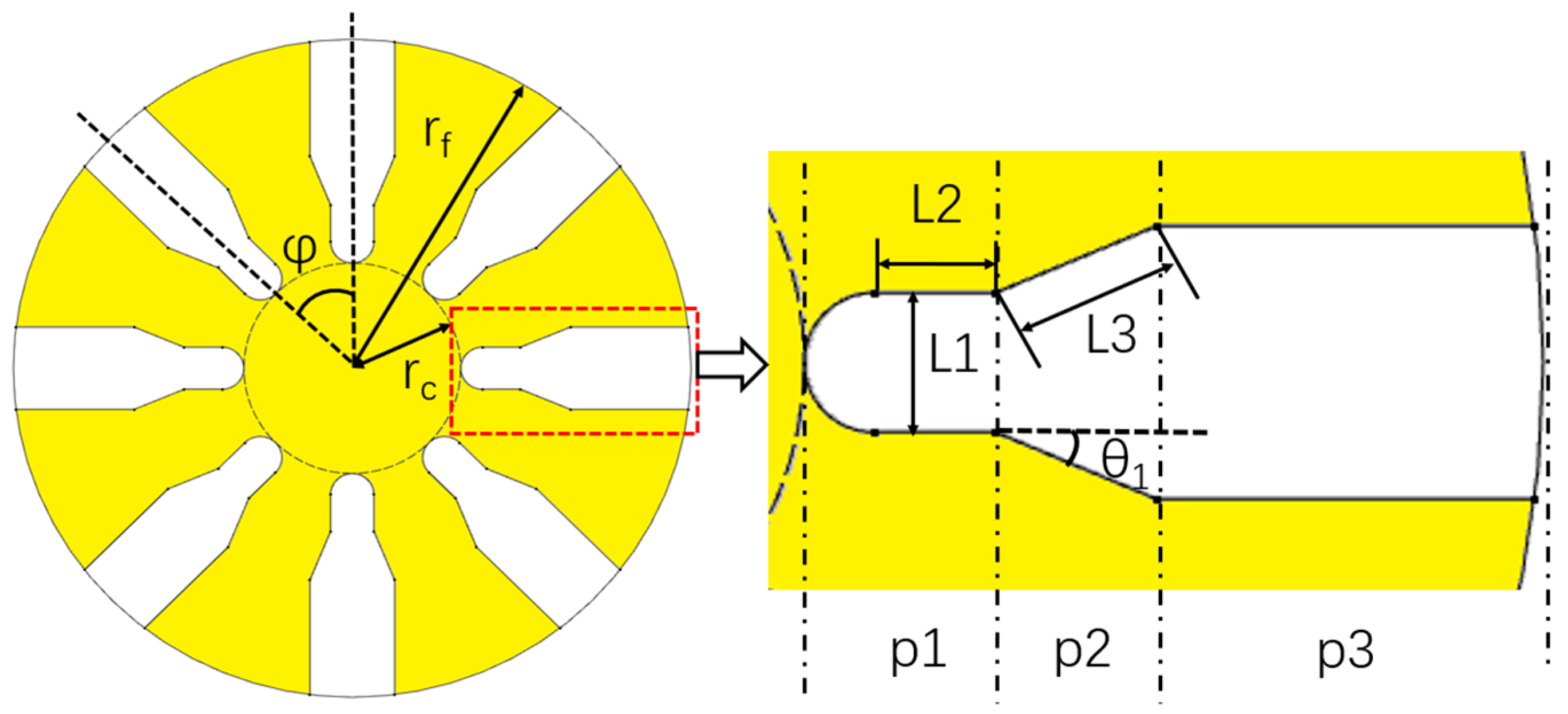





| Parameter | Value |
|---|---|
| rf | 62.5 μm |
| rc | 20 μm |
| φ | 45° |
| L1 | 8 μm |
| L2 | 7 μm |
| L3 | 10 μm |
| θ | 22.5° |
| nclad | 1.444 |
| ncore | 1.450 |
| λ | 1310 nm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xue, M.; Qin, H.; Li, S.; Hao, Y.; Yuan, L. A Detailed Study of a Resonant-Assisted Segmented Cladding Fiber for Large Mode Area Applications. Photonics 2025, 12, 84. https://doi.org/10.3390/photonics12010084
Xue M, Qin H, Li S, Hao Y, Yuan L. A Detailed Study of a Resonant-Assisted Segmented Cladding Fiber for Large Mode Area Applications. Photonics. 2025; 12(1):84. https://doi.org/10.3390/photonics12010084
Chicago/Turabian StyleXue, Minmin, Hao Qin, Suwen Li, Yuqi Hao, and Libo Yuan. 2025. "A Detailed Study of a Resonant-Assisted Segmented Cladding Fiber for Large Mode Area Applications" Photonics 12, no. 1: 84. https://doi.org/10.3390/photonics12010084
APA StyleXue, M., Qin, H., Li, S., Hao, Y., & Yuan, L. (2025). A Detailed Study of a Resonant-Assisted Segmented Cladding Fiber for Large Mode Area Applications. Photonics, 12(1), 84. https://doi.org/10.3390/photonics12010084





