An Efficient Hybrid Method for Calculating the Focal Field of a Cassegrain Antenna
Abstract
1. Introduction
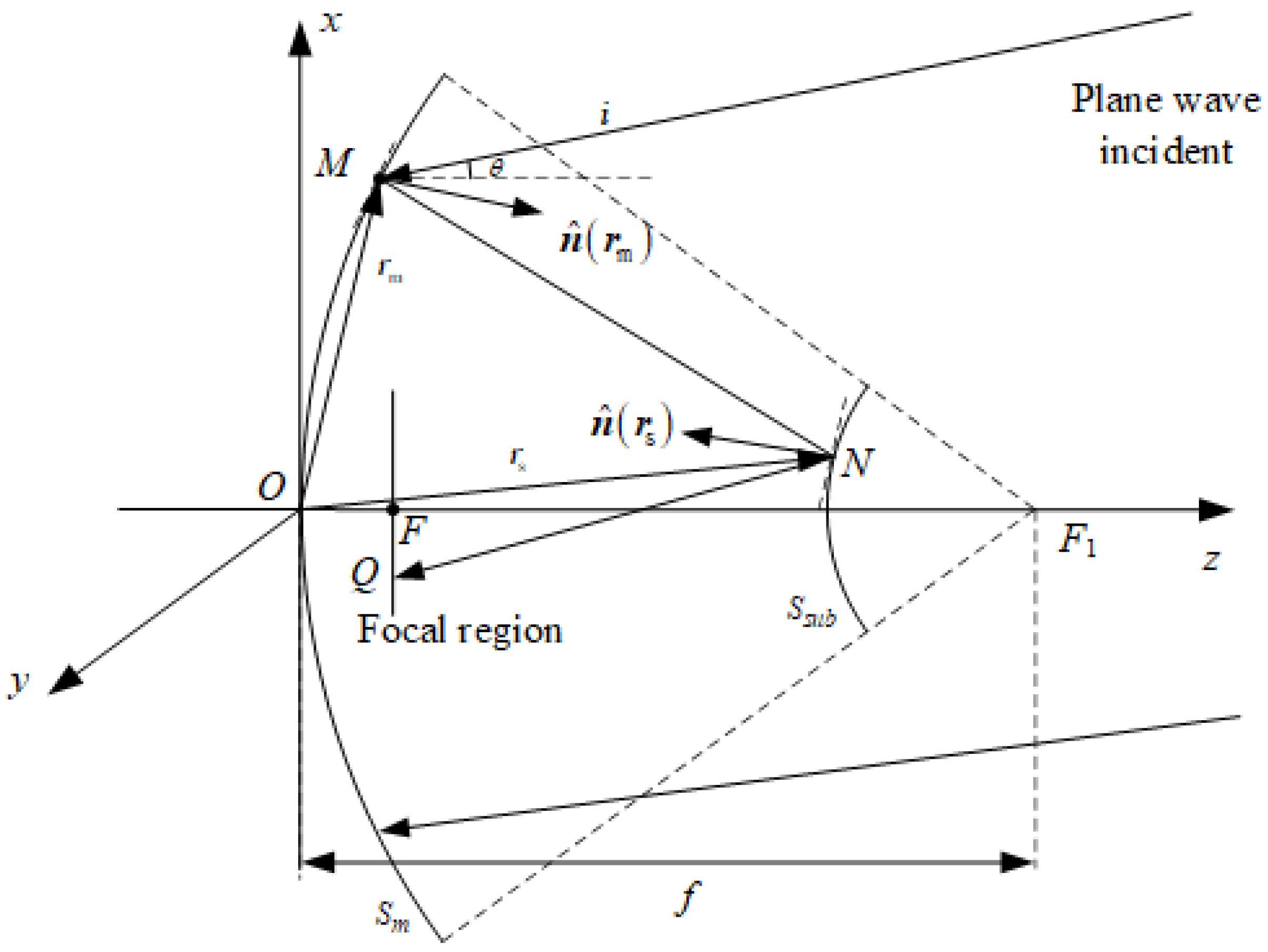
2. Traditional Induced Current Method
3. Proposed Method
4. Results
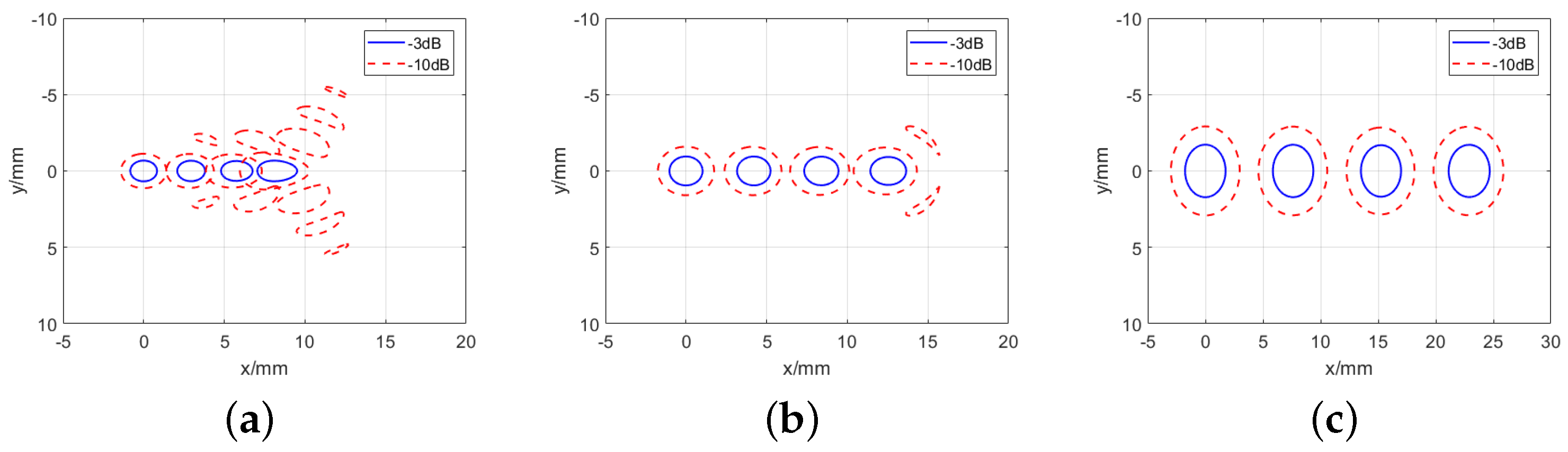
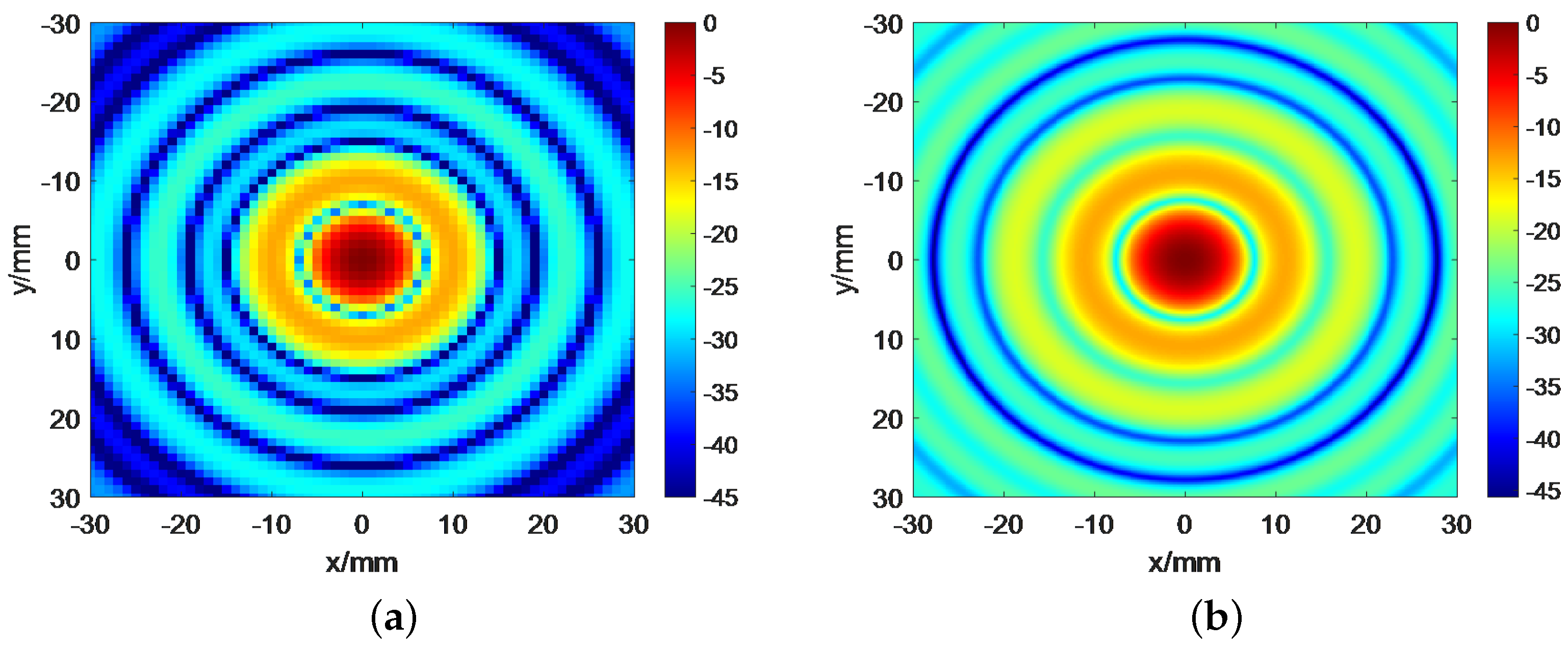
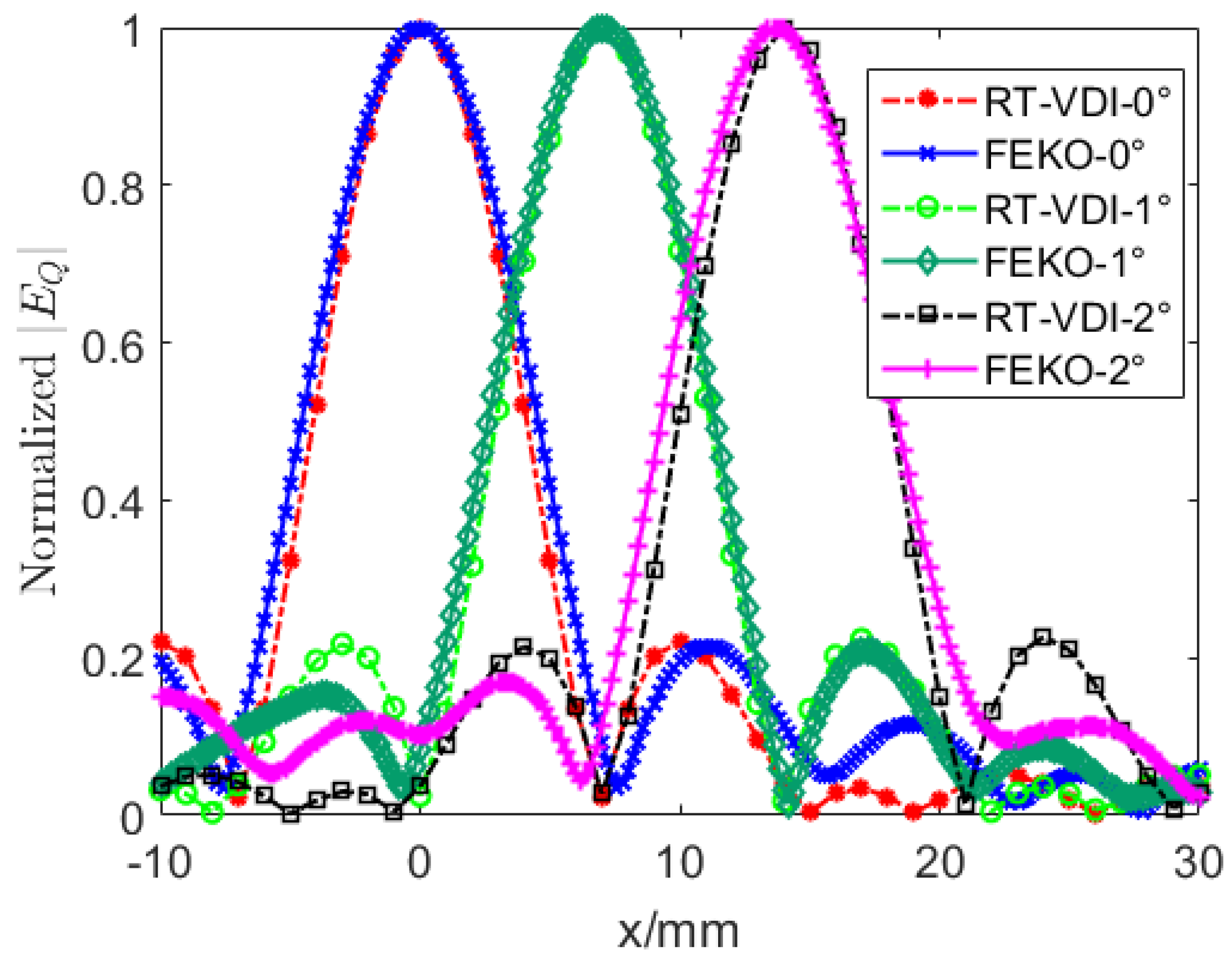
4.1. Accuracy
4.2. Efficiency
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Balasubramanian, M.; Campbell, S.D.; Werner, D.H.; Hand, T.H. A Shaped Reflector Antenna Design Approach for Contoured Beam Synthesis With Surface Curvature Constraints. IEEE Trans. Antennas Propag. 2024, 72, 1297–1307. [Google Scholar] [CrossRef]
- van den Biggelaar, A.; Al-Rawi, A.; Johannsen, U.; Smolders, A. 64 Element Active Phased Array as Focal Plane Array Feed for Reflector Antennas for mm-Wave Wireless Communications. In Proceedings of the 2020 14th European Conference on Antennas and Propagation (EuCAP), Copenhagen, Denmark, 15–20 March 2020; pp. 1–5. [Google Scholar] [CrossRef]
- Wang, J.; Ge, J.; Zhou, Y.; Xia, H.; Yang, X. Design of a High-Isolation 35/94-GHz Dual-Frequency Orthogonal-Polarization Cassegrain Antenna. IEEE Antennas Wirel. Propag. Lett. 2017, 16, 1297–1300. [Google Scholar] [CrossRef]
- Shi, Q.; Du, B.; Tian, J.; Niu, S.; Wu, Y. Design of a Ka Band Phased Array Feed Based on the Focal Plane Field Sampling for Edge Beam. In Proceedings of the 2023 Cross Strait Radio Science and Wireless Technology Conference (CSRSWTC), Guilin, China, 10–13 November 2023; pp. 1–3. [Google Scholar] [CrossRef]
- Chen, Q.; Fan, Y. Design of Focal Plane Array with Lens for W-band Short Range Passive Imaging. In Proceedings of the 2019 IEEE Asia-Pacific Microwave Conference (APMC), Singapore, 10–13 December 2019; pp. 859–861. [Google Scholar] [CrossRef]
- Gunaratne, T.K.; Bruton, L.; Agathoklis, P. Broadband Beamforming of Focal Plane Array (FPA) Signals Using Real-Time Spatio-Temporal 3D FIR Frustum Digital Filters. IEEE Trans. Antennas Propag. 2011, 59, 2029–2040. [Google Scholar] [CrossRef]
- Pakkathillam, J.K.; Sivaprakasam, B.T.; Poojali, J.; Krishnamurthy, C.V.; Arunachalam, K. Tailoring Antenna Focal Plane Characteristics for a Compact Free-Space Microwave Complex Dielectric Permittivity Measurement Setup. IEEE Trans. Instrum. Meas. 2021, 70, 6000412. [Google Scholar] [CrossRef]
- Wu, Y.; Liang, W.; Ma, C.; Wang, M.; Zheng, H.; Li, E. Thin Absorber with Dispersive Medium Simulated by Conformal Hybrid Explicit Implicit FDTD Method. IEEE Antennas Wirel. Propag. Lett. 2024, 23, 2461–2465. [Google Scholar] [CrossRef]
- Wang, Y.Y.; Zhan, Q.; Feng, H.; Wang, Y.D.; Sun, B.; Yin, W.Y. A Novel Matrix-Free Finite Element Method for Time-Harmonic Maxwell’s Equations. IEEE Trans. Antennas Propag. 2024, 72, 2609–2619. [Google Scholar] [CrossRef]
- Huo, J.; Xu, L.; Shi, X.; Yang, Z. An Accelerated Shooting and Bouncing Ray Method Based on GPU and Virtual Ray Tube for Fast RCS Prediction. IEEE Antennas Wirel. Propag. Lett. 2021, 20, 1839–1843. [Google Scholar] [CrossRef]
- Dong, C.L.; Guo, L.X.; Meng, X. An Accelerated Algorithm Based on GO-PO/PTD and CWMFSM for EM Scattering From the Ship Over a Sea Surface and SAR Image Formation. IEEE Trans. Antennas Propag. 2020, 68, 3934–3944. [Google Scholar] [CrossRef]
- Bian, Z.; Li, J.; Guo, L.; Luo, X. Analyzing the Electromagnetic Scattering Characteristics of a Hypersonic Vehicle Based on the Inhomogeneity Zonal Medium Model. IEEE Trans. Antennas Propag. 2021, 69, 971–982. [Google Scholar] [CrossRef]
- Ling, H.; Chou, R.C.; Lee, S.W. Shooting and bouncing rays: Calculating the RCS of an arbitrarily shaped cavity. IEEE Trans. Antennas Propag. 1989, 37, 194–205. [Google Scholar] [CrossRef]
- Li, J.; Li, K.; Guo, L.X.; Ren, Z.L. A Bi-Iterative Model for Electromagnetic Scattering From a PEC Object Partially Buried in Rough Sea Surface. IEEE Geosci. Remote Sens. Lett. 2018, 15, 493–497. [Google Scholar] [CrossRef]
- Wang, H.; Miao, J.; Jiang, J.; Wang, R. The focal region electric field distribution of an offset paraboloid. In Proceedings of the 2012 International Symposium on Communications and Information Technologies (ISCIT), Gold Coast, Australia, 2–5 October 2012; pp. 632–637. [Google Scholar] [CrossRef]
- Naeini, M.A.; Bruton, L.T. The 3D on-axis space-time scalar impulse response field of the parabolic reflector in the focal plane. In Proceedings of the 2011 IEEE Pacific Rim Conference on Communications, Computers and Signal Processing, Victoria, BC, Canada, 23–26 August 2011; pp. 394–398. [Google Scholar] [CrossRef]
- Budé, R.X.F.; Elsakka, A.; Johannsen, U.; Smolders, A.B. Wide-Scan Focal Plane Arrays for mmWave Point-to-Multipoint Communications. IEEE Open J. Antennas Propag. 2022, 3, 112–123. [Google Scholar] [CrossRef]
- Letrou, C.; Boag, A. An MLPO algorithm for fast evaluation of the focal plane fields of reflector antennas. In Proceedings of the 2012 6th European Conference on Antennas and Propagation (EUCAP), Prague, Czech Republic, 26–30 March 2012; pp. 3436–3437. [Google Scholar] [CrossRef][Green Version]
- Chen, B.; Tong, C. Near-Field Scattering Prediction Based on Refined Time-Domain High-Frequency Method. IEEE Antennas Wirel. Propag. Lett. 2019, 18, 1194–1198. [Google Scholar] [CrossRef]
- Rahim, T.; Mughal, M.J.; Naqvi, Q.A.; Faryad, M. Focal Region Field of a Paraboloidal Reflector Coated with Isotropic Chiral Medium. Prog. Electromagn.-Res.-Pier 2009, 94, 351–366. [Google Scholar] [CrossRef][Green Version]
- TICRA Software. Available online: https://www.ticra.com/software/grasp/ (accessed on 11 July 2024).


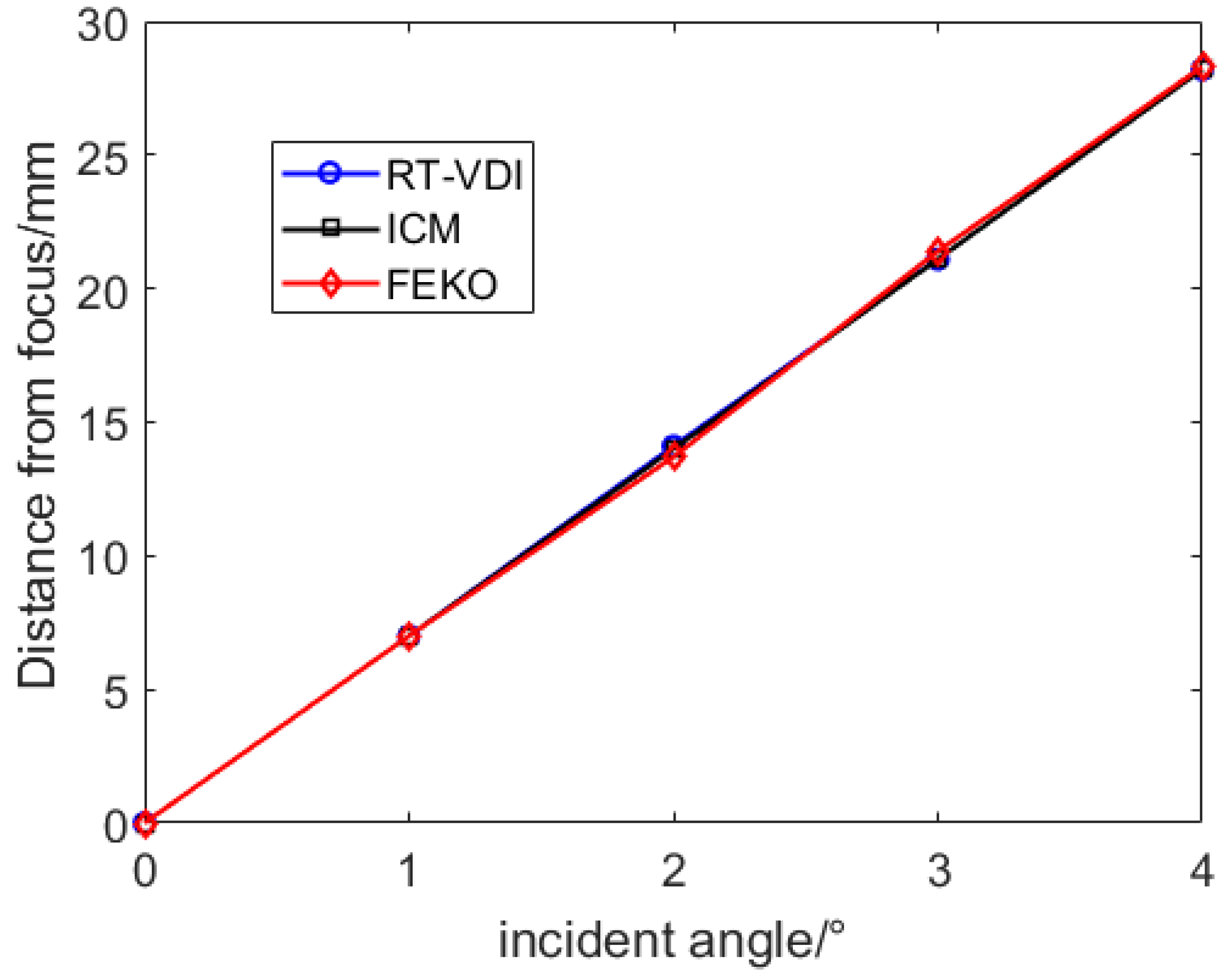
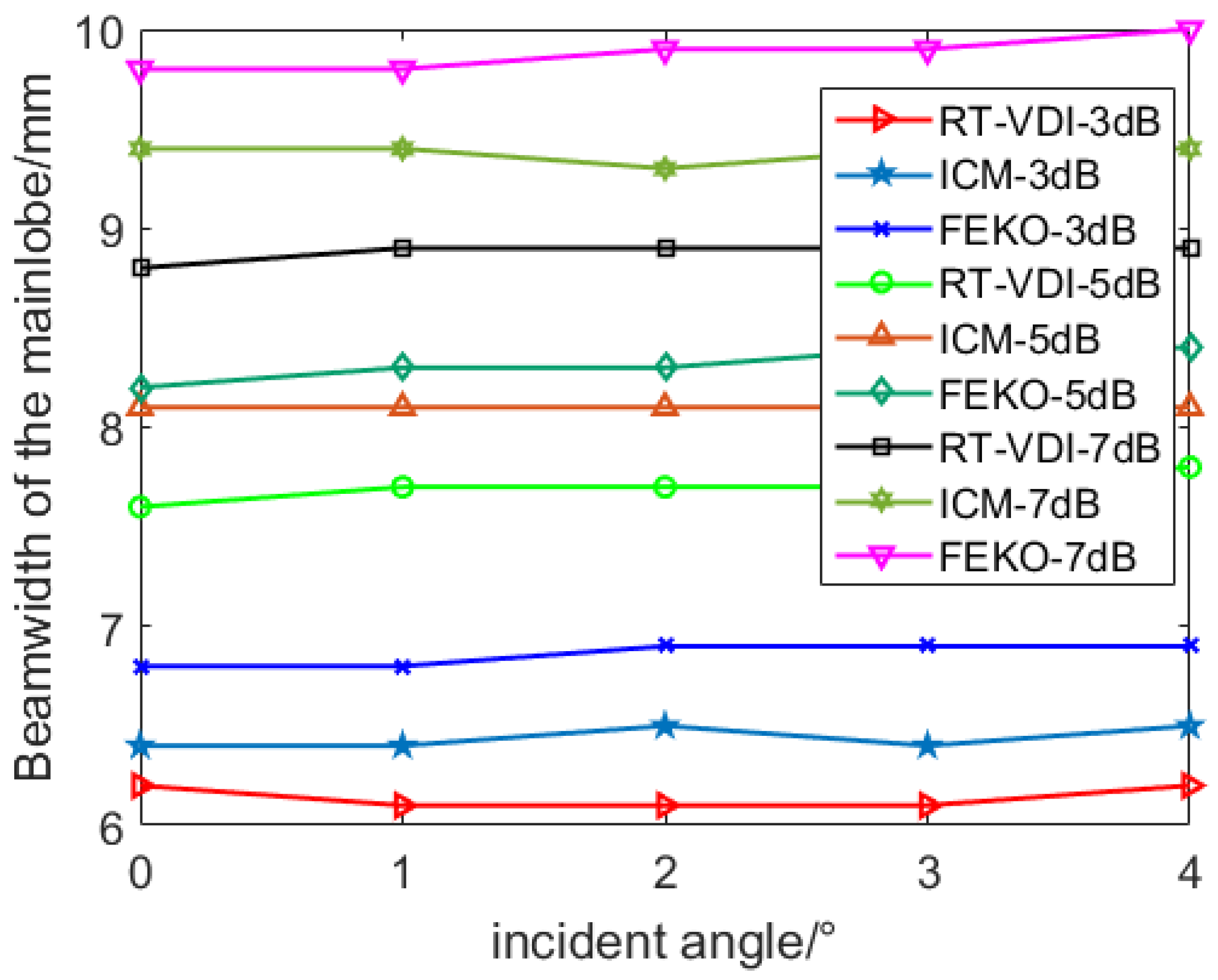
| Traditional Method | RT-VDI | Speedup | |
|---|---|---|---|
| 1 | 2.133 s | 1.066 s | 2.0 |
| 4 | 30.242 s | 11.887 s | 2.54 |
| 2.5 | 2473.40 s | 768.628 s | 3.22 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lian, H.; Jiang, Y.; Lu, D.; Xiao, H.; Fan, H. An Efficient Hybrid Method for Calculating the Focal Field of a Cassegrain Antenna. Photonics 2024, 11, 832. https://doi.org/10.3390/photonics11090832
Lian H, Jiang Y, Lu D, Xiao H, Fan H. An Efficient Hybrid Method for Calculating the Focal Field of a Cassegrain Antenna. Photonics. 2024; 11(9):832. https://doi.org/10.3390/photonics11090832
Chicago/Turabian StyleLian, Hongfei, Yanwen Jiang, Dawei Lu, Huaitie Xiao, and Hongqi Fan. 2024. "An Efficient Hybrid Method for Calculating the Focal Field of a Cassegrain Antenna" Photonics 11, no. 9: 832. https://doi.org/10.3390/photonics11090832
APA StyleLian, H., Jiang, Y., Lu, D., Xiao, H., & Fan, H. (2024). An Efficient Hybrid Method for Calculating the Focal Field of a Cassegrain Antenna. Photonics, 11(9), 832. https://doi.org/10.3390/photonics11090832





