Rapid Autofocus Method Based on LED Oblique Illumination for Metaphase Chromosome Microscopy Imaging System
Abstract
1. Introduction
2. Materials and Methods
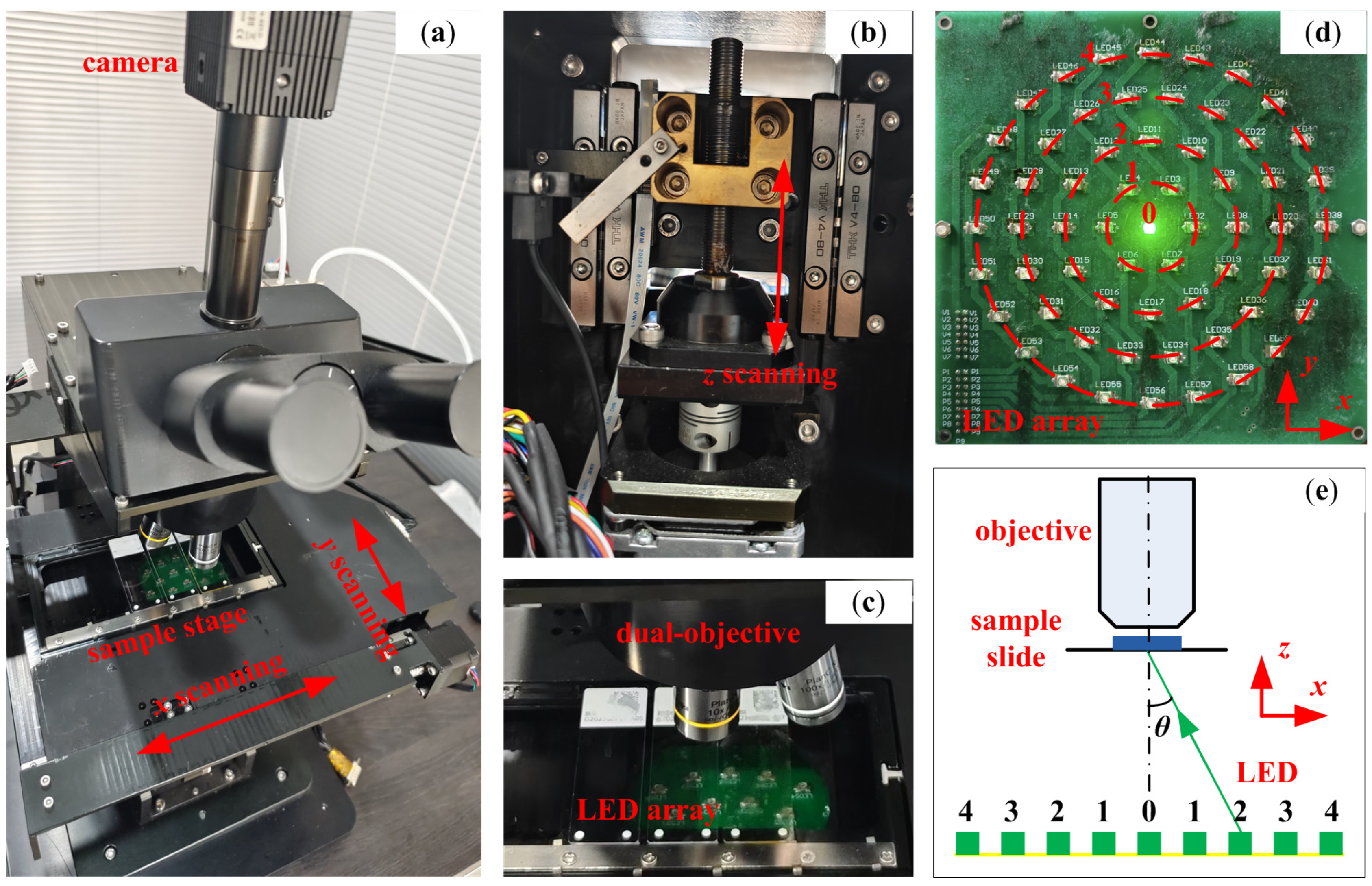
2.1. Hardware Platform
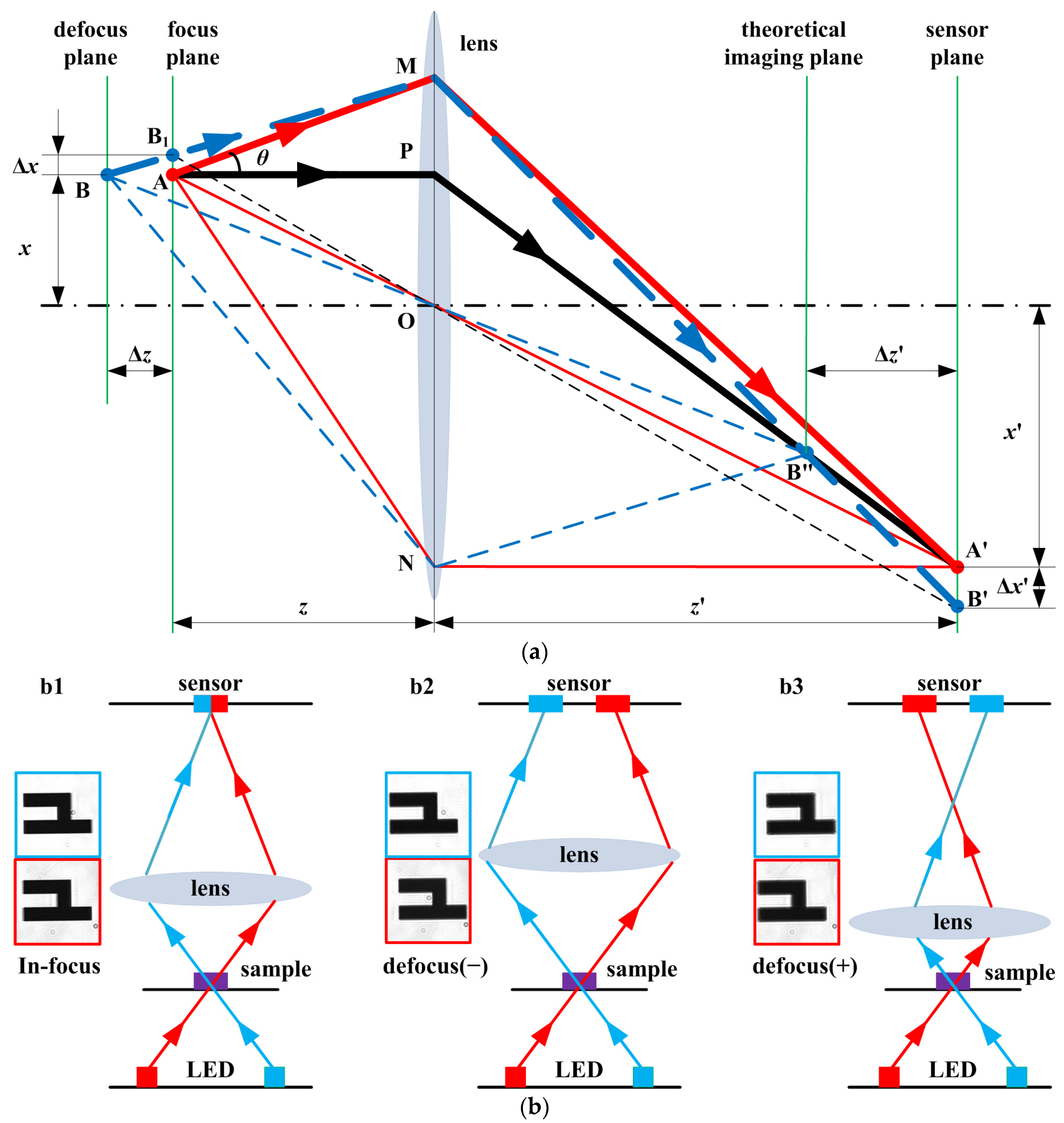
2.2. Rapid Autofocus Method Based on LED Oblique Illumination for Dual-Objective Unit
3. Experimental Results
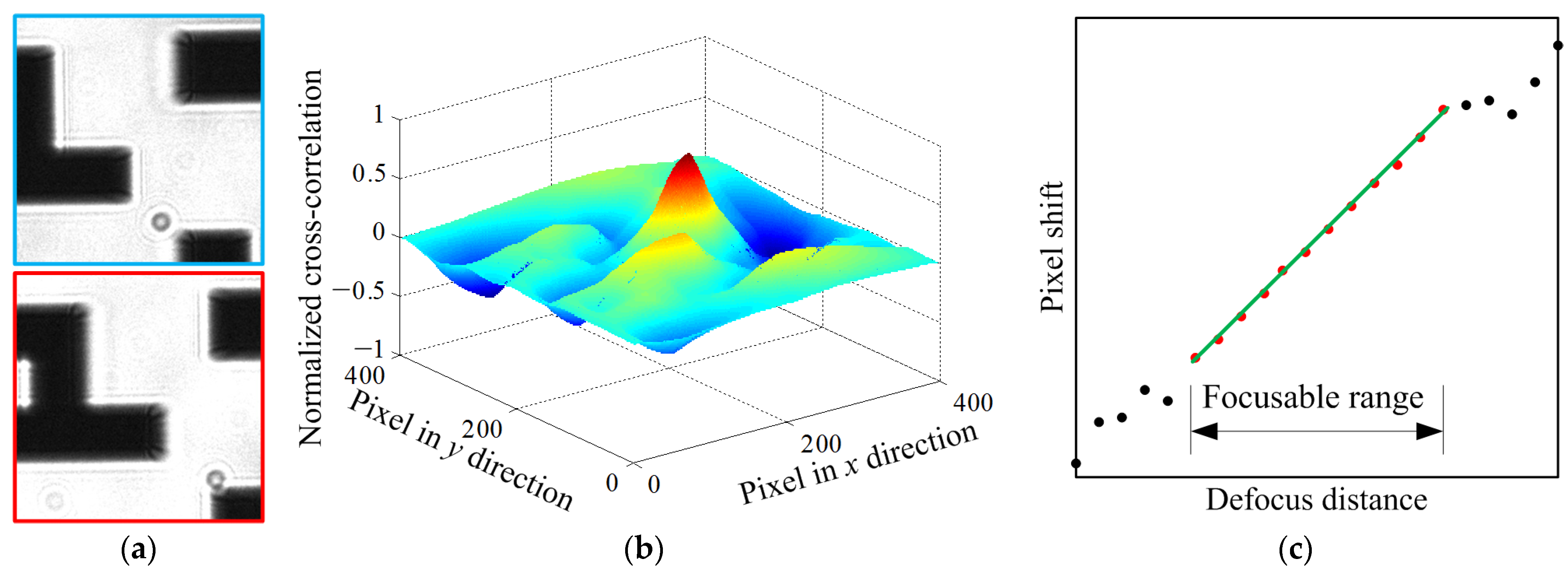
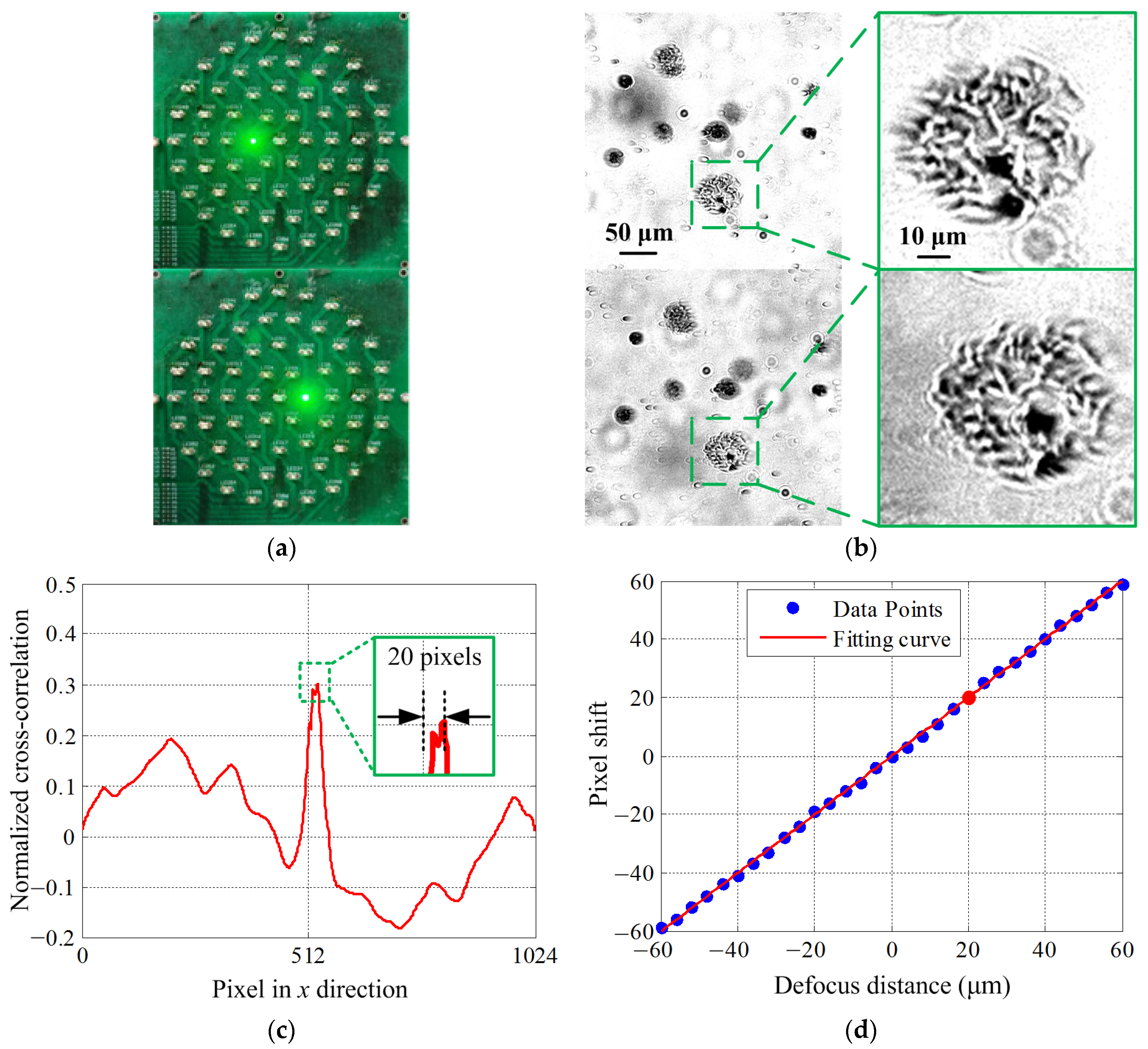
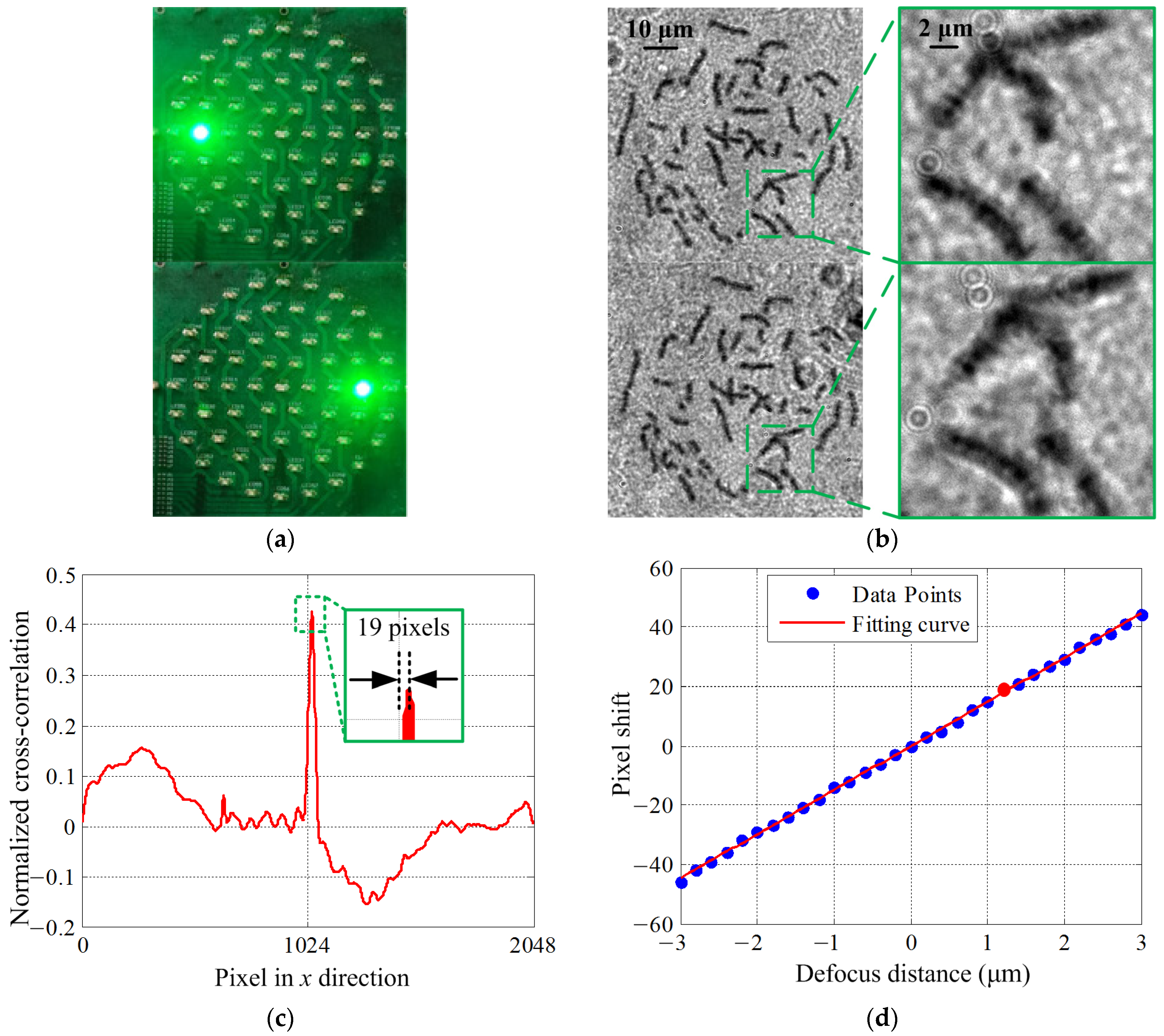
3.1. Dual-Objective Focus Curve Fitting
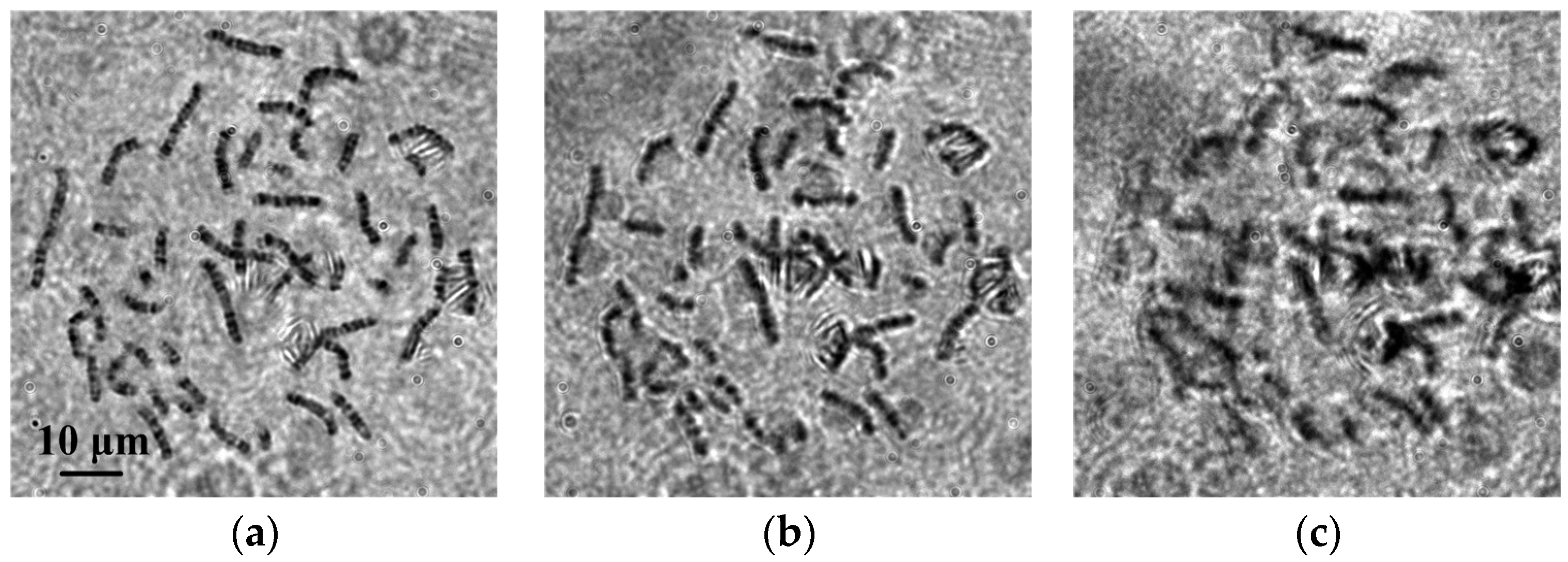
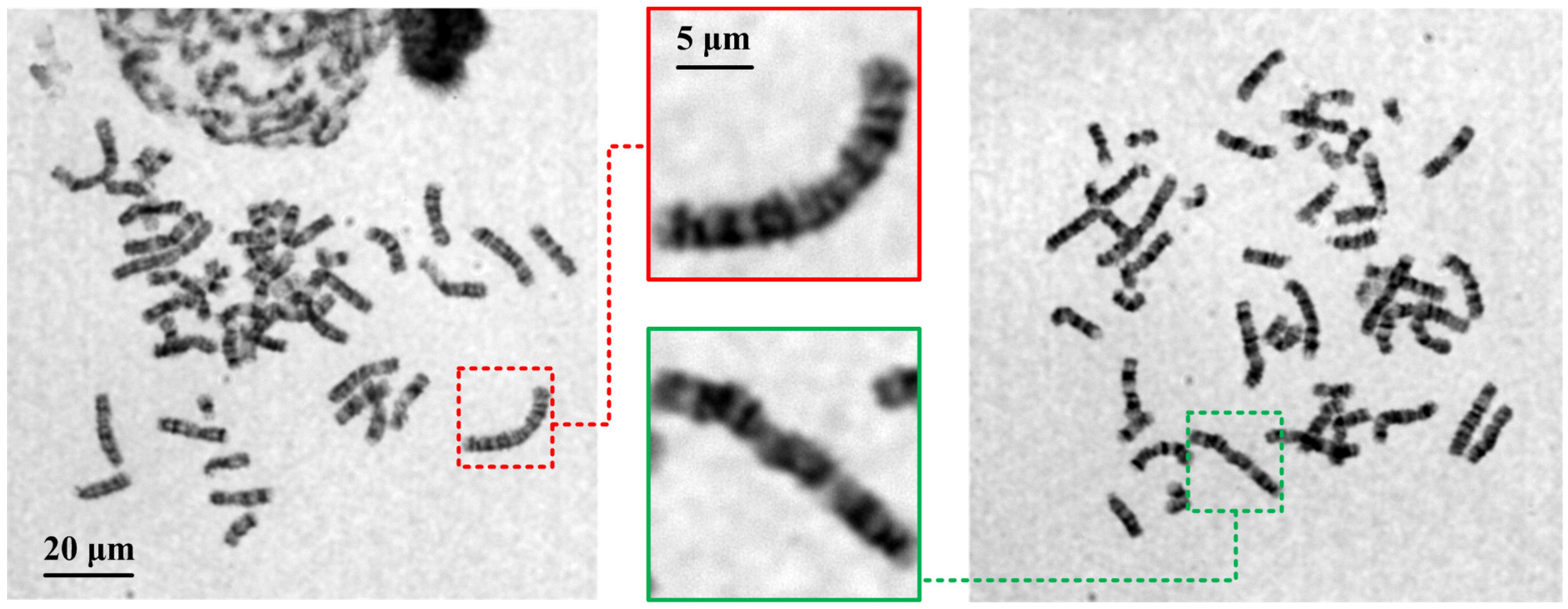
3.2. Chromosome Whole-Slide Autofocusing Experiments
3.3. Evaluation of Autofocusing Performance
4. Conclusions and Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Natarajan, A.T. Chromosome aberrations: Past, present and future. Mutat. Res. 2002, 504, 3–16. [Google Scholar] [CrossRef] [PubMed]
- Levy, B.; Wapner, R. Prenatal diagnosis by chromosomal microarray analysis. Fertil. Steril. 2018, 109, 201–212. [Google Scholar] [CrossRef] [PubMed]
- Theisen, A.; Shaffer, L.G. Disorders caused by chromosome abnormalities. Appl. Clin. Genet. 2010, 3, 159–174. [Google Scholar] [PubMed]
- Madian, N.; Jayanthi, K.B. Analysis of human chromosome classification using centromere position. Measurement 2014, 47, 287–295. [Google Scholar] [CrossRef]
- Arora, T.; Dhir, R. A review of metaphase chromosome image selection techniques for automatic karyotype generation. Med. Biol. Eng. Comput. 2016, 54, 1147–1157. [Google Scholar] [CrossRef]
- Grisan, E.; Poletti, E.; Ruggeri, A. Automatic segmentation and disentangling of chromosomes in Q-band prometaphase images. IEEE Trans. Inf. Technol. Biomed. 2009, 13, 575–581. [Google Scholar] [CrossRef]
- Furukawa, A. The implementation of artificial intelligence to the low-cost metaphase finder. Radiat. Prot. Dosim. 2023, 199, 1460–1464. [Google Scholar] [CrossRef]
- Wang, X.; Li, S.; Liu, H.; Wood, M.; Chen, W.R.; Zheng, B. Automated identification of analyzable metaphase chromosomes shown on microscopy digital images. J. Biomed. Inf. 2008, 41, 264–271. [Google Scholar] [CrossRef]
- Bian, Z.; Guo, C.; Jiang, S.; Zhu, J.; Wang, R.; Song, P.; Zhang, Z.; Hoshino, K.; Zheng, G. Autofocusing technologies for whole slide imaging and automated microscopy. J. Biophotonics 2020, 13, e202000227. [Google Scholar] [CrossRef]
- Hu, J.; Zhong, B.; Jin, Z.; Wang, Z.; Sun, L. Adaptive predictive scanning method based on a high-precision automatic microscopy system. Appl. Opt. 2019, 58, 7305–7310. [Google Scholar] [CrossRef]
- Liu, C.-S.; Song, R.-C.; Fu, S.-J. Design of a laser-based autofocusing microscope for a sample with a transparent boundary layer. Appl. Phys. B 2019, 125, 199. [Google Scholar] [CrossRef]
- Song, J.; Liu, M. A new methodology in constructing no-reference focus quality assessment metrics. Pattern Recognit. 2023, 142, 109688. [Google Scholar] [CrossRef]
- Qiu, Y.; Chen, X.; Li, Y.; Chen, W.R.; Zheng, B.; Li, S.; Liu, H. Evaluations of auto-focusing methods under a microscopy imaging modality for metaphase chromosome image analysis. Anal. Cell. Pathol. 2013, 36, 37–44. [Google Scholar] [CrossRef]
- Yilmaz, H.; Turan, M.K. FahamecV1: A Low Cost Automated Metaphase Detection System. Eng. Technol. Appl. Sci. Res. 2017, 7, 2160–2166. [Google Scholar] [CrossRef]
- Furukawa, A.; Minamihisamatsu, M.; Hayata, I. Low-cost metaphase finder system. Health Phys. 2010, 98, 269–275. [Google Scholar] [CrossRef]
- Furukawa, A. The project of another low-cost metaphase finder. Radiat. Prot. Dosim. 2016, 172, 238–243. [Google Scholar] [CrossRef]
- Montalto, M.C.; McKay, R.R.; Filkins, R.J. Autofocus methods of whole slide imaging systems and the introduction of a second-generation independent dual sensor scanning method. J. Pathol. Inform. 2011, 2, 44. [Google Scholar] [CrossRef]
- Guo, K.; Liao, J.; Bian, Z.; Heng, X.; Zheng, G. InstantScope: A low-cost whole slide imaging system with instant focal plane detection. Biomed. Opt. Express 2015, 6, 3210–3216. [Google Scholar] [CrossRef]
- Li, C.; Liu, K.; Guo, X.; Xiao, Y.; Zhang, Y.; Huang, Z.L. Robust autofocus method based on patterned active illumination and image cross-correlation analysis. Biomed. Opt. Express 2024, 15, 2697–2707. [Google Scholar] [CrossRef]
- Liao, J.; Bian, L.; Bian, Z.; Zhang, Z.; Patel, C.; Hoshino, K.; Eldar, Y.C.; Zheng, G. Single-frame rapid autofocusing for brightfield and fluorescence whole slide imaging. Biomed. Opt. Express 2016, 7, 4763–4768. [Google Scholar] [CrossRef]
- Liao, J.; Jiang, Y.; Bian, Z.; Mahrou, B.; Nambiar, A.; Magsam, A.W.; Guo, K.; Wang, S.; Cho, Y.K.; Zheng, G. Rapid focus map surveying for whole slide imaging with continuous sample motion. Opt. Lett. 2017, 42, 3379–3382. [Google Scholar] [CrossRef] [PubMed]
- Liao, J.; Wang, Z.; Zhang, Z.; Bian, Z.; Guo, K.; Nambiar, A.; Jiang, Y.; Jiang, S.; Zhong, J.; Choma, M.; et al. Dual light-emitting diode-based multichannel microscopy for whole-slide multiplane, multispectral and phase imaging. J. Biophoton. 2018, 11, e201700075. [Google Scholar] [CrossRef] [PubMed]
- Jiang, S.; Bian, Z.; Huang, X.; Song, P.; Zhang, H.; Zhang, Y.; Zheng, G. Rapid and robust whole slide imaging based on LED-array illumination and color-multiplexed single-shot autofocusing. Quant. Imaging Med. Surg. 2019, 9, 82331–82831. [Google Scholar] [CrossRef]
- Guo, C.; Bian, Z.; Jiang, S.; Murphy, M.; Zhu, J.; Wang, R.; Song, P.; Shao, X.; Zhang, Y.; Zheng, G. OpenWSI: A low-cost, high-throughput whole slide imaging system via single-frame autofocusing and open-source hardware. Opt. Lett. 2020, 45, 260–263. [Google Scholar] [CrossRef]
- Guo, C.; Bian, Z.; Alhudaithy, S.; Jiang, S.; Tomizawa, Y.; Song, P.; Wang, T.; Shao, X. Brightfield, fluorescence, and phase-contrast whole slide imaging via dual-LED autofocusing. Biomed. Opt. Express 2021, 12, 4651–4660. [Google Scholar] [CrossRef]
- Xin, K.; Jiang, S.; Chen, X.; He, Y.; Zhang, J.; Wang, H.; Liu, H.; Peng, Q.; Zhang, Y.; Ji, X. Low-cost whole slide imaging system with single-shot autofocusing based on color-multiplexed illumination and deep learning. Biomed. Opt. Express 2021, 12, 5644–5657. [Google Scholar] [CrossRef]
- Zheng, G.; Horstmeyer, R.; Yang, C. Wide-field, high-resolution Fourier ptychographic microscopy. Nat. Photonics 2013, 7, 739. [Google Scholar] [CrossRef]
- Lewis, J.P. Fast normalized cross-correlation. Vis. Interface 1995, 10, 120–123. [Google Scholar]
- Strojnik, M.; Bravo-Medina, B.; Martin, R.; Wang, Y. Ensquared energy and optical centroid efficiency in optical sensors: Part 1, Theory. Photonics 2023, 10, 254. [Google Scholar] [CrossRef]
- Born, M.; Wolf, E. Principles of Optics, 7th ed.; Cambridge University Press: Cambridge, UK, 1999. [Google Scholar]
- Strojnik, M.; Martin, R.; Wang, Y. Ensquared energy and optical centroid efficiency in optical sensors: Part 2, Primary Aberrations. Photonics 2024, 11, 855. [Google Scholar] [CrossRef]
- Qiu, Y.; Chen, X.; Li, Y.; Zheng, B.; Li, S.; Chen, W.R.; Liu, H. Impact of the optical depth of field on cytogenetic image quality. J. Biomed. Opt. 2012, 17, 096017. [Google Scholar] [CrossRef] [PubMed]
- Brenner, J.F.; Dew, B.S.; Horton, J.B.; King, T.; Neurath, P.W.; Selles, W.D. An automated microscope for cytologic research a preliminary evaluation. J. Histochem. Cytochem. 1976, 24, 100–111. [Google Scholar] [CrossRef] [PubMed]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, C.; Ding, F.; Ma, Z.; Tang, Y. Rapid Autofocus Method Based on LED Oblique Illumination for Metaphase Chromosome Microscopy Imaging System. Photonics 2024, 11, 1091. https://doi.org/10.3390/photonics11111091
Yu C, Ding F, Ma Z, Tang Y. Rapid Autofocus Method Based on LED Oblique Illumination for Metaphase Chromosome Microscopy Imaging System. Photonics. 2024; 11(11):1091. https://doi.org/10.3390/photonics11111091
Chicago/Turabian StyleYu, Changliang, Fangqiu Ding, Zhenyu Ma, and Yuguo Tang. 2024. "Rapid Autofocus Method Based on LED Oblique Illumination for Metaphase Chromosome Microscopy Imaging System" Photonics 11, no. 11: 1091. https://doi.org/10.3390/photonics11111091
APA StyleYu, C., Ding, F., Ma, Z., & Tang, Y. (2024). Rapid Autofocus Method Based on LED Oblique Illumination for Metaphase Chromosome Microscopy Imaging System. Photonics, 11(11), 1091. https://doi.org/10.3390/photonics11111091




