An Optimized Error Compensation Method for Phase Measurement Profilometry
Abstract
:1. Introduction
2. The Proposed Method
2.1. Wrapped Phase Retrieval
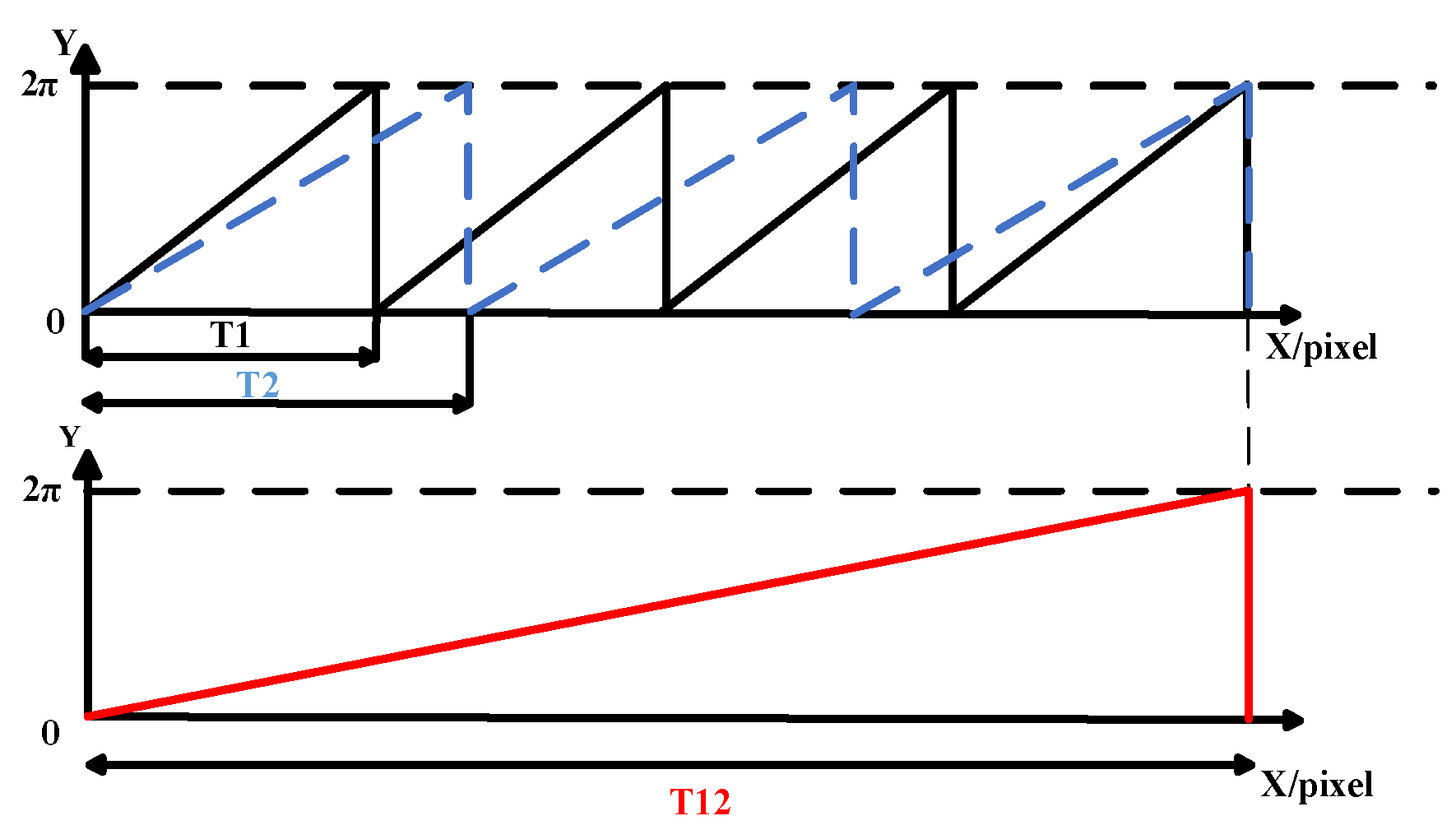
2.2. Phase Unwrapped
2.3. System Calibration
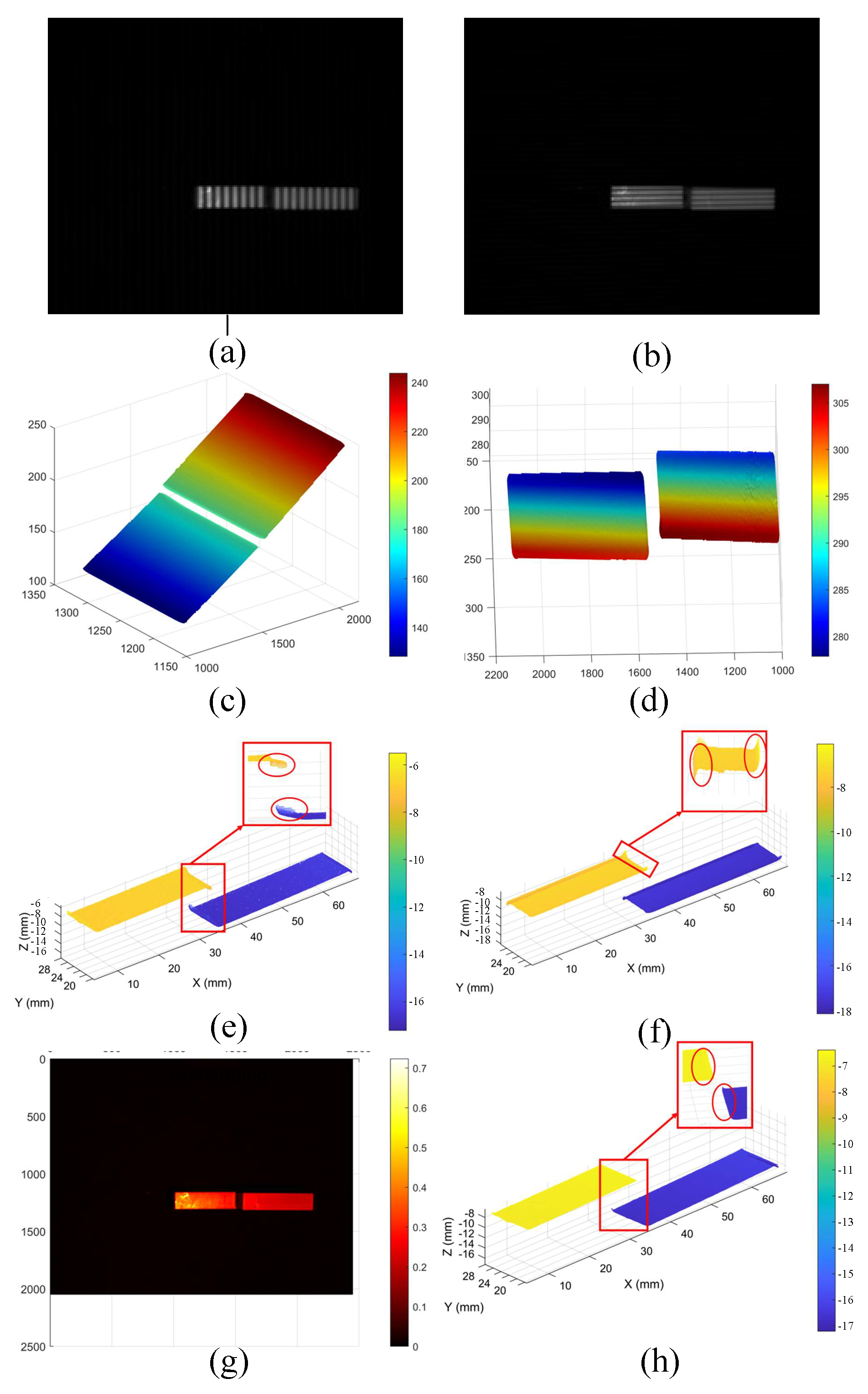
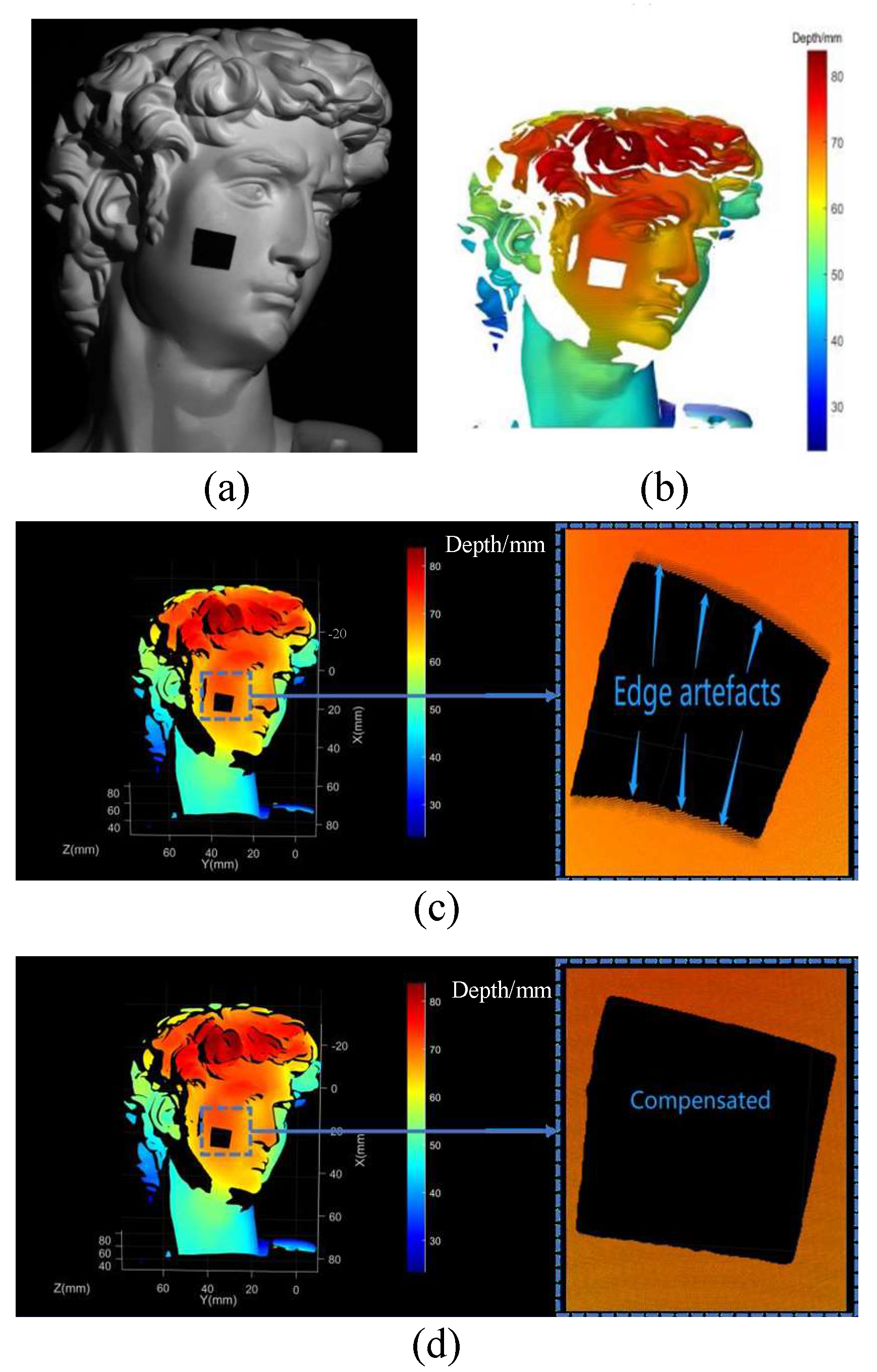
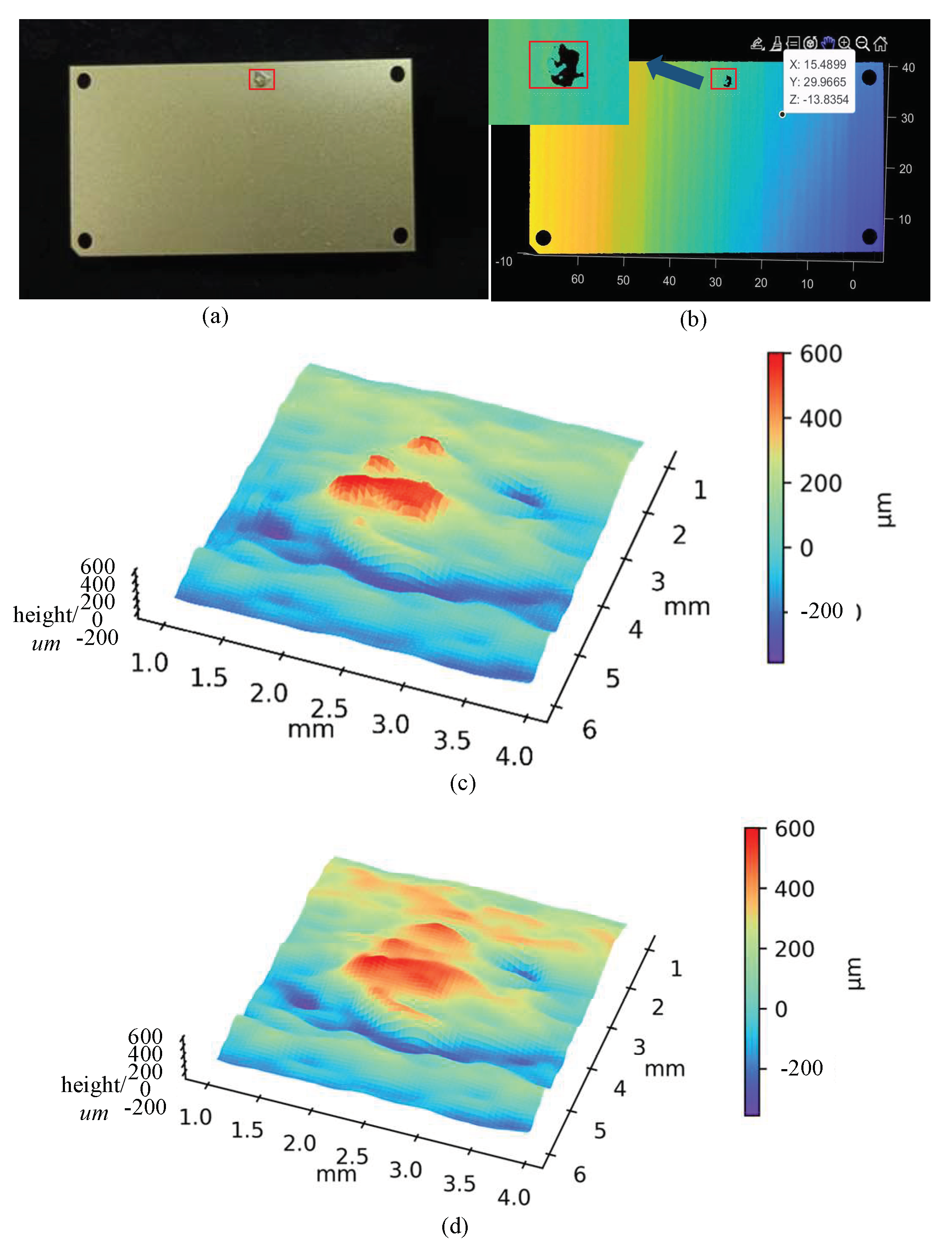
2.4. Edge Artefacts Compensation
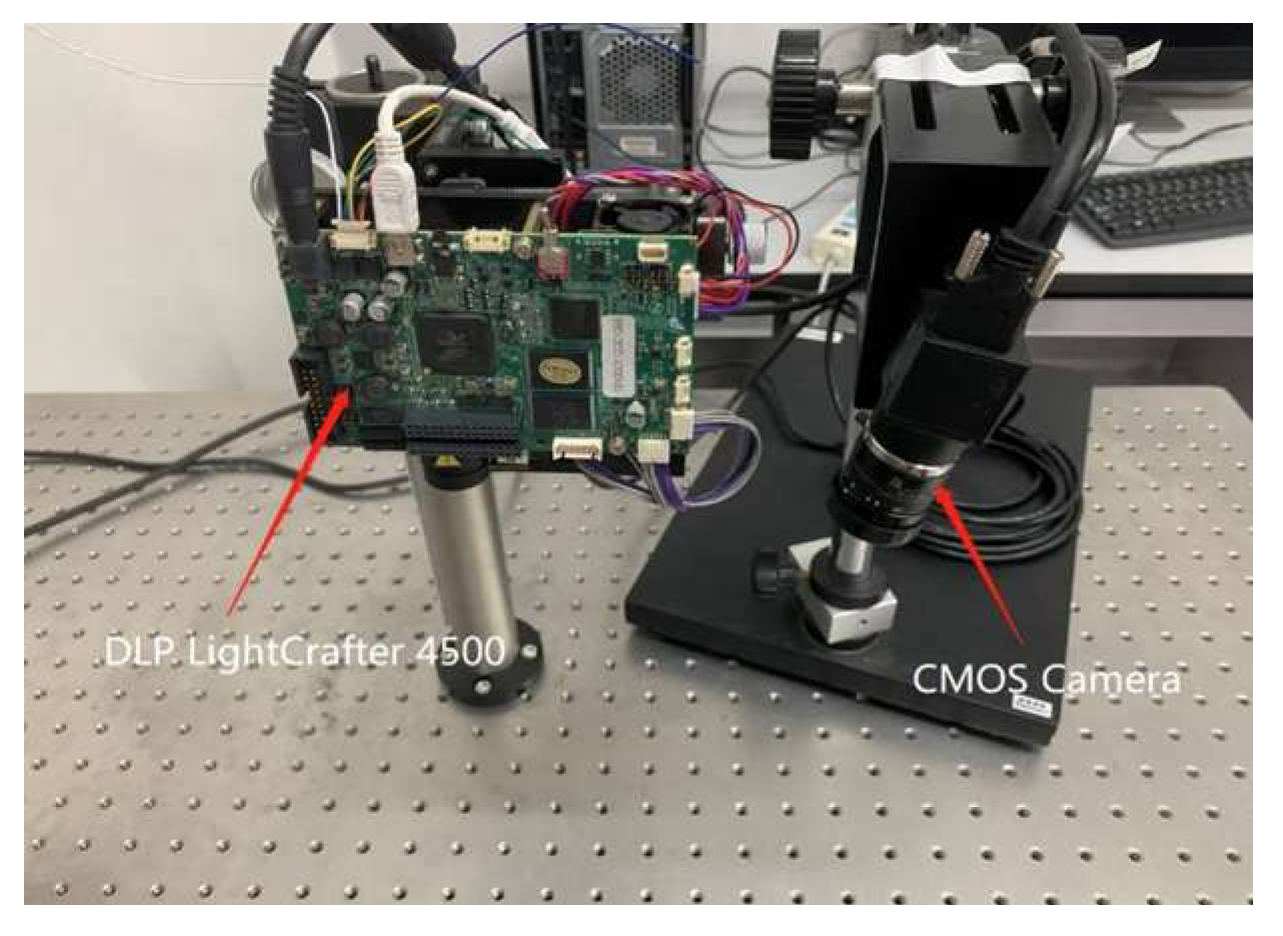
3. Experiments
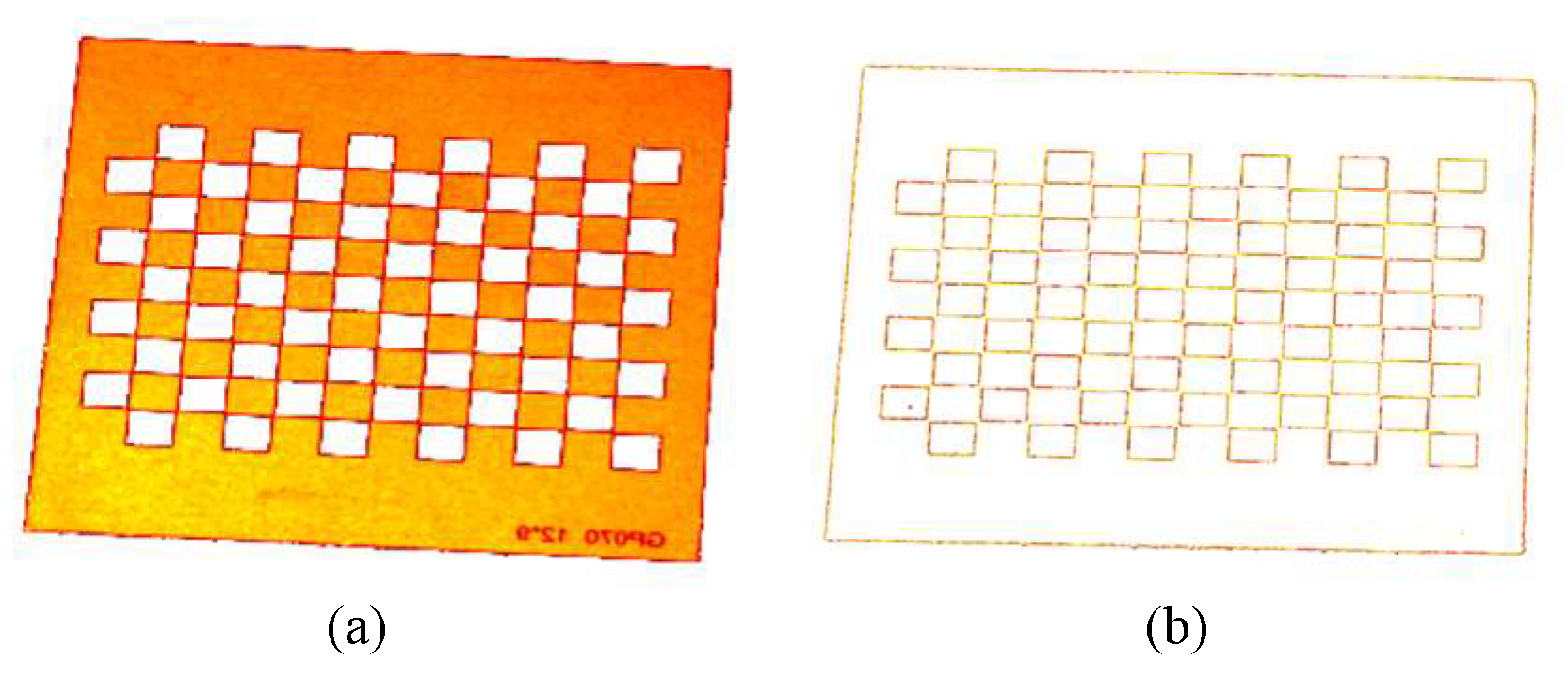
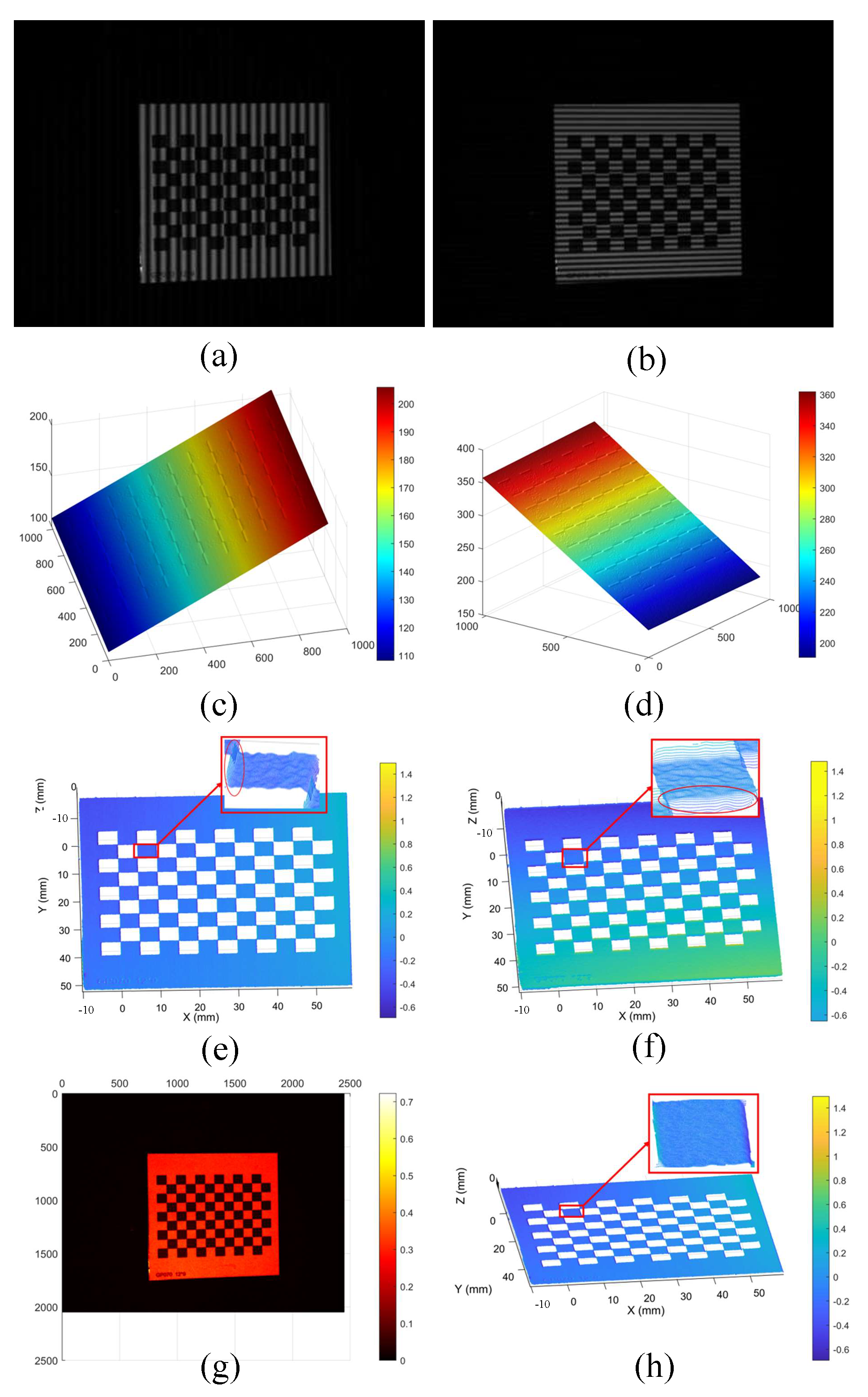
3.1. Reconstruction of Checkerboard
3.2. Reconstruction of Ceramic Blocks
3.3. Reconstruction of Plaster Sculpture
3.4. Reconstruction of Workpiece
4. Discussion
- (1)
- This study ignores the influence brought about by edge artefacts during system calibration. The phased elimination of artefacts in the circular calibration plate was introduced in [13], which can solve the error effects caused by edge artefacts to some extent. The method of fitting the phase of the calibration plate plane mentioned further improves the calibration accuracy [28]. These methods are all compensated based on phase results and cannot be applied correctly to objects with complex surfaces. Therefore, a combination method for error compensation for the reconstruction results can be further investigated.
- (2)
- Although the number of fringe patterns used by the phase-shifting method is reduced by using a Hilbert transform derived from the work in [20], twelve images still need to be projected, which is permissible for static measurements but not well-suited to dynamic measurements. Therefore, a combination of defocusing techniques in fringe projection profilometry can be employed to increase the projection rate for dynamic measurements of the object surface.
- (3)
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ma, R.; Li, J.; He, K.; Tang, T.; Zhang, Y.; Gao, X. Application of Moire Profilometry in Three-Dimensional Profile Reconstruction of Key Parts in Railway. Sensors 2022, 22, 2498. [Google Scholar] [CrossRef] [PubMed]
- Land, W.S.; Zhang, B.; Ziegert, J.; Davies, A. In-Situ Metrology System for Laser Powder Bed Fusion Additive Process. Procedia Manuf. 2015, 1, 393–403. [Google Scholar] [CrossRef]
- Zuo, C.; Tao, T.; Feng, S.; Huang, L.; Asundi, A.; Chen, Q. Micro Fourier Transform Profilometry (ΜFTP): 3D Imaging at 10,000 Fps. In Proceedings of the Conference on Lasers and Electro-Optics/Pacific Rim, Hong Kong, China, 29 July–3 August 2018; OSA: Hong Kong, China, 2018; p. Th2K.1. [Google Scholar]
- Fulvio, G.D.; Frontoni, E.; Mancini, A.; Zingaretti, P. Multi-Point Stereovision System for Contactless Dimensional Measurements. J Intell Robot Syst 2016, 81, 273–284. [Google Scholar] [CrossRef]
- Meza, J.; Contreras-Ortiz, S.H.; Romero, L.A.; Marrugo, A.G. Three-Dimensional Multimodal Medical Imaging System Based on Freehand Ultrasound and Structured Light. Opt. Eng. 2021, 60, 054106. [Google Scholar] [CrossRef]
- Chen, Q.; Han, M.; Wang, Y.; Chen, W. An Improved Circular Fringe Fourier Transform Profilometry. Sensors 2022, 22, 6048. [Google Scholar] [CrossRef]
- Laloum, D.; Printemps, T.; Lorut, F.; Bleuet, P. Correction of Absorption-Edge Artifacts in Polychromatic X-Ray Tomography in a Scanning Electron Microscope for 3D Microelectronics. Rev. Sci. Instrum. 2015, 86, 013703. [Google Scholar] [CrossRef]
- Zuo, C.; Huang, L.; Zhang, M.; Chen, Q.; Asundi, A. Temporal Phase Unwrapping Algorithms for Fringe Projection Profilometry: A Comparative Review. Opt. Lasers Eng. 2016, 85, 84–103. [Google Scholar] [CrossRef]
- Chen, C.; Gao, N.; Wang, X.; Zhang, Z. Adaptive Projection Intensity Adjustment for Avoiding Saturation in Three-Dimensional Shape Measurement. Opt. Commun. 2018, 410, 694–702. [Google Scholar] [CrossRef]
- Zhang, L.; Chen, Q.; Zuo, C.; Feng, S. High-Speed High Dynamic Range 3D Shape Measurement Based on Deep Learning. Opt. Lasers Eng. 2020, 134, 106245. [Google Scholar] [CrossRef]
- Zhang, W.; Li, W.; Yan, J.; Yu, L.; Pan, C. Adaptive Threshold Selection for Background Removal in Fringe Projection Profilometry. Opt. Lasers Eng. 2017, 90, 209–216. [Google Scholar] [CrossRef]
- Brakhage, P.; Heinze, M.; Notni, G.; Kowarschik, R. Influence of the Pixel Size of the Camera in 3D Measurements with Fringe Projection. In Optical Measurement Systems for Industrial Inspection III; Osten, W., Kujawinska, M., Creath, K., Eds.; SPIE: Munich, Germany, 2003; p. 478. [Google Scholar]
- Yue, H.; Dantanarayana, H.G.; Wu, Y.; Huntley, J.M. Reduction of Systematic Errors in Structured Light Metrology at Discontinuities in Surface Reflectivity. Opt. Lasers Eng. 2019, 112, 68–76. [Google Scholar] [CrossRef]
- Winiarski, B.; Gholinia, A.; Mingard, K.; Gee, M.; Thompson, G.; Withers, P.J. Correction of Artefacts Associated with Large Area EBSD. Ultramicroscopy 2021, 226, 113315. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Fu, Y.; Zhuan, Y.; Zhong, K.; Guan, B. High Dynamic Range Real-Time 3D Measurement Based on Fourier Transform Profilometry. Opt. Laser Technol. 2021, 138, 106833. [Google Scholar] [CrossRef]
- Zhang, H.; Zhang, Q.; Li, Y.; Liu, Y. High Speed 3D Shape Measurement with Temporal Fourier Transform Profilometry. Appl. Sci. 2019, 9, 4123. [Google Scholar] [CrossRef]
- Wang, Y.; Cai, J.; Liu, Y.; Chen, X.; Wang, Y. Motion-Induced Error Reduction for Phase-Shifting Profilometry with Phase Probability Equalization. Opt. Lasers Eng. 2022, 156, 107088. [Google Scholar] [CrossRef]
- Qian, J.; Tao, T.; Feng, S.; Chen, Q.; Zuo, C. Motion-Artifact-Free Dynamic 3D Shape Measurement with Hybrid Fourier-Transform Phase-Shifting Profilometry. Opt. Express 2019, 27, 2713. [Google Scholar] [CrossRef]
- Huang, P.S. Phase Error Compensation for a 3-D Shape Measurement System Based on the Phase-Shifting Method. Opt. Eng. 2007, 46, 063601. [Google Scholar] [CrossRef]
- Deng, J.; Li, J.; Feng, H.; Ding, S.; Xiao, Y.; Han, W.; Zeng, Z. Efficient Intensity-Based Fringe Projection Profilometry Method Resistant to Global Illumination. Opt. Express 2020, 28, 36346–36360. [Google Scholar] [CrossRef]
- Liao, Y.-H.; Xu, M.; Zhang, S. Digital Image Correlation Assisted Absolute Phase Unwrapping. Opt. Express 2022, 30, 33022. [Google Scholar] [CrossRef]
- Fei, L.; Jiaxin, L.; Junlin, L.; Chunqiao, H. Full-Frequency Phase Unwrapping Algorithm Based on Multi-Frequency Heterodyne Principle. Laser Optoelectron. Prog. 2019, 56, 011202. [Google Scholar] [CrossRef]
- Reich, C.; Ritter, R.; Thesing, J. White Light Heterodyne Principle for 3D-Measurement; Loffeld, O., Ed.; SPIE: Munich, Germany, 1997; pp. 236–244. [Google Scholar]
- Feng, S.; Zuo, C.; Zhang, L.; Tao, T.; Hu, Y.; Yin, W.; Qian, J.; Chen, Q. Calibration of Fringe Projection Profilometry: A Comparative Review. Opt. Lasers Eng. 2021, 143, 106622. [Google Scholar] [CrossRef]
- Zhang, Z. A Flexible New Technique for Camera Calibration. IEEE Trans. Pattern Anal. Mach. Intell. 2000, 22, 1330–1334. [Google Scholar] [CrossRef]
- Huang, P.S. Novel Method for Structured Light System Calibration. Opt. Eng. 2006, 45, 083601. [Google Scholar] [CrossRef]
- Zhang, S. Flexible and High-Accuracy Method for Uni-Directional Structured Light System Calibration. Opt. Lasers Eng. 2021, 143, 106637. [Google Scholar] [CrossRef]
- Takeda, M.; Mutoh, K. Fourier Transform Profilometry for the Automatic Measurement of 3-D Object Shapes. Appl. Opt. 1983, 22, 3977. [Google Scholar] [CrossRef]
- Wang, J.; Su, R.; Leach, R.; Lu, W.; Zhou, L.; Jiang, X. Resolution Enhancement for Topography Measurement of High-Dynamic-Range Surfaces via Image Fusion. Opt. Express 2018, 26, 34805–34819. [Google Scholar] [CrossRef] [PubMed]




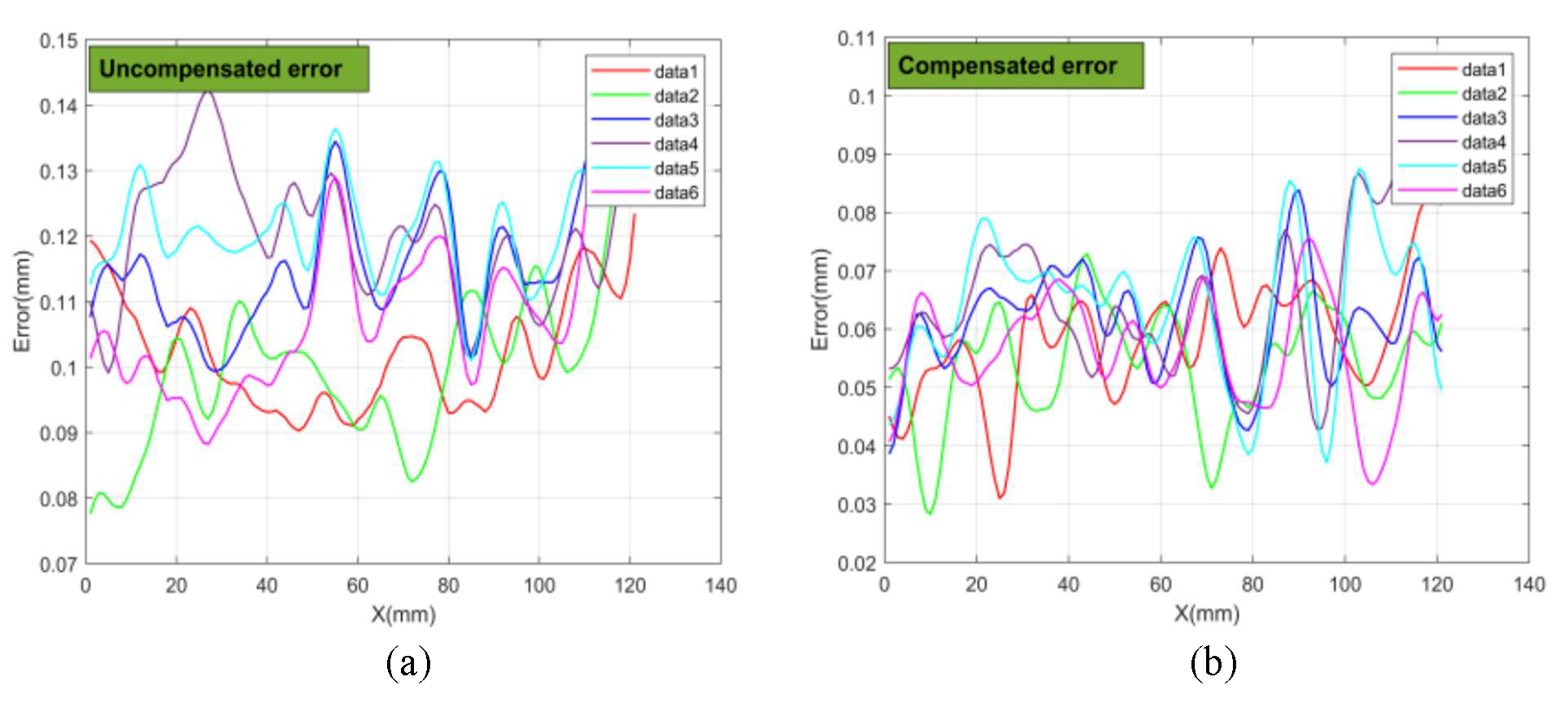
| Uncompensated (RMSE) | Compensated (RMSE) | |
|---|---|---|
| 1 | 0.1654 | 0.0943 |
| 2 | 0.1695 | 0.0898 |
| 3 | 0.1598 | 0.0924 |
| 4 | 0.1563 | 0.0793 |
| 5 | 0.1752 | 0.0921 |
| 6 | 0.1799 | 0.1011 |
| Mean | 0.1677 | 0.0915 |
| Uncompensated (RMSE) | Compensated (RMSE) | |
|---|---|---|
| 1 | 0.1022 | 0.0598 |
| 2 | 0.1208 | 0.0550 |
| 3 | 0.1168 | 0.0624 |
| 4 | 0.1217 | 0.0676 |
| 5 | 0.1213 | 0.0658 |
| 6 | 0.1094 | 0.0576 |
| Mean | 0.1154 | 0.0614 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, B.; Xu, Y.; Zhang, C.; Tang, J.; Tang, D.; Kong, C.; Jin, J. An Optimized Error Compensation Method for Phase Measurement Profilometry. Photonics 2023, 10, 1036. https://doi.org/10.3390/photonics10091036
Guo B, Xu Y, Zhang C, Tang J, Tang D, Kong C, Jin J. An Optimized Error Compensation Method for Phase Measurement Profilometry. Photonics. 2023; 10(9):1036. https://doi.org/10.3390/photonics10091036
Chicago/Turabian StyleGuo, Benjun, Yuanping Xu, Chaolong Zhang, Jianfeng Tang, Dan Tang, Chao Kong, and Jin Jin. 2023. "An Optimized Error Compensation Method for Phase Measurement Profilometry" Photonics 10, no. 9: 1036. https://doi.org/10.3390/photonics10091036
APA StyleGuo, B., Xu, Y., Zhang, C., Tang, J., Tang, D., Kong, C., & Jin, J. (2023). An Optimized Error Compensation Method for Phase Measurement Profilometry. Photonics, 10(9), 1036. https://doi.org/10.3390/photonics10091036





