Sensitivity Modal Analysis of Long Reflective Multimode Interferometer for Small Angle Detection and Temperature
Abstract
1. Introduction
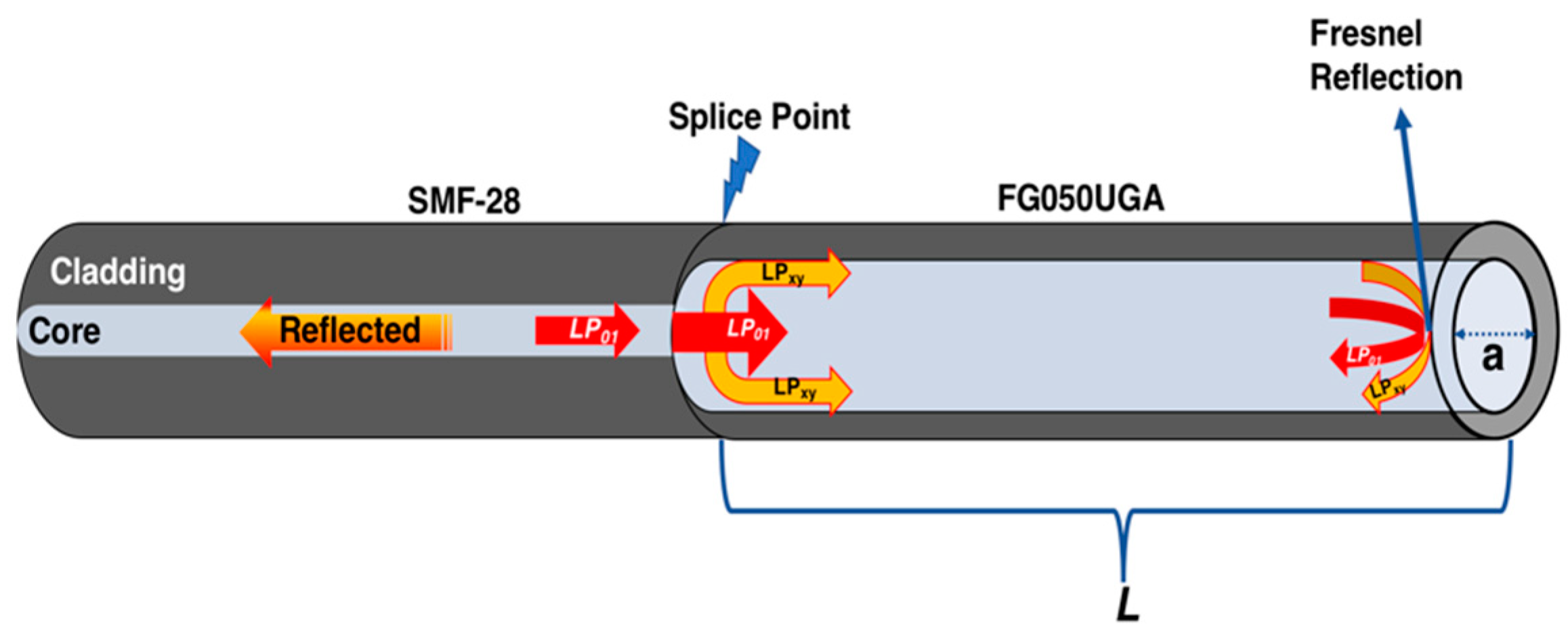
2. Principle of Operation
3. Experimental Setup
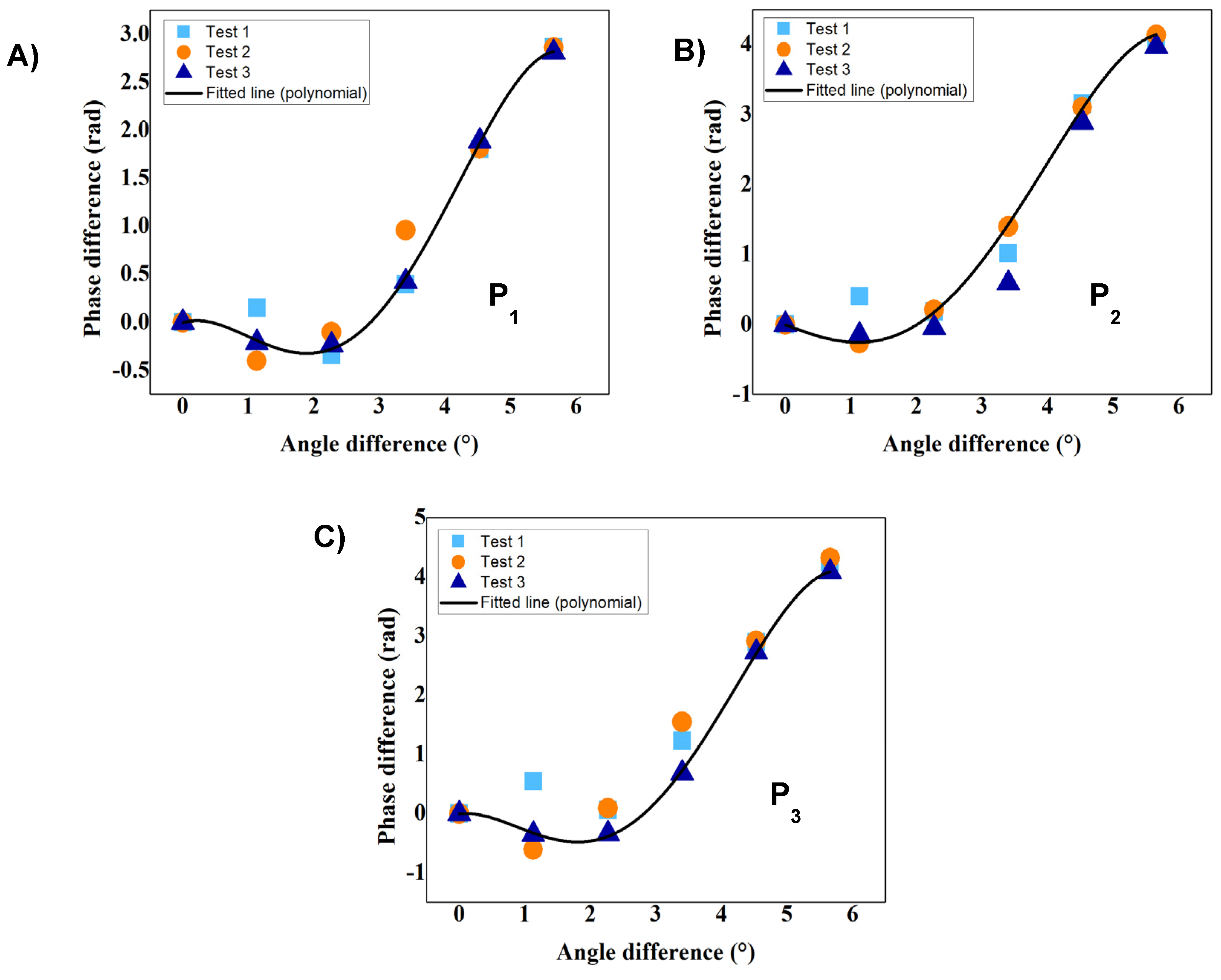
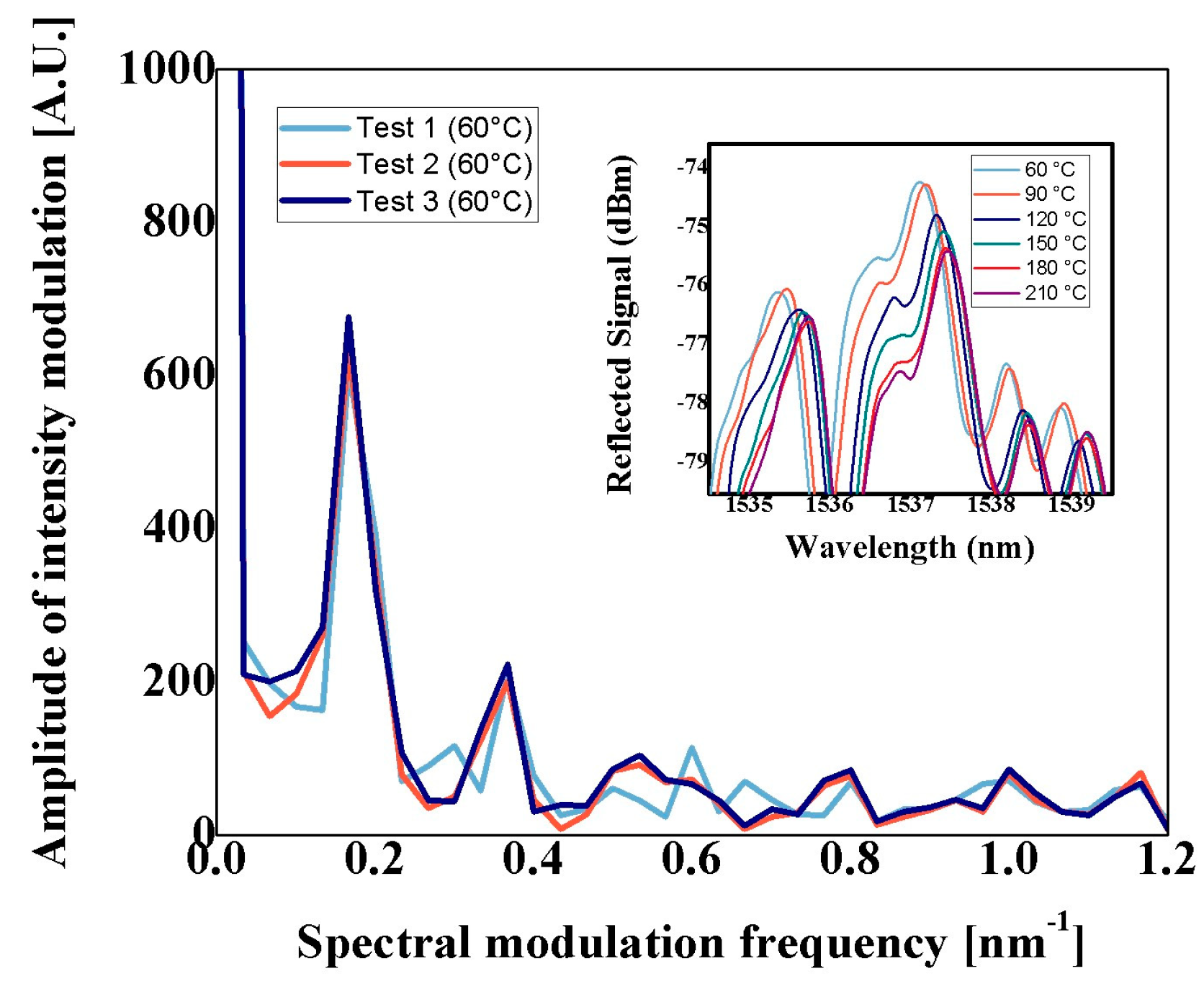
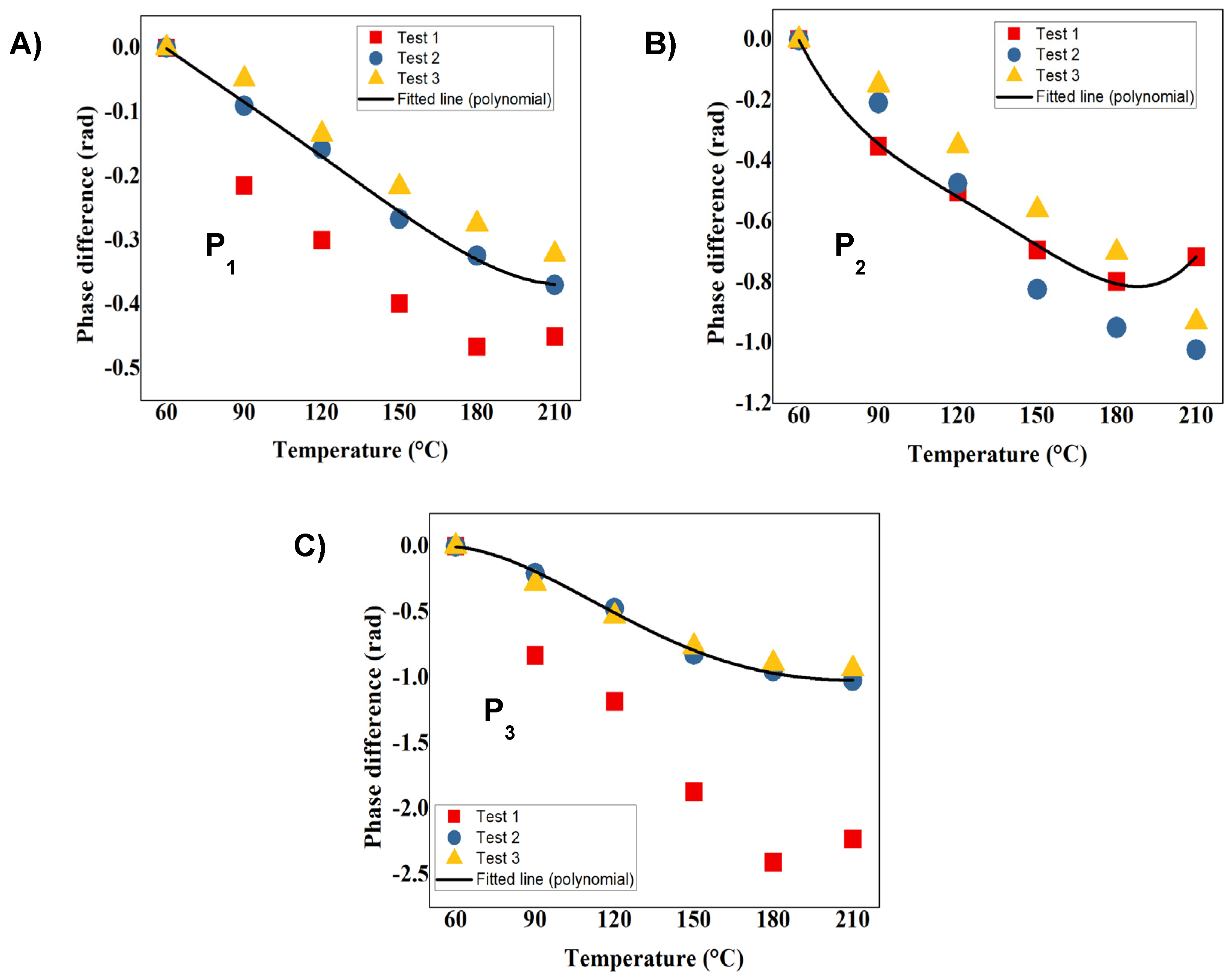
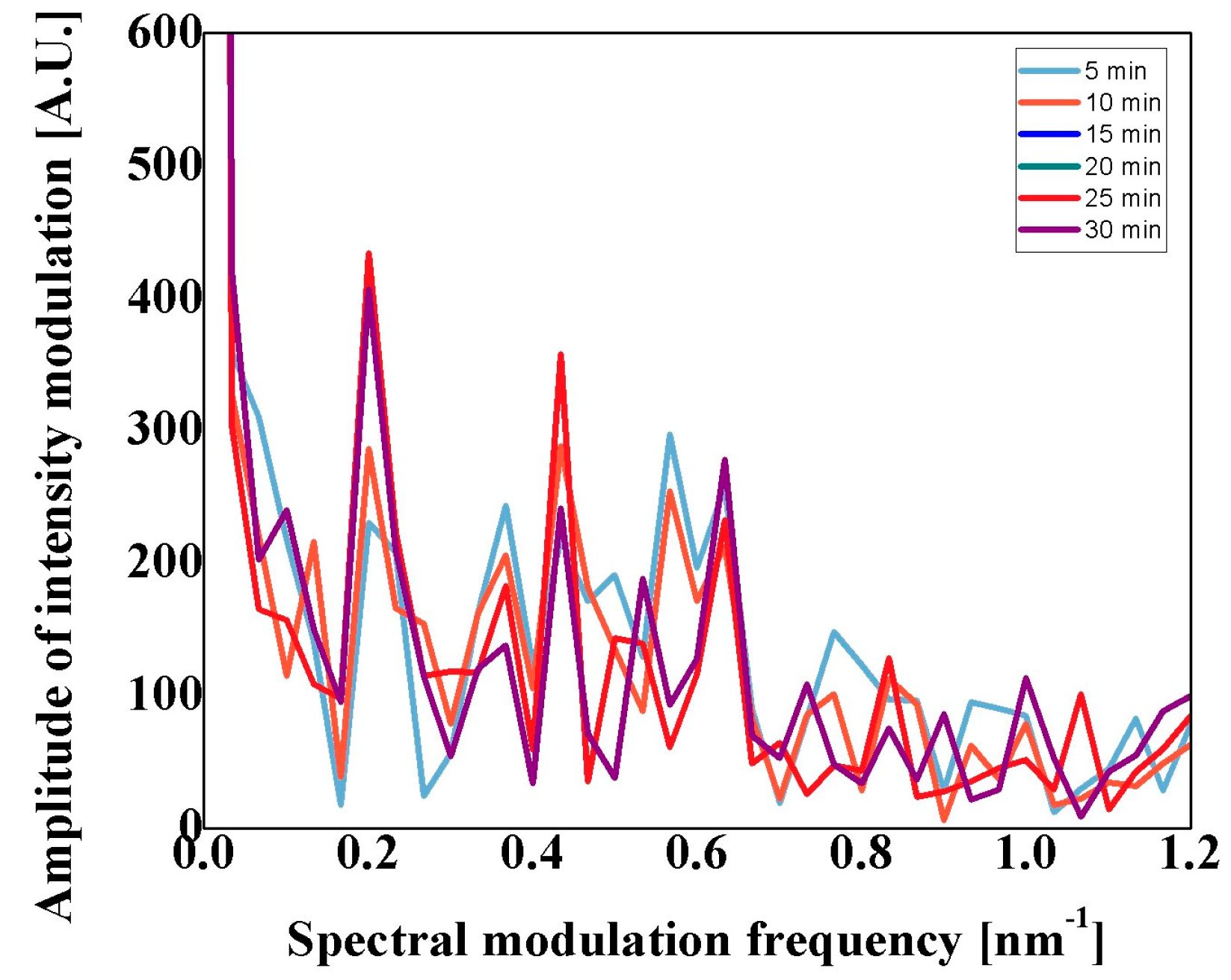
4. Results
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Mehta, A.; Mohammed, W.; Johnson, E.G. Multimode interference-based fiber-optic displacement sensor. IEEE Photon. Technol. Lett. 2003, 15, 1129–1131. [Google Scholar] [CrossRef]
- Wang, Q.; Farrell, G. All-fiber multimode-interference-based refractometer sensor: Proposal and design. Opt. Lett. 2006, 31, 317–319. [Google Scholar] [CrossRef]
- Wang, K.; Dong, X.; Kohler, M.H.; Kienle, P.; Bian, Q.; Jakobi, M.; Koch, A.W. Advances in Optical Fiber Sensors Based on Multimode Interference (MMI): A Review. IEEE Sens. J. 2020, 21, 132–142. [Google Scholar] [CrossRef]
- Zhou, X.; Chen, K.; Mao, X.; Peng, W.; Yu, Q. A reflective fiber-optic refractive index sensor based on multimode interference in a coreless silica fiber. Opt. Commun. 2015, 340, 50–55. [Google Scholar] [CrossRef]
- Wang, K.; Dong, X.; Kienle, P.; Fink, M.; Kurz, W.; Köhler, M.H.; Jakobi, M.; Koch, A.W. Optical Fiber Sensor for Temperature and Strain Measurement Based on Multimode Interference and Square-Core Fiber. Micromachines 2021, 12, 1239. [Google Scholar] [CrossRef] [PubMed]
- Guzmán-Sepúlveda, J.R.; Guzmán-Cabrera, R.; Castillo-Guzmán, A.A. Optical Sensing Using Fiber-Optic Multimode Interference Devices: A Review of Nonconventional Sensing Schemes. Sensors 2021, 21, 1862. [Google Scholar] [CrossRef]
- Wang, P.; Zhao, H.; Wang, X.; Farrell, G.; Brambilla, G. A Review of Multimode Interference in Tapered Optical Fibers and Related Applications. Sensors 2018, 18, 858. [Google Scholar] [CrossRef]
- Huang, J.; Wang, W.; Xu, X.; Zhou, S.; Tang, C.; Gao, F.; Chen, J. Ultraviolet ultranarrow second-order magnetic plasmon induced reflection of lifted 3D metamaterials for slow light and optical sensing. Results Phys. 2023, 47, 106354. [Google Scholar] [CrossRef]
- Wang, S.; Yang, Y.; Mohanty, L.; Jin, R.-B.; Lu, P. Ultrasensitive Fiber Optic Inclinometer Based on Dynamic Vernier Effect Using Push–Pull Configuration. IEEE Trans. Instrum. Meas. 2022, 71, 3196445. [Google Scholar] [CrossRef]
- Zhang, C.; Zhang, S.; Cao, J. In-place fiber-optic inclinometer based on a vertical cantilever beam and dual FBGs. Opt. Laser Technol. 2023, 159, 108933. [Google Scholar] [CrossRef]
- Lin, W.; Zhou, S.; Shao, L.; Vai, M.I.; Shum, P.-P.; Xu, W.; Zhao, F.; Yu, F.; Liu, Y.; Liu, Y.; et al. A Temperature Independent Inclinometer Based on a Tapered Fiber Bragg Grating in a Fiber Ring Laser. Sensors 2021, 21, 2892. [Google Scholar] [CrossRef]
- Liu, S.; Liu, N.; Hou, M.; Guo, J.; Li, Z.; Lu, P. Direction-independent fiber inclinometer based on simplified hollow core photonic crystal fiber. Opt. Lett. 2013, 38, 449–451. [Google Scholar] [CrossRef] [PubMed]
- Lee, C.-L.; Shih, W.-C.; Hsu, J.-M.; Horng, J.-S. Asymmetrical dual tapered fiber Mach-Zehnder interferometer for fiber-optic directional tilt sensor. Opt. Express 2014, 22, 24646–24654. [Google Scholar] [CrossRef]
- Wang, H.; Zheng, L.; Zhang, J.; Liu, J. Orientation-dependent fiber-optic inclinometer based on core-offset michelson interferometer. Sci. Rep. 2022, 12, 7849. [Google Scholar] [CrossRef] [PubMed]
- Da Silveira, C.R.; Jorge, P.A.S.; Costa, J.W.A.; Giraldi, M.T.M.R.; Santos, J.L.; Frazão, O. In-fiber Michelson interferometer inclinometer. In Proceedings of the 24th International Conference on Optical Fibre Sensors, Curitiba, Brazil, 28 September–2 October 2015; pp. 201–204. [Google Scholar] [CrossRef]
- Li, J.; Qiao, X.; Rong, Q.; Sun, A. A Compact Fiber Inclinometer Using a Thin-Core Fiber with Incorporated an Air-Gap Microcavity Fiber Interferometer. Sensors 2016, 16, 92. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Ma, X.; Chen, K.; Wang, E.; Yu, Z.; Yu, Q. A high-resolution dynamic fiber-optic inclinometer. Sens. Actuators A Phys. 2018, 283, 305–312. [Google Scholar] [CrossRef]
- Su, D.; Qiao, X.; Yang, H.; Rong, Q.; Bai, Z.; Wang, Y.; Feng, Z. Temperature-Independent Fiber Inclinometer Based on Orthogonally Polarized Modes Coupling Using a Polarization-Maintaining Fiber Bragg Grating. Sensors 2014, 14, 20930–20939. [Google Scholar] [CrossRef]
- Zheng, D.; Cai, Z.; Floris, I.; Madrigal, J.; Pan, W.; Zou, X.; Sales, S. Temperature-insensitive optical tilt sensor based on a single eccentric-core fiber Bragg grating. Opt. Lett. 2019, 44, 5570–5573. [Google Scholar] [CrossRef]
- Shao, L.-Y.; Albert, J. Compact fiber-optic vector inclinometer. Opt. Lett. 2010, 35, 1034–1036. [Google Scholar] [CrossRef]
- Amaral, L.M.N.; Frazao, O.; Santos, J.L.; Ribeiro, A.B.L. Fiber-Optic Inclinometer Based on Taper Michelson Interferometer. IEEE Sens. J. 2011, 11, 1811–1814. [Google Scholar] [CrossRef]
- Li, C.-M.; Hsiao, Y.-L.; Lee, C.-F.; Lee, C.-L. In-fiber optical airflow sensor based on a tapered fiber interferometric cantilever. In Proceedings of the 17th Opto-Electronics and Communications Conference, Busan, Republic of Korea, 2–6 July 2012. [Google Scholar] [CrossRef]
- Wahl, M.S.; Wilhelmsen, Ø.; Hjelme, D.R. Addressing Challenges in Fabricating Reflection-Based Fiber Optic Interferometers. Sensors 2019, 19, 4030. [Google Scholar] [CrossRef]
- Silva, S.; Frazão, O.; Santos, J.; Malcata, F. A reflective optical fiber refractometer based on multimode interference. Sens. Actuators B Chem. 2012, 161, 88–92. [Google Scholar] [CrossRef]
- Xu, W.; Shen, Y.; Yu, C.; Dong, B.; Zhao, W.; Wang, Y. Long modal interference in multimode fiber and its application in vital signs monitoring. Opt. Commun. 2020, 474, 126100. [Google Scholar] [CrossRef]
- Jauregui-Vazquez, D.; Haus, J.W.; Negari, A.B.H.; Sierra-Hernandez, J.M.; Hansen, K. Bitapered fiber sensor: Signal analysis. Sens. Actuators B Chem. 2015, 218, 105–110. [Google Scholar] [CrossRef]
- Mina, D.G.; Haus, J.W.; Chong, A.; Khanolkar, A.; Sarangan, A.; Hansen, K. Bi-tapered fiber sensor using visible to near infrared light. Sens. Actuators A Phys. 2017, 263, 285–290. [Google Scholar] [CrossRef]
- Li, E.; Wang, X.; Zhang, C. Fiber-optic temperature sensor based on interference of selective higher-order modes. Appl. Phys. Lett. 2006, 89, 091119. [Google Scholar] [CrossRef]
- Li, E. Sensitivity-Enhanced Fiber-Optic Strain Sensor Based on Interference of Higher Order Modes in Circular Fibers. IEEE Photon-Technol. Lett. 2007, 19, 1266–1268. [Google Scholar] [CrossRef]
- Gutierrez-Rivera, M.; Jauregui-Vazquez, D.; Sierra-Hernandez, J.; Garcia-Mina, D.; Lopez-Dieguez, Y.; Estudillo-Ayala, J.; Rojas-Laguna, R. Low-pressure fiber-optic sensor by polyester Fabry-Perot cavity and its phase signal processing analysis. Sens. Actuators A Phys. 2020, 315, 112338. [Google Scholar] [CrossRef]
- Guzman-Sepulveda, J.R. Temporal coherence characteristics of fiber optics multimode interference devices. Optik 2023, 283, 170895. [Google Scholar] [CrossRef]
- Husain, M.A.; Hasan, Z.H. Reduced Equations of Slope-Deflection Method in Structural Analysis. Int. J. Appl. Mech. Eng. 2021, 26, 51–62. [Google Scholar] [CrossRef]
- Li, X.; Prinz, F. Analytical and Experimental Study on Noncontact Sensing with Embedded Fiber-Optic Sensors in Rotating Metal Parts. J. Light. Technol. 2004, 22, 1720–1727. [Google Scholar] [CrossRef]
- Cardoso, V.H.R.; Caldas, P.; Giraldi, M.T.R.; Fernandes, C.S.; Frazão, O.; Costa, J.C.W.A.; Santos, J.L. A Simple Optical Sensor Based on Multimodal Interference Superimposed on Additive Manufacturing for Diameter Measurement. Sensors 2022, 22, 4560. [Google Scholar] [CrossRef] [PubMed]
- Guo, T.; Liu, F.; Guan, B.-O.; Albert, J. Polarimetric multi-mode tilted fiber grating sensors. Opt. Express 2014, 22, 7330–7336. [Google Scholar] [CrossRef] [PubMed]




| Optical Fiber Structure | Sensitivity of Angle Detection | Angle Detection Range | Sensitivity of Temperature | Temperature Range | Ref. |
|---|---|---|---|---|---|
| Two Fabry-Perot in parallel | 0.909 nm/° to 35.96 nm/° | ±2.5° ±0.2° | x | x | [9] |
| Vertical cantilever beam and dual FBGs | ∼ 0.1 nm/° | ± 30 ° | x | x | [10] |
| Tapered fiber Bragg grating | 0.849 dBm/° and 0.583 dBm/° | 0° to 90° | 12 pm/°C | 0 °C to 90 °C | [11] |
| Multimode Interference using Square-Core Fiber | x | x | −15.3 pm/°C | 30 °C to 80 °C | [5] |
| Michelson | 0.55 nm/° | 0° to 50° | x | x | [14] |
| Reflective Multimode Interferometer (RMMI) | 0.75 rad/°, 1.19 rad/° and 1.52 rad/° | 0° to 3.4° | 0.003 rad/°C, 0.0062 rad/°C and 0.0065 rad/°C | 60 °C to 210 °C | This work |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lozano-Hernandez, T.; Estudillo-Ayala, J.M.; Jauregui-Vazquez, D.; Sierra-Hernandez, J.M.; Rojas-Laguna, R. Sensitivity Modal Analysis of Long Reflective Multimode Interferometer for Small Angle Detection and Temperature. Photonics 2023, 10, 706. https://doi.org/10.3390/photonics10070706
Lozano-Hernandez T, Estudillo-Ayala JM, Jauregui-Vazquez D, Sierra-Hernandez JM, Rojas-Laguna R. Sensitivity Modal Analysis of Long Reflective Multimode Interferometer for Small Angle Detection and Temperature. Photonics. 2023; 10(7):706. https://doi.org/10.3390/photonics10070706
Chicago/Turabian StyleLozano-Hernandez, Tania, Julian M. Estudillo-Ayala, Daniel Jauregui-Vazquez, Juan M. Sierra-Hernandez, and Roberto Rojas-Laguna. 2023. "Sensitivity Modal Analysis of Long Reflective Multimode Interferometer for Small Angle Detection and Temperature" Photonics 10, no. 7: 706. https://doi.org/10.3390/photonics10070706
APA StyleLozano-Hernandez, T., Estudillo-Ayala, J. M., Jauregui-Vazquez, D., Sierra-Hernandez, J. M., & Rojas-Laguna, R. (2023). Sensitivity Modal Analysis of Long Reflective Multimode Interferometer for Small Angle Detection and Temperature. Photonics, 10(7), 706. https://doi.org/10.3390/photonics10070706





