Meshless Local Petrov–Galerkin Formulation of Inverse Stefan Problem via Moving Least Squares Approximation
Abstract
1. Introduction
2. Statement of the Problem
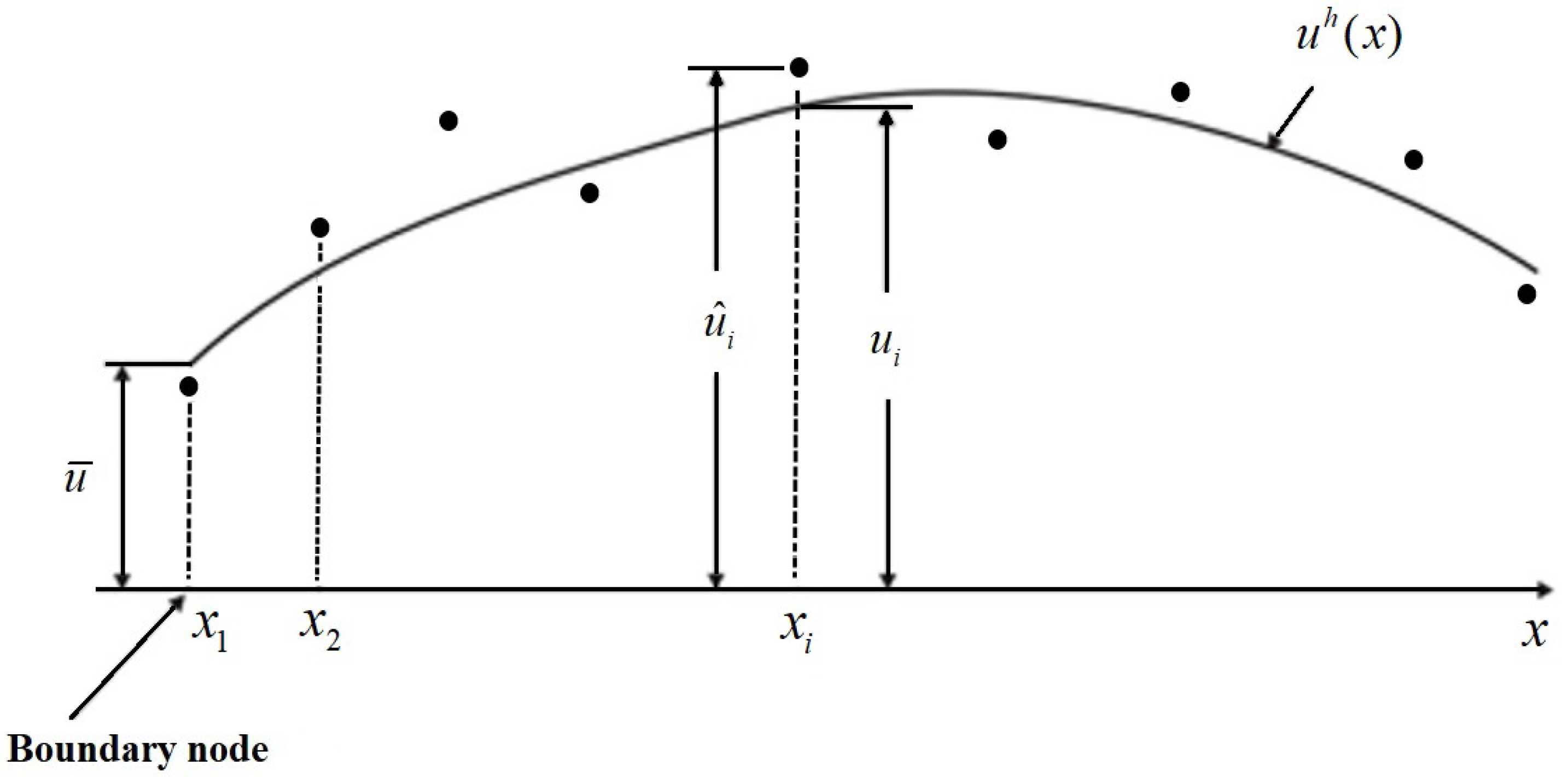
3. The MLS Approximation Technique
4. The Time Discretization of the Problem
5. The Local Weak Form Formulation
6. MLPG Discretization
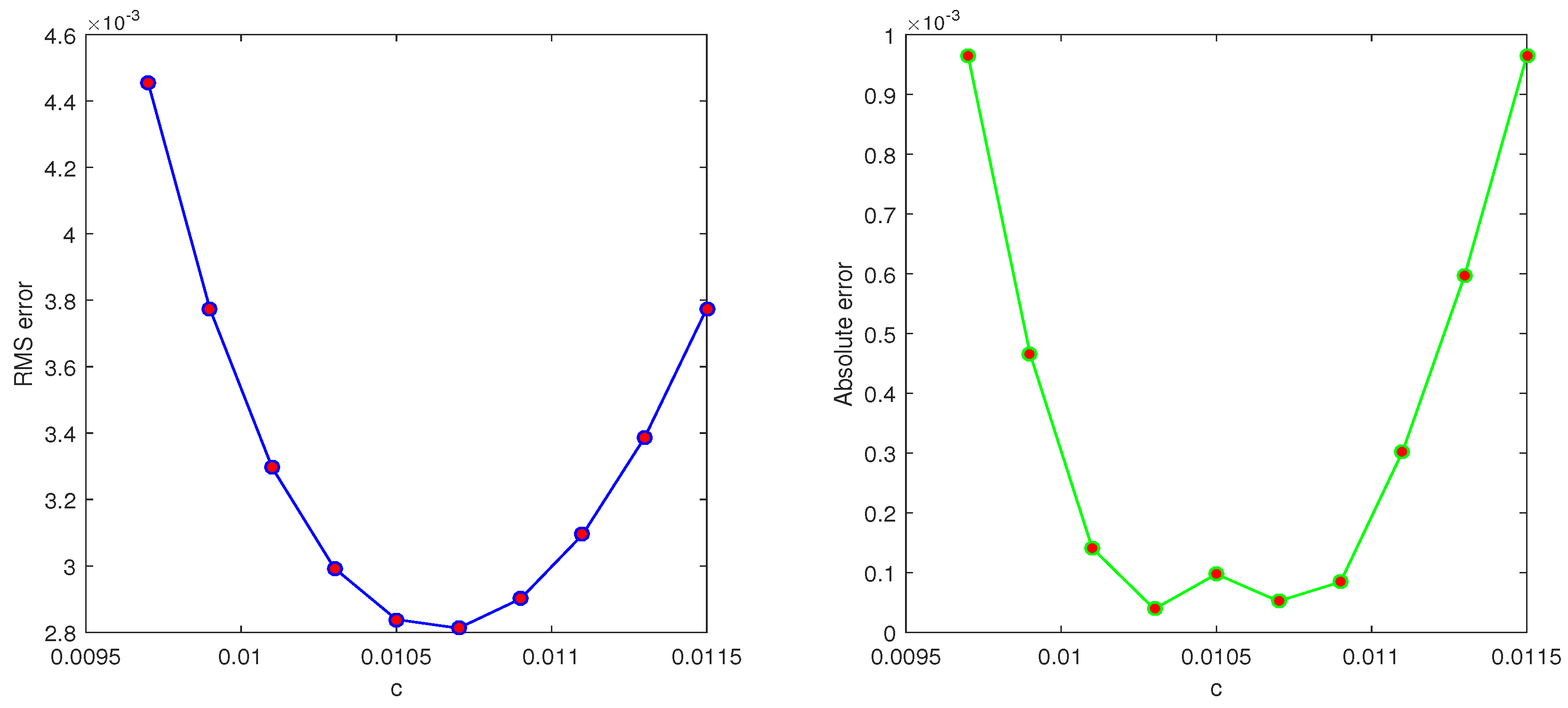
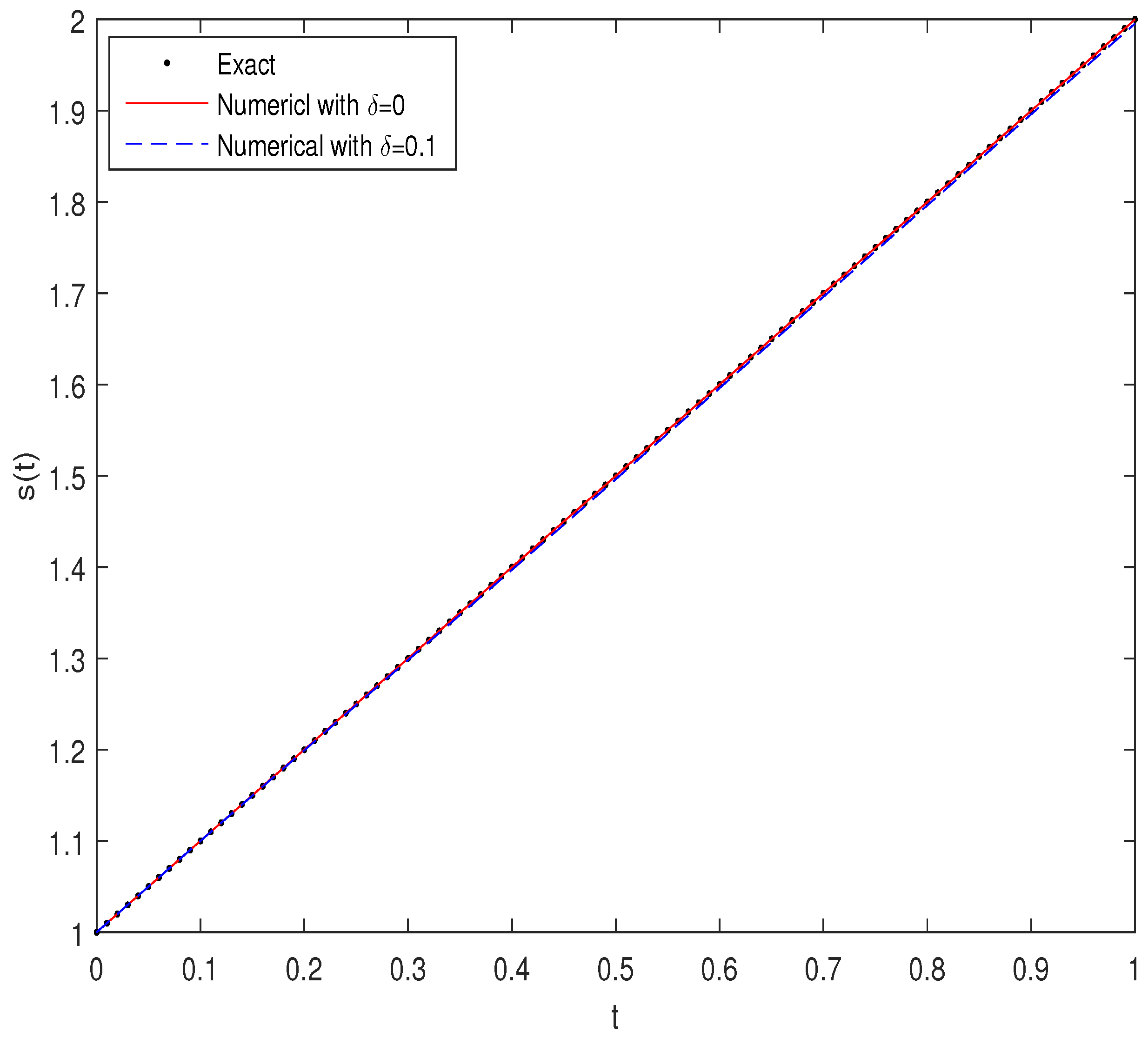
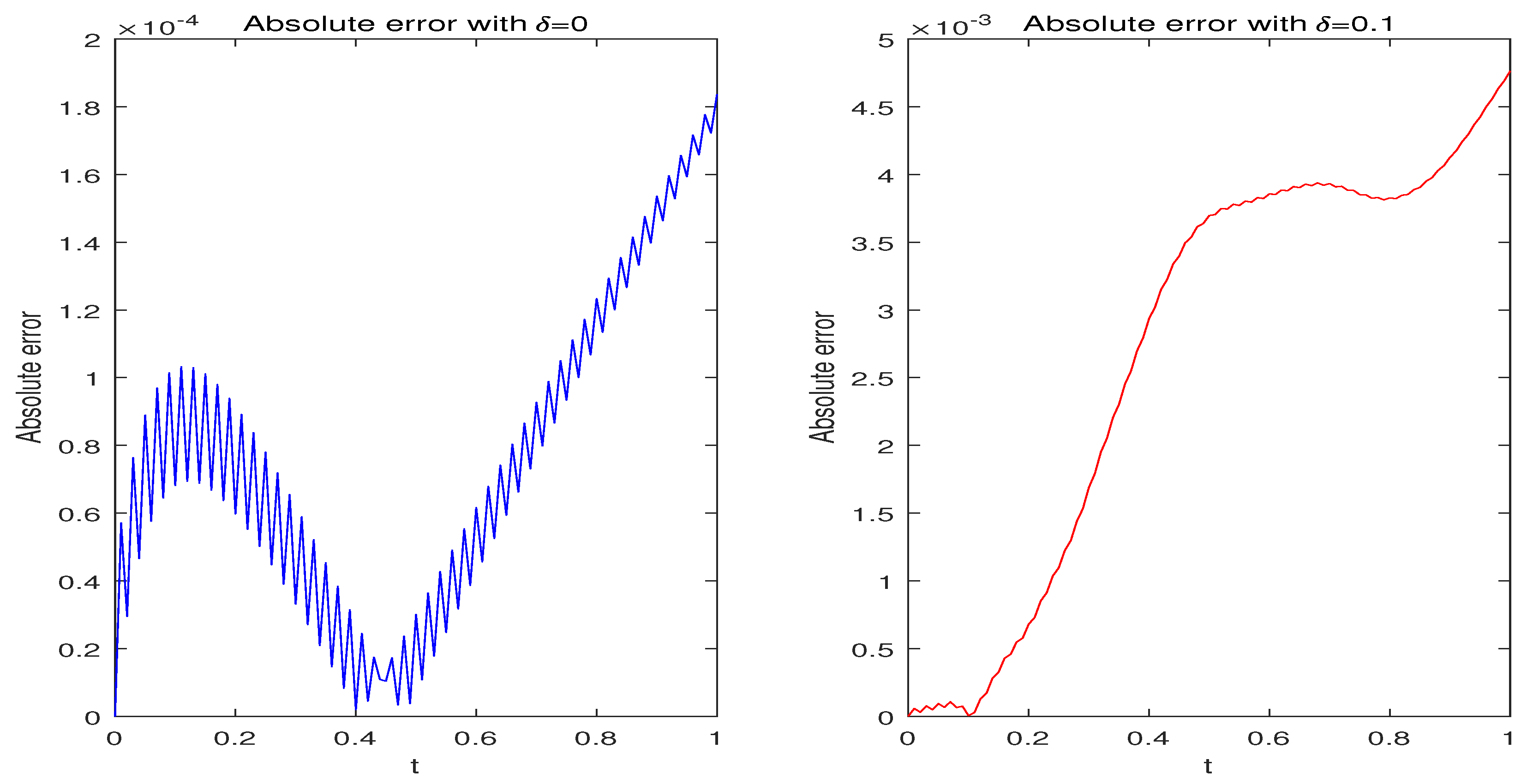
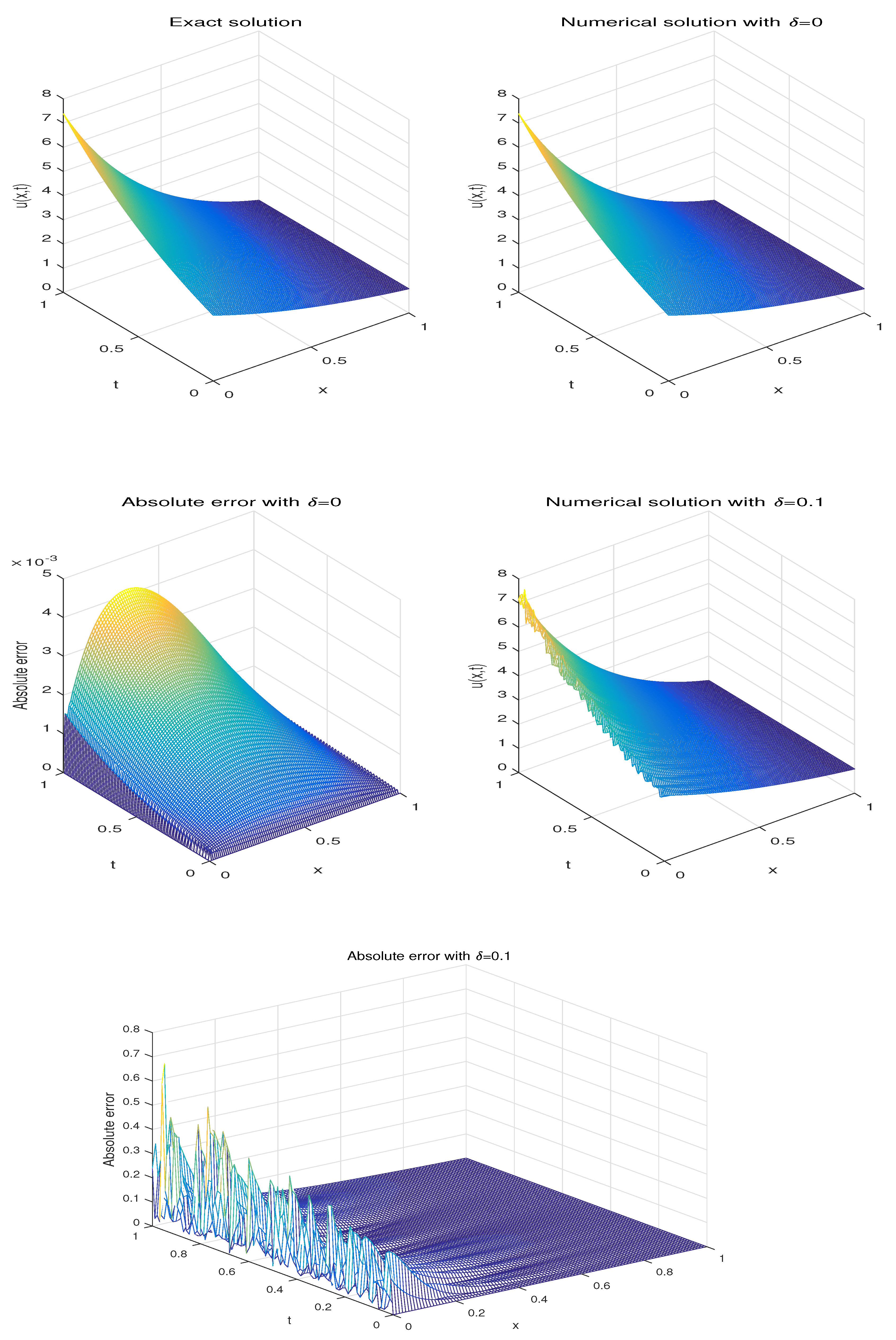
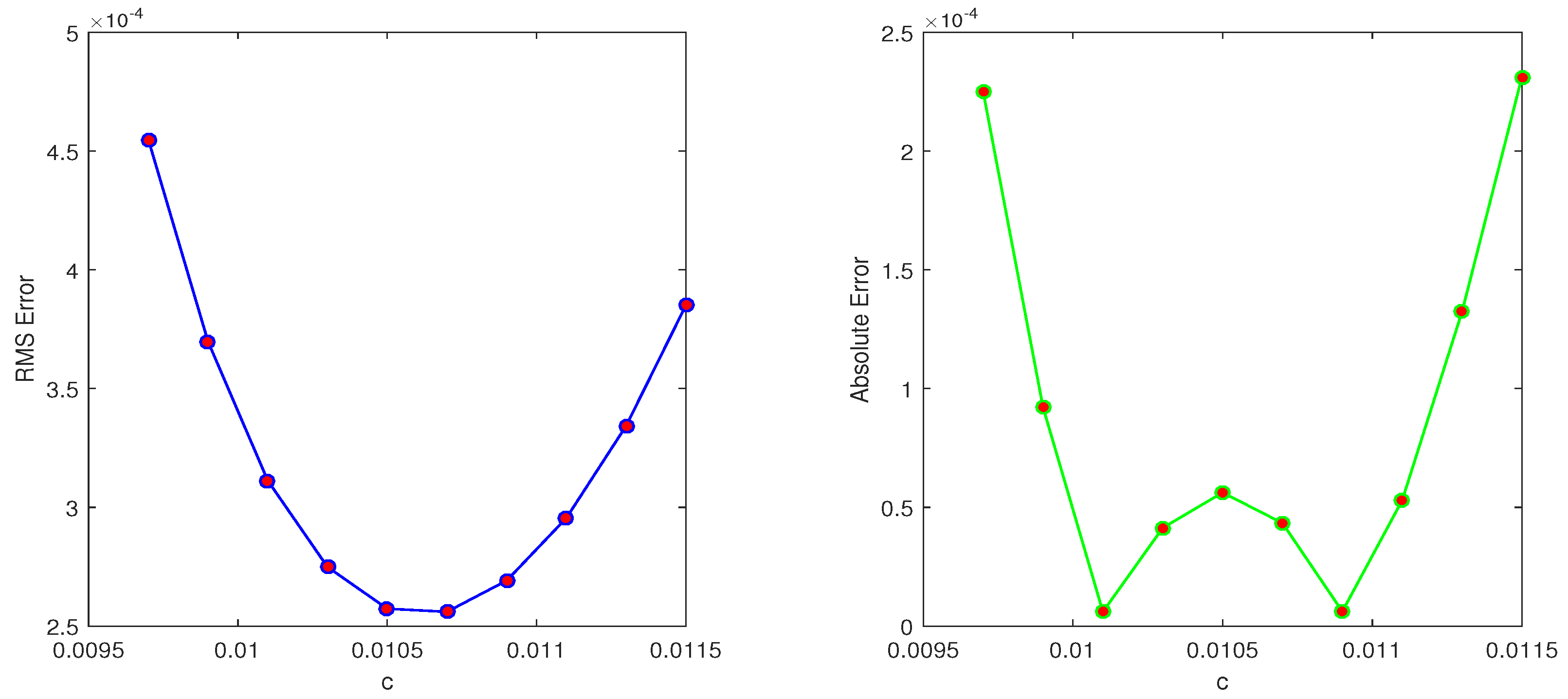
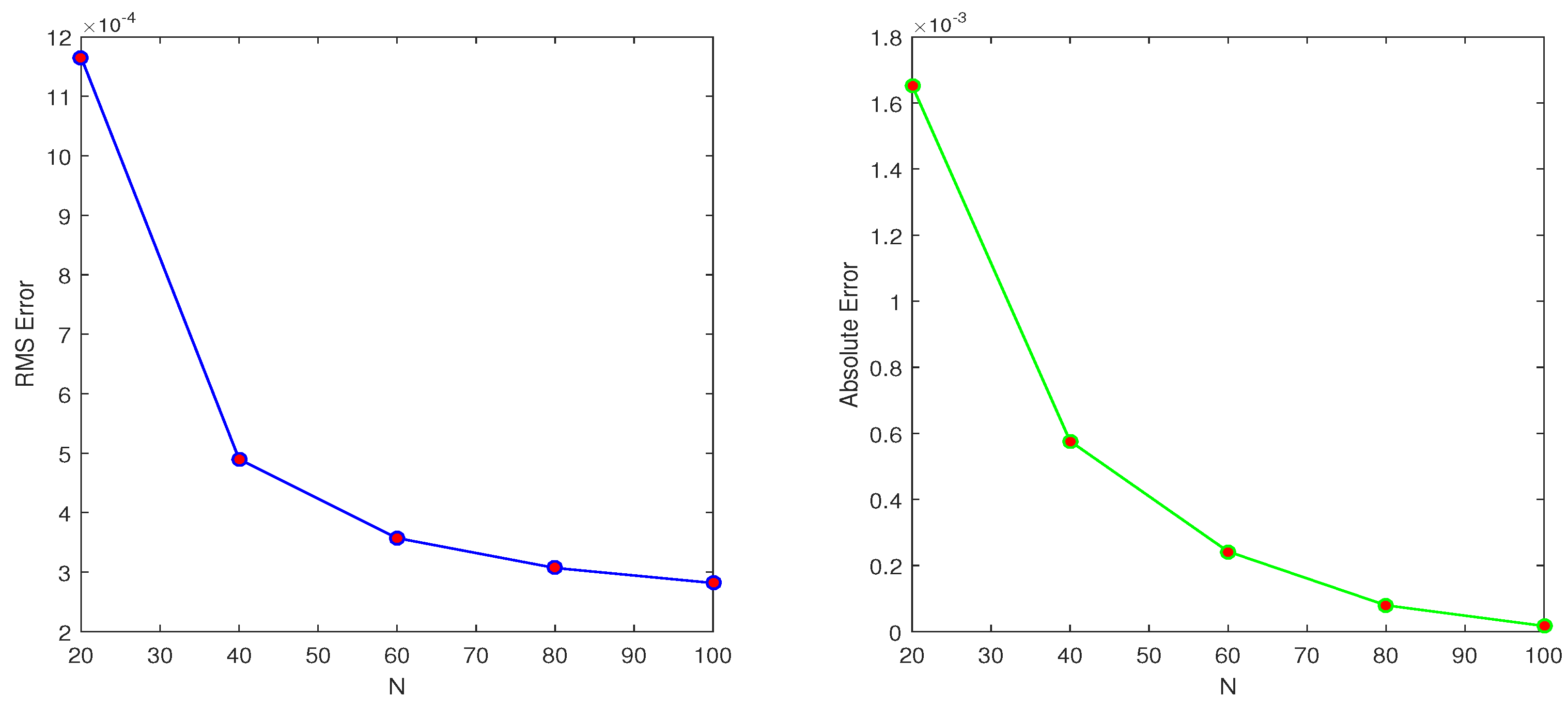
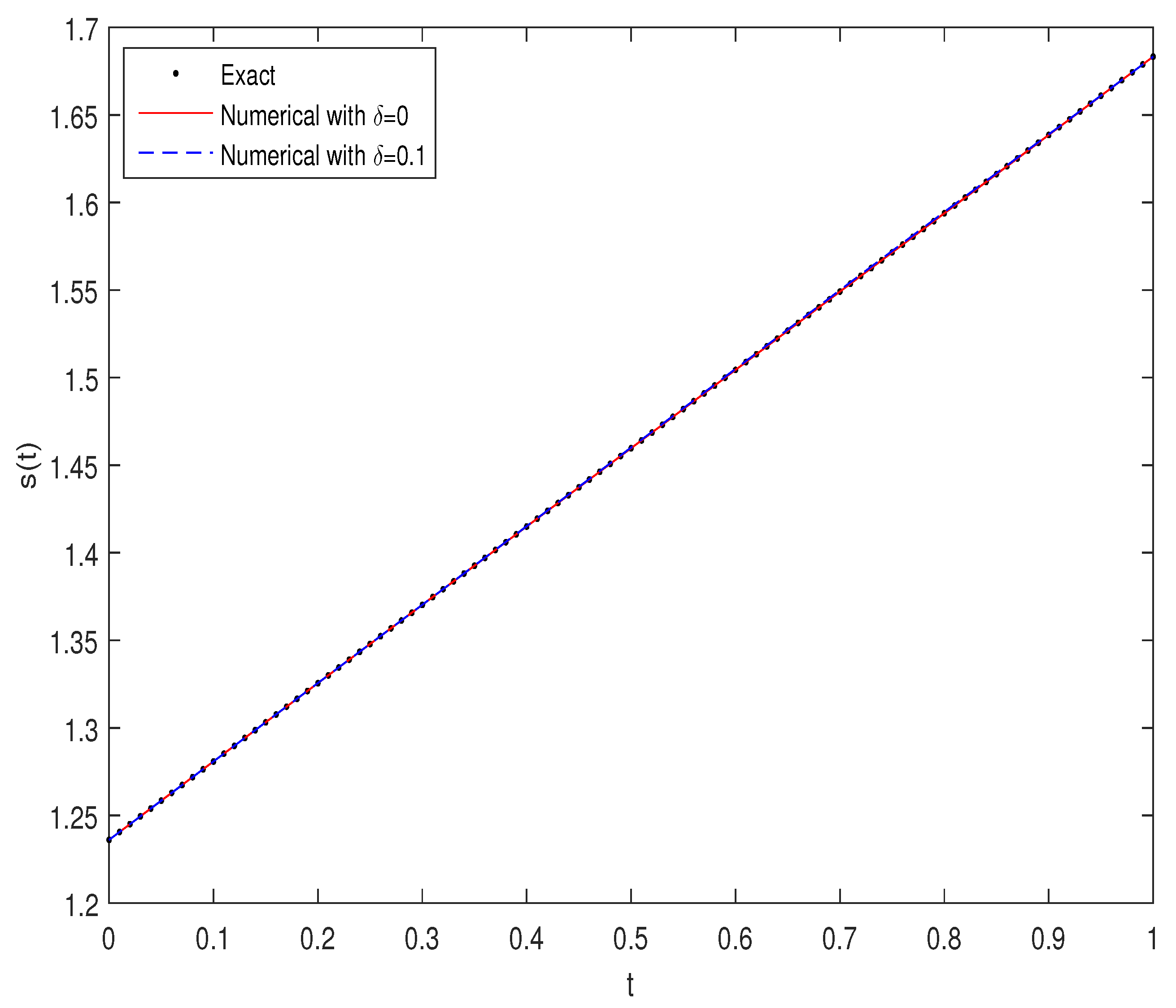
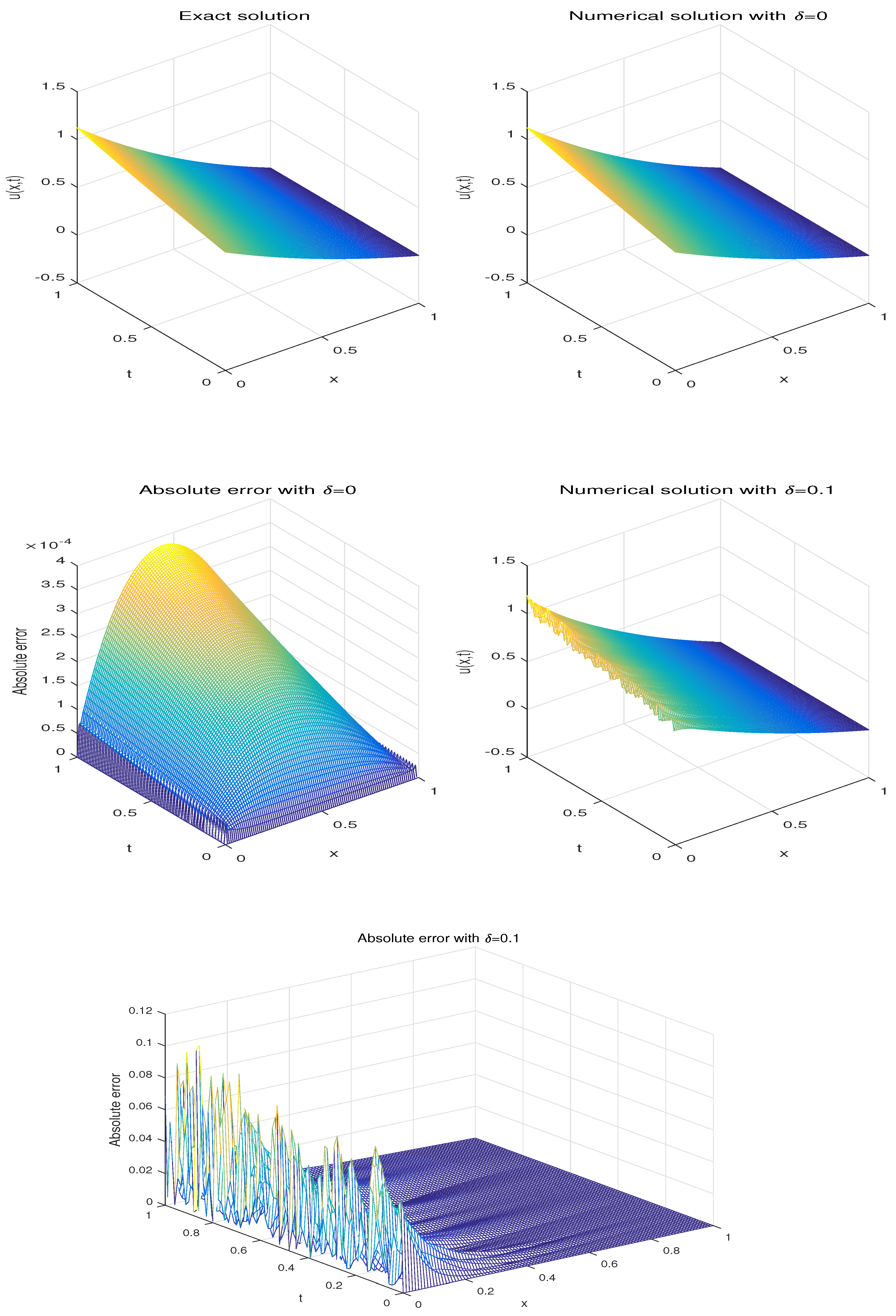
7. Numerical Experiments
8. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Crank, J. Free and Moving Boundary Problems; Clarendon Press: Oxford, UK, 1984. [Google Scholar]
- Gupta, S. The Classical Stefan Problem. Basic Concepts, Modelling and Analysis; Elsevier: Amsterdam, The Netherlands, 2003. [Google Scholar]
- Meirmanov, A. The Stefan Problem; Walter de Gruyter: Berlin, Germany, 1992. [Google Scholar]
- Ozisik, M. Heat Conduction; Wiley: New York, NY, USA, 1980. [Google Scholar]
- Rubinstein, L. The Stefan Problem; AMS: Providence, RI, USA, 1971. [Google Scholar]
- Hetmaniok, E.; Slota, D.; Wituła, R.; Zielonka, A. Solution of the One-Phase Inverse Stefan Problem by Using the Homotopy Analysis Method. Appl. Math. Model. 2015, 39, 6793–6805. [Google Scholar] [CrossRef]
- Onyejekwe, O.N. The Solution of One-Phase Inverse Stefan Problem by Homotopy Analysis Method. Appl. Math. Sci. 2014, 8, 2635–2644. [Google Scholar] [CrossRef]
- Liu, C.S. Solving two typical inverse Stefan problems by using the Lie-group shooting method. Int. J. Heat Mass Transf. 2011, 54, 1941–1949. [Google Scholar] [CrossRef]
- Zabaras, N.; Ruan, Y. A deforming finite element method analysis of inverse Stefan problems. Int. J. Numer. Methods Eng. 1989, 28, 295–313. [Google Scholar] [CrossRef]
- Slota, D. Direct and inverse one-phase Stefan problem solved by the variational iteration method. Comput. Math. Appl. 2007, 54, 1139–1146. [Google Scholar] [CrossRef][Green Version]
- Grzymkowski, R.; Slota, D. Stefan problem solved by Adomian decomposition method. Int. J. Comput. Math. 2005, 82, 851–856. [Google Scholar] [CrossRef]
- Grzymkowski, R.; Slota, D. One-phase inverse Stefan problem solved by Adomian decomposition method. Comput. Math. Appl. 2006, 51, 33–40. [Google Scholar] [CrossRef][Green Version]
- Slota, D. The application of the homotopy perturbation method to one-phase inverse Stefan problem. Int. J. Comput. Math. 2010, 37, 587–592. [Google Scholar] [CrossRef]
- Johansson, B.T.; Lesnic, D.; Reeve, T. A method of fundamental solutions for the one-dimensional inverse Stefan problem. Appl. Math. Model. 2011, 35, 4367–4378. [Google Scholar] [CrossRef]
- Asfirane, S.; Hlioui, S.; Amara, Y.; Gabsi, M. Study of a hybrid excitation synchronous machine: Modeling and experimental validation. Math. Comput. Appl. 2019, 24, 34. [Google Scholar] [CrossRef]
- Bacciocchi, M.; Tarantino, A. Natural frequency analysis of functionally graded orthotropic cross–ply plates based on the finite element method. Math. Comput. Appl. 2019, 24, 52. [Google Scholar] [CrossRef]
- Liu, G.; Gu, Y. An Introduction to Meshfree Methods and Their Programing; Springer: Berlin, Germany, 2005. [Google Scholar]
- Belytschko, T.; Lu, Y.Y.; Gu, L. Element-free Galerkin methods. Int. J. Numer. Methods Eng. 1994, 37, 229–256. [Google Scholar] [CrossRef]
- Belytschko, T.; Lu, Y.Y.; Gu, L. Element free Galerkin methods for static and dynamic fracture. Int. J. Solids Struct. 1995, 32, 2547–2570. [Google Scholar] [CrossRef]
- Peng, M.; Cheng, Y. A boundary element-free method (BEFM) for two-dimensional potential problems. Eng. Anal. Boundary Elem. 2009, 33, 77–82. [Google Scholar] [CrossRef]
- Peng, M.; Li, D.; Cheng, Y. The complex variable element-free Galerkin (CVEFG) method for elasto-plasticity problems. Eng. Struct. 2011, 33, 127–135. [Google Scholar] [CrossRef]
- Peng, M.; Liu, P.; Cheng, Y. The complex variable element-free Galerkin (CVEFG) method for two-dimensional elasticity problems. Int. J. Appl. Mech. 2009, 1, 367–385. [Google Scholar] [CrossRef]
- Ren, H.; Cheng, Y. The interpolating element-free Galerkin (IEFG) method for two-dimensional potential problems. Eng. Anal. Boundary Elem. 2012, 36, 873–880. [Google Scholar] [CrossRef]
- Zhang, Z.; Hao, S.; Liew, K.; Cheng, Y. The improved element-free Galerkin method for two-dimensional elastodynamics problems. Eng. Anal. Boundary Elem. 2013, 37, 1576–1584. [Google Scholar] [CrossRef]
- Uddin, M.; Ali, H.; Taufiq, M. On the Approximation of a nonlinear biological population model using localized radial basis function method. Math. Comput. Appl. 2019, 24, 54. [Google Scholar] [CrossRef]
- Kansa, E. Multiquadrics—A scattered data approximation scheme with applications to computational fluid-dynamics. I. Surface approximations and partial derivative estimates. Comput. Math. Appl. 1990, 19, 127–145. [Google Scholar] [CrossRef]
- Dehghan, M.; Shokri, A. A numerical method for solution of the two dimensional sine-Gordon equation using the radial basis functions. Math. Comput. Simul. 2008, 79, 700–715. [Google Scholar] [CrossRef]
- Abbasbandy, S.; Ghehsareh, H.R.; Hashim, I. Numerical analysis of a mathematical model for capillary formation in tumor angiogenesis using a meshfree method based on the radial basis function. Eng. Anal. Boundary Elem. 2012, 36, 1811–1818. [Google Scholar] [CrossRef]
- Abbasbandy, S.; Shirzadi, A. MLPG method for two-dimensional diffusion equation with Neumann’s and non-classical boundary conditions. Appl. Numer. Math. 2011, 61, 170–180. [Google Scholar] [CrossRef]
- Atluri, S.; Zhu, T. A new meshless local Petrov–Galerkin (MLPG) approach in computational mechanics. Comput. Mech. 1998, 22, 117–127. [Google Scholar] [CrossRef]
- Atluri, S.; Zhu, T. A new meshless local Petrov–Galerkin (MLPG) approach to nonlinear problems in computer modeling and simulation. Comput. Model. Simul. Eng. 1998, 3, 187–196. [Google Scholar]
- Atluri, S.; Zhu, T. New concepts in meshless methods. Int. J. Numer. Meth. Eng. 2000, 13, 537–556. [Google Scholar] [CrossRef]
- Atluri, S.; Zhu, T. The meshless local Petrov–Galerkin (MLPG) approach for solving problems in elasto-statics. Comput. Mech. 2000, 25, 169–179. [Google Scholar] [CrossRef]
- Dai, B.; Cheng, Y. An improved local boundary integral equation method for two-dimensional potential problems. Int. J. Appl. Mech. 2010, 2, 421–436. [Google Scholar] [CrossRef]
- Dehghan, M.; Mirzaei, D. The meshless local Petrov–Galerkin (MLPG) method for the generalized two-dimensional non-linear Schrödinger equation. Eng. Anal. Boundary Elem. 2008, 32, 747–756. [Google Scholar] [CrossRef]
- Dehghan, M.; Mirzaei, D. Meshless local Petrov–Galerkin (MLPG) method for the unsteady magnetohydrodynamic (MHD) flow through pipe with arbitrary wall conductivity. Appl. Numer. Math. 2009, 59, 1043–1058. [Google Scholar] [CrossRef]
- Gu, Y.; Liu, G. A meshless local Petrov–Galerkin (MLPG) method for free and forced vibration analyses for solids. Comput. Mech. 2001, 27, 188–198. [Google Scholar] [CrossRef]
- Lancaster, P.; Salkauskas, K. Surface generated by moving least squares methods. Math. Comput. 1981, 37, 141–158. [Google Scholar] [CrossRef]
- Shirzadi, A.; Ling, L.; Abbasbandy, S. Meshless simulations of the two-dimensional fractional-time convection-diffusion-reaction equations. Eng. Anal. Boundary Elem. 2012, 36, 1522–1527. [Google Scholar] [CrossRef]
- Shirzadi, A.; Sladek, V.; Sladek, J. A local integral equation formulation to solve coupled nonlinear reaction-diffusion equations by using moving least square approximation. Eng. Anal. Boundary Elem. 2013, 37, 8–14. [Google Scholar] [CrossRef]
- Hu, D.; Long, S.; Liu, K.; Li, G. A modified meshless local Petrov–Galerkin method to elasticity problems in computer modeling and simulation. Eng. Anal. Boundary Elem. 2006, 30, 399–404. [Google Scholar] [CrossRef]
- Liu, K.; Long, S.; Li, G. A simple and less-costly meshless local Petrov–Galerkin (MLPG) method for the dynamic fracture problem. Eng. Anal. Boundary Elem. 2006, 30, 72–76. [Google Scholar]
- Grzymkowski, R.; Pleszczyński, M.; Słota, D. Comparing the Adomian decomposition method and Runge–Kutta method for solutions of the Stefan problem. Int. J. Comput. Math. 2006, 83, 409–417. [Google Scholar] [CrossRef]


| RMS Error | RMS Error | Absolute Error | Absolute Error | |
|---|---|---|---|---|
| t | for | for | for | for |
| with | with | with | with | |
| 0 | 0 | 0 | 0 | 0 |
| 0.1 | ||||
| 0.2 | ||||
| 0.3 | ||||
| 0.4 | ||||
| 0.5 | ||||
| 0.6 | ||||
| 0.7 | ||||
| 0.8 | ||||
| 0.9 | ||||
| 1.0 |
| RMS Error | RMS Error | Absolute Error | Absolute Error | |
|---|---|---|---|---|
| t | for | for | for | for |
| with | with | with | with | |
| 0 | 0 | 0 | 0 | 0 |
| 0.1 | ||||
| 0.2 | ||||
| 0.3 | ||||
| 0.4 | ||||
| 0.5 | ||||
| 0.6 | ||||
| 0.7 | ||||
| 0.8 | ||||
| 0.9 | ||||
| 1.0 |
| ADM | RKM | PM | ||
|---|---|---|---|---|
| ADM | RKM | PM | ||
|---|---|---|---|---|
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Karami, A.; Abbasbandy, S.; Shivanian, E. Meshless Local Petrov–Galerkin Formulation of Inverse Stefan Problem via Moving Least Squares Approximation. Math. Comput. Appl. 2019, 24, 101. https://doi.org/10.3390/mca24040101
Karami A, Abbasbandy S, Shivanian E. Meshless Local Petrov–Galerkin Formulation of Inverse Stefan Problem via Moving Least Squares Approximation. Mathematical and Computational Applications. 2019; 24(4):101. https://doi.org/10.3390/mca24040101
Chicago/Turabian StyleKarami, A., Saeid Abbasbandy, and E. Shivanian. 2019. "Meshless Local Petrov–Galerkin Formulation of Inverse Stefan Problem via Moving Least Squares Approximation" Mathematical and Computational Applications 24, no. 4: 101. https://doi.org/10.3390/mca24040101
APA StyleKarami, A., Abbasbandy, S., & Shivanian, E. (2019). Meshless Local Petrov–Galerkin Formulation of Inverse Stefan Problem via Moving Least Squares Approximation. Mathematical and Computational Applications, 24(4), 101. https://doi.org/10.3390/mca24040101






