Tensors in Newtonian Physics and the Foundations of Classical Continuum Mechanics
Abstract
:1. Introduction
- Tensor representation of various characteristics of mechanical processes and states, in the first place, stresses and strains;
- The structure of mappings of these tensors and equations linking them, including differential and integral connections used in constitutive relations of media;
- The basic hypothesis for the construction of constitutive relations of bodies at finite deformations, the general reduced forms of relations, bases of their classification (specialization for classes of bodies and processes), possibilities of their experimental verification and confirmation.
2. Newtonian Objectivity
2.1. Objective Tensors and Diagrams
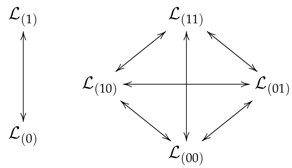
2.2. Mappings of Objective Tensors
3. Development of Foundations of Classical Continuum Mechanics
3.1. Generalized Theory of Strain and Stress Tensor Measures
3.2. Constitutive Relations: Foundations of the General Theory
3.3. Notions of Process Image and Five-Dimensional Isotropy at Finite Strains
4. Conclusions
Acknowledgments
Conflicts of Interest
References
- Sedov, L.I. Continuum Mechanics; Nauka: Moscow, Russia, 1973. [Google Scholar]
- Ilyushin, A.A. Continuum Mechanics; Moscow University Press: Moscow, Russia, 1990. [Google Scholar]
- Truesdell, C. A First Course in Rational Continuum Mechanics; The Johns Hopkins University: Baltimore, MD, USA, 1972. [Google Scholar]
- Germain, P. Continuum Mechanics; Vysshaya Shkola: Moscow, Russia, 1983. [Google Scholar]
- Jaric, J. Mehanika Kontinuuma; IRO Gradevinska Knjiga: Beograd, Russia, 1988. [Google Scholar]
- Pobedrya, B.E.; Georgievskii, D.V. Foundations of Continuum Mechanics; Fizmatlit: Moscow, Russia, 2006. [Google Scholar]
- Gurtin, M.E.; Fried, E.; Anand, L. The Mechanics and Thermodinamics of Continua; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Eglit, M.E. Lectures on the Basics of Continuum Mechanics; Book House “Librokom”: Moscow, Russia, 2013. [Google Scholar]
- Brovko, G.L. Foundations of Continuum Mechanics; Lomonosov Moscow State University: Moscow, Russia, 2013. [Google Scholar]
- Ilyushin, A.A. On dependence between stresses and small strains in continuum mechanics. Appl. Math. Mech. 1954, 18, 641–666. [Google Scholar]
- Ilyushin, A.A. Plasticity. Foundations of General Mathematical Theory; Academy of Sciences of the USSR: Moscow, Russia, 1963. [Google Scholar]
- Noll, W. A mathematical theory of the mechanical behavior of continuous media. Arch. Ration. Mech. Anal. 1958, 2, 197–226. [Google Scholar] [CrossRef]
- Truesdell, C.A.; Noll, W. The Nonlinear Field Theories of Mechanics; Springer: Berlin, Germany, 1965. [Google Scholar]
- Alexandrov, P.S. Problems of Hilbert. Collection; Nauka: Moscow, Russia, 1969. [Google Scholar]
- Ilyushin, A.A. Works (1946–1966). Plasticity; Fizmatlit: Moscow, Russia, 2004. [Google Scholar]
- Ilyushin, A.A.; Lensky, V.S. Strength of Materials; Fizmatgiz: Moscow, Russia, 1959. [Google Scholar]
- Astarita, G.; Marrucci, G. Principles of Non-Newtonian Fluid Mechanics; McGraw-Hill: London, UK, 1974. [Google Scholar]
- Hill, R. Aspects of invariance in solid mechanics. Adv. Appl. Mech. 1978, 18, 1–75. [Google Scholar]
- Ogden, R.W. On Eulerian and Lagrangean objectivity in continuum mechanics. Arch. Mech. 1984, 36, 207–218. [Google Scholar]
- Tolokonnikov, L.A. Mechanics of Solids; Vysshaya Shkola: Moscow, Russia, 1979. [Google Scholar]
- Lourie, A.I. Nonlinear Theory of Elasticity; Nauka: Mockow, Russia, 1980. [Google Scholar]
- Pozdeyev, A.A.; Trusov, P.V.; Nyashin, Y.I. Large Elastic-Plastic Deformations; Nauka: Moscow, Russia, 1986. [Google Scholar]
- Levitas, V.I. Large Elastic-Plastic Deformations of Materials at High Pressure; Naukova Dumka: Kyiv, Ukraine, 1987. [Google Scholar]
- Kondaurov, V.I.; Nikitin, L.V. Theoretical Foundations of Rheology of Geomaterials; Nauka: Moscow, Russia, 1990. [Google Scholar]
- Chernykh, K.F. Nonlinear Elasticity (Theory and Applications); Solo: St. Petersburg, Russia, 2004. [Google Scholar]
- Zubchaninov, V.G. Mechanics of Processes in Plastic Media; Fizmatlit: Moscow, Russia, 2010. [Google Scholar]
- Markin, A.A.; Sokolova, M.Y. Thermomechanics of Elastic-Plastic Deformations; Fizmatlit: Moscow, Russia, 2013. [Google Scholar]
- Hencky, H. The elastic behavior of vulcanized rubber. J. Appl. Mech. 1933, 1, 45–53. [Google Scholar] [CrossRef]
- Seth, B.R. Generalized strain measures with applications to physical problems. In Second Order Effects in Elasticity, Plasticity and Fluid Dynamics; Reiner, M., Abir, D., Eds.; Pergamon Press: Oxford, UK, 1964; pp. 162–172. [Google Scholar]
- Zhao, Z. Logarithmic strain and plastic constitutive equation at finite strain. In Proceedings of the International Conference on Nonlinear Mechanics, Shanghai, China, 28–31 October 1985; Science Press: Beijing, China, 1985; pp. 651–656. [Google Scholar]
- Novozhilov, V.V.; Chernykh, K.F. On “true” stress and strain measures in nonlinear mechanics of deformable bodies. Proc. Russ. Acad. Sci. Mech. Rigid Solid Bodies 1987, 5, 73–80. [Google Scholar]
- Man, C.S.; Guo, Z.H. A basis-free formula for time rate of Hill’s strain tensors. Int. J. Solids Struct. 1993, 30, 2819–2842. [Google Scholar]
- Xiao, H.; Bruhns, O.T.; Meyers, A. Large strain responses of elastic-perfect plasticity and kinematic hardening plasticity with the logarithmic rate: Swift effect in torsion. Int. J. Plast. 2001, 17, 211–235. [Google Scholar] [CrossRef]
- Zaremba, S. Sur une forme perfectionée de la théorie de la relaxation. Bull. Int. Acad. Sci. Cracovie 1903, 19A, 594–614. [Google Scholar]
- Jaumann, G. Geschlossenes system physikalischer und chemischer differenzialgesetze. Sitzber. Akad. Wiss. Wien 1911, 120, 385–530. [Google Scholar]
- Oldroyd, J.G. On the formulation of rheological equations of state. Proc. R. Soc. Lond. A 1950, 200, 523–541. [Google Scholar]
- Cotter, B.A.; Rivlin, R.S. Tensors assotiated with time-dependent stress. Q. Appl. Math. 1955, 13, 177–188. [Google Scholar] [CrossRef]
- Truesdell, C. Hypo-elasticity. J. Ration. Mech. Anal. 1955, 4, 83–133. [Google Scholar] [CrossRef]
- Sedov, L.I. The concepts of different rates of change of tensors. Appl. Math. Mech. 1960, 24, 393–398. [Google Scholar]
- Sedov, L.I. Introduction to Continuum Mechanics; Fizmatgiz: Moscow, Russia, 1962. [Google Scholar]
- Lehmann, T. Some remarks on a general class of material laws for large elasto-plastic deformations. Eng. Arch. 1972, 41, 279–310. [Google Scholar]
- Kolarov, D.; Baltov, A.; Boncheva, N. Mechanics of Plastic Media; Mir: Moscow, Russia, 1979. [Google Scholar]
- Dienes, J.K. On the analysis of rotation and stress rate in deforming bodies. Acta Mech. 1979, 32, 217–232. [Google Scholar] [CrossRef]
- Nagtegaal, J.C.; de Jong, J.E. Some aspects of nonisotropic work hardening in finite strain plasticity. In Plasticity of Metals at Finite Strain: Theory, Experiment and Computation; Stanford University: Stanford, CA, USA, 1982; pp. 65–102. [Google Scholar]
- Dafalias, Y.F. Corotational rates for kinematic hardening at large plastic deformations. Trans. ASME J. Appl. Mech. 1983, 50, 561–565. [Google Scholar] [CrossRef]
- Atluri, S.N. On constitutive relations at finite strain: Hypo-elasticity and elasto-plasticity with isotropic or kinematic hardening. Comput. Methods Appl. Mech. Eng. 1984, 43, 137–171. [Google Scholar] [CrossRef]
- Markin, A.A.; Tolokonnikov, L.A. Measures and constitutive equations of finite elastoplastic deformation. Appl. Probl. Strength Plast. 1987, 32–37. [Google Scholar]
- Latorre, M.; Montans, F.J. Stress and strain mapping tensors and general work-conjugacy in large strain continuum mechanics. Appl. Math. Model. 2016, 40, 3938–3950. [Google Scholar] [CrossRef]
- Neff, P.; Eidel, B.; Martin, R.J. Geometry of logarithmic strain measures in solid mechanics. Arch. Ration. Mech. Anal. 2016, 222, 507–572. [Google Scholar] [CrossRef]
- Marsden, J.E.; Hughes, T.J.R. Mathematical Foundations of Elasticity; Dover Publications: New York, NY, USA, 1994. [Google Scholar]
- Zhang, M.; Montans, F.J. A simple formulation for large-strain cyclic hyperelasto-plasticity using elastic correctors. Theory and algorithmic implementation. Int. J. Plast. 2019, 113, 185–217. [Google Scholar] [CrossRef]
- Dubrovin, B.A.; Novikov, S.P.; Fomenko, A.T. Contemporary Geometry; Nauka: Moscow, Russia, 1979. [Google Scholar]
- Postnikov, M.M. Lectures on Geometry; Nauka: Moscow, Russia, 1986. [Google Scholar]
- Lang, S. Algebra; Mir: Moscow, Russia, 1968. [Google Scholar]
- Dieudonne, J.; Carrol, J.; Mamford, D. Geometric Theory of Invariants; Mir: Moscow, Russia, 1974. [Google Scholar]
- Vinberg, E.B.; Gorbatsevich, V.V.; Onishchik, A.L. The structure of lie groups and lie algebras. Results Sci. Technol. Mod. Probl. Math. Fundam. Dir. 1989, 41, 5–258. [Google Scholar]
- Skornyakov, L.A. General Algebra; Nauka: Moscow, Russia, 1990. [Google Scholar]
- Kostrikin, A.I. Introduction to Algebra; Fizmatlit: Moscow, Russia, 2004. [Google Scholar]
- Vinberg, E.B. Course of Algebra; Faktorial Press: Moscow, Russia, 2002. [Google Scholar]
- Vinberg, E.B. Representations of classical groups in tensors. In Mathematical Encyclopedia; Sovetskaya Enciklopedia: Moscow, Russia, 1984; pp. 595–598. [Google Scholar]
- Zhelobenko, D.P.; Stern, A.I. Representations of Lie Groups; Nauka: Moscow, Russia, 1983. [Google Scholar]
- Gurevich, G.B. Foundations of the Theory of Algebraic Invariants; OGIZ GITTL: Moscow, Russia, 1948. [Google Scholar]
- Lankaster, P. Theory of Matrices; Nauka: Moscow, Russia, 1982. [Google Scholar]
- Spencer, E. Theory of Invariants; Mir: Moscow, Russia, 1974. [Google Scholar]
- Brovko, G.L. Notions of process image and five-dimensional isotropy of material properties at finite strains. Rep. Acad. Sci. USSR 1989, 308, 565–570. [Google Scholar]
- Brovko, G.L. Properties and integration of some time derivatives of tensor processes in continuum mechanics. Proc. Russ. Acad. Sci. Mech. Rigid Solid Bodies 1990, 1, 54–60. [Google Scholar]
- Brovko, G.L. Material and spatial representations for constitutive relations of deformable media. Appl. Math. Mech. 1990, 54, 814–824. [Google Scholar] [CrossRef]
- Brovko, G.L. On a family of holonomic tensor measures of deformations and stresses. Bull. Mosc. Univ. 1992, 4, 86–91. [Google Scholar]
- Brovko, G.L. Invariance Types of Tensors, Tensor Processes and Their Transforms in Classical Continuum Mechanics. In Proceedings of the Fifth International Seminar on Geometry, Continuum and Microstructures, Sinaia, Romania, 26–28 September 2001; Editura Academiei Romane: Bucuresti, Romania, 2002; pp. 13–24. [Google Scholar]
- Brovko, G.L. On general principles of the theory of constitutive relations in classical continuum mechanics. J. Eng. Math. 2013, 78, 37–53. [Google Scholar] [CrossRef]
- Brovko, G.L. Elements of the Mathematical Apparatus of Continuum Mechanics; Fizmatlit: Moscow, Russia, 2015. [Google Scholar]
- Brovko, G.L. Constitutive Relations of Continuum Mechanics. The Development of Mathematical Apparatus and the Foundations of the General Theory; Nauka: Moscow, Russia, 2017. [Google Scholar]
- Brovko, G.L. General reduced forms of constitutive relations of classical continuum mechanics. Bull. Mosc. Univ. 2018, 2, 67–71. [Google Scholar] [CrossRef]
- Finoshkina, A.S. The use of new objective derivatives in the simplest models of hypoelasticity and plastic flow with kinematic hardening. Bull. Tula State Univ. 2000, 6, 160–166. [Google Scholar]
- Brovko, G.L.; Ivanova, O.A.; Finoshkina, A.S. On geometrical and analytical aspects in formulations of problems of classic and non-classic continuum mechanics. In Operator Theory: Advances and Applications; Birkhäuser Verlag: Basel, Switzerland, 2009; pp. 51–79. [Google Scholar]
- Brovko, G.L.; Ivanova, O.A. Modeling of properties and motions of inhomogeneous one-dimensional continuum with complicated structure of Cosserat type. Proc. Russ. Acad. Sci. Mech. Rigid Solid Bodies 2008, 1, 22–36. [Google Scholar]
- Ivanova, O.A. Model of equipped rod with viscoelastic internal interactions. Mech. Compos. Mater. Struct. 2018, 24, 70–81. [Google Scholar]
- Brovko, G.L.; Kuzichev, S.A. Stability of forced torsional vibrations of an equipped rod. Bull. Mosc. Univ. 2010, 1, 57–62. [Google Scholar] [CrossRef]
- Brovko, G.L.; Grishayev, A.G.; Ivanova, O.A. Continuum models of discrete heterogeneous structures and saturated porous media: Constitutive relations and invariance of internal interactions. J. Phys. Conf. Ser. 2007, 62, 1–22. [Google Scholar] [CrossRef]
- Faskheyev, I.O. Modeling of Mechanical Processes in Saturated Porous Media with Account of Interactive Forces. Ph.D. Thesis, Lomonosov Moscow State University, Moscow, Russia, 2017. [Google Scholar]
- Shutkin, A.S. Approaches to generalization of the constitutive relations of deformable solids to the finite deformation region. Mech. Compos. Mater. Struct. 2010, 16, 166–180. [Google Scholar]
- Muravlev, A.V.; Devyatov, A.S. Development of the theory of elastic-plastic processes of Ilyushin and experimental-theoretical methods of research of viscoplastic properties of materials at finite deformations. Probl. Mech. Eng. Autom. Int. J. 2016, 1, 84–90. [Google Scholar]
- Ovchinnikova, N.V. On tensor measures of stresses and strains used in ANSYS to solve elastic-plastic problems under finite strains. Bull. Mosc. Univ. 2017, 5, 31–36. [Google Scholar]
- Tunguskova, Z.G. Analytical representation of the stress tensor in the problem of the shift of hypoelastic body using corotational derivatives of a certain type. In Elasticity and Anelasticity; Moscow University Press: Moscow, Russia, 2016; pp. 260–262. [Google Scholar]
- Martynova, E.D.; Stetsenko, N.S. The use of one-parameter Gordon–Showalter family of objective derivatives to describe finite deformations of viscoelastic bodies. Bull. Mosc. Univ. 2017, 6, 64–68. [Google Scholar]
- Bykov, D.L.; Martynova, E.D. Identification of characteristics of viscoelastic materials under repeated compression after unloading by numerical-graphical method. Proc. Russ. Acad. Sci. Mech. Rigid Solid Bodies 2018, 2, 3–9. [Google Scholar]
- Martynova, E.D. Processes of torsion of cylindrical specimens made from an incompressible viscoelastic materials of Maxwell type. Appl. Math. Mech. 2019, 83, 95–106. [Google Scholar]
© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brovko, G.L. Tensors in Newtonian Physics and the Foundations of Classical Continuum Mechanics. Math. Comput. Appl. 2019, 24, 79. https://doi.org/10.3390/mca24030079
Brovko GL. Tensors in Newtonian Physics and the Foundations of Classical Continuum Mechanics. Mathematical and Computational Applications. 2019; 24(3):79. https://doi.org/10.3390/mca24030079
Chicago/Turabian StyleBrovko, George L. 2019. "Tensors in Newtonian Physics and the Foundations of Classical Continuum Mechanics" Mathematical and Computational Applications 24, no. 3: 79. https://doi.org/10.3390/mca24030079
APA StyleBrovko, G. L. (2019). Tensors in Newtonian Physics and the Foundations of Classical Continuum Mechanics. Mathematical and Computational Applications, 24(3), 79. https://doi.org/10.3390/mca24030079




