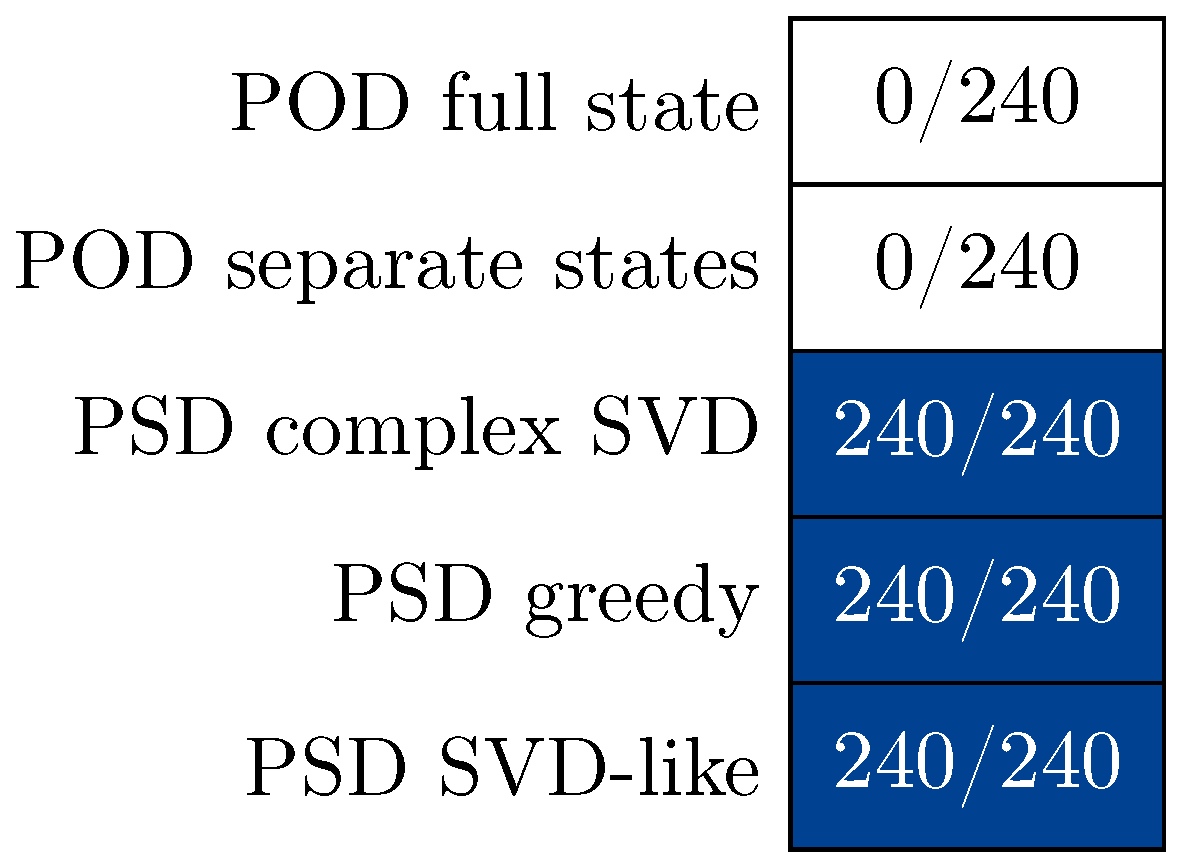
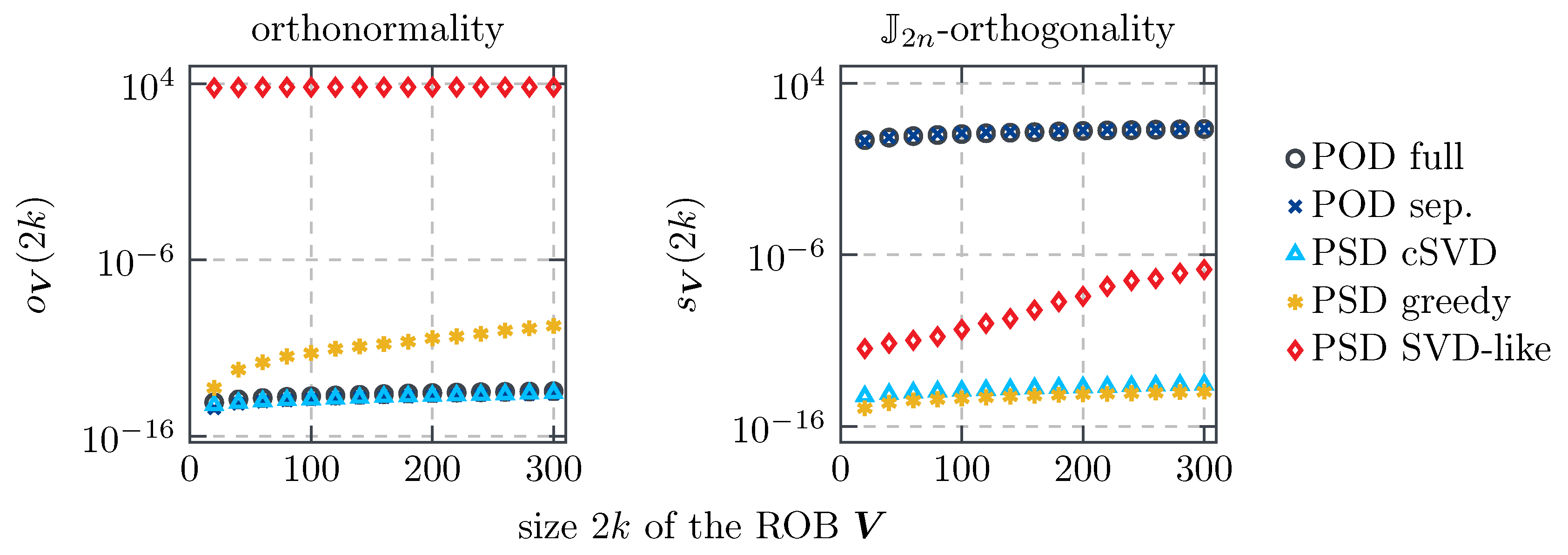
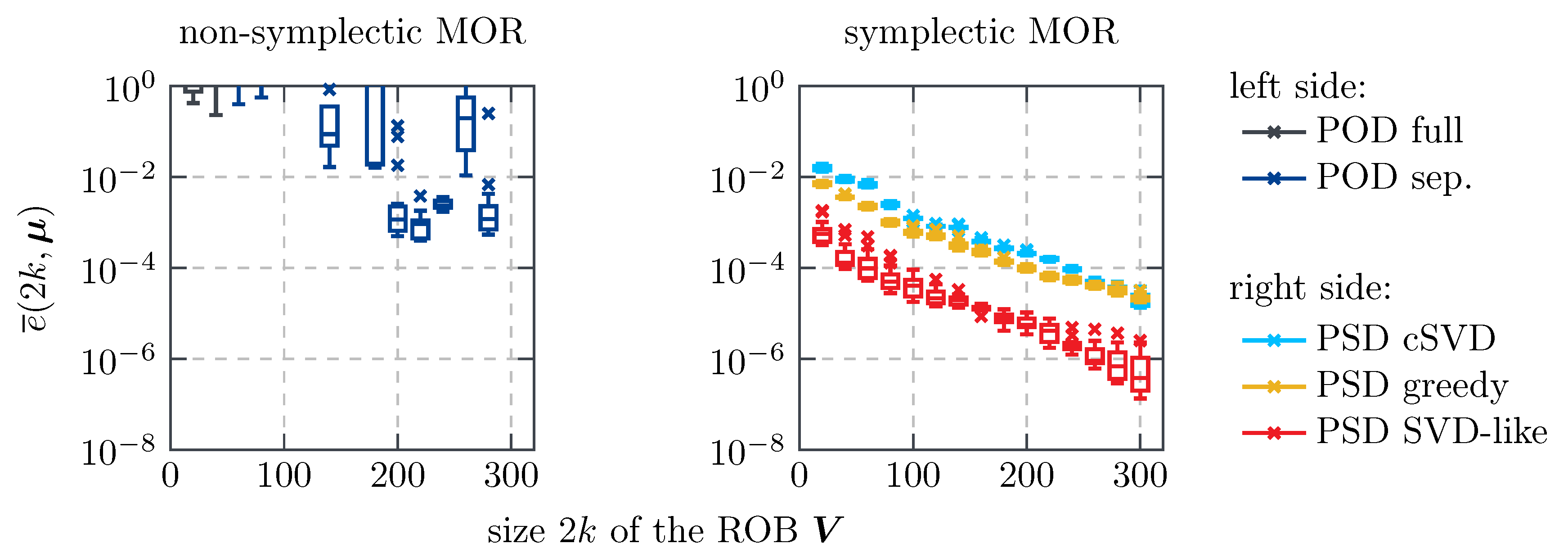
We introduced the symplectic MOR for finite-dimensional Hamiltonian systems in the previous section. This approach requires a symplectic ROB which is yet not further specified. In the following, we discuss the Proper Symplectic Decomposition (PSD) as a data-driven basis generation approach. To this end, we classify symplectic ROBs as orthogonal and non-orthogonal. The PSD is investigated for these two classes in
Section 3.1 and
Section 3.2 separately. For symplectic, orthogonal ROBs, we prove that an optimal solution can be derived based on an established procedure, the PSD Complex SVD. For symplectic, non-orthogonal ROBs, we provide a new basis generation method, the PSD SVD-like decomposition.
We pursue the approach to generate an ROB from a collection of snapshots of the system [
16]. A snapshot is an element of the so-called solution manifold
which we aim to approximate with a low-dimensional surrogate
where
is a solution of the full model (
5),
is the ROB and
is the solution of the reduced system (
7). In [
4], the Proper Symplectic Decomposition (PSD) is proposed as a snapshot-based basis generation technique for symplectic ROBs. The idea is to derive the ROB from a minimization problem which is suggested in analogy to the very well established Proper Orthogonal Decomposition (POD, also Principal Component Analysis) [
1].
Classically, the POD chooses the ROB
to minimize the sum over squared norms of all
residuals
of the orthogonal projection
of the
single snapshots
measured in the 2-norm
with the constraint that the ROB
is orthogonal, i.e.,
We summarize this in a more compact (matrix-based) formulation in the following definition.
3.1. Symplectic, Orthonormal Basis Generation
The foremost problem of the PSD is that there is no explicit solution procedure known so far due to the high nonlinearity and possibly multiple local optima. This is an essential difference to the POD as the POD allows to find a global minimum by solving an eigenvalue problem [
1].
Current solution procedures for the PSD restrict to a certain subset of symplectic matrices and derive an optimal solution for this subset which might be suboptimal in the class of symplectic matrices. In the following, we show that this subclass almost exclusively restricts to symplectic, orthonormal ROBs.
Definition 7 (Symplectic, orthonormal ROB)
. We call an ROB symplectic, orthonormal (also orthosymplectic, e.g., in [5]) if it is symplectic w.r.t. and and is orthonormal, i.e., the matrix has orthonormal columns In the following, we show an alternative characterization of a symplectic and orthonormal ROB. Therefore, we extend the results given, e.g., in [
17] for square matrices
in the following Proposition 4 to the case of rectangular matrices
. This was also partially addressed in ([
4], Lemma 4.3.).
Proposition 4 (Characterization of a symplectic matrix with orthonormal columns)
. The following statements are equivalent for any matrix
- (i)
is symplectic with orthonormal columns,
- (ii)
- (iii)
is symplectic and it holds .
We remark that these matrices are characterized in [
4] to be elements in
where
is the symplectic Stiefel manifold and
is the Stiefel manifold.
Proof. “(i) ⇒ (ii)”: Let
be a symplectic matrix with orthonormal columns. We rename the columns to
with
and
. The symplecticity of the matrix written in terms of
and
reads
Expressed in terms of the columns
of the matrices
, this condition reads for any
and the orthonormality of the columns of
implies
For a fixed
, it is easy to show with
that
is of unit length
Thus,
and
are both unit vectors which fulfill
. By the Cauchy–Schwarz inequality, it holds
if and only if the vectors are parallel. Thus, we infer
, which is equivalent to
. Since this holds for all
, we conclude that
is of the form proposed in (
15).
“(ii) ⇒ (iii)”: Let
be of the form (
15). Direct calculation yields
which shows that
is symplectic. Thus, the symplectic inverse
exists. The following calculation shows that it equals the transposed
“(iii) ⇒ (i)”: Let
be symplectic with
. Then, we know that
has orthonormal columns since
□
Proposition 4 essentially limits the symplectic, orthonormal ROB
to be of the form (
15). Later in the current section, we see how to solve the PSD for ROBs of this type. In
Section 3.2, we are interested in ridding the ROB
of this requirement to explore further solution methods of the PSD.
As mentioned before, the current solution procedures for the PSD almost exclusively restrict to the class of symplectic, orthonormal ROBs introduced in Proposition 4. This includes the Cotangent Lift [
4], the Complex SVD [
4], partly the nonlinear programming algorithm from [
4] and the greedy procedure presented in [
5]. We briefly review these approaches in the following proposition.
Proposition 5 (Symplectic, orthonormal basis generation)
. The Cotangent Lift (CT), Complex SVD (cSVD) and the greedy procedure for symplectic basis generation all derive a symplectic and orthonormal ROB. The nonlinear programming (NLP) admits a symplectic, orthonormal ROB if the coefficient matrix in ([4], Algorithm 3) is symplectic and has orthonormal columns, i.e., it is of the form . The methods can be rewritten with , where the different formulations of readwhere - (i)
are matrices that fulfilwhich is technically equivalent to and (see (15)) for and , - (ii)
are the basis vectors selected by the greedy algorithm,
- (iii)
is an ROB computed from CT or cSVD and , , stems from the coefficient matrix computed by the NLP algorithm.
Proof. All of the listed methods derive a symplectic ROB of the form
which satisfies (
15). By Proposition 4, these ROBs are each a symplectic, orthonormal ROB. □
In the following, we show that PSD Complex SVD is the solution of the PSD in the subset of symplectic, orthonormal ROBs. This was partly shown in [
4] which yet lacked the final step that, restricting to orthonormal, symplectic ROBs, a solution of
solves
and vice versa. This proves that the PSD Complex SVD is not only near optimal in this set but indeed optimal. Furthermore, the proof we show is alternative to the original and naturally motivates an alternative formulation of the PSD Complex SVD which we call the POD of
in the following. To begin with, we reproduce the definition of PSD Complex SVD from [
4].
Definition 8 (PSD Complex SVD)
. We define the complex snapshot matrixwhich is derived with the imaginary unit . The PSD Complex SVD is a basis generation technique that requires the auxiliary complex matrix to fulfiland builds the actual ROB withThe solution of (18) is known to be based on the left-singular vectors of which can be explicitly computed with a complex version of the SVD. We emphasize that we denote this basis generation procedure as PSD Complex SVD in the following to avoid confusions with the usual complex SVD algorithm.
Proposition 6 (Minimizing PSD in the set of symplectic, orthonormal ROBs)
. Given the snapshot matrix , we augment this with “rotated” snapshots to . We assume that is such that we obtain a gap in the singular values of , i.e., . Then, minimizing the PSD in the set of symplectic, orthonormal ROBs is equivalent to the following minimization problemClearly, this is equivalent to the POD (12) applied to the snapshot matrix . We, thus, call this procedure the POD of in the following. A minimizer can be derived with the SVD as it is common for POD [1]. Proof. The proof proceeds in three steps: we show
- (i)
that is a pair of left- and right-singular vectors of to the singular value if and only if also is a pair of left- and right-singular vectors of to the same singular value ,
- (ii)
that a solution of the POD of is a symplectic, orthonormal ROB, i.e., ,
- (iii)
that the POD of is equivalent to the PSD for symplectic, orthonormal ROBs.
We start with the first step (i). Let
be a pair of left- and right-singular vectors of
to the singular value
. We use that the left-singular (or right-singular) vectors of
are a set of orthonormal eigenvectors of
(or
). To begin with, we compute
where we use
. Thus, we can reformulate the eigenvalue problems of
and, respectively,
as
Thus,
is necessarily another pair of left- and right-singular vectors of
with the same singular value
. We infer that the left-singular vectors
,
, ordered by the magnitude of the singular values in a descending order can be written as
For the second step (ii), we remark that the solution of the POD is explicitly known to be any matrix which stacks in its columns
left-singular vectors of the snapshot matrix
with the highest singular value [
1]. Due to the special structure (
21) of the singular vectors for the snapshot matrix
, a minimizer of the POD of
necessarily adopts this structure. We are allowed to rearrange the order of the columns in this matrix and thus the result of the POD of
can always be rearranged to the form
Note that it automatically holds that
and
since, in both products, we use the left-singular vectors from the columns of the matrix
from (
21) which is known to be orthogonal from properties of the SVD. Thus, (
15) holds and we infer from Proposition 4 that the POD of
indeed is solved by a symplectic, orthonormal ROB.
For the final step (iii), we define the orthogonal projection operators
Both are idempotent and symmetric, thus
. Due to
, it further holds
Thus, it follows
and with
where we use in the last step that for two matrices
,
for
, it holds
for the Frobenius norm
.
Since it is equivalent to minimize a function
or a multiple
of it for any positive constant
, minimizing
is equivalent to minimizing
. Additionally, for an ROB of the form
, the constraint of orthonormal columns is equivalent to the requirements in (
15). Thus, to minimize the PSD in the class of symplectic, orthonormal ROBs is equivalent to the POD of
(
19). □
Remark 6. We remark that, in the same fashion as the proof of step (iii) in Proposition 6, it can be shown that, restricting to symplectic, orthonormal ROBs, a solution of is a solution of and vice versa, which is one detail that was missing in [4] to show the optimality of PSD Complex SVD in the set of symplectic, orthonormal ROBs. We next prove that PSD Complex SVD is equivalent to POD of
from (
19) and thus also minimizes the PSD in the set of symplectic, orthonormal bases. To this end, we repeat the optimality result from [
4] and extend it with the results of the present paper.
Proposition 7 (Optimality of PSD Complex SVD)
. Let denote the set of symplectic bases with the structure . The PSD Complex SVD solves in .
Proof. See ([
4], Theorem 4.5). □
Proposition 8 (Equivalence of POD of Ys and PSD Complex SVD)
. PSD Complex SVD is equivalent to the POD of . Thus, PSD Complex SVD yields a minimizer of the PSD for symplectic, orthonormal ROBs.
Proof. By Proposition 7, PSD Complex SVD minimizes (
19) in the set
of symplectic bases with the structure
. Thus, (
16) holds with
which is equivalent to the conditions on
required in (
15). By Proposition 4, we infer that
equals the set of symplectic, orthonormal bases.
Furthermore, we can show that, in the set , a solution of is a solution of and vice versa (see Remark 6). Thus, PSD Complex SVD minimizes the PSD for the snapshot matrix in the set of orthonormal, symplectic matrices and PSD Complex SVD and the POD of solve the same minimization problem. □
We emphasize that the computation of a minimizer of (
19) via PSD Complex SVD requires less memory storage than the computation via POD of
. The reason is that the complex formulation uses the complex snapshot matrix
which equals
floating point numbers while the solution with the POD of
method artificially enlarges the snapshot matrix to
which are
floating point numbers. Still, the POD of
might be computationally more efficient since it is a purely real formulation and thereby does not require complex arithmetic operations.
3.2. Symplectic, Non-Orthonormal Basis Generation
In the next step, we want to give an idea how to leave the class of symplectic, orthonormal ROBs. We call a basis generation technique symplectic, non-orthonormal if it is able to compute a symplectic, non-orthonormal basis.
In Proposition 5, we briefly showed that most existing symplectic basis generation techniques generate a symplectic, orthonormal ROB. The only exception is the NLP algorithm suggested in [
4]. It is able to compute a non-orthonormal, symplectic ROB. The algorithm is based on a given initial guess
which is a symplectic ROB, e.g., computed with PSD Cotangent Lift or PSD Complex SVD. Nonlinear programming is used to leave the class of symplectic, orthonormal ROBs and derive an optimized symplectic ROB
with the symplectic coefficient matrix
for some
. Since this procedure searches a solution spanned by the columns of
, it is not suited to compute a global optimum of the PSD which we are interested in the scope of this paper.
In the following, we present a new, non-orthonormal basis generation technique that is based on an SVD-like decomposition for matrices
presented in [
6]. To this end, we introduce this decomposition in the following. Subsequently, we present first theoretical results which link the symplectic projection error with the “singular values” of the SVD-like decomposition which we call symplectic singular values. Nevertheless, the optimality with respect to the PSD functional (
14) of this new method is yet an open question.
Proposition 9 (SVD-like decomposition
[
6])
. Any real matrix can be decomposed as the product of a symplectic matrix , a sparse and potentially non-diagonal matrix and an orthogonal matrix with![Mca 24 00043 g007]() with and and where we indicate the block row and column dimensions in by small letters. The diagonal entries , , of the matrix are related to the pairs of purely imaginary eigenvalues of with
with and and where we indicate the block row and column dimensions in by small letters. The diagonal entries , , of the matrix are related to the pairs of purely imaginary eigenvalues of with Remark 7 (Singular values)
. We call the diagonal entries , , of the matrix from Proposition 9 in the following the symplectic singular values. The reason is the following analogy to the classical SVD.
The classical SVD decomposes as where , are each orthogonal matrices and is a diagonal matrix with the singular values on its diagonal , . The singular values are linked to the real eigenvalues of with . Furthermore, for the SVD, it holds due to the orthogonality of and , respectively, and .
A similar relation can be derived for an SVD-like decomposition from Proposition 9. Due to the structure of the decomposition (22) and the symplecticity of , it holds![Mca 24 00043 g008]() This analogy is why we call the diagonal entries , , of the matrix symplectic singular values.
This analogy is why we call the diagonal entries , , of the matrix symplectic singular values. The idea for the basis generation now is to select pairs of columns of in order to compute a symplectic ROB. The selection should be based on the importance of these pairs which we characterize by the following proposition by linking the Frobenius norm of a matrix with the symplectic singular values.
Proposition 10. Let with an SVD-like decomposition with from Proposition 9. The Frobenius norm of can be rewritten aswhere is the i-th column of for . In the following, we refer to each as the weighted symplectic singular value. Proof. We insert the SVD-like decomposition
and use the orthogonality of
to reformulate
which is equivalent to (
24). □
It proves true in the following Proposition Proposition 11 that we can delete single addends
in (
24) with the symplectic projection used in the PSD if we include the corresponding pair of columns in the ROB. This will be our selection criterion in the new basis generation technique that we denote PSD SVD-like decomposition.
Definition 9 (PSD SVD-like decomposition)
. We compute an SVD-like decomposition (22) as of the snapshot matrix and define as in Proposition 9. In order to compute an ROB with columns, find the k indices which have large contributions in (24) withTo construct the ROB, we choose the k pairs of columns from corresponding to the selected indices such that The special choice of the ROB is motivated by the following theoretical result which is very analogous to the results known for the classical POD in the framework of orthogonal projections.
Proposition 11 (Projection error by neglegted weighted symplectic singular values)
. Let be an ROB constructed with the procedure described in Definition 9 to the index set with from Proposition 9. The PSD functional can be calculated bywhich is the cumulative sum of the squares of the neglected weighted symplectic singular values. Proof. Let
be an ROB constructed from an SVD-like decomposition
of the snapshot matrix
with the procedure described in Definition 9. Let
be defined as in Proposition 9 and
be the set of indices selected with (
25).
For the proof, we introduce a slightly different notation of the ROB
. The selection of the columns
of
is denoted with the selection matrix
based on
which allows us to write the ROB as the matrix–matrix product
. Furthermore, we can select the neglected entries with
.
We insert the SVD-like decomposition and the representation of the ROB introduced in the previous paragraph in the PSD which reads
where we use the orthogonality of
and the symplecticity of
in the last step. We can reformulate the product of Poisson matrices and the selection matrix as
Thus, we can further reformulate the PSD as
where
are the weighted symplectic singular values from (
24). In the last step, we use that the resultant diagonal matrix in the braces sets all rows of
with indices
to zero for
. Thus, the last step can be concluded analogously to the proof of Proposition 10.
A direct consequence of Proposition 11 is that the decay of the PSD functional is proportional to the decay of the sum over the neglected weighted symplectic singular values
from (
24). In the numerical example
Section 4.2.1, we observe an exponential decrease of this quantities which induces an exponential decay of the PSD functional. □
Remark 8 (Computation of the SVD-like decomposition)
. To compute an SVD-like decompostion (22) of , several approaches exist. The original paper [6] derives a decomposition based on the product which is not good for a numerical computation since errors can arise from cancellation. In [3], an implicit version is presented that does not require the computation of the full product but derives the decomposition implicitly by transforming . Furthermore, Ref. [18] introduces an iterative approach to compute an SVD-like decomposition which computes parts of an SVD-like decomposition with a block-power iterative method. In the present case, we use the implicit approach [3]. To conclude the new method, we display the computational steps in Algorithm 1. All methods in this algorithm are standard MATLAB® functions except for SVD_like_decomp which is supposed to return the matrices , , and integers of the SVD-like decomposition (22). The matrix is not required and thus, replaced with ∼ as usual in MATLAB® notation.
| Algorithm 1: PSD SVD-like decomposition in MATLAB® notation. |
| Input: snapshot matrix , size of the ROB |
| Output: symplectic ROB |
| 1 | // compute SVD-like decomposition, Q is not required |
| 2 | // extract symplectic singular values |
| 3 | // compute squares of the 2-norm of each column of S |
| 4 | // weighted sympl. singular values |
| 5 | // append weighted symplectic singular values |
| 6 | // find indices of k highest weighted symplectic singular values |
| 7 | // select columns with indices and |
3.3. Interplay of Non-Orthonormal and Orthonormal ROBs
We give further results on the interplay of non-orthonormal and orthonormal ROBs. The fundamental statement in the current section is the Orthogonal SR decomposition [
6,
19].
Proposition 12 (Orthogonal SR decomposition)
. For each matrix with , there exists a symplectic, orthogonal matrix , an upper triangular matrix and a strictly upper triangular matrix such that We remark that a similar result can be derived for the case
[
6], but it is not introduced since we do not need it in the following.
Proof. Let
with
. We consider the QR decomposition
where
is an orthogonal matrix and
is upper triangular. The original Orthogonal SR decomposition ([
19], Corollary 4.5.) for the square matrix states that we can decompose
as a symplectic, orthogonal matrix
, an upper triangular matrix
, a strictly upper triangular matrix
and two (possibly) full matrices
Since
is upper triangular, it does preserve the (strictly) upper triangular pattern in
and
and we obtain the (strictly) upper triangular matrices
from
□
Based on the Orthogonal SR decomposition, the following two propositions prove bounds for the projection errors of PSD which allows an estimate for the quality of the respective method. In both cases, we require the basis size to satisfy or , respectively. This restriction is not limiting in the context of symplectic MOR as in all application cases .
Similar results have been presented in ([
20], Proposition 3.11) for PSD Cotangent Lift. In comparison to these results, we are able to extend the bound to the case of PSD Complex SVD and thereby improve the bound for the projection error by a factor of
.
Proposition 13. Let be a minimizer of POD with basis vectors and be a minimizer of the PSD in the class of orthonormal, symplectic matrices with basis vectors. Then, the orthogonal projection errors of and satisfy Proof. The Orthogonal SR decomposition (see Proposition 12) guarantees that a symplectic, orthogonal matrix
and
exist with
. Since both matrices
and
are orthogonal and
, we can show that
yields a lower projection error than
with
Let
be a minimizer of the PSD in the class of symplectic, orthonormal ROBs. By definition of
, it yields a lower projection error than
. Since both ROBs are symplectic and orthonormal, we can exchange the symplectic inverse with the transposition (see Proposition 4, (iii)). This proves the assertion with
□
Proposition 13 proves that we require at most twice the number of basis vectors to generate a symplectic, orthonormal basis with an orthogonal projection error at least as small as the one of the classical POD. An analogous result can be derived in the framework of a symplectic projection which is proven in the following proposition.
Proposition 14. Assume that there exists a minimizer of the general PSD for a basis size with potentially non-orthonormal columns. Let be a minimizer of the PSD in the class of symplectic, orthogonal bases of size . Then, we know that the symplectic projection error of is less than or equal to the one of , i.e., Proof. Let
be a minimizer of PSD with
. By Proposition 12, we can determine a symplectic, orthogonal matrix
and
with
. Similar to the proof of Proposition 13, we can bound the projection errors. We require the identity
With this identity, we proceed analogously to the proof of Proposition 13 and derive for a minimizer
of PSD in the class of symplectic, orthonormal ROBs
□
Proposition 14 proves that we require at most twice the number of basis vectors to generate a symplectic, orthonormal basis with a symplectic projection error at least as small as the one of a (potentially non-orthonormal) minimizer of PSD.
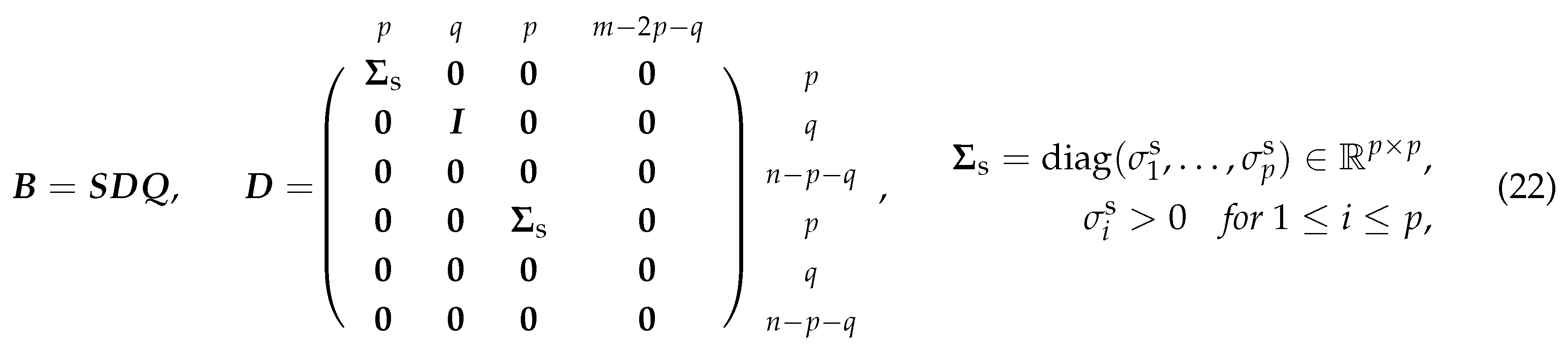 with and and where we indicate the block row and column dimensions in by small letters. The diagonal entries , , of the matrix are related to the pairs of purely imaginary eigenvalues of with
with and and where we indicate the block row and column dimensions in by small letters. The diagonal entries , , of the matrix are related to the pairs of purely imaginary eigenvalues of with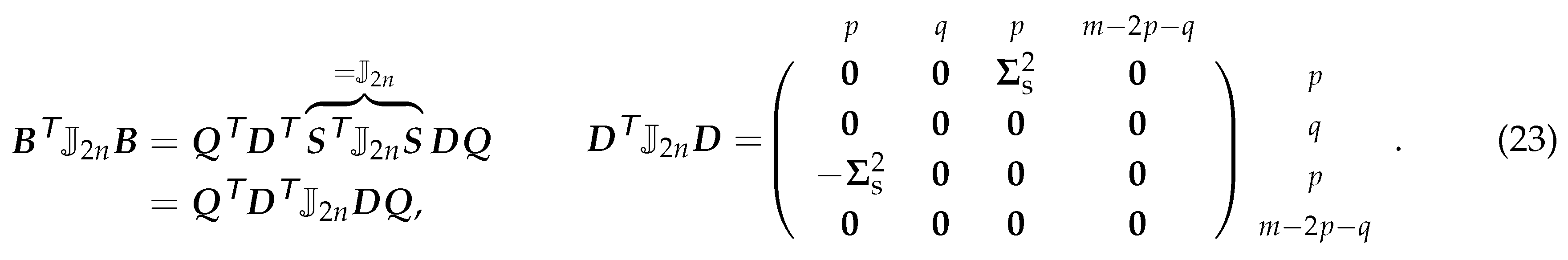 This analogy is why we call the diagonal entries , , of the matrix symplectic singular values.
This analogy is why we call the diagonal entries , , of the matrix symplectic singular values.