High-Order Methods Applied to Nonlinear Magnetostatic Problems
Abstract
:1. Introduction
2. Modeling Methods
2.1. Finite Element Method
2.2. Spectral Element Method
2.3. Isogeometric Analysis
3. Problem Formulation
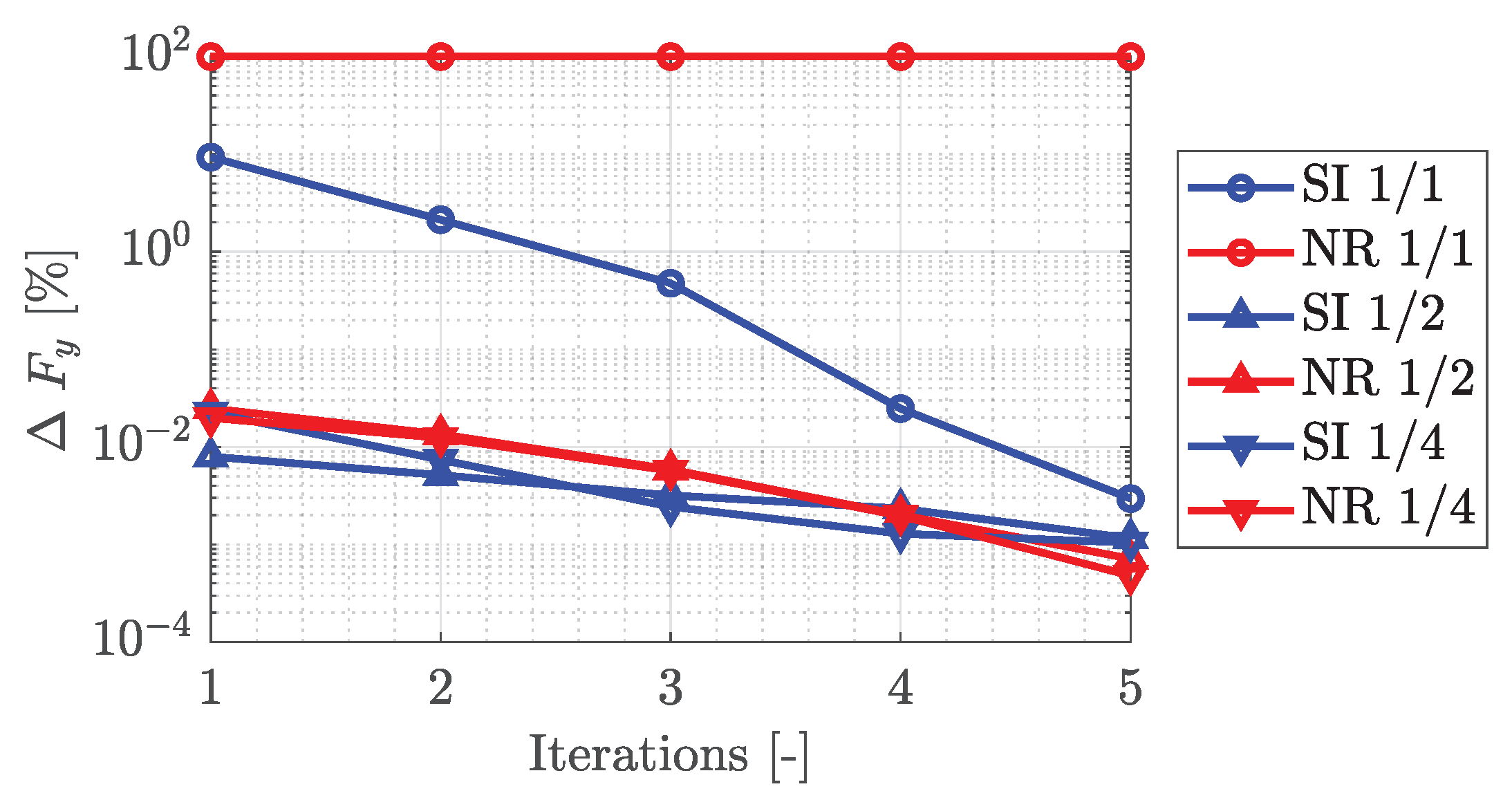
3.1. Successive Iterations (SI)
3.2. Newton-Raphson
4. Post-Processing
4.1. Force Calculation
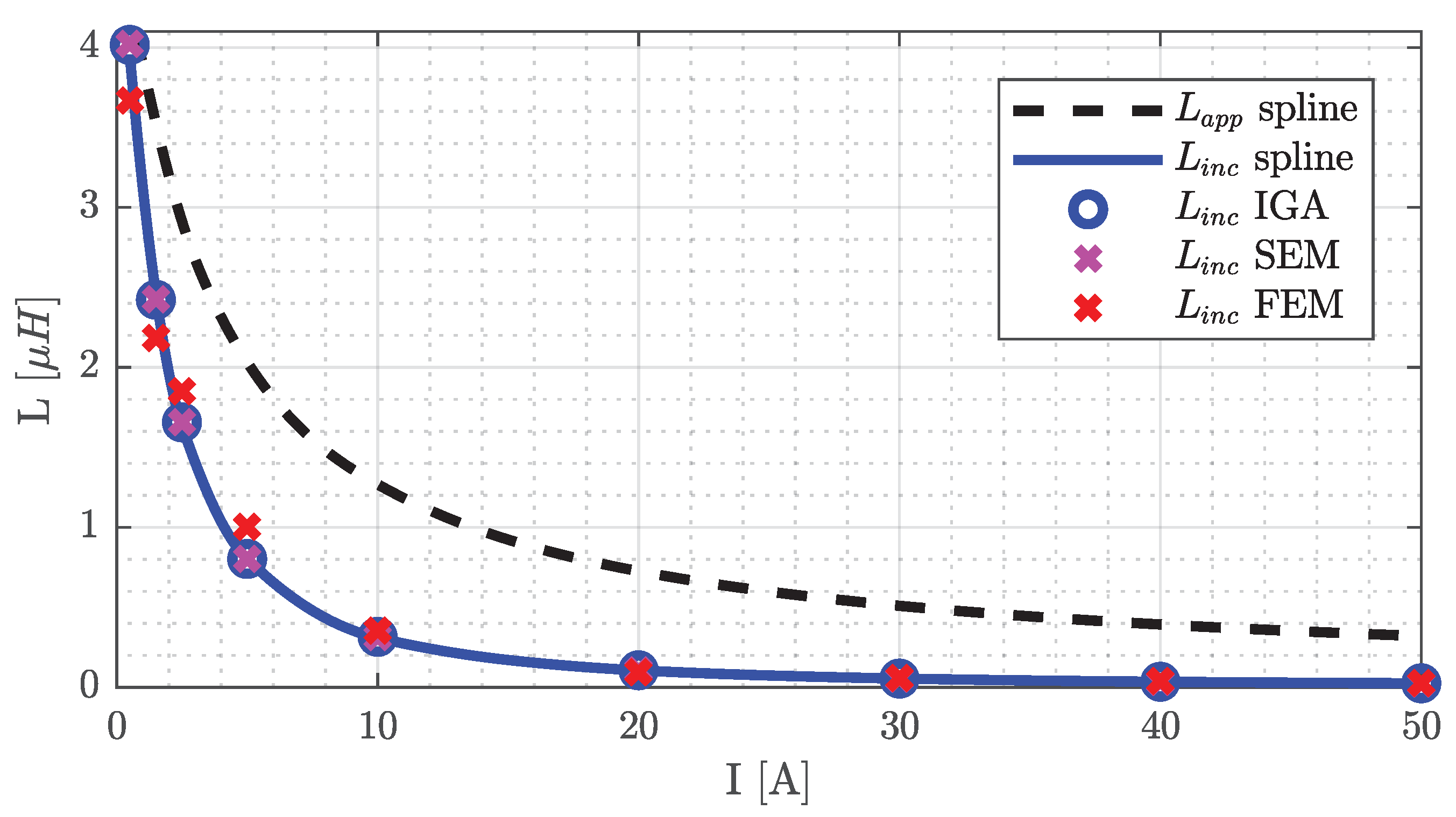
4.2. Flux Linkage and Inductances Calculation
5. Results
5.1. Benchmark I
5.2. Benchmark II
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Van Beek, T.A.; Jansen, J.W.; Gysen, B.L.J.; Lomonova, E.A. Comparison of direct and indirectly liquid-cooled coreless linear actuators with multi-layer coils. In Proceedings of the 11th International Symposium on Linear Drives for Industry Applications (LDIA), Osaka, Japan, 6–8 September 2017; pp. 1–6. [Google Scholar]
- Bao, J.; Gysen, B.L.J.; Boynov, K.; Paulides, J.J.H.; Lomonova, E.A. Torque Ripple Reduction for 12-Stator/10- Rotor-Pole Variable Flux Reluctance Machines by Rotor Skewing or Rotor Teeth Non-Uniformity. IEEE Trans. Magn. 2017, 53, 1–5. [Google Scholar] [CrossRef]
- Djelloul-Khedda, Z.; Boughrara, K.; Dubas, F.; Ibtiouen, R. Nonlinear Analytical Prediction of Magnetic Field and Electromagnetic Performances in Switched Reluctance Machines. IEEE Trans. Magn. 2017, 53, 1–11. [Google Scholar] [CrossRef]
- Mohammed, B.; Boughrara, K.; Dubas, F.; Ibtiouen, R. Two-Dimensional Exact Subdomain Technique of Switched Reluctance Machines with Sinusoidal Current Excitation. Math. Comput. Appl. 2018, 23, 59. [Google Scholar]
- Gysen, B.L.J.; Ilhan, E.; Meessen, K.J.; Paulides, J.J.H.; Lomonova, E.A. Modeling of flux switching permanent magnet machines with Fourier analysis. IEEE Trans. Magn. 2010, 46, 1499–1502. [Google Scholar] [CrossRef]
- Lee, S.; Lee, J.; Cho, S. Isogeometric Shape Optimization of Ferromagnetic Materials in Magnetic Actuators. IEEE Trans. Magn. 2016, 52, 1–8. [Google Scholar] [CrossRef]
- Dang Manh, N.; Evgrafov, A.; Gravesen, J.; Lahaye, D. Iso-geometric shape optimization of magnetic density separators. COMPEL Int. J. Comput. Math. Electr. Electron. Eng. 2014, 33, 1416–1433. [Google Scholar] [CrossRef]
- Gangl, P.; Amstutz, S.; Langer, U. Topology Optimization of Electric Motor Using Topological Derivative for Nonlinear Magnetostatics. IEEE Trans. Magn. 2016, 52, 1–4. [Google Scholar] [CrossRef]
- Ramakrishnan, K.; Curti, M.; Zarko, D.; Mastinu, G.; Paulides, J.J.H.; Lomonova, E.A. Comparative analysis of various methods for modelling surface permanent magnet machines. IET Electr. Power Appl. 2017, 11, 540–547. [Google Scholar] [CrossRef]
- Kopriva, D.A. Implementing Spectral Methods for Partial Differential Equations; Springer: Dordrecht, The Netherlands, 2009. [Google Scholar]
- Vázquez, R. A new design for the implementation of isogeometric analysis in Octave and Matlab: GeoPDEs 3.0. Comput. Math. Appl. 2016, 72, 523–554. [Google Scholar] [CrossRef]
- De Gersem, H. Coupled finite element - spectral element discretization for models with circular inclusions and far field domains. In Proceedings of the 4th International Conference on Computation in Electromagnetics, Bournemouth, UK, 8–11 April 2002; p. 27. [Google Scholar]
- Curti, M.; van Beek, T.A.; Jansen, J.W.; Gysen, B.L.J.; Lomonova, E.A. General Formulation of the Magnetostatic Field and Temperature Distribution in Electrical Machines Using Spectral Element Analysis. IEEE Trans. Magn. 2018, 54, 1–9. [Google Scholar] [CrossRef]
- Bontinck, Z.; Corno, J.; Gersem, H.D.; Kurz, S.; Pels, A.; Schöps, S.; Wolf, F.; de Falco, C.; Dölz, J.; Vázquez, R.; et al. Recent Advances of Isogeometric Analysis in Computational Electromagnetics. arXiv, 2017; arXiv:1709.06004. [Google Scholar]
- Mahariq, I. On the application of the spectral element method in electromagnetic problems involving domain decomposition. Turk. J. Electr. Eng. Comput. Sci. 2017, 25, 1059–1069. [Google Scholar] [CrossRef]
- Gyselinck, J.; Vandevelde, L.; Melkebeek, J.; Dular, P. Complementary two-dimensional finite element formulations with inclusion of a vectorized Jiles-Atherton model. COMPEL Int. J. Comput. Math. Electr. Electron. Eng. 2004, 23, 959–967. [Google Scholar] [CrossRef]
- Flux 12.2 User’s Guide. 2016. Available online: https://altairhyperworks.com/product/flux (accessed on 29 January 2019).
- Trefethen, L.N. Spectral Methods in MATLAB; Society for Industrial and Applied Mathematics: Philadelphia, PA, USA, 2000. [Google Scholar]
- Kopriva, D.A.; Gassner, G. On the quadrature and weak form choices in collocation type discontinuous Galerkin spectral element methods. J. Sci. Comput. 2010, 44, 136–155. [Google Scholar] [CrossRef]
- Curti, M.; Paulides, J.J.H.; Lomonova, E.A. Magnetic Modeling of a Linear Synchronous Machine with the Spectral Element Method. IEEE Trans. Magn. 2017, 53, 1–6. [Google Scholar] [CrossRef]
- Cottrell, J.A.; Hughes, T.J.; Bazilevs, Y. Isogeometric Analysis: Toward Integration of CAD and FEA; John Wiley & Sons: Hoboken, NJ, USA, 2009. [Google Scholar]
- Buffa, A.; Sangalli, G.; Vázquez, R. Isogeometric methods for computational electromagnetics: B-spline and T-spline discretizations. J. Comput. Phys. 2014, 257, 1291–1320. [Google Scholar] [CrossRef]
- Da Veiga, L.B.; Buffa, A.; Sangalli, G.; Vázquez, R. Mathematical analysis of variational isogeometric methods. Acta Numer. 2014, 23, 157–287. [Google Scholar] [CrossRef]
- Dlala, E.; Arkkio, A. Analysis of the Convergence of the Fixed-Point Method Used for Solving Nonlinear Rotational Magnetic Field Problems. IEEE Trans. Magn. 2008, 44, 473–478. [Google Scholar] [CrossRef]
- Dlala, E.; Arkkio, A. General formulation for the Newton-Raphson method and the fixed-point method in finite-element programs. In Proceedings of the XIX International Conference on Electrical Machines, Rome, Italy, 6–8 September 2010; pp. 1–5. [Google Scholar]
- Yan, S.; Jin, J.M.; Wang, C.F.; Kotulski, J.D. Numerical study of a time-domain finite element method for nonlinear magnetic problems in three dimensions. Prog. Electromagn. Res. 2015, 153, 69–91. [Google Scholar] [CrossRef]
- Fujiwara, K.; Okamoto, Y.; Kameari, A.; Ahagon, A. The Newton-Raphson method accelerated by using a line search—Comparison between energy functional and residual minimization. IEEE Trans. Magn. 2005, 41, 1724–1727. [Google Scholar] [CrossRef]
- Li, S.; Cui, X. An edge-based smoothed finite element method for nonlinear magnetostatic and eddy current analysis. Appl. Math. Model. 2018, 62, 287–302. [Google Scholar] [CrossRef]
- Vande Sande, H.; Boonen, T.; De Gersem, H.; Henrotte, F.; Hameyer, K. A hybrid Picard-Newton acceleration scheme for non-linear timeharmonic problems. In Proceedings of the 6th International Conference on Computational Electromagnetics, Aachen, Germany, 4–6 April 2006; pp. 1–2. [Google Scholar]
- Kim, D.H.; Lowther, D.A.; Sykulski, J.K. Efficient force calculations based on continuum sensitivity analysis. IEEE Trans. Magn. 2005, 41, 1404–1407. [Google Scholar]
- Kim, D.H.; Lowther, D.A.; Sykulski, J.K. Efficient Global and Local Force Calculations Based on Continuum Sensitivity Analysis. IEEE Trans. Magn. 2007, 43, 1177–1180. [Google Scholar] [CrossRef]
- Bottesi, O.; Alberti, L.; Sabariego, R.V.; Gyselinck, J. Finite element small-signal simulation of electromagnetic devices considering eddy currents in the laminations. IEEE Trans. Magn. 2017, 53, 1–8. [Google Scholar] [CrossRef]
- El-Murr, G.; Giaouris, D.; Finch, J.W. Online cross-coupling and self incremental inductances determination of salient permanent magnet synchronous machines. In Proceedings of the 5th IET International Conference on Power Electronics, Machines and Drives (PEMD 2010), Brighton, UK, 19–21 April 2010; pp. 1–4. [Google Scholar]
- Li, G.J.; Zhu, Z.Q.; Jewell, G. Performance investigation of hybrid excited switched flux permanent magnet machines using frozen permeability method. IET Electr. Power Appl. 2015, 9, 586–594. [Google Scholar] [CrossRef]
- Lin, Z.; Reay, D.; Williams, B.; He, X. High-performance current control for switched reluctance motors based on on-line estimated parameters. IET Electr. Power Appl. 2010, 4, 67–74. [Google Scholar] [CrossRef]
- Hemmati, S.; Kojoori, S.S.; Saied, S.; Lipo, T.A. Modelling and experimental validation of internal short-circuit fault in salient-pole synchronous machines using numerical gap function including stator and rotor core saturation. IET Electr. Power Appl. 2013, 7, 391–399. [Google Scholar] [CrossRef]
- Wang, L.; Aleksandrov, S.; Tang, Y.; Paulides, J.J.H.; Lomonova, E.A. Fault-tolerant electric drive and space-phasor modulation of flux-switching permanent magnet machine for aerospace application. IET Electr. Power Appl. 2017, 11, 1416–1423. [Google Scholar] [CrossRef]
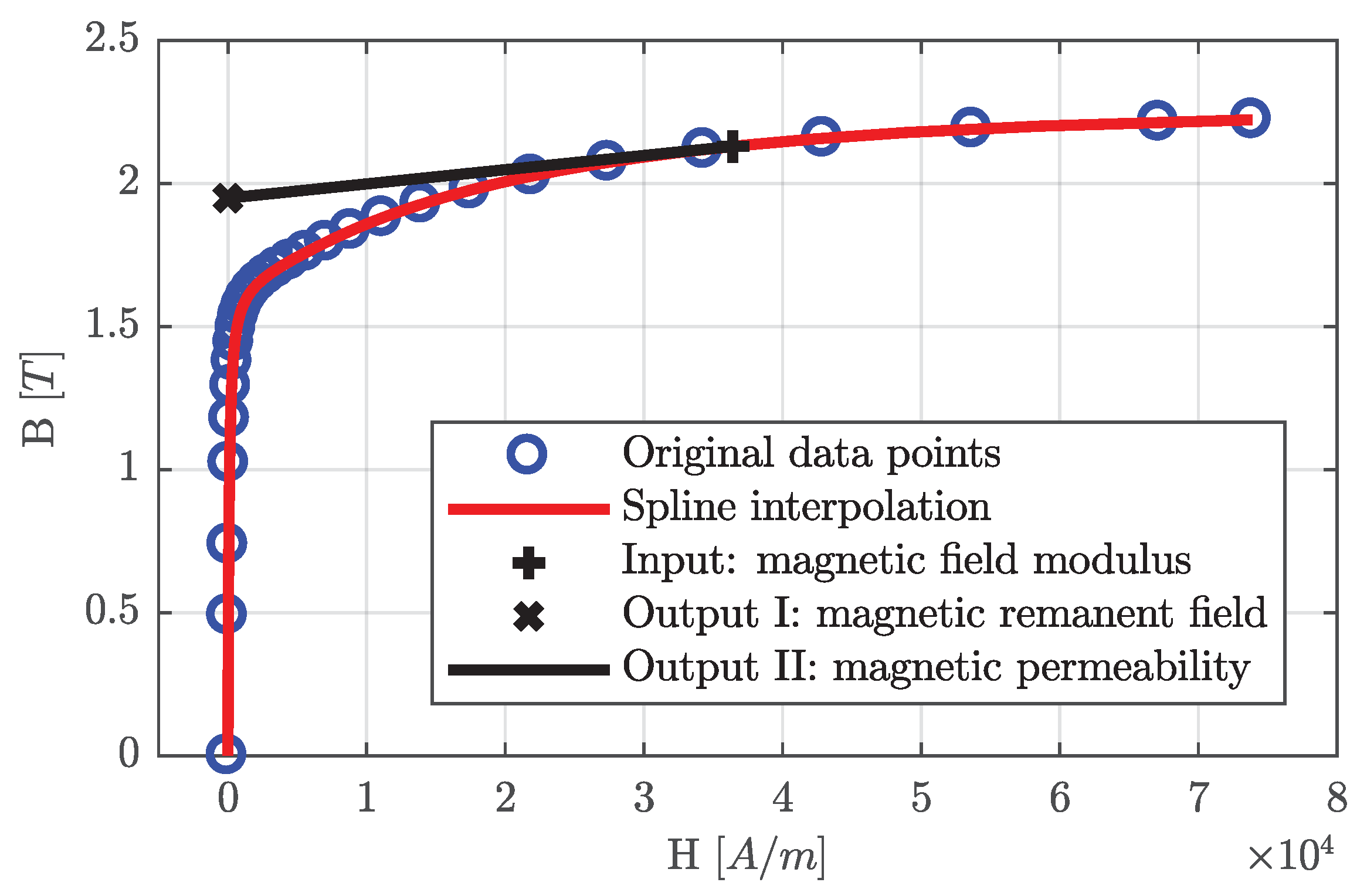
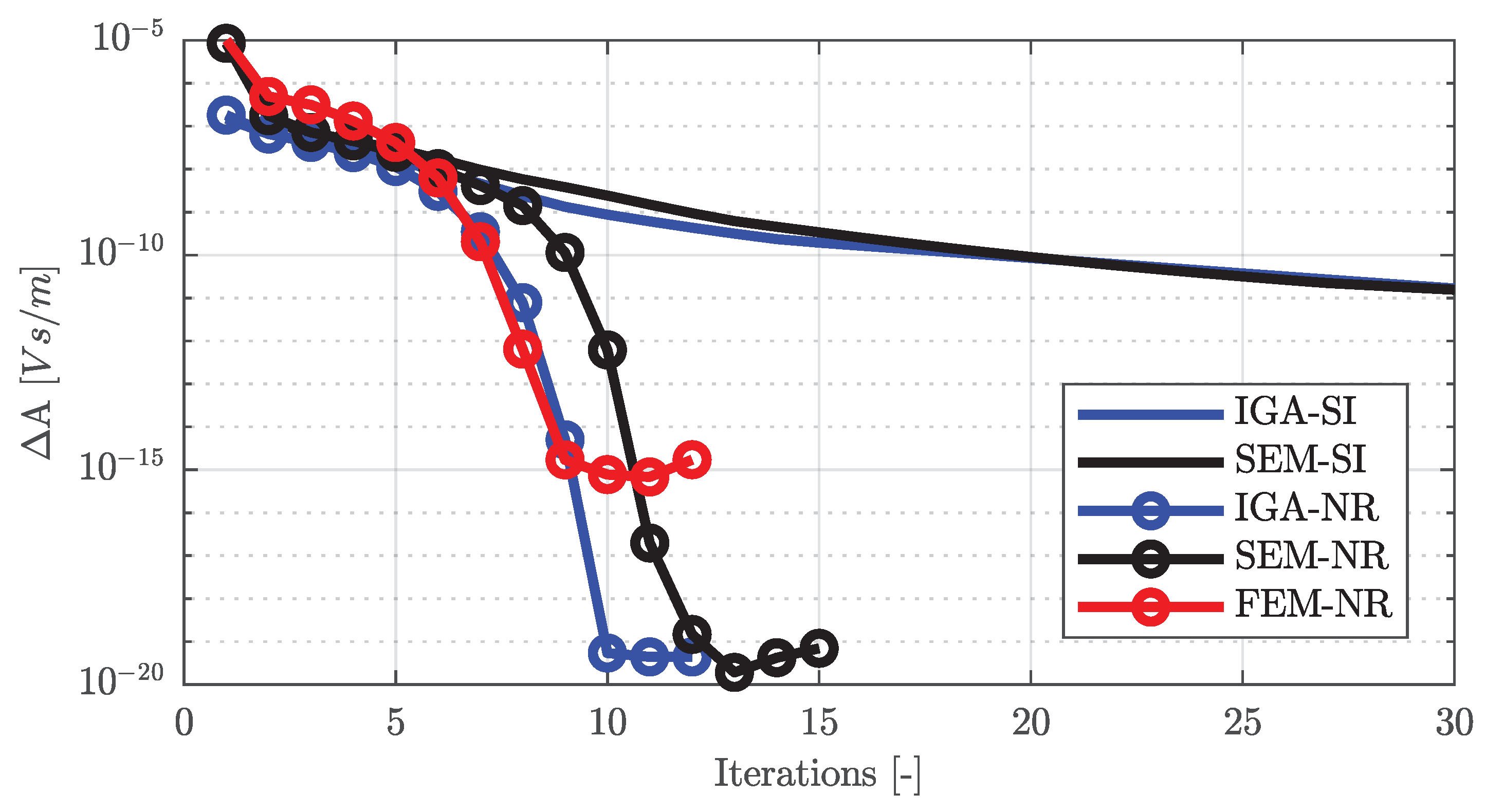
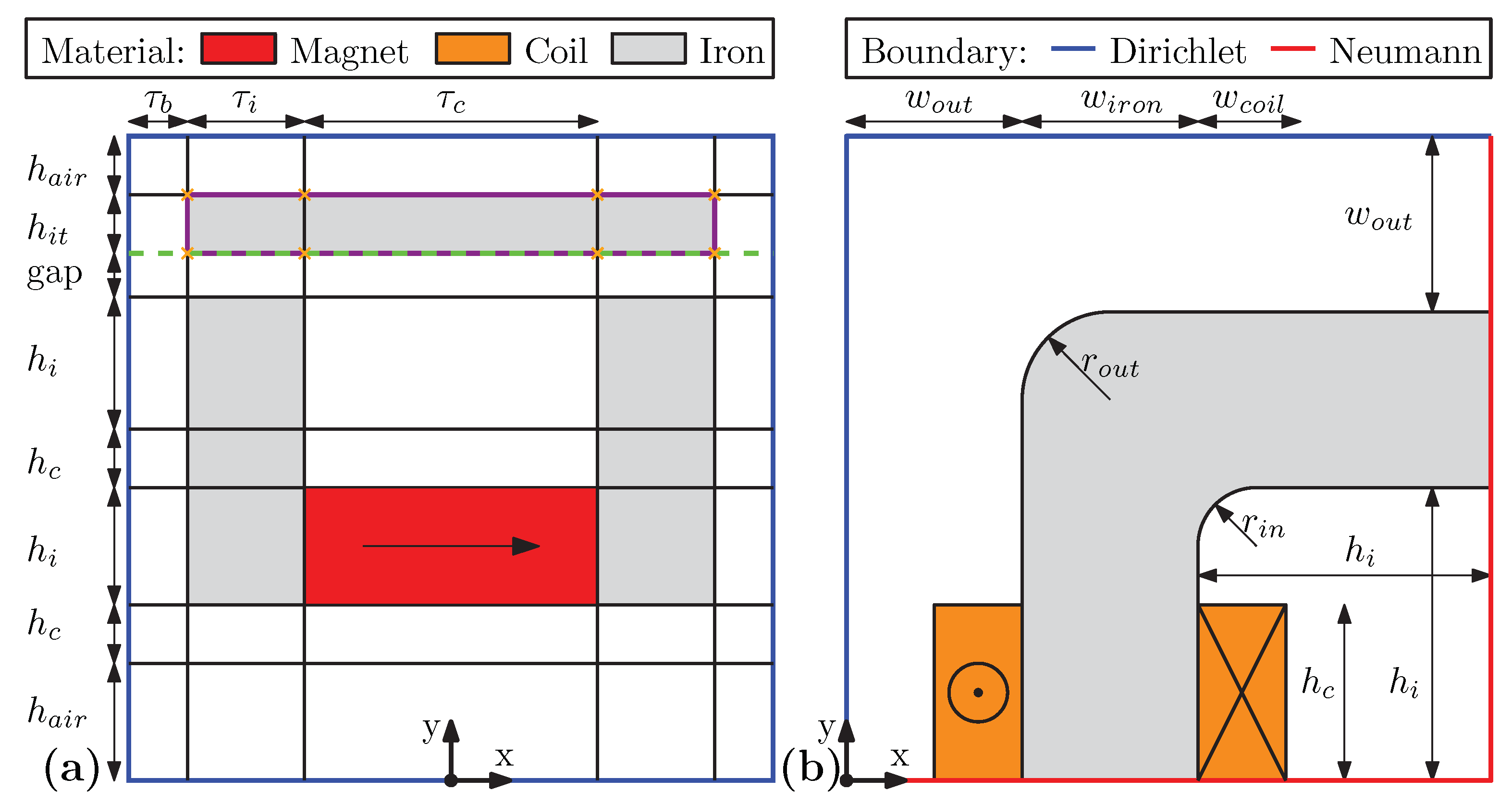
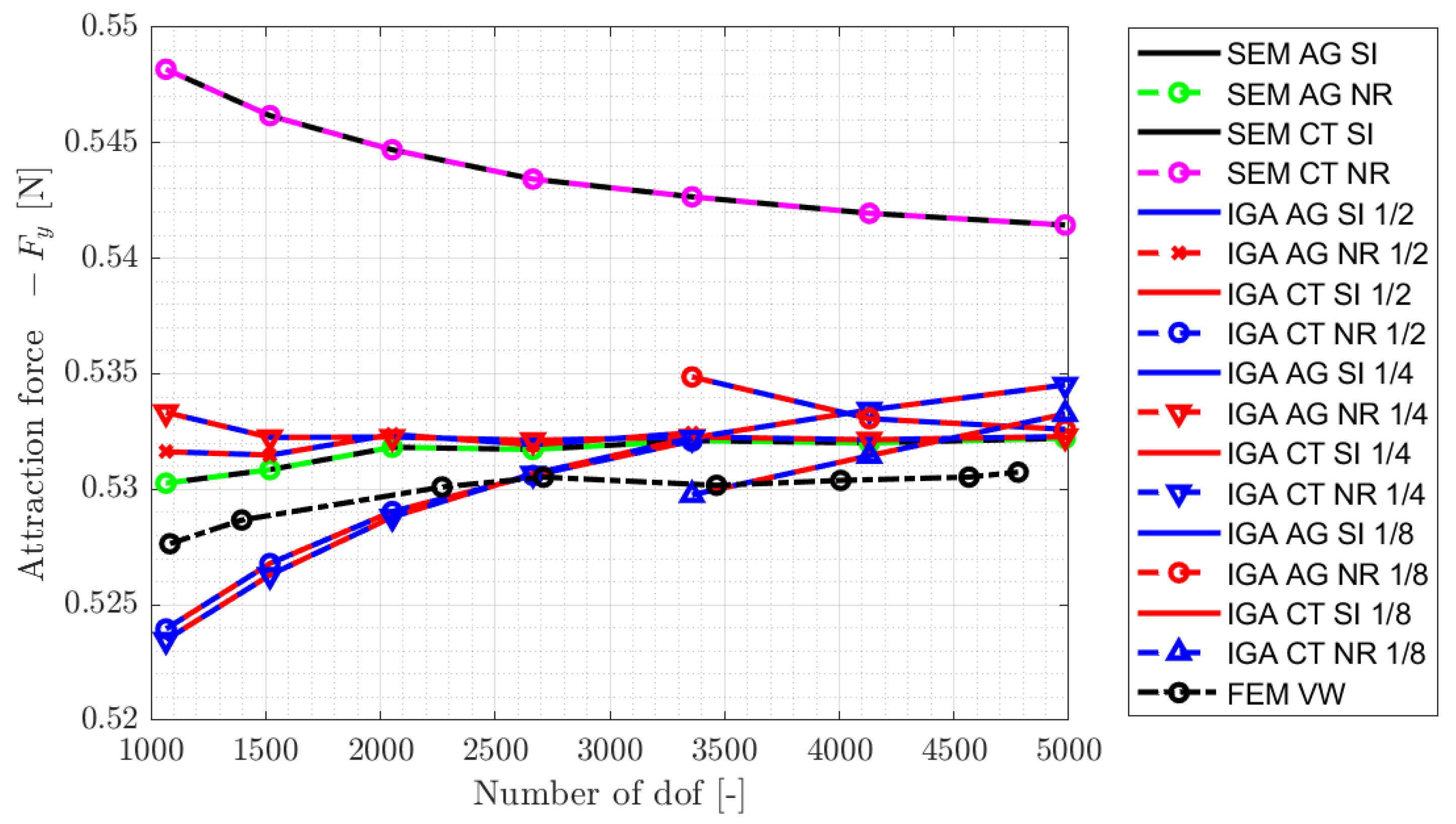
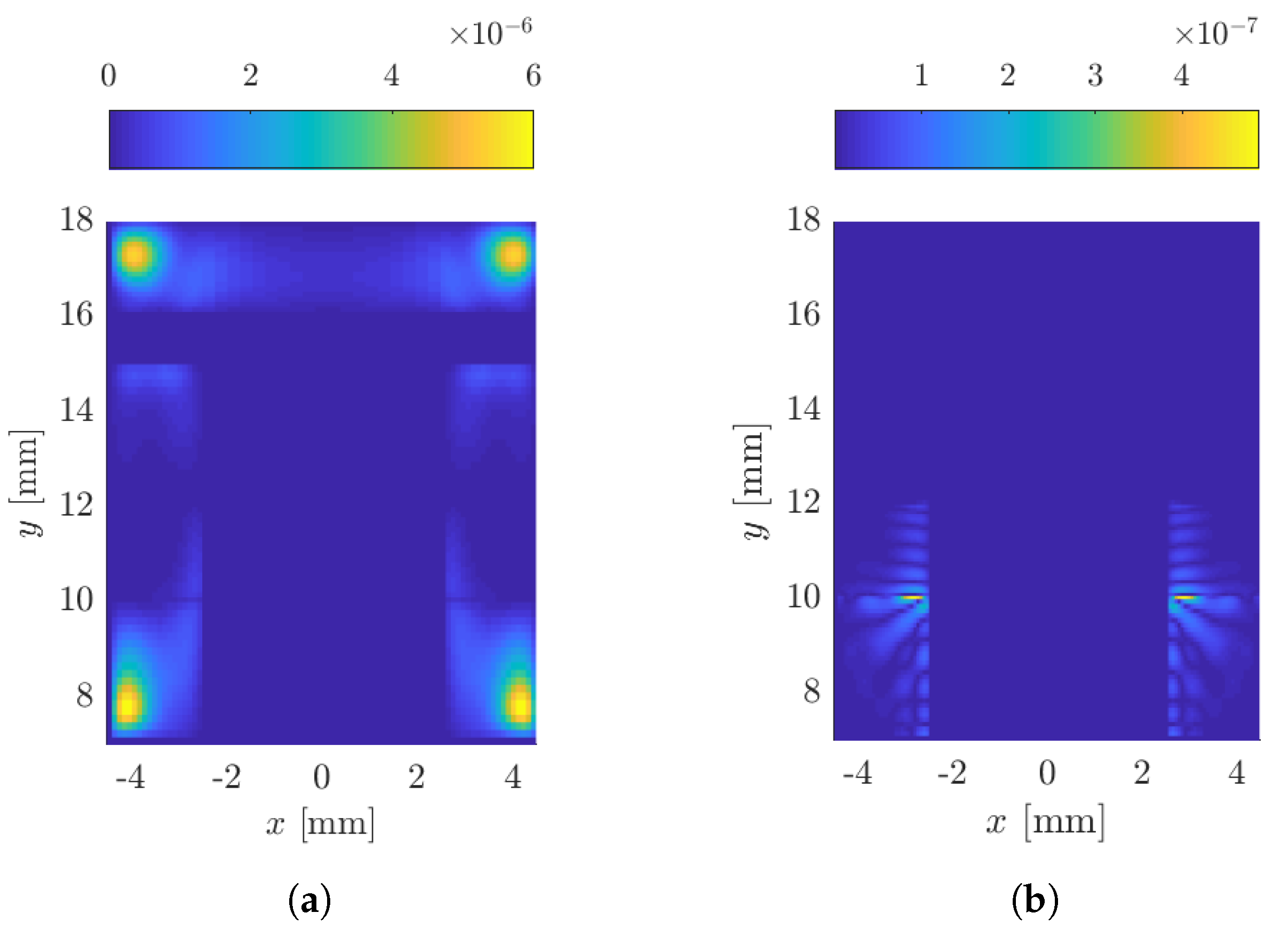
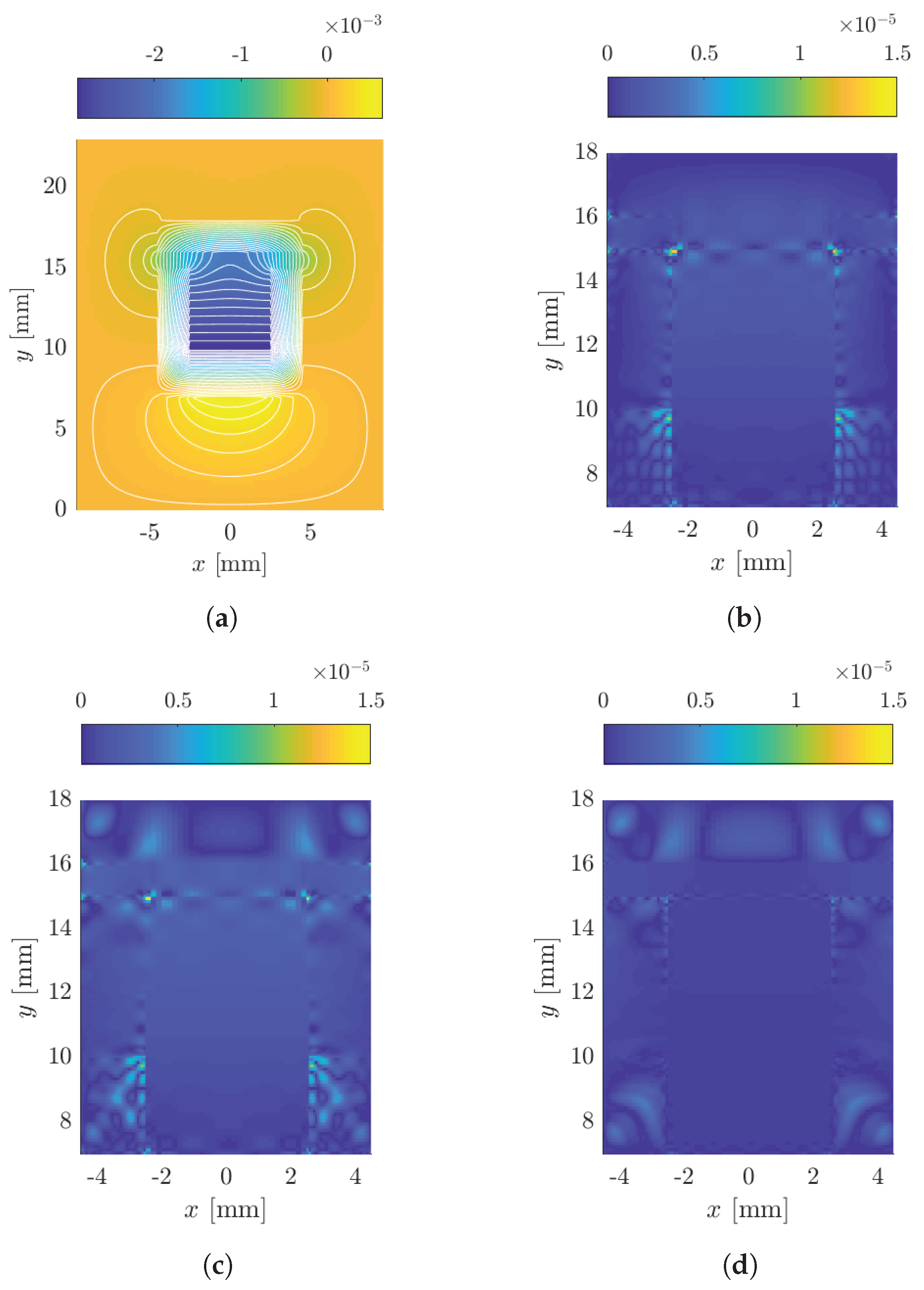
| Benchmark I Parameter | Value | Benchmark II Parameter | Value |
|---|---|---|---|
| 5 mm | 10 mm | ||
| 2 mm | 10 mm | ||
| 5 mm | 12 mm | ||
| 5 mm | 2 mm | ||
| 2 mm | 5 mm | ||
| 3 mm | 5 mm | ||
| 2 mm | 18 mm | ||
| gap | 1 mm |
| Method | Calculation | [N/mm] | [N/mm] |
| FEM | VW | ||
| IGA | MST - CT | ||
| MST - AG | |||
| SEM | MST - CT | ||
| MST - AG | |||
| Difference | Regarding from | FEM(VW) [%] | IGA(AG) [%] |
| FEM | VW | - | 0.157 |
| IGA | MST - CT | 0.780 | 0.624 |
| MST - AG | 0.130 | - | |
| SEM | MST - CT | 2.203 | 2.458 |
| MST - AG | 0.213 | 0.036 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Friedrich, L.A.J.; Curti, M.; Gysen, B.L.J.; Lomonova, E.A. High-Order Methods Applied to Nonlinear Magnetostatic Problems. Math. Comput. Appl. 2019, 24, 19. https://doi.org/10.3390/mca24010019
Friedrich LAJ, Curti M, Gysen BLJ, Lomonova EA. High-Order Methods Applied to Nonlinear Magnetostatic Problems. Mathematical and Computational Applications. 2019; 24(1):19. https://doi.org/10.3390/mca24010019
Chicago/Turabian StyleFriedrich, Léo A. J., Mitrofan Curti, Bart L. J. Gysen, and Elena A. Lomonova. 2019. "High-Order Methods Applied to Nonlinear Magnetostatic Problems" Mathematical and Computational Applications 24, no. 1: 19. https://doi.org/10.3390/mca24010019
APA StyleFriedrich, L. A. J., Curti, M., Gysen, B. L. J., & Lomonova, E. A. (2019). High-Order Methods Applied to Nonlinear Magnetostatic Problems. Mathematical and Computational Applications, 24(1), 19. https://doi.org/10.3390/mca24010019






