Application of Approximate Analytical Technique Using the Homotopy Perturbation Method to Study the Inclination Effect on the Thermal Behavior of Porous Fin Heat Sink
Abstract
:1. Introduction
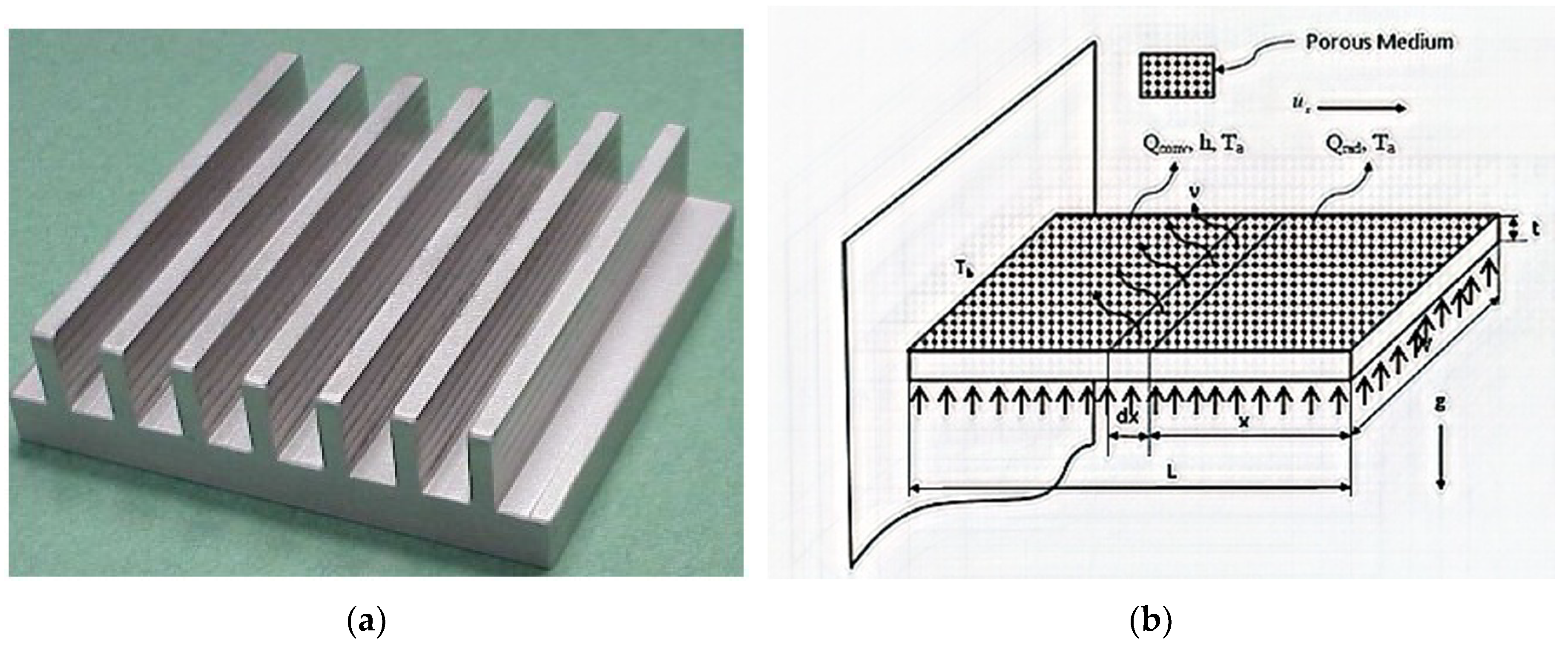
2. Problem Formulation
- Porous media is homogeneous and saturated with single-phase fluid.
- The interaction between the saturated fluid and medium is governed by Darcy’s model.
- Thermo-physical characteristics of the porous fin with that of the fluid are constant.
- Fin tip is adiabatic.
3. Method of Solution using HPM
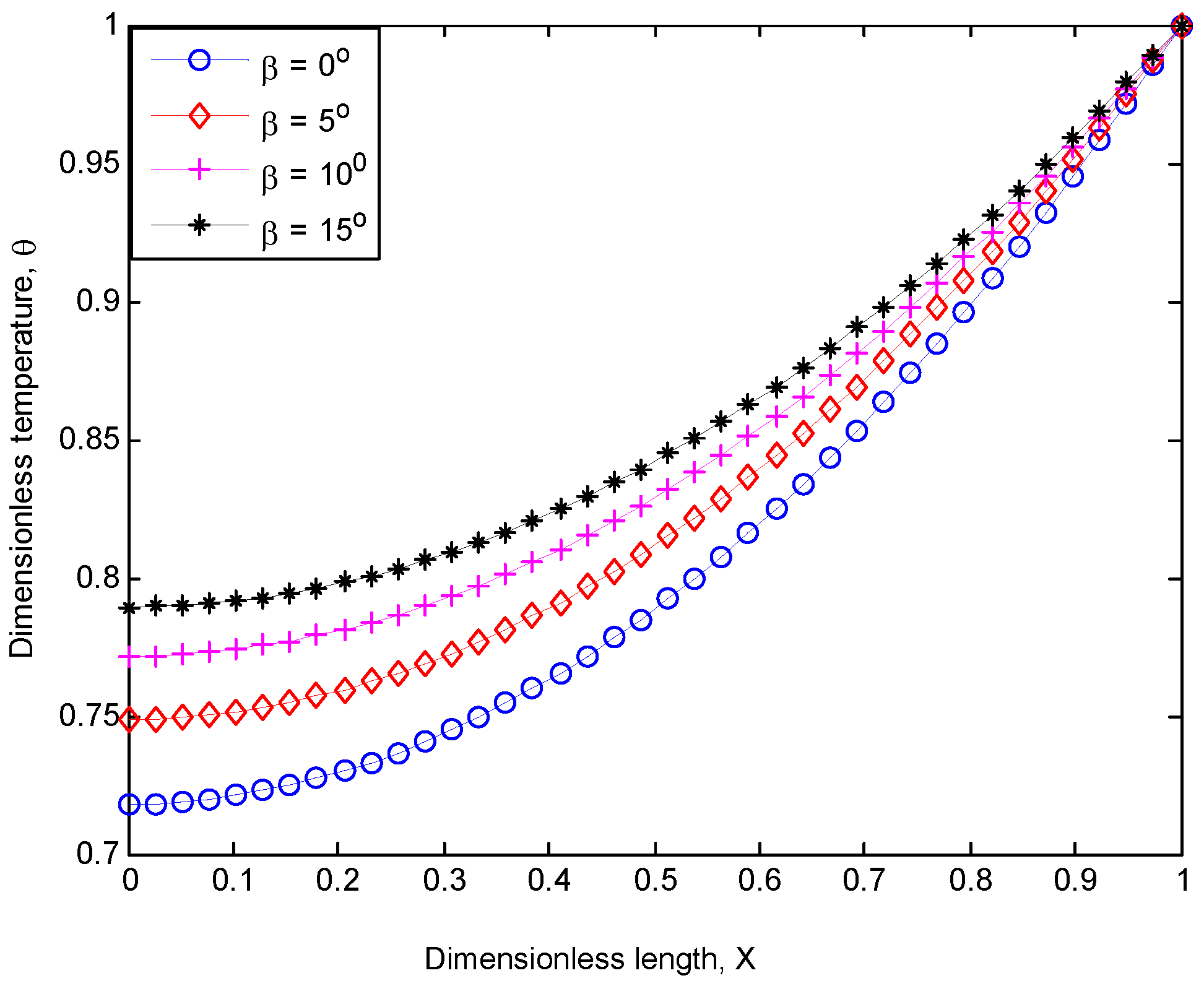
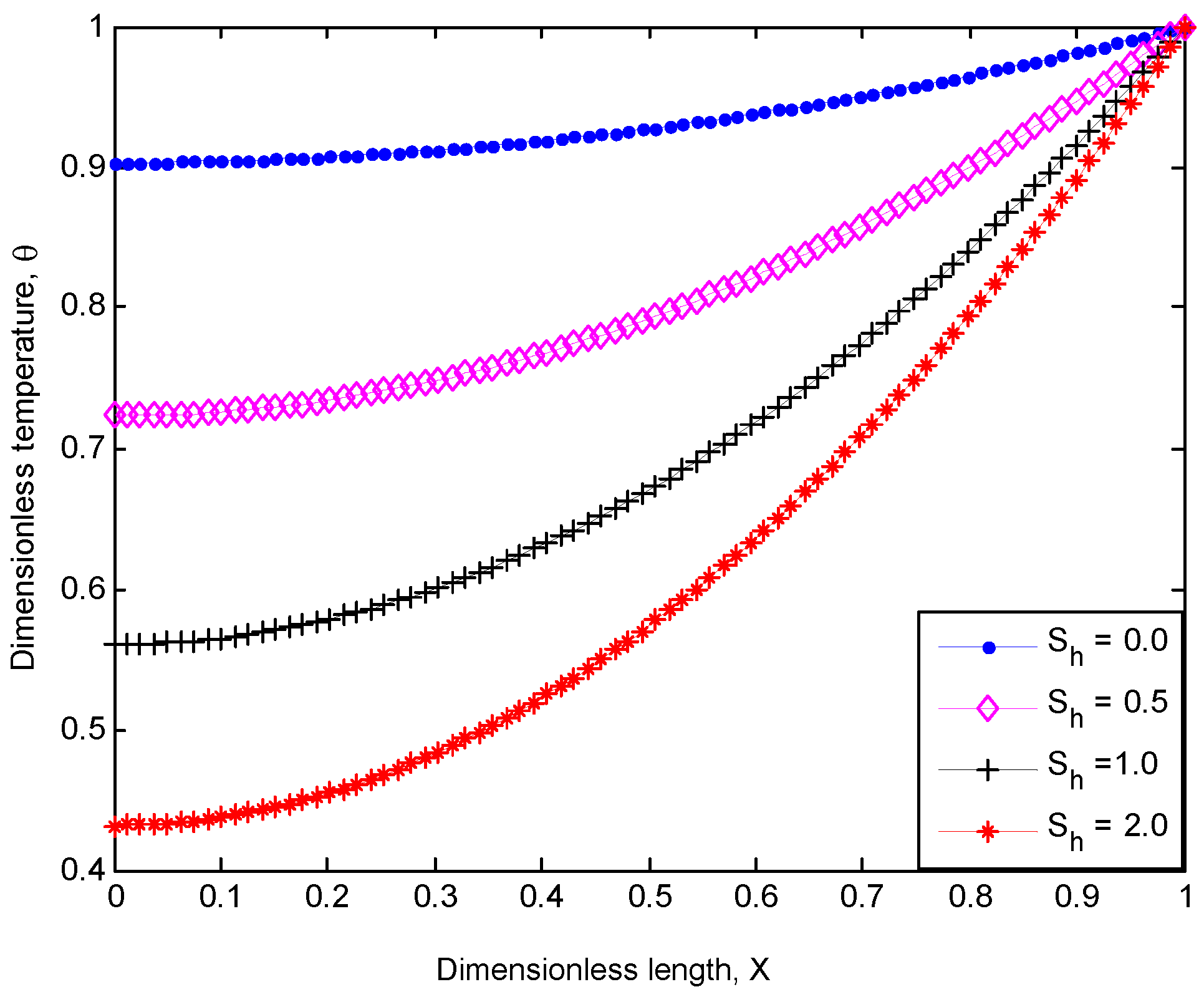
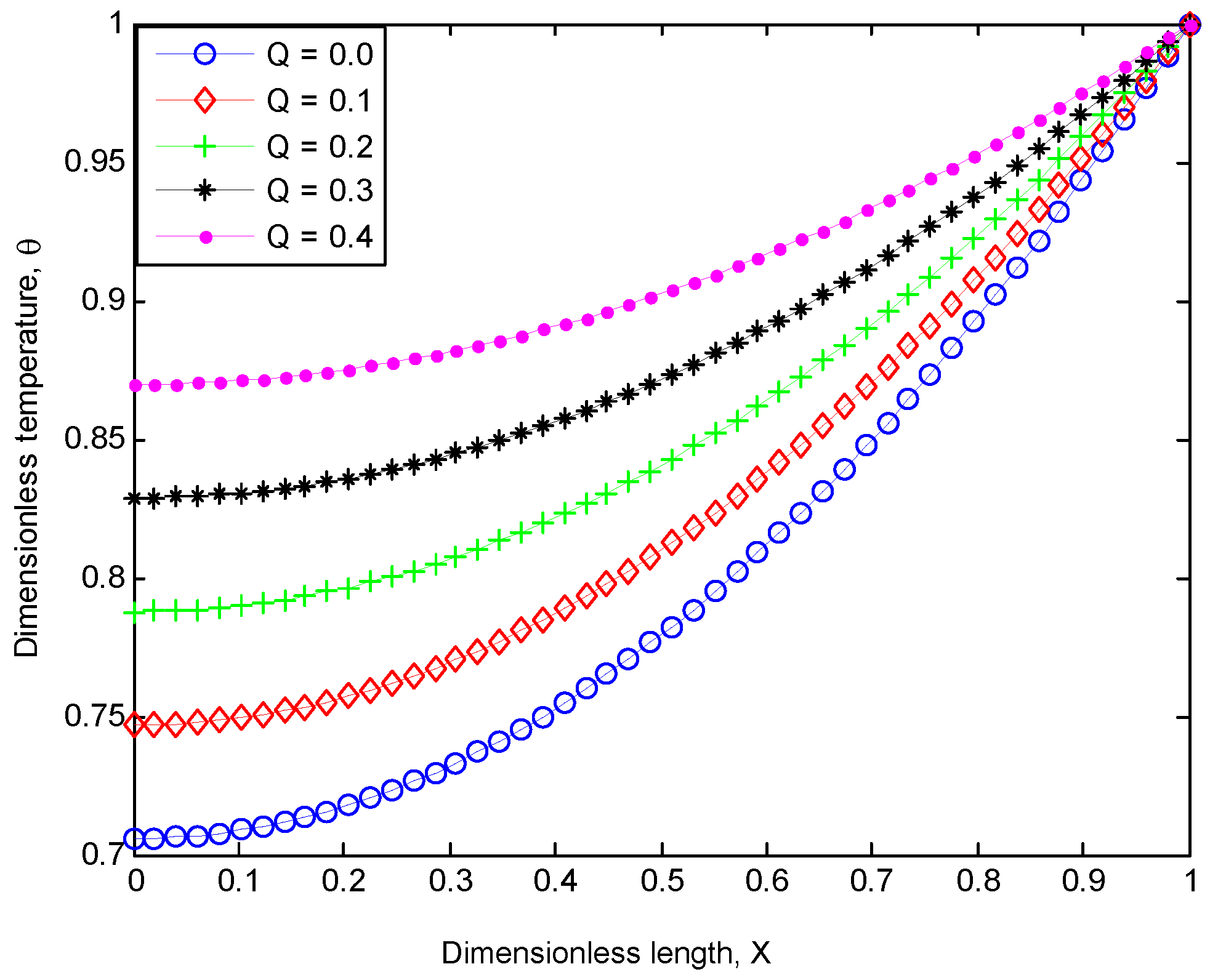
4. Results and Discussion
5. Conclusions
Author Contributions
Acknowledgements
Conflict of Interest
Abbreviations
| Terminology | |
| A | Fin cross-sectional area |
| Ab | Base area of the fin |
| As | Fin surface area |
| heff | Heat coefficient at fin base |
| cp | Specific heat of the fluid passing through the porous fin |
| K | Permeability |
| M | Thermo-geometric parameter |
| Saturated fluid mass flowage | |
| Nu | Nusselt number |
| P | Fin perimeter |
| t | Fin thickness |
| q | Rate of heat transfer |
| X | Dimensionless length |
| q | Internal heat generation |
| Gr | Grashoff’s number |
| β | Inclination angle. |
| Sh | Porosity term. |
| M | Convective heat parameter |
| T | Temperature |
| Ta | Ambient temperature |
| Tb | Temperature at the base of the fin |
| V | Average velocity of the fluid passing through the porous fin |
| Greek Symbols | |
| β | Inclination angle |
| θ | Temperature (Dimensionless) |
| η | Fin efficiency |
| βth | Coefficient of thermal expansion |
| υ | Kinematic viscosity |
| ρ | Fluid density |
| Subscripts | |
| s | Solid properties |
| f | Fluid properties |
| eff | Effective porous properties |
References
- Kiwan, S.; Al-Nimr, M.A. Using Porous Fins for Heat Transfer Enhancement. J. Heat Transf. 2000, 123, 790–795. [Google Scholar] [CrossRef]
- Ghasemi, S.E.; Hatami, M.; Ganji, D.D. Thermal analysis of convective fin with temperature-dependent thermal conductivity and heat generation. Case Stud. Thermal Eng. 2014, 4, 1–8. [Google Scholar] [CrossRef]
- Das, R. Estimation of parameters in a fin with temperature-dependent thermal conductivity and radiation. Proc. Inst. Mech. Eng. Part E J. Process Mech. Eng. 2016, 230, 474–485. [Google Scholar] [CrossRef]
- Kiwan, S.; Zeitoun, O. Natural convection in a horizontal cylindrical annulus using porous fins. Int. J. Numer. Methods Heat Fluid Flow 2008, 18, 618–634. [Google Scholar] [CrossRef]
- Gorla, R.S.R.; Bakier, A.Y. Thermal analysis of natural convection and radiation in porous fins. Int. Commun. Heat Mass Transf. 2011, 38, 638–645. [Google Scholar] [CrossRef]
- Saedodin, S.; Sadeghi, S. Temperature Distribution in Long Porous Fins in Natural Convection Condition. Middle East J. Sci. Res. 2013, 13, 812. [Google Scholar]
- Alshuraiaan, B.; Khanafer, K. The effect of the position of the heated thin porous fin on the laminar natural convection heat transfer in a differentially heated cavity. Int. Commun. Heat Mass Transf. 2016, 78, 190–199. [Google Scholar] [CrossRef]
- Sobamowo, M.G.; Kamiyo, O.M.; Adeleye, O.A. Thermal performance analysis of a natural convection porous fin with temperature-dependent thermal conductivity and internal heat generation. Therm. Sci. Eng. Prog. 2017, 1, 39–52. [Google Scholar] [CrossRef]
- Shateri, A.R.; Salahshour, B. Comprehensive thermal performance of convection–radiation longitudinal porous fins with various profiles and multiple nonlinearities. Int. J. Mech. Sci. 2018, 136, 252–263. [Google Scholar] [CrossRef]
- Oguntala, G.A.; Abd-Alhameed, R.A. Haar Wavelet Collocation Method for Thermal Analysis of Porous Fin with Temperature-Dependent Thermal Conductivity and Internal Heat Generation. J. Appl. Comput. Mech. 2017, 3, 185–191. [Google Scholar]
- Oguntala, G.; Abd-Alhameed, R.A.; Sobamowo, G.; Danjuma, I. Performance, Thermal Stability and Optimum Design Analyses of Rectangular Fin with Temperature-Dependent Thermal Properties and Internal Heat Generation. J. Comput. Appl. Mech. 2018, 49, 37–43. [Google Scholar]
- Ma, J.; Sun, Y.; Li, B.; Chen, H. Spectral collocation method for radiative–conductive porous fin with temperature dependent properties. Energy Convers. Manag. 2016, 111, 279–288. [Google Scholar] [CrossRef]
- Oguntala, G.; Abd-Alhameed, R.A. Thermal Analysis of Convective-Radiative Fin with Temperature-Dependent Thermal Conductivity Using Chebychev Spectral Collocation Method. J. Appl. Comput. Mech. 2018, 4, 87–94. [Google Scholar]
- Oguntala, G.A.; Abd-Alhameed, R.A.; Sobamowo, G.M.; Eya, N. Effects of particles deposition on thermal performance of a convective-radiative heat sink porous fin of an electronic component. Therm. Sci. Eng. Prog. 2018, 6, 177–185. [Google Scholar] [CrossRef]
- Oguntala, G.; Abd-Alhameed, R.A.; Sobamowo, G.; Abdullahi, H.S. Improved thermal management of computer microprocessors using cylindrical-coordinate micro-fin heat sink with artificial surface roughness. Eng. Sci. Technol. Int. J. 2018, 21, 736–744. [Google Scholar] [CrossRef]
- Ma, J.; Sun, Y.; Li, B. Simulation of combined conductive, convective and radiative heat transfer in moving irregular porous fins by spectral element method. Int. J. Therm. Sci. 2017, 118, 475–487. [Google Scholar] [CrossRef]
- Jayesimi, L.; Oguntala, G. Prediction of Temperature distribution in Straight Fin with Variable Thermal Conductivity and Internal Heat Generation using Legendre Wavelet Collocation Method. J. Comput. Appl. Mech. 2017, 48, 217–224. [Google Scholar]
- Roy, P.K.; Mondal, H.; Mallick, A. A decomposition method for convective–radiative fin with heat generation. Ain Shams Eng. J. 2015, 6, 307–313. [Google Scholar] [CrossRef]
- Bhanja, D.; Kundu, B.; Mandal, P.K. Thermal Analysis of Porous Pin Fin Used for Electronic Cooling. Proc. Eng. 2013, 64, 956–965. [Google Scholar] [CrossRef]
- Torabi, M.; Yaghoobi, H. Series Solution for Convective-Radiative Porous Fin Using Differential Transformation Method. J. Porous Media 2013, 16, 341–349. [Google Scholar] [CrossRef]
- Moradi, A.; Hayat, T.; Alsaedi, A. Convection-radiation thermal analysis of triangular porous fins with temperature-dependent thermal conductivity by DTM. Energy Convers. Manag. 2014, 77, 70–77. [Google Scholar] [CrossRef]
- Oguntala, G.; Abd-Alhameed, R.A. Performance of convective-radiative porous fin heat sink under the influence of particle deposition and adhesion for thermal enhancement of electronic components. Karbala Int. J. Mod. Sci. 2018, 4, 297–312. [Google Scholar] [CrossRef]
- Coşkun, S.B.; Atay, M.T. Fin efficiency analysis of convective straight fins with temperature-dependent thermal conductivity using variational iteration method. Appl. Therm. Eng. 2008, 28, 2345–2352. [Google Scholar] [CrossRef]
- Hoshyar, H.; Ganji, D.D.; Abbasi, M. Determination of Temperature Distribution for Porous Fin with Temperature-Dependent Heat Generation by Homotopy Analysis Method. J. Appl. Mech. Eng. 2015, 4, 153. [Google Scholar]
- Mosayebidorcheh, S.; Sheikholeslami, M.; Hatami, M.; Ganji, D.D. Analysis of Turbulent MHD Couette Nanofluid Flow and Heat Transfer Using Hybrid DTM–FDM. Particuology 2016, 26, 95–101. [Google Scholar] [CrossRef]
- Amirkolaei, R.S.; Ganji, D.D.; Slarian, H. Determination of Temperature Distribution for Porous Fin Which is Exposed to Uniform Magnetic Field to a Vertical Isothermal Surface by Homotopy Analysis Method and Collocation Method. Indian J. Sci. Res. 2014, 1, 215–222. [Google Scholar]
- Hoshyar, H.; Rahimipetroudi, I.; Ganji, D.D.; Majidian, A.R. Thermal performance of porous fins with temperature-dependent heat generation via the homotopy perturbation method and Collocation method. J. Appl. Math. Comput. Mech. 2015, 14, 53–65. [Google Scholar] [CrossRef]
- Tari, I.; Mehrtash, M. Natural convection heat transfer from horizontal and slightly inclined plate-fin heat sinks. Appl. Therm. Eng. 2013, 61, 728–736. [Google Scholar] [CrossRef]
- Ayaskanta, A.; Huang, L.; Simon, T.; Yeom, T.; North, M.; Cui, T. Heat Transfer Enhancement of a Heat Sink by Inclined Synthetic Jets for Electronics Cooling. In Proceedings of the ASME 2013 7th International Conference on Energy Sustainability and the ASME 2013 11th International Conference on Fuel Cell Science, Engineering and Technology, Minneapolis, MN, USA, 14–19 July 2013. [Google Scholar]
- Oguntala, G.; Abd-Alhameed, R.A.; Sobamowo, G. On the effect of magnetic field on thermal performance of convective-radiative fin with temperature-dependent thermal conductivity. Karbala Int. J. Mod. Sci. 2018, 4, 1–11. [Google Scholar] [CrossRef]

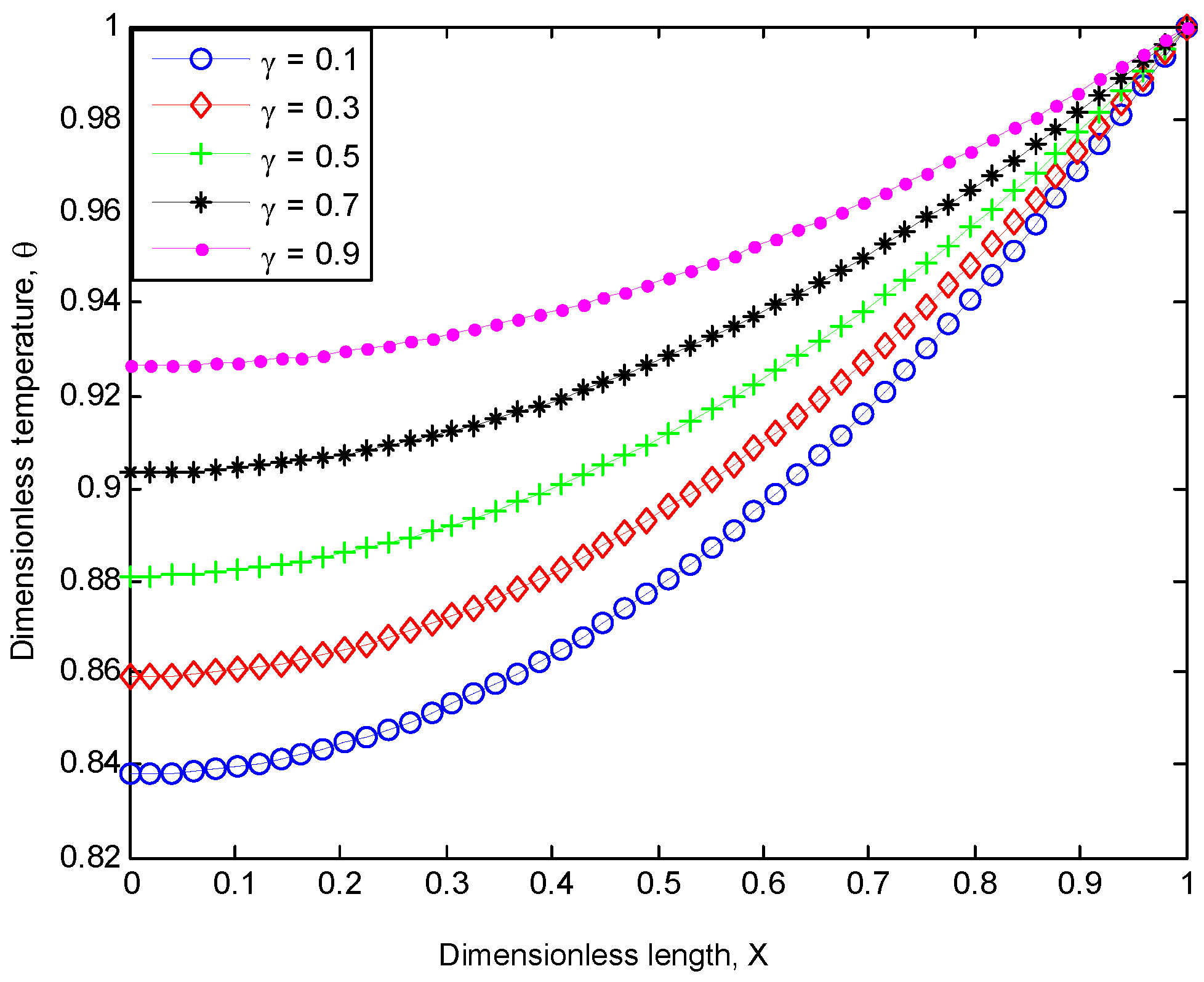
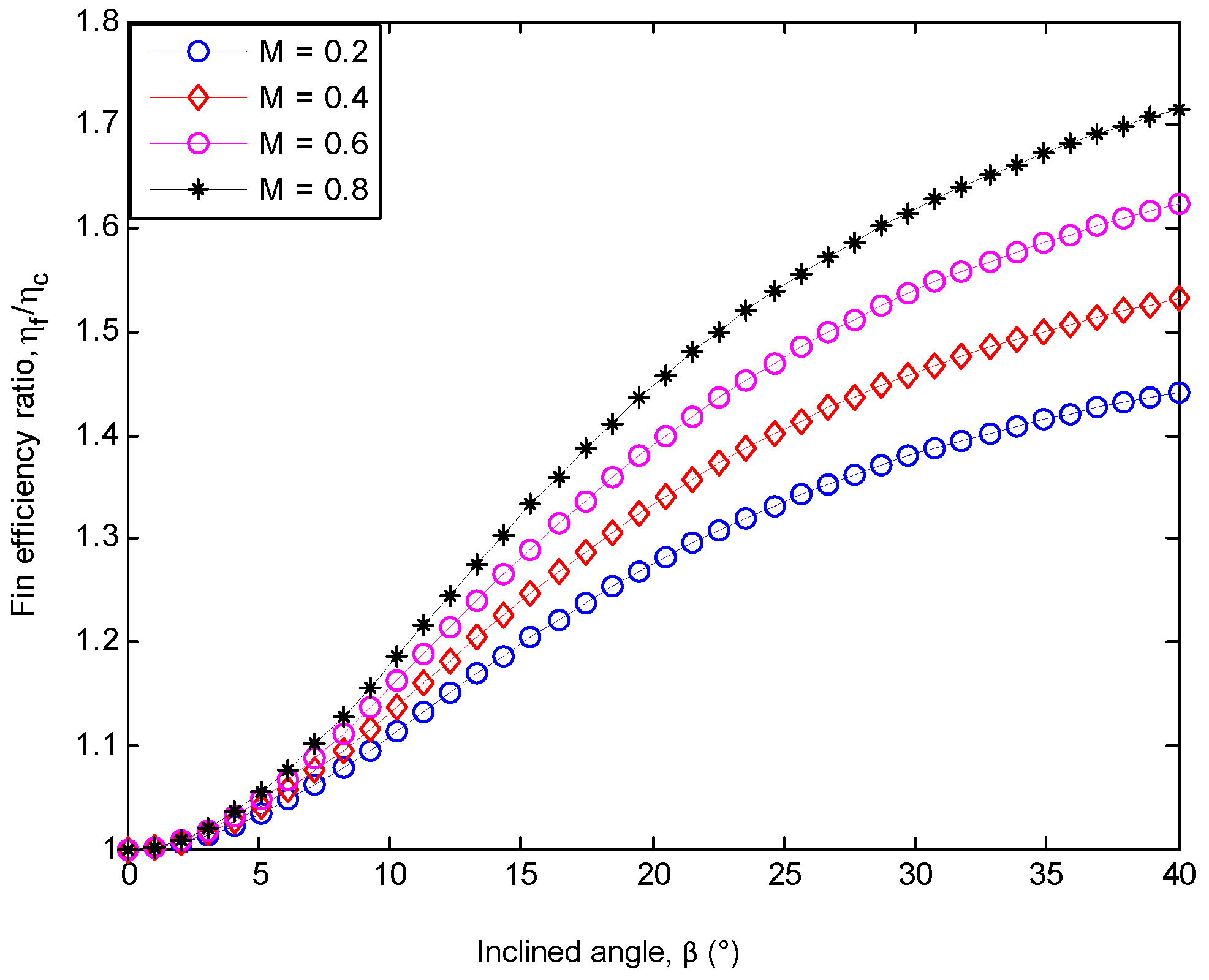

| x | Numerical Method (Runge-Kutta) | HPM (Present Study) | Absolute Error |
|---|---|---|---|
| 0.00 | 0.863499231 | 0.863499664 | 0.000000433 |
| 0.05 | 0.863828568 | 0.863829046 | 0.000000478 |
| 0.10 | 0.864817090 | 0.864817539 | 0.000000449 |
| 0.15 | 0.866466182 | 0.866465743 | 0.000000439 |
| 0.20 | 0.868776709 | 0.868776261 | 0.000000448 |
| 0.25 | 0.871751555 | 0.871751104 | 0.000000451 |
| 0.30 | 0.875393859 | 0.875393404 | 0.000000455 |
| 0.35 | 0.879707472 | 0.879707010 | 0.000000462 |
| 0.40 | 0.884696967 | 0.884696500 | 0.000000467 |
| 0.45 | 0.890367650 | 0.890367181 | 0.000000469 |
| 0.50 | 0.896725569 | 0.896725096 | 0.000000473 |
| 0.55 | 0.903777531 | 0.903777060 | 0.000000471 |
| 0.60 | 0.911531120 | 0.911530658 | 0.000000462 |
| 0.65 | 0.919994710 | 0.919994259 | 0.000000451 |
| 0.70 | 0.929177488 | 0.929177056 | 0.000000432 |
| 0.75 | 0.939089476 | 0.939089079 | 0.000000397 |
| 0.80 | 0.949741555 | 0.949741203 | 0.000000352 |
| 0.85 | 0.961145491 | 0.961145189 | 0.000000302 |
| 0.90 | 0.973313964 | 0.973313764 | 0.000000200 |
| 0.95 | 0.986260599 | 0.986260549 | 0.000000005 |
| 1.00 | 1.000000000 | 1.000000000 | 0.000000000 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Oguntala, G.; Sobamowo, G.; Ahmed, Y.; Abd-Alhameed, R. Application of Approximate Analytical Technique Using the Homotopy Perturbation Method to Study the Inclination Effect on the Thermal Behavior of Porous Fin Heat Sink. Math. Comput. Appl. 2018, 23, 62. https://doi.org/10.3390/mca23040062
Oguntala G, Sobamowo G, Ahmed Y, Abd-Alhameed R. Application of Approximate Analytical Technique Using the Homotopy Perturbation Method to Study the Inclination Effect on the Thermal Behavior of Porous Fin Heat Sink. Mathematical and Computational Applications. 2018; 23(4):62. https://doi.org/10.3390/mca23040062
Chicago/Turabian StyleOguntala, George, Gbeminiyi Sobamowo, Yinusa Ahmed, and Raed Abd-Alhameed. 2018. "Application of Approximate Analytical Technique Using the Homotopy Perturbation Method to Study the Inclination Effect on the Thermal Behavior of Porous Fin Heat Sink" Mathematical and Computational Applications 23, no. 4: 62. https://doi.org/10.3390/mca23040062
APA StyleOguntala, G., Sobamowo, G., Ahmed, Y., & Abd-Alhameed, R. (2018). Application of Approximate Analytical Technique Using the Homotopy Perturbation Method to Study the Inclination Effect on the Thermal Behavior of Porous Fin Heat Sink. Mathematical and Computational Applications, 23(4), 62. https://doi.org/10.3390/mca23040062







