Surface Roughness Modeling Using Q-Sequence
Abstract
:1. Introduction
2. Q-Sequence
3. Modifying the Q-Sequence
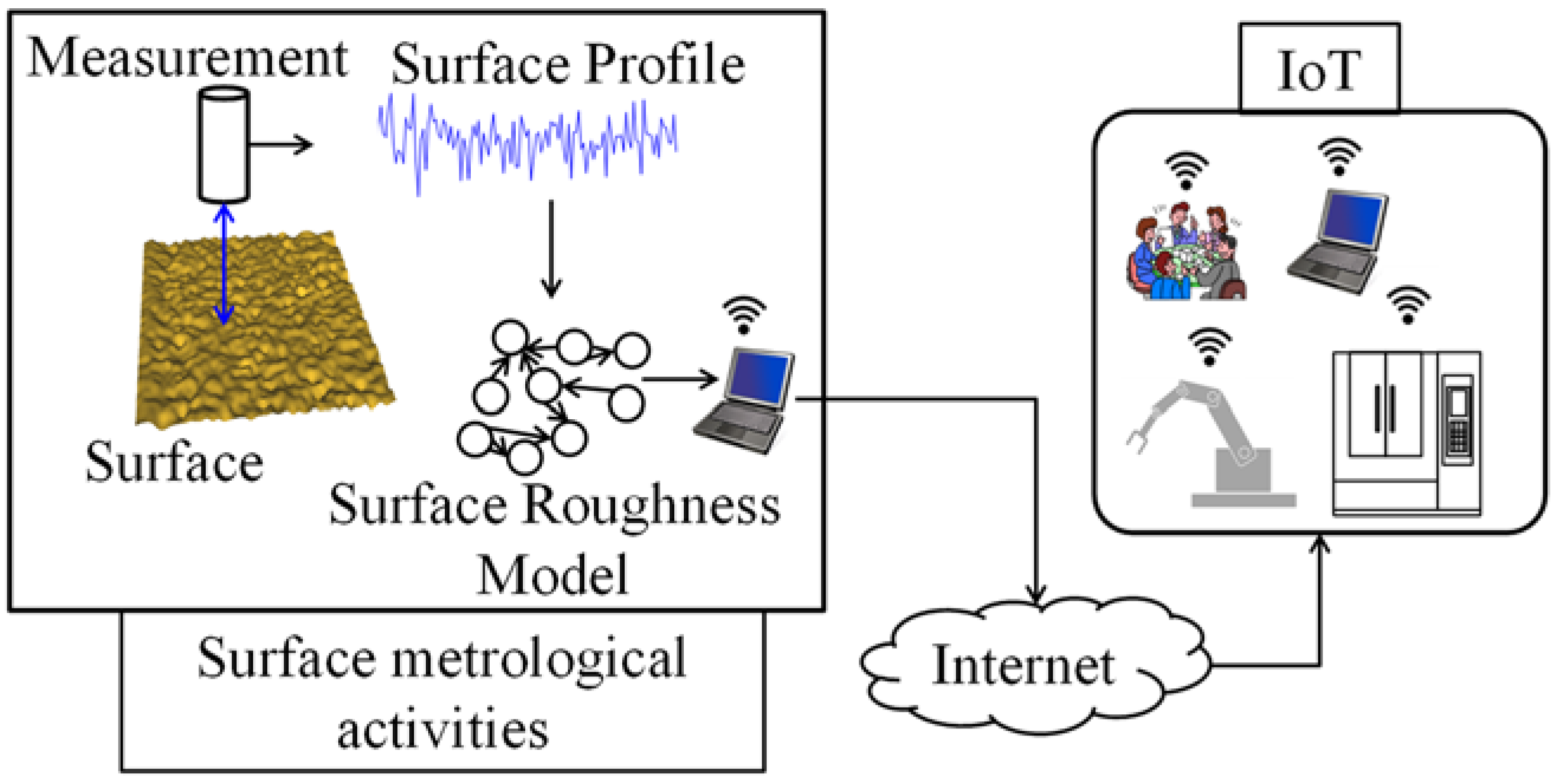
4. Modeling Surface Roughness
5. Discussion and Concluding Remarks
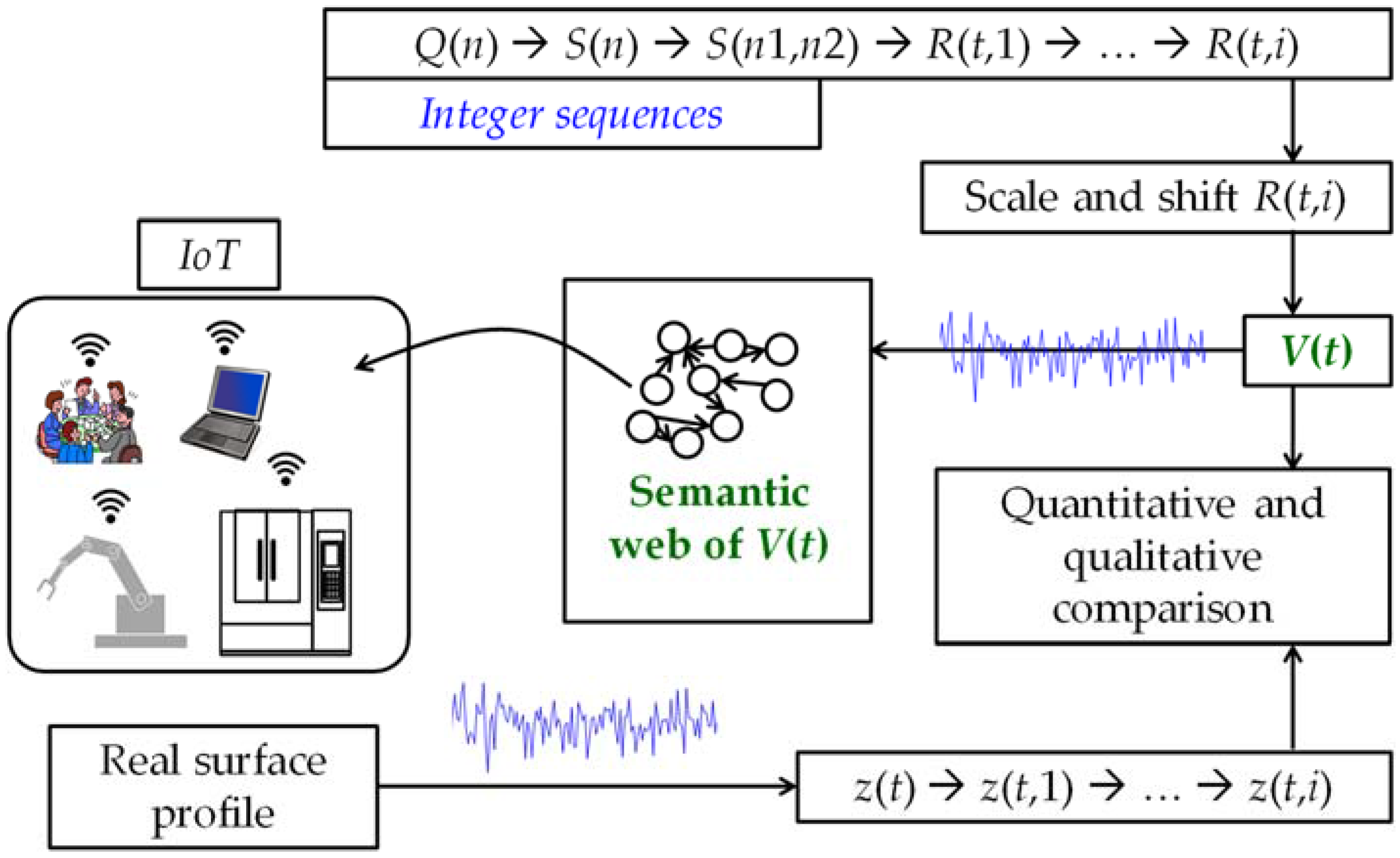
- Step 1
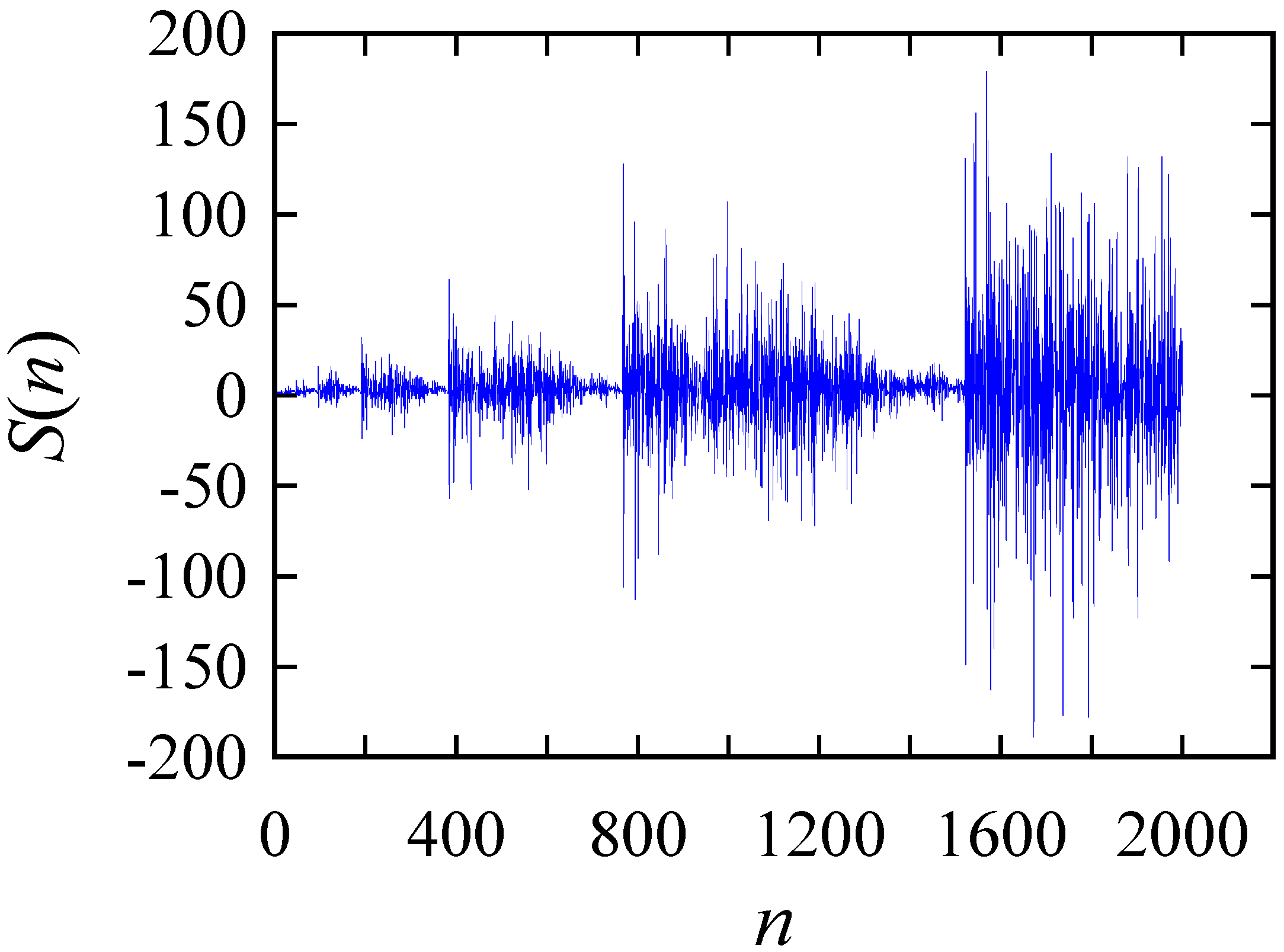
- Generate Q(n) (Equation (1)).
- Step 2
- Generate S(n) (Equation (2)).
- Step 3
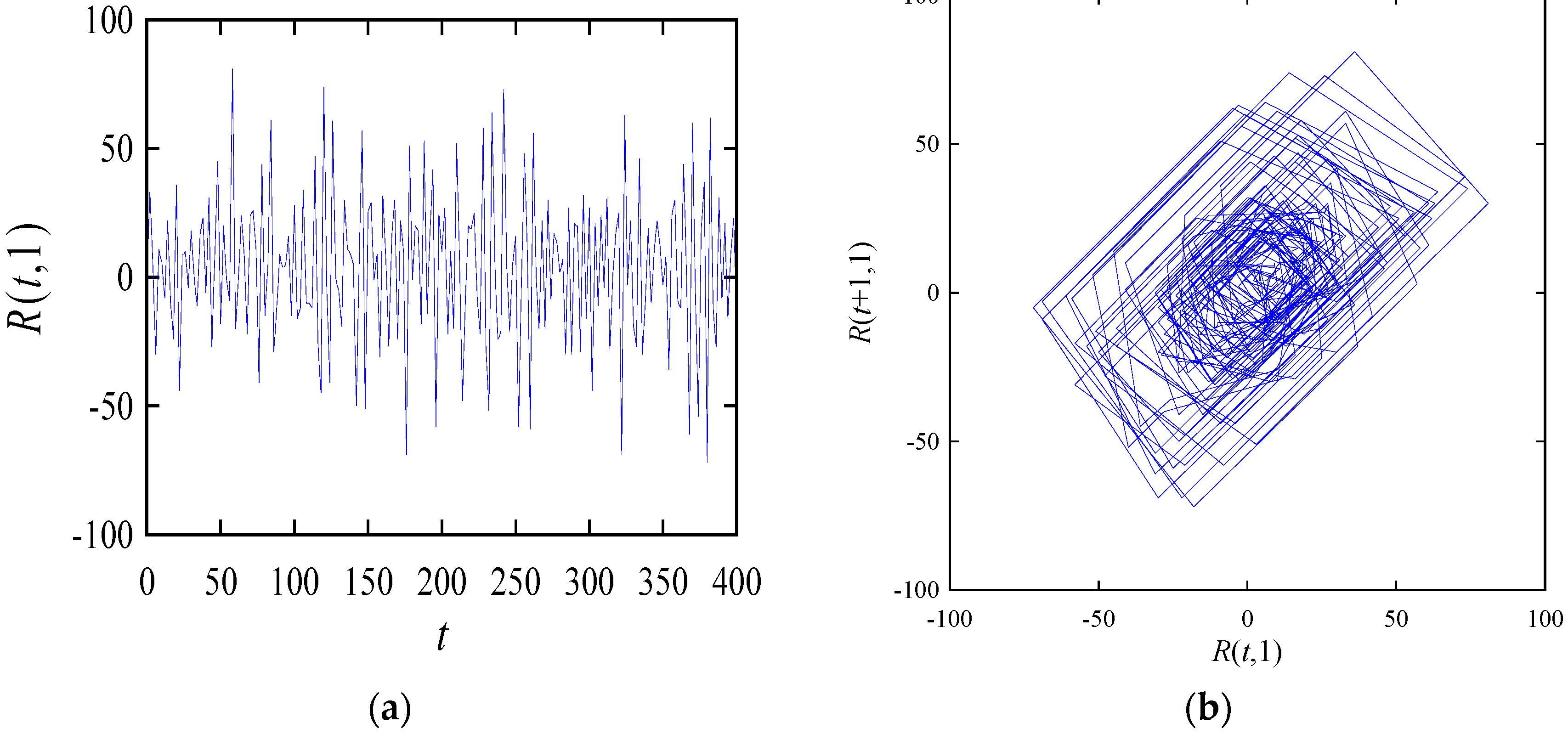
- Select a segment of S(n), S(n1,n2) (Equation (3)).
- Step 4
- Expand S(n1,n2) by a linear interpolation operation and generate R(t,1) (Equation (4)).
- Step 5
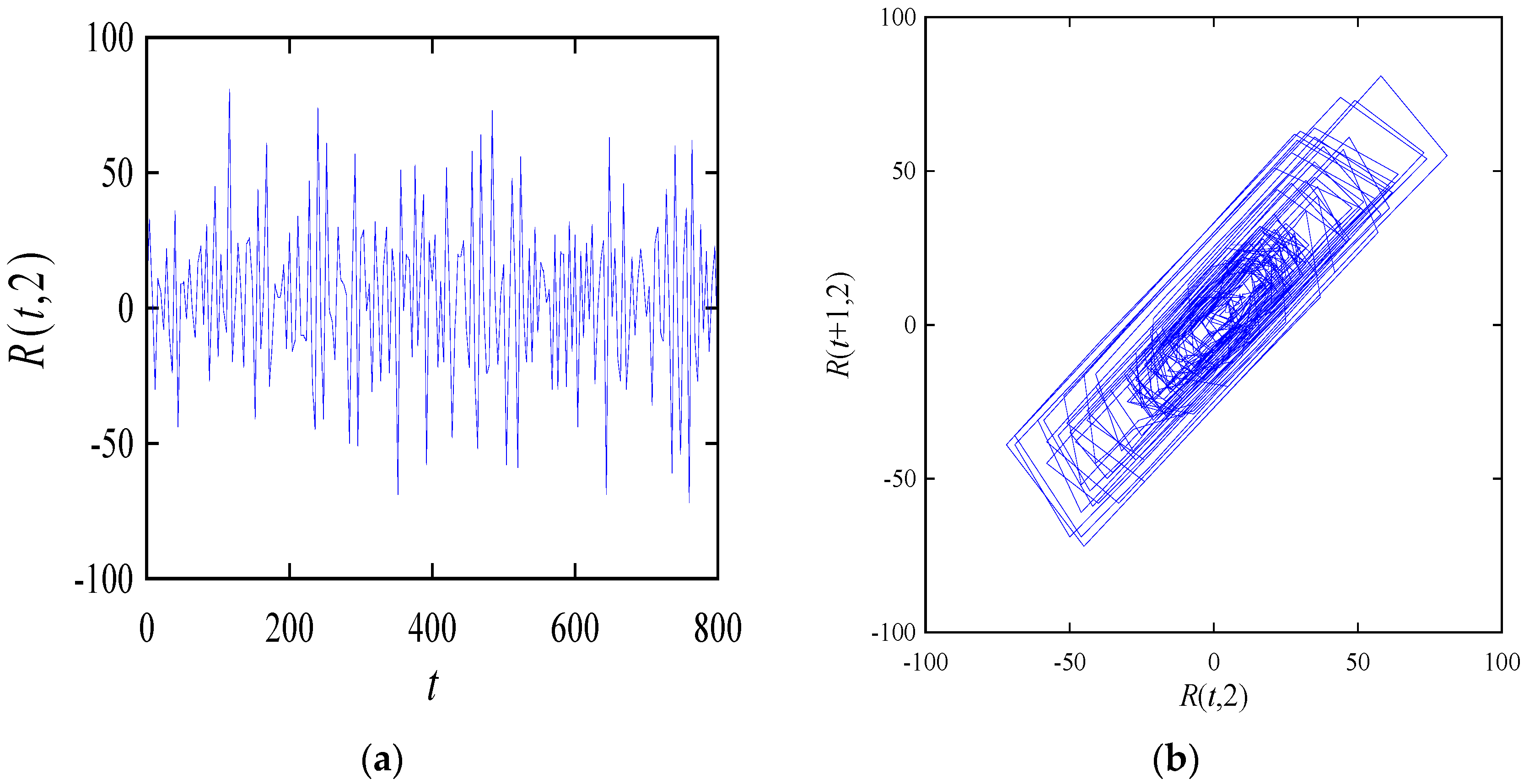
- Expand R(t,1) by successive linear interpolation and generate R(t,i), i = 1, 2, … (Equation (5)).
- Step 6
- Select one of the R(t,i), ∃i ∈{1, 2, …}.
- Step 7
- Scale and shift R(t,i) selected in Step 6 and generate V(t) (Equation (6)).
- Step 8
- Compare V(t) with the given surface profile z(t) or with one of its (z(t)’s) linear interpolated profiles, z(t,j), j = 1, 2, etc.
- Step 9
- If the comparison is satisfactory in terms of qualitative and quantitative measures, then accept V(t) as a model of the roughness profile. Create semantic web to use V(t) in the framework of IoT. Otherwise, go back to Step 3 and continue.
Acknowledgments
Conflicts of Interest
References
- ISO TC 213. Available online: http://www.iso.org/iso/home/store/catalogue_tc/catalogue_tc_browse.htm?commid=54924&published=on (accessed on 15 January 2017).
- Jusko, O.; Neugebauer, M.; Reimann, H.; Bernhardt, R. Recent progress in CMM-based form measurement. Int. J. Autom. Technol. 2015, 9, 170–175. [Google Scholar]
- Woźniak, A.; Krajewski, G. CMM dynamic properties of the scanning measurement of a 2D profile. Int. J. Autom. Technol. 2015, 9, 530–533. [Google Scholar]
- Ito, S.; Jia, Z.; Goto, S.; Hosobuchi, K.; Shimizu, Y.; He, G.; Gao, W. An electrostatic force probe for surface profile measurement in noncontact condition. Int. J. Autom. Technol. 2013, 7, 714–719. [Google Scholar]
- Elrawemi, M.; Blunt, L.; Muhamedsalih, H.; Gao, F.; Fleming, L. Implementation of in Process Surface Metrology for R2R flexible PV barrier films. Int. J. Autom. Technol. 2015, 9, 312–321. [Google Scholar]
- Whitehouse, D. A new look at surface metrology. Wear 2009, 266, 560–565. [Google Scholar] [CrossRef]
- Wang, X.; Shi, T.; Liao, G.; Zhang, Y.; Hong, Y.; Chen, K. Using Wavelet Packet Transform for Surface Roughness Evaluation and Texture Extraction. Sensors 2017, 17, 933. [Google Scholar] [CrossRef] [PubMed]
- Sharif Ullah, A.M.M.; Fuji, A.; Kubo, A.; Tamaki, J.; Kimura, M. On the surface metrology of bimetallic components. Mach. Sci. Technol. 2015, 19, 339–359. [Google Scholar] [CrossRef]
- Bui, S.H.; Vorburger, T.V. Surface metrology algorithm testing system. Precis. Eng. 2007, 31, 218–225. [Google Scholar] [CrossRef]
- Li, T.; Blunt, L.A.; Jiang, X.; Zeng, W. An Information Model for Surface Metrology. Procedia CIRP 2013, 10, 251–258. [Google Scholar] [CrossRef]
- Sharif Ullah, A.M.M.; Tamaki, J.; Kubo, A. Modeling and Simulation of 3D Surface Finish of Grinding. Adv. Mater. Res. 2010, 126–128, 672–677. [Google Scholar] [CrossRef]
- Sharif Ullah, A.M.M.; Harib, K.H. Simulation of Cutting Force using Nonstationary Gaussian Process. J. Intell. Manuf. 2010, 21, 681–691. [Google Scholar] [CrossRef]
- Pawlus, P. Simulation of Stratified Surface Topographies. Wear 2008, 264, 457–463. [Google Scholar] [CrossRef]
- Sharif Ullah, A.M.M.; Arai, N.; Watanabe, M. Concept Map and Internet-aided Manufacturing. Procedia CIRP 2013, 12, 378–383. [Google Scholar] [CrossRef]
- Weyer, S.; Schmitt, M.; Ohmer, M.; Gorecky, D. Towards Industry 4.0—Standardization as the crucial challenge for highly modular, multi-vendor production systems. IFAC-PapersOnLine 2015, 48, 579–584. [Google Scholar] [CrossRef]
- Monostori, L. Cyber-physical Production Systems: Roots, Expectations and R&D challenges. Procedia CIRP 2014, 17, 9–13. [Google Scholar]
- Ramos, L. Semantic Web for manufacturing, trends and open issues: Toward a state of the art. Comput. Ind. Eng. 2015, 90, 444–460. [Google Scholar] [CrossRef]
- Lee, J.; Bagheri, B.; Kao, H.-A. A Cyber-Physical Systems architecture for Industry 4.0-based manufacturing systems. Manuf. Lett. 2015, 3, 18–23. [Google Scholar] [CrossRef]
- Wu, J.-J. Simulation of rough surfaces with FFT. Tribol. Int. 2000, 33, 47–58. [Google Scholar] [CrossRef]
- Higuchi, M.; Yamaguchi, T.; Yano, A.; Yamamoto, N.; Ueshima, R.; Matumori, N.; Yoshizawa, I. Development of Design Technology of Porous Superfinishing Stone Using Fractal Geometry (2nd Report)—Geometric Modeling of Stone Topography and Design Support System. J. Jpn. Soc. Precis. Eng. 2001, 67, 428–432. (In Japanese) [Google Scholar] [CrossRef]
- Ullah, A.M.M.S.; Harib, K.H. Knowledge extraction from time series and its application to surface roughness simulation. Inf. Knowl. Syst. Manag. 2006, 5, 117–134. [Google Scholar]
- Uchidate, M.; Yanagi, K.; Yoshida, I.; Shimizu, T.; Iwabuchi, A. Generation of 3D random topography datasets with periodic boundaries for surface metrology algorithms and measurement standards. Wear 2011, 271, 565–570. [Google Scholar] [CrossRef]
- Sharif Ullah, A.M.M.; Chowdhury, M.A.K.; Kubo, A. A Surface Generation Mechanism of Grinding. Appl. Mech. Mater. 2017, 860, 13–18. [Google Scholar] [CrossRef]
- Hofstadter, D.R. Gödel, Escher, Bach: An Eternal Golden Braid; Vintage Books: New York, NY, USA, 1980; pp. 137–138. [Google Scholar]
- Pinn, K. Order and chaos in Hofstadter’s Q(n) sequence. Complexity 1999, 4, 41–46. [Google Scholar] [CrossRef]
- Kantz, H.; Schreiber, T. Nonlinear Time Series Analysis; Cambridge University Press: Cambridge, UK, 2002. [Google Scholar]
- NIST Surface Roughness Database. Available online: http://physics.nist.gov/VSC/jsp/Database.jsp (accessed on 12 January 2017).
- Sharif Ullah, A.M.M.; Shamsuzzaman, M. Fuzzy Monte Carlo Simulation using point-cloud-based probability-possibility transformation. Simulation 2013, 89, 860–875. [Google Scholar] [CrossRef]
- Ullah, A.M.M.S.; Shahinur, S.; Haniu, H. On the Mechanical Properties and Uncertainties of Jute Yarns. Materials 2017, 10, 450. [Google Scholar] [CrossRef]
- Shahinur, S.; Ullah, A.M.M.S. Quantifying the Uncertainty Associated with the Material Properties of a Natural Fiber. Procedia CIRP 2017, 61, 541–546. [Google Scholar] [CrossRef]


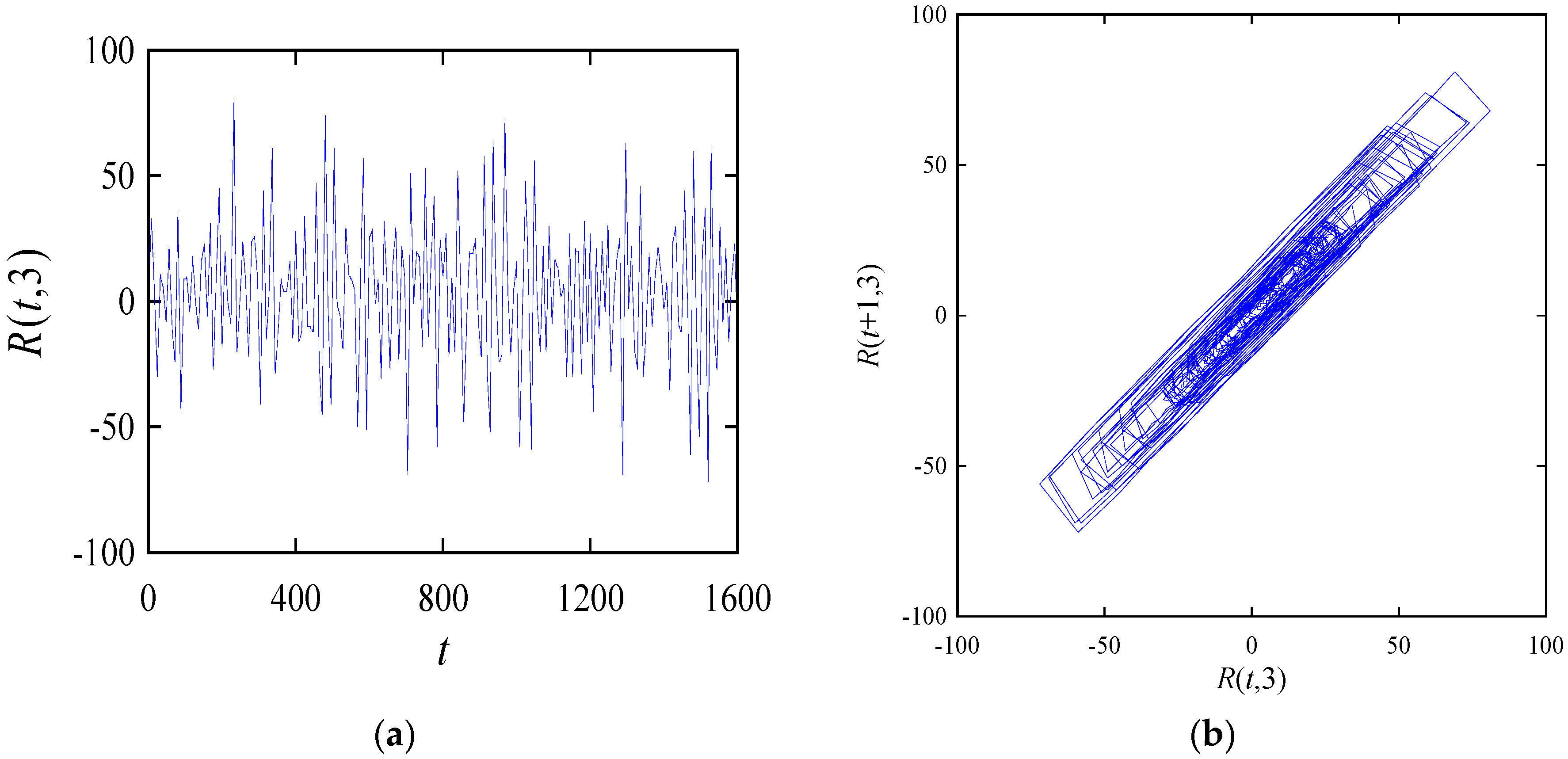


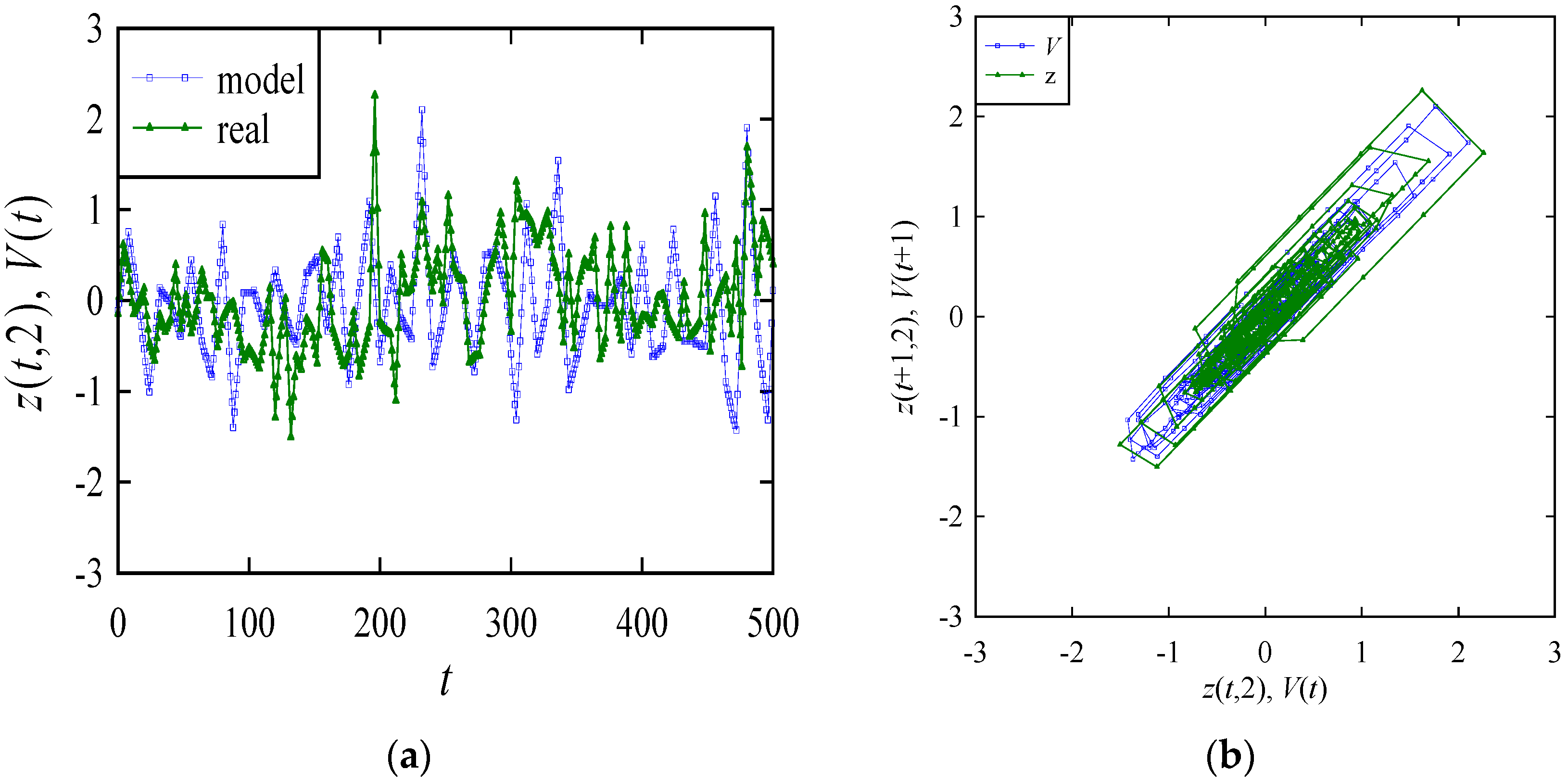
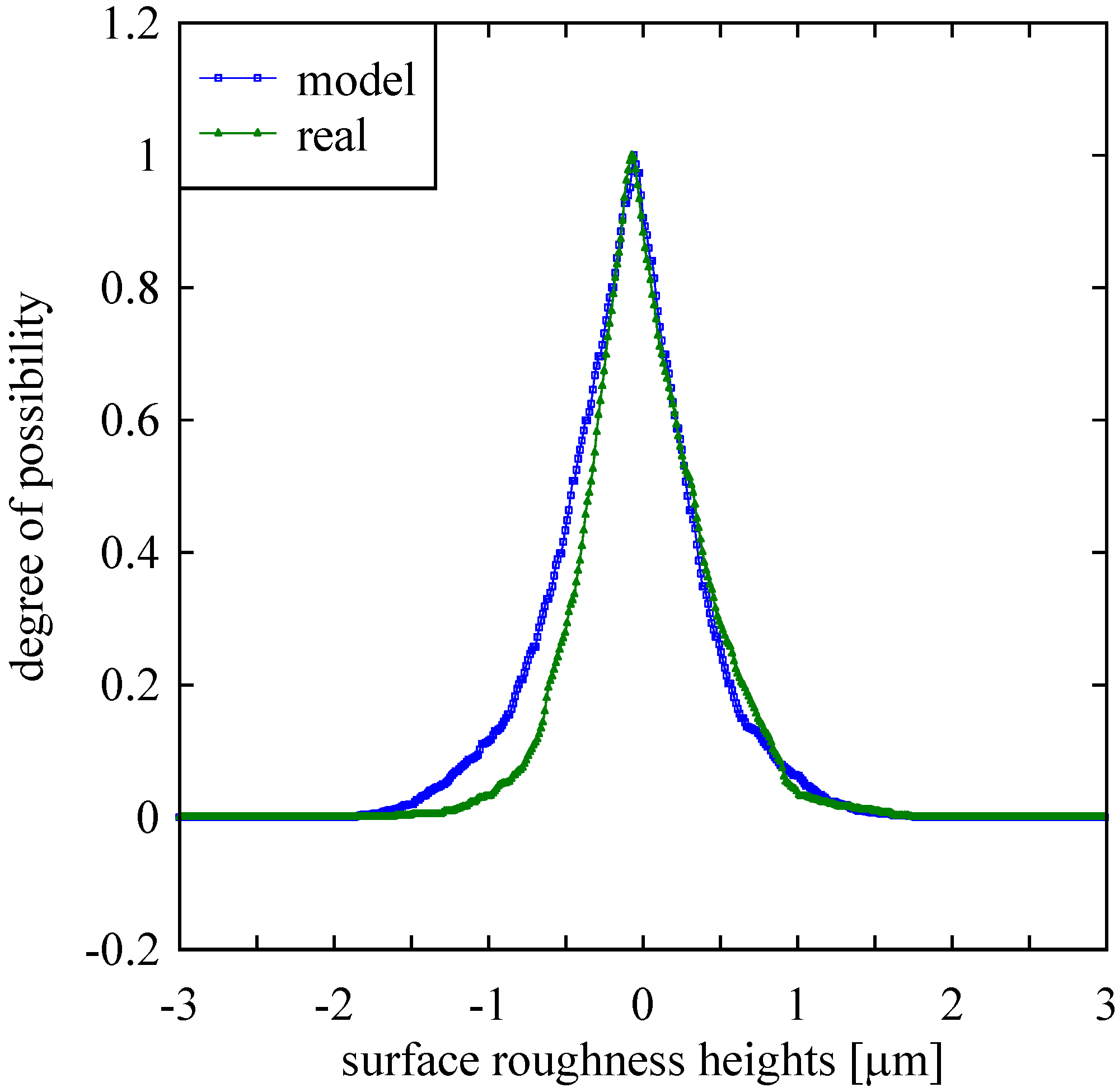
| Parameters | Model (V(t)) | Real (z(t,2)) |
|---|---|---|
| Ra (μm) | 0.471 | 0.419 |
| Rz (μm) | 4.281 | 4.281 |
| Entropy (Bits) | 3.209 | 4.063 |
© 2017 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ullah, A.M.M.S. Surface Roughness Modeling Using Q-Sequence. Math. Comput. Appl. 2017, 22, 33. https://doi.org/10.3390/mca22020033
Ullah AMMS. Surface Roughness Modeling Using Q-Sequence. Mathematical and Computational Applications. 2017; 22(2):33. https://doi.org/10.3390/mca22020033
Chicago/Turabian StyleUllah, A.M.M. Sharif. 2017. "Surface Roughness Modeling Using Q-Sequence" Mathematical and Computational Applications 22, no. 2: 33. https://doi.org/10.3390/mca22020033
APA StyleUllah, A. M. M. S. (2017). Surface Roughness Modeling Using Q-Sequence. Mathematical and Computational Applications, 22(2), 33. https://doi.org/10.3390/mca22020033






