Exponentially Fitted Finite Difference Schemes for Reaction-Diffusion Equations
Abstract
:1. Introduction
2. Exponentially Fitted Finite Difference Formulation
2.1. Pure Diffusion
Stability of the Method
2.2. Reaction-Diffusion
3. Numerical Examples
3.1. Diffusion Equation
3.2. Nonlinear Diffusion
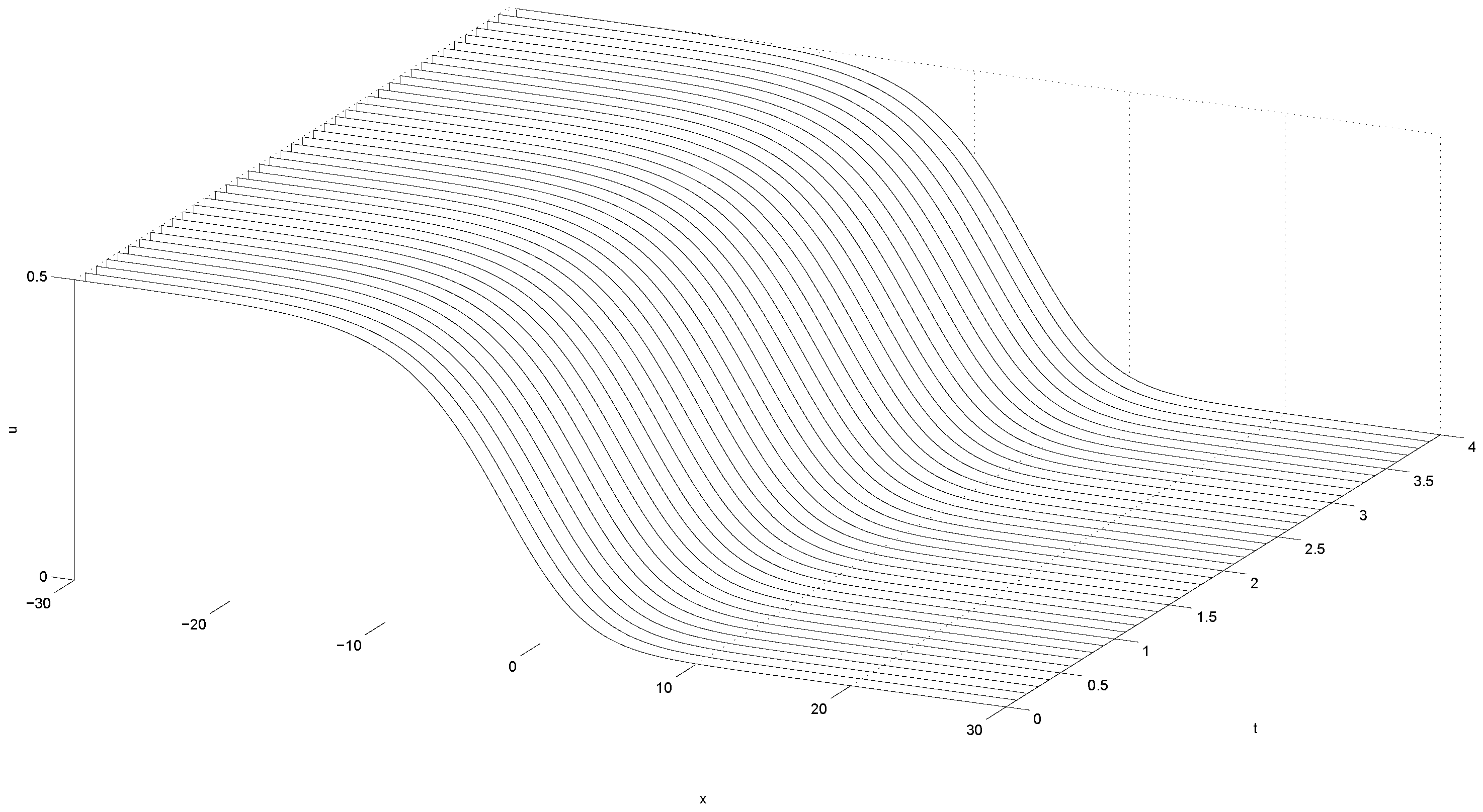
3.3. Fisher Equation
3.4. Coupled Nonlinear System
3.5. Stiff Coupled System
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Abbreviations
| MDPI | Multidisciplinary Digital Publishing Institute |
| DOAJ | Multidisciplinary Digital Publishing Institute |
| TLA | Three letter acronym |
| LD | linear dichroism |
References
- Hundsdorfer, W.; Verwer, J. Numerical Solution of Time-Dependent Advection-Diffusion-Reaction Equations; Springer Series in Computational Mathematics; Springer-Verlag: Berlin/Heidelberg, Germany, 2003. [Google Scholar]
- Alvarez-Ramirez, J.; Valdes-Parada, F.J.; Alvarez, J.; Ochoa-Tapia, J.A. A Green’s function formulation for finite-differences schemes. Chem. Eng. Sci. 2007, 62, 3083–3091. [Google Scholar] [CrossRef]
- Mickens, R.E. Nonstandard Finite Difference Schemes for Differential Equations. J. Differ. Equ. Appl. 2002, 8, 823–847. [Google Scholar]
- Gautschi, W. Numerical integration of ordinary differential equations based on trigonometric polynomials. Numer. Math. 1961, 3, 381–397. [Google Scholar] [CrossRef]
- Paternoster, B. Present state-of-the-art in exponential fitting. A contribution dedicated to Liviu Ixaru on his 70th birthday. Comput. Phys. Commun. 2012, 183, 2499–2512. [Google Scholar] [CrossRef]
- Anastassi, Z.; Simos, T. A family of two-stage two-step methods for the numerical integration of the Schrödinger equation and related IVPs with oscillating solution. J. Math. Chem. 2009, 45, 1102–1129. [Google Scholar] [CrossRef]
- Erdogan, U.; Ozis, T. A smart nonstandard finite difference scheme for second order nonlinear boundary value problems. J. Comput. Phys. 2011, 230, 6464–6474. [Google Scholar] [CrossRef]
- Gurski, K. A simple construction of nonstandard finite-difference schemes for small nonlinear systems applied to SIR models. Comput. Math. Appl. 2013, 66, 2165–2177. [Google Scholar] [CrossRef]
- Roos, H.G.; Stynes, M.; Tobiska, L. Robust Numerical Methods for Singularly Perturbed Differential Equations: Convection-Diffusion-Reaction and Flow Problems; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2008; Volume 24. [Google Scholar]
- Öziş, T.; Erdoğan, U. An exponentially fitted method for solving Burgers’ equation. Int. J. Numer. Methods Eng. 2009, 79, 696–705. [Google Scholar] [CrossRef]
- Marušić, M. On ϵ uniform convergence of exponentially fitted methods. Math. Commun. 2014, 19, 545–559. [Google Scholar]
- Erdogan, U.; Kocak, H. Numerical study of the asymptotics of the second Painlevé equation by a functional fitting method. Math. Methods Appl. Sci. 2013, 36, 2347–2352. [Google Scholar] [CrossRef]
- Ramos, H.; Vigo-Aguiar, J. On the frequency choice in trigonometrically fitted methods. Appl. Math. Lett. 2010, 23, 1378–1381. [Google Scholar] [CrossRef]
- Erdogan, U. Improved upwind discretization of the advection equation. Numer. Methods Partial Differ. Equ. 2014, 30, 773–787. [Google Scholar] [CrossRef]
- Ixaru, L.; Berghe, G. Exponential fitting. In Mathematics and its Applications; Kluwer Academic Publishers: Amsterdam, The Netherlands, 2004. [Google Scholar]
- Ascher, U.M. Numerical Methods for Evolutionary Differential Equations; Society for Industrial and Applied Mathematics: Philadelphia, PA, USA, 2008. [Google Scholar]
- Uddin, R. Comparison of the nodal integral method and nonstandard finite-difference schemes for the fisher equation. SIAM J. Sci. Comput. 2000, 22, 1926–1942. [Google Scholar] [CrossRef]
- Ehrhardt, M.; Mickens, R.E. A nonstandard finite difference scheme for convection–diffusion equations having constant coefficients. Appl. Math. Comput. 2013, 219, 6591–6604. [Google Scholar]
- Wazwaz, A.M. Exact solutions to nonlinear diffusion equations obtained by the decomposition method. Appl. Math. Comput. 2001, 123, 109–122. [Google Scholar] [CrossRef]
- Cattani, C.; Kudreyko, A. Mutiscale Analysis of the Fisher Equation. Comput. Sci. Appl. ICCSA 2008 2008, 5072, 1171–1180. [Google Scholar]
- Mittal, R.C.; Arora, G. Efficient numerical solution of Fisher’s equation by using B-spline method. Int. J. Comput. Math. 2010, 87, 3039–3051. [Google Scholar] [CrossRef]
- Feng, X. Exact wave front solutions to two generalized coupled nonlinear physical equations. Phys. Lett. A 1996, 213, 167–176. [Google Scholar] [CrossRef]
- El-Wakil, S.; Abdou, M. New applications of Adomian decomposition method. Chaos Solitons Fractals 2007, 33, 513–522. [Google Scholar] [CrossRef]
- Abbas, M.; Majid, A.A.; Ismail, A.I.M.; Rashid, A. Numerical method using cubic B-spline for a strongly coupled reaction-diffusion system. PLoS ONE 2014, 9. [Google Scholar] [CrossRef] [PubMed]
- Cao, H.Y.; Sun, Z.Z. A second-order linearized difference scheme for a strongly coupled reaction-diffusion system. Numer. Methods Partial Differ. Equ. 2008, 24, 9–23. [Google Scholar] [CrossRef]
- Boyd, J. Chebyshev and Fourier Spectral Methods; Dover books on mathematics; DOVER PUBN Incorporated: New York, NY, USA, 2001. [Google Scholar]
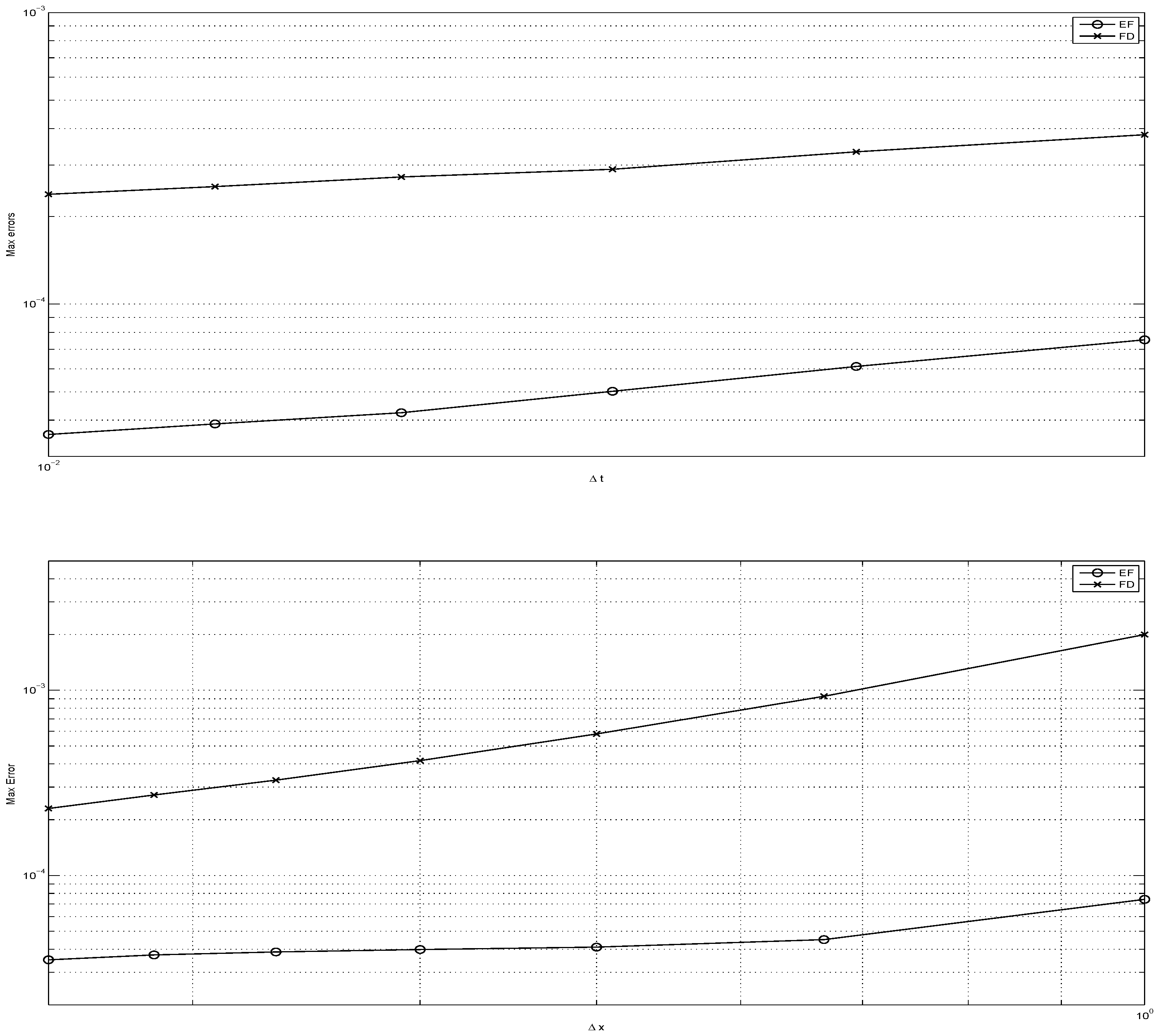
| Space Step Size | |||||
|---|---|---|---|---|---|
| Time Step Size | |||||
| 9.43 × | 9.43 × | 2.22 × | 4.44 × | 3.88 × | |
| EF | 1.43 × | 8.99 × | 1.09 × | 3.59 × | 3.17 × |
| Classical FD | 2.52 × | 1.59 × | 1.95 × | 1.12 × | 1.55 × |
| Space Step Size | ||||
|---|---|---|---|---|
| Time Step Size | ||||
| EF | 2.8 × | 2.8 × | 7.0 × | 7.0 × |
| Classical explicit FD | 4.5 × | 7.08 × | 1.1 × | 5.0 × |
| x | Wavelet | B-Spline | Present | Exact | Errors | Errors of |
|---|---|---|---|---|---|---|
| Method | Solution | of B-Spline | Present Method | |||
| −20 | 0.498678 | 0.499412 | 0.49941337 | 0.499413331 | 1.35 × | 3.48 × |
| −16 | 0.498525 | 0.498146 | 0.49814210 | 0.498142011 | 4.01 × | 9.32 × |
| −12 | 0.494757 | 0.494149 | 0.49414023 | 0.494140001 | 8.86 × | 2.31 × |
| −8 | 0.481776 | 0.481763 | 0.48175680 | 0.481755704 | 7.28 × | 1.09 × |
| −4 | 0.445508 | 0.445372 | 0.44540611 | 0.445397724 | 2.53 × | 8.38 × |
| 2 | 0.279025 | 0.280082 | 0.27998956 | 0.279941256 | 1.41 × | 4.83 × |
| 6 | 0.11698 | 0.117196 | 0.11699390 | 0.116963394 | 2.33 × | 3.05 × |
| 10 | 0.025927 | 0.025881 | 0.02598319 | 0.025974116 | 9.30 × | 9.07 × |
| 14 | 0.003695 | 0.003559 | 0.00362421 | 0.003622347 | 6.29 × | 1.86 × |
| 18 | 0.000409 | 0.000395 | 0.00040592 | 0.000405692 | 1.12 × | 2.24 × |
| Space Step Size | |||
|---|---|---|---|
| Implicit EF | 7.39 × | 6.37 × | 4.35 × |
| Implicit FD | 1.79 × | 3.62 × | 2.48 × |
| Implicit B-spline [24] | 5.06 × | 5.61 × | 2.15 × |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Erdoğan, U.; Akarbulut, K.; Tan, N.Ö. Exponentially Fitted Finite Difference Schemes for Reaction-Diffusion Equations. Math. Comput. Appl. 2016, 21, 32. https://doi.org/10.3390/mca21030032
Erdoğan U, Akarbulut K, Tan NÖ. Exponentially Fitted Finite Difference Schemes for Reaction-Diffusion Equations. Mathematical and Computational Applications. 2016; 21(3):32. https://doi.org/10.3390/mca21030032
Chicago/Turabian StyleErdoğan, Utku, Kenan Akarbulut, and Neşet Özkan Tan. 2016. "Exponentially Fitted Finite Difference Schemes for Reaction-Diffusion Equations" Mathematical and Computational Applications 21, no. 3: 32. https://doi.org/10.3390/mca21030032
APA StyleErdoğan, U., Akarbulut, K., & Tan, N. Ö. (2016). Exponentially Fitted Finite Difference Schemes for Reaction-Diffusion Equations. Mathematical and Computational Applications, 21(3), 32. https://doi.org/10.3390/mca21030032





