Design Criteria for Generating Physiologically Relevant In Vitro Models in Bioreactors
Abstract
:1. Introduction
1.1. In Vivo and In Vitro

1.2. Classifying the Microenvironment
| Method | Description | Advantages | Disadvantages |
|---|---|---|---|
| Monolayer monocultures | Only one cell type plated in flat dishes | Cheap, standard | Loss of phenotype, absence of 3D architecture changes cell shape |
| Tissue slice culture | Tissue slices are cultured in plates or on supports | In vivo environment is better preserved | Require hyperoxic conditions, viability limited in time |
| Co-cultures | Monolayer cultures containing more than one cell type | Cell function is improved | Difficult to analyse the contribution of each cell type to co-culture function |
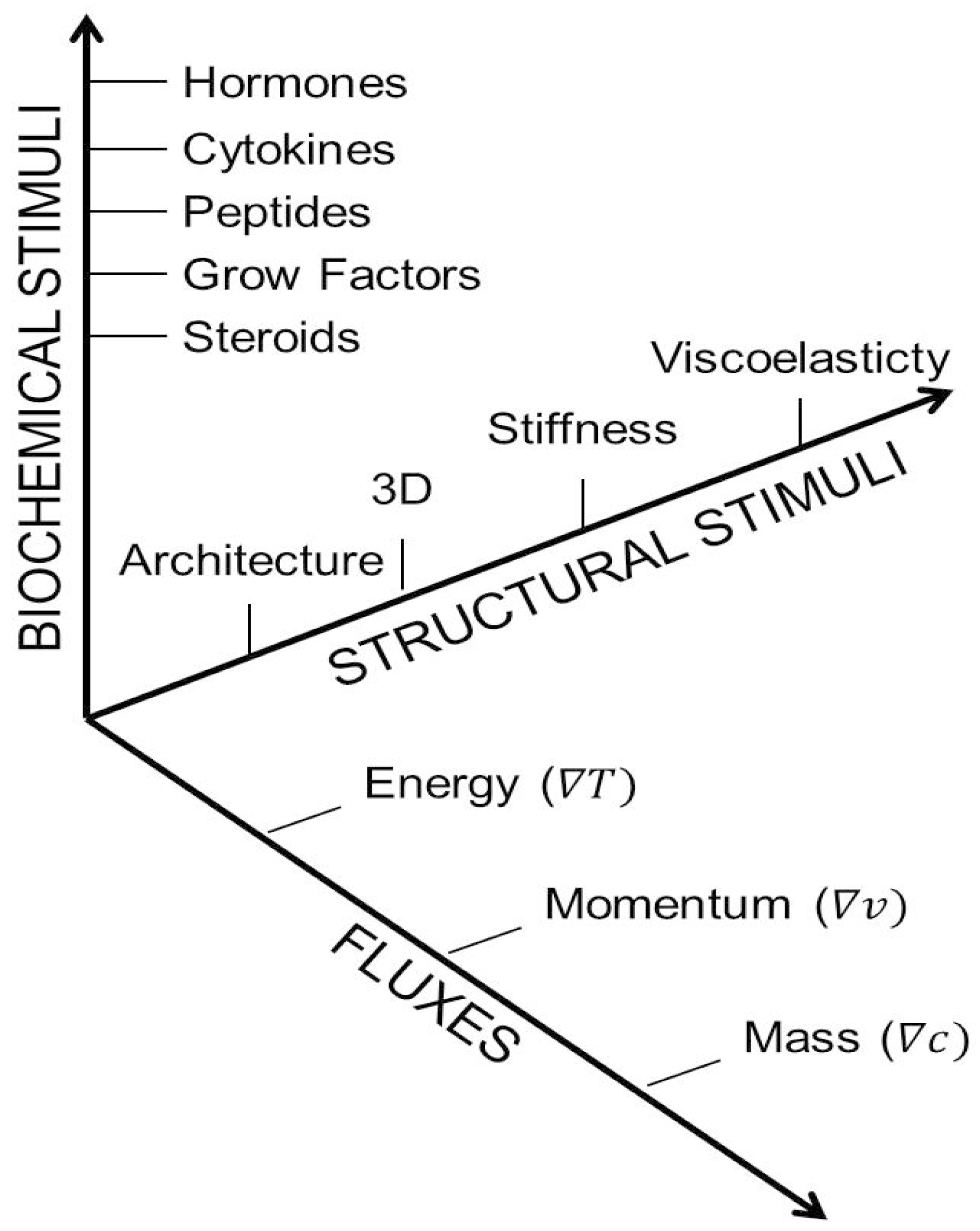
2. Mimicking Physiological Systems: The Rule of Ten
3. Scaling: Nano, Micro and Milli
| Micro-Fluidics | Milli-Fluidics |
|---|---|
| Low shear by reduction of flow rate | Low shear, high flow rates |
| Low nutrient turnover | High nutrient turnover |
| High surface to volume ratio | Low surface to volume ratio |
| Fiddly to assemble | Easy to assemble |
| Presence of air bubbles | No air bubbles |
| Low fluid volumes, saving on reagents | Higher volumes of media and reagents |
| Easy quantification of cell products | Cell products may be harder to quantify |
4. Modelling 3D In Vitro Cell Cultures: Hepatocyte-Laden Hydrogels as a Reference
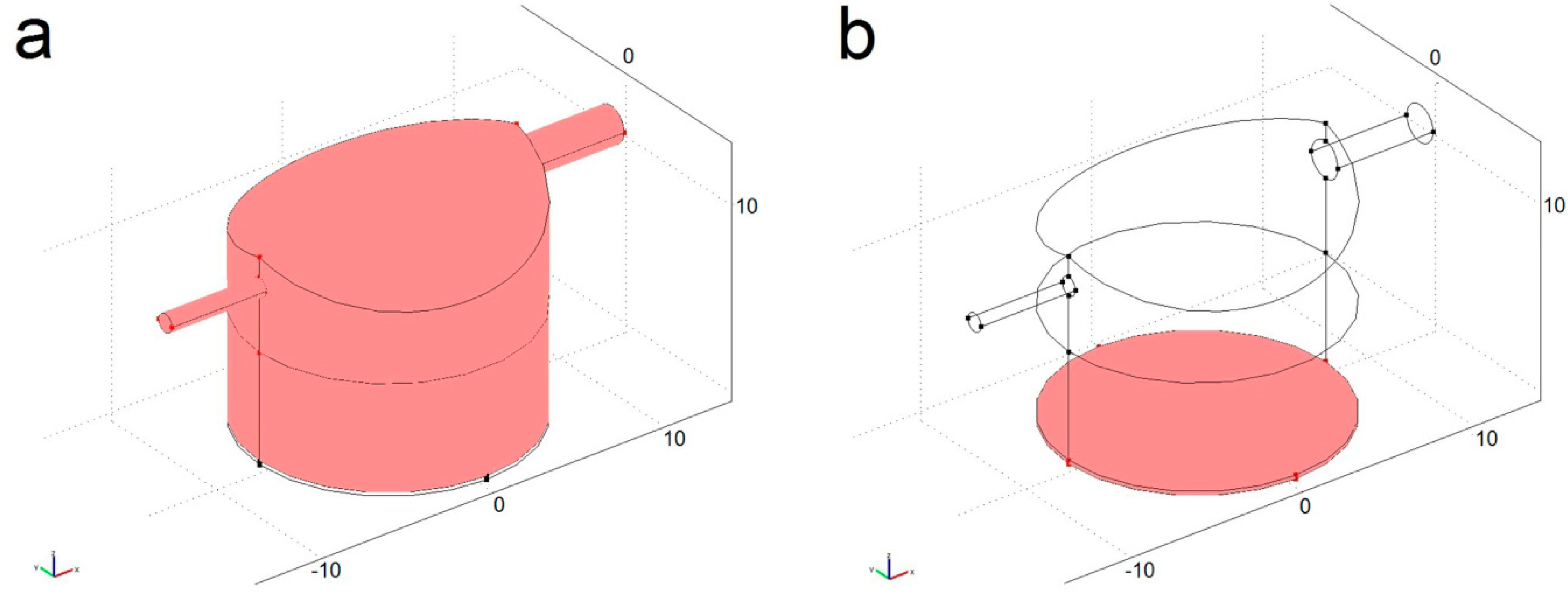
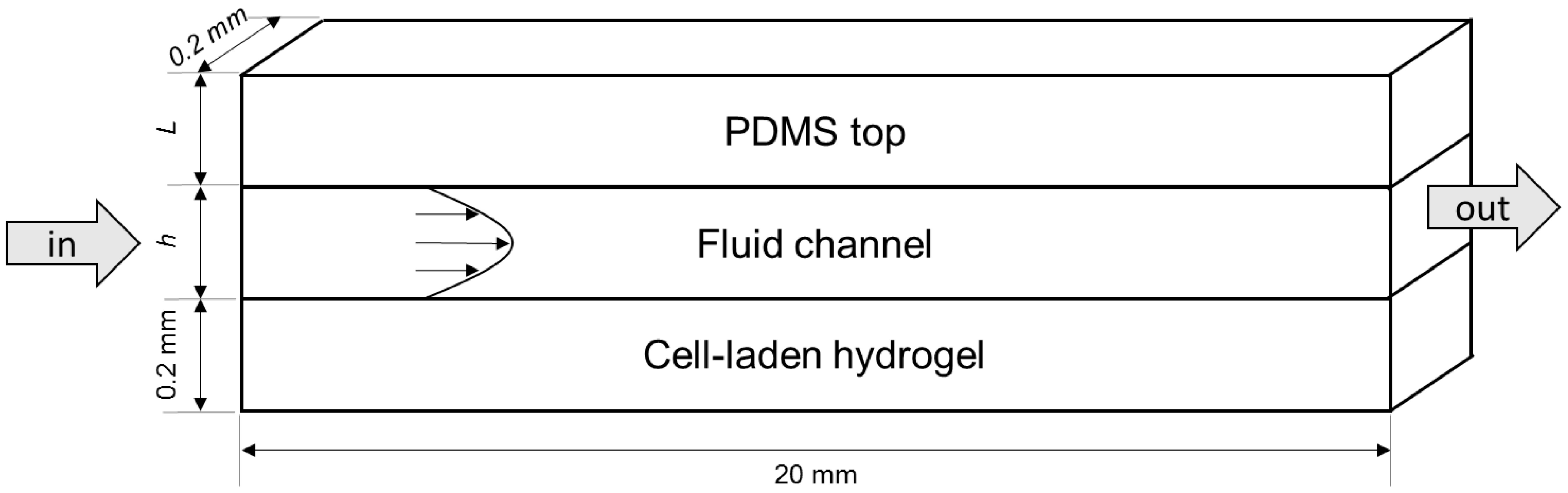
4.1. Modelled Configurations
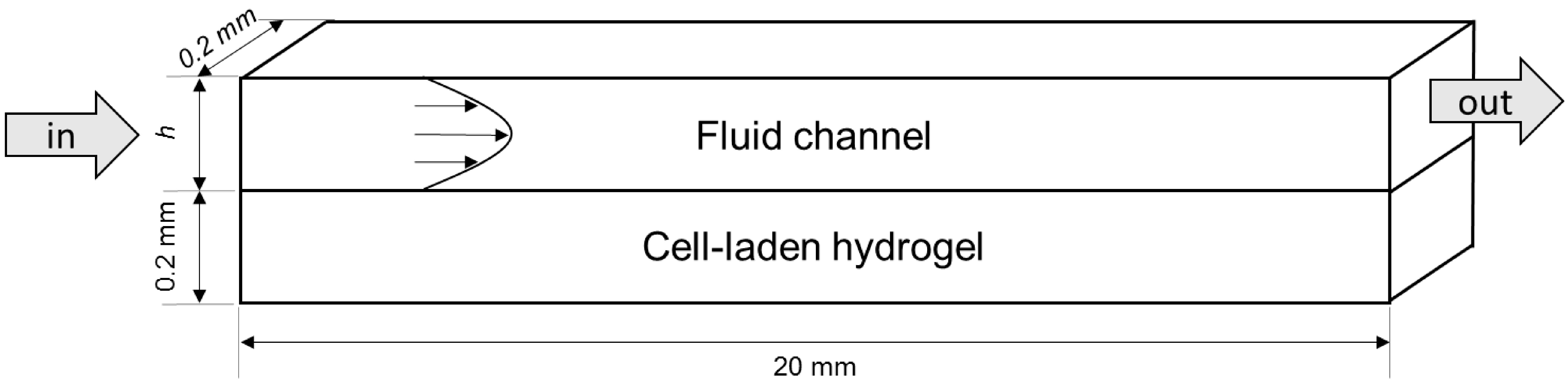
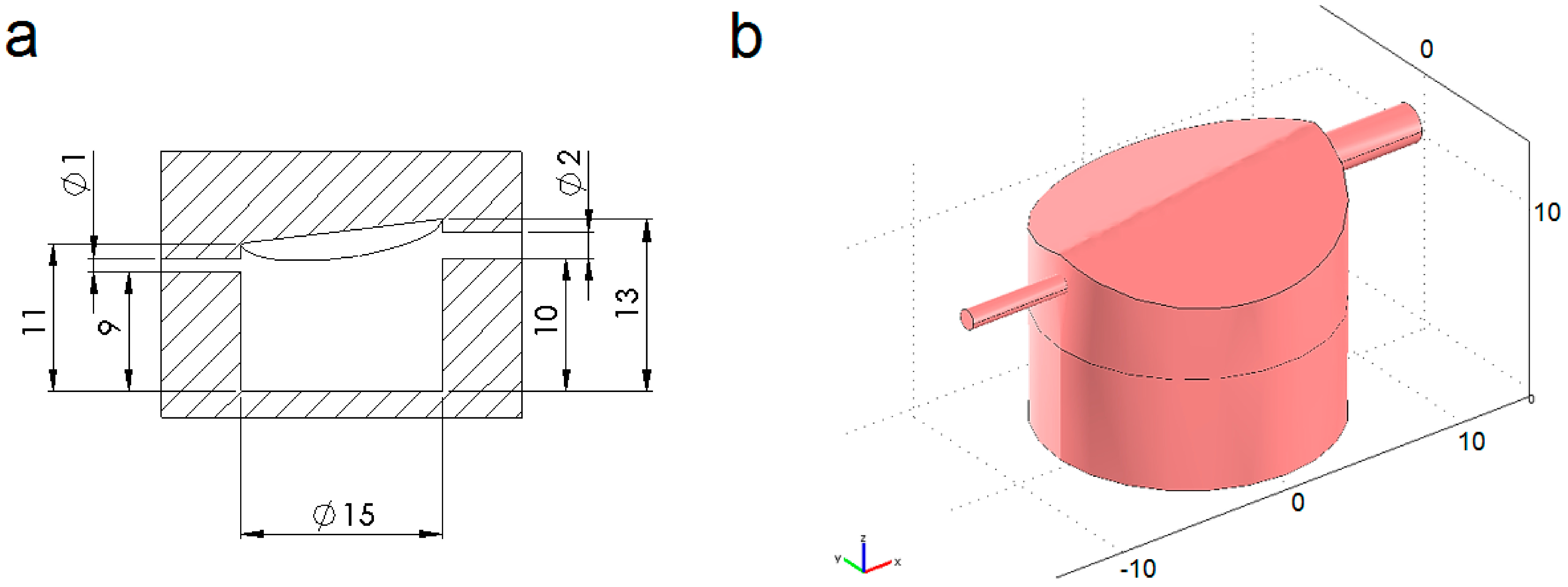
4.2. Computational Mass Transport and Flow Model
4.2.1. Oxygen Transport and Consumption
| Parameter | Symbol | Value | Units | References |
|---|---|---|---|---|
| Henry’s constant for oxygen | 1.32 × 10−3 | mol∙m−3∙mmHg−1 | [50] | |
| Oxygen partial pressure in atmosphere | 159 | mmHg | [51] | |
| Oxygen concentration in culture medium entering the system | 0.21 | mol/m3 | [31,32,52,53] | |
| Oxygen diffusion in aqueous media | 3 × 10−9 | m2/s | [31,52,54] | |
| Oxygen diffusion in the hydrogel construct | 1 × 10−9 | m2/s | [52,54,55,56,57,58] | |
| Hepatocyte maximum oxygen consumption rate | Ω | 4.8 × 10−17 | mol∙cell−1∙s−1 | [49] |
| Michaelis-Menten constant for oxygen consumption | Km | 7.39 × 10−3 | mol/m3 | [45,59,60] |
| Critical oxygen concentration to account for cell necrosis | ccr | 2.64 × 10−3 | mol/m3 | [61] |
4.2.2. Fluid Dynamics
4.2.3. Model Implementation
| Model | Surface | Boundary Condition |
|---|---|---|
| Oxygen convection and diffusion | System side walls | Insulation/symmetry (n · (−D∇c + cu) = 0) |
| Interface between the hydrogel construct and the fluid sub-domain | Continuity | |
| Fluid domain inlet | Constant oxygen concentration (c = 0.21 mol/m3) | |
| Fluid domain outlet | Convective flux (n · (−D∇c) = 0) | |
| Navier-Stokes | Solid-liquid interfaces | No slip (µ = 0) |
| Fluid domain inlet | Normal inflow velocity (vin) | |
| Fluid domain outlet | Pressure, no viscous stress (p0 = 0) |
4.3. CFD Model Refinement: Oxygen Diffusion through PDMS Walls
4.4. Results and Discussion
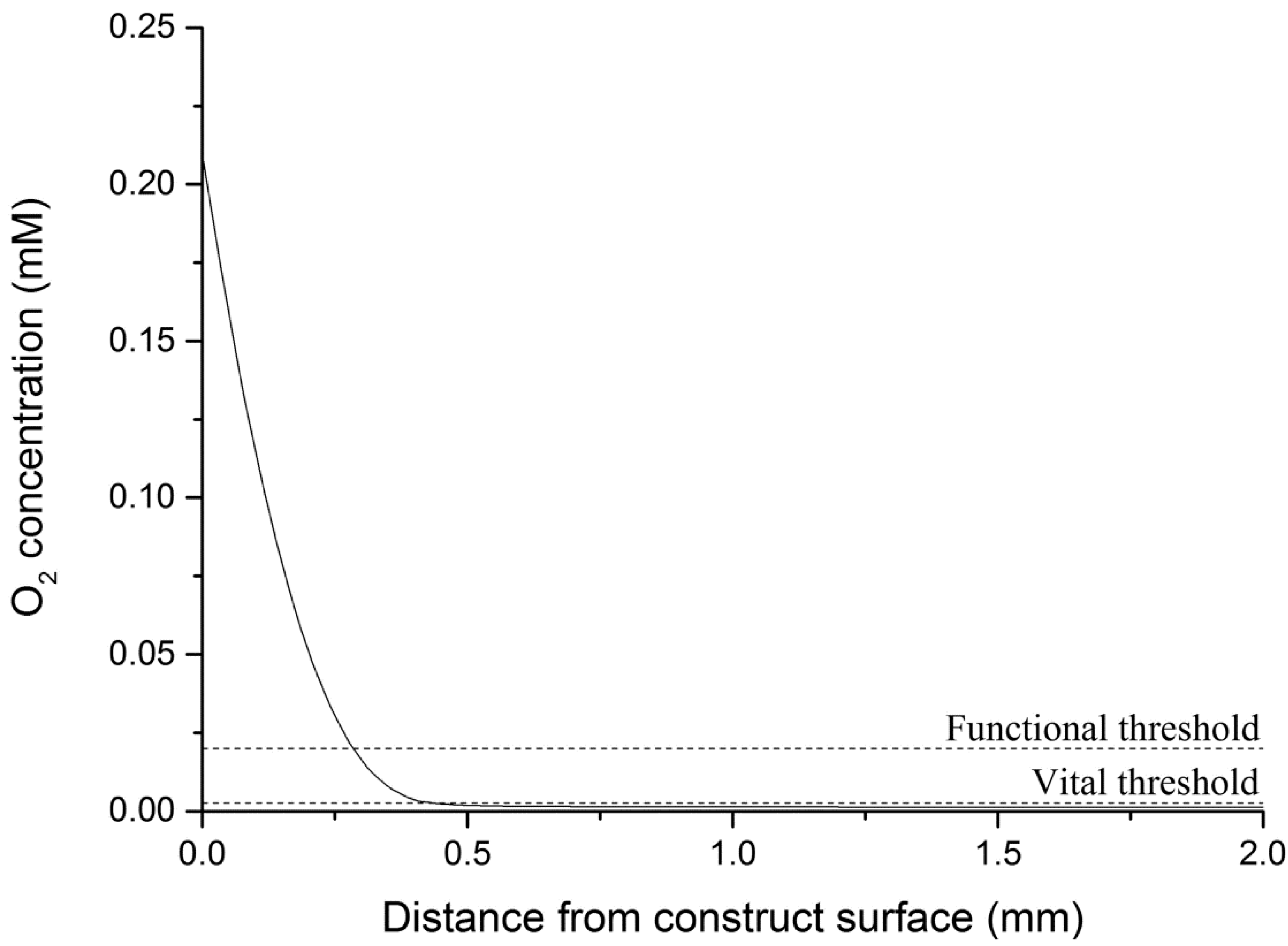
4.4.1. Theoretical Maximum Thickness for Functional Physiologically Relevant Hepatocyte Constructs
4.4.2. Fluid Dynamics in Micro- and Milli-Fluidic Systems
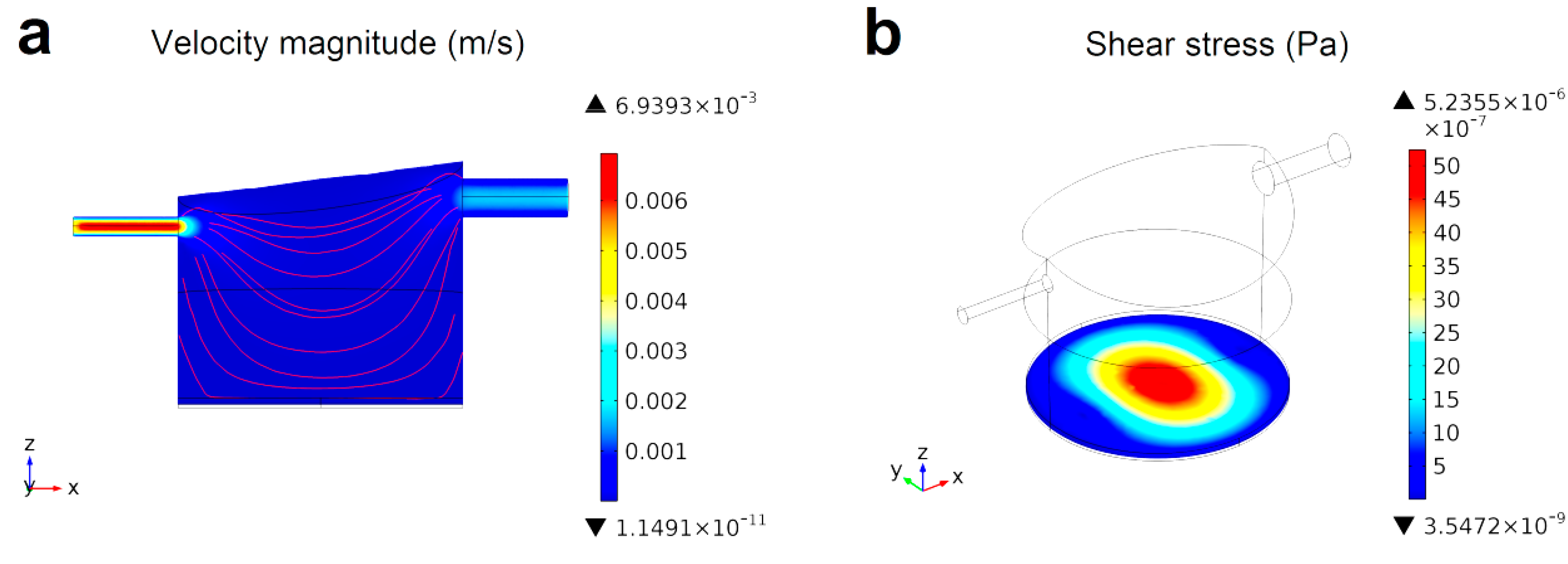
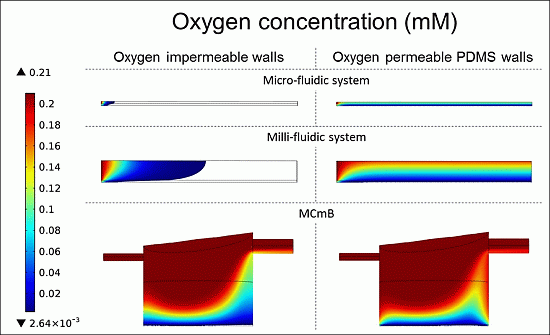
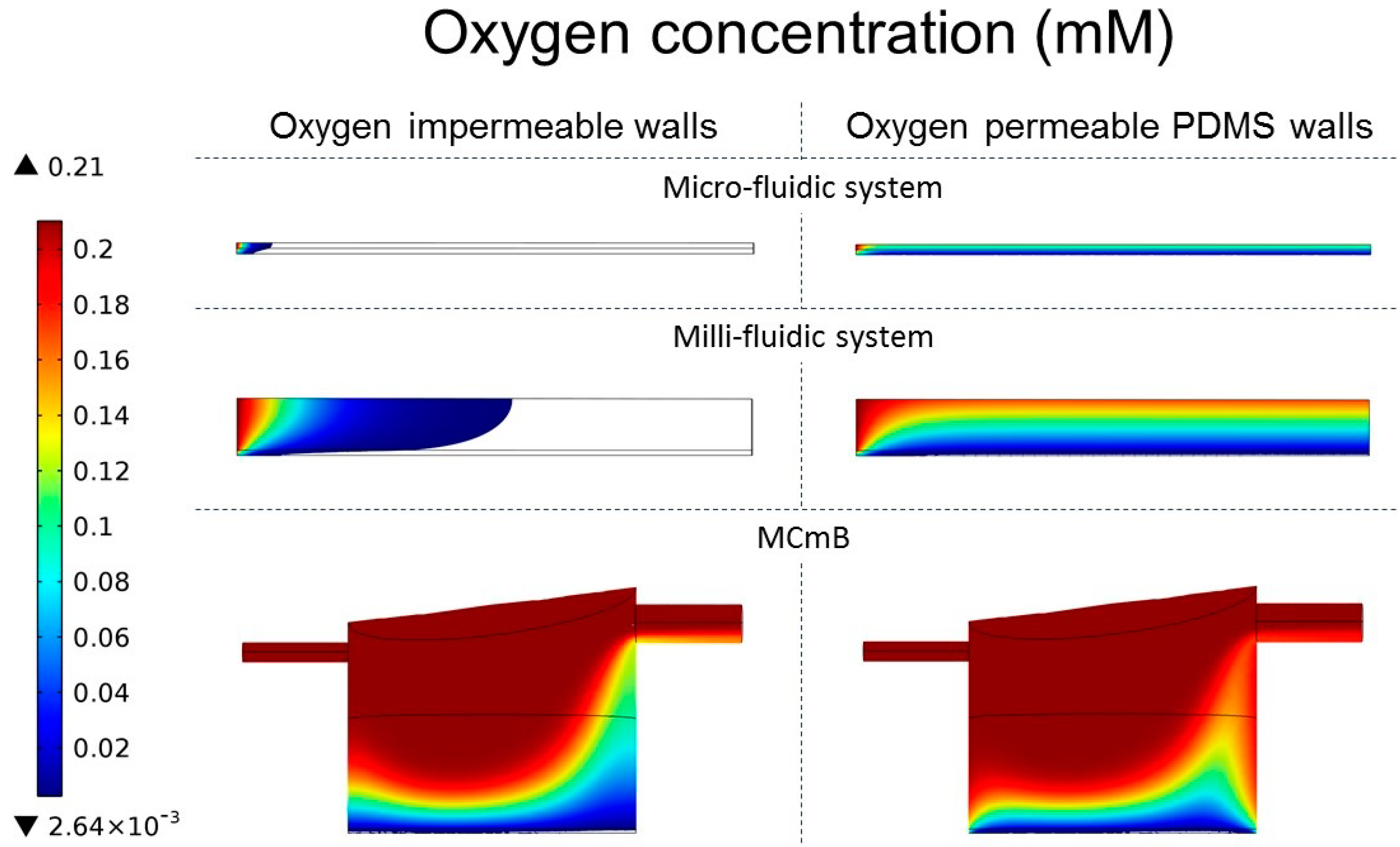
4.4.3. Oxygen Concentration Profiles in Micro- and Milli-Fluidic Systems
| Modelled System | Micro-Fluidic | Milli-Fluidic | MCmB | |||
|---|---|---|---|---|---|---|
| OIW | OPW | OIW | OPW | OIW | OPW | |
| Viable cells (%) | 2.6 | 100 | 17.8 | 78 | 60.5 | 85.4 |
| Configuration | Flow Rate (m3/s) | Rin (mol/s) | Cell Number | Rcons (mol/s) | Rin/Rcons |
|---|---|---|---|---|---|
| Micro-fluidic | 2.84 × 10−14 | 1.42 × 10−13 | 5.60 × 104 | 4.48 × 10−13 | 0.3 |
| Milli-fluidic | 6.40 × 10−13 | 3.20 × 10−12 | 5.60 × 104 | 4.48 × 10−13 | 7.1 |
| MCmB | 3.00 × 10−9 | 1.50 × 10−8 | 2.47 × 106 | 1.98 × 10−11 | 758.3 |
5. The Allometric Approach
6. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Elliott, N.T.; Yuan, F. A review of three-dimensional in vitro tissue models for drug discovery and transport studies. J. Pharmcol. Sci. 2011, 100, 59–74. [Google Scholar] [CrossRef]
- Cimetta, E.; Godier-Furnémont, A.; Vunjak-Novakovic, G. Bioengineering heart tissue for in vitro testing. Curr. Opin. Biotechnol. 2013, 24, 926–932. [Google Scholar] [CrossRef] [PubMed]
- Rouwkema, J.; Gibbs, S.; Lutolf, M.P.; Martin, I.; Vunjak-Novakovic, G.; Malda, J. In vitro platforms for tissue engineering: Implications for basic research and clinical translation. J. Tissue Eng. Regen. Med. 2011, 5, e164–e167. [Google Scholar] [CrossRef] [PubMed]
- Astashkina, A.; Mann, B.; Grainger, D.W. A critical evaluation of in vitro cell culture models for high-throughput drug screening and toxicity. Pharmacol. Ther. 2012, 134, 82–106. [Google Scholar] [CrossRef] [PubMed]
- Nerem, R.M. Tissue engineering: The hope, the hype, and the future. Tissue Eng. 2006, 12, 1143–1150. [Google Scholar] [CrossRef] [PubMed]
- Shoji, Y.; Nakashima, H. Nutraceutics and delivery systems. J. Drug Target. 2004, 12, 385–391. [Google Scholar] [CrossRef] [PubMed]
- Seok, J.; Warren, H.S.; Cuenca, A.G.; Mindrinos, M.N.; Baker, H.V.; Xu, W.; Richards, D.R.; Gao, H.; Hennessy, L.; Finnerty, C.C.; et al. Genomic responses in mouse models poorly mimic human inflammatory diseases. Proc. Natl. Acad. Sci. USA 2013, 110, 3507–3512. [Google Scholar] [CrossRef] [PubMed]
- Bracken, M.B. Why animal studies are often poor predictors of human reactions to exposure. J. R. Soc. Med. 2009, 102, 120–122. [Google Scholar] [CrossRef] [PubMed]
- Alberts, B.; Johnson, A.; Lewis, J.; Raff, M.; Roberts, K.; Walte, P. Molecular Biology of the Cell, 4th ed.; Garland Science: New York, NY, USA, 2002. [Google Scholar]
- Di Nardo, P.; Minieri, M.; Ahluwalia, A. Engineering the Stem Cell Niche and the Differentiative Micro- and Macroenvironment: Technologies and Tools for Applying Biochemical, Physical and Structural Stimuli and Their Effects on Stem Cells. In Stem Cell Engineering; Springer: Heidelberg, Germany, 2011; pp. 41–59. [Google Scholar]
- Patzer, J.F. Oxygen consumption in a hollow fiber bioartificial liver. Artif. Organs 2004, 28, 83–98. [Google Scholar] [CrossRef] [PubMed]
- Schyschka, L.; Sánchez, J.J.M.; Wang, Z.; Burkhardt, B.; Müller-Vieira, U.; Zeilinger, K.; Bachmann, A.; Nadalin, S.; Damm, G.; Nussler, A.K.; et al. Hepatic 3D cultures but not 2D cultures preserve specific transporter activity for acetaminophen-induced hepatotoxicity. Arch. Toxicol. 2013, 87, 1581–1593. [Google Scholar] [CrossRef] [PubMed]
- Francioli, S.E.; Candrian, C.; Martin, K.; Heberer, M.; Martin, I.; Barbero, A. Effect of three-dimensional expansion and cell seeding density on the cartilage-forming capacity of human articular chondrocytes in type II collagen sponges. J. Biomed. Mater. Res. A 2010, 95, 924–931. [Google Scholar] [CrossRef] [PubMed]
- Iwasa, J.; Ochi, M.; Uchio, Y.; Katsube, K.; Adachi, N.; Kawasaki, K. Effects of cell density on proliferation and matrix synthesis of chondrocytes embedded in atelocollagen gel. Artif. Organs 2003, 27, 249–255. [Google Scholar] [CrossRef] [PubMed]
- Loeve, S. About a Definition of Nano: How to Articulate Nano and Technology? Int. J. Philos. Chem. 2010, 6, 3–18. [Google Scholar]
- Lee, M.Y.; Kumar, R.A.; Sukumaran, S.M.; Hogg, M.G.; Clark, D.S.; Dordick, J.S. Three-dimensional cellular microarray for high-throughput toxicology assays. Proc. Natl. Acad. Sci. USA 2008, 105, 59–63. [Google Scholar] [CrossRef] [PubMed]
- Sung, J.H.; Shuler, M.L. In vitro microscale systems for systematic drug toxicity study. Bioprocess Biosyst. Eng. 2010, 33, 5–19. [Google Scholar] [CrossRef] [PubMed]
- Sung, J.H.; Esch, M.B.; Shuler, M.L. Integration of in silico and in vitro platforms for pharmacokinetic-pharmacodynamic modeling. Expert Opin. Drug Metab. Toxicol. 2010, 6, 1063–1081. [Google Scholar] [CrossRef] [PubMed]
- Wei, C.W.; Cheng, J.Y.; Young, T.H. Elucidating in vitro cell-cell interaction using a microfluidic coculture system. Biomed. Microdevices 2006, 8, 65–71. [Google Scholar] [CrossRef] [PubMed]
- Baudoin, R.; Corlu, A.; Griscom, L.; Legallais, C.; Leclerc, E. Trends in the development of microfluidic cell biochips for in vitro hepatotoxicity. Toxicol. In Vitro 2007, 21, 535–544. [Google Scholar] [CrossRef] [PubMed]
- Tingley, S. High-throughput cell culture: A real-world evaluation. Innov. Pharm. Technol. 2006, 54, 54–58. [Google Scholar]
- Tilles, A.W.; Baskaran, H.; Roy, P.; Yarmush, M.L.; Toner, M. Effects of oxygenation and flow on the viability and function of rat hepatocytes cocultured in a microchannel flat-plate bioreactor. Biotechnol. Bioeng. 2001, 73, 379–389. [Google Scholar] [CrossRef] [PubMed]
- Park, J.; Li, Y.; Berthiaume, F.; Toner, M.; Yarmush, M.L.; Tilles, A.W. Radial flow hepatocyte bioreactor using stacked microfabricated grooved substrates. Biotechnol. Bioeng. 2008, 99, 455–467. [Google Scholar] [CrossRef] [PubMed]
- McBeath, R.; Pirone, D.M.; Nelson, C.M.; Bhadriraju, K.; Chen, C.S. Cell shape, cytoskeletal tension, and RhoA regulate stem cell lineage commitment. Dev. Cell 2004, 6, 483–495. [Google Scholar] [CrossRef] [PubMed]
- Francis, K.; Palsson, B.O. Effective intercellular communication distances are determined by the relative time constants for cyto/chemokine secretion and diffusion. Proc. Natl. Acad. Sci. USA 1997, 94, 12258–12262. [Google Scholar] [CrossRef] [PubMed]
- Kilian, K.A.; Bugarija, B.; Lahn, B.T.; Mrksich, M. Geometric cues for directing the differentiation of mesenchymal stem cells. Proc. Natl. Acad. Sci. USA 2010, 107, 4872–4877. [Google Scholar] [CrossRef] [PubMed]
- Lundholt, B.K.; Scudder, K.M.; Pagliaro, L. A simple technique for reducing edge effect in cell-based assays. J. Biomol. Screening 2003, 8, 566–570. [Google Scholar] [CrossRef]
- Toepke, M.W.; Beebe, D.J. PDMS absorption of small molecules and consequences in microfluidic applications. Lab Chip 2006, 6, 1484–1486. [Google Scholar] [CrossRef] [PubMed]
- Regehr, K.J.; Domenech, M.; Koepsel, J.T.; Carver, K.C.; Ellison-Zelski, S.J.; Murphy, W.L.; Schuler, L.A.; Alarid, E.T.; Beebe, D.J. Biological implications of polydimethylsiloxane-based microfluidic cell culture. Lab Chip 2009, 9, 2132–2139. [Google Scholar] [CrossRef] [PubMed]
- Mehta, K.; Mehta, G.; Takayama, S.; Linderman, J. Quantitative Inference of Cellular Parameters From Microfluidic Cell Culture Systems. Biotechnol. Bioeng. 2009, 103, 966–974. [Google Scholar] [CrossRef] [PubMed]
- Mazzei, D.; Guzzardi, M.A.; Giusti, S.; Ahluwalia, A. A low shear stress modular bioreactor for connected cell culture under high flow rates. Biotechnol. Bioeng. 2010, 106, 127–137. [Google Scholar] [PubMed]
- Martin, Y.; Vermette, A. Bioreactors for tissue mass culture: Design, characterization, and recent advances. Biomaterials 2005, 26, 7481–7503. [Google Scholar] [CrossRef] [PubMed]
- Freshney, I.R. Culture of Animal Cells: A Manual of Basic Technique and Specialized Applications, 6th ed.; Wiley-Blackwell: Hoboken, NJ, USA, 2010. [Google Scholar]
- Coleman, W.B.; Presnell, S.C. Plasticity of the hepatocyte phenotype in vitro: Complex phenotypic transitions in proliferating hepatocyte cultures suggest bipotent differentiation capacity of mature hepatocytes. Hepatology 1996, 24, 1542–1546. [Google Scholar] [PubMed]
- Guillouzo, A. Liver cell models in in vitro toxicology. Environ. Health Perspect. 1998, 106, 511–532. [Google Scholar] [CrossRef] [PubMed]
- Nahmias, Y.; Berthiaume, F.; Yarmush, M.L. Integration of technologies for hepatic tissue engineering. Adv. Biochem. Eng. Biotechnol. 2007, 103, 309–329. [Google Scholar] [PubMed]
- Smith, M.K.; Mooney, D.J. Hypoxia leads to necrotic hepatocyte death. J. Biomed. Mater. Res. A 2007, 80, 520–529. [Google Scholar] [CrossRef] [PubMed]
- Tanaka, Y.; Yamato, M.; Okano, T.; Kitamori, T.; Sato, K. Evaluation of effects of shear stress on hepatocytes by a microchip-based system. Meas. Sci. Technol. 2006, 17, 3167–3170. [Google Scholar] [CrossRef]
- Nakatsuka, H.; Sokabe, T.; Yamamoto, K.; Sato, Y.; Hatakeyama, K.; Kamiya, A.; Ando, J. Shear stress induces hepatocyte PAI-1 gene expression through cooperative Sp1/Ets-1 activation of transcription. Am. J. Physiol. Gastrointest. Liver Physiol. 2006, 291, G26–G34. [Google Scholar] [CrossRef] [PubMed]
- Rutkowski, J.M.; Swartz, M.A. A driving force for change: Interstitial flow as a morphoregulator. Trends Cell Biol. 2007, 17, 44–50. [Google Scholar] [CrossRef] [PubMed]
- Swartz, M.A.; Fleury, M.E. Interstitial flow and its effects in soft tissues. Annu. Rev. Biomed. Eng. 2007, 9, 229–256. [Google Scholar] [CrossRef] [PubMed]
- Zahorodny-Burke, M.; Nearingburg, B.; Elias, A.L. Finite element analysis of oxygen transport in microfluidic cell culture devices with varying channel architectures, perfusion rates, and materials. Chem. Eng. Sci. 2011, 66, 6244–6253. [Google Scholar] [CrossRef]
- Kim, M.C.; Lam, R.H.W.; Thorsen, T.; Asada, H.H. Mathematical analysis of oxygen transfer through polydimethylsiloxane membrane between double layers of cell culture channel and gas chamber in microfluidic oxygenator. Microfluid. Nanofluidics 2013, 15, 285–296. [Google Scholar] [CrossRef]
- Ahluwalia, A.; Mazzei, D.; Vinci, B.; Vozzi, G. Improved Bioreactor Chamber. EP 2318510 A2, 11 May 2011. [Google Scholar]
- Tan, G.D.S.; Toh, G.W.; Birgersson, E.; Robens, J.; van Noort, D.; Leo, H.L. A thin-walled polydimethylsiloxane bioreactor for high-density hepatocyte sandwich culture. Biotechnol. Bioeng. 2013, 110, 1663–1673. [Google Scholar] [CrossRef] [PubMed]
- Buchwald, P. FEM-based oxygen consumption and cell viability models for avascular pancreatic islets. Theor. Biol. Med. Model. 2009, 6, 5. [Google Scholar] [CrossRef] [PubMed]
- Comsol, A. COMSOL Multiphysics Modeling Guide; Version 3.5a; COMSOL AB: Stockholm, Sweden, 2009. [Google Scholar]
- Silbey, R.J.; Alberty, R.A.; Bawendi, M.G. Physical Chemistry; John Wiley & Sons: Hoboken, NJ, USA, 2005. [Google Scholar]
- Nyberg, S.L.; Remmel, R.P.; Mann, H.J.; Peshwa, M.V.; Hu, W.S.; Cerra, F.B. Primary hepatocytes outperform Hep G2 cells as the source of biotransformation functions in a bioartificial liver. Ann. Surg. 1994, 220, 59–67. [Google Scholar] [PubMed]
- Kutty, M.N. Site Selection for Aquaculture: Chemical Features of Water; African Regional Aquaculture Centre: Port Harcourt, Nigeria, 1987. [Google Scholar]
- Frisancho, A.R. Functional adaptation to high altitude hypoxia. Science 1975, 187, 313–319. [Google Scholar] [CrossRef] [PubMed]
- Buchwald, P. A local glucose-and oxygen concentration-based insulin secretion model for pancreatic islets. Theor. Biol. Med. Model. 2011, 8, 20. [Google Scholar] [CrossRef] [PubMed]
- Oller, A.R.; Buser, C.W.; Tyo, M.A.; Thilly, W.G. Growth of mammalian cells at high oxygen concentrations. J. Cell Sci. 1989, 94, 43–49. [Google Scholar] [PubMed]
- Haselgrove, J.C.; Shapiro, I.M.; Silverton, S.F. Computer modeling of the oxygen supply and demand of cells of the avian growth cartilage. Am. J. Physiol. 1993, 265, C497–C506. [Google Scholar] [PubMed]
- Mehmetoglu, U.; Ates, S.; Berber, R. Oxygen diffusivity in calcium alginate gel beads containing Gluconobacter suboxydans. Artif. Cells Blood Substit. Immobil. Biotechnol. 1996, 24, 91–106. [Google Scholar] [CrossRef] [PubMed]
- Hulst, A.C.; Hens, H.J.H.; Buitelaar, R.M.; Tramper, J. Determination of the effective diffusion coefficient of oxygen in gel materials in relation to gel concentration. Biotechnol. Tech. 1989, 3, 199–204. [Google Scholar] [CrossRef]
- Ehsan, S.M.; George, S.C. Nonsteady state oxygen transport in engineered tissue: Implications for design. Tissue Eng. A 2013, 19, 1433–1442. [Google Scholar] [CrossRef]
- Van Stroe-Biezen, S.A.M.; Everaerts, F.M.; Janssen, L.J.J.; Tacken, R.A. Diffusion coefficients of oxygen, hydrogen peroxide and glucose in a hydrogel. Anal. Chim. Acta 1993, 273, 553–560. [Google Scholar] [CrossRef]
- Foy, B.D.; Rotem, A.; Toner, M.; Tompkins, R.G.; Yarmush, M.L. A device to measure the oxygen uptake rate of attached cells: Importance in bioartificial organ design. Cell Transplant. 1994, 3, 515–527. [Google Scholar] [PubMed]
- Allen, J.W.; Bhatia, S.N. Formation of steady-state oxygen gradients in vitro: Application to liver zonation. Biotechnol. Bioeng. 2003, 82, 253–262. [Google Scholar] [CrossRef] [PubMed]
- De Groot, H.; Littauer, A.; Noll, T. Metabolic and pathological aspects of hypoxia in liver cells. In Oxygen Sensors Tissues; Springer: Heidelberg, Germany, 1988; pp. 49–64. [Google Scholar]
- Vinci, B.; Murphy, E.; Iori, E.; Marescotti, M.C.; Avogaro, A.; Ahluwalia, A. Flow-regulated glucose and lipid metabolism in adipose tissue, endothelial cell and hepatocyte cultures in a modular bioreactor. Biotechnol. J. 2010, 5, 618–626. [Google Scholar] [CrossRef] [PubMed]
- Vinci, B.; Duret, C.; Klieber, S.; Gerbal-Chaloin, S.; Sa-Cunha, A.; Laporte, S.; Suc, B.; Maurel, P.; Ahluwalia, A.; Daujat-Chavanieu, M.; et al. Modular bioreactor for primary human hepatocyte culture: Medium flow stimulates expression and activity of detoxification genes. Biotechnol. J. 2011, 6, 554–564. [Google Scholar] [CrossRef] [PubMed]
- Vinci, B.; Murphy, E.; Iori, E.; Meduri, F.; Fattori, S.; Marescotti, M.C.; Castagna, M.; Avogaro, A.; Ahluwalia, A. An in vitro model of glucose and lipid metabolism in a multicompartmental bioreactor. Biotechnol. J. 2012, 7, 117–126. [Google Scholar] [CrossRef] [PubMed]
- Vinci, B.; Cavallone, D.; Vozzi, G.; Mazzei, D.; Domenici, C.; Brunetto, M.; Ahluwalia, A. In vitro liver model using microfabricated scaffolds in a modular bioreactor. Biotechnol. J. 2010, 5, 232–241. [Google Scholar] [CrossRef] [PubMed]
- Guzzardi, M.A.; Domenici, C.; Ahluwalia, A. Metabolic control through hepatocyte and adipose tissue cross-talk in a multicompartmental modular bioreactor. Tissue Eng. A 2011, 17, 1635–1642. [Google Scholar] [CrossRef]
- Giulitti, S.; Magrofuoco, E.; Elvassore, N. Optimal periodic perfusion strategy for robust long-term microfluidic cell culture. Lab Chip 2013, 13, 4430–4441. [Google Scholar] [CrossRef] [PubMed]
- Nishikawa, M.; Kojima, N.; Komori, K.; Yamamoto, T.; Fujii, T.; Sakai, Y. Enhanced maintenance and functions of rat hepatocytes induced by combination of on-site oxygenation and coculture with fibroblasts. J. Biotechnol. 2008, 133, 253–260. [Google Scholar] [CrossRef] [PubMed]
- Skolimowski, M.; Nielsen, M.W.; Emnéus, J.; Molin, S.; Taboryski, R.; Sternberg, C.; Dufva, M.; Geschke, O. Microfluidic dissolved oxygen gradient generator biochip as a useful tool in bacterial biofilm studies. Lab Chip 2010, 10, 2162–2169. [Google Scholar] [CrossRef] [PubMed]
- Cimetta, E.; Flaibani, M.; Mella, M.; Serena, E.; Boldrin, L.; de Coppi, P.; Elvassore, N. Enhancement of viability of muscle precursor cells on 3D scaffold in a perfusion bioreactor. Int. J. Artif. Organs 2007, 30, 415–428. [Google Scholar] [PubMed]
- Brandrup, J.; Immergut, E.H.; Grulke, E.A.; Akihiro, A.; Bloch, D.R. Polymer Handbook; Interscience Publishers: New York, NY, USA, 2004. [Google Scholar]
- Sayed-Ahmed, M.E.; Saif-Elyazal, A.; Iskander, L. Laminar Flow and Heat Transfer of Herschel-Bulkley Fluids in a Rectangular Duct; Finite-Element Analysis. Tikrit J. Sci. Eng. 2009, 12, 99–107. [Google Scholar]
- Weibel, E.R.; Bacigalupe, L.D.; Schmitt, B.; Hoppeler, H. Allometric scaling of maximal metabolic rate in mammals: Muscle aerobic capacity as determinant factor. Respir. Physiol. Neurobiol. 2004, 140, 115–132. [Google Scholar] [CrossRef] [PubMed]
- Elert, G. The Physics Factbook. Available online: http://hypertextbook.com/facts (accessed on 11 May 2014).
- NASA-STD-3000, Man-Systems Integration Standards. Available online: http://msis.jsc.nasa.gov (accessed on 11 May 2014).
- Sohlenius-Sternbeck, A.K. Determination of the hepatocellularity number for human, dog, rabbit, rat and mouse livers from protein concentration measurements. Toxicol. In Vitro 2006, 20, 1582–1586. [Google Scholar] [CrossRef] [PubMed]
- Sbrana, T.; Ahluwalia, A. Engineering Quasi-Vivo in vitro organ models. Adv. Exp. Med. Biol. 2012, 745, 138–153. [Google Scholar] [PubMed]
- Vozzi, F.; Mazzei, D.; Vinci, B.; Vozzi, G.; Sbrana, T.; Ricotti, L.; Forgione, N.; Ahluwalia, A. A flexible bioreactor system for constructing in vitro tissue and organ models. Biotechnol. Bioeng. 2011, 108, 2129–2140. [Google Scholar] [CrossRef] [PubMed]
- Haycock, J.; Ahluwalia, A.; Wilkinson, M.J. Cellular In Vitro Testing: Methods and Protocols; Pan Stanford Publishing Pte. Ltd.: Singapore, Singapore, 2014. [Google Scholar]
© 2014 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Mattei, G.; Giusti, S.; Ahluwalia, A. Design Criteria for Generating Physiologically Relevant In Vitro Models in Bioreactors. Processes 2014, 2, 548-569. https://doi.org/10.3390/pr2030548
Mattei G, Giusti S, Ahluwalia A. Design Criteria for Generating Physiologically Relevant In Vitro Models in Bioreactors. Processes. 2014; 2(3):548-569. https://doi.org/10.3390/pr2030548
Chicago/Turabian StyleMattei, Giorgio, Serena Giusti, and Arti Ahluwalia. 2014. "Design Criteria for Generating Physiologically Relevant In Vitro Models in Bioreactors" Processes 2, no. 3: 548-569. https://doi.org/10.3390/pr2030548
APA StyleMattei, G., Giusti, S., & Ahluwalia, A. (2014). Design Criteria for Generating Physiologically Relevant In Vitro Models in Bioreactors. Processes, 2(3), 548-569. https://doi.org/10.3390/pr2030548