Intensification of Multiphase Reactions in Petroleum Processing: A Simulation Study of SK Static Mixer Using NaClO for H2S Removal
Abstract
1. Introduction
2. Materials and Methods
2.1. Physical Model and Boundary Conditions
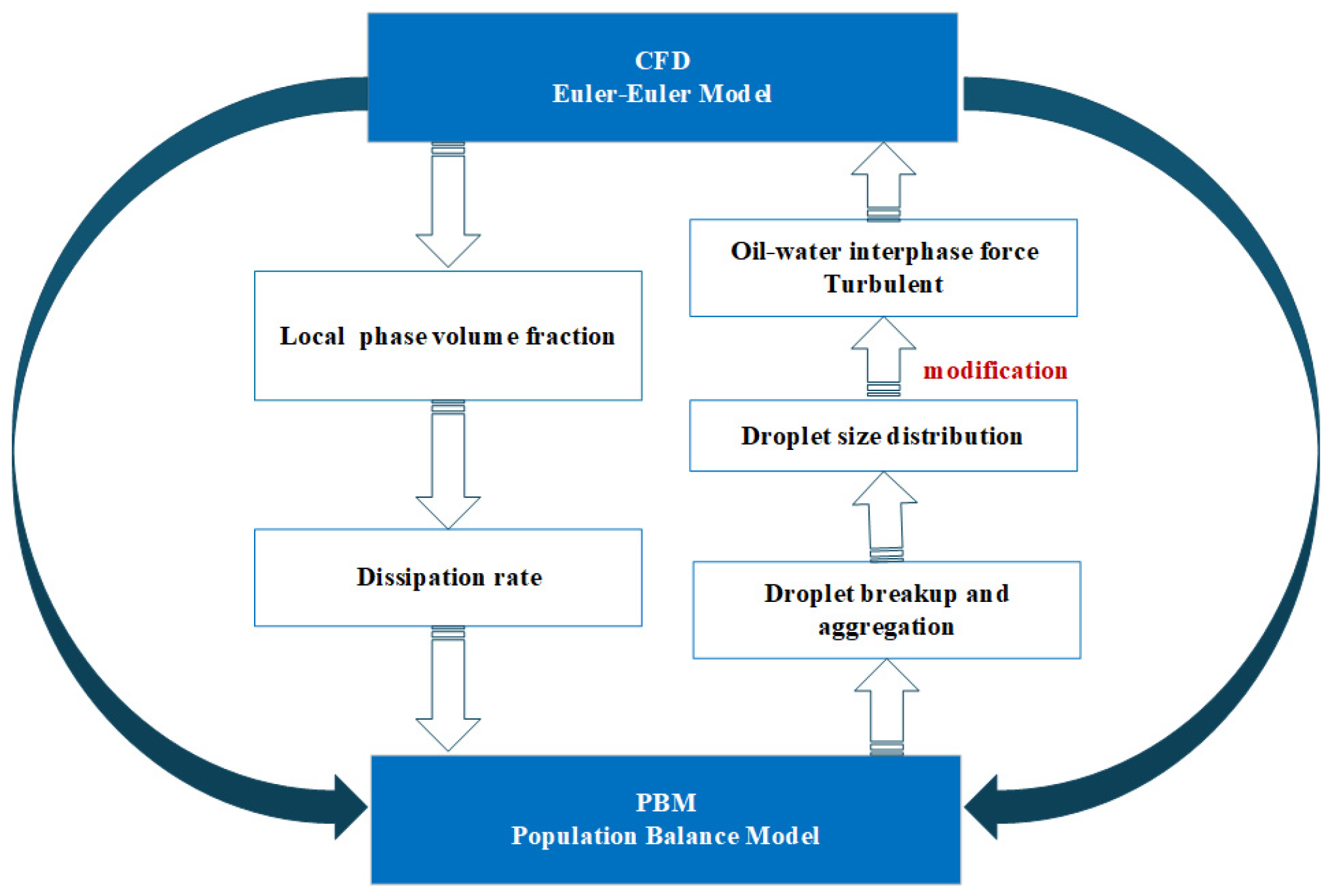
2.2. Computational Method and Assumptions
- The acceleration of gravity is 9.81 m/s2 in the direction of the z-axis.
- In this study, the volumetric flow rate of crude oil significantly exceeds that of the aqueous phase. Therefore, crude oil is set as the continuous phase and water is set as the dispersed phase during oil-water mixing.
- Mass transfer occurs exclusively at the oil-water interface.
2.3. Characterization Methods
2.3.1. Hydrogen Sulfide Removal Efficiency
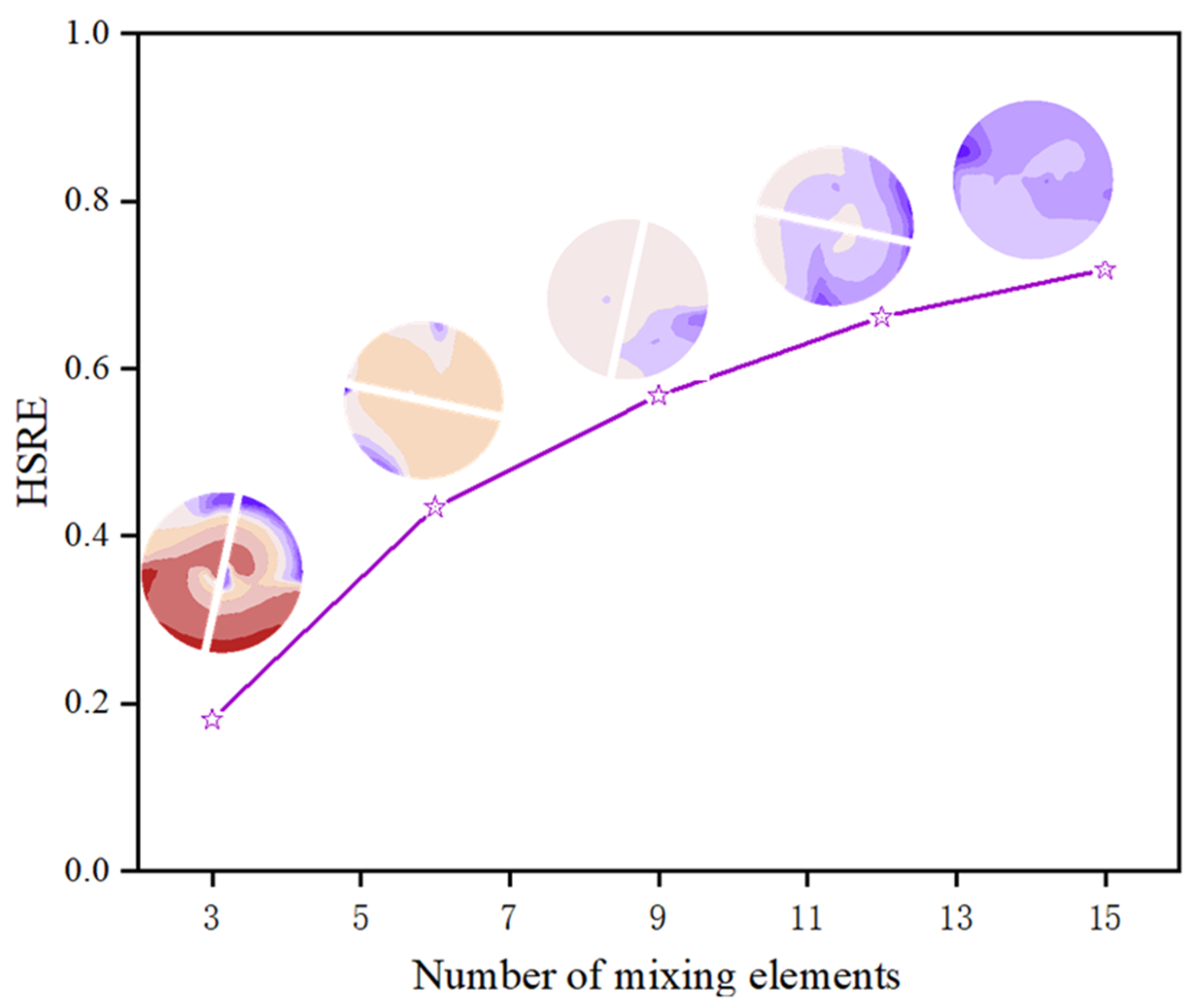
2.3.2. Mixing Effect
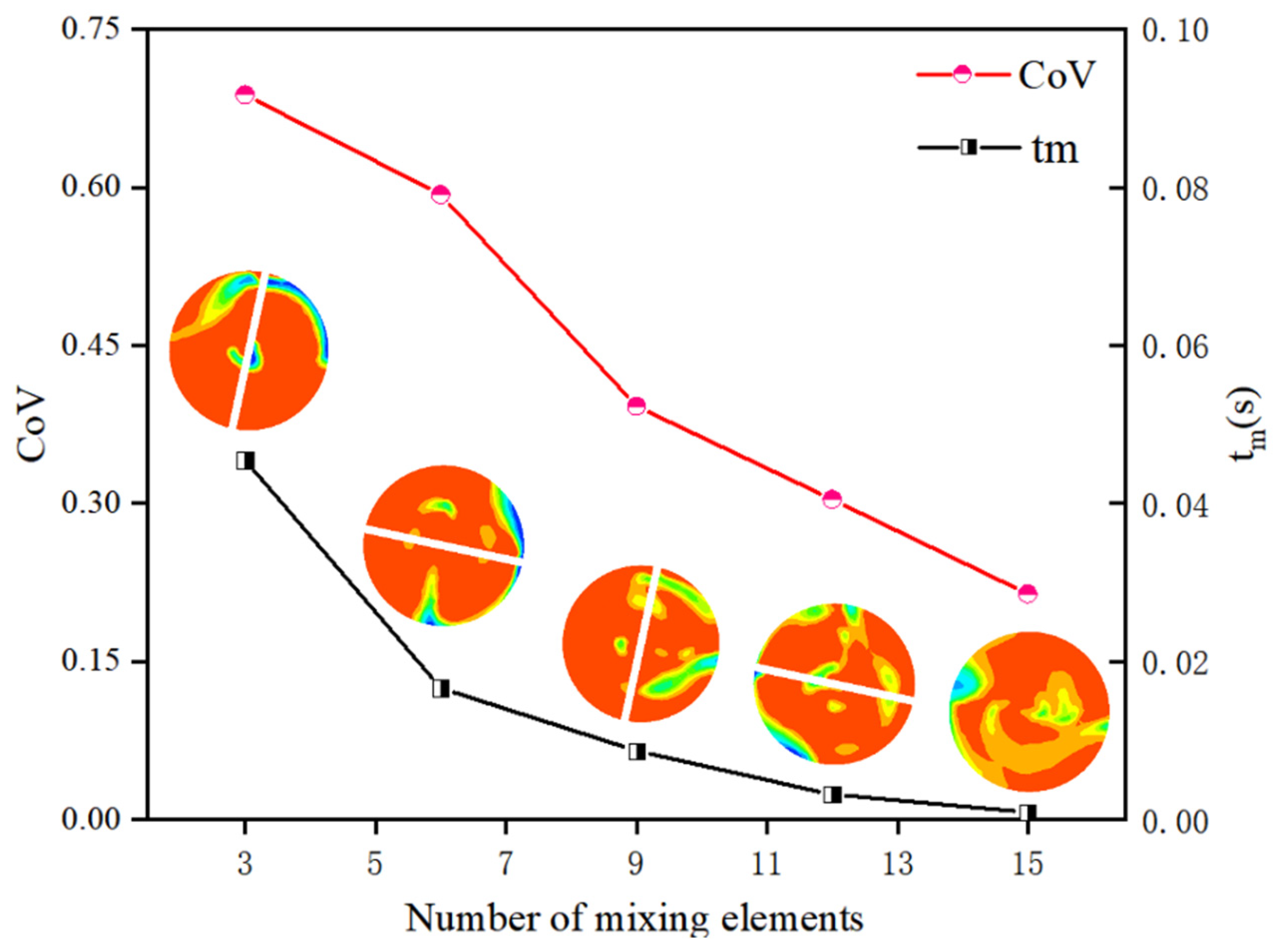
- The coefficient of variation (CoV) is used to evaluate the mixing effectiveness, representing the deviation of the crude oil volume fraction at the sampling point from the average volume fraction of crude oil across the entire cross-section. A lower CoV indicates a better mixing effect [43].where N is the number of grids in the static mixer cross-section, and, in this paper, we take N to be 2000. is the volume fraction of oil-phase components in each grid. is the average volume fraction of crude oil at the entire outlet plane.
- Mixing efficiency was further assessed using the micro-mixing time (tm, in seconds). This parameter indicates the time required to achieve homogeneous mixing of crude oil and water at a microscopic level. A lower tm value reflects a higher mixing efficiency of the static mixer [44].where represents the kinematic viscosity of the continuous phase fluid, m2/s. denotes the energy dissipation rate per unit time in the mixer (calculated by simulation software, m2/s3).
2.3.3. Droplet Dispersion Effect
2.3.4. Differential Pressure
2.4. Grid Irrelevance Test
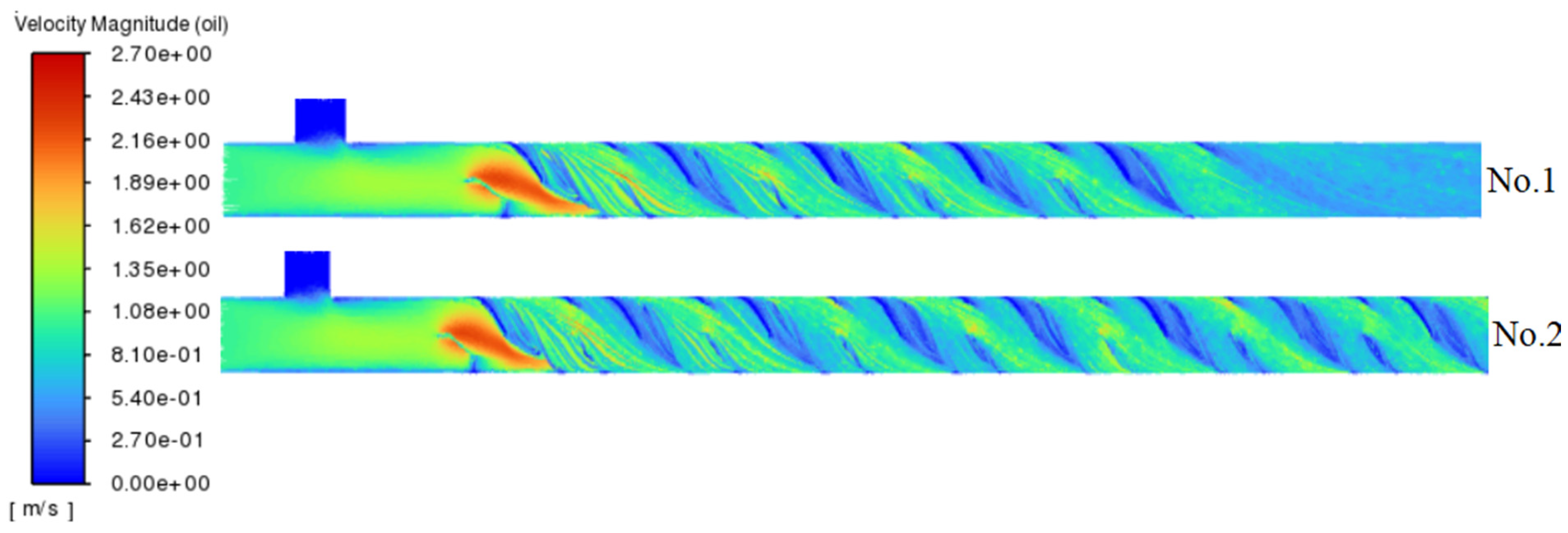
2.5. Model Validation
3. Results and Discussion
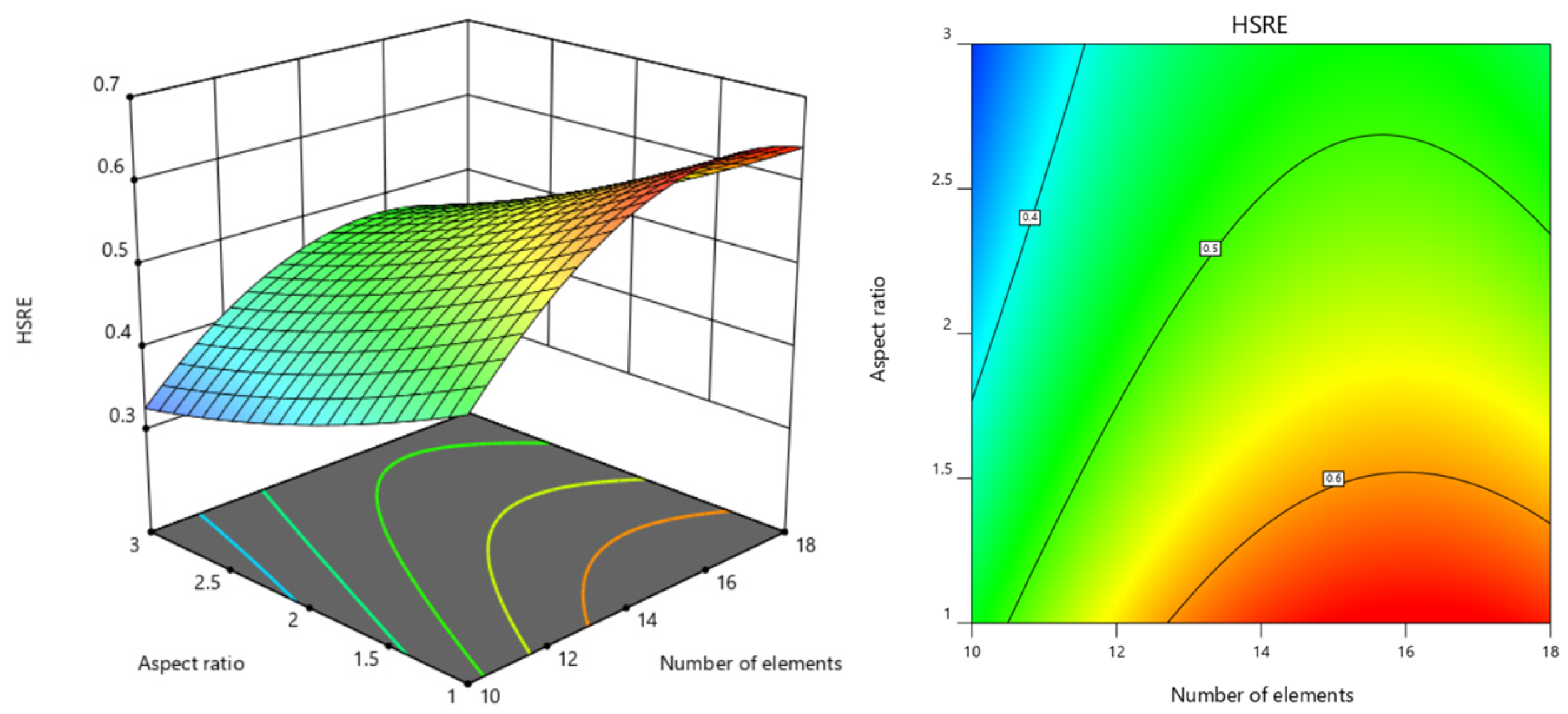
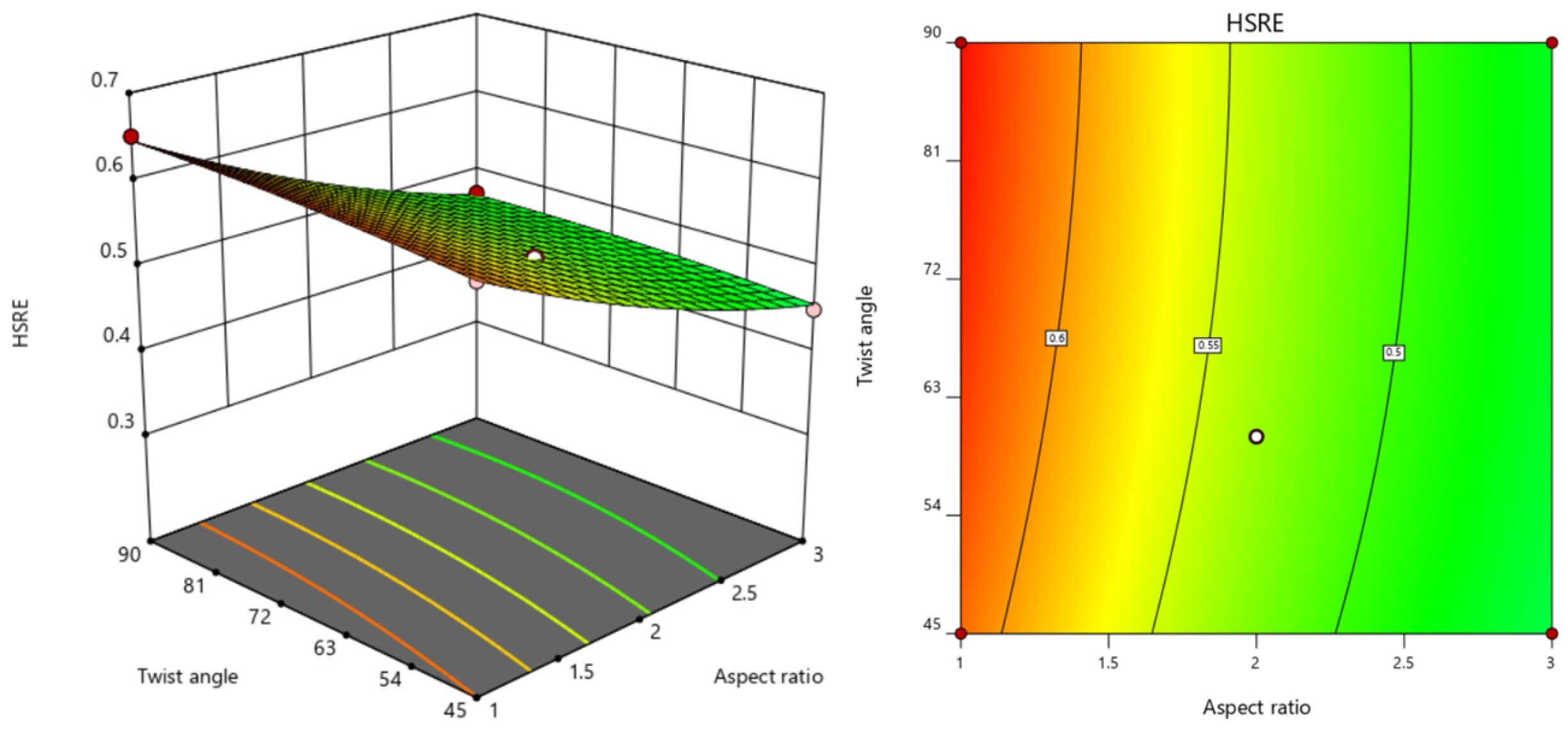
3.1. Structural Optimization of SK Static Mixer
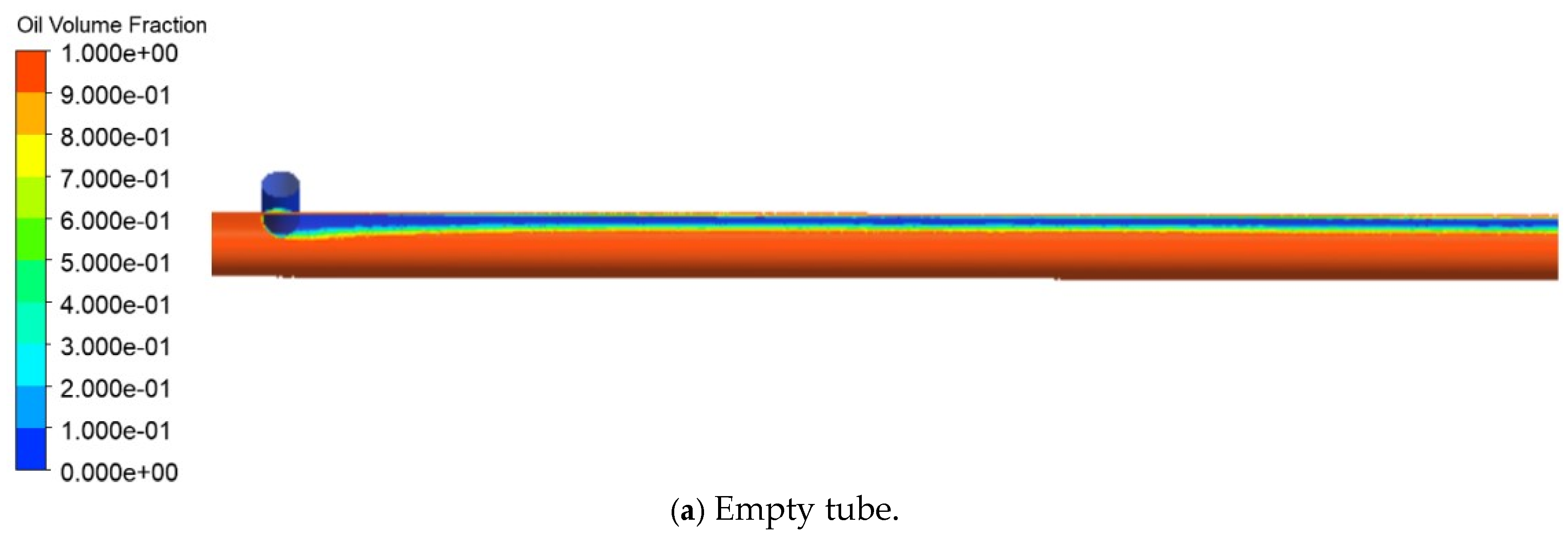
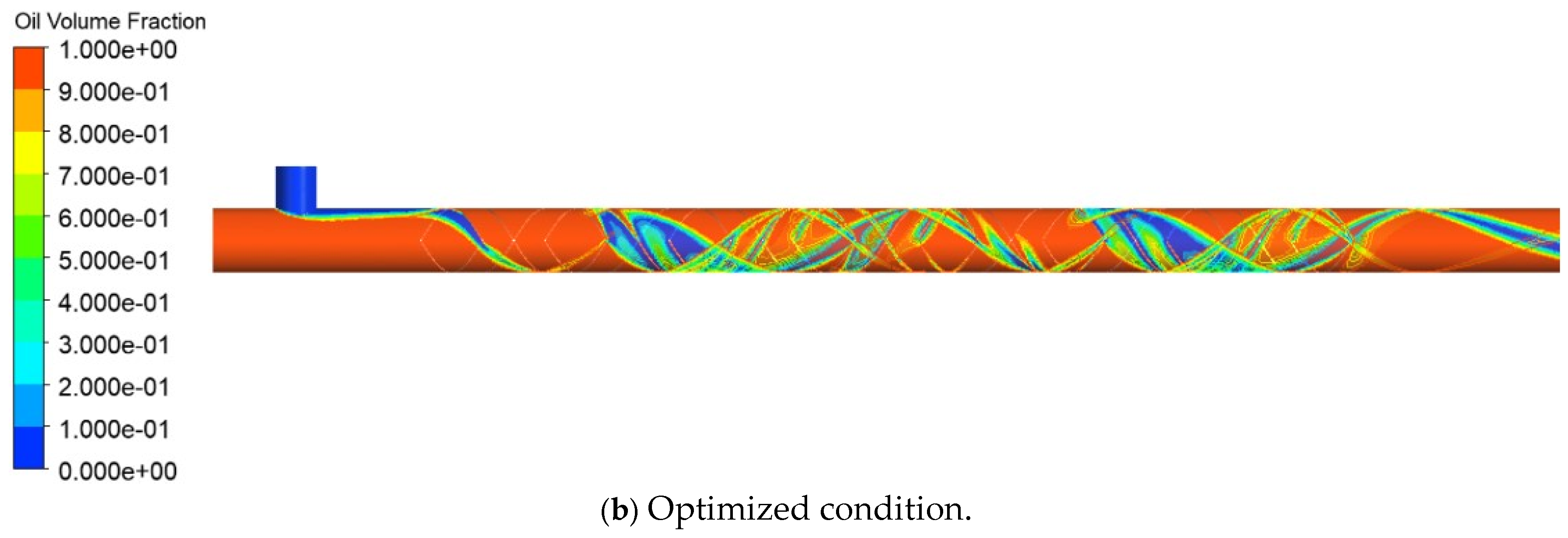
3.2. Static Mixer Performance
3.2.1. Hydrogen Sulfide Removal Efficiency
3.2.2. Mixing Effect
3.2.3. Differential Pressure
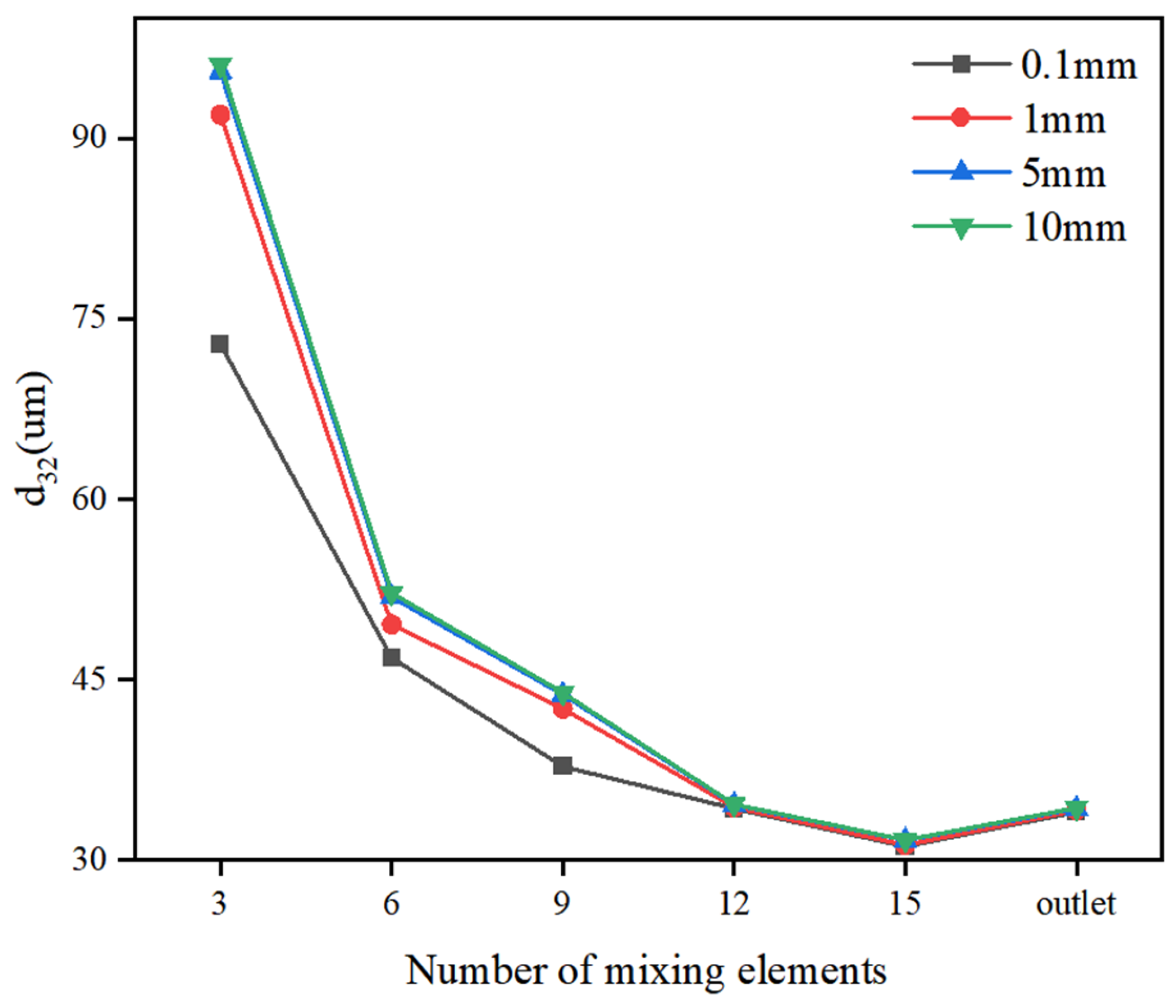
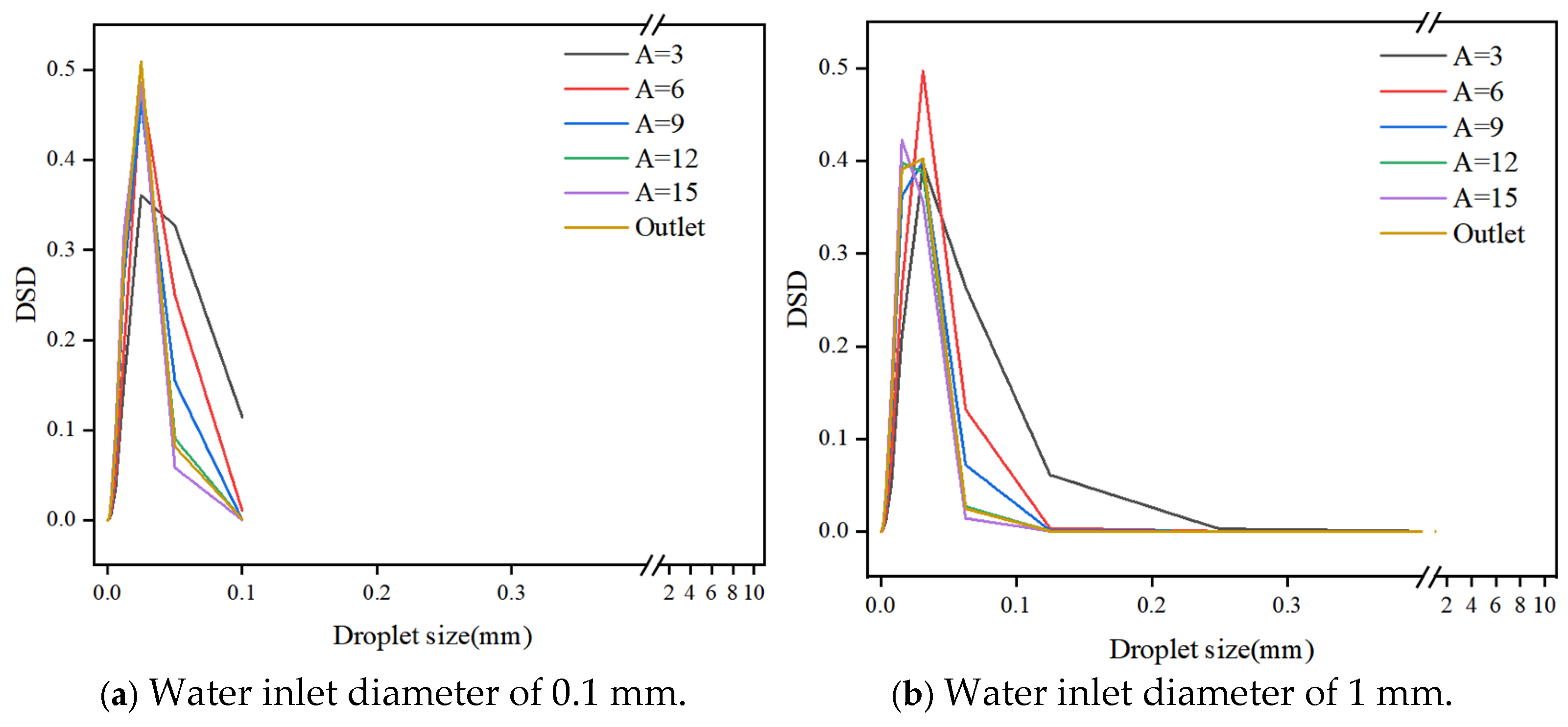
3.3. Water Inlet Diameter
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Sun, H.; Zhong, L.; Zhu, Y.; Zhu, J.; Li, Z.; Zhang, Z.; Zhou, Y. Assessing sulfate-reducing bacteria influence on oilfield safety: Hydrogen sulfide emission and pipeline corrosion failure. Eng. Fail. Anal. 2024, 164, 108646. [Google Scholar] [CrossRef]
- Marriott, R.A.; Pirzadeh, P.; Marrugo-Hernandez, J.J.; Raval, S. Hydrogen sulfide formation in oil and gas. Can. J. Chem. 2016, 94, 406–413. [Google Scholar] [CrossRef]
- Guzenkova, A.S.; Artamonova, I.V.; Guzenkov, S.A.; Ivanov, S.S. Steel Corrosion in Hydrogen Sulfide Containing Oil Field Model Media. Metallurgist 2021, 65, 517–521. [Google Scholar] [CrossRef]
- Sun, H.; Zhong, L.; Zhu, Y.; Zhu, J.; Zhou, Y. Risk prediction for hydrogen sulfide emission based on sulfate-reducing bacteria in the water flooding oilfield. Phys. Fluids 2024, 36, 057119. [Google Scholar] [CrossRef]
- Almasvandi, M.H.; Rahimi, M.; Tagheie, Y. Microfluidic cold stripping of H2S from crude oil in low temperature and natural gas consumption. J. Nat. Gas Sci. Eng. 2016, 34, 499–508. [Google Scholar] [CrossRef]
- Vilmain, J.-B.; Courousse, V.; Biard, P.-F.; Azizi, M.; Couvert, A. Kinetic study of hydrogen sulfide absorption in aqueous chlorine solution. Chem. Eng. Res. Des. 2014, 92, 191–204. [Google Scholar] [CrossRef]
- Busca, G.; Pistarino, C. Technologies for the abatement of sulphide compounds from gaseous streams: A comparative overview. J. Loss Prev. Process Ind. 2003, 16, 363–371. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, H.; Zhang, X.; Lu, F.; Wang, W.; Zhang, Y.; Nie, Q.; He, P. Dual roles of odor cleaners and pollutant producers for chemical scrubbing and biological treatment: Evidence in a food waste anaerobic digestion plant. Chem. Eng. J. 2024, 496, 153898. [Google Scholar] [CrossRef]
- Taylor, R.A.; Penney, W.R.; Vo, H.X. Scale-up Methods for Fast Competitive Chemical Reactions in Pipeline Mixers. Ind. Eng. Chem. Res. 2005, 44, 6095–6102. [Google Scholar] [CrossRef]
- Shen, Y. Study on Technology of Removing Hydrogen Sulfide from Crude Oil. Master’s Thesis, China University of Petroleum (East China) Shandong, Qingdao, China, 2010. [Google Scholar]
- Khinast, J.G.; Bauer, A.; Bolz, D.; Panarello, A. Mass-transfer enhancement by static mixers in a wall-coated catalytic reactor. Chem. Eng. Sci. 2003, 58, 1063–1070. [Google Scholar] [CrossRef]
- Madhuranthakam, C.M.R.; Pan, Q.; Rempel, G.L. Continuous process for production of hydrogenated nitrile butadiene rubber using a Kenics® KMX static mixer reactor. AlChE J. 2009, 55, 2934–2944. [Google Scholar] [CrossRef]
- Li, D.; Xiong, K.; Yang, Z.; Liu, C.; Feng, X.; Lu, X. Process intensification of heterogeneous photocatalysis with static mixer: Enhanced mass transfer of reactive species. Catal. Today 2011, 175, 322–327. [Google Scholar] [CrossRef]
- Al Taweel, A.M.; Azizi, F.; Sirijeerachai, G. Static mixers: Effective means for intensifying mass transfer limited reactions. Chem. Eng. Process. Process Intensif. 2013, 72, 51–62. [Google Scholar] [CrossRef]
- Ren, X.; Mei, Y.; Feng, M.; Li, B.; Lyu, W.; Wen, Y.; Wang, C.; He, D. Process intensification of removing arsenic from industrial phosphoric acid by Keltics static mixer. CIESC J. 2018, 69, 218–225. [Google Scholar]
- Biard, P.-F.; Dang, T.T.; Bocanegra, J.; Couvert, A. Intensification of the O3/H2O2 advanced oxidation process using a continuous tubular reactor filled with static mixers: Proof of concept. Chem. Eng. J. 2018, 344, 574–582. [Google Scholar] [CrossRef]
- Gong, H.; Gao, L.; Nie, K.; Wang, M.; Tan, T. A new reactor for enzymatic synthesis of biodiesel from waste cooking oil: A static-mixed reactor pilot study. Renew. Energy 2020, 154, 270–277. [Google Scholar] [CrossRef]
- Chetpattananondh, P.; Tabtimmuang, A.; Prasertsit, K. Enhanced Glycerolysis of Fatty Acid Methyl Ester by Static Mixer Reactor. ACS Omega 2024, 9, 39703–39714. [Google Scholar] [CrossRef]
- Liu, Y.; Liu, M.; Huang, Z.; Wang, H.; Yuan, P.; Xu, X.; Yang, J. Numerical simulation of the mixing and salt washing effects of a static mixer in an electric desalination process. Processes 2024, 12, 883. [Google Scholar] [CrossRef]
- Lupo, M.; Sofia, D.; Barletta, D.; Poletto, M. Calibration of DEM Simulation of Cohesive Particles. Chem. Eng. Tran. 2019, 74, 379–384. [Google Scholar]
- Haddadi, M.M.; Hosseini, S.H.; Rashtchian, D.; Ahmadi, G. CFD modeling of immiscible liquids turbulent dispersion in Kenics static mixers: Focusing on droplet behavior. Chin. J. Chem. Eng. 2020, 28, 348–361. [Google Scholar] [CrossRef]
- Deng, J.; Wu, J.; Han, L.; Zhou, Y. CFD-PBM coupled modeling of liquid-liquid interphase mass transfer behaviors inside the Kenics static mixer. Powder Technol. 2024, 445, 120119. [Google Scholar] [CrossRef]
- Cao, Q.; Zhou, J.; Qian, Y.; Yang, S. Three-Dimensional Model on Liquid–Liquid Mass Transfer of the Kenics Static Mixer: Considering Dynamic Droplet Size Distribution. Ind. Eng. Chem. Res. 2023, 62, 10507–10522. [Google Scholar] [CrossRef]
- Meng, H.; Meng, T.; Yu, Y.; Wang, Z.; Wu, J. Experimental and numerical investigation of turbulent flow and heat transfer characteristics in the Komax static mixer. Int. J. Heat Mass Transf. 2022, 194, 123006. [Google Scholar] [CrossRef]
- Albertazzi, J.; Florit, F.; Busini, V.; Rota, R. A novel Static Mixer for photochemical reactions. Chem. Eng. Process. Process Intensif. 2022, 182, 109201. [Google Scholar] [CrossRef]
- Moon, D.H.; Park, S.S.; Kang, S.-P.; Lee, W.; Park, K.T.; Chun, D.H.; Rhim, G.B.; Hwang, S.-M.; Youn, M.H.; Jeong, S.K. Determination of kinetic factors of CO2 mineralization reaction for reducing CO2 emissions in cement industry and verification using CFD modeling. Chem. Eng. J. 2021, 420, 129420. [Google Scholar] [CrossRef]
- Xie, Y.; Liu, J.; Li, J.; Yang, B. Study on Ozone Oxidation Regeneration of Ce3+ Enhanced by a Static Mixer. J. Chem. Eng. Chin. Univ. 2017, 31, 1318–1326. [Google Scholar]
- Santana, H.S.; Tortola, D.S.; Silva, J.L.; Taranto, O.P. Biodiesel synthesis in micromixer with static elements. Energy Convers. Manag. 2017, 141, 28–39. [Google Scholar] [CrossRef]
- Townsend-Small, A.; Edgar, A.; Fernandez, J.M.; Jackson, A.; Currit, N. High rates of hydrogen sulfide emissions measured from marginal oil wells near Austin and San Antonio, Texas. Environ. Res. Commun. 2024, 6, 091007. [Google Scholar] [CrossRef]
- Ghalwa, N.A.; Tamos, H.; ElAskalni, M.; El Agha, A.R. Generation of sodium hypochlorite (NaOCl) from sodium chloride solution using C/PbO2 and Pb/PbO2 electrodes. Int. J. Min. Met. Mater. 2012, 19, 561–566. [Google Scholar] [CrossRef]
- Li, B.; Huang, C.; Liu, L.Y.; Yao, L.; Ning, B.; Yang, L. Separation efficiency prediction of non-Newtonian oil-water swirl-vane separators in offshore platform based on GA-BP neural network. Ocean Eng. 2024, 296, 116984. [Google Scholar] [CrossRef]
- Lei, J.; Zhang, J. Numerical simulation of aerated flow in tunnel based on CFD-PBM coupled model. Ocean. Eng. 2024, 313, 119407. [Google Scholar] [CrossRef]
- Gu, D.; Wen, L.; Xu, H.; Ye, M. Study on hydrodynamics characteristics in a gas-liquid stirred tank with a self-similarity impeller based on CFD-PBM coupled model. J. Taiwan Inst. Chem. Eng. 2023, 143, 104688. [Google Scholar] [CrossRef]
- Meng, H.; Wang, J.; Yu, Y.; Wang, Z.; Wu, J. CFD-PBM Numerical Study on Liquid-Liquid Dispersion in the Q-Type Static Mixer. Ind. Eng. Chem. Res. 2021, 60, 18121–18135. [Google Scholar] [CrossRef]
- Luo, H.; Svendsen, H.F. Theoretical model for drop and bubble breakup in turbulent dispersions. AlChE J. 2004, 42, 1225–1233. [Google Scholar] [CrossRef]
- Tan, G.; Qian, K.; Jiang, S.; Wang, J.; Wang, J. CFD-PBM Investigation on Droplet Size Distribution in a Liquid-Liquid Stirred Tank: Effect of Impeller Type. Ind. Eng. Chem. Res. 2023, 62, 4109–4121. [Google Scholar] [CrossRef]
- Abrahamson, J. Collision Rates of Small Particles In a VigorouslyI Turbulent Fluid. Chem. Eng. Sci. 1975, 30, 1371–1379. [Google Scholar] [CrossRef]
- Saffman, P.G.; Turner, J.S. On the collision of drops in turbulent clouds. J. Fluid Mech. 2006, 1, 16–30. [Google Scholar] [CrossRef]
- Bouras, H.; Haroun, Y.; Philippe, R.; Augier, F.; Fongarland, P. CFD modeling of mass transfer in Gas-Liquid-Solid catalytic reactors. Chem. Eng. Sci. 2021, 233, 116378. [Google Scholar] [CrossRef]
- Deising, D.; Marschall, H.; Bothe, D. A unified single-field model framework for Volume-Of-Fluid simulations of interfacial species transfer applied to bubbly flows. Chem. Eng. Sci. 2016, 139, 173–195. [Google Scholar] [CrossRef]
- Haroun, Y.; Legendre, D.; Raynal, L. Volume of fluid method for interfacial reactive mass transfer: Application to stable liquid film. Chem. Eng. Sci. 2010, 65, 2896–2909. [Google Scholar] [CrossRef]
- Woo, M.; Tischer, S.; Deutschmann, O.; Wörner, M. A step toward the numerical simulation of catalytic hydrogenation of nitrobenzene in Taylor flow at practical conditions. Chem. Eng. Sci. 2021, 230, 116132. [Google Scholar] [CrossRef]
- Li, H.; Yu, X.; Song, Y.; Li, Q.; Lu, S. Experimental and numerical investigation on optimization of foaming performance of the kenics static mixer in compressed air foam system. Eng. Appl. Comput. Fluid Mech. 2023, 17, 2183260. [Google Scholar] [CrossRef]
- Fournier, M.-C.; Falk, L.; Villermaux, J. A new parallel competing reaction system for assessing micromixing effciency-determination of micromixing time by a simple model. Chem. Eng. Sci. 1996, 51, 5187–5192. [Google Scholar] [CrossRef]
- Xie, R.; Li, J.; Jin, Y.; Zou, D.; Chen, M. Simulation of drop breakage in liquid–liquid system by coupling of CFD and PBM: Comparison of breakage kernels and effects of agitator configurations. Chin. J. Chem. Eng. 2019, 27, 1001–1014. [Google Scholar] [CrossRef]
- Wang, X.; Guo, W.; Wu, J. Experimental and numerical study on liquid liquiddispersion in static mixer. CIESC J. 2012, 63, 767–774. [Google Scholar]
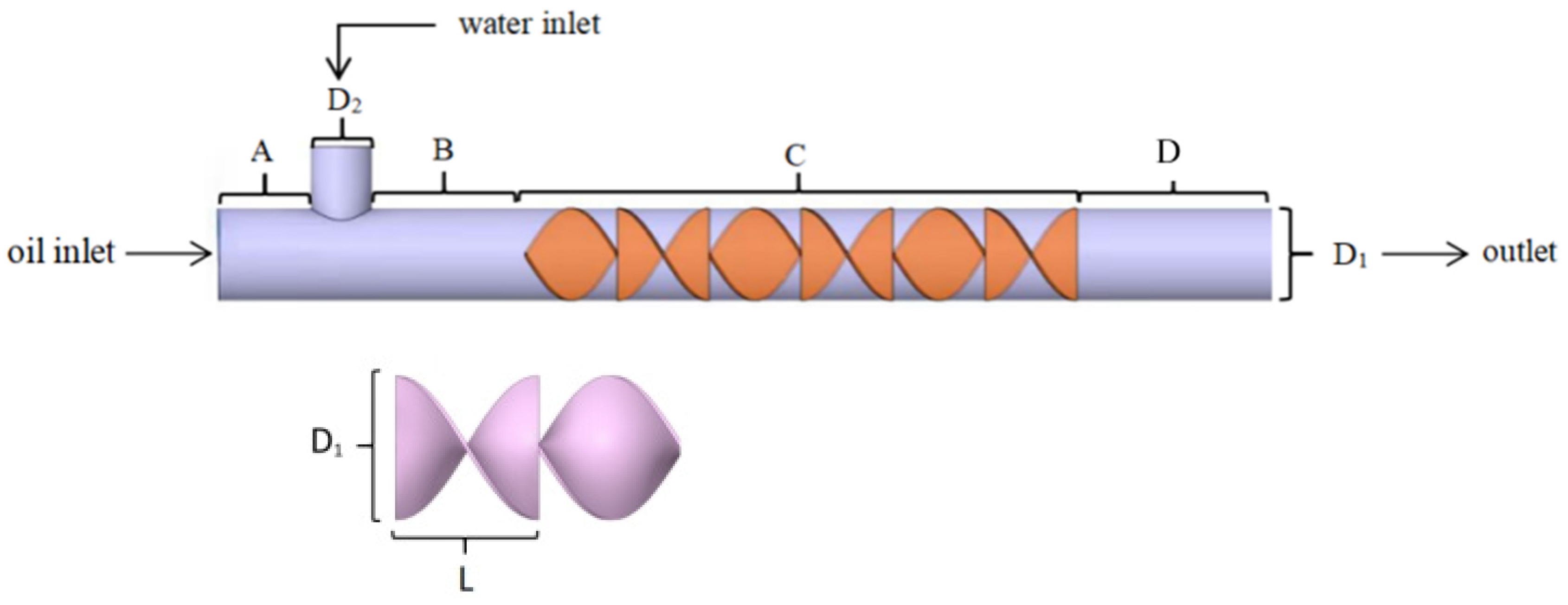







| Parameters | Value |
|---|---|
| Length of Zone A | 15 mm |
| Length of Zone B | 30 mm |
| Length of Zone D | 30 mm |
| Diameter of crude oil inlet D1 | 15 mm |
| Diameter of solvation inlet D2 | 10 mm |
| Properties | Crude Oil | Sodium Hypochlorite Solution |
|---|---|---|
| Density (293 K) | 810 kg/m3 | 998.2 kg/m3 |
| Viscosity (293 K) | 0.01 kg/(m·s) | 0.001 kg/(m·s) |
| Concentration of H2S | 100 mg/kg | 0 mg/kg |
| Concentration of NaClO | 0 g/kg | 10 g/kg |
| Oil–water interfacial tension | 0.01 N/m | |
| Oil-to- Water Ratios | Oil Phase H2S Content at Inlet | Oil Phase H2S Content at Outlet (Experiment) | Oil Phase H2S Content at Outlet (Simulation) | Error (%) |
|---|---|---|---|---|
| 25:1 | 15.48 | 5.29 | 6.25 | 18.22 |
| 30:1 | 15.46 | 5.86 | 6.99 | 19.23 |
| 35:1 | 16.10 | 7.21 | 7.52 | 4.29 |
| 40:1 | 16.95 | 9.19 | 9.11 | 0.84 |
| 45:1 | 25.40 | 18.52 | 14.89 | 19.60 |
| No. | Water Velocity (m/s) | Toluene Velocity (m/s) | Experimental Results (mm) | Simulation Results (mm) | Error (%) |
|---|---|---|---|---|---|
| 2 | 0.27 | 0.21 | 1.99 | 2.08 | 4.42 |
| 5 | 0.30 | 0.71 | 1.69 | 1.85 | 9.42 |
| 7 | 0.15 | 0.36 | 3.1 | 3.48 | 1.21 |
| 10 | 0.18 | 0.85 | 2.35 | 2.27 | 3.32 |
| Code | Factors | Levels | ||
|---|---|---|---|---|
| A | Number of elements | 10 | 14 | 18 |
| B | Aspect ratio | 1 | 2 | 3 |
| C | Twist angle (°) | 45 | 60 | 90 |
| No. | A | B | C | HSRE |
|---|---|---|---|---|
| 1 | 10 | 1 | 60 | 0.4462 |
| 2 | 18 | 1 | 60 | 0.6504 |
| 3 | 10 | 3 | 60 | 0.3077 |
| 4 | 18 | 3 | 60 | 0.4674 |
| 5 | 10 | 2 | 45 | 0.3811 |
| 6 | 18 | 2 | 45 | 0.5062 |
| 7 | 10 | 2 | 90 | 0.4012 |
| 8 | 18 | 2 | 90 | 0.5161 |
| 9 | 14 | 1 | 45 | 0.6141 |
| 10 | 14 | 3 | 45 | 0.4504 |
| 11 | 14 | 1 | 90 | 0.6511 |
| 12 | 14 | 3 | 90 | 0.4711 |
| 13 | 14 | 2 | 60 | 0.5321 |
| Source | Sum of Squares (SS) | Degrees of Freedom (df) | Mean Square (MS) | F | p | Significance |
|---|---|---|---|---|---|---|
| Model | 1252 | 9 | 139.2 | 22.58 | 0.01321 | Yes |
| A | 419.2 | 1 | 419.2 | 68.03 | 0.003732 | Yes |
| B | 535.5 | 1 | 535.5 | 86.90 | 0.002614 | Yes |
| C | 9.614 | 1 | 9.614 | 1.560 | 0.3002 | No |
| AB | 4.951 | 1 | 4.951 | 0.8034 | 0.4361 | No |
| AC | 2.254 | 1 | 2.254 | 0.3658 | 0.5880 | No |
| BC | 0.9474 | 1 | 0.9474 | 0.1537 | 0.7212 | No |
| A2 | 145.7 | 1 | 145.7 | 23.65 | 0.01661 | Yes |
| B2 | 5.616 | 1 | 5.616 | 0.9114 | 0.4102 | No |
| C2 | 0.5007 | 1 | 0.5007 | 0.08126 | 0.7942 | No |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, M.; Liu, J.; Chen, Y.; Huang, Z.; Wang, H.; Yuan, P.; Xu, X.; Yang, J. Intensification of Multiphase Reactions in Petroleum Processing: A Simulation Study of SK Static Mixer Using NaClO for H2S Removal. Processes 2025, 13, 1515. https://doi.org/10.3390/pr13051515
Gao M, Liu J, Chen Y, Huang Z, Wang H, Yuan P, Xu X, Yang J. Intensification of Multiphase Reactions in Petroleum Processing: A Simulation Study of SK Static Mixer Using NaClO for H2S Removal. Processes. 2025; 13(5):1515. https://doi.org/10.3390/pr13051515
Chicago/Turabian StyleGao, Mengmeng, Jiacheng Liu, Ying Chen, Zibin Huang, Hongfu Wang, Peiqing Yuan, Xinru Xu, and Jingyi Yang. 2025. "Intensification of Multiphase Reactions in Petroleum Processing: A Simulation Study of SK Static Mixer Using NaClO for H2S Removal" Processes 13, no. 5: 1515. https://doi.org/10.3390/pr13051515
APA StyleGao, M., Liu, J., Chen, Y., Huang, Z., Wang, H., Yuan, P., Xu, X., & Yang, J. (2025). Intensification of Multiphase Reactions in Petroleum Processing: A Simulation Study of SK Static Mixer Using NaClO for H2S Removal. Processes, 13(5), 1515. https://doi.org/10.3390/pr13051515






