Study of the Macro-Mesoscopic Shear Anisotropic Mechanical Behavior of Reservoir Shale
Abstract
1. Introduction
2. Materials and Methods
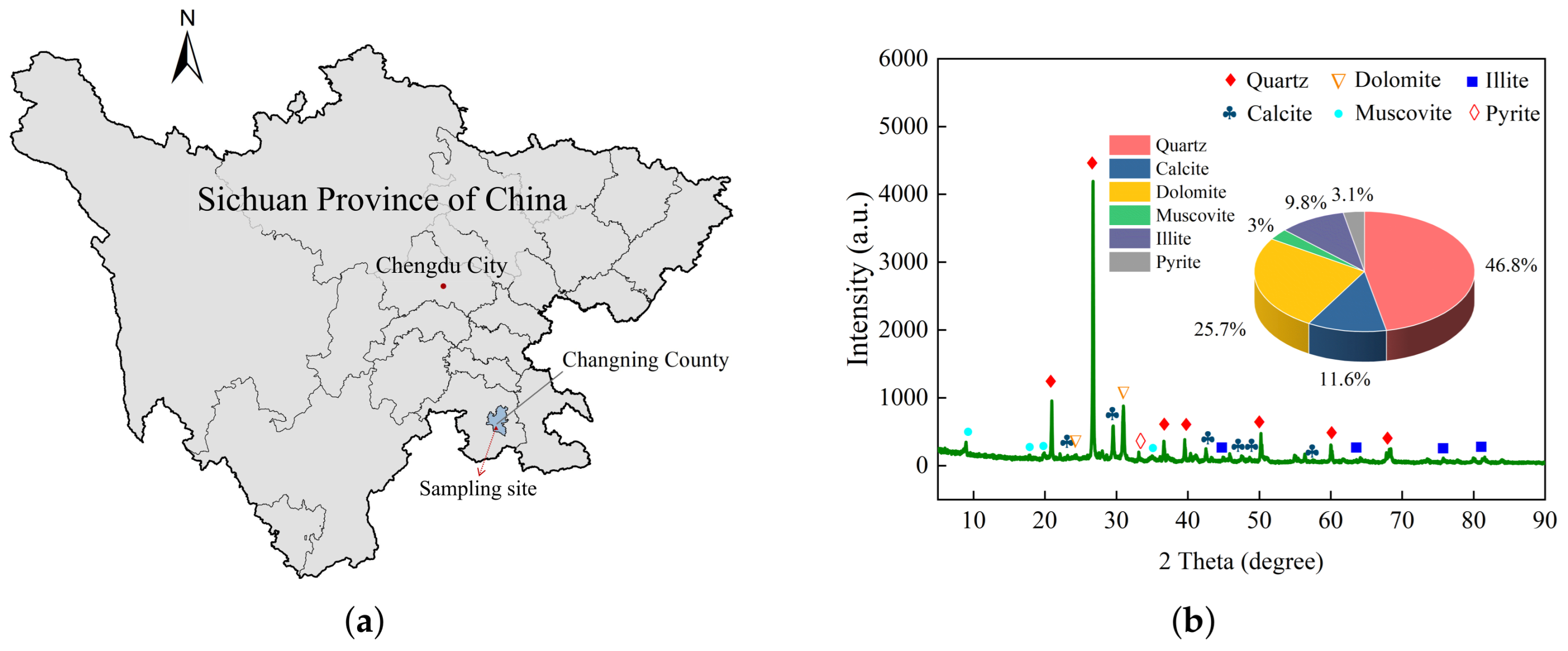
2.1. Microscopic Experimental Methods
2.2. Macroscopic and Mesoscopic Experimental Methods
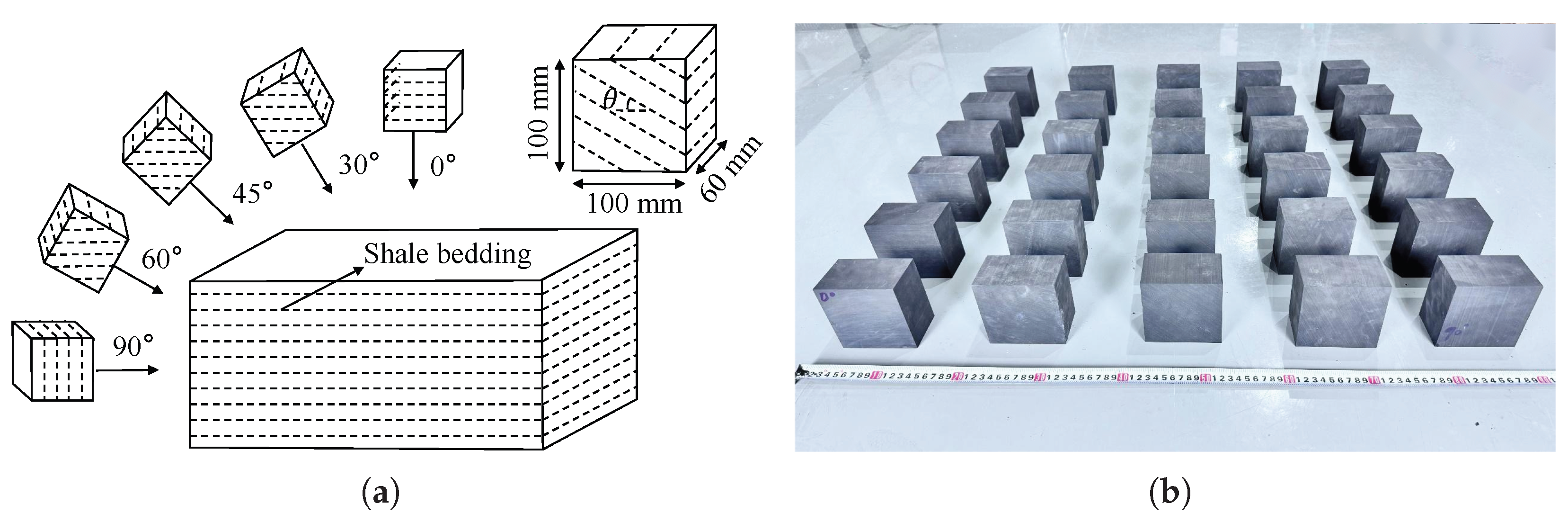
2.2.1. Macroscale Direct Shear Test Method
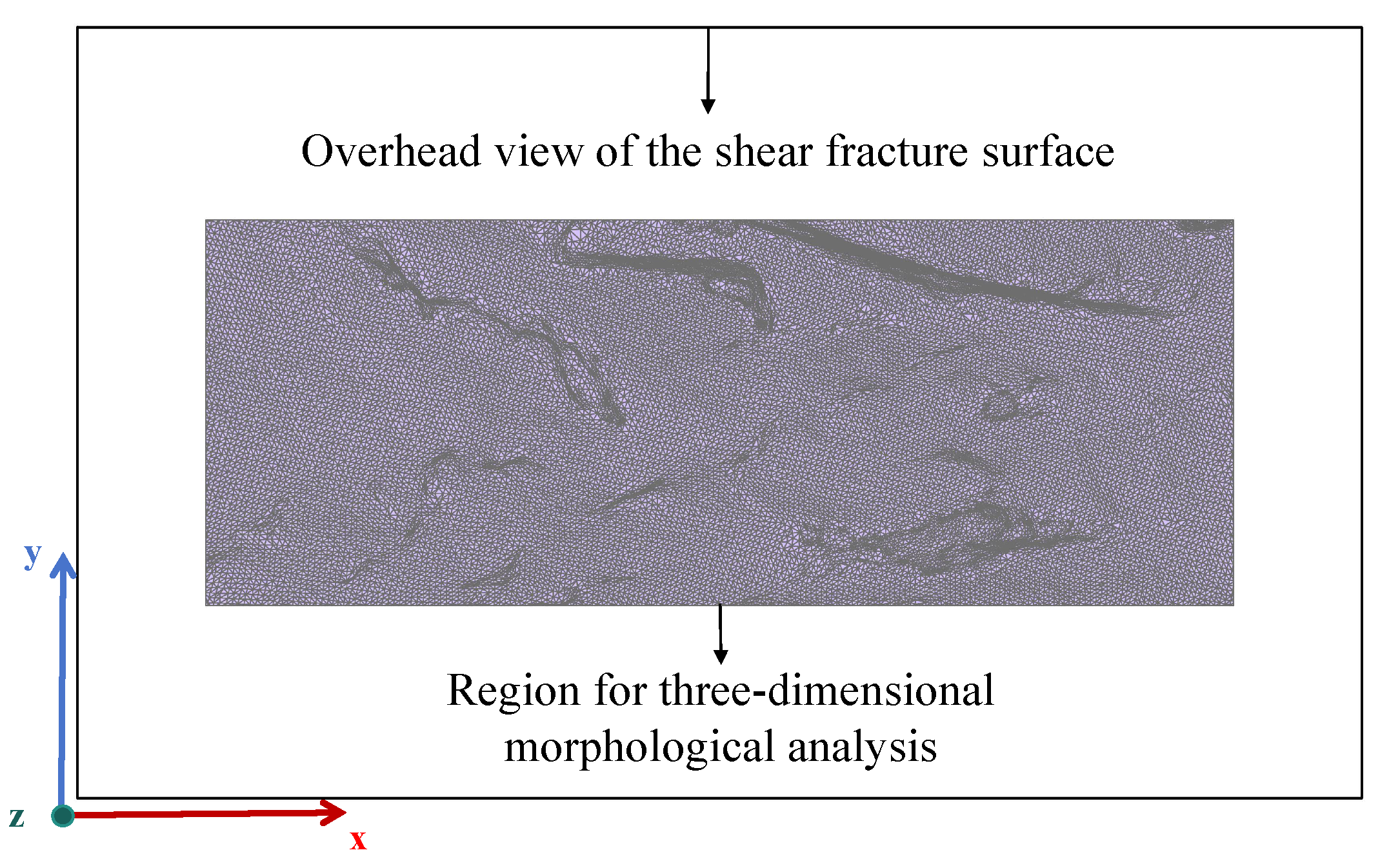
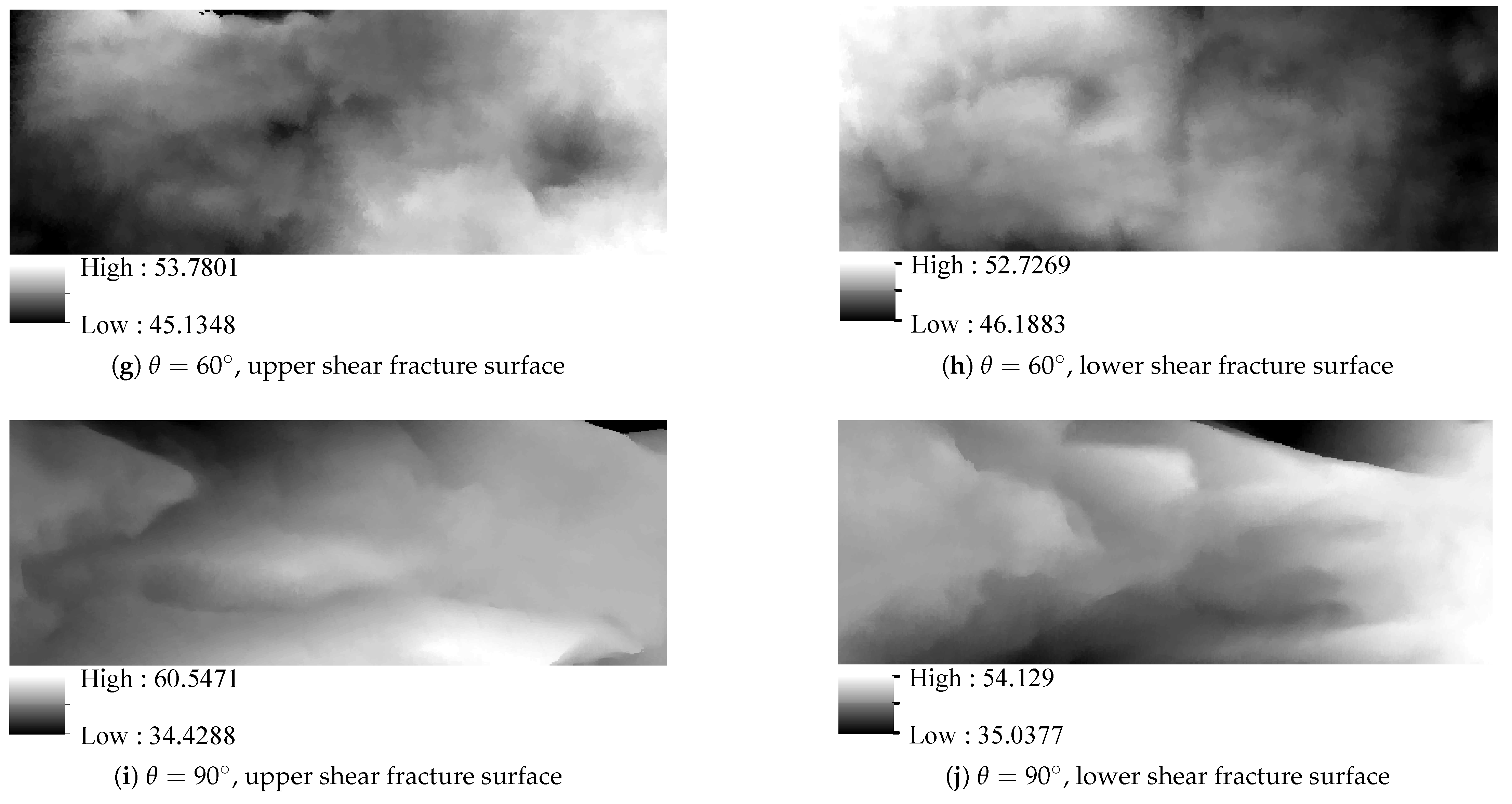
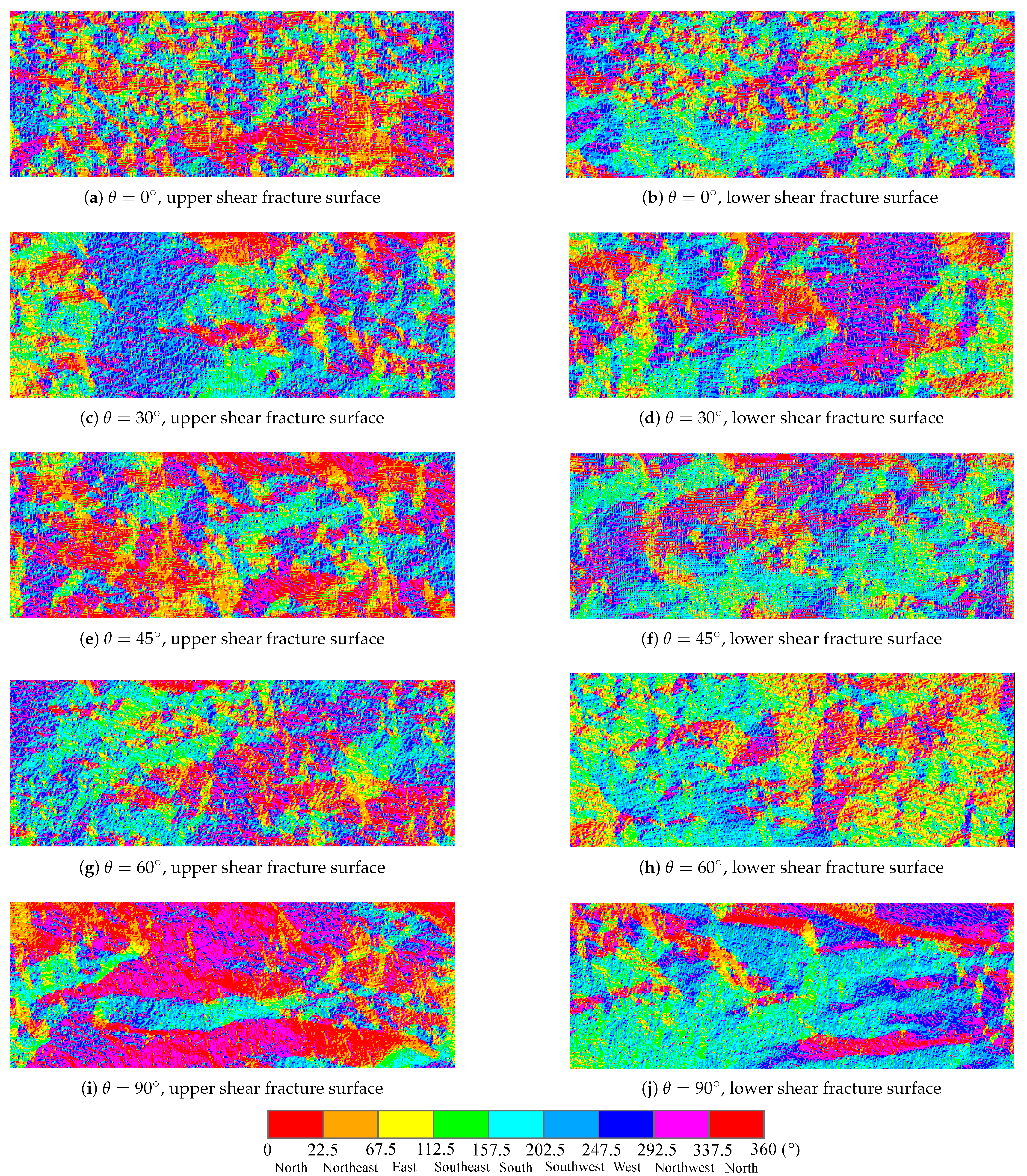
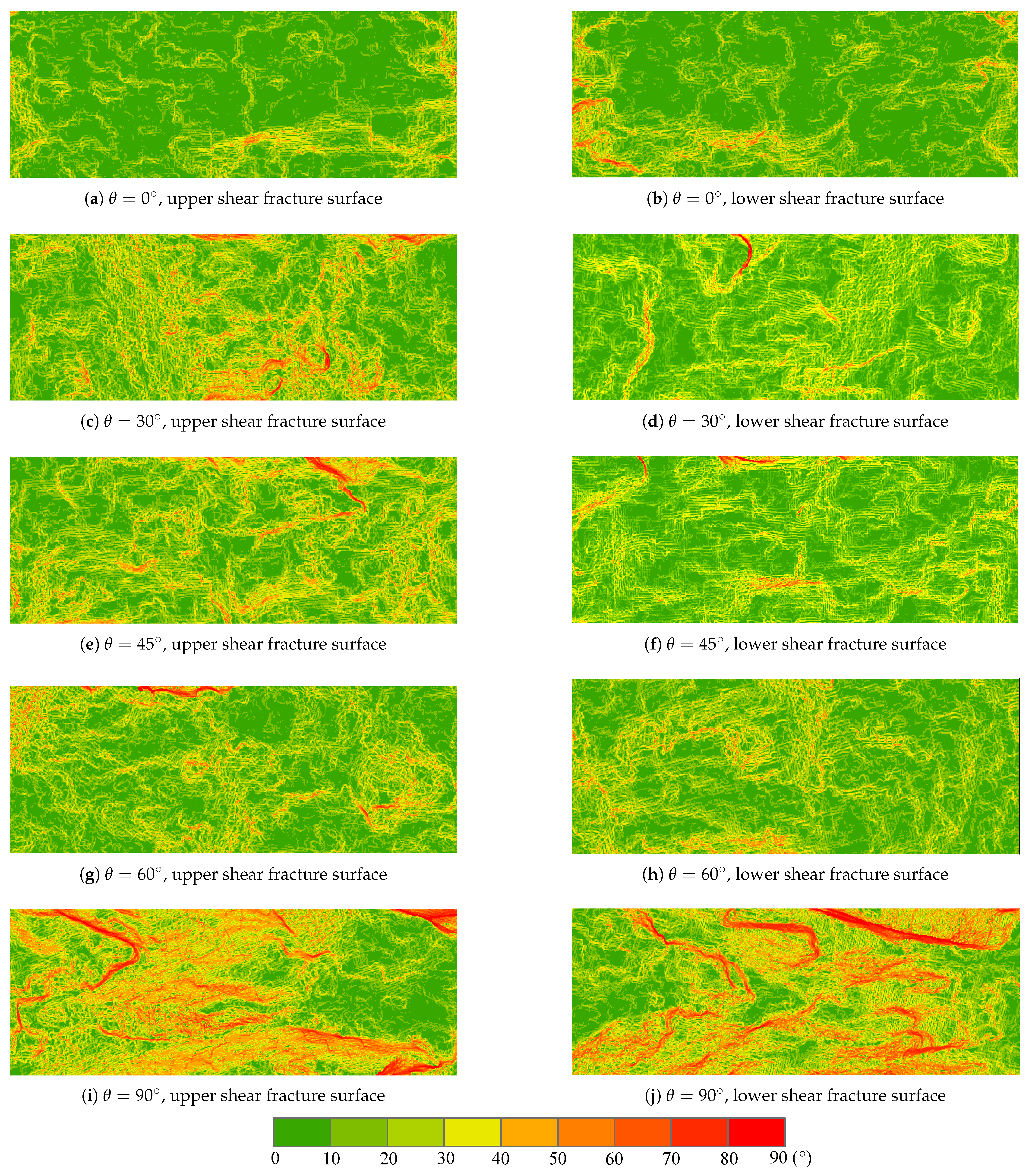
2.2.2. Quantitative Characterization Method of Shear Fracture Surface Morphology
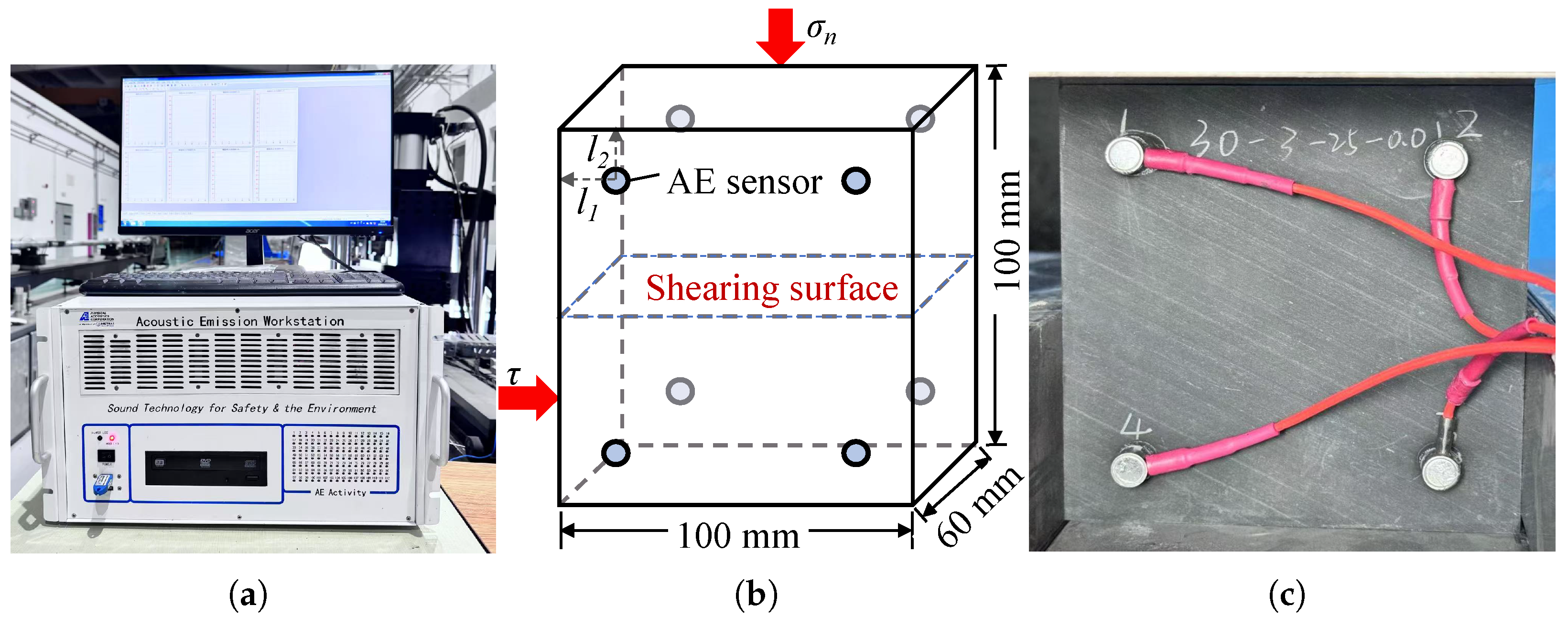
2.2.3. Mesoscale Acoustic Emission Monitoring Method
3. Results
3.1. Microscopic Structure Analysis of Shale
3.2. Macroscopic Shear Anisotropic Mechanical Properties
3.2.1. Shear Stress–Shear Strain Curve
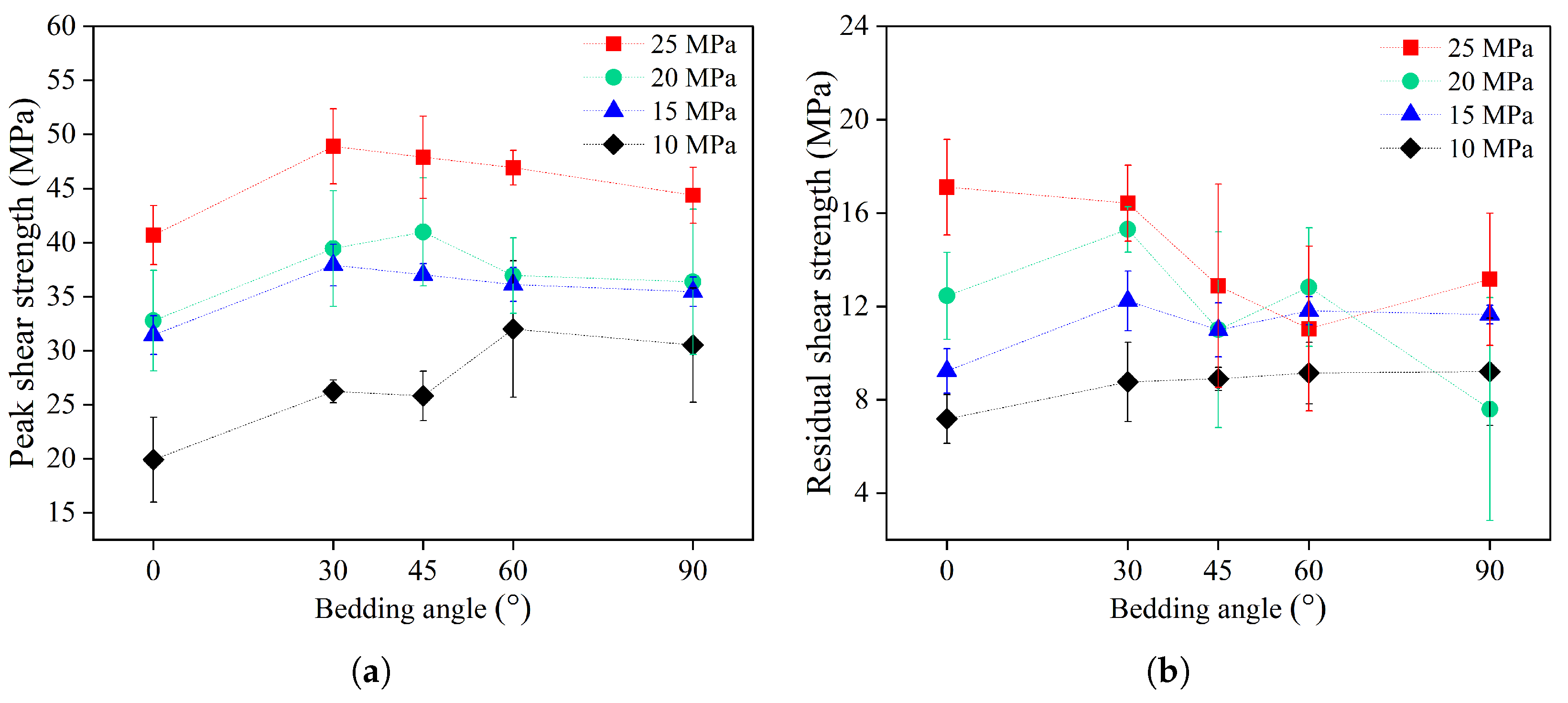
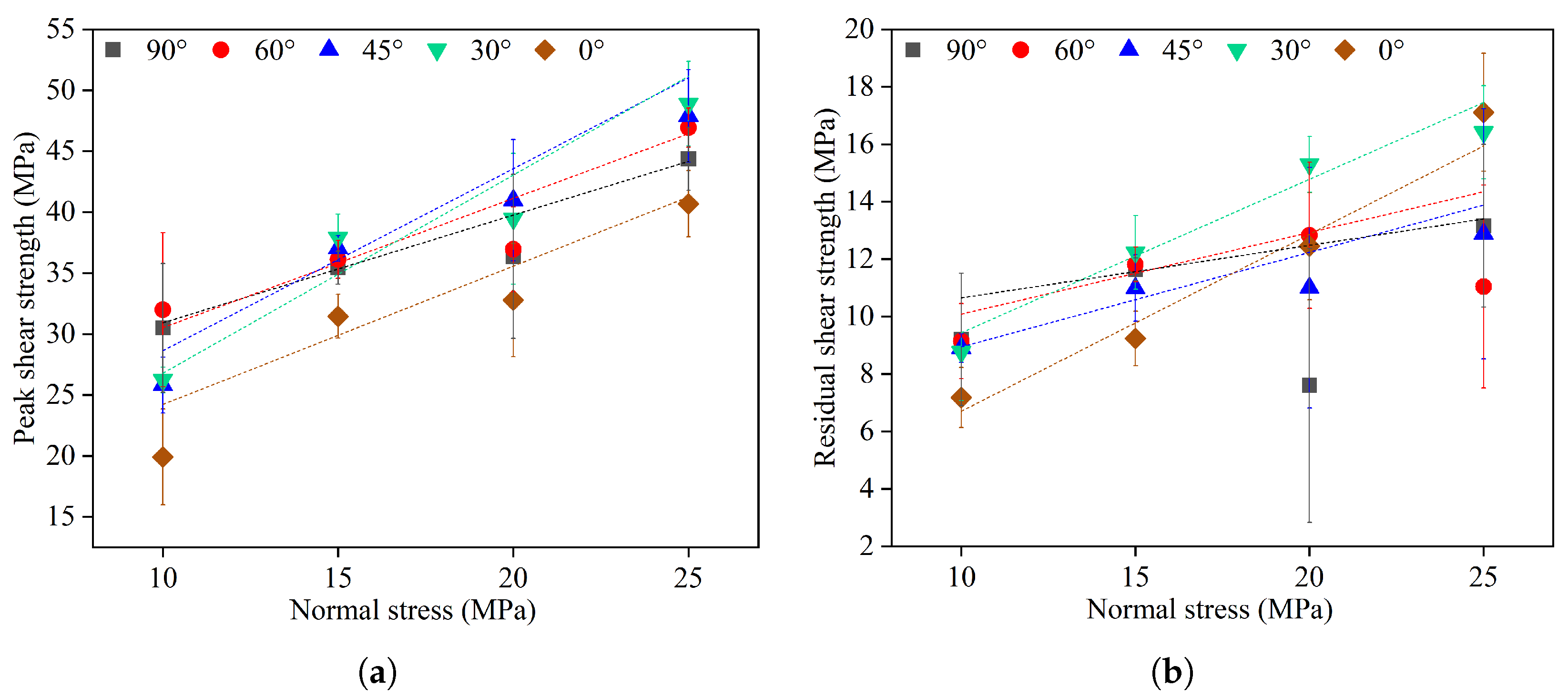
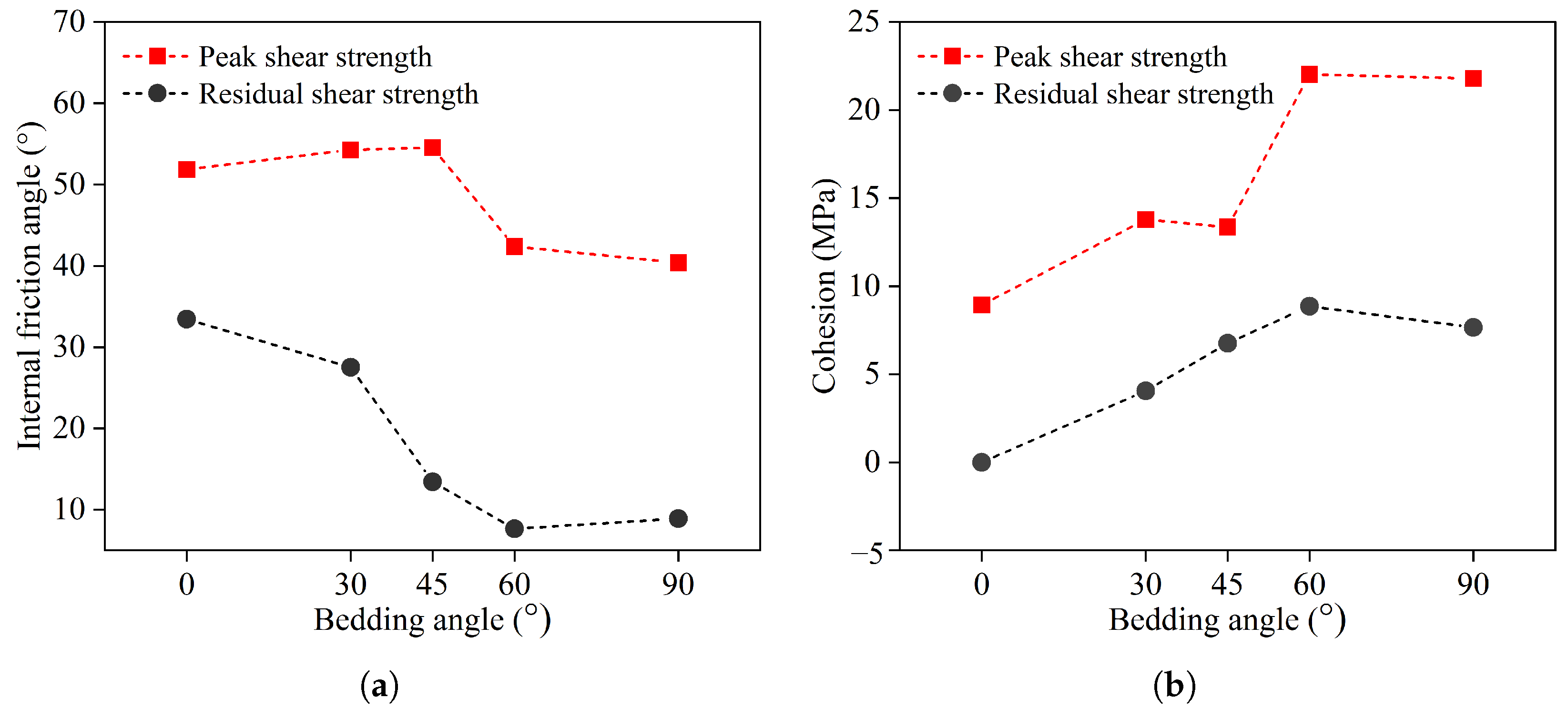
3.2.2. Macroscopic Shear Strength Parameters
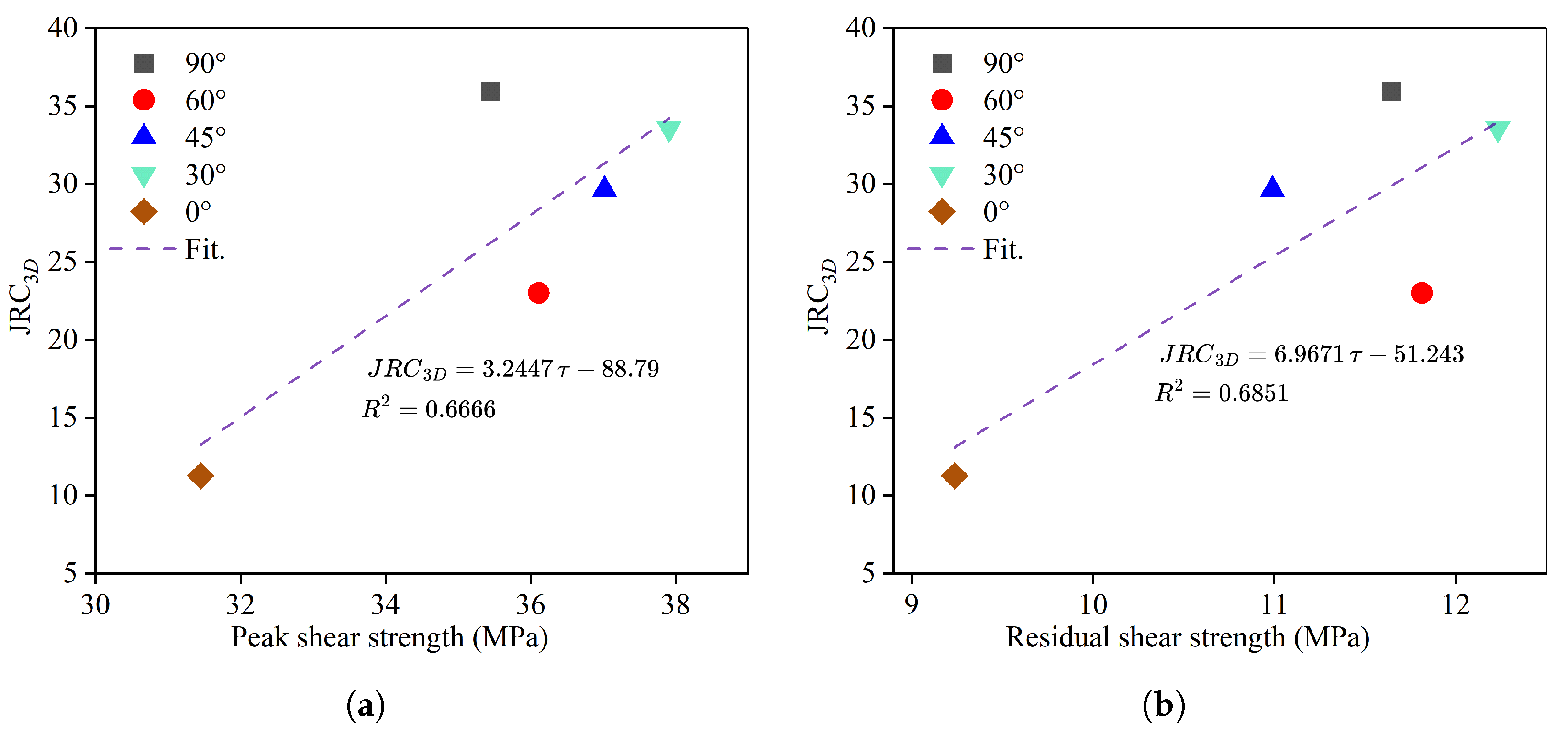
3.3. Shear Failure Patterns and Shear Fracture Surface Morphology
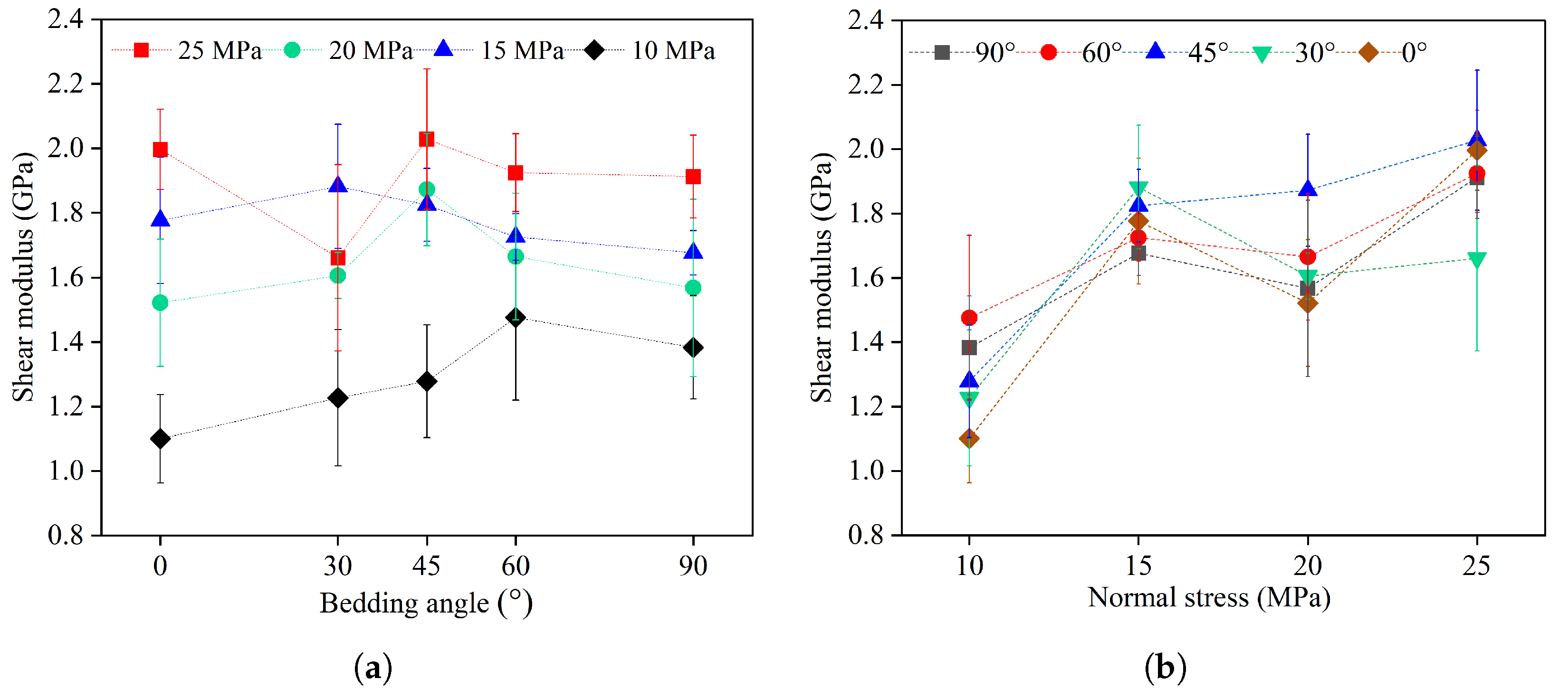
3.4. Mesoscopic Shear Anisotropic Mechanical Properties
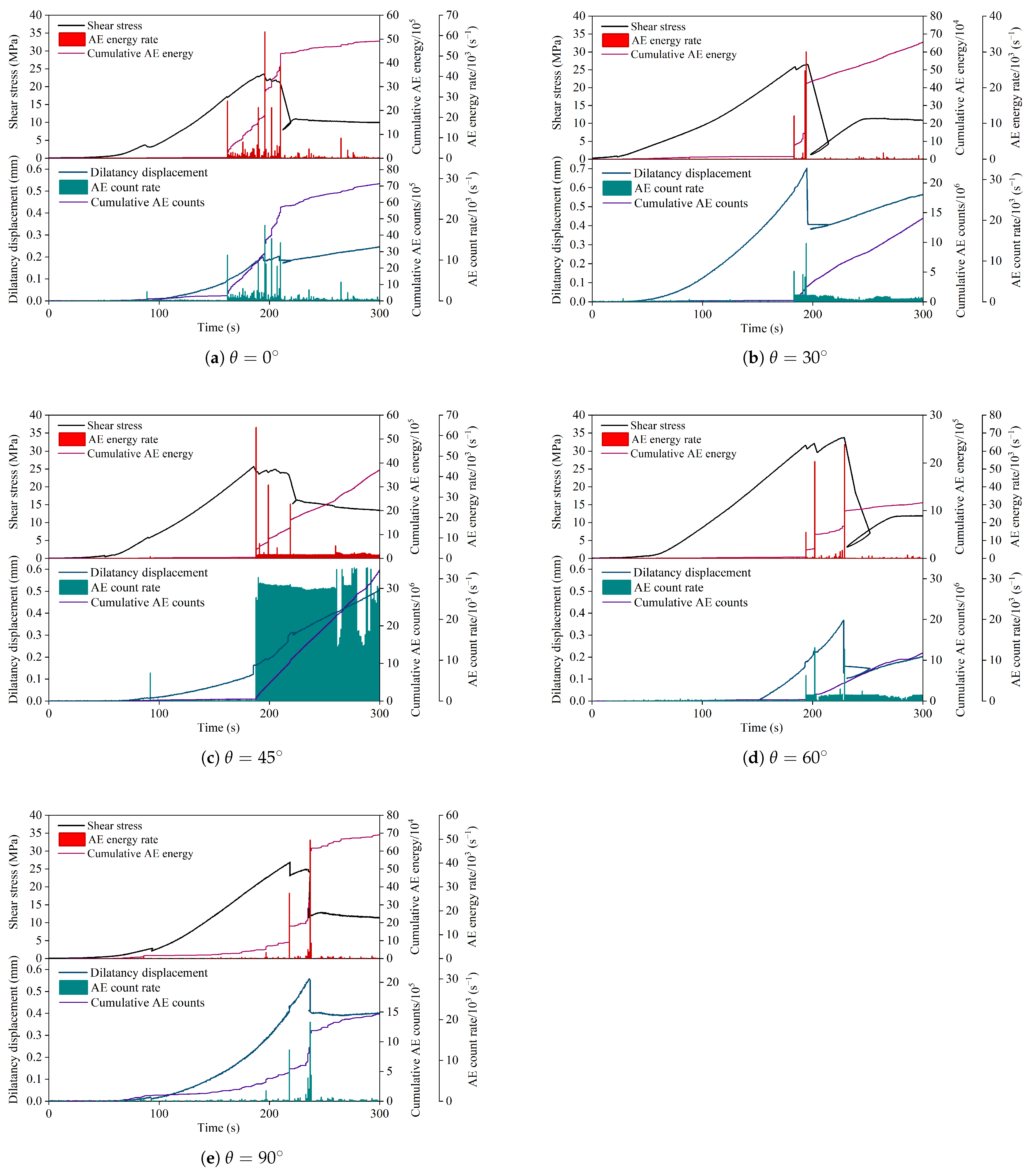
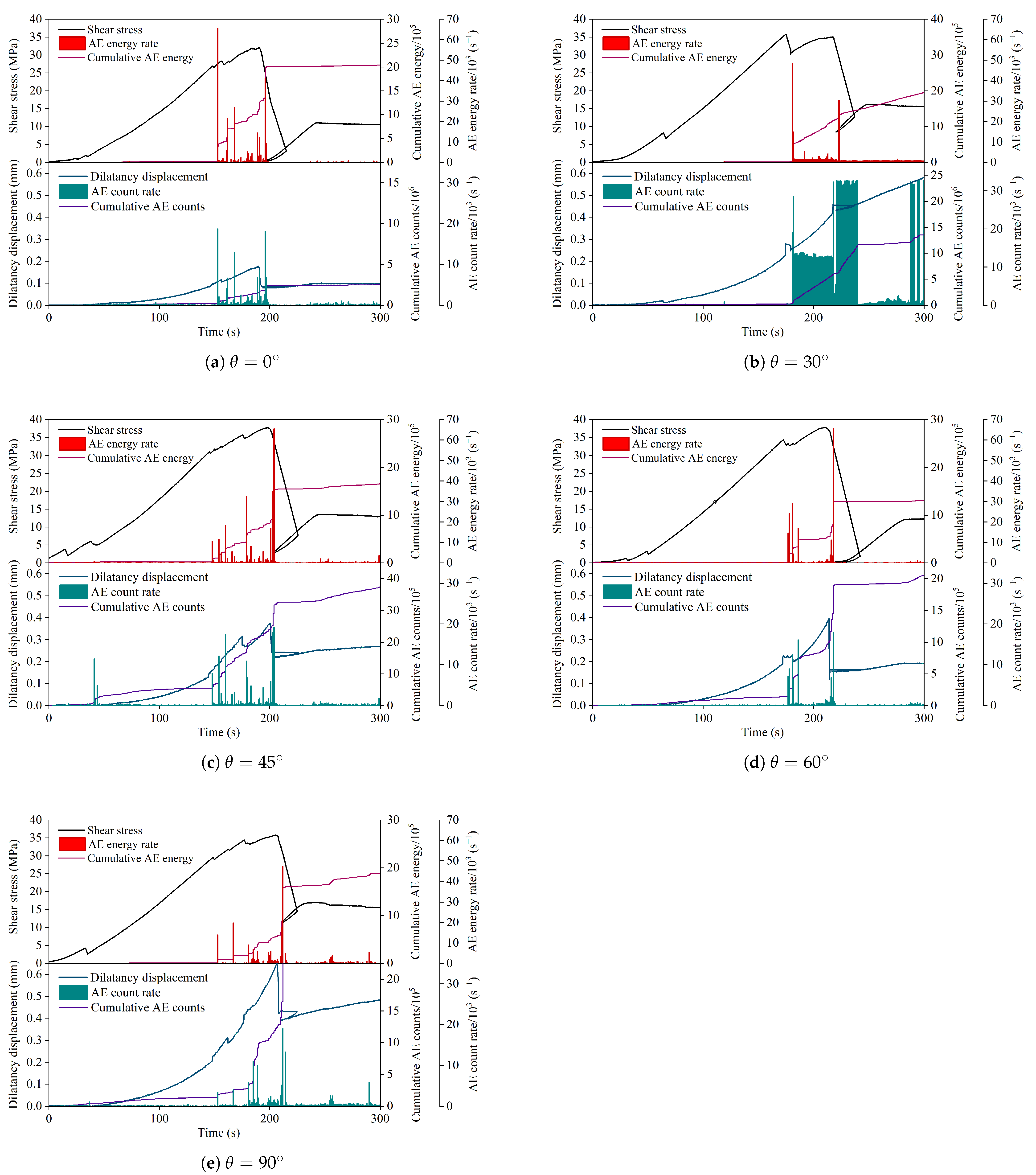
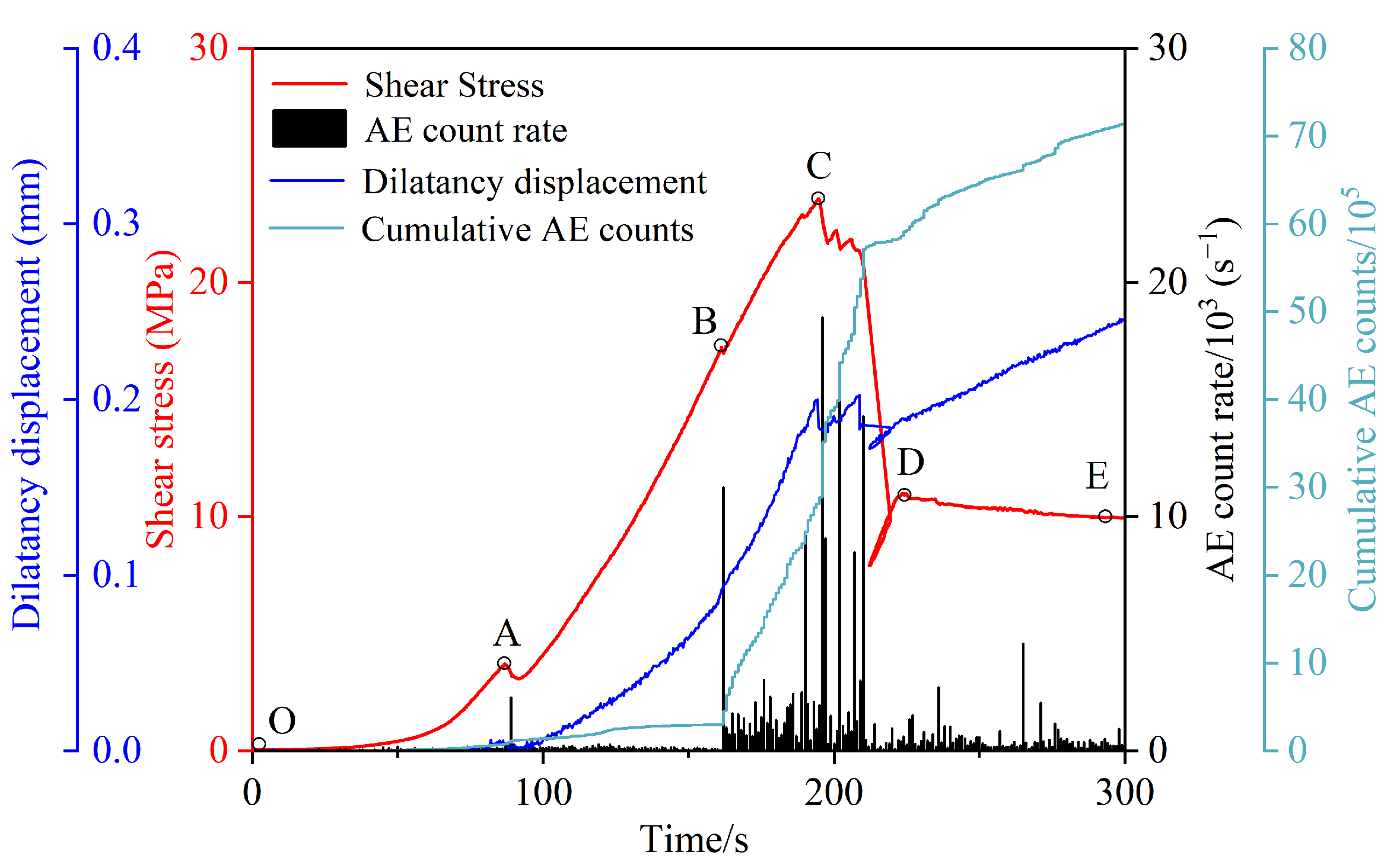
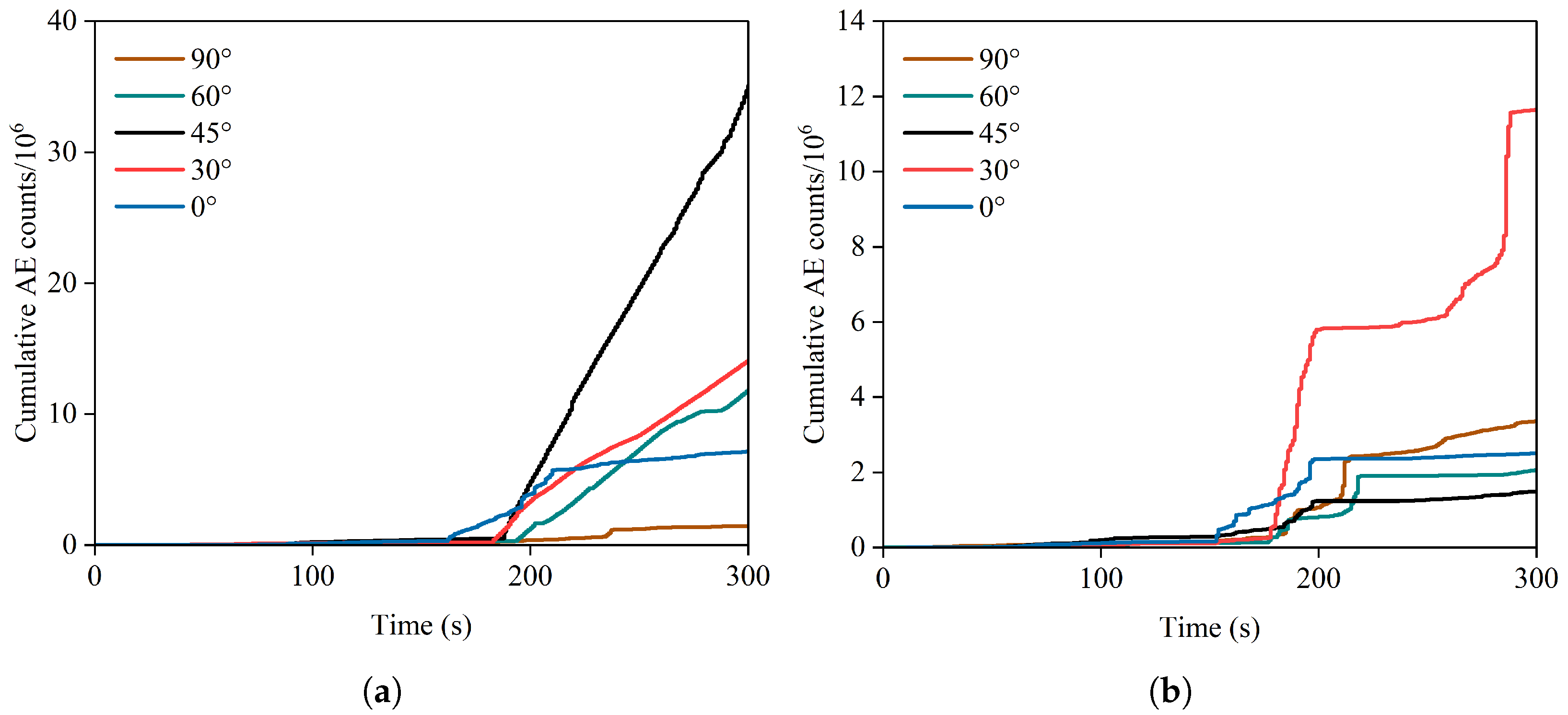
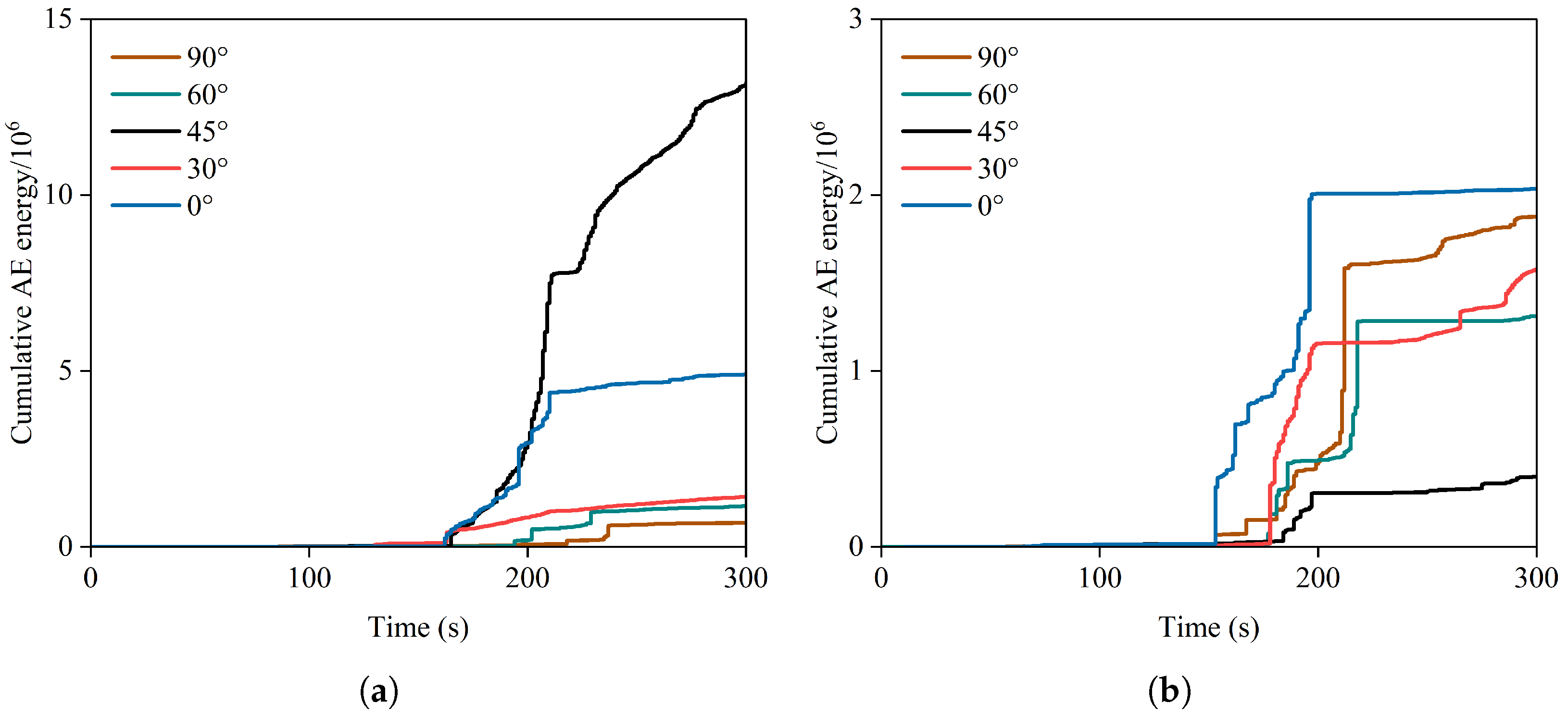
3.4.1. Mesoscopic Characterization of the Progressive Shear Failure Process
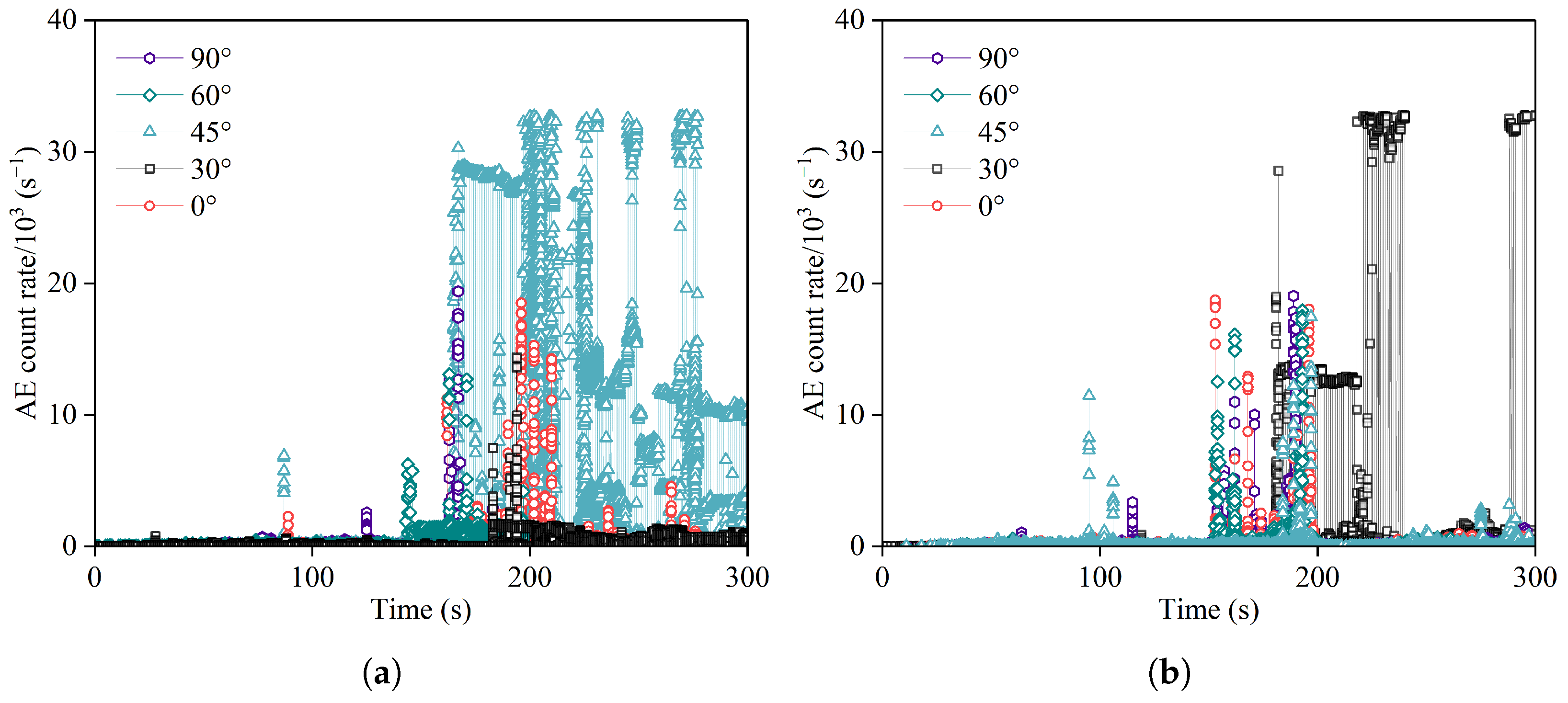
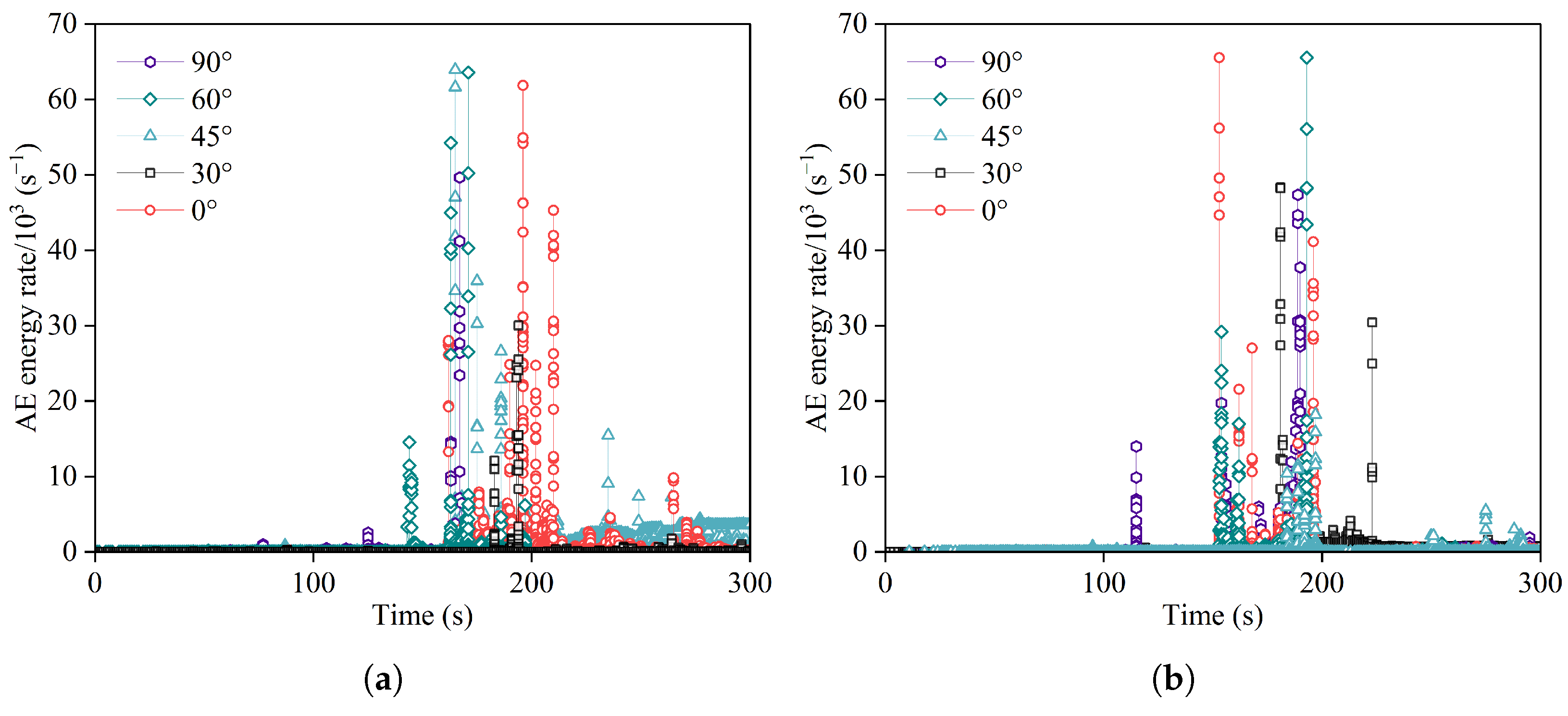
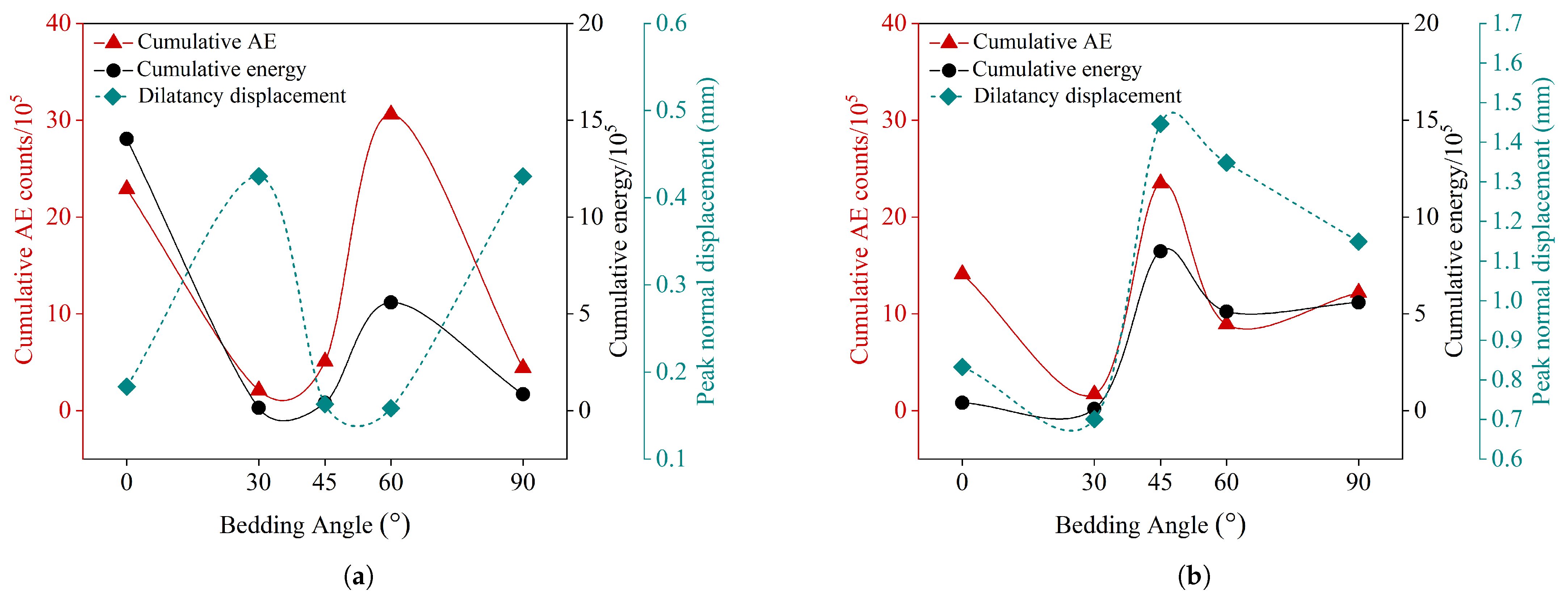
3.4.2. Stress and Bedding Effects on Acoustic Emission Parameters During Progressive Shear Failure
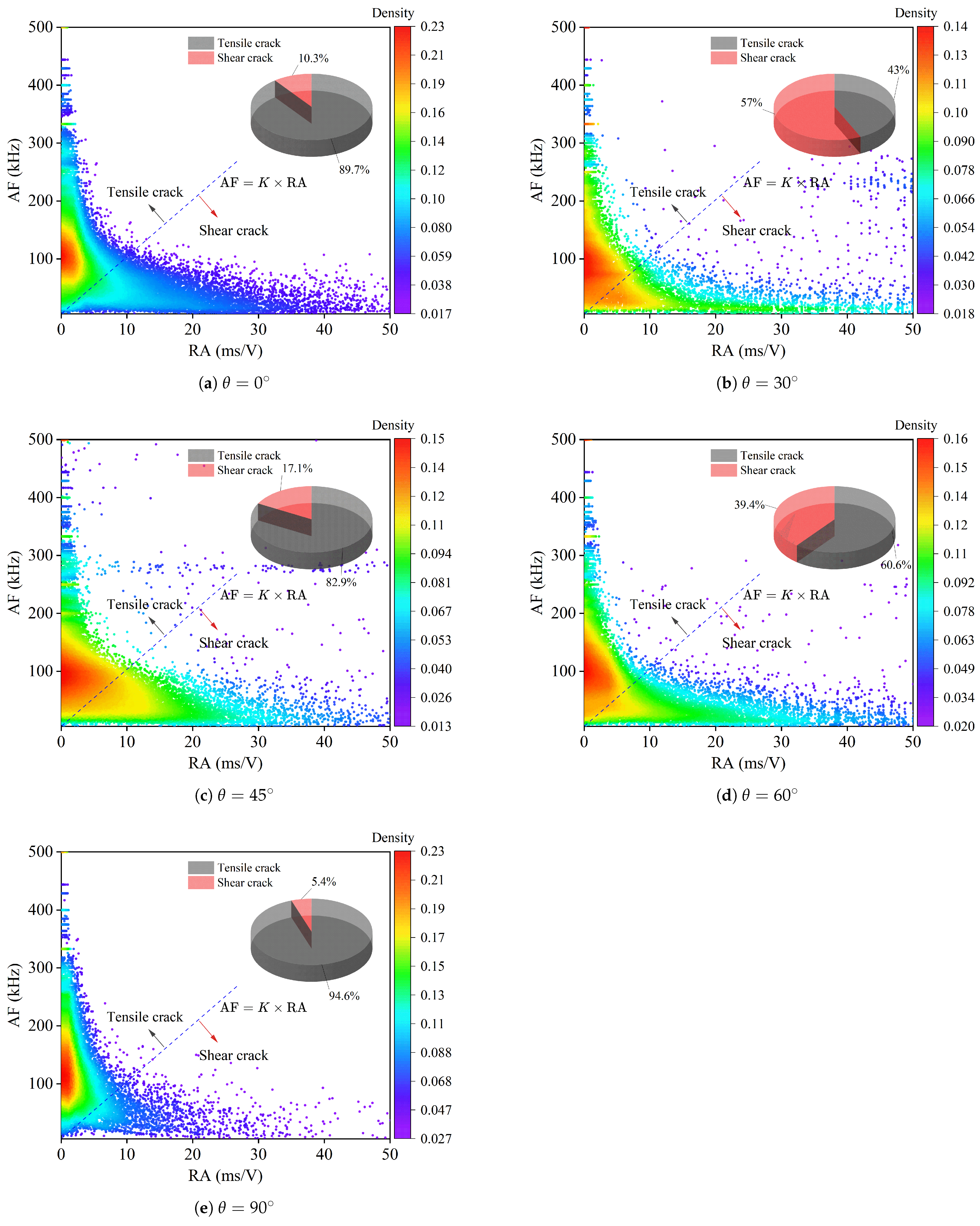
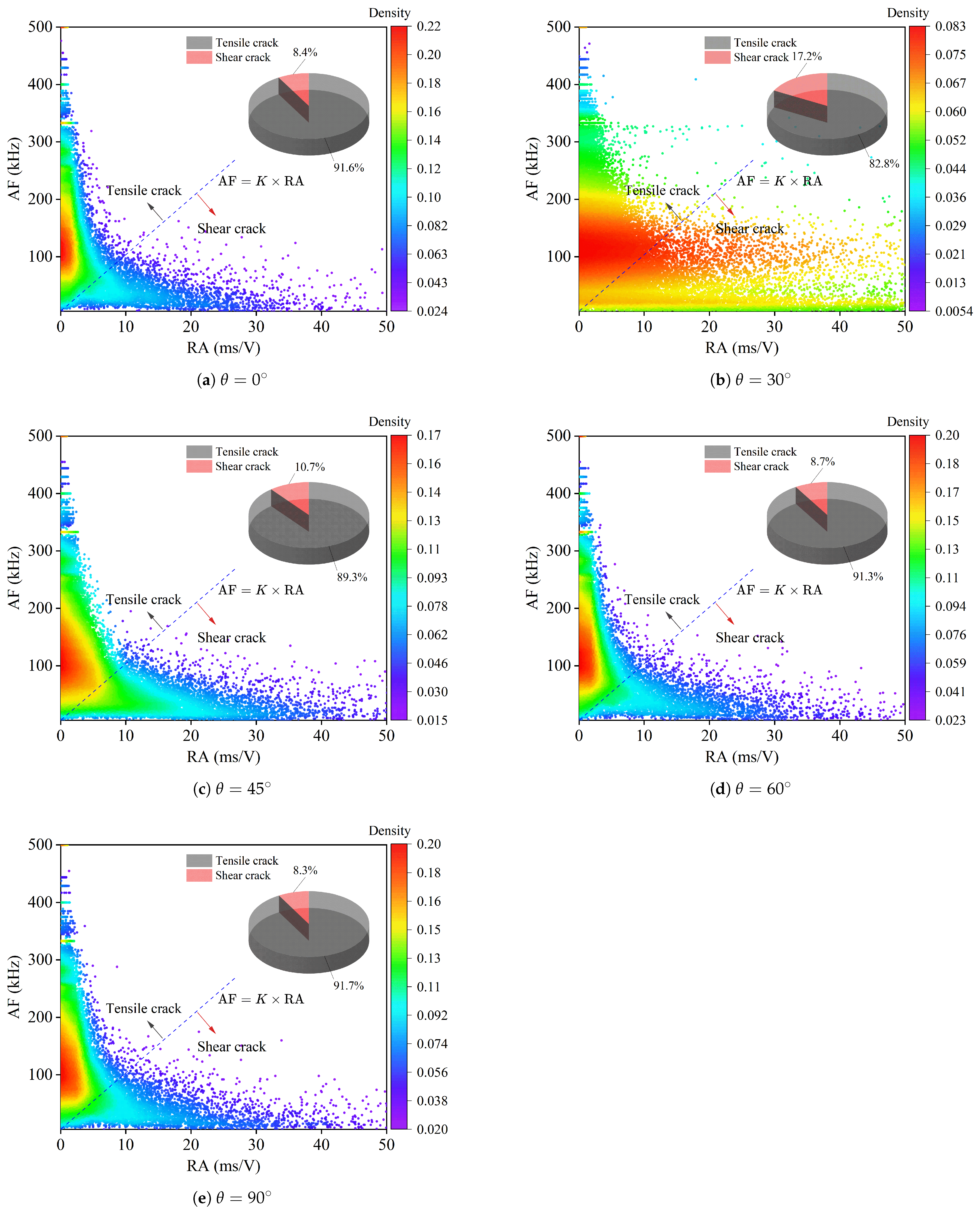
3.4.3. Stress and Bedding Effects on the Evolution of Tensile and Shear Fractures
4. Discussion
4.1. Anisotropy of Macroscopic Shear Parameters
4.2. Anisotropy of Mesoscopic Shear Parameters
4.3. Engineering Implications and Prospects
5. Conclusions
- (1)
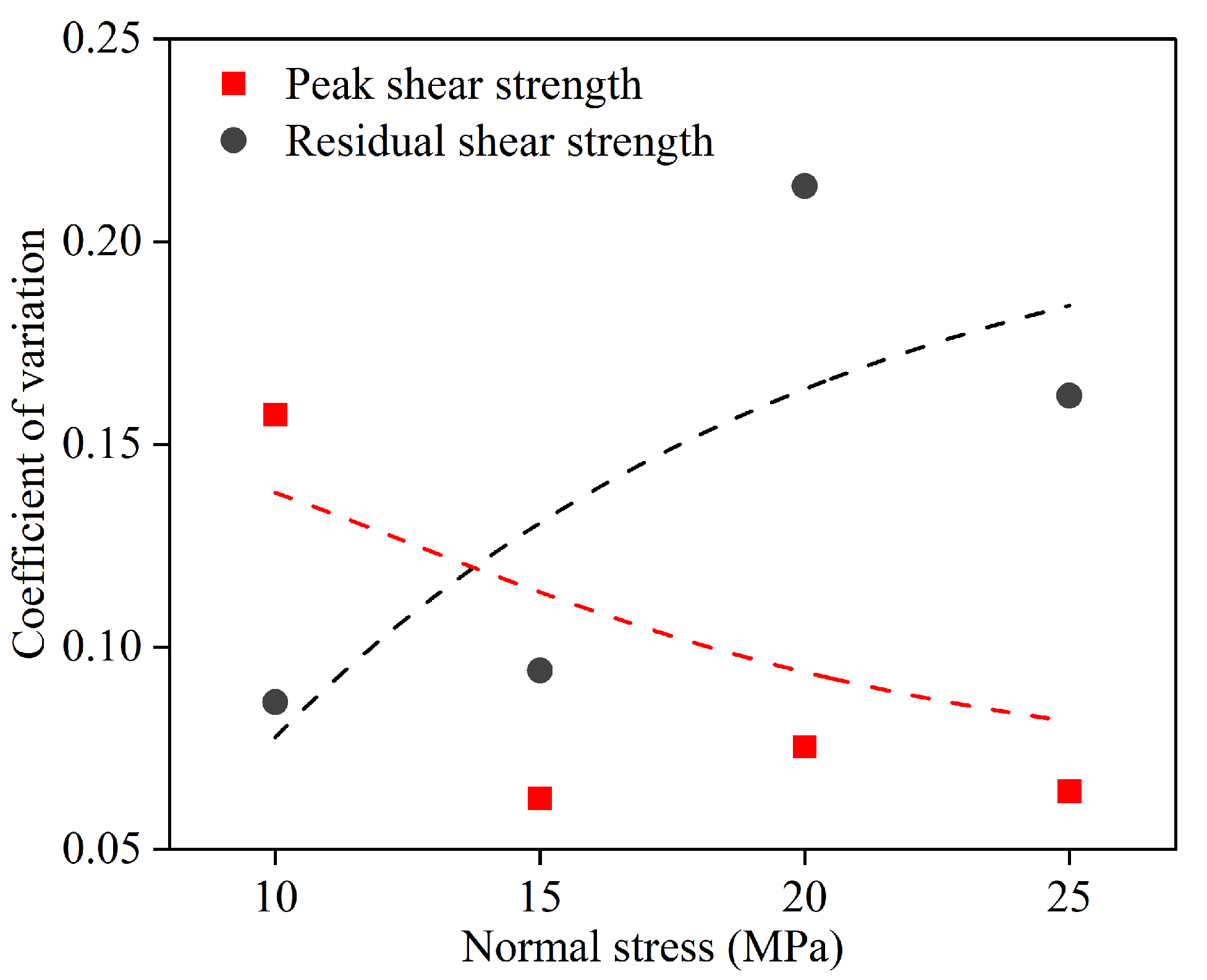
- The peak and residual shear strengths of shale at various bedding angles exhibit a fluctuating increasing trend with increasing normal stress. Under all normal stress conditions, shale with bedding angles of to generally shows higher peak shear strength. When the normal stress is low, the anisotropy of peak shear strength is more pronounced than that of residual shear strength. As the normal stress increases, the bedding-induced anisotropy of peak and residual shear strengths exhibits opposite trends. The anisotropy of peak shear strength tends to decrease in a fluctuating manner, while that of residual shear strength increases with fluctuations.
- (2)
- Both the peak and residual internal friction angles of shale bedding planes are greater than those of the shale matrix, whereas the cohesion values of the bedding planes are lower than those of the matrix. The peak internal friction angle reaches its maximum at a bedding angle of , while the peak cohesion attains its maximum at a bedding angle of . As the bedding angle increases from to , the bedding effect on the anisotropy of peak shear strength parameters (internal friction angle and cohesion) shows a staged evolution characterized by “plateau and step-like” transitions. Moreover, the bedding-induced anisotropy of the internal friction angle and cohesion for peak and residual shear strength exhibits opposite trends.
- (3)
- At lower normal stresses, the cumulative AE ringing count curves for shale shear failure follow an “S”-shaped pattern for bedded and matrix shear, differing from the piecewise linear pattern observed in bedded-matrix coupled shear. Under such conditions, macro- and mesoscale damage evolution is inconsistent but becomes increasingly aligned as normal stress increases, indicating a transition of the shear failure mechanism from non-coordinated to coordinated. Combined with acoustic emission (AE) monitoring, the microcrack evolution during shear can be evaluated in real-time, providing technical support for fracture mechanism analysis and fracture-type regulation, thereby enhancing the safety and controllability of shale gas extraction and geological sequestration projects.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Sun, C.; Nie, H.; Dang, W.; Chen, Q.; Zhang, G.; Li, W.; Lu, Z. Shale Gas Exploration and Development in China: Current Status, Geological Challenges, and Future Directions. Energy Fuels 2021, 35, 6359–6379. [Google Scholar] [CrossRef]
- Josh, M.; Esteban, L.; Delle Piane, C.; Sarout, J.; Dewhurst, D.; Clennell, M. Laboratory characterisation of shale properties. J. Pet. Sci. Eng. 2012, 88–89, 107–124. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, Y.; Zou, R.; Zou, R.; Huang, L.; Liu, Y.; Meng, Z.; Wang, Z.; Lei, H. A systematic review of CO2 injection for enhanced oil recovery and carbon storage in shale reservoirs. Int. J. Hydrogen Energy 2023, 48, 37134–37165. [Google Scholar] [CrossRef]
- Liang, X.; Zhang, J.; Zhang, H.; Xu, Z.; Zhang, D.; Zhu, D. Major discovery and high-efficiency development strategy of shallow shale gas: A case study of Taiyang shale gas field. China Pet. Explor. 2021, 26, 21–37. (In Chinese) [Google Scholar]
- Liang, X.; Xu, Z.; Zhang, Z.; Wang, W.; Zhang, J.; Lu, H.; Zhang, L.; Zou, C.; Wang, G.; Mei, J.; et al. Breakthrough of shallow shale gas exploration in Taiyang anticline area and its significance for resource development in Zhaotong, Yunnan Province, China. Pet. Explor. Dev. 2020, 47, 12–29. [Google Scholar] [CrossRef]
- Lu, Y. Accumulation and exploration enlightenment of shallow normal-pressure shale gas in southeastern Sichuan Basin, SW China. Pet. Explor. Dev. 2023, 50, 1308–1319. [Google Scholar] [CrossRef]
- Liu, Y.; Ma, T.; Wu, H.; Chen, P. Investigation on mechanical behaviors of shale cap rock for geological energy storage by linking macroscopic to mesoscopic failures. J. Energy Storage 2020, 29, 101326. [Google Scholar] [CrossRef]
- Zhang, Q.; Yue, X. Comparative Analysis of Shale Reservoir Exploitation Conditions in Southeast Sichuan. Multipurp. Util. Miner. Resour. 2022, 43, 109–119. [Google Scholar] [CrossRef]
- Ajibona, A.; Pandey, R.; Khanal, A.; Zenali, S.M.; Abdelaziz, S.L. Pycnometric evaluation of shale caprocks during underground hydrogen storage in depleted reservoirs. Int. J. Hydrogen Energy 2025, 101, 1312–1328. [Google Scholar] [CrossRef]
- Hou, L.; Elsworth, D.; Wang, J.; Zhou, J.; Zhang, F. Feasibility and prospects of symbiotic storage of CO2 and H2 in shale reservoirs. Renew. Sustain. Energy Rev. 2024, 189, 113878. [Google Scholar] [CrossRef]
- Huang, H.; Dong, M. CO2 containment and storage in organic-rich strata—State of the art and future directions. Int. J. Greenh. Gas Control 2024, 132, 104047. [Google Scholar] [CrossRef]
- Yan, C.; Deng, J.; Cheng, Y.; Li, M.; Feng, Y.; Li, X. Mechanical properties of gas shale during drilling operations. Rock Mech. Rock Eng. 2017, 50, 1753–1765. [Google Scholar] [CrossRef]
- Li, W.; Rezakhani, R.; Jin, C.; Zhou, X.; Cusatis, G. A multiscale framework for the simulation of the anisotropic mechanical behavior of shale. Int. J. Numer. Anal. Methods Geomech. 2017, 41, 1494–1522. [Google Scholar] [CrossRef]
- He, J.; Li, X.; Yin, C.; Zhang, Y.; Lin, C. Propagation and Characterization of the Micro Cracks Induced by Hydraulic Fracturing in Shale. Energy 2020, 191, 116449. [Google Scholar] [CrossRef]
- Sarout, J.; Guéguen, Y. Anisotropy of elastic wave velocities in deformed shales: Part 1—Experimental results. Geophysics 2008, 73, D75–D89. [Google Scholar] [CrossRef]
- Gao, Q.; Tao, J.; Hu, J.; Yu, X.B. Laboratory study on the mechanical behaviors of an anisotropic shale rock. J. Rock Mech. Geotech. Eng. 2015, 7, 213–219. [Google Scholar] [CrossRef]
- Ghazvinian, A.; Vaneghi, R.G.; Hadei, M.; Azinfar, M. Shear behavior of inherently anisotropic rocks. Int. J. Rock Mech. Min. Sci. 2013, 61, 96–110. [Google Scholar] [CrossRef]
- Mashhadiali, N.; Molaei, F. Theoretical and experimental investigation of a shear failure model for anisotropic rocks using direct shear test. Rock Mech. Min. Sci. 2023, 170, 105561. [Google Scholar] [CrossRef]
- Wu, Y.; Li, Y.; Luo, S.; Lu, M.; Zhou, N.; Wang, D.; Zhang, G. Multiscale elastic anisotropy of a shale characterized by cross-scale big data nanoindentation. Int. J. Rock Mech. Min. Sci. 2020, 134, 104458. [Google Scholar] [CrossRef]
- Amann, F.; Button, E.A.; Evans, K.F.; Gischig, V.S.; Blümel, M. Experimental study of the brittle behavior of clay shale in rapid unconfined compression. Rock Mech. Rock Eng. 2011, 44, 415–430. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, L.; Liu, D.; Zuo, J.; Liu, S.; Dong, H. Application of micro-CT and digital volume correlation for investigating the macro-mesoscopic failure mechanism of shale under uniaxial loading: Insights into fracture behavior relevant to shale gas recovery. Bull. Eng. Geol. Environ. 2025, 84, 82. [Google Scholar] [CrossRef]
- Heng, S.; Guo, Y.; Yang, C.; Daemen, J.J.; Li, Z. Experimental and theoretical study of the anisotropic properties of shale. Int. J. Rock Mech. Min. Sci. 2015, 74, 58–68. [Google Scholar] [CrossRef]
- Lu, H.; Xie, H.; Luo, Y.; Ren, L.; Zhang, R.; Li, C.; Wang, J.; Yang, M. Failure characterization of Longmaxi shale under direct shear mode loadings. Int. J. Rock Mech. Min. Sci. 2021, 148, 104936. [Google Scholar] [CrossRef]
- Lei, B.; Zuo, J.; Coli, M.; Yu, X.; Li, Y.; Liu, H. Investigation on failure behavior and hydraulic fracturing mechanism of Longmaxi shale with different bedding properties. Comput. Geotech. 2024, 167, 106081. [Google Scholar] [CrossRef]
- Zhou, P.; Xie, H.; Wang, J.; Zou, B.; Hu, J.; Li, C. Thermal effects on mechanical and failure behaviors of anisotropic shale subjected to direct shear. Rock Mech. Geotech. Eng. 2024, 17, 2307–2327. [Google Scholar] [CrossRef]
- Li, T. Study on Anisotropic Mechanical Behavior of Reservoir Shale in Shear Failure. Master’s Thesis, Chongqing University, Chongqing, China, 2021. (In Chinese). [Google Scholar] [CrossRef]
- Fan, Z.; Ren, L.; Xie, H.; Zhang, R.; Li, C.; Lu, H.; Zhang, A.; Zhou, Q.; Ling, W. 3D anisotropy in shear failure of a typical shale. Pet. Sci. 2023, 20, 212–229. [Google Scholar] [CrossRef]
- Ye, C.; Xie, H.; Wu, F.; Hu, J.; Ren, L.; Li, C. Asymmetric failure mechanisms of anisotropic shale under direct shear. Int. J. Rock Mech. Min. Sci. 2024, 183, 105941. [Google Scholar] [CrossRef]
- Hu, J.; Yang, S.; Fu, D.; Rui, R.; Yu, Y.; Chen, Z. Rock mechanics of shear rupture in shale gas reservoirs. J. Nat. Gas Sci. Eng. 2016, 36, 943–949. [Google Scholar] [CrossRef]
- Li, N.; Zhang, S.; Zou, Y.; Ma, X.; Zhang, Z.; Li, S.; Chen, M.; Sun, Y. Acoustic Emission Response of Laboratory Hydraulic Fracturing in Layered Shale. Rock Mech. Rock Eng. 2018, 51, 3395–3406. [Google Scholar] [CrossRef]
- Niandou, H.; Shao, J.; Henry, J.; Fourmaintraux, D. Laboratory investigation of the mechanical behaviour of Tournemire shale. Int. J. Rock Mech. Min. Sci. 1997, 34, 3–16. [Google Scholar] [CrossRef]
- Yang, S.Q.; Yin, P.F.; Li, B.; Yang, D.S. Behavior of transversely isotropic shale observed in triaxial tests and Brazilian disc tests. Int. J. Rock Mech. Min. Sci. 2020, 133, 104435. [Google Scholar] [CrossRef]
- Li, C.; Xie, H.; Wang, J. Anisotropic characteristics of crack initiation and crack damage thresholds for shale. Int. J. Rock Mech. Min. Sci. 2020, 126, 104178. [Google Scholar] [CrossRef]
- Jia, Y.; Tang, J.; Lu, Y.; Lu, Z. Laboratory geomechanical and petrophysical characterization of Longmaxi shale properties in Lower Silurian Formation, China. Mar. Pet. Geol. 2021, 124, 104800. [Google Scholar] [CrossRef]
- Zhao, C.; Liu, J.; Xu, D.; Zhang, L.; Lyu, C.; Ren, Y. Investigation on Mechanical Properties, AE Characteristics, and Failure Modes of Longmaxi Formation Shale in Changning, Sichuan Basin, China. Rock Mech. Rock Eng. 2023, 56, 1239–1272. [Google Scholar] [CrossRef]
- Heng, S.; Li, X.; Liu, X.; Chen, Y. Experimental study on the mechanical properties of bedding planes in shale. J. Nat. Gas Sci. Eng. 2020, 76, 103161. [Google Scholar] [CrossRef]
- Fan, Z.; Xie, H.; Ren, L.; Zhang, R.; He, R.; Li, C.; Zhang, Z.; Wang, J.; Xie, J. Anisotropy in shear-sliding fracture behavior of layered shale under different normal stress conditions. J. Cent. South Univ. 2022, 29, 3678–3694. [Google Scholar] [CrossRef]
- Zhang, A.; Zhang, R.; Lu, H.; Yang, Z.; Zhang, Z.; Ren, L. Anisotropy in shear failure of shale: An insight from microcracking to macrorupture. Measurement 2025, 243, 116391. [Google Scholar] [CrossRef]
- Wu, S.; Ge, H.; Li, T.; Wang, X.; Li, N.; Zou, Y.; Gao, K. Characteristics of fractures stimulated by supercritical carbon dioxide fracturing in shale based on acoustic emission monitoring. Int. J. Rock Mech. Min. Sci. 2022, 152, 105065. [Google Scholar] [CrossRef]
- Liu, D.; Wang, Z.; Zhang, X.; Wang, Y.; Zhang, X.; Li, D. Experimental investigation on the mechanical and acoustic emission characteristics of shale softened by water absorption. J. Nat. Gas Sci. Eng. 2018, 50, 301–308. [Google Scholar] [CrossRef]
- Xie, Y.; Hou, M.Z.; Li, C. Anisotropic characteristics of acoustic emission and the corresponding multifractal spectrum during progressive failure of shale under cyclic loading. Int. J. Rock Mech. 2023, 165, 105364. [Google Scholar] [CrossRef]
- Du, K.; Li, X.; Tao, M.; Wang, S. Experimental study on acoustic emission (AE) characteristics and crack classification during rock fracture in several basic lab tests. Int. J. Rock Mech. Min. Sci. 2020, 133, 104411. [Google Scholar] [CrossRef]
- Qi, S.; Zheng, B.; Wu, F.; Huang, X.; Guo, S.; Zhan, Z.; Zou, Y.; Barla, G. A new dynamic direct shear testing device on rock joints. Rock Mech. Rock Eng. 2020, 53, 4787–4798. [Google Scholar] [CrossRef]
- Zheng, B.; Qi, S.; Huang, X.; Guo, S.; Wang, C.; Zhan, Z.; Luo, G. An Advanced Shear Strength Criterion for Rock Discontinuities Considering Size and Low Shear Rate. Appl. Sci. 2020, 10, 4095. [Google Scholar] [CrossRef]
- Zheng, B.; Qi, S.; Luo, G.; Liu, F.; Huang, X.; Guo, S.o. Characterization of discontinuity surface morphology based on 3D fractal dimension by integrating laser scanning with ArcGIS. Bull. Eng. Geol. Environ. 2021, 80, 2261–2281. [Google Scholar] [CrossRef]
- Luo, G.; Qi, S.; Zheng, B. Rate Effect on the Direct Shear Behavior of Granite Rock Bridges at Low to Subseismic Shear Rates. J. Geophys. Res. Solid Earth 2022, 127, e2022JB024348. [Google Scholar] [CrossRef]
- Liu, J.; Yang, D.; Hu, J.; Yu, B.; Nie, Z. Experimental Study on the Mechanical Properties and ionion Characteristics of Different Bedding High-Rank Coals. ACS Omega 2023, 8, 22168–22177. [Google Scholar] [CrossRef] [PubMed]
- Luo, G.; Qi, S.; Zheng, B. A comparative study of progressive failure of granite and marble rock bridges under direct shearing. Sci. Rep. 2024, 14, 10865. [Google Scholar] [CrossRef]
- Zhao, X.; Cai, M. A mobilized dilation angle model for rocks. Int. J. Rock Mech. Min. Sci. 2010, 47, 368–384. [Google Scholar] [CrossRef]
- Zheng, L.; Wang, L.; Zhu, L.; Fan, B.; Dong, M.; Sun, Z.; An, C. Analytical model of the shear behaviors of bolted rough joints based on the dilation effect and surface abrasion. Eng. Geol. 2021, 294, 106399. [Google Scholar] [CrossRef]
- Zhang, X.; Hu, B.; Li, J.; Huang, L.; Zhang, Z.; Li, B.; Zhong, Z.; Wan, C. Acid corrosion deterioration of carbonaceous shale: Mechanism revealed from shear properties and macro-micro structure. Constr. Build. Mater. 2025, 474, 141062. [Google Scholar] [CrossRef]
- Barton, N. Review of a new shear-strength criterion for rock joints. Eng. Geol. 1973, 7, 287–332. [Google Scholar] [CrossRef]
- Grasselli, G. Shear Strength of Rock Joints Based on Quantified Surface Description. Ph.D. Thesis, EPFL, Lausanne, Switzerland, 2001. [Google Scholar] [CrossRef]
- Grasselli, G.; Egger, P. Constitutive law for the shear strength of rock joints based on three-dimensional surface parameters. Int. J. Rock Mech. Min. Sci. 2003, 40, 25–40. [Google Scholar] [CrossRef]
- Huan, J.; He, M.; Zhang, Z.; Kong, X.; Shang, W. Reverse calculation and quantitative estimation of JRC3D for rough rock fracture surfaces in various shear directions. Phys. Chem. Earth 2024, 134, 103587. [Google Scholar] [CrossRef]
- Zheng, B.; Qi, S.; Guo, S.; Liang, N.; Luo, G.; Zhang, X.; Lu, W.; Jin, C.; Li, Y.; Yu, X.; et al. Experimental Study of Direct Shear Properties of Anisotropic Reservoir Shale. Energies 2024, 17, 1977. [Google Scholar] [CrossRef]
- Zhao, J.; Zhang, D. Dynamic microscale crack propagation in shale. Eng. Fract. Mech. 2020, 228, 106906. [Google Scholar] [CrossRef]

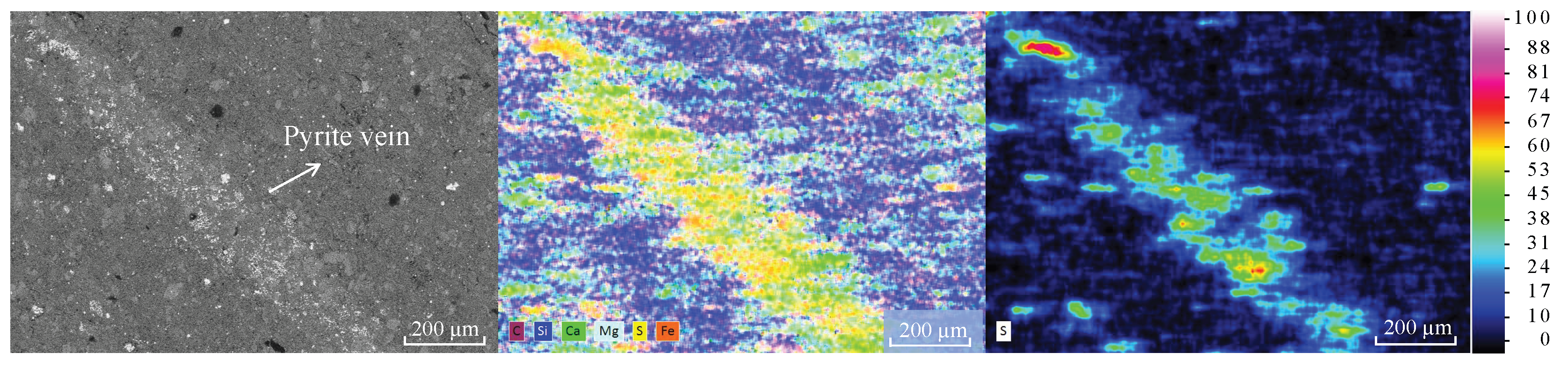
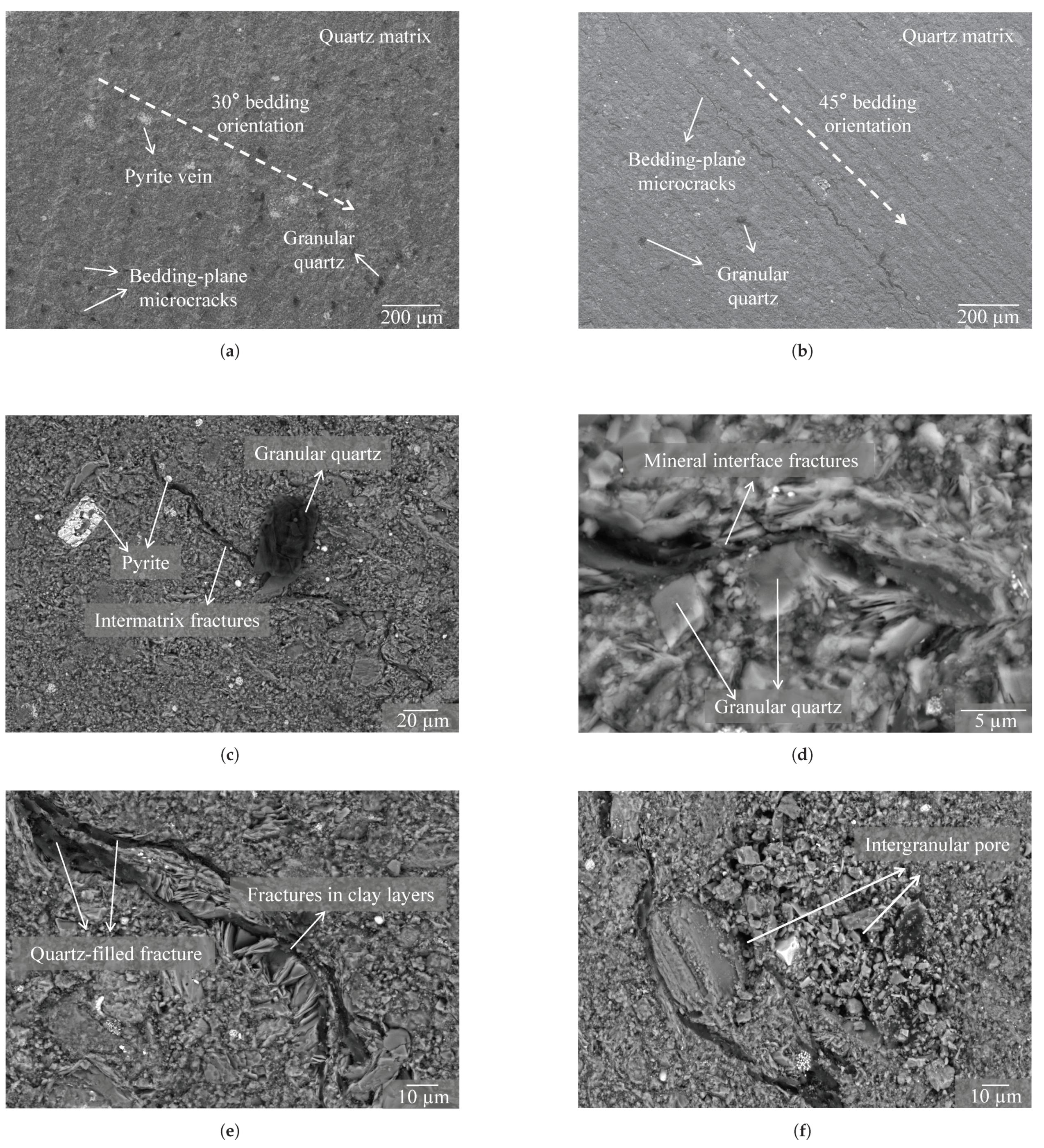




| Bedding Angle (°) | Normal Stress (MPa) | Average Peak Strength (MPa) | Fitting Formula | Internal Friction Angle (°) | Cohesion (MPa) |
|---|---|---|---|---|---|
| 0° | 10 | 19.91 | 51.85 | 8.93 | |
| 15 | 31.45 | ||||
| 20 | 32.78 | ||||
| 25 | 40.68 | ||||
| 30° | 10 | 26.23 | 54.28 | 13.79 | |
| 15 | 37.91 | ||||
| 20 | 39.45 | ||||
| 25 | 48.90 | ||||
| 45° | 10 | 25.81 | 54.54 | 13.35 | |
| 15 | 37.02 | ||||
| 20 | 40.97 | ||||
| 25 | 47.89 | ||||
| 60° | 10 | 31.99 | 42.38 | 22.02 | |
| 15 | 36.11 | ||||
| 20 | 36.94 | ||||
| 25 | 46.92 | ||||
| 90° | 10 | 30.51 | 40.38 | 21.79 | |
| 15 | 35.45 | ||||
| 20 | 36.37 | ||||
| 25 | 44.37 |
| Bedding Angle (°) | Normal Stress (MPa) | Average Residual Strength (MPa) | Fitting Formula | Internal Friction Angle (°) | Cohesion (MPa) |
|---|---|---|---|---|---|
| 0° | 10 | 7.18 | 33.44 | 0 | |
| 15 | 9.24 | ||||
| 20 | 12.45 | ||||
| 25 | 17.11 | ||||
| 30° | 10 | 8.76 | 27.52 | 4.06 | |
| 15 | 12.23 | ||||
| 20 | 15.30 | ||||
| 25 | 16.42 | ||||
| 45° | 10 | 8.90 | 13.45 | 6.76 | |
| 15 | 10.99 | ||||
| 20 | 11.00 | ||||
| 25 | 12.88 | ||||
| 60° | 10 | 9.14 | 7.65 | 8.86 | |
| 15 | 11.81 | ||||
| 20 | 12.83 | ||||
| 25 | 11.05 | ||||
| 90° | 10 | 9.20 | 8.92 | 7.66 | |
| 15 | 11.65 | ||||
| 20 | 7.60 | ||||
| 25 | 13.17 |
| Resource | Bedding Angle (°) | c (MPa) | (°) |
|---|---|---|---|
| This work (cuboid sample) | 90° | 21.79 | 40.38 |
| 0° | 8.93 | 51.85 | |
| [38] (cubic sample) | 90° | 25.89 | 39.08 |
| 0° | 17.44 | 43.88 | |
| [27] (cubic sample) | 90° | 29.83 | 44.89 |
| 0° | 23.85 | 46.50 | |
| [23] (cubic sample) | 90° | 31.15 | 27.11 |
| 0° | 19.49 | 32.94 | |
| [36] (cylindrical sample) | 90° | 36.22 | 16.18 |
| 0° | 31.22 | 8.93 | |
| [12] (cylindrical sample) | 90° | 25.22 | 32.24 |
| 0° | 4.91 | 25.90 | |
| [22] (cylindrical sample) | 90° | 16.04 | 35.34 |
| 0° | 8.92 | 32.31 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, Z.; Zheng, B.; Qi, S.; Guo, S.; Luo, G.; Wang, T.; Jiao, J. Study of the Macro-Mesoscopic Shear Anisotropic Mechanical Behavior of Reservoir Shale. Processes 2025, 13, 1404. https://doi.org/10.3390/pr13051404
Zhu Z, Zheng B, Qi S, Guo S, Luo G, Wang T, Jiao J. Study of the Macro-Mesoscopic Shear Anisotropic Mechanical Behavior of Reservoir Shale. Processes. 2025; 13(5):1404. https://doi.org/10.3390/pr13051404
Chicago/Turabian StyleZhu, Zifang, Bowen Zheng, Shengwen Qi, Songfeng Guo, Guangming Luo, Tao Wang, and Jianrui Jiao. 2025. "Study of the Macro-Mesoscopic Shear Anisotropic Mechanical Behavior of Reservoir Shale" Processes 13, no. 5: 1404. https://doi.org/10.3390/pr13051404
APA StyleZhu, Z., Zheng, B., Qi, S., Guo, S., Luo, G., Wang, T., & Jiao, J. (2025). Study of the Macro-Mesoscopic Shear Anisotropic Mechanical Behavior of Reservoir Shale. Processes, 13(5), 1404. https://doi.org/10.3390/pr13051404






