Study on the Influence of Reynolds Number on Heat Exchange Performance and Nusselt Number of Spray Coil Heat Exchanger
Abstract
1. Introduction
2. Materials and Methods
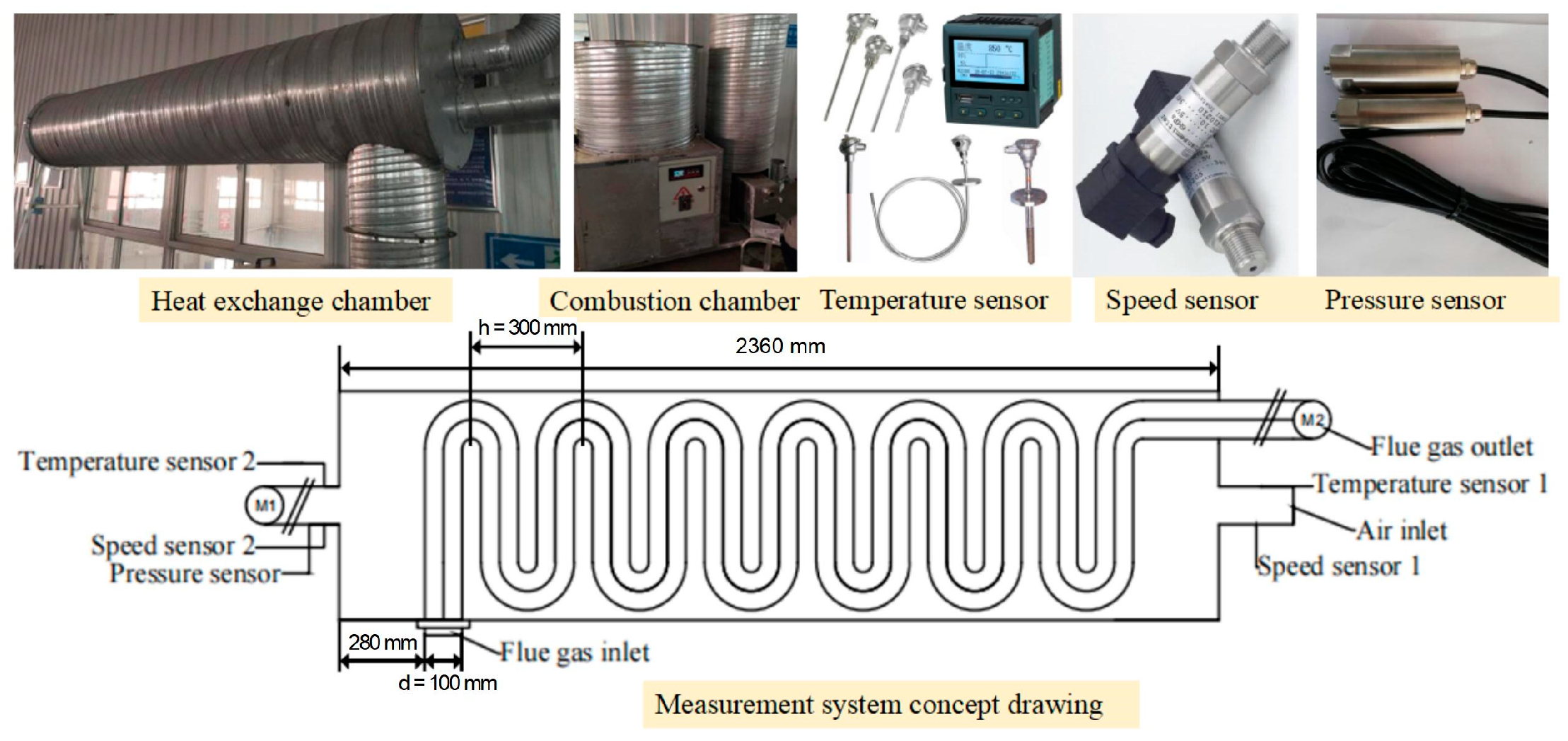
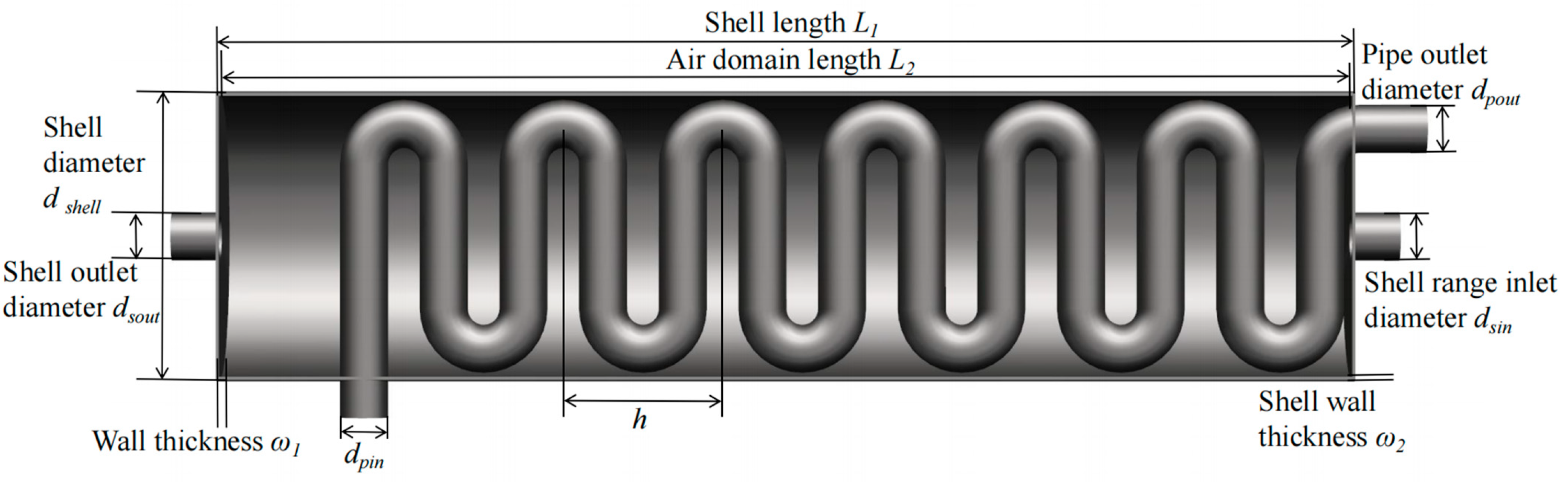
2.1. Geometric Model and Experimental Device
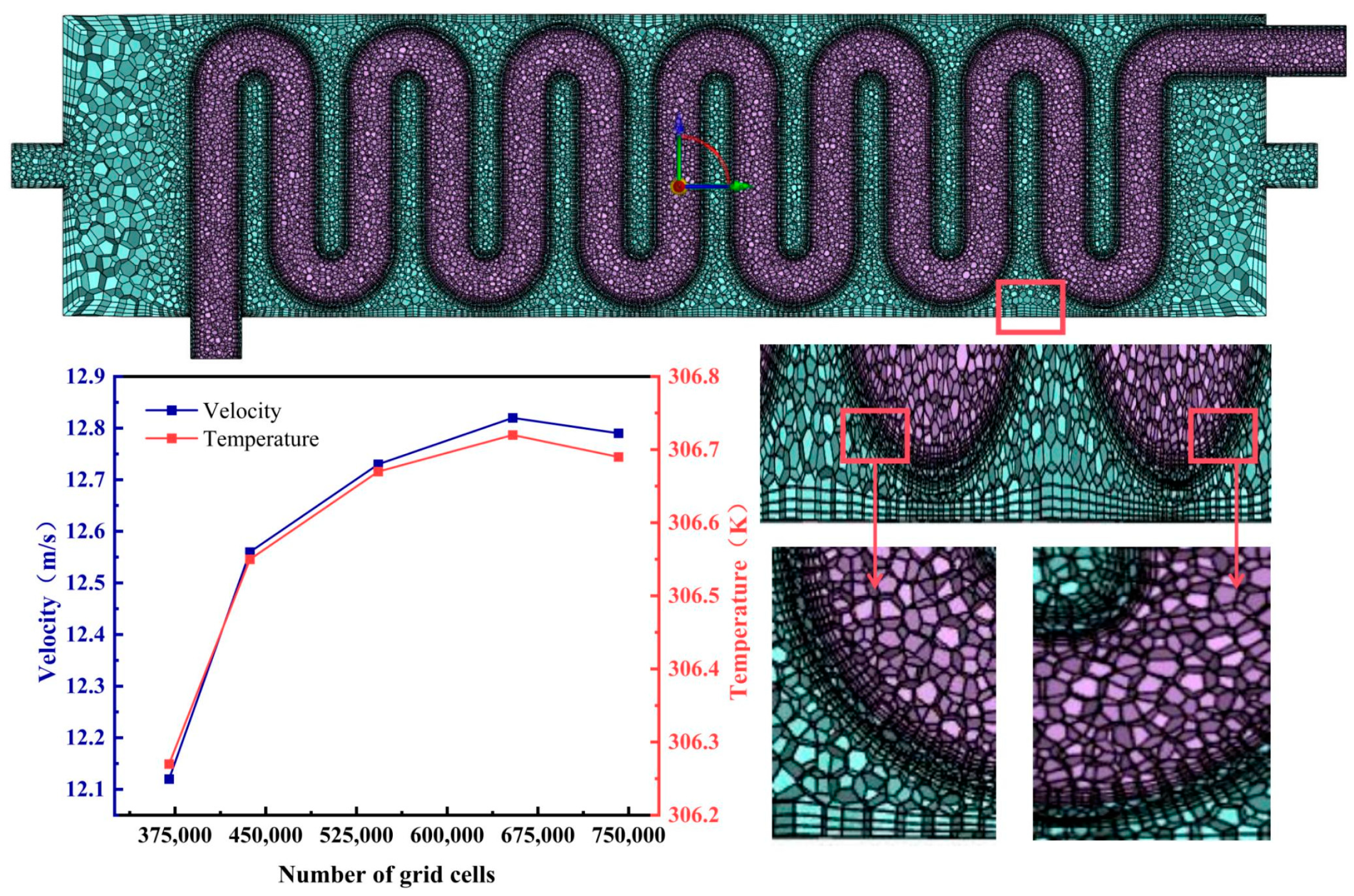
2.2. Mesh Generation and Independence Testing
2.3. Flow Control Equations and Numerical Models
2.4. Experimental Procedure
3. Results and Discussion
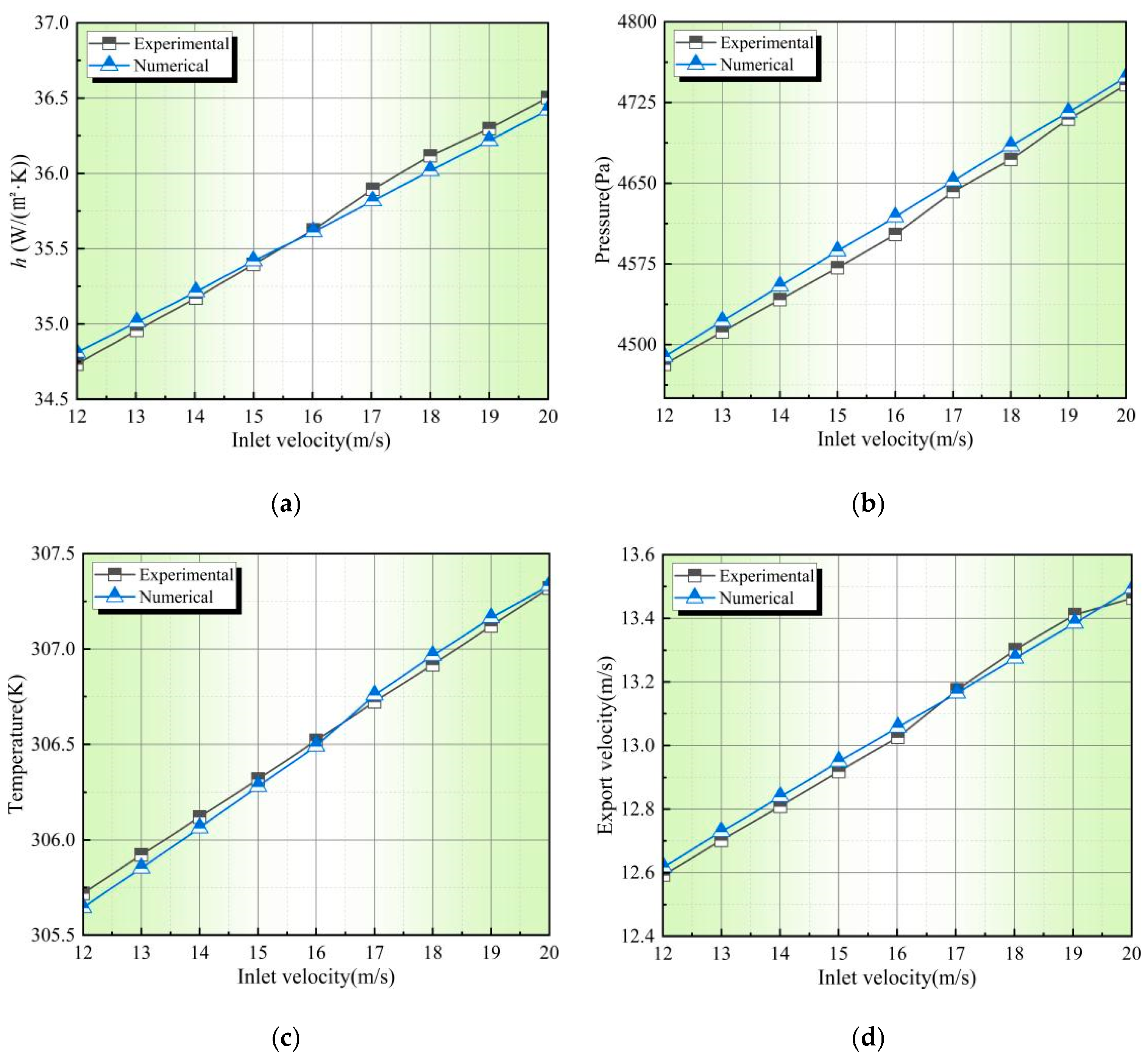
3.1. Reliability Verification of Numerical Results
3.2. Analysis of Heat Transfer Performance in Shell of Heat Exchanger
3.3. Analysis of Flow Performance in Shell of Heat Exchanger
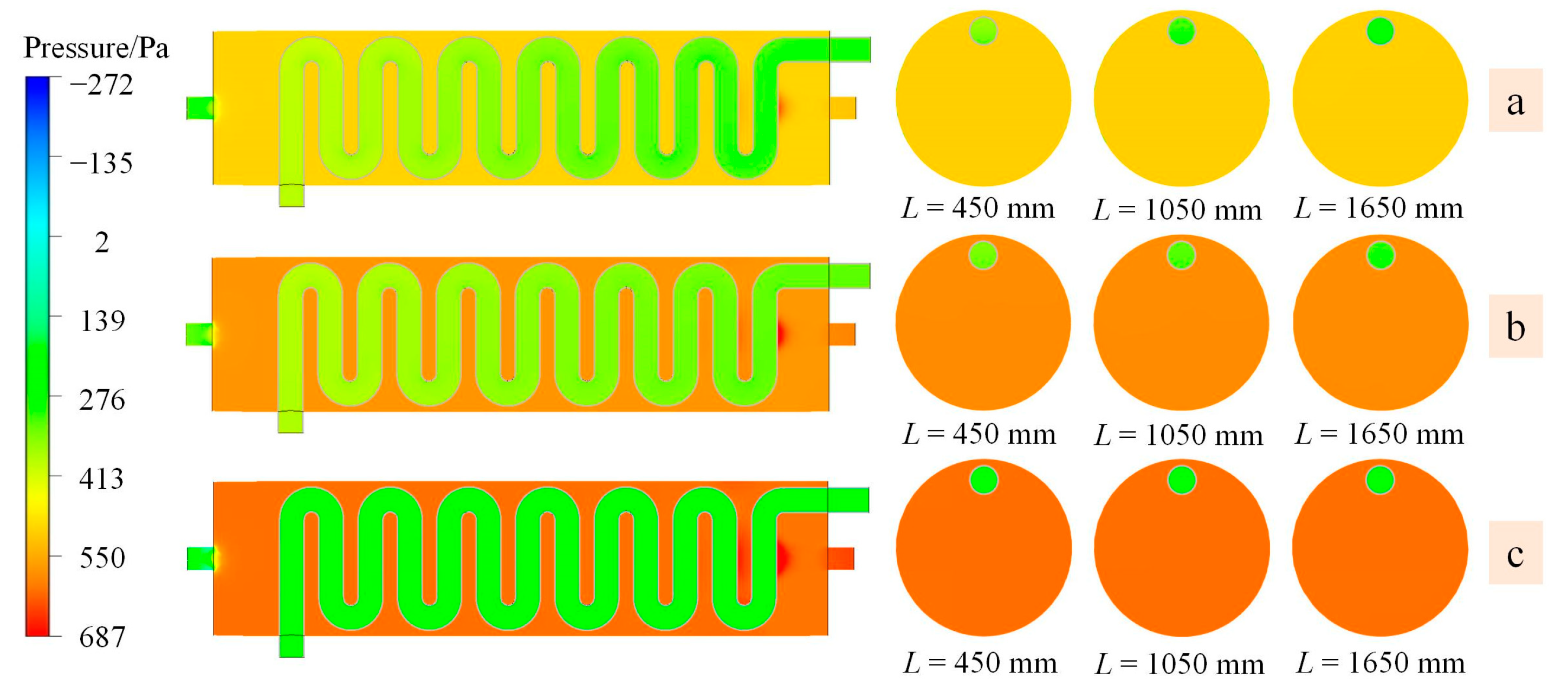
3.4. Analysis of Pressure Performance in Shell of Heat Exchanger
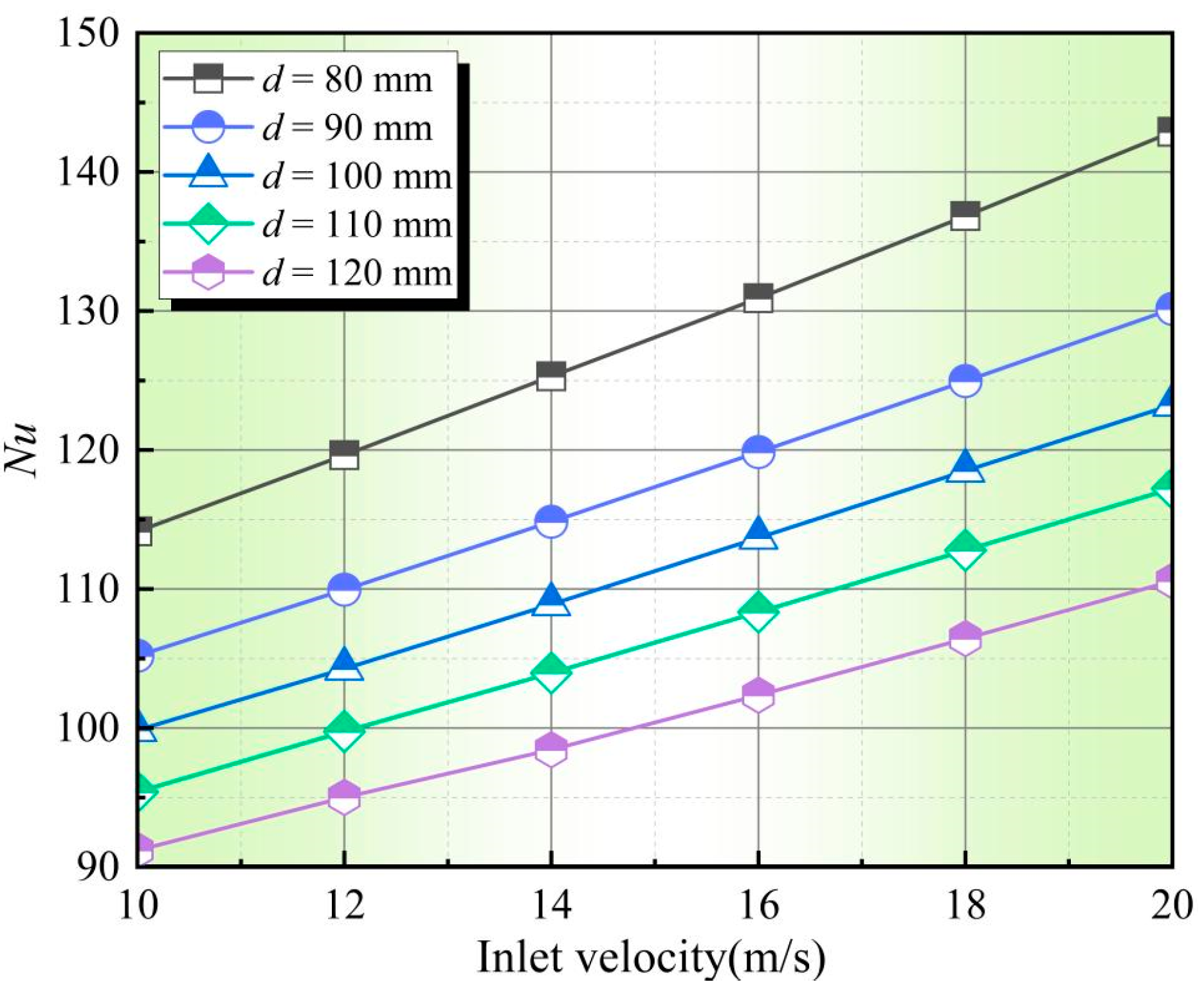
3.5. Effect of Shell and Tube Diameter Parameters on Heat Transfer
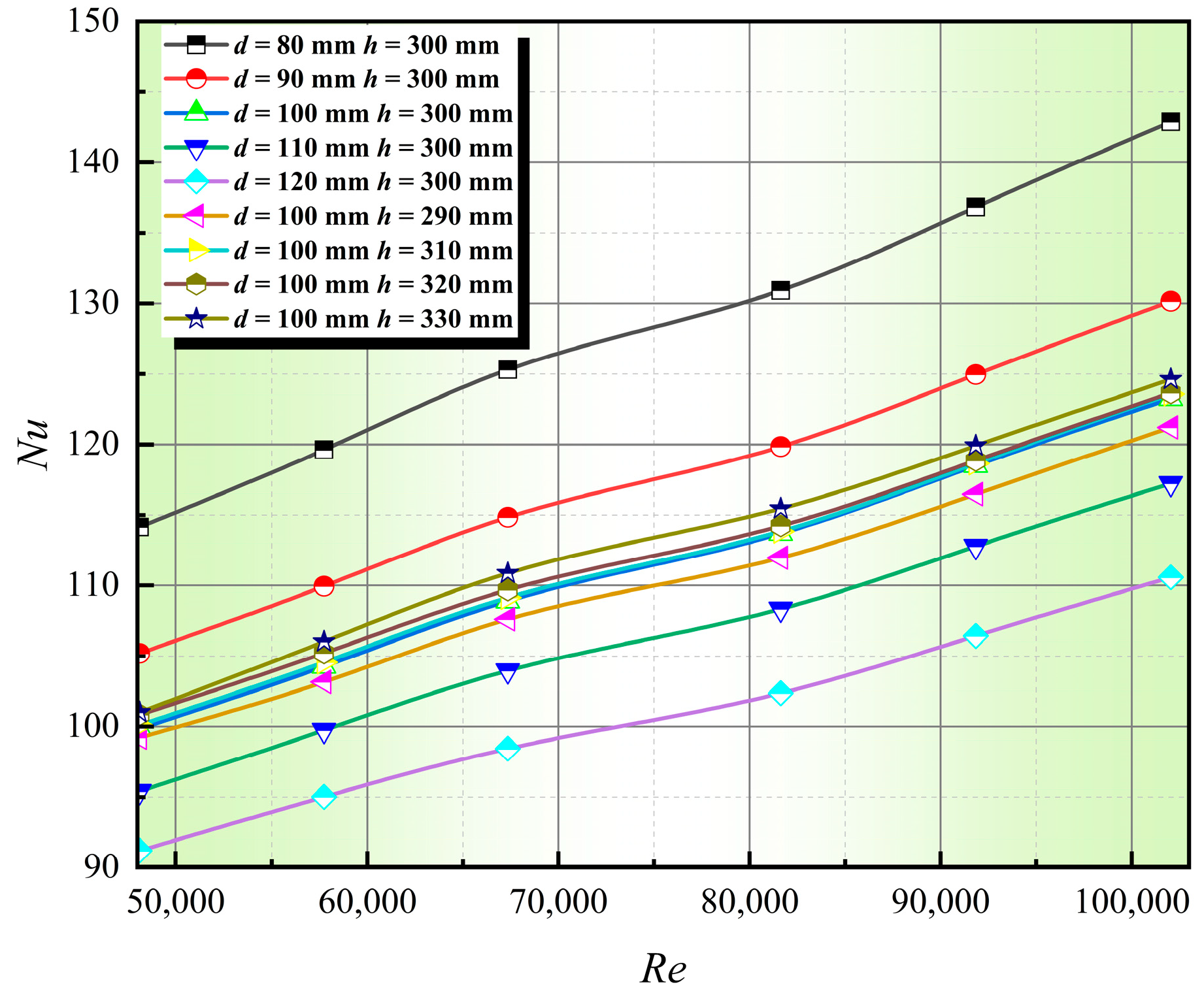
3.6. Effect of Serpentine Bump Spacing on Heat Transfer
3.7. Influence of Re on Nu
4. Conclusions
- (1)
- The temperature and velocity on the air-inlet side of the serpentine heat exchanger are lower compared to other regions, forming a heat transfer dead zone. The primary reason for this phenomenon is that the air-inlet side is located at the end of the serpentine tube, where the high-temperature flue gas entering the tube continuously dissipates heat along its path until it reaches the end where the air enters. By that point, the temperature has significantly decreased, resulting in limited heat transfer in this area and creating a heat transfer dead zone. Increasing the distance between the serpentine protrusions expands the heat transfer area, making heat transfer more uniform, while also enlarging the Nusselt number (Nu) and enhancing convective heat transfer efficiency. With a serpentine protrusion spacing of h = 330 mm, under the same flow rate, Nu increased by a maximum of 23.39%.
- (2)
- The air inlet-velocity within the shell significantly influences the performance of the heat exchanger. Increasing the velocity can raise Re, thereby strengthening convective heat transfer and improving Nu. However, excessively high inlet velocities can enlarge the negative pressure area before the shell outlet, leading to increased pressure and uneven heat transfer in localized areas. When exploring the optimal air-inlet velocity, one can start experimenting from low velocities and gradually increase the inlet flow rate, continuing until the heat transfer efficiency reaches a maximum and stabilizes, achieving the optimal inlet air velocity condition.
- (3)
- The diameter of the serpentine tubes shows an inverse relationship with Nu. Enlarging the tube diameter increases the heat exchange area but reduces the heat transfer rate per unit area. This reduction leads to a decrease in the convective heat transfer coefficient, subsequently decreasing Nu. Through comprehensive analysis, when d = 80 mm and h = 300 mm, the highest percentage increase in Nu concerning Re is 25.17%. At this point, the convective heat transfer strength is at its peak, resulting in optimal heat transfer performance.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Han, F. The status quo and prospects of my country’s renewable energy development. Renew. Energy Resour. 2010, 28, 137–140. [Google Scholar]
- Peng, H.; Yao, K.; Cao, X.; Jiang, S.; Wang, Y.; Peng, F. Experimental study on the combustion characteristics of two different biomass briquettes. Acta Energiae Solaris Sin. 2016, 37, 1002–1008. [Google Scholar]
- Li, M.; Zhang, W.; Niu, Q.; Nie, C. Performance test of biomass stirling cogeneration system in rice drying. J. Eng. Thermophys. 2015, 36, 1847–1852. [Google Scholar]
- Fan, Z.; Ci, W.; Li, H.; Zhao, F.; Zhang, Z. Development of a new type of biomass hot blast stove. Agric. Equip. Veh. Eng. 2017, 55, 82–84. [Google Scholar]
- Li, W.; Li, Z.; Han, W.; Li, D.; Yan, S.; Zhou, J. Study of The Flow Characteristics of Pumped Media in The Confined Morphology of A Fer-rofluid Pump with Annular Microscale Constraints. J. Fluids Eng. 2025, 147, 021201. [Google Scholar] [CrossRef]
- Li, W.; Li, Z.; Han, W.; Tan, S.; Yan, S.; Wang, D.; Yang, S. Time-mean equation and multi-field coupling numerical method for low-Reynolds-number turbulent flow in ferrofluid. Phys. Fluids 2023, 35, 125145. [Google Scholar] [CrossRef]
- Tu, F.; Ma, S.; Gao, S.; Liu, Q.; Mao, J. Numerical simulation of the influence of incoming flow velocity distribution on the performance of radial heat pipe heat exchangers. J. Cent. South Univ. (Nat. Sci. Ed.) 2013, 44, 3904–3910. [Google Scholar]
- Liu, B.; Zhang, J.; Tan, X. The effect of jet impingement velocity on heat transfer under rotation effect. J. Eng. Thermophys. 2015, 36, 366–370. [Google Scholar]
- Hua, H.J.; Al-Obaidi, A.S.M.; Meng, C.W.; Hui, K.J.L.N. Effect of supply and exhaust air velocity on the enthalpy and temperature exchange efficiency of a paper heat exchanger. MATEC Web Conf. 2021, 335, 03006. [Google Scholar] [CrossRef]
- Li, S.; Lu, L.; Chen, J.; Chai, W.; Jiang, Y.; Zhang, H. Simulation study on the influence of structural parameters on the tube side heat transfer of the wound tube heat exchanger. Energy Conserv. Technol. 2017, 35, 498–504. [Google Scholar]
- Wang, S.; Jian, G.; Xiao, J.; Wang, J.; Wen, J. Numerical simulation study on multi-objective optimization of structural parameters of wound tube heat exchanger. J. Xi’an Jiaotong Univ. 2017, 51, 9–15. [Google Scholar]
- Lu, C.; Li, Z.; Wang, Q.; Liu, X. Numerical analysis of the effects of fin structure and PCM physical parameters on the thermal storage process of energy storage furnace. Therm. Power Eng. 2020, 35, 114–121. [Google Scholar]
- Zhao, X.; Li, J.; Xu, J.; Zhu, X.; Liao, Q. Numerical simulation of flow and heat transfer characteristics of three-dimensional rectangular externally finned tubes. J. Eng. Thermophys. 2019, 40, 907–915. [Google Scholar]
- Lazova, M.; Kaya, A.; Billiet, M.; Lecompte, S.; Manolakos, D.; De Paepe, M. Experimental Assessment of a Helical Coil Heat Exchanger Operating at Subcritical and Supercritical Conditions in a Small-Scale Solar Organic Rankine Cycle. Energies 2017, 10, 619. [Google Scholar] [CrossRef]
- Jiang, H.; Jiang, T.; Tian, H.; Wu, Q.; Deng, C.; Zhang, R. Heat Transfer Simulation and Structural Optimization of Spiral Fin-and-Tube Heat Exchanger. Electronics 2024, 13, 4639. [Google Scholar] [CrossRef]
- Patidar, N.; Makrariya, A.; Rasheed, R.H.; Singh, N.K.; Alsayah, A.M.; Alshukri, M.J.; Rathore, R.K. Model to identify comfortable cloth fabric for human body during thermal stress due to physical exercise. Mater. Res. Express 2024, 11, 085402. [Google Scholar] [CrossRef]
- Rathore, R.K.; Patel, P.; Singh, N.K.; Kaimkuriya, A.; Gajghat, R.H.; Kumbhalkar, M.A.; Verma, K. EES analysis of low-grade thermal energy organic-rankine-vapor compression-refrigeration system. AIP Conf. Proc. 2023, 2839, 020031. [Google Scholar]
- Yang, Y.; Ma, Q.; Zuo, Y. Heat transfer characteristics and parameter optimization of the flow channel of herringbone plate heat exchanger. Trans. Chin. Soc. Agric. Eng. 2019, 35, 210–215. [Google Scholar]
- Li, W.; Li, Z.; Han, W.; Li, Y.; Yan, S.; Zhao, Q.; Gu, Z. Pumping-velocity variation mechanisms of a ferrofluid micropump and structural optimization for reflow inhibition. Phys. Fluids 2023, 35, 052005. [Google Scholar]
- Li, W.; Li, Z.; Han, W.; Li, Y.; Yan, S.; Zhao, Q.; Chen, F. Measured viscosity characteristics of Fe3O4 ferrofluid in magnetic and thermal fields. Phys. Fluids 2023, 35, 012002. [Google Scholar] [CrossRef]
- Chen, F.; Zhang, J.; Li, Z.; Yan, S.; Li, W.; Yan, Z.; Liu, X. Effect of the surface coating of carbonyl iron particles on the dispersion stability of magnetorheological fluid. Sci. Rep. 2024, 14, 11358. [Google Scholar] [CrossRef] [PubMed]
- Li, W.; Li, Z.; Qin, Z.; Yan, S.; Wang, Z.; Peng, S. Influence of the solution pH on the design of a hydro-mechanical magneto-hydraulic sealing device. Eng. Fail. Anal. 2022, 135, 106091. [Google Scholar] [CrossRef]
- Xu, L.; Kan, K.; Zheng, Y.; Liu, D.; Binama, M.; Xu, Z.; Yan, X.; Guo, M.; Chen, H. Rotating stall mechanism of pump-turbine in hump region: An insight into vortex evolution. Energy 2024, 292, 130579. [Google Scholar] [CrossRef]
- Li, W.; Li, Z.; Han, W.; Wang, Y.; Zhao, J.; Zhou, J. Morphologic transformation of ferrofluid during micropump driving under field control. Ann New York Acad Sci. 2025, 1543, 194–203. [Google Scholar] [CrossRef] [PubMed]
- Liu, D.; Li, Z.; Xu, L.; Li, J.; Yang, Y.; Wang, X.; Pang, J.; Liu, X. Vortex motion in vaneless space and runner passage of pump-turbine in S-shaped region. Phys. Fluids 2024, 36, 0194239. [Google Scholar] [CrossRef]
- Ren, Z.; Li, D.; Wang, H.; Liu, J.; Li, Y. Computational model for predicting the dynamic dissolution and evolution behaviors of gases in liquids. Phys. Fluids 2022, 34, 103310. [Google Scholar] [CrossRef]
- Wang, X.; Zheng, N.; Liu, P.; Liu, Z.; Liu, W. Analysis of flow and heat transfer performance of wave-baffled rod heat exchanger. J. Eng. Thermo-Phys. 2016, 37, 1758–1762. [Google Scholar]
- Liu, J.; Liu, W. Numerical simulation of heat transfer and flow in a rod baffle heat exchanger using deep groove spiral bellows. J. Eng. Thermophys. 2015, 36, 151–153. [Google Scholar]
- Yang, S.; Tao, W. Heat Transfer, 5th ed.; Higher Education Press: Beijing, China, 2019; pp. 344–350. [Google Scholar]
- Ren, Z.; Li, D.; Zhou, W.; Li, Z.; Wang, H.; Liu, J.; Li, Y.; Khoo, B.C. Gas–liquid mass-transfer characteristics during dissolution and evolution in quasi-static and dynamic processes. Int. J. Multiph. Flow 2024, 180, 104970. [Google Scholar]
- Qin, X.; Wang, S.; Yuan, Y. Heat Transfer Coefficient Estimation and Performance Evaluation of Shell and Tube Heat Exchanger Using Flue Gas. Processes 2021, 9, 939. [Google Scholar] [CrossRef]
- Bahadori, A. Prediction of compressed air transport properties at elevated pressures and high temperatures using simple method. Appl. Energy 2010, 88, 1434–1440. [Google Scholar] [CrossRef]
- Zhou, W.; Jin, X.; Ding, L.; Ma, J.; Su, H.; Zhao, A. Research on vibration signal decomposition of cracked rotor-bearing system with double-disk based on CEEMDAN-CWT. Appl. Acoust. 2024, 227, 110254. [Google Scholar] [CrossRef]
- Jin, X.; Zhou, W.; Ma, J.; Su, H.; Liu, S.; Gao, B. Analysis on the vibration signals of a novel double-disc crack rotor-bearing system with single defect in inner race. J. Sound Vib. 2025, 595, 118729. [Google Scholar] [CrossRef]
- Zhou, W.; Qiu, N.; Wang, L.; Gao, B.; Liu, D. Dynamic analysis of a planar multi-stage centrifugal pump rotor system based on a novel coupled model. J. Sound Vib. 2018, 434, 237–260. [Google Scholar] [CrossRef]



| Material | Cu | Steel |
|---|---|---|
| (kg/m3) | 8930 | 7750 |
| W (m·K) | 370 | 31.1 |
| T/°C | ||||
|---|---|---|---|---|
| 500 | 0.457 | 1.185 | 6.560 | 3.408 |
| 600 | 0.407 | 1.241 | 7.420 | 3.790 |
| 700 | 0.363 | 1.239 | 8.247 | 4.007 |
| 800 | 0.303 | 1.237 | 9.442 | 4.307 |
| 900 | 0.252 | 1.235 | 10.621 | 4.609 |
| 1000 | 0.204 | 1.233 | 11.854 | 4.907 |
| 1100 | 0.153 | 1.231 | 12.078 | 5.206 |
| T/°C | ||||
|---|---|---|---|---|
| 10 | 1.247 | 1.005 | 2.51 | 17.6 |
| 20 | 1.205 | 1.005 | 2.59 | 18.1 |
| 30 | 1.165 | 1.005 | 2.67 | 18.6 |
| 40 | 1.128 | 1.005 | 2.76 | 19.1 |
| 50 | 1.093 | 1.005 | 2.83 | 19.6 |
| 60 | 1.060 | 1.005 | 2.90 | 20.1 |
| 70 | 1.029 | 1.009 | 2.96 | 20.6 |
| 80 | 1.000 | 1.009 | 3.05 | 21.1 |
| 90 | 0.972 | 1.009 | 3.13 | 21.5 |
| 100 | 0.946 | 1.009 | 3.21 | 21.9 |
| 120 | 0.898 | 1.009 | 3.34 | 22.8 |
| Parameter | T | V | P | hc |
|---|---|---|---|---|
| Numerical simulation | 306.67 | 12.93 | 4491 | 35.03 |
| Experimental test | 317.421 | 13.581 | 4721.136 | 36.113 |
| Relative error % | 3.39 | 4.79 | 4.87 | 3.00 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Han, T.; Li, Q.; Shang, L.; Chen, X.; Zhou, F.; Li, W. Study on the Influence of Reynolds Number on Heat Exchange Performance and Nusselt Number of Spray Coil Heat Exchanger. Processes 2025, 13, 588. https://doi.org/10.3390/pr13020588
Han T, Li Q, Shang L, Chen X, Zhou F, Li W. Study on the Influence of Reynolds Number on Heat Exchange Performance and Nusselt Number of Spray Coil Heat Exchanger. Processes. 2025; 13(2):588. https://doi.org/10.3390/pr13020588
Chicago/Turabian StyleHan, Tianding, Qifei Li, Lin Shang, Xiangyu Chen, Feng Zhou, and Wangxu Li. 2025. "Study on the Influence of Reynolds Number on Heat Exchange Performance and Nusselt Number of Spray Coil Heat Exchanger" Processes 13, no. 2: 588. https://doi.org/10.3390/pr13020588
APA StyleHan, T., Li, Q., Shang, L., Chen, X., Zhou, F., & Li, W. (2025). Study on the Influence of Reynolds Number on Heat Exchange Performance and Nusselt Number of Spray Coil Heat Exchanger. Processes, 13(2), 588. https://doi.org/10.3390/pr13020588





