Bubble Dynamics in Sustainable Technologies: A Review of Growth, Collapse, and Heat Transfer

Abstract

The study of bubble growth and collapse is of great significance in the context of sustainability due to its influence on numerous energy-related processes and technologies. Understanding the dynamics of bubble behavior is vital for optimising heat transfer efficiency, which has an energetic role in improving the performance of sustainable systems such as nuclear reactors, thermal inkjet printing, and nucleate boiling. Indeed, researchers can progress strategies to enhance the efficiency of these technologies by analysing the parameters influencing bubble growth and collapse, which can lead to reduced energy consumption and environmental impact. Although several theoretical models and experimental investigations have been achieved in the past to inspect bubble growth and collapse, a thorough review and critical assessment of the studies conducted have not yet been achieved. This review aims to provide a comprehensive understanding of the relationship between bubble dynamics and sustainability, highlighting the potential for further research and development in this area. Specifically, the scope and limitations of past research on bubble growth and collapse is conducted to fill this gap in the open literature. The review covers both numerical and experimental studies of bubble growth and collapse in a wide set of innovative industrial applications including nuclear reactors, thermal inkjet printing, nucleate boiling, hydrodynamic erosion, and ultrasonic and medicinal therapy. The current review also attempts to illustrate and evaluate the numerical methods used and underlines the most relevant results from the studies that were looked at in order to provide researchers with a clear picture of the growth and collapse of bubbles in different applications. The results give a precise understanding of the dynamics of bubble growth and collapse and the related temperature change and cumulative heat transmission from the thermal boundary layer. Additionally, it has been demonstrated that simulation-based models can effectively predict transport coefficients. However, the review observes a number of limitations of the past research on bubble growth and collapse. Due to numerical instability, very little work with respect to dynamic modelling has been carried out on the mechanisms of bubble collapse. Accordingly, a number of recommendations are made for the improvement of heat transmission during bubble growth and collapse. Specifically, future criteria for the highest heat transmission will demand more precise experimental and numerical approaches.

1. Introduction

The growth and collapse of bubbles in fluids can have significant impacts on a variety of physical processes and engineering applications, including subcooled nucleate boiling, bubble condensation, and liquid cavitation. In particular, as the liquid is being boiled, the bubbles will rise and promote the mixing of the liquid’s molecules and heat transmission. The procedures are intrinsically complicated due to the occurrence of phase-change heat transfer in a moving interface, which is characterised by the variable radius of the bubble. This makes the operations more difficult [1].

It is crucial to have a thorough appreciation of the bubble dynamics that take place throughout nucleate boiling in order to accomplish operative and reliable heat transfer in several industrial processes, such as distillation columns and refrigeration [2]. Specifically, bubbles are created on a surface immersed in a liquid that is below its boiling point during the subcooled nucleate boiling process. Due to the heat transfer from the surface, the liquid boils at the bubble site with the expansion of the bubbles. The bubble separates from the surface as the bubble reaches a sufficient size, rises to the surface, and then bursts. High-speed liquid jets may be created when the bubble bursts, which could recover heat transmission from the surface [3]. Throughout the boiling process, it is well acknowledged that changes in the shape of the bubbles and the dynamics of the contact lines can have a noteworthy influence on heat transmission. Due to the controlling role that they play in heat transmission and hydrodynamics, the bubble departure frequency, departure diameter, and growth rate are the subjects of a significant amount of research [4].

As the phenomenon of bubble condensation happens, a bubble may condense and collapse when it touches the boundary between a liquid’s surface and a gas phase. A fast-moving liquid jet is created when a bubble bursts, which can erode or damage surrounding surfaces. The collapse of bubbles is required in some applications, such as inkjet printing, since it can facilitate the production of tiny liquid droplets that are extruded from nozzles [5].

Cavitation, a physical phenomenon in liquids, is the act of rupturing a liquid by lessening the pressure at a relatively fixed liquid temperature. As the liquid pressure drops below its vapor pressure, bubbles start to develop and eventually collapse, which is known as liquid cavitation. These bubbles have the potential to expand and then burst, sending high velocity liquid jets and shockwaves toward nearby objects. Pumps, turbines, and propellers may experience cavitation in engineering applications, resulting in decreased performance or even failure [6,7]. Cavitation methods are specifically ultrasonic or ultrasound cavitation techniques. Under high-intensity sound wave irradiation (in industrial processes between 20 and 50 kHz), acoustic cavitation happens in liquids. Yet, the passageway of a liquid through a constriction, such as an orifice plate, throttling valve, venturi, etc., produces hydrodynamic cavitation [8]. The cavitation process is very attractive because it produces large amounts of local energy in the bulk liquid at a low cost, has a great degree of flexibility, and minimises environmental damage [9]. In nucleate boiling, bubble condensation, and liquid cavitation processes, the growth and collapse of bubbles play a substantial role in the overall behavior of systems. Understanding the dynamics of bubble growth and collapse can help engineers to design more efficient and reliable systems and improve our understanding of fundamental physical processes.

Both numerical and experimental studies were carried out concerning the growth and collapse of bubbles in different industrial applications. The ability of numerical modelling to forecast dynamic behavior and comprehend the mechanisms of heat transfer involved in nucleate boiling heat transfer has proven to be very promising. This potential can be ascribed to the rapid advancement of computational resources and the enhancement of various multi-phase models.

Both direct and indirect measurements of the bubble-induced pressure pulses have resulted in the development of a number of different methods over the years. Acoustic transients, such as shockwaves, micro-jets, turbulence, shear forces, etc., released during the collapse of a bubble are collected utilising a hydrophone at a distance from the centre of the collapse. These transients are then utilised to estimate the pressure that was exerted during the collapse [10,11]. During the collapsing bubble-driven pumping, the following effects are caused by a rise in the viscosity of the fluid: (1) an upsurge in the stability of the bubble oscillations, which restricts the creation of a jet that is vital in the operation of the pump; and (2) an upsurge in the viscous pressure head that has to be confronted in order to push the liquid through the hole [12].

The authors believe that the body of published research does not offer a thorough analysis of the advancements made in bubble growth and collapse. In this area of research, the drive of this review is to judgmentally evaluate the conducted studies of bubble growth and collapse illustrating the scope and limitations, innovative configurations, numerical methods, in addition to drawing some of the foremost findings from the existing open literature and identifying a number of unresolved questions for future studies. The scope of this review encompasses a broad diversity of subject areas, one of which is the configuration of experimental or numerical studies on bubble growth and collapse. This article goes into considerable detail about technology strategies for bubble growth and collapse, covering research, application, and development techniques. The consequences of this review may deliver a map for further investigations as they would make it easier for scientists to understand the numerous advancements made in bubble growth and collapse. On top of this, this review attempts to offer a comprehensive understanding of the link between bubble dynamics and sustainability, emphasizing the potential for further research and development in this region.

2. Review Method

The current review is presented to deliver an outline of research that has examined the scope and limitations of recent innovations and developments concerning the growth and collapse of bubbles. This overview facilitates a comprehensive understanding of the topic. The review is further augmented by a detailed examination of existing published studies. Different databases, including ScienceDirect, SpringerLink, Scopus, ResearchGate, and Google Scholar, were used to find related studies and peer reviewed content. The keywords designed to filter and catch related papers included: bubble growth, collapse, cavitation, hydrodynamics, bubble dynamics, and phase change. Although there were no restrictions on the publication dates of the articles, priority was given to the inclusion of the most successful current published studies between 2004 and 2023 to discourse the most innovative investigations.

3. Bubble Growth Studies

Indeed, it is essential to have a proper knowledge of the bubble dynamics that occur during boiling to achieve successful heat transfer in a wide set of applications. It is widely known that during boiling operations, variations in the bubble form and the dynamics of the contact lines may significantly affect the transport of bubbles [13]. Ebullition factors, including the bubble departure diameter, bubble departure frequency, and growth rate are the focus of a plethora of studies because of the controlling impact they have in heat transmission and hydrodynamics. In most situations where heterogeneous bubble nucleation happens on a solid surface, it is well known that the wettability of the surface will control the bubble shape during the whole ebullition cycle. This section focuses on presenting the associated studies of bubble growth and their influences on heat transmission within a wide set of applications with a critical evaluation. The next sections discuss the relative bubble growth studies that are classified as the liquid–gas and solid–gas studies.

3.1. Liquid–Gas Studies

Agarwal et al. [14] utilised a Volume of Fluid (VOF) based interface approach to model the film boiling and bubble production in water at 219 bars and 373 °C, while satisfying an isothermal horizontal surface. Together with an interface mass transfer model, the Navier–Stokes and thermal energy correlations were resolved. The models account for the impacts of interface mass transfer, surface tension, and conforming latent heat as well as the impact of temperature on the conveyed thermal characteristics (specific heat and thermal conductivity) of vapor. The findings provided a clear understanding of film boiling and yielded quantitative data on irregular periodic bubble release forms as well as on temporal and spatial variable film thickness. In comparison to simulations with constant fluid characteristics, the calculations also accurately anticipated the transport coefficients on the horizontal surface, which were strongly affected by the fluctuations in fluid characteristics.

Mukherjee and Dhir [15] numerically examined the heat transfer and bubble dynamics related to lateral bubble merger when nucleate boiling transitions from partial to fully developed. The 3-dimensional Navier/Stokes equation, the continuity equation, and the energy equation are all resolved by the SIMPLE approach. The Level-Set Method (LSM) was employed, and the liquid vapor interface was captured. The LSM involves solving a partial differential equation (PDE) that tracks the growth of the interface corresponding to time. Numerous bubble mergers in a line and in a plane were calculated, and the wall heat transfer and bubble dynamics were contrasted to the case study of a single bubble. The outcomes demonstrated that the total wall heat transmission is greatly increased by the merger of several bubbles. This improved wall heat transfer results from colder liquid being drawn toward the wall throughout contraction after bubble merger, which traps a layer of liquid between the bubble bases.

In 2005, a connected Level-Set and VOF technique for the simulation of incompressible two-phase flows with surface tension was presented by Tomar et al. [16]. The paired algorithm saves bulk while accurately capturing the intricate interfaces. For various superheat levels, a planar simulation of bubble development in water at a critical pressure was conducted. Analysis has been done on how superheat affects the frequency of bubble formation. Additionally, simulations of bubble formation and film boiling in the refrigerant R134a are carried out at pressures close to and far from the critical pressure points. The ways in which saturation pressure affects frequently bubble development have been investigated. When water is heated to over 15 K, a divergence from the sporadic bubble release is seen. Additionally, the impact of heat flow on instability was studied. For water in close-to-critical state, it has been discovered that a drop in super-heat between 15 K and 10 K causes fluctuations that affect the length of the ebullition cycle.

In 2010, a computer simulation of a vapor bubble boiling in a sub-cooled pool was achieved by Wu and Dhir [17]. By contrasting the findings of the bubble growth domain, while considering bubble departure diameter, the numerical process combining level-set function with moving mesh technique was proven to be effective. For various sub-cooling and varied gravity levels, the estimates of bubble dynamics and heat transmission are shown. The drop in gravity for a certain sub-cooling reduced the heat transfer around the bubble.

In 2016, the progression dynamics of a bubble population were studied by Marchal et al. [18] in a yield stress fluid matrix (a liquid medium (bitumen). A uniform generation of gas was used to drive the bubble population. The situation involves the gradual expansion of bitumen barrels over an extended period of time, inside of which radioactive salts were distributed. Radioactivity results in the creation of hydrogen in a consistent volume throughout the radiolysis of bitumen chains. The results ascertained that the utilised fluids can deform indefinitely if they are subjected to stress over a specific value. Furthermore, it has been demonstrated that, in the event that the population is stationary, yield stress interrupts the classic paradigm of nucleation, bubble formation, and Ostwald ripening in a Newtonian fluid. The study by Marchal et al. [18] was characterised by the use of two different nucleation models (threshold nucleation, continuous nucleation) to explore the impact of the yield stress on the most important metrics of the settling time to the steady state, the max. swelling, and the stationary swelling, as represented in Figure 1, Figure 2 and Figure 3, respectively. These figures indicate that the two nucleation models produce the same steady state swelling of up to 6 Pa of the yield stress (Figure 2).

Goel et al. [19] used high-speed vision tests with sub-cooled de-mineralized water at 1 atm under nucleate pool boiling conditions to evaluate the bubble departure features such as the bubble departure frequency and diameters. To achieve the parametric examinations of bubble departure behavior, the water pool was designed with dimensions of 300 × 135 × 250 mm and four distinct heating components. The results indicated that the degree of sub-cooling (T_sub = 520 K), super-heat (T_sat = 110 K), as well as heat flux, parameters of heater configuration, heater inclination, and heater surface roughness were studied. It was revealed that the heater size, inclination angle, and wall super-heat all increased the departure diameter while liquid sub-cooling and surface roughness dampened it. It was also exposed that the departure rate reduced with an upsurge in heater size but enlarged wall superheat and inclination angle.

Thermal cavitating flows in liquid nitrogen surrounding a 2D hydrofoil were inspected by Zhang et al. [20]. The mass transfer homogeneous cavitation model connected to the energy equation and the RNG k-ε turbulence model with an adapted turbulent eddy viscosity were utilised to account for thermal effects. The Clausius-Clapeyron equation was utilised to enhance the saturated vapour pressure in the cavitation model process. Particularly in the thermal area, the enhanced Zwart–Gerber–Belamri model comes to an agreement with the experimental data of Hord et al. of NASA, as established by the expected pressure and temperature within the cavity under cryogenic circumstances. The cavitation dynamics during the phase-change process were significantly affected by the temperature effect, which may cause a delay or suppression in the onset and development of cavitation behavior.

Pahk et al. [21] invented the creation and dynamic performance of a boiling vapor-bubble that happens under boiling histotripsy insolation. Unambiguously, the researchers observed how the bubble expands and contracts over time. Numerical and experimental studies were attained using a high-speed camera to examine the dynamic of bubbles that were created in optically transparent tissue imitating gel phantoms and subjected to 2.0 MHz of the field of a High Intensity Focused Ultrasound (HIFU) transducer. The results indicated that the shockwave’s asymmetry and water vapor transport can cause incorrect bubble formation, that might be accountable for HIFU-tempted tissue decellularization.

Using in situ synchrotron radiography, Lu et al. [22] studied bubble development, intermetallic compounds (IMCs) dissolution, and their connections throughout heating of an Al-5 wt.% Mn alloy. As the melt was heated, the pores underwent a slow transition from an irregular shape to a subglobose shape, and then eventually a transformation into spherical bubbles. The radii (r) of bubbles 1 and 3 become smaller as time (t) progresses, and the connection between r and t may be effectively described by a Gaussian function in terms of its shape (Figure 4a). Bubbles 2, 4, and 5 all exhibit a rise in their radii with time, with bubble 5 exhibiting the most rapid expansion rate among the three (Figure 4b). With a rise in temperature, the hydrogen should become more soluble in the aluminum melt, causing the bubble size to diminish throughout the course of the heating process and eventually vanish altogether.

In 2020, Zeng et al. [23] exhibited concrete proof for the development of growth styles, shape, mass transfer channels, and lateral growth rate when thickening hydrates on gas (CH₄-C₃H₈) bubbles are suspended in water with inhibitors. These findings were found during the process of thickening growth. During the thickening growth process, an identical and dense-hydrate coating was detected on the bubble as well as at the gas-liquid planar contact. This happened when an inhibitor was not present. The heterogeneous nature of the film instigated a number of circular and cross-shaped craters to perform on its surface. These craters represented as a new conduit for the transfer of mass and sped up the initial thickening and expansion of the hydrate. The hydrate’s viscosity was created in the reaction setup and the capacity of the aqueous solution to spread over the reactor wall was instituted to be connected with the enlargement of the morphology of the hydrate.

Allred et al. [24] created a conceptual framework for the wetting and de-wetting processes that occur throughout bubble generation relying on dynamic contact angles. To accurately illustrate the impact of surface wettability on contact angle dynamics and contact lines throughout bubble development and departure, this structure is integrated into VOF simulation. Computers were used to conduct these simulations. It has been established that the early stages of bubble formation are controlled by the declining contact angle. This happens as the contact line becomes farther and farther from a bubble’s centre. The primary wetting parameter that controls the greatest departure size and contact diameter is the receding contact angle. Better reduced-order models were then constructed, which resulted in correlations between the greatest departure diameter and contact diameter as functions of the dynamic contact angles, independent of fluid properties.

Zhao et al. [25] employed a phase change lattice Boltzmann to assess the impacts of 2-computational fields with constrained space and a free outlet boundary while observing conjugate heat transfer on the whole nucleate boiling. The model introduced was tested, notably in the enhanced convective outlet boundary for multi-phase flow in detailed information, using Laplace’s law and the droplet wetting process. A thorough investigation was carried out into the dynamic behaviors of the whole nucleate boiling process, considering the nucleation, growth, coalescence, departure, ascent, and ultimate rupture. Although growth and departure were significantly assisted when more isolated bubbles coalesced, the dynamic flow behaviors of bubbles’ coalescence are essentially similar. Additionally, the researchers demonstrated that the volume of escalating bubbles in the free outlet field increased over time. It was found that the pressure impact between the inside of the bubble and its surroundings was the main cause of this.

Winder et al. [26] used a stainless-steel framework called the SNS target module which is designed to hold mercury. In order to lessen stresses and prevent cavitation, bubbles of helium are inserted into the mercury. This gas makes it more challenging to precisely guesstimate the physical reaction of the target module. It is essential to have the skill to simulate the reaction of the target vessel before endeavoring to estimate its life. A forceful model for mercury with injected gas bubbles has been offered with the intention of delivering a high-accuracy mercury–vessel reply behavior without the obligatory level of processing expenditures to screen individual bubbles.

Li et al. [27] appraised the influence of thermodynamic effects on the cavitation flow in a liquid oxygen turbo-pump. To couple the physical parameters with temperature, the thermodynamic model based on the Zwart–Gerber–Belamri cavitation model and the shear stress transport k–ω turbulence model were utilised to numerically simulate the entire flow passage. Referring to numerical studies, cavitation mostly occurs at the head of the impeller inlet’s central blades and at the leading edge of the inducer inlet’s suction surface. At low cavitation numbers (σ = 0.07), thermodynamic impacts meaningfully lessen the vapor-phase volume fraction of the cavitation zone; yet, at high cavitation numbers, they increase it.

In 2022, the pool boiling tests were employed on vertical surfaces by Zhou et al. [28] in sub-cooled and nearly saturated conditions. Deploying a high-speed camera with great time-based and latitudinal resolution, the behavior of bubbles on micro-pin-finned surfaces was considered. Plentiful forms of bubble growth on many surfaces were created as a result of this research, which observed the impacts of the micro-pin-fins on the bubble creation and collapse. When scheming the bubble development rate, the influences of heat transfer from the micro-pin-fins and thermal boundary layer were considered. Further investigation was done to see how surface tension, reaction forces, and inertia affected the movement of bubbles. Following these components, a model for the growth and motion of bubbles was shaped. In this aspect, the micro-pin-fins used three key mechanisms to withstand bubble collapse. These include the inertia deduced by the variation in bubble growth rate, the pin influence on the contact radius, and the response force caused by the local differential pressure.

Lai et al. [29] formed a model for the construction of a single bubble in liquid hydrogen, while bearing in mind how temperature is disseminated throughout the bubble. By simultaneously resolving the Rayleigh Plesset equation, the thermal equilibrium correlation, the thermal diffusion correlation, and the heat conduction correlation in semi-infinite space, one may define and forecast the formation of a single bubble in liquid hydrogen. The model investigates the growth pattern of the bubble radius, the bubble radius growth rate, the vapor pressure, the thickness of the thermal boundary layer, and the temperature differential between the boundary and the centre; it inspects how variables like the liquid’s temperature and the surrounding pressure affected the growth of a single bubble in liquid hydrogen. It was possible to examine if a single bubble may transit from experiencing dynamic expansion to facing thermal growth by comparing the important moments of the numerous physical metrics described above.

Cheng et al. [30] built an examination setup with an observable flow channel in order to study bubble generation in vertical rectangular narrow channels. At the Onset of Nucleate Boiling location, bubbles were discovered at one atmosphere in sub-cooled flow boiling water. Two different common bubble development schemes were chosen to be simulated using numerical methods. During the experiment, thermocouples and a high-speed camera were utilised to capture the bubble shapes as well as the wall temperatures. Approximately 30% of the total amount of evaporation is attributed to the microlayer evaporation that takes place close to the base of the bubble.

3.2. Solid–Gas Studies

Hu et al. [31] outlined the Phase Field Model (PFM) of gas bubble growth that comprises micro-structure dependent cluster dynamics to discover the performance of gas bubble swelling in the recrystallization zone of a solid matrix Uranium Monolithic (UMo) fuel. The PFM is an influential numerical approach for simulating multi-phase flows with complex interface dynamics. It can precisely capture the interface between various fluids, handle complex geometries, and allow for easy implementation of boundary conditions. The PFM includes solving the model’s equations that recognize the progress of the scalar field representing the interface, along with the motion’s equations for the fluid-phases. This model was then utilised to discover gas bubble growth. The phase field coupled model permits the systematic simulation of the effect that the rate of defect creation, the rate of clustering, the rate of interstitial emission, and the rate of sink rates on grain boundaries have on the advance of gas bubbles. The findings specified that interstitial clustering is the prominent key physical process that leads to rapid gas bubble swelling kinetics in the recrystallization zone.

Zhou et al. [32] utilised molecular dynamic simulation to search for the dynamic progress features of a helium (He) bubble throughout spallation in single crystal aluminum (a solid matrix) and its consequences on void nucleation growth and thermomechanical features. Before and after the bubbles merge, the connection between bubble radius and time is nearly linear, and the expansion rates that are parallel to those rates were instituted. The expansion towards adjoining bubbles sources an overlap of plastic deformation zones, which endorses local melting. This happens due to the bubbles’ proximity to one another. The bubble avoids the nucleation and development of voids in the surrounding area, and when the expanding bubble comes into contact with developed voids, it absorbs them. This suppression becomes more operative as the bubble size or density is bigger, but it becomes less operative as the shock force grows stronger. When compared to the sample without He bubbles in solid state, this one has a much lower number of nucleated voids and a meaningfully smaller average radius of voids. Following the melting process, there is a destruction of the continued nucleation of voids, which grounds the radius to rise somewhat, while concurrently causing a lesser drop in quantity.

In 2020, the growth process of nano-solid structured cobalt foams was investigated by Arévalo-Cid et al. [33]. These nanostructured cobalt foams were generated via hydrogen bubble template electrodeposition. In order to accomplish this goal, cobalt foams have been created utilising a diversity of electro-deposition periods and inspected using a variability of characterization approaches, namely confocal microscopy in reflection mode and scanning electron microscopy (SEM). Throughout the creation of the foam, agar-agar was mixed into the electrolytic bath to examine the significance of the chemical additives on the growth process and concluding qualities of the foams. Figure 5 introduces the combination of agar occasioned in a successful adjustment of the micro-structure of the final foams. This resulted in a denser, more porous structure that had a lesser pore area and a slower rate of growth, which decoded into improved mechanical features.

In 2022, the time evolution of an inter-granular bubble was reconstructed by Prudil et al. [34] utilising the Included Phase Model (IPM). The IPM involves resolving a number of partial differential equations that define the dynamics of the dispersed phase, including transport, diffusion, and reaction processes. The model incorporated complicated interface morphology while accounting for the creation and associated transit of gas atoms and vacancies. Interfacial energy, internal energy, and elasticity are the driving forces behind these two processes. The four processes that the model predicts ultimately resulted in the generation of linked high-aspect ratio bubbles on the grain face are over-pressure, bubble development, coarsening, and coalescence. The results compared favorably with both the CAGR/UOX/SWELL SEM data and the current SIFGRS model when sets of 250 bubbles were simulated over time using the model’s computing efficiency. Analysis of the simulation’s results revealed that the two-point autocorrelation function captured the development of an inter-connected network, perhaps leading to improved release thresholds. The findings of this investigation further showed the significance of bubble density as a measure of the bubble structure created by coalescence within a solid matrix. Furthermore, this study ascertained that the IPM is a powerful numerical technique for simulating multi-phase flows. It can accurately capture the dynamics of the dispersed phase, and its impacts on the continuous phase, while handling high volume fractions and complex physical phenomena.

Table 1. Outline of research on bubble growth indifferent applications.

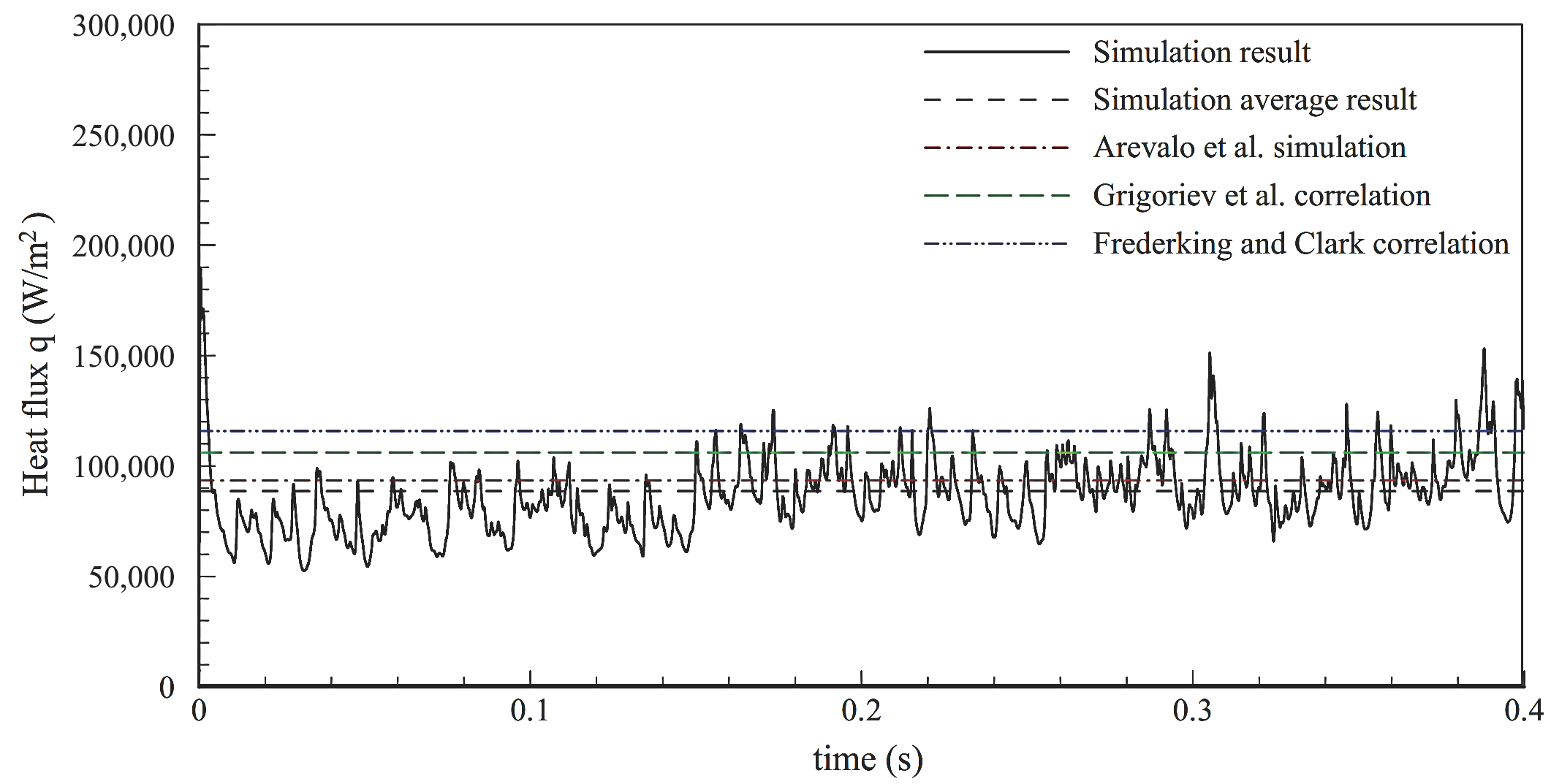
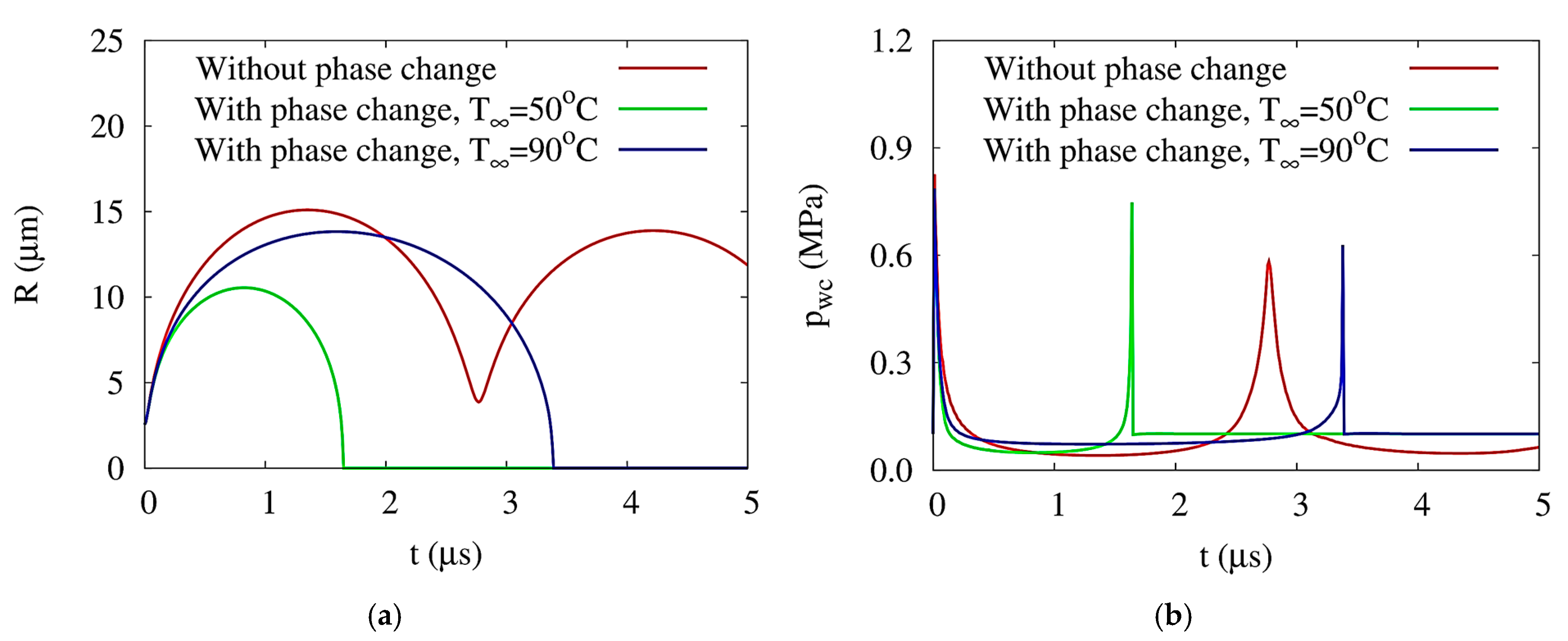
Authors (Year) [Reference]	Sector/Area of Process Engineering	Configuration	Medium	Type of Study	Parameters Under Investigation	Highlighted Findings
Agarwal et al. (2004) [14]	Thermal conductivity and specific heat of vapor	Film boiling and bubble formation in water.	Water	Numerical	Effect of film thickness.	It is discovered that the scale and position of minimal film thickness are related to the location and magnitude of greatest heat transfer from the wall surface.
Mukherjee and Dhir (2005) [15]	Bubble dynamics and wall heat transfer	Lateral bubble merger throughout transition from partial to fully-developed nucleate boiling.	Water	Numerical	Effect of merger of multiple bubbles.	The overall wall heat transmission is considerably increased when numerous bubbles merge.
Tomar et al. (2005) [16]	Incompressible two phase flows with surface tension	Modelling incompressible two phase flows with surface tension.	Water	Numerical	The impact of heat flux on the instability.	When the superheat is reduced from 15 to 10 K for water that is almost at critical condition, oscillations with sub-harmonics affect the length of the ebullition cycle.
Wu and Dhir (2010) [17]	Bubble boiling in a sub-cooled pool	Vapor bubble in sub-cooled pool boiling.	Water	Numerical	Effect of gravity.	The heat transfer around the bubble reduces while gravity dropping for a given sub-cooling.
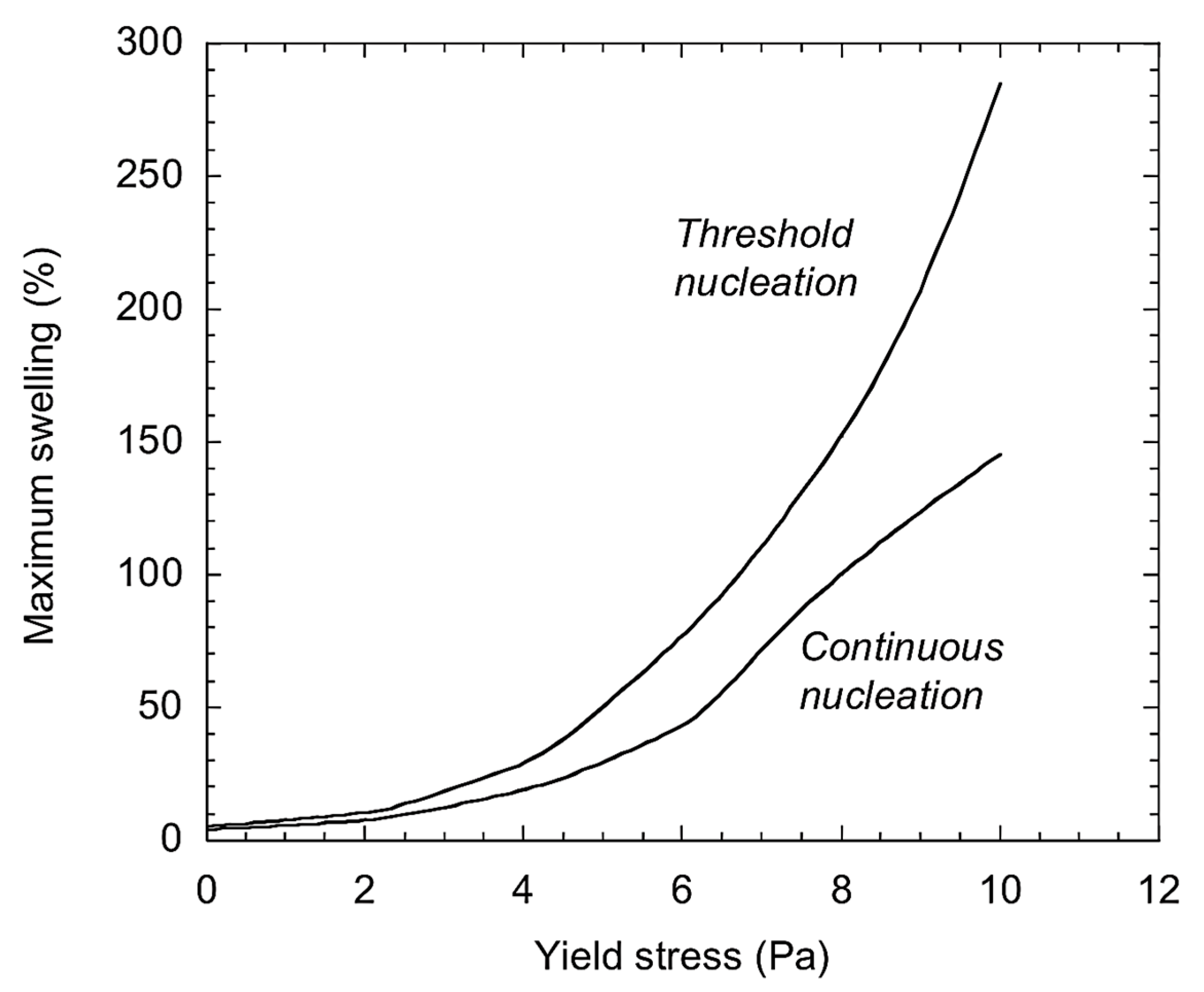
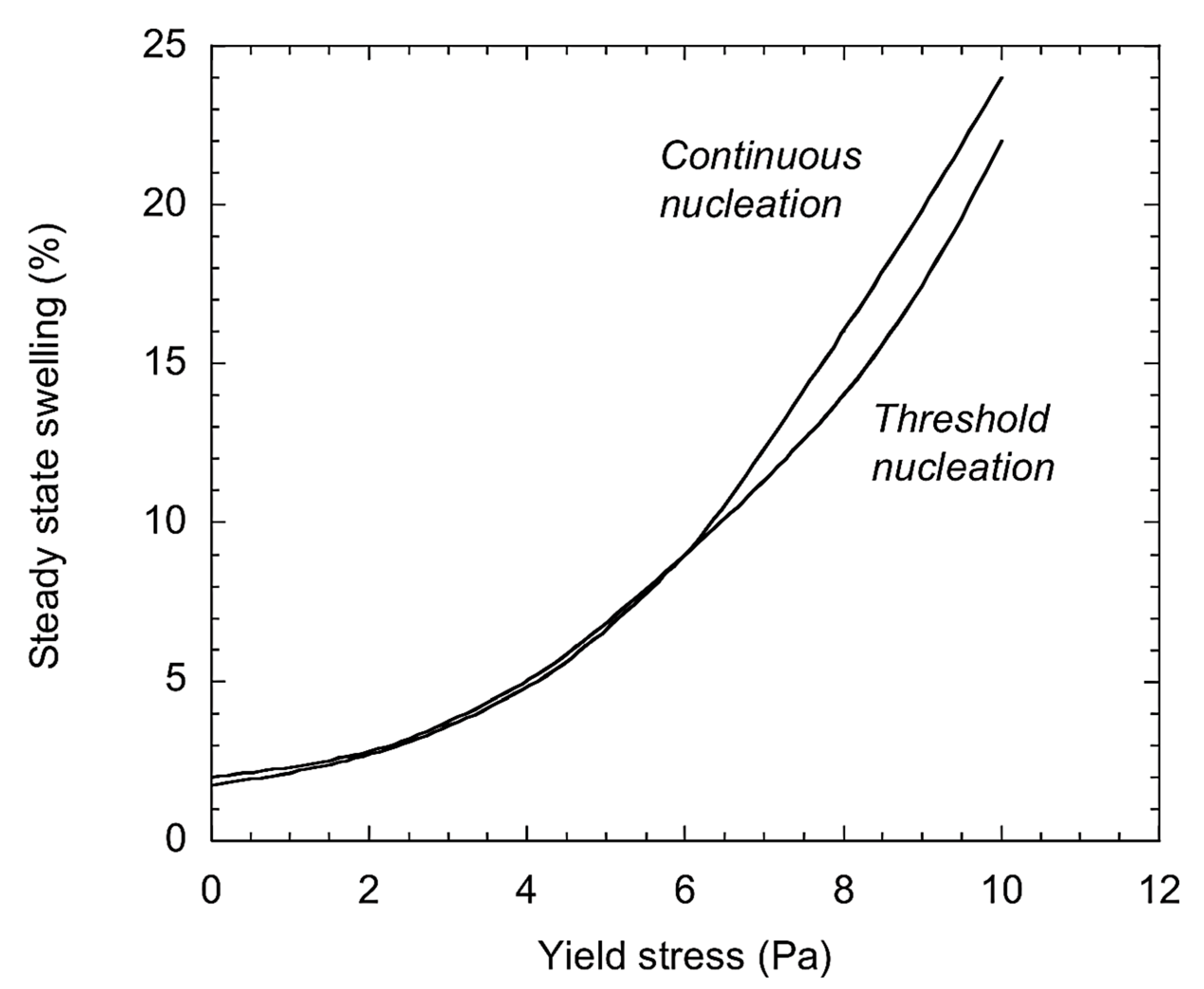
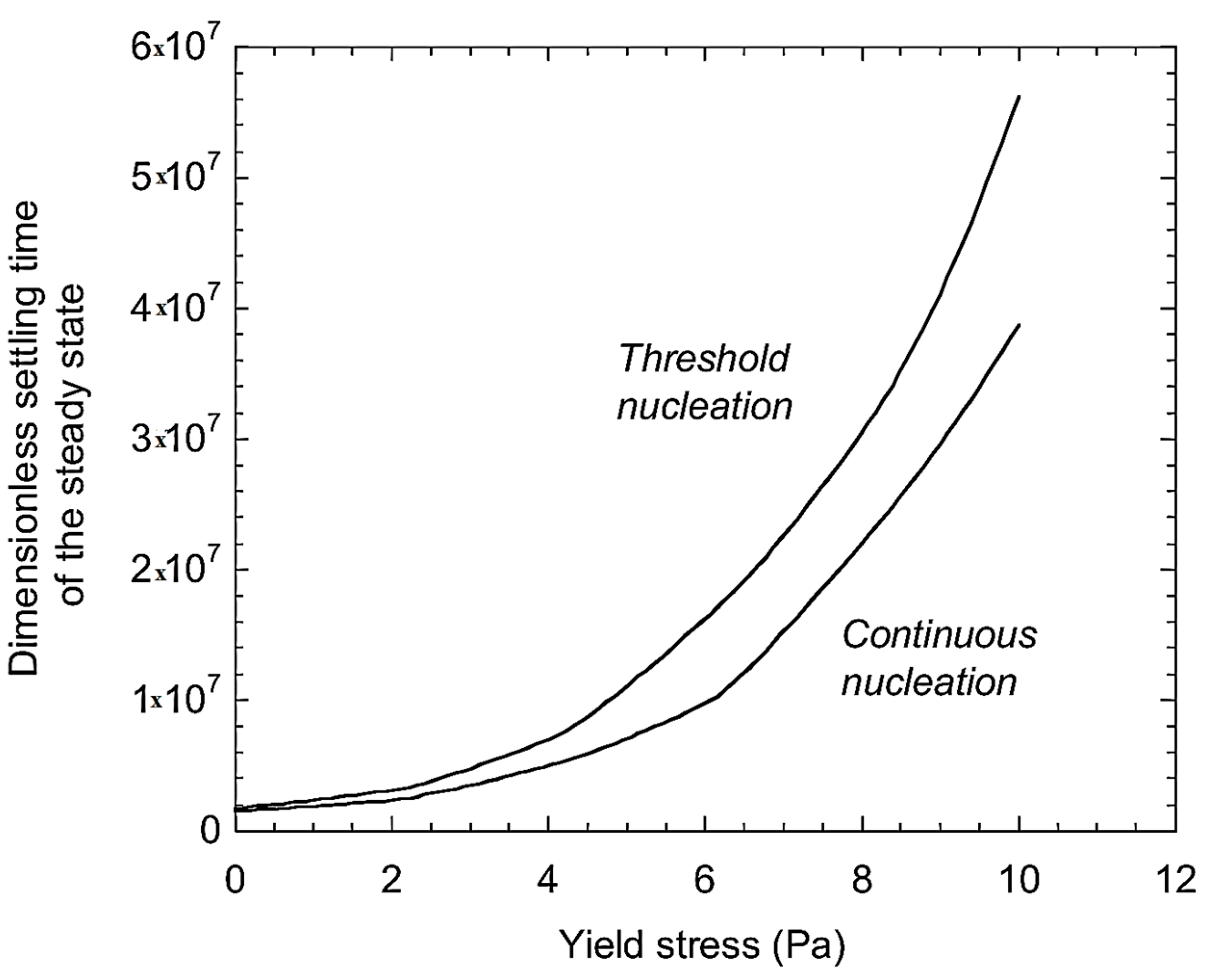
Marchal et al. (2016) [18]	Macroscopic swelling of bitumen drums	In a fluid matrix under yield stress, consistent gas generation drives the population of bubbles.	Yield stress fluids	Numerical	Impact of yield stress value.	In a static population (no movement owing to buoyancy), bitumen’s yield stress disrupts the conventional state of nucleation, bubble development, and Ostwald ripening in a Newtonian fluid.
Goel et al. (2017) [19]	Water pool	Sub-cooled demineralized water at one atmosphere.	Water	Experimental	Effect of wall super-heat, heater size and the inclination angle.	It was discovered that the heater size, inclination angle, and wall superheat all increased the departure diameter while liquid sub-cooling and surface roughness dampened it.
Zhang et al. (2018) [20]	Thermal cavitating flows.	Cavitating flows in liquid nitrogen around a 2D hydrofoil.	Liquid nitrogen.	Numerical	Thermal effects.	The cavitation dynamics during the phase-change process are greatly affected by the temperature effect, which may deduce a delay or suppression in the onset and evolution of cavitation behavior.
Pahk et al. (2019) [21]	Boiling histotripsy exposure	Boiling histotripsy insolation causes a vapor bubble to boil.	Viscoelastic medium	Experimental and Numerical	Shockwave asymmetry and water vapor transportation.	Asymmetric shockwave and water vapor transfer may cause corrected bubble development, which may cause HIFU-induced tissue decellularization.
Lu et al. (2020) [22]	In-situ synchrotron radiography for heating an Al-5 wt.%Mn alloy	In situ synchrotron radiography, bubble development, IMC dissolution, and interactions occur throughout heating of an Al-5 wt.% Mn alloy.	Intermetallic compounds	Experimental	Effects of bubble existence.	A logistic model may explain IMC dissolution, which bubbles can speed up.
Zeng et al. (2020) [23]	Hydrates in different reaction systems	On a gas bubble (CH4-C3H8) floating in water with inhibitors, hydrates are thickening in growth.	Kinetic inhibitors added in the water	Experimental	Hydrate growth mode impacts the inhibitor concentration.	Since hydrate particles do not form films, they will not hinder flow substantially.
Allred et al. (2021) [24]	Boiling surface wetting	During bubble development, wetting and dewetting processes depend on the dynamic contact angles.	Wetting surface	Numerical	Contact angle.	The early stages of bubble development are controlled by the receding contact angle, which also establishes the greatest contact diameter and departure size as the contact line moves away from the bubble centre.
Zhao et al. (2021) [25]	Nucleate boiling	Boiling of the whole nucleate.	Convective and confinement space with solid wall boundary condition	Numerical	The pressure difference.	Coupled pressure from the bubble’s interior and surroundings causes the volume of bubbles in the free outlet domain to increase.
Winder et al. (2021) [26]	Sudden energy input	Mercury is filled with helium bubbles to lessen stresses and cavitation in the SNS target module.	Structure containing liquid and gas	Numerical	The energy deposit and helium gas injection into the mercury.	The protons’ deposited energy has the potential to cause harm to the target. It is possible to lessen the harm by injecting helium gas into the mercury. On the other hand, the addition of this gas makes the bodily reaction more complicated.
Li et al. (2021) [27]	Turbo-pump.	Cavitation flow of a liquid oxygen turbopump.	Liquid oxygen.	Numerical	The influence of thermodynamic effects.	At low cavitation numbers, the thermodynamic effects significantly decrease the vapor-phase volume fraction of the cavitation zone. Hitherto, at large cavitation numbers, they increase it.
Zhou et al. (2022) [28]	Nucleate pool boiling	Experiments on vertical surfaces with pools boiling in subcooled, almost saturated environments.	Vertical surfaces	Experimental and Numerical	Location of the bubble, size of the micro-pin-fins, pin effect, and force of response.	The formation of bubble and motion, as well as its departure time and diameter, are all influenced by the size of the micro-pin-fins. Through the pin effect and response force, micro-pin-fins encourage bubble departure.
Lai et al. (2022) [29]	Liquid hydrogen delivery	Liquid hydrogen single bubble growth model with internal temperature distribution.	Liquid hydrogen	Numerical	The impact of ambient pressure and super-heat on the development of a single bubble in liquid hydrogen.	Comparing the crucial times of the various physical indicators outlined above sheds light on the process by which a single bubble might go from experiencing dynamic expansion to experiencing thermal growth.
Cheng et al. (2022) [30]	Compact type reactors	Bubble development in small, vertical, rectangular channels.	Vertical rectangular narrow flow channels	Experimental and Numerical	Investigate the behaviour of bubble growth in vertical rectangular narrow channels.	Approximately thirty percent of the entire quantity of evaporation may be attributed to the micro-layer evaporation that occurs at the bubble’s base.
Hu et al. (2020) [31]	Polycrystalline UMo fuels	Gas bubble evolution incorporating cluster dynamics based on microstructure.	Irradiated UMo fuels	Numerical	Rates of defect clustering, interstitial emission, defect production, and sinking.	Gas bubble swelling kinetics rises with defect formation, interstitial emission, and clustering rates, but decreases with grain boundary defect sink rates.
Zhou et al. (2020) [32]	Spallation of aluminium	During spallation, a helium bubble forms in a single Al crystal.	Aluminium	Numerical	Size, density, and shock resistance of the bubbles.	The bubble prevents the formation and growth of near voids, and its expansion absorbs grown voids. Suppression increases with bubble size or density and decreases with shock strength.
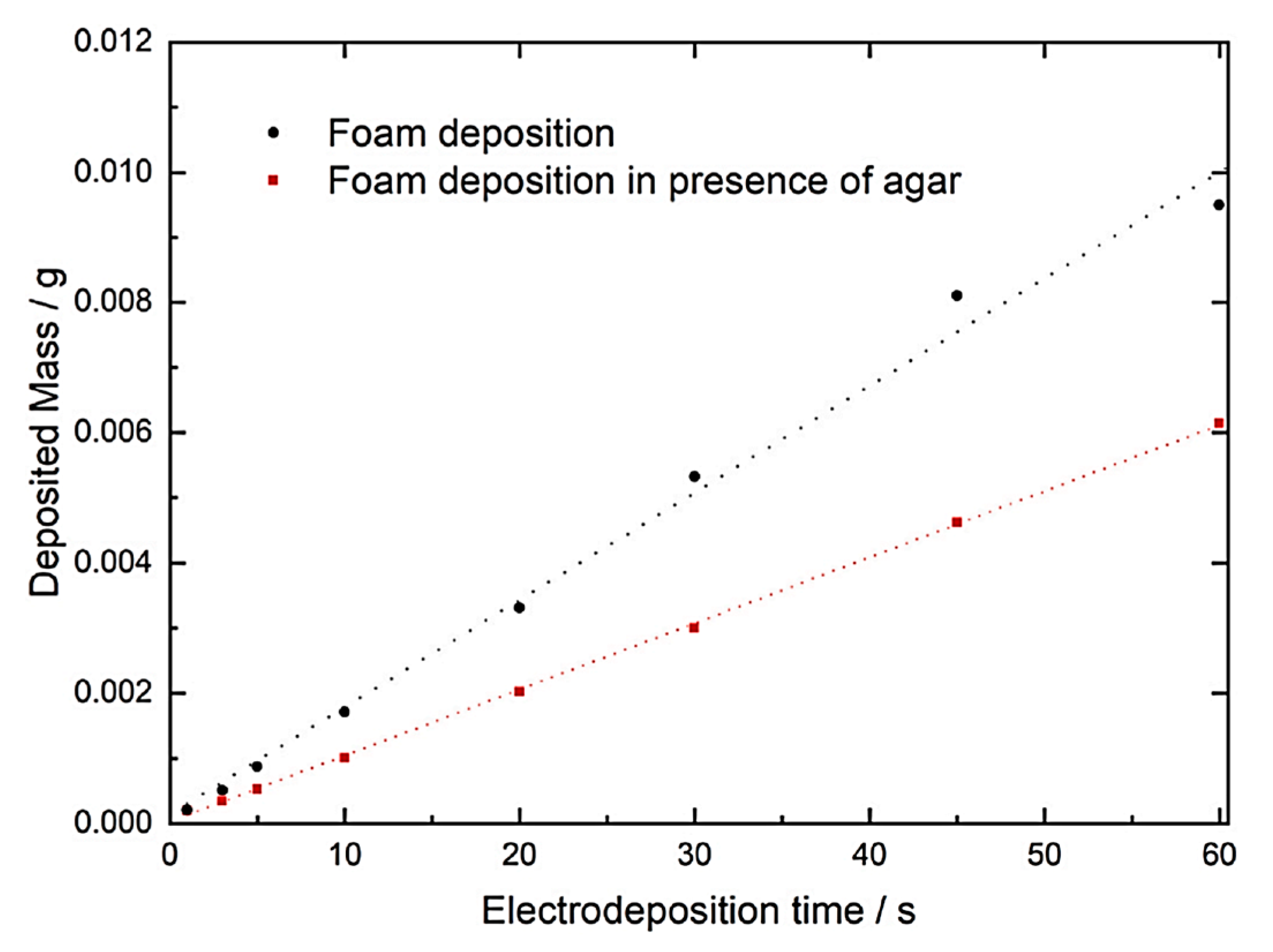
Arévalo-Cid et al. (2020) [33]	Hydrogen bubble template electrodeposition	Cobalt foams with nanostructures created via hydrogen bubble template electrodeposition.	Nanostructured cobalt foams	Experimental	The addition of agar.	Agar has effectively affected the micro-structure of the final foams, providing a denser porous structure with smaller pore area and slower growth rate, which improves mechanical qualities.
Prudil et al. (2022) [34]	Populations of intergranular fission gas bubbles	Bubble development, coarsening, and coalescence lead to connected, high-aspect-ratio bubbles on the grain face.	Oxide fuels	Numerical	Populations of intergranular fission gas bubbles that are significant.	Bubble density is a metric of bubble structure shaped by coalescence, and the two point auto-correlation function records the expansion of a connected network, that might decrease release thresholds.

depicts the summarization of the associated research on bubble growth studies while distinguishing the most interesting results.

According to the studies that were previously addressed, the creation of corrected bubbles may be the source of HIFU-induced tissue decellularization due to the potential imbalance in shockwaves and the transfer of water vapor. One may have a better understanding of the procedure by which a single bubble converts from dynamic development to thermal growth by linking the critical moments of the aforementioned physical indications. Furthermore, a cluster dynamics and phase field model can be stretched to train the accompanying growth of defect and second phase precipitates often detected in treated materials.

4. Bubble Collapse Studies

Plentiful academics have, over the past few decades, attempted to recognize the fluid dynamical mechanisms in charge of the impulses connected to the cavitation collapse of bubbles. However, it has been proved that at the three distinct processes—including the shockwave, the splashing properties, and the impinging liquid jet—are at work here. This section proposes conducting a critical assessment of the associated studies on bubble collapse in a wide range of industrial applications.

In 2013, Gong and Cheng [35] conducted numerical simulations in two dimensions using the novel phase-change lattice Boltzmann method (LBM) to study the nucleation of water on a micro-heater with constant heat flux and constant wall temperature, respectively. Under conditions of constant wall temperature, the effects of gravity, contact angle, and superheat on bubble departure diameter and release duration were tested. The analysis included the three-phase movement of the vapor bubble’s contact line, as well as the flow fields and temperature profiles both within and outside the bubble during the boiling process.

In 2015, using wavelets and Fourier transforms to analyse the piezoelectric waveforms, Navarrete et al. [36] investigated the dissipation of acoustic energy. The main experimental findings show that as compression progresses: (1) the liquid meniscus attains the conical zone in cavitation conditions; (2) the liquid meniscus undertakes a symmetrical alteration from two dimensions to three dimensions, becoming a “bulb with a nozzle”, where the instabilities are drawn and controlled; (3) the nozzle is maintained as part of the new meniscus that persists in pushing the gas pocket; and (4) furthermore, as the collapse process proceeds, the bulb eventually changes into a cloud of bubbles, and the bubble structures display their distinct expansion–contraction rate. The authors arrived at the conclusion that the breaking of a conical bubble activates a variety of distinct processes for the emission of light after looking at the dynamics and acoustics.

In 2016, Gong and Klaseboer [37] developed a fluid–solid integrated algorithmic scheme to consider the relationship between a deformable viscoelastic solid and a collapsing bubble. A Zener type viscoelastic model was utilised using the linked Boundary Element (BE) and Finite Element Method (FEM) to achieve this. The FEM was utilised to determine the viscoelastic solid response to the spontaneous pressure brought on by the bubble’s collapse, whereas the boundary element method was utilised to mimic bubble growth, contraction, and collapse. The Zener type viscoelastic model was employed to appropriately accommodate for the change in a viscoelastic solid’s stiffness over time as a result of pressure. The study indicated how variables, including the solid’s viscoelastic properties, the range of max. bubble sizes, and the locations of the bubbles affect the viscoelastic dynamic response of a solid.

In 2016, Tang et al. [38] conducted a study on the impact of an ultrasonic field on the deflation and condensation of vapor bubbles in a sub-cooled pool that was at rest. The effects of the ultrasonic field were examined in this study. When outcomes were introduced at liquid sub-cooling temperatures of 15–30 K, the vapor bubbles progressively disintegrated, but at temperatures over 40 K, they collapsed into a significant number of smaller bubbles. The limit of liquid sub-cooling for bubble break was lowered to 20–26 K while utilising ultrasonic vibration, causing capillary waves to appear on the bubble surface. The enhanced sensitivity of bubbles to the ultrasonic vibration made this possible. Additionally, the region of the bubble that was in touch with the cold bulk liquid was extended due to the capillary wave’s existence. The temperature boundary layer close to the vapor–liquid interface was also disturbed by the capillary wave’s presence, which augmented the condensation process. Accordingly, at the same amount of liquid sub-cooling, the liquid’s inertial shock to the vapor bubble with ultrasonic vibration was a bit more robust than what would have been detected in the absence of ultrasonic vibration, as proved in Figure 6.

In an underwater launching test, Wang et al. [39] testified to a distinct internal collapse mechanism that happens from the tail to the head of an axisymmetric projectile. This collapse phenomenon was discrete from other collapse scenarios and is on occasion seen in water tunnel experiments. The cavities swiftly contract upstream, which results in high-impact pressure. Moreover, fast re-entry jets occur, which causes substantial instability. Referring to the findings of the analysis, this phenomenon is associated with sudden changes in the cavitation number and vehicle speed. The quick acceleration of the vehicle causes the creation of thin cavitation bubbles, and the consequent rapid condensation of vapor during the deceleration phase results in the formation of an extra body force at the point where the cavity is closed. Hence, a major amount of velocity that is engaged toward the wall is created, and the layer of liquid water that is moving toward the wall remains to travel toward it and collide with it as an interior collapse. Additionally, numerical calculations were achieved with the effect of slowing down the development of the cavity. Referring to the findings, an enlargement in pressure was a crucial component, which brought about a nonlinear shift in the cavity’s overall length.

Qin and Alehossein [40] introduced a model of heat transfer allowing for the use of the Rayleigh/Plesset (RP) correlation and Computational Fluid Dynamics (CFD) with the aim of precisely forecasting the change of temperature and heat transfer that happens throughout a bubble’s collapse. CFD comprises solving a number of equations that outlines the dynamics of the fluid phases and their collaborations, while utilising numerical approaches. A two-phase compressible CFD model was formed to correctly mimic the bubble collapse to establish the changes in velocity, temperature, and pressure distribution between the liquid and the bubble. After being revised to take into account the impacts of radiation and conduction, the RP equation’s conclusions mostly settled with those of the numerical CFD. Further investigation was achieved on the temperature rise and collapse of bubbles, the rate of heat transmission by radiation and conduction, and the cumulative heat transfer of bubbles of altered sizes. It was detected that the bubble burst more violently as the initial max. bubble size was increased. The max. temperature was extended inside the bubble, which quickly enlarged, as depicted in Figure 7, along with the amount of heat that was accumulated and stored. The authors also confirmed the superiority of CFD as an influential instrument for appreciating and predicting the behaviour of complex multi-phase flows. The simulations are carefully validated against experimental data.

Li et al. [41] calculated the dynamic bubble pressure using the Potential Flow Theory (PFT) and BEM. PFT is an analytical technique that describes fluid flow as an irrational and inviscid fluid motion. This technique assumes that the fluid particles move in such a way that the velocity vector at each point is the gradient of a scalar potential function. The pressure was specifically estimated using the Bernoulli equation and the auxiliary function approach. The potential function satisfying Laplace’s equation is defined such that the fluid velocity and pressure at any point in the flow can be obtained from the potential function. The BEM involves discretizing the boundary of the domain into a set of components and approximating the solution to the governing equations within each element. Bubble-induced pressure had two sources, and dynamic pressure had two portions. The first component, $\mathsf{\rho }$g, monitored the uneven pressure between bubble gas and ambient flow, which contributes to dynamic pressure. The second term of decomposed pressure, pm, is created via bubble motion, which evaluates jet impact. $\mathsf{\rho }$g fluctuation mirrored gas pressure. After the jet impact, pm at the wall centre peaks and subsequently drops owing to jet velocity and may increase when the toroidal bubble migrates into the wall.

Rowlatt and Lind [42] prolonged the Spectral Element Marker Particle (SEMP) technique to integrate a third fluid phase to investigate the bubble collapse problems in a fluid–fluid interface. The method is thought to be validated when a two-phase bubble explodes close to a stiff barrier. SEMP involves resolving a number of partial differential correlations that define the dynamics of the fluid and solid phases, including momentum conservation, mass conservation, and energy conservation. A wide set of fluid parameters and geometric arrangements were considered for understanding the impact of bubble collapse in bioengineering applications. In an effort to gain visions into the flow dynamics behind sono-poration and microbubble improved targeted drug administration, the authors considered a straightforward model of collaboration between the bubbles and cells. The bubbles deflated into spherical shapes at higher interface heights away from the rigid wall as a direct outcome of the compact contact that existed between the stiff wall and the bubbles. Indeed, SEMP has allowed for accurate modelling of the dynamics of the fluid–solid phases and can handle complex geometries.

Ye and Zhu [43] suggested the effect of a near wall acoustic bubble collapse micro-jet on an aluminum 1060 sheet, the cavitation threshold formula, and micro-jet velocity formulation. The Johnson Cook rate equation was used to create a 3-dimensional fluid–solid coupling model of the micro-jet effect on a wall. The utilised ultrasonic pressure amplitude was far higher than the liquid cavitation threshold, causing cavitation in the liquid. Micro-jets caused surface micro-pits. Clearly, micro-jet velocity affected the wall pressure. Ultrasonic cavitation examination evaluated tiny pits generated by micro-jet on aluminum 1060. Inversion analysis showed that pit diameter-to-depth ratio affected equivalent stress, equivalent strain, impact strength, and micro-jet velocity.

In 2017, calculations were made by Pavlov [44] to acquire the kinetics of a bubble’s collapse under the influence of an abruptly utilised external pressure. The bubble was initially in a state of thermal and mechanical balance with the nearby liquid. The condensation of vapor inside the bubble correlates to the bubble’s compression creating a pressure of saturated vapor inside the bubble at the interface temperature. Adiabatic processes are likely to cause a temperature increase in the main body of the bubble. Both the heat fluxes field and the temperature in the instant area of contact were deliberated. The mass flow coming from the bubble was calculated from the thermal balance. The assumption used in the calculation of the liquid’s pressure field was that the liquid is both incompressible and non-viscous. Several equations associated with the radius of bubble, compression rate, and internal vapor pressure were developed.

In 2017, a numerical simulation of the collapse of a cavitation bubble enclosed inside a diesel droplet was performed by Ming et al. [45] using the volume of fluid (VOF) method of the open source CFD toolbox OpenFOAM. The outcome exhibited that the collapse process was composed of several phases of rebound and collapse, which is alike to the vibration process of a damping spring oscillator. Figure 8 depicts that the pace of collapse accelerates as the pressure of the environment around it rises, whereas Figure 9 depicts that the collapse’s rate accelerates as the pressure of the saturated vapor falls. The impact of the surrounding pressure on the collapse process is far greater than the impact of the saturation vapor pressure.

Rosselló et al. [46] created a water hammer to achieve managed bubble collapses. A laser-generated bubble is enlarged and then squeezed utilising an electro-mechanical piston. It permits high energy concentrations in collapses and autonomous regulation of system parameters. To expand experimental findings, simulations of prototype bubble dynamics were conducted. The expansion ratio, consisting of the increased bubble radius over the equilibrium bubble radius, was the most important metric for collapse strength.

Phan et al. [47] developed an algorithm for compressible interface flows with and without mass transfers. The mathematical technique was built upon dual time fully compressible multi-phase homogeneous mixture flow modelling. The authors measured the boundaries that showed a substantial change in velocity, density, pressure, and temperature while deploying a compressive high-resolution interface capturing technique. It was possible to observe the formation of the wave-like boundary between the liquid and vapor around the sphere as the film boiled. The average heat fluxes generated by the simulation were contrasted against forecasted ones using analytical correlations and numerical data. Also considered were the impacts of the superheated wall on the heat fluxes. A pattern similar to the one seen in the previously published data was discovered as a result of a bigger heat flux on the sphere caused by a rise in the superheated wall’s temperature (Figure 10).

Kerabchi et al. [51] introduced computational results while considering the impact of depths between 0 and 10 m on acoustic cavitation. The study provided particular attention to the impact of the ultrasonic wave’s attenuation in regard to the dramatic conditions that emerged inside the bubbles as they burst. The liquid’s surface tension, compressibility, viscosity, depth, and attenuation of the ultrasonic wave are all reflected by the mathematical model that was utilised. The greatest pressure (p_max) and bubble temperature (T_max) at the bubble collapse were instituted to meaningfully decrease when the depth of the water was increased up to 10 m, with the ultrasonic wave attenuation significantly contributing to the overall reduction event. However, when the bubbles were under the same pressure, this was the case. Lower temperature (10 °C) and higher frequency (1000 kHz) caused the decrease in T_max and p_max with depth to be more dramatic; in these conditions, losses beyond 72% in T_max and up to 94% in p_max (as opposed to values at z = 0) were achieved at z = 10 m. When the temperature and frequency were lower (10 °C) and higher (1000 kHz), the decrease in T_max and p_max with depth was more dramatic.

In 2019, a Smoothed Particle Hydrodynamics (SPH) solver was established by Joshi et al. [52] to model the deflation of a single cavitation bubble near an elastic plastic material and examine the origins of plasticity which resulted in material degradation. The results highlighted the significance of the material deformation caused by the micro-impact jets and the shockwave produced during the collapse. An event dominated by shockwaves has the potential to degrade material at a far faster rate than a micro-jet hit. It was found that, in the case of the strain rate of stainless steel A2205, there is a significant impact on plastic deformation that, if ignored, may cause an over-estimation of the plastic deformation by as much as 60%. Additionally, they demonstrated that the biggest plastic strain happens at a radial offset from the symmetry axis, even though the impact pressure is the strongest below the collapsed bubble. This is due to the effects of inertia, which signify an influence on the position and the size of the plastic domain inside the material.

Brujan et al. [53] examined the dynamic of a laser-prompted cavitation bubble utilising 100,000 frame per second high-speed photography. A bubble near a vertical solid wall collapsed into a flat jet. Both radial and planar jets were created in the transition zone. The planar jet penetration and secondary cavitation construction creation were seen throughout bubble oscillation when the bubble diameter at max. expansion was lower than the distance between parallel solid walls. Planar jet height grew with bubble size relative to stiff wall distance. The jet velocity decreased as the bubble size and distance from the vertical wall increased.

In 2019, a unique experimental setup was reported by Hopfes et al. [54]. This novel experimental setup made use of a shock tube and applied a jellylike mixture as a water-like carrier medium. The effects of an instantaneous pressure rise on air bubbles of mm size that were injected in gelatin were investigated from two distinct angles. First, they demonstrated that individual bubbles in the gelatin behaved correspondingly to bubbles in water throughout the collapse of the structure, and that the concentration of the gelatin did not greatly impact the behavior of the bubbles. They also investigated four primary forms of bubble interactions that may also be defined by non-dimensional factors. It was observed that if there was a big size ratio between two bubbles, the interaction between them would either be controlled by the larger bubble if the bubbles were separated or would result in a prominent liquid jet if the bubbles were near to each other.

Bardia and Trujillo [55] offered a mathematical study of the intermediary bubble collapse. In contrast to thermally generated or inertia controlled collapse, intermediary bubble collapse was influenced by both liquid/vapor interfacial heat transfer and surrounding liquid advection. The bubble pressure and interfacial temperature were constantly shifting all the way through the process of this particular kind of collapse, which was one of the most identifying characteristics of this form of collapse. To be more specific, the work demonstrated in a quantitative manner that the collapse behavior shifted, when the process was happening, by a regular rate of pressurization in the adjacent liquid phase, rather than the commonly deployed approximated method of a step change. This was in contrast to the previous approximation, which assumed that the change would occur all at once.

Gan et al. [56] investigated a hull girder constructed to mimic a real warship and subsequently exposed to underwater explosion loads to see how it deformed. Experimental and computational techniques were both used in this study. The study also considered how a non-spherical underwater explosion bubble might affect things. The numerical computation was carried out by Coupled Eulerian–Lagrangian (CEL) method of CFD, and the experiment was carried out in an underwater environment with explosions that occurred in a swimming pool. Analysis of the associated results led to the conclusion that when detonation distances increase, the hull girder’s deformation form shifts from rigid-body motion to whipping, leading to the creation of a plastic hinge. The dynamic reactions of the hull girder will be the same for a range of charge weights when measured at the same dimensionless distance if the charge weights are within a given range.

Chudnovskii et al. [57] directed an examination of the boiling of a substantially sub-cooled liquid in which they explained how the liquid’s local heating by concentrated laser radiation via a thin optical fibre caused the boiling to begin. They investigated the growth of a vapor–gas bubble established at the surface of an optical fibre tip extensively. They presented estimations of these jets’ velocities, which appear to be in perfect agreement with the experiment.

Using a tank of water inside a cold room, Yuan et al. [58] studied the dynamics of a collapsed bubble close to a floating ice cake and its potential to be harmed by a high-speed jet and shockwave. A cryostat produced an ice cake from clean water, which was subsequently managed and positioned in a water tank inside a frigid room. The bubble was simultaneously created at a distance underneath the floating ice-cake by an electric spark that was produced at the same time. The two of them were interacting while being observed and captured by a fast camera. After seizing the bubble’s dynamics, which comprise the bubble jet and the shockwave, the authors calculated the negative impacts of bubble loads on the ice, counting fractures, breakage, and movement. Relying on the level of damage and the propagation of fractures, it was detected that crevasse patterns, radial and circumferential crack patterns, and radial crack patterns were produced on the ice. However, the most distinctive type of damage pattern was crevasse patterns.

Han et al. [59] deliberated the association between two bubbles of diverse sizes as well as the consequence of a wall on the process of bubble collapse using the Volumetric Optical Force (VOFE) microscopy method. VOFE microscopy is a prevailing experimental procedure used to train complex multi-phase flows in three dimensions. VOF microscopy contains and presents particles into the fluid and illuminates them with a laser beam. The laser light exerts optical forces on the particles, which can be utilised to operate their motion and track their trajectories. Thus, VOFE permits the quantification of the velocity, acceleration, and trajectory of individual particles or droplets within the flow. The authors observed connections between two bubbles of diverse sizes in free water without a wall in between. The authors guaranteed that these bubbles would move close to the wall and close to each other as they collapsed when there was a wall nearby. This has specifically occurred concurrently. They revealed that, in both a single bubble system and a double bubble system, the presence of a wall would increase the time necessary for the bubble to rupture. However, the wall’s existence can result in a more powerful process of the bubble collapsing and then rebounding. By inspecting the bubble’s centre of displacement, they revealed that the double bubble system may upsurge the dislocation of both bubbles. The VOFE technique is restricted to studying small-scale flows and requires careful calibration and validation against other experimental procedures to guarantee accurateness and reliability of the results.

Fursenko et al. [60] delivered a simulation of vapor bubble collapse close to a micro/fiber submerged in a sub-cooled liquid to suggest the process of high velocity liquid jet creation. Numerical simulations showed that jet generation is driven by bubble surface dynamics, not bubble shrinkage. The jet’s ideal fibre thickness was discovered.

Tan and Yeo [61] introduced a particle velocity estimate model relying on mass loss. Micro-abrasive particles measuring 5–50 μm were subjected to 20 kHz ultrasonic irradiation in deionized water for 10 min. In a well-controlled experiment, targets positioned 0.5 mm from the ultrasonic horn surface captured pushed particles. A reverse solid particle erosion model may estimate particle impact velocity from specimen mass loss. The results revealed particle velocity was 8–40 m/s and depended on the particle size and ultrasonic amplitude.

Orthaber et al. [62] conducted a number of tests in which they inspected the behavior of a single cavitation bubble on two sides of an oil–water interface. They looked at the specifics of the interaction between bubbles and surfaces (deformation, penetration). The anisotropy (the property of being directionally dependent) parameter makes the correct prediction that the bubble would permanently shoot to the interface if it was formed in low-density liquid. On the other hand, the bubble will continually shoot away from the interface if it was formed within high-density liquid. They expanded the scope of the study to include the correlations between a variety of bubble features and the anisotropy parameter.

Zhang et al. [63] performed an experiment to investigate the dynamics of a laser-prompted cavitation bubble that was near to the edge of a solid wall. The authors used high-speed photography equipment. Three typical scenarios were identified for the purpose of categorizing the phenomenon, relying on the deformation of the bubble interface that happened throughout the process of the bubble collapsing. The movements of the bubble centroid and interface in various directions were identified using a numerical analysis of the captured high-speed photographs. There was visible neck development in the middle of the bubble when it was extremely near to the edge. The drive of the bubble interface at the edge was hampered by this clear neck. In this case, the edge increased the time needed for the bubble to burst by up to 22% over Rayleigh’s entire time requirement (Rayleigh number is large, somewhere around 10⁶ to 10⁸).

Ezzatneshan and Vaseghnia [64] appraised the collapse dynamics of cavitation bubbles of various liquids in the vicinity of the solid surface with variable wettability conditions using a computational methodology based on the pseudo-potential multiphase lattice Boltzmann method (LBM). The simulation of the Redlich–Kwong–Soave equation of state (EoS) with an acentric factor was used to address the physical features of water (H2O), liquid nitrogen (LN2), and liquid hydrogen (LH2). The H₂O, LN₂, and LH₂ fluids were considered while evaluating the cavitation bubble collapse close to the solid wall. Also, the effect of the surface’s wettability on the dynamics of the collapse was tested. The findings showed that the water’s cavitation bubble collapses faster when it comes across a strong liquid jet, but the LN2 bubble collapses more gently because of its bigger bubble radius at the rebound.

Huang et al. [65] explored the acoustic waves caused by the collapse and oscillation of a single bubble. The Schlieren Method was employed throughout the trials to document both the temporal growth of the bubble formations and the equivalent acoustic waves. A three-dimensional (3D) model was employed throughout the computational simulation to train the dynamics of a single bubble. The acoustic waves were the main topics of this study. The results showed that compression waves were produced as a result of the bubble’s rapid growth. These waves progressively steepened into a single shockwave that propagated outward through the liquid, and during the final phase of the collapse of the bubble, another strong shockwave was produced. The liquid field’s pressure and velocity increased following the shockwave. The liquid’s pressure dropped as a result of the rarefaction wave, and its spatial distribution became dispersive. As the distance over which the acoustic waves travel increased, the pressure that they exert and the effect they have on the liquid’s speed were observed to increase.

In 2021, to better understand the method of air entrainment used in the hydraulic aspect to lessen cavitation, Xu et al. [66] examined the influence of a single air bubble on the volume of noise produced by the collapse of a cavitation bubble. When a rising air bubble interacted with an underwater low-voltage electric discharge unit, it resulted in the formation of a cavitation bubble. For this analysis, only circumstances where the hydrophone probe, cavitation bubble centroid, and air bubble centroid were all almost on the identical horizontal line were taken into account. Referring to the findings, an air bubble’s size, roundness, and distance all considerably impacted the cavitation bubble’s direction of collapse and the sound generated pressure. The results also revealed a strong correlation between the direction of collapse and the associated pressure that the collapse produced.

Peng et al. [67] outlined the concept of the collapse of a single and a dual cavitation bubble while utilising the Thermal Lattice Boltzmann (TLBM) and double distribution function (DDF) approach. This approach uses a lattice-based model to simulate the movement of fluid particles and their interactions with solid particles and interfaces. Despite LBM including a thermal lattice model that defines the transfer of heat between fluid and solid phases, LBM affords an exceptional density-to-viscosity ratio. The approach also involves solving a number of partial differential correlations that define the dynamics of the fluid–solid phases, including mass conservation, momentum conservation, energy conservation, and temperature evolution. The correlations were solved using mathematical approaches such as the lattice Boltzmann method, and the simulation findings were authorized against experimental data. The outcomes of the simulation were in decent consistency with those of the theoretical analysis and the experiments. Peng et al. [67] dignified the max. collapse pressure, the lifetime of the cavitation bubble, the maximum collapse temperature, and the max. collapse velocity as corresponding to the distance between the cavitation bubble and the rigid barrier. Moreover, investigations were achieved to determine how the viscosity of the fluid and the starting pressure differential affect the max. temperature at which a system can collapse. The outcomes of the LBM simulation completely reproduced the slowing influence of a hard boundary on the cavitation bubble, the distance between the cavitation bubble, and the hard barrier growth; this resulted in the growth of the max. collapse pressure, max. collapse temperature, and max. collapse velocity.

In 2021, the behavior of cavitation bubbles when they collapse close to walls under conditions of high ambient pressure was highlighted by Trummler et al. [68]. Generic setups with various stand-off lengths were analysed using mathematical simulation with a solver that incorporates phase change. The findings showed that the stand-off distance and the collapse dynamics, the production of micro-jets, the rebound, and the maximum wall pressure were significantly related. The pressure impact data gathered at the wall may be used to establish a connection between cavitation-related material damage and the corresponding collapse processes. The findings on six different grid resolutions were contrasted, and it was exposed that, while the peak pressures were meaningfully dependent on the resolution, the key collapse characteristics were already documented on the coarsest resolution.

Long et al. [69] observed and investigated the collapse and formation of cavitation bubbles created throughout the pulsed-laser ablation of submerged targets in three different liquid environments: water, ethanol, and n-butanol, utilising a stroboscopic shadowgraph system. These bubbles were produced when submerged targets were being ablated by a pulsed laser. The images of collapsed bubble transitory phases were obtained. The bubble volume was at its lowest at this phase. Both ethanol and n-butanol might undergo cavitation-induced reactions, which generated gaseous molecules that prevented the bubbles from collapsing explosively. In contrast, the penultimate step of collapse was when cavitation bubbles produced by a laser in water acquired their lowest radii, leading to a higher upsurge in localized pressure and temperature that might cause a second round of etching.

Wang et al. [70] investigated the association between the various physical factors and the max. dimensionless radius of the bubbles by employing single factor analysis in addition to multiple regression analysis. Only a very weak linear relationship exists between the following quantities, for example, between density and viscosity, and between sound velocity and surface tension. Additionally, the regression models developed based on the random forest and neural network types of machine learning algorithms were utilised to forecast how the combined physical factors affect the severity of the bubble collapse. The outcomes confirmed that each of these models was workable and efficient. Additionally, the strength of the collapse was clearly influenced by the viscosity and surface tension, whereas density and sound velocity had the least effect.

Gai et al. [71] adapted the Conventional Lattice Boltzmann Method (CLBM) to allow for the statistical analysis of ice nucleation resulting from the collapse of a cavitation bubble. To examine cavitation bubble dynamics, including growth and collapse, the authors examined two distinct real-world ice nucleation application scenarios: scenario I used a solid barrier, while scenario II involved a pressured environment. Some of the significant system variables whose impacts on the max. collapse pressure (p_max) were inspected included the stand-off distance (λ), initial bubble size (R₀), and differential pressure (Δp). The findings showed that scenario II yields a substantially higher p_max than scenario I, leading to more direct initiation of ice nucleation.

In 2021, a three-dimensional computational tool based on the multiphase lattice Boltzmann method (LBM) with multiple relaxation time (MRT) was used by Ezzatneshan and Vaseghnia [72] to study the dynamics of cavitation bubble clusters produced by sound near a solid wall under various wetting circumstances. To correctly impose the physical features of real fluids, the Peng–Robinson–Stryjek–Vera equation of state with an acentric component was integrated into the LBM. The obtained findings for the cavitation bubble cluster dynamics driven by sound showed that the pressure pulse cannot reach the lower bubbles due to the shielding effect of top bubbles. As such, the destruction of the top layer bubbles has a greater impact on the cluster core and the bubbles close to the solid surface than it does on the acoustic field.

In 2022, to better perceive the association between the cavitation bubble’s proximity to the wall and the collapse features, Xu et al. [73] created models with various distances from the benzamide wall to the cavitation bubble. The bubble placed exactly in the middle of two parallel walls experienced a major rate of volume change at the beginning of the collapse, according to the research. The bubble’s rate of volume change during the acceleration phase is proportional to its proximity to the wall and, thus, the pressure at its peak is higher. The initial spherical bubble regularly changed into an ellipsoid shape, and the surface of the bubble closest to the wall had a rather smooth texture. The wall penetrated the area with the strongest pressure field created by the collapse of the bubble when d was smaller than 1.59 R₀.

In 2022, the dynamic behavior of bubble collapses, pressure loads, and water jets throughout the collapse of the bubble between walls and a free surface was mathematically inspected by Nguyen et al. [74] using a VOF-based simulation approach. This was done by mathematically analysing the pressure loads, water jets, and bubble collapses that occurred when the bubble collapsed close to the walls. The mathematical approach was relying on the compressible Navier–Stokes correlations, which were represented in a conservative formula. These equations explain how compressible viscous fluids flow. Investigations of spherical and non-spherical bubbles, as well as grid dependence studies of a spark-generated bubble were conducted. This study modelled the burst of a bubble. The outcomes indicated that the dynamics of the bubble during its collapse were highly consistent between the experiments and simulation’s results. The inspection of a single bubble that was situated near to a wall was done utilising a range of standoff distances. The pressure loads caused by the jets interacting with the walls were evaluated and analysed. A more difficult situation of bubble collapse along an inclined wall and free surface was also developed and experienced.

Yang et al. [75] evaluated the influences of wall temperature on the collapse of a bubble and formed a robust model for a cavitation bubble close to a cooled or heated wall. The process of the micro-jet and the cavitation bubble itself affecting the solid wall heat transfer, as well as the thermodynamic behavior traits of the cavitation bubble collapse close to the wall, were resolved by the researchers. This makes it possible to better realize how the micro-jet affects the cavitation bubble. By modifying the wall’s temperature, T_w, the cavitation’s thermal effects could also be influenced. To further explore the heat transfer intensity, a dimensionless temperature parameter was added. Research and analysis were conducted on the impact that p, and R₀ have on the heat transfer. The outcomes showed that the ideal values of p, R₀, and T_w could be utilised to recover heat conduction and device cooling or heating treatments on several surfaces.

He et al. [76] looked into the thermodynamics of bubble interactions using the double distribution function thermal lattice Boltzmann technique. Strong and weak interaction regimes of double cavitation bubbles were methodically explored, and the whole development and collapse processes during an interaction were effectively replicated. Also, the essential lengths between two equal-sized bubbles that allow for the distinction of various interaction types were proposed. When there are two unequal-sized bubbles in a liquid film, imbalanced forces operate on them, making the film harder to rupture than when the bubbles are equal in size. This means that the coalescence regime requires a smaller starting distance between the bubbles.

Ezzatneshan et al. [77] used a multiphase lattice Boltzmann method (LBM) to investigate the beginning of the boiling phenomena and the bubble dynamics that resulted. The researchers used two equations of state (EoS), the Peng–Robinson (P–R) and the Redlich–Kwong–Soave (R–K–S). Their predictive power for the nucleation, growth, and departure of bubbles was measured in comparison to the existing literature data. The influence of the wettability and form of the micro-heater surface, namely the grooved and cavity areas, was appraised in relation to the detached bubble’s separation time and velocity under various flow conditions. The region with larger temperature gradients—the groove and cavity’s sharply angled edges—were where the boiling phenomena originated, according to the results. The bubble continues to develop on the hydrophilic heated surface’s sharp edges where there are not many dry spots.

The implication of a dual distribution function multiphase lattice Boltzmann technique (DDF-MLBM) to examine the collapse of cavitation bubbles in cryogenic liquids was addressed by Ezzatneshan et al. [78]. The exact difference method (EDM) was used in the scheme to apply fluid–solid adhesion forces and interparticle interactions, while counting the energy equation. The Peng–Robinson (PR) equation of state was utilised in conjunction with an acentric factor to properly simulate phase shifts and the molecular complexity of cryogenic fluids such as liquid nitrogen (LN₂) and hydrogen (LH₂). The research observed the impacts of pressure, temperature, collapse duration, and the separation between a cavitation bubble and a nearby solid wall. The results indicated how vital the distances between the cavitation bubbles in the cluster and between the bubbles and the surrounding solid surface are in defining micro-jet velocity.

Table 2. Summary of studies on bubble collapse in different applications.

Authors (Year) [Reference]	Sector/Area of Process Engineering	Configuration	Medium	Type of Study	Parameters Under Investigation	Highlighted Findings
Navarrete et al. (2015) [36]	Biomedicine	Collapse of a conical bubble.	The liquid meniscus	Experimental	Effect of collapse on various light emission.	When a conical bubble bursts, a number of light-emitting mechanisms—thermal, chemical, and electrical in nature—are engaged.
Gong and Klaseboer (2016) [37]	Biomedicine or marine industry	Interaction between a deforming viscoelastic solid and a bubble that is in the process of bursting.	Viscoelastic solid	Numerical	The standoff distance, bubble size, and form, as well as the viscosity of the plate.	The outcomes showed that standoff distance and bubble size have a substantial impact on viscoelastic solid deformation and bubble collapse.
Tang et al. (2016) [38]	Bubble condensation with and without ultrasonic	The process of vapor bubbles condensing and bursting in an otherwise still and cold pool with the application of ultrasonic field.	Vapor bubble surface	Experimental	Impact of capillary wave.	The existence of the capillary wave enlarged the area of the bubble’s contact with the cold bulk and disturbed the thermal boundary layer near the vapor liquid interface, which aided in the condensation process.
Wang et al. (2016) [39]	Underwater vertical launching	The axisymmetric projectile experiences a phenomenon known as internal collapse that moves forward from the tail to the head.	Water	Experimental and Numerical	Effect of increasing pressure.	A crucial element that causes a non-linear variation in the cavity length is the pressure rise.
Qin and Alehossein (2016) [40]	Water jet drilling and rock cutting	The fluctuation in temperature and the transfer of heat that occurs when a bubble bursts.	Water	Experimental and Numerical	Bubble size and temperature.	The bubble crumbled more quickly as the original maximum bubble size grew. The inside bubble’s maximum temperature rose sharply, and the total transmitted heat also rose greatly.
Li et al. (2016) [41]	Ship propellers and hydroturbine	The fluctuating pressure that is caused as a bubble deflates.	Water	Experimental and Numerical	The impacts of the stand-off parameter, which is denoted by γ, the strength parameter, which is denoted by, and the ratio of specific heats, which is denoted by κ.	The maximum pm progressively reduces as γ rises from 1 to 1.4. A crucial standoff parameter, γc, exists. The pressure peak of pg rises as κ rises, whereas the pressure peak of pm falls.
Rowlatt and Lind (2017) [42]	Bioengineering	Near a fluid–fluid contact, bubbles collapse.	Stiff wall	Numerical	Interface heights.	Due to the lessened contact between the bubble and stiff wall, the bubble collapsed spherically at increasing interface heights from the rigid wall.
Ye and Zhu (2017) [43]	Propeller blades	Acoustic bubble collapse micro-jet near the wall.	Al 1060	Numerical	Micro-jet velocity.	The wall pressure rises with increasing micro-jet velocity.
Pavlov (2017) [44]	Implosion phenomenon	The bursting of a vapor bubble caused by the immediate application of an external pressure.	Water	Numerical	Effect of vapor pressure.	Vapor offers shock absorption to the compression pressure and lengthens the period until the empty bubble bursts. In this situation, the temperature increases rapidly and the vapor pressure peaks.
Ming et al. (2017) [45]	Fuel jet when supercavitation phenomenon	The process of the collapse of the cavitation bubble that was occurring within the diesel droplet.	Water droplet	Numerical	Environmental pressure and saturation vapor pressure.	As ambient pressure rises, the rate of collapse accelerates, while saturation vapor pressure falls, the rate of collapse accelerates.
Rosselló et al. (2018) [46]	Water hammering	A device that uses water to create well-ordered collapses of a single cavitation bubble is called a water hammer.	Water	Experimental	The expansion ratio.	The expansion ratio and the equilibrium bubble radius, is the most important metric in relation to collapse strength.
Phan et al. (2018) [47]	Free surface flow	Compressible interface flows with and without mass transfers.	Water	Numerical	Superheated wall temperature.	The super-heated wall temperature increases heat flow on the sphere, comparable to previously reported findings.
Kerabchi et al. (2018) [51]	Lab-scale sonoreactors	Concentrated acoustic cavitation.	Liquids subjected to ultrasound	Numerical	The influence of depth, ranging from 0 to 10 m, on acoustic cavitation, with a particular emphasis on this factor.	The drop in Tmax and pmax with depth was more severe at 1000 kHz frequency and 10 °C, with 72% losses in Tmax and 94% in pmax at z = 10 m.
Joshi et al. (2019) [52]	Cavitation erosion	Cavitation bubble collapsing in close proximity to an elastic-plastic substance.	Elasto-visco-plastic material	Numerical	Shockwave and Strain rate.	Shockwave impacts erode material more than micro-jet impacts. Strain rate overestimates plastic deformation by 60%.
Brujan et al. (2019) [53]	Medicine	The dynamics of a cavitation bubble created by a laser in the centre of two hard, horizontal parallel walls.	Microfluidics	Experimental	Size of the bubble, size of the bubble in relation to other bubbles, and the starting position of the bubble.	Planar jet height grows with bubble size relative to stiff wall distance. The jet velocity declines as the bubble size and distance from the vertical wall increase.
Hopfes et al. (2019) [54]	Material science to medical applications	Gelatine was filled with air bubbles that were mm-sized and subjected to an immediate pressure rise.	Gelatinous fluids	Experimental	During the collapse, there was motion of single bubbles of gelatine, each of which had a distinct concentration.	Single gelatine bubbles collapse similarly to water bubbles, and gelatine concentration does not influence their behaviour.
Bardia and Trujillo (2019) [55]	Heat exchanger and petroleum industries	Intermediate bubble collapse.	Homogeneous surrounding liquid phase	Numerical and Theoretical	Effects of the slow but steady increase in pressure.	Instead of a step shift, a progressive rate of liquid phase pressurization affects the collapse behaviour.
Gan et al. (2019) [56]	Underwater explosion	A hull girder that replicates the construction of a genuine warship and is subjected to the stresses of an underwater explosion.	Water	Experimental and Numerical	Examined how the charge weight and the distance from the detonation effect the interactions between loads from an underwater explosion and a hull girder.	Deformation characteristics are the same over a particular charge weight range.
Chudnovskii et al. (2020) [57]	Liquid aerosol above the sea surface	Liquid that has been significantly subcooled begins to boil as a result of local heating.	Subcooled liquid	Experimental	Radiation power and duration.	The relationship between maximum bubble size and absorbed radiation power (and time, if pulsed) is nonlinear.
Yuan et al. (2020) [58]	Floating ice cake	A bubble bursts close to an ice cake that’s drifting.	Cake	Experimental	Effect of bubble collapse on ice.	Referring to damage degree and crack growth, three damage patterns may be created on ice: crevasse, radial and circumferential, and radial.
Han et al. (2020) [59]	Chemical industry and medicine	Two different-sized bubbles interact and the wall affects bubble collapse.	Water	Numerical	The presence of the wall as well as the quantity of the bubbles.	Walls delay bubble collapse. Walls might enhance the collapse and rebound of the bubble. The number of bubbles does not directly affect collapse times.
Fursenko et al. (2020) [60]	Biological tissues and surgery	Near the microfibre submerged in a subcooled liquid, a vapor bubble collapses.	Micro-fibre immersed in a liquid	Experimental	The jet velocity and intensity.	Jet velocity is maximum during formation and declines with time. Jet speed depends on fibre radius. Maximum jet intensity be contingent on initial vapor bubble radius and fibre thickness. Water jet speed directly affects gas bubble volume decrease.
Tan and Yeo (2020) [61]	Ultrasonic device	Microabrasive particles with an average diameter of 5 μm to 50 μm were subjected to 20 kHz of strong ultrasonic irradiation in a deionized water medium.	Deionized water medium	Experimental	The influence on particle velocity of both the particle size and the ultrasonic amplitude.	Particle velocity is 8–40 m/s, depending on particle size and ultrasonic amplitude.
Orthaber et al. (2020) [62]	Biology, chemistry, and medicine	Dynamics of a single cavitation bubble at the oil–water contact.	Oil-water	Experimental and Numerical	Effect of liquid type (light or dense).	In the bubble develops in the lighter liquid, it jets toward the interface; if the denser liquid, it jets away.
Zhang et al. (2020) [63]	Biomedical treatment	Using a high-speed photography equipment, the dynamics of a laser-induced cavitation bubble collapsing close to a stiff wall.	Near the edge of a rigid wall	Experimental	Bubble location.	When a bubble is near to the edge, the interface’s mobility is constrained by a distinct neck in the centre. The edge might delay bubble collapse by 22% of the Rayleigh time.
Ezzatneshan and Vaseghnia (2020) [64]	Different wettability conditions	Collapse dynamics of cavitation bubbles of various liquids.	Water, liquid nitrogen, and liquid hydrogen.	Numerical	Wettability effect of the surface.	The water’s cavitation bubble collapses faster due to a powerful liquid jet, whereas the LN2 bubble takes a longer time to collapse due to its bigger bubble radius at the rebound.
Huang et al. (2021) [65]	Noise and erosion	The collapse and oscillation of a single bubble produce acoustic waves.	Water	Experimental and Numerical	Rarefaction wave pressure of acoustic waves.	After a shockwave, liquid velocity and pressure rise. Rarefaction waves disperse and lower liquid pressure. Acoustic waves’ pressure and influence on liquid velocity decrease with distance.
Xu et al. (2021) [66]	Ships	Air entrainment cavitation bubble collapse to reduce cavitation.	Water	Experimental	The roundness, size, and distance of an air bubble.	The collapse pressure determines the collapse direction.
Peng et al. (2021) [67]	Medicine	Single and dual bubbles of cavitation seen in close proximity to a stiff border that has a significant density-to-viscosity ratio.	Near a rigid boundary	Numerical	The impacts of the gap in space between the bubble of cavitation and the solid border.	As the bubble’s distance from the hard boundary grows, so will its maximum collapse pressure, velocity, and temperature. Cavitation bubble lifespan decreases.
Trummler et al. (2021) [68]	Ships	The way cavitation bubbles collapse in close proximity to walls when there is a lot of atmospheric pressure.	Vertical wall	Numerical	Effect of stand-off distance.	Stand-off distance affects collapse dynamics, micro-jet generation, rebound, and wall pressure.
Long et al. (2021) [69]	Biomedical	Throughout the pulsed laser ablation of sub-merged targets in three liquids, cavitation bubbles were created.	Three organic liquids	Numerical	Effect of liquid media on cavitation.	In ethanol and n-butanol, cavitation-induced reactions produce gaseous gases that prevent bubble collapse. In water, cavitation bubbles may be crushed into a microscopic area, increasing localized temperature and pressure and triggering a second etching.
Wang et al. (2021) [70]	Ships	Bubble collapse strength.	Water	Numerical	Viscosity, surface tension, density and sound velocity.	Viscosity and surface tension affect collapse strength more than density and sound speed.
Gai et al. (2022) [71]	Ice formation	Ice formation brought on by the cavitation bubble collapsing.	Water	Numerical	The stand-off distance, differential pressure, and initial bubble size on the maximum collapse pressure.	Scenario II’s greater pmax initiates ice nucleation more easily than scenario I.
Xu et al. (2022) [73]	Biomedical	Various separations (d) between the cavitation bubble and the benzamide wall.	Benzamide wall	Numerical	Influence of time on maximum pressure.	When one stays in the field of maximum pressure for a longer period of time, the pressure at its peak will be higher.
Nguyen et al. (2022) [74]	Ships	Bubble collapse near walls and a free surface: dynamic behaviour of water jets, pressure loads, and bubble collapses.	Water	Experimental and Numerical	Water jets and pressure loads.	The pressure stresses that were produced as a result of the jets colliding with the walls.
Yang et al. (2022) [75]	Heating or cooling system	Near the heated/cooled wall, a cavitation bubble develops.	Water	Numerical	The mechanism of influence that the micro-jet and the cavitation bubble itself have on one another.	The values of λ, Δp, R0 and Tw that are ideal may be employed to improve heat transmission and bring about heating or cooling treatments on a variety of various surfaces.
Ezzatneshan et al. (2024) [78]	Cryogenic fluids.	Collapse of cavitation bubbles in cryogenic liquids.	Cryogenic liquids	Numerical	The influence of the distance between a cavitation bubble with an adjacent solid wall.	The collapsing bubble cluster’s micro-jet velocity and collapse intensity are both enhanced by raisng the solid surface’s contact angle.

shows a summary of the accompanying research of bubble collapse while demonstrating the most important conclusions.

Despite the fact that it has a very tiny influence on the contour of the bubble, the analysis of a large number of previous studies revealed that the viscosity of the fluid may lower the pressure that is created by the bubble right before it bursts. The bubble deflated into a spherical shape as it moved further from the rigid wall’s interface heights as a further effect of the reduced contact between the bubble and the hard wall. Although nonlinear, the relationship between the max. bubble size and power is connected to the radiation power absorbed. In addition, the max. bubble size is proportionate to the absorbed radiation power.

5. Bubble Growth and Collapse Studies

This section develops the evaluation of the bubble growth and collapse studies that were encountered to enhance the heat transmission of specific set of industries.

In 2017, a Sharp-Interface Level-Set (SILS) approach was addressed by Lee and Son [79] for modelling the development and contraction of a compressible vapor bubble. This method utilises a level set function to characterize the interface and a number of partial differential correlations to express the dynamics of the fluid and solid phases. Referring to the findings, it was revealed that the temperature of the surrounding environment was an extensive factor in describing the pattern of bubble development and collapse. Throughout the phase of bubble expansion, it was also detected that the bubble’s pressure does not fall to a level lesser than the saturation pressure corresponding to the phase. The impacts of ambient temperature and phase change on the temporal discrepancies of the mathematical outcomes for the bubble growth and burst close to a wall, considering the radius of bubble and pressure of wall against time, are exhibited in Figure 11. Figure 11 shows that the bubble growth and collapse in close vicinity to a wall established that a re-entrant liquid jet was designed as a result of irregular liquid inertia and was influenced by the phase change and ambient liquid temperature. This phenomenon was observed when the bubbles formed near the wall. Furthermore, it was determined that the SILS Approach is a prevailing numerical technique for simulating complex multi-phase flows that involve fluid–solid interactions. It permits accurate modelling of the sharp interface between the fluid and solid phases and can handle complex geometries.

Wang et al. [80] utilised a comprehensive set of ordinary differential equations to observe bubble production and collapse in micro-gravity situations. A dimensionless fitting constantly considers both the area changes of the moving vapor/liquid interface and the diffusive nature of the interface layer was added. By comparing predicted temporal fluctuations of bubble radii with actual data, one may define the fitting coefficients for bubble production and collapse in water and ethanol. The original conditions of a sharp lessening in temperature caused some “fluctuations” in the simulated bubble radii during the initial phase of bubble collapse. The “re-bound influence” of pressure equilibrium in the bubble, which occurred due to the original state, was linked to these “fluctuations”.

In 2019, to power micro-synthetic jets, Sourtiji and Peles [81] calculated the viability of using bubble growth and collapse over a wide set of operational frequencies between 0.1 and 2.5 Hz and a heating rate between 3 and 4.5 W. A primary micro-channel was linked to a micro heater that was placed inside a chamber with dimensions of 3.5 mm in radius and 220 μm in height. The nozzle’s aperture was 300 μm. The artificial jet was formed by switching the micro-heater on and off at regular intervals, which led to the implosion and explosion of bubbles inside the container. The authors captured successive images of bubble nucleation, growth, and eventual collapse using high speed DSLR photography and a microscope. The characterization of the synthetic jet comprised the use of a momentum coefficient. It was indicated that the synthetic jet’s average value was more than unity over a wide set of operational frequencies, which recommends that it could progress the performance of a number of micro-systems, including the micro-mixing that takes place in microfluidic devices.

In 2019, continued visual observations were prepared by Sun et al. [82] of the morphological development of a hydrate shell on suspended methane bubbles in super-saturated water. Referring to recently discovered information and results, the hydrate shell’s dynamic growth progressively went through three phases: rapid development, ongoing collapse, and growing stasis. These stages can be further separated into their individual sub-stages. A novel mechanistic model for the development of hydrate shells on bubble surfaces was established. This model measured the mechanical features of hydrate collapse as well as the inter-coupling of several hydrate-forming mechanisms and multi-phase mass transfer processes. This model was created based on the surface properties of hydrated bubbles at various phases. The model not only described the growth of hydrates on each side of the hydrate shell, it also provided the first quantifiable account of the capillary holes within the hydration shell.

Zimnyakov et al. [83] employed image analysis to pinpoint when foam samples started to form at various temperatures. The transition divided the collaborative of surface film cells into sub-ensembles that are developing and depopulating. With the depopulation of unstable sub-ensembles, a specific rate of cell rearrangements exponentially dropped and got closer to a fixed value during the growth. The association between the temperature dependent “macroscopic” growth collapse rate for average cell size, a “microscopic” rate parameter for individual cell growth–collapse, and the probability density function for cell size distribution was established for growth.

In 2020, a cavitation bubble produced by the nanosecond pulsed laser ablation of a solid at shallow liquid depths was explored by Nguyen et al. [84]. When the bubble radius was between 0.4 and 9 times the max. the liquid was presented. Photo-elasticity laser stroboscopic videography was utilised to track bubble dynamics. The bubble breaks through liquid when the liquid depth is slighter than the bubble radius. The duration of the bubbles is shortened but no further shock was felt. The bubbles expanded during contraction and flattened during expansion at liquid depths that are half or less of the max. bubble radius. The bubbles became toroidal at the lowest contraction, emitting more shockwaves.

Puncochar et al. [85] proposed a simple and straightforward physical model for the description of the bubble formation process. This concept covered bubble expansion, bubble ascent, bubble separation, and bubble deformation. Buoyancy, inertia, and elasticity are the three effects that were considered. The relationship between the final form of the bubble and its Weber number was assessed during the detachment procedure using the energy conservation concept. For this investigation, the bubble shapes, including oblate spheroid, prolate spheroid, spherical cap, and lens shape were taken into account. The data that were gathered are examined and contrasted with earlier studies.

Table 3. Outline of studies on bubble growth and collapse in different applications.

Authors (Year) [Reference]	Sector/Area of Process Engineering	Configuration	Medium	Type of Study	Parameters Under Investigation	Highlighted Results/Findings
Lee and Son (2017) [79]	Ultrasonic cleaning and medical treatment	The expansion and subsequent deflation of a compressible vapor bubble.	Liquid-vapor phase change	Numerical	The influence of the ambient temperature, the phase shift, and the wall on the development and collapse of the compressible bubble.	It was demonstrated that the ambient temperature had a substantial influence on the bubble formation and collapse patterns. Additionally, it was noted that throughout the bubble growth phase, the bubble pressure did not descent lower the comparable saturation pressure.
Wang et al. (2018) [80]	Subcooled boiling	The expansion and contraction of bubbles when subjected to microgravity.	Water	Numerical	Behaviour of bubble radii.	The bubble radii exhibited some “variations” throughout the early stages of bubble collapse, which may be ascribed to the “re-bound effect” of pressure balance in the bubble due to the starting condition of a sharp decrease in temperature.
Sourtiji and Peles (2019) [81]	Microbiology and micro-sensors	Micro synthetic jets that function by using bubble development and collapse throughout a spectrum of operational frequencies (0.1–2.5 Hz) and heating powers.	Microfluidics	Experimental	Operating frequencies.	The average value is greater than unity over a wide range of operational frequencies, proposing that this jet may advance the functionality of a number of micro-systems, including micro-mixing in microfluidic devices.
Sun et al. (2019) [82]	System sub-cooling	Variation in the morphology of the hydrate shell that forms on methane bubbles floating in supersaturated water.	Water	Experimental	Effect of continuous collapse and hydrate shell.	The opposition between the hydrate shell’s critical collapse pressure and the bubbles’ differential pressure has a significant impact on how frequently continuous collapse occurs.
Zimnyakov et al. (2019) [83]	Fresh constrained foams	The surface film cells that are located at the interface between the new liquid foam and the confining wall.	Liquid foam	Numerical and Analytical	Effect of splitting process.	The averaged specific rate of local re-arrangements in the surface film cells and clusters of bulk bubbles decays exponentially during the splitting process until it reaches a constant value. This happens as the rate of re-arrangements in the clusters of surface film cells and bulk bubbles slows down.
Nguyen et al. (2020) [84]	Nanoparticles synthesis	A nanosecond pulsed laser is used to ablate a solid at varying depths of a finite liquid, which causes a bubble of cavitation to form.	Finite liquid	Experimental	liquid depth.	The secondary shockwaves grow more concentric as the liquid depth rises, and a larger impulse is produced into the solid target as a result of this change.
Puncochar et al. (2022) [85]	Water desalination	Process of bubble production and subsequent deformation.	Water	Numerical	Buoyancy, inertia, elasticity.	The principle of energy conservation is used throughout the detachment process in order to define the association that exists between the final form of the bubble and its Weber number.

shows different applications of the accompanying studies that delineated the bubble growth and collapse.

In the publications that were analysed above, it was found that ambient temperature was a key aspect in defining the pattern of bubble development and collapse. A re-entrant liquid jet arises as a result to the irregular liquid inertia and is substantially influenced by the phase shift and ambient liquid temperature, as seen by the bubble expansion and collapse close to the wall. The fact that the bubbles became larger before collapsing close to the wall served as evidence for this. Additionally, the secondary shockwaves become enlarged as the liquid depth increases, and a durable impulse is generated into the solid target.

6. Evaluation of the Numerical Methods Used in Bubble Growth and Collapse Studies

Many industrial and environmental activities involve binary and multi-phase flow phenomena. These procedures entail the simultaneous flow of multiple phases (gas, liquid, and solid) in varied arrangements. To accurately represent the complicated physical interactions between the phases in the numerical simulation of these processes, specialized models and techniques are needed. The current review recognizes the utilization of different numerical methods to mimic multi-phase flow phenomena. Each method has its advantages and shortcomings, depending on the specific presentation and the level of accurateness needed. Some of the commonly used numerical techniques for multi-phase flow simulation are discussed below:

The Finite Volume Method (FVM) is a generally utilised mathematical method for resolving complex multi-phase flow problems. It is a conventional approach that can handle complex geometries and irregular boundaries. FVM can also handle non-uniform grids and is computationally effective. However, FVM needs careful selection of numerical schemes to avoid numerical instability [86]. Specifically, FVM is an adaptable method that can be used in both liquid–gas and solid–gas systems as a result of its capacity to handle complicated geometries, non-uniform grids, and multi-phase interactions. However, the careful selection of numerical patterns is important in both cases to guarantee stability and precision in the simulations.

The Level-Set Method (LSM) is a general numerical method for pursuing the development of a free surface or interface between two fluids in a multi-phase flow. It indicates the interface as a level set of a higher dimension corresponding to the computational domain. The LSM delivers a number of benefits, including the ability to handle complex interface topologies and geometries, and making it simple to apply boundary conditions. The dynamics of the interface, such as merging and splitting events, can also be precisely captured [87].

The numerical method known as the Phase-Field Model (PFM) is utilised to mimic multiphase flows that entail the progress of a diffuse interface between various fluids. The interface between the two fluids is characterised by a non-conserved scalar field in the model’s mathematical foundation. The PEM delivers a number of benefits, including the ability to handle complex interface topologies and geometries and make boundary conditions simple to implement. Furthermore, it may faithfully depict the dynamics of the interface, such as coalescence and break-up processes [88].

A numerical technique known as the Included Phase Model (IPM) is utilized to simulate multiphase flows involving the presence of bubbles in a fluid. Instead of representing the dispersed phase as discrete particles, it signifies it as a continuum and uses a series of transport equations to chart its behavior. The IPM offers many benefits, including the ability to handle high volume fractions of the dispersed phase and the ability to consider the impacts of the dispersed phase on the continuous phase. Moreover, it can record intricate physical processes like turbulence modulation [89].

A powerful tool for modelling complicated multi-phase flows in which the collaboration between distinct phases is important is CFD. Multi-phase flows can be simulated using a variety of CFD techniques, counting the Eulerian–Eulerian, Eulerian–Lagrangian, and Eulerian–Granular approaches. The specific parameters of the multi-phase flow being simulated determine the CFD that should be used [90].

The complex multi-phase flow phenomenon can be accomplished using two analytical and numerical methods called the Potential Flow Theory (PFT) and the Boundary Element Method (BEM). Understanding the underlying notions regulating fluid dynamics is made easier by PFT. The BEM is a mathematical approach to solve partial differential equations that contain discretization within the domain of interest’s borders. When dealing with complex geometries, where more standard numerical tactics like the Finite Element or Finite Difference approaches can become computationally expensive, BEM is particularly helpful. It is crucial to remember that both PFT and BEM methods include restrictions and presumptions that might not always hold true in practical settings. Thus, suspicious validation of the results against experimental data is necessary to safeguard the accurateness and reliability of the simulation results [91].

Spectral Element Marker Particle (SEMP) is a mathematical method used in general to simulate complex multi-phase flows that include the interaction between fluid and solid phases to accurately model fluid and solid dynamics. SEMP discretizes the fluid and solid domains, which permits a high order of accuracy and efficient computation of the solution. SEMP has several benefits over other numerical techniques, such as its ability to accurately capture the dynamics of the fluid–solid interface and the interaction between the particles [92].

The Finite Element Method (FEM) is a mathematical approach that can solve complicated multi-phase flow problems by dividing the domain into small subdomains. The FEM can handle complex geometries and asymmetrical boundaries and can provide accurate results. However, the FEM is computationally expensive, particularly for large-scale problems [93].

A numerical method called the Thermal Lattice Boltzmann Method (TLBM) is utilised to model intricate multiphase flows involving heat transfer. This technique is based on a mesoscopic approach that simulates the behavior of individual particles within a lattice to address fluid flow problems. In comparison to other numerical methods, LBM provides a number of benefits, including the ability to precisely model the dynamics of multi-phase flows and heat transfer events. Also, it is computationally active and enables the simulation of intricate geometries. To settle the correctness of the results, it is essential to compare the simulation results to experimental data [94].

Smoothed Particle Hydrodynamics (SPH) is a meshless numerical method that can simulate multi-phase flow phenomena with complicated geometries and boundaries. SPH is computationally efficient and can handle large deformations and complex free-surface flows. However, SPH requires a large number of particles to achieve accurate results, which can raise computational cost [95].

A prevalent numerical approach to simulate free-surface flows is Volume of Fluid (VOF). It is capable of handling complicated geometries and boundaries as well as properly capturing the interface between several phases. To accurately capture the contact, VOF may need a fine grid resolution, which can be computationally expensive, especially for large-scale problems [96].

A numerical method known as Sharp-Interface Level-Set (SILS) is also used to model complex multi-phase flows that incorporate the collaboration of the fluid and solid phases. The approach relies on the Level-Set Technique, which numerically tracks the boundary between two fluids in a flow. In comparison to other numerical methods, the SILS Approach has several benefits, such as the ability to accurately represent the sharp boundary between fluid and solid phases. Moreover, complex multi-phase flows incorporating surface tension and other interfacial phenomena can be simulated using this method. To ensure the correctness of the simulation outcomes, the outcomes should be contrasted with experimental data [97].

The various discussed numerical methods, including the Finite Volume Method (FVM), Level-Set Method (LSM), Phase-Field Model (PFM), Included Phase Model (IPM), Computational Fluid Dynamics (CFD), Potential Flow Theory (PFT), Boundary Element Method (BEM), Spectral Element Marker Particle (SEMP), Thermal Lattice Boltzmann Method (TLBM), Smoothed Particle Hydrodynamics (SPH), Volume of Fluid (VOF), and Sharp-Interface Level-Set (SILS) are all useful tools to simulate both liquid–gas and solid–gas systems. Each method affords exceptional strengths, particularly when capturing the complexities of multiphase flows. These include an accurate modeling of fluid–solid interactions, managing complex geometries, and efficiently tracking phase interfaces. Despite some numerical methods being prominent in specific cases—like SPH for large deformations or VOF for free-surface flows—others can provide detailed contexts for perceiving the dynamics in both types of systems, such as CFD and IPM. This flexibility can highlight the prosperity of these numerical methods to be used in a wide sector of applications in fluid dynamics and materials science.

7. Recommendations for Further Research

The following are some suggestions that may be derived from the thermal performance analysis of each research study conducted, as well as from a careful examination of the information shown in Table 1, Table 2 and Table 3. In other words, the review came up with the limitations of past research on bubble growth and collapse that should be resolved following these suggestions:

There is a necessity to utilise the Bayesian approach to modelling bubble growth and collapse because it is still extremely pertinent today.

As the use of high-speed underwater acoustic communications shifts from military to commercial applications, it has absorbed a lot of attention. The implementation of these vehicles necessitates a speed variation in the cavitation flow, which introduces a significant challenge. Furthermore, deceleration may contribute to the worsening of cavitation instability, making it an important factor that has to be researched further. Thus, more exact experimental and numerical methodologies are necessary in the future to quantify the necessities of the maximum heat transfer.

The creation of bubbles with variable amounts of gas within them and the subsequent investigation of how this affects the behavior of the bubbles, as well as the investigation of how a bursting bubble interacts with a flexible substance, are both interesting possibilities for future research.

8. Conclusions

These are the most important conclusions that can be made:

The interaction between the ultimate form of the bubble and its Weber number is assessed throughout the detachment process using the energy conservation concept.

Throughout the splitting process, the average specific rate of local rearrangements in surface film cells and bulk bubble clusters decreases rapidly until it achieves a fixed value. This occurs as the intensity of rearrangements in the bulk bubbles and clusters of surface film cells declines.

A key element in deciding whether continuous collapse takes place is the degree of competitiveness between the critical collapse pressure of the hydrate shell and the bubble differential pressure.

It was found that the pattern of bubble formation and collapse was significantly influenced by the ambient temperature.

Collapse strength is most strongly influenced by viscosity, followed by surface tension, and then by density and sound velocity, in that order of diminishing importance.

Inside the bubble of ethanol and n-butanol, cavitation-induced reactions result in the production of gaseous by-products that prevent the bubble from collapsing.

The dynamics of the collapse, the growth of the micro-jet, the rebound, and the maximum wall pressure are all significantly influenced by the stand-off distance.

The shockwave sources a growth in the velocity and pressure of the liquid field. The rarefaction waves trigger a reduction in the liquid’s pressure, which causes a dispersive change in the liquid’s spatial distribution.

A visible neck will form in the middle of the bubble when the bubble is near the edge. This apparent neck will restrict the bubble interface’s mobility close to the edge.

Bubble density, a metric of bubble structure created by coalescence, and the two/point autocorrelation function show the expansion of a linked network, that may result in lower release thresholds.

The micro-layer that forms at the bubble bottom is responsible for around thirty percent of the entire quantity of evaporation.

Numerical methods can accurately simulate complex physical interactions between different phases while providing detailed information on the flow field and phase distribution.

Careful selection of numerical schemes is important to avoid numerical instability.