Numerical Study of the Soil Temperature Field Affected by Natural Gas Pipeline Leakage
Abstract
1. Introduction
2. Model Establishment
2.1. Mathematical Model
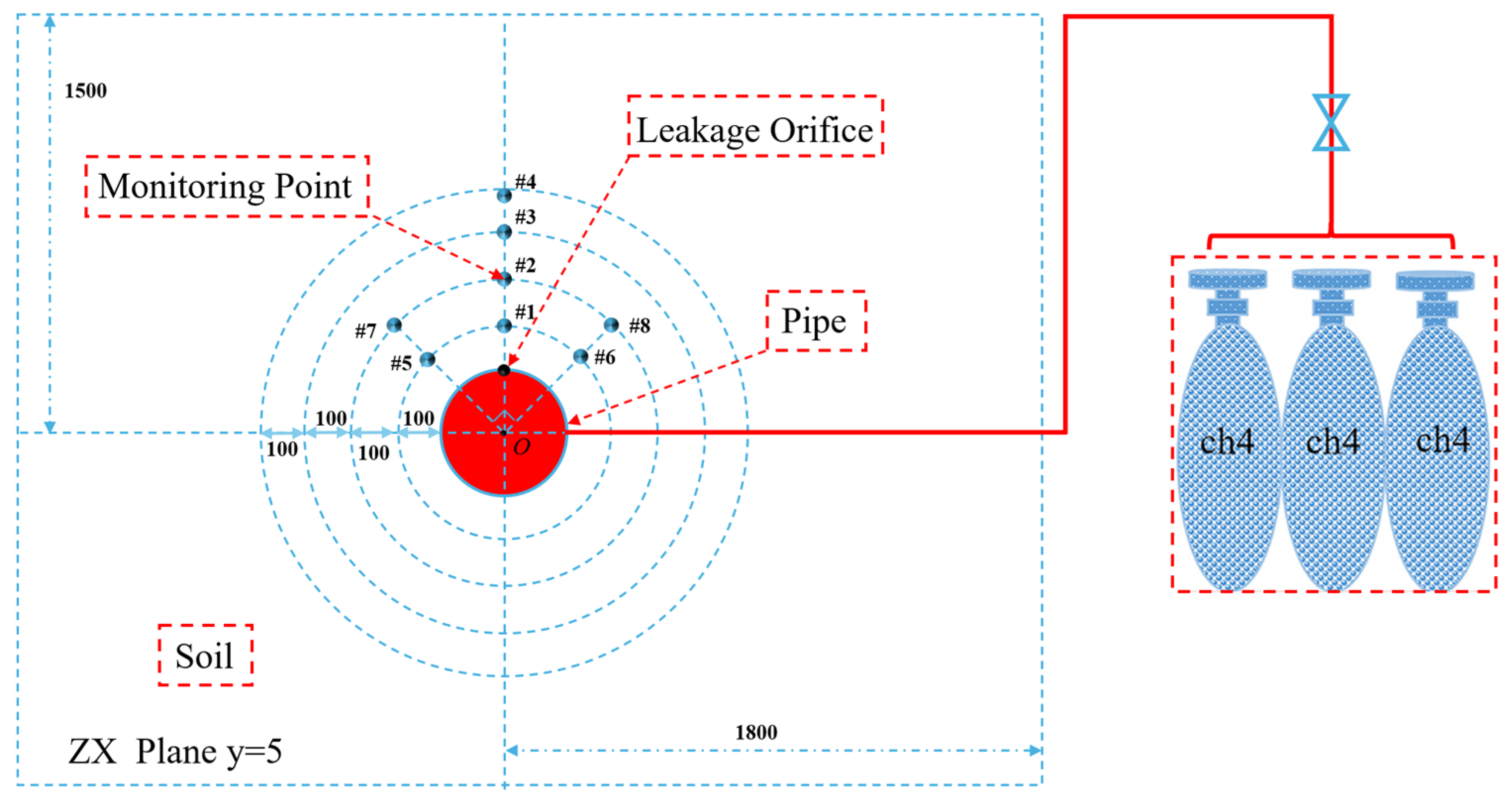
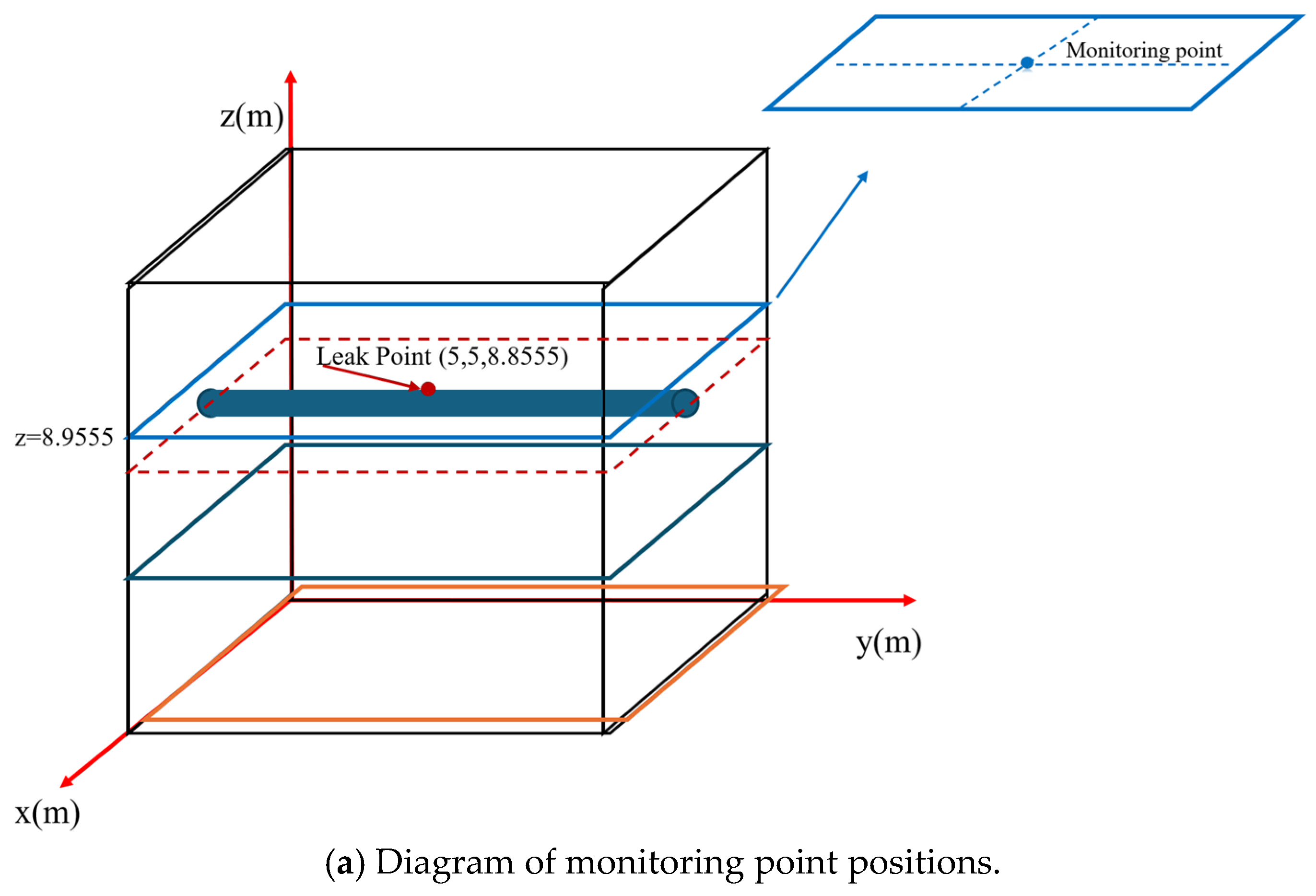
2.2. Physical Model
2.3. Mesh Division
2.4. Mesh Independence Verification
3. Numerical Results of the Temperature Field
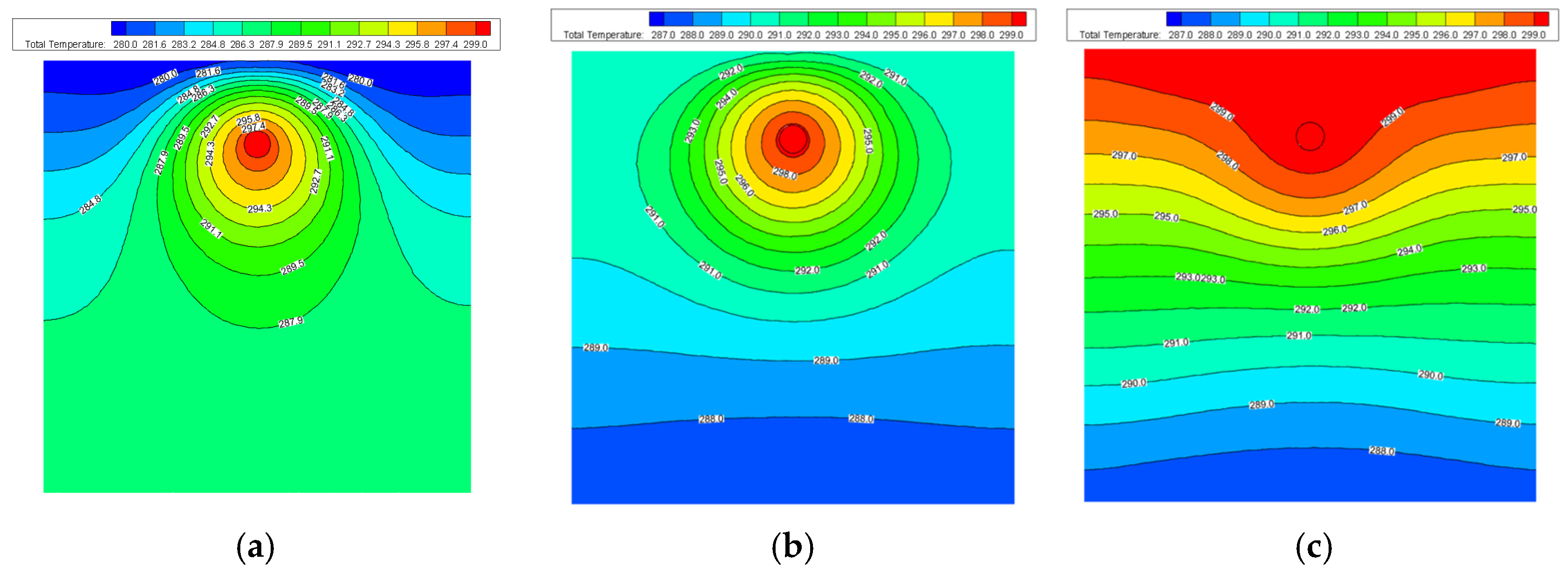
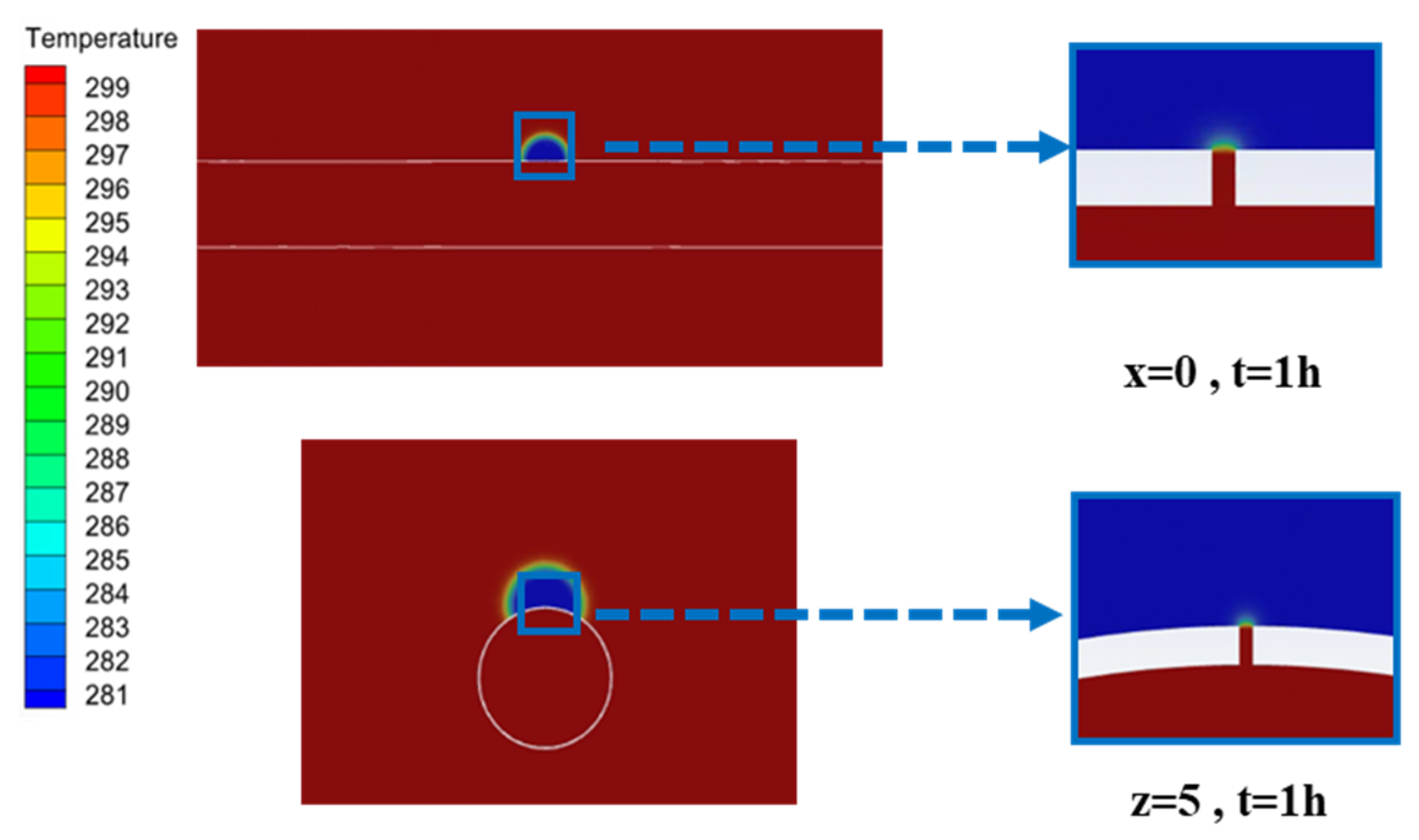
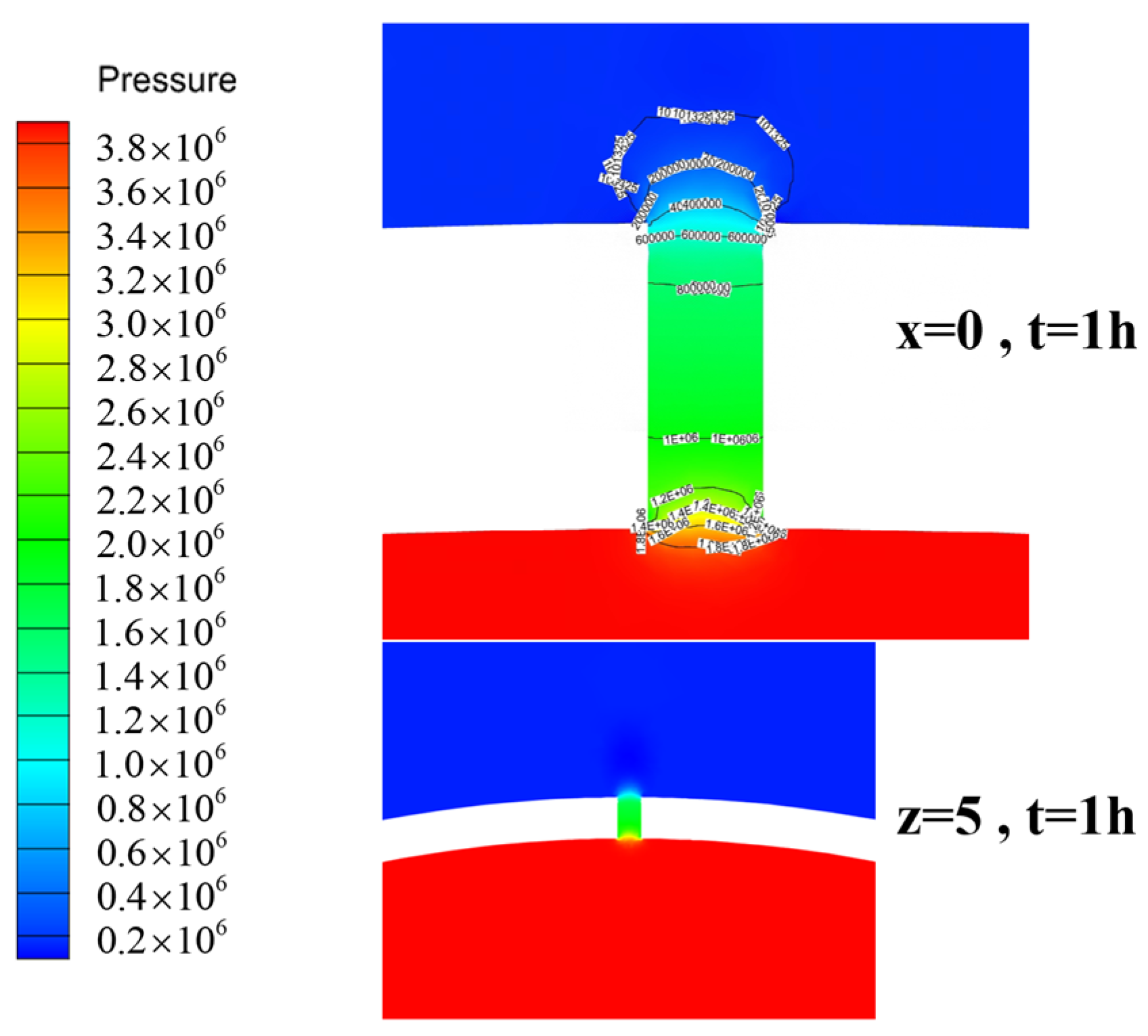
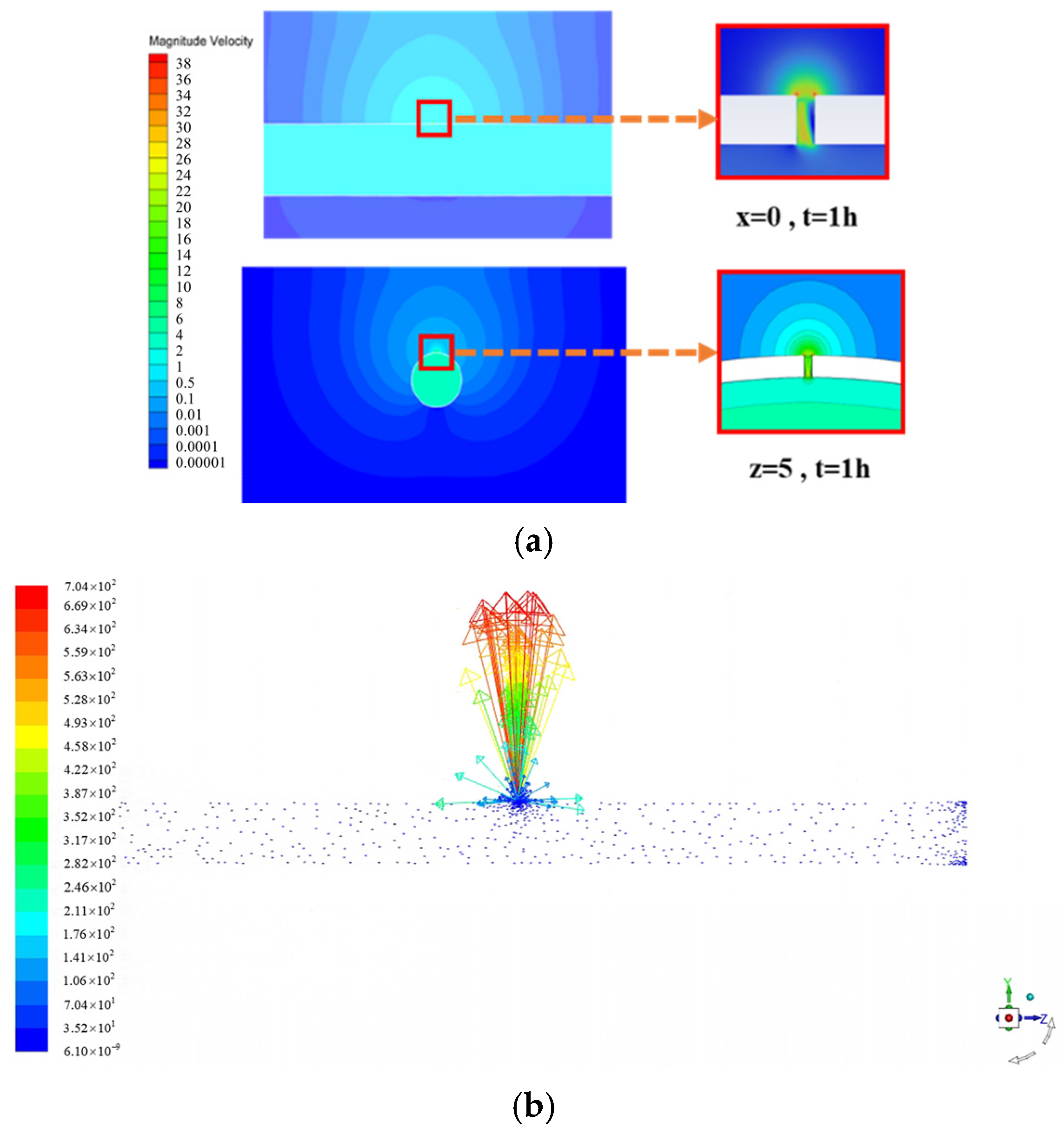
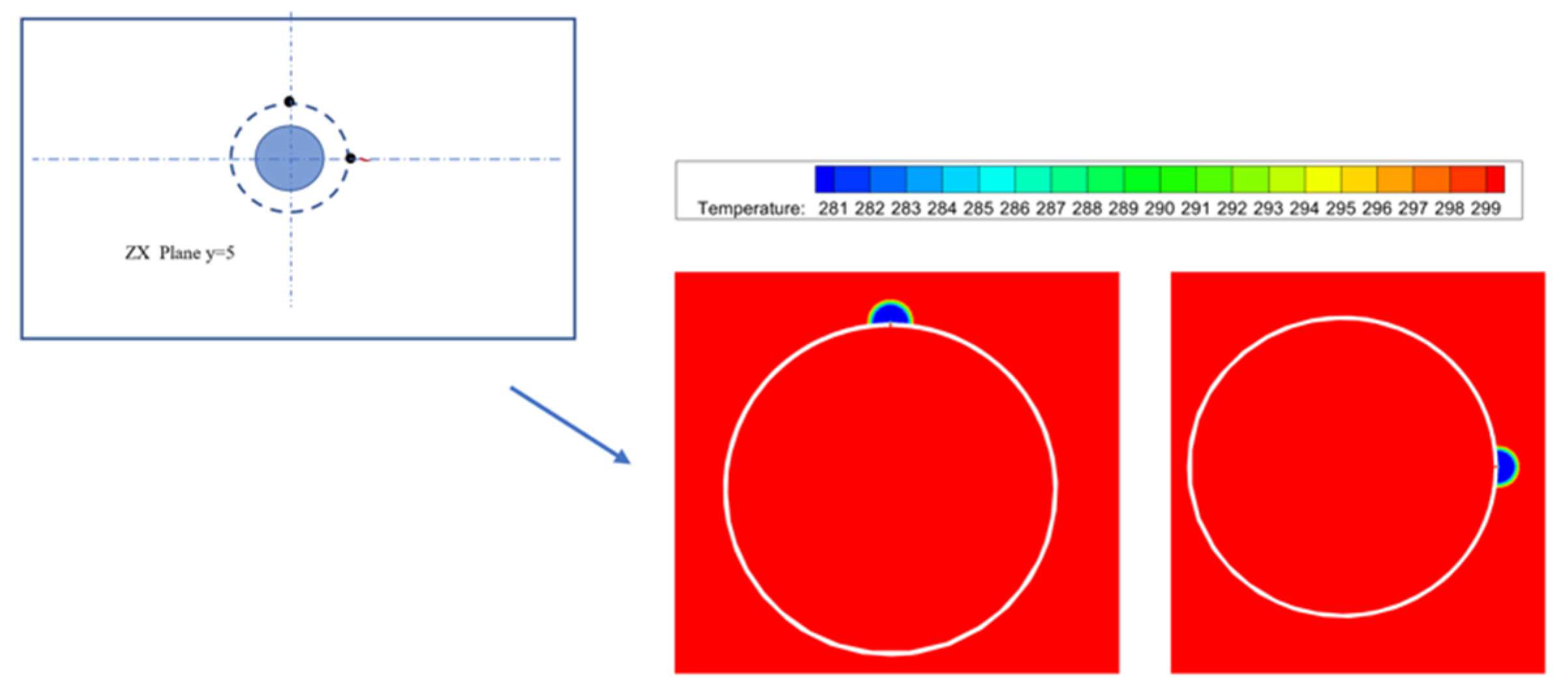
3.1. Results of the Flow Field and Temperature Field Due to Natural Gas Pipeline Leakage
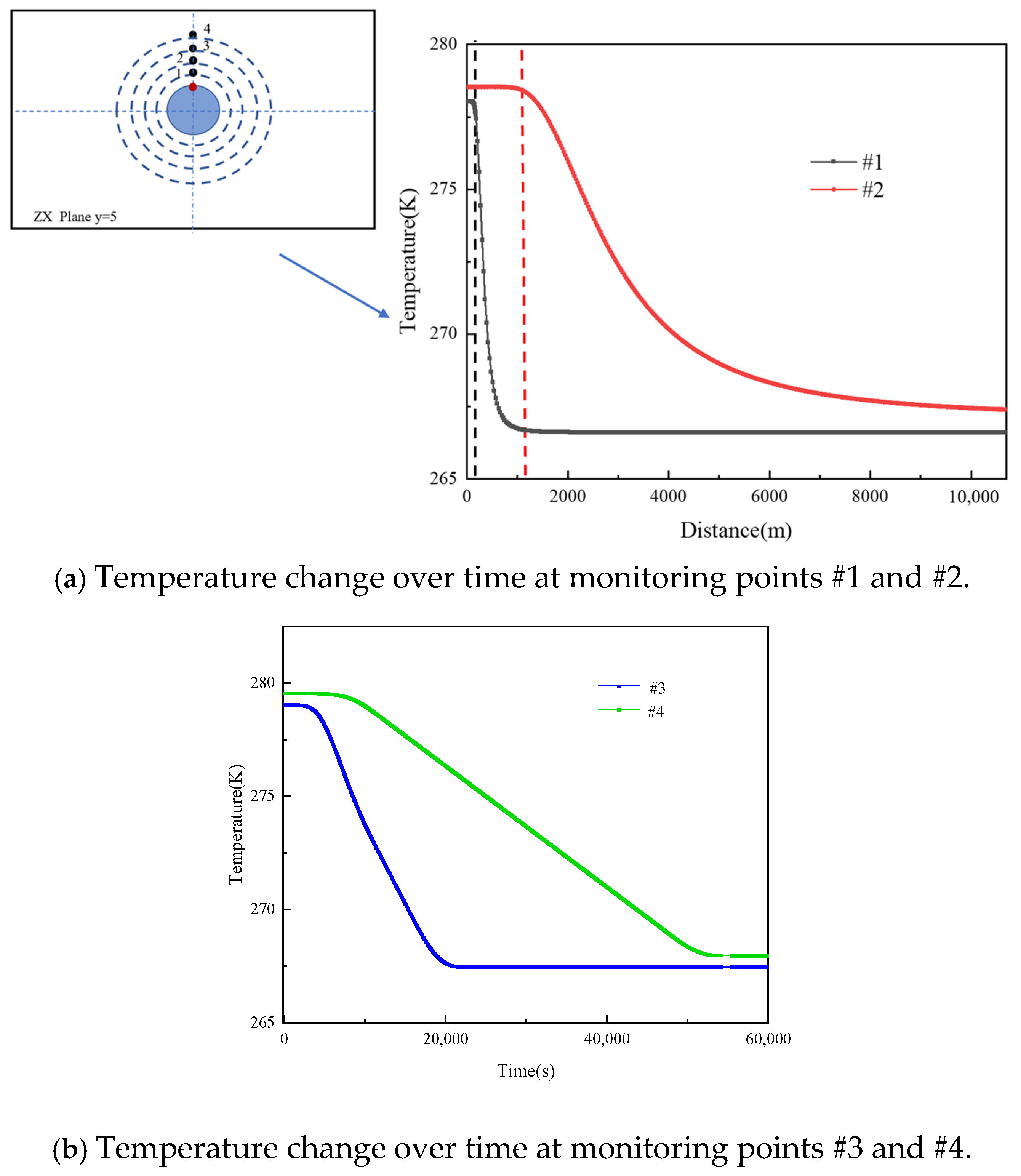
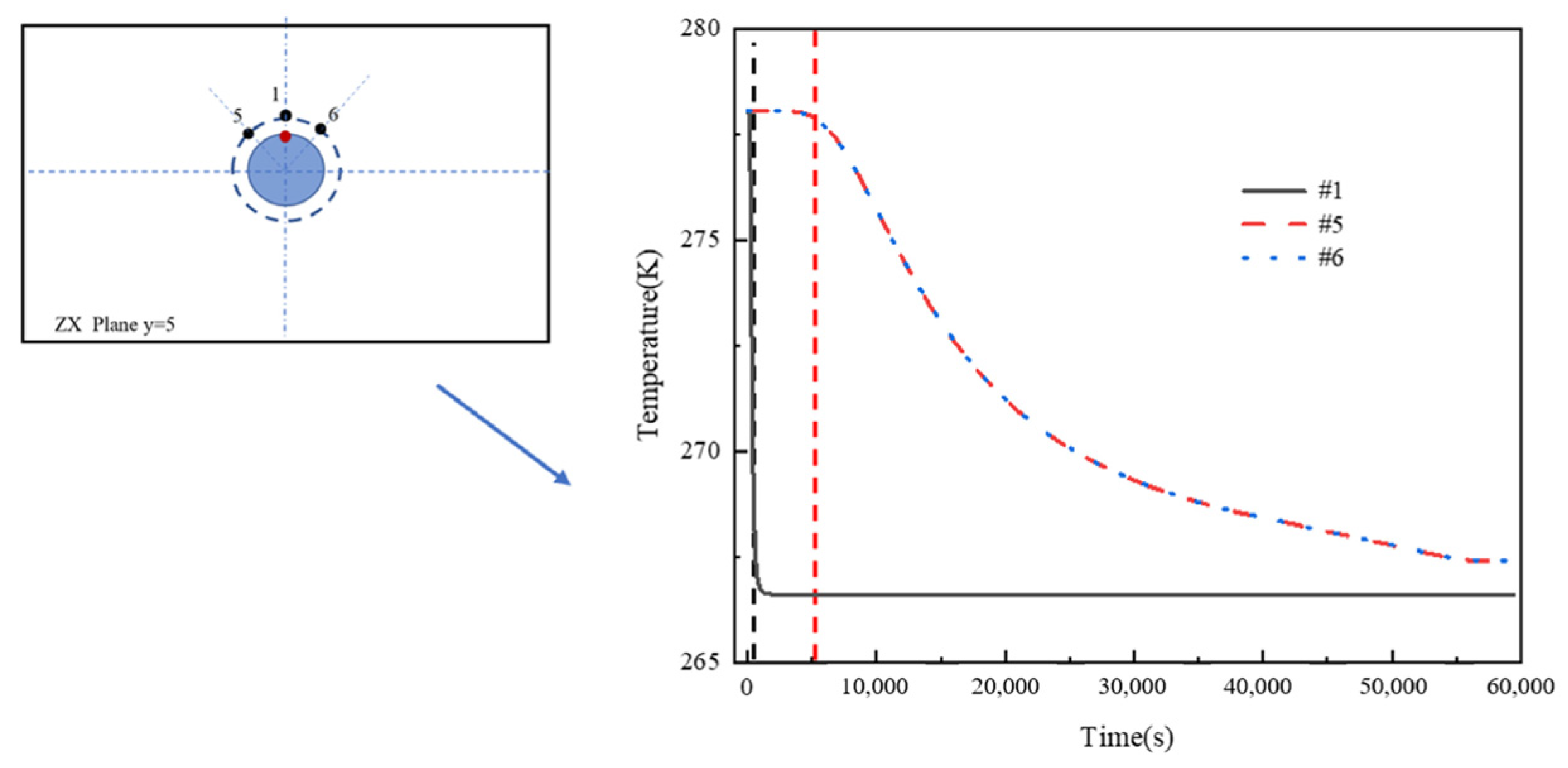
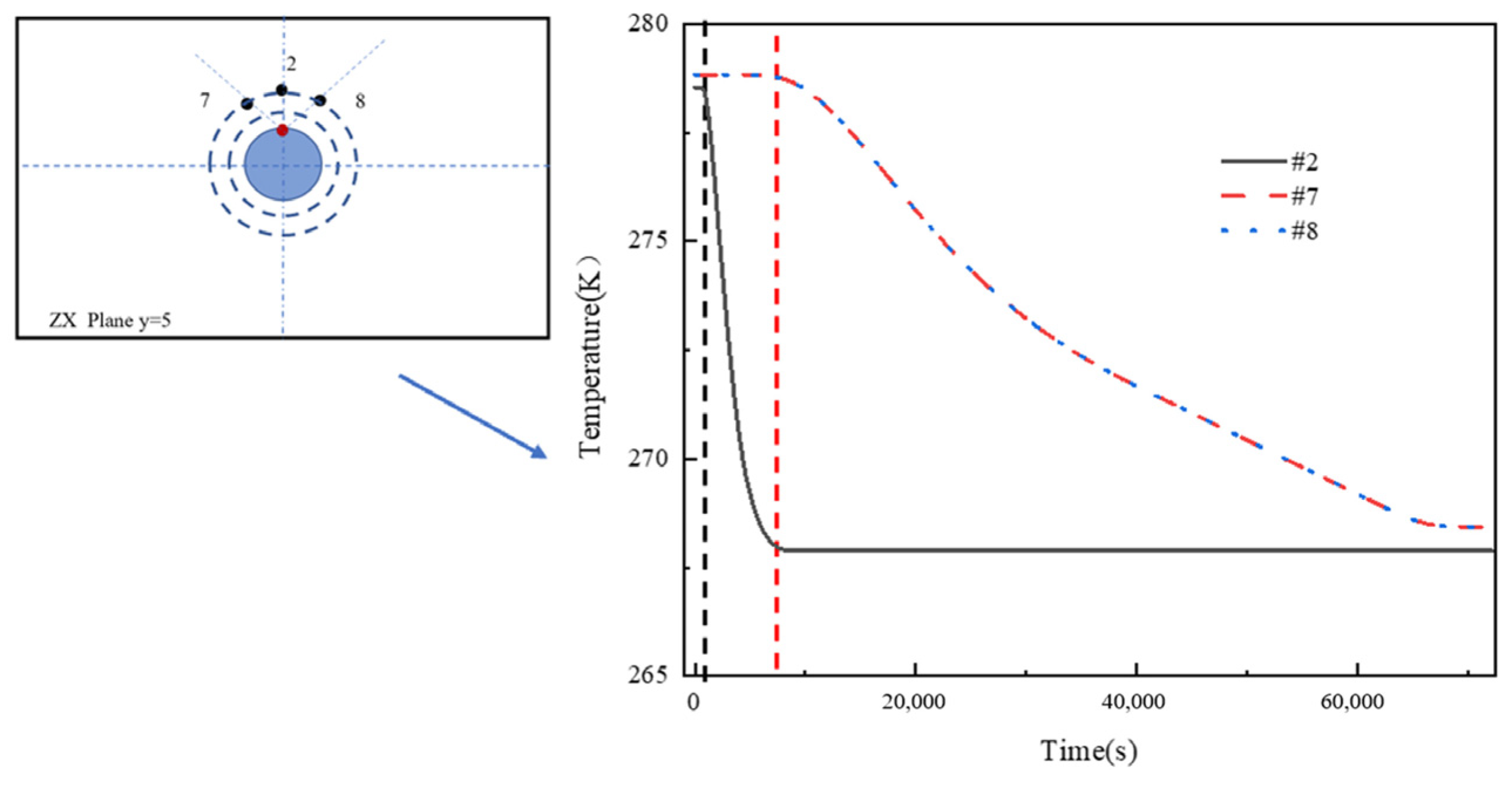
3.2. Results of the Sensor Monitored Temperature with Time Variation
4. Effects of Different Leakage Factors on the Temperature Field
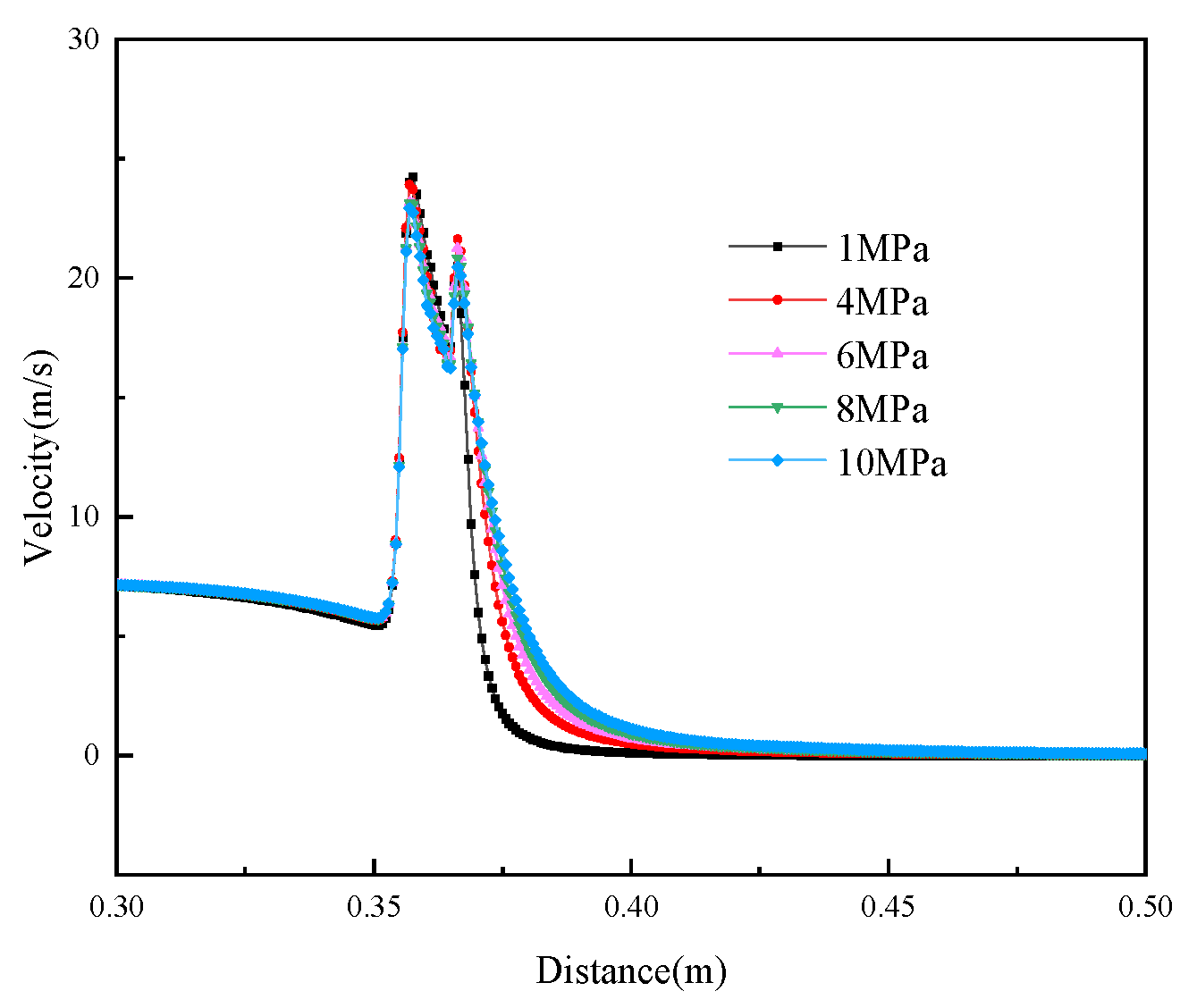
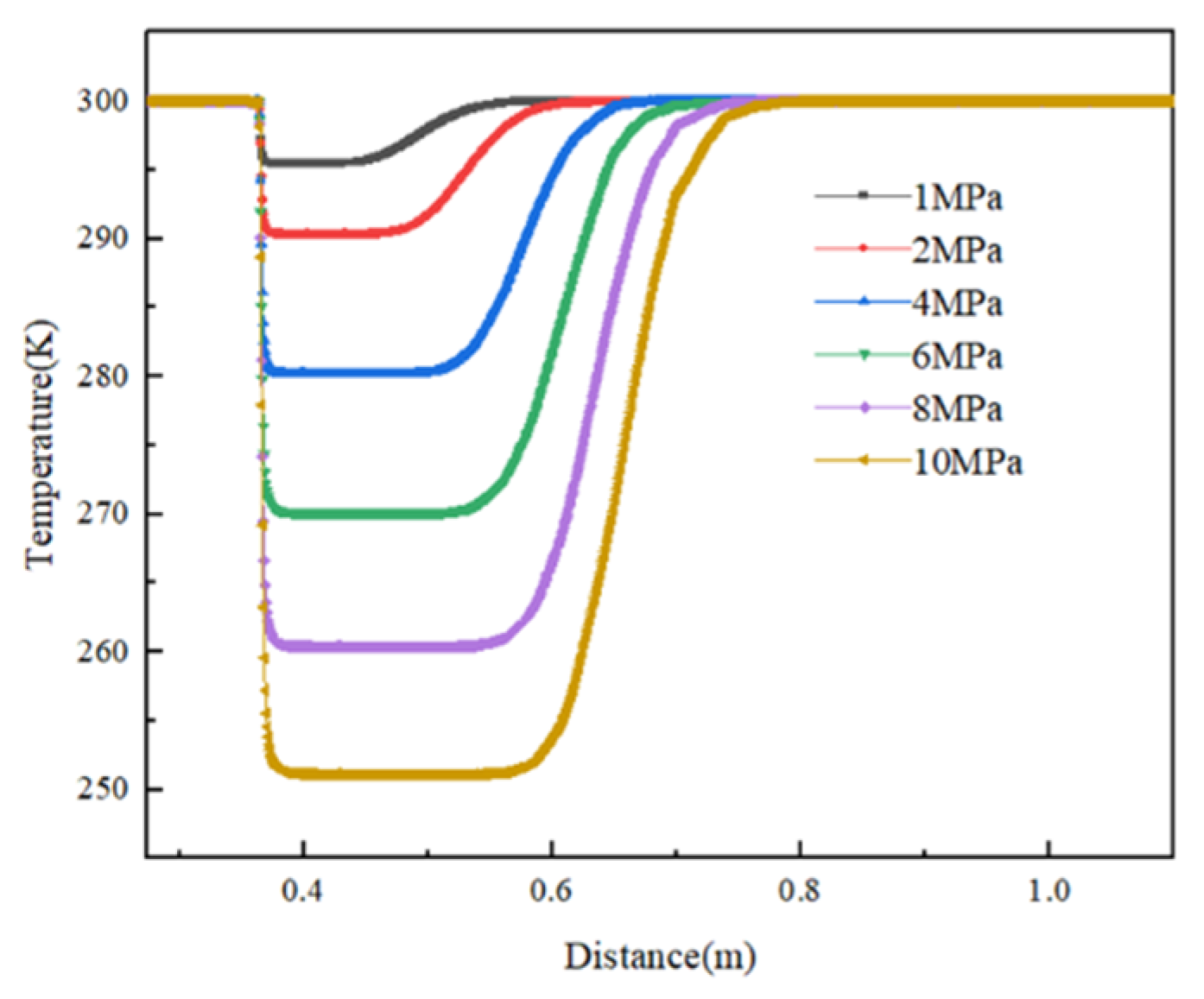
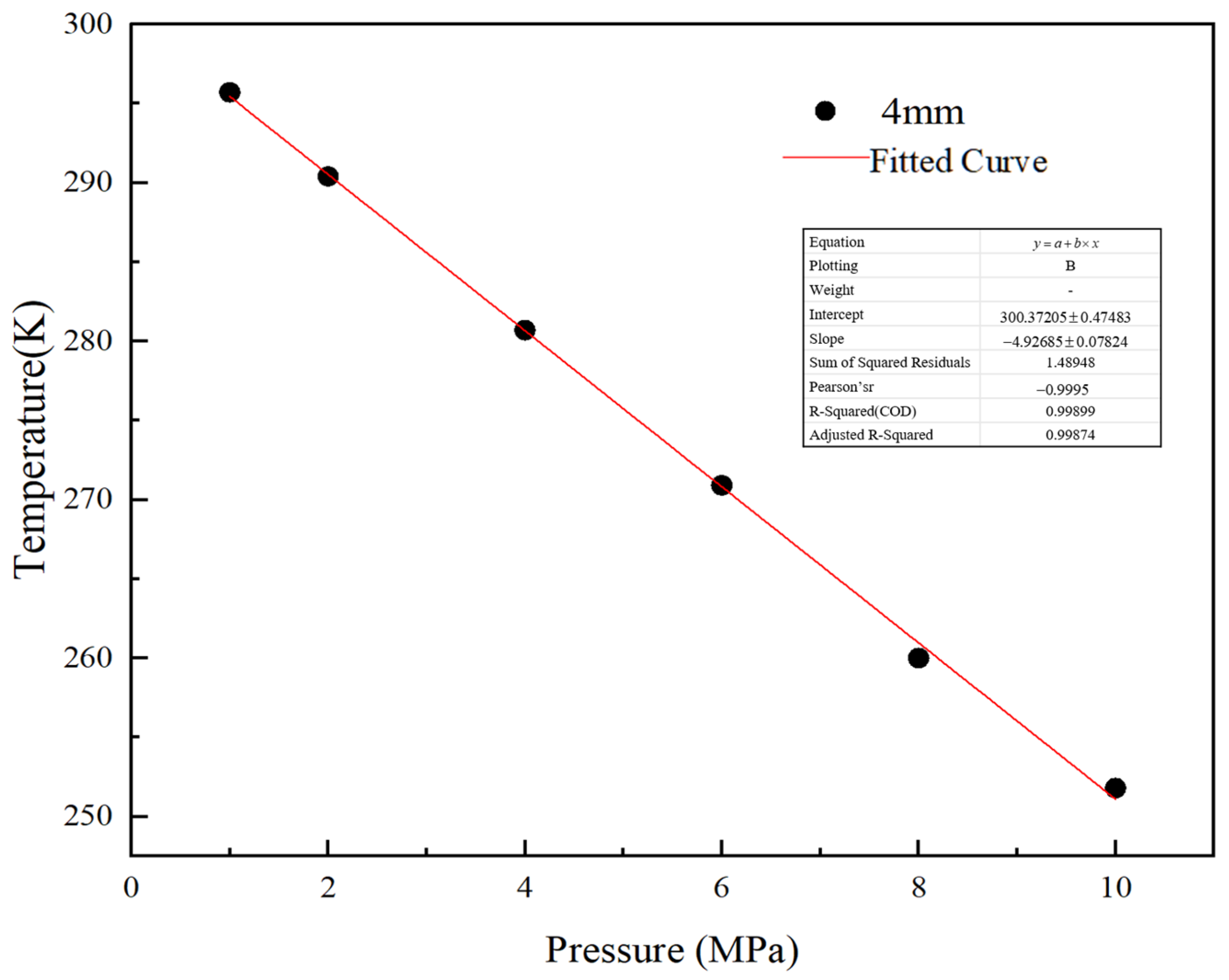
4.1. Pipeline Outlet Pressure
4.2. Leak Orifice Diameter
4.3. Different Leakage Directions
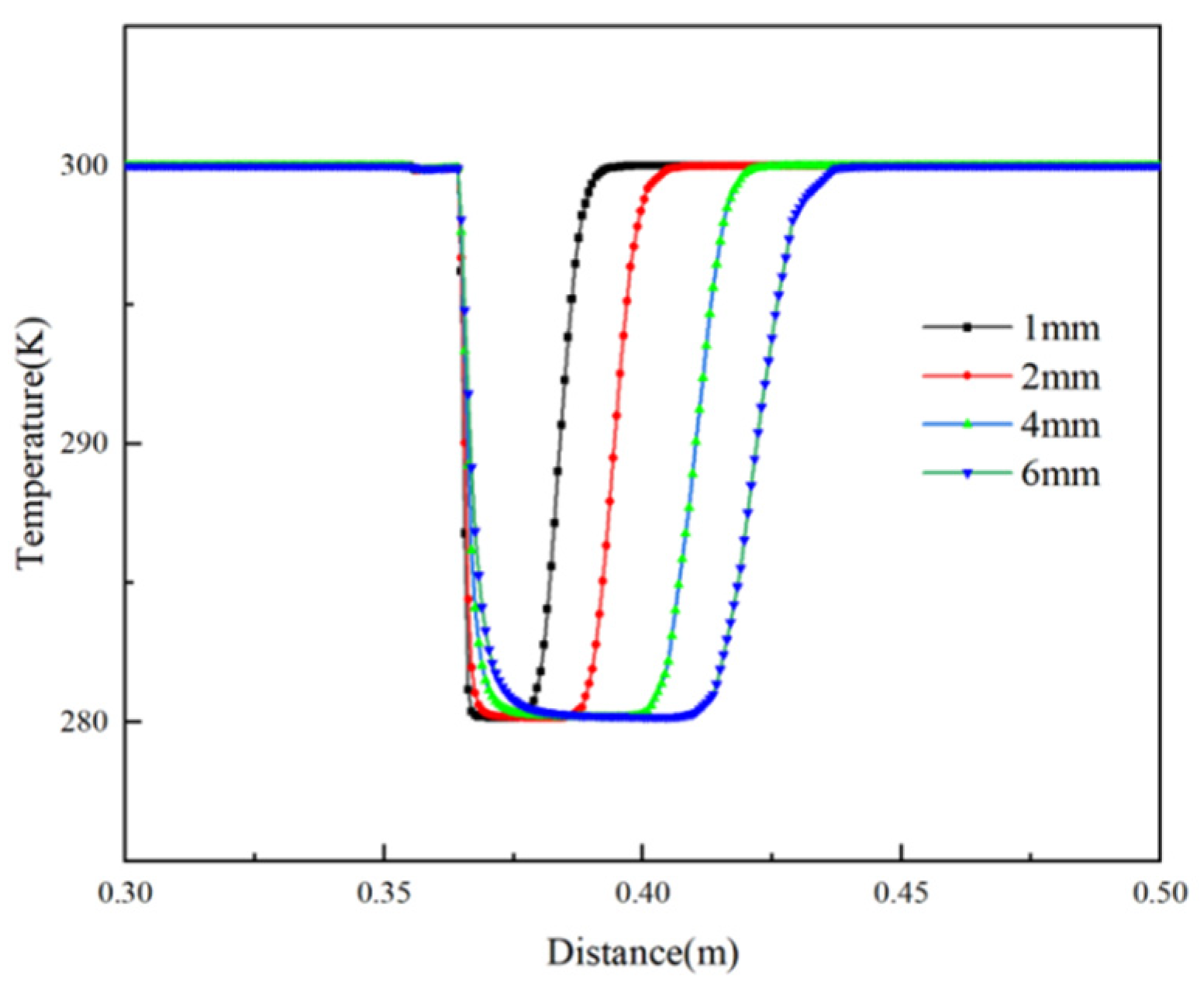
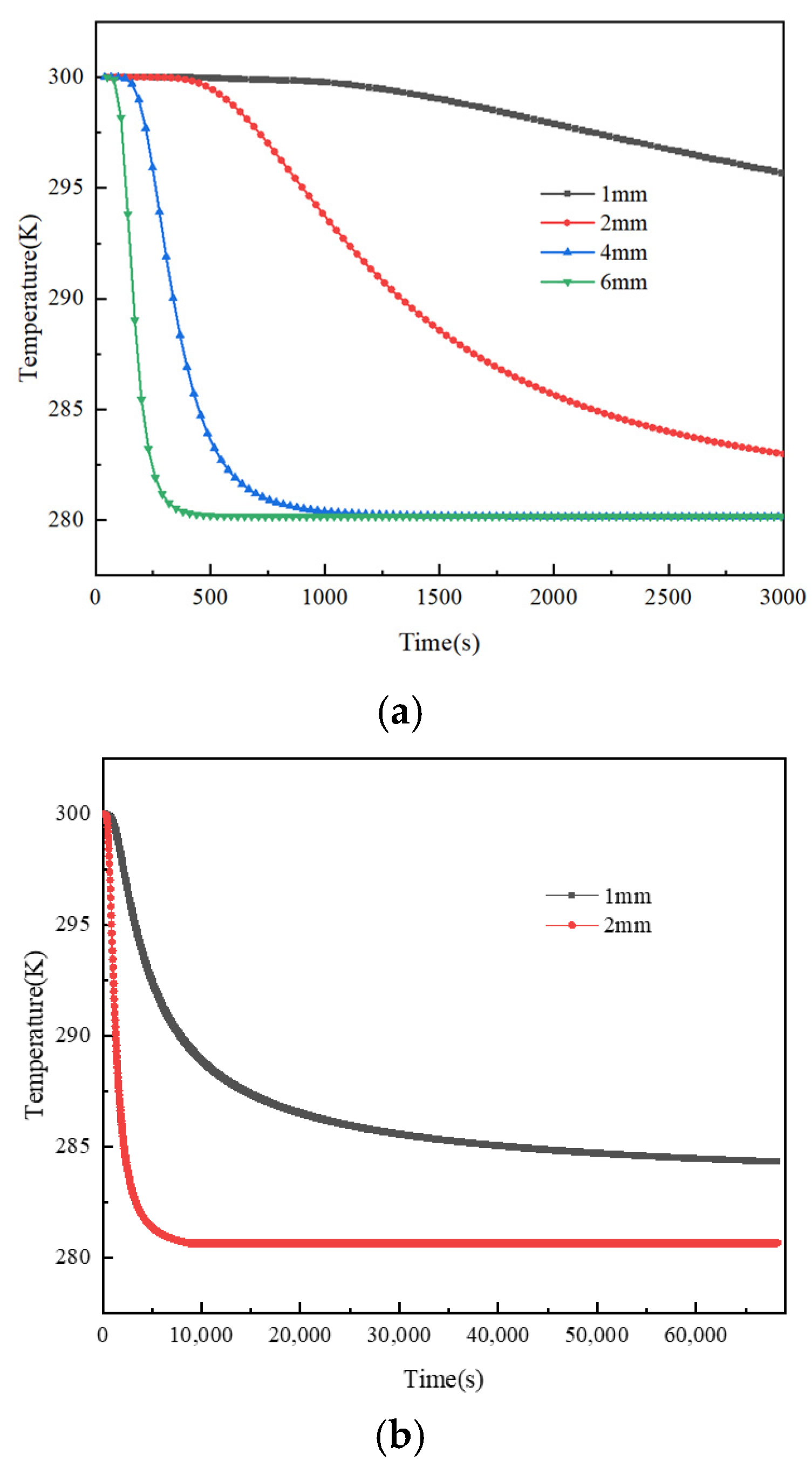
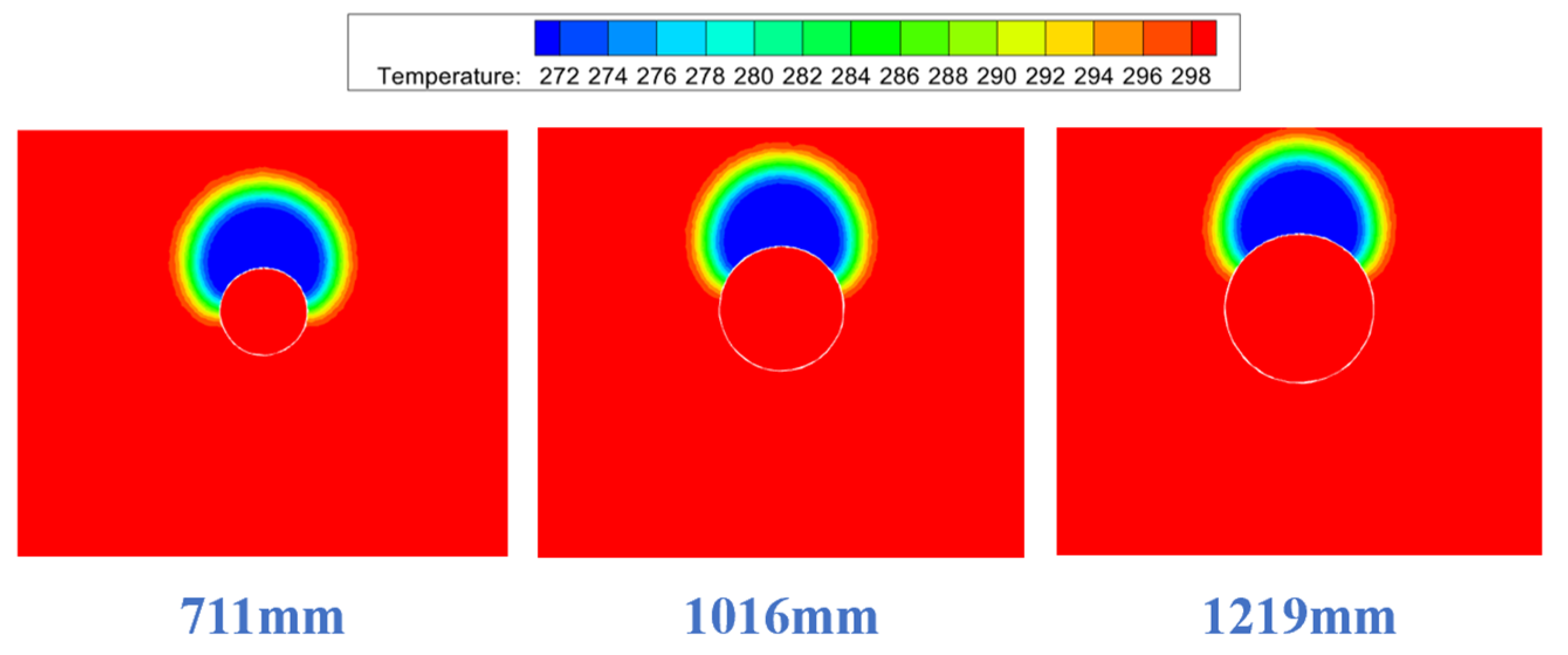
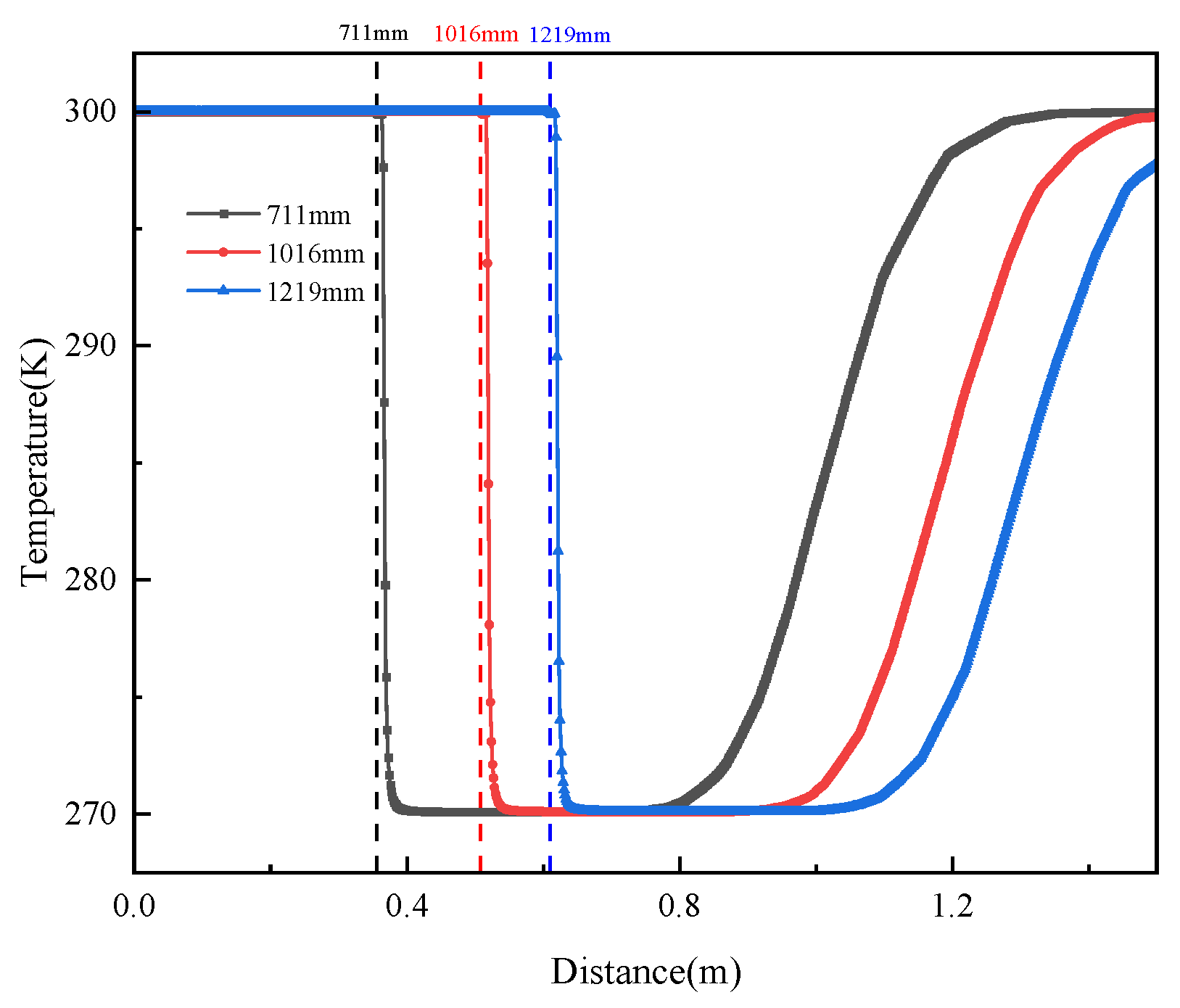
4.4. Different Pipeline Diameters
5. Conclusions
6. Discussion and Recommendations
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhang, Y.; Zhao, X.; Wang, S.; He, Z. Study of wind speed effect on laser detection in natural gas pipeline leaking. DEStech Trans. Mater. Sci. Eng. 2017. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Jin, H.; Zhang, L.; Liang, W.; Ding, Q. Integrated leakage detection and localization model for gas pipelines based on the acoustic wave method. J. Loss Prev. Process Ind. 2014, 27, 74–88. [Google Scholar] [CrossRef]
- Hou, Z.; Zhu, X. An EKF-based method and experimental study for small leakage detection and location in natural gas pipelines. Appl. Sci. 2019, 9, 3193. [Google Scholar] [CrossRef]
- Liu, C.; Li, Y.; Fan, L.; Xu, M. Leakage monitoring research and design for natural gas pipelines based on dynamic pressure waves. J. Process Control 2017, 50, 66–76. [Google Scholar] [CrossRef]
- Pan, X.; Karim, M.N. Modelling and monitoring of natural gas pipelines: New method for leak detection and localization estimation. Comput. Aided Chem. Eng. 2015, 37, 1787–1792. [Google Scholar]
- Yang, J.; Zhang, J.-H.; Mei, S.; Liu, D.; Zhao, F. Numerical simulation of sudden gas pipeline leakage in urban block. Energy Procedia 2017, 105, 4921–4926. [Google Scholar] [CrossRef]
- Hu, M.; Li, H.; Chen, L.; Xie, P.; Zhang, Z.; Yang, M.; Wu, M.; Zhang, Q. Numerical simulation on the leakage and diffusion of the natural gas of the underground pipeline in the soil. J. Phys. Conf. Ser. 2021, 2083, 032082. [Google Scholar] [CrossRef]
- Liu, C.; Liao, Y.; Shaoxiong, W.; Li, Y. Quantifying leakage and dispersion behaviors for sub-sea natural gas pipelines. Ocean Eng. 2020, 216, 108107. [Google Scholar] [CrossRef]
- Yao, L.; Zhang, Y.; He, T.; Luo, H. Natural gas pipeline leak detection based on acoustic signal analysis and feature reconstruction. Appl. Energy 2023, 352, 121975. [Google Scholar] [CrossRef]
- Li, M.; Liu, B.; Chen, T.; Liu, R.; Guo, Y.; Hou, K. On-site leakage locating of underground natural gas pipeline based on Ne tracer by miniature time-of-flight mass spectrometry. Talanta 2023, 254, 124170. [Google Scholar] [CrossRef] [PubMed]
- Li, H.; Chu, L.; Lu, J.; Liu, Q.; Li, F.; Zhang, K. SVD-VMD algorithm and its application in leak detection of natural gas pipeline. Pet. Sci. Technol. 2022, 41, 230–255. [Google Scholar] [CrossRef]
- Xingyuan, M.; Hong, Z.; Zhaoyuan, X. Leakage detection in natural gas pipeline based on unsupervised learning and stress perception. Process Saf. Environ. Prot. 2023, 170, 76–88. [Google Scholar]
- Zhu, L.; Wang, D.; Yue, J.; Lu, J.; Li, G. Leakage detection method of natural gas pipeline combining improved variational mode decomposition and Lempel–Ziv complexity analysis. Trans. Inst. Meas. Control 2022, 44, 2865–2876. [Google Scholar] [CrossRef]
- Runchang, C.; Shan, C.; Yang, W.D.K.; Khoo, B.C. Modelling and quantification of measurement uncertainty for leak localisation in a gas pipeline. Meas. Sens. 2022, 24, 100443. [Google Scholar]
- Lu, J.; Fu, Y.; Yue, J.; Zhu, L.; Wang, D.; Hu, Z. Natural gas pipeline leak diagnosis based on improved variational modal decomposition and locally linear embedding feature extraction method. Process Saf. Environ. Prot. 2022, 164, 857–867. [Google Scholar] [CrossRef]
- Bu, F.; Chen, S.; Liu, Y.; Guan, B.; Wang, X.; Shi, Z.; Hao, G. CFD analysis and calculation models establishment of leakage of natural gas pipeline considering real buried environment. Energy Rep. 2022, 8, 3789–3808. [Google Scholar] [CrossRef]
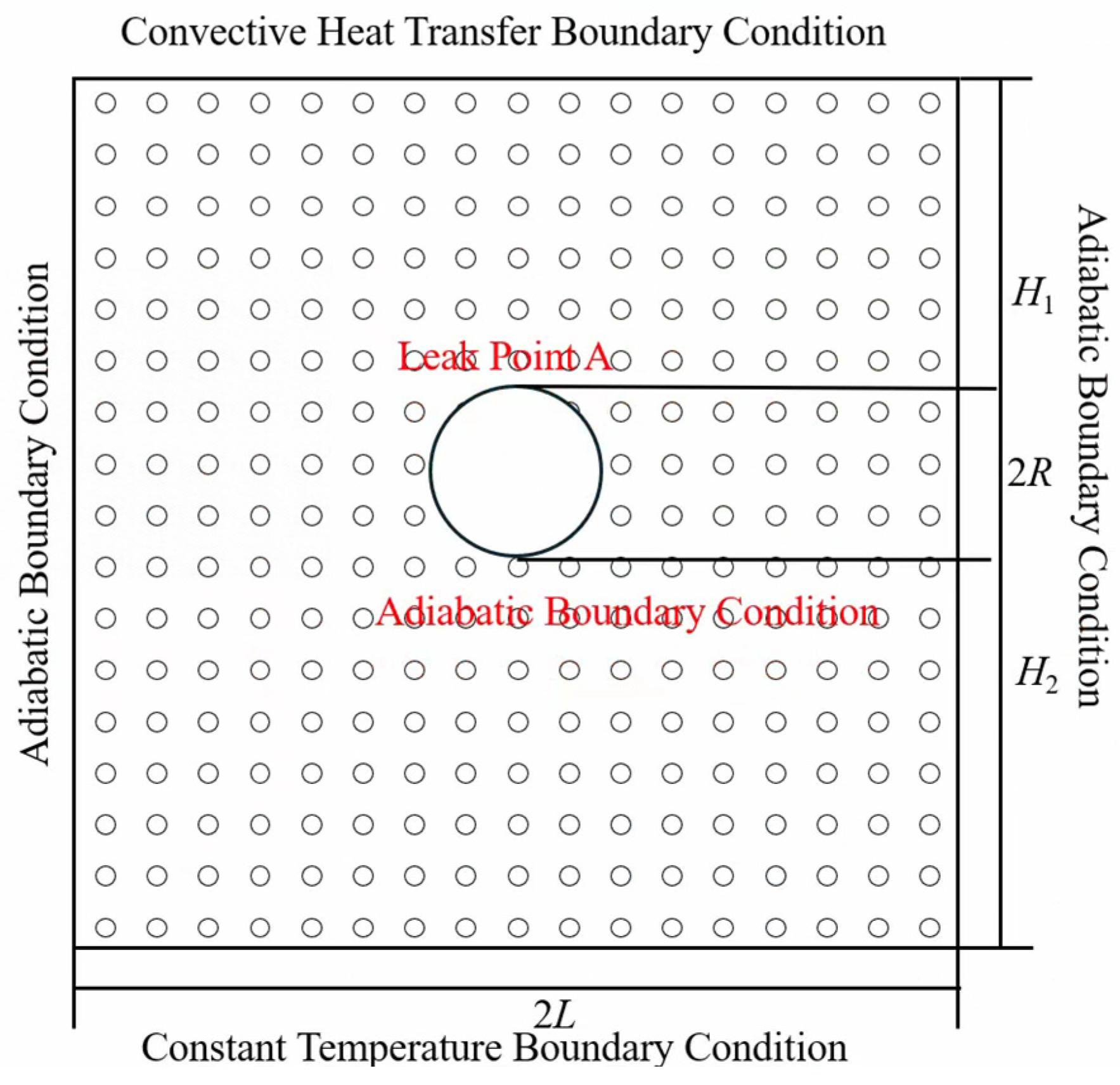

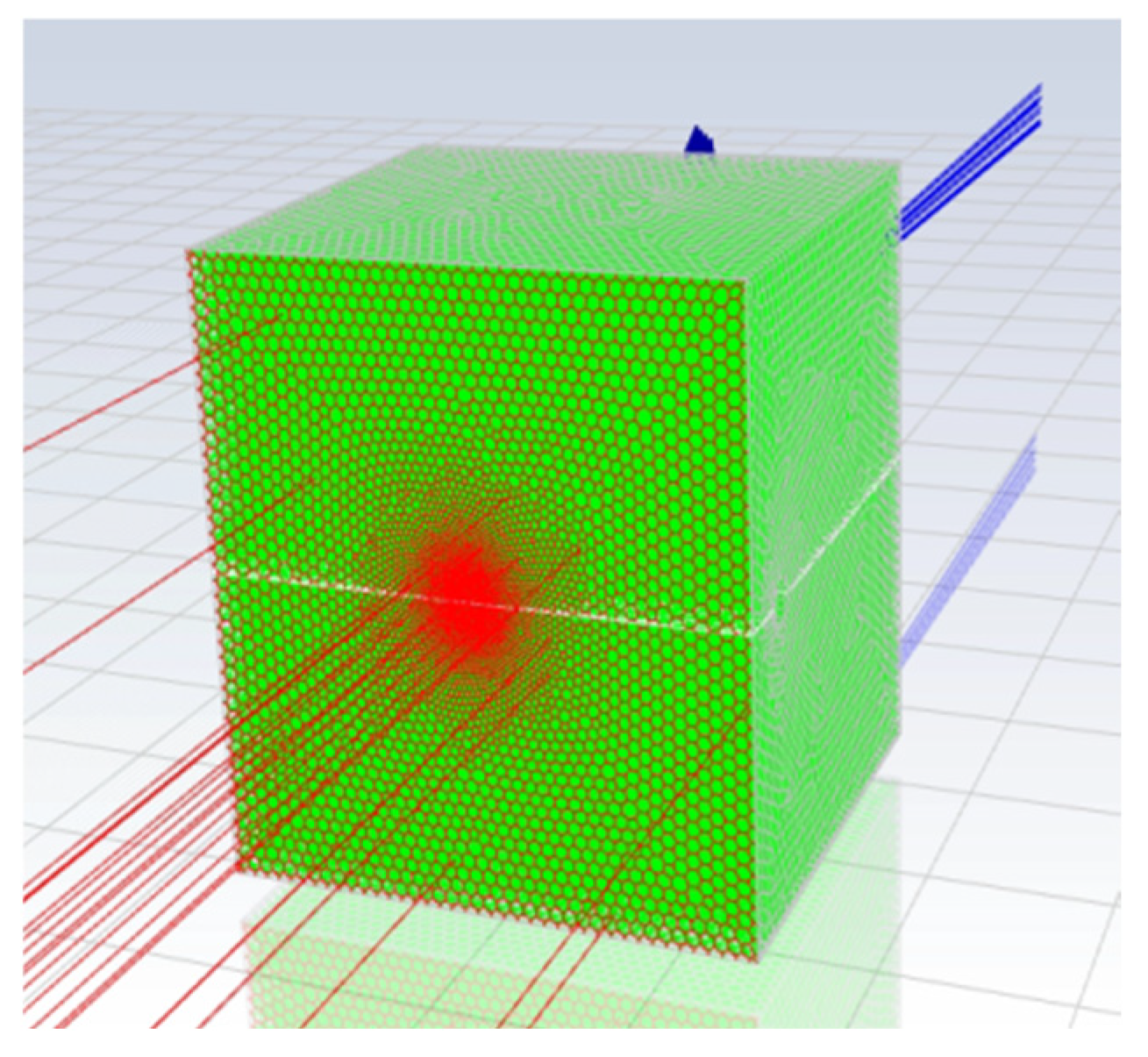
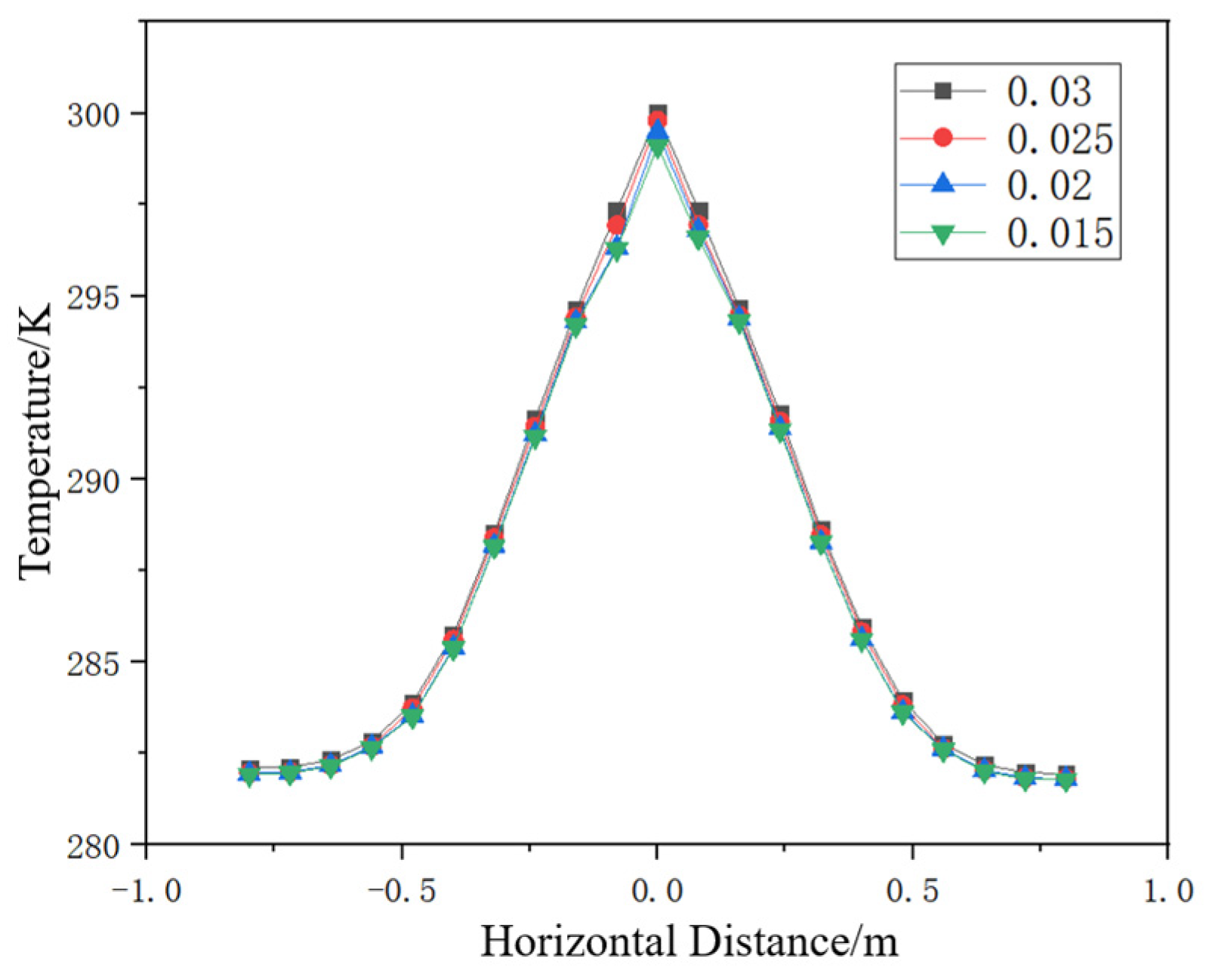
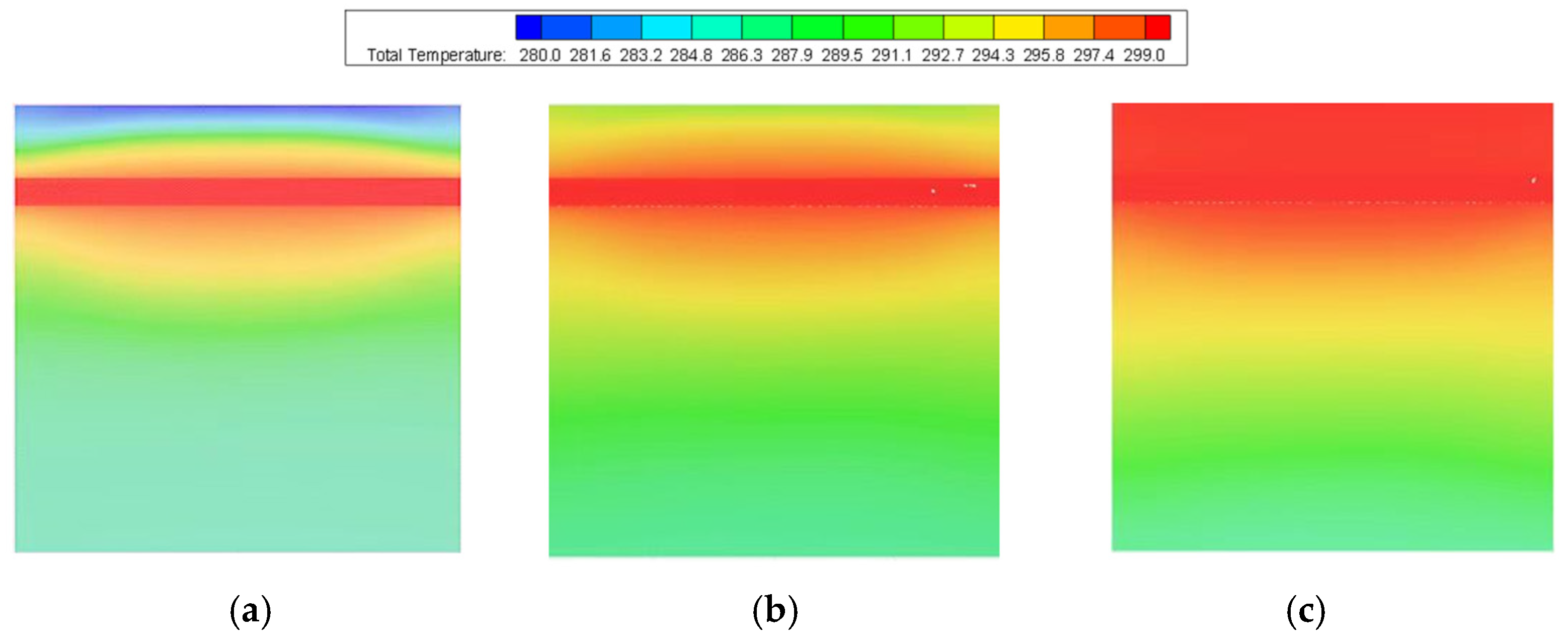




| Boundary Position | Boundary Naming |
|---|---|
| Pipeline Inlet | Inlet-Pipeline |
| Pipeline Outlet | Outlet-Pipeline |
| Ground Surface | Outlet-Top |
| Perimeter of the Computational Domain | Outlet-Around |
| Bottom of the Computational Domain | Outlet-Bottom |
| Interface Between Fluid in the Leakage Hole and Soil Region | Holefluid-Soilfluid |
| Interface Between Fluid in the Leakage Hole and Soil Region | Holefluid-Pipefluid |
| Outer Wall of the Pipeline | Wall-Outside |
| Inner Wall of the Pipeline | Wall-Inside |
| Side Wall of the Leakage Hole | Wall-Hole |
| No. | Minimum Element Size/mm | Maximum Element Size/mm | Mesh Count | Minimum Inverse Orthogonal Quality | Leakage Velocity /(m/s) |
|---|---|---|---|---|---|
| 1 | 2.5 | 500 | 246,040 | 0.08 | 10.516 |
| 2 | 2 | 500 | 250,512 | 0.24 | 16.532 |
| 3 | 1.5 | 500 | 256,881 | 0.41 | 17.876 |
| 4 | 1 | 500 | 114,659 | 0.35 | 20.361 |
| 5 | 1 | 300 | 289,793 | 0.21 | 20.164 |
| 6 | 1 | 100 | 966,446 | 0.20 | 20.157 |
| 7 | 0.5 | 100 | 968,095 | 0.26 | 20.284 |
| 8 | 0.3 | 100 | 907,170 | 0.32 | 20.080 |
| 9 | 0.1 | 100 | 930,152 | 0.32 | 20.045 |
| 10 | 0.05 | 100 | 988,188 | 0.23 | 20.448 |
| Case | Pressure (MPa) | Leak Diameter (mm) | Burial Depth (m) | Pipeline Diameter (mm) | Direction of Leakage |
|---|---|---|---|---|---|
| Case1 | 1 | 4 | 1.5 | 711 | Top |
| Case2 | 2 | 4 | 1.5 | 711 | Top |
| Case3 | 4 | 4 | 1.5 | 711 | Top |
| Case4 | 6 | 4 | 1.5 | 711 | Top |
| Case5 | 8 | 4 | 1.5 | 711 | Top |
| Case6 | 10 | 4 | 1.5 | 711 | Top |
| Case7 | 4 | 1 | 1.5 | 711 | Top |
| Case8 | 4 | 2 | 1.5 | 711 | Top |
| Case9 | 4 | 6 | 1.5 | 711 | Top |
| Case10 | 4 | 1 | 1.5 | 711 | Top |
| Case11 | 6 | 2 | 1.5 | 711 | Top |
| Case12 | 6 | 6 | 1.5 | 711 | Top |
| Case13 | 4 | 4 | 1.5 | 711 | Side |
| Case14 | 6 | 4 | 1.5 | 711 | Top |
| Case15 | 2 | 6 | 1.5 | 1016 | Top |
| Case16 | 4 | 6 | 1.5 | 1016 | Top |
| Case17 | 6 | 6 | 1.5 | 1016 | Top |
| Case18 | 8 | 6 | 1.5 | 1016 | Top |
| Case19 | 6 | 2 | 1.5 | 1016 | Top |
| Case20 | 6 | 4 | 1.5 | 1016 | Top |
| Case21 | 6 | 6 | 1.5 | 1219 | Top |
| Monitoring Points | Coordinates |
|---|---|
| Point 1 | (0, 0.465, 5) |
| Point 2 | (0, 0.565, 5) |
| Point 3 | (0, 0.665, 5) |
| Point 4 | (0, 0.765, 5) |
| Point 5 | (0.329, 0.329, 5) |
| Point 6 | (−0.329, 0.329, 5) |
| Point 7 | (0.4, 0.4, 5) |
| Point 8 | (−0.4, 0.4, 5) |
| Monitoring Points | Initial Sensing Times (s) |
|---|---|
| Point 1 | 13 |
| Point 5 | 1561 |
| Point 6 | 1554 |
| Monitoring Points | Initial Sensing Times (s) |
|---|---|
| Point 2 | 332 |
| Point 7 | 4351 |
| Point 8 | 4346 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chang, W.; Gu, X.; Zhang, X.; Gou, Z.; Zhang, X.; Xiong, Z. Numerical Study of the Soil Temperature Field Affected by Natural Gas Pipeline Leakage. Processes 2025, 13, 36. https://doi.org/10.3390/pr13010036
Chang W, Gu X, Zhang X, Gou Z, Zhang X, Xiong Z. Numerical Study of the Soil Temperature Field Affected by Natural Gas Pipeline Leakage. Processes. 2025; 13(1):36. https://doi.org/10.3390/pr13010036
Chicago/Turabian StyleChang, Weichun, Xiaolong Gu, Xiahua Zhang, Zenian Gou, Xin Zhang, and Zhiyi Xiong. 2025. "Numerical Study of the Soil Temperature Field Affected by Natural Gas Pipeline Leakage" Processes 13, no. 1: 36. https://doi.org/10.3390/pr13010036
APA StyleChang, W., Gu, X., Zhang, X., Gou, Z., Zhang, X., & Xiong, Z. (2025). Numerical Study of the Soil Temperature Field Affected by Natural Gas Pipeline Leakage. Processes, 13(1), 36. https://doi.org/10.3390/pr13010036






