Risk Analysis of Wellbore Instability of “Felt Layer” in Qiudong Sag Based on Monte Carlo Method
Abstract
1. Introduction
2. Wellbore Stability Analysis Model
2.1. Vertical Wellbore Wall Stress Distribution
2.2. Collapse Pressure Calculation Model
2.3. Fracture Pressure Calculation Model
3. Construction of Reliability Evaluation Method
3.1. Reliability Evaluation Method of Wellbore Instability Risk
- (1)
- : the structure satisfies functional requirements, indicating that the wellbore is in a stable state.
- (2)
- : the structure fails, leading to wellbore collapse or fracture.
- (3)
- : the structure is in a limit state, indicating that the wellbore is in a critical state.
3.2. Monte Carlo Simulations Method
- (1)
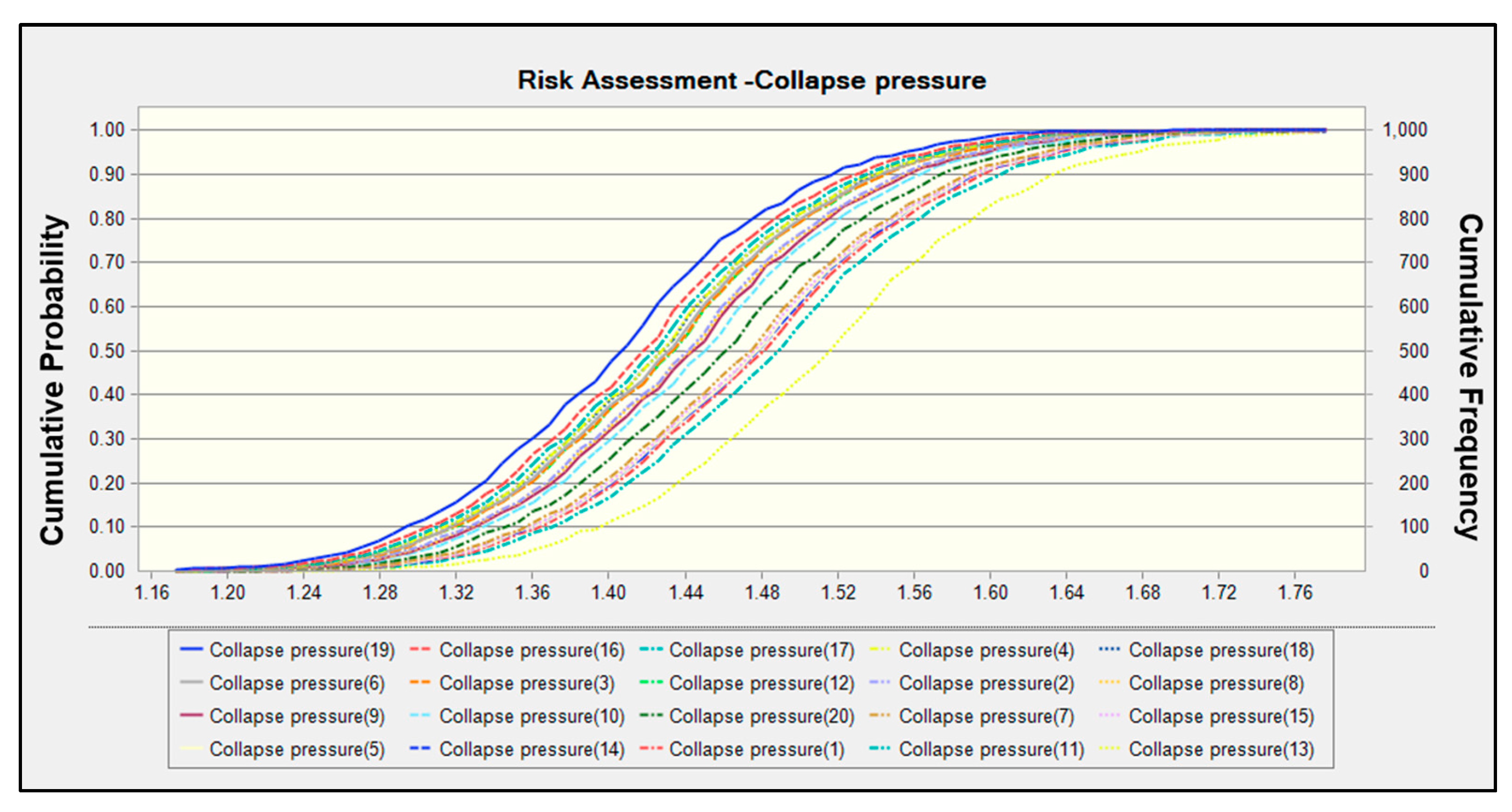
- Combining the probability distributions of geological mechanical parameters and employing the Monte Carlo simulation method, the simulation was conducted with 4000 iterations. During each iteration, 4000 random numbers, consistent with the characteristics of the probability distributions of geological mechanical parameters, were generated. These random numbers were then input into the models for formation collapse pressure and fracture pressure calculations. As a result, 4000 computation outcomes were obtained.
- (2)
- Statistically analyzing the computation results and based on the principles of normal information diffusion, the probability distributions of collapse pressure and fracture pressure were determined for different probability distributions of geological mechanical parameters. The mean, variance, and coefficient of variation for both collapse pressure and fracture pressure were obtained.
- (3)
- By comparing and analyzing the coefficients of variation in the probability distributions of collapse pressure and fracture pressure obtained under different probability distributions of geological mechanical parameters, we discuss the sensitivity of the random reliability variables of wellbore stability.
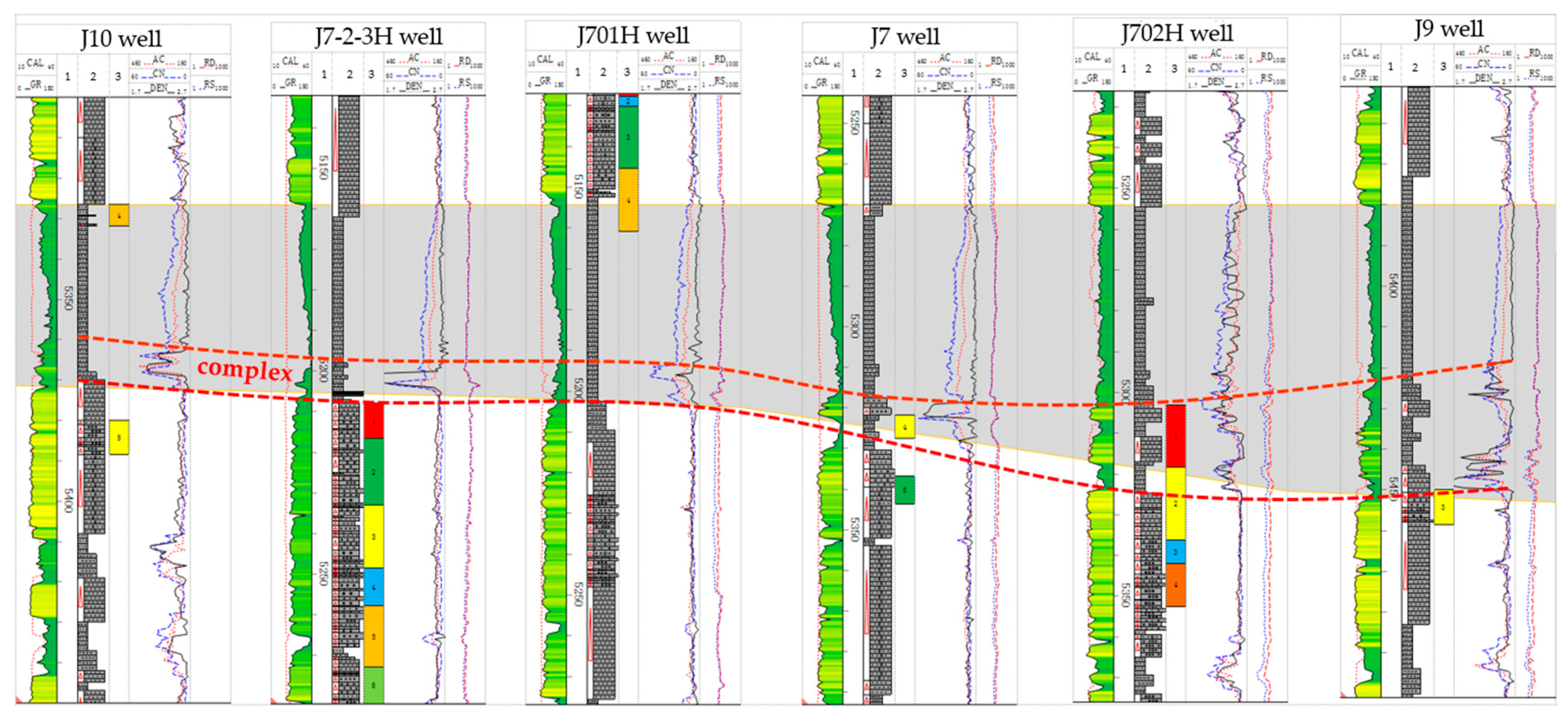
4. Applicable Analysis
4.1. Uncertainty Analysis of Input Parameters
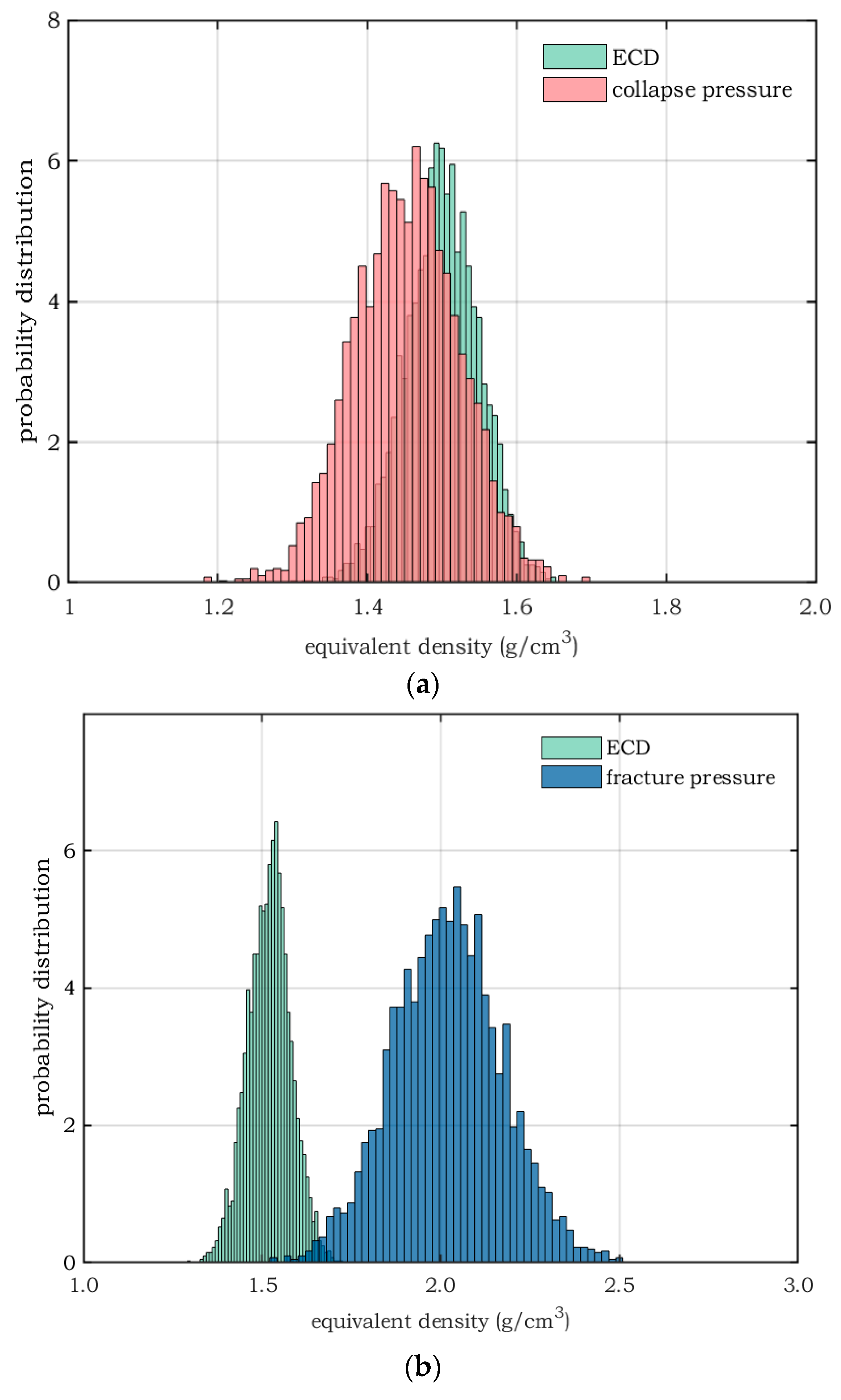
4.2. Quantitative Evaluation Results of Wellbore Stability
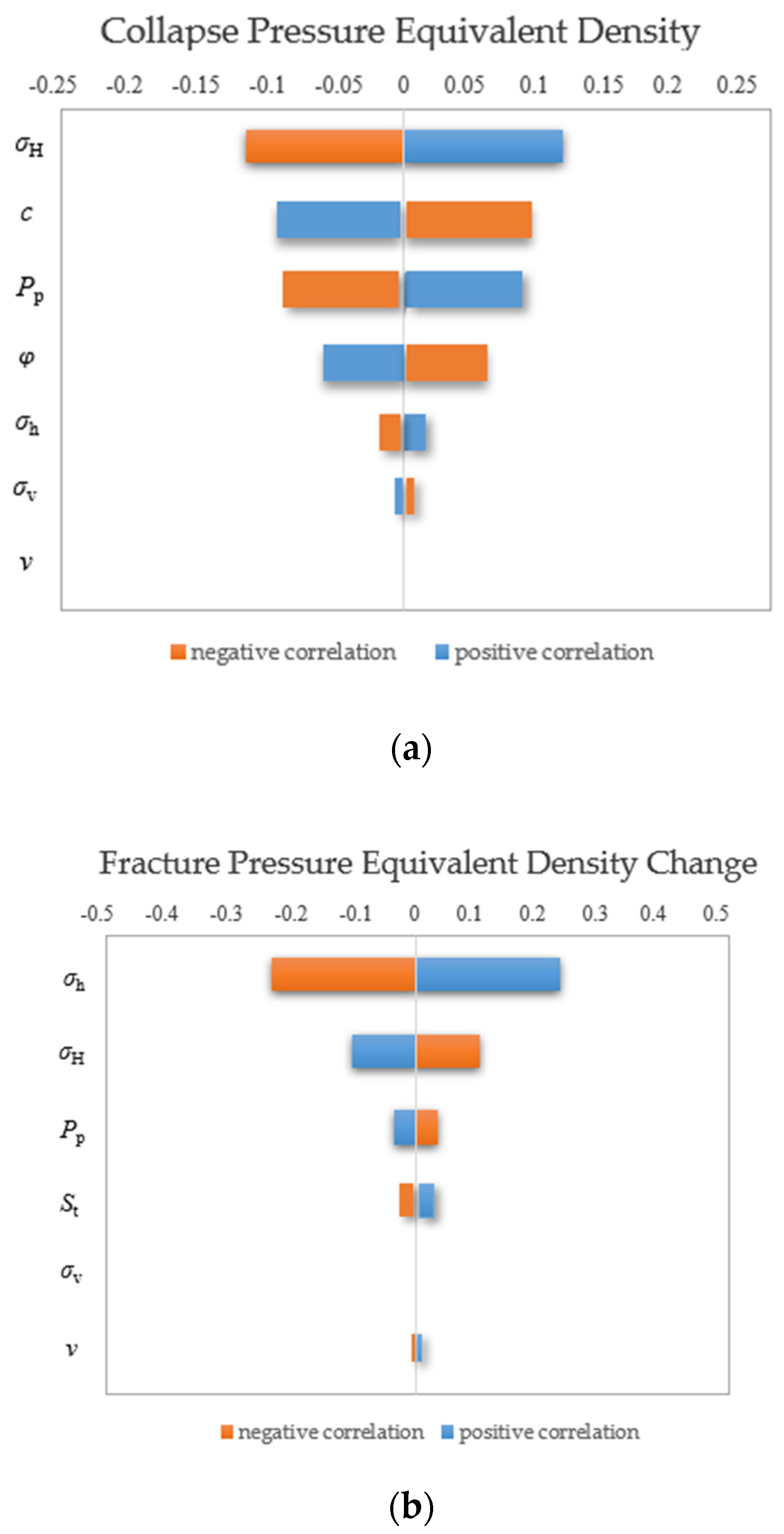
4.3. Parameter Mean Sensitivity Analysis
4.4. Risk Analysis of Wellbore Instability
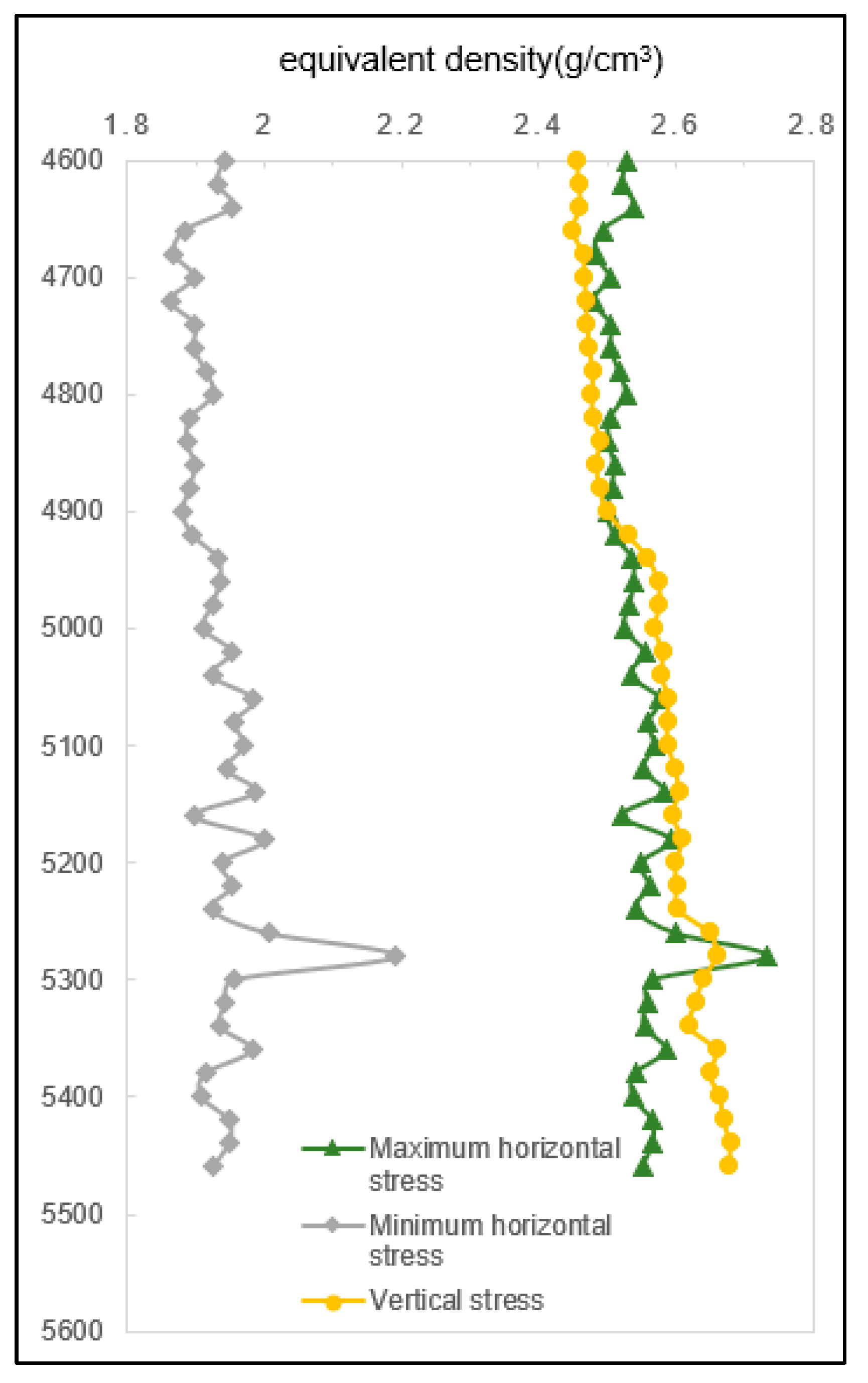
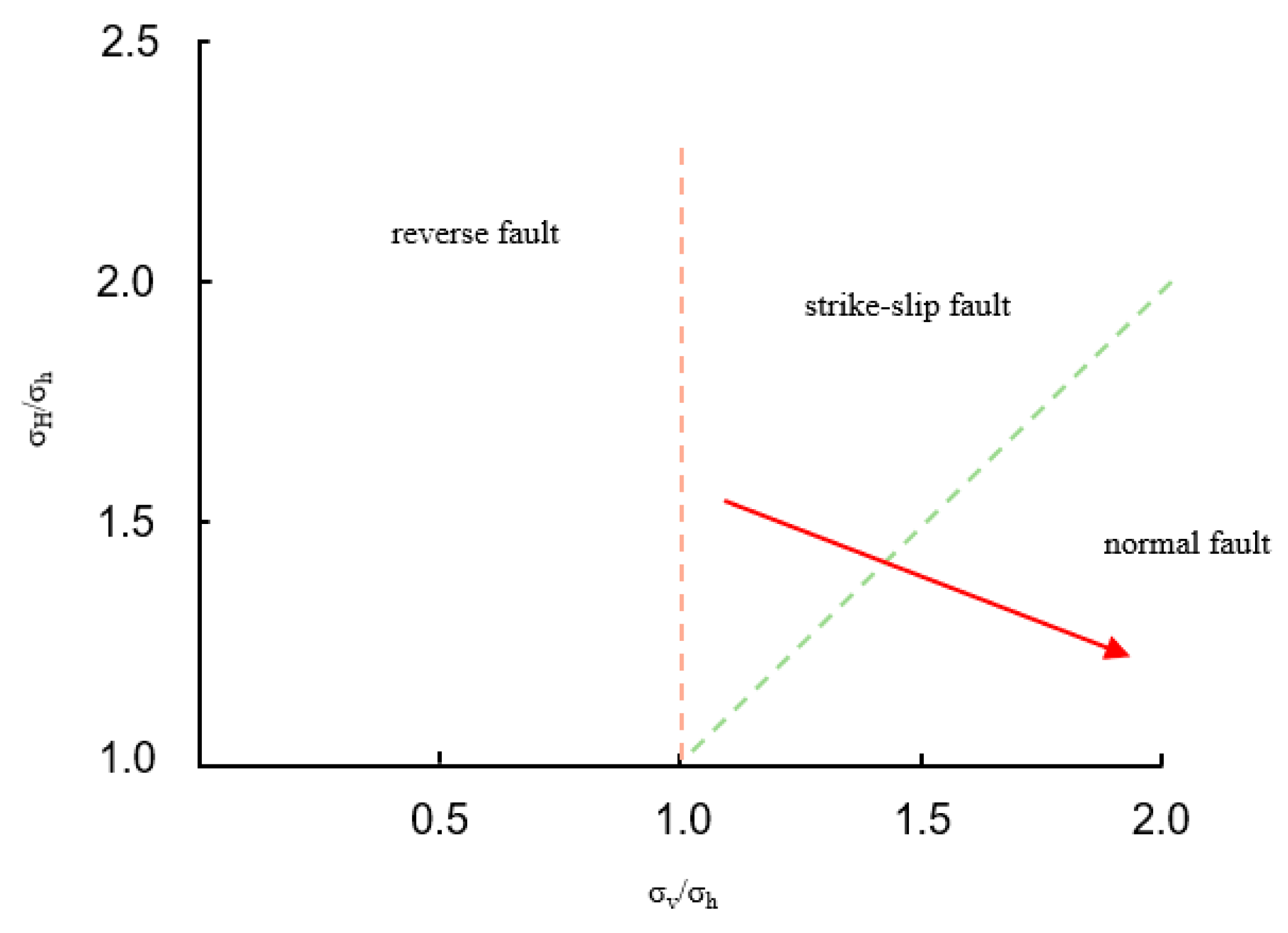
4.4.1. Geostress State Analysis
4.4.2. Two-Dimensional Simulation Validation
5. Conclusions
- (1)
- A wellbore stability analysis model was established, and Monte Carlo methods were used to simulate the reliability and instability probability of the wellbore. Quantitative analysis and calculations for wellbore stability were conducted based on the specific distribution characteristics of lithological parameters in the research area. The evaluation results obtained from the quantitative risk calculation model were consistent with the actual conditions. The case analysis validated the reliability and accuracy of wellbore instability risk analysis based on the Monte Carlo method.
- (2)
- The sensitivity ranking of geological and rock mechanics parameters under a normal distribution is as follows: geostress > pore pressure > rock strength. The use of two-dimensional simulation experiments clarified the impact of accurately determining the magnitude of geostress and reducing its uncertainty in wellbore stability analysis. This, in turn, enhances the accuracy of wellbore stability assessments.
- (3)
- In the deep formations of the Qiudong Depression, the stress field around the wellbore gradually transitions from a potential slip type to a potential normal faulting type. Under conditions of normal stress, with the increase in wellbore inclination, the stability of the wellbore gradually deteriorates. Straight wells exhibit better stability compared to directional or horizontal wells. Accurately analyzing and evaluating the impact of stress distribution in deep formations on wellbore stability is crucial for ensuring the economic and rapid drilling of deep wells.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lou, Y.; Jin, Y. Rock Mechanics and Petroleum Engineering; Petroleum Industry Publications: Beijing, China, 2006. [Google Scholar]
- Chen, M.; Jin, Y.; Zhang, G. Petroleum Related Rock Mechanics; Science Press: Beijing, China, 2008. [Google Scholar]
- Ottesen, S.; Zheng, R.H.; McCann, R.C. SPE/IADC 52864: Borehole Stability Assessment Using Quantitative Risk Analysis. In Drilling Conference; International Association of Drilling Contractors: Huston, TC, USA, 1999; Volume 2, pp. 313–316. [Google Scholar]
- de Fontoura, S.A.; Holzberg, B.B.; Teixeira, É.C.; Frydman, M. Probabilistic analysis of wellbore stability during drilling. In Proceedings of the SPE/ISRM Rock Mechanics Conference, Irving, TX, USA, 20–23 October 2002. [Google Scholar]
- Mostafavi, V.; Aadnoy, B.S.; Hareland, G. Model—Based uncertainty assessment of wellbore stability analyses and downhole pressure estimations. In Proceedings of the 45th U.S. Rock Mechanics/Geomechanics Symposium, San Francisco, CA, USA, 26–29 June 2011. [Google Scholar]
- Udegbunam, J.E.; Aadnøy, B.S.; Fjelde, K.K. Uncertainty evaluation of wellbore stability model predictions. J. Pet. Sci. Eng. 2014, 124, 254–263. [Google Scholar] [CrossRef]
- Gholami, R.; Rabiei, M.; Rasouli, V.; Aadnøy, B.S.; Fakhari, N. Application of quantitative risk assessment in wellbore stability analysis. J. Pet. Sci. Eng. 2015, 135, 185–200. [Google Scholar] [CrossRef]
- Plazas, F.; Calderon, Z.; Quintero, Y. Wellbore stability analysis:a stochastic approach applied to a Colombian Cretaceous Formation. In Proceedings of the SPE Latin American and Caribbean Petroleum Engineering Conference, Quito, Ecuador, 18–20 November 2015. [Google Scholar]
- Kinik, K.; Wojtanowicz, A.K.; Gumus, F. Probabilistic assessment of the temperature-induced effective fracture pressures. SPE Drill. Complet. 2016, 31, 40–52. [Google Scholar] [CrossRef]
- Zhang, L.; Yan, X.; Tian, Z.; Yang, H.; Yang, X. A collapse pressure analysis in reservoir drilling based on the reliability method. Acta Pet. Sin. 2012, 33, 477–482. [Google Scholar]
- Wei, K.; Ma, J.; Guan, Z. Method for determining the unstable area of wellbore and analysis of influencing factors. Mech. Pract. 2014, 36, 54–58. [Google Scholar]
- Ma, T.; Tang, T.; Chen, P.; Yang, C. Uncertainty evaluation of safe mud weight window utilizing the reliability assessment method. Energies 2019, 12, 942. [Google Scholar] [CrossRef]
- Zhang, L.; Bian, Y.; Zhang, S.; Yan, Y. A new analytical model to evaluate uncertainty of wellbore collapse pressure based on advantageous synergies of different strength criteria. Rock Mech. Rock Eng. 2019, 52, 2649–2664. [Google Scholar] [CrossRef]
- Zheng, D.; Miska, S.; Ozbayoglu, E.; Zhang, J. Combined experimental and well log study of anisotropic strength of shale. In Proceedings of the SPE Annual Technical Conference and Exhibition, San Antonio, TX, USA, 16–18 October 2023. [Google Scholar]
- Long, Z.; Zhong, J.; Ding, J.; Zeng, X. Wellbore stability of slimhole in deep shale-gas horizontal wells, Yongchuan southern area. Nat. Gas Technol. Econ. 2023, 17, 22–29. [Google Scholar]
- Lin, H.; Deng, J.; Xie, T.; Huo, H.; Chen, Z. Influence of Formation Anisotropy on Wellbore Stability of Hard and Brittle Mudstone. J. Northeast. Pet. Univ. 2021, 45, 85–94+10–11. [Google Scholar]
- Zheng, D.; Ozbayoglu, E.; Miska, S.; Zhang, J. Experimental study of anisotropic strength properties of shale. In Proceedings of the ARMA US Rock Mechanics/Geomechanics Symposium, Atlanta, GA, USA, 25–28 June 2023. [Google Scholar]
- Xu, S.; Liu, R.; Meng, X.; Liu, B.; Sun, Z.; Fu, J.; Zhai, X.; Zhang, J. Evaluation Method of Wellbore Stability in Mudstone Horizontal Section Based on Prediction Model of Collapse Angle and Collapse Depth of Borehole. Oil Drill. Prod. Technol. 2023, 45, 136–142. [Google Scholar]
- Zhao, G.; Jin, W.; Gong, J. Structural Reliability Theory; China Architecture & Building Press: Beijing, China, 2000. [Google Scholar]
- Zhang, L.; Zhang, J.; Xu, Y.; Li, X. Reliability Theory in Geotechnical Engineering; Tongji University Press: Shanghai, China, 2011. [Google Scholar]
- Zhang, L.L.; Zhang, J.; Xu, Y.; Li, X. Analysis of API formulas and calculation of casing strength based on reliability theory. Pet. Sin. 2007, 28, 122–126. [Google Scholar]
- Liao, H.; Guan, Z.; Yan, Z.; Ma, G. Assessment method of casing failure risk based on structure reliability theory. Acta Pet. Sin. 2010, 31, 161–164. [Google Scholar]
- Sheng, Y.; Guan, Z.; Xu, Y.; Wang, Q.; Zhang, B. Exploration of Uncertainty Analysis Methods for Wellbore Stability Issues. Fault-Block Oil Gas Field 2017, 24, 847–850+866. [Google Scholar]
- Ye, J. The Application of ANSYS in Structural Reliability Analysis. Civ. Eng. Archit. Guangdong 2008, 4, 27–28. [Google Scholar]
- Guan, Z.; Shegn, Y. The quantitative method of ECD uncertainty based on Uncertainty Theory. Drill. Complet. Fluid 2018, 35, 62–67. [Google Scholar]
- Wang, Q. Quantitative evaluation method of wellbore stability based on reliability theory. Sci. Technol. Eng. 2022, 22, 11880–11886. [Google Scholar]
- Deng, J.; Zhang, H. Mechanical Mechanisms of Borehole Instability in Drilling Engineering; Petroleum Industry Press: Beijing, China, 1998. [Google Scholar]
- Li, Y.; Fu, Y.; Tang, G.; She, Z.; Zhang, J. Influence of Ground Stress Types on the Stability of Directional Wellbore Walls. Nat. Gas Ind. 2012, 32, 78–80. [Google Scholar]

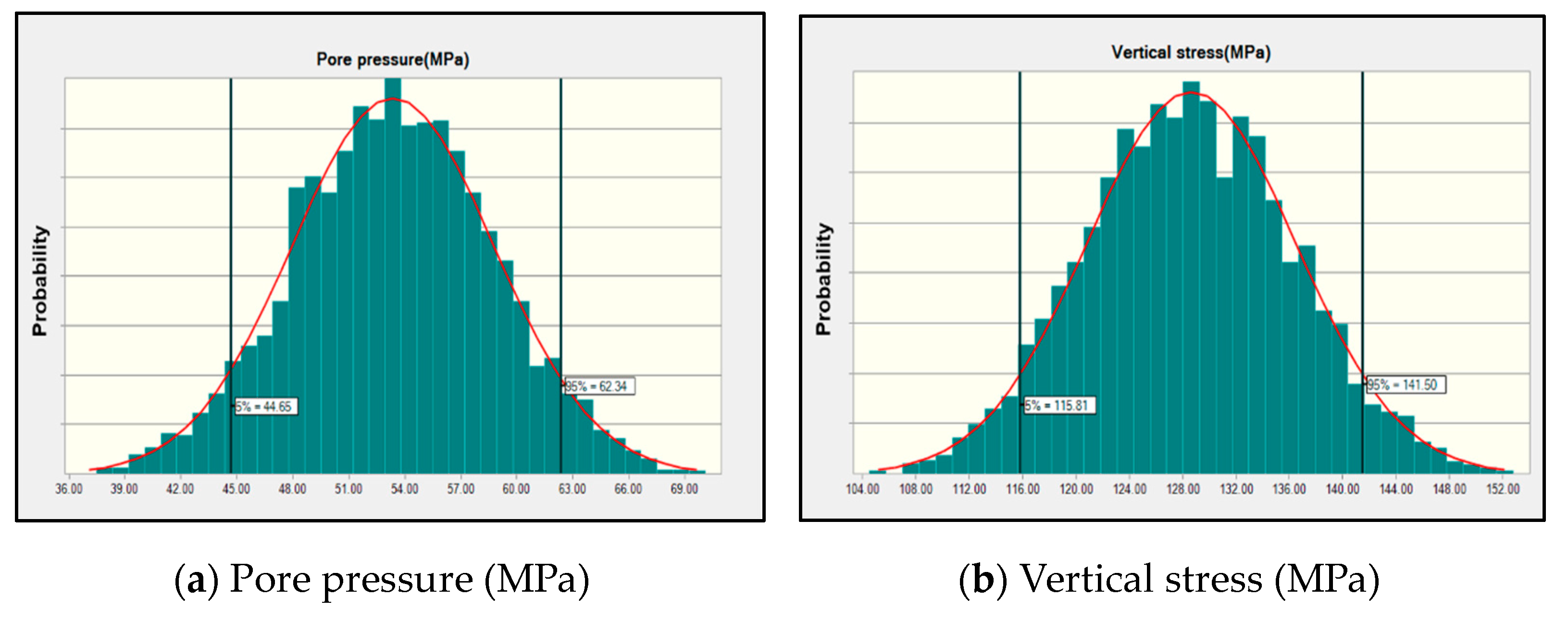
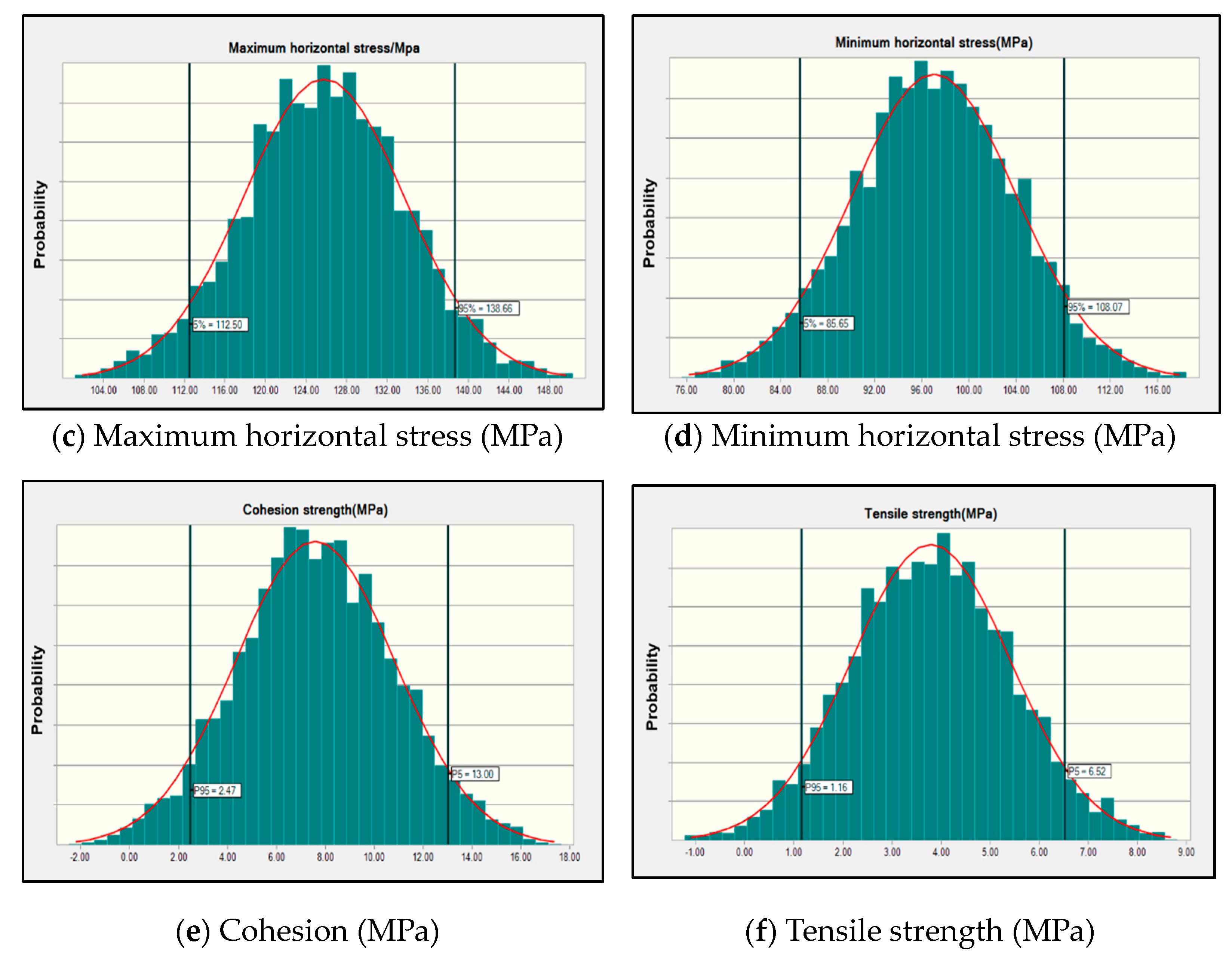
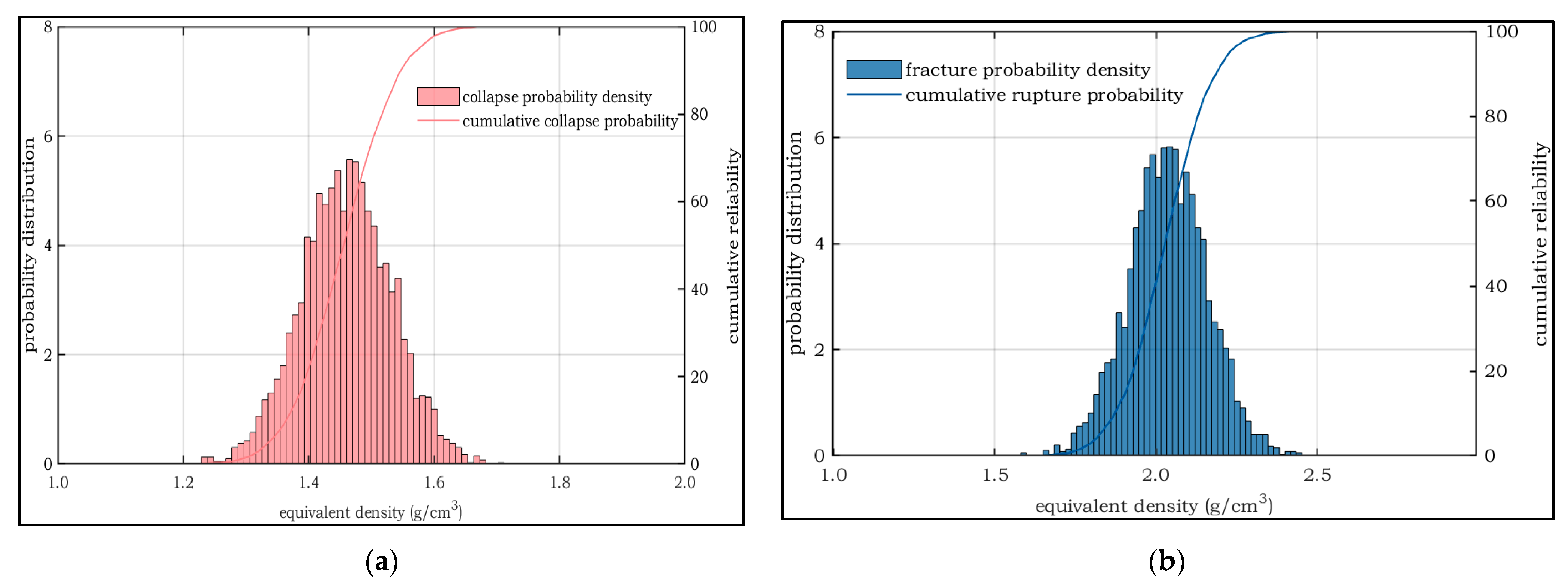



| Random Variable | Mean | Standard Deviation | Coefficient of Variation | Cumulative Probability | |
|---|---|---|---|---|---|
| 5% | 95% | ||||
| Vertical stress/MPa | 128.65 | 7.80 | 0.06 | 115.81 | 141.50 |
| Maximum horizontal stress/MPa | 125.75 | 7.93 | 0.06 | 112.50 | 138.66 |
| Minimum horizontal stress/MPa | 97.03 | 6.93 | 0.07 | 85.65 | 108.07 |
| Pore pressure/MPa | 53.35 | 5.41 | 0.10 | 44.65 | 62.34 |
| Cohesion/MPa | 7.58 | 3.25 | 0.43 | 2.45 | 12.98 |
| Rock internal friction angle/° | 41.51 | 2.9 | 0.07 | 36.91 | 46.22 |
| Tensile strength/MPa | 3.79 | 1.62 | 0.43 | 1.10 | 6.52 |
| Amount | Mean g/cm3 | Standard Deviation g/cm3 | Coefficient of Variation | Cumulative Probability | Minimum Value g/cm3 | Maximum Value g/cm3 | |
|---|---|---|---|---|---|---|---|
| 5% | 95% | ||||||
| 4000 | 1.45 | 0.07 | 0.0483 | 1.27 | 1.64 | 1.11 | 1.78 |
| Amount | Mean g/cm3 | Standard Deviation g/cm3 | Coefficient of Variation | Cumulative Probability | Minimum Value g/cm3 | Maximum Value g/cm3 | |
|---|---|---|---|---|---|---|---|
| 5% | 95% | ||||||
| 4000 | 2.04 | 0.15 | 0.0735 | 1.98 | 2.94 | 1.51 | 2.57 |
| Name | Depth /m | Maximum Horizontal Stress /MPa | Minimum Horizontal Stress /MPa | Vertical Stress /MPa |
|---|---|---|---|---|
| J3801H | 2687 | 68.09 | 57.21 | 59.75 |
| Jf101H | 2894.16 | 71.19 | 59.75 | 60.30 |
| 3257.97 | 80.52 | 70.19 | 68.68 | |
| J7H | 4699.57 | 125.96 | 107.43 | 103.1 |
| J10 | 5366 | 135.54 | 101.09 | 140.17 |
| 5383.27 | 134.31 | 115.51 | 144.13 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jia, R.; Zhu, L.; Song, X.; Chen, J. Risk Analysis of Wellbore Instability of “Felt Layer” in Qiudong Sag Based on Monte Carlo Method. Processes 2024, 12, 578. https://doi.org/10.3390/pr12030578
Jia R, Zhu L, Song X, Chen J. Risk Analysis of Wellbore Instability of “Felt Layer” in Qiudong Sag Based on Monte Carlo Method. Processes. 2024; 12(3):578. https://doi.org/10.3390/pr12030578
Chicago/Turabian StyleJia, Ruixue, Liang Zhu, Xiaoping Song, and Jian Chen. 2024. "Risk Analysis of Wellbore Instability of “Felt Layer” in Qiudong Sag Based on Monte Carlo Method" Processes 12, no. 3: 578. https://doi.org/10.3390/pr12030578
APA StyleJia, R., Zhu, L., Song, X., & Chen, J. (2024). Risk Analysis of Wellbore Instability of “Felt Layer” in Qiudong Sag Based on Monte Carlo Method. Processes, 12(3), 578. https://doi.org/10.3390/pr12030578






