Hydraulic Support Liquid Supply System Adaptive Pump Controlled Pressure Stabilization Control Under Strong Time-Varying Load
Abstract
1. Introduction
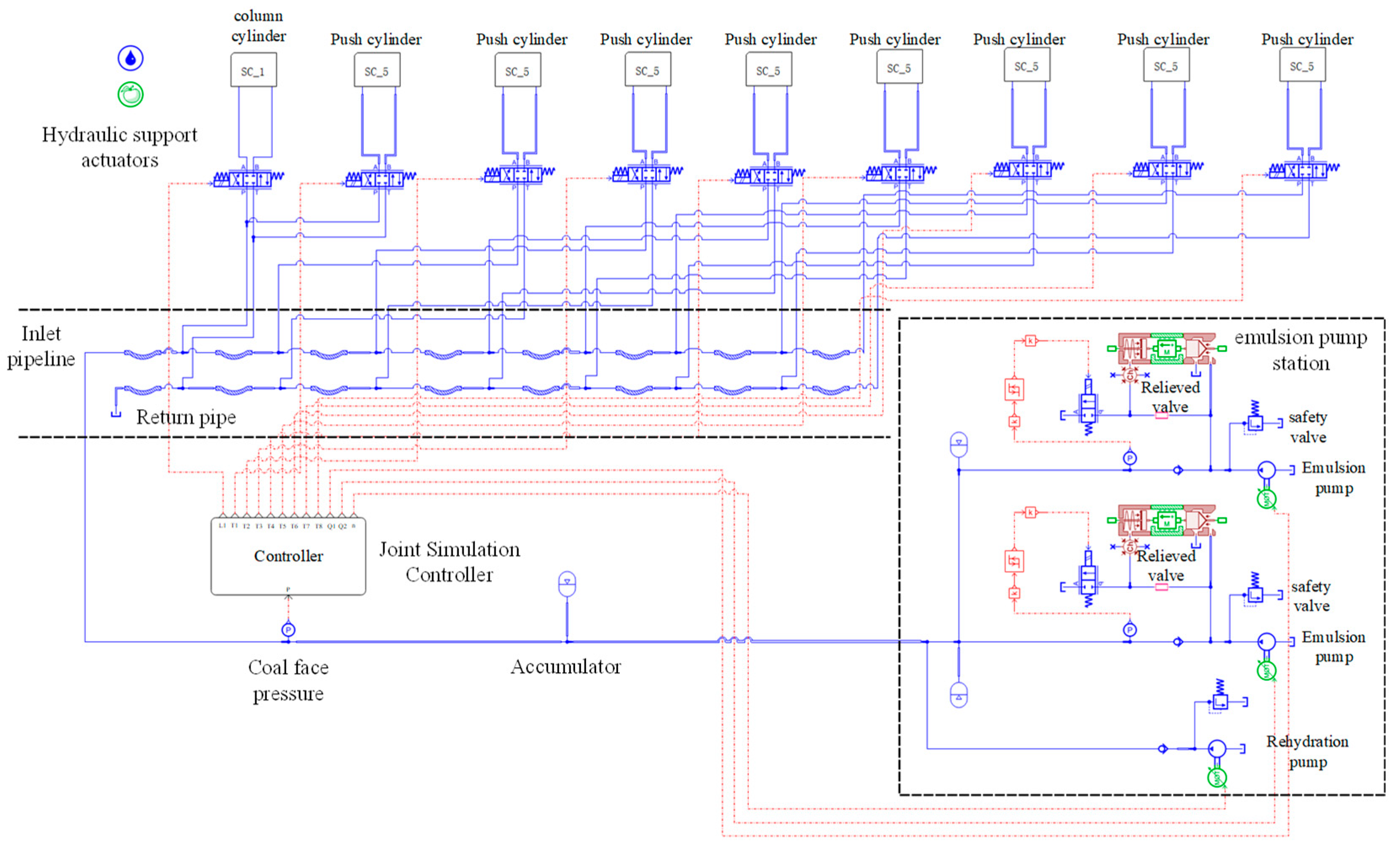
2. Liquid Supply System Configuration and Control Principle
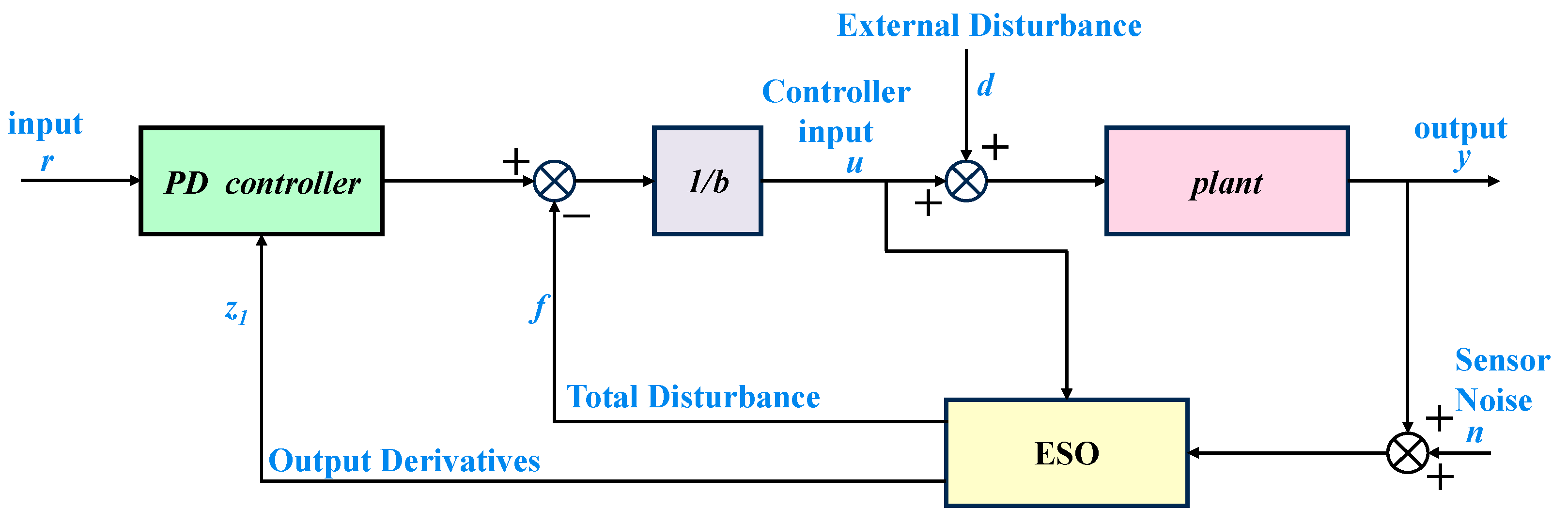
3. Controller Design
3.1. Configure System Configuration Parameters
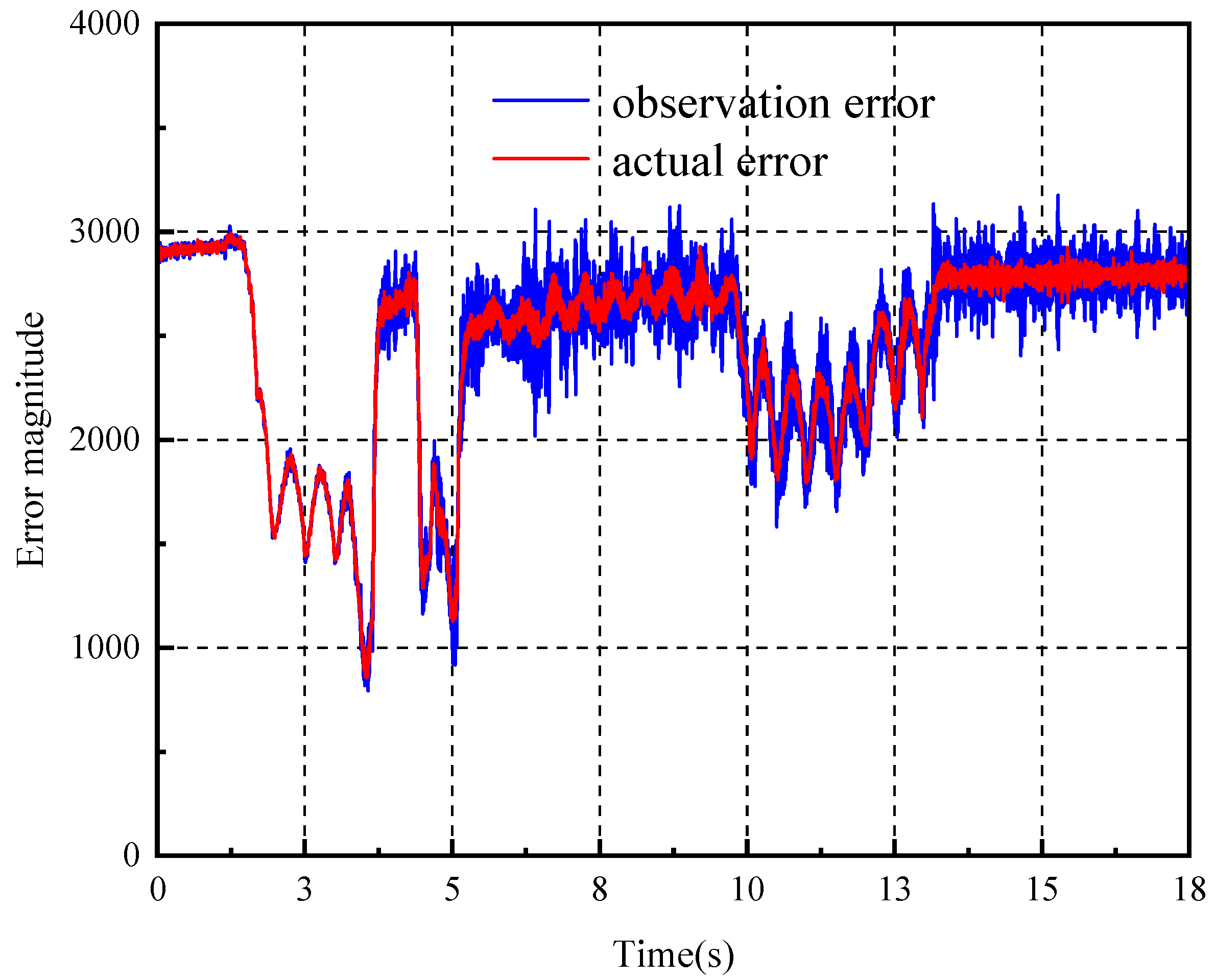
3.2. Extended State Observer Design
4. Control Characteristic Analysis
4.1. Simulation Design
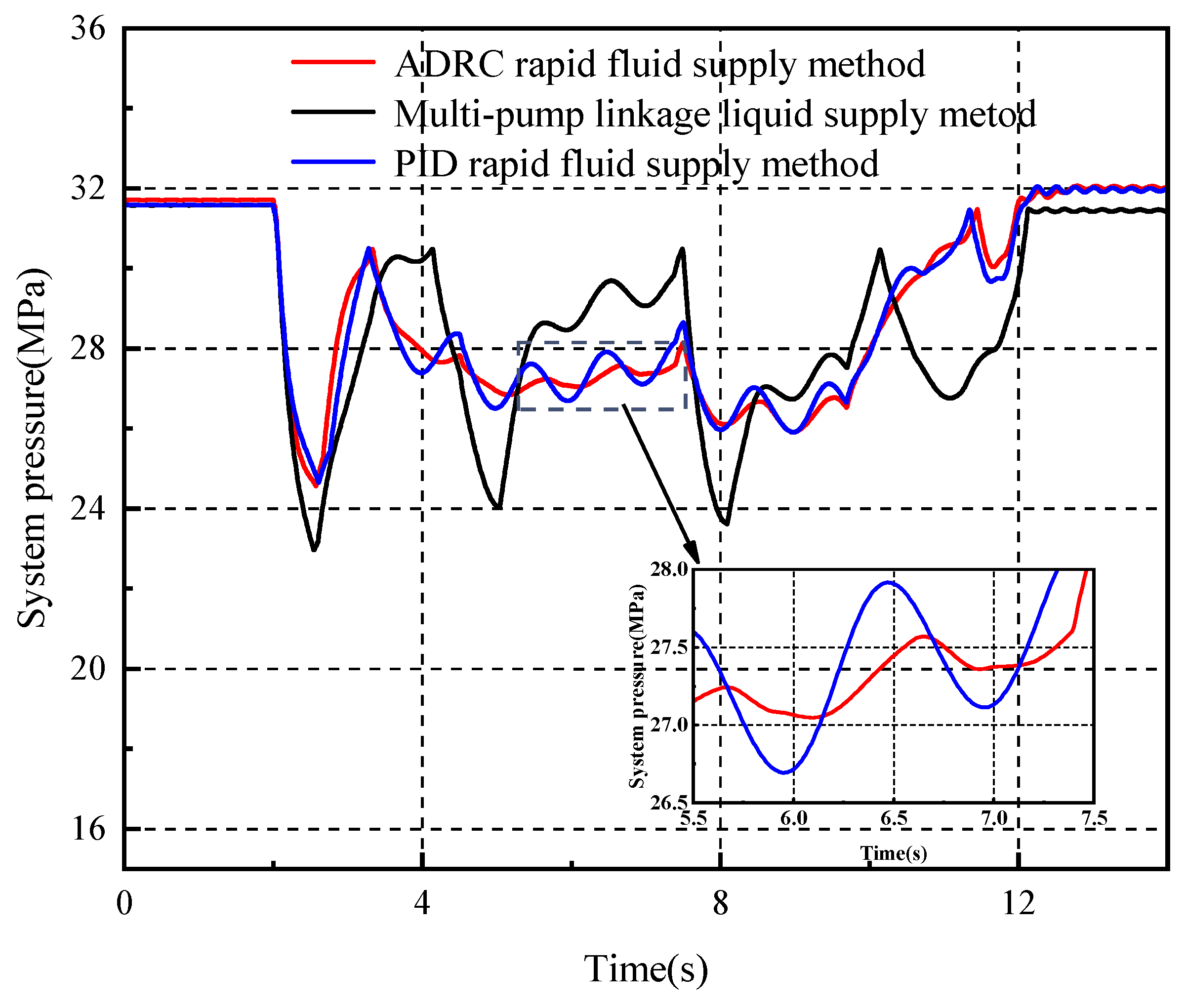
4.2. Simulation Result
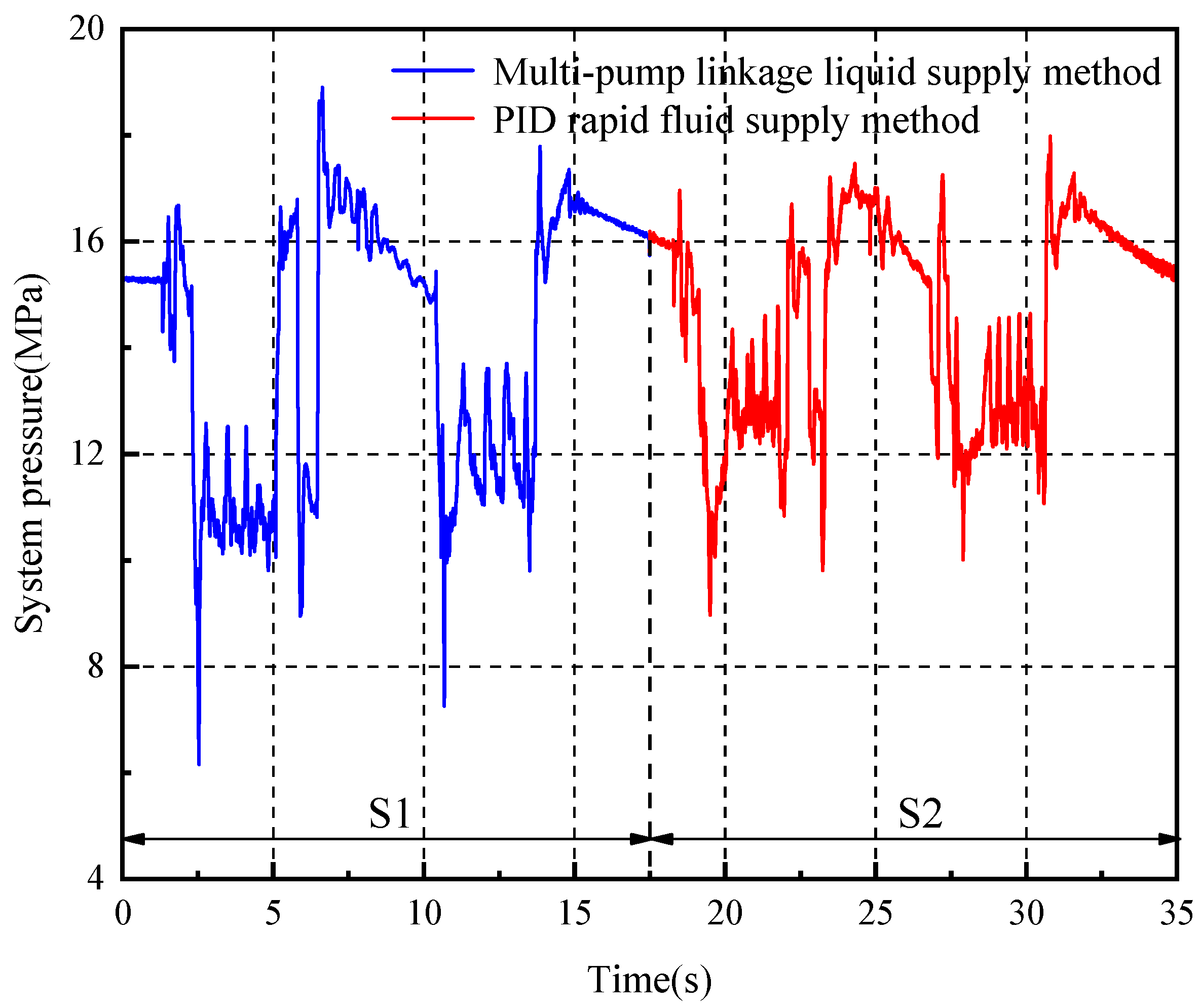
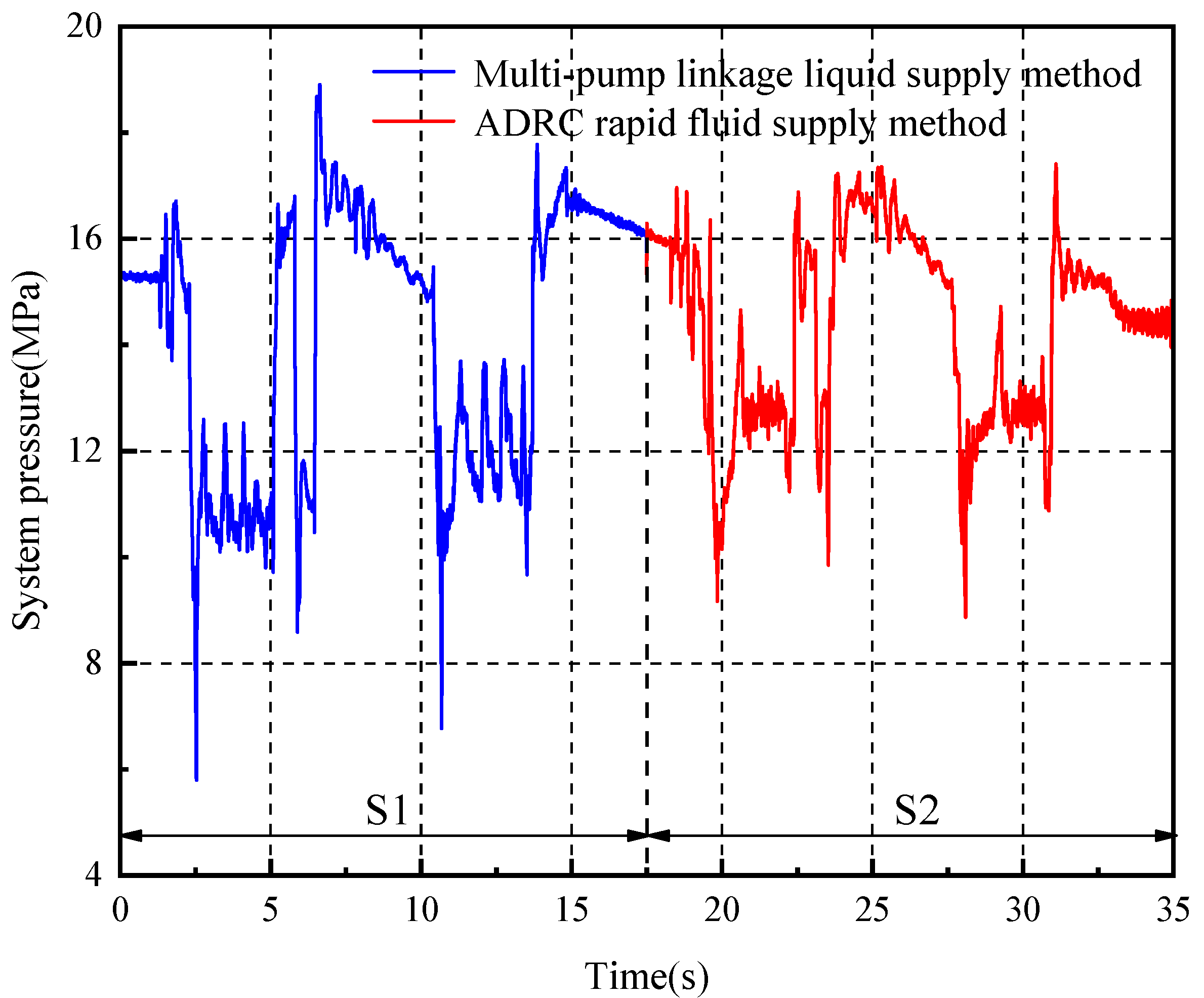
5. Experiment
5.1. Design of Experiment
5.2. Experimental Result
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wang, C.; Wang, W.; Guo, Y.; Zhao, D. Multi-condition simulation analysis of a sliding hydraulic support system based on elastoplastic mechanics. Sci. Rep. 2024, 14, 10663. [Google Scholar] [CrossRef] [PubMed]
- Zeng, X.; Wang, D. Optimal tracking control of the coal mining face fluid supply system via adaptive dynamic programming. Sci. Rep. 2023, 13, 20002. [Google Scholar] [CrossRef] [PubMed]
- Liu, X.; Yang, Z.; Yang, C.P.; Geng, J.G. 1250 L/min high flow high-end intelligent emulsion pump system to assist 10 million tons of coal face in Hongliulin Coal Mine. Intell. Min. 2023, 4, 44–47. [Google Scholar]
- Wang, G.F. Hydraulic Support Control Technology, 1st ed.; Beijing University of Aeronautics and Astronautics Press: Beijing, China, 2010; pp. 1–10. [Google Scholar]
- Zeng, Q.L.; Xu, W.Q.; Gao, K.D. Measurement Method and Experiment of Hydraulic Support Group Attitude and Straightness Based on Binocular Vision. IEEE Trans. Instrum. Meas. 2023, 72, 7502814. [Google Scholar] [CrossRef]
- Mamcic, S.; Bogdevicius, M. Simulation of dynamic processes in hydraulic accumulators. Transport 2010, 25, 215–221. [Google Scholar] [CrossRef]
- Van de Ven, J.D. Constant pressure hydraulic energy storage through a variable area piston hydraulic accumulator. Appl. Energy 2013, 105, 262–270. [Google Scholar] [CrossRef]
- Ma, Y.; Zhang, D.S.; Zhao, S.; Zhang, S. Research and application of liquid supply system with distributed energy accumulators in fully-mechanized mining face. Coal Sci. Technol. 2024, 52, 238–247. [Google Scholar]
- Li, Z.H. Research and analysis on main supply and return system of hydraulic support for large mining height. Mech. Manag. Dev. 2018, 33, 46–48. [Google Scholar]
- Shu, F.; Wang, Y.; Li, J. Simulate calcultion about pressure loss in hydraulic pipe system of roof supports. Coal Technol. 2009, 28, 26–28. [Google Scholar]
- Zhao, X. Pressure Loss and Dynamic Characteristics of Emulsion Supply and Return Pipeline for Hydraulic Support; Taiyuan University of Science and Technology: Taiyuan, China, 2018. [Google Scholar]
- Li, W.; Wei, J. Control strategy for multi-pump emulsion power station in coal mine face. J. Coal Sci. Eng. 2011, 17, 443–446. [Google Scholar] [CrossRef]
- Cao, L.; Xu, G.; Su, G.; Cui, J.; Cai, Y.; Cao, H. Design of automatic pressurizing device of hydraulic support initial force. Energy Sci. Eng. 2020, 8, 2868–2877. [Google Scholar] [CrossRef]
- Tian, C.; Su, C.; Zhao, W. Application of PLC in Variable Frequency Constant Pressure Control System for Mine Emulsion Pump Station. J. Phys. Conf. Ser. 2021, 1982, 012187. [Google Scholar] [CrossRef]
- Bordeasu, D.; Prostean, O.; Filip, I.; Vasar, C. Adaptive Control Strategy for a Pumping System Using a Variable Frequency Drive. Machines 2023, 11, 688. [Google Scholar] [CrossRef]
- Du, B.H. Research on Adapt Liquid Supply System of Hydraulic Support Running Double Pumps in Fully Mechanized Mining Face; Taiyuan University of Science and Technology: Taiyuan, China, 2023. [Google Scholar]
- Tan, C.; Qi, N.; Zhou, X.; Liu, X.; Yao, X.; Wang, Z.; Si, L. A pressure control method for emulsion pump station based on elman neural network. Comput. Intell. Neurosci. 2015, 2015, 684096. [Google Scholar] [CrossRef] [PubMed]
- Li, R.; Wei, W.S.; Lai, Y.H. Long-distance intelligent liquid supply for coal mining faces based on liquid demand prediction. J. Phys. Conf. Ser. 2023, 2561, 012018. [Google Scholar] [CrossRef]
- Tian, C.; Wei, X.W.; Zheng, Y. The intelligent control of emulsion pump station. J. Phys. Conf. Ser. 2021, 1881, 022044. [Google Scholar] [CrossRef]
- Tian, C.; Wei, X.W.; Yang, C.; Su, C. Optimization Technology of frequency conversion constant pressure control system of mine emulsion pump station in electrical engineering and automation Specialty. Adv. Civ. Eng. 2021, 2021, 5569994. [Google Scholar] [CrossRef]
- Peng, Y.; Kou, Z.; Wu, J.; Luo, J.; Liu, H.; Zhang, B. Research on a Pressure Control Method for a Liquid Supply System Based on Online Updating of a Radial Basis Function Neural Network. Processes 2023, 12, 57. [Google Scholar] [CrossRef]
- Si, M.; Wu, B.; Wang, Z. Research on large flow intelligent liquid supply system in fully mechanized working face. Mine Autom. 2022, 48, 66–72. [Google Scholar]
- Wang, G.F.; Zhang, L.; Li, S.B.; Li, S.; Feng, Y.H.; Meng, L.Y.; Nan, B.F.; Du, M.; Fu, Z.; Li, R.; et al. Progresses in theory and technological development of unmanned smart mining system. J. China Coal Soc. 2023, 48, 34–53. [Google Scholar]
- Zhang, W.Q. Research on Intelligent Linkage Control between Frequency Conversion and Electromagnetic Unloading of Emulsion Pump. Coal Mine Mach. 2013, 34, 196–198. [Google Scholar]
- Cao, C.; Zhao, J.; Gao, K.; Wang, H.; Li, R.; Liu, H.; Zhang, H. Rapid Pump-controlled Replenishment Pressure-stabilizing Method of the Hydraulic Support Fluid Supply System. Coal Sci. Technol. 2024, 1–12. Available online: http://kns.cnki.net/kcms/detail/11.2402.TD.20240613.1528.007.html (accessed on 23 November 2024).
- Fu, X.; Wang, R.F.; Zhao, Y.S. Simulation of hydraulic system of working face support and Research on stabilized pressure fluid supply technology. J. China Coal Soc. 2018, 43, 1471–1478. [Google Scholar]
- Zeng, X.T.; Wang, D.L. Analysis of the Influence of Accumulator Configuration on Stable Liquid Supply. Int. J. Simul. Model. 2023, 1, 52–63. [Google Scholar] [CrossRef]
- Wang, Y.; Zhao, J.; Zhang, H.; Wang, H.; Wang, J. An Output Cooperative Controller for a Hydraulic Support Multi-Cylinder System Based on Neural Network Compensation. Mathematics 2024, 12, 1866. [Google Scholar] [CrossRef]
- Abhilash, P.; Spandan, R.; Simone, B. Wide-Area Damping Control Resilience Towards Cyber-Attacks: A Dynamic Loop Approach. IEEE Trans. Smart Grid 2021, 12, 3438–3447. [Google Scholar]
- Xie, B.; Yang, Y. Study on Working Characteristics of 4-Column Hydraulic Support in Lifting–Lowering–Moving State Based on Microcontact Theory and Rigid–Flexible–Mechanical–Hydraulic Coupling Simulation. Actuators 2024, 13, 193. [Google Scholar] [CrossRef]
- Ren, B.; Ding, K.; Wang, L.; Wang, S.; Jiang, C.; Guo, J. Research on an Intelligent Mining Complete System of a Fully Mechanized Mining Face in Thin Coal Seam. Sensors 2023, 23, 9034. [Google Scholar] [CrossRef]




| Parameter Name | Parameter Value |
|---|---|
| Emulsion bulk modulus | 1900 MPa |
| The first emulsion pump flow | 400 L/min |
| The first emulsion unloading valve set pressure | 31.5–26.5 MPa |
| The second emulsion pump flow | 400 L/min |
| The second emulsion unloading valve set pressure | 30.5–25.5 MPa |
| Gas volume of accumulator | 18.4 L |
| Pipe diameter | 65 mm |
| Parameter Name | Parameter Value |
|---|---|
| Emulsion bulk modulus | 1900 MPa |
| The first emulsion pump flow | 125 L/min |
| The first emulsion unloading valve set pressure | 17–15 MPa |
| The second emulsion pump flow | 80 L/min |
| The second emulsion unloading valve set pressure | 16–13 MPa |
| Gas volume of accumulator | 18 L |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cao, C.; Gao, K.; Wang, H.; Pan, Y.; Deng, Z.; Xu, H.; Huang, D.; Zhao, X.; Zhao, J. Hydraulic Support Liquid Supply System Adaptive Pump Controlled Pressure Stabilization Control Under Strong Time-Varying Load. Processes 2024, 12, 2774. https://doi.org/10.3390/pr12122774
Cao C, Gao K, Wang H, Pan Y, Deng Z, Xu H, Huang D, Zhao X, Zhao J. Hydraulic Support Liquid Supply System Adaptive Pump Controlled Pressure Stabilization Control Under Strong Time-Varying Load. Processes. 2024; 12(12):2774. https://doi.org/10.3390/pr12122774
Chicago/Turabian StyleCao, Chao, Kai Gao, Hao Wang, Yanzhao Pan, Zhendong Deng, Haoyan Xu, Di Huang, Xinglong Zhao, and Jiyun Zhao. 2024. "Hydraulic Support Liquid Supply System Adaptive Pump Controlled Pressure Stabilization Control Under Strong Time-Varying Load" Processes 12, no. 12: 2774. https://doi.org/10.3390/pr12122774
APA StyleCao, C., Gao, K., Wang, H., Pan, Y., Deng, Z., Xu, H., Huang, D., Zhao, X., & Zhao, J. (2024). Hydraulic Support Liquid Supply System Adaptive Pump Controlled Pressure Stabilization Control Under Strong Time-Varying Load. Processes, 12(12), 2774. https://doi.org/10.3390/pr12122774







