Investigating Erosion of String in Underground Hydrogen Storage under High Flow Velocity
Abstract
:1. Introduction
2. Establishment of Numerical Model
2.1. Control Equations
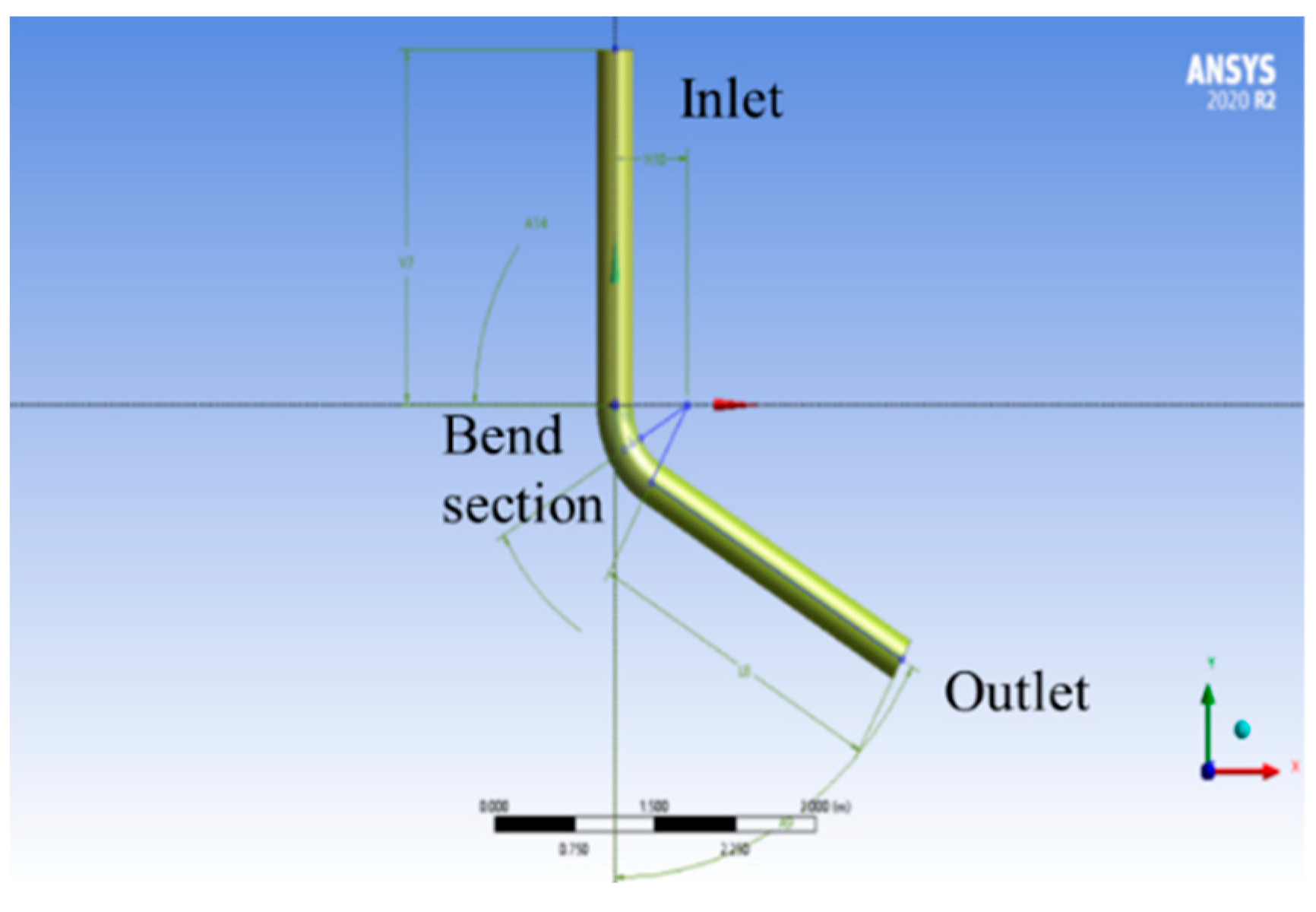
2.2. Physical Model
2.3. Computational Parameters and Working Conditions Setting
2.4. Model Validation
3. Results and Discussion
- (1)
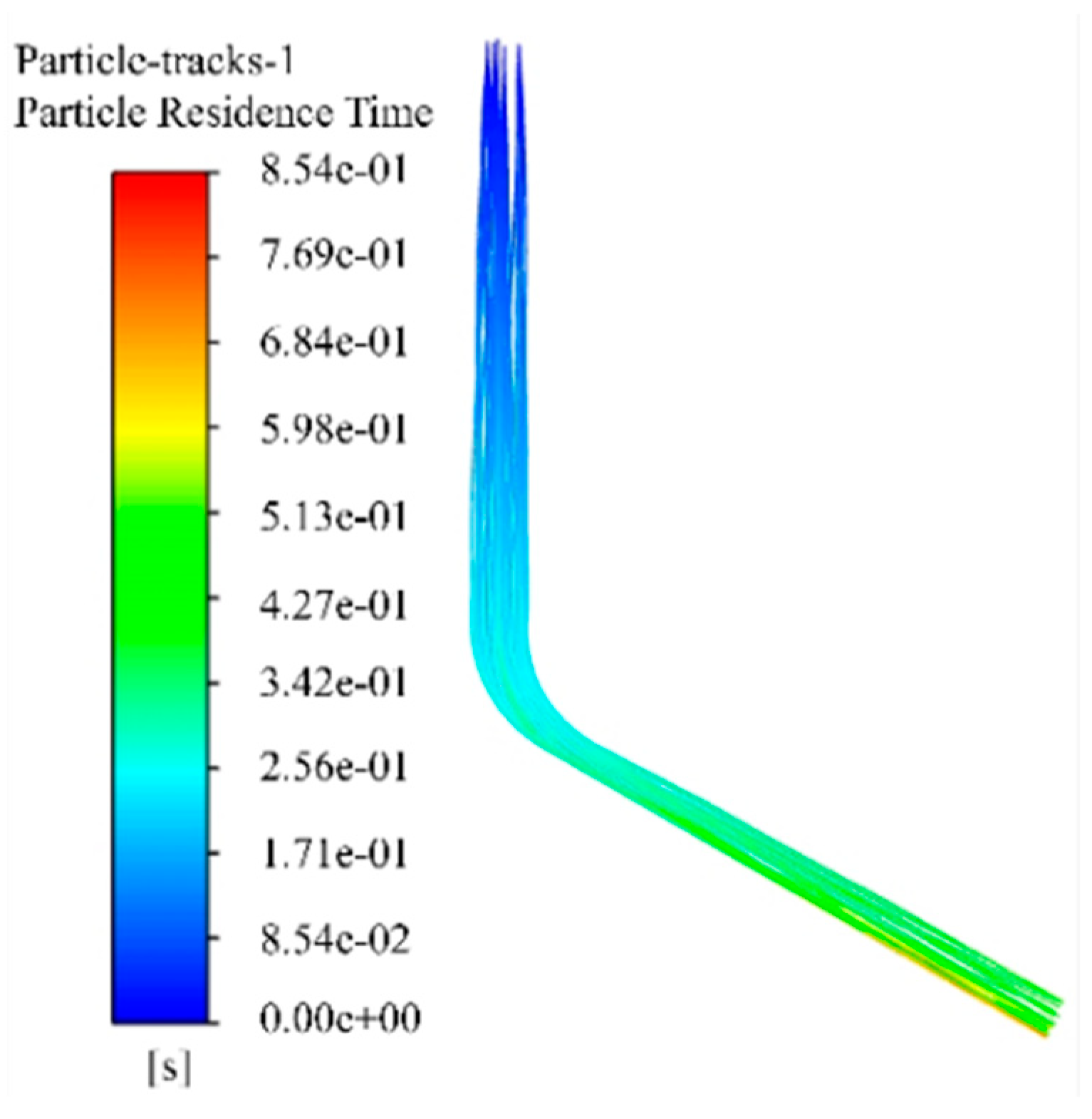
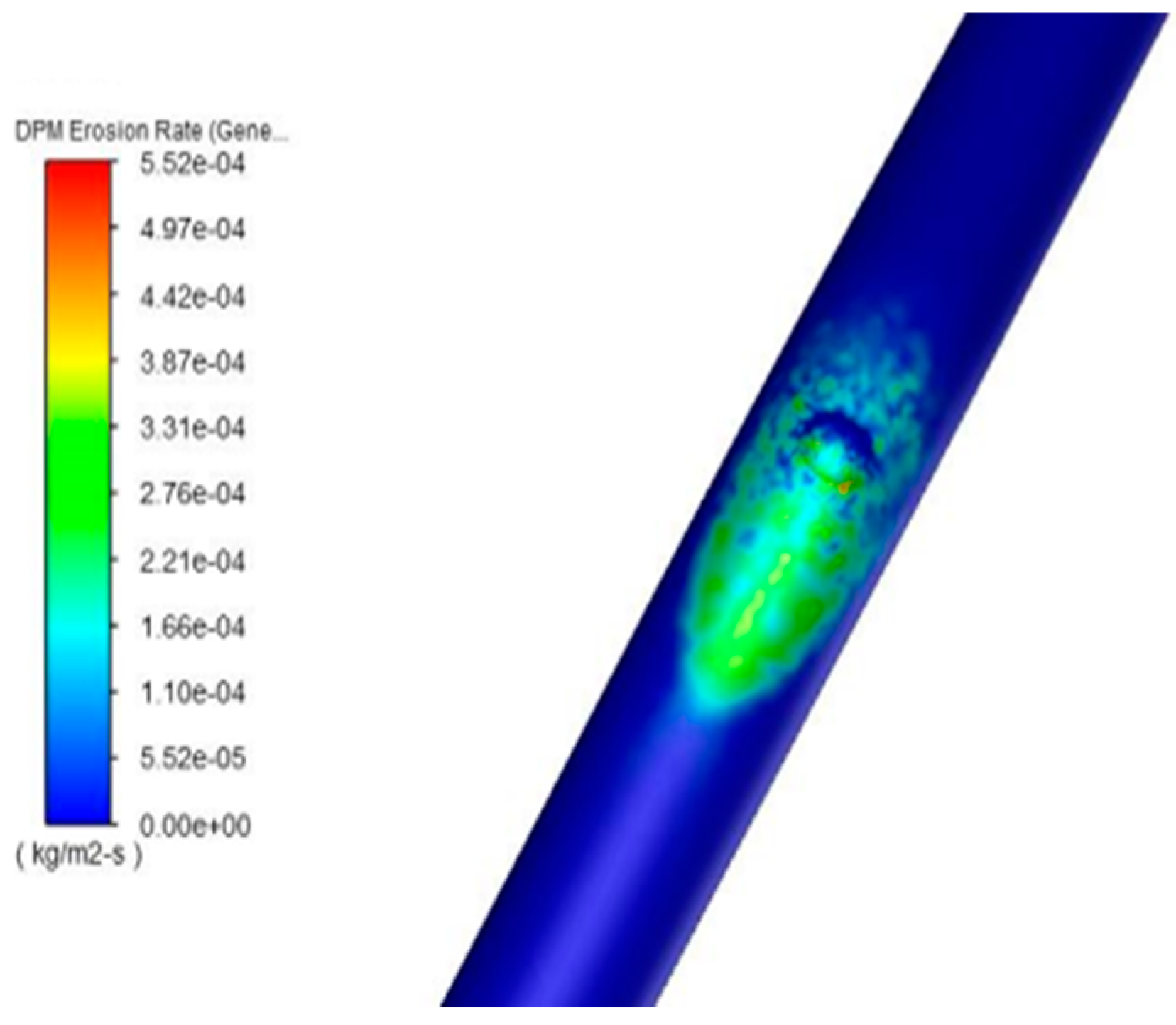
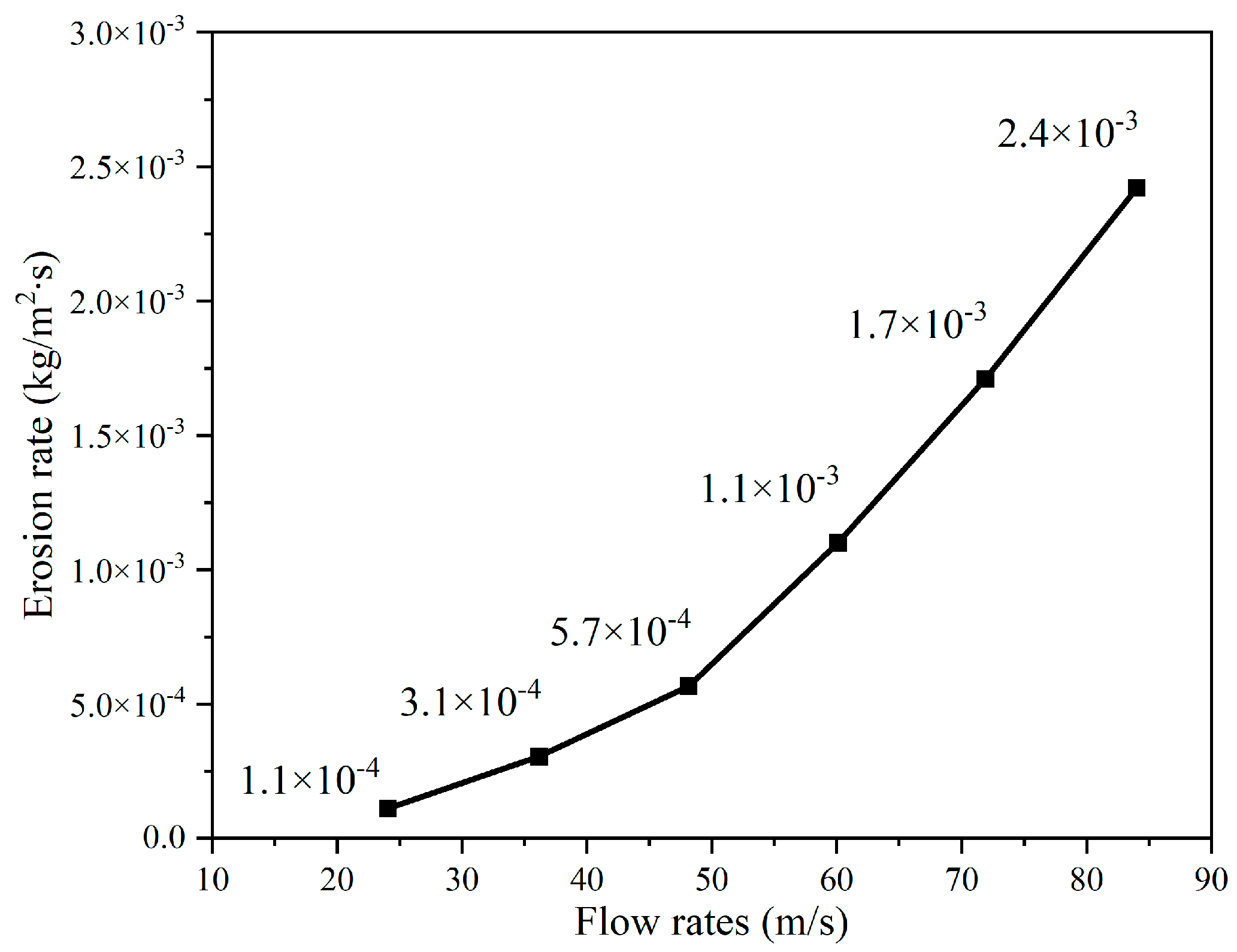
- Flow Rate: A bend pipe with a diameter of Φ0.27 × 0.02 is selected, the volume fraction of discrete phase particles is 1%, and the particle diameter is 2 × 10−4 m. The maximum erosion wear rate curves are obtained at six flow rates of 24 m/s, 36 m/s, 48 m/s, 60 m/s, 72 m/s, and 84 m/s. The maximum erosion rates are 1.12 × 10−4 kg/m2·s, 3.06 × 10−4 kg/m2·s, 5.67 × 10−4 kg/m2·s, 1.10 × 10−3 kg/m2·s, 1.71 × 10−3 kg/m2·s, and 2.42 × 10−3 kg/m2·s, respectively. As shown in Figure 5, the maximum erosion rate increases with the increase in flow rate. As the particle speed increases, the degree of erosion also increases, the erosion rate gradually increases, and the erosion rate increases significantly at high flow rates.
- (2)
- Particle Diameter: A bend pipe with a diameter of Φ0.27 × 0.02 is selected, and the volume fraction of discrete phase particles is 1%. At a speed of 48 m/s, four different particle diameters of 5 × 10−5 m, 1 × 10−4 m, 2 × 10−4 m, and 5 × 10−4 m are added to perform numerical simulations, and the maximum erosion wear rate curve is obtained. As shown in Figure 6, when the speed and other external conditions are fixed, the maximum erosion wear rate of the string is the smallest at 4.63 × 10−4 kg/m2·s when the particle size is 50 μm; when the particle size is 500 μm, the maximum erosion wear rate of the string is the largest at 1.10 × 10−3 kg/m2·s. Under the particle size of 100–200 μm, the maximum erosion rate has a small increase, and it can be seen that the maximum erosion rate increases with the increase in particle size.
- (3)
- Pipe Material: A bend pipe with a diameter of Φ0.27 × 0.02 is selected, and the volume fraction of discrete phase particles is 1%. At a flow rate of 48 m/s, N80 and P110, two different materials are added, respectively, for numerical simulation, and the maximum erosion wear rate histogram is obtained. The maximum erosion rate of the P110 material is 5.67 × 10−4 kg/m2·s, and the maximum erosion rate of the N80 material is 1.03 × 10−3 kg/m2·s. As can be seen from Figure 7, under the hydrogen medium, the N80 pipe material is more prone to corrosion than the P110 pipe material.
- (4)
- Corrosion Defect Factors: A bend pipe with a diameter of Φ0.27 × 0.02 is selected, the volume fraction of solid particles is 1%, the flow rate is 48 m/s, and the particle diameter is 2 × 10−4 m. Different corrosion defects such as double corrosion defects in the bend pipe section, three corrosion defects in the bend pipe section, and large-area corrosion defects in the bend pipe section are added to the bend pipe model for numerical simulation. The maximum erosion wear rates under different corrosion defects are 7.39 × 10−4 kg/m2·s, 1.02 × 10−3 kg/m2·s, and 5.06 × 10−3 kg/m2·s, respectively. As can be seen from Figure 8, the maximum erosion rate caused to the pipeline varies under the influence of different numbers of corrosion defects. Among them, the bend pipe with three corrosion defects has the highest maximum erosion rate, and the risk of failure is relatively high at this time. Therefore, regular inspections should be carried out to see whether there are multiple corrosion defects inside the pipeline and timely protection measures should be taken to avoid leakage accidents.
4. Conclusions
- (1)
- Drawing from an analysis of injection and extraction methods for underground energy storage pipelines and factors influencing erosion, a high-velocity gas flow model and an erosion-wear model were developed for strings. This paper examines the influence of variables like internal flow velocity, particle size, pipeline material, and wall corrosion defects on the pressure distribution near the inclined pipeline section wall, as well as the maximum erosion wear rate caused by high-speed airflow scouring.
- (2)
- The maximum erosion rate increases with the increase in particle speed, and the degree of erosion becomes larger and larger, and the erosion rate gradually increases. The maximum erosion rate of the P110 pipe material is 5.67 × 10−4 kg/m2·s, and that of the N80 pipe material is 1.03 × 10−3 kg/m2·s. The N80 pipe material is more susceptible to erosion than the P110 pipe material.
- (3)
- Under the influence of corrosion defect factors, the maximum erosion wear rates of the double corrosion defects in the bend pipe section, the three corrosion defects in the bend pipe section, and the large-area corrosion defects in the bend pipe section are 7.39 × 10−4 kg/m2·s, 1.02 × 10−3 kg/m2·s, and 8.57 × 10−4 kg/m2·s, respectively. Among them, the bend pipe with three corrosion defects has the highest maximum erosion rate, and the risk of failure is relatively high at this time. Therefore, regular inspections should be carried out to see whether there are multiple corrosion defects inside the pipeline and timely protection measures should be taken to avoid leakage accidents.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| CFD | Computational fluid dynamics |
| DPM | Discrete phase model |
| RNG | Renormalization-group |
| SM | Body force |
| ρ | Relative density |
| μeff | Effective dynamic viscosity |
| μt | Turbulent viscosity |
| p | Static pressure |
| p0 | Static pressure fluid incompressible |
| Reynolds stress | |
| Kronecker integral term | |
| k | Turbulent energy |
| ε | Turbulent energy dissipation rate |
| D | Internal diameter |
References
- Juez-Larré, J.; Machado, C.G.; Groenenberg, R.M.; Belfroid, S.S.; Yousefi, S.H. A detailed comparative performance study of underground storage of natural gas and hydrogen in the Netherlands. Int. J. Hydrogen Energy 2023, 48, 28843–28868. [Google Scholar] [CrossRef]
- Yousefi, H. Design Considerations for Developing an Underground Hydrogen Storage Facility in Porous Reservoirs. Ph.D. Thesis, University of Twente, Enschede, The Netherlands, 2021. [Google Scholar]
- Prinzhofer, A.; Moretti, I.; Françolin, J.; Pacheco, C.; d’Agostino, A.; Werly, J.; Rupin, F. Natural hydrogen continuous emission from sedimentary basins: The example of a Brazilian H2-emitting structure. Int. J. Hydrogen Energy 2019, 44, 5676–5685. [Google Scholar] [CrossRef]
- Bai, M.; Song, K.; Xu, B.; Sun, J.; Feng, F.; Chen, Z. Feasibility, limitation and prospect of H2 underground storage. Geol. Rev. 2014, 60, 748–754. [Google Scholar]
- Li, J. Development status and prospect of underground gas storage in China. Oil Gas Storage Transp. 2022, 41, 780–786. [Google Scholar]
- Fang, D.; Hou, Z.; Yue, Y.; Ren, L.; Chen, Q. A New Concept of Multifunctional Salt Cavern Hydrogen Storage Applied to the Integration of Hydrogen Energy Industry. Adv. Eng. Sci. 2022, 54, 128–135. [Google Scholar]
- Fu, P.; Luo, M.; Xia, Y.; Li, G.; Ban, F. Research On the Status Quo and Difficulties of Hydrogen Underground Storage Technology. China Well Miner. Salt 2020, 51, 19–23. [Google Scholar]
- Wang, Z.; Yang, H.; Zhang, Y. Research Progress on Erosion Theory and Prediction of Gas Storage String. Surf. Technol. 2023, 52, 91–110. [Google Scholar]
- Xu, R. Simulation analysis of vertical gas well production string erosion model. Compr. Corros. Control 2020, 34, 58–66. [Google Scholar]
- He, Z.; Yi, W.; Li, J.; Sun, P.; Liu, P.; Jiang, J. Analysis of Erosion Law and Ultimate Gas Injection and Production Capacity of Injection and Production Pipe String in Wen 23 Gas Storage. Pet. Pipes Instrum. 2020, 6, 46–49. [Google Scholar]
- Liu, W.; Zhang, Z.; Chen, J.; Jiang, D.; Wu, F.; Fan, J.; Li, Y. Feasibility evaluation of large-scale underground hydrogen storage in bedded salt rocks of China: A case study in Jiangsu province. Energy 2020, 198, 117348. [Google Scholar] [CrossRef]
- Wu, L.; Xiang, F.; Shi, B.; Zhang, Y.; Zhang, X.; Zhai, X.; Lou, Y. Optimization of erosion resistant structure of gas pipeline bends based on numerical simulation. Mater. Prot. 2021, 54, 50–57. [Google Scholar]
- Meng, W.; Zhang, J.; Zhang, C.; Huang, L.; Zhang, R.; Zhang, L.; Ren, S. Coupling test of steel erosion and CO2 corrosion for underground pipe columns. J. China Univ. Pet. 2021, 5, 104–110. [Google Scholar]
- Sletfjerding, E.; Gudmundsson, J.S. Friction factor directly from roughness measurements. J. Energy Resour. Technol. 2003, 125, 126–130. [Google Scholar] [CrossRef]
- Liu, M.; Wang, J.; Yan, Y.; Xie, W.; Yang, X.; Yan, X.; Zhang, Y. Numerical simulation of fluid state along the well string of underground gas storage. Pet. Drill. Prod. Technol. 2017, 39, 449–454. [Google Scholar]
- Zeng, L.; Zhang, G.A.; Guo, X.P. Erosion–corrosion at different locations of X65 carbon steel elbow. Corros. Sci. 2014, 85, 318–330. [Google Scholar] [CrossRef]
- Mazumder, Q.H.; Shirazi, S.A.; McLaury, B.S.; Shadley, J.R.; Rybicki, E.F. Development and validation of a mechanistic model to predict solid particle erosion in multiphase flow. Wear 2005, 259, 203–207. [Google Scholar] [CrossRef]
- Tabakoff, W.; Kotwal, R.; Hamed, A. Erosion study of different materials affected by coal ash particles. Wear 1979, 52, 161–173. [Google Scholar] [CrossRef]
- Suzki, M.; Inaba, K.; Yammoto, M. Numerical simulation of sand erosion in a square-section 90-degree bend. J. Fluid Sci. Technol. 2008, 3, 868–880. [Google Scholar] [CrossRef]
- Tijsseling, A.S. Fluid-structure interaction in liquid-filled pipe systems: A review. J. Fluids Struct. 1996, 10, 109–146. [Google Scholar] [CrossRef]
- Zhang, S.; Yan, Y.; Shi, L.; Li, L.; Zhao, L.; Wang, R.; Yan, X. A semi-empirical model for underground gas storage injection-production string time series remaining useful life analysis in process safety operation. Process Saf. Environ. Prot. 2021, 154, 1–17. [Google Scholar] [CrossRef]
- Pan, L.; Oldenburg, C.M.; Freifeld, B.M.; Jordan, P.D. Modeling the Aliso Canyon underground gas storage well blowout and kill operations using the coupled well-reservoir simulator T2 Well. J. Pet. Sci. Eng. 2018, 161, 158–174. [Google Scholar] [CrossRef]
- Zhang, S.; Yan, Y.; Sheng, Z.; Yan, X. Uncertainty failure risk quantitative assessments for underground gas storage near-wellbore area. J. Energy Storage 2021, 36, 102393. [Google Scholar] [CrossRef]
- Bai, M.; Shen, A.; Meng, L.; Zhu, J.; Song, K. Well completion issues for underground gas storage in oil and gas reservoirs in China. J. Pet. Sci. Eng. 2018, 171, 584–591. [Google Scholar] [CrossRef]
- Dharmananda, K.; Kingsbury, N.; Singh, H. Underground gas storage: Issues beneath the surface. In Proceedings of the SPE Asia Pacific Oil and Gas Conference and Exhibition, Perth, Australia, 18–20 October 2004; SPE: Richardson, TX, USA, 2004; p. SPE-88491. [Google Scholar]
- Stack, M.M.; Abdelrahman, S.M. A CFD model of particle concentration effects on erosion–corrosion of Fe in aqueous conditions. Wear 2011, 273, 38–42. [Google Scholar] [CrossRef]
- Michalski, J.; Bünger, U.; Crotogino, F.; Donadei, S.; Schneider, G.S.; Pregger, T.; Heide, D. Hydrogen generation by electrolysis and storage in salt caverns: Potentials, economics and systems aspects with regard to the German energy transition. Int. J. Hydrogen Energy 2017, 42, 13427–13443. [Google Scholar] [CrossRef]
- Zhou, Q.; Zhang, J. Overview of underground hydrogen storage technology research. Oil Gas New Energy 2022, 34, 1–6. [Google Scholar]
- Zhang, H.; Tan, Y.; Yang, D.; Trias, F.X.; Jiang, S.; Sheng, Y.; Oliva, A. Numerical investigation of the location of maximum erosive wear damage in elbow: Effect of slurry velocity, bend orientation and angle of elbow. Powder Technol. 2012, 217, 467–476. [Google Scholar] [CrossRef]
- Tan, Y.; Zhang, H.; Yang, D.; Jiang, S.; Song, J.; Sheng, Y. Numerical simulation of concrete pumping process and investigation of wear mechanism of the piping wall. Tribol. Int. 2012, 46, 137–144. [Google Scholar] [CrossRef]
- Amid, A.; Mignard, D.; Wilkinson, M. Seasonal storage of hydrogen in a depleted natural gas reservoir. Int. J. Hydrogen Energy 2016, 41, 5549–5558. [Google Scholar] [CrossRef]
- Ugarte, E.R.; Salehi, S. A review on well integrity issues for underground hydrogen storage. J. Energy Resour. Technol. 2022, 144, 042001. [Google Scholar] [CrossRef]
- Xue, W.; Wang, Y.; Chen, Z.; Liu, H. An integrated model with stable numerical methods for fractured underground gas storage. J. Clean. Prod. 2023, 393, 136268. [Google Scholar] [CrossRef]
- Li, J.; Li, J.; Wang, Y.; Zhao, J.; Li, H.; Yu, B. Research progress and development trend exploration of key technologies for hydrogen energy storage and transportation. Oil Gas Storage Transp. 2023, 42, 856–871. [Google Scholar]
- Cheng, C. Numerical Simulation Study on Erosion Law of Test String in Offshore Gas Well. Master’s Thesis, Southwest Petroleum University, Chengdu, China, 2015. [Google Scholar]



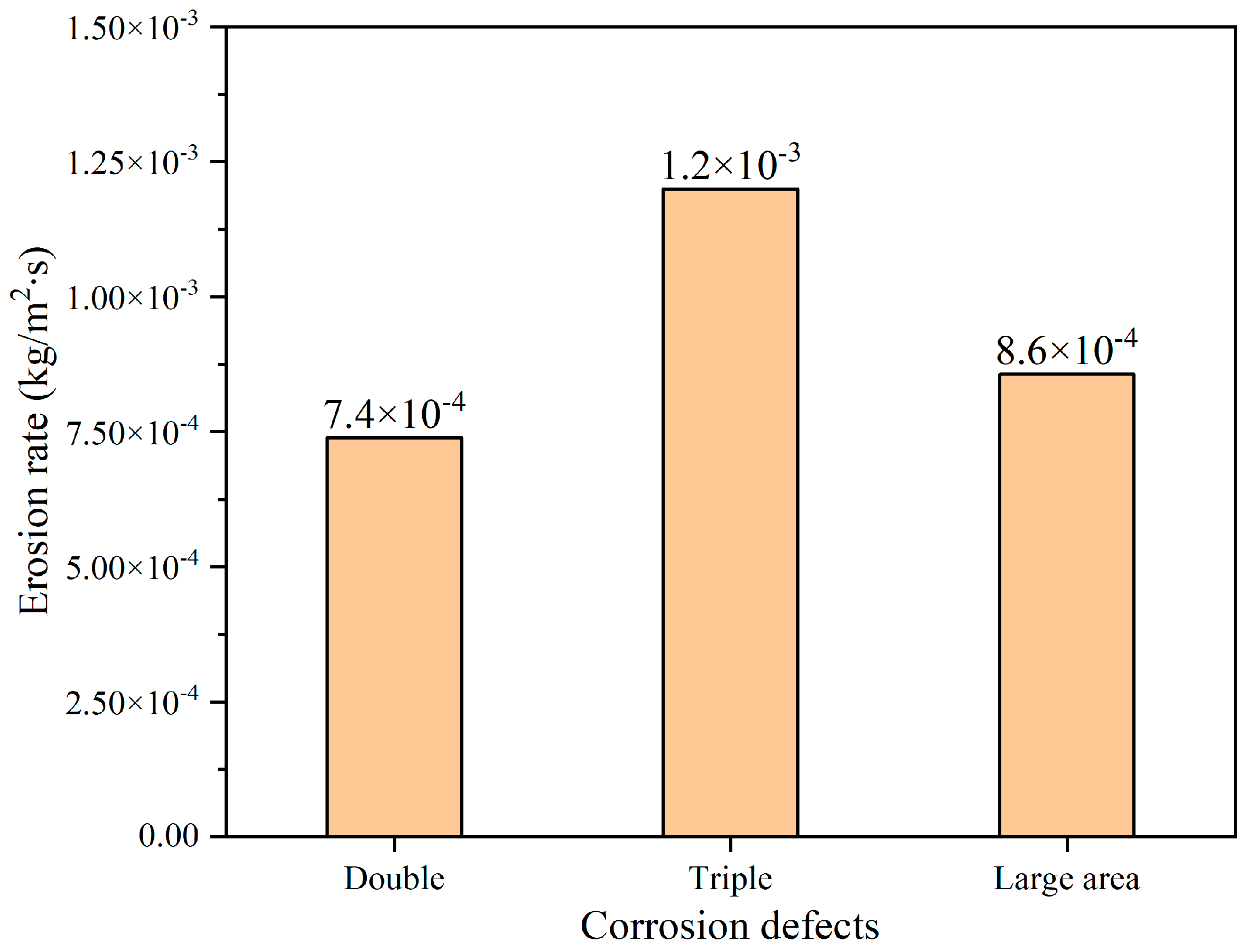
| Boundary | Continuous Phase | Discrete Phase |
|---|---|---|
| Model | κ-ε Model | DPM Model |
| Inlet | Velocity Inlet | Escape |
| Outlet | Free Outflow | Escape |
| Wall | No-slip Boundary | Reflection |
| Number | Pipe Diameter (m) | Pipe Material | Particle Diameter (μm) | Flow Speed (m/s) | Corrosion Defect Factors |
|---|---|---|---|---|---|
| 1 | 0.27 | N80 | 200 | 48 | No corrosion defects |
| 2 | 0.34 | N80 | 200 | 48 | No corrosion defects |
| 3 | 0.27 | P110 | 200 | 48 | No corrosion defects |
| 4 | 0.27 | N80 | 50 | 48 | No corrosion defects |
| 5 | 0.27 | N80 | 100 | 48 | No corrosion defects |
| 6 | 0.27 | N80 | 500 | 48 | No corrosion defects |
| 7 | 0.27 | N80 | 200 | 24 | No corrosion defects |
| 8 | 0.27 | N80 | 200 | 36 | No corrosion defects |
| 9 | 0.27 | N80 | 200 | 60 | No corrosion defects |
| 10 | 0.27 | N80 | 200 | 72 | No corrosion defects |
| 11 | 0.27 | N80 | 200 | 84 | No corrosion defects |
| 12 | 0.27 | N80 | 200 | 48 | Double corrosion defects in the bent pipe section |
| 13 | 0.27 | N80 | 200 | 48 | Triple corrosion defects in the bent pipe section |
| 14 | 0.27 | N80 | 200 | 48 | Large area corrosion defects in the bent pipe section |
| Cumulative Duration (h) | Experimental Erosion Weight Loss (mg) | Simulated Erosion Weight Loss (mg) | Error (%) |
|---|---|---|---|
| 1 | 1.56 | 1.65 | 4.2 |
| 2 | 3.16 | 3.29 | 4.3 |
| 3 | 5.45 | 4.94 | 1.7 |
| 4 | 6.66 | 6.58 | 1.2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, L.; Li, L.; Luo, J.; Han, Z.; Xie, S.; Yu, T.; Liu, Q. Investigating Erosion of String in Underground Hydrogen Storage under High Flow Velocity. Processes 2023, 11, 2894. https://doi.org/10.3390/pr11102894
Zhu L, Li L, Luo J, Han Z, Xie S, Yu T, Liu Q. Investigating Erosion of String in Underground Hydrogen Storage under High Flow Velocity. Processes. 2023; 11(10):2894. https://doi.org/10.3390/pr11102894
Chicago/Turabian StyleZhu, Lixia, Lifeng Li, Jinheng Luo, Ziyue Han, Shuyi Xie, Tao Yu, and Qing Liu. 2023. "Investigating Erosion of String in Underground Hydrogen Storage under High Flow Velocity" Processes 11, no. 10: 2894. https://doi.org/10.3390/pr11102894
APA StyleZhu, L., Li, L., Luo, J., Han, Z., Xie, S., Yu, T., & Liu, Q. (2023). Investigating Erosion of String in Underground Hydrogen Storage under High Flow Velocity. Processes, 11(10), 2894. https://doi.org/10.3390/pr11102894






