Research and Modelling the Ability of Waste from Water and Wastewater Treatment to Remove Phosphates from Water
Abstract
:1. Introduction
2. Materials and Methods
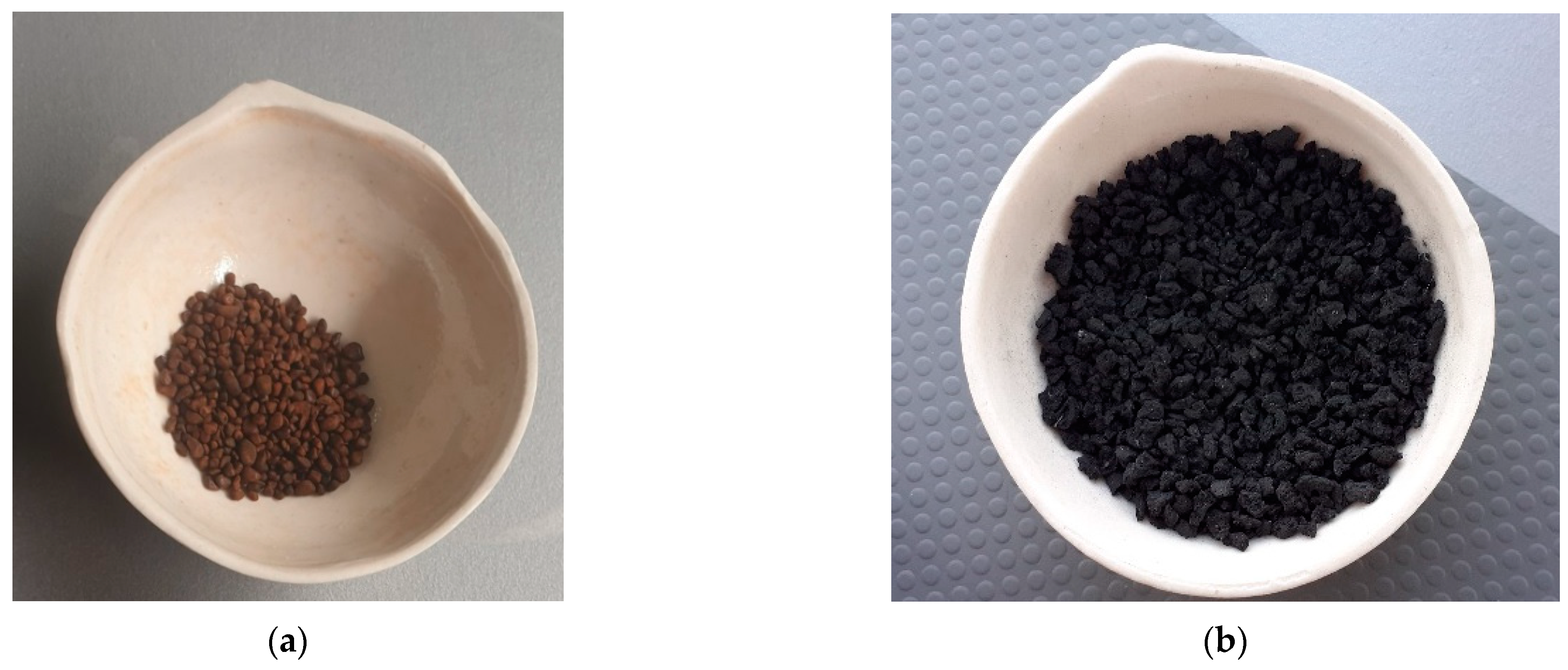
2.1. Selected Materials
2.2. Methodology for Determining the Composition of the Materials
2.3. Experiments Methodology
2.3.1. Batch Tests
2.3.2. Column Research
2.4. Process Modelling
2.4.1. Adsorption Equilibrium
2.4.2. Kinetics Analysis
3. Results and Discussion
3.1. Composition of the Materials
3.2. Modelling Results
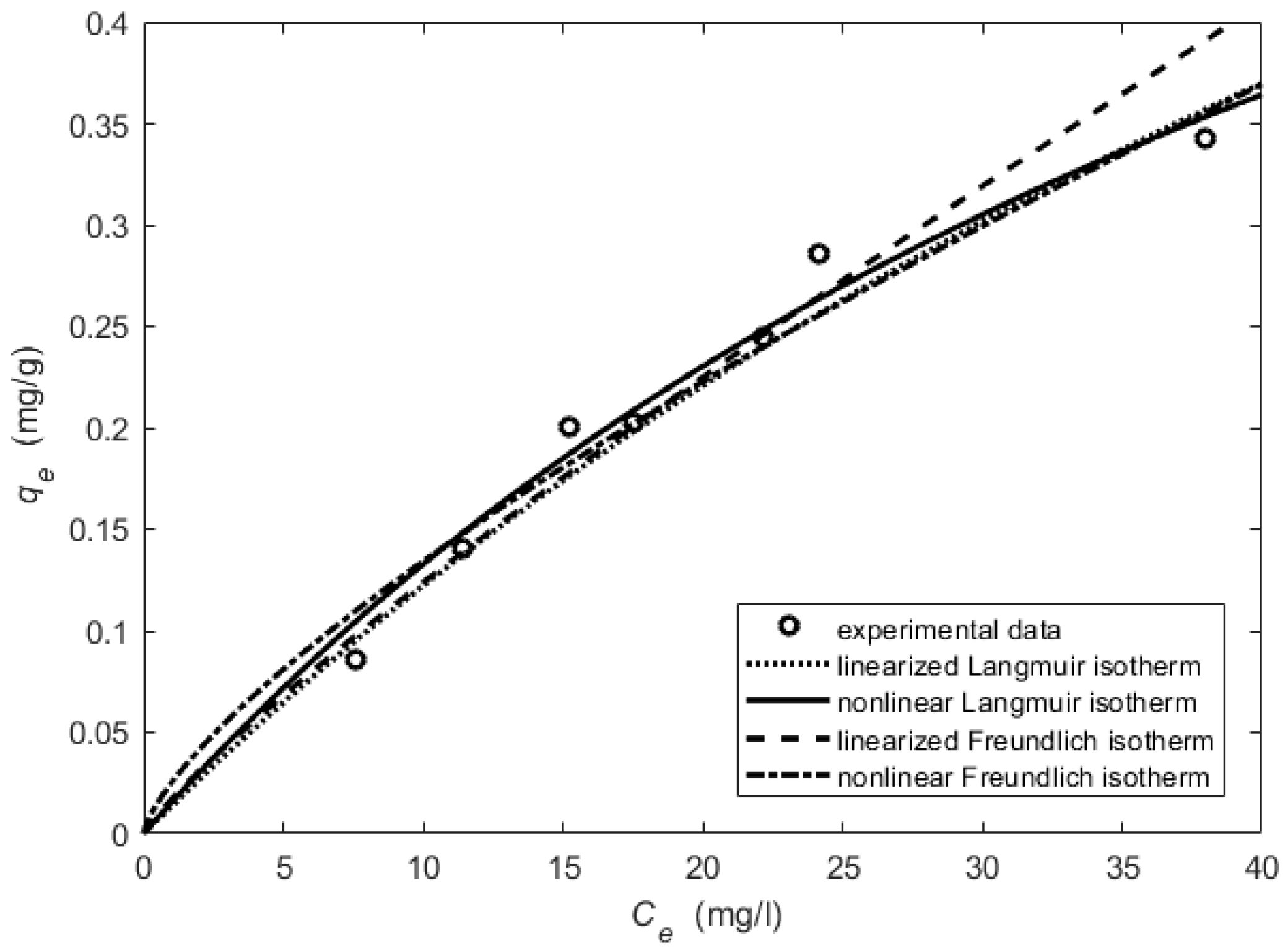
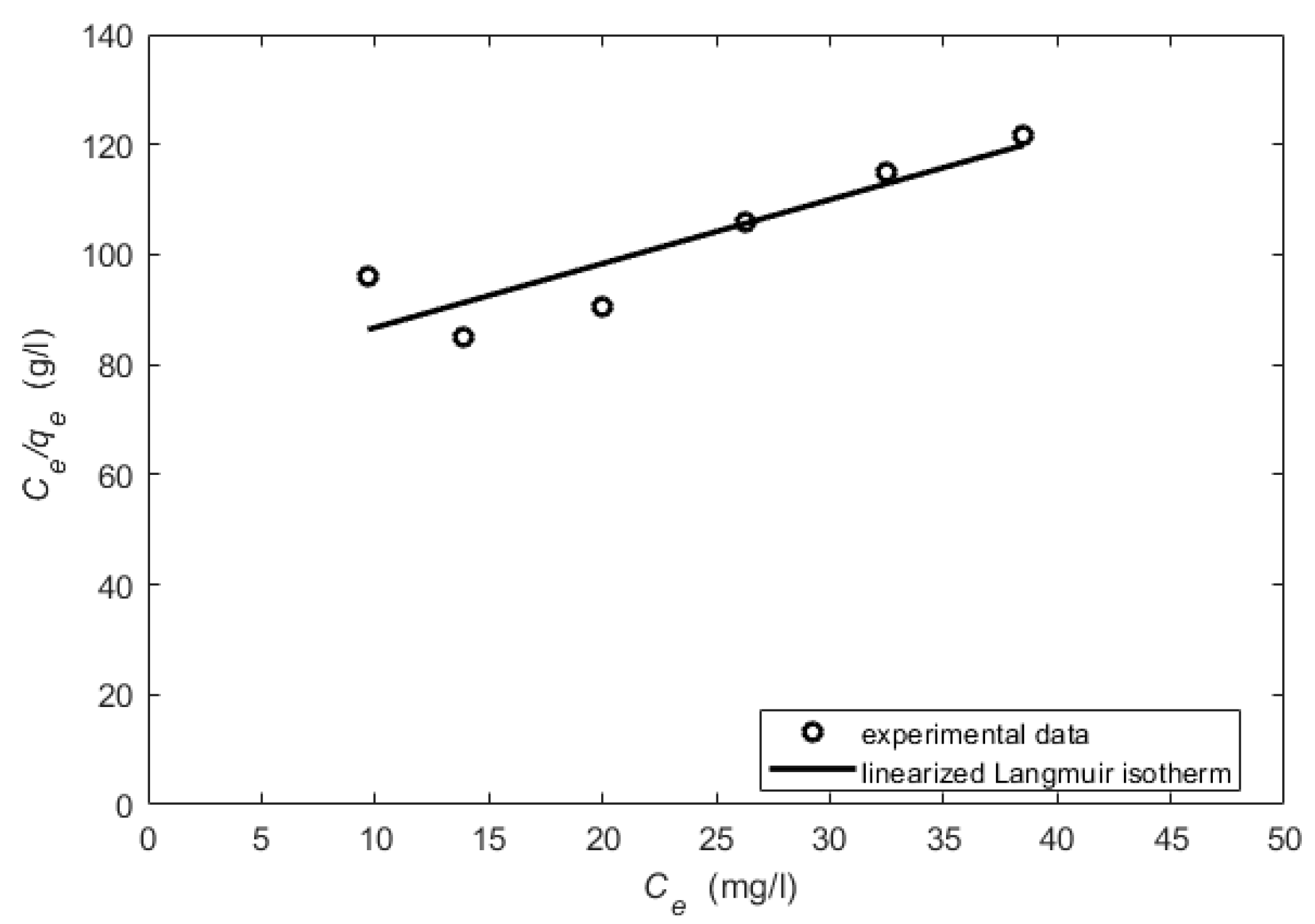
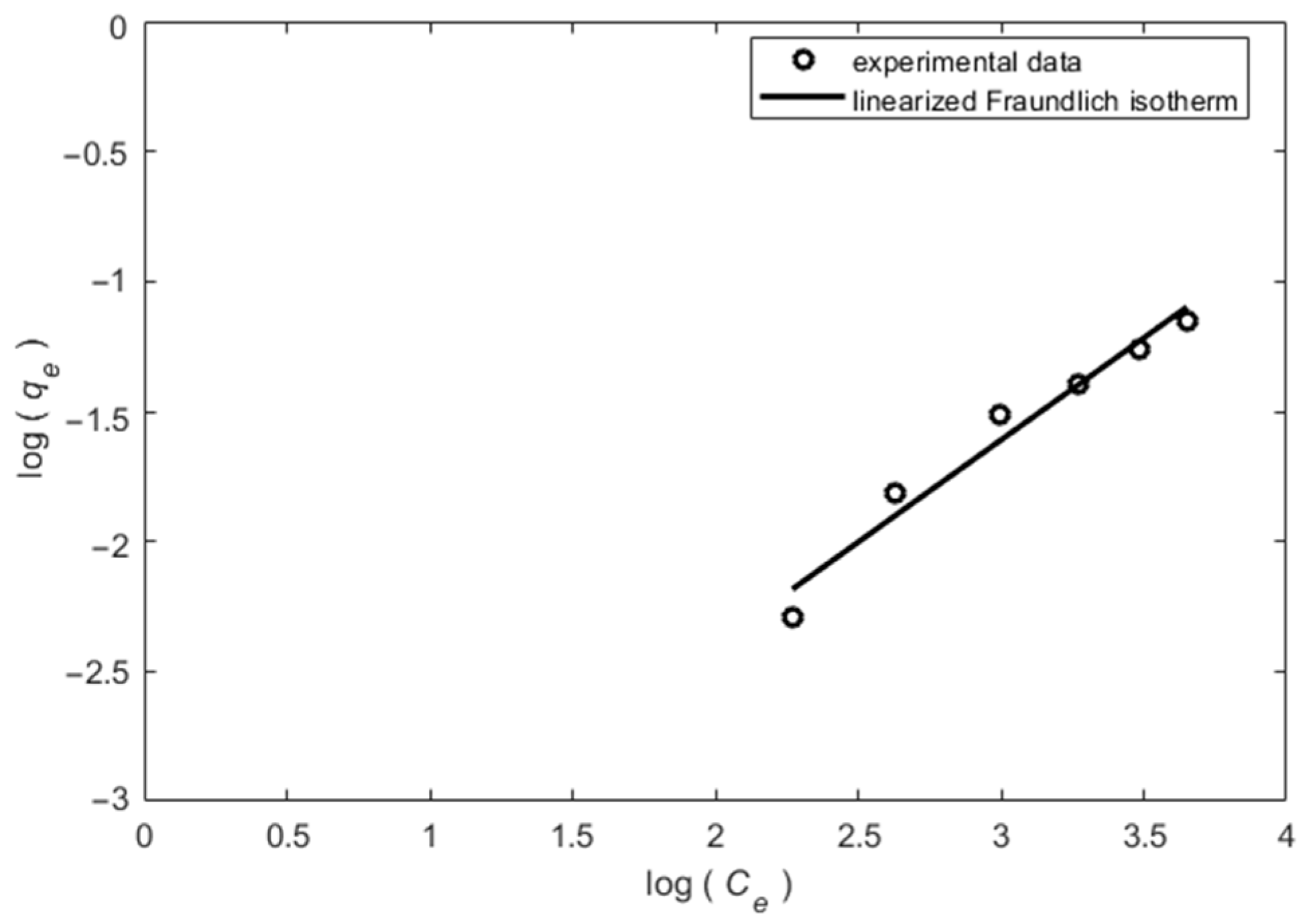
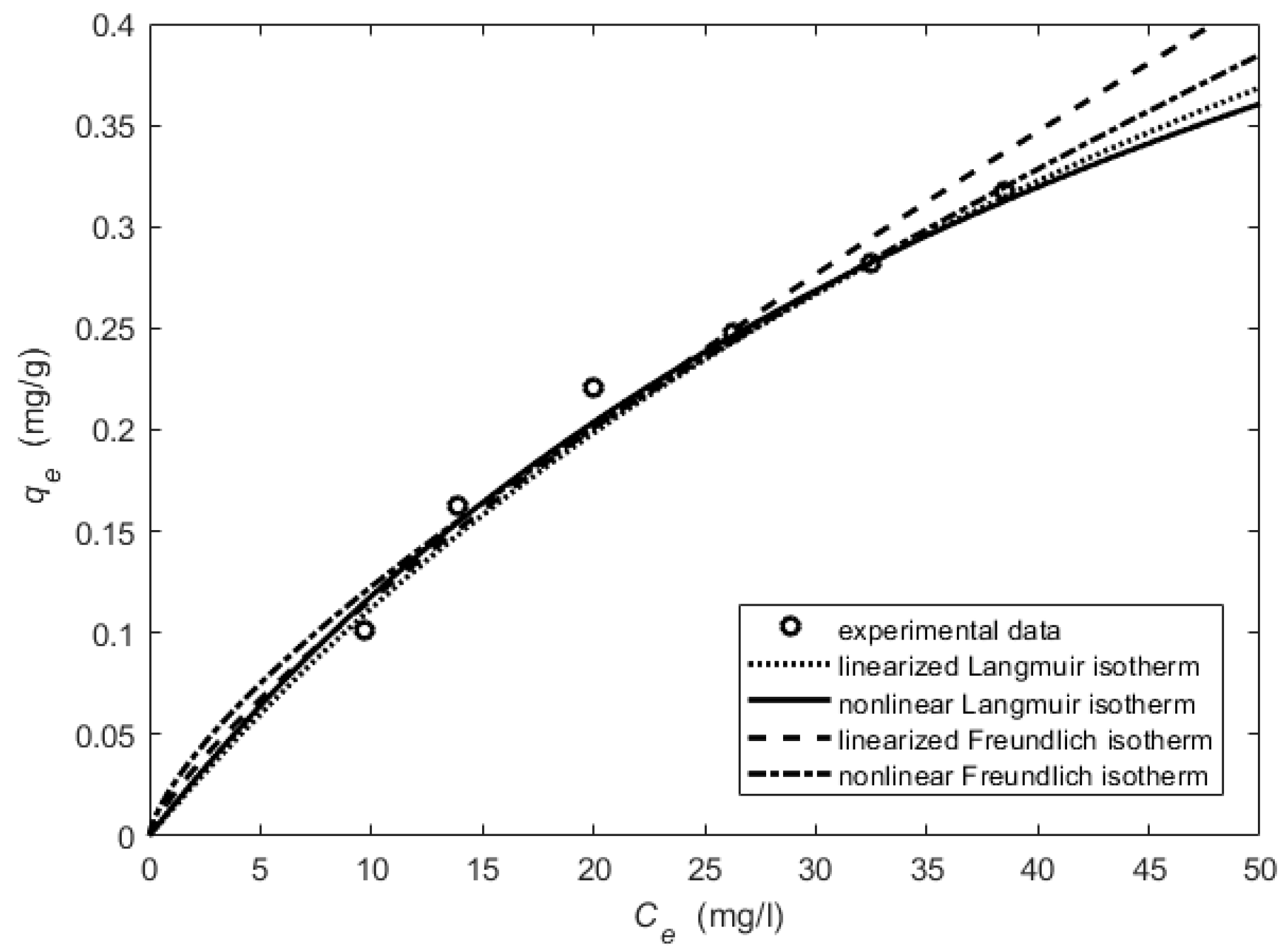
3.2.1. Adsorption Equilibrium
3.2.2. OQS Adsorbent Results
3.2.3. ISS Adsorbent Results
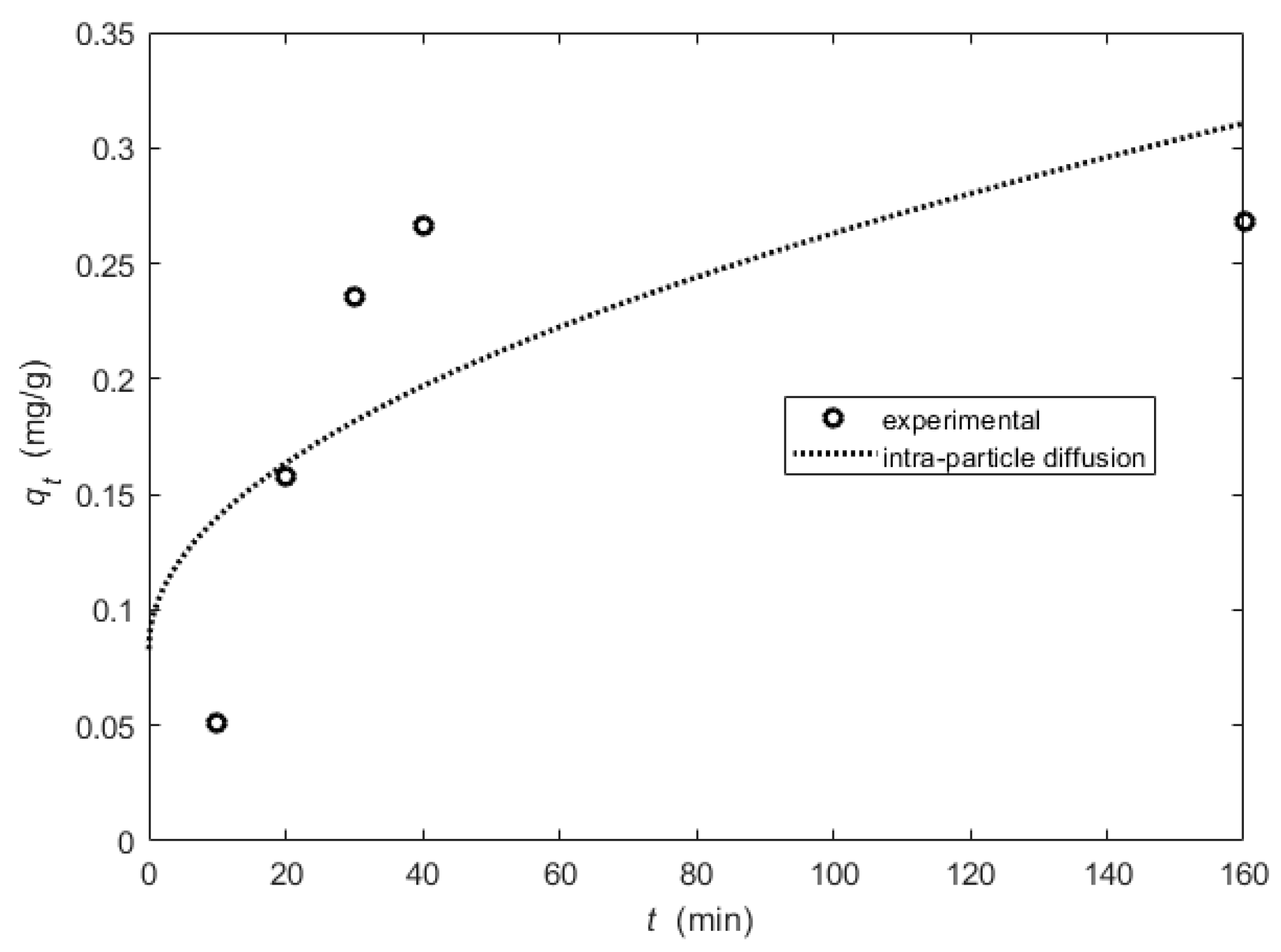
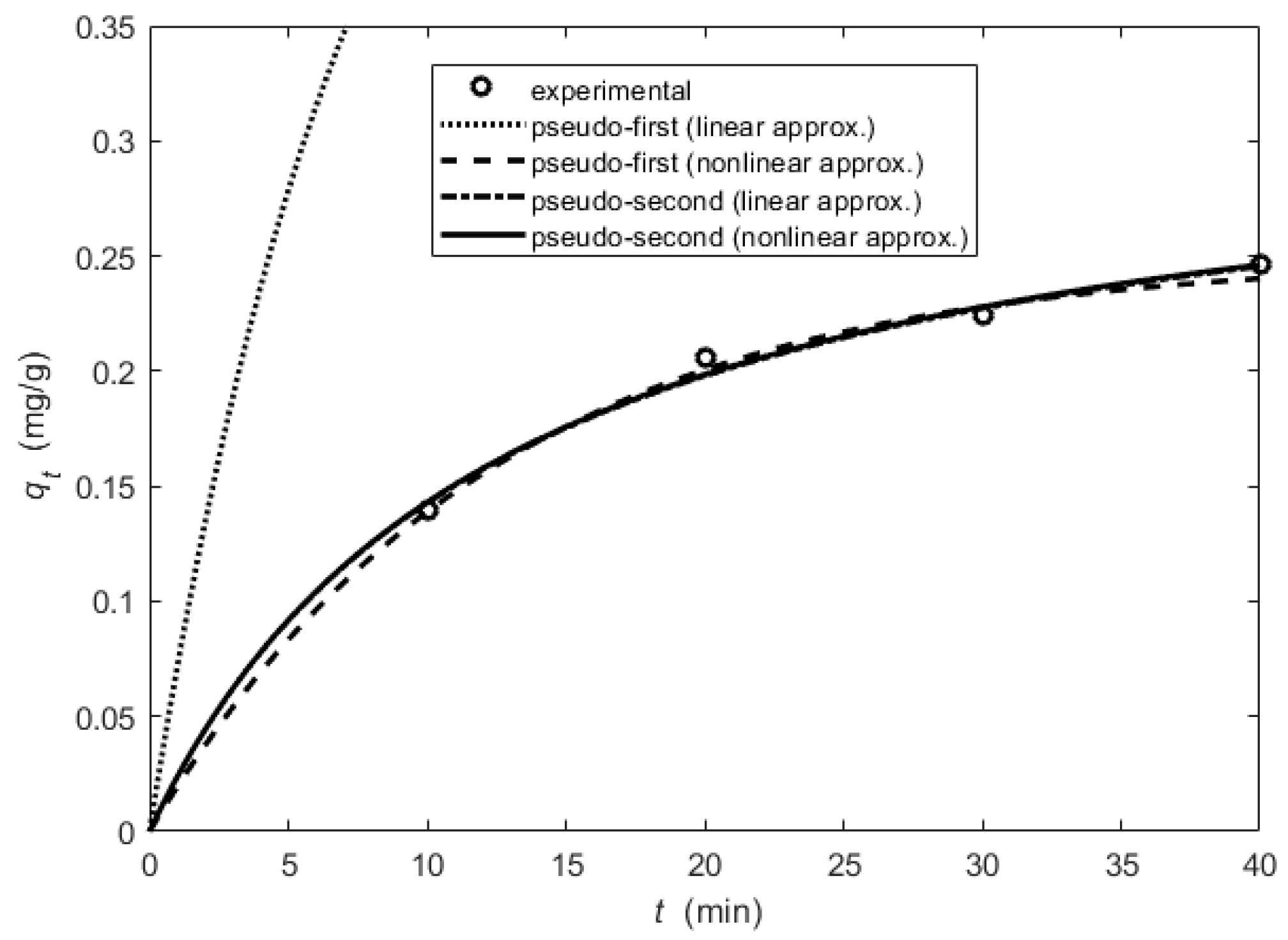
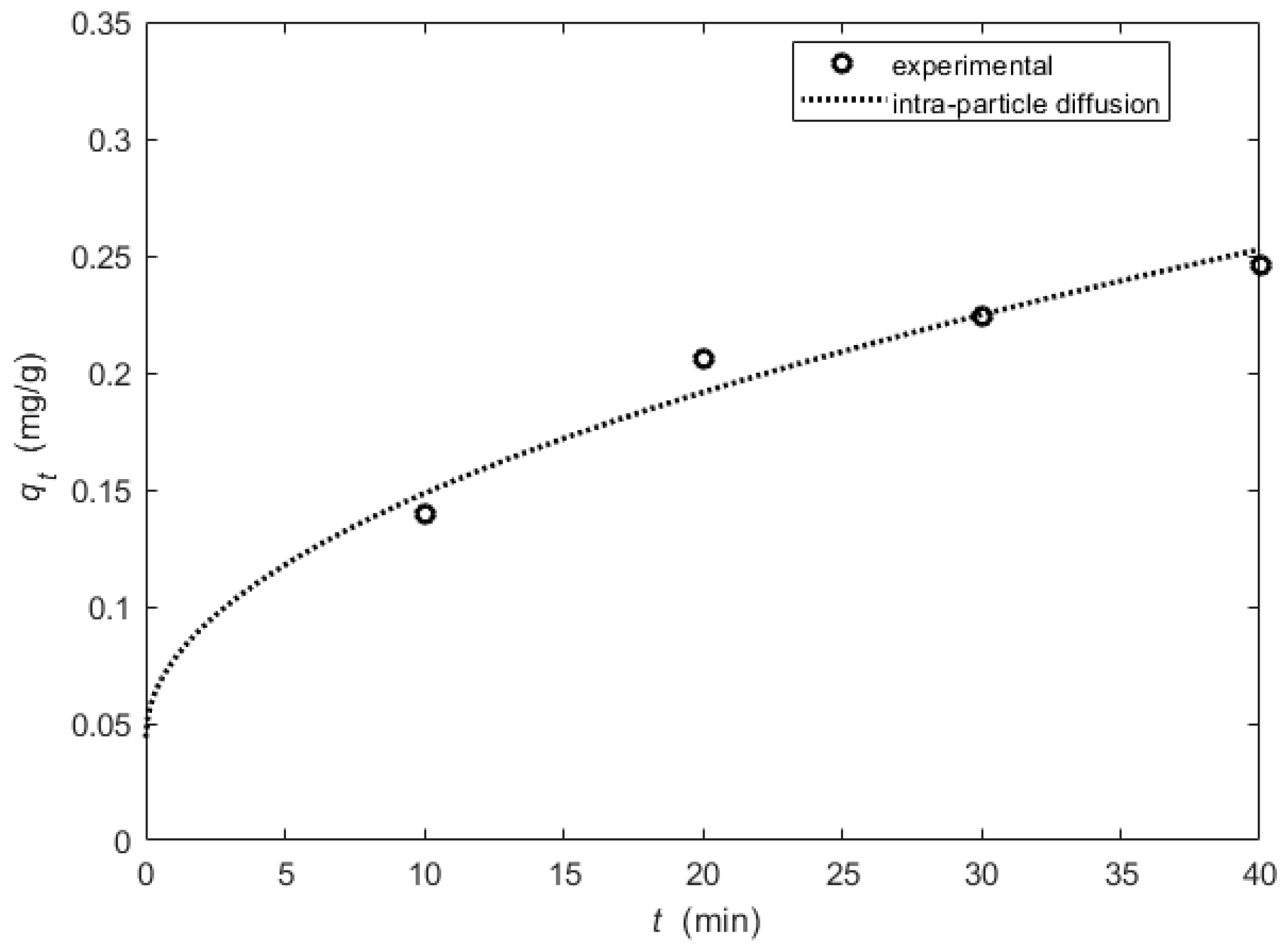
3.2.4. Kinetics Analysis
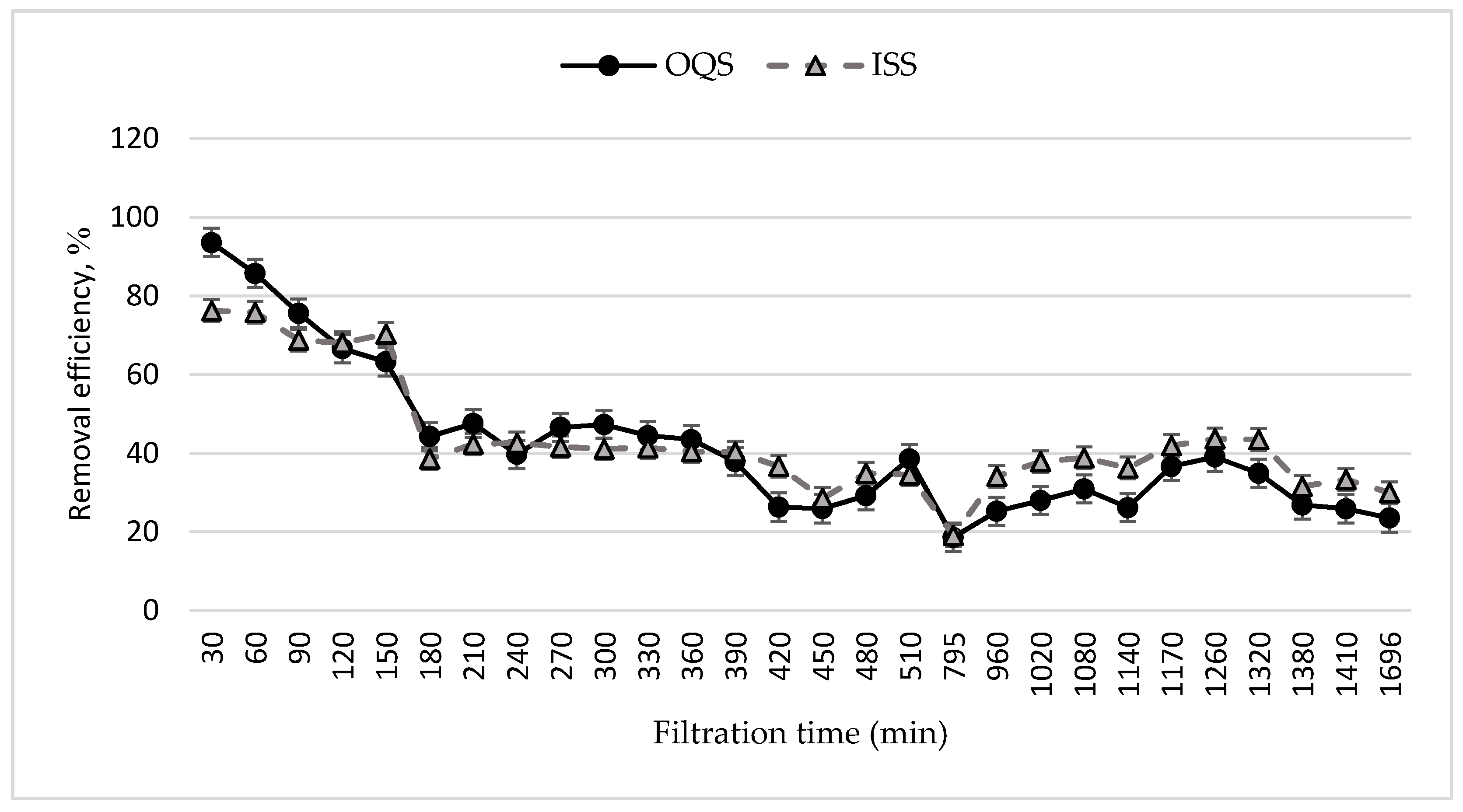
3.3. Column Filtration Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Environment Agency Reform of Regulatory System to Control Small Sewage Discharges from Septic Tanks and Small Sewage Treatment Plants in England; Environment Agency: Bristol, UK, 2015.
- McDowell, R.W.; Noble, A.; Pletnyakov, P.; Haggard, B.E.; Mosley, L.M. Global mapping of freshwater nutrient enrichment and periphyton growth potential. Sci. Rep. 2020, 10, 1–13. [Google Scholar] [CrossRef] [PubMed]
- Farkas, K.; Walker, D.I.; Adriaenssens, E.M.; McDonald, J.E.; Hillary, L.S.; Malham, S.K.; Jones, D.L. Viral indicators for tracking domestic wastewater contamination in the aquatic environment. Water Res. 2020, 181, 115926. [Google Scholar] [CrossRef]
- Dodds, W.K.; Smith, V.H. Nitrogen, phosphorus, and eutrophication in streams. Inland Waters 2016, 6, 155–164. [Google Scholar] [CrossRef]
- Gupta, V.K.; Ali, I. Wastewater Treatment by Biological Methods. In Environmental Water; Elsevier: Amsterdam, The Netherlands, 2013; pp. 179–204. [Google Scholar]
- Mažeikienė, A.; Grubliauskas, R. Biotechnological wastewater treatment in small-scale wastewater treatment plants. J. Clean. Prod. 2021, 279, 123750. [Google Scholar] [CrossRef]
- Du, X.; Han, Q.; Li, J.; Li, H. The behavior of phosphate adsorption and its reactions on the surfaces of Fe–Mn oxide adsorbent. J. Taiwan Inst. Chem. Eng. 2017, 76, 167–175. [Google Scholar] [CrossRef]
- Adera, S.; Drizo, A.; Twohig, E.; Jagannathan, K.; Benoit, G. Improving Performance of Treatment Wetlands: Evaluation of Supplemental Aeration, Varying Flow Direction, and Phosphorus Removing Filters. Water Air Soil Pollut. 2018, 229, 1–15. [Google Scholar] [CrossRef]
- Li, Y.; He, X.; Hu, H.; Zhang, T.; Qu, J.; Zhang, Q. Enhanced phosphate removal from wastewater by using in situ generated fresh trivalent Fe composition through the interaction of Fe(II) on CaCO3. J. Environ. Manag. 2018, 221, 38–44. [Google Scholar] [CrossRef]
- Hamisi, R.; Renman, A.; Renman, G. Performance of an On-Site Wastewater Treatment System Using Reactive Filter Media and a Sequencing Batch Constructed Wetland. Sustainability 2019, 11, 3172. [Google Scholar] [CrossRef] [Green Version]
- Bali, M.; Gueddari, M. Removal of phosphorus from secondary effluents using infiltration–Percolation process. Appl. Water Sci. 2019, 9, 3. [Google Scholar] [CrossRef] [Green Version]
- Erickson, A.J.; Gulliver, J.S.; Weiss, P.T. Capturing phosphates with iron enhanced sand filtration. Water Res. 2012, 46, 3032–3042. [Google Scholar] [CrossRef]
- Wilfert, P.; Kumar, P.S.; Korving, L.; Witkamp, G.J.; Van Loosdrecht, M.C.M. The Relevance of Phosphorus and Iron Chemistry to the Recovery of Phosphorus from Wastewater: A Review. Environ. Sci. Technol. 2015, 49, 9400–9414. [Google Scholar] [CrossRef] [PubMed]
- Chakraborty, T.; Gabriel, M.; Amiri, A.S.; Santoro, D.; Walton, J.; Smith, S.; Ray, M.B.; Nakhla, G. Carbon and Phosphorus Removal from Primary Municipal Wastewater Using Recovered Aluminum. Environ. Sci. Technol. 2017, 51, 12302–12309. [Google Scholar] [CrossRef] [PubMed]
- Lyngsie, G.; Penn, C.J.; Pedersen, H.L.; Borggaard, O.K.; Hansen, H.C.B. Modelling of phosphate retention by Ca- and Fe-rich filter materials under flow-through conditions. Ecol. Eng. 2015, 75, 93–102. [Google Scholar] [CrossRef]
- Kefeni, K.K.; Mamba, B.B.; Msagati, T.A.M. Application of spinel ferrite nanoparticles in water and wastewater treatment: A review. Sep. Purif. Technol. 2017, 188, 399–422. [Google Scholar] [CrossRef]
- Eveborn, D.; Gustafsson, J.P.; Elmefors, E.; Yu, L.; Eriksson, A.K.; Ljung, E.; Renman, G. Phosphorus in soil treatment systems: Accumulation and mobility. Water Res. 2014, 64, 42–52. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Huang, W.; Zhang, L.; Gao, J.; Li, J.; Zhang, J.; Zheng, Z. Removal of dissolved inorganic phosphorus with modified gravel sand: Kinetics, equilibrium, and thermodynamic studies. Desalin. Water Treat. 2016, 57, 3074–3084. [Google Scholar] [CrossRef]
- Huang, Y.; Yang, J.K.; Keller, A.A. Removal of arsenic and phosphate from aqueous solution by metal (hydr-)oxide coated sand. ACS Sustain. Chem. Eng. 2014, 2, 1128–1138. [Google Scholar] [CrossRef] [Green Version]
- Chowdhury, R.B.; Moore, G.A.; Weatherley, A.J.; Arora, M. Key sustainability challenges for the global phosphorus resource, their implications for global food security, and options for mitigation. J. Clean. Prod. 2017, 140, 945–963. [Google Scholar] [CrossRef]
- Kvartenko, O.; Sabliy, L.; Kovalchuk, N.; Lysytsya, A. The use of the biological method for treating iron containing underground waters. J. Water Land Dev. 2018, 39, 77–82. [Google Scholar] [CrossRef] [Green Version]
- Baltrėnaitė-Gedienė, E.; Leonavičienė, T.; Baltrėnas, P. Comparison of CU(II), MN(II) and ZN(II) adsorption on biochar using diagnostic and simulation models. Chemosphere 2020, 245, 125562. [Google Scholar] [CrossRef]
- Pehlivan, E.; Cetin, S.; Yanik, B.H. Equilibrium studies for the sorption of zinc and copper from aqueous solutions using sugar beet pulp and fly ash. J. Hazard. Mater. 2006, 135, 193–199. [Google Scholar] [CrossRef] [PubMed]
- Rout, P.R.; Bhunia, P.; Dash, R.R. Modeling isotherms, kinetics and understanding the mechanism of phosphate adsorption onto a solid waste: Ground burnt patties. J. Environ. Chem. Eng. 2014, 2, 1331–1342. [Google Scholar] [CrossRef]
- Prapagdee, S.; Tawinteung, N. Effects of biochar on enhanced nutrient use efficiency of green bean, Vigna radiata L. Environ. Sci. Pollut. Res. 2017, 24, 9460–9467. [Google Scholar] [CrossRef] [PubMed]
- Mateus, D.M.R.; Vaz, M.M.N.; Pinho, H.J.O. Fragmented limestone wastes as a constructed wetland substrate for phosphorus removal. Ecol. Eng. 2012, 41, 65–69. [Google Scholar] [CrossRef]
- Rout, P.R.; Bhunia, P.; Dash, R.R. Effective utilization of a sponge iron industry by-product for phosphate removal from aqueous solution: A statistical and kinetic modelling approach. J. Taiwan Inst. Chem. Eng. 2015, 46, 98–108. [Google Scholar] [CrossRef]
- Moharami, S.; Jalali, M. Removal of phosphorus from aqueous solution by Iranian natural adsorbents. Chem. Eng. J. 2013, 223, 328–339. [Google Scholar] [CrossRef]
- Vidal, B.; Hedström, A.; Herrmann, I. Phosphorus reduction in filters for on-site wastewater treatment. J. Water Process. Eng. 2018, 22, 210–217. [Google Scholar] [CrossRef]
- Tian, Y.; Cui, L.; Lin, Q.; Li, G.; Zhao, X. The sewage sludge biochar at low pyrolysis temperature had better improvement in urban soil and turf grass. Agronomy 2019, 9, 156. [Google Scholar] [CrossRef] [Green Version]




| Element | OQS | ISS |
|---|---|---|
| Amount (mg/g) | ||
| Fe | 267.5 | 13.1 |
| Si | 120.5 | 61.4 |
| Mn | 70.5 | 0.4 |
| Ca | 25.0 | 58.6 |
| Al | 24.0 | 18.9 |
| P | 20.0 | 31.7 |
| S | 0.5 | 3.2 |
| C | 0.16 | 167 |
| Model | Parameters | Process Indicator | Initial PO4-P Concentration (mg/L) |
|---|---|---|---|
| OQS | |||
| Linearized Langmuir model | mg/g L/mg | 9–45 | |
| Nonlinear Langmuir model | mg/g L/mg | 9–45 | |
| Linearized Freundlich model | mg/g | 9–45 | |
| Nonlinear Freundlich model | mg/g | 9–45 | |
| ISS | |||
| Linearized Langmuir model | mg/g L/mg | 11–45 | |
| Nonlinear Langmuir model | mg/g L/mg | 11–45 | |
| Linearized Freundlich model | mg/g | 11–45 | |
| Nonlinear Freundlich model | mg/g | 11–45 | |
| Model | Parameters | ARE (%) | |
|---|---|---|---|
| OQS | |||
| Linearized Langmuir model | mg/g L/mg | 0.96 | 6.2% |
| Nonlinear Langmuir model | mg/g L/mg | 0.97 | 6.9% |
| Linearized Freundlich model | mg/g | 0.92 | 7.0% |
| Nonlinear Freundlich model | mg/g | 0.96 | 8.2% |
| ISS | |||
| Linearized Langmuir model | mg/g L/mg | 0.97 | 4.3% |
| Nonlinear Langmuir model | mg/g L/mg | 0.98 | 4.1% |
| Linearized Freundlich model | mg/g | 0.96 | 5.7% |
| Nonlinear Freundlich model | mg/g | 0.97 | 5.1% |
| qe,exp | Pseudo-First-Order Linear Form (10) | Pseudo-First-Order Nonlinear Fit (7) | ||||||
|---|---|---|---|---|---|---|---|---|
| qe,calc | ARE | qe,calc | ARE | |||||
| 0.29 | 0.11 | 0.0132 | <0 | 78.19% | 0.288 | 0.044 | 0.87 | 26.94% |
| qe,exp | Pseudo-Second-Order Linear Form (11) | Pseudo-Second-Order Nonlinear Fit (8) | ||||||
|---|---|---|---|---|---|---|---|---|
| qe,calc | ARE | qe,calc | ARE | |||||
| 0.29 | 0.32 | 0.133 | 0.72 | 28.96% | 0.34 | 0.147 | 0.77 | 33.88% |
| qe,exp | Pseudo-First-Order Linear Form (10) | Pseudo-First-Order Nonlinear Fit (7) | ||||||
|---|---|---|---|---|---|---|---|---|
| qe,calc | ARE | qe,calc | ARE | |||||
| 0.25 | 0.57 | 0.134 | <0 | >100% | 0.25 | 0.081 | 0.99 | 1.77% |
| qe,exp | Pseudo-Second-Order Linear Form (11) | Pseudo-Second-Order Nonlinear Fit (8) | ||||||
|---|---|---|---|---|---|---|---|---|
| qe,calc | ARE | qe,calc | ARE | |||||
| 0.25 | 0.323 | 0.245 | 0.99 | 1.91% | 0.324 | 0.244 | 0.99 | 1.94% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Šarko, J.; Leonavičienė, T.; Mažeikienė, A. Research and Modelling the Ability of Waste from Water and Wastewater Treatment to Remove Phosphates from Water. Processes 2022, 10, 412. https://doi.org/10.3390/pr10020412
Šarko J, Leonavičienė T, Mažeikienė A. Research and Modelling the Ability of Waste from Water and Wastewater Treatment to Remove Phosphates from Water. Processes. 2022; 10(2):412. https://doi.org/10.3390/pr10020412
Chicago/Turabian StyleŠarko, Julita, Teresė Leonavičienė, and Aušra Mažeikienė. 2022. "Research and Modelling the Ability of Waste from Water and Wastewater Treatment to Remove Phosphates from Water" Processes 10, no. 2: 412. https://doi.org/10.3390/pr10020412
APA StyleŠarko, J., Leonavičienė, T., & Mažeikienė, A. (2022). Research and Modelling the Ability of Waste from Water and Wastewater Treatment to Remove Phosphates from Water. Processes, 10(2), 412. https://doi.org/10.3390/pr10020412






