The Outlines of a Possible Pension System Funded with Human Capital

Abstract

The broadly used pay-as-you-go (PAYG) pension system is intrinsically wrong. The essence of the problem is that the PAYG system distributes the yield of raising children, i.e., of human capital investment (which is essentially the pension contribution), in such a way that it disregards the extent to which individuals have contributed to this, and even whether it has occurred at all. This error can be corrected if we take the pension contribution to be the yield on an investment of human capital, and as such use this to pay back the costs and expenses of the raising of the contribution payer—overall to those who paid these costs and expenses at the time. Accordingly, the central question of my study is whether it is possible to construct a consistent pension system based on the above foundations, and how my ideas may be inserted into the Diamond–Samuelson model. The method of the study was logical analysis and the construction of a theoretical mathematical model. The results of the study show that it is possible to construct a public pension system that operates according to a different logic than today’s system, a system which is free from the effects of demographic fluctuations, which does not motivate the refusal to have children, and which will remain self-sufficient under all circumstances. The study achieves this by presenting a possible pension system of this kind in detail. Via the suitable modification of the Diamond–Samuelson model, I have succeeded in showing that the pension system I am proposing increases the willingness to have children up to the social optimum, in contrast to the fully (but traditionally) funded and PAYG systems. This system currently only exists in theory and may be regarded as a major theoretical innovation, which naturally has certain (although not particularly extensive) antecedents. Its introduction could enable the resolution of the contradictions of existing pension systems and could also provide a solution to the as yet unsolved problem of the increasingly expensive regeneration of human capital, and as such, its potential practical implications are immeasurable.

Notice

This study is the third part of a longer manuscript consisting of three parts, which builds to a major extent on the first two parts, the English translation of which can be found in the related Supporting Materials. It is expedient to begin by reading these. These two parts (which are also understandable individually) have already been published, the first originally in both Hungarian and English, and the second only in Hungarian, and I have cited them in the References as Banyár (2019b, 2020).

1. Introduction

The most popular pension system worldwide today is the non-funded, pay-as-you-go (PAYG) pension system, created in an ad-hoc manner by Roosevelt. The “official ideology” of this system was provided by Samuelson (1958) almost two decades after its introduction, greatly reassuring its operators, who until then had thought they were running a Ponzi scheme (Blackburn 2003) and felt that Samuelson had shown this was not the case (although a article in The Economist (2017) praised Samuelson as the inventor of a “good” Ponzi scheme.) According to Samuelson, the members of different generations had signed a social contract according to which current active workers maintain the current older generation, in exchange for which they can count on future active workers (the children of today, or those not yet born) to also maintain them when they get old. In the model that underpins the theory, Samuelson put forward the abstraction that the cost of raising children is zero (their consumption forms part of their parents’ consumption).

In 2014, I presented the argument (Banyár 2014) that if we modify Samuelson’s model a little and introduce the consumption of children, then we come to a totally different pension system, which still shows many similarities to the PAYG system. A decisive difference, however, is that this system is not unfunded; rather, it is funded using human capital (HC). As a result, the most important problem of the PAYG system, its dependence on demographics, is eliminated. I have demonstrated the errors of the PAYG system, and the principles according to which an HC system should be constructed, in several studies (Banyár 2016a, 2017, 2019a, 2019b, 2019c). However, the focus of these studies was always a description of the problems associated with PAYG, and I was only able to explain in a very limited manner how an HC system could actually be constructed. Below, I shall attempt to correct this omission and to present how an HC system could be constructed. To this end, I shall utilize elements that could also be useful in a well-operating PAYG system, which I have explained in greater detail in Banyár (2020), although I have also dealt with some of these in my previous works. These elements are: the introduction of individual pension accounts (Banyár and Mészáros 2009); automatic age limit indexing; the introduction of a quasi-mandatory, fully funded pension pillar; and the continuous, as opposed to parallel, disbursement of the pensions acquired from this and the HC (or PAYG) system (Banyár 2016b).

In support of my argument, moving beyond Banyár (2014), in which I modified the original Samuelsonesque model, in Appendix A I have inserted the costs of raising a child into the classic Diamond–Samuelson model, and shown how the HC model affects the number of children (it increases it up to the social optimum) and young age consumption (it also increases it, but more importantly it makes it independent from the number of children), in comparison to the no pension model and PAYG models.

To facilitate the orientation of the reader, I have briefly summarized the most important characteristics of the traditional PAYG system and the HC system I am proposing, which I have discussed in detail in the Supplementary Materials, and the conclusions of which are developed in this paper, in two tables.

Table 1. The main characteristics of the pay-as-you-go (PAYG) and human capital (HC) systems.

PAYG	HC
A “pure” system, which is viable without other pension systems.	Only imaginable as a composite system; people with no or few children still require a traditionally funded pension subsystem.
According to the old approach: non-funded—the only one of its kind from among the possible pension systems.	(Fully) funded, like all possible and rational pension systems. The capital is the human capital of the raised, new generation, which is repaid to the investors by its owners (with interest).
According to the new approach: the capital behind the system is an implicit sovereign debt, which is huge, 2–4 times the annual GDP, because of which its value is often kept secret, although within the EU it has been mandatory to indicate this since 2017.	In view of the above, the implicit (and explicit) sovereign debt behind the system is 0.
The basis of the pension is the contribution payment capacity of the new generation, but this has not been built into the system, meaning the level of individual pensions is not dependent on this.	The basis of the pension is the social security contribution payment capacity of the new generation, which has been explicitly built into the system, meaning that the level of individual pensions is dependent on the investment made towards the creation of contribution-payers.
Motivates depopulation because it makes it economically irrational to raise children.	Compared to the existing situation, it encourages the maintaining of a stable population, but in a general sense it makes it economically neutral whether people raise children or provide for their pension in some other manner.
Based on a contradiction, because although it assumes and requires an increasing population, it in fact encourages a decrease in population, meaning it is highly vulnerable to demographic changes that are mistakenly regarded as exogenic factors.	Logically coherent, neutral to demographic changes (that are regarded as endogenic by the system); will adapt to the changes whether the population increases or decreases. Only the ratio of systems funded with human and traditional capital will change.
Economic policy is seemingly free to determine the volume of pension to be distributed, and thereby the level of the average pension.	The volume of the pension is more or less given as the estimated value of the investment in human capital and the interest on that investment, which people pay as a contribution during a determined period of their working lives.
Often realizes an unfair distribution of burdens between generations.	Represents a fair distribution of burdens between generations.

compares the PAYG and HC systems, and

Table 2. The specialties of the HC system I am proposing in this paper, which would also be worthwhile introducing in traditional PAYG systems.

Traditional PAYG System	Reformed PAYG System	HC System as Proposed Here
No individual account	Individual account, where the paid contribution is accumulated and valorized.	Individual account, where the paid contribution is accumulated and valorized.
Defined benefit (DB) in character—the contribution is determined based on an index series (usually the period of employment, the level of contributions of a chosen period, etc.).	Defined contribution (DC) in character. The pension is determined exclusively based on total contributions and life expectancy. Specifically, a Non-financial Defined Contribution (NDC) or point system (practically: a defined contribution that resembles defined benefit).	DC in character, and specifically a point system. But in this case, keeping records of contributions serves for the recording of obligations, not entitlements, meaning that they generate no points. The entitlements, which are recorded using points, are proportionate to the period and results (the child’s qualifications) of child-raising.
Individual pension—rights are acquired by everyone based on individual performance.	Family pension. The acquisition of rights by spouses during their marriage is a joint performance, which is halved if they become divorced, and which is inherited by the widow on the death of the spouse. In view of the joint acquisition of rights, the pension is a joint annuity. There is no separate widow’s pension.	Family pension. The acquisition of rights by spouses during their marriage is a joint performance. Joint annuity, without a separate widow’s pension.
Fixed age of retirement that is corrected at great intervals.	Continuous retirement age indexing based on changes (practically: increases) in life expectancy.	Continuous retirement age indexing based on changes (practically: increases) in life expectancy.
Has no funded subsystem, but there are also funded pension systems operating parallel to it that are not integrated into the system.	Has a subsystem that is funded (traditionally, not with human capital), and which is proportionally the same for everybody.	Has a subsystem that is funded (traditionally, not with human capital), but the size of this changes on an individual basis, depending on the number of children.
The pensions of the PAYG and funded pension systems are paid out parallel to each other.	(Potentially, because no such international example exists as yet,) the pensions received from the funded and PAYG systems are paid out consecutively in such a manner that the level of the pension is equalized during the full pension period. Upon reaching the normal age of retirement, the capital of the funded system is first spent as a phased withdrawal (or possibly saved), following which payment of the higher pension (in view of the higher age limit) begins from the state system upon reaching the higher age of retirement.	The pensions received from the funded and PAYG systems are paid consecutively in the manner explained previously.

presents the elements that I explained in detail in Banyár (2020) (the English translation of which may be found in the Supplementary Materials) which I have built into my proposal for the HC system described here, but which could also be applied within the framework of a reformed PAYG system. In fact, some of these are already being used in certain countries.

In the literature, numerous authors have already attempted to link the raising of children and the pension system. Demény (1987) was the first to propose this, but his solution was extremely simple, although this is precisely what made it attractive to many, even today. Werding (2014), Giday and Szilvia (2018) and the Botos and Katalin’s (2020) put forward complex proposals. Their recommendations differ from those set down in this paper with relation to the principle (in my opinion mistaken) that the raising of children is equivalent to the payment of contributions. In contrast, I regard contribution payment as the return on the initial investment of human capital, which children are obliged to (re)pay, but which does not make them eligible to receive a pension. In this regard, I am fundamentally following Hyzl et al. (2005), who formulated an early version of what is described below. The thoughts presented here also differ from the proposals for linking the pension system to the raising of children put forward by practically all other authors with respect to the fact that they regard the goal of such a system to be to encourage an increase in the number of births. This is clearly indicated by the title of the article by Regős (2015): Can Fertility be Increased with a Pension Reform? I, however, believe that we must create a pension system that remains in balance regardless of the number of births. I shall attempt to do so below.

2. Should We Disburse the Profits of Child-Raising or Not?

First of all it is important to note that the current paper uses the term “child-raising” throughout, rather than “having a child”. By doing so, I would like to emphasize the fact that only efforts aimed at raising a child count, and it is not even relevant whether someone is the biological parent of the raised child. In other words, if someone is the biological parent of several children but does not raise them, they are not due any kind of pension. Accordingly, it is also inconsequential if someone cannot have children for biological reasons, because the opportunity to raise children via adoption is nevertheless open to them.

It is in theory very simple to link the raising of children to pensions—children should maintain their parents in their old age, just as their parents theoretically used to do for them. Thus, when we call for the two to be linked, there immediately arises a simple and obvious solution, the alternative of which is for the state to pay out the profits of child-raising among pensioners, while applying risk balancing. In the following, I examine whether such a solution is expedient.

A legislative declaration, such as the one already in existence in China (Constitution 2004, Article 49), and also in Hungary’s Fundamental Law (“Article XVI … [3] Parents shall be obliged to care for their minor children … [4] Adult children shall be obliged to care for their needy parents”) is sufficient to achieve the simple and obvious solution. This may be truly interpreted in this manner in China, but certainly not in Hungary, or at least not for the moment. However, the issue also has theoretical followers.

For example, in his logic Pál Demény, who was the first to internationally raise the linking of child-raising and pensions, recommends a similar, but much less strict system (Demény 1987). He suggested that part of the child’s contribution should be transferred to his/her pensioner parents, meaning that a direct link is established between the child’s contribution payments and the parents’ pension. This was criticized and also further developed by Julian L. Simon, according to whom parents and their children should sign a contract whereby parents finance the university studies of their child, in return for which the child will provide the parent with a pension (Simon 1988).

Truly, nothing could encourage the high-quality raising of children more than if parents were to strive to raise their children in such a way that they will be able to earn as much as possible. However, this method would introduce an uncertainty into pensions, and would make both burdens and profits highly uneven. If someone’s parents were to die early, they would not have to pay anything, but if they live a long life, they would have to pay a great deal. Parents may already have reached retirement age while their child is still studying, so regardless of how well they may have raised their child, they will not have a pension. Some children may refrain from making the required payments, the child may die, etc. It is therefore expedient to balance both burdens and profits, i.e., to operate the HC system similarly to today’s state pension system, and accordingly I shall refrain from examining the simple solution below but shall instead put forward a proposal for a system that disburses profits.

3. The Cost of Raising a Child

Since the logic of the HC system (which should have intrinsically been the logic of the PAYG system) is that the pension is the return on the raising of children, as a form of capital investment, a prerequisite of the system’s operation is that we assess as exactly as possible the cost of raising a child. This is also what we shall do here, although for the moment only at a theoretical level, without concrete numbers.

It is obvious that the cost of raising individual children requires significantly different material and temporal investments, and it is also difficult to distinguish which of these are necessary, superfluous or possibly have a negative return. The extent of state funding received towards this end also differs in view of the fact that child-raising subsidies are neither automatic, nor of a uniform level, for instance, in the case of income-dependent tax concessions.

However, the individual costs are in fact unimportant, just as in the case of other “goods”, where, at least in principle, a uniform market price prevails. Of course, human capital has no market, but using the market price as an analogy we are able to establish a standard cost, with monitoring, continuous measurements and corrections. During the course of measurement, we must take into account the child’s average consumption (food, clothing, accommodation, etc.), the cost of their education and medical care and the time parents1 spend on raising them. An important separate issue is what share of this is borne by the “public” (i.e., the average taxpayer) and by the parents. The public’s share is primarily realized via nursery school, school, healthcare and transfers relating to the child (family subsidies, child-related tax concessions, funding towards the purchase of a family home or car, etc.).

An important question of principle also arises here: should we take the fact that the pension is the repayment of the costs of child-raising literally, or in a broad sense? If we take it literally, then it is very important to assess the average costs as precisely as possible, and in fact, if we regard these as an investment, then we must also calculate and assign some kind of return to them. If we interpret it in a broad sense, however, then it is sufficient to calculate the distribution of the costs in relation to private contributions (essentially the money and time spent by parents) and public contributions.

As a first step, it is expedient to satisfy ourselves with the broad approach. Therefore, we assume (which should be calculated in reverse) that the current average level of the (state) pension, multiplied by the average number of years spent in retirement, is roughly equivalent to the value, with interest, of the cost of raising a child (two per married couple). This is admittedly a practical approach, not a theoretical one. The justification is that if we embark on a program of precise assessment, a set of extremely difficult questions will be opened, which are capable of making the system highly complex, and could pose a barrier to its realization. Nevertheless, the possibility of later, precise assessments must be left open, as must the opportunity to gradually adjust the system to this, since we must strive for theoretical solutions, but must be aware of the fact that there will occasionally be a need for practical (and possibly temporary) simplification (just as in all social systems).

The principle of the non-broad approach would otherwise be as follows: a general balance is realized between the human capital investment and the total contribution volume paid by the raised child. This may be defined as a very general balance equation (let us call it the GBE), according to which

the cost (with interest) of raising a generation = the discounted value of the
total contribution volume expected to be paid by the generation (in the
manner set down in the contribution payment regulations)

As a result of the above, a condition for the system’s operation is that these costs are continuously measured and calculated. When the basic parameters are set at the launch of the system, we may begin by applying the abovementioned broad estimate, but will have to calculate increasingly precise values as time goes on (which is also an important issue with relation to the system’s legitimacy).

During the application of the GBE, we must obviously also take into account the fact that some children will never become contribution-payers, because they unfortunately die as children, or are born disabled or become disabled. This, however, is one of the intrinsic risk factors of raising children, and accordingly it is expedient also to include in the equation deceased children and the costs of raising disabled children.

It may also occur that a child who has been raised and has become a contribution-payer dies during the course of their active career, and accordingly this mortality must also be taken into account on the right side of the GBE. Children who have remained alive must (partly) repay the costs of being raised in their place.

Furthermore, some kind of equitable interest rate must also be applied. A logical candidate is the average wage index, since this may be regarded as the rate at which the value of human capital increases each year on average.

A result of the calculation that is already necessary initially is the ratio of individual (i) and public (p) efforts with relation to raising a child, where i + p = 1. This ratio must also be continuously measured and actualized. In addition, individual efforts are in the most part not financial, but are made in the form of time spent, which may be converted into money using opportunity cost, for instance, how much the parent would have earned if they had been employed at a workplace during this time. Public expenditure must include the transfers received with relation to the child (the various maternity leave and childcare benefits), the tax concessions utilized, the costs of the child’s free education and healthcare, etc. These may of course change with time in line with changes in regulations. In the beginning, in Hungary, we recommend the application of a ratio of 1.9:1 = 0.66–0.34, which is an estimate based on the Hungarian data currently available (Gál and Márton 2019). This means that according to surveys, in Hungary the individual effort with relation to raising children is 1.9 times that of community effort, meaning that parents spend 1.9 times a much time and money on raising their children (on average) than they are repaid by society for doing so, i.e., what may be regarded as the taxpayers’ contribution. The community effort is a result of the fact that in Hungary, as in most developed countries, social protection schemes reward families with children in a number of ways, including child-care specific benefits, unconditional basic income, publicly subsidized childcare, tax credits for families raising children, child or family allowances and the provision of maternity and paternity leave.

4. Repaying the Cost of Being Raised—The Place of Contributions within the HC System

4.1. Uniform Contribution Rate with No Upper Threshold, Uniform Contribution Payment Period

Based on the GBE, we may also determine the level of contributions. Two parameters are important here: for what period must contributions be paid, and with relation to what proportion of income?

Above, we have already discarded the idea that a child must repay exactly the amount that their parents and society have spent on him/her. Instead, we recommend the use of the “results approach” used in the case of venture capital investment, from which it follows that it is expedient for the contribution to be a set percentage of income (just as occurs today) and there is no justification for imposing an upper threshold on the contribution level.

However, in the case of the HC system, it is not justified for the period of the contribution payment to be the full lifetime spent in active employment, as is the case in the PAYG system. Because today this is primarily what supports the fact that the service provided should also be in proportion to this. In this HC system, however, the pension is exclusively dependent on child-raising efforts, and not on contributions. The contribution does not belong to the investment “side”, but exclusively to the system’s return “side”.

In other words, repayment is the obligation of all raised children, and this must be set down from some perspective. If the amount is not fixed, then the total period of contribution payment could be. Of course, what this fixed period should be is a parameter that may be chosen relatively freely. The restriction is that the lower it is, the higher the contribution rate (based on the GBE). Hereafter, we recommend using a period of 30 or 35 years.

4.2. Handling Exceptions—If Someone Is Unable to Pay Contributions for a Sufficient Period

There of course exist a number of cases in which someone is unable or unwilling to pay their contributions until the end of the uniform contribution payment period, meaning they are unable or unwilling to repay the cost of having been raised. The most important cases include if someone dies at working age, if they become temporarily ill for a long period, or are permanently disabled, unemployed, are raising a child, move abroad or launch a business from which they receive no income in the form of a wage. Within the system, we may handle these cases in three ways. We may regard them as part of the system’s risks and distribute them among the other participants, we may attempt to compensate for them somehow or we may exclude the affected parents from receiving pension services. The question is, in which category should we place these individual cases? Below, we shall attempt to fit the most important cases into these three categories and to justify this categorization.

4.2.1. The Distribution of Risk

It is expedient to handle exceptions that are beyond the individual’s control by distributing the risk within the risk community. Early death and disability are clearly such cases. The probability of these occurring must be included on the right side of the GBE—this is the method by which the risk is distributed. It is questionable whether illness and unemployment should also be included in this category. An argument for doing so is that these can (generally) also not be controlled by the individual, whereas an argument against it is the fact that even in this case, they still have some form of income into which the contribution may be incorporated. In the first case, this is also included in the probabilities found on the right side of the GBE, whereas in the latter case these two factors are moved to the compensation category.

It is not really expedient to apply risk distribution in the case of someone raising a child (some other solution must be applied instead) because by doing so, in contrast to the PAYG system, which is unfair to people who raise children, the HC system would risk making the opposite mistake: becoming unfair with respect to people who do not raise children. In such a case it would place a double burden on them: they do not receive a pension as a result of having raised a child, in addition to which they would also have to pay part of their parents’ contributions (i.e., what their parents owe to their own parents).

In this context it is interesting and somewhat ironic that this study regards the greatest fault of the PAYG system to be that it distributes the risk of not raising children, but Hans-Werner Sinn, who also formulated arguments in favor of linking the raising of children to the pension system, regards it as its greatest advantage—see Sinn (1998).

4.2.2. Compensating the Non-Payment of Contributions

Overall, it may be stated that we must strive to handle the majority of exceptions through compensation, because by doing so the contribution rate will not increase, nor will the pension decrease, and fewer people will have to be excluded from receiving a pension.

Illness, Unemployment

In the case of long-term but temporary illness or unemployment, compensation may come from two sources, and these are non-exclusive. The required pension contribution may be built into their sickness benefit or unemployment benefit. In such cases, the basis for the contribution is the same income that forms the basis for the sickness benefit or unemployment benefit. By doing so, we of course still distribute the risk of contribution non-payment, but not among the contribution-payers, but among those from whom we collect the consideration relating to these two benefits.

The other possibility is that we extend the contribution payment period and extend it into the pension payment period for a time. In practice, this means that we deduct the missing contributions from the pension benefit with relation to the missing years. In view of the fact that a pension can have two sources, the HC and FF (fully funded) systems, and the latter would precede the former, meaning the primary source for the missing contributions would be the savings accumulated within the FF system. In such cases, the level of contribution may be the value of the average contribution paid until that time, valorized using the average wage index (but of course not exceeding the value of the pension).

Contribution Jointly with Spouse, and/or Grandparent’s Pension from the Parent’s Pension

When someone is raising a child (and the child is still so small that one of the parents is unable to work parallel to raising the child) and this period conflicts with the contribution payment period (which we otherwise strive to avoid when planning the system), the parent in question may only receive a temporary exemption from having to pay a contribution, but their obligations remain. This situation may be handled in several ways, among which it is expedient to establish a kind or order of priority, meaning we must begin by applying the first method, then move on to the second method once we have depleted all the possibilities afforded by the first. The third method may be chosen freely above a certain number of children (e.g., three). These methods are:

• If the spouse of the parent who is raising the child full time is working, then the contribution paid by the working spouse must be regarded as a joint contribution. This means that we accept the one-year contribution payment of the spouse as covering them both, but only for a 6-month period, meaning that the contribution payment period of both parents will be extended by six months. This also means that the parent who remains home with the child (generally the mother), does not fall behind with relation to fulfilling his/her commitments compared to the parent who continues to work during this period (usually the father), meaning the system is already intrinsically more equitable than the current one. The logic of the method is absolutely parallel to the family pension system mentioned in the introduction and detailed in Banyár (2020). In such cases, the level of contribution is given. As a supplementary solution, it is worth allowing the working spouse to pay a larger than necessary contribution during this period. The higher the contribution paid, the more years the system regards as having been paid in contributions from the mandatory number, also with regard to both parents.
• If despite this there still remain a few years of contribution payment obligation when the parent reaches the age of retirement, then the contribution may be deducted from the pension in the same manner as described in the point above, as recommended in the case of unemployment or long-term illness. Here too, the level of contribution is equal to the valorized average of the level of contributions paid during the individual’s working career, as above in the case of illness. If their contribution was partly paid in their place by their spouse, it is this level of contribution that will be included in the average calculation for the given period.
• The introduction of a full-time mother program on a market basis, without state funding. In view of its length, this solution is detailed separately below.

Full-Time Mother Program on a Market Basis, without State Funding

In most cases, people will fall behind with their contribution payments with relation to raising children because they raise a large number of children and simply do not have the time to work (or at least, not for a long enough time), and as a result will be unable to repay the costs of their having been raised. If someone gradually finds themselves in such a position and would not like their pensions to decrease to an uncertain extent, there comes the third method, whereby they come to an agreement with a (generally childless) external sponsor (ES) with relation to the fact that the latter will pay a regular sum appropriate to the average cost of raising a child with respect to one (or more) of the children, until they are fully raised.

In the case of such an agreement, if the parents have not yet fulfilled their contribution payment obligation, a sum equivalent to the monthly average contribution must be deducted from the monthly sum paid by the ES in the interests of fulfilling their contribution payment obligation. The relationship between the average monthly cost of raising a child and the average monthly contribution can be easily calculated using the GBE.

The ES is motivated to conclude such an agreement in the interests of purchasing pension points as a result (one for each child sponsored until they are fully raised), because by doing so raising the child will not count towards the parents’ pension, but towards the ES’s pension. This means that by doing so the ES can participate in the raising of the new generation without actually raising a child; it can entrust this to people who are more willing to do so than they are. Accordingly, this could essentially enable the establishment of a “market-based, full-time mother program” without the need for state funding. The role of the state would be restricted to, for instance, facilitating contact between “supply and “demand” via a special website.

It is important to note that by entering into such an agreement the ES also undertakes a kind of “investment” risk, just as parents also generally do, because, in view of reasons to be explained later, pension points would only be due with respect to children who acquire at least a secondary school leaving certificate level of education. Therefore, if the ES sponsors a child who will be incapable of this, then their investment will be worth nothing.

The question may occur: why not make things simpler by simply accepting the raising of one of the children as contribution payment? The answer is that this solution may create the equivalence of rights and obligations at an individual level, but it would cause financial problems at the system level. In this instance, the missing contribution would have to be deducted from the contributions already paid by current pensioners and would therefore increase the later pension of current active workers without them having worked for it, since the contribution payments of the child raised in lieu of the payment of contributions would now be providing them (current active workers) with a pension. This would essentially include the same mistaken solution into the HC system which generally characterizes the PAYG system, and which we are precisely aiming to correct with the HC system. This is true despite the fact that there will otherwise be a kind of generational “hiccup” in the system in view of the fact that there may be a significant variance in the age at which people have children (particularly in the case of men), and because in certain cases the payment of contributions may extend into retirement age. However, in theory these cases will balance each other out in the long term.

Income Not Derived from Employment

Unfortunately, this problem is a general problem relating to today’s system of employment pensions and affects both the PAYG and HC systems, and we do not provide a general solution to the problem here, but simply indicate that some kind of solution must be found for cases in which someone’s income is not derived from a working wage, and as a result they do not pay any contributions. This is also problematic because they may raise a child and will become eligible for a pension as a result. However, they may pay the unpaid contributions in installments out of this pension, as detailed above. Another possibility is that we introduce some kind of contribution payment for such autonomous people, either in the form of a flat rate, or dependent on income at some level.

When Children Move Abroad

If a child moves to another country, works there and pays contributions there, then the cost of raising them does not generate a return at home; it is as if they had never been born, and accordingly—in the first instance—no pension benefits will be due with respect to such children, and parents must be excluded from receiving a pension (with respect to the child in question). However, it may not necessarily be expedient to act in this manner, even though for practical reasons we do suggest that the risk of moving abroad should not be distributed among the risk community.

Moving abroad is the best indication of the extent to which the ideology of the PAYG system is false, because according to the system in such a case there is absolutely no problem here; the child who has moved abroad pays into the social security system there, based on which they will become eligible to receive a pension there. It is, however, obvious that what in fact happens in this case is that the social security balance of host countries is improved by workers who move there, whereas the social security balance of the countries they leave behind becomes worse. It might be stated that the worsening social security balance of the host country could be saved—at least for a time—using this method, to the detriment of the sending countries. It means that the cost of investing in children is borne by one country, but its profits are reaped by the other country.

This is in itself a sufficient argument for switching from the PAYG system to the HC system, because this uncovers yet another failure, and in fact an implicit background assumption, of the PAYG system—the fact that it assumes a closed economy, one from/to which employees do not come and go at will. Meaning that, for instance, the PAYG system and the EU’s “freedoms” are not compatible with each other, or rather only to the extent that they favor developed EU countries in a one-sided manner, since the workforces of less developed EU countries are fundamentally flowing into these better developed countries (Banyár 2014).

The problem could, however, in theory be solved if the net sending countries affected were to initiate negotiations for EU internal regulations that ensured that the pension contributions of young people who emigrate (or at least part of them) continue to be paid into the social security system of the sending country for a certain period. If such an agreement is successfully concluded, then parents whose children move abroad will not have to be excluded from the pension system. The most expedient would be a regulation of this nature that extends to the whole of the EU, which could be jointly initiated by the net source countries of intra-EU migration. Later, similar agreements may be initiated with other countries based on the intra-EU agreement. Without an agreement (or if someone emigrates to a country with which no such agreement exists), the following method may be applied.

4.2.3. Exclusion from Pension Services

If, however, such an international agreement is not possible, it is recommended that exclusion and not risk distribution be applied with relation to children who move abroad, since this is the result of a conscious decision, and not analogous to a child becoming disabled or dying. There are naturally also certain technical problems in this instance, which must be solved later, because working abroad may also be temporary, after which people return home, in which case exclusion from receiving pension services is not an expedient solution.

It is prudent for young people who have moved abroad for the long term to expect to have to compensate their parents for their lost pensions themselves, via direct transfers, meaning that when they calculate the profits and expenses associated with moving to another country, they should also take this into account on the expenses side (and the attention of all young people must be drawn to this in a timely manner).

Children moving abroad is the main cause for exclusion from pension services, but other, hopefully very rare, cases may also occur. These include if the fact that the child becomes unable to pay contributions is a direct result of the actions of the parent(s) or the person who raises them, e.g., if they become disabled or a criminal. Of course, in the majority of such cases this is not the fault of the parent and accordingly it must be duly assessed, possibly via an individual decision with the involvement of the courts, whether or not this is in fact the case in a concrete instance.

5. The Distribution of Contributions: Pension Services within the HC System

The GBE not only determines the level of contributions, but also the level of pension services, providing we determine the most important principles with relation to distribution, and based on those the following rules.

5.1. Current Financing May Remain as the Principle for Distribution

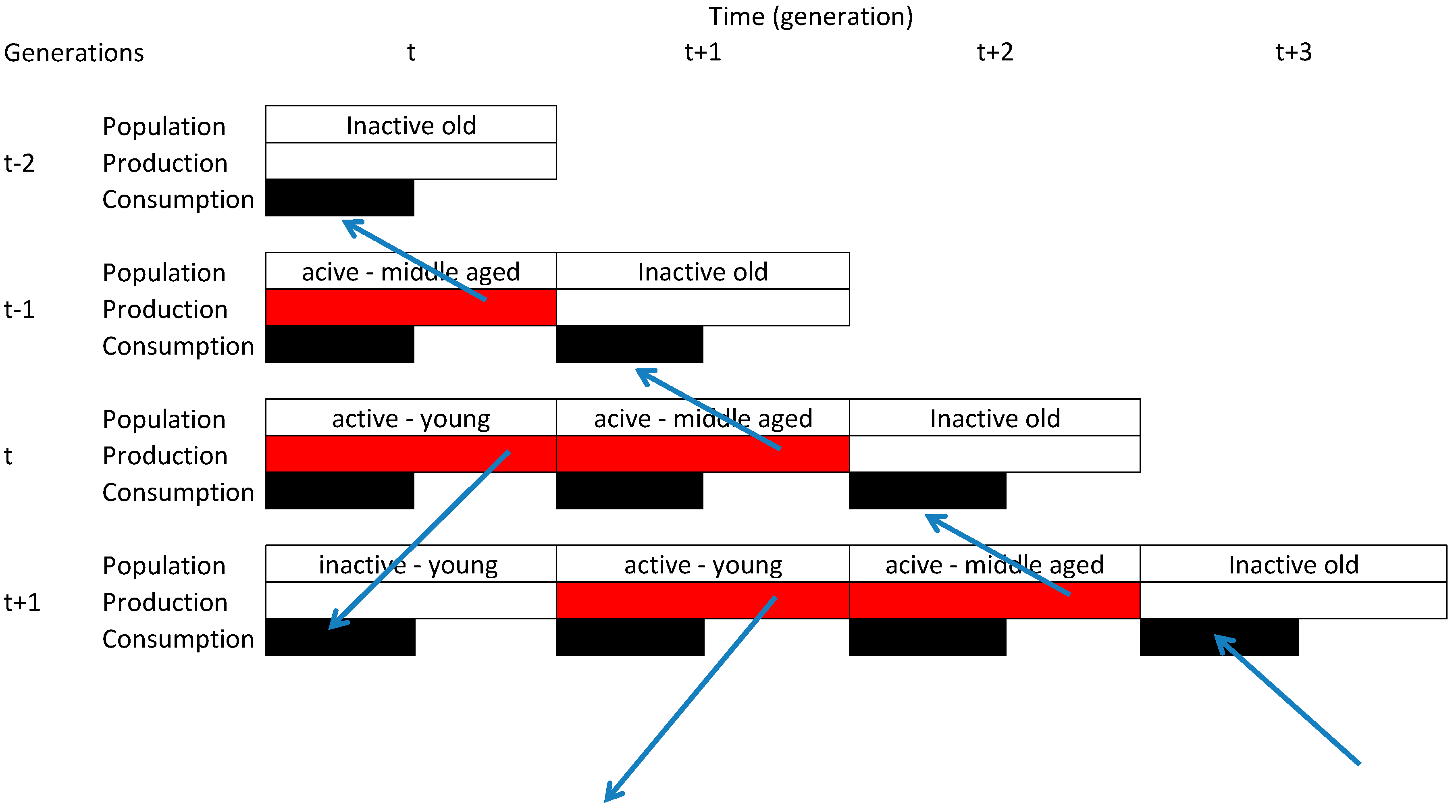
The first principle may be that each year it is expedient to disburse the contributions that have been paid during that year in the form of a pension, since these contributions now represent a kind of “dividend” (and of course also “partial repayment”). In this respect, the HC system is similar to the PAYG system. It fundamentally requires no separate financial reserve in view of the fact that its actual reserve is human capital (in an acknowledged manner, and in this regard, it differs radically from the PAYG system).

5.2. Pension Benefit Indexing Is Automatic, but There Are Conditions to This

Pension benefits do not need to be indexed separately in view of the fact that they are automatically indexed in relation to changes in the volume of contributions paid in during the given year. If the system’s parameters are set up well, then the automatic index will be the wage index (with minor fluctuations), since the volume of contributions will increase parallel to this, regardless of any of its other unchanged factors. Many things will change, of course, but these changes are partly neutralized automatically, and may in part be neutralized through clever regulation.

Changes in the number of active workers will be automatically neutralized in view of the fact that this is also mirrored in the number of people who are eligible to receive a pension. If fewer children are born, the HC system will have fewer eligible participants, and more people will receive services from the FF system (or rather, in practice they will receive services for a shorter period from the HC system and for a longer period via the FF system, according to the concrete proposal).

The automatic age limit indexing proposed in Banyár (2020) and mentioned in the introduction (meaning that we always increase the age of retirement to ensure that the expected remaining lifetime at that age threshold remains a fixed value) enables us to neutralize the increased lifetime effect, as a result of which we would have to distribute the same volume of contributions over an increasingly longer period. If we can count on the number of years in retirement remaining constant on average, then the pension services will not change as a result of an increase in lifetime, because the ratio of years spent paying contributions and years spent receiving pension services remains constant. If, for instance, we set the expected years receiving a pension at 15 or 17.5 and the number of years of contribution payment to 30 or 35 (as recommended above), then their ratio will be a constant 2, meaning the pension received with relation to a raised child (per head in case of a married couple) will remain stable at roughly twice the average contribution (at least in the case of contributions paid for one person—see explanation regarding family pensions).

5.3. The Recommended Method for Distributing Contributions into Pensions Is a Kind of Points System

It is expedient for pensions to be such that the pensions received by various generations of retirees do not diverge as they do within the current Hungarian PAYG system. This means that someone receives the same pension in a given year with respect to having raised the same number of children regardless of whether they retired 20 years ago or whether the pension has only recently been determined for them. This problem is solved very well by the German points system, and accordingly we recommend its adaptation.

However, the German points system currently serves a classic PAYG system, meaning that points may be received with relation to the payment of contributions. Within the HC system, however, eligibility for a pension is not acquired in exchange for the payment of contributions, but only with respect to raising children, and accordingly the points (let us call them “pension points”) can only be distributed based on an investment towards raising a child.

5.4. Principles behind the Distribution of Pension Points

The primary principle is that we must differentiate between points that are awarded based on individual (i) and community (c) contributions. The ratio of individual and community pensions points awarded with respect to the raising of a child is i/c.

5.4.1. Pension Points (Based on an Individual Contribution) That Are Due Directly with Respect to Raising Children

For the sake of simplicity, let us determine the value of a pension point as being due with respect to an individual contribution towards the raising of a child until it reaches the age of 18, providing the child has acquired at least a secondary school leaving certificate.

I believe it is worth considering the possibility that no pension points should be due for levels of education lower than a secondary school leaving certificate, or that in such cases it should only be possible to acquire points following a separate procedure. The justification for this is that in today’s economic conditions it is practically certain that a child who does not even reach the level of a secondary school leaving certificate will never become a contribution-payer, meaning that an investment in such a child will not provide a return. From a different perspective, however, this regulation would strongly motivate both parents and children to acquire at least a secondary school leaving certificate, even after the age of 18. In such cases, parents would receive their single pension point at a later date. If it transpires that a child who lacks a secondary school leaving certificate has nevertheless become a good contribution-payer, then following a sufficiently long period (to be defined later) of performing as a good contribution-payer, it should be made possible for a request to be submitted enabling the parents to receive their pension point.

It is not fully clear whether we should differentiate further between children based on their level of education, or according to what criteria we should differentiate between (adult) children. In favor of differentiation based on the level of education is the fact that there are major differences between people who only have a secondary school leaving certificate and people with higher education diplomas with relation to average income (and accordingly, the level of contribution paid). An argument against this differentiation, however, is the fact that this could give rise to large individual differences, which could lead to tensions. It is almost certain that in the case of such a differentiation unemployed university graduates, with respect to whom their parents receive higher-than-average pensions, and people with only secondary school leaving certificates, who are exceptional contribution-payers but whose parents receive much more modest pensions, will be paraded on television.

For this reason, we recommend considering the supplementation of this differentiation with a bonus system, according to which we intrinsically increase the pension point linked to a secondary school leaving certificate in proportion to the time required to achieve a higher level of education. In other words, a parent (both parents together) whose child acquires a basic level (bachelor’s) degree should receive 21/18 pension points in view of the fact that to acquire a bachelor’s degree parents must maintain their child at least until the age of 21, and parents whose child achieves a master’s degree should receive 23/18 pension points for similar reasons. The fact that someone only acquires a bachelor’s degree at, for instance, the age of 28 and lives with their parents until that time need not, and in fact must not, be rewarded by the system with a higher number of points.

It is expedient to split the (one whole or somewhat larger) pension point received with respect to one child between those participating in the raising of that child on a pro rata temporis basis (or at least, when the system begins operating, it should not be examined whether or not the contribution fluctuates according to the age of the child). If a married couple raise the child together, they share the point equally. If a parent has raised their child alone, they receive the whole point. If they are divorced, but continue to raise the child together, then they will also continue to share the point equally; if they do not share in the raising of the child equally, then they will share the pension point on a pro rata basis for the period during which they are not raising the child together. For instance, if someone is only paying alimony, then they and their partner-parent will share the fraction points in a ratio of 0.3–0.7 (it is expedient to determine and continuously maintain the precise figure via assessments). If a parent is not even paying alimony, then the parent who is raising the child will receive everything with relation to the raising of the child.

The points of a parent who has died will be transferred to the parent with whom they have been raising the child until that time. If the child is later adopted by another parent, then the adopting parent will from then on receive fraction points on a pro rata basis.

The same rule applies in the case of children who are not the parent’s biological child but are adopted. No pension points are due with respect to fostered children, in consideration of whom the state pays a fostering allowance.

It would be logical for pension points to be due with respect to the actual level of contribution payments, but in many cases, this is impossible, since the parent may already have retired some time ago, but the child is not yet paying contributions, or because the level of contributions may change continuously, or even fluctuate wildly in line with the level of payments realized by the child. It would not be expedient for all this to be mirrored in pension services. At the same time, it is absolutely justified, and easily derivable from the basic principles of the recommended system, that parents whose children earn an outstanding income, and therefore pay an outstandingly high level of contributions, should also receive a higher pension. However, the demand for someone’s pension to be reduced simply because their child’s career (and therefore their contribution) has encountered a fracture, or because they have given up their highly paying workplace to take on a job that generates a high social profit, but is badly paid, is problematic. In view of the above, we do not recommend directly linking pensions to children’s contribution payments (as suggested by Demény (1987) and those who accept his logic), but it is instead expedient to introduce a kind of bonus system (without a malus system). This means that parents should not lose any additional pension they may have received even if the child’s salary is reduced in the meantime. However, this also means that bonuses may only increase the level of pensions tentatively. One possibility is that parents should receive additional fraction points with respect to their child if the child’s income is, for instance, at least double the average contribution for a period of three years. This additional fraction point could be proportional to how many times the contribution exceeded the average contribution (provided it is at least twice the average) and could increase continuously in accordance with the period during which the contribution is higher than double the average. The determination of the precise values requires a separate calculation, which it is only worth formulating once a consensus has developed with relation to the above proposals.

5.4.2. Pension Points That Are Based on Community Contributions

All taxpayers, whether they raise children directly or not, also receive pension points with respect to the level of their community contributions, i.e., the “c” part of the total number of points with respect to raised children. In order to determine the number of points that may be distributed and the ratio of distribution, several problems must be solved.

In view of the fact that it is recommended that points are distributed continuously (based on a suitable set of records) each year, it is clear how many points may be distributed in total with respect to the number of children—a total of 1/18 point per year per child, although some of these will later be regarded as invalid because of a lack of a secondary school leaving certificate. However, this will hopefully not be significant, and accordingly we may disregard it during the calculation of the c part. Since this is equivalent to the “i” part of the points, this community point multiplied by c/i must still be distributed among all taxpayers in proportion to their tax payments. However as one of the reviewers of the paper has correctly pointed out, in countries such as Israel that have a childless tax, the tax behaves much more like an individual targeted child-raising contribution than child-raising funding provided via general taxes (meaning it behaves as i rather than c), and in such special cases the accumulation of points must be amended accordingly.

Unfortunately, we do not possess (and in theory cannot possess) a set of records concerning who pays what level of taxes, since there are many types of tax, from personal income tax to consumer tax. For this reason, in order to distribute community points, the ratio of taxes paid by individuals must be estimated in some way, meaning we must apply some kind of proxy. In addition, it is expedient to narrow down the period during which points are acquired through paying taxes, because for instance a pensioner also pays taxes, but by that time it will be difficult to amend the number of pension points accordingly. It is expedient to limit the acquisition of points to the period of active employment only, or the contribution payment.

The payment of contributions could be an obvious proxy for tax payment, since it is proportional to earnings and personal income tax, and the value-added tax paid with respect to consumption is probably also proportional to wages. However, there are two arguments against this idea:

• Above, we recommended that the payment of contributions should not extend to an individual’s whole active career, but to a shorter period (e.g., 30 or 35 years). Of course, it is also possible to generate a good estimate of the ratio of taxes paid during the course of a whole career based on a shorter, although sufficiently long, period, but it is expedient to choose as long a period as possible, because the approach will be all the more correct.
• People could easily confuse this acquisition of rights with the acquisition of rights that occurs within the current PAYG system, and it would be difficult to explain to them that in this case we are talking about something completely different.

Particularly in view of the latter argument, we recommend an estimation based on the amount of personal income tax paid, data which are also readily available with respect to everyone.

Based on the above, we arrive at a logical rule for the distribution of community points—every year, the community points for that year must be distributed among active workers who have paid personal income tax for that year, in proportion to their tax payments.

6. Interaction between the System’s Elements

In order for the above elements to interact well, they must be configured well with respect to each other. For instance, it must be determined when contributions or savings onto individual accounts should be paid, and how the HC system and FF subsystem should be linked to each other.

6.1. Normal Retirement Age

In view of the fact that we have recommended that the FF and HC subsystems should not be parallel, but consecutive, two retirement ages are required, as well as an age limit for the payment of contributions, all of which are interrelated. The centrally important age limit is “the” normal age of retirement, to which the other two may be associated. The normal age of retirement is the age limit for people who have raised “enough” children. What counts as enough may partly be determined based on the GBE but may in part be determined freely. The more children we include, the higher the starting pension, and vice versa. It is expedient to determine this in such a way that its level corresponds roughly to what enables the achievement of the current average pension. This requires a certain amount of calculation, and for the sake of simplicity we shall hereafter assume that this is one child per head, or two per married couple. We might also express this by stating that the normal age of retirement covers people whose pension points, based on their individual contribution, reaches 1.

Important to note, that in the above, for practical reasons and temporarily, we have already put forward a proposal with respect to the fact that this logic should be reversed and the current average pension should be set to a level that corresponds to the raising of one child (two per couple). However, the “correct” logic, which should be gradually introduced later, is the one described above.

The normal age of retirement is, as we know, the age at which the expected remaining lifetime is a previously set value. We recommend that this value should be 15 years (which in Hungary today means an age of retirement of a little over 65 years—based on Table 1, in 2017 this was somewhere around 67.5 years).

6.2. Increased Retirement Age

We have proposed that people who do not raise children should have a higher age of retirement. It should be higher to an extent that ensures that their pension level is similar to that of people who have acquired at least one pension point. This may be achieved by setting the “increased retirement age” to the age at which the last part of the expected remaining lifetime begins in the case of a normal age of retirement. This may be calculated based on c and the ratio of all taxpayers to taxpayers with children. This therefore applies to people who have a zero number of individual pension points. A weighted average of the two retirement ages would apply to people with a transitional number of pension points. Based on

Table 3. Life expectancy (based on the 2017 Hungarian unisex mortality table) and what percentage of the life expectancy (LE) at age 67 exceeds the given age.

Age (x)	LE	% of Expected Remaining Lifetime	Age (x)	LE	% of Expected Remaining Lifetime	Age (x)	LE	% of Expected Remaining Lifetime
67	15.31	100%	75	10.34	54%	83	6.24	20.4%
68	14.67	94%	76	9.77	49%	84	5.85	17.3%
69	14.04	87%	77	9.19	44%	85	5.44	14.6%
70	13.41	81%	78	8.65	40%	86	5.09	12.1%
71	12.79	76%	79	8.12	35%	87	4.76	9.9%
72	12.15	70%	80	7.62	31%	88	4.48	8.0%
73	11.54	64%	81	7.12	27.3%	89	4.21	6.4%
74	10.94	59%	82	6.67	23.7%	90	3.94	5.0%

Source: mortality.org + own calculations.

, this increased retirement age would be somewhere around the age of 82, if this ratio were 0.34 × 0.7 = 0.24, for instance. If the state increases this to for instance 0.5 via benefits for people with children, then the age limit would be 76.

Nota bene, that no one should be fooled by the fact that 82 does no split the 15 years following the age of 67 to a ratio of 76:24, because an expected remaining lifetime at the age of 67 does not mean that we will live for another 15 years. Many people will live for much longer.

In the Table 3 the calculation was performed using a period life table because in Hungary, similarly to most countries, it is the only one available. In future, however, if age limit indexing is introduced, it would be expedient to switch to using the cohort life table (or the “projected” mortality rate table, as I referred to it in Banyár (2016b)), i.e., we should not start out from a mortality “snapshot” but use the remaining life expectancy of the given cohort, and of course regularly prepare the related mortality tables. For the differences between the two tables see Ayuso et al. (2021).

Naturally, people who go into retirement later for whatever reason receive a proportionately higher pension. This may be achieved by recalculating their pension points. It may be stated that pension points (whether individual or community) apply to the normal retirement age. In the case of people who retire later (or later than the age of retirement that is valid in their particular case), their pension points must be multiplied by the amount by which the expected remaining lifetime at the normal age of retirement exceeds the expected remaining lifetime at the time when the individual actually goes into retirement.

6.3. The Age Limit for the Commencement of Contribution Payments

Having determined a predetermined period of contribution payments that is shorter than the active career is justified, it is not absolutely clear when the payment of contributions should actually begin. There are two clear options—it should begin immediately, together with the active career (as is currently the case within the PAYG system); or it should begin later and last until the normal age of retirement. There is no justification for the payment of contributions ending later than the normal retirement age, because in principle everyone can retire at that time—although some will begin depleting their pension savings. At this time, the age limit for the start of contribution payments must be reverse-calculated based on this. All other cases fall somewhere between these two.

From among the possibilities, such an intermediate case is most justified in which the end of the contribution payment period falls close to the normal retirement age. The reasons are as follows.

• In most cases, salaries are generally higher in the second half of the career than they are in the first half, meaning people can in part pay a higher level of contributions, and in part will find it easier to live without those missing resources. We might state that at this time the human capital investment realized by the parents (and society) begins to bear fruit.
• It is expedient not to also burden the first phase of an active working career with additional contribution payments, since this is usually the period during which the majority of people undertake to have children, which is already a major expense in itself. Accordingly, the system also encourages the investment in human capital by exempting young people from having to pay other expenses to some extent during a period that is most logical for this purpose. By doing so, it otherwise also shepherds them towards starting a family relatively (compared to today’s high ages) early.
• It is however expedient not to “push” the contribution payment period fully onto the normal age of retirement, but instead to leave a certain “margin”—5 years, for instance—in view of the fact that there may be deferments to contribution payments, when the individual suspends them.

In summary, the recommended age limit for the commencement of contribution payments = normal retirement age − contribution payment period (e.g., 30 years) − contribution payment margin (e.g., 5 years). Accordingly, this age limit will be indexed together with the normal retirement age. In the above example, if the age of retirement is 67, then the age limit for the commencement of contribution payments is 32.

6.4. Making Raising Children and Savings Financially Independent

In view of the fact that within the HC system raising children would continue to be a voluntary decision, people who do not undertake to do so would not receive a pension from the state system. It is, however, expedient for the state to also facilitate the pensions of these people, meaning it should operate a fully funded defined contribution (DC) system for them. The accumulation of savings within this system cannot, however, occur at the last moment when, possibly in contrast to original plans, it transpires that someone will not be having children after all. On the contrary, it is worth departing from the assumption that someone will not have a child, and then make continuous amendments if it transpires that they will be having one.

Accordingly, savings for pension purposes (payment into the FF system) should begin at the earliest opportunity, at the beginning of the active working career. Based on the above, everyone additionally has a good opportunity to do so at this time, since they will not yet have to pay pension contributions and, in view of the relatively long savings period, will be able to accumulate a large sum of capital until retirement with relatively small payments.

However, an individual will have less need for these savings if they have a child, and no need at all if a second child is born. For this reason, we recommend that the mandatory level of savings (the sum deducted from salaries) should be reduced by half on the birth of the first child and cease to be mandatory following the birth of the second child (assuming married couples). If someone wishes to, they may of course continue to accumulate savings even after the birth of their second child.

These elements—the timing of savings and contribution payments—and the above rules for their mutual interaction achieve the following effects:

• The fact that the payment of contributions begins later than the active career also means that we leave young people with a stage of life in which they do not need to pay contributions and can instead deal with more important things, e.g., raising children. This means that this solution on the one hand shepherds young people towards having children, and on the other hand makes the initial years of this easier for them (providing they get down to the “project” in a timely manner).
• If they do not have children, then they may put aside the money they save by not doing so towards their pension, since in this case their state pension will only begin 13–15 years later than everyone else’s.
• However, if they do have a child after all, then they will not have to bother with these savings, because money will inherently become available for the “project”.
• As a result, the issue of whether they accumulate savings or decide to have children will be financially neutral to them, which would be a major step forward compared to the current situation, in which undertaking to have children clearly means a financial step backwards.

6.5. The Functioning of Annuities within the FF System

At the beginning of the normal retirement age, the money accumulated within a state FF system set up in this manner would not begin to be disbursed in the form of a life annuity but would simply be distributed evenly over the number of months remaining until the commencement of state pension services (e.g., 13 years × 12 months = 156 equal parts). The pensioner will receive this sum each month, plus the interest accumulated in the meantime, unless they decide to continue working past the age of retirement and do not wish to withdraw the money. This sum (or rather the sum not spent by its owner) will become inheritable in such a manner that the default heir will be the spouse/registered partner, who by default will also be able to spend it on their pension.

However, it is by no means certain that the capital accumulated within the FF system will be paid out with respect to a pension or inheritance, because it is recommended that these savings are given a kind of guaranteeing role. If, for instance, someone has not fully repaid the costs of having been raised (meaning they have not paid contributions for a long enough period), then that sum may also be deducted from the money that has accumulated within the FF system.

7. Transition from Current System to New System

The above system may be introduced without problems for young people who are just developing an individual pension structure and are able to adapt to any system. It is, however, problematic to introduce it with respect to people who have lived a significant part of their lives adapting to a totally different pension philosophy.

Because of this, it may be expedient to introduce the system in such a manner that we make the keeping of an individual pension savings account mandatory for people under a certain age, e.g., 55 (while people over this age limit will continue using the current pension system) who are raising fewer than two children, and then continuously increase the age of retirement in their case (which will become the increased retirement age within the final system), at which time they will be able to go into retirement compared to people with two children, in such a way that they also receive a pension from the HC system. One possibility could be a one-year increase every two years (in addition to normal retirement age indexing), until the increased retirement age reaches the theoretical value. This also means that during the transitional phase people have 2 years available to make up for their one year of missing the pension out of their own savings. People who are scheduled to retire in 2 years’ time will only have one year accumulated but will only have to make up for one year, etc., meaning everyone would be able to adapt gradually to the new system.

A system of this kind cannot be introduced without a high level of social and political support. It must be agreed with not only by the current governing authorities, but also by the government which will probably replace it in the future. However, a political accord of this nature can only be rooted in a broad acceptance on the part of those affected (i.e., active voters under a given age), the first step of which is that they become aware of the concept at all and understand the principles behind the new system. Therefore, this is the first step in the realization of the HC system, and also the greatest difficulty in relation to its actual implementation—its broad dissemination among the general public.