Appendix A. Determination of Annuity Payouts and Bequests
We first define the following notations:
22| c = Payout Age |
| d = Life-annuity Age |
| e = Number of years payouts funded by RA, i.e., |
| i = Annual effective interest rate |
| v = Discount factor = 1/(1+i) |
| = Bequest payable at age g |
| = First installment from RA to LIF at age 55 |
| = Second installment from RA to LIF at Payout Age |
| = Monthly curtate future lifetime random variable at age g |
| = Monthly payout estimate at age 55 |
| P = Monthly payout |
| = Balance of RA at age g |
| = Aggregate amount of payouts from LIF up to age g |
We adopt the actuarial framework in [
3] to determine the monthly payouts of the Unified Plan based on the actuarial equivalence principle. The key input variables are the return of the Life Income Fund and the mortality rates. The fund return rate is specified by the government as the plan sponsor. While various mortality tables are used in [
3], we adopt the U.S. MP2014 table in this paper. For sensitivity of the results with respect to mortality assumption, see [
3].
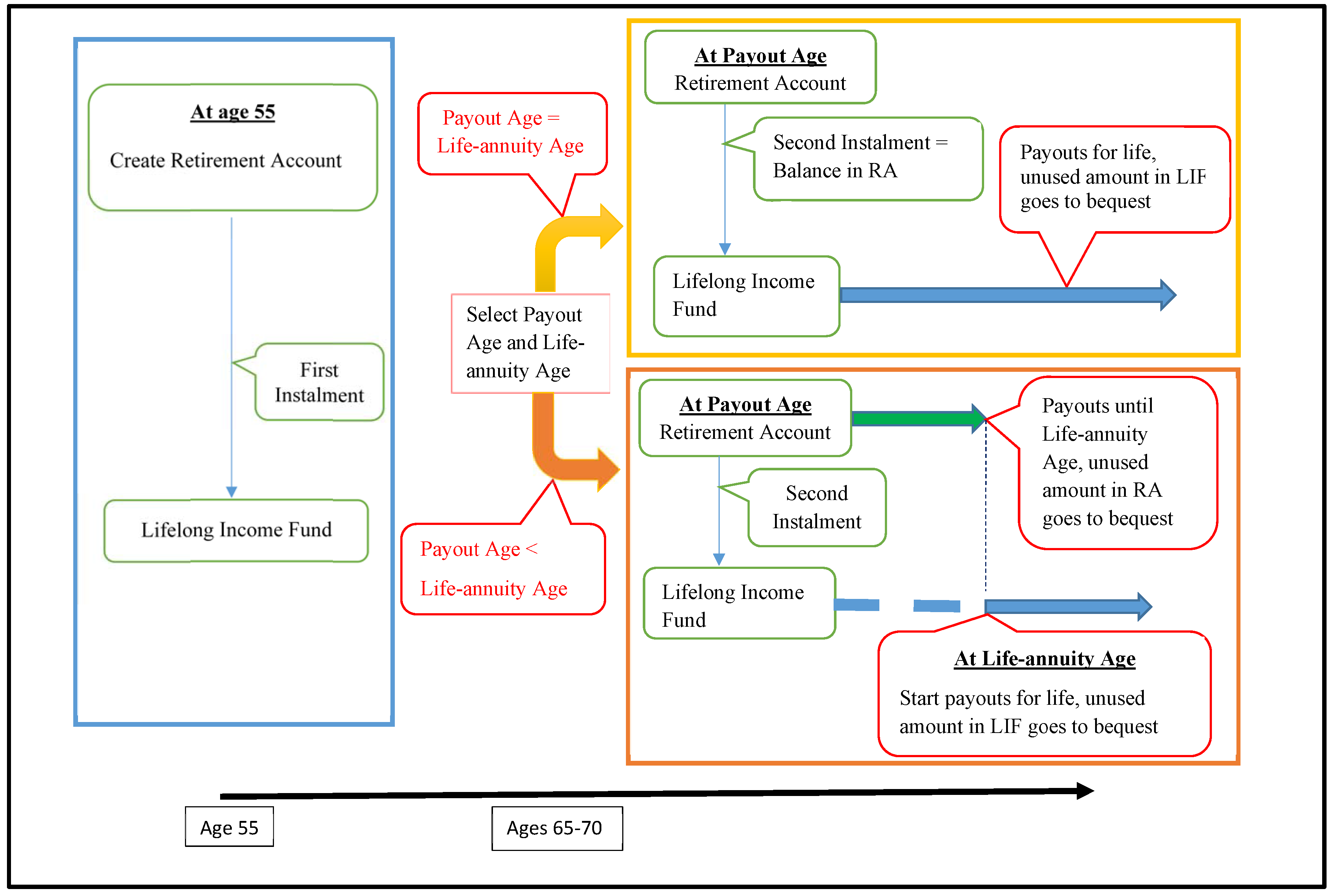
Under the Unified Plan, the cash outflows consist of the monthly payouts P starting at the Payout Age c until death at age g, with possible bequest payable upon death. If c is less than the Life-annuity Age d, the payouts are funded by the RA from age c to age d and then by the LIF from age d until death. The minimum value of c is the Payout Eligibility Age (currently set to be 65), and it cannot exceed 70. In contrast, the value of d may be from c to 90. If , the annuity payouts are entirely funded by the LIF for life and the configuration is similar to the Standard Plan.
As per the plan design, if a member dies at age g before reaching age c, the bequest consists of the balance of his RA and his first installment to the LIF, i.e., , for . If a member lives to start receiving monthly payouts and chooses , a second installment , which is the balance in his RA, is transferred to the LIF. Thus, his RA is depleted and the monthly payouts are funded by the LIF. If he dies after age c, the bequest is the difference between the sum of two installments and the aggregate amount of payouts from the LIF , i.e., for .
If his choice is
,
to the LIF is only part of the RA balance, as the rest is used to fund the annuity-certain up to age
d. If he dies between ages
c and
d, the bequest to his beneficiaries is the sum of two installments plus the balance of RA, i.e.,
for
; otherwise, the bequest is the difference between the summation of two installments and the aggregate amount of payouts from the LIF. In summary, we can write the bequest
as
for
and
for
.
We now determine the amount
to be transferred from the RA to the LIF at age 55, and the initial estimate of the monthly payout
. As the age-parameter configuration is determined at age
, our strategy is to determine the value of
so that no transfer from the LIF to the RA is required should other age parameters be chosen later. To this effect, we adopt the minimum value of
c and the maximum value of
d for the determination of
, i.e.,
and
.
23 Under this configuration, the present value of the future loss of the LIF at age 55, denoted by
, is given by
where
for
.
Let
be the term (in years) of the monthly decreasing term insurance benefit covered by
after age 90. Invoking the equivalence principle that the actuarial present value of cash inflows equals the actuarial present value of cash outflows,
,
and
can be obtained simultaneously by solving a system of equations and inequalities, so that
subject to
and the annuity-certain constraint
.
24At age 65, if the member really chooses the age-parameter configuration of and , and no new money is contributed to the RA, the monthly payout is . In other cases, we have to re-calculate the monthly payouts.
If the member chooses the configurations of , the RA is closed after is transferred from the RA to the LIF. The payouts are then funded by the LIF for life. Under the configuration of , the monthly payouts are funded from two sources. From ages c to d, the payouts are paid from the RA as an annuity-certain and from age d onward they are paid from the LIF as a life-annuity. However, the payouts have to be level throughout the two periods so that members receive a stable monthly income. To meet this condition, and P have to be determined jointly according to actuarial principles.
Note that
can be regarded as the cost of purchasing two insurance products at age 55: a
-year term insurance with death benefit
and a
-year pure endowment insurance policy with survival benefit
, where
can be determined by solving the following equation
Thus, if the CPF member survives to age c, the balance in the LIF is actuarially equivalent to the survival benefit of a pure endowment insurance policy.
Let
be the present value of the future loss of the LIF at age
c and
be the term of the monthly decreasing term insurance covered by LIF at age
d. The variable
is then given by:
where
and
for
. Based on the equivalence principle,
P,
, and
can be evaluated by solving
subject to
and
where
is the balance in the RA at age
c, inclusive of any new money amount.
Finally, we note that if , is the RA balance and P can be calculated directly after solving for . If , however, and P have to be determined jointly by iteration.
Appendix B. Determination of LIF Components
As discussed in
Section 2, the annuity fund LIF is a notional account directly funding the life-annuity payouts and possible bequests, resulting in simplicity in analyzing the benefits and costs provided by the LIF at different stages of the plan under different plan parameter configurations.
The Unified Plan can be divided into three stages. Stage 1 is from ages 55 to
c, Stage 2 is from ages
c to
d and Stage 3 is from age
d to death. If
, Stages 2 and 3 are collapsed into a single stage. As discussed in
Appendix A,
transferred from the RA to the LIF at age 55 is the cost to cover a term insurance and a pure endowment insurance with maturity at age
c. If the member lives to age
c, the LIF balance is equivalent to the survival benefit
of a pure endowment insurance. Otherwise, the bequest payable is the sum of the death benefit
from the LIF and the balance in the RA at the time of death.
If
in Stage 2, the amount
at age
c is used to cover the costs of two insurance products: an
e-year term insurance with death benefit
and an
e-year pure endowment insurance with survival benefit
. Hence,
from which we can solve for
. If the member lives to age
d, the LIF balance is equivalent to the survival benefit
of the pure endowment insurance. Otherwise, the amount of bequest payable is the sum of
from the LIF and the balance in the RA at the time of death. From age
d onward (Stage 3), the reserve
at age
d is used to fund two insurance products: a life-annuity with monthly payments
P and a decreasing
-year term insurance with bequest
for
and zero for
.
However, if
the amount
is used to fund the life-annuity and decreasing term insurance. In summary, we have the following result: