Adding Shocks to a Prospective Mortality Model
Abstract
1. Introduction
2. Proposed Stochastic Mortality Model
2.1. Specification
2.2. Log-Likelihood Determination
2.3. Parameter Estimation
2.4. Calculating Prospective Residual Life Expectancies
3. Numerical Application
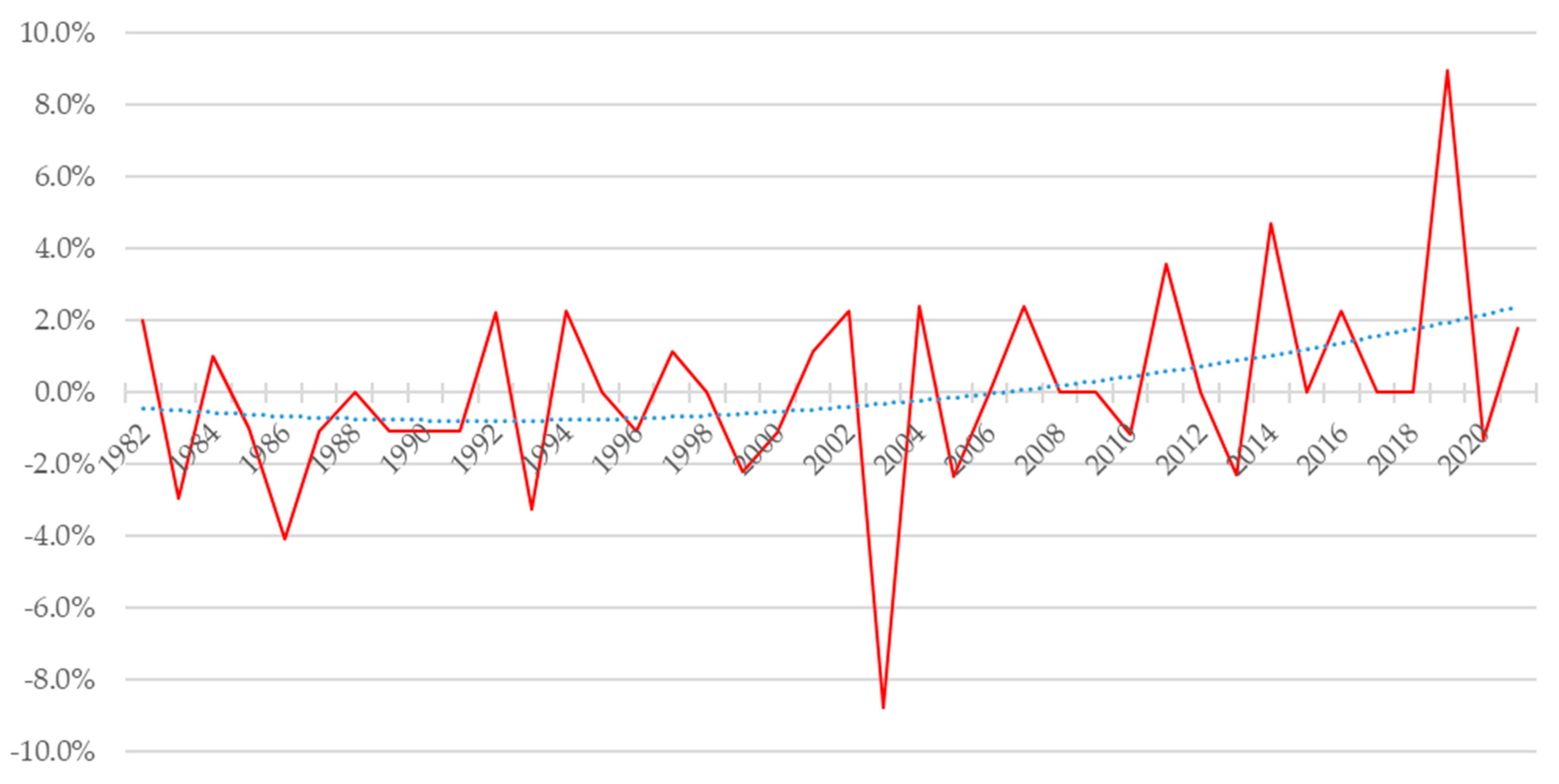
3.1. Model Adjustment
3.1.1. Estimation of Gamma Distribution Parameters
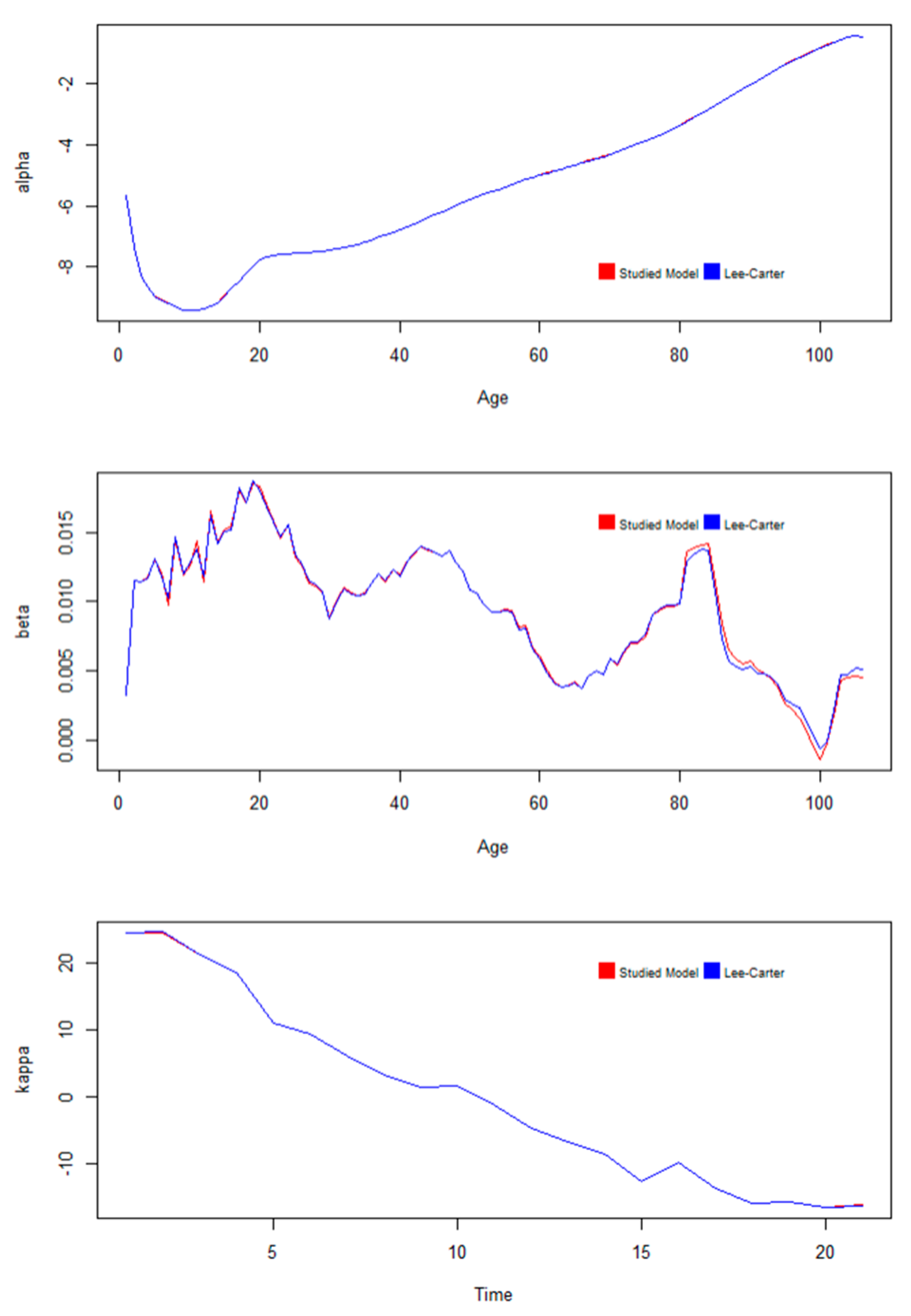
3.1.2. Estimation of Model Parameters
3.1.3. Extrapolation of Time Coefficients
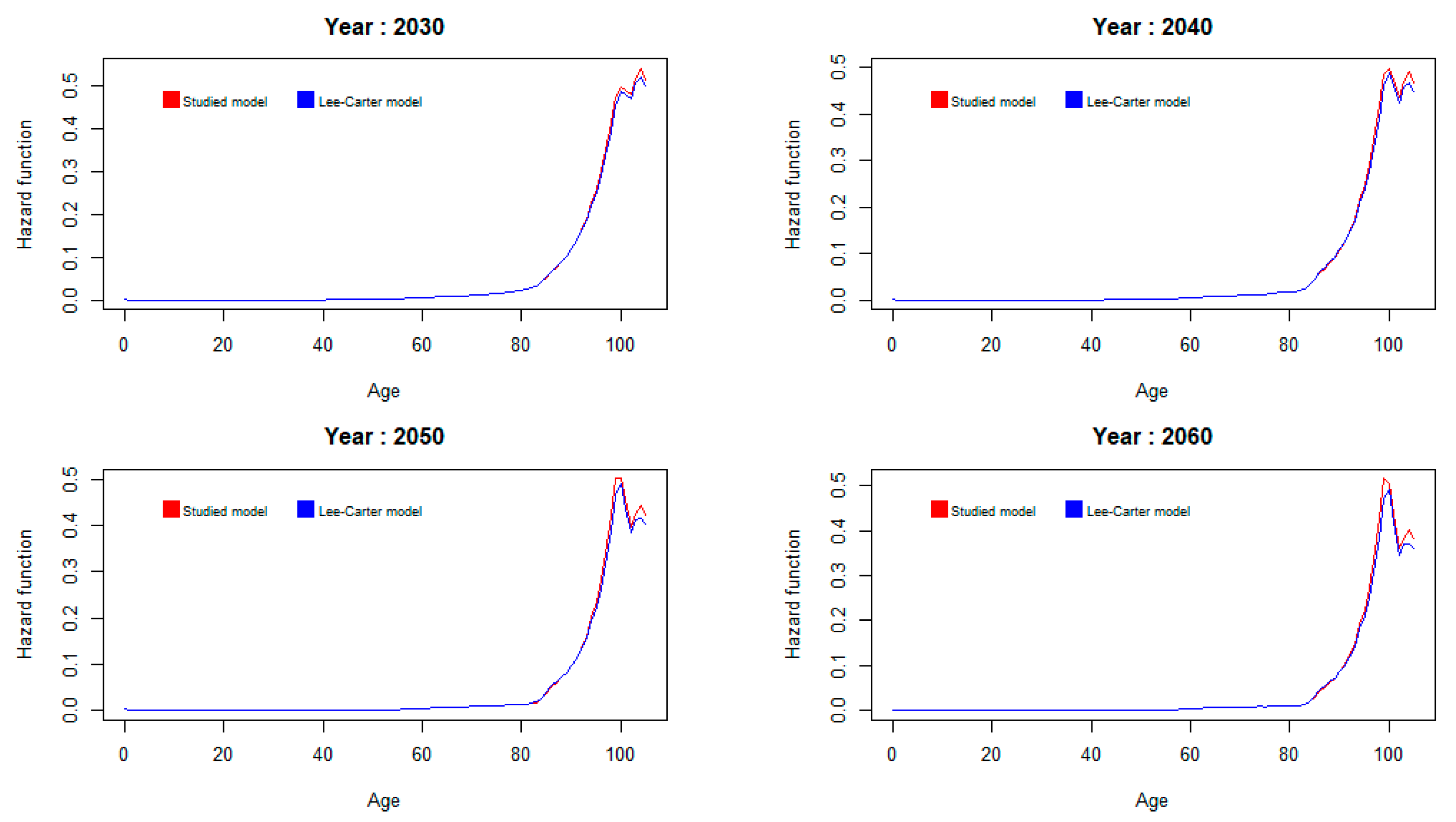
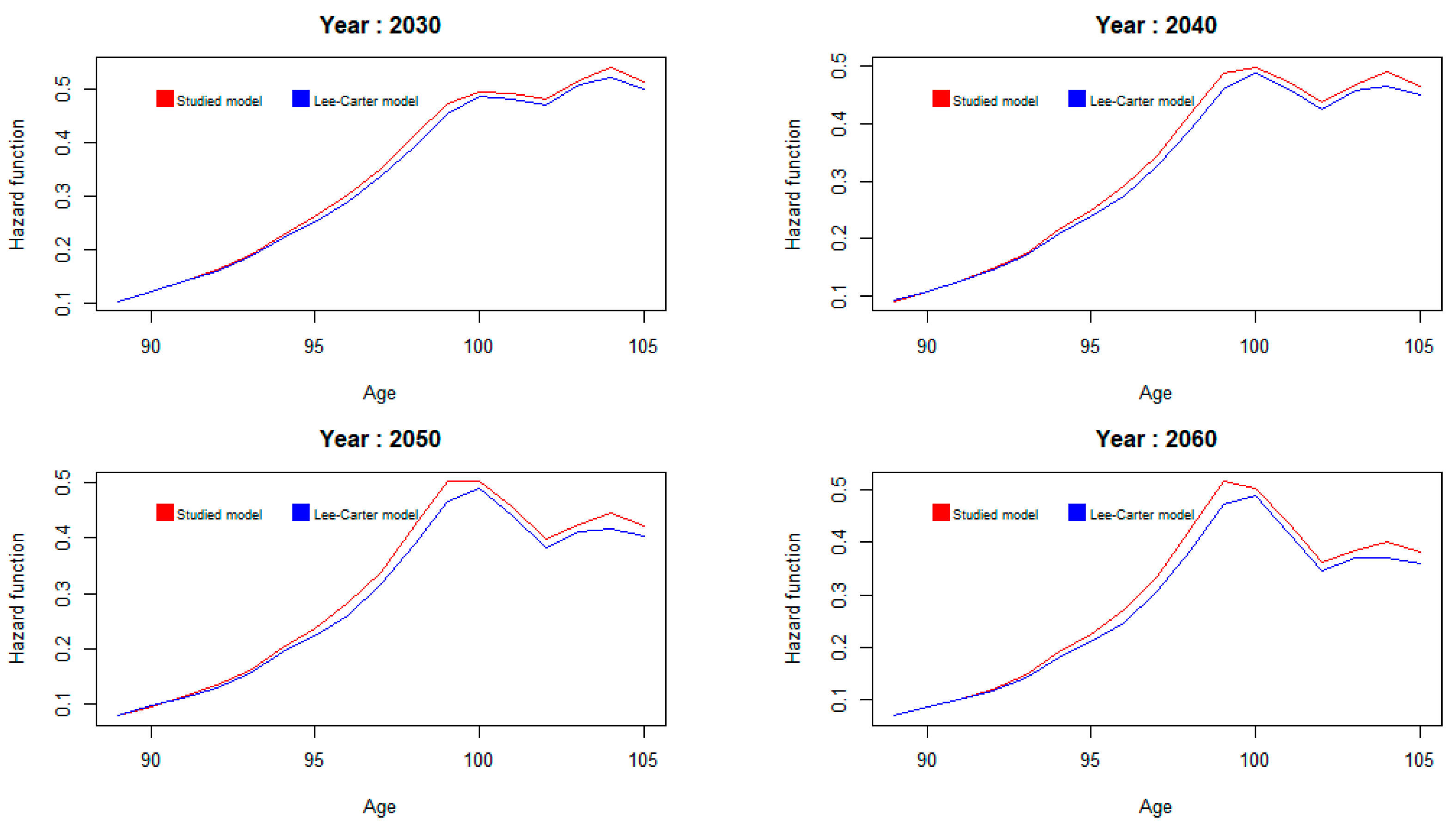
3.2. Projected Mortality Forces
3.3. Estimating Prospective Residual Life Expectancies
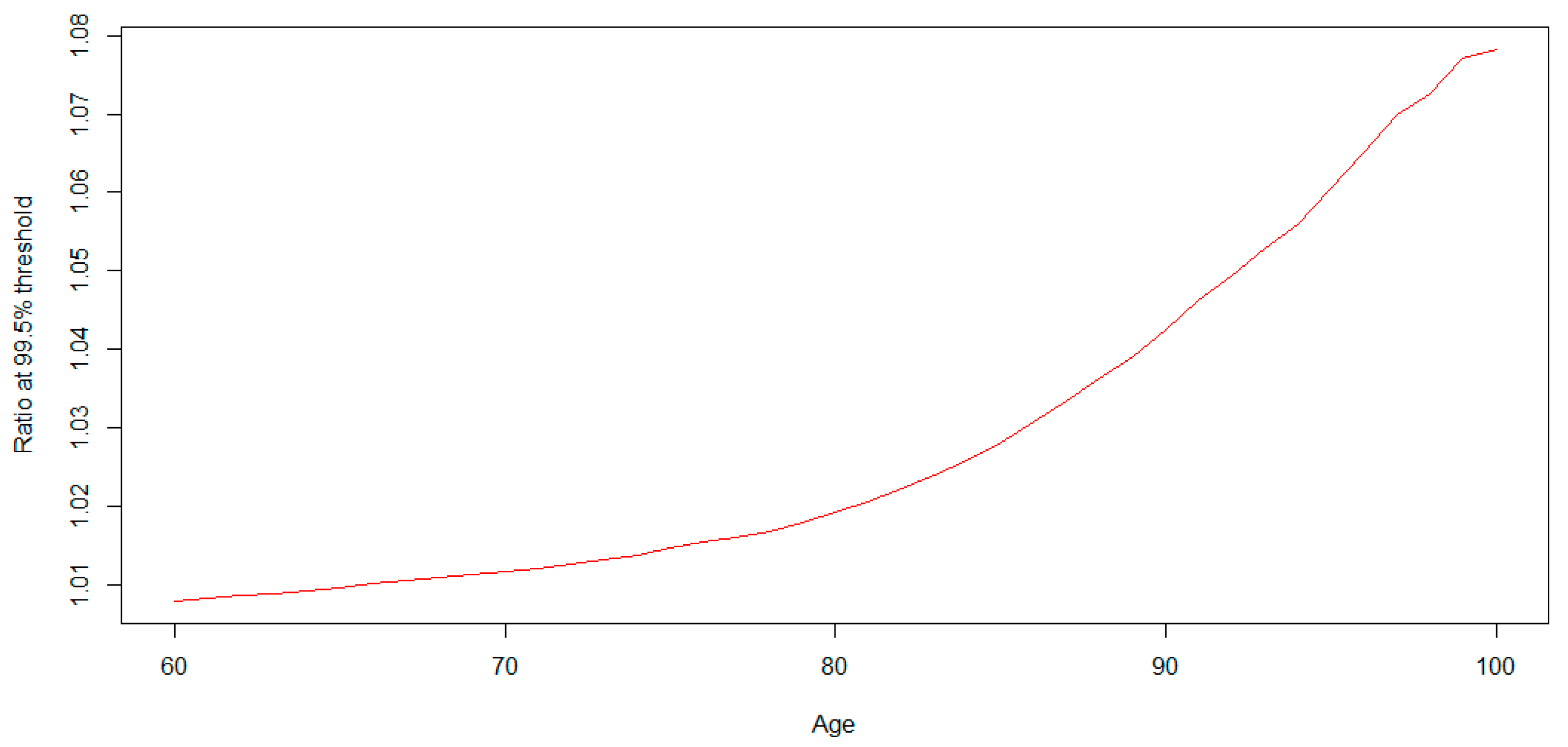
3.4. Sensitivity to Frailty Parameter
- -
- the severity of the COVID-19 pandemic remains below the Solvency 2 bicentennial event. It is associated with a 10-fold higher probability of occurrence.
- -
- the calibration of frailty with a volatility of 5.5% is consistent with that of the Solvency 2 standard formula for mortality risk.
3.5. Consequences for the Capital Requirement of an Annuity Plan
4. Conclusions and Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
| Alpha | ||||||||
|---|---|---|---|---|---|---|---|---|
| Age | Model Studied | LC Reference Model | Age | Model Studied | LC Reference Model | Age | Model Studied | LC Reference Model |
| 0 | −5.6542 | −5.6553 | 36 | −7.0173 | −7.0186 | 72 | −4.0422 | −4.0456 |
| 1 | −7.3447 | −7.3457 | 37 | −6.9390 | −6.9396 | 73 | −3.9592 | −3.9625 |
| 2 | −8.2790 | −8.2804 | 38 | −6.8474 | −6.8480 | 74 | −3.8668 | −3.8713 |
| 3 | −8.6679 | −8.6695 | 39 | −6.7630 | −6.7633 | 75 | −3.7867 | −3.7882 |
| 4 | −8.9672 | −8.9721 | 40 | −6.6729 | −6.6733 | 76 | −3.6851 | −3.6862 |
| 5 | −9.0765 | −9.0803 | 41 | −6.5862 | −6.5867 | 77 | −3.5790 | −3.5803 |
| 6 | −9.1958 | −9.2028 | 42 | −6.4878 | −6.4880 | 78 | −3.4774 | −3.4791 |
| 7 | −9.2892 | −9.2924 | 43 | −6.3872 | −6.3877 | 79 | −3.3674 | −3.3679 |
| 8 | −9.4087 | −9.4124 | 44 | −6.2849 | −6.2854 | 80 | −3.2163 | −3.2216 |
| 9 | −9.3935 | −9.4009 | 45 | −6.1800 | −6.1803 | 81 | −3.0838 | −3.0881 |
| 10 | −9.4223 | −9.4329 | 46 | −6.0917 | −6.0922 | 82 | −2.9510 | −2.9556 |
| 11 | −9.3577 | −9.3662 | 47 | −5.9813 | −5.9817 | 83 | −2.8178 | −2.8236 |
| 12 | −9.2768 | −9.2809 | 48 | −5.8840 | −5.8845 | 84 | −2.6937 | −2.7014 |
| 13 | −9.1674 | −9.1742 | 49 | −5.7947 | −5.7952 | 85 | −2.5725 | −2.5850 |
| 14 | −8.9411 | −8.9443 | 50 | −5.7068 | −5.7077 | 86 | −2.4381 | −2.4498 |
| 15 | −8.6907 | −8.6949 | 51 | −5.6157 | −5.6166 | 87 | −2.3015 | −2.3126 |
| 16 | −8.4690 | −8.4721 | 52 | −5.5399 | −5.5411 | 88 | −2.1619 | −2.1733 |
| 17 | −8.2022 | −8.2047 | 53 | −5.4596 | −5.4605 | 89 | −2.0266 | −2.0390 |
| 18 | −7.9805 | −7.9814 | 54 | −5.3712 | −5.3722 | 90 | −1.8871 | −1.9001 |
| 19 | −7.7424 | −7.7441 | 55 | −5.2853 | −5.2866 | 91 | −1.7522 | −1.7651 |
| 20 | −7.6642 | −7.6654 | 56 | −5.2062 | −5.2078 | 92 | −1.6216 | −1.6351 |
| 21 | −7.6042 | −7.6051 | 57 | −5.1270 | −5.1289 | 93 | −1.4936 | −1.5072 |
| 22 | −7.5935 | −7.5952 | 58 | −5.0598 | −5.0611 | 94 | −1.3657 | −1.3777 |
| 23 | −7.5824 | −7.5837 | 59 | −4.9902 | −4.9925 | 95 | −1.2453 | −1.2594 |
| 24 | −7.5520 | −7.5540 | 60 | −4.9156 | −4.9178 | 96 | −1.1239 | −1.1367 |
| 25 | −7.5513 | −7.5531 | 61 | −4.8551 | −4.8570 | 97 | −1.0154 | −1.0262 |
| 26 | −7.5195 | −7.5211 | 62 | −4.7853 | −4.7877 | 98 | −0.9056 | −0.9177 |
| 27 | −7.4982 | −7.4995 | 63 | −4.7165 | −4.7182 | 99 | −0.8059 | −0.8166 |
| 28 | −7.4795 | −7.4816 | 64 | −4.6552 | −4.6571 | 100 | −0.7093 | −0.7208 |
| 29 | −7.4393 | −7.4409 | 65 | −4.5875 | −4.5894 | 101 | −0.6286 | −0.6345 |
| 30 | −7.3880 | −7.3899 | 66 | −4.5141 | −4.5163 | 102 | −0.5389 | −0.5481 |
| 31 | −7.3594 | −7.3607 | 67 | −4.4558 | −4.4580 | 103 | −0.4581 | −0.4679 |
| 32 | −7.3008 | −7.3012 | 68 | −4.3774 | −4.3802 | 104 | −0.4095 | −0.4185 |
| 33 | −7.2536 | −7.2544 | 69 | −4.2982 | −4.3005 | 105 | −0.4625 | −0.4652 |
| 34 | −7.1764 | −7.1771 | 70 | −4.2170 | −4.2209 | |||
| 35 | −7.1106 | −7.1110 | 71 | −4.1381 | −4.1415 | |||
| Beta | ||||||||
|---|---|---|---|---|---|---|---|---|
| Age | Model Studied | LC Reference Model | Age | Model Studied | LC Reference Model | Age | Model Studied | LC Reference Model |
| 0 | 0.0033 | 0.0033 | 36 | 0.0120 | 0.0120 | 72 | 0.0070 | 0.0071 |
| 1 | 0.0115 | 0.0115 | 37 | 0.0115 | 0.0115 | 73 | 0.0070 | 0.0071 |
| 2 | 0.0114 | 0.0114 | 38 | 0.0123 | 0.0123 | 74 | 0.0075 | 0.0077 |
| 3 | 0.0118 | 0.0117 | 39 | 0.0119 | 0.0118 | 75 | 0.0090 | 0.0091 |
| 4 | 0.0130 | 0.0131 | 40 | 0.0129 | 0.0129 | 76 | 0.0094 | 0.0094 |
| 5 | 0.0121 | 0.0118 | 41 | 0.0134 | 0.0135 | 77 | 0.0097 | 0.0097 |
| 6 | 0.0097 | 0.0102 | 42 | 0.0140 | 0.0140 | 78 | 0.0096 | 0.0097 |
| 7 | 0.0145 | 0.0146 | 43 | 0.0137 | 0.0137 | 79 | 0.0099 | 0.0098 |
| 8 | 0.0119 | 0.0121 | 44 | 0.0135 | 0.0136 | 80 | 0.0136 | 0.0129 |
| 9 | 0.0126 | 0.0127 | 45 | 0.0132 | 0.0133 | 81 | 0.0139 | 0.0134 |
| 10 | 0.0144 | 0.0138 | 46 | 0.0136 | 0.0137 | 82 | 0.0141 | 0.0137 |
| 11 | 0.0114 | 0.0118 | 47 | 0.0128 | 0.0127 | 83 | 0.0142 | 0.0137 |
| 12 | 0.0165 | 0.0162 | 48 | 0.0122 | 0.0122 | 84 | 0.0115 | 0.0107 |
| 13 | 0.0143 | 0.0142 | 49 | 0.0108 | 0.0108 | 85 | 0.0083 | 0.0073 |
| 14 | 0.0151 | 0.0151 | 50 | 0.0106 | 0.0106 | 86 | 0.0065 | 0.0057 |
| 15 | 0.0155 | 0.0151 | 51 | 0.0099 | 0.0099 | 87 | 0.0059 | 0.0054 |
| 16 | 0.0180 | 0.0182 | 52 | 0.0093 | 0.0093 | 88 | 0.0056 | 0.0052 |
| 17 | 0.0171 | 0.0171 | 53 | 0.0093 | 0.0093 | 89 | 0.0057 | 0.0054 |
| 18 | 0.0185 | 0.0187 | 54 | 0.0095 | 0.0094 | 90 | 0.0051 | 0.0049 |
| 19 | 0.0183 | 0.0180 | 55 | 0.0093 | 0.0092 | 91 | 0.0049 | 0.0048 |
| 20 | 0.0170 | 0.0169 | 56 | 0.0082 | 0.0080 | 92 | 0.0044 | 0.0046 |
| 21 | 0.0158 | 0.0158 | 57 | 0.0082 | 0.0081 | 93 | 0.0039 | 0.0041 |
| 22 | 0.0146 | 0.0146 | 58 | 0.0067 | 0.0066 | 94 | 0.0026 | 0.0030 |
| 23 | 0.0156 | 0.0156 | 59 | 0.0061 | 0.0059 | 95 | 0.0023 | 0.0027 |
| 24 | 0.0132 | 0.0134 | 60 | 0.0050 | 0.0049 | 96 | 0.0016 | 0.0024 |
| 25 | 0.0126 | 0.0127 | 61 | 0.0042 | 0.0041 | 97 | 0.0008 | 0.0014 |
| 26 | 0.0113 | 0.0115 | 62 | 0.0039 | 0.0038 | 98 | −0.0005 | 0.0004 |
| 27 | 0.0111 | 0.0112 | 63 | 0.0040 | 0.0039 | 99 | −0.0013 | −0.0006 |
| 28 | 0.0107 | 0.0108 | 64 | 0.0042 | 0.0042 | 100 | −0.0002 | −0.0001 |
| 29 | 0.0088 | 0.0088 | 65 | 0.0038 | 0.0038 | 101 | 0.0018 | 0.0022 |
| 30 | 0.0103 | 0.0102 | 66 | 0.0046 | 0.0046 | 102 | 0.0044 | 0.0047 |
| 31 | 0.0111 | 0.0110 | 67 | 0.0050 | 0.0051 | 103 | 0.0046 | 0.0048 |
| 32 | 0.0107 | 0.0106 | 68 | 0.0047 | 0.0047 | 104 | 0.0046 | 0.0053 |
| 33 | 0.0105 | 0.0104 | 69 | 0.0059 | 0.0059 | 105 | 0.0046 | 0.0051 |
| 34 | 0.0106 | 0.0105 | 70 | 0.0055 | 0.0055 | |||
| 35 | 0.0114 | 0.0113 | 71 | 0.0063 | 0.0064 | |||
| Kappa | |||||
|---|---|---|---|---|---|
| Age | Model Studied | LC Reference Model | Age | Model Studied | LC Reference Model |
| 2000 | 24.4565 | 24.4761 | 2030 | −43.8008 | −43.8043 |
| 2001 | 24.4627 | 24.5265 | 2031 | −45.9908 | −45.9945 |
| 2002 | 21.2073 | 21.2070 | 2032 | −48.1809 | −48.1847 |
| 2003 | 18.4710 | 18.4083 | 2033 | −50.3709 | −50.3750 |
| 2004 | 10.9651 | 10.9513 | 2034 | −52.5610 | −52.5652 |
| 2005 | 9.4399 | 9.4231 | 2035 | −54.7510 | −54.7554 |
| 2006 | 6.0386 | 6.0366 | 2036 | −56.9410 | −56.9456 |
| 2007 | 3.1599 | 3.1578 | 2037 | −59.1311 | −59.1358 |
| 2008 | 1.4649 | 1.4631 | 2038 | −61.3211 | −61.3260 |
| 2009 | 1.6981 | 1.6984 | 2039 | −63.5112 | −63.5163 |
| 2010 | −1.1863 | −1.1865 | 2040 | −65.7012 | −65.7065 |
| 2011 | −4.7123 | −4.7140 | 2041 | −67.8912 | −67.8967 |
| 2012 | −6.6593 | −6.6691 | 2042 | −70.0813 | −70.0869 |
| 2013 | −8.5651 | −8.5639 | 2043 | −72.2713 | −72.2771 |
| 2014 | −12.6304 | −12.6243 | 2044 | −74.4614 | −74.4673 |
| 2015 | −9.8526 | −9.8338 | 2045 | −76.6514 | −76.6575 |
| 2016 | −13.5803 | −13.5743 | 2046 | −78.8414 | −78.8478 |
| 2017 | −15.8335 | −15.8386 | 2047 | −81.0315 | −81.0380 |
| 2018 | −15.6887 | −15.6650 | 2048 | −83.2215 | −83.2282 |
| 2019 | −16.4993 | −16.4493 | 2049 | −85.4116 | −85.4184 |
| 2020 | −16.1564 | −16.2296 | 2050 | −87.6016 | −87.6086 |
| 2021 | −24.0904 | −24.0924 | 2051 | −89.7916 | −89.7988 |
| 2022 | −26.2805 | −26.2826 | 2052 | −91.9817 | −91.9891 |
| 2023 | −28.4705 | −28.4728 | 2053 | −94.1717 | −94.1793 |
| 2024 | −30.6606 | −30.6630 | 2054 | −96.3618 | −96.3695 |
| 2025 | −32.8506 | −32.8532 | 2055 | −98.5518 | −98.5597 |
| 2026 | −35.0406 | −35.0435 | 2056 | −100.7418 | −100.7499 |
| 2027 | −37.2307 | −37.2337 | 2057 | −102.9319 | −102.9401 |
| 2028 | −39.4207 | −39.4239 | 2058 | −105.1219 | −105.1304 |
| 2029 | −41.6108 | −41.6141 | 2059 | −107.3120 | −107.3206 |
| 2060 | −109.5020 | −109.5108 | |||
| 1 | It should be remembered that Lee and Carter’s initial model is not a probabilistic model, and simply proposes a parsimonious decomposition of interactions between age and year in the structure of mortality rates across a country. |
| 2 | https://cran.r-project.org/web/packages/Rsolnp/index.html (accessed on 31 December 2023). |
| 3 | https://cran.r-project.org/web/packages/demography/index.html (accessed on 31 December 2023). |
| 4 | https://www.ressources-actuarielles.net/C1256F13006585B2/0/39B54166464089AFC12572B0003D88C2/$FILE/20230921_FP.pdf?OpenElement (accessed on 31 December 2023). |
| 5 | https://actudactuaires.typepad.com/laboratoire/2021/03/mortalit%C3%A9-en-france-en-2020-suite.html (accessed on 31 December 2023). |
| 6 | The SCR is the minimum capital required to control the probability of ruin at one year in the sense of the economic balance sheet at the level of 0.5%. |
| 7 | EU Delegated Regulation n°2015/35: https://eur-lex.europa.eu/legal-content/FR/TXT/?uri=CELEX:32015R0035 (accessed on 31 December 2023). |
References
- Agalva, Élisabeth, and Nathalie Blanpain. 2021. Projections de Population 2021–2070. Insee Résultats. Paris: INSEE. [Google Scholar]
- Barbi, Elisabetta. 1999. Eterogeneità Della Popolazione e Sopravvivenza Umana: Prospettive Metodologiche ed Applicazioni alle Generazioni Italiane 1870–1895. Ph.D. thesis, Dipartimento Statistico, Università degli Studi di Firenze, Florence, Italy; 91p. [Google Scholar]
- Barbi, Elisabetta, Graciella Casellic, and Jacques Vallin. 2003. Hétérogénéité des générations et âge extrême de la vie. Population 58: 45–68. [Google Scholar] [CrossRef]
- Bongaarts, John. 2004. Long-range trends in adults mortality: Models and projection methods. Demography 42: 23–49. [Google Scholar] [CrossRef] [PubMed]
- Brouhns, Natacha, Michael Denuit, and Jeroen K. Vermunt. 2002. A Poisson log-bilinear regression approach to the construction of projected lifetables. Insurance, Mathematic and Economics 31: 373–93. [Google Scholar] [CrossRef]
- Carannante, Maria, Valeria D’Amato, Steven Haberma, and Massimilliano Menzietti. 2023. Frailty-based Lee–Carter family of stochastic mortality models. Quality and Quantity. [Google Scholar] [CrossRef]
- Currie, Ian, Maria Durban, and Paul Eilers. 2003. Using P-splines to extrapolate two-dimensional Poisson data. Paper presented at the 18th International Workshop on Statistical Modelling, Leuven, Belgium, July 7–11. [Google Scholar]
- Debonneuil, Edouard. 2015. Parametric age-dependent mortality model, for applications to retirement portfolios. In Actuarial Thesis. Lyon: ISFA. [Google Scholar]
- Ghalanos, Alexios, and Stefan Theussl. 2015. Rsolnp: General Non-Linear Optimization. Available online: https://cran.r-project.org/package=Rsolnp (accessed on 31 December 2023).
- Guette, Vivien. 2010. La prise en compte des catastrophes dans la modélisation de la mortalité. In Actuary Thesis. Lyon: ISFA. [Google Scholar]
- Guilbaud, Corentin. 2018. Nouveaux modèles d’analyse et de projection de la mortalité, application a la population française. In Mémoire d’Actuaire. Paris: Dauphine. [Google Scholar]
- Hyndman, Rob. 2023. Demography: Forecasting Mortality, Fertility, Migration and Population Data. Available online: https://cran.r-project.org/package=demography/index.html (accessed on 31 December 2023).
- Juillard, Marc, and Frederic Planchet. 2006. Mesure de l’incertitude tendancielle sur la mortalité—Application à un régime de rentes. Assurances et Gestion des Risques 75: 357–74. [Google Scholar]
- Juillard, Marc, Frédéric Planchet, and Pierre E. Thérond. 2008. Perturbations extrêmes sur la dérive de mortalité anticipée. Assurances et Gestion des Risques 76: 1–11. [Google Scholar]
- Lee, Ronald D., and Lawrence R. Carter. 1992. Modeling and forecasting us mortality. Journal of the American Statistical Association 87: 659–71. [Google Scholar]
- Planchet, Frédéric, and Pierre E. Thérond. 2011. Modélisation Statistique des Phénomènes de Durée—Applications Actuarielles. Paris: Economica. [Google Scholar]
- Plat, Richard. 2009. On stochastic mortality modelling. Insurance: Mathematics and Economics 45: 393–404. [Google Scholar]
- Robben, Jens, and Katrien Antonio. 2023. Catastrophe risk in a stochastic multi-population mortality model. arXiv arXiv:2306.15271. [Google Scholar]
- Thatcher, A. R. 1999. The Long-term Pattern of Adult Mortality and the Highest Attained Age. Journal of the Royal Statistical Society 162: 5–43. [Google Scholar] [CrossRef] [PubMed]
- Vaupel, James W., Kenneth Manton, and Eric Stallard. 1979. The impact of heterogeneity in individual frailty on the dynamics of mortality. Demography 16: 439–54. [Google Scholar] [CrossRef] [PubMed]
- Ye, Yinyu. 1987. Interior Algorithms for Linear, Quadratic, and Linearly Constrained Non-Linear Programming. Ph.D. thesis, Department of EES Stanford University, Stanford, CA, USA. [Google Scholar]

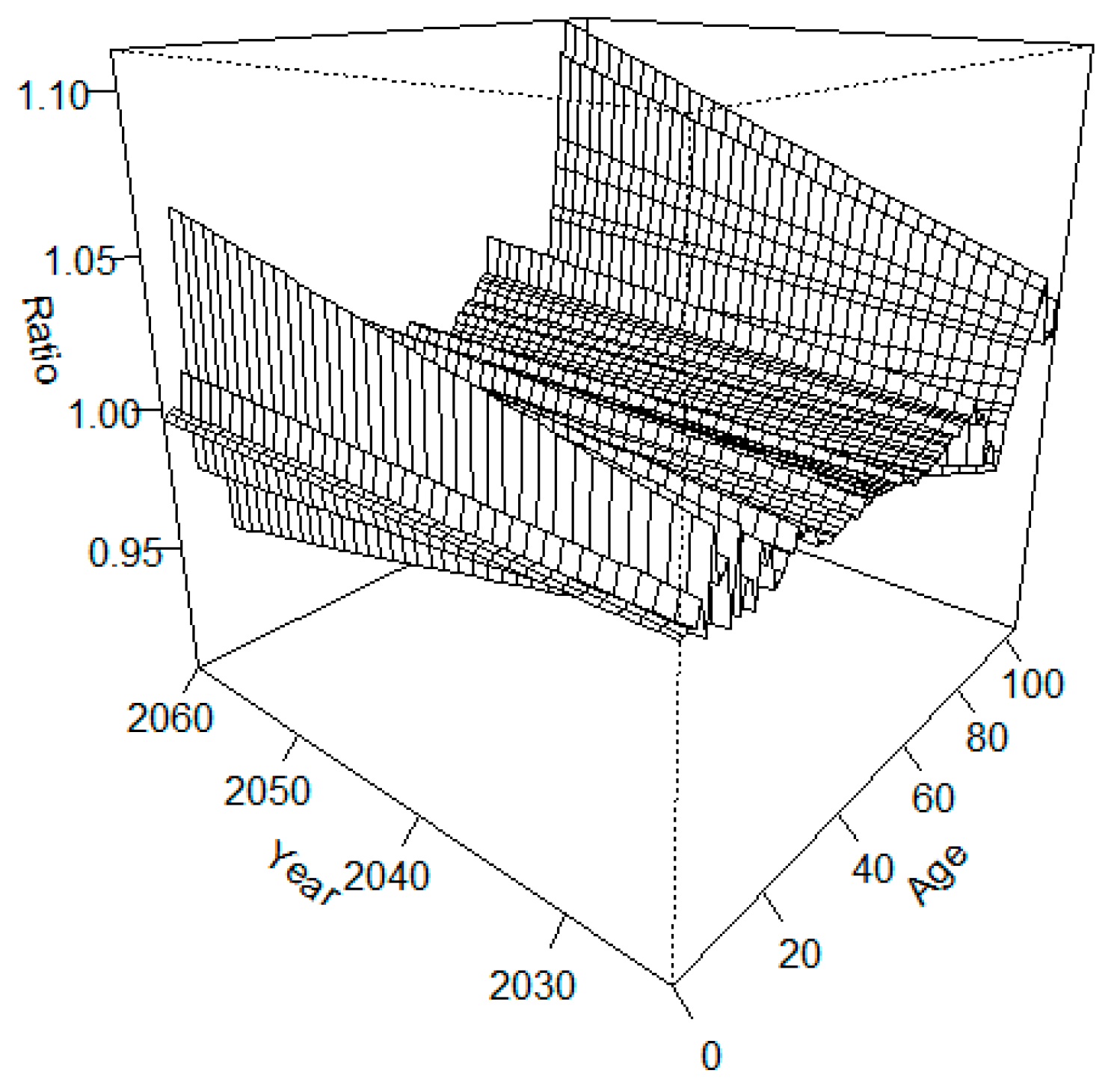
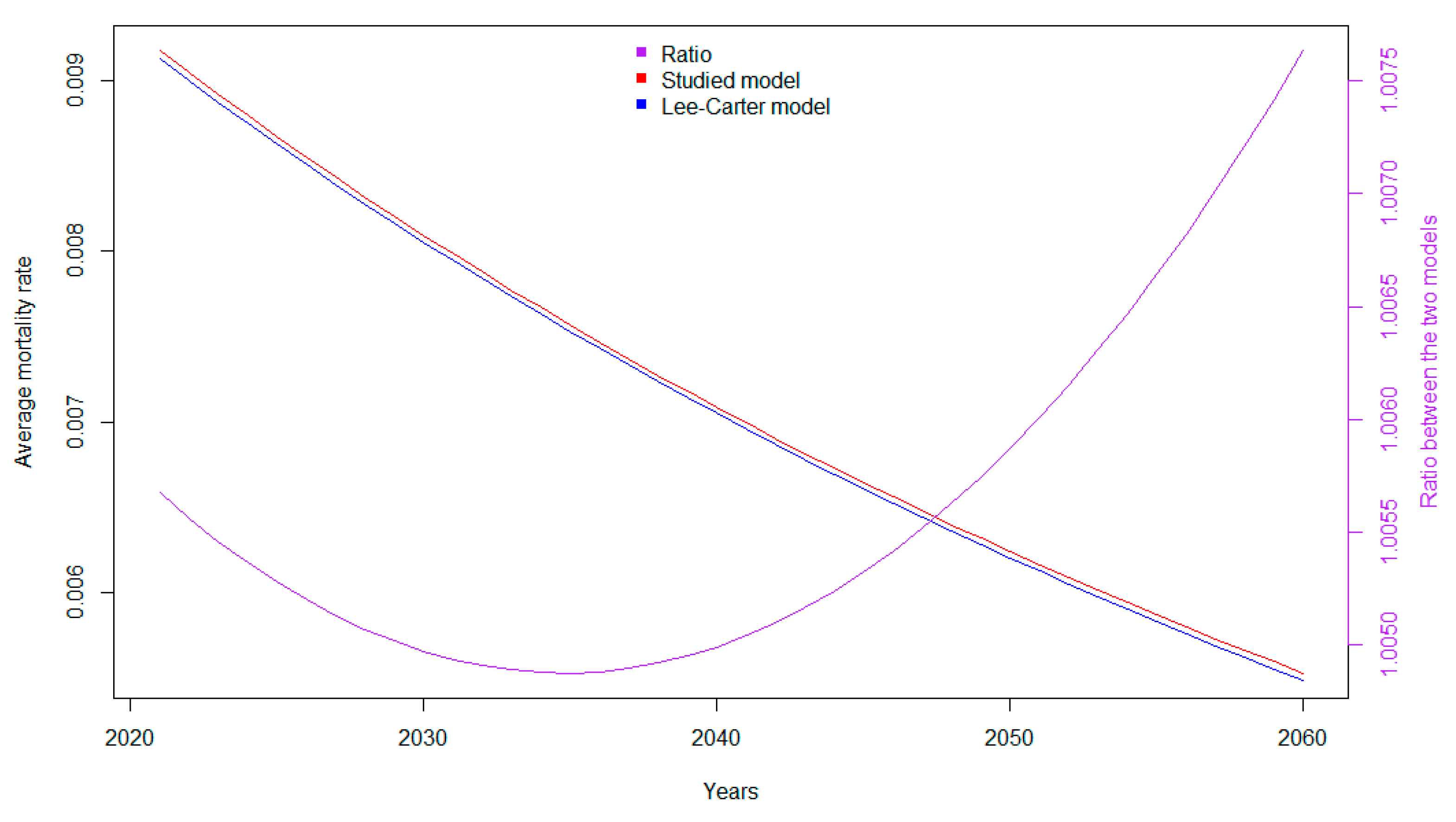

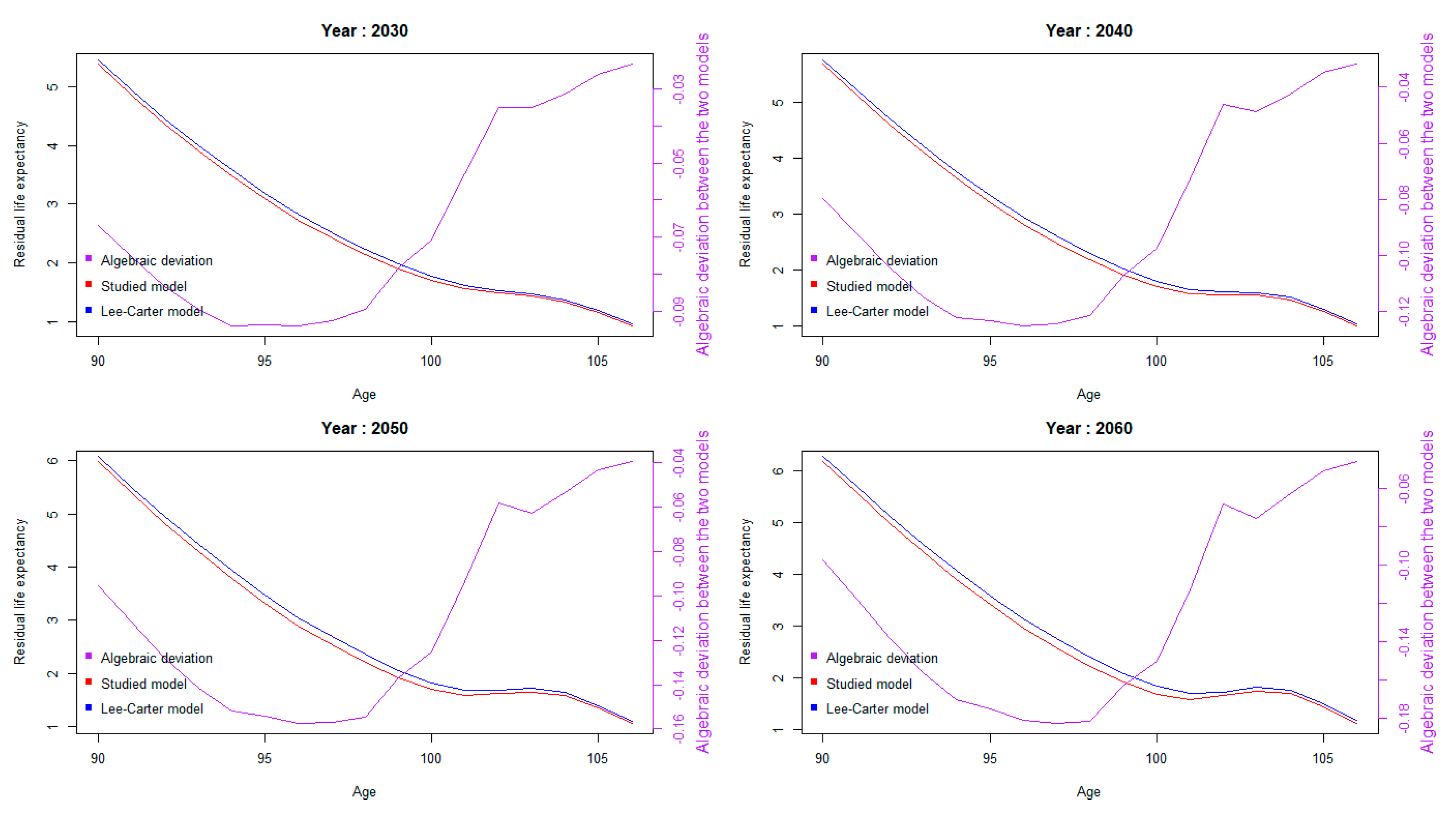

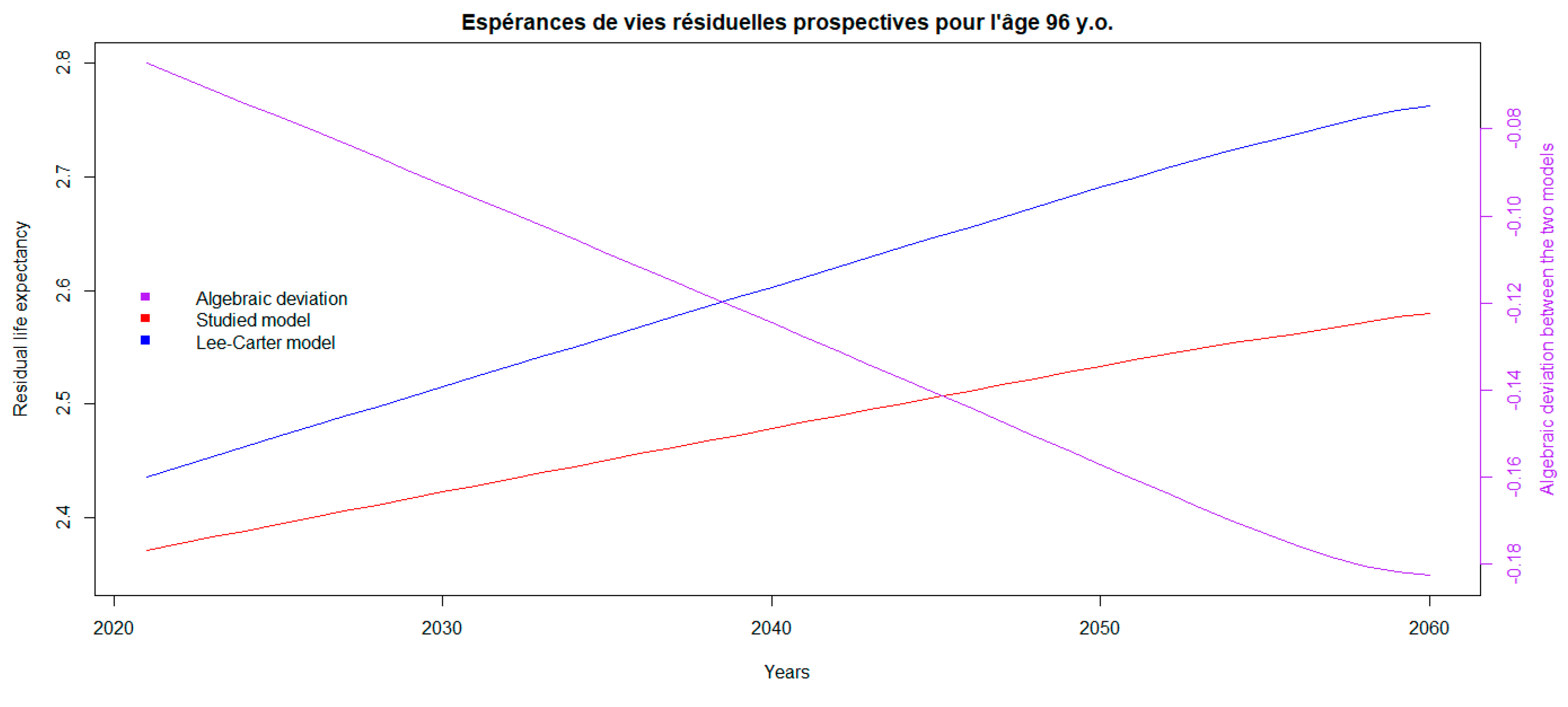

| m | p | |
|---|---|---|
| Model studied | −2.19 | 4401.98 |
| Lee–Carter reference model | −2.19 | 4402.33 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Planchet, F.; Gautier de La Plaine, G. Adding Shocks to a Prospective Mortality Model. Risks 2024, 12, 57. https://doi.org/10.3390/risks12030057
Planchet F, Gautier de La Plaine G. Adding Shocks to a Prospective Mortality Model. Risks. 2024; 12(3):57. https://doi.org/10.3390/risks12030057
Chicago/Turabian StylePlanchet, Frédéric, and Guillaume Gautier de La Plaine. 2024. "Adding Shocks to a Prospective Mortality Model" Risks 12, no. 3: 57. https://doi.org/10.3390/risks12030057
APA StylePlanchet, F., & Gautier de La Plaine, G. (2024). Adding Shocks to a Prospective Mortality Model. Risks, 12(3), 57. https://doi.org/10.3390/risks12030057






