Regulation and De-Risking: Theoretical and Empirical Insights
Abstract
1. Introduction
2. Literature, Research Issues and Hypotheses
2.1. Why Regulation
- How should capital be defined?
- How much capital is sufficient?
- How liquid should capital be?
- Should there be a maximum level of leverage?
- Against which risks capital should be held?
- Should capital requirements be a percentage of face value or be risk weighted?
- How should systemic issues such as pro-cyclicality be addressed?
2.2. Theoretical Perspectives
2.3. Empirical Research
3. Method and Research Hypotheses
4. Data and Descriptive Statistics
5. Statistical Analysis and Interpretation of Results
6. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
| 1 |
|
| 2 | In focusing on VaR, our concern is market risk as regulated under Basel-inspired regulations. Other types of risk such as Credit Risk and Operational risk also have regulatory capital requirements but are not examined in our research. Arguably, Market Risk is the most identifiable with systematic risk, as per the Capital Asset Pricing Model. |
| 3 | See Note 9 concerning Silicon Valley Bank. |
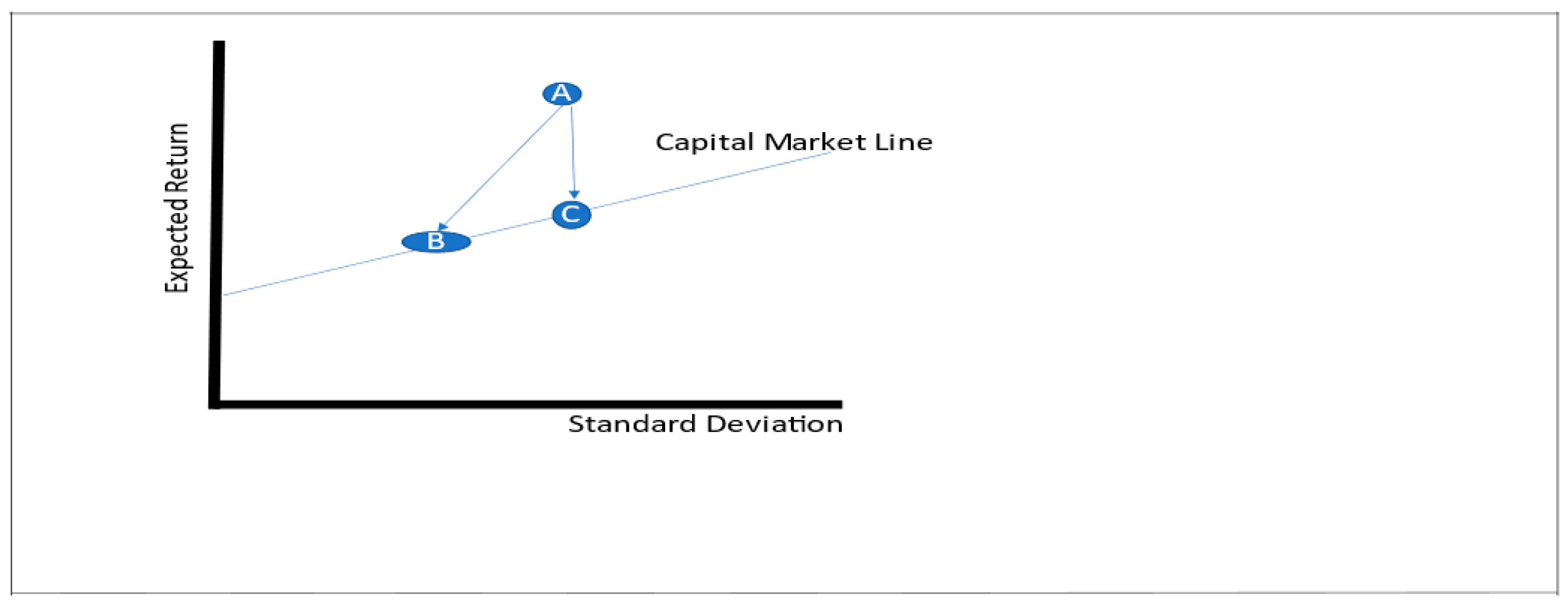
| 4 | The work of Kaplanski and Levy (2015) is distinct in positing more than one CML, i.e., one with and without regulatory impositions. While intriguing, we ask, how can there be more than one Capital Market Line? Moreover, in their work, a constraint is imposed upon VaR, which is curious. Subject to model approval, VaR is not regulated; rather, the amount of capital required to support a level of VaR is regulated. |
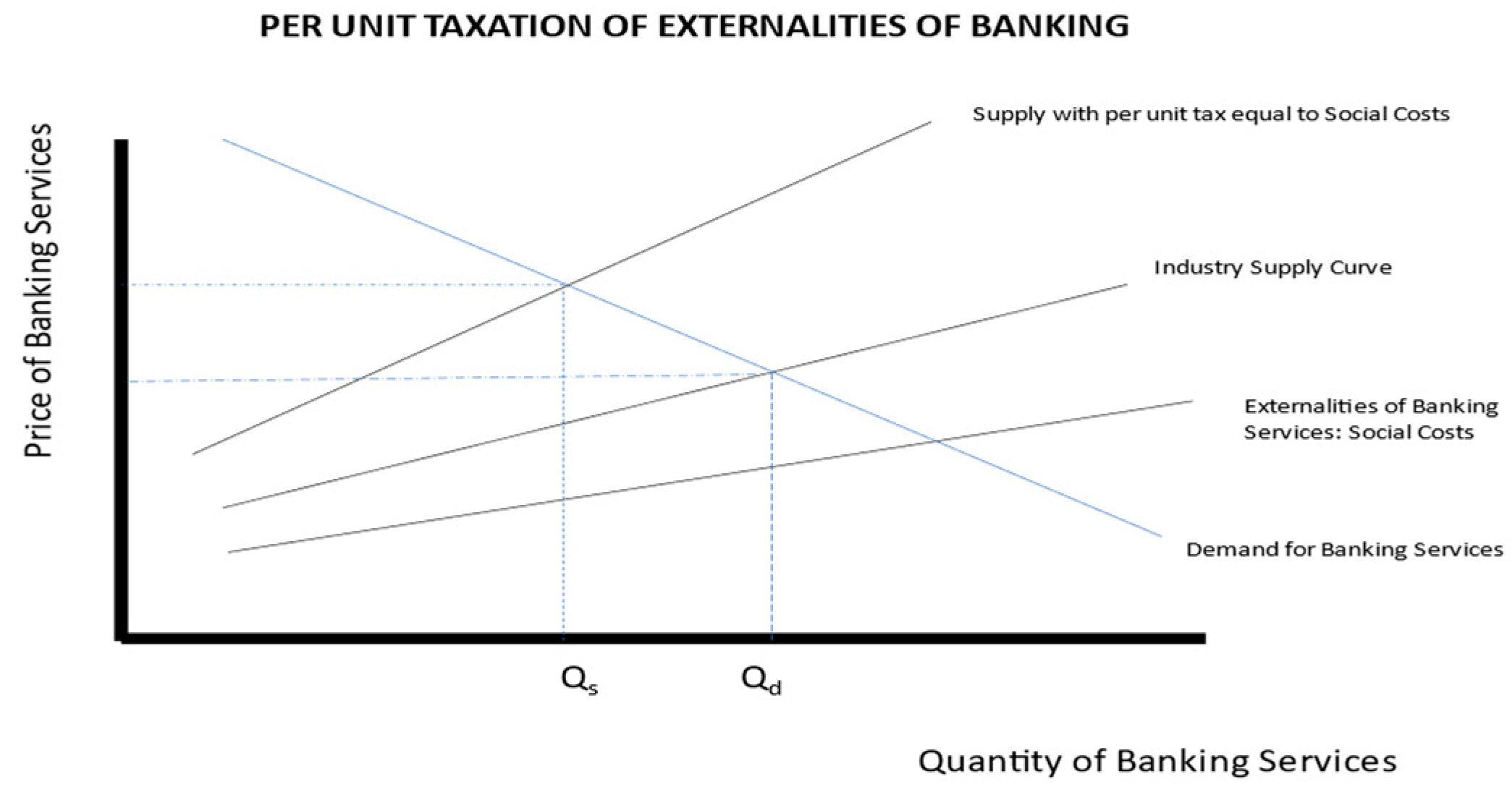
| 5 | We do not discuss the optimality of aligning private benefits with social costs through other means such as restricting the quantity of asset creation and lending by banks. How regulation has affected the nature and scope of credit creation are beyond the scope of the present research. |
| 6 | We examine the impact of the various Basel accords regulating the inputs to the creation of market risk through trading. As per the previous Note 5, examining the creation of credit risk and even examining how the two types of risk are jointly affected by regulation may be an area for future research. |
| 7 | See Note 1. |
| 8 | In contrast, according to the option valuation theory, unanticipated redistributions to wealth are possible, see (Copeland and Weston 1992, pp. 507–9). |
| 9 | According to some experts, the recent protection of large deposits of the non-systemically important Silicon Valley Bank in the USA is yet another example of moral hazard and lax oversight (Wall Street Journal, https://www.wsj.com/articles/did-esg-help-sink-svb-progressive-climate-bank-bailout-federal-reserve-treasury-biden-insurance-9db64b0b (accessed on 21 September 2021). |
| 10 | Non-linearity of systemic risk means that the “macro-prudential” exposure facing the economy may exceed the aggregate risks facing individual institutions. |
| 11 | We follow the approach found in chapter 8 of Mathematical Optimisation and Economic Theory by Michael D. Intriligator, Philadelphia, USA: Society for Industrial and Applied Mathematics (Intriligator 1971). |
| 12 | We assume that a bank is a “price-taker” with regard to the cost of debt and equity according to its capital structure. |
| 13 | We note that banks are required by their regulators to report VaR along with related statistics. Though it has been argued that banks may have an incentive to under-estimate their exposure, according to research, the opposite is possible as well as diversification benefits are under-estimated (Pérignon et al. 2008). Notwithstanding such concerns, as officially reported metrics, we assume the validity of the reported VaR. |
| 14 | Although the various major financial centres where these banks are head-quartered may have implemented the Basel Accords in not entirely consistent manners hypothetically leaving scope for regulatory arbitrage, all these banks operate globally. The major regulators moreover regularly compare how their respective institutions and non-headquartered institutions measure their risk exposures. |
| 15 | Adjusted Beta vs. Raw Beta—The beta of a share may be presented as either an Adjusted Beta or a Raw Beta. A Raw Beta is obtained from the linear regression of a stock’s historical data. Raw Beta, also known as Historical Beta, is based on the observed relationship between the security’s return and the returns of an index. The Adjusted Beta is an estimate of a security’s future Beta. Adjusted Beta is derived from historical data but modified by the assumption that a security’s true Beta will move towards the market average, of 1. The formula used to adjust Beta is (0.67) × Raw Beta + (0.33) × 1.0. All Betas are computed using the relevant markets-exchanges on which they trade. |
| 16 | The regulatory changes moving from Basel I to II to III are well known but importantly with the last phase, there has been a greater focus on the quality of capital and its liquidity reflecting the lessons of the 2008 financial crisis. |
| 17 | ). |
| 18 | To address the inherent funding risk of banks, creating illiquid assets from liquid deposits, two new liquidity constraints were introduced under Basel III: The Liquidity Coverage Ratio (LCR) to promote the short-term resilience of banks and the Net Stable Funding Ratio (NSFR) to incentivise a stable and reliable source of funds. Compared to capital regulation, there has been less theoretical and empirical investigation of their respective impacts. To the extent that the two ratios encourage banks to behave differently than they would otherwise, holding more liquid assets for stress events, there may be a cost in impeding the transformation function but measuring the impact is difficult (Elliot et al. 2012). According to research on the EU’s largest banks, both the LCR and the NSFR increased capital requirements by reducing bank fragility (Chiaramonte and Casub 2017). Both ratios may lead to holding greater capital, but this may have occurred for other reasons as well. If Liquidity Regulation makes banks safer, then the cost of both debt and equity should fall if everything else were equal…but it is not. Assessing the impact of these two ratios hinges on whether the cost of capital is appropriate to its risks both individually as well as for the sector, but controlling for other effects would be difficult. |
| 19 | While acknowledging the many limitations of VaR, we utilise it as a widely accepted summary measure of market risk (See, Lesnevski et al. (2007)). Its identification, measurement, reporting and management are regulatory requirements. Credit risk and Operational Risk while also falling within the Basel agenda are not examined in this research. |
| 20 | Some smaller but prominent institutions, such as Standard & Chartered, through their antecedents have been involved in edible oils and minerals on behalf of their clients going back to the 19th Century. The origins of Rabo Bank of the Netherlands Bank began with agricultural credit unions. |
| 21 | As reported by Consultancy Coalition, the commodity trading performance of the top 12 banks improved somewhat in 2019 through involvement in metals and petroleum markets (Reuters, 21 February 2020. https://www.reuters.com/article/banks-commodities-revenue/top-banks-2019-commodities-revenue-climbs-11-consultancy-coalition-idUSL8N2AL2R4, accessed on 3 March 2020). |
| 22 | This situation is unlikely as banks tend to set position limits according to VaR. If VaR were to fall because of a secular reduction in volatility, permitted positions might be increased. VaR is regulated by stipulating the required capital. |
| 23 | Further, the risk of bankruptcy is not rewarded by higher returns with the CAPM framework (Dichev 1998). |
References
- Abbas, Faisal, Omar Masood, Shoaib Ali, and Sohail Rizwan. 2021. How Do Capital Ratios Affect Bank Risk-Taking: New Evidence from the United States. Sage Open Journal 11: 2158244020979678. [Google Scholar] [CrossRef]
- Admati, Anat R., and Martin F. Hellwig. 2013. Does Debt Discipline Bankers? An Academic Myth about Bank Indebtedness. Available online: https://admati.people.stanford.edu/sites/g/files/sbiybj1846/f/publications/3031_1.pdf (accessed on 12 September 2021).
- Admati, Anat R., Peter M. DeMarzo, Martin F. Hellwig, and Paul C. Pfleiderer. 2012. Debt Overhang and Capital Regulation (March 23). Rock Center for Corporate Governance at Stanford University Working Paper No. 114, MPI Collective Goods Preprint, No. 2012/5. Available online: https://ssrn.com/abstract=2031204 (accessed on 2 February 2022).
- Admati, Anat R., Peter M. DeMarzo, Martin Hellwig, and Paul Pfleiderer. 2013. Fallacies, Irrelevant Facts, and Myths in the Discussion of Capital Regulation: Why Bank Equity Is Not Expensive. Stanford Graduate School of Business Working Paper No. 2065. Available online: https://www.gsb.stanford.edu/faculty-research/working-papers/fallacies-irrelevant-facts-myths-discussion-capital-regulation-why (accessed on 2 February 2022).
- Alexander, Gordon J., and Alexandre M. Baptista. 2006. Does the Basel Capital Accord reduce bank fragility? An assessment of the value-at-risk approach. Journal of Monetary Economics, Elsevier, Amsterdam 53: 1631–60. [Google Scholar] [CrossRef]
- Allen, Franklin, Elena Carletti, and Agnese Leonetto. 2011. Deposit Insurance and Risk Taking. Oxford Review of Economic Policy 27: 464–78. [Google Scholar] [CrossRef]
- Armour, John, Dan Awrey, Paul Davies, Luca Enriques, Jeffrey N. Gordon, Colin Mayer, and Jennifer Payne. 2016. Principles of Financial Regulation. Oxford: Oxford University Press. [Google Scholar]
- Arthur, Terry, and Philip Booth. 2010. Does Britain Need a Financial Regulator. Paris: IEA. [Google Scholar]
- Asal, Maher. 2015. Estimating the Cost of Equity Capital of the Banking. Journal of Applied Finance & Banking 5: 69–96. [Google Scholar]
- Baker, Malcolm, and Jeffrey Wurgler. 2015. Do Strict Capital Requirements Raise the Cost of Capital? Bank Regulation, Capital Structure, and the Low-Risk Anomaly. American Economic Review 105: 315–20. [Google Scholar] [CrossRef]
- Barnes, Samantha. 2014. Is This the End of Commodity Trading in Banks? International Banker. July. Available online: https://internationalbanker.com/brokerage/end-commodity-trading-banks/ (accessed on 2 February 2022).
- Basak, Suleyman, and Alexander Shapiro. 2001. Value-at-Risk-Based Risk Management: Optimal Policies and Asset Prices. The Review of Financial Studies 14: 371–405. Available online: http://www.jstor.org/stable/2696745 (accessed on 2 February 2022). [CrossRef]
- Boyd, John H., and Gianni. De Nicolo. 2005. The Theory of Bank Risk Taking and Competition Revisited. Journal of Finance 60: 1329–43. [Google Scholar] [CrossRef]
- Brandao-Marques, Luis, Ricardo Correa, and Horacio Sapriza. 2020. Government support, regulation, and risk taking in the banking sector. Journal of Banking & Finance 112: 105284. [Google Scholar]
- Chan-Lau, Jorge Antonio, Estelle Liu, and Jochen Schmittmann. 2012. Equity Returns in the Banking Sector in the Wake of the Great Recession and the European Sovereign Debt Crisis (July 1). Available online: https://ssrn.com/abstract=2101635 (accessed on 2 February 2022).
- Chiaramonte, Laura, and Barbara Casub. 2017. Capital and liquidity ratios and financial distress. Evidence from the European banking industry. The British Accounting Review 49: 138–61. [Google Scholar] [CrossRef]
- Copeland, Thomas E., and J. Fred Weston. 1992. Financial Theory and Corporate Policy: Pearson New International Edition, 3rd ed. Boston: Addison Wesley. ISBN 0-201-10648-5. [Google Scholar]
- Culp, Christopher L., and Andrea M. P. Neves. 2017. Shadow Banking, Risk Transfer, and Financial Stability. Journal of Applied Corporate Finance 29: 45–64. [Google Scholar] [CrossRef]
- Daníelsson, Jón, Hyun Song Shin, and Jean-Pierre Zigrand. 2004. The impact of risk regulation on price dynamics. Journal of Banking and Finance 28: 1069–87. [Google Scholar] [CrossRef]
- Demirguc-Kunt, Asli, and Harry Huizinga. 2010. Bank activity and funding strategies: The impact on risk and returns. Journal of Financial Economics 98: 626–50. [Google Scholar] [CrossRef]
- Dichev, Ilia D. 1998. Is the Risk of Bankruptcy a Systematic Risk? The Journal of Finance 53: 1131–47. Available online: http://www.jstor.org/stable/117389 (accessed on 21 August 2021). [CrossRef]
- Dombret, Andreas, Yalin Gündüz, and Joerg Rocholl. 2017. Will German Banks Earn Their Cost of Capital? Bundesbank Discussion Paper, No. 01/2017. Frankfurt: Deutsche Bundesbank. [Google Scholar] [CrossRef]
- Eichberger, Jürgen, and Martin Summer. 2010. Bank Capital, Liquidity and Systemic Risk. Journal of the European Economic Association 3: 547–55. [Google Scholar] [CrossRef]
- Elliott, Douglas, Suzanne Salloy, and Andre Oliveira Santos. 2012. Assessing the Cost of Financial Regulation. September. IMF Working Paper No. 12/233. Washington, DC: International Monetary Fund. Available online: https://ssrn.com/abstract=2164587/233 (accessed on 2 February 2022).
- Gropp, Reint E., Thomas Mosk, Steven Ongena, and Carlo Wix. 2016. Bank Response to Higher Capital Requirements: Evidence from a Quasi-Natural Experiment. IWH Discussion Papers 33/2016. Halle: Halle Institute for Economic Research (IWH). [Google Scholar]
- Guo, Rui, Ying Jiang, Ao Li, Zhigang Qiu, and Hefei Wang. 2021. A model of delegation with a VaR constraint. Finance Research Letters 42: 101895. [Google Scholar] [CrossRef]
- Haldane, Aandrew, and Robert M. May. 2011. Systemic risk in banking ecosystems. Nature 469: 351–55. [Google Scholar] [CrossRef]
- Hanson, Samuel G., Anil K. Kashyap, and Jeremy C. Stein. 2011. A Macroprudential Approach to Financial Regulation. Journal of Economic Perspectives 25: 3–28. [Google Scholar] [CrossRef]
- Hirtle, Beverly, Anna Kovner, and Matthew Plosser. 2020. The Impact of Supervision on Bank Performance. The Journal of Finance 75: 2765–808. [Google Scholar] [CrossRef]
- Hogan, Thomas L. 2015. Capital and risk ratios in commercial banking: A comparison of capital and risk-based capital ratios. The Quarterly Review of Economics and Finance 57: 32–45. [Google Scholar] [CrossRef]
- Hoque, Hafiz, Dimitris Andriosopoulos, Kostas Andriosopoulos, and Douady Raphael. 2015. Bank regulation, risk and return: Evidence from the credit and sovereign debt crises. Journal of Banking and Finance 50: 455–74. [Google Scholar] [CrossRef]
- Intriligator, Michael D. 1971. Mathematical Optimization and Economic Theory Classics in Applied Mathematics. Philadelphia: Society for Industrial and Applied Mathematics. [Google Scholar]
- Jiang, Hai, Jinyi Zhang, and Chen Sun. 2020. How does capital buffer affect bank risk-taking? New evidence from China using quantile regression. China Economic Review 60: 101300. [Google Scholar] [CrossRef]
- Kahane, Yehuda. 1977. Capital adequacy and the regulation of financial intermediaries. Journal of Banking and Finance 1: 207–17. [Google Scholar] [CrossRef]
- Kaplanski, Guy, and Haim Levy. 2015. Value-at-risk capital requirement regulation, risk taking and asset allocation: A mean–variance analysis. The European Journal of Finance 21: 215–41. [Google Scholar] [CrossRef]
- Kern, Alexander. 2019. Principles of Banking Regulation. Cambridge: Cambridge University Press. [Google Scholar] [CrossRef]
- Kim, Daesik, and Anthony M. Santomero. 1988. Risk in banking and capital regulation. Journal of Finance 43: 1219–33. [Google Scholar] [CrossRef]
- King, Michael R. 2009. The cost of equity for global banks: A CAPM perspective from 1990 to 2009. BIS Quartery Review 59–73. Available online: https://www.bis.org/publ/qtrpdf/r_qt0909g.pdf (accessed on 2 February 2022).
- Klein, Michael A. 1971. A Theory of the Banking Firm. Journal of Money, Credit and Banking 3: 205–18. [Google Scholar] [CrossRef]
- Kobrak, Christopher, and Michael Troege. 2015. From Basel to bailouts: Forty years of international attempts to bolster bank safety. Financial History Review 22: 133–56. [Google Scholar] [CrossRef]
- Koehn, Michael, and Anthony M. Santomero. 1980. Regulation of bank capital and portfolio risk. Journal of Finance 35: 1235–50. [Google Scholar] [CrossRef]
- Leippold, Markus, Paolo Vanini, and Silvan Ebnoether. 2006. Optimal credit limit management under different information regimes. Journal of Banking & Finance 30: 463–87. [Google Scholar]
- Lesnevski, Vadim, Barry L. Nelson, and Jeremy Staum. 2007. Simulation of Coherent Risk Measures Based on Generalized Scenarios. Management Science 53: 1756–69. [Google Scholar] [CrossRef]
- Maccario, Aurelio, Andrea Sironi, and Cristiano Zazzara. 2002. “Is banks’ Cost of Equity Capital Different across Countries? Evidence from the G10 Countries Major Banks”, Libera Università Internazionale degli Studi Sociali (LUISS) Guido Carli, Working Paper. Available online: https://papers.ssrn.com/sol3/papers.cfm?abstract_id=335721 (accessed on 10 October 2021).
- McCauley, Robert N., and Steven A. Zimmer. 1991. Cost of Capital for Industry and Banks. Economics 26: 14–18. Available online: http://www.jstor.org/stable/23485845 (accessed on 10 October 2021).
- Megaw, Nicholas, and Kate Beioley. 2018. Big UK banks wade back into wealth management. Financial Times, August 12. Available online: https://www.ft.com/content/bd99a084-9c84-11e8-9702-5946bae86e6d (accessed on 2 February 2022).
- Miles, David, Jing Yang, and Gilberto Marcheggiano. 2012. Optimal Bank Capital. The Economic Journal 123: 1–37. [Google Scholar] [CrossRef]
- Modigliani, Franco, and Merton Miller. 1958. The Cost of Capital, Corporate Finance and the Theory of Investment. American Economic Review 48: 261–97. [Google Scholar]
- Neri, Massimiliano. 2012. The Unintended Consequences of the Basel III Liquidity Risk Regulation (June 30). Available online: https://ssrn.com/abstract=2096821 (accessed on 2 February 2022).
- Pandit, Vikram. 2010. We Must Rethink Basel or Growth Will Suffer. Financial Times, November 10. [Google Scholar]
- Pelligrinia, Carlo Bellavite, Michele Meolib, and Giovanni Urga. 2017. Money market funds, shadow banking and systemic risk in United Kingdom. Finance Research Letters 21: 163–71. [Google Scholar] [CrossRef]
- Pérignon, Christophe, Zi Yin Deng, and Zhi Jun Wang. 2008. Do banks overstate their Value-at-Risk? Journal of Banking & Finance 32: 783–94. [Google Scholar] [CrossRef]
- Shahzad, Syed Jawad Hussain, Thi Hong Van Hoang, and Jose Arreola-Hernandez. 2019. Risk spillovers between large banks and the financial sector: Asymmetric evidence from Europe. Finance Research Letters 28: 153–59. [Google Scholar] [CrossRef]
- Shrieves, Ronald E., and Drew Dahl. 1992. The relationship between risk and capital in commercial banks. Journal of Banking & Finance 16: 439–57. [Google Scholar] [CrossRef]
- Van Der Weide, Mark E., and Jeffery. Y. Zhang. 2019. Bank Capital Requirements after the Financial Crisis. In The Oxford Handbook of Banking, 3rd ed. Edited by Allen N. Berger, Philip Molyneux and John O. S. Wilson. Oxford: Oxford Academic. [Google Scholar]
- Verdoes, T. 2013. Basel III Capital Requirements: Costs and Benefits for Banks and Societies. Leidenlawblog. Available online: http://leidenlawblog.nl/articles/basel-iii-capital-requirements-costs-and-benefits-for-banks-and-societies (accessed on 15 October 2021).
- Weigand, Robert A. 2016. The performance and risk of banks in the U.S., Europe and Japan post financial crisis. Investment Management and Innovation 13: 75–93. [Google Scholar] [CrossRef]
- Yang, Jing, and Kostas Tsatsaronis. 2012. Bank stock returns, leverage and the business cycle. BIS Quarterly Review. March. Available online: https://ideas.repec.org/a/bis/bisqtr/1203g.html (accessed on 2 February 2022).
- Zhang, Zong-yi, Jun Wu, and Qiong-fang Liu. 2008. Impacts of Capital Adequacy Regulation on Risk-taking Behaviour of Banking. Systems Engineering–Theory and Practice 28: 183–89. [Google Scholar] [CrossRef]



| Ticker | Full Name | Ticker | Full Name |
|---|---|---|---|
| JPM | JP Morgan | PNC | PNC FINANCIAL SERVICES GROUP |
| BAC | Bank of America | BNP | BNP Paribas |
| RY | Royal Bank of Canada | CM | Canadian Imperial Bank of Commerce |
| TD | Toronto Dominion Bank | ISP | Intesa Sanpaolo SpA |
| WFC | Wells Fargo Bank | INGA | ING Groep NV |
| C | Citibank | ACA | Credit Agricole |
| BNS | Bank of Nova Scotia | FRC | First Republic Bank |
| HSBA | HSBC Holdings | NA | National Bank of Canada |
| TFC | Truist Financial Corp. | LLOY | Lloyds Banking Group PLC |
| USB | U.S. Bancorp | BARC | Barclays PLC |
| BMO | Bank of Montreal |
| Data Series and Frequency | |
|---|---|
| Alpha | Daily |
| Adjusted Beta | Daily |
| Cost of Debt | Quarterly |
| Cost of Equity | Quarterly |
| Debt to Equity Ratio | Quarterly |
| Return on Equity | Quarterly |
| VaR by Asset Classes | Quarterly |
| Weighted Average Cost of Capital | Quarterly |
| Financial Statistics for Selected Banks | ||||||
|---|---|---|---|---|---|---|
| Metric | Descriptive Statistics | 2000 to 2020 | Pre-Basel II 2000 to 2009 | Basel II to Basel III Transition 2009 to 2016 | Basel III Post 2016 | Percent Change Pre-Basel II to Post Basel III |
| Alpha | Mean | 0.0001 | 0.0003 | 0.0002 | −0.0002 | |
| Stdev. | 0.0010 | 0.0009 | 0.0012 | 0.0007 | ||
| Adjusted Beta | Mean | 1.08 | 0.98 | 1.21 | 1.07 | 8.87% |
| Stdev. | 0.26 | 0.21 | 0.31 | 0.16 | ||
| Cost of Debt | Mean | 2.21% | 3.52% | 1.55% | 1.24% | −104.49% |
| Stdev. | 1.38 | 1.16 | 0.74 | 0.80 | ||
| Cost of Equity | Mean | 11.62% | 9.43% | 13.88% | 11.48% | 19.67% |
| Stdev. | 3.58 | 1.97 | 4.01 | 2.56 | ||
| Debt to Equity Ratio | Mean | 3.31 | 4.05 | 3.14 | 2.24 | −59.25% |
| Stdev. | 3.61 | 4.63 | 3.02 | 1.29 | ||
| Return on Equity | Mean | 11.28% | 14.23% | 9.17% | 9.98% | −35.48% |
| Stdev. | 8.25 | 8.59 | 8.26 | 6.06 | ||
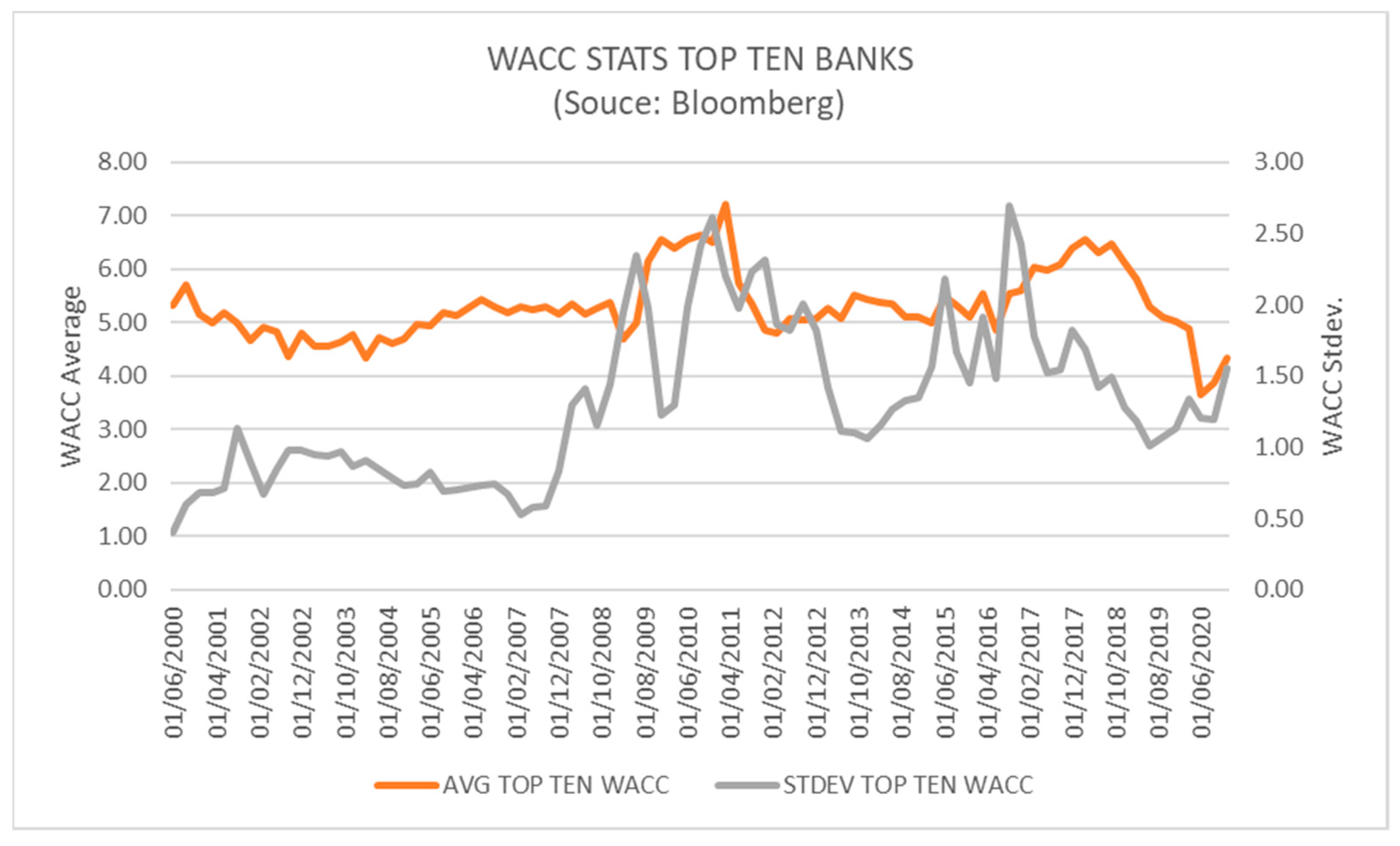
| WACC | Mean | 5.02 | 4.44 | 5.59 | 5.20 | 15.66% |
| Stdev. | 2.73 | 2.49 | 3.08 | 2.41 | ||
| Fall in WACC 2000 versus 2020 | |||||
|---|---|---|---|---|---|
| BANK | BANK | BANK | |||
| JPM | −34% | HSBA | −108% | ISP | −62% |
| BAC | −48% | TFC | −6% | INGA | −123% |
| RY | 17% | USB | −26% | ACA | −261% |
| TD | 9% | BMO | 13% | FRC | −54% |
| WFC | −67% | PNC | −5% | NA | 27% |
| C | −82% | BNP | −274% | LLOY | 47% |
| BNS | 7% | CM | −3% | BARC | −187% |
| Summary Statistics on VaR for Selected Banks | ||||||
|---|---|---|---|---|---|---|
| Metric | Descriptive Statistics | 2000 to 2020 | Pre-Basel II 2000 to 2009 | Basel II to Basel III Transition 2009 to 2016 | Basel III Post 2016 | Percentage Change Pre-Basel II versus Post Basel III |
| Total VaR (USD, Millions) | Mean | 66.22 | 72.84 | 72.11 | 51.56 | −35% |
| Total VaR (USD, Millions) | Stdev. | 61.87 | 68.40 | 65.54 | 36.41 | −63% |
| Rate VaR (USD, Millions) | Mean | 51.47 | 75.26 | 48.93 | 36.01 | −74% |
| Rate VaR (USD, Millions) | Stdev. | 56.08 | 69.13 | 58.71 | 32.38 | −76% |
| Equity VaR (USD, Millions) | Mean | 19.26 | 30.24 | 18.99 | 12.49 | −88% |
| Equity VaR (USD, Millions) | Stdev. | 18.15 | 23.16 | 18.23 | 8.59 | −99% |
| FX VaR (Millions) | Mean | 11.43 | 14.77 | 13.80 | 7.13 | −73% |
| FX VaR (Millions) | Stdev. | 13.14 | 13.54 | 16.27 | 6.83 | −68% |
| Commodity VaR (Millions) | Mean | 9.03 | 13.04 | 8.64 | 6.50 | −70% |
| Commodity VaR (Millions) | Stdev. | 9.61 | 12.30 | 8.41 | 7.50 | −49% |
| Z-Test for Difference in Mean WACC and Mean VaR during Pre-Basel II, II to III Transition and Post Basel III Periods | |||
|---|---|---|---|
| Changes in WACC Z-Test for Sample of Two Means | One-Tail Z Value | P(Z ≤ z) One-Tail | Z Critical One-Tail 95% |
| WACC: Pre Basel II vs. Transition II to III | −2.6743 | 0.0037 | 1.6449 |
| WACC: Pre Basel II vs. Post Basel III | −1.7447 | 0.0405 | 1.6449 |
| WACC: Transition Basel II to III vs. Post Basel III | 0.8000 | 0.2119 | 1.6449 |
| VaR: Pre Basel II vs. Transition | −2.8335 | 0.0023 | 1.6449 |
| VaR: Pre Basel II vs. Post Basel III | 10.5448 | 0.0000 | 1.6449 |
| VaR: Transition Basel II to III vs. Post Basel III | 12.7669 | 0.0000 | 1.6449 |
| Bank | Observations | Coefficient on WACC | T-Statistic on WACC | Coefficient on D/E | T-Statistic on D/E | Left-Tail T-Distribution WACC | Left-Tail T-Distribution D/E | Standard Error Y-Estimate | p-Value on WACC | p-Value on D/E | WACC | D/E | F-Statistic | F-Significance | Adjusted R-Squared | ||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Lower 95% | Upper 95% | Lower 95% | Upper 95% | ||||||||||||||
| JPM | 79 | 3.80 | 1.39 | 17.63 | 5.94 | 80.11% | 94.69% | 52.49 | 0.1695 | 2.585 × 10−08 | −1.66 | 9.27 | 11.72 | 23.54 | 129.19 | 3.546 × 10−25 | 75.44% |
| BAC | 80 | −5.78 | −1.58 | 29.07 | 6.19 | 82.00% | 94.90% | 52.68 | 0.1191 | 2.585 × 10−08 | −13.08 | 1.52 | 19.72 | 38.41 | 91.44 | 4.586 × 10−21 | 68.44% |
| TD | 39 | −1.20 | −1.44 | 17.00 | 4.93 | 80.72% | 93.64% | 8.17 | 0.1572 | 1.729 × 10−05 | −2.89 | 0.49 | 10.02 | 38.41 | 158.82 | 1.379 × 10−18 | 86.58% |
| WFC | 68 | excluded | n.a. | 13.66 | 7.14 | n.a. | 95.57% | 36.53 | n.a. | 8.664 × 10−10 | n.a. | n.a. | 9.84 | 17.49 | 50.91 | 9.298 × 10−10 | 41.69% |
| CITI | 79 | 4.62 | 1.52 | 19.35 | 7.88 | 81.48% | 95.98% | 57.19 | 0.1326 | 1.744 × 10−11 | −1.43 | 10.68 | 14.46 | 24.24 | 197.13 | 8.359 × 10−31 | 82.15% |
| PNC | 49 | excluded | n.a. | 3.17 | 14.50 | n.a. | 97.81% | 2.66 | n.a. | 3.611 × 10−19 | n.a. | n.a. | 2.73 | 3.60 | 210.37 | 5.643 × 10−19 | 79.34% |
| BNP | 57 | excluded | n.a. | 3.14 | 16.53 | n.a. | 98.08% | 18.61 | n.a. | 3.345 × 10−23 | n.a. | n.a. | 2.76 | 3.52 | 273.22 | 5.412 × 10−23 | 81.20% |
| ACA | 55 | 13.56 | 12.97 | excluded | n.a. | 97.55% | n.a. | 18.52 | 3 × 10−18 | n.a. | 11.46 | 15.65 | n.a. | n.a. | 168.21 | 4.476 × 10−18 | 73.85% |
| Average 30 Volatility during Basel Implementation Periods | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| Periods | Commodity Index | Euro Index | USD Index | FTSE 100 | Nasdaq Index | Dow Jones Industrial Index | Nikkei Index | US Treasury Index | EU Rate Index |
| Pre Basel II-2000 to 2009 | 16.41 | 5.28 | 9.31 | 17.51 | 31.03 | 7.05 | 23.39 | 4.73 | 3.00 |
| Basel II to Basel III Transition 2009 to 2016 | 15.69 | 7.17 | 10.16 | 17.34 | 18.93 | 6.01 | 22.29 | 4.23 | 3.01 |
| Basel III Post 2016 | 12.39 | 5.29 | 8.31 | 15.13 | 19.01 | 5.50 | 17.62 | 3.89 | 2.85 |
| Percent Change First and Last Periods | −28% | 0% | −11% | 15% | −49% | −10% | −28% | −20% | −5% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Haar, L.; Gregoriou, A. Regulation and De-Risking: Theoretical and Empirical Insights. Risks 2023, 11, 104. https://doi.org/10.3390/risks11060104
Haar L, Gregoriou A. Regulation and De-Risking: Theoretical and Empirical Insights. Risks. 2023; 11(6):104. https://doi.org/10.3390/risks11060104
Chicago/Turabian StyleHaar, Lawrence, and Andros Gregoriou. 2023. "Regulation and De-Risking: Theoretical and Empirical Insights" Risks 11, no. 6: 104. https://doi.org/10.3390/risks11060104
APA StyleHaar, L., & Gregoriou, A. (2023). Regulation and De-Risking: Theoretical and Empirical Insights. Risks, 11(6), 104. https://doi.org/10.3390/risks11060104







