Assessing the Impact of Credit Risk on Equity Options via Information Contents and Compound Options
Abstract
:1. Introduction
2. Firm’s Claims as Compound Options
Call and Put Equity Options as a –Fold Compound Options on Asset Value
3. Information Content Ratios as Measure of Impact of Credit Risk
4. Data and Estimation Methodology
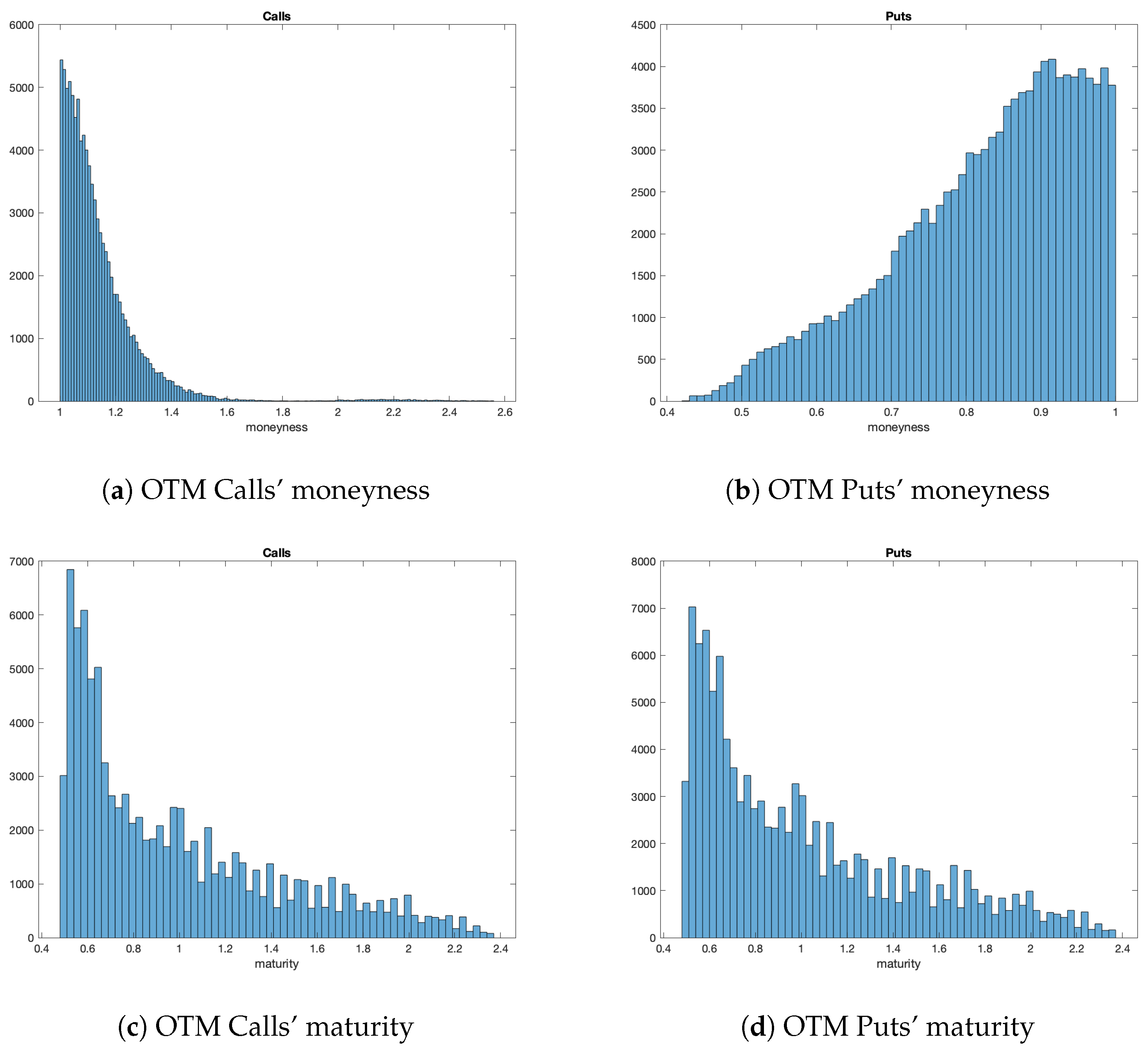
4.1. Dataset
4.2. Estimation of the Model Parameters
5. Empirical Tests
5.1. Cross Sectional Differences in Calls and Puts
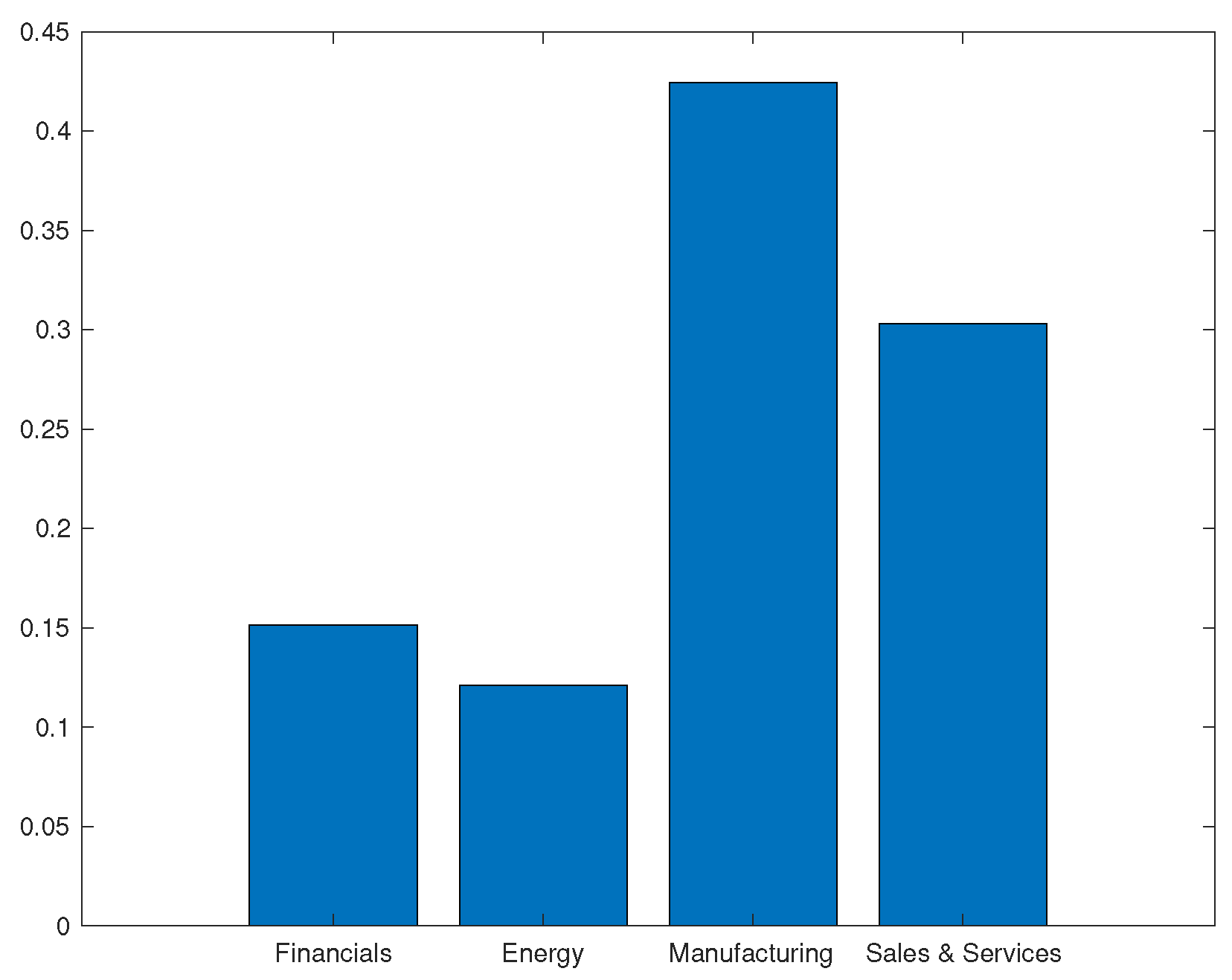
5.2. Sub-Samples Analysis Based on Sectors
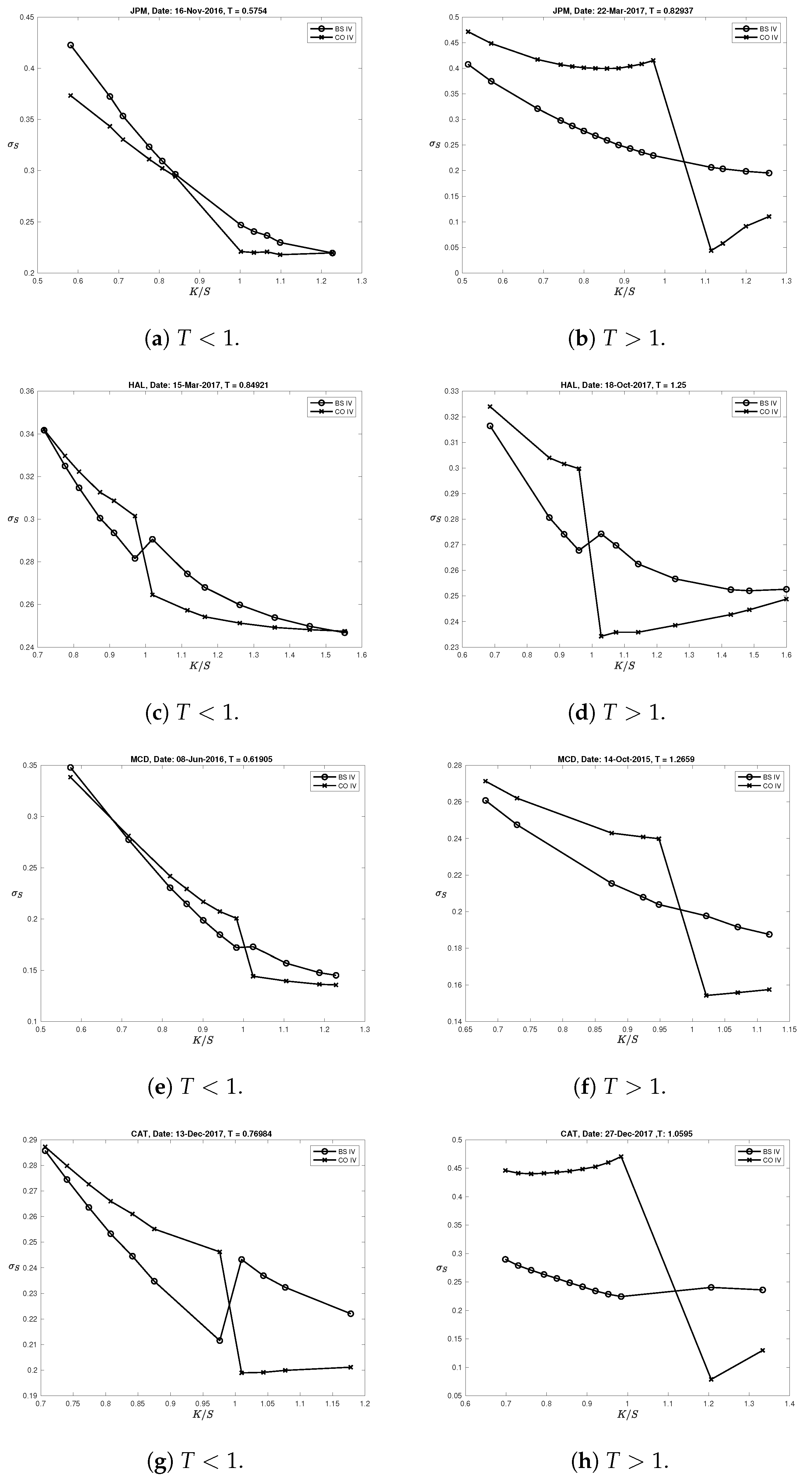
5.3. Explaining the Skew
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Sample Availability
| 1 | https://www.risk.net/derivatives/1505339/jp-morgan-chase-launches-equity-default-swaps (accessed on 11 October 2023). |
| 2 | |
| 3 | Shorting bonds is even more difficult in the cash market as the repo market for corporate bond is often illiquid, and the tenor of the agreement is usually very short. |
| 4 | These are the assumptions in Merton (1974) and Geske (1977). Specifically: (A.1) there are no transactions costs, taxes, or problems with indivisibilities of assets; (A.2) there are a sufficient number of investors with comparable wealth levels so that each investor believes that he can buy and sell as much of an asset as he wants at the market price; (A.3) there exists an exchange market for borrowing and lending at the same rate of interest; (A.4) short-sales of all assets, with full use of the proceeds, is allowed; (A.5) trading in assets takes place continuously in time. |
| 5 | That is the value of equity before paying the bond. E.g., if the continuation value of the equity is 20 and the face value of debt is 30, then equity is worthless (). |
| 6 | |
| 7 | To be precise, the option’s payoff consistent with the compound option model of equity should be
|
| 8 | In Information Theory, information content (or surprisal) of a signal is the amount of information gained when it is sampled. It is defined as minus the log-probability of the event: the less likely the event, the greater is the “surprise” associated if it happens. See Cover and Thomas (2006) for further details. |
| 9 | Usually, the -entropy of a discrete random variable X is defined as as the chosen base is usually . Here, instead, having a base , the minus is not necessary as the function is already positive. |
| 10 | Unreported empirical tests show that are indeed approximately constant for the sample. By construction, is already bounded in ; moreover it is the probability of the intersection of the option expiring ITM and the firm surviving up to . Therefore, as the probability of the intersection is smaller of the probability of the single events, it should not surprise that is quite small and stable. |
| 11 | This estimation technique is based on Brigo and Mercurio (2006) and is further discussed in Maglione (2022), Section 3.3. We refer to these references for further details. |
| 12 | Other values of loss given default have been investigated as a robustness and results are available upon request. |
| 13 | More specifically, after estimating the asset volatility surface from options, the average value is used to compute the asset value such that (4) holds. Subsequently, the model implied market value of debt is obtained. |
| 14 | The compound option model of default used here is able to model default only by the mean of financial leverage: if at reimbursement dates the equity of the firm is not large enough to repay the face value of the liability due, then the firm defaults. It should be clear that real-world default may occur not only in case of excessive financial leverage. Indeed, other sources of default are investigated in Carr and Wu (2017). What we refer as ‘apparent’ leverage effect is the possibility of observing a sizeable and similar skew both in the Black–Scholes and compound option implied volatilities when a firm is highly levered: since the compound options accounts for financial leverage, observing a large skew after having accounted for the latter may suggest that put option price the possibility of a large fall in asset prices for reasons other than leverage. |
References
- Anderson, Ronald, and Suresh Sundaresan. 1996. Design and valuation of debt contracts. Review of Financial Studies 9: 37–68. [Google Scholar] [CrossRef]
- Andreou, Panayiotis C. 2015. Effects of market default risk on index option risk-neutral moments. Quantitative Finance 15: 2021–40. [Google Scholar] [CrossRef]
- Black, Fischer. 1976. Studies of stock price volatility changes. In Proceedings of the 1976 Meeting of the Business and Economic Statistics Section. Washington, DC: American Statistical Association, pp. 177–81. [Google Scholar]
- Black, Fischer, and John C. Cox. 1976. Valuing corporate securities: Some effects of bond indenture provisions. Journal of Finance 31: 351–67. [Google Scholar] [CrossRef]
- Black, Fischer, and Myron Scholes. 1973. The pricing of options and corporate liabilities. Journal of Political Economy 81: 637–54. [Google Scholar] [CrossRef]
- Blanco, Roberto, Simon Brennan, and Ian. W. Marsh. 2005. An empirical analysis of the dynamic relation between investment-grade bonds and credit default swaps. Journal of Finance 60: 2255–81. [Google Scholar] [CrossRef]
- Brigo, Damiano, and Fabio Mercurio. 2006. Interest Rate Models: Theory and Practice. Berlin and Heidelberg: Springer Finance. [Google Scholar]
- Burkovska, Olena, Maximilian Gass, Kathrin Glau, Mirco Mahlstedt, Wim Schoutens, and Barbara Wohlmuth. 2018. Calibration to american options: Numerical investigation of the de-americanization method. Quantitative Finance 18: 1091–113. [Google Scholar] [CrossRef] [PubMed]
- Campbell, John. Y., and Glen. B. Taksler. 2003. Equity volatility and corporate bond yields. Journal of Finance 58: 2321–49. [Google Scholar] [CrossRef]
- Cao, Charles, Fan Yu, and Zhaodong Zhong. 2010. The information content of option-implied volatility for credit default swap valuation. Journal of Financial Markets 13: 321–43. [Google Scholar] [CrossRef]
- Carr, Peter, and Liuren Wu. 2007. Theory and evidence on the dynamic interactions between sovereign credit default swaps and currency options. Journal of Banking and Finance 31: 2383–403. [Google Scholar] [CrossRef]
- Carr, Peter, and Liuren Wu. 2010. Stock options and credit default swaps: A joint framework for valuation and estimation. Journal of Financial Econometrics 8: 409–49. [Google Scholar] [CrossRef]
- Carr, Peter, and Liuren Wu. 2011. A simple robust link between american puts and credit protection. Review of Financial Studies 24: 473–505. [Google Scholar] [CrossRef]
- Carr, Peter, and Liuren Wu. 2017. Leverage effect, volatility feedback, and self-exciting market disruptions. Journal of Financial and Quantitative Analysis 52: 2119–56. [Google Scholar] [CrossRef]
- Carr, Peter, and Vadim Linetsky. 2006. A jump to default extended CEV model: An application of Bessel processes. Finance and Stochastics 10: 303–30. [Google Scholar] [CrossRef]
- Christie, Andrew A. 1982. The stochastic behavior of common stock variances: Value, leverage and interest rate effects. Journal of Financial Economics 10: 407–32. [Google Scholar] [CrossRef]
- Collin-Dufresne, Pierre, Robert S. Goldstein, and Fan Yang. 2012. On the relative pricing of long-maturity index options and collateralized debt obligations. Journal of Finance 67: 1983–2014. [Google Scholar] [CrossRef]
- Collin-Dufresne, Pierre, Robert S. Goldstein, and J. Spencer Martin. 2001. The determinants of credit spread changes. Journal of Finance 56: 2177–207. [Google Scholar] [CrossRef]
- Cover, Thomas M., and Joy A. Thomas. 2006. Elements of Information Theory. Hoboken: Wiley-Blackwell. [Google Scholar]
- Cremers, K. J. Martijn, Joost Driessen, and Pascal Meanhout. 2008a. Explaining the level of credit spreads: Option-implied jump risk premia in a firm value model. Review of Financial Studies 21: 2209–42. [Google Scholar] [CrossRef]
- Cremers, K. J. Martijn, Joost Driessen, Pascal Meanhout, and David Weinbaum. 2008b. Individual stock-option prices and credit spreads. Journal of Banking and Finance 32: 2706–15. [Google Scholar] [CrossRef]
- Culp, Christopher L., Yoshio Nozawa, and Pietro Veronesi. 2018. Option-based credit spreads. American Economic Review 108: 454–88. [Google Scholar] [CrossRef]
- Duffie, Darrell, and Kenneth J. Singleton. 1999. Modeling term structures of defaultable bonds. Review of Financial Studies 12: 687–720. [Google Scholar] [CrossRef]
- Elton, Edwin J., J. Gruber Martin, Deepak Agrawal, and Christopher Mann. 2001. Explaining the rate spread on corporate bonds. Journal of Finance 56: 247–77. [Google Scholar] [CrossRef]
- Ericsson, Jan, Kris Jacobs, and Rodolfo Ovied. 2009. The determinants of credit default swap premia. Journal of Financial and Quantitative Analysis 44: 109–32. [Google Scholar] [CrossRef]
- Geske, Robert 1977. The valuation of corporate liabilities as compound options. Journal of Financial and Quantitative Analysis 12: 541–52.
- Geske, Robert. 1979. The valuation of compound options. Journal of Financial Economics 7: 63–81. [Google Scholar] [CrossRef]
- Geske, Robert, Avanidhar Subrahmanyam, and Yi Zhou. 2016. Capital structure effects on the prices of equity call options. Journal of Financial Economics 36: 1639–52. [Google Scholar] [CrossRef]
- Huang, Jing-Zhi, and Ming Huang. 2012. How much of the corporate-treasury yield spread is due to credit risk? Review of Asset Pricing Studies 2: 153–202. [Google Scholar] [CrossRef]
- Hull, John, and Alan White. 1995. The impact of default risk on the prices of options and other derivative securities. Journal of Banking and Finance 9: 299–322. [Google Scholar] [CrossRef]
- Hull, John C., Izzy Nelkenand, and Alan D. White. 2004. Merton’s model, credit risk and volatility skews. Journal of Credit Risk 1: 3–28. [Google Scholar] [CrossRef]
- Jarrow, Robert A., and Stuart M. Turnbull. 1995. Pricing derivatives on financial securities subject to credit risk. Journal of Finance 50: 53–85. [Google Scholar] [CrossRef]
- Johnson, Herb, and René Stulz. 1987. The pricing of options with default risk. Journal of Finance 42: 267–80. [Google Scholar] [CrossRef]
- Leland, Hayne, and Klaus Bjerre Toft. 1996. Optimal capital structure, endogenous bankruptcy, and theterm structure of credit spreads. Journal of Finance 51: 987–1019. [Google Scholar] [CrossRef]
- Leland, Hayne E. 1994. Corporate debt value, bond covenants, and optimal capital structure. Journal of Finance 49: 1213–52. [Google Scholar] [CrossRef]
- Maglione, Federico. 2022. Credit spreads, leverage and volatility: A cointegration approach. Computation 10: 155. [Google Scholar] [CrossRef]
- Mella-Barral, Pierre, and William Perraudin. 1997. Strategic debt service. Journal of Finance 52: 531–66. [Google Scholar] [CrossRef]
- Mendoza-Arriga, Rafael, Peter Carr, and Vadim Linetsky. 2010. Time-changed Markov processes in unified credit-equity modeling. Mathematical Finance 20: 527–69. [Google Scholar] [CrossRef]
- Merton, Robert C. 1974. On the pricing of corporate debt: The risk structure of interest rates. Journal of Finance 29: 449–70. [Google Scholar]
- Merton, Robert C. 1977. On the pricing of contingent claims and the Modigliani-Miller theorem. Journal of Financial Economics 5: 241–9. [Google Scholar] [CrossRef]
- Strebulaev, Ilya A., and Toni M. Whited. 2012. Dynamic Models and Structural Estimation in Corporate Finance. Boston: Now Publishers Inc. [Google Scholar]
- Toft, Klaus Bjerre, and Brian Prucyk. 1997. Options on leveraged equity: Theory and empirical tests. Journal of Finance 53: 1151–80. [Google Scholar] [CrossRef]
- Vasquez, Aurelio, and Xiao Xiao. 2023. Default risk and option returns. Management Science. [Google Scholar] [CrossRef]


| Ticker | SIC | Division |
|---|---|---|
| AAPL | 3663 | Manufacturing |
| ABT | 2834 | Manufacturing |
| ACN | 8742 | Services |
| ALL | 6331 | Finance, Insurance and Real Estate |
| AMGN | 2836 | Manufacturing |
| AMZN | 5961 | Wholesale Trade |
| BA | 3721 | Manufacturing |
| BAC | 6020 | Finance, Insurance and Real Estate |
| BMY | 2834 | Manufacturing |
| C | 6199 | Finance, Insurance and Real Estate |
| CAT | 3531 | Manufacturing |
| CL | 2844 | Manufacturing |
| CMCSA | 4841 | Transportation, Communications, Electric, Gas and Sanitary service |
| COF | 6141 | Finance, Insurance and Real Estate |
| COP | 1311 | Mining |
| COST | 5399 | Wholesale Trade |
| CSCO | 3576 | Manufacturing |
| CVS | 5912 | Retail Trade |
| CVX | 2911 | Manufacturing |
| DD | 2821 | Manufacturing |
| DIS | 4888 | Transportation, Communications, Electric, Gas and Sanitary service |
| EMR | 3823 | Manufacturing |
| EXC | 4911 | Transportation, Communications, Electric, Gas and Sanitary service |
| F | 3711 | Manufacturing |
| FDX | 4513 | Transportation, Communications, Electric, Gas and Sanitary service |
| GD | 3721 | Manufacturing |
| GE | 4911 | Transportation, Communications, Electric, Gas and Sanitary service |
| HAL | 1389 | Mining |
| HD | 5211 | Wholesale Trade |
| IBM | 7370 | Services |
| INTC | 3674 | Manufacturing |
| JNJ | 2834 | Manufacturing |
| JPM | 6020 | Finance, Insurance and Real Estate |
| KO | 2086 | Manufacturing |
| LLY | 2834 | Manufacturing |
| LOW | 5211 | Wholesale Trade |
| MCD | 5812 | Retail Trade |
| MDT | 3845 | Manufacturing |
| MMM | 2670 | Manufacturing |
| MO | 2111 | Manufacturing |
| MON | 5169 | Retail Trade |
| MRK | 2834 | Manufacturing |
| MS | 6211 | Finance, Insurance and Real Estate |
| MSFT | 7372 | Services |
| ORCL | 7370 | Services |
| OXY | 1311 | Mining |
| PEP | 2080 | Manufacturing |
| PFE | 2834 | Manufacturing |
| PG | 2840 | Manufacturing |
| PM | 2111 | Manufacturing |
| RTN | 3812 | Manufacturing |
| SLB | 1389 | Mining |
| SO | 4911 | Transportation, Communications, Electric, Gas and Sanitary service |
| SPG | 6798 | Finance, Insurance and Real Estate |
| T | 4812 | Transportation, Communications, Electric, Gas and Sanitary service |
| TGT | 5331 | Wholesale Trade |
| TWX | 8748 | Services |
| TXN | 3674 | Manufacturing |
| UNH | 6324 | Finance, Insurance and Real Estate |
| UNP | 4011 | Transportation, Communications, Electric, Gas and Sanitary service |
| USB | 6020 | Finance, Insurance and Real Estate |
| UTX | 3724 | Manufacturing |
| VZ | 4812 | Transportation, Communications, Electric, Gas and Sanitary service |
| WFC | 6020 | Finance, Insurance and Real Estate |
| WMT | 5331 | Retail Trade |
| XOM | 1311 | Mining |
| Calls | Puts | |||
|---|---|---|---|---|
| Correlation (w/o CDS) | −0.0307 | −0.0053 | −0.0897 | 0.1291 |
| p-value | 0.000 *** | 0.155 | 0.000 *** | 0.000 *** |
| Correlation (with CDS) | −0.0723 | −0.0857 | −0.0751 | 0.1250 |
| p-value | 0.000 *** | 0.000 *** | 0.000 *** | 0.000 *** |
| (a): regressed onto(both constructed on calls). | |||||
| Regressand | Adj-: | 0.7416 | |||
| Regressors | Coefficient | Robust Standard Error | t-stat | p-value | |
| 0.7936 | 0.0050 | 160.07 | 0.000 | *** | |
| 0.0018 | 0.0001 | 29.42 | 0.000 | *** | |
| (b): regressed onto (both constructed on puts). | |||||
| Regressand | Adj-: | 0.9054 | |||
| Regressors | Coefficient | Robust Standard Error | t-stat | p-value | |
| 0.8357 | 0.0145 | 57.63 | 0.000 | *** | |
| −0.0002 | 0.00004 | −4.21 | 0.000 | *** | |
| (a): (constructed on calls) regressed onto . | |||||
| Regressand | Adj-: | 0.0309 | |||
| Regressors | Coefficient | Robust Standard Error | t-stat | p-value | |
| 0.0002 | 0.0004 | 0.53 | 0.630 | ||
| 0.0017 | 0.0006 | 2.74 | 0.071 | * | |
| Industry−FE | ✓ | ||||
| Year−FE | ✓ | ||||
| (b): (constructed on calls and CDSs) regressed onto . | |||||
| Regressand | Adj-: | 0.1120 | |||
| Regressors | Coefficient | Robust Standard Error | t-stat | p-value | |
| 0.0066 | 0.0013 | 5.16 | 0.014 | ** | |
| 0.0029 | 0.0011 | 2.59 | 0.081 | * | |
| Industry−FE | ✓ | ||||
| Year−FE | ✓ | ||||
| (c): (constructed on puts) regressed onto . | |||||
| Regressand | Adj-: | 0.4967 | |||
| Regressors | Coefficient | Robust Standard Error | t-stat | p-value | |
| 0.0253 | 0.0015 | 16.72 | 0.000 | *** | |
| −0.0060 | 0.0020 | −3.07 | 0.054 | * | |
| Industry−FE | ✓ | ||||
| Year−FE | ✓ | ||||
| (d): (constructed on puts and CDSs) regressed onto . | |||||
| Regressand | Adj-: | 0.5326 | |||
| Regressors | Coefficient | Robust Standard Error | t-stat | p-value | |
| 0.0236 | 0.0019 | 12.41 | 0.001 | *** | |
| −0.0070 | 0.0023 | −2.98 | 0.059 | * | |
| Industry−FE | ✓ | ||||
| Year−FE | ✓ | ||||
| (a): Extra-information provided by CDSs when calls are used to infer credit risk. | |||||
| Regressand | Adj-: | 0.3948 | |||
| Regressors | Coefficient | Robust Standard Error | t-stat | p-value | |
| 0.0064 | 0.0014 | 4.74 | 0.018 | ** | |
| −0.0002 | 0.0122 | −0.17 | 0.875 | ||
| Industry−FE | ✓ | ||||
| Year−FE | ✓ | ||||
| (b): Extra-information provided by CDSs when puts are used to infer credit risk. | |||||
| Regressand | Adj-: | 0.0557 | |||
| Regressors | Coefficient | Robust Standard Error | t-stat | p-value | |
| 0.0024 | 0.0008 | 2.98 | 0.058 | * | |
| −0.0018 | 0.0009 | −1.90 | 0.153 | ||
| Industry−FE | ✓ | ||||
| Year−FE | ✓ | ||||
| (a): Financials | |||||
| Regressand | Adj-: | 0.5205 | |||
| Regressors | Coefficient | Robust Standard Error | t-stat | p-value | |
| 0.0255 | 0.0008 | 32.20 | 0.000 | *** | |
| −0.0092 | 0.0012 | −7.50 | 0.000 | *** | |
| Year−FE | ✓ | ||||
| (b): Mining, Energy and Utilities | |||||
| Regressand | Adj-: | 0.3916 | |||
| Regressors | Coefficient | Robust Standard Error | t-stat | p-value | |
| 0.0200 | 0.0013 | 15.72 | 0.000 | *** | |
| −0.0013 | 0.0005 | −2.69 | 0.007 | *** | |
| Year−FE | ✓ | ||||
| (c): Manufacturing | |||||
| Regressand | Adj-: | 0.3541 | |||
| Regressors | Coefficient | Robust Standard Error | t-stat | p-value | |
| 0.0249 | 0.0031 | 8.08 | 0.000 | *** | |
| −0.0040 | 0.0006 | −6.87 | 0.000 | *** | |
| Year−FE | ✓ | ||||
| (d): Retail, Wholesale and Services | |||||
| Regressand | Adj-: | 0.1081 | |||
| Regressors | Coefficient | Robust Standard Error | t-stat | p-value | |
| 0.0009 | 0.0001 | 14.38 | 0.000 | *** | |
| 0.0001 | 0.00002 | 4.91 | 0.000 | *** | |
| Year−FE | ✓ | ||||
| Financials | Energy and Utilities | Manufacturing | Sales and Services | |
|---|---|---|---|---|
| 1.3436 | 0.4478 | 0.2237 | 0.2286 | |
| 0.0265 | 0.0072 | 0.0008 | 0.0033 |
| (a): Predictive regression for short-term skew. | |||||
| Regressand | (between): | 0.0036 | |||
| (within): | 0.0004 | ||||
| Regressors | Coefficient | Robust Standard Error | t-stat | p-value | |
| −0.4308 | 0.9730 | −0.44 | 0.659 | ||
| −0.0035 | 0.0009 | −3.81 | 0.000 | *** | |
| firm−FE | ✓ | ||||
| year−FE | ✓ | ||||
| (b): Predictive regression for long-term skew. | |||||
| Regressand | (between): | 0.3096 | |||
| (within): | 0.0004 | ||||
| Regressors | Coefficient | Robust Standard Error | t-stat | p-value | |
| 0.3360 | 0.0956 | 3.51 | 0.001 | *** | |
| −0.0108 | 0.0017 | −6.36 | 0.000 | *** | |
| firm−FE | ✓ | ||||
| year−FE | ✓ | ||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Maglione, F.; Mancino, M.E. Assessing the Impact of Credit Risk on Equity Options via Information Contents and Compound Options. Risks 2023, 11, 183. https://doi.org/10.3390/risks11100183
Maglione F, Mancino ME. Assessing the Impact of Credit Risk on Equity Options via Information Contents and Compound Options. Risks. 2023; 11(10):183. https://doi.org/10.3390/risks11100183
Chicago/Turabian StyleMaglione, Federico, and Maria Elvira Mancino. 2023. "Assessing the Impact of Credit Risk on Equity Options via Information Contents and Compound Options" Risks 11, no. 10: 183. https://doi.org/10.3390/risks11100183
APA StyleMaglione, F., & Mancino, M. E. (2023). Assessing the Impact of Credit Risk on Equity Options via Information Contents and Compound Options. Risks, 11(10), 183. https://doi.org/10.3390/risks11100183







