Bivariate Copulas Based on Counter-Monotonic Shock Method
Abstract
1. Introduction
2. Proposed Family of Copulas
2.1. The Model
- 1.
- and are counter-monotonic; that is, and .
- 2.
- and U are independent.
2.2. New Approach-Based Copula
- Generate three independent values , and from uniform [0, 1].
- Set and .
- The desired pair is .
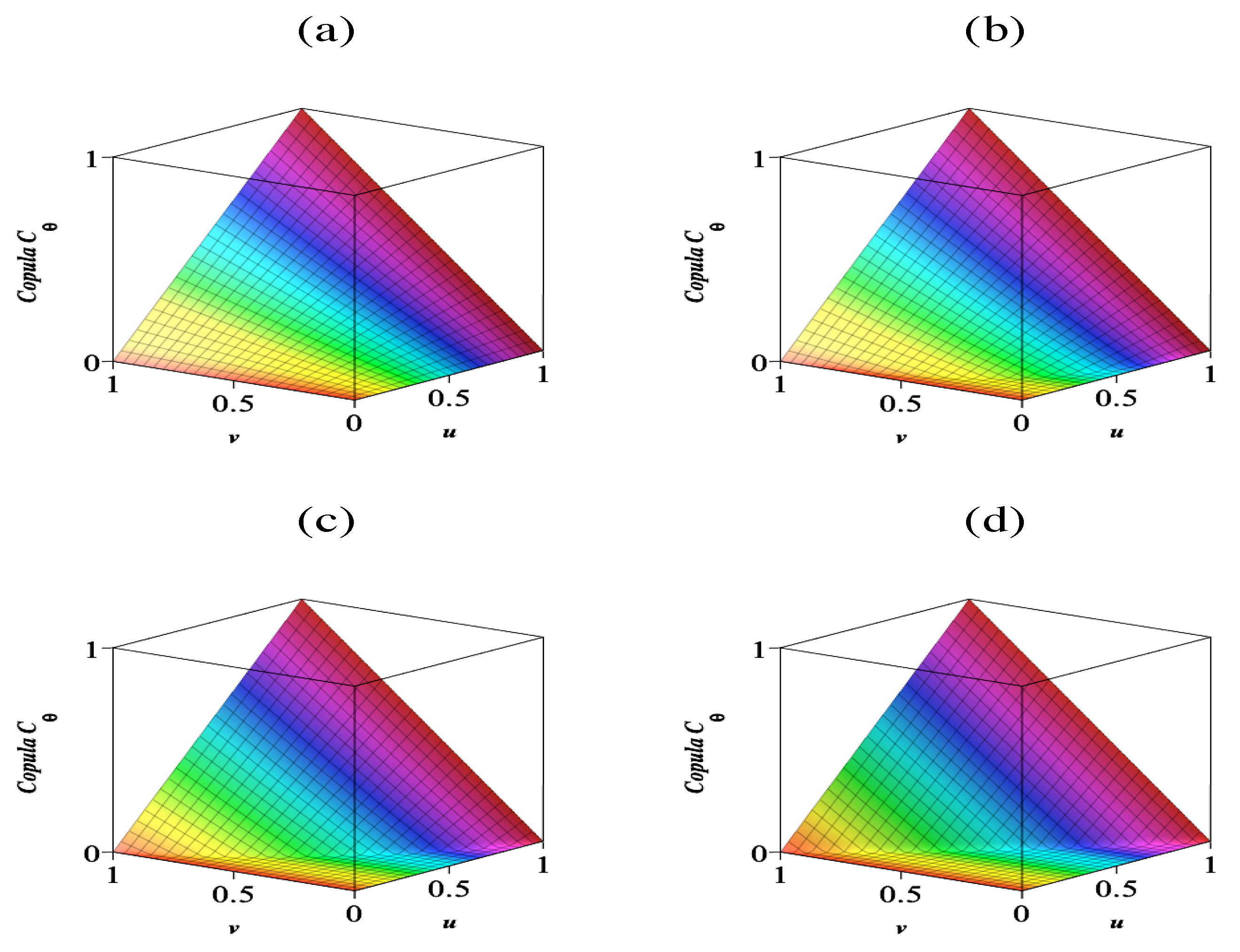
3. Properties of the Copula
3.1. Singularity
3.2. Density Function Corresponding to
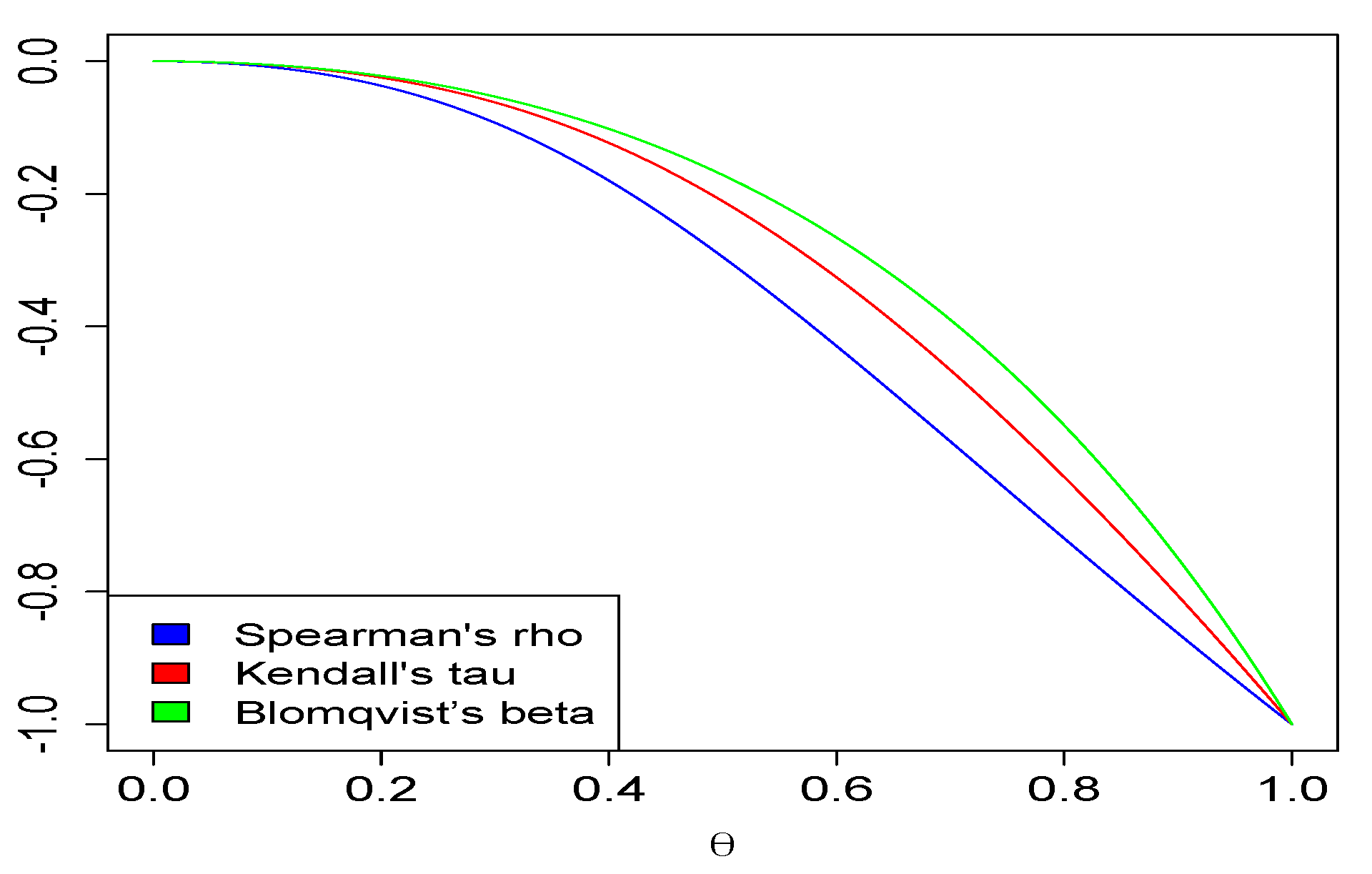
3.3. Concordance Measures of
3.4. Convexity Properties of
3.5. Mixed Moment of
4. Parameter Estimation
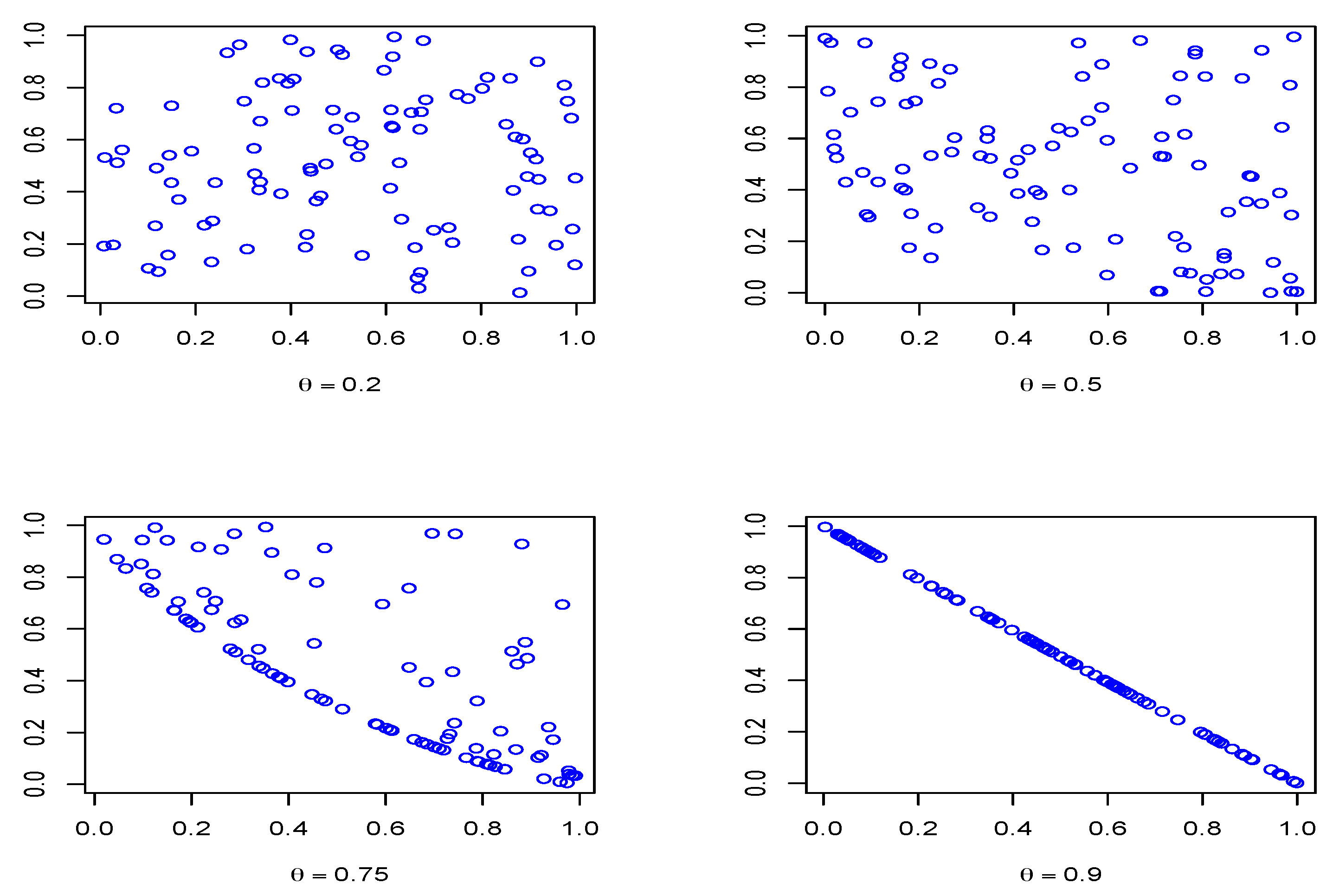
5. Simulation Study
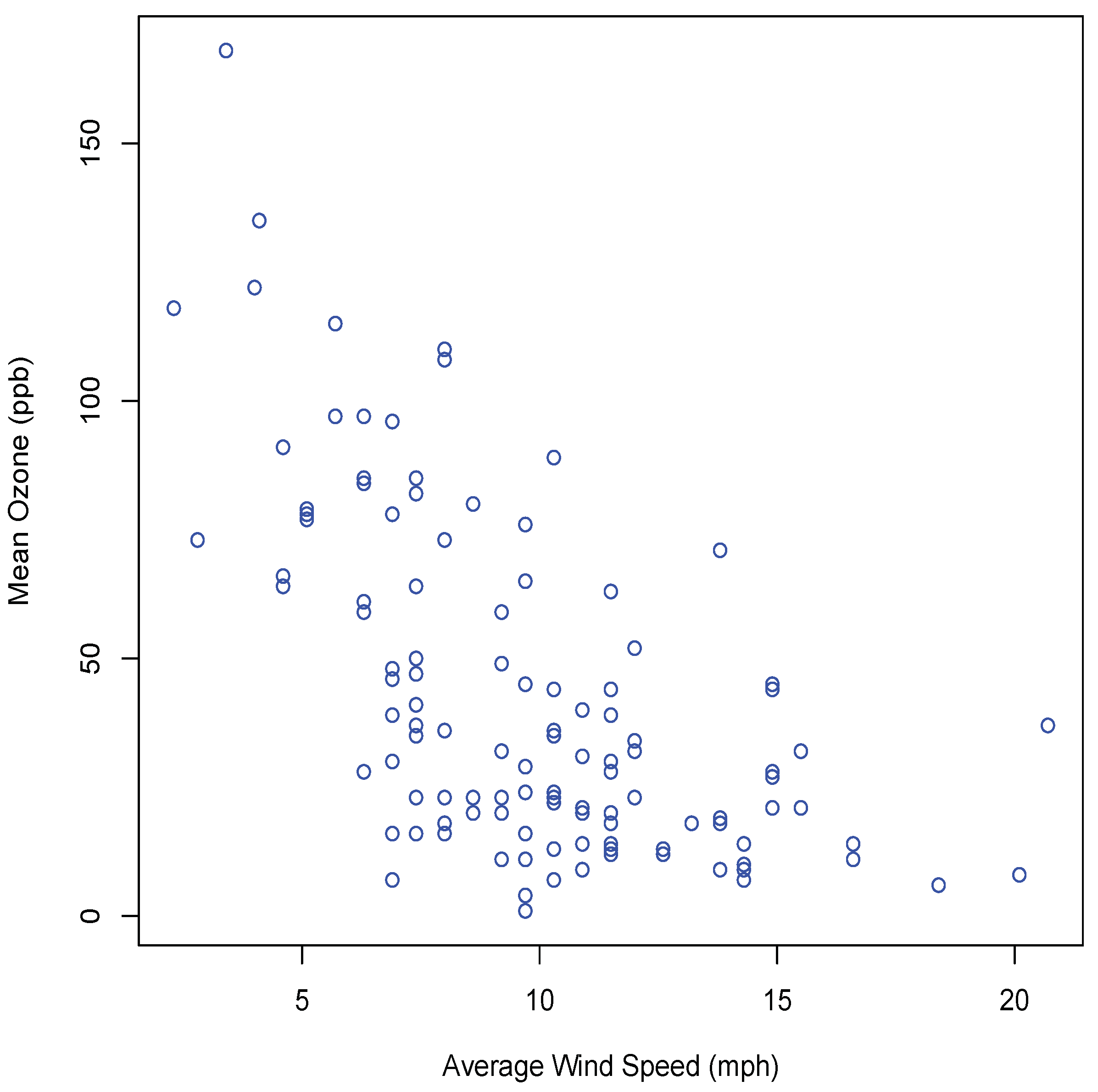
6. Real Data Study
7. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Almongy, Hisham Mohamed, Ehab Mohamed Almetwally, and Amaal Elsayed Mubarak. 2021. Marshall–Olkin Alpha Power Lomax Distribution: Estimation Methods, Applications on Physics and Economics. Pakistan Journal of Statistics and Operation Research 17: 137–53. [Google Scholar] [CrossRef]
- Bentoumi, Rachid, Farid El Ktaibi, and Mhamed Mesfioui. 2021. A New Family of Bivariate Exponential Distributions with Negative Dependence Based on Counter-Monotonic Shock Method. Entropy 23: 548. [Google Scholar] [CrossRef] [PubMed]
- Cuadras, Carles M., and Joan Augé. 1981. A continuous general multivariate distribution and its properties. Communications in Statistics-Theory and Methods 10: 339–53. [Google Scholar] [CrossRef]
- Chambers, John M., William S. Cleveland, Beat Kleiner, and Paul A. Tukey. 1983. Graphical Methods for Data Analysis. London: Taylor & Francis/CRC. [Google Scholar]
- Capéraà, Philippe, and Christian Genest. 1993. Spearman’s is larger than Kendall’s for positively dependent random variables. Journal of Nonparametric Statistics 2: 183–94. [Google Scholar] [CrossRef]
- Dolati, Ali, Soheyla Mohseni, and Manuel Úbeda-Flores. 2014. Some results on a transformation of copulas and quasi-copulas. Information Sciences 257: 176–82. [Google Scholar] [CrossRef]
- Durante, Fabrizio. 2009. Construction of non-exchangeable bivariate distribution functions. Statistical Papers 50: 383–91. [Google Scholar] [CrossRef]
- El-Morshedy, Mahmoud, Ziyad Ali Alhussain, Doaa Atta, Ehab M. Almetwally, and Mohammed. S. Eliwa. 2020. Bivariate Burr X Generator of Distributions: Properties and Estimation Methods with Applications to Complete and Type-II Censored Samples. Mathematics 14: 264. [Google Scholar] [CrossRef]
- Eliwa, Mohammed. S., and Mahmoud El-Morshedy. 2020. Bivariate odd Weibull-G family of distributions: Properties, Bayesian and non-Bayesian estimation with bootstrap confidence intervals and application. Journal of Taibah University for Science 8: 331–45. [Google Scholar] [CrossRef]
- Ferguson, Thomas S. 1996. A Course in Large Sample Theory. London: Chapman & Hall/CRC. [Google Scholar]
- Fredricks, Gregory A., and Roger B. Nelsen. 2007. On the Relationship between Spearman’s Rho and Kendall’s Tau for Pairs of Continuous Random Variables. Journal of Statistical Planning and Inference 137: 2143–50. [Google Scholar] [CrossRef]
- Genest, Christian, Bruno Rémillard, and David Beaudoin. 2009. Goodness-of-fit tests for copulas: A review and a power study. Insurance: Mathematics and Economics 44: 199–213. [Google Scholar] [CrossRef]
- Genest, Christian, Mhamed Mesfioui, and Juliana Schulz. 2018. A new bivariate Poisson common shock model covering all possible degrees of dependence. Statistics and Probability Letters 140: 202–9. [Google Scholar] [CrossRef]
- Haj Ahmad, Hanan, and Ehab M. Almetwally. 2020. Marshall–Olkin Generalized Pareto Distribution: Bayesian and Non Bayesian Estimation. Pakistan Journal of Statistics and Operation Research 16: 21–33. [Google Scholar] [CrossRef]
- Khoudraji, Abdelhaq. 1995. Contributions à l’étude des Copules et à la Modélisation des Valeurs Extrêmes Bivariées. Ph.D. thesis, Université de Laval, Québec, QC, Canada. [Google Scholar]
- Kole, Erik, Kees Koedijk, and Marno Verbeek. 2007. Selecting copulas for risk management. Journal of Banking & Finance 31: 2405–23. [Google Scholar]
- Lehmann, Erich Leo. 1966. Some concepts of dependence. The Annals of Mathematical Statistics 37: 1137–53. [Google Scholar] [CrossRef]
- Liebscher, Eckhard. 2008. Construction of asymmetric multivariate copulas. Journal of Multivariate Analysis 99: 2234–50. [Google Scholar] [CrossRef]
- Marshall, Albert W., and Ingram Olkin. 1967. A multivariate exponential distribution. Journal of the American Statistical Association 62: 30–44. [Google Scholar] [CrossRef]
- Mesfioui, Mhamed, and Abdelouahid Tajar. 2005. On the properties of some nonparametric concordance measures in the discrete case. Nonparametric Statistics 17: 541–54. [Google Scholar] [CrossRef]
- Nelsen, Roger B. 2006. An Introduction to Copulas. New York: Springer. [Google Scholar]
- Ruiz-Rivas, Carmen, and Carles M. Cuadras. 1988. Inference properties of a one-parameter curved exponential family of distributions with given marginals. Journal of Multivariate Analysis 27: 447–56. [Google Scholar] [CrossRef]


| n | Bias() | MSE() | 95% CI | |||
|---|---|---|---|---|---|---|
| 50 | ||||||
| 100 | ||||||
| 200 | ||||||
| 300 | ||||||
| 400 | ||||||
| 50 | ||||||
| 100 | ||||||
| 200 | ||||||
| 300 | ||||||
| 400 | ||||||
| 50 | ||||||
| 100 | ||||||
| 200 | ||||||
| 300 | ||||||
| 400 | ||||||
| 50 | ||||||
| 100 | ||||||
| 200 | ||||||
| 300 | ||||||
| 400 | ||||||
| 50 | ||||||
| 100 | ||||||
| 200 | ||||||
| 300 | ||||||
| 400 | ||||||
| 50 | ||||||
| 100 | ||||||
| 200 | ||||||
| 300 | ||||||
| 400 | ||||||
| 50 | ||||||
| 100 | ||||||
| 200 | ||||||
| 300 | ||||||
| 400 | ||||||
| 50 | ||||||
| 100 | ||||||
| 200 | ||||||
| 300 | ||||||
| 400 | ||||||
| 50 | ||||||
| 100 | ||||||
| 200 | ||||||
| 300 | ||||||
| 400 |
| Weibull | Lognormal | Gamma | Beta4 | GEVD | |
|---|---|---|---|---|---|
| AIC | 625.408 | 631.862 | 624.490 | 626.947 | 624.942 |
| BIC | 630.916 | 637.370 | 629.997 | 637.961 | 633.203 |
| Weibull | Lognormal | Gamma | Beta4 | GEVD | |
|---|---|---|---|---|---|
| AIC | 1089.221 | 1091.766 | 1087.075 | 1090.803 | 1093.552 |
| BIC | 1094.728 | 1097.273 | 1092.583 | 1101.817 | 1101.813 |
| Average Wind | Mean Ozone Level | |||
|---|---|---|---|---|
| KS | AD | KS | AD | |
| Test statistic | 0.073 | 0.481 | 0.080 | 0.738 |
| p-value | 0.537 | 0.766 | 0.420 | 0.527 |
| Shape | 7.17 | 1.70 | ||
| Scale | 1.375 | 24.770 | ||
| KS | Cramér–von Mises | |
|---|---|---|
| Test statistic | ||
| p-value | 0.584 | 0.219 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
El Ktaibi, F.; Bentoumi, R.; Sottocornola, N.; Mesfioui, M. Bivariate Copulas Based on Counter-Monotonic Shock Method. Risks 2022, 10, 202. https://doi.org/10.3390/risks10110202
El Ktaibi F, Bentoumi R, Sottocornola N, Mesfioui M. Bivariate Copulas Based on Counter-Monotonic Shock Method. Risks. 2022; 10(11):202. https://doi.org/10.3390/risks10110202
Chicago/Turabian StyleEl Ktaibi, Farid, Rachid Bentoumi, Nicola Sottocornola, and Mhamed Mesfioui. 2022. "Bivariate Copulas Based on Counter-Monotonic Shock Method" Risks 10, no. 11: 202. https://doi.org/10.3390/risks10110202
APA StyleEl Ktaibi, F., Bentoumi, R., Sottocornola, N., & Mesfioui, M. (2022). Bivariate Copulas Based on Counter-Monotonic Shock Method. Risks, 10(11), 202. https://doi.org/10.3390/risks10110202






