Angiogenesis Dynamics: A Computational Model of Intravascular Flow Within a Structural Adaptive Vascular Network
Abstract
1. Introduction
2. Materials and Methods
2.1. Numerical Model of Angiogenesis
2.2. Hemodynamics and Intravascular Flow
2.3. Capillary Structure Adaptation
2.4. In Vivo Angiogenesis
2.5. Computational Implementation
3. Results and Discussion
3.1. Angiogenesis Network
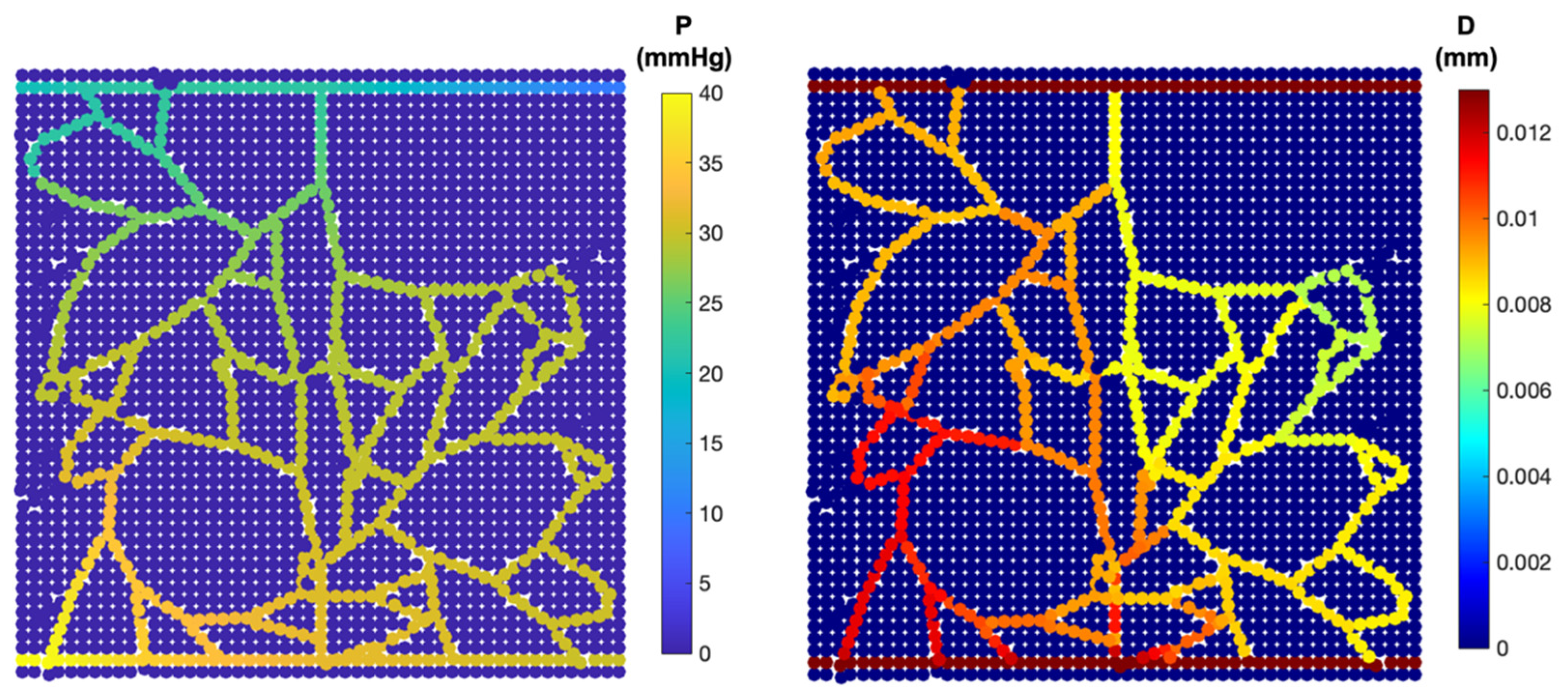
3.2. Intravascular Flow
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hamm, R. Failure of Wounds to Heal. In Diagnosis for Physical Therapists: A Symptom-Based Approach; Davenport, T.E., Kulig, K., Sebelski, C.A., Gordon, J., Watts, H.G., Eds.; McGraw-Hill Education: New York, NY, USA, 2013. [Google Scholar]
- Tonnesen, M.G.; Feng, X.; Clark, R.A. Angiogenesis in wound healing. J. Investig. Dermatol. Symp. Proc. 2000, 5, 40–46. [Google Scholar] [CrossRef] [PubMed]
- Costa, C.; Incio, J.; Soares, R. Angiogenesis and chronic inflammation: Cause or consequence? Angiogenesis 2007, 10, 149–166. [Google Scholar] [CrossRef] [PubMed]
- Martin, A.; Komada, M.R.; Sane, D.C. Abnormal angiogenesis in diabetes mellitus. Med. Res. Rev. 2003, 23, 117–145. [Google Scholar] [CrossRef] [PubMed]
- Szekanecz, Z.; Besenyei, T.; Paragh, G.; Koch, A.E. Angiogenesis in rheumatoid arthritis. Autoimmunity 2009, 42, 563–573. [Google Scholar] [CrossRef]
- Carmeliet, P.; Jain, R.K. Angiogenesis in cancer and other diseases. Nature 2000, 407, 249–257. [Google Scholar] [CrossRef]
- Del Amo, C.; Borau, C.; Gutiérrez, R.; Asín, J.; García-Aznar, J.M. Quantification of angiogenic sprouting under different growth factors in a microfluidic platform. J. Biomech. 2016, 49, 1340–1346. [Google Scholar] [CrossRef]
- Ferrara, N.; Gerber, H.P.; LeCouter, J. The biology of VEGF and its receptors. Nat. Med. 2003, 9, 669–676. [Google Scholar] [CrossRef]
- Chen, T.T.; Luque, A.; Lee, S.; Anderson, S.M.; Segura, T.; Iruela-Arispe, M.L. Anchorage of VEGF to the extracellular matrix conveys differential signaling responses to endothelial cells. J. Cell Biol. 2010, 188, 595–609. [Google Scholar] [CrossRef]
- Johnson, K.E.; Wilgus, T.A. Vascular Endothelial Growth Factor and Angiogenesis in the Regulation of Cutaneous Wound Repair. Adv. Wound Care 2014, 3, 647–661. [Google Scholar] [CrossRef]
- Nishida, N.; Yano, H.; Nishida, T.; Kamura, T.; Kojiro, M. Angiogenesis in cancer. Vasc. Health Risk Manag. 2006, 2, 213–219. [Google Scholar] [CrossRef]
- Hellström, M.; Phng, L.-K.; Hofmann, J.J.; Wallgard, E.; Coultas, L.; Lindblom, P.; Alva, J.; Nilsson, A.-K.; Karlsson, L.; Gaiano, N.; et al. Dll4 signalling through Notch1 regulates formation of tip cells during angiogenesis. Nature 2007, 445, 776–780. [Google Scholar] [CrossRef] [PubMed]
- Arima, S.; Nishiyama, K.; Ko, T.; Arima, Y.; Hakozaki, Y.; Sugihara, K.; Koseki, H.; Uchijima, Y.; Kurihara, Y.; Kurihara, H. Angiogenic morphogenesis driven by dynamic and heterogeneous collective endothelial cell movement. Development 2011, 138, 4763–4776. [Google Scholar] [CrossRef] [PubMed]
- Jakobsson, L.; Franco, C.A.; Bentley, K.; Collins, R.T.; Ponsioen, B.; Aspalter, I.M.; Rosewell, I.; Busse, M.; Thurston, G.; Medvinsky, A.; et al. Endothelial cells dynamically compete for the tip cell position during angiogenic sprouting. Nat. Cell Biol. 2010, 12, 943–953. [Google Scholar] [CrossRef]
- Fleury, V.; Schwartz, L. Modelisation of 3-D microvasculature by interlaced diffusion limited aggregation. Fractals 2000, 8, 255–259. [Google Scholar] [CrossRef]
- Chapman, S.C.; Collignon, J.; Schoenwolf, G.C.; Lumsden, A. Improved method for chick whole-embryo culture using a filter paper carrier. Dev. Dyn. 2001, 220, 284–289. [Google Scholar] [CrossRef]
- Maibier, M.; Reglin, B.; Nitzsche, B.; Xiang, W.; Rong, W.W.; Hoffmann, B.; Djonov, V.; Secomb, T.W.; Pries, A.R. Structure and hemodynamics of vascular networks in the chorioallantoic membrane of the chicken. Am. J. Physiol. Circ. Physiol. 2016, 311, H913–H926. [Google Scholar] [CrossRef]
- Richard, S.; Brun, A.; Tedesco, A.; Gallois, B.; Taghi, N.; Dantan, P.; Seguin, J.; Fleury, V. Direct imaging of capillaries reveals the mechanism of arteriovenous interlacing in the chick chorioallantoic membrane. Commun. Biol. 2018, 1, 235. [Google Scholar] [CrossRef]
- Pries, A.R.; Reglin, B.; Secomb, T.W. Structural adaptation of microvascular networks: Functional roles of adaptive responses. Am. J. Physiol. Circ. Physiol. 2001, 281, H1015–H1025. [Google Scholar] [CrossRef]
- Pries, A.R.; Secomb, T.W. Making microvascular networks work: Angiogenesis, remodeling, and pruning. Physiology 2014, 29, 446–455. [Google Scholar] [CrossRef]
- Delp, M.D.; Colleran, P.N.; Wilkerson, M.K.; McCurdy, M.R.; Muller-Delp, J. Structural and functional remodeling of skeletal muscle microvasculature is induced by simulated microgravity. Am. J. Physiol. Circ. Physiol. 2000, 278, H1866–H1873. [Google Scholar] [CrossRef]
- Stéphanou, A.; McDougall, S.R.; Anderson, A.R.A.; Chaplain, M.A.J. Mathematical modelling of the influence of blood rheological properties upon adaptative tumour-induced angiogenesis. Math. Comput. Model. 2006, 44, 96–123. [Google Scholar] [CrossRef]
- Chaplain, M.A.; Anderson, A.R. Mathematical modelling, simulation and prediction of tumour-induced angiogenesis. Invasion Metastasis 1996, 16, 222–234. [Google Scholar] [PubMed]
- Levine, H.A.; Pamuk, S.; Sleeman, B.D.; Nilsen-Hamilton, M. Mathematical modeling of capillary formation and development in tumor angiogenesis: Penetration into the stroma. Bull. Math. Biol. 2001, 63, 801–863. [Google Scholar] [CrossRef]
- Plank, M.J.; Sleeman, B.D.; Jones, P.F. A mathematical model of tumour angiogenesis, regulated by vascular endothelial growth factor and the angiopoietins. J. Theor. Biol. 2004, 229, 435–454. [Google Scholar] [CrossRef]
- Anderson, A.R.; Chaplain, M.A. Continuous and discrete mathematical models of tumor-induced angiogenesis. Bull. Math. Biol. 1998, 60, 857–899. [Google Scholar] [CrossRef]
- Perfahl, H.; Byrne, H.; Chen, T.; Estrella Suarez, M.V.; Alarcon, T.; Lapin, A.; Gatenby, R.; Gillies, R.; Maini, P.; Reuss, M.; et al. Multiscale Modelling of Vascular Tumour Growth in 3D: The Roles of Domain Size and Boundary Conditions. PLoS ONE 2011, 6, e14790. [Google Scholar] [CrossRef]
- Stepanova, D.; Byrne, H.M.; Maini, P.K.; Alarcón, T. A multiscale model of complex endothelial cell dynamics in early angiogenesis. PLoS Comput. Biol. 2021, 17, e1008055. [Google Scholar] [CrossRef]
- Welter, M.; Rieger, H. Interstitial Fluid Flow and Drug Delivery in Vascularized Tumors: A Computational Model. PLoS ONE 2013, 8, e70395. [Google Scholar] [CrossRef]
- Santos-Oliveira, P.; Correia, A.; Rodrigues, T.; Ribeiro-Rodrigues, T.M.; Matafome, P.; Rodríguez-Manzaneque, J.C.; Seiça, R.; Girão, H.; Travasso, R.D.M. The Force at the Tip—Modelling Tension and Proliferation in Sprouting Angiogenesis. PLOS Comput. Biol. 2015, 11, e1004436. [Google Scholar] [CrossRef]
- Travasso, R.D.; Corvera Poire, E.; Castro, M.; Rodriguez-Manzaneque, J.C.; Hernandez-Machado, A. Tumor angiogenesis and vascular patterning: A mathematical model. PLoS ONE 2011, 6, e19989. [Google Scholar] [CrossRef]
- Das, A.; Lauffenburger, D.; Asada, H.; Kamm, R. A hybrid continuum-discrete modelling approach to predict and control angiogenesis: Analysis of combinatorial growth factor and matrix effects on vessel-sprouting morphology. Philos. Trans. Ser. A Math. Phys. Eng. Sci. 2010, 368, 2937–2960. [Google Scholar] [CrossRef] [PubMed]
- Milde, F.; Bergdorf, M.; Koumoutsakos, P. A Hybrid Model for Three-Dimensional Simulations of Sprouting Angiogenesis. Biophys. J. 2008, 95, 3146–3160. [Google Scholar] [CrossRef] [PubMed]
- Moreira-Soares, M.; Coimbra, R.; Rebelo, L.; Carvalho, J.; Travasso, R.D.M. Angiogenic Factors produced by Hypoxic Cells are a leading driver of Anastomoses in Sprouting Angiogenesis—A computational study. Sci. Rep. 2018, 8, 8726. [Google Scholar] [CrossRef] [PubMed]
- Jafari Nivlouei, S.; Soltani, M.; Carvalho, J.; Travasso, R.; Salimpour, M.R.; Shirani, E. Multiscale modeling of tumor growth and angiogenesis: Evaluation of tumor-targeted therapy. PLOS Comput. Biol. 2021, 17, e1009081. [Google Scholar] [CrossRef]
- Jafari Nivlouei, S.; Soltani, M.; Shirani, E.; Salimpour, M.R.; Travasso, R.; Carvalho, J. A multiscale cell-based model of tumor growth for chemotherapy assessment and tumor-targeted therapy through a 3D computational approach. Cell Prolif. 2022, 55, e13187. [Google Scholar] [CrossRef]
- Anderson, A.R.A.; Chaplain, M.A.J.; McDougall, S. A Hybrid Discrete-Continuum Model of Tumour Induced Angiogenesis. In Modeling Tumor Vasculature: Molecular, Cellular, and Tissue Level Aspects and Implications; Jackson, T.L.L., Ed.; Springer: New York, NY, USA, 2012; pp. 105–133. [Google Scholar]
- Soltani, M. Capillary network formation and structure in a modified discrete mathematical model of angiogenesis. Biomed. Phys. Eng. Express 2021, 8, 015023. [Google Scholar] [CrossRef]
- Soltani, M.; Chen, P. Numerical Modeling of Interstitial Fluid Flow Coupled with Blood Flow through a Remodeled Solid Tumor Microvascular Network. PLoS ONE 2013, 8, e67025. [Google Scholar] [CrossRef]
- Guerra, A.; Belinha, J.; Natal Jorge, R. A preliminary study of endothelial cell migration during angiogenesis using a meshless method approach. Int. J. Numer. Methods Biomed. Eng. 2020, 36, e3393. [Google Scholar] [CrossRef]
- Guerra, A.; Belinha, J.; Mangir, N.; Macneil, S.; Natal Jorge, R. Simulation of the process of angiogenesis: Quantification and assessment of vascular patterning in the chicken chorioallantoic membrane. Comput. Biol. Med. 2021, 136, 104647. [Google Scholar] [CrossRef]
- Guerra, A.; Belinha, J.; Mangir, N.; MacNeil, S.; Natal Jorge, R. Sprouting Angiogenesis: A Numerical Approach with Experimental Validation. Ann. Biomed. Eng. 2020, 49, 871–884. [Google Scholar] [CrossRef]
- Dinis, L.M.J.S.; Natal Jorge, R.M.; Belinha, J. Analysis of 3D solids using the natural neighbour radial point interpolation method. Comput. Methods Appl. Mech. Eng. 2007, 196, 2009–2028. [Google Scholar] [CrossRef]
- Belinha, J.; Dinis, L.M.J.S.; Natal Jorge, R.M. The natural radial element method. Int. J. Numer. Methods Eng. 2013, 93, 1286–1313. [Google Scholar] [CrossRef]
- Belinha, J. Meshless Methods in Biomechanics—Bone Tissue Remodelling Analysis; Springer International Publishing: Cham, Switzerland, 2014. [Google Scholar]
- Price, R.J.; Skalak, T.C. Circumferential Wall Stress as a Mechanism for Arteriolar Rarefaction and Proliferation in a Network Model. Microvasc. Res. 1994, 47, 188–202. [Google Scholar] [CrossRef] [PubMed]
- Mangir, N.; Dikici, S.; Claeyssens, F.; MacNeil, S. Using ex Ovo Chick Chorioallantoic Membrane (CAM) Assay To Evaluate the Biocompatibility and Angiogenic Response to Biomaterials. ACS Biomater. Sci. Eng. 2019, 5, 3190–3200. [Google Scholar] [CrossRef]
- Mangır, N.; Eke, G.; Hasirci, N.; Chapple, C.R.; Hasirci, V.; MacNeil, S. An estradiol releasing, proangiogenic hydrogel as a candidate material for use in soft tissue interposition. Neurourol. Urodyn. 2019, 38, 1195–1202. [Google Scholar] [CrossRef]
- Vermolen, F.J.; Javierre, E. A finite-element model for healing of cutaneous wounds combining contraction, angiogenesis and closure. J. Math. Biol. 2011, 65, 967–996. [Google Scholar] [CrossRef]
- Karayiannakis, A.J.; Zbar, A.; Polychronidis, A.; Simopoulos, C. Serum and drainage fluid vascular endothelial growth factor levels in early surgical wounds. Eur. Surg. Res. 2003, 35, 492–496. [Google Scholar] [CrossRef]
- Kamei, M.; Brian Saunders, W.; Bayless, K.J.; Dye, L.; Davis, G.E.; Weinstein, B.M. Endothelial tubes assemble from intracellular vacuoles in vivo. Nature 2006, 442, 453–456. [Google Scholar] [CrossRef]

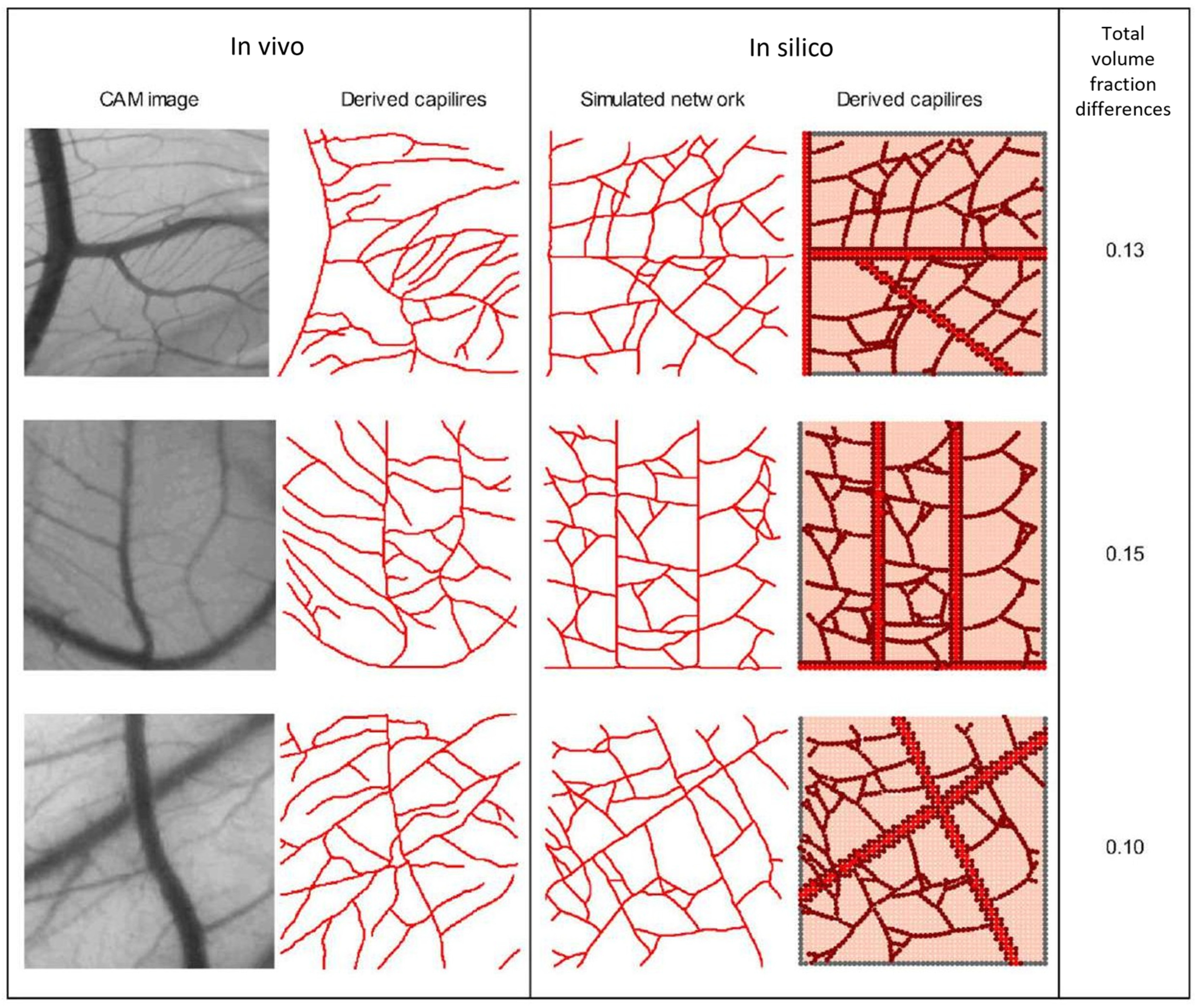
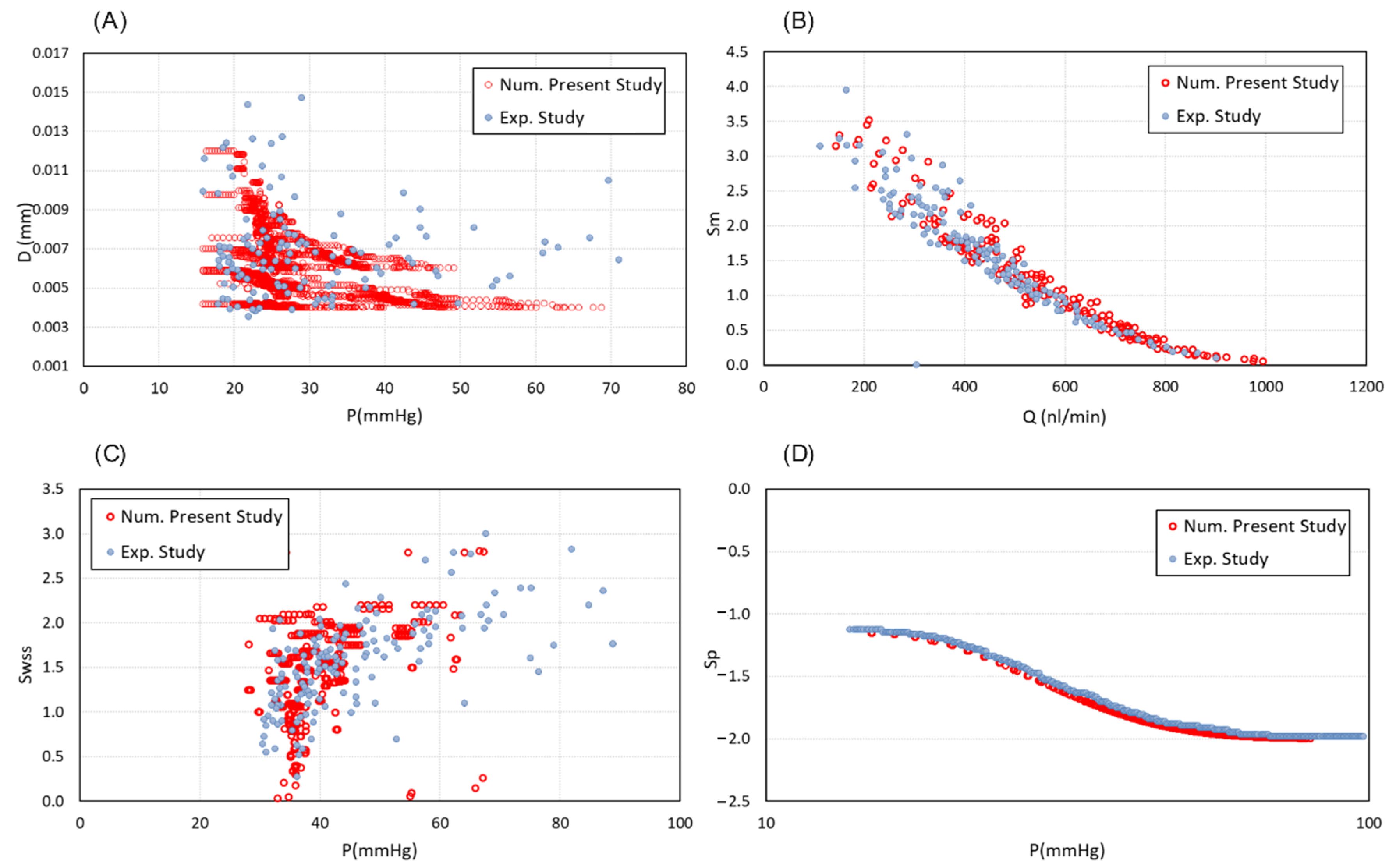
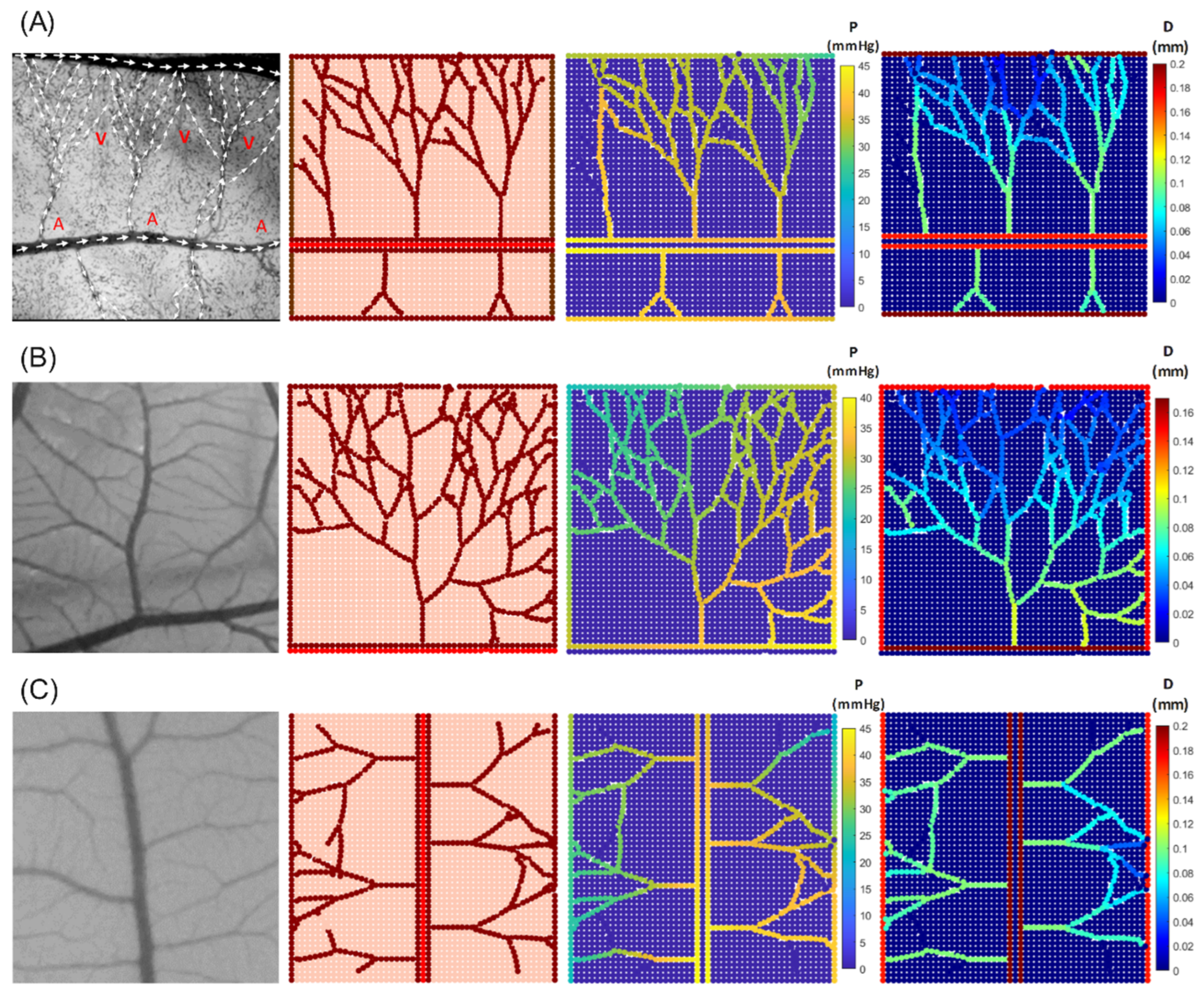
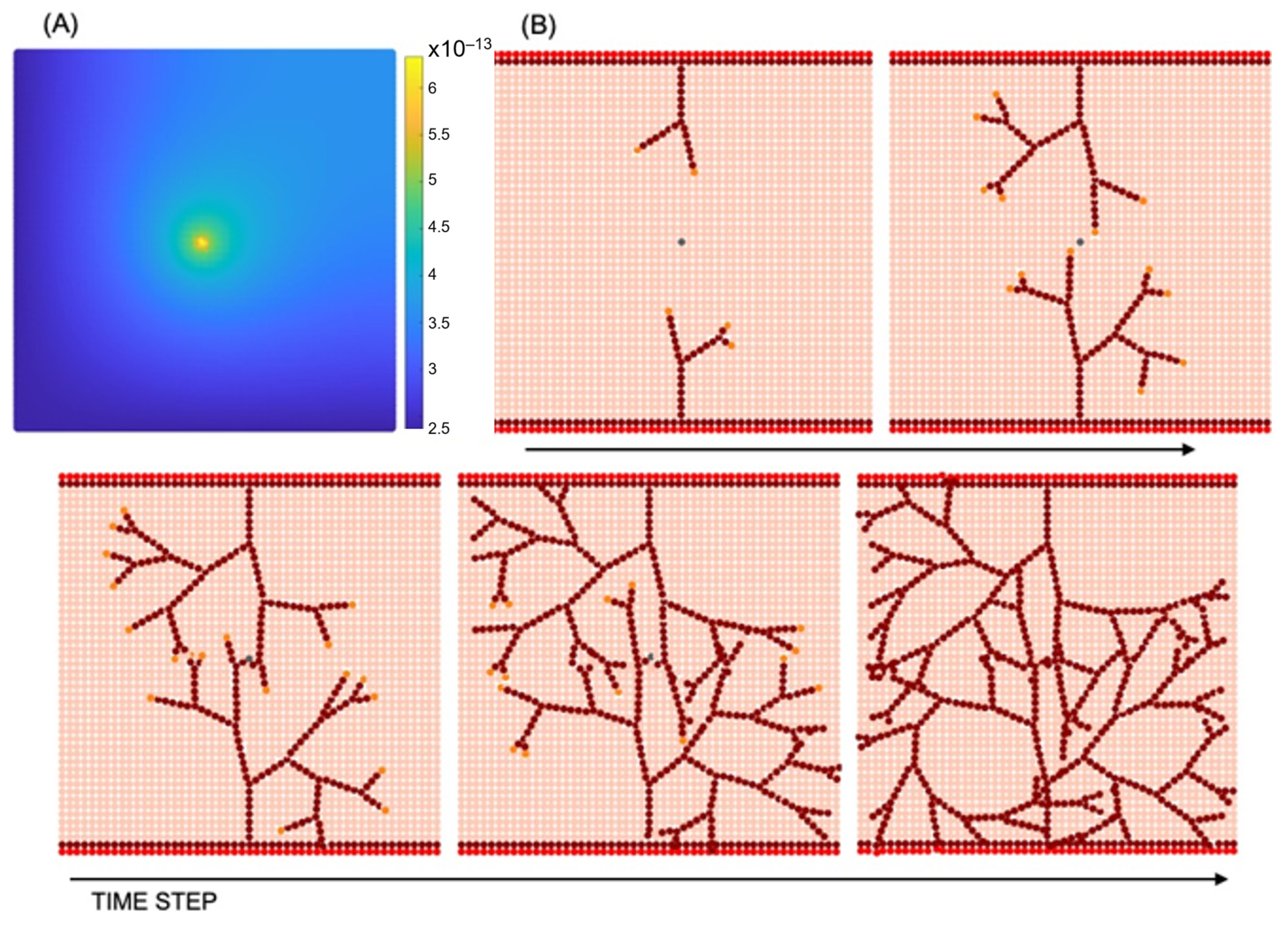

| Parameters | Description | Value | References |
|---|---|---|---|
| Angiogenesis | |||
| VEGF diffusion coefficient | mm2 s−1 | [49,50] | |
| VEGF release | g mm−3 | [40,42] | |
| Capillary network adaption | |||
| Reference value for shear stress | [19] | ||
| Scaling parameter for sensitivity to intravascular pressure | 0.68 ± 0.04 | [19] | |
| Scaling parameter for sensitivity to metabolic signal | 0.7 ± 0.06 | [19] | |
| Basal shrinking tendency coefficient | 1.72 ± 0.15 | [19] | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nivlouei, S.J.; Guerra, A.; Belinha, J.; Mangir, N.; MacNeil, S.; Salgado, C.; Monteiro, F.J.; Natal Jorge, R. Angiogenesis Dynamics: A Computational Model of Intravascular Flow Within a Structural Adaptive Vascular Network. Biomedicines 2024, 12, 2845. https://doi.org/10.3390/biomedicines12122845
Nivlouei SJ, Guerra A, Belinha J, Mangir N, MacNeil S, Salgado C, Monteiro FJ, Natal Jorge R. Angiogenesis Dynamics: A Computational Model of Intravascular Flow Within a Structural Adaptive Vascular Network. Biomedicines. 2024; 12(12):2845. https://doi.org/10.3390/biomedicines12122845
Chicago/Turabian StyleNivlouei, Sahar Jafari, Ana Guerra, Jorge Belinha, Naside Mangir, Sheila MacNeil, Christiane Salgado, Fernando Jorge Monteiro, and Renato Natal Jorge. 2024. "Angiogenesis Dynamics: A Computational Model of Intravascular Flow Within a Structural Adaptive Vascular Network" Biomedicines 12, no. 12: 2845. https://doi.org/10.3390/biomedicines12122845
APA StyleNivlouei, S. J., Guerra, A., Belinha, J., Mangir, N., MacNeil, S., Salgado, C., Monteiro, F. J., & Natal Jorge, R. (2024). Angiogenesis Dynamics: A Computational Model of Intravascular Flow Within a Structural Adaptive Vascular Network. Biomedicines, 12(12), 2845. https://doi.org/10.3390/biomedicines12122845









