Aortic Remodeling Kinetics in Response to Coarctation-Induced Mechanical Perturbations
Abstract
1. Introduction
2. Material and Methods
2.1. Stress-Mediated G&R Formulation
2.2. Strain Energy Density Function for Vessel Constituents
2.3. Simulation of Stress Mediated G&R
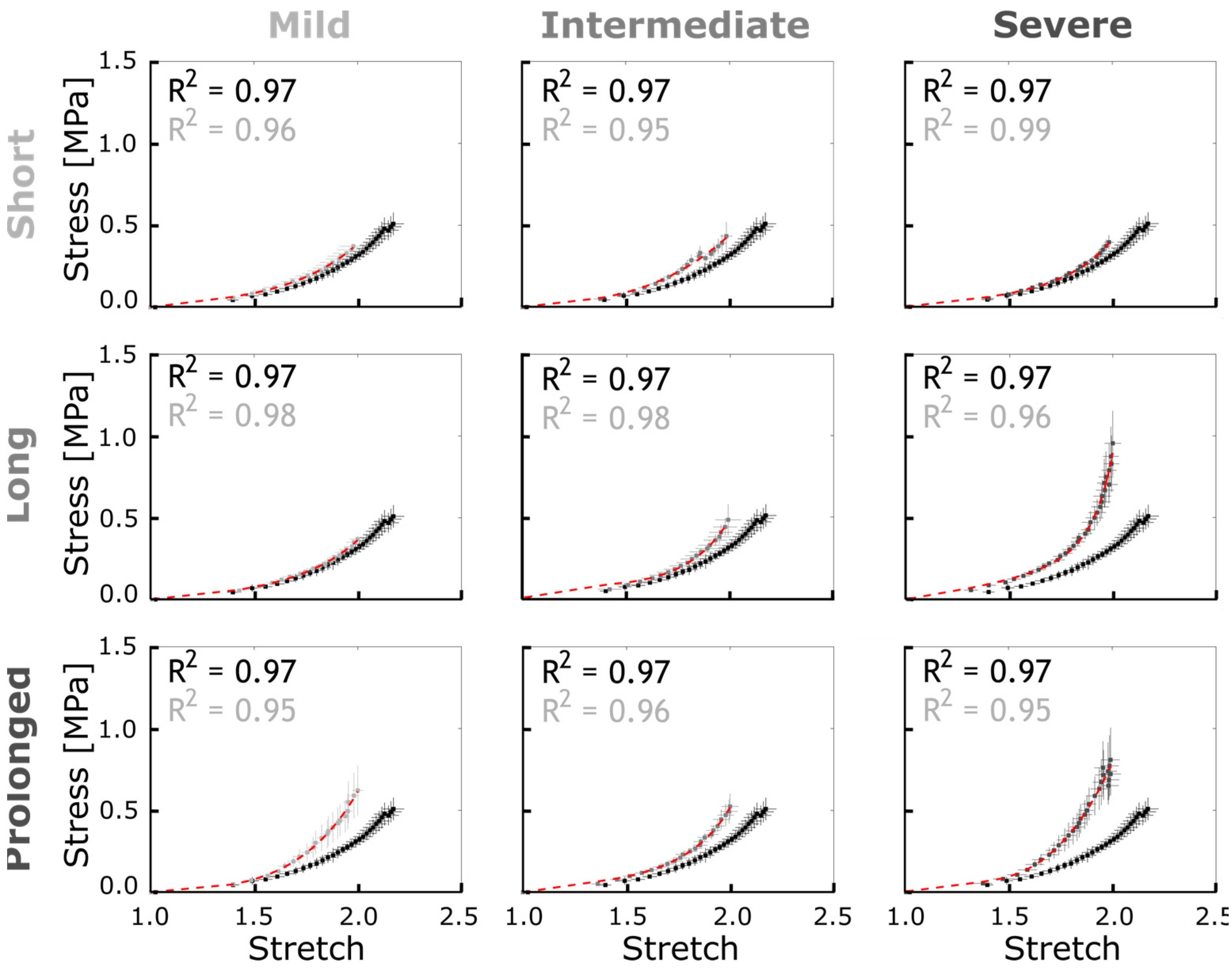
2.4. G&R Model Fitting
2.5. Empirical Quantification of Hypertension Precursors
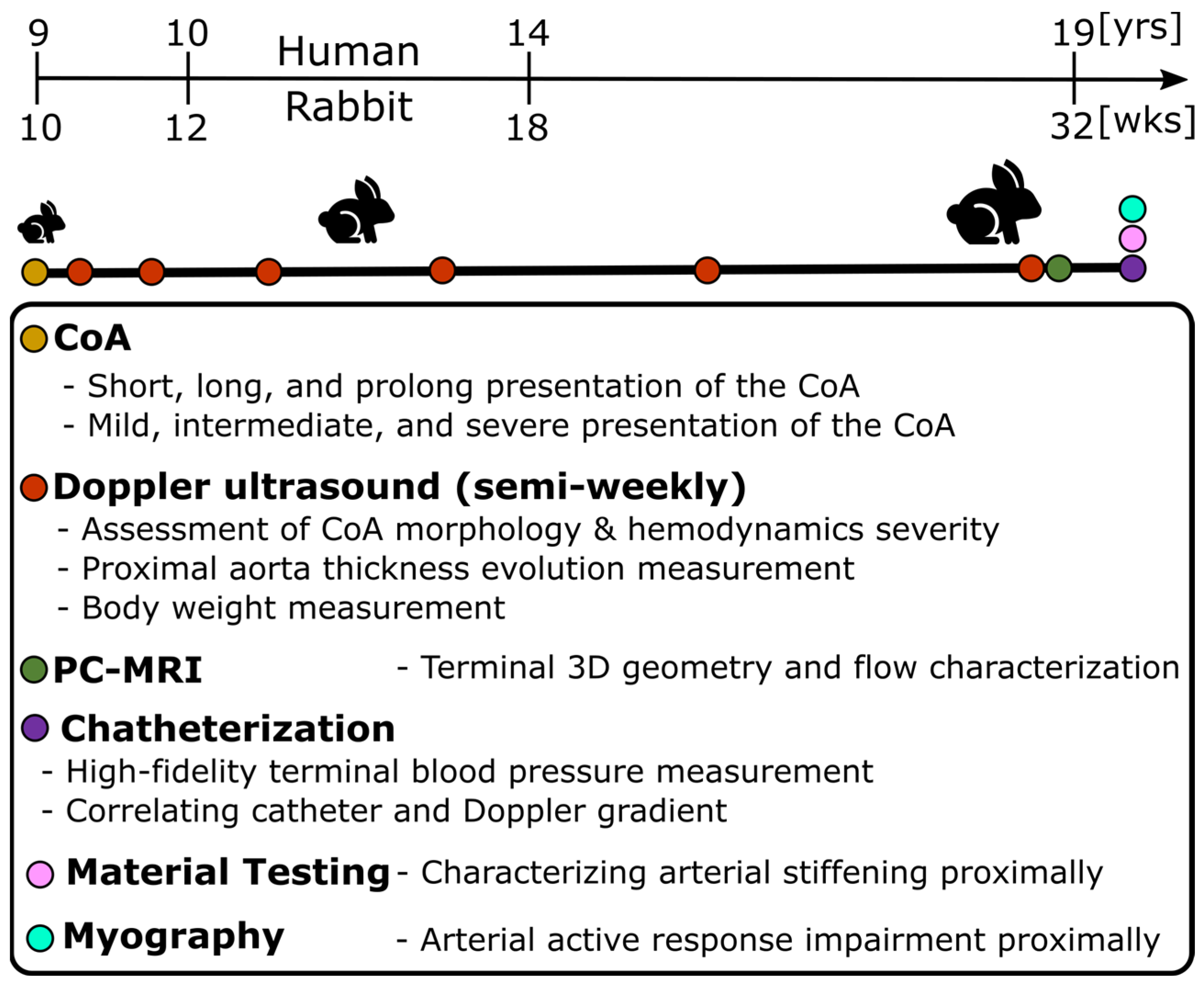
2.5.1. Experimental Protocol
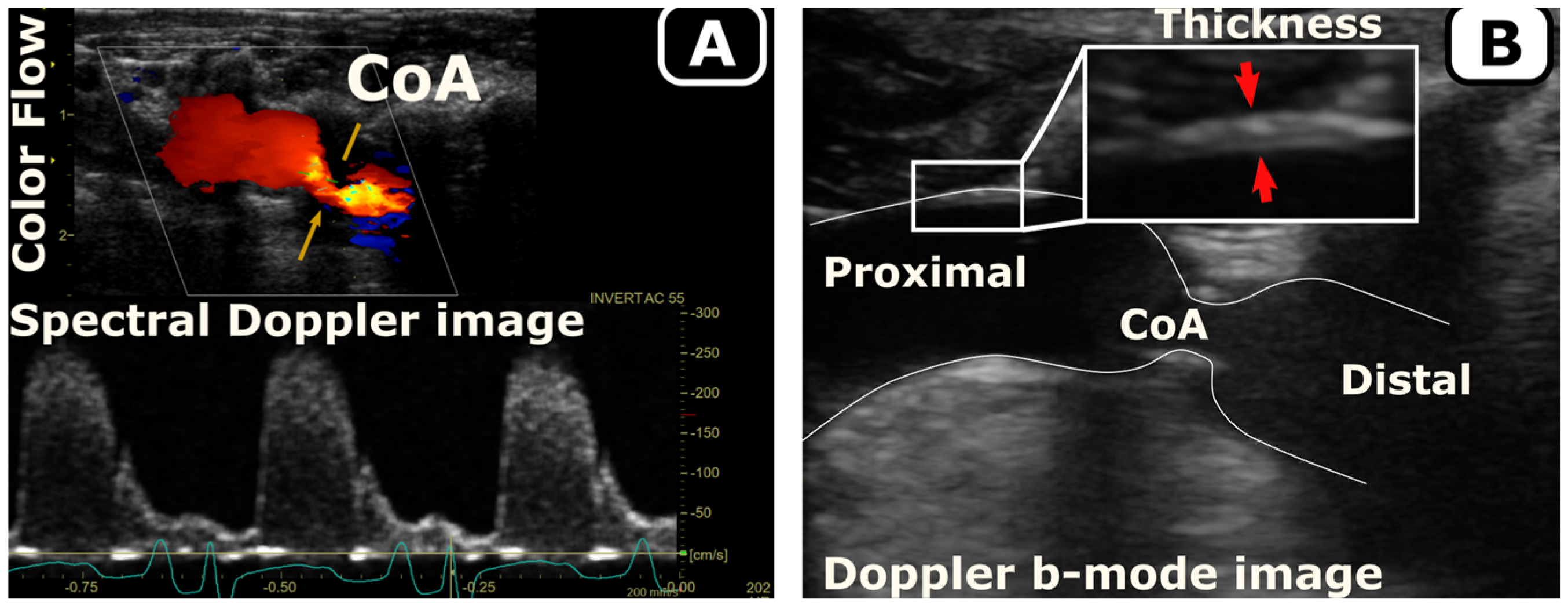
2.5.2. Temporal Monitoring of Morphology and Hemodynamics
2.5.3. Vascular Stiffening
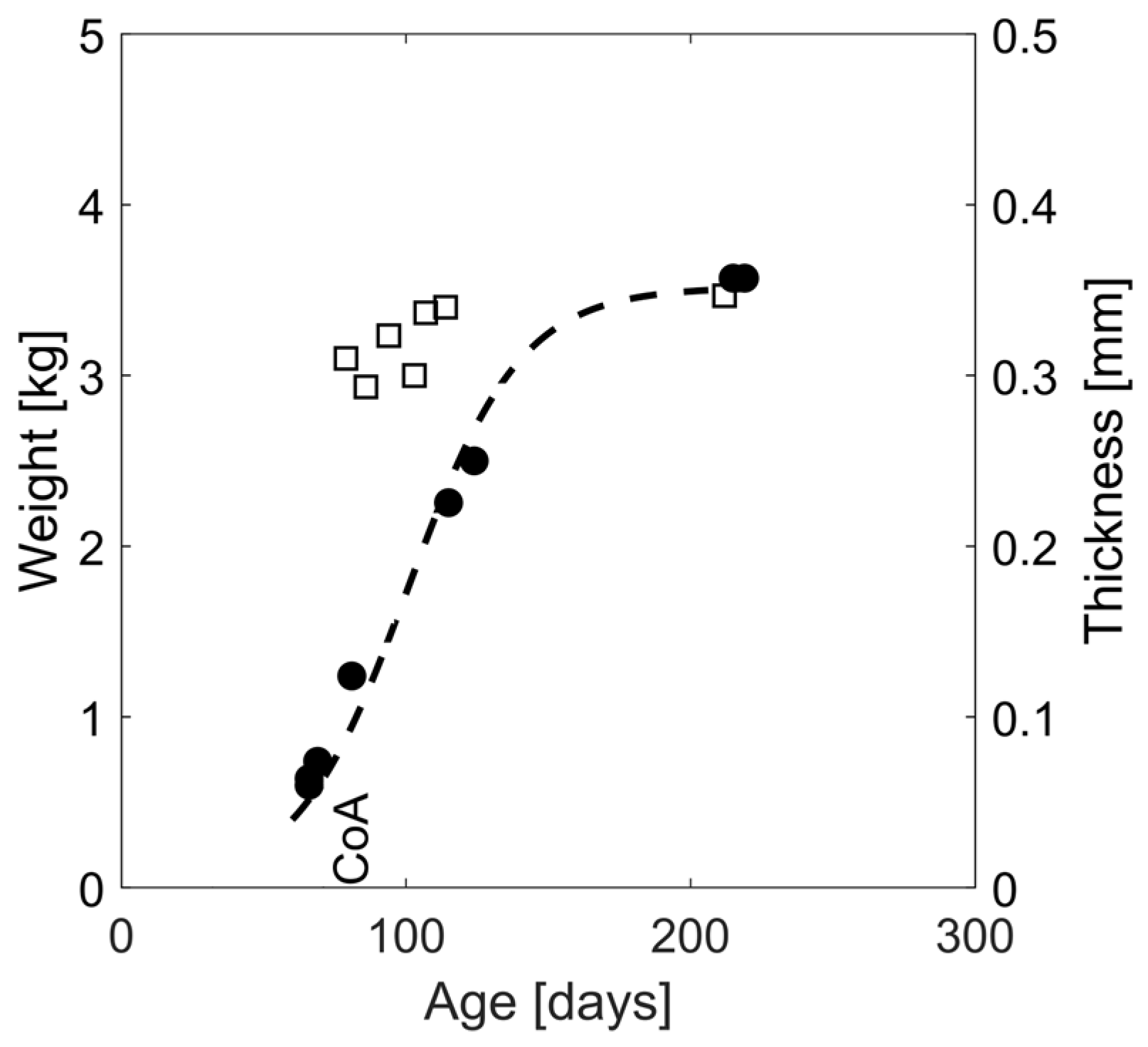
2.5.4. Vascular Thickening
2.5.5. Vascular Dysfunction
2.5.6. WSS and IWS Evolution
2.6. Statistical Analysis
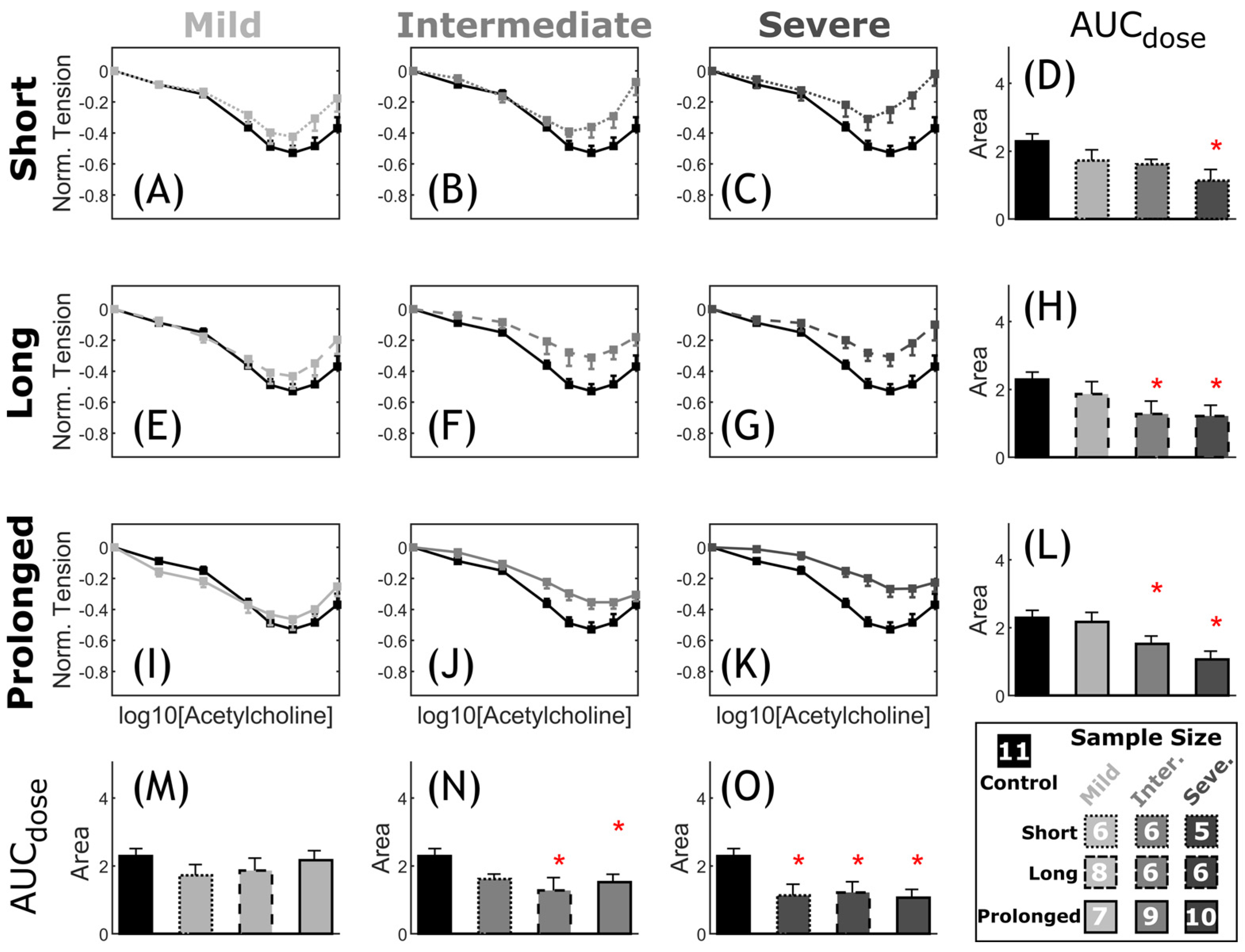
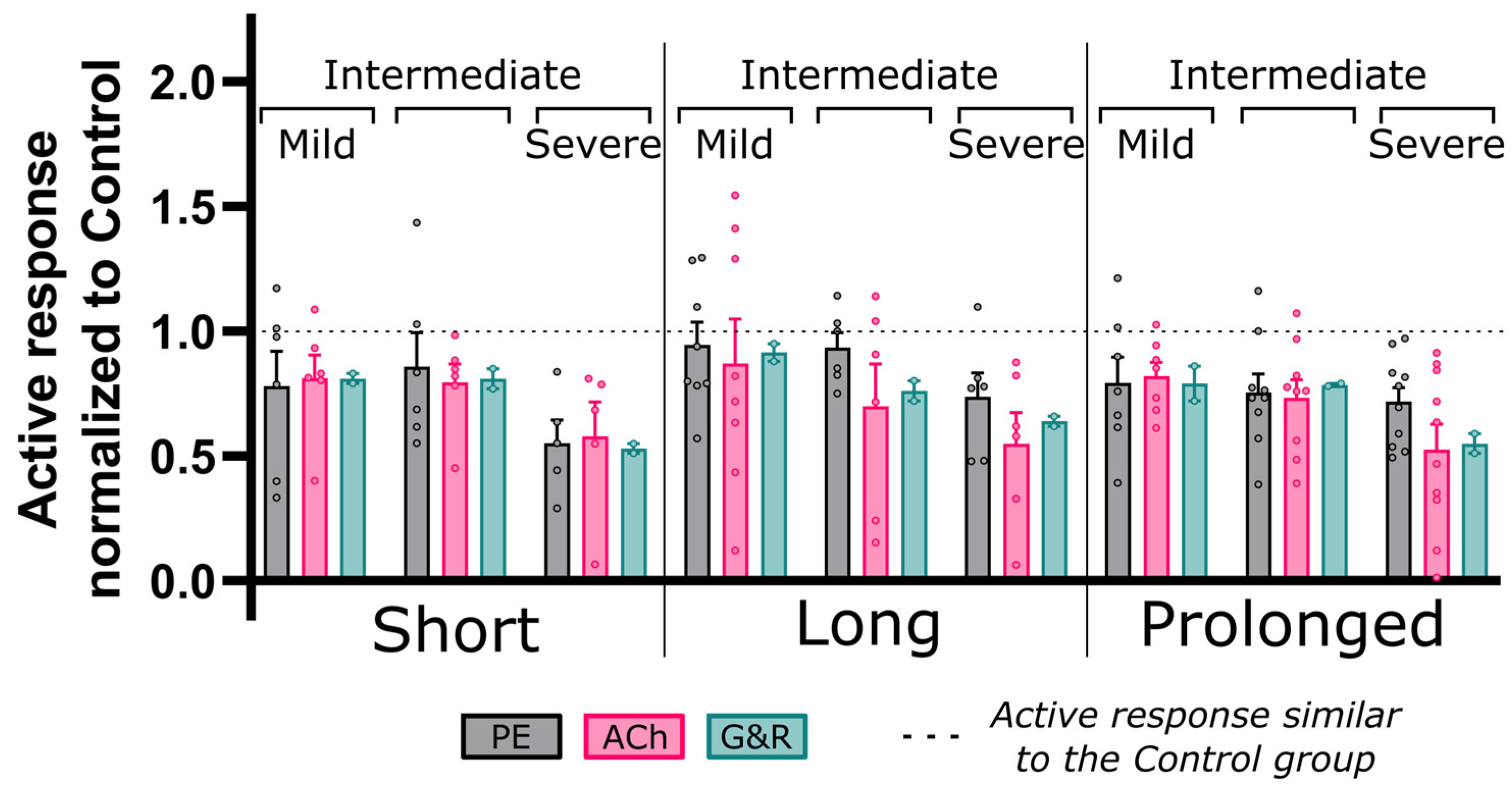
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| AA | Ascending aorta |
| BP | Blood pressure |
| CFPG | Continuous-flow pressure gradient |
| CoA | Coarctation of the aorta |
| IWS | Intramural wall stress |
| MBE | Modified Bernoulli equation |
| PC-MRI | Phase-contrast magnetic resonance image |
| ROM | Reduced-order model |
| SBE | Simplified Bernoulli equation |
| SBE-RP | SBE minus recovered pressure term |
| WSS | Wall shear stress |
References
- Ferencz, C.; Rubin, J.D.; McCarter, R.J.; Brenner, J.I.; Neill, C.A.; Perry, L.W.; Hepner, S.I.; Downing, J.W. Congenital Heart Disease: Prevalence at Livebirth: The Baltimore-Washington Infant Study. Am. J. Epidemiol. 1985, 121, 31–36. [Google Scholar] [CrossRef]
- Torok, R.D.; Campbell, M.J.; Fleming, G.A.; Hill, K.D. Coarctation of the Aorta: Management from Infancy to Adulthood. World J. Cardiol. 2015, 7, 765–775. [Google Scholar] [CrossRef]
- Feltes, T.F.; Bacha, E.; Beekman, R.H.; Cheatham, J.P.; Feinstein, J.A.; Gomes, A.S.; Hijazi, Z.M.; Ing, F.F.; de Moor, M.; Morrow, W.R.; et al. Indications for Cardiac Catheterization and Intervention in Pediatric Cardiac Disease. Circulation 2011, 123, 2607–2652. [Google Scholar] [CrossRef]
- Cohen, M.; Fuster, V.; Steele, P.M.; Driscoll, D.; McGoon, D.C. Coarctation of the Aorta. Long-Term Follow-up and Prediction of Outcome after Surgical Correction. Circulation 1989, 80, 840–845. [Google Scholar] [CrossRef]
- Sendzikaite, S.; Sudikiene, R.; Tarutis, V.; Lubaua, I.; Silis, P.; Rybak, A.; Jankauskiene, A.; Litwin, M. Prevalence of Arterial Hypertension, Hemodynamic Phenotypes, and Left Ventricular Hypertrophy in Children after Coarctation Repair: A Multicenter Cross-Sectional Study. Pediatr. Nephrol. 2020, 35, 2147–2155. [Google Scholar] [CrossRef]
- LaDisa, J.F., Jr.; Bozdag, S.; Olson, J.; Ramchandran, R.; Kersten, J.R.; Eddinger, T.J. Gene Expression in Experimental Aortic Coarctation and Repair: Candidate Genes for Therapeutic Intervention? PLoS ONE 2015, 10, e0133356. [Google Scholar] [CrossRef]
- LaDisa, J.F.J.; Tomita-Mitchell, A.; Stamm, K.; Bazan, K.; Mahnke, D.K.; Goetsch, M.A.; Wegter, B.J.; Gerringer, J.W.; Repp, K.; Palygin, O.; et al. Human Genotyping and an Experimental Model Reveal NPR-C as a Possible Contributor to Morbidity in Coarctation of the Aorta. Physiol. Genom. 2019, 51, 177–185. [Google Scholar] [CrossRef]
- Menon, A.; Wendell, D.C.; Wang, H.; Eddinger, T.J.; Toth, J.M.; Dholakia, R.J.; Larsen, P.M.; Jensen, E.S.; LaDisa, J.F., Jr. A Coupled Experimental and Computational Approach to Quantify Deleterious Hemodynamics, Vascular Alterations, and Mechanisms of Long-Term Morbidity in Response to Aortic Coarctation. J. Pharmacol. Toxicol. Methods 2012, 65, 18–28. [Google Scholar] [CrossRef]
- Menon, A.; Eddinger, T.J.; Wang, H.; Wendell, D.C.; Toth, J.M.; LaDisa, J.F., Jr. Altered Hemodynamics, Endothelial Function, and Protein Expression Occur with Aortic Coarctation and Persist after Repair. Am. J. Physiol. Heart Circ. Physiol. 2012, 303, H1304-18. [Google Scholar] [CrossRef]
- Ghorbannia, A.; Ellepola, C.D.; Woods, R.K.; Ibrahim, E.-S.H.; Maadooliat, M.; Ramirez, H.M.; LaDisa, J.F.J. Clinical, Experimental, and Computational Validation of a New Doppler-Based Index for Coarctation Severity Assessment. J. Am. Soc. Echocardiogr. 2022, 35, 1311–1321. [Google Scholar] [CrossRef]
- Panzer, J.; Bové, T.; Vandekerckhove, K.; De Wolf, D. Hypertension after Coarctation Repair-a Systematic Review. Transl. Pediatr. 2022, 11, 270–279. [Google Scholar] [CrossRef]
- Valentín, A.; Holzapfel, G.A. Constrained Mixture Models as Tools for Testing Competing Hypotheses in Arterial Biomechanics: A Brief Survey. Mech. Res. Commun. 2012, 42, 126–133. [Google Scholar] [CrossRef]
- Valentín, A.; Humphrey, J.D. Evaluation of Fundamental Hypotheses Underlying Constrained Mixture Models of Arterial Growth and Remodelling. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2009, 367, 3585–3606. [Google Scholar] [CrossRef]
- Humphrey, J.D. Mechanisms of Arterial Remodeling in Hypertension. Hypertension 2008, 52, 195–200. [Google Scholar] [CrossRef]
- Cyron, C.J.; Humphrey, J.D. Vascular Homeostasis and the Concept of Mechanobiological Stability. Int. J. Eng. Sci. 2014, 85, 203–223. [Google Scholar] [CrossRef]
- Humphrey, J.D.; Rajagopal, K.R. A Constrained Mixture Model for Growth and Remodeling of Soft Tissues. Math. Model. Methods Appl. Sci. 2002, 12, 407–430. [Google Scholar] [CrossRef]
- Teng, Z.; Yuan, J.; Feng, J.; Zhang, Y.; Brown, A.J.; Wang, S.; Lu, Q.; Gillard, J.H. The Influence of Constitutive Law Choice Used to Characterise Atherosclerotic Tissue Material Properties on Computing Stress Values in Human Carotid Plaques. J. Biomech. 2015, 48, 3912–3921. [Google Scholar] [CrossRef]
- Ogden, R.W. Non-Linear Elastic Deformations; Dover Civil and Mechanical Engineering; Dover Publications: Mineola, NY, USA, 1997; ISBN 9780486696485. [Google Scholar]
- Wilson, J.S.; Baek, S.; Humphrey, J.D. Parametric Study of Effects of Collagen Turnover on the Natural History of Abdominal Aortic Aneurysms. Proc. R. Soc. A Math. Phys. Eng. Sci. 2013, 469, 20120556. [Google Scholar] [CrossRef]
- Wu, J.; Shadden, S.C. Coupled Simulation of Hemodynamics and Vascular Growth and Remodeling in a Subject-Specific Geometry. Ann. Biomed. Eng. 2015, 43, 1543–1554. [Google Scholar] [CrossRef]
- Rhodin, J.A.G. Architecture of the Vessel Wall. In Comprehensive Physiology; Wiley: New York, NY, USA, 2014; pp. 1–31. ISBN 978-0-470-65071-4. [Google Scholar]
- Halayko, A.J.; Solway, J. Invited Review: Molecular Mechanisms of Phenotypic Plasticity in Smooth Muscle Cells. J. Appl. Physiol. 2001, 90, 358–368. [Google Scholar] [CrossRef]
- Albinsson, S.; Bhattachariya, A.; Hellstrand, P. Stretch-Dependent Smooth Muscle Differentiation in the Portal Vein-Role of Actin Polymerization, Calcium Signaling, and MicroRNAs. Microcirculation 2014, 21, 230–238. [Google Scholar] [CrossRef]
- Westerhof, N.; Stergiopulos, N.; Noble, M.I.M. Snapshots of Hemodynamics an Aid for Clinical Research and Graduate Education; Springer: New York, NY, USA, 2005. [Google Scholar]
- Van Varik, B.; Rennenberg, R.; Reutelingsperger, C.; Kroon, A.; de Leeuw, P.; Schurgers, L. Mechanisms of Arterial Remodeling: Lessons from Genetic Diseases. Front. Genet. 2012, 3, 290. [Google Scholar] [CrossRef]
- Lindström, S.B.; Stålhand, J.; Klarbring, A. Modeling of the Mechanobiological Adaptation in Muscular Arteries. Eur. J. Mech.-A/Solids 2017, 64, 165–177. [Google Scholar] [CrossRef]
- Figueroa, C.A.; Baek, S.; Taylor, C.A.; Humphrey, J.D. A Computational Framework for Fluid-Solid-Growth Modeling in Cardiovascular Simulations. Comput. Methods Appl. Mech. Eng. 2009, 198, 3583–3602. [Google Scholar] [CrossRef]
- Baek, S.; Valentín, A.; Humphrey, J.D. Biochemomechanics of Cerebral Vasospasm and Its Resolution: II. Constitutive Relations and Model Simulations. Ann. Biomed. Eng. 2007, 35, 1498–1509. [Google Scholar] [CrossRef]
- Rachev, A.; Hayashi, K. Theoretical Study of the Effects of Vascular Smooth Muscle Contraction on Strain and Stress Distributions in Arteries. Ann. Biomed. Eng. 1999, 27, 459–468. [Google Scholar] [CrossRef]
- Ogden, R.W. Nonlinear Elasticity, Anisotropy, Material Stability and Residual Stresses in Soft Tissue BT—Biomechanics of Soft Tissue in Cardiovascular Systems; Holzapfel, G.A., Ogden, R.W., Eds.; Springer: Vienna, Austria, 2003; pp. 65–108. ISBN 978-3-7091-2736-0. [Google Scholar]
- Taber, L.A. A Model for Aortic Growth Based on Fluid Shear and Fiber Stresses. J. Biomech. Eng. 1998, 120, 348–354. [Google Scholar] [CrossRef]
- Rachev, A. A Model of Arterial Adaptation to Alterations in Blood Flow. J. Elast. Phys. Sci. Solids 2000, 61, 83–111. [Google Scholar] [CrossRef]
- Conn, A.R.; Gould, N.I.M.; Toint, P. A Globally Convergent Augmented Lagrangian Algorithm for Optimization with General Constraints and Simple Bounds. SIAM J. Numer. Anal. 1991, 28, 545–572. [Google Scholar] [CrossRef]
- Holzapfel, G.A.; Gasser, T.C.; Ogden, R.W. A New Constitutive Framework for Arterial Wall Mechanics and a Comparative Study of Material Models. J. Elast. Phys. Sci. Solids 2000, 61, 1–48. [Google Scholar] [CrossRef]
- Touyz, R.M.; Alves-Lopes, R.; Rios, F.J.; Camargo, L.L.; Anagnostopoulou, A.; Arner, A.; Montezano, A.C. Vascular Smooth Muscle Contraction in Hypertension. Cardiovasc. Res. 2018, 114, 529–539. [Google Scholar] [CrossRef]
- DePaola, N.; Gimbrone, M.A., Jr.; Davies, P.F.; Dewey, C.F. Vascular Endothelium Responds to Fluid Shear Stress Gradients. Arterioscler. Thromb. 1992, 12, 1254–1257. [Google Scholar] [CrossRef]
- Stout, K.K.; Daniels, C.J.; Aboulhosn, J.A.; Bozkurt, B.; Broberg, C.S.; Colman, J.M.; Crumb, S.R.; Dearani, J.A.; Fuller, S.; Gurvitz, M.; et al. 2018 AHA/ACC Guideline for the Management of Adults With Congenital Heart Disease: A Report of the American College of Cardiology/American Heart Association Task Force on Clinical Practice Guidelines. Circulation 2019, 139, e698–e800. [Google Scholar] [CrossRef]
- Masoud, I.; Shapiro, F.; Kent, R.; Moses, A. A Longitudinal Study of the Growth of the New Zealand White Rabbit: Cumulative and Biweekly Incremental Growth Rates for Body Length, Body Weight, Femoral Length, and Tibial Length. J. Orthop. Res. Off. Publ. Orthop. Res. Soc. 1986, 4, 221–231. [Google Scholar] [CrossRef]
- Dutta, S.; Sengupta, P. Rabbits and Men: Relating Their Ages. J. Basic Clin. Physiol. 2018, 29, 427–435. [Google Scholar] [CrossRef]
- Humphrey, J.D. Towards a Theory of Vascular Growth and Remodeling BT—Mechanics of Biological Tissue; Holzapfel, G.A., Ogden, R.W., Eds.; Springer: Berlin/Heidelberg, Germany, 2006; pp. 3–15. ISBN 978-3-540-31184-3. [Google Scholar]
- Latorre, M.; Humphrey, J.D. A Mechanobiologically Equilibrated Constrained Mixture Model for Growth and Remodeling of Soft Tissues. Z. Fur. Angew. Math. Und Mech. 2018, 98, 2048–2071. [Google Scholar] [CrossRef]
- Laurent, S.; Boutouyrie, P. Arterial Stiffness and Stroke in Hypertension: Therapeutic Implications for Stroke Prevention. CNS Drugs 2005, 19, 1–11. [Google Scholar] [CrossRef]
- Tardy, Y.; Resnick, N.; Nagel, T.; Gimbrone, M.A.; Dewey, C.F. Shear Stress Gradients Remodel Endothelial Monolayers in Vitro via a Cell Proliferation-Migration-Loss Cycle. Arterioscler. Thromb. Vasc. Biol. 1997, 17, 3102–3106. [Google Scholar] [CrossRef]
- Gimbrone, M.A.J.; García-Cardeña, G. Vascular Endothelium, Hemodynamics, and the Pathobiology of Atherosclerosis. Cardiovasc. Pathol. Off. J. Soc. Cardiovasc. Pathol. 2013, 22, 9–15. [Google Scholar] [CrossRef]
- López, D.; Rodríguez-Sinovas, A.; Agulló, L.; Inserte, J.; Cabestrero, A.; García-Dorado, D. Acidic Reoxygenation Protects against Endothelial Dysfunction in Rat Aortic Rings Submitted to Simulated Ischemia. Am. J. Physiol.-Heart Circ. Physiol. 2008, 295, H2409–H2416. [Google Scholar] [CrossRef]
- Ghorbanniahassankiadeh, A.; Marks, D.S.; LaDisa, J.F., Jr. Correlation of Computational Instantaneous Wave-Free Ratio With Fractional Flow Reserve for Intermediate Multivessel Coronary Disease. J. Biomech. Eng. 2021, 143, 051011. [Google Scholar] [CrossRef]
- LaDisa, J.F.; Ghorbannia, A.; Marks, D.S.; Mason, P.; Otake, H. Advancements and Opportunities in Characterizing Patient-Specific Wall Shear Stress Imposed by Coronary Artery Stenting. Fluids 2022, 7, 325. [Google Scholar] [CrossRef]





| Parameter | Description | Reference | Value |
|---|---|---|---|
| Collagen turnover rate | Rhobin et al. [21] | 1/80 [1/days] | |
| SM turnover rate | Rhobin et al. [21] | 1/80 [1/days] | |
| Initial elastin decay kinetics | Halayko et al. [22] | 1 | |
| Remodeled elastin decay kinetics | Halayko et al. [22] | 0 | |
| SM pre-stretch | Holzapfel et al. [34] | 1.3 | |
| Maximum stress capacity of SM in the fully contractile state | Conn et al. [33] | 100 [kPa] | |
| Phenotypic modulation stimulus parameter at normotensive condition | Lindstrom et al. [26] | 0.8326 | |
| Phenotypic modulation stimulus parameter at hypertensive condition | Touyz et al. [35] | [0.1, 100] | |
| Wu et al. [20] | |||
| Wu et al. [20] | |||
| Wu et al. [20] | |||
| Pre-stretch for collagen | Rachev et al. [32] | 1.08 | |
| Pre-stretch for elastin | Rachev et al. [32] | 1.4 | |
| G&R collagen kinetic parameter for hoop stress | DePaola et al. [36] | ||
| G&R collagen kinetic parameter for WSS | DePaola et al. [36] | ||
| G&R SM kinetic parameter for hoop stress | DePaola et al. [36] | ||
| G&R SM kinetic parameter for WSS | DePaola et al. [36] | ||
| Basal net production rate of collagen | Halayko et al. [22] | [0, 10] | |
| Basal net production rate of SM | Halayko et al. [22] | [0, 10] | |
| Basal net production rate of elastin | Halayko et al. [22] | 0 |
| Rabbit Group | Constitutive Parameters | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Duration (Weeks) | Severity (Peak-to-Peak CoA Gradient) | Collagen * | Smooth Muscle * | Elastin ** | ||||||
| c1 *** | c2 | c3 | c1 | c2 | c3 | c1 | c2 | c3 | ||
| Short (~2) | Mild (<13 †) | 0.0 | 597 ± 91 | 11.7 ± 4.8 | 0.0 | 11.5 ± 3.3 | 2.3 ± 1.4 | 56.2 ± 21 | 0.0 | NA |
| Intermediate (13–20) | 0.0 | 646 ± 101 | 6.40 ± 2.1 | 0.0 | 13.2 ± 4.3 | 3.7 ± 1.8 | 67.4 ± 18 | 0.0 | NA | |
| Severe (≥20) | 0.0 | 613 ± 94 | 7.70 ± 2.0 | 0.0 | 13.0 ± 3.9 | 1.9 ± 1.0 | 58.7 ± 23 | 0.0 | NA | |
| Long (~5) | Mild (<13) | 0.0 | 629 ± 97 | 10.5 ± 3.8 | 0.0 | 10.1 ± 4.2 | 3.7 ± 1.9 | 59.3 ± 8 | 0.0 | NA |
| Intermediate (13–20) | 0.0 | 673 ± 113 | 9.70 ± 4.0 | 0.0 | 14.2 ± 4.8 | 4.8 ± 2.1 | 63.8 ± 16 | 0.0 | NA | |
| Severe (≥20) | 0.0 | 716 ± 117 | 14.9 ± 5.0 | 0.0 | 18.9 ± 4.3 | 7.2 ± 2.8 | 72.9 ± 31 | 0.0 | NA | |
| Prolonged (~22) | Mild (<13) | 0.0 | 692 ± 88 | 12.7 ± 3.6 | 0.0 | 13.8 ± 5.1 | 11.7 ± 4.8 | 65.4 ± 21 | 0.0 | NA |
| Intermediate (13–20) | 0.0 | 708 ± 122 | 13.2 ± 4.5 | 0.0 | 15.1 ± 3.8 | 11.7 ± 4.8 | 61.4 ± 17 | 0.0 | NA | |
| Severe (≥20) | 0.0 | 721 ± 42 | 14.0 ± 5.2 | 0.0 | 15.2 ± 4.2 | 11.7 ± 4.8 | 68.1 ± 24 | 0.0 | NA | |
| Rabbit Group | Constitutive Parameters | |||||
|---|---|---|---|---|---|---|
| Duration (Weeks) | Severity (Peak-to-Peak CoA Gradient) | Collagen | Smooth Muscle | |||
| (×10−6) | (×10−6) | (×10−6) | (×10−6) | C′ | ||
| Short (~2) | Mild (<13 †) | 3.10 ± 2.0 * | 1.80 ± 1.2 | 5.30 ± 3.0 | 3.90 ± 1.5 | 10.1 ± 3.0 |
| Intermediate (13–20) | 5.20 ± 1.1 | 2.90 ± 1.2 | 10.2 ± 4.5 | 4.10 ± 2.0 | 8.80 ± 2.1 | |
| Severe (≥20) | 3.70 ± 0.9 | 1.70 ± 0.5 | 7.10 ± 2.7 | 4.50 ± 1.8 | 10.0 ± 2.8 | |
| Long (~5) | Mild (<13) | 4.30 ± 1.6 | 2.30 ± 1.2 | 5.60 ± 3.1 | 5.60 ± 2.7 | 9.60 ± 2.5 |
| Intermediate (13–20) | 4.10 ± 2.1 | 2.00 ± 0.8 | 7.60 ± 2.7 | 4.60 ± 1.1 | 11.6 ± 1.7 | |
| Severe (≥20) | 5.00 ± 1.7 | 3.50 ± 1.3 | 9.60 ± 1.5 | 3.60 ± 1.6 | 8.60 ± 2.1 | |
| Prolonged (~22) | Mild (<13) | 5.40 ± 2.5 | 2.80 ± 1.2 | 5.60 ± 2.2 | 3.60 ± 1.1 | 7.60 ± 2.7 |
| Intermediate (13–20) | 4.60 ± 1.3 | 3.10 ± 1.9 | 12.60 ± 2.8 | 5.60 ± 2.9 | 9.60 ± 3.0 | |
| Severe (≥20) | 6.30 ± 2.2 | 3.40 ± 1.7 | 10.60 ± 3.0 | 4.60 ± 2.7 | 10.6 ± 1.9 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ghorbannia, A.; Maadooliat, M.; Woods, R.K.; Audi, S.H.; Tefft, B.J.; Chiastra, C.; Ibrahim, E.S.H.; LaDisa, J.F. Aortic Remodeling Kinetics in Response to Coarctation-Induced Mechanical Perturbations. Biomedicines 2023, 11, 1817. https://doi.org/10.3390/biomedicines11071817
Ghorbannia A, Maadooliat M, Woods RK, Audi SH, Tefft BJ, Chiastra C, Ibrahim ESH, LaDisa JF. Aortic Remodeling Kinetics in Response to Coarctation-Induced Mechanical Perturbations. Biomedicines. 2023; 11(7):1817. https://doi.org/10.3390/biomedicines11071817
Chicago/Turabian StyleGhorbannia, Arash, Mehdi Maadooliat, Ronald K. Woods, Said H. Audi, Brandon J. Tefft, Claudio Chiastra, El Sayed H. Ibrahim, and John F. LaDisa. 2023. "Aortic Remodeling Kinetics in Response to Coarctation-Induced Mechanical Perturbations" Biomedicines 11, no. 7: 1817. https://doi.org/10.3390/biomedicines11071817
APA StyleGhorbannia, A., Maadooliat, M., Woods, R. K., Audi, S. H., Tefft, B. J., Chiastra, C., Ibrahim, E. S. H., & LaDisa, J. F. (2023). Aortic Remodeling Kinetics in Response to Coarctation-Induced Mechanical Perturbations. Biomedicines, 11(7), 1817. https://doi.org/10.3390/biomedicines11071817






