Reliability Measures and Profit Exploration of Windmill Water-Pumping Systems Incorporating Warranty and Two Types of Repair
Abstract
:1. Introduction
2. Materials and Methods
2.1. Notation
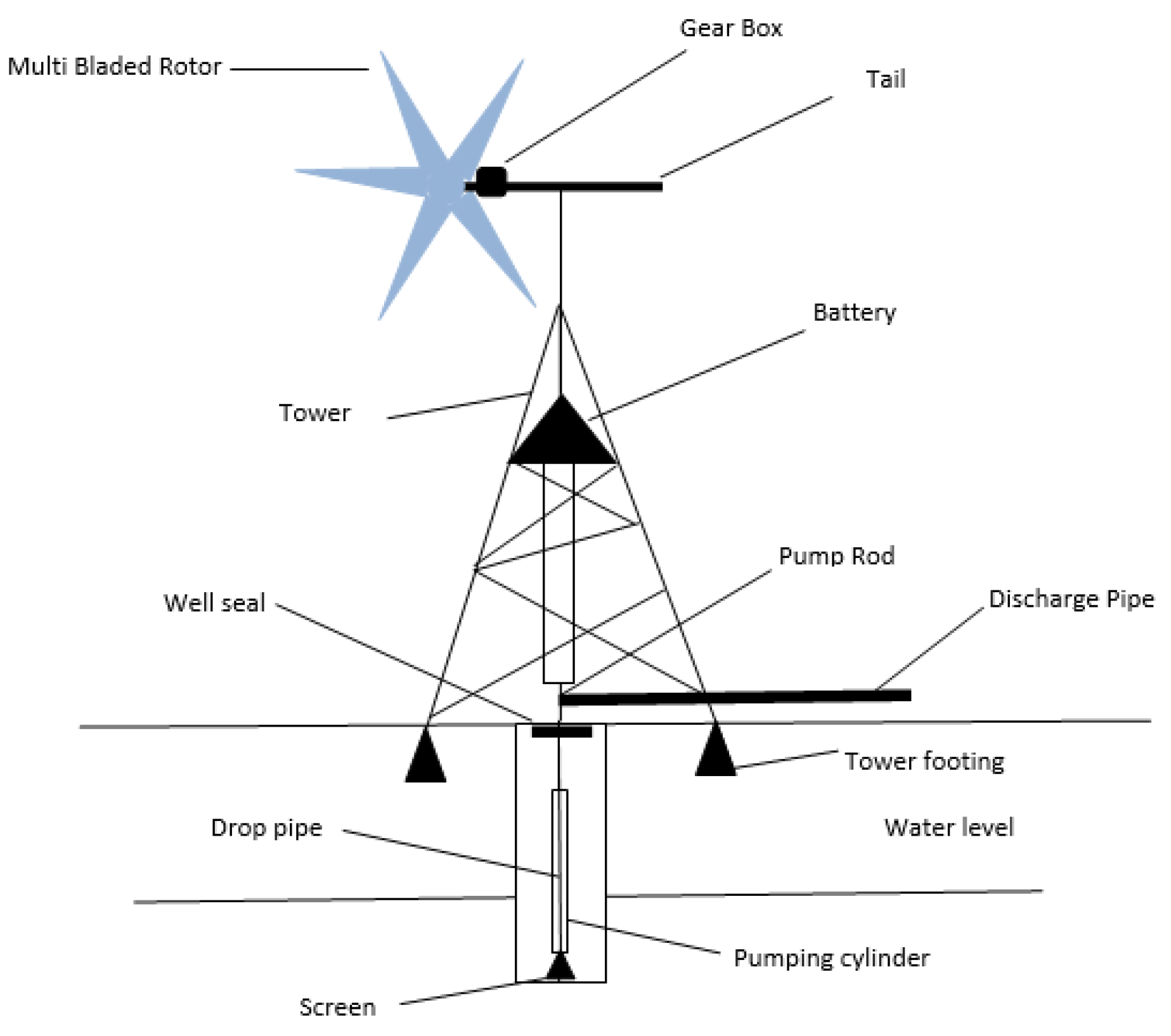
2.2. System Description
- Initially, the system is in good working condition.
- The system has a fixed warranty period.
- The gearbox is repaired by two types of repair policy via the Gumbel–Hougaard family of Copula and the other components are repaired generally.
- The system is partially working when k blades are in a failed state, but two of them should not be adjacent.
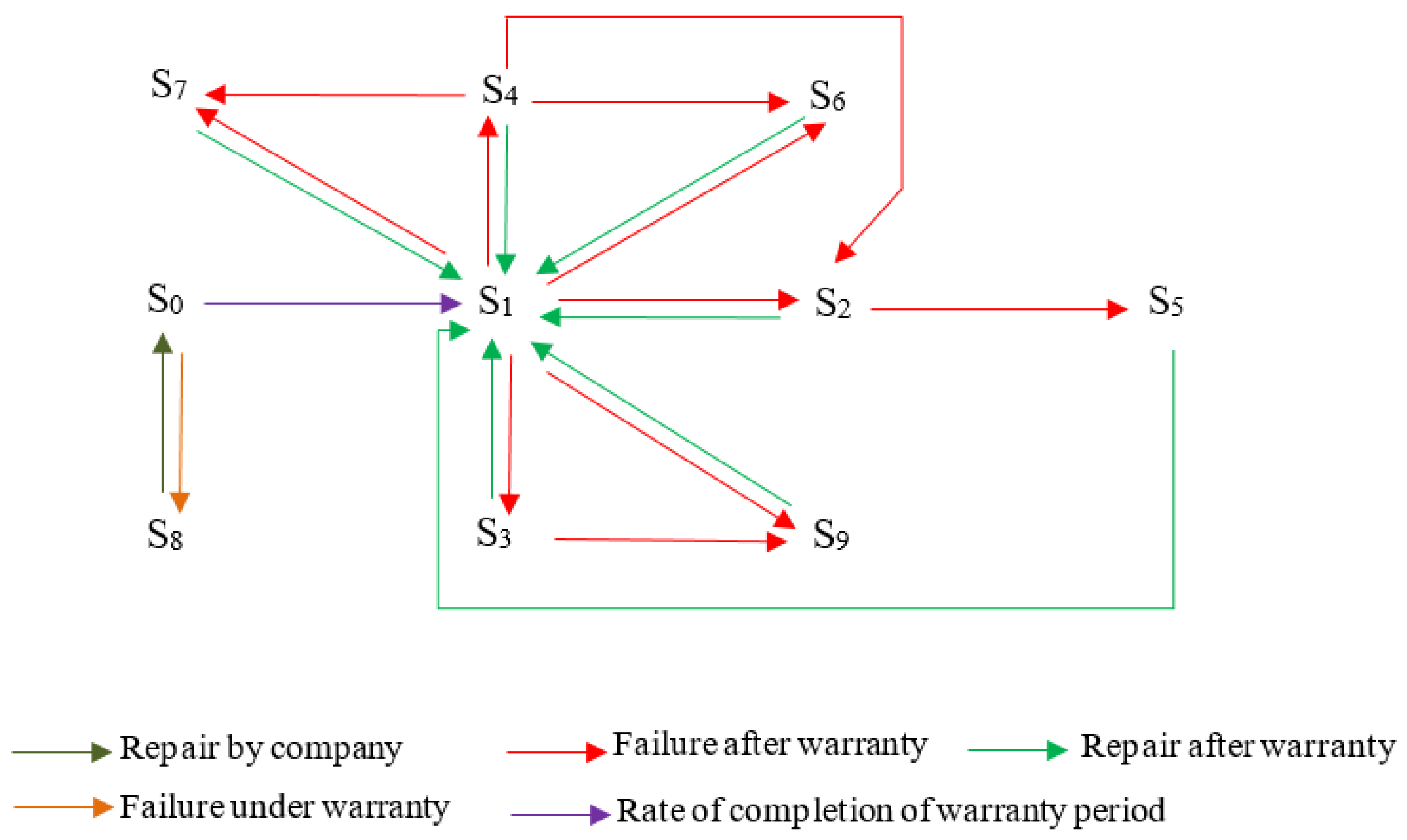
| S0 | The initial state in which the system is in good condition as per the assumptions. |
| S1 | The good working state of the system when the warranty period is over. |
| S2 | The degraded state of the system when k-out-of-n blades have been failed but in which two blades should not be adjacent. |
| S3 | The degraded state of the system due to minor failure in the pumping system. |
| S4 | Partially failed state of the combined system due to failure in the gearbox. |
| S5 | Failed state of the system due to k+1 faulty blades. |
| S6 | Failed state of the system due to break or error in the shaft. |
| S7 | Failed state of the system caused by battery discharge. |
| S8 | Failed state of the system when the system can fail due to any failure under the warranty period and in this system is repaired by the company free of charge. |
| S9 | Failed state of the system because of any major failure in the pumping system. |
3. Mathematical Model
3.1. Formulation of the Model
3.2. Solution of the Model
3.3. Working State and Failed State Probability of the System
4. Numerical Example
4.1. Availability Analysis
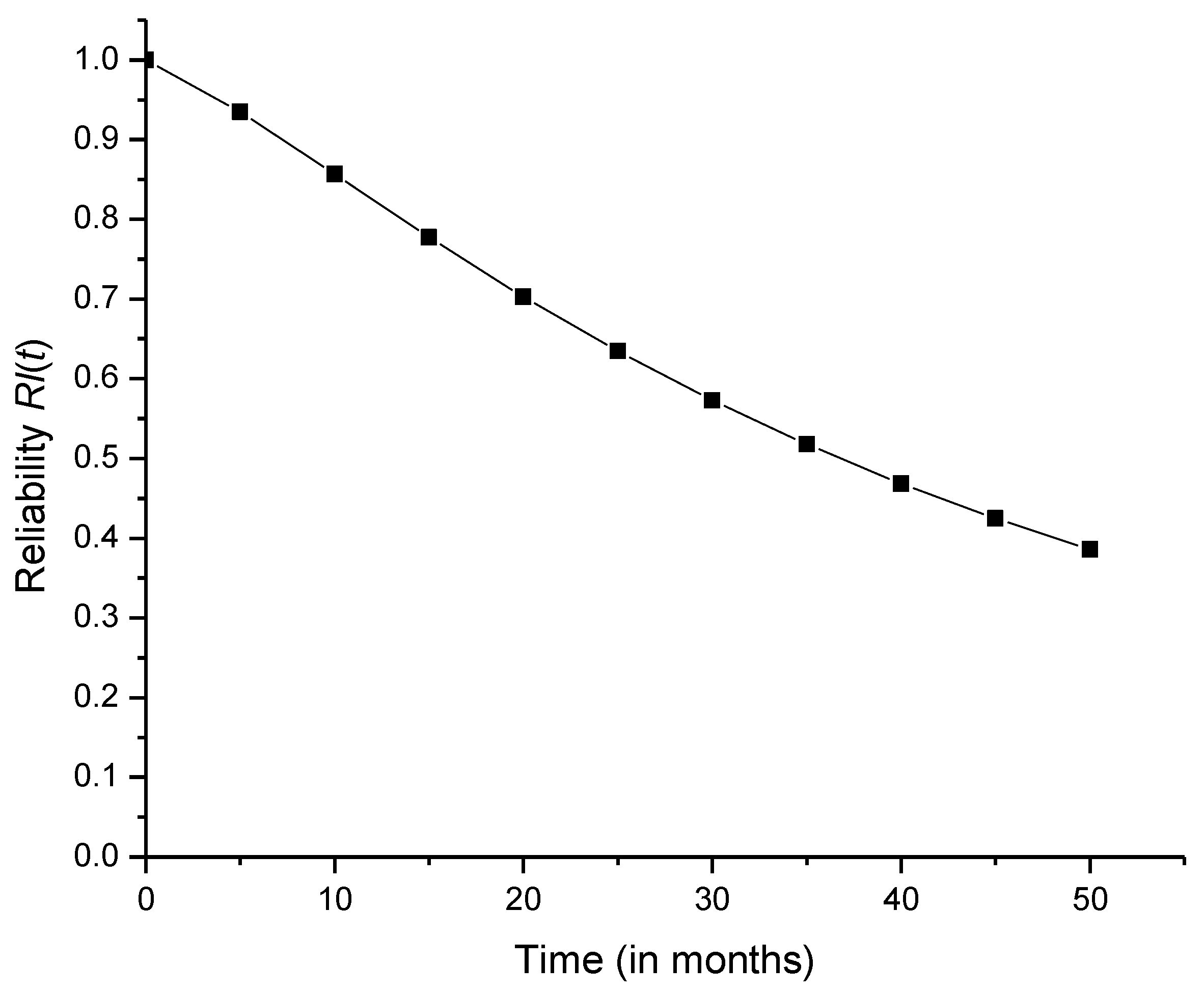
4.2. Reliability Analysis
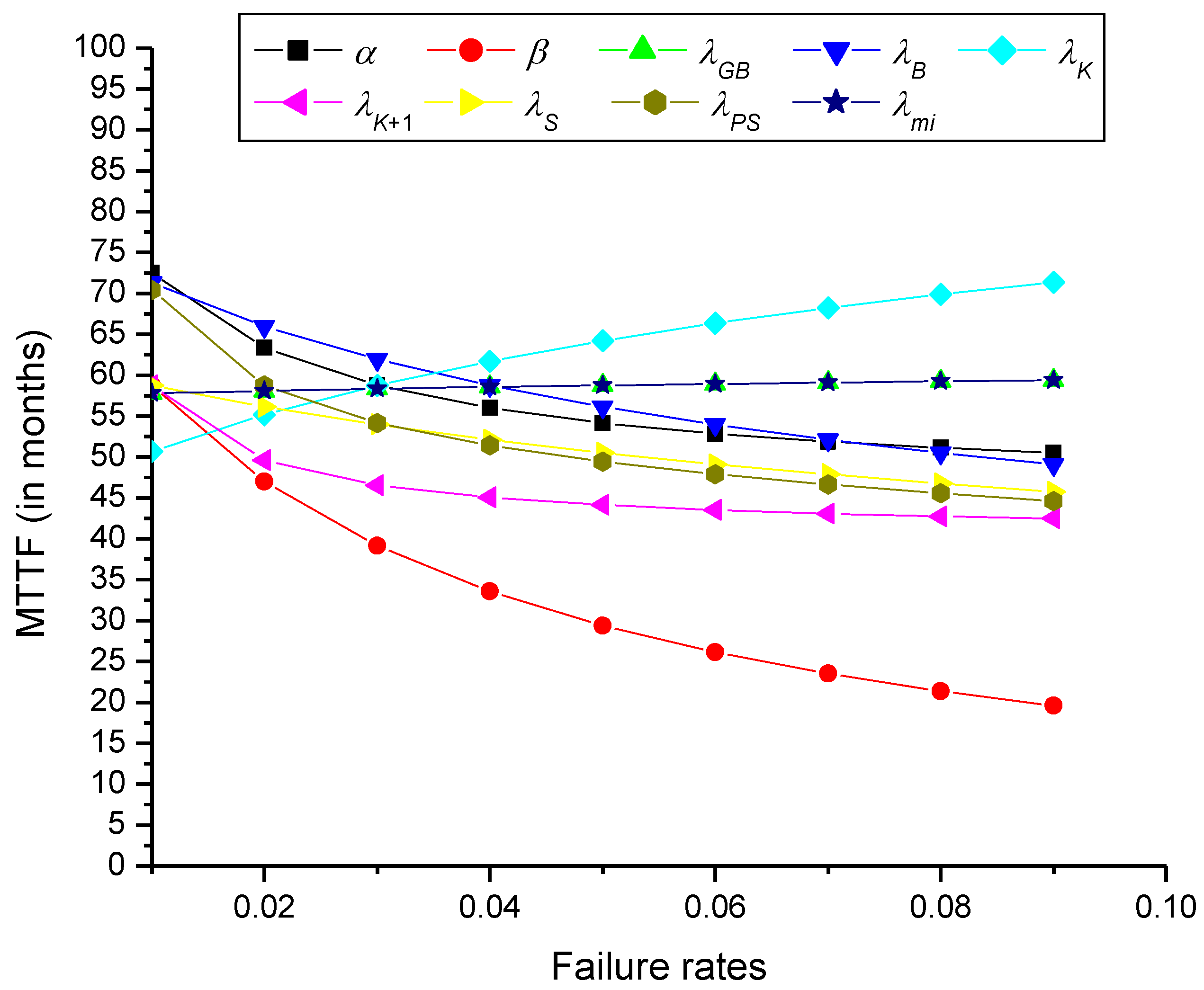
4.3. Mean Time to Failure (MTTF)
4.4. Expected Profit
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bragg, G.M.; Schmidt, W.L. Performance matching and optimization of wind powered water pumping systems. Energy Convers. 1979, 19, 33–39. [Google Scholar] [CrossRef]
- Gopal, C.; Mohanraj, M.; Chandramohan, P.; Chandrasekar, P. Renewable energy source water pumping systems—A literature review. Renew. Sustain. Energy Rev. 2013, 25, 351–370. [Google Scholar] [CrossRef]
- Aliyu, M.; Hassan, G.; Said, S.A.; Siddiqui, M.U.; Alawami, A.T.; Elamin, I.M. A review of solar-powered water pumping systems. Renew. Sustain. Energy Rev. 2018, 87, 61–76. [Google Scholar] [CrossRef]
- Argaw, N.E.W.A.Y.; Foster, R.; Ellis, A. Renewable Energy for Water Pumping Applications in Rural Villages; New Mexico State University: Lass Cruces, NM, USA, 2003. [Google Scholar]
- Liu, Z.; Wu, D.; Liu, Y.; Han, Z.; Lun, L.; Gao, J.; Cao, G. Accuracy analyses and model comparison of machine learning adopted in building energy consumption prediction. Energy Explor. Exploit. 2019, 37, 1426–1451. [Google Scholar] [CrossRef] [Green Version]
- Imani, M.; Ghoreishi, S.F. Scalable inverse reinforcement learning through multi-fidelity Bayesian optimization. IEEE Trans. Neural Netw. Learn. Syst. 2021, 1–8. [Google Scholar] [CrossRef]
- Xu, Z.; Saleh, J.H. Machine learning for reliability engineering and safety applications: Review of current status and future opportunities. Reliab. Eng. Syst. Saf. 2021, 211, 107530. [Google Scholar] [CrossRef]
- Badran, O. Wind turbine utilization for water pumping in Jordan. J. Wind Eng. Ind. Aerodyn. 2003, 91, 1203–1214. [Google Scholar] [CrossRef]
- Mohamed, R.M. General characteristics of mechanical wind water pumping system and the possibility of applying system in Egypt. In Proceedings of the First International Conference on Energy Solutions for Electric and water Supply in Rural, Cairo, Egypt, 7–10 October 2015; pp. 59–69. [Google Scholar]
- Odesola, I.F.; Adinoyi, L.G.; Student, P.G. Development of wind powered water pump. Int. J. Eng. Sci. 2017, 7, 10341–10345. [Google Scholar]
- Oi, A. Design and Simulation of Photovoltaic Water Pumping System. Ph.D. Thesis, California Polytechnic State University, San Luis Obispo, CA, USA, 2005; pp. 1–113. [Google Scholar]
- Browning, J.A. U.S. Patent No. 3,952,723; U.S. Patent and Trademark Office: Washington, DC, USA, 1976.
- Vick, B.D.; Neal, B.A. Analysis of off-grid hybrid wind turbine/solar PV water pumping systems. Sol. Energy 2012, 86, 1197–1207. [Google Scholar] [CrossRef] [Green Version]
- Bouzidi, B. Viability of solar or wind for water pumping systems in the Algerian Sahara regions–Case study Adrar. Renew. Sustain. Energy Rev. 2011, 15, 4436–4442. [Google Scholar] [CrossRef]
- Odeh, I.; Yohanis, Y.G.; Norton, B. Economic viability of photovoltaic water pumping systems. Sol. Energy 2006, 80, 850–860. [Google Scholar] [CrossRef]
- Mohsen, M.S.; Akash, B.A. Potentials of wind energy development for water pumping in Jordan. Renew. Energy 1998, 14, 441–446. [Google Scholar] [CrossRef]
- Awad, A.S. Practical design and testing of wind driven water pumping systems. Int. J. Mech. Eng. Technol. 2019, 10, 1419–1430. [Google Scholar]
- Poompavai, T.; Kowsalya, M. Control and energy management strategies applied for solar photovoltaic and wind energy fed water pumping system: A review. Renew. Sustain. Energy Rev. 2019, 107, 108–122. [Google Scholar] [CrossRef]
- Argaw, N. Renewable Energy Water Pumping Systems Handbook; National Renewable Energy Lab.: Golden, CO, USA, 2004.
- Rehman, S.; Sahin, A.Z. A wind-solar PV hybrid power system with battery backup for water pumping in remote localities. Int. J. Green Energy 2016, 13, 1075–1083. [Google Scholar] [CrossRef]
- Zio, E.; Sansavini, G. Reliability analysis of multi-state networks: Making Monte Carlo simulation feasible through biasing. Nato Secur. Through Sci. Ser. D Inf. Commun. Secur. 2007, 13, 121. [Google Scholar]
- Liu, Y.W.; Kapur, K.C. New models and measures for reliability of multi-state systems. In Performability Engineering; Springer: London, UK, 2008; pp. 431–445. [Google Scholar]
- Natvig, B. Multistate System Reliability; Wiley Encyclopedia of Operations Research and Management Science; John Wiley & Sons: Hoboken, NJ, USA, 2010; pp. 1–7. [Google Scholar]
- Lin, Y. Reliability evaluation of a multistate network under routing policy. In Proceedings of the 2011 9th International Conference on Reliability, Maintainability and Safety, Guiyang, China, 12–15 June 2011; pp. 222–230. [Google Scholar]
- Lin, Y.K.; Chang, P.C. System reliability of a manufacturing network with reworking action and different failure rates. Int. J. Prod. Res. 2012, 50, 6930–6944. [Google Scholar] [CrossRef]
- Lin, Y.K.; Huang, C.F.; Liao, Y.C.; Yeh, C.C. System reliability for a multistate intermodal logistics network with time windows. Int. J. Prod. Res. 2017, 55, 1957–1969. [Google Scholar] [CrossRef]
- Wenbin, Z.; Frenkel, I.; Guixiang, S.; Bolvashenkov, I.; Kammermann, J.; Herzog, H.G.; Khvatskin, L. Markov reward approach and reliability associated cost model for machine tools maintenance-planning optimization. Int. J. Math. Eng. Manag. Sci. 2019, 4, 824–840. [Google Scholar] [CrossRef]
- Lin, T.; Pham, H. Reliability and cost-benefit analysis for two-stage intervened decision-making systems with interdependent decision units. Int. J. Math. Eng. Manag. Sci. 2019, 4, 531–541. [Google Scholar] [CrossRef]
- Rushdi, A.M.A. Utilization of symmetric switching functions in the symbolic reliability analysis of multi-state k-out-of-n systems. Int. J. Math. Eng. Manag. Sci. 2019, 4, 306–326. [Google Scholar] [CrossRef]
- Deng, C.; Miao, J.; Ma, Y.; Wei, B.; Feng, Y. Reliability analysis of chatter stability for milling process system with uncertainties based on neural network and fourth moment method. Int. J. Prod. Res. 2020, 58, 2732–2750. [Google Scholar] [CrossRef]
- Sarsour, W.M.; Sabri, S.R.M. Forecasting the long-run behaviour of the stock price of some selected companies in the Malaysian construction sector: A Markov chain approach. Int. J. Math. Eng. Manag. Sci. 2020, 5, 296–308. [Google Scholar]


| Symbol | Description |
|---|---|
| t/s | Time scale/Laplace transform variable. |
| Laplace transformation of . | |
| Failure rates of battery/shaft/gearbox/pumping cylinder/minor error in pumping system. | |
| Failure rate for k blades not to two adjacent/k + 1 blades. | |
| g | Average failure rate after warranty. |
| w | Warranty period. |
| l | Total expected life of the system. |
| Rate of completion of warranty. | |
| Failure rate during the warranty period. | |
| Repair rates for the degraded states S2 and S3. | |
| , | Repair rate for the degraded state S4 and y is the elapse repair time for this state. |
| q | The joint probability (failed state S4, to normal state S0) according to the Gumbel–Hougaard family is given as: . |
| Repair rate for completely failed state Si+4; i = 1 … 5. | |
| The probability that the system is in state Si, where i = 0 … 4. | |
| The probability that the system is the state Sj+4, and xi is the elapse repair time where i = 1, 2, …5. | |
| Probability of working state of the system. | |
| Probability of failed state of the system. | |
| Ep(t) | Expected profit during the interval [0, t). |
| K1, K2 | Revenue and service cost per unit time. |
| Time (t in Months) | Availability Pup(t) |
|---|---|
| 0 | 1.00000 |
| 5 | 0.97452 |
| 10 | 0.93721 |
| 15 | 0.88624 |
| 20 | 0.82708 |
| 25 | 0.76383 |
| 30 | 0.69943 |
| 35 | 0.63596 |
| 40 | 0.57483 |
| 45 | 0.51697 |
| 50 | 0.46293 |
| Time (t in Months) | Reliability Rl |
|---|---|
| 0 | 1.00000 |
| 5 | 0.93502 |
| 10 | 0.85651 |
| 15 | 0.77743 |
| 20 | 0.70283 |
| 25 | 0.63452 |
| 30 | 0.57290 |
| 35 | 0.51776 |
| 40 | 0.46862 |
| 45 | 0.42492 |
| 50 | 0.38608 |
| Failure Rates | MTTF | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| α | β | λGB | λB | λK | λK+1 | λS | λPS | λmi | |
| 0.01 | 72.50000 | 58.75000 | 57.81250 | 71.32353 | 50.69445 | 58.75000 | 58.75000 | 70.39474 | 57.81250 |
| 0.02 | 63.33333 | 47.00000 | 58.08823 | 65.97222 | 55.16917 | 49.60937 | 56.15079 | 58.75000 | 58.08823 |
| 0.03 | 58.75000 | 39.16667 | 58.33334 | 61.93609 | 58.75000 | 46.56250 | 53.97727 | 54.16667 | 58.33334 |
| 0.04 | 56.00000 | 33.57143 | 58.55263 | 58.75000 | 61.70635 | 45.03906 | 52.12450 | 51.42045 | 58.55263 |
| 0.05 | 54.16667 | 29.37500 | 58.75000 | 56.15079 | 64.20454 | 44.12500 | 50.52083 | 49.45652 | 58.75000 |
| 0.06 | 52.85714 | 26.11111 | 58.92857 | 53.97727 | 66.35375 | 43.51562 | 49.11538 | 47.91667 | 58.92857 |
| 0.07 | 51.87500 | 23.50000 | 59.09091 | 52.12450 | 68.22917 | 43.08036 | 47.87088 | 46.64286 | 59.09091 |
| 0.08 | 51.11111 | 21.36364 | 59.23913 | 50.52083 | 69.88461 | 42.75391 | 46.75926 | 45.55288 | 59.23913 |
| 0.09 | 50.50000 | 19.58333 | 59.37500 | 49.11538 | 71.35989 | 42.50000 | 45.75893 | 44.59876 | 59.37500 |
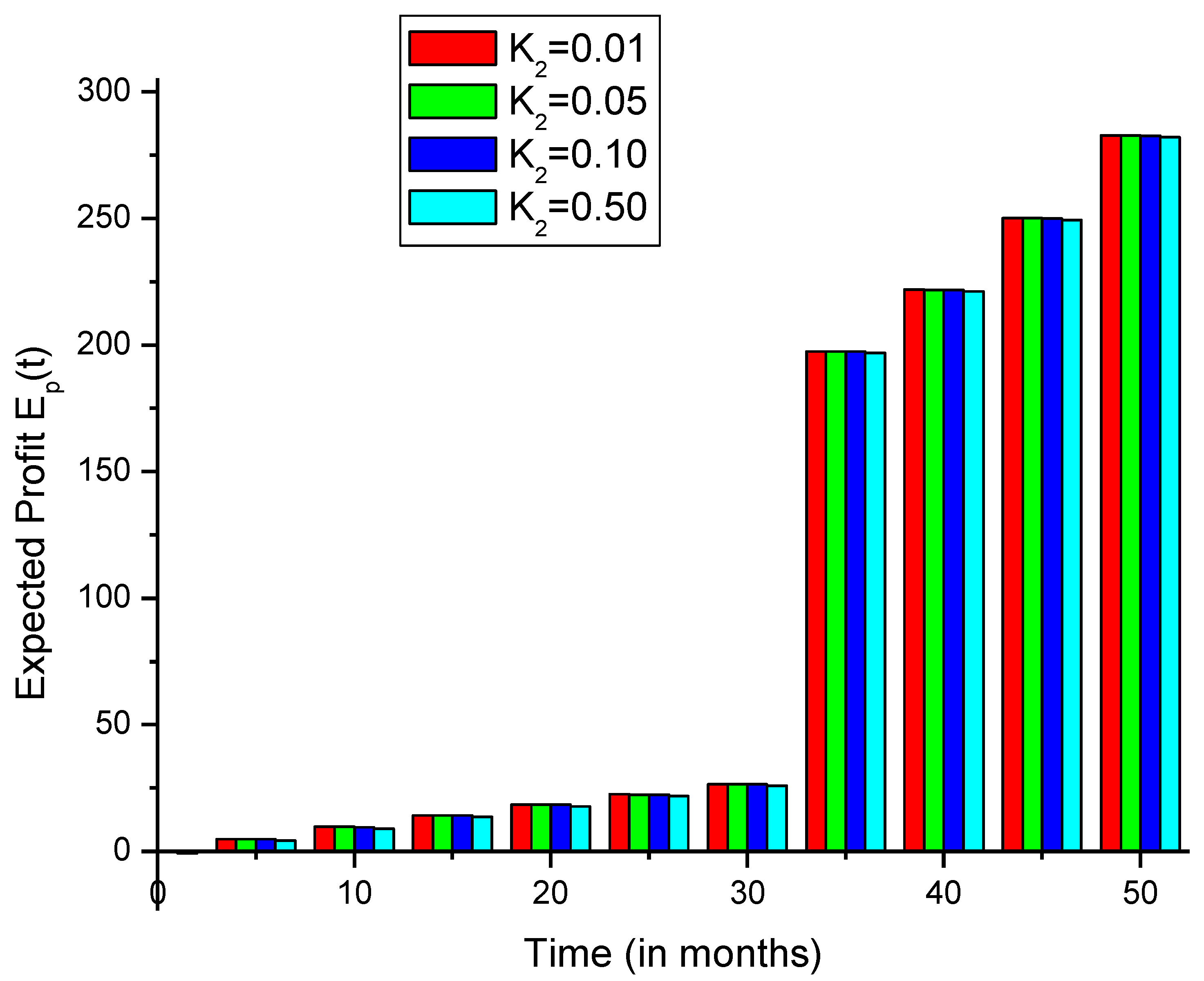
| Time (t) (In Months) | Expected Profit | |||||||
|---|---|---|---|---|---|---|---|---|
| K2 = 0.01 | K2 = 0.05 | K2 = 0.1 | K2 = 0.5 | |||||
| w = 12 | w = 24 | w = 12 | w = 24 | w = 12 | w = 24 | w = 12 | w = 24 | |
| 0 | −0.01440 | −0.0108 | −0.07200 | −0.05400 | −0.14400 | −0.108 | −0.72000 | −0.540 |
| 5 | 4.91808 | 4.92168 | 4.86048 | 4.87847 | 4.78848 | 4.82448 | 4.21248 | 4.39247 |
| 10 | 9.70443 | 9.70803 | 9.64683 | 9.66483 | 9.57483 | 9.61083 | 8.99883 | 9.17883 |
| 15 | 14.26750 | 14.27110 | 14.20990 | 14.22790 | 14.13790 | 14.17390 | 13.56190 | 13.74190 |
| 20 | 18.55286 | 18.55646 | 18.49526 | 18.51326 | 18.42326 | 18.45926 | 17.84726 | 18.02726 |
| 25 | 22.55368 | 22.55728 | 22.49608 | 22.51408 | 22.42408 | 22.46008 | 21.84808 | 22.02808 |
| 30 | 26.60545 | 26.60905 | 26.54785 | 26.56585 | 26.47585 | 26.51185 | 25.89985 | 26.07985 |
| 35 | 197.55858 | 197.56218 | 197.50098 | 197.51898 | 197.42898 | 197.46498 | 196.85298 | 197.03298 |
| 40 | 221.91050 | 221.91410 | 221.85290 | 221.87090 | 221.78090 | 221.81690 | 221.20490 | 221.38490 |
| 45 | 250.14638 | 250.14998 | 250.08878 | 250.10678 | 250.01678 | 250.05278 | 249.44078 | 249.62078 |
| 50 | 282.84736 | 282.85096 | 282.78976 | 282.80775 | 282.71776 | 282.75376 | 282.14176 | 282.32176 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Goyal, N.; Ram, M.; Kumar, A.; Bisht, S.; Klochkov, Y. Reliability Measures and Profit Exploration of Windmill Water-Pumping Systems Incorporating Warranty and Two Types of Repair. Mathematics 2021, 9, 822. https://doi.org/10.3390/math9080822
Goyal N, Ram M, Kumar A, Bisht S, Klochkov Y. Reliability Measures and Profit Exploration of Windmill Water-Pumping Systems Incorporating Warranty and Two Types of Repair. Mathematics. 2021; 9(8):822. https://doi.org/10.3390/math9080822
Chicago/Turabian StyleGoyal, Nupur, Mangey Ram, Akshay Kumar, Soni Bisht, and Yury Klochkov. 2021. "Reliability Measures and Profit Exploration of Windmill Water-Pumping Systems Incorporating Warranty and Two Types of Repair" Mathematics 9, no. 8: 822. https://doi.org/10.3390/math9080822
APA StyleGoyal, N., Ram, M., Kumar, A., Bisht, S., & Klochkov, Y. (2021). Reliability Measures and Profit Exploration of Windmill Water-Pumping Systems Incorporating Warranty and Two Types of Repair. Mathematics, 9(8), 822. https://doi.org/10.3390/math9080822









