Unsteady MHD Mixed Convection Flow in Hybrid Nanofluid at Three-Dimensional Stagnation Point
Abstract
1. Introduction
2. Mathematical Modeling
3. Stability Analysis
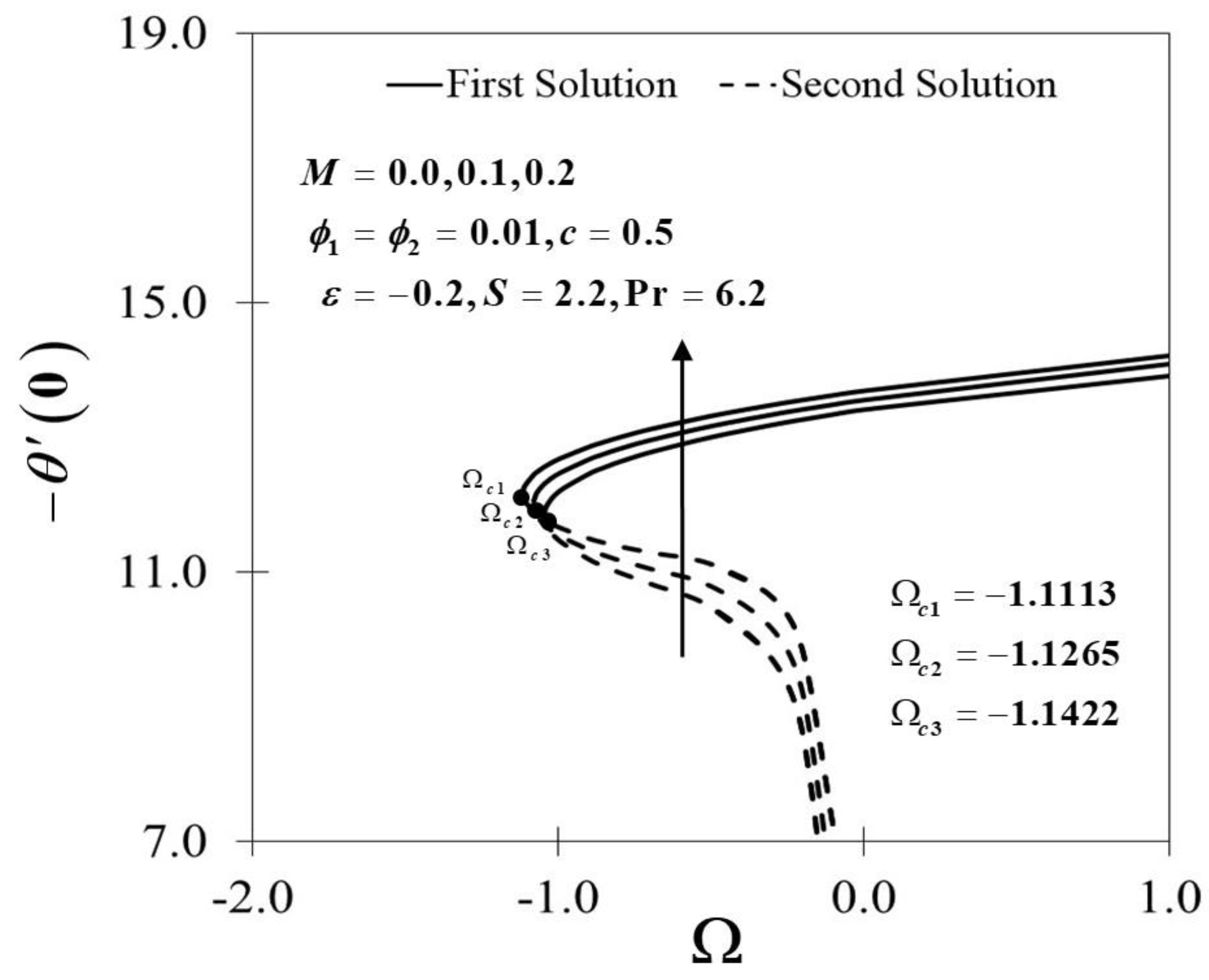
4. Discussion and Results
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| Roman letters | |
| constants | |
| transverse magnetic field | |
| strength of the magnetic field | |
| local skin friction coefficients | |
| specific heat at constant pressure | |
| dimensionless velocity function | |
| functions | |
| local Grashof number | |
| thermal conductivity of the fluid | |
| characteristic length of the sheet surface | |
| magnetic coefficient | |
| local Nusselt number | |
| heat capacitance of the fluid | |
| Prandtl number | |
| surface heat flux | |
| Rex, Rey | local Reynolds number in the and axes, respectively |
| mass flux parameter | |
| time | |
| T | fluid temperature |
| variable temperature | |
| reference temperature | |
| surrounding temperature | |
| velocity components along the and axes, respectively | |
| velocities of the ambient (inviscid) fluid in the and axes, respectively | |
| constant mass flux velocity | |
| Cartesian coordinates | |
| Greek letters | |
| thermal expansion coefficient | |
| constant | |
| unsteadiness parameter | |
| nanoparticle volume fractions for Al2O3 (alumina) | |
| nanoparticle volume fractions for Cu (copper) | |
| similarity variable | |
| dynamic viscosity of the fluid | |
| kinematic viscosity of the fluid | |
| dimensionless temperature | |
| density of the fluid | |
| dimensionless time variable | |
| shear stresses or skin frictions in and axes, respectively | |
| eigenvalue | |
| smallest eigenvalue | |
| mixed convection parameter | |
| Subscripts | |
| base fluid | |
| nanofluid | |
| hybrid nanofluid | |
| solid component for Al2O3 (alumina) | |
| solid component for Cu (copper) | |
| Superscript | |
| differentiation with respect to | |
References
- Marston, P.G.; Dawson, A.M.; Montgomery, D.B.; Williams, J.E.C. Superconducting MHD Magnet Engineering Program. In Advances in Cryogenic Engineering; Springer: Boston, MA, USA, 1980. [Google Scholar]
- Katagiri, M. Unsteady magnetohydrodynamic flow at the forward stagnation point. J. Phys. Soc. Jpn. 1969, 27, 1662–1668. [Google Scholar] [CrossRef]
- Pavlov, K.B. Magnetohydrodynamic flow of an incompressible viscous fluid caused by deformation of a plane surface. Magn. Gidrodin. 1974, 4, 146–147. [Google Scholar]
- Takhar, H.S.; Ali, M.; Gupta, A.S. Stability of magnetohydrodynamic flow over a stretching sheet. In Liquid Metal Magnetohydrodynamics; Springer: Dordrecht, The Netherlands, 1989; pp. 465–471. [Google Scholar]
- Deswita, L.; Junoh, M.M.; Ali, F.; Nazar, R.; Pop, I. Magnetohydrodynamic slip flow and heat transfer over a nonlinear shrinking surface in a heat generating fluid. Int. J. Eng. Technol. 2020, 9, 534–540. [Google Scholar] [CrossRef]
- Fayyadh, M.M.; Naganthran, K.; Md Basir, M.F.; Hashim, I.; Roslan, R. Radiative MHD sutterby nanofluid flow past a moving sheet: Scaling group analysis. Mathematics 2020, 8, 1430. [Google Scholar] [CrossRef]
- Zainal, N.A.; Nazar, R.; Naganthran, K.; Pop, I. MHD flow and heat transfer of hybrid nanofluid over a permeable moving surface in the presence of thermal radiation. Int. J. Numer. Methods Heat Fluid Flow. 2020. [Google Scholar] [CrossRef]
- Nisar, K.S.; Khan, U.; Zaib, A.; Khan, I.; Baleanu, D. Exploration of aluminium and titanium alloys in the stream-wise and secondary flow directions comprising the significant impacts of magnetohydrodynamic and hybrid nanofluid. Crystals 2020, 10, 679. [Google Scholar] [CrossRef]
- Zainal, N.A.; Nazar, R.; Naganthran, K.; Pop, I. MHD mixed convection stagnation point flow of a hybrid nanofluid past a vertical flat plate with convective boundary condition. Chin. J. Phys. 2020, 66, 630–644. [Google Scholar] [CrossRef]
- Ramachandran, N.; Chen, T.S.; Armaly, B.F. Mixed convection in stagnation flows adjacent to vertical surfaces. J. Heat Transfer. 1988, 110, 373–377. [Google Scholar] [CrossRef]
- Merkin, J.H. Mixed convection boundary layer flow on a vertical surface in a saturated porous medium. J. Eng. Math. 1980, 14, 301–313. [Google Scholar] [CrossRef]
- Merkin, J.H. On dual solutions occurring in mixed convection in a porous medium. J. Eng. Math. 1986, 20, 171–179. [Google Scholar] [CrossRef]
- Oztop, H.F.; Al-Salem, K.; Pop, I. MHD mixed convection in a lid-driven cavity with corner heater. Int. J. Heat Mass Transf. 2011, 54, 3494–3504. [Google Scholar] [CrossRef]
- Daniel, Y.S.; Daniel, S.K. Effects of buoyancy and thermal radiation on MHD flow over a stretching porous sheet using homotopy analysis method. Alex. Eng. J. 2015, 54, 705–712. [Google Scholar] [CrossRef]
- Jamaludin, A.; Naganthran, K.; Nazar, R.; Pop, I. MHD mixed convection stagnation-point flow of Cu-Al2O3/water hybrid nanofluid over a permeable stretching/shrinking surface with heat source/sink. Eur. J. Mech. B/Fluids 2020, 84, 71–80. [Google Scholar] [CrossRef]
- Asadi, A.; Asadi, M.; Rezaniakolaei, A.; Rosendahl, L.A.; Afrand, M.; Wongwises, S. Heat transfer efficiency of Al2O3-MWCNT/thermal oil hybrid nanofluid as a cooling fluid in thermal and energy management applications: An experimental and theoretical investigation. Int. J. Heat Mass Transf. 2018, 117, 474–486. [Google Scholar] [CrossRef]
- Esfe, M.H.; Esfandeh, S.; Saedodin, S.; Rostamian, H. Experimental evaluation, sensitivity analyzation and ANN modeling of thermal conductivity of ZnO-MWCNT/EG-water hybrid nanofluid for engineering applications. Appl. Therm. Eng. 2017, 125, 673–685. [Google Scholar] [CrossRef]
- Bahiraei, M.; Mazaheri, N.; Rizehvandi, A. Application of a hybrid nanofluid containing graphene nanoplatelet–platinum composite powder in a triple-tube heat exchanger equipped with inserted ribs. Appl. Therm. Eng. 2019, 149, 588–601. [Google Scholar] [CrossRef]
- Xian, H.W.; Azwadi, N.; Sidik, C.; Aid, S.R.; Ken, T.L.; Asako, Y. Review on Preparation Techniques, Properties and Performance of Hybrid Nanofluid in Recent Engineering Applications. J. Adv. Res. Fluid Mech. Therm. Sci. 2018, 45, 1–13. [Google Scholar]
- Gupta, M.; Singh, V.; Kumar, S.; Kumar, S.; Dilbaghi, N. Up to date review on the synthesis and thermophysical properties of hybrid nanofluids. J. Clean. Prod. 2018, 190, 169–192. [Google Scholar] [CrossRef]
- Suresh, S.; Venkitaraj, K.P.; Selvakumar, P.; Chandrasekar, M. Synthesis of Al2O3-Cu/water hybrid nanofluids using two-step method and its thermophysical properties. Colloids Surf. A Physicochem. Eng. Asp. 2011, 388, 41–48. [Google Scholar] [CrossRef]
- Suresh, S.; Venkitaraj, K.P.; Selvakumar, P. Synthesis, characterisation of Al2O3-Cu nanocomposite powder and water-based nanofluids. Adv. Mater. Res. 2011, 328–330, 1560–1567. [Google Scholar] [CrossRef]
- Khashi’ie, N.S.; Arifin, N.M.; Pop, I. Non-Darcy mixed convection of hybrid nanofluid with thermal dispersion along a vertical plate embedded in a porous medium. Int. Commun. Heat Mass Transf. 2020, 118, 104866. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Groşan, T.; Pop, I. Mixed convection of a hybrid nanofluid flow along a vertical surface embedded in a porous medium. Int. Commun. Heat Mass Transf. 2020, 114, 104565. [Google Scholar] [CrossRef]
- Mehryan, S.A.M.; Izadpanahi, E.; Ghalambaz, M.; Chamkha, A.J. Mixed convection flow caused by an oscillating cylinder in a square cavity filled with Cu–Al2O3/water hybrid nanofluid. J. Therm. Anal. Calorim. 2019, 137, 965–982. [Google Scholar] [CrossRef]
- Zainal, N.A.; Nazar, R.; Naganthran, K.; Pop, I. Impact of anisotropic slip on the stagnation-point flow past a stretching/shrinking surface of the Al2O3-Cu /H2O hybrid nanofluid. Appl. Math. Mech. 2020, 41, 1401–1416. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Hybrid nanofluid flow and heat transfer over a permeable biaxial stretching/shrinking sheet. Int. J. Numer. Methods Heat Fluid Flow. 2019, 30, 3497–3513. [Google Scholar] [CrossRef]
- Hiemenz, K. Die Grenzschicht an einem in den gleichförmigen Flüssigkeitsstrom eingetauchten geraden Kreiszylinder. Dinglers Polytech. J. 1911, 326, 321–324. [Google Scholar]
- Libby, P.A. Heat and mass transfer at a general three-dimensional stagnation point. AIAA J. 1967, 5, 507–517. [Google Scholar] [CrossRef][Green Version]
- Chiam, T.C. Stagnation-point flow towards a stretching plate. J. Phys. Soc. Jpn. 1994, 63, 2443–2444. [Google Scholar] [CrossRef]
- Takhar, H.S.; Soundalgekar, V.M.; Gupta, A.S. Mixed convection of an incompressible viscous fluid in a porous medium past a hot vertical plate. Int. J. Nonlinear Mech. 1990, 25, 723–728. [Google Scholar] [CrossRef]
- Chamkha, A.J. Hydromagnetic mixed convection stagnation flow with suction and blowing. Int. Commun. Heat Mass Transf. 1998, 25, 417–426. [Google Scholar] [CrossRef]
- Abdelkhalek, M.M. The skin friction in the MHD mixed convection stagnation point with mass transfer. Int. Commun. Heat Mass Transf. 2006, 33, 249–258. [Google Scholar] [CrossRef]
- Jamaludin, A.; Nazar, R.; Pop, I. Three-dimensional mixed convection stagnation-point flow over a permeable vertical stretching/shrinking surface with a velocity slip. Chin. J. Phys. 2017, 55, 1865–1882. [Google Scholar] [CrossRef]
- Naganthran, K.; Basir, M.F.M.; Alharbi, S.O.; Nazar, R.; Alwatban, A.M.; Tlili, I. Stagnation point flow with time-dependent bionanofluid past a sheet: Richardson extrapolation technique. Processes 2019, 7, 722. [Google Scholar] [CrossRef]
- Zainal, N.A.; Nazar, R.; Naganthran, K.; Pop, I. Unsteady stagnation point flow of hybrid nanofluid past a convectively heated stretching/shrinking sheet with velocity slip. Mathematics 2020, 8, 1649. [Google Scholar] [CrossRef]
- Khashi’ie, N.S.; Arifin, N.; Pop, I. Mixed convective stagnation point flow towards a vertical Riga plate in hybrid Cu-Al2O3/water nanofluid. Mathematics 2020, 8, 912. [Google Scholar] [CrossRef]
- Khashi’ie, N.S.; Arifin, N.; Hafidzuddin, E.H.; Wahi, N.; Pop, I. Mixed convective stagnation point flow of a thermally stratified hybrid Cu-Al2O3/water nanofluid over a permeable stretching/shrinking sheet. ASM Sci. J. 2019, 12, 17–25. [Google Scholar]
- Zainal, N.A.; Nazar, R.; Naganthran, K.; Pop, I. Unsteady three-dimensional MHD non-axisymmetric Homann stagnation point flow of a hybrid nanofluid with stability analysis. Mathematics 2020, 8, 784. [Google Scholar] [CrossRef]
- Noor, A.; Nazar, R.; Naganthran, K. Unsteady mixed convection flow at a three-dimensional stagnation point. Int. J. Numer. Methods Heat Fluid Flow 2021, 31, 236–250. [Google Scholar] [CrossRef]
- Takabi, B.; Salehi, S. Augmentation of the heat transfer performance of a sinusoidal corrugated enclosure by employing hybrid nanofluid. Adv. Mech. Eng. 2014, 6, 147059. [Google Scholar] [CrossRef]
- Ghalambaz, M.; Rosca, N.C.; Rosca, A.V.; Pop, I. Mixed convection and stability analysis of stagnation-point boundary layer flow and heat transfer of hybrid nanofluids over a vertical plate. Int. J. Numer. Methods Heat Fluid Flow 2020, 30, 3737–3754. [Google Scholar] [CrossRef]
- Eswara, A.T.; Nath, G. Effect of large injection rates on unsteady mixed convection flow at a three-dimensional stagnation point. Int. J. Nonlinear Mech. 1999, 34, 85–103. [Google Scholar] [CrossRef]
- Krishnaswamy, R.; Nath, G. Compressible boundary-layer flow at a three-dimensional stagnation point with massive blowing. Int. J. Heat Mass Transf. 1982, 25, 1639–1649. [Google Scholar] [CrossRef]
- Oztop, H.F.; Abu-Nada, E. Numerical study of natural convection in partially heated rectangular enclosures filled with nanofluids. Int. J. Heat Fluid Flow 2008, 29, 1326–1336. [Google Scholar] [CrossRef]
- Cheng, E.H.W.; Özişik, M.N.; Williams, J.C., III. Nonsteady three-dimensional stagnation-point flow. J. Appl. Mech. 1971, 38, 282–287. [Google Scholar] [CrossRef]
- Merrill, K.; Beauchesne, M.; Previte, J.; Paullet, J.; Weidman, P. Final steady flow near a stagnation point on a vertical surface in a porous medium. Int. J. Heat Mass Transf. 2006, 49, 4681–4686. [Google Scholar] [CrossRef]
- Weidman, P.D.; Kubitschek, D.G.; Davis, A.M.J. The effect of transpiration on self-similar boundary layer flow over moving surfaces. Int. J. Eng. Sci. 2006, 44, 730–737. [Google Scholar] [CrossRef]
- Harris, S.D.; Ingham, D.B.; Pop, I. Mixed convection boundary-layer flow near the stagnation point on a vertical surface in a porous medium: Brinkman model with slip. Transp. Porous Media 2009, 77, 267–285. [Google Scholar] [CrossRef]
- Shampine, L.F.; Gladwell, I.; Thompson, S. Solving ODEs with Matlab; Cambridge University Press: Cambridge, UK, 2003. [Google Scholar]
- Devi, S.P.A.; Devi, S.S.U. Numerical investigation of hydromagnetic hybrid Cu-Al2O3/water nanofluid flow over a permeable stretching sheet with suction. Int. J. Nonlinear Sci. Numer. Simul. 2016, 17, 249–257. [Google Scholar] [CrossRef]
- Devi, S.S.U.; Devi, S.P.A. Numerical investigation of three-dimensional hybrid Cu–Al2O3/water nanofluid flow over a stretching sheet with effecting Lorentz force subject to Newtonian heating. Can. J. Phys. 2016, 94, 490–496. [Google Scholar] [CrossRef]
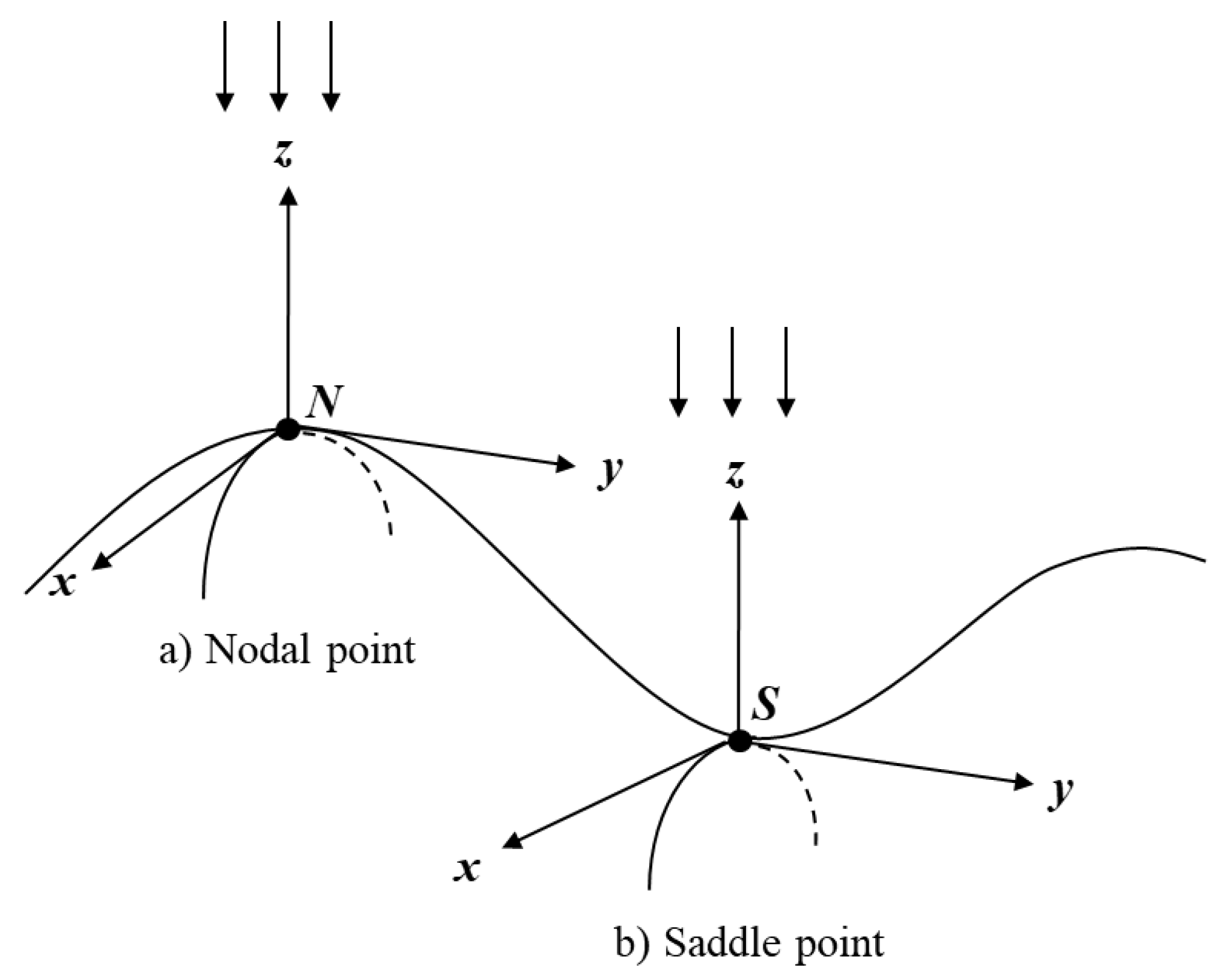

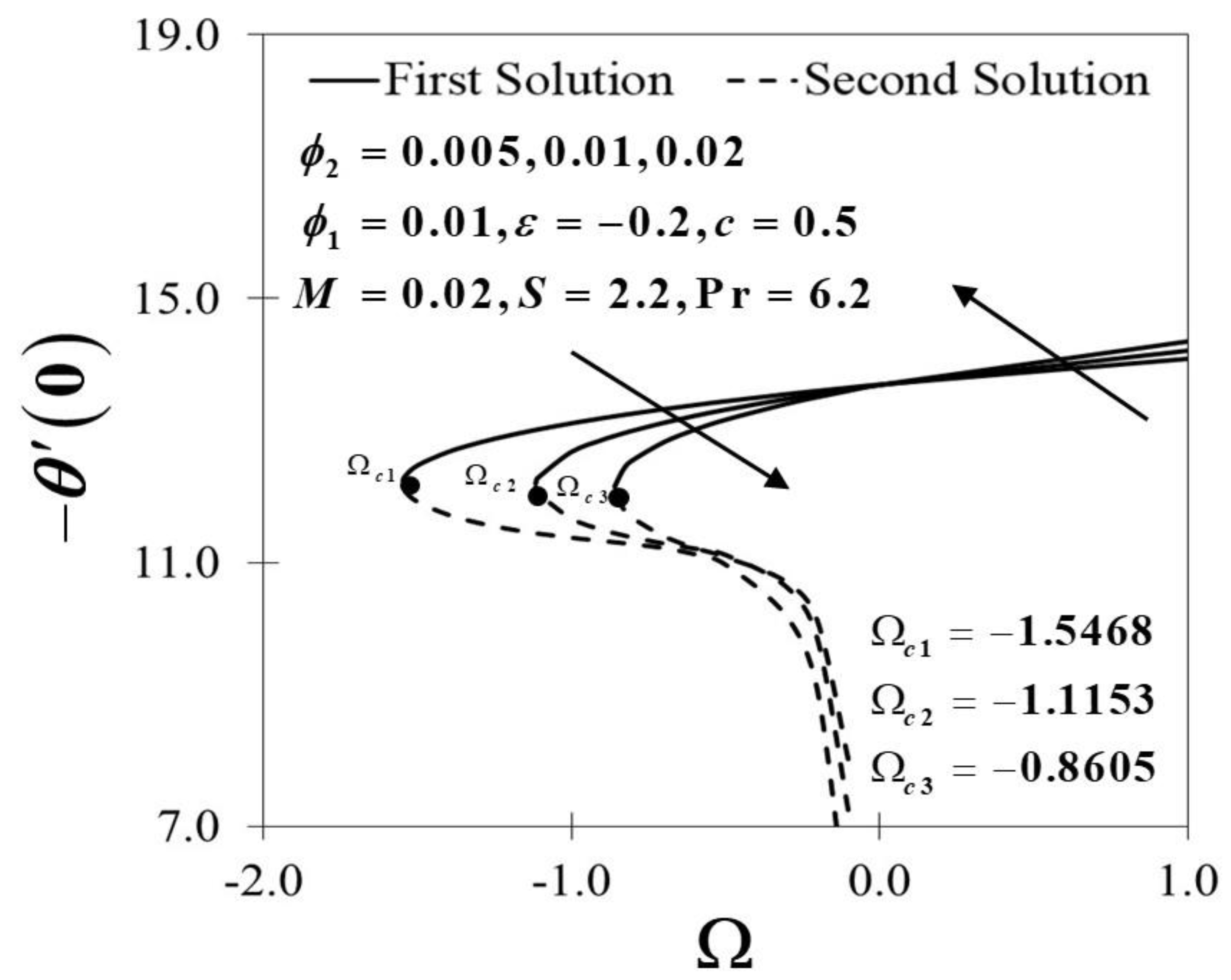
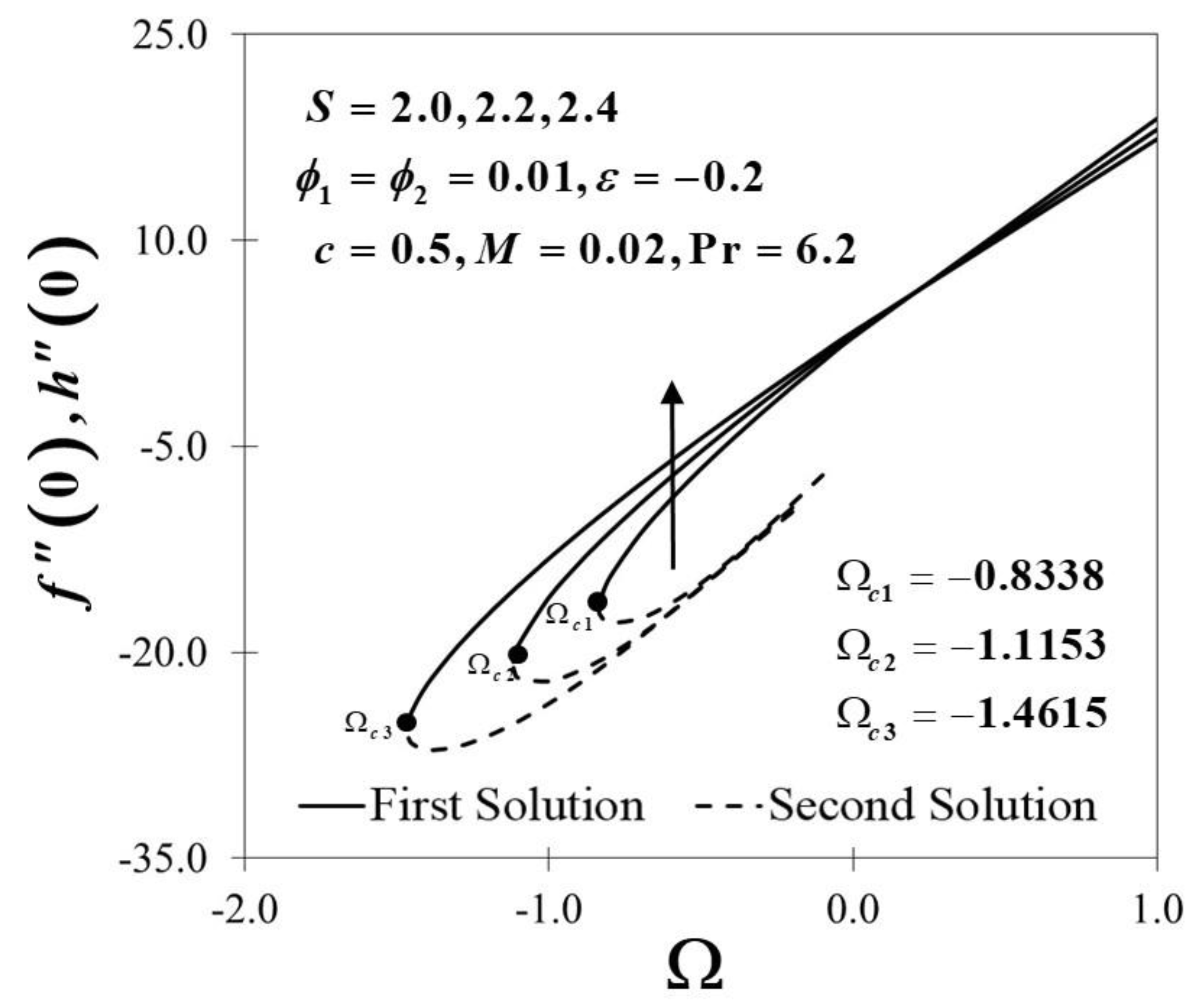



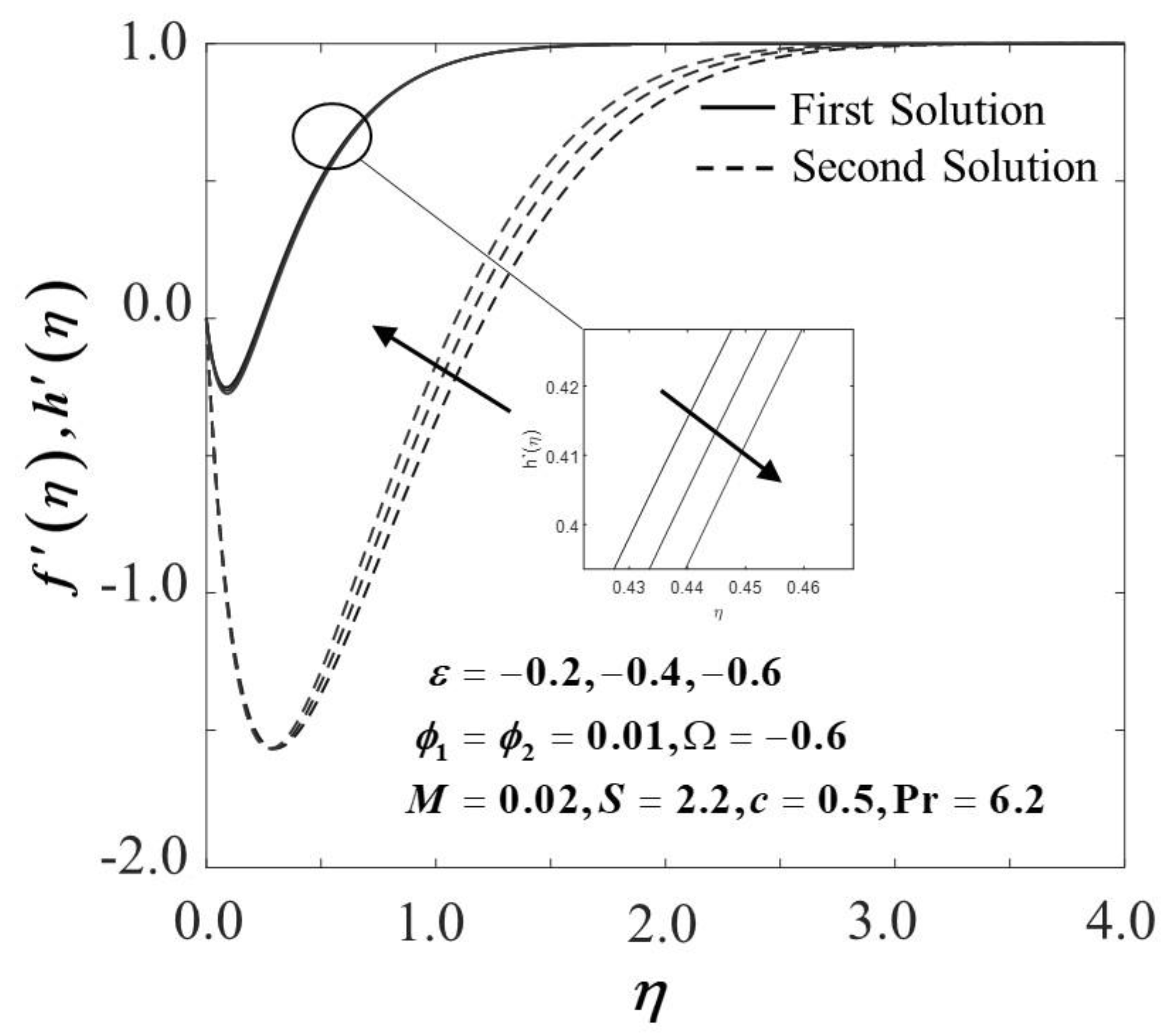




| Properties | Al2O3–Cu/H2O |
|---|---|
| Density | |
| Thermal capacity | |
| Dynamic viscosity | |
| Electrical conductivity | |
| Thermal conductivity |
| Physical Properties | Cu | Al2O3 | H2O |
|---|---|---|---|
| 400 | 40 | 0.613 | |
| 8933 | 3970 | 997.1 | |
| 385 | 765 | 4179 | |
| 1.67 | 0.85 | 21 |
| Present Result | Noor et al. [40] | Eswara and Nath [43] | ||||
|---|---|---|---|---|---|---|
| 1.00 | 1.311938 | 1.311938 | 1.31194 | 1.31194 | 1.3128 | 1.3128 |
| 0.75 | 1.288629 | 1.164316 | 1.28863 | 1.16432 | 1.2885 | 1.1642 |
| 0.50 | 1.266866 | 0.998111 | 1.26687 | 0.99811 | 1.2677 | 0.9980 |
| 0.25 | 1.247612 | 0.805137 | 1.24761 | 0.80514 | 1.2475 | 0.8050 |
| 0.00 | 1.232588 | 0.570465 | 1.23259 | 0.57047 | 1.2324 | 0.5706 |
| −0.25 | 1.225129 | 0.267950 | 1.22513 | 0.26795 | 1.2249 | 0.2671 |
| −0.50 | 1.230195 | −0.111500 | 1.23020 | −0.11150 | 1.2302 | −0.1110 |
| −0.75 | 1.247319 | −0.482131 | 1.24732 | −0.48219 | 1.2489 | −0.4975 |
| −1.00 | 1.271539 | −0.794493 | 1.27277 | −0.80950 | 1.2762 | −0.8226 |
| Present Result | Noor et al. [40] | |
|---|---|---|
| 1.00 | 0.665378 | 0.66538 |
| 0.75 | 0.623085 | 0.62308 |
| 0.50 | 0.579670 | 0.57967 |
| 0.25 | 0.536212 | 0.53621 |
| 0.00 | 0.495866 | 0.49587 |
| −0.25 | 0.467776 | 0.46778 |
| −0.50 | 0.470589 | 0.47059 |
| −0.75 | 0.507541 | 0.50755 |
| −1.00 | 0.562037 | 0.56595 |
First Solution | Second Solution | |
|---|---|---|
| −0.8 | 1.6793 | −2.9220 |
| −0.83 | 1.0506 | −2.3993 |
| −0.84 | 0.7756 | −2.1585 |
| −0.848 | 0.5036 | −1.9130 |
| −0.855 | 0.1818 | −1.6126 |
| −0.856 | 0.1217 | −1.5557 |
| −0.857 | 0.0552 | −1.4919 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zainal, N.A.; Nazar, R.; Naganthran, K.; Pop, I. Unsteady MHD Mixed Convection Flow in Hybrid Nanofluid at Three-Dimensional Stagnation Point. Mathematics 2021, 9, 549. https://doi.org/10.3390/math9050549
Zainal NA, Nazar R, Naganthran K, Pop I. Unsteady MHD Mixed Convection Flow in Hybrid Nanofluid at Three-Dimensional Stagnation Point. Mathematics. 2021; 9(5):549. https://doi.org/10.3390/math9050549
Chicago/Turabian StyleZainal, Nurul Amira, Roslinda Nazar, Kohilavani Naganthran, and Ioan Pop. 2021. "Unsteady MHD Mixed Convection Flow in Hybrid Nanofluid at Three-Dimensional Stagnation Point" Mathematics 9, no. 5: 549. https://doi.org/10.3390/math9050549
APA StyleZainal, N. A., Nazar, R., Naganthran, K., & Pop, I. (2021). Unsteady MHD Mixed Convection Flow in Hybrid Nanofluid at Three-Dimensional Stagnation Point. Mathematics, 9(5), 549. https://doi.org/10.3390/math9050549







