Mathematical Analysis of the Process Forces Effect on Collet Chuck Holders
Abstract
1. Introduction
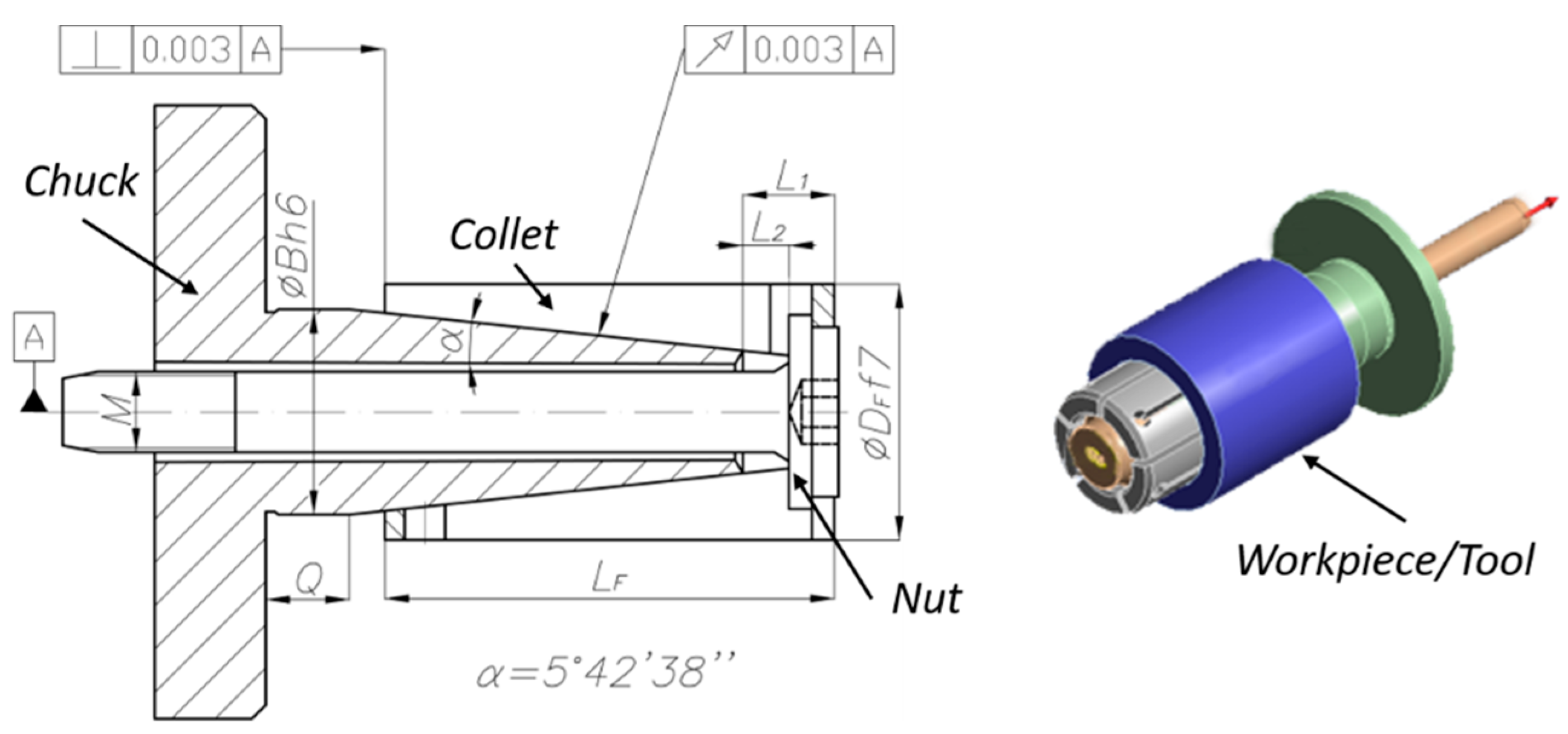
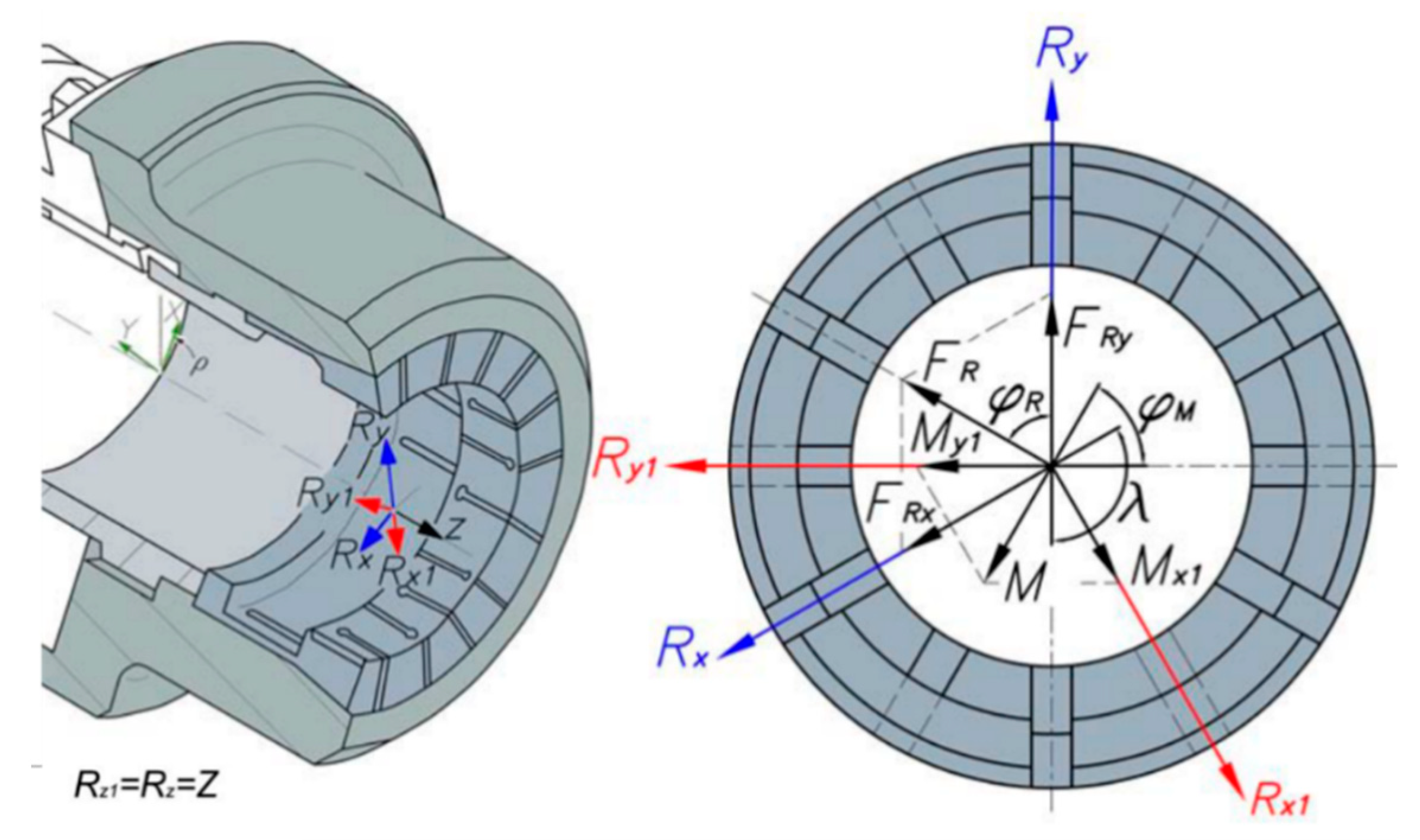
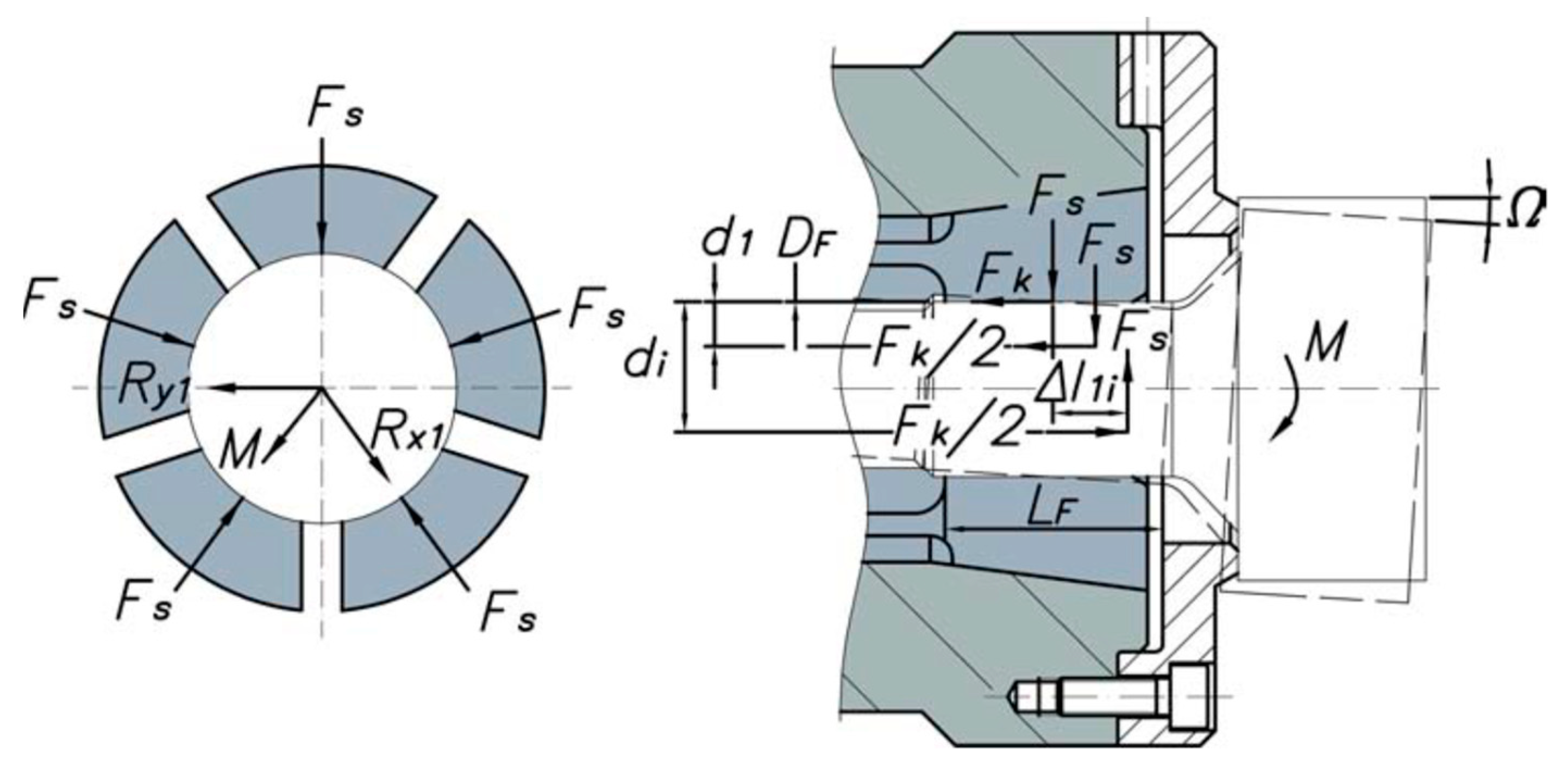
2. Determination of the Loads on the Collet Contact Elements
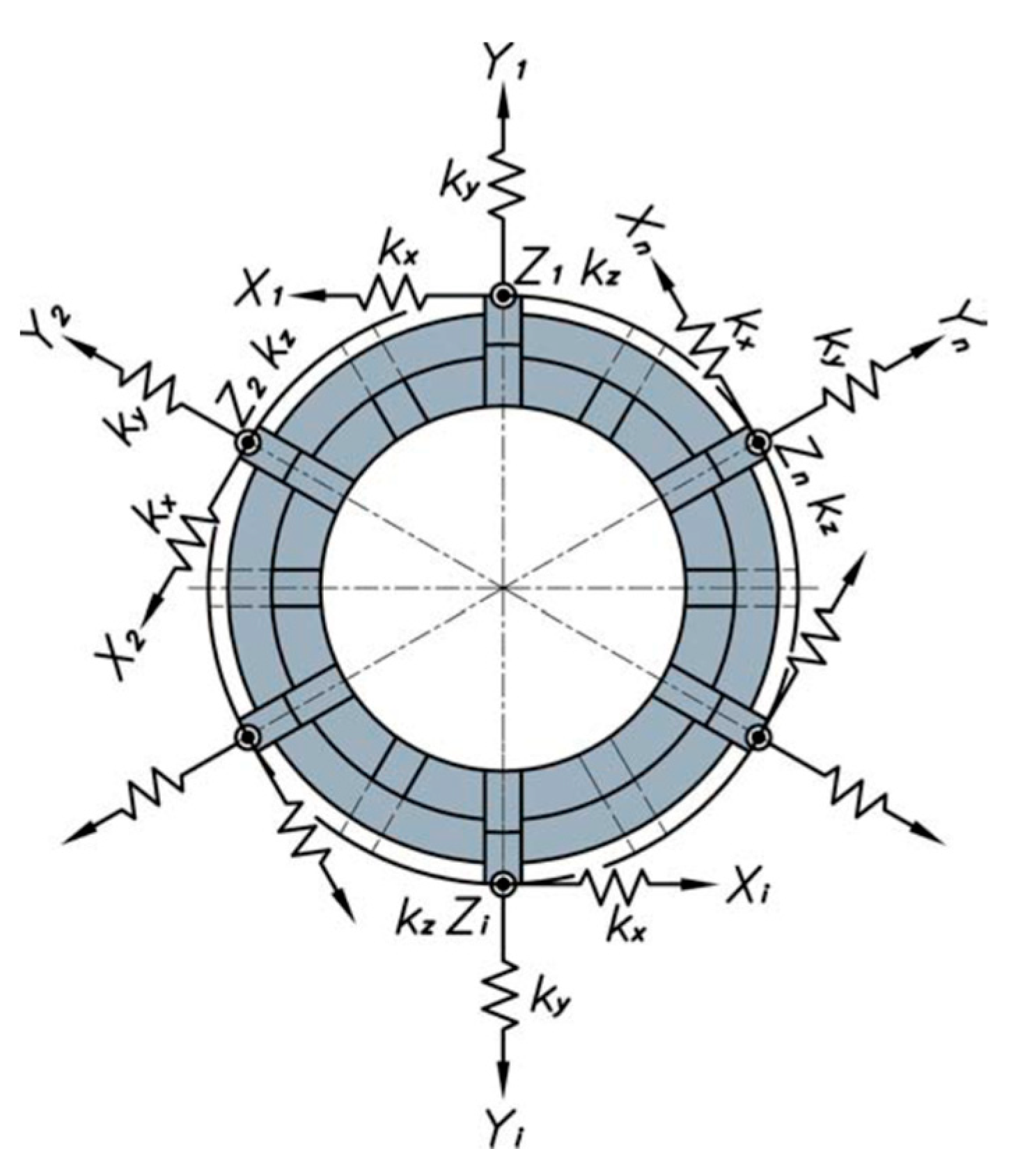
3. Strains by Radial Forces
4. Strains by Bending Moments
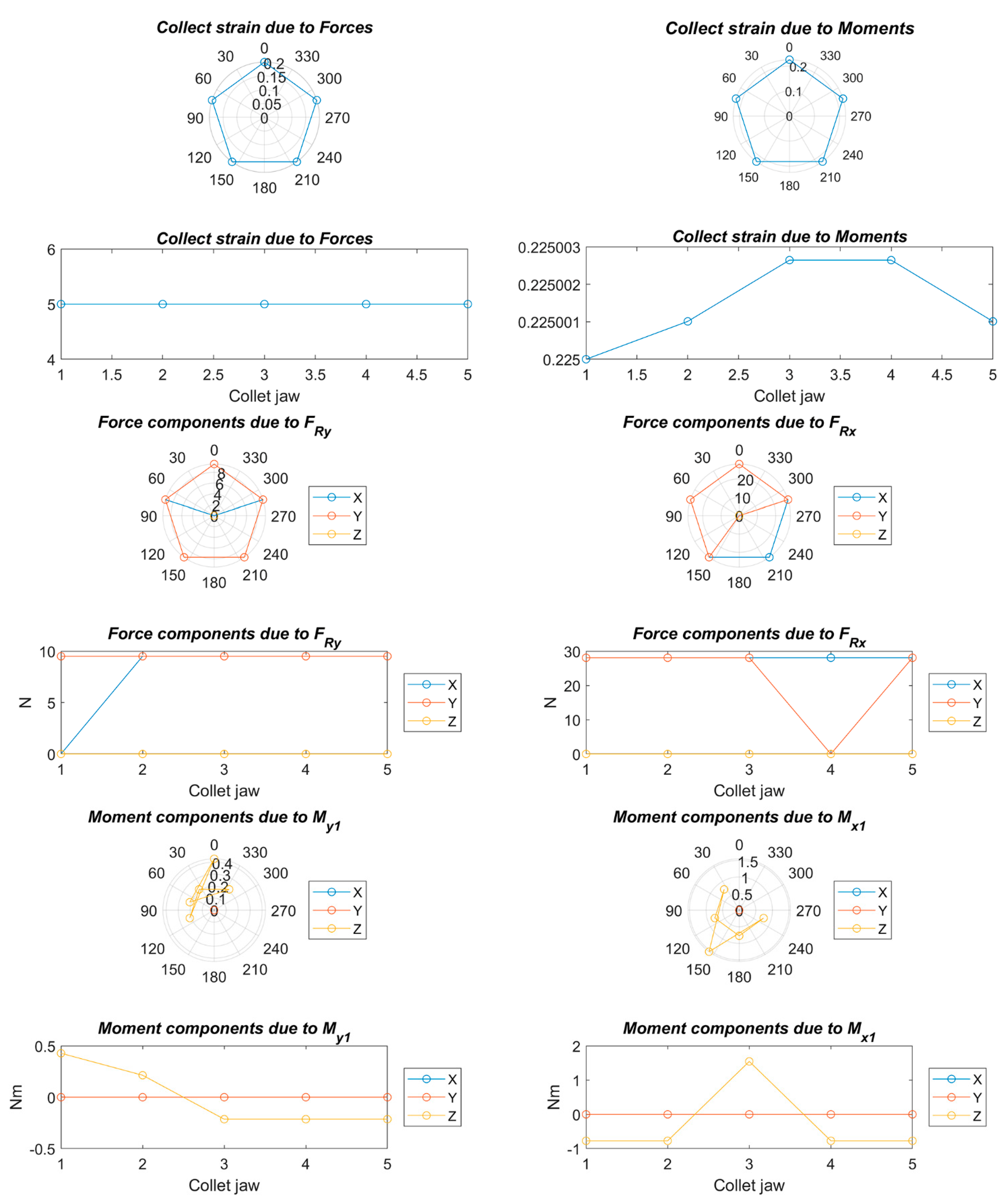
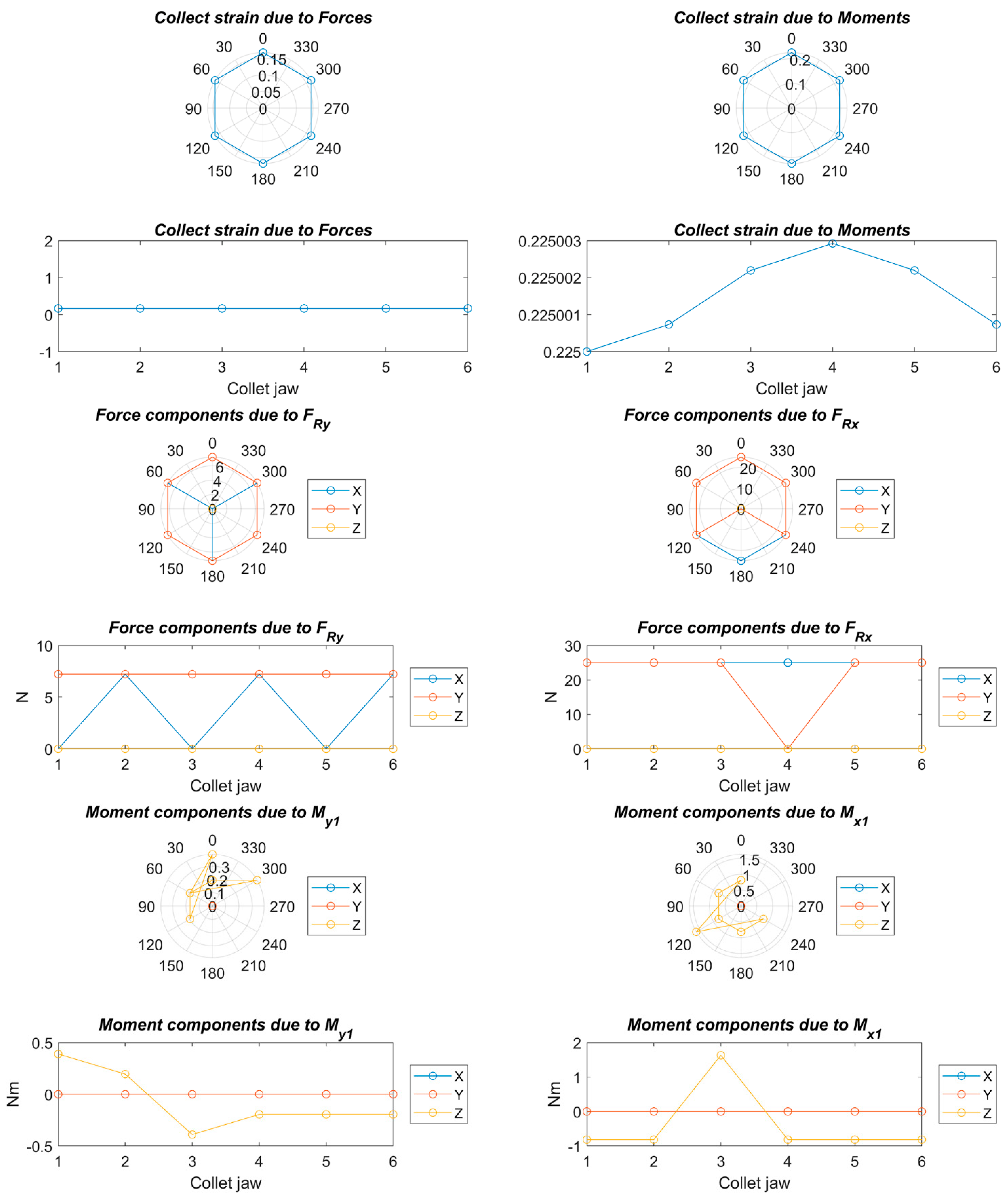
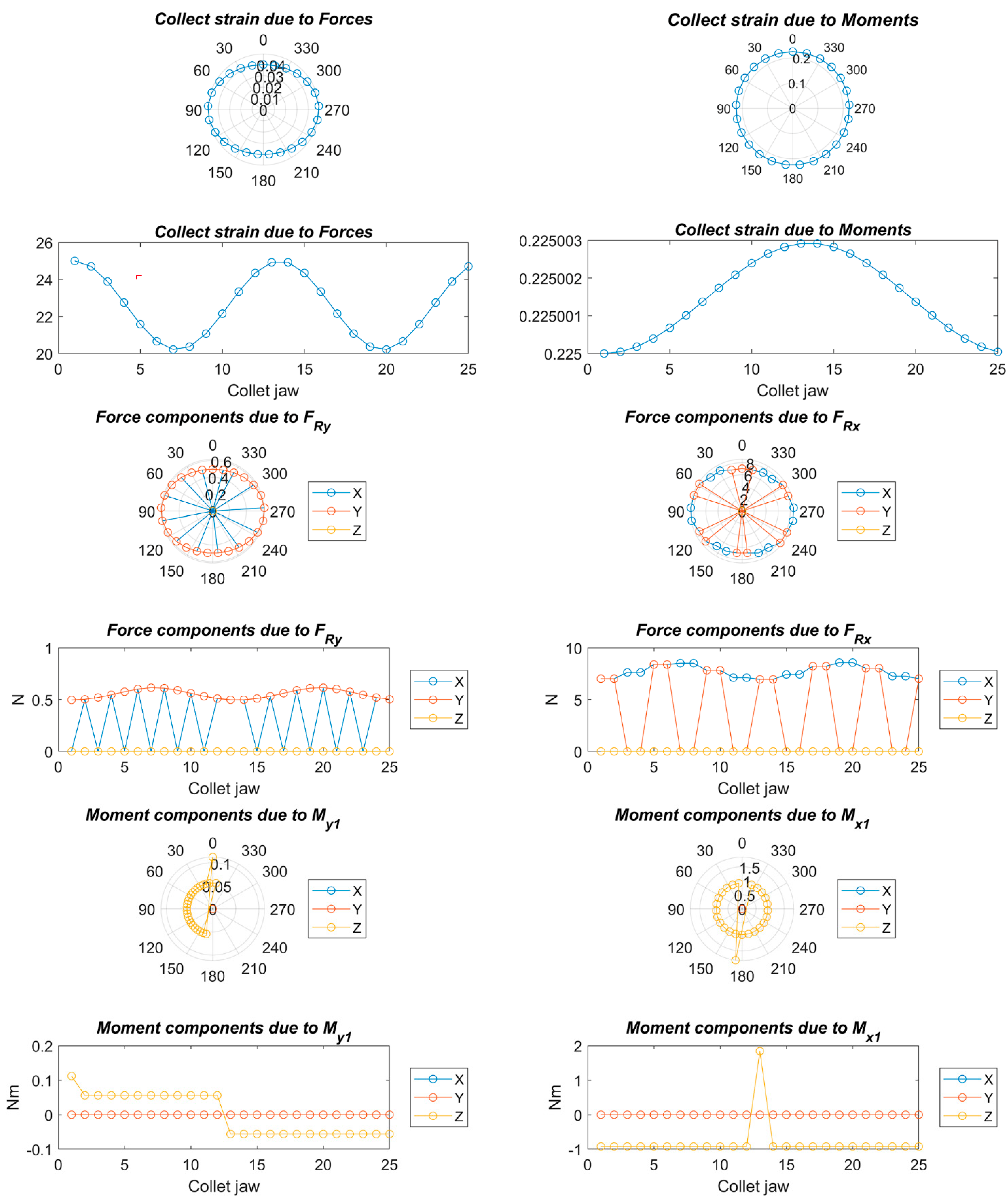
5. Results
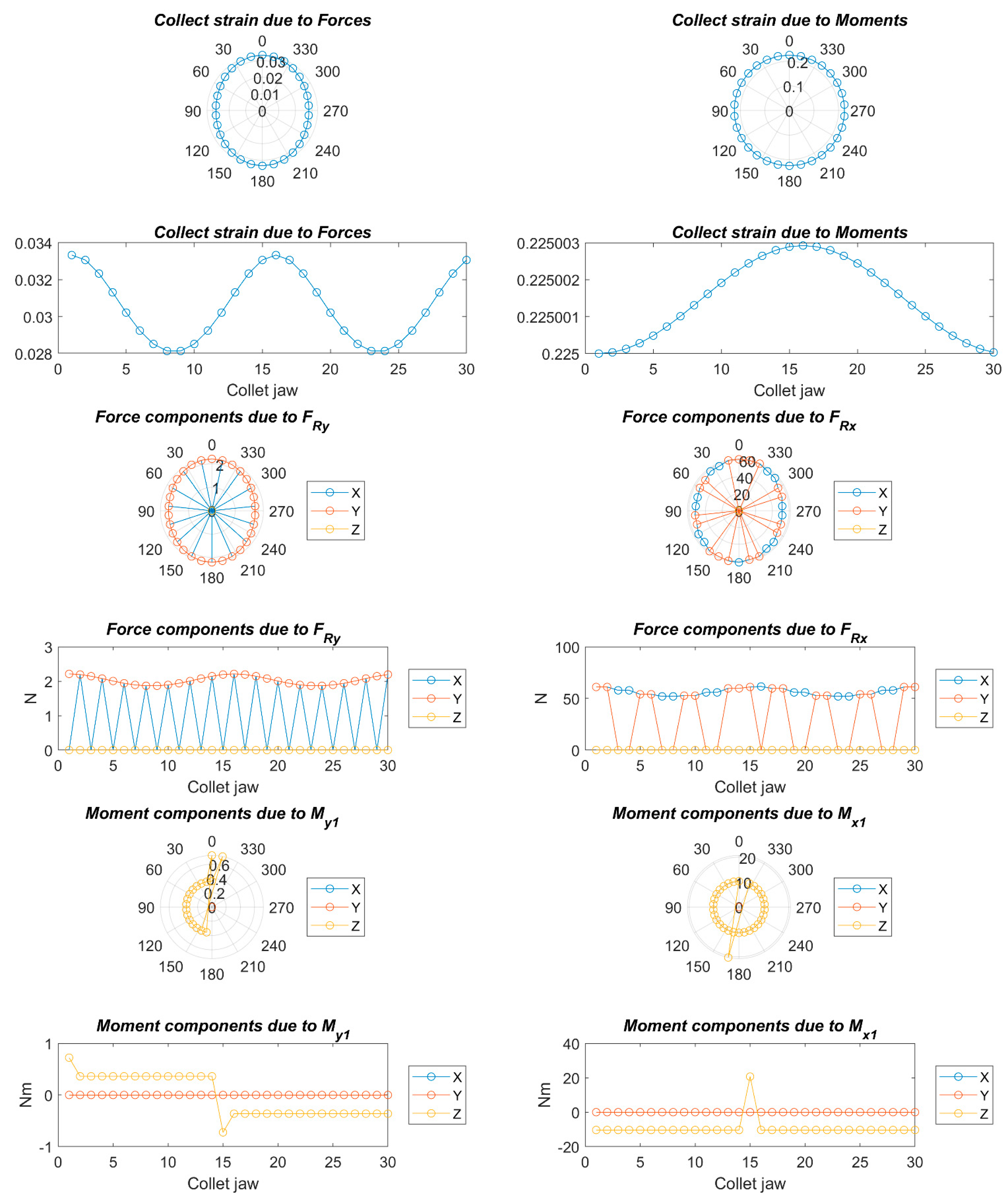
5.1. Strains Due to Radial Forces
5.2. Strains due to Bending Moments
5.3. Total Strains
6. Conclusions
- The stiffness of the collet chuck holder, and thus the equipment that presents a lower structural rigidity will reduce the variations in the clamping force due to the centrifugal forces caused by a high rotational speed.
- The stiffness of the workpiece is always satisfied when the workpiece is more rigid than the contact elements or collet jaws, which is fulfilled in the case of metal workpieces. In less common cases, in which the workpiece is less rigid than the contact elements or collet jaws, the rotational speed has no influence.
- It is important that the collet stiffness is uniform to minimize the deformations that affect the final product. For this reason, it is very common to use vulcanized collets.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- VDI-Fachbereich Produktionstechnik und Fertigungsverfahren VDI 3106: Determination of permissible speed (rpm) of lathe chucks (jaw chucks). In VDI manual Production Technology and Manufacturing Methods—Volume 3: Production Equipment; VDI-Gesellschaft Produktion und Logistik: Düsseldorf, Germany, 2004.
- Anselmetti, B. Generation of functional tolerancing based on positioning features. Comput. Des. 2006, 38, 902–919. [Google Scholar] [CrossRef]
- Soriano, E.; Rubio, H.; García-Prada, J.C. Analysis of the clamping mechanisms of collet chucks holders for turning. In New Trends in Mechanism and Machine Science; Springer: Dordrecht, The Netherlands, 2013; Volume 7, pp. 391–398. [Google Scholar] [CrossRef]
- Nyamekye, K.; Mudiam, S.S. A model for predicting the initial static gripping force in lathe chucks. Int. J. Adv. Manuf. Technol. 1992, 7, 286–291. [Google Scholar] [CrossRef]
- Rahman, M.; Tsutsumi, M. Effect of spindle speed on clamping force in turning. J. Mater. Process. Technol. 1993, 38, 407–415. [Google Scholar] [CrossRef]
- Ema, S.; Marui, E. Chucking Performance of a Wedge-Type Power Chuck. Trans. ASME J. Eng. Ind. 1994, 116, 70–77. [Google Scholar] [CrossRef]
- Rivin, E.I.; Agapiou, J.; Brecher, C.; Clewett, M.; Erickson, R.; Huston, F.; Kadowaki, Y.; Lenz, E.; Moriwaki, T.; Pitsker, A.; et al. Tooling Structure: Interface between Cutting Edge and Machine Tool. CIRP Ann. 2000, 49, 591–634. [Google Scholar] [CrossRef]
- Soriano, E.; Rubio, H.; García-Prada, J.C. Models for Determining the Static Stiffness of Collet Sleeves. In New Advances in Mechanisms, Transmissions and Applications; Springer: Dordrecht, The Netherlands, 2014; Volume 17, pp. 375–383. [Google Scholar]
- Xu, C.; Zhang, J.; Wu, Z.; Yu, D.; Feng, P. Dynamic modeling and parameters identification of a spindle–holder taper joint. Int. J. Adv. Manuf. Technol. 2013, 67, 1517–1525. [Google Scholar] [CrossRef]
- Xu, C.; Zhang, J.; Feng, P.; Yu, D.; Wu, Z. Characteristics of stiffness and contact stress distribution of a spindle–holder taper joint under clamping and centrifugal forces. Int. J. Mach. Tools Manuf. 2014, 82–83, 21–28. [Google Scholar] [CrossRef]
- Liu, G.; Hong, J.; Wu, W.; Sun, Y. Investigation on the influence of interference fit on the static and dynamic characteristics of spindle system. Int. J. Adv. Manuf. Technol. 2018, 99, 1953–1966. [Google Scholar] [CrossRef]
- Heras, E.S.; Rubio, H.; Bustos, A.; García Prada, J.C. Automatic Expanding Mandrel with Air Sensing Device: Design and Analysis. Appl. Sci. 2020, 10, 2551. [Google Scholar] [CrossRef]
- Mai, T.D.; Ryu, J. Effects of Leading-Edge Modification in Damaged Rotor Blades on Aerodynamic Characteristics of High-Pressure Gas Turbine. Mathematics 2020, 8, 2191. [Google Scholar] [CrossRef]
- Liang, Z.; Cui, C.; Meng, K.; Xin, Y.; Pei, H.; Li, H. New Analytical Solutions for Longitudinal Vibration of a Floating Pile in Layered Soils with Radial Heterogeneity. Mathematics 2020, 8, 1294. [Google Scholar] [CrossRef]
- Fetecau, C.; Ellahi, R.; Sait, S.M. Mathematical Analysis of Maxwell Fluid Flow through a Porous Plate Channel Induced by a Constantly Accelerating or Oscillating Wall. Mathematics 2021, 9, 90. [Google Scholar] [CrossRef]
- Rezvani, S.; Kim, C.-J.; Park, S.S.; Lee, J. Simultaneous Clamping and Cutting Force Measurements with Built-In Sensors. Sensors 2020, 20, 3736. [Google Scholar] [CrossRef]
- Santos, E.; Richter, H. Design and Analysis of Novel Actuation Mechanism with Controllable Stiffness. Actuators 2019, 8, 12. [Google Scholar] [CrossRef]
- Fadyushin, I.L.; Musykant, Y.A.; Messheryakov, A.I. Tooling for cnc machine tool. Mashinostorenie Publ. 1990. [Google Scholar]
- Rotberg, J.; Lenz, E.; Levin, M. Drill and clamping interface in high-performance drilling. Int. J. Adv. Manuf. Technol. 1998, 14, 229–238. [Google Scholar] [CrossRef]
- X-tra Long Tool Holders, Elderfield & Hall: Knoxville, TN, USA, 1999.
- V-Flange Holders, Fitz-Rite Products, Inc.: Troy, MI, USA, 2000.
- Soriano, E.; Ramirez, M.B.; Rubio, H. Model for determining the clamping force in expanding mandrels for high-speed turning. Int. Rev. Mech. Eng. 2012, 6, 384–389. [Google Scholar]
- Namazi, M.; Altintas, Y.; Abe, T.; Rajapakse, N. Modeling and identification of tool holder–spindle interface dynamics. Int. J. Mach. Tools Manuf. 2007, 47, 1333–1341. [Google Scholar] [CrossRef]
- Xu, C.; Zhang, J.; Yu, D.; Wu, Z.; Feng, P. Dynamics prediction of spindle system using joint models of spindle tool holder and bearings. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2015, 229, 3084–3095. [Google Scholar] [CrossRef]
- Criado, V.; Feito, N.; Cantero Guisández, J.L.; Díaz-Álvarez, J. A New Cutting Device Design to Study the Orthogonal Cutting of CFRP Laminates at Different Cutting Speeds. Materials (Basel) 2019, 12, 4074. [Google Scholar] [CrossRef]
- Moufki, A.; Devillez, A.; Dudzinski, D.; Molinari, A. Thermomechanical modelling of oblique cutting and experimental validation. Int. J. Mach. Tools Manuf. 2004, 44, 971–989. [Google Scholar] [CrossRef]
- Sutter, G.; Molinari, A. Analysis of the Cutting Force Components and Friction in High Speed Machining. J. Manuf. Sci. Eng. 2005, 127, 245–250. [Google Scholar] [CrossRef]

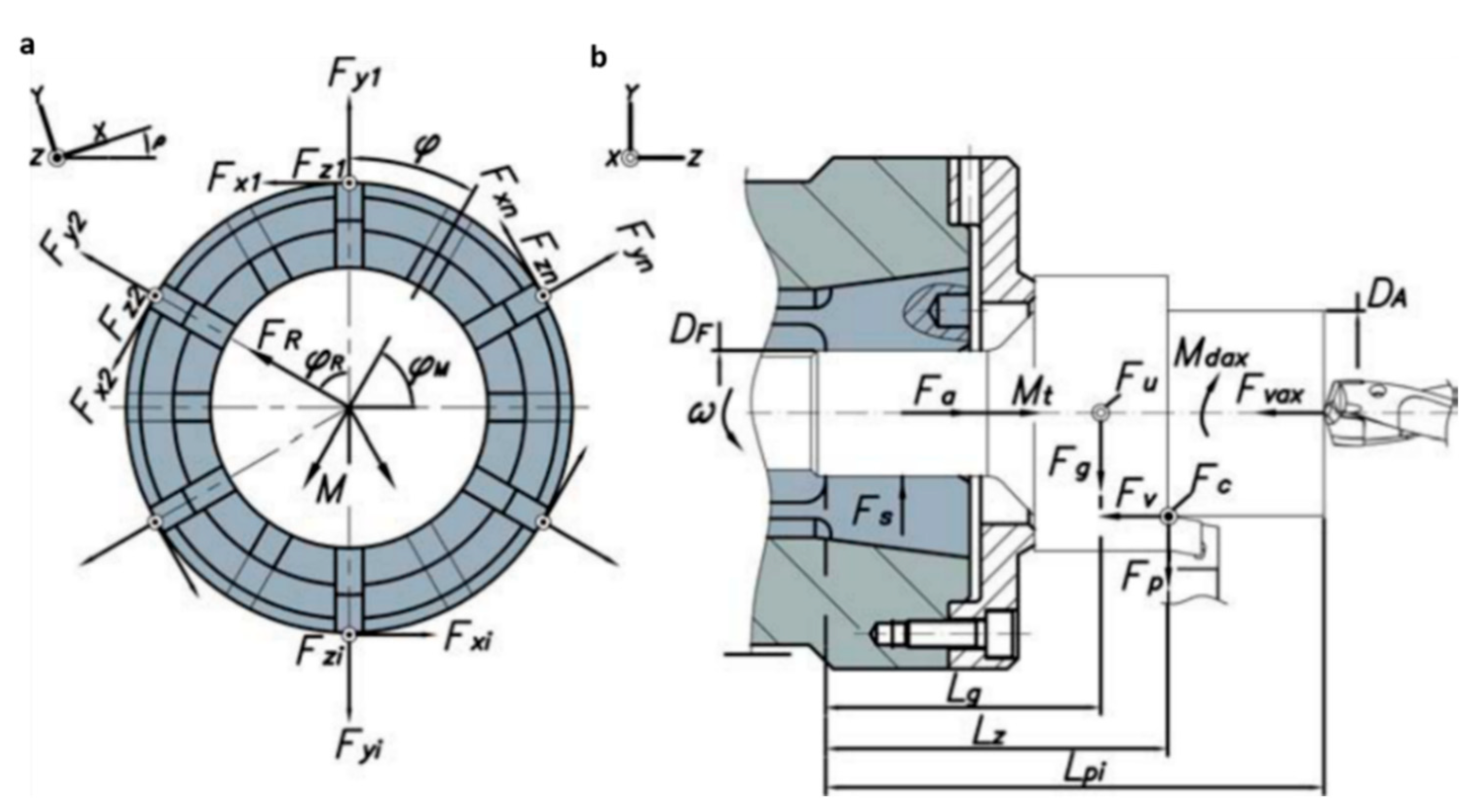

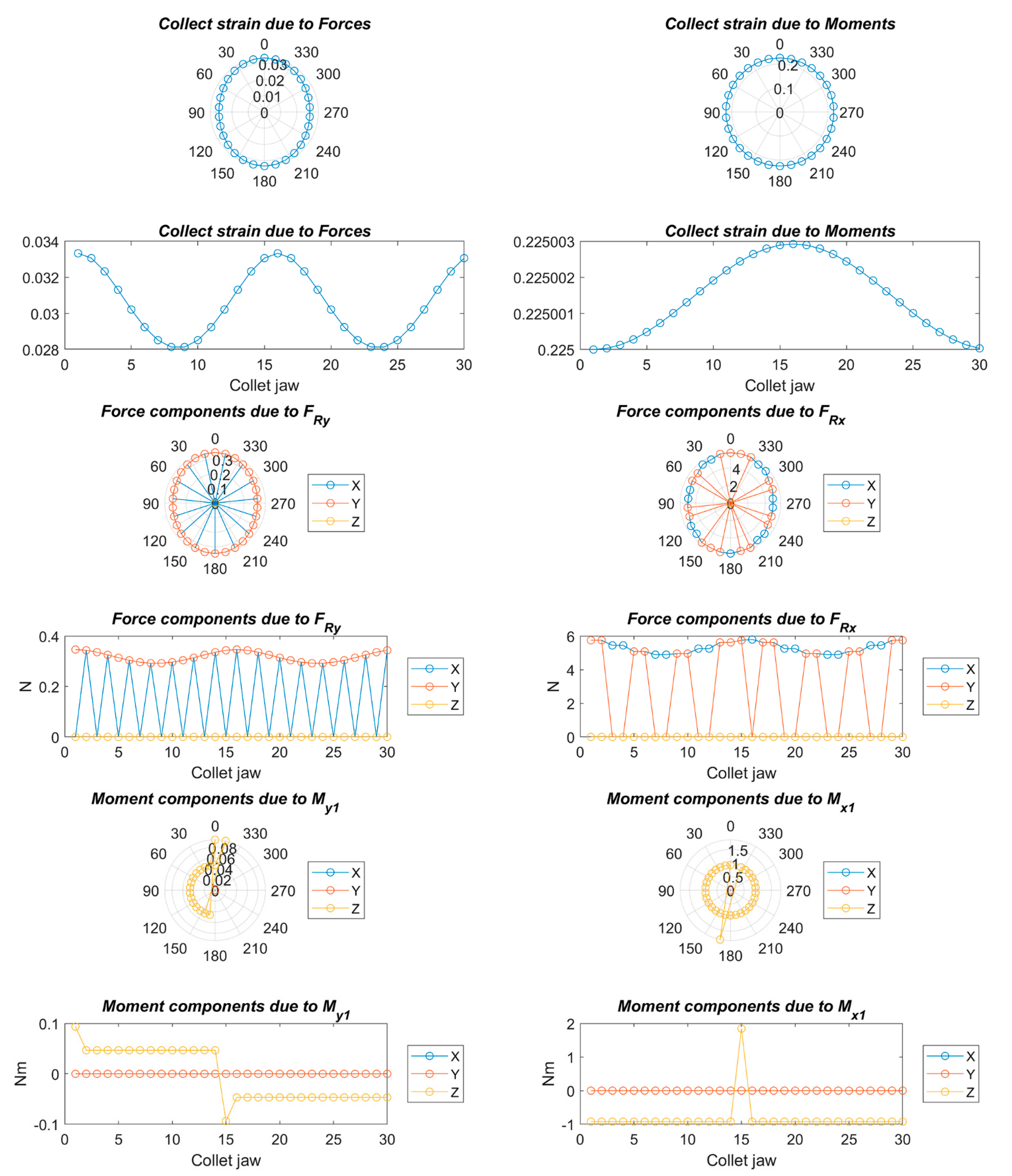
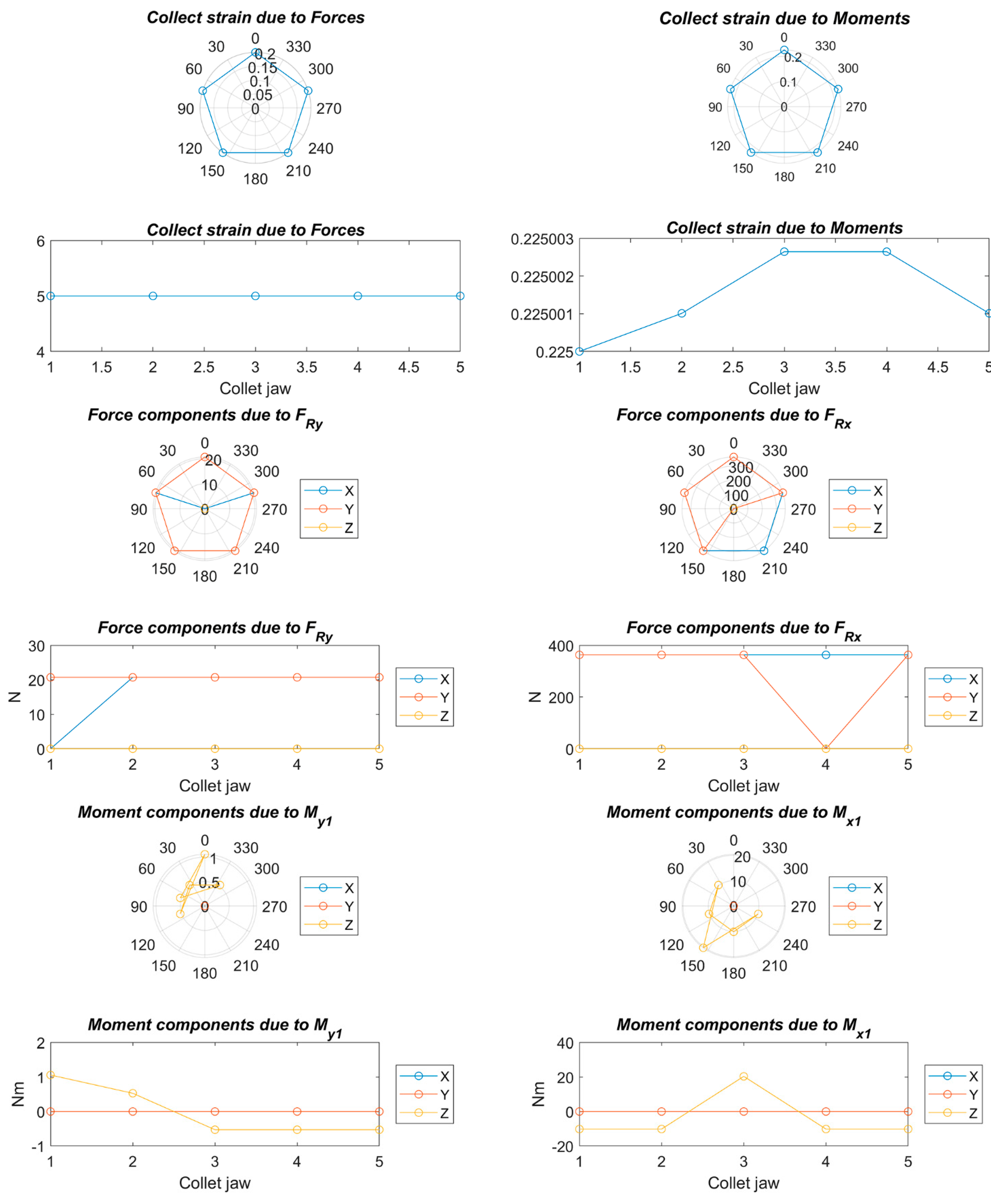
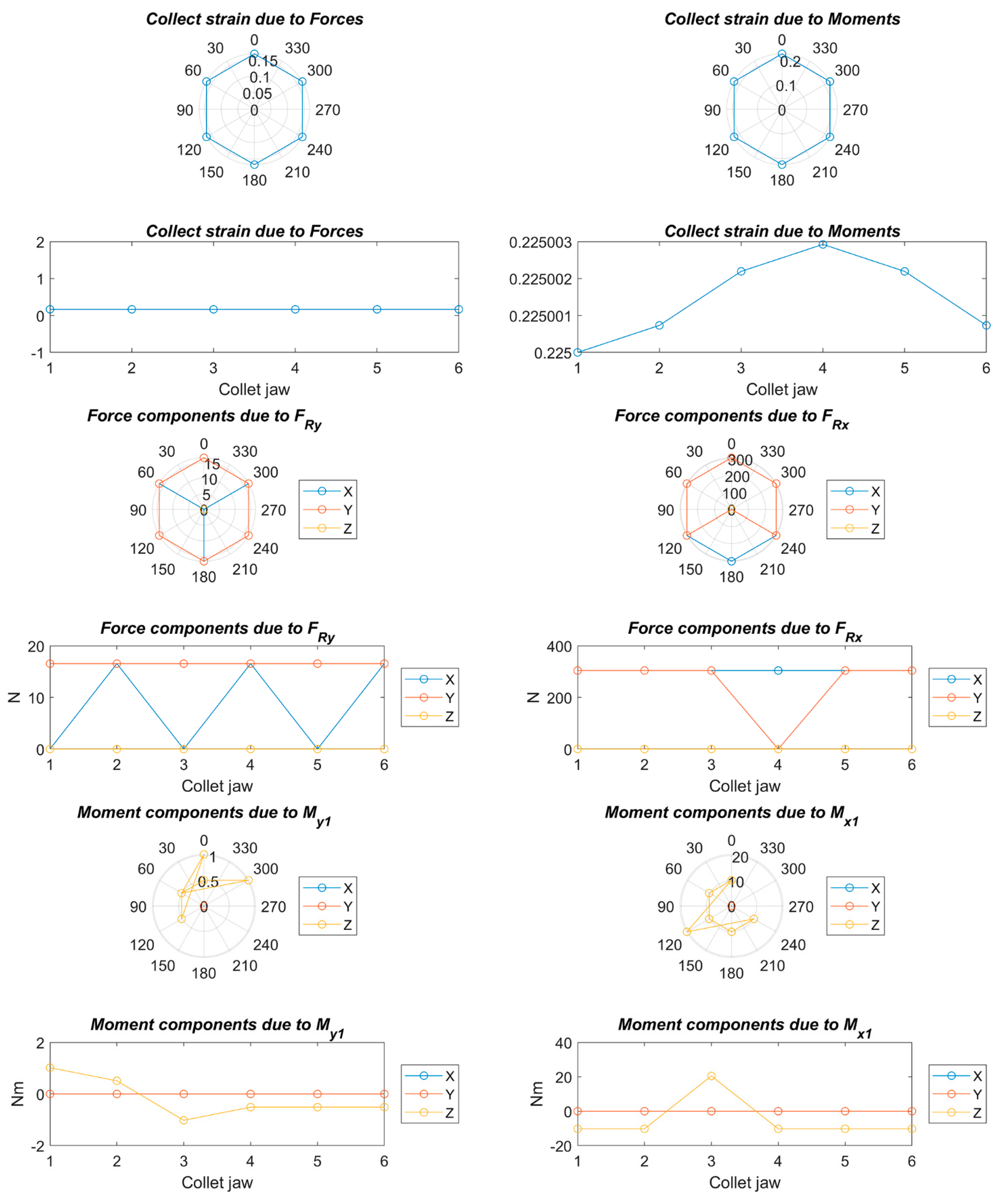
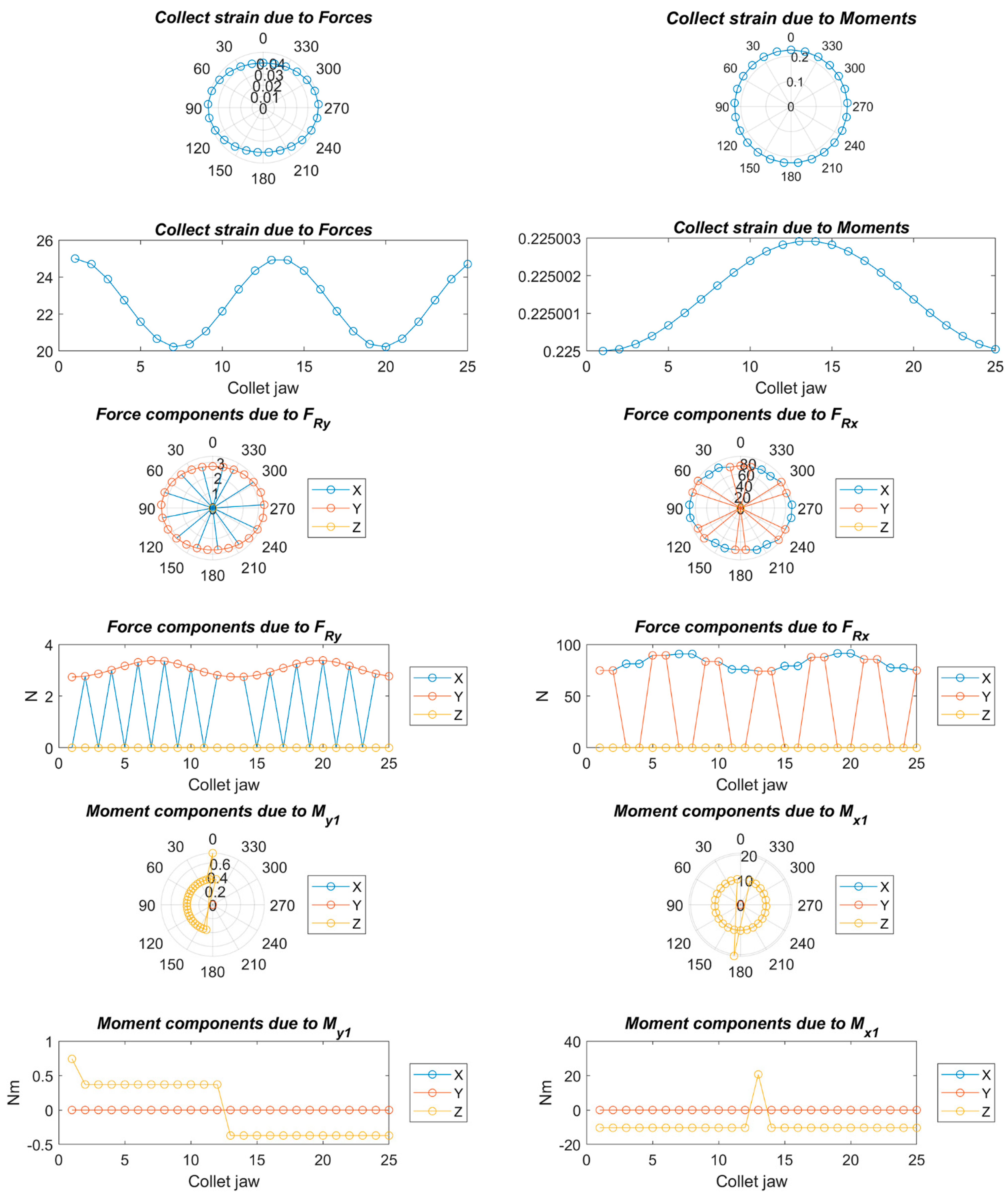
| Process | Fa(N) Feed Force | Fc = Fu(N) Cutting Force | Fp(N) Thrust Force | Fg (N) Weight | Mdax (Nm) Drilling Torque | χH Machine Bed Angle | nc Collet Jaws | ρ = ϑ Collet Jaws Angle | C = kx/ky Stiffness Relation | Lg(m) Gravity Center | Lz(m) Application Point | ||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Min | Max | Min | Max | Min | Max | Min | Max | ||||||||
| Orthogonal | 90 | 440 | 125 | 205 | 0 | 0 | 50 | 0 | 0 | 0 | 5 | 72 | 1 | 0.02 | 0.025 |
| 90 | 440 | 125 | 205 | 0 | 0 | 50 | 0 | 0 | 0 | 6 | 60 | 1 | 0.02 | 0.025 | |
| 90 | 440 | 125 | 205 | 0 | 0 | 50 | 0 | 0 | 0 | 25 | 14.40 | 0.8 | 0.02 | 0.025 | |
| 90 | 440 | 125 | 205 | 0 | 0 | 50 | 0 | 0 | 0 | 30 | 12 | 1.2 | 0.02 | 0.025 | |
| Oblique | 503 | 1042 | 758 | 1800 | 17 | 56 | 50 | 0 | 0 | 0 | 5 | 72 | 1 | 0.02 | 0.025 |
| 503 | 1042 | 758 | 1800 | 17 | 56 | 50 | 0 | 0 | 0 | 6 | 60 | 1 | 0.02 | 0.025 | |
| 503 | 1042 | 758 | 1800 | 17 | 56 | 50 | 0 | 0 | 0 | 25 | 14.40 | 0.8 | 0.02 | 0.025 | |
| 503 | 1042 | 758 | 1800 | 17 | 56 | 50 | 0 | 0 | 0 | 30 | 12 | 1.2 | 0.02 | 0.025 | |
| Process | FRx (N) | FRy (N) | Mx1 (Nm) | My1 (Nm) | ||||
|---|---|---|---|---|---|---|---|---|
| Min | Max | Min | Max | Min | Max | Min | Max | |
| Orthogonal | 140.45 | 220.45 | 47.55 | 47.55 | 3.43 | 5.43 | 0.95 | 0.95 |
| 150 | 230 | 43.30 | 43.30 | 3.63 | 5.63 | 0.87 | 0.87 | |
| 173.43 | 253.65 | 12.43 | 11.53 | 4.09 | 6.10 | 0.25 | 0.23 | |
| 173.91 | 253.91 | 10.40 | 10.40 | 4.10 | 6.10 | 0.21 | 0.21 | |
| Oblique | 773.45 | 1815.45 | 64.55 | 103.55 | 19.26 | 45.31 | 1.38 | 2.35 |
| 783 | 1825 | 60.30 | 99.30 | 19.45 | 45.5 | 1.29 | 2.27 | |
| 806.65 | 1848.4 | 25.53 | 68.43 | 19.92 | 45.97 | 0.66 | 1.65 | |
| 806.91 | 1848.9 | 27.40 | 66.40 | 19.93 | 45.98 | 0.63 | 1.61 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Soriano-Heras, E.; Rubio, H.; Bustos, A.; Castejon, C. Mathematical Analysis of the Process Forces Effect on Collet Chuck Holders. Mathematics 2021, 9, 492. https://doi.org/10.3390/math9050492
Soriano-Heras E, Rubio H, Bustos A, Castejon C. Mathematical Analysis of the Process Forces Effect on Collet Chuck Holders. Mathematics. 2021; 9(5):492. https://doi.org/10.3390/math9050492
Chicago/Turabian StyleSoriano-Heras, Enrique, Higinio Rubio, Alejandro Bustos, and Cristina Castejon. 2021. "Mathematical Analysis of the Process Forces Effect on Collet Chuck Holders" Mathematics 9, no. 5: 492. https://doi.org/10.3390/math9050492
APA StyleSoriano-Heras, E., Rubio, H., Bustos, A., & Castejon, C. (2021). Mathematical Analysis of the Process Forces Effect on Collet Chuck Holders. Mathematics, 9(5), 492. https://doi.org/10.3390/math9050492









