Zero-Hopf Bifurcation in a Generalized Genesio Differential Equation
Abstract
1. Introduction and Statement of the Main Results
2. Preliminaries
2.1. Results from Averaging Theory
- (i)
- are locally Lipschitz in x and Q is twice differentiable with respect to
- (ii)
- Let be a bounded and open set, for each , there exists such that and.
3. Proof of Proposition 1 and Theorem 1
4. Numerical Verification of the Analytic Results Obtained
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Cardin, P.T.; Llibre, J. Transcritical and zero-Hopf bifurcations in the Genesio system. Nonlinear Dyn. 2016, 88, 547–553. [Google Scholar] [CrossRef]
- Sanders, J.A.; Verhulst, F. Averaging Methods in Nonlinear Dynamical Systems; Springer: New York, NY, USA, 2007. [Google Scholar]
- Buzzi, C.; Llibre, J.; Medrado, J. Hopf and zero-Hopf bifurcations in the Hindmarsh-Rose system. Nonlinear Dyn. 2016, 83, 1549–1556. [Google Scholar] [CrossRef]
- Castellanos, V.; Llibre, J.; Quilantan, I. Simultaneous periodic orbits bifurcating from two zero-Hopf equilibria in a tritrophic food chain model. J. Appl. Math. Phys. 2013, 1, 31–38. [Google Scholar] [CrossRef][Green Version]
- Llibre, J. Periodic orbits in the zero-hopf bifurcation of the Rössler system. Rom. Astron. J. 2014, 24, 49–60. [Google Scholar]
- Llibre, J.; Oliveira, R.D.; Valls, C. On the integrability and the zero-Hopf bifurcation of a Chen-Wang differential system. Nonlinear Dyn. 2015, 80, 353–361. [Google Scholar] [CrossRef]
- Llibre, J.; Mereu, A.C.; Teixeira, M.A. Limit cycles of the generalized polynomial Liénard differential equations. Math. Proc. Camb. Philos. Soc. 2010, 148, 363–383. [Google Scholar] [CrossRef]
- Feddaoui, A.; Llibre, J.; Chemseddine, B.; Makhlouf, A. Periodic solutions for differential systems in ℝ3 and ℝ4. Appl. Math. Nonlinear Sci. 2020. [Google Scholar] [CrossRef]
- Buică, A.; Llibre, J. Averaging methods for finding periodic orbits via Brouwer degree. Bull. Sci. Math. 2004, 128, 7–22. [Google Scholar] [CrossRef]
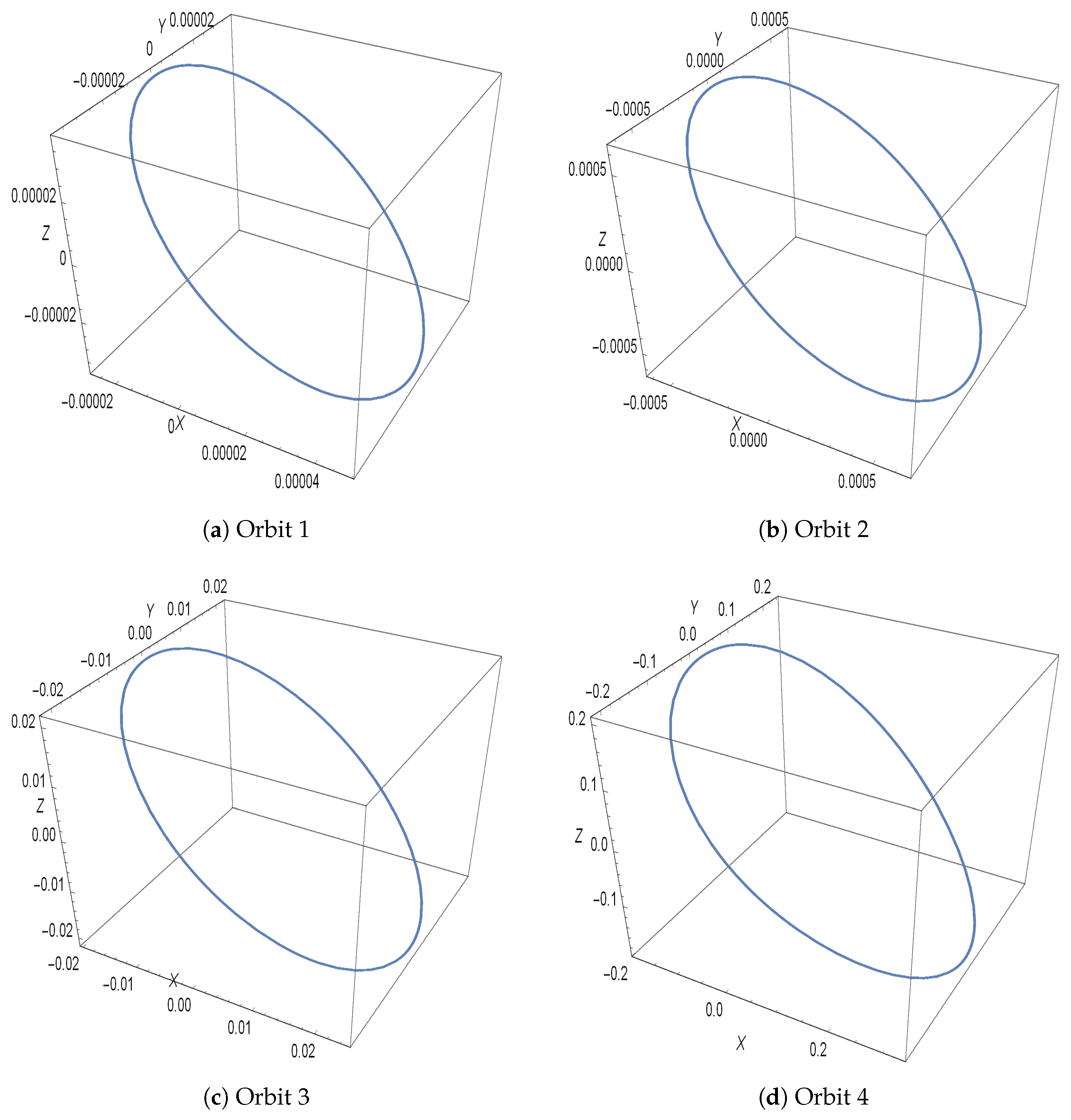
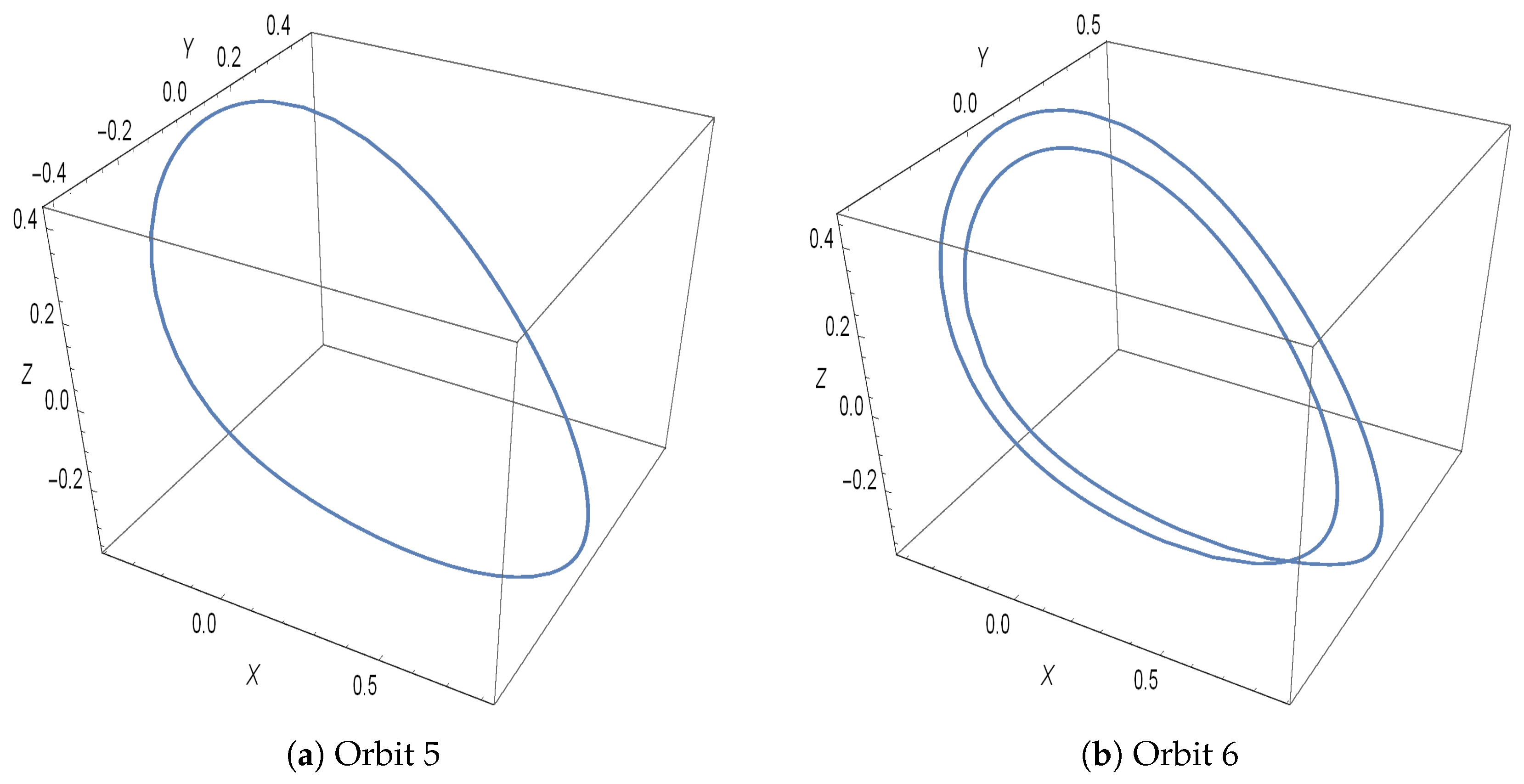
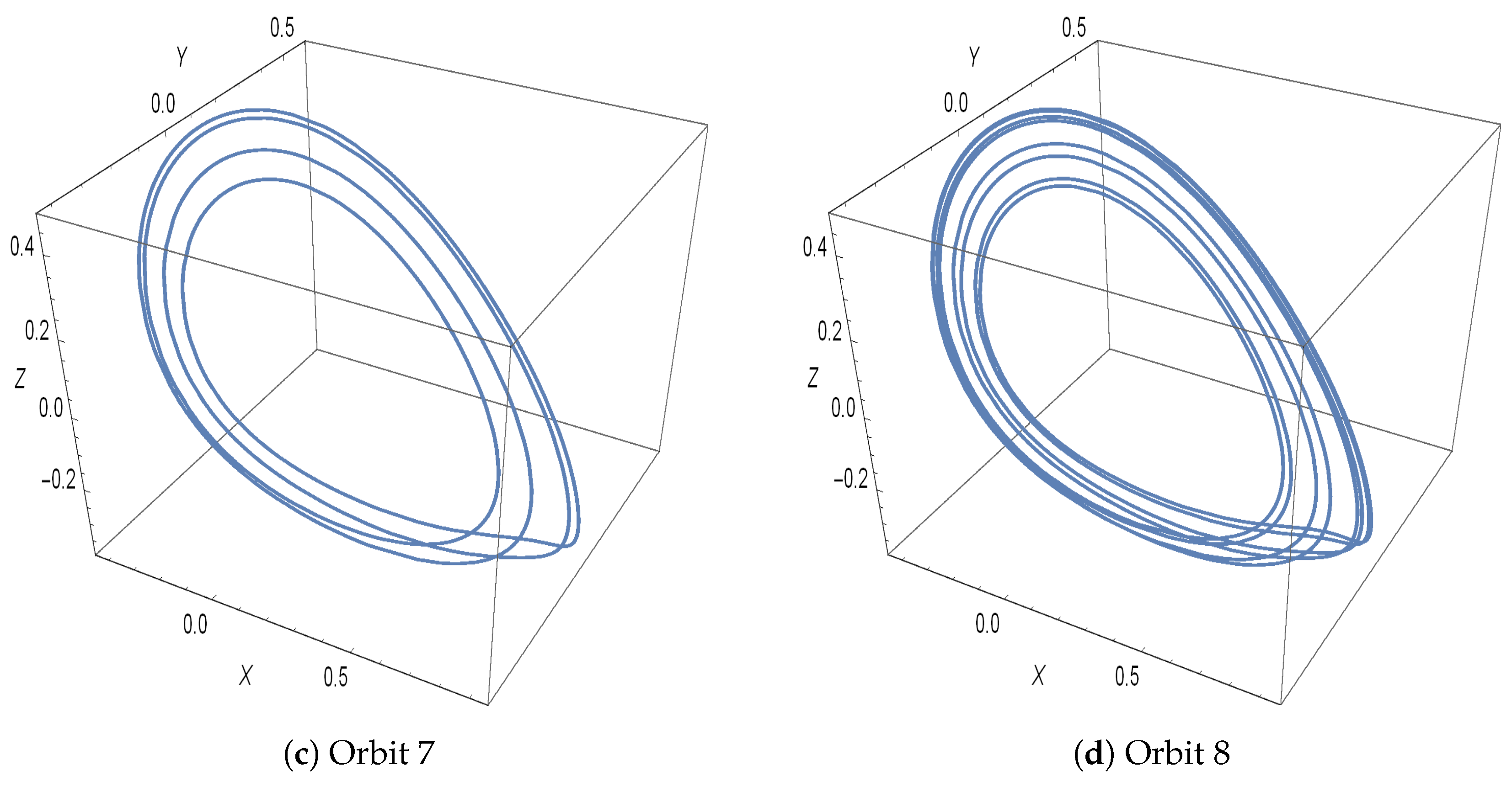
| T | |||||
|---|---|---|---|---|---|
| Orbit 1 | |||||
| Orbit 2 | |||||
| Orbit 3 | |||||
| Orbit 4 |
| T | |||||
|---|---|---|---|---|---|
| Orbit 5 | |||||
| Orbit 6 | |||||
| Orbit 7 | |||||
| Orbit 8 |
| Bifurcation | n | |||
|---|---|---|---|---|
| 1 | – | – | ||
| 2 | – | |||
| 3 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Diab, Z.; Guirao, J.L.G.; Vera, J.A. Zero-Hopf Bifurcation in a Generalized Genesio Differential Equation. Mathematics 2021, 9, 354. https://doi.org/10.3390/math9040354
Diab Z, Guirao JLG, Vera JA. Zero-Hopf Bifurcation in a Generalized Genesio Differential Equation. Mathematics. 2021; 9(4):354. https://doi.org/10.3390/math9040354
Chicago/Turabian StyleDiab, Zouhair, Juan L. G. Guirao, and Juan A. Vera. 2021. "Zero-Hopf Bifurcation in a Generalized Genesio Differential Equation" Mathematics 9, no. 4: 354. https://doi.org/10.3390/math9040354
APA StyleDiab, Z., Guirao, J. L. G., & Vera, J. A. (2021). Zero-Hopf Bifurcation in a Generalized Genesio Differential Equation. Mathematics, 9(4), 354. https://doi.org/10.3390/math9040354








